Analysis of Yield Threshold in a Functionally Graded Variable Thickness Rotating Disc Using the Tresca Yield Criterion

1. Introduccian

The rapid industrial advancement [1,2,3,4,5,6] in recent years has increased the human need for materials with optimal properties and special capabilities [7,8,9,10,11,12,13,14,15]. One category of new materials developed by scientists in this field is Functionally Graded Materials (FGM). FGM are composite materials that exhibit gradual and continuous variations in composition, structure, and properties in different directions within the component. The concept of Functionally Graded Materials was first introduced in 1984 [16] , and since then, research has continued to develop materials with high efficiency and heat resistance using the technology of gradual changes.

Rotating equipment plays a crucial role as one of the main components in various industries [17,18,19,20,21]. The industry of rotating equipment requires research and investigation in different areas due to its strategic role in various industries and the numerous parameters that significantly affect the performance and efficiency of the industrial processes.

Among the rotating components that have widespread applications and play a vital role is the rotating disc. The increasing use of rotating discs in diverse industries such as Power Generation Industry and Turbine Manufacturing, automotive [13,22,23,24,25,26,27,28,29], maritime, and others highlights its crucial importance in the industry [30,31,32,33,34,35].

Considering the significance of rotating discs in various industries, the analysis of these discs under different loading conditions becomes essential. Therefore, this research aims to provide a proper analysis to study the yielding behavior of variable thickness rotating discs.

Timoshenko and Goodier were the first to propose a closed-form set of stress equations for a rotating disk[36]. Gamer published four articles in the years 1983-1985, focusing on the deformation and stress distribution in rotating discs under different boundary conditions [37,38,39,40]. They based their analyses on the Tresca yield criterion and performed the flow law analysis. In Gamer’s analyses, density and thickness were assumed to have constant distributions, [37] and they investigated the elastoplastic behavior in a rotating cylinder. Their analysis, based on the Tresca yield criterion, compared the stress distribution under two conditions: fully plastic and linear hardening.

Furthermore, the influence of density on the elastoplastic behavior of a hollow rotating disc with variable thickness was examined by Guven [41]. Guven then[42] analyzed an assumed disc under external pressure, focusing on angles corresponding to the yield threshold. Finally, Argso and his colleagues [43] investigated the velocity of homogenous variable-thickness discs.

Various research studies have been conducted on rotating disc and cylindrical structures made of functionally graded materials (FGM) due to the advantages they offer. Akis and Eraslan [44] investigated the yielding thresholds of hollow rotating shafts made of functionally graded materials using the Tresca yield criterion. They considered the elastic modulus and yield stress as power functions of the radial coordinate. Later, Argeso and Eraslan[45], based on the Tresca yield criterion, presented an exact solution for the elastoplastic analysis of a rotating functionally graded shaft.

Birman and Byrd [46] studied the stress in a functionally graded rotating disc, considering the Young’s modulus and Poisson’s ratio as power functions. They used the finite difference method for numerical analysis. Akis and Eraslan [47] performed an analytical elastoplastic analysis of a functionally graded rotating cylinder and utilized the ideal plasticity associated with the Tresca yield criterion for the plastic deformation analysis.

Bayat and his colleagues [48] analyzed a functionally graded rotating disc with variable thickness. They considered the material properties and disc profile as power functions along the radial direction. Madan and Saha [49] investigated the effect of functionally graded materials on the yield threshold of a thick-walled rotating cylinder. They also compared the yield criteria of von Mises and Tresca. Furthermore, they provided an exact solution for elastoplastic analysis of a thick-walled functionally graded rotating cylinder under pressure, considering the elastic modulus, density, and yield stress as power functions of the radial coordinates.

Ting-Dai and Hong-Dai[50]assumed a functionally graded hollow rotating disc with variable rotational velocity. They considered the elastic modulus and density of the rotating disc as variables along the radial direction. Ma and Hao [51] analyzed the elastoplastic deformation of a rotating disc beyond the yield limit. They examined the effects of various parameters, including cross-sectional profiles, and material properties on the critical velocitys of the disc. Peng and Li [52] analyzed the elastoplastic deformation of a rotating disc at velocitys exceeding the yield limit. They studied the effects of various parameters, including the cross-sectional profiles and material properties, on the critical velocitys of the disc. Additionally, they provided a numerical solution for the elastoplastic deformation of a functionally graded rotating disc, considering linear hardening.

Lomakin and his colleagues [53] analyzed the hollow rotating disc using the von Mises yield criterion along with the flow law to study the fields of stress and strain in the elastoplastic region.

The main objective of this research is to analyze the yielding threshold of a functionally graded hollow rotating disc with variable thickness. This analysis takes into account the variations in elastic modulus, density, and yield stress. The Tresca yield criterion is utilized to investigate the yielding conditions.

2. Equations of Motion

there is a hollow disk made of functionally graded materials, which is sufficiently thin and large, with inner radius a and outer radius b, rotating at an angular velocity $\omega$. Considering the geometry of the problem, the formulation and analysis are performed in cylindrical coordinates $(z,\theta ,r)$. The thickness of the disk section, elastic modulus, density, and yield stress are assumed to be power functions (1) of the radial coordinate:

(1)

In order,$h_0$ the values of thickness, Young’s modulus $E_0$ are given by equations (1). ${\rho }_0$density, and ${\sigma }_{Y_0}$ yield stress at the outer radius $(r=b)$ are also provided. The parameters $n_t$,$n_E$,$n_{\rho }$ and $n_{\sigma }$ represent the geometric and material properties and are defined as per equations (2).

(2)

The values of ${\delta }_t$ , ${\delta }_E$,${\delta }_{\rho }$ and ${\delta }_{\sigma }$ are constant. If in equations (2), the material and geometric properties are represented in a general form as P, then the variation of p and its value at the outer radius with $p_0$ is shown dimensionless as $\overline{p}=p/p_0$. The results for the variation of material and geometric properties are depicted in Figure 1 for the dimensionless radius$\overline{r}$.

The equation of motion for the rotating disk, considering the effect of thickness, is expressed as equation (3).

(3)

The components of radial and hoop stresses, ${\sigma }_r$ and ${\sigma }_{\theta }$, in equation (3) are represented. It should be noted that the volumetric force due to weight ($\rho g$) has been neglected. Now, the radial and hoop displacements, u and v, are considered. Due to axial symmetry, there is no hoop displacement, and in other words, $v=0$. Therefore, the stress equations in cylindrical coordinates are as follows:

(4)

Using Hooke’s law for the state of plane stress and displacement, strain equations (4) are employed, and the stress-displacement relationship is obtained in the form of equations (5).

(5)

By substituting the stress components into equation (3), the equation of motion the disk is transformed into the form of equation (6).

(6)

The analytical solution of the second-order differential equation (6) has a general solution in the form of equation (7):

(7)

In which $C_1$ and $C_2$ are integration constants. Also, the constant parameters in equation (7) are defined as equations (8).

(8)

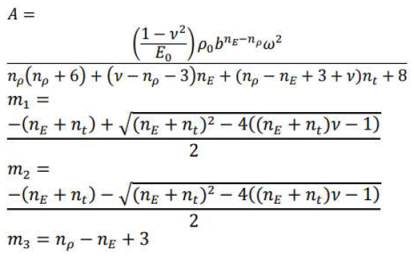
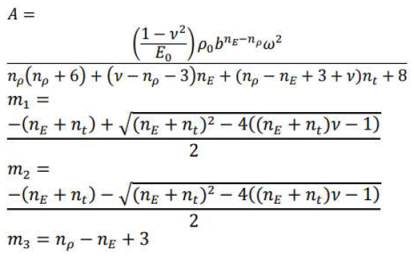
By substituting the displacement equation (7) into the strain equations (5), the radial and hoop stresses are obtained in terms of the constants $C_1$ and $C_2$ as equations (9).

(9)

The constants $C_1$ and $C_2$ are obtained. For a hollow disk, the radial stress at the inner boundaries ${\left({\sigma }_r\right)}_{r=a}$ and the exterior ${\left({\sigma }_r\right)}_{r=b}$ is zero. Therefore, by applying the boundary conditions for the disk, the constants $C_1$ and $C_2$ are obtained in equation (10).

(10)

In which R is a constant parameter defined in equation (10) as follows:

(11)

In order to obtain general solutions, the derived equations are made dimensionless using equations (12).

(12)

3. Analyze yielding state

In order to determine the angular velocities corresponding to the yielding threshold and examine the yielding conditions, the Tresca yielding criterion has been employed. The utilization of the Tresca yielding criterion necessitates establishing the order of principal stresses ${\sigma }_r$ and ${\sigma }_{\theta }$. On the other hand, the order of principal stresses is dependent on the numerical values of the power parameters ($n_t$, $n_p$,$n_E$ and $n_{\sigma }$) and the ratio of radii $(r/b)$. Therefore, for monitoring the initiation of yielding, a dimensionless variable $\Psi$ based on the Tresca criterion is utilized. This variable is defined by the equation (13).

(13)

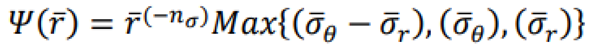
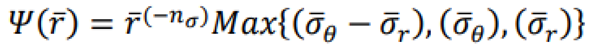
The aforementioned criterion indicates that yielding initiates from points where $\Psi =1$, and at the onset of yielding, the dimensionless function $\Psi$ attains its maximum value.

For the analysis of the behavior of the rotating functionally graded material (FGM) disk, depending on the values of the power parameters, yielding may initiate from the inner radius, the outer radius, both simultaneously, or the intermediate region between the inner and outer radii.

3.1. State 1: Initiating yielding from the inner radius

Yielding occurs when $\Psi \left(\overline{a}\right)=1$ and the function $\Psi$ has its maximum absolute value at the inner radius. By utilizing the stress equations (9) and applying the boundary condition ${\left({\overline{\sigma }}_r\right)}_{\overline{r}=\overline{a}=0}$, the dimensionless terminal rotational velocity ${\Omega }_{e1}$ can be defined as per the equation (14):

(14)

The constant S is defined as equation (15).

(15)

Where H is expressed as equation (16).

(16)

3.2. State 2: Initiating yielding from the outer radius

In this case, yielding occurs when $\Psi \left(1\right)=1$ and the function $\Psi$ has its maximum absolute value at the outer radius. By using the stress equations (9) and applying the boundary condition ${\left({\overline{\sigma }}_r\right)}_{\overline{r}=\overline{b}}=0$, the dimensionless terminal rotational velocity ${\omega }_{e_2}$ is defined as an equation (17):

(17)

In which the constant $S_b$ is defined as equation (18):

(18)

3.3. State 3: Simultaneous yielding initiation from both inner and outer radii.

In this case, yielding commences simultaneously from the inner and outer radii when both $\Psi \left(\overline{a}\right)=1$ and $\Psi \left(1\right)=1$ are satisfied, and the function $\Psi$ must have maximum absolute values at both the inner and outer radii. Considering the boundary conditions of the disc and using equations (14) and(17), the critical dimensionless angular velocity ${\omega }_{c_r}$ and critical power parameter $n_{c_r}$ can be obtained by solving the system of equations (19).

(19)

3.4. State 4: Yielding initiation from a radius between the inner and outer radii.

To initiate yielding from a radius between the inner and outer radii, the dimensionless function $\Psi$ at the yielding initiation point $r_{ep}$ must be equal to 1 and have the maximum absolute value at this point. For this reason, equation (20) must hold true to initiate yielding from the boundary between the inner and outer radii.

(20)

Equation (20) is used to calculate the required terminal rotational velocity for initiating plastic flow and the location of yielding initiation point ${\overline{r}}_{ep}$ for a specific power parameter n.

4. Conclusions

In this paper, an analytical study of the threshold of yielding in a variable thickness rotating disk made of functionally graded materials (FGM) based on the Tresca criterion is presented. The thickness of the disk cross-section, modulus of elasticity, density, and yield stress were assumed to be power functions of the radial coordinate. The effects of various parameters on the initiation of yielding in the rotating disk were investigated in this study. Furthermore, emphasizing that yielding always initiates from the inner radius in homogeneous rotating disks, different scenarios were considered for the initiation of yielding and the propagation of plastic flow in the target rotating disk. The results clearly demonstrate the significance of considering thickness variations in the analysis of the threshold of yielding in the target rotating disk.

For the results of this draft, please consider contacting the first author of this paper.