Prerpints.org logo
Preprint
Article

Forest site and stand structure affecting the distribution of Emerald Ash Borer, Agrilus planipennis Fairmaire, 1888 (Coleoptera: Buprestidae) in Eastern Ukraine

Altmetrics

Downloads

125

Views

47

Comments

0

A peer-reviewed article of this preprint also exists.

supplementary.zip (144.96KB )

Submitted:

05 February 2024

Posted:

06 February 2024

You are already at the latest version

Alerts
Abstract
Emerald ash borer (EAB), Agrilus planipennis, an invasive phloem-boring beetle, was detected in the Luhansk Region of Ukraine in 2019. In the following years, it expanded to a large part of the Kharkiv region and the parks of Kyiv. Previous research proved that the climate in Luhansk and neighboring regions is favorable for EAB, and the lack of a host plant (Fraxinus sp.) cannot prevent pest expansion in Ukraine. Considering the necessity to detect inhabited trees in time, our current research aimed to identify the most attractive EAB forest subcompartments considering forest site conditions and stand structure. MaxEnt model predicted the potential distribution of EAB with high accuracy (AUC = 0.842). The most significant five variables provided 82.9% to the model, particularly «Age of trees, years», «Area of forest subcompartment, ha», «Mean height of trees, m», «The proportion of Fraxinus excelsior in the stand composition, %», and «Hygrotope index (humidity level), point». A very high probability of EAB expansion in the Luhansk region (>90 %) is predicted at 20 % of the forest area with Fraxinus sp. in the stand composition. The probability of EAB spread above 30% is predicted for 75 % of such area. To improve the forecasting accuracy, it could be necessary to consider the data on road localization along which the pest can spread passively, as well as dominate wind speed.
Keywords: 
Subject: Biology and Life Sciences  -   Forestry

1. Introduction

The Emerald ash borer (EAB), Agrilus planipennis Fairmaire, 1888 (Coleoptera: Bu-prestidae) is a phloem-boring beetle, originating from the temperate regions of Northeast Asia [1], inadvertently found its way into North America and European Russia in the 1990s, likely introduced through infested ash material. The full extent of the catastrophe only became apparent in the early 2000s following the initial widespread outbreaks [2]. On its native range, EAB colonized Fraxinus mandshurica Rupr. and F. chinensis Roxb.) without significant damage [3]. EAB displays a broader host range in secondary ranges, infesting all Fraxinus species and even non-ash host, cultivated olive (Olea europaea L.) [4,5]. Over the past two decades, the range of EAB has expanded and continues to increase. Numerous kinds of research were devoted to predicting the ecological, economic, and social impacts of EAB, and management efforts based on studies of its host preference, cold tolerance, and many other features summarized in well-known reviews [3,6]. Despite the diligent efforts of scientists and practitioners, EAB relentless spread is evident, currently documented in 36 US states, five Canadian provinces, and 20 regions of Russia [6].
In 2019, EAB was first identified in Ukraine, specifically in the Luhansk Region [7,8]. However, the various larval instars and exit holes suggest that the pest likely infiltrated the region in 2017, or potentially even earlier, potentially originating from the neighboring Voronezh region of Russia.
Within two years, EAB had expanded its reach over 300 km westward from the initial detection site, colonizing hundreds of trees both F. excelsior and F. pennsylvanica [9]. By the end of 2021, the pest was detected in most of the Luhansk region and the neighboring part of the Kharkiv region [9]. In 2022, EAB was identified in the parks of Kyiv [10], and by 2023, it had spread to a significant part of the Kharkiv region [11].
However. field research in 2022–2023 was hindered due to the region's involvement in active hostilities.
Consequently, during these years, investigations focused on the seasonal development of EAB and climate variables influencing its successful survival. The wide range of bioclimatic variables in EAB regions indicates this pest's high ecological adaptability [9]. The westward spread of EAB from the Luhansk region to the west was predicted using the MaxEnt model, and a comparative analysis of the most significant bioclimatic indicators was conducted between its natural and invasive ranges. Climate favorableness for EAB in Luhansk and neighboring regions further underscores the urgency of addressing this invasive threat [9].
A sufficient number of host trees is the second important condition (besides climate) for EAB spread [12]. In Ukraine, ash forests encompass approximately 150,000 hectares, or 2.4 % of the total forested area [13]. Among them, F. excelsior stands are dominant, covering nearly 87% or 130,000 hectares, while the remainder is predominantly F. pennsylvanica. The majority of ash stands (93.6% or 121,700 hectares) are interspersed with other broadleaved tree species with different proportions of ash. Both ash species are also presented in roadside shelter belts and urban stands in Ukraine [14], although comprehensive data regarding the total area of these plantings are currently unavailable. Consequently, ash as a host tree for EAB is widespread throughout Ukraine.
The primary challenge of effective EAB management is early detection, but this becomes intricate. In young trees, EAB colonizes trunks and branches with a bark thickness ranging from 1.5 to 5 mm [15]. This bark provides sufficient protection for larvae and pupae from desiccation, extreme temperatures, predators, and parasitoids, typically characterizing stems and branches with a diameter of 5–10 cm [15].
Conversely, in larger-diameter trees, EAB first inhabits individual branches of high trees, and the crowns of inhabited trees do not differ from uninhabited ones [17,18]. To reveal such trees from the ground (without tree felling) becomes possible only after the woodpeckers begin to peck the galleries [11].
Assessing EAB in the early stages of tree colonization on cut sample branches is labour-intensive [17], prompting the need to identify trees or stands most attractive to the pest, where EAB abundance and outbreak area may rapidly increase [12].
Existing publications suggest that EAB exhibits a preference for trees with open or thinly populated canopies for mating and egg-laying [18,19]. The density of the population, the speed of development, and the overall survival of individuals are greater exposed to higher levels of light [15], especially those situated at the edges of forests [1,20].
Therefore, our current research aimed to identify the most attractive forest subcompartments for EAB, considering forest site conditions and forest structure.

2. Materials and Methods

2.1. Study region

The entry point of the Emerald Ash Borer (EAB) into Ukraine was documented in the Luhansk region (Figure 1).
This region is situated within the steppe Atlantic-continental climatic region, characterized by the highest continentality and aridity. Geobotanically, the area is classified as diverse feather-grass, fescue-feather-grass, and worm-wood steppes. Based on forest typological zoning, it falls within the Donetsk Bayrak forest region, and according to comprehensive forestry zoning, it belongs to the Forest-Steppe, Left-Bank Dnieper Forest-Steppe District, and Northern (Bayrak) Steppe [21,22]. The region experiences scorching and arid summers marked by drought and hot, dry winds, as well as cold and low-snow winters featuring regular thaws, fog, and ice-covered ground, uneven distribution of precipitation in the year, and the growing season, dust storms and snowstorms. The maximum air temperature in July reaches +39 °C (+42 °C on August 12, 2010), the minimum in January drops to -38 °C (-41.9 °C on January 8, 1935). Frequent thaws occur in winter, with temperatures rising to +15 °C. The annual precipitation is 445 mm, and droughts are recorded on average once every three years. During the growing season, easterly winds prevail [23], a factor contributing to EAB spread westward.
Forests cover 11.5% of the territory of the Luhansk region, primarily in small are-as along rivers, on ravine slopes, and in gullies. Over 70 tree species grow in the forests of the Luhansk region. The largest areas are occupied by Common oak (Quercus robur L.) at 38.1%, Scots pine (Pinus sylvestris L.) at 30.9%, Black locust (Robinia pseudoacacia L.) at 8.9%, European ash (F. excelsior) at 5.4%, and American green ash (F. pennsylvanica) at 3.0% [24]. The shelterbelts cover 30.3 thousand hectares with species composition including Black locust – 39.8%, Common oak – 19.8%, American green ash – 14.7%, and European ash – 8.0%. Over half of the shelterbelts were planted in the 1940s-1950s [25].

2.2. Modeling the spread of the emerald ash borer

The spread of A. planipennis was projected using a niche model generated in MaxEnt software, version 3.4.4 [26,27]. This software was chosen due to its capability to handle presence-only data and accommodate a limited number of presence points [28]. Information regarding the presence of EAB in the Luhansk region was obtained during forest inspection in 2020-2021 (the first years of the pest’s invasion into the Lugansk region) covering latitudes from 49°49'03.0"N to 49°14'03.6"N, and longitudes from 38°51'43.7"E to 39°21'57.2"E.
Variables representing forest site conditions and stand characteristics (Table 1) were obtained from the Database of Production Association “Ukrderzhlisproekt”.
Variables Limits
Hygrotope index (humidity level) 0, 1, 2, 3, 4, 5
Trophotope index (A,B,C,D) 1, 2, 3, 4
The proportion of Fraxinus excelsior in the stand composition 10–100%
The presence of any Fraxinus species in the stand composition 1/0
Age of trees, years 30–110
Mean height of trees, m 6–25 m
Mean diameter of trees, cm (DBH) 4–36 cm
Relative density of stocking 0.5–0.9
Site index class 1–5
Area of forest subcompartment 0.5–59.5 ha
Number of non-forested lands neighbouring subcompartment 0–8
Combination of soil richness levels (trophotope – nutritional richness for trees) – (A, B, C, D), and humidity levels (hygrotope) – 0, 1, 2, 3, 4, 5 characterize each forest subcom-partment, aligning with the Ukrainian forest typology [22]. Group A ("bor") is characterized by sandy soils as well as oligotrophic (nutrient-poor) vegetation (Pinus spp., Betula spp.). At the same time, group B ("subor") features sandy clay soils and mesotrophic (moderately fertile) vegetation (Quercus spp., Populus spp., Picea spp.). Clay loam soils together with megatrophic flora (Acer spp., Tilia spp., Alnus sp., and Fraxinus spp.) define group C (“sugrud”), and clay soils, conducive to the optimal development of mesotrophic and megatrophic (highly fertile ) vegetation and the lack of oligotrophic are characteristic for group D (“grud”). Six soil moisture regimes (potential hygrotope) are identified: 0 –very xeric, 1 – xeric, 2 –submesic, 3 – mesic, 4 – subhygric, and 5 – hygric (wetland) [22].
In preparation for MaxEnt, all parameters were used as real. Trophotope classes A, B, C, and D were converted to 1, 2, 3, and 4, respectively. Several consecutive operations were executed using QGIS 3.3.2:
– attributive data (variables for each subcompartment as XLSS file) and subcompart-ment geometry (Esri shp format) were joined in one vector layer for the whole Luhansk region;
– subcompartments with Fraxinus sp. presence were selected;
– vector layer projection was converted to EPSG:3856 (Pseudo-Mercator);
– values of each variable (columns, or fields) in the attribute table (see Table 1) were converted to raster images in GeoTIFF format;
– all raster images obtained were converted to ASC files for further use in MaxEnt 3.4.4.
For forest plots inspected with unknown coordinates, we used the centroids of respective compartments and then exported them in a CSV file for further use in MaxEnt 3.4.4.
The variables listed in Table 1 served as predictors, and the algorithm identified the most important variables based on the preset regularization parameters. The default setting of “cross-validation” was selected for replicates of the Species Distribution Models (SDMs), as it effectively utilized all available data and maximized the utility of the limited dataset [31]. The forest layer for the map (ESA WorldCover 10m v200) was sourced from the dataset Google Earth Engine [29]. QGIS 3.3.2 was utilized for map creation [30].
To assess model performance, the area under the curve (AUC) parameter of the ROC (receiver-operating characteristic) was employed [31]. AUC reflects the probability of accurately predicting the existence of emerald ash borer in forest subcompartments. If the AUC value approached 0.5 for the experimental data, the model demonstrated performance equivalent to a random model. Conversely, if the AUC reached 1.0, the model exhibited a flawless fit, indicating the absence of false-negative results [27,31]. The impact of variables on the obtained model was assessed through both contribution and permutation coefficients. For verification, we employed the jack-knife test, generating three various models for each variable. The first model is without any variable, while the second and third ones comprise one and all the variables respectively.

3. Results

MaxEnt accurately forecasted the potential distribution of the emerald ash borer, yielding a commendable test under the curve (AUC) value of 0.842 accompanied by a standard deviation of 0.018. Additionally, the jack-knife test underscored consistently high AUC values for each variable (Table 2).
Based on the calculations, the age of trees exhibits the highest significance in constructing the EAB range model, accounting for 38.9% of the model's influence. However, it also displays the highest permutation in young age categories (Figure 2a). The permutation represents a considerably smaller correlation between the selected and other variables (Figure 2b).
Variable “Area of forest subcompartment” takes the second place in terms of contribution to the model (Table 2). The influence of the subcompartment area on EAB spread (Figure 3) was almost independent of other variables. Pest foci are found more often in the subcompartments, which are characteristic of the sparsely forested Luhansk region.
Variable “Mean height of trees, m” takes the third place in terms of contribution to the model (Table 2).
At the same time, in the case of taking into account all variables, a high permutation of the height effect on the probability of EAB colonization was computed (Figure 4a) in the case of an increase in height of more than 30 m. In the case of taking into account the correlation of the variable "Mean height of trees" with other variables, the permutation is much smaller (Figure 4b). In both cases, the maximum probability of colonization by EAB falls on the age of trees of about 25 years.
The percentage of Fraxinus excelsior in the stand composition contributes 10,6 % to EAB prediction model with rather low permutation (see Table 2). With an increase in the proportion of Fraxinus excelsior in the stand composition, the likelihood of pest spread rises, both when all other environmental variables are maintained under standard sample conditions (Figure 5a), and when considering correlations amongst the selected and other variables (Figure 5b).
Among the remaining variables, “Hygrotope index (humidity level)” contributes 8.3 % to the model of EAB spread, but has a rather high permutation – 13,5 % (see Table 2). In both calculation variants, the probability (POP) of pest distribution increases in drier conditions (Figures 6a, 6b).
The mentioned five variables describe 82.9% of the contribution to the modeling of the probability of EAB presence (see Table 2).
The model revealed a significantly elevated likelihood of EAB spread in the Luhansk region (>90 %) at the 20 % of forest area with Fraxinus sp. in the stand composition, particularly probability >30% – at the 75 % of forest area with Fraxinus sp. (Table 3; Figure 7).
Coordinates of settlements in the selected fragment
Name Latitude Longitude
Hannivka 38.787006 49.342424
Kuriachivka 38.811778 49.320327
Pidhorivka 38.826286 49.297213
Starobil'sk 38.897531 49.268241
Butkivka 38.902156 49.390202
Lyman 38.950438 49.332158

4. Discussion

The survival success of a non-indigenous species in establishing itself in a new region is contingent upon favorable climatic conditions [32,33], a sufficient number of host trees [34], and a minimum of competitors or entomophagous [35,36]. Therefore, various approaches to predicting the EAB spread have considered climatic conditions favorable for pest survival and propagation [33,37,38,39], the spread of host plant [34,40], landscape and stand characteristics [41], the possibility of active moth migration along the roads and passive spread with wood chips [42], other trunk fragments with bark and various combinations [6].
It has been demonstrated that EAB is well-adapted to temperature variations [6]. Particularly in Ukraine, using MaxEnt model and 19 Bioclimatic variables [9] resulted in high accuracy (AUC = 0.988) predicting EAB penetrations for 87% (Luhansk), 48% (Kharkiv) and 32% (Donetsk).
Field inspection confirmed, that EAB spread in 2020–2021 in most of the Luhansk region and in the south-east of the Kharkiv [14].
Despite limited access to forests due to military operations, the borer was discovered in the parks of Kyiv in 2022 [10], and in 2023 – in a significant part of the Kharkiv region, including in urban plantings of Kharkiv [11].
An analysis shows that Fraxinus sp. is rather abundant in the forest (see Figure 1), as well as in the shelter belts and urban plantings of Ukraine [13,24], suggesting that the lack of a host plant does not hinder the expansion of EAB in Ukraine. At the same time, in the Luhansk region, Fraxinus sp. in the forest is represented on 12.3 thousand hectares, in Kharkiv region on 7 thousand hectares, in Kyiv region on 6.1 thousand hectares, and in the Sumy region on 12.8 thousand hectares [13]. However, there are regional variations, such as EAB's absence in the Sumy region [14] but is present in Kyiv [10]. Non-published information about the mass decline of F. pennsylvanica Marshall. in the shelter belts along the Kharkiv-Kyiv highway suggests that the pest spreads mainly in this way.
When implementing MaxEnt, 11 variables that characterize the forest stands of the Luhansk region populated with EAB in 2020–2021 were used.
The high reliability of the EAB spread prediction model was statistically confirmed (AUC value of 0.842), and the contribution of individual variables was evaluated (see Table 2). The most significant five variables provided 82.9% to the model, particularly «Age of trees, years», «Area of forest subcompartment, ha», «Mean height of trees, m», «The proportion of Fraxinus excelsior in the stand composition, %», and «Hygrotope index (humidity level), point».
The variable «Age of trees, years» (38.9%) has the largest contribution to the model (see Table 2, Figure 2) because EAB colonizes trees of all ages but favors parts of the trunk and branches with thin bark. Hence, colonization in the upper trunks and crowns of trees over 60 years old is often impossible to see from the ground.
It is for this reason that the contribution of the variable "mean diameter of trees" ranks one of the last among the considered indicators (see Table 2). However, it usually correlates with the age of trees [22].
The more frequent EAB spread in forest subcompartments of smaller areas (see Figure 2) can be explained by the fact that the study region belongs to the steppe zone, and the size of forest subcompartments is often quite small. In such subcompartments, the trees are better lit and heated, which benefits EAB. The presence of EAB in urban plantings is consistent with information from other regions about its preference for more illuminated trees [15], particularly at the forest edges [1,20], and sparse crowns for mating and laying eggs [18,19], as well as with EAB detection in the parks of St. Petersburg [43], Moscow [44], Kyiv [10] and Kharkiv [11].
The significance of the variable “Mean height of trees” (Figure 3) can be explained by the dependency of the available bark surface of the trunk and branches for colonization by EAB on the tree height. The probability of EAB colonizing trees increases in stands with a larger proportion of ash (Figure 4) because Fraxinus sp. is the main host tree of this insect.
The higher probability of EAB spread in drier conditions (Figure 5) is consistent with information about the preference of many phytophages for warmer and illuminated environments, as well as with greater weakening of trees and their greater susceptibility to colonization by phytophages under conditions of moisture scarcity [45]. Thus, it has been found that EAB prefers to colonize trees with sparse crowns, and its larvae develop more slowly in healthy trees [18,19]. In more lightened trees, higher EAB population density, development rates, and survival were noted [15], particularly at the edges [1,20].
The contribution of the remaining six analyzed variables to the model for predicting EAB spread was 17.3 %.
Our modeling using Maxent has revealed a notably high probability of EAB distribution in the Luhansk region (>90 %) is predicted at 20 % of the forest area with Fraxinus sp. in the stand composition. Particularly the probability of EAB invading above 30% is predicted for 75 % of forest area with Fraxinus sp. in the stand composition (Table 3; Figure 7).
At the same time, significant adjustments to the forecast can be made by data on EAB presence in the forest belts containing F. pennsylvanica. Unfortunately, access to many of them in the region is limited due to military operations.
The swift penetration of EAB into Kyiv within such a brief timeframe indicates an increased threat of further spread of the pest to the West. Improving the forecasting accuracy may involve considering data on road localization along where the pest can disperse passively, as well as dominate wind speed. Leveraging pairwise distances among places where EAB is present, as indicated in [46], could prove to be a beneficial method for determining the likelihood of EAB spread.

5. Conclusions

The MaxEnt model for predicting the spread of the Emerald Ash Borer (EAB) in the forest stands of the Luhansk region, populated with EAB in 2020–2021, demonstrated high reliability, as statistically confirmed (AUC value of 0.842).
The most impactful five variables provided 82.9% to the model, particularly «Age of trees, years», «Area of forest subcompartment, ha», «Mean height of trees, m», «The proportion of Fraxinus excelsior in the stand composition, %», and «Hygrotope index (humidity level), point».
A very high probability of EAB expansion in the Luhansk region (>90 %) is predicted at 20 % of the forest area with Fraxinus sp. in the stand composition. The probability of EAB spread above 30% is predicted for 75 % of such area.
To enhance forecasting accuracy, it is recommended to consider data on road localization, which serves as a passive pathway for pest spread, along with an evaluation of dominant wind speed.

Supplementary Materials

The following supporting information can be downloaded at: Preprints.org, Table S1: Results of MaxEnt modeling of EAB spread.

Author Contributions

conceptualization, V.M.; Data curation, T.K., Y.S.; Formal analysis, V.M., T.K., N.V., Y.S. and J.H.; Investigation, V.M., T.K., N.V., Y.S. and K.D.; Methodology, V.M., O.B., T.K., K.D. and J.H.; Software, O.B.; Supervision, J.H.; Validation, O.B. and N.V.; Visualization, O.B.; Writing – original draft, V.M., N.V. and K.D.; Writing – review & editing, V.M., Y.S., K.D. and J.H.

Funding

This research was funded by Funding: Project EVA 4.0 Advanced Research Supporting the Forestry and Wood-Processing Sector’s Adaptation to Global Change and the Fourth Industrial Revolution, CZ.02.1.01/0.0/0.0/16_019/0000803 Czech University of Life Sciences Prague. K.D. was funded by the Swedish Foundation for Strategic Research (SSF) project UKR22-0060, Knut and Alice Wallenberg’s foundation project KAW 2022.0211. The research of K.D. was funded in part by the Swedish Research Council Formas (project no. 2023-01065).

Acknowledgments

KD, Y.S. and O.B. were supported by the Ministry of Education and Science of Ukraine within joint Ukrainian-Latvian project No M/92-2023

Conflicts of Interest

The authors declare no conflicts of interest.

References

  1. Wang, X.Y.; Yang, Z.Q.; Gould, J.R.; Zhang, Y.N.; Liu, G.J.; Liu, E.S. The biology and ecology of the emerald ash borer, Agrilus planipennis, in China. J. Insect Sci. 2010, 10, 128. [Google Scholar] [CrossRef]
  2. Haack, R.A.; Baranchikov, Y.; Bauer, L.S.; Poland, T.M. Emerald ash borer biology and invasion history. In Biology and Control of Emerald Ash Borer; Van Driesche, R.G., Reardon, R.C., Eds.; FHTET-2014-09 Department of Agriculture, Forest Service, Forest Health Technology Enterprise Team: Morgantown, WV, USA, 2015; Chapter 1; pp. 1–13.
  3. Herms, D.A.; McCullough, D.G. Emerald ash borer invasion of North America: History, biology, ecology, impacts, and management. Annu. Rev. Entomol. 2014, 59, 13–30. [Google Scholar] [CrossRef]
  4. Cipollini, D.; Rigsby, C.M.; Peterson, D.L. Feeding and development of emerald ash borer (Coleoptera: Buprestidae) on cultivated olive, Olea europaea. J. Econ. Entomol. 2017, 110, 1935–1937. [Google Scholar] [CrossRef]
  5. Orlova-Bienkowskaja, M.J.; Bienkowski, A.O. Southern range expansion of the emerald ash borer, Agrilus planipennis, in Russia threatens ash and olive trees in the Middle East and southern Europe. Forests 2022, 13, 541. [Google Scholar] [CrossRef]
  6. Sun, J.; Koski, T.M.; Wickham, J.D.; Baranchikov, Y.N.; Bushley, K. E. Emerald Ash Borer Management and Research: Decades of Damage and Still Expanding. Annual Review of Entomology 2024, 69, 239–258. [Google Scholar] [CrossRef]
  7. Drogvalenko, A.N.; Orlova-Bienkowskaja, M.J.; Bienkowski, A.O. Record of the Emerald ash borer (Agrilus planipennis) in Ukraine is confirmed. Insects 2019, 10, 338. [Google Scholar] [CrossRef]
  8. Orlova-Bienkowskaja, M.J.; Drogvalenko, A.N.; Zabaluev, I.A.; Sazhnev, A.S.; Peregudova, E.Y.; Mazurov, S.G.; Komarov, E.V.; Struchaev, V.V.; Martynov, V.V.; Nikulina, T.V.; et al. Current range of Agrilus planipennis Fairmaire, an alien pest of ash trees, in European Russia and Ukraine. Ann. For. Sci. 2020, 77, 1–14. [Google Scholar] [CrossRef]
  9. Meshkova, V.; Borysenko, O.; Kucheryavenko, T.; Skrylnyk, Y.; Davydenko, K.; Holusa, J. Potential westward spread of emerald ash borer, Agrilus planipennis Fairmaire, 1888 (Coleoptera: Buprestidae) from Eastern Ukraine. Forests 2023, 14, 736. [Google Scholar] [CrossRef]
  10. Strygun O.O.; Fedorenko, V.P.; Chumak, P.Y.; Vygera, S.M.;, Honcharenko, O.M., Anyol, O.H. Emerald ash borer (Agrilus planipennis Fairmaire) in Kyiv parks. In: Plant Protection and Quarantine in the 21st Century: Problems and Prospects. Proc. of the International scientific-practical conference dedicated to the anniversaries of the outstanding phytopathologists doctors of biological sciences, professors V.K. Panteleev and M.M. Rodygin (Kharkiv, State Biotechnological University, October 20−21, 2022), Kharkiv: 2022. 189−201. (ISBN 978-614-581-554-6).
  11. Skrylnyk, Y.Y., Kucheryavenko, T.V., Zinchenko, O.V. Distribution of the emerald ash borer Agrilus planipennis Fairmaire, 1888 (Coleoptera: Buprestidae) in the Kharkiv region. In: Plant Protection and Quarantine in the 21st Century: Problems and Prospects. Proc. of the International scientific-practical conference dedicated to the anniversaries of the outstanding entomologists doctors of biological sciences, professors O.O. Migulin and O.V. Zakharenko (Kharkiv, State Biotechnological Univer-sity, October 19−20, 2023). Zhytomyr, Ruta, 2023. 142–145. ISBN 978-617-581-597-7.
  12. Dang, Y.; Wei, K.; Wang, X.; Duan, J.J.; Jennings, D.E.; Poland, T.M. Introduced plants induce outbreaks of a native pest and facilitate invasion in the plants' native range: Evidence from the emerald ash borer. J. Ecol. 2022, 110, 593–604. [Google Scholar] [CrossRef]
  13. Tkach, V.; Rumiantsev, M.; Luk’yanets, V.; Kobets, O.; Pozniakova, S.; Obolonyk, I.; Sydorenko, S. Common ash (Fraxinus excelsior L.) in Ukrainian forests and its successful natural regeneration. For. Stud. | Metsanduslikud Uurim. 2020, 73, 26–42, ISSN 1406-9954. Journal homepage: Available online: http://mi.emu.ee/forestry.studies (accessed on 1 December 2023). [CrossRef]
  14. Davydenko, K.; Skrylnyk, Y.; Borysenko, O.; Menkis, A.; Vysotska, N.; Meshkova, V.; Olson, A.; Elfstrand, M.; Vasaitis, R. Invasion of Emerald ash borer Agrilus planipennis and ash dieback pathogen Hymenoscyphus fraxineus in Ukraine–A concerted action. Forests 2022, 13, 789. [Google Scholar] [CrossRef]
  15. Timms, L.L.; Smith, S.M.; De Groot, P. Patterns in the within-tree distribution of the emerald ash borer Agrilus planipennis (Fairmaire) in young, green-ash plantations of south-western Ontario, Canada. Agric. For. Entomol. 2006, 8, 313–321. [Google Scholar] [CrossRef]
  16. Cappaert, D.; McCullough, D.G.; Poland, T.M.; Siegert, N.W. Emerald ash borer in North America: A research and regulatory challenge. Am. Entomol. 2005, 51, 152–165. [Google Scholar] [CrossRef]
  17. Turgeon, J.J.; Fidgen, J.G.; Ryall, K.L.; Scarr, T.A. Estimates of emerald ash borer (Coleoptera: Buprestidae) larval galleries in branch samples from asymptomatic urban ash trees (Oleaceae). The Canadian Entomologist 2016, 148(3), 361–370. [CrossRef]
  18. Tluczek, A.R.; McCullough, D.G.; Poland, T.M. Influence of host stress on emerald ash borer (Coleoptera: Buprestidae) adult density, development, and distribution in Fraxinus pennsylvanica trees. Environ. Entomol. 2011, 40, 357–366. [Google Scholar] [CrossRef]
  19. Duan, J.J.; Watt, T.; Taylor, P.; Larson, K.; Lelito, J.P. Effects of ambient temperature on egg and larval development of the invasive emerald ash borer (Coleoptera: Buprestidae): Implications for laboratory rearing. J. Econ. Entomol. 2013, 106, 2101–2108. [Google Scholar] [CrossRef]
  20. Caitano, B.; Chaves, T.P.; Dodonov, P.; Delabie, J.H.C. Edge effects on insects depend on life history traits: A global meta-analysis. J. Insect Conserv. 2020, 24, 233–240. [Google Scholar] [CrossRef]
  21. National Atlas of Ukraine [Maps]. Kyiv: DNVP Cartography, 2007. 440 p.
  22. Ostapenko, B.F.; Ulanovsky, M.S. Typological Diversity of Forests in Ukraine. Steppe. Kharkiv: Kharkiv State Agrarian University, 1999. 157 p.
  23. Zubov A. R., Zubova L. G. The climate of Luhansk and its applied aspects: monograph. Luhansk: Publishing house of V. Dahl Luhansk National University. 2016. 180 p.
  24. Bondar, O.B.; Tkach, L.I.; Chuykova, O.O.; Zolotarova, A.S. Typological diversity of forests in the Siversky Donets river basin in the territory of Luhansk region. Ukrainian Journal of Ecology, 2017, 7(3), 120–127.
  25. Bila, Yu.; Tupchiy, O.; Yuhnovskyi, V. Components of optimizing protective forest density in agro-landscapes of the northern (“bayrak”) Steppe in Ukraine. Modern challenges and current issues in forestry education, science, and production: Proceedings of the II International Scientific and Practical Internet Conference (Bila Tserkva, April 15, 2022). Bila Tserkva National Agrarian University, 2022, 34–36.
  26. Phillips, S.J. 2014. A Brief Tutorial on Maxent [Internet]. Available online: http:// www.cs.princeton.edu/~schapire/maxent/ (accessed on 23 June 2023).
  27. Phillips, S.J.; Dudik, M.; Schapire, R.E. Maxent Software for Modeling Species Niches and Distributions. Available online: http://biodiversityinformatocs.amnh.org/open_source/maxent/ MaxEnt version 3.4.4 (accessed on 1 December 2023).
  28. Elith, J.; Graham, C.H. Do They? How Do They? WHY Do They Differ? On Finding Reasons for Differing Performances of Species Distribution Models. Ecography 2009, 32, 66–77. Available online: https://www.jstor.org/stable/30244651 (accessed on 4 August 2022). [CrossRef]
  29. Zanaga, D.; Van De Kerchove, R.; Daems, D.; De Keersmaecker, W.; Brockmann, C.; Kirches, G.; Wevers, J.; Cartus, O.; Santoro, M.; Fritz, S.; Lesiv, M.; Herold, M.; Tsendbazar, N.E.; Xu, P.; Ramoino, F.; Arino, O. 2022. ESA WorldCover 10 m 2021 v200. [CrossRef]
  30. QGIS 3.28.1. Available online: https://qgis.org/en/site/ (accessed on 23 September 2023).
  31. Van Erkel, A.R.; Peter, M. Receiver operating characteristic (ROC) analysis: Basic principles and applications in radiology. Eur. J. Radiol. 1998, 27, 88–94. [Google Scholar] [CrossRef]
  32. Orlova-Bienkowskaja, M.J.; Bieńkowski, A.O. Minimum winter temperature as a limiting factor of the potential spread of Agrilus planipennis, an alien pest of ash trees, in Europe. Insects 2020, 11, 25. [Google Scholar] [CrossRef]
  33. Orlova-Bienkowskaja, M.J.; Bieńkowski, A.O. Low heat availability could limit the potential spread of the Emerald ash borer to Northern Europe (Prognosis Based on Growing Degree Days per Year). Insects 2022, 13, 52. [Google Scholar] [CrossRef]
  34. Dang, Y.Q.; Zhang, Y.L.; Wang, X.Y.; Xin, B.; Quinn, N.F.; Duan, J.J. Retrospective analysis of factors affecting the distribution of an invasive wood-boring insect using native range data: The importance of host plants. J. Pest Sci. 2021, 94, 981–990. [Google Scholar] [CrossRef]
  35. Liu, H.; Bauer, L.S.; Miller, D.L.; Zhao, T.; Gao, R.; Song, L.; Luan, Q.; Jin, R.; Gao, C. Seasonal abundance of Agrilus planipennis (Coleoptera: Buprestidae) and its natural enemies Oobius agrili (Hymenoptera: Encyrtidae) and Tetrastichus planipennisi (Hymenoptera: Eulophidae) in China. Biol. Control. 2007, 42, 61–71. [Google Scholar] [CrossRef]
  36. Liebhold, A.M.; Brockerhoff, E.G.; Kalisz, S.; Nuñez, M.A.; Wardle, D.A.; Wingfield, M.J. Biological invasions in forest ecosys-tems. Biol. Invasions 2017, 19, 3437–3458. [Google Scholar] [CrossRef]
  37. Liang, L.; Fei, S. Divergence of the potential invasion range of emerald ash borer and its host distribution in North America under climate change. Clim. Chang. 2014, 122, 735–746. [Google Scholar] [CrossRef]
  38. Webb, C.R.; Mona, T.; Gilligan, C.A. Predicting the potential for spread of emerald ash borer (Agrilus planipennis) in Great Britain: What can we learn from other affected areas? Plants People Planet 2021, 3, 402–413. [Google Scholar] [CrossRef]
  39. Sobek-Swant, S.; Crosthwaite, J.C.; Lyons, D.B.; Sinclair, B.J. Could phenotypic plasticity limit an invasive species? Incomplete reversibility of mid-winter deacclimation in emerald ash borer. Biol. Invasions 2012, 14, 115–125. [Google Scholar] [CrossRef]
  40. Valenta, V.; Moser, D.; Kuttner, M.; Peterseil, J.; Essl, F. A high-resolution map of emerald ash borer invasion risk for south-ern central Europe. Forests 2015, 6, 3075–3086. [Google Scholar] [CrossRef]
  41. BenDor, T.K.; Metcalf, S.S.; Fontenot, L.E.; Sangunett, B.; Hannon, B. Modeling the spread of the emerald ash borer. Ecol. Model. 2006, 197, 221–236. [Google Scholar] [CrossRef]
  42. Flø, D.; Krokene, P.; Økland, B. Invasion potential of Agrilus planipennis and other Agrilus beetles in Europe: Import pathways of deciduous wood chips and MaxEnt analyses of potential distribution areas. EPPO Bull. 2015, 45, 259–268. [Google Scholar] [CrossRef]
  43. Selikhovkin, A.V.; Popovichev, B.G.; Merkuryev, S.A.; Volkovitsh, M.G.; Vasaitis, R.; Musolin, D.L. Invasive populations of Emerald ash borer Agrilus planipennis Fairmaire, 1888 (Coleoptera: Buprestidae) in Saint Petersburg, Russia: A “Hitchhiker”? Insects 2021, 13, 191. [Google Scholar] [CrossRef] [PubMed]
  44. Orlova-Bienkowskaja, M.J.; Bienkowski, A.O. The life cycle of the emerald ash borer Agrilus planipennis in European Russia and comparisons with its life cycles in Asia and North America. Agric. For. Entomol. 2016, 18, 182–188. [Google Scholar] [CrossRef]
  45. Allison, J.D.; ·Paine, T.D.; ·Slippers, B.; Wingfield, M.J. (Editors). Forest Entomology and Pathology. Volume 1: Entomology. Springer, 2023. 810 pp. ISBN 978-3-031-11552-3 ISBN 978-3-031-11553-0 (eBook). [CrossRef]
  46. Orlova-Bienkowskaja, M.J.; Bieńkowski, A.O. Modeling long-distance dispersal of emerald ash borer in European Russia and prognosis of spread of this pest to neighboring countries within next 5 years. Ecol. Evol. 2018, 8, 9295–9304. [Google Scholar] [CrossRef] [PubMed]
Preprints 98208 g001
Figure 1. Forest subcompartments containing European ash within the territory of Ukraine (specific emphasis on the Luhansk region— the location where the EAB first entered and underwent field survey).
Figure 1. Forest subcompartments containing European ash within the territory of Ukraine (specific emphasis on the Luhansk region— the location where the EAB first entered and underwent field survey).
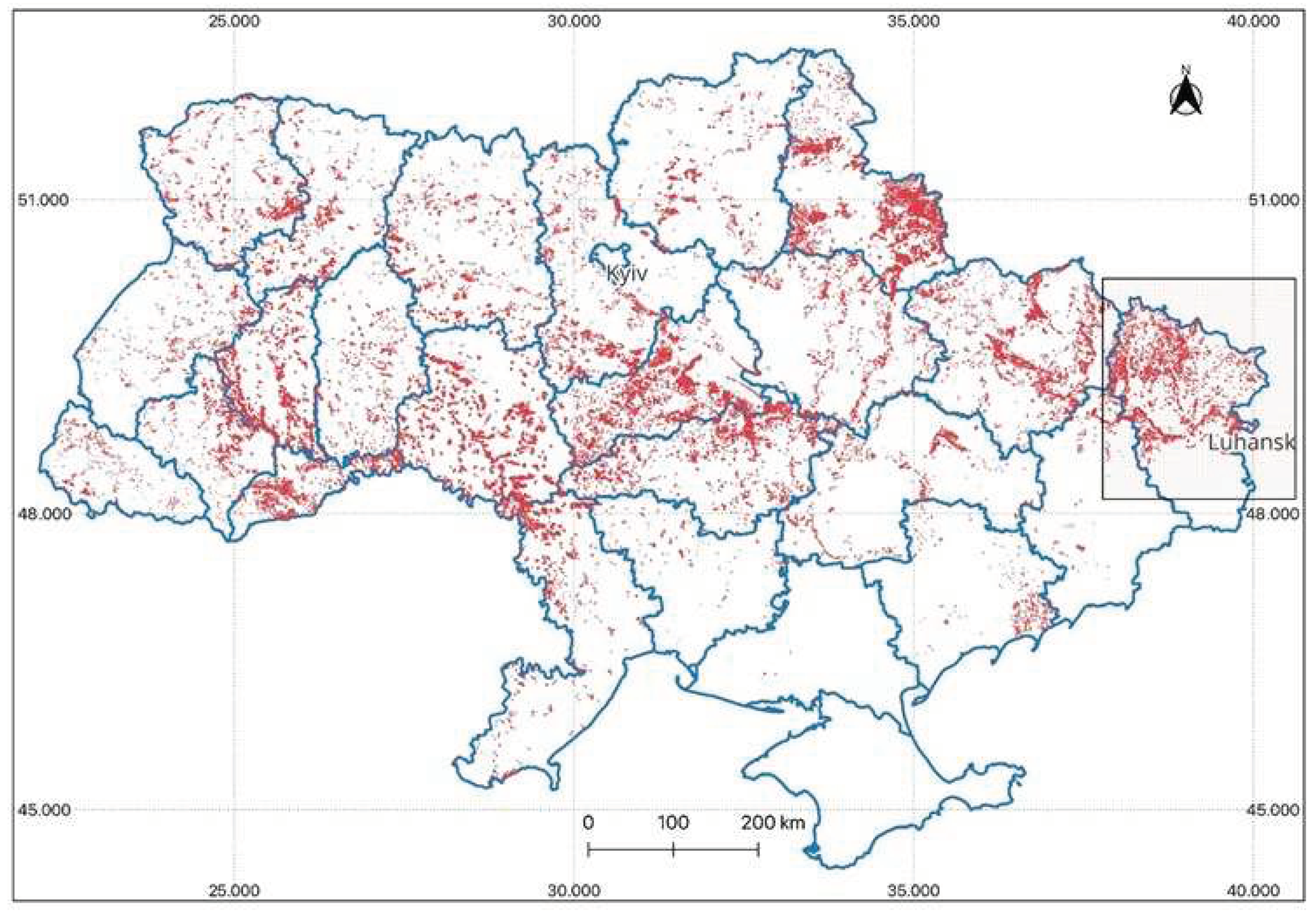
Preprints 98208 g001
Preprints 98208 g002
Figure 2. Outcome curves of variable «Age of trees, years» which holds significant value in constructing the spread model. Here, POP (predicted probability) of pest existence. The blue area illustrates the range of POP values when the variable changes. Curve (a) depicts how the POP shifted as the variable altered under standard sample conditions. The curve (b) demonstrates the dependence of POP both on the selected variable and on effects resulting from correlations between the selected variable and other elements.
Figure 2. Outcome curves of variable «Age of trees, years» which holds significant value in constructing the spread model. Here, POP (predicted probability) of pest existence. The blue area illustrates the range of POP values when the variable changes. Curve (a) depicts how the POP shifted as the variable altered under standard sample conditions. The curve (b) demonstrates the dependence of POP both on the selected variable and on effects resulting from correlations between the selected variable and other elements.
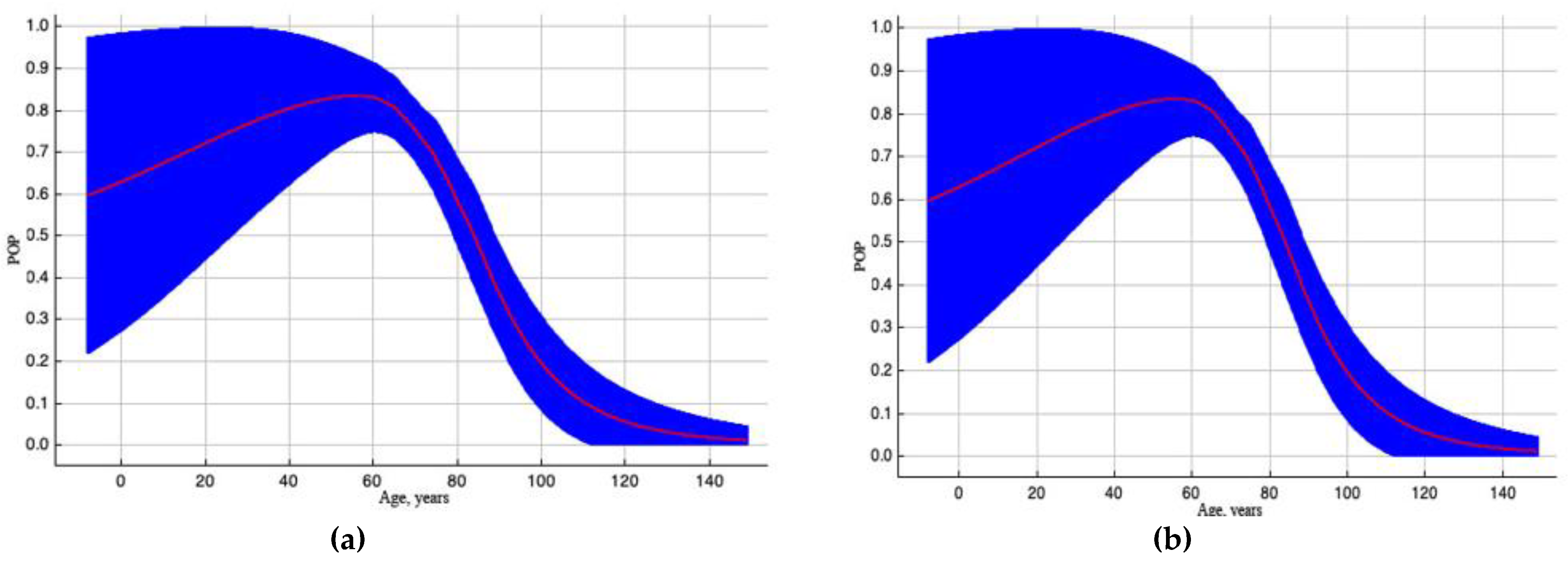
Preprints 98208 g002
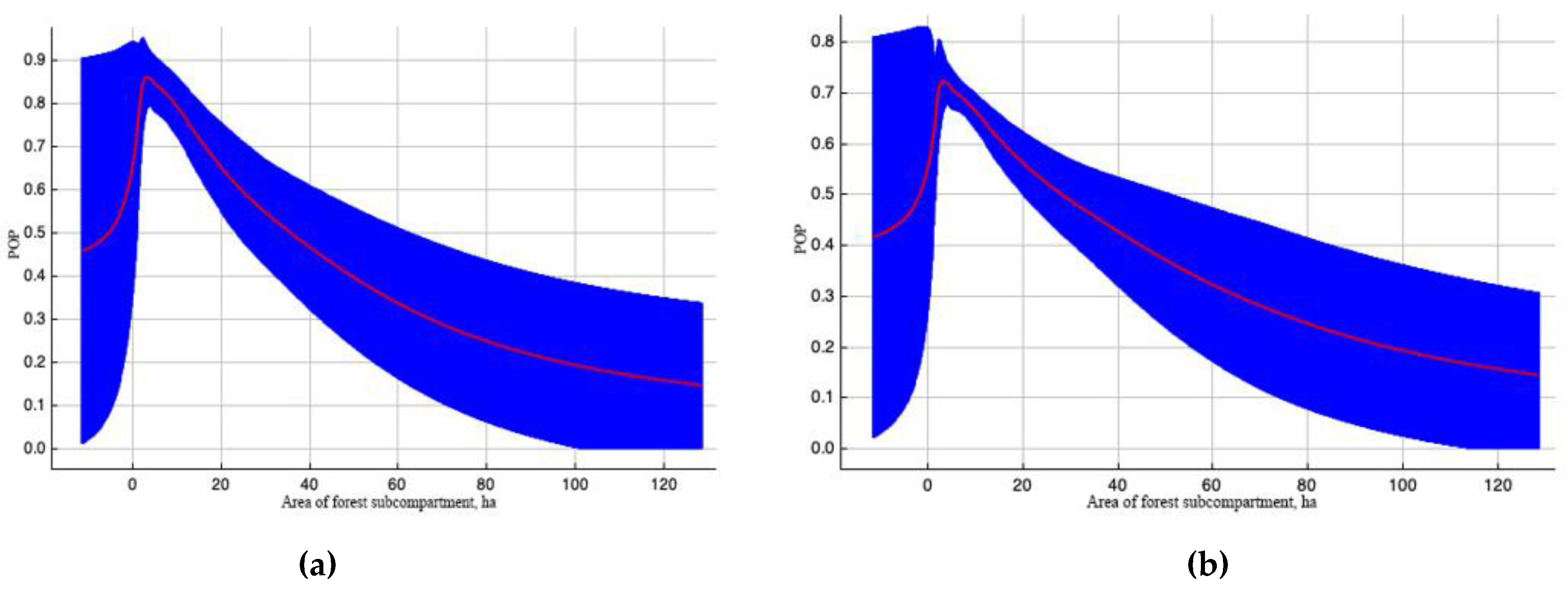
Preprints 98208 g003
Figure 3. Outcome curves of variable «Area of forest subcompartment, ha» with significant value in forming the EAB scale model. Here, POP (predicted probability) of pest existence. The blue area shows the range of POP values when the variable changes. Curve (a) depicts how the POP shifted as the variable altered under standard sample conditions. The curve (b) demonstrates the dependence of POP both on the selected variable and on effects resulting from correlations between the selected variable and other elements.
Figure 3. Outcome curves of variable «Area of forest subcompartment, ha» with significant value in forming the EAB scale model. Here, POP (predicted probability) of pest existence. The blue area shows the range of POP values when the variable changes. Curve (a) depicts how the POP shifted as the variable altered under standard sample conditions. The curve (b) demonstrates the dependence of POP both on the selected variable and on effects resulting from correlations between the selected variable and other elements.
Preprints 98208 g003
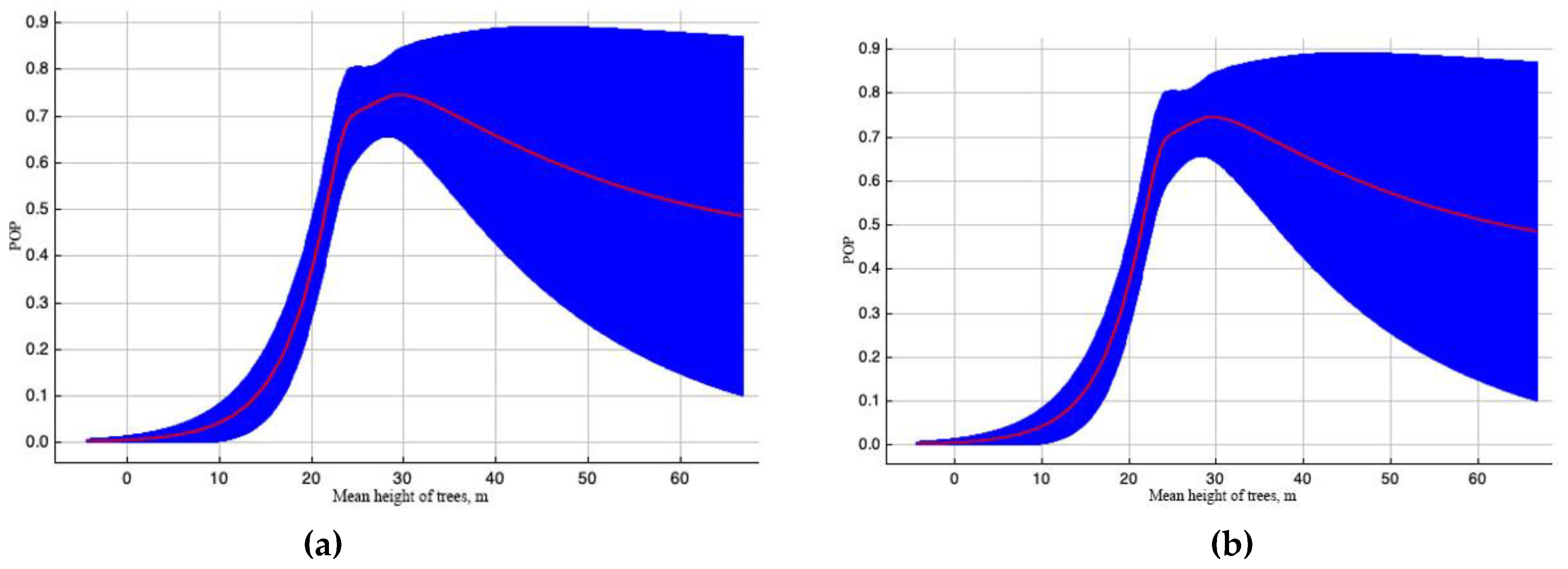
Preprints 98208 g004
Figure 4. Outcome curves of variable «Mean height of trees, m» with great value in constructing the EAB range model. Here, POP (predicted probability) of pest existence. The blue area shows the range of POP values when the variable changes. The curve (a) depicts how the POP shifted as the variable altered under standard sample conditions. The curve (b) demonstrates the dependence of POP both on the selected variable and on effects resulting from correlations between the selected variable and other elements.
Figure 4. Outcome curves of variable «Mean height of trees, m» with great value in constructing the EAB range model. Here, POP (predicted probability) of pest existence. The blue area shows the range of POP values when the variable changes. The curve (a) depicts how the POP shifted as the variable altered under standard sample conditions. The curve (b) demonstrates the dependence of POP both on the selected variable and on effects resulting from correlations between the selected variable and other elements.
Preprints 98208 g004
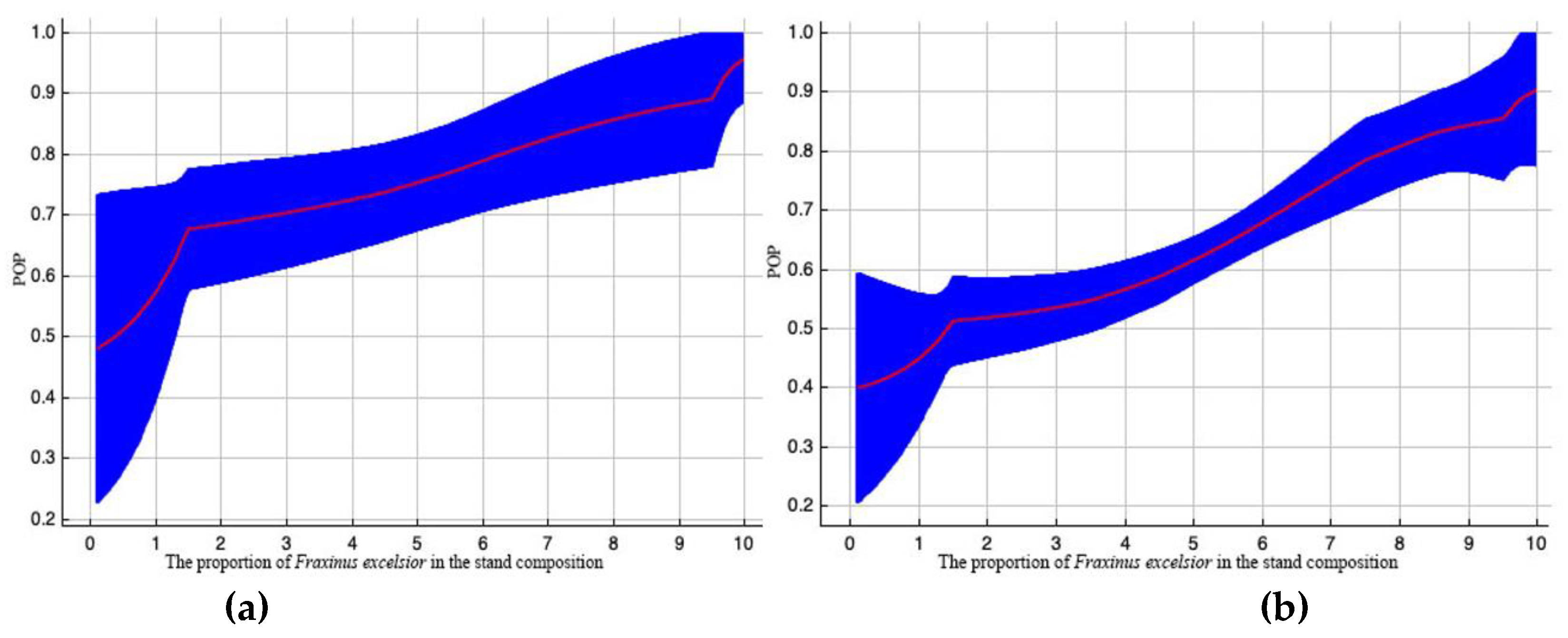
Preprints 98208 g005
Figure 5. Outcome curves of the variable «The proportion of Fraxinus excelsior in the stand composition» with significant value in constructing the EAB range model. Here, POP (predicted probability) of pest existence. The blue area demonstrates the distribution of POP values when the variable is altered. Curve (a) depicts how the POP shifted as the variable altered under standard sample conditions. The curve (b) shows the dependence of POP both on the selected variable and on and on effects resulting from correlations between the selected variable and other elements.
Figure 5. Outcome curves of the variable «The proportion of Fraxinus excelsior in the stand composition» with significant value in constructing the EAB range model. Here, POP (predicted probability) of pest existence. The blue area demonstrates the distribution of POP values when the variable is altered. Curve (a) depicts how the POP shifted as the variable altered under standard sample conditions. The curve (b) shows the dependence of POP both on the selected variable and on and on effects resulting from correlations between the selected variable and other elements.
Preprints 98208 g005
Preprints 98208 g006
Figure 6. Outcome curves of the variable «Hygrotope index (humidity level), point» with great value in constructing the EAB range model. Here, POP (predicted probability) of pest existence. The blue area shows the range of POP values when the variable alters. Curve (a) demonstrates how the POP shifted as the variable altered under standard sample conditions. Curve (b) depicts the dependence of POP both on the selected variable and on and on effects resulting from correlations between the selected variable and other elements.
Figure 6. Outcome curves of the variable «Hygrotope index (humidity level), point» with great value in constructing the EAB range model. Here, POP (predicted probability) of pest existence. The blue area shows the range of POP values when the variable alters. Curve (a) demonstrates how the POP shifted as the variable altered under standard sample conditions. Curve (b) depicts the dependence of POP both on the selected variable and on and on effects resulting from correlations between the selected variable and other elements.
Preprints 98208 g006
Preprints 98208 g007
Figure 7. The expected spreading area for the Emerald Ash Borer (EAB) was estimated through maximum entropy modeling. The segment with the maximum probability of EAB infestation has been enlarged. Dark dots denote points where the beetle was registered, while light dots indicate points where EAB was absent in 2021. The color of forest fragments corresponds to the predicted likelihood of EAB distribution in each forest subcompartment (see the legend).
Figure 7. The expected spreading area for the Emerald Ash Borer (EAB) was estimated through maximum entropy modeling. The segment with the maximum probability of EAB infestation has been enlarged. Dark dots denote points where the beetle was registered, while light dots indicate points where EAB was absent in 2021. The color of forest fragments corresponds to the predicted likelihood of EAB distribution in each forest subcompartment (see the legend).
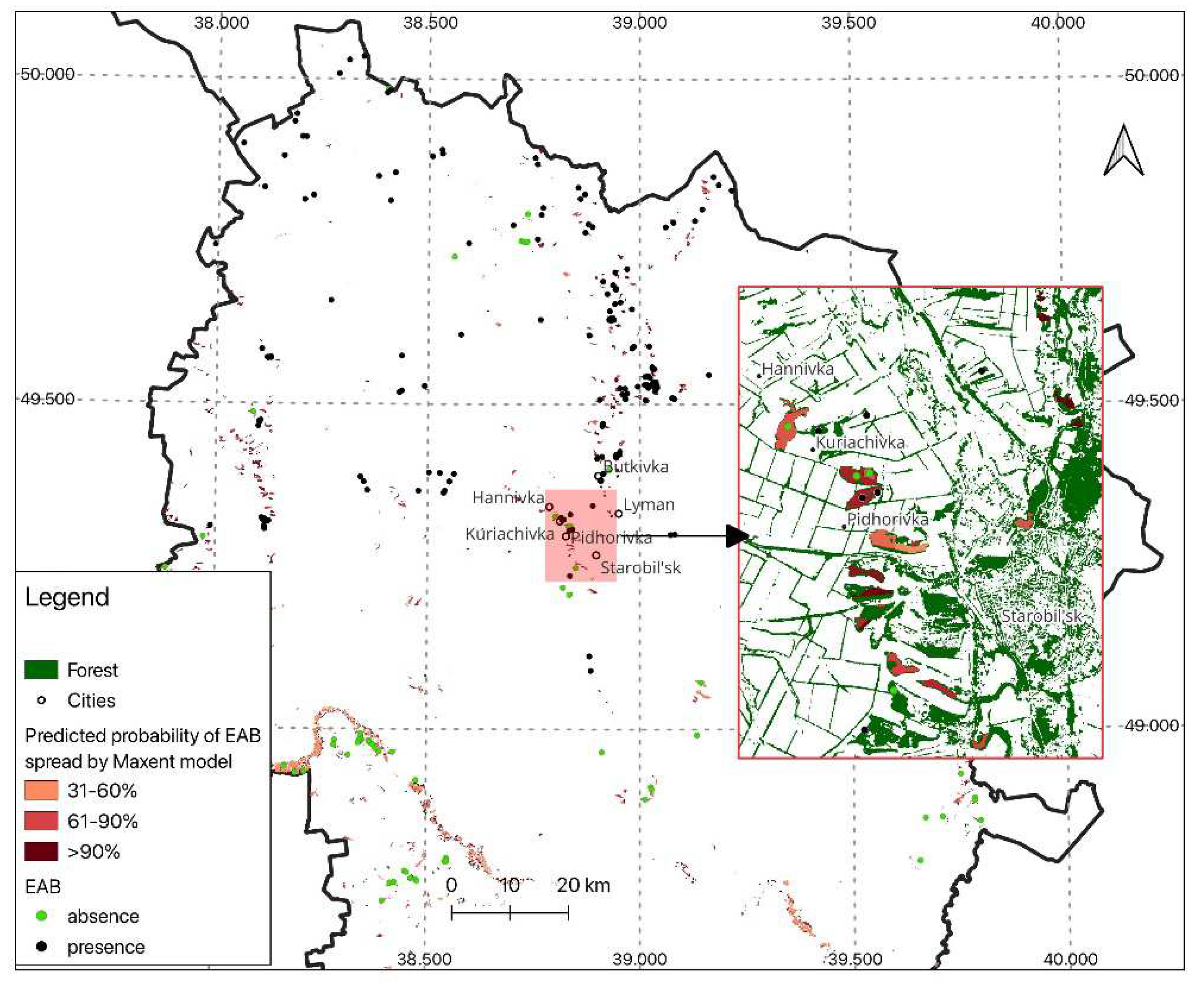
Preprints 98208 g007
Table 2. The MaxEnt probability of EAB existence is primarily influenced by variables, which are presented in descending order of significance.
Table 2. The MaxEnt probability of EAB existence is primarily influenced by variables, which are presented in descending order of significance.
Variables AUC Contribution, % Permutation, % Aggregated contribution, %
Age of trees, years 0.7 38.9 31.4 38.9
Area of forest subcompartment, ha 0.6 13.9 11.2 52.8
Mean height of trees, m 0.7 11.2 17.1 64.0
The proportion of Fraxinus excelsior in the stand composition, % 0.6 10.6 4.3 74.6
Hygrotope index (humidity level), point 0.6 8.3 13.5 82.9
Site index class, point 0.5 5.8 8.3 88.6
Number of non-forested lands neighboring subcompartment, point 0.6 5.3 3.6 93.9
Relative density of stocking, unit fraction 0.6 2.1 2.6 96.0
Mean diameter of trees, cm (DBH) 0.6 1.8 5.5 97.8
Trophotope index (soil richness level), point 0.5 1.4 1.0 99.1
The presence of any Fraxinus species in the stand composition, 1/0 0.6 0.9 1.4 100.0
Note: AUC (Area Under the Curve) is calculated for each variable, serving as a metric to assess the model's performance.
Table 3. Distribution of predicted probability of EAB presence by MaxEnt 3.4.4.
Table 3. Distribution of predicted probability of EAB presence by MaxEnt 3.4.4.
Probability Area, ha Proportion of area, % Cumulative
probability		 Cumulative
proportion, %
0–30 4007 25 0–30 25
31–60 3286 20 31–60 20
61–90 5691 35 31–90 55
>90 3280 20 >30 75
Total 16264 100 – 100
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content.
Copyright: This open access article is published under a Creative Commons CC BY 4.0 license, which permit the free download, distribution, and reuse, provided that the author and preprint are cited in any reuse.
Forest site and stand structure affecting the distribution of Emerald Ash Borer, Agrilus planipennis Fairmaire, 1888 (Coleoptera: Buprestidae) in Eastern Ukraine

Valentyna Meshkova

et al.

,

2024

Invasive Populations of Emerald Ash Borer Agrilus planipennis Fairmaire, 1888 (Coleoptera: Buprestidae) in Saint Petersburg, Russia: a “Hitchhiker”?

Andrey V. Selikhovkin

et al.

,

2021

Distribution of Insect in Protected Forest Area in Ambon City

fransina latumahina

,

2021

Prerpints.org logo

Preprints.org is a free preprint server supported by MDPI in Basel, Switzerland.

facebook logotwitter logolinkedin logo
MDPI Initiatives
Important Links
Subscribe

Disclaimer

Terms of Use

Privacy Policy

© 2024 MDPI (Basel, Switzerland) unless otherwise stated