Preprint
Article
Quinn’s Law of Fluid Dynamics Pressure-Driven Fluid Flow through Closed Conduits
Altmetrics
Downloads
842
Views
493
Comments
0
This version is not peer-reviewed
Submitted:
16 October 2019
Posted:
18 October 2019
You are already at the latest version
Alerts
Abstract
In this paper we develop from first principles a unique law pertaining to the flow of fluids through closed conduits. This law, which we call “Quinn’s Law”, may be described as follows: When fluids are forced to flow through closed conduits under the driving force of a pressure gradient, there is a linear relationship between the normalized dimensionless pressure gradient, PQ, and the normalized dimensionless fluid current, CQ. The relationship is expressed mathematically as: PQ = k1 +k2CQ. This linear relationship remains the same whether the conduit is filled with or devoid of solid obstacles. The law differentiates, however, between a packed and an empty conduit by virtue of the tortuosity of the fluid path, which is seamlessly accommodated within the normalization framework of the law itself. When movement of the fluid is very close to being at rest, i.e., very slow, this relationship has the unique minimum constant value of k1, and as the fluid acceleration increases, it varies with a slope of k2 as a function of normalized fluid current. Quinn’s Law is validated herein by applying it to the data from published classical studies of measured permeability in both packed and empty conduits, as well as to the data generated by home grown experiments performed in the author’s own laboratory.
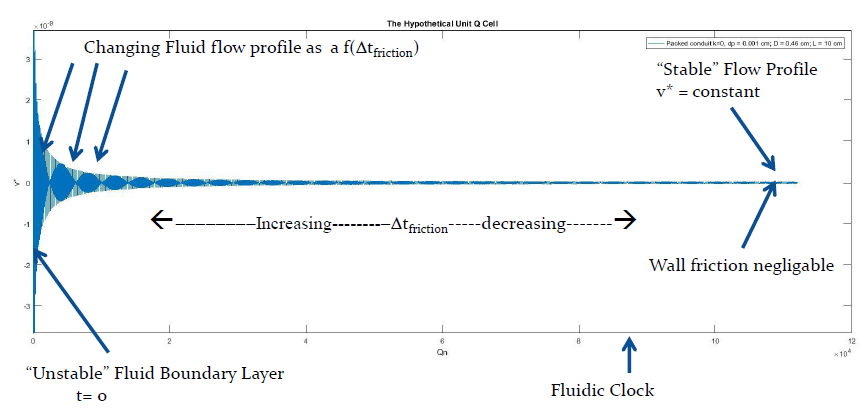
Keywords:
Subject: Physical Sciences - Fluids and Plasmas Physics
Copyright: This open access article is published under a Creative Commons CC BY 4.0 license, which permit the free download, distribution, and reuse, provided that the author and preprint are cited in any reuse.
MDPI Initiatives
Important Links
© 2024 MDPI (Basel, Switzerland) unless otherwise stated




