A Proof of the Riemann Hypothesis Based on a New Expression of the Completed Zeta Function

Preprint

Article

A Proof of the Riemann Hypothesis Based on a New Expression of the Completed Zeta Function

This version is not peer-reviewed.

Weicun Zhang^*

This version is not peer-reviewed.

Altmetrics

Downloads

8306

Views

72334

Comments

Submitted:

04 November 2024

Posted:

04 November 2024

Read the latest preprint version here

Abstract

Based on the Hadamard product $\xi(s)= \xi(0)\prod_{\rho}(1-\frac{s}{\rho})$, a new expression of $\xi(s)$ is obtained by paring $\rho$ and $\bar{\rho}$ $$\xi(s)= \xi(0)\prod_{i=1}^{\infty}(1-\frac{s}{\rho_i})(1-\frac{s}{\bar{\rho}_i})=\xi(0)\prod_{i=1}^{\infty}\Big{(}\frac{\beta_i^2}{\alpha_i^2+\beta_i^2}+\frac{(s-\alpha_i)^2}{\alpha_i^2+\beta_i^2}\Big{)}$$ where $\xi(0)=\frac{1}{2}$, $\rho_i=\alpha_i+j\beta_i$ and $\bar{\rho}_i=\alpha_i-j\beta_i$ are the complex conjugate zeros of $\xi(s)$, $0<\alpha_i<1$ and $\beta_i\neq 0$ are real numbers, $\beta_i$ are in order of increasing $|\beta_i|$, i.e., $|\beta_1|\leq|\beta_2|\leq|\beta_3|\leq \cdots$.\\ Then, by the functional equation $\xi(s)=\xi(1-s)$, we have $$\xi(0)\prod_{i=1}^{\infty}\Big{(}\frac{\beta_i^2}{\alpha_i^2+\beta_i^2}+\frac{(s-\alpha_i)^2}{\alpha_i^2+\beta_i^2}\Big{)} =\xi(0)\prod_{i=1}^{\infty}\Big{(}\frac{\beta_i^2}{\alpha_i^2+\beta_i^2}+\frac{(1-s-\alpha_i)^2}{\alpha_i^2+\beta_i^2}\Big{)}$$ i.e., $$\prod_{i=1}^{\infty}\Big{(}1+\frac{(s-\alpha_i)^2}{\beta_i^2}\Big{)}=\prod_{i=1}^{\infty}\Big{(}1+\frac{(1-s-\alpha_i)^2}{\beta_i^2}\Big{)}$$ which, by Lemma 3, is equivalent to $$\alpha_i= \frac{1}{2}, i =1, 2, 3, \cdots, \infty$$ Thus, we conclude that the Riemann Hypothesis is true.

Keywords:

Subject:

Computer Science and Mathematics - Signal Processing

This preprints belongs to the Collection

Preprints.org 2023 Most Popular Preprints Award Winner Collection

1. Introduction

The Riemann Hypothesis [1] is one of the most important unsolved problems in mathematics. Although many efforts and achievements have been made towards proving this celebrated hypothesis, it still remains an open problem [2,3]. The Riemann zeta function is originally defined in the half-plane

ℜ (s) > 1

by the absolutely convergent series [2]

ζ (s) = \sum_{n = 1}^{\infty} \frac{1}{n^{s}}, ℜ (s) > 1

(1)

The connection between the above-defined Riemann zeta function and prime numbers was discovered by Euler, i.e., the famous Euler product

ζ (s) = \sum_{n = 1}^{\infty} \frac{1}{n^{s}} = \prod_{p} {(1 - p^{- s})}^{- 1}, ℜ (s) > 1

(2)

where p runs over the prime numbers.

Riemann showed in his paper in 1859 how to extend the zeta function to the whole complex plane

C

by analytic continuation, i.e.

ζ (s) = \frac{Γ (1 - s)}{2 π i} \int_{\infty}^{\infty} \frac{{(- x)}^{s}}{e^{x} - 1} \cdot \frac{d x}{x}

(3a)

where “

\int_{\infty}^{\infty}

” is the symbol adopted by Riemann to represent the contour integral from

+ \infty

+ \infty

around a domain which includes the value 0 but no other point of discontinuity of the integrand in its interior.

Or equivalently,

ζ (s) = \frac{π^{s / 2}}{Γ (s / 2)} {\frac{1}{s (s - 1)} + \int_{1}^{\infty} (x^{\frac{s}{2} - 1} + x^{- \frac{s}{2} - \frac{1}{2}}) \cdot (\frac{θ (x) - 1}{2}) d x}

(3b)

where

θ (x) = \sum_{- \infty}^{\infty} e^{- n^{2} π x}

is the Jaccobi theta function,

Γ

is the Gamma function in the following Weierstrass expression

\frac{1}{Γ (s)} = s \cdot e^{γ s} \prod_{n = 1}^{\infty} (1 + \frac{s}{n}) e^{- s / n}

(4)

where

γ

is the Euler-Mascheroni constant.

As shown by Riemann,

ζ (s)

extends to

C

as a meromorphic function with only a simple pole at

s = 1

, with residue 1, and satisfies the following functional equation

π^{- \frac{s}{2}} Γ (\frac{s}{2}) ζ (s) = π^{- \frac{1 - s}{2}} Γ (\frac{1 - s}{2}) ζ (1 - s)

(5)

The Riemann zeta function

ζ (s)

has zeros at the negative even integers:

- 2

- 4

- 6

- 8

, ⋯ and one refers to them as the trivial zeros. The other zeros of

ζ (s)

are the complex numbers, i.e., non-trivial zeros [2].

In 1896, Hadamard [4] and Poussin [5] independently proved that no zeros could lie on the line

ℜ (s) = 1

, together with the functional equation

ξ (s) = ξ (1 - s)

and the fact that there are no zeros with real part greater than 1, this showed that all non-trivial zeros must lie in the interior of the critical strip

0 < ℜ (s) < 1

. Later on, Hardy (1914) [6], Hardy and Littlewood (1921) [7] showed that there are infinitely many zeros on the critical line

ℜ (s) = \frac{1}{2}

To give a summary of the related research on the RH, we have the following results on the properties of the non-trivial zeros of

ζ (s)

[4,5,6,7,8,9].

Lemma 1: Non-trivial zeroes of

ζ (s)

, noted as

ρ = α + j β

, have the following properties

1): The number of non-trivial zeroes is infinity;
2): $β \neq 0$ ;
3): $0 < α < 1$ ;
4): $ρ, \bar{ρ}, 1 - \bar{ρ}, 1 - ρ$ are all non-trivial zeroes.

As further study, a completed zeta function

ξ (s)

is defined as

ξ (s) = \frac{1}{2} s (s - 1) π^{- \frac{s}{2}} Γ (\frac{s}{2}) ζ (s)

(6)

It is well-known that

ξ (s)

is an entire function of order 1. This implies

ξ (s)

is analytic, and can be expressed as infinite polynomial, in the whole complex plane

C

. In addition, replacing s with

1 - s

in Eq.(6), and combining Eq.(5), we obtain the following functional equation

ξ (s) = ξ (1 - s)

(7)

Considering the definition of

ξ (s)

, and recalling Eq.(4), the trivial zeros of

ζ (s)

are canceled by the poles of

Γ (\frac{s}{2})

. The zero of

s - 1

and the pole of

ζ (s)

cancel; the zero

s = 0

and the pole of

Γ (\frac{s}{2})

cancel [9,10]. Thus, all the zeros of

ξ (s)

are exactly the nontrivial zeros of

ζ (s)

. Then we have the following Lemma 2.

Lemma 2: The zeros of

ξ (s)

coincide with the non-trivial zeros of

ζ (s)

Accordingly, the following two statements of the RH are equivalent.

Statement 1: All the non-trivial zeros of

ζ (s)

have real part equal to

\frac{1}{2}

Statement 2: All the zeros of

ξ (s)

have real part equal to

\frac{1}{2}

To prove the RH, a natural thinking is to estimate the numbers of non-trivial zeros of

ζ (s)

inside or outside some certain areas according to Argument Principle. Along this train of thought, there are many research works. Let

N (T)

denote the number of non-trivial zeros of

ζ (s)

inside the rectangle:

0 < α < 1, 0 < β \leq T

, and let

N_{0} (T)

denote the number of non-trivial zeros of

ζ (s)

on the line

α = \frac{1}{2}, 0 < β \leq T

. Selberg proved that there exist positive constants c and

T_{0}

, such that

N_{0} (T) > c N (T), (T > T_{0})

[11], later on, Levinson proved that

c \geq \frac{1}{3}

[12], Lou and Yao proved that

c \geq 0.3484

[13], Conrey proved that

c \geq \frac{2}{5}

[14], Bui, Conrey and Young proved that

c \geq 0.41

[15], Feng proved that

c \geq 0.4128

[16], Wu proved that

c \geq 0.4172

[17].

On the other hand, many non-trivial zeros have been calculated by hand or by computer programs. Among others, Riemann found the first three non-trivial zeros [18]. Gram found the first 15 zeros based on Euler-Maclaurin summation [19]. Titchmarsh calculated the 138^th to 195^th zeros using the Riemann-Siegel formula [20,21]. Here are the first three (pairs of) non-trivial zeros:

\frac{1}{2} \pm j 14.1347251; \frac{1}{2} \pm j 21.0220396; \frac{1}{2} \pm j 25.0108575

The idea of this paper is originated from Euler’s work on proving the following famous equality

1 + \frac{1}{2^{2}} + \frac{1}{3^{2}} + \frac{1}{4^{2}} + \frac{1}{5^{2}} + \dots = \frac{π^{2}}{6}

(8)

This interesting result is deduced by comparing the like terms of two types of infinite expressions, i.e., infinite polynomial and infinite product, as shown in the following

\begin{matrix} \frac{sin x}{x} & = 1 - \frac{x^{2}}{3!} + \frac{x^{4}}{5!} - \frac{x^{6}}{7!} + \dots = (1 - \frac{x^{2}}{π^{2}}) (1 - \frac{x^{2}}{4 π^{2}}) (1 - \frac{x^{2}}{9 π^{2}}) \dots \end{matrix}

(9)

Then the author of this paper conjectured that

ξ (s)

should be factored into

(1 + \frac{{(s - α_{i})}^{2}}{β_{i}^{2}})

or something like that, which was verified by paring

ρ_{i}

and

{\bar{ρ}}_{i}

in the Hadamard product of

ξ (s)

, i.e.

(1 - \frac{s}{ρ_{i}}) (1 - \frac{s}{{\bar{ρ}}_{i}}) = \frac{β_{i}^{2}}{α_{i}^{2} + β_{i}^{2}} (1 + \frac{{(s - α_{i})}^{2}}{β_{i}^{2}})

The Hadamard product of

ξ (s)

as shown in Eq.(10) was first proposed by Riemann, however, it was Hadamard who showed the validity of this infinite product expansion [22].

ξ (s) = ξ (0) \prod_{ρ} (1 - \frac{s}{ρ})

(10)

where

ξ (0) = \frac{1}{2}

ρ

runs over all zeros of the completed zeta function

ξ (s)

Hadamard pointed out that to ensure the absolute convergence of the infinite product expansion,

ρ

and

1 - ρ

are paired. Later in Section 3, we will show that

ρ

and

\bar{ρ}

can also be paired to ensure the absolute convergence of the infinite product expansion.

2. Lemmas

In this section, we first explain the concept of the real multiplicity of a zero of

ξ (s)

. And then we prove Lemma 3 to support the proof of the RH.

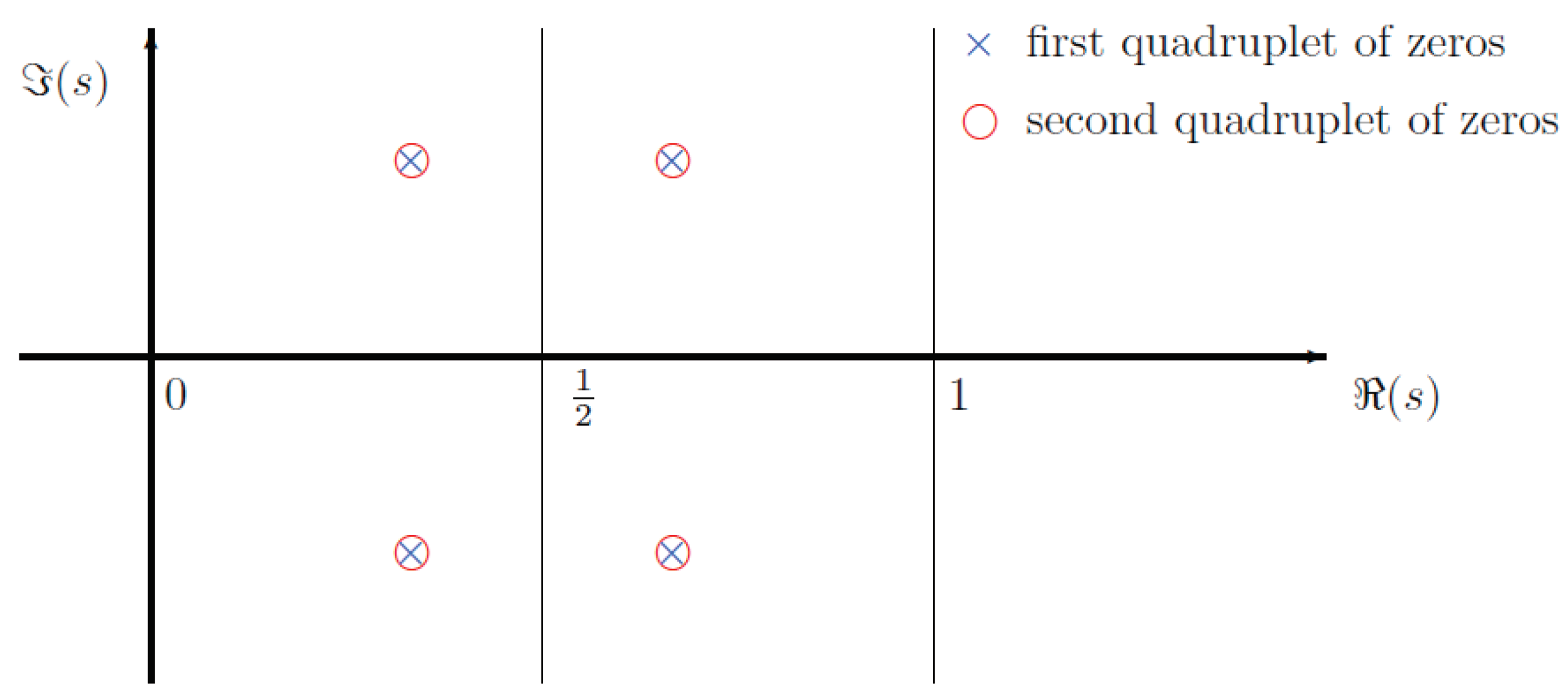
Multiple zeros of $ξ (s)$ and their real multiplicities: As shown in Figure 1, the multiple zeros of

ξ (s)

are defined in terms of the quadruplet, i.e.,

ρ, \bar{ρ}, 1 - ρ, 1 - \bar{ρ}

There are two different expressions of factors of

ξ (s) / ξ (1 - s)

for the multiple zeros in Figure 1, respectively, i.e.,

(1 + \frac{{(s - α_{1})}^{2}}{β_{1}^{2}})^{2} / (1 + \frac{{(1 - s - α_{1})}^{2}}{β_{1}^{2}})^{2}

, or

(1 + \frac{{(s - α_{1})}^{2}}{β_{1}^{2}}) (1 + \frac{{(s - α_{2})}^{2}}{β_{2}^{2}}) / (1 + \frac{{(1 - s - α_{1})}^{2}}{β_{1}^{2}}) (1 + \frac{{(1 - s - α_{2})}^{2}}{β_{2}^{2}})

with

α_{1} + α_{2} = 1, β_{1}^{2} = β_{2}^{2}

To exclude the latter expression, we stipulate that zero

ρ_{i}

related factors of

ξ (s) / ξ (1 - s)

take the unique form of

(1 + \frac{{(s - α_{i})}^{2}}{β_{i}^{2}})^{m_{i}} / (1 + \frac{{(1 - s - α_{i})}^{2}}{β_{i}^{2}})^{m_{i}}

, where

m_{i} \geq 1

is the real multiplicity of

ρ_{i}

, here “real” means unique and unchangeable. In Figure 1, the real multiplicity of

ρ_{1}

is 2, i.e.,

m_{1} = 2

Remark: Although the real multiplicity

m_{i}

of zero

ρ_{i}

is unknown, it is an objective existence, unique, and unchangeable. This is the key point in the proof of Lemma 3.

Lemma 3: Given two absolutely convergent infinite products

f (s) = \prod_{i = 1}^{\infty} (1 + \frac{{(s - α_{i})}^{2}}{β_{i}^{2}})^{m_{i}}

(11)

and

f (1 - s) = \prod_{i = 1}^{\infty} (1 + \frac{{(1 - s - α_{i})}^{2}}{β_{i}^{2}})^{m_{i}}

(12)

where s is a complex variable,

ρ_{i} = α_{i} + j β_{i}

and

{\bar{ρ}}_{i} = α_{i} - j β_{i}

are the complex conjugate zeros of

ξ (s)

0 < α_{i} < 1

and

β_{i} \neq 0

are real numbers,

m_{i} \geq 1

is the real multiplicity of

ρ_{i}

0 < | β_{1} | \leq | β_{2} | \leq | β_{3} | \leq \dots

Then we have

f (s) = f (1 - s) \Leftrightarrow \{\begin{matrix} α_{i} = \frac{1}{2} \\ 0 < | β_{1} | < | β_{2} | < | β_{3} | < \dots \\ i = 1, 2, 3, \dots, \infty \end{matrix}

(13)

where “

\Leftrightarrow

” is the equivalent sign.

Proof: First of all, we have the following fact:

(1 + \frac{{(s - α)}^{2}}{β^{2}})^{m} = (1 + \frac{{(1 - s - α)}^{2}}{β^{2}})^{m} \Leftrightarrow {(s - α)}^{2} = {(1 - s - α)}^{2} \Leftrightarrow α = \frac{1}{2}

(14)

where

m \geq 1

is positive integer,

α \neq 0

and

β \neq 0

are real numbers.

Next, the proof is based on the divisibility of infinite products with reference to the divisibility of polynomials. It is obvious that

\begin{matrix} f (s) = f (1 - s) & \Leftrightarrow \prod_{i = 1}^{\infty} (1 + \frac{{(s - α_{i})}^{2}}{β_{i}^{2}})^{m_{i}} = \prod_{i = 1}^{\infty} (1 + \frac{{(1 - s - α_{i})}^{2}}{β_{i}^{2}})^{m_{i}} \\ \Leftrightarrow (1 + \frac{{(s - α_{l})}^{2}}{β_{l}^{2}})^{m_{l}} f_{l} (s) = (1 + \frac{{(1 - s - α_{l})}^{2}}{β_{l}^{2}})^{m_{l}} f_{l} (1 - s) \end{matrix}

(15)

where

f_{l} (s) = \prod_{\begin{matrix} i \in I ∖ {l} \end{matrix}} (1 + \frac{{(s - α_{i})}^{2}}{β_{i}^{2}})^{m_{i}}

(16)

f_{l} (1 - s) = \prod_{\begin{matrix} i \in I ∖ {l} \end{matrix}} (1 + \frac{{(1 - s - α_{i})}^{2}}{β_{i}^{2}})^{m_{i}}

(17)

with

I = {1, 2, 3, \dots, \infty}

, and “l” is an arbitrary element of set

I

. In brief,

i \in I ∖ {l}

means that i runs over the elements of

I

excluding “l”.

Then we have

\begin{matrix} (1 + \frac{{(s - α_{l})}^{2}}{β_{l}^{2}})^{m_{l}} f_{l} (s) = (1 + \frac{{(1 - s - α_{l})}^{2}}{β_{l}^{2}})^{m_{l}} f_{l} (1 - s) \\ \Rightarrow \\ \{\begin{matrix} (1 + \frac{{(s - α_{l})}^{2}}{β_{l}^{2}})^{m_{l}} | (1 + \frac{{(1 - s - α_{l})}^{2}}{β_{l}^{2}})^{m_{l}} f_{l} (1 - s) \\ (1 + \frac{{(1 - s - α_{l})}^{2}}{β_{l}^{2}})^{m_{l}} | (1 + \frac{{(s - α_{l})}^{2}}{β_{l}^{2}})^{m_{l}} f_{l} (s) \end{matrix} \end{matrix}

(18)

where “|” is the divisible sign.

Next, we exclude the possibility of

(1 + \frac{{(s - α_{l})}^{2}}{β_{l}^{2}})^{m_{l}} | f_{l} (1 - s)

and

(1 + \frac{{(1 - s - α_{l})}^{2}}{β_{l}^{2}})^{m_{l}} | f_{l} (s)

in Eq.(18) with the help of the real multiplicities of zeros of

ξ (s)

Considering

(1 + \frac{{(s - α_{l})}^{2}}{β_{l}^{2}}), 0 < α_{l} < 1, β_{l} \neq 0

, is irreducible over the field R of real numbers, we know that

\begin{matrix} (1 + \frac{{(s - α_{l})}^{2}}{β_{l}^{2}})^{m_{l}} | f_{l} (1 - s) \Rightarrow (1 + \frac{{(s - α_{l})}^{2}}{β_{l}^{2}}) | f_{l} (1 - s) \\ \Rightarrow (by Lemma 6) \\ (1 + \frac{{(s - α_{l})}^{2}}{β_{l}^{2}}) | (1 + \frac{{(1 - s - α_{i})}^{2}}{β_{i}^{2}}), i \neq l \\ \Rightarrow (1 + \frac{{(1 - s - α_{i})}^{2}}{β_{i}^{2}}) = k (1 + \frac{{(s - α_{l})}^{2}}{β_{l}^{2}}), i \neq l \\ \Rightarrow (by comparing the like terms in the above polynomial equation) \\ α_{i} + α_{l} = 1, β_{i}^{2} = β_{l}^{2}, k = 1, i \neq l \end{matrix}

Similarly,

\begin{matrix} (1 + \frac{{(1 - s - α_{l})}^{2}}{β_{l}^{2}})^{m_{l}} | f_{l} (s) \Rightarrow (1 + \frac{{(1 - s - α_{l})}^{2}}{β_{l}^{2}}) | f_{l} (s) \\ \Rightarrow (by Lemma 6) \\ (1 + \frac{{(1 - s - α_{l})}^{2}}{β_{l}^{2}}) | (1 + \frac{{(s - α_{i})}^{2}}{β_{i}^{2}}), i \neq l \\ \Rightarrow (1 + \frac{{(s - α_{i})}^{2}}{β_{i}^{2}}) = k (1 + \frac{{(1 - s - α_{l})}^{2}}{β_{l}^{2}}), i \neq l \\ \Rightarrow (by comparing the like terms in the above polynomial equation) \\ α_{i} + α_{l} = 1, β_{i}^{2} = β_{l}^{2}, k = 1, i \neq l \end{matrix}

As explained in the situation of Figure 1,

α_{i} + α_{l} = 1, β_{i}^{2} = β_{l}^{2}, i \neq l

means that

α_{i} \pm j β_{i}

and

α_{l} \pm j β_{l}

are the same zeros in terms of quadruplet, i.e.,

ρ, \bar{ρ}, 1 - ρ

, and

1 - \bar{ρ}

, which contradicts the definition of real multiplicities of zeros of

ξ (s)

Thus, in order to keep the real multiplicities of zeros of

ξ (s)

unchanged,

(1 + \frac{{(s - α_{l})}^{2}}{β_{l}^{2}})^{m_{l}}

can not divide

f_{l} (1 - s)

(1 + \frac{{(1 - s - α_{l})}^{2}}{β_{l}^{2}})^{m_{l}}

can not divides

f_{l} (s)

. In addition,

(1 + \frac{{(s - α_{l})}^{2}}{β_{l}^{2}})

is irreducible, then we know that

(1 + \frac{{(s - α_{l})}^{2}}{β_{l}^{2}})^{m_{l}}

and

f_{l} (1 - s)

are relatively prime,

(1 + \frac{{(1 - s - α_{l})}^{2}}{β_{l}^{2}})^{m_{l}}

and

f_{l} (s)

are relatively prime. Consequently, by Lemma 7, we obtain from Eq.(18) the following result.

\begin{matrix} (1 + \frac{{(s - α_{l})}^{2}}{β_{l}^{2}})^{m_{l}} f_{l} (s) = (1 + \frac{{(1 - s - α_{l})}^{2}}{β_{l}^{2}})^{m_{l}} f_{l} (1 - s) \\ \Rightarrow \\ (1 + \frac{{(s - α_{l})}^{2}}{β_{l}^{2}})^{m_{l}} | (1 + \frac{{(1 - s - α_{l})}^{2}}{β_{l}^{2}})^{m_{l}} \\ (1 + \frac{{(1 - s - α_{l})}^{2}}{β_{l}^{2}})^{m_{l}} | (1 + \frac{{(s - α_{l})}^{2}}{β_{l}^{2}})^{m_{l}} \\ \Rightarrow \\ (1 + \frac{{(s - α_{l})}^{2}}{β_{l}^{2}})^{m_{l}} = k (1 + \frac{{(1 - s - α_{l})}^{2}}{β_{l}^{2}})^{m_{l}} \\ \Rightarrow (k = 1, by comparing the highest - order terms in the above polynomial equation) \\ (1 + \frac{{(s - α_{l})}^{2}}{β_{l}^{2}})^{m_{l}} = (1 + \frac{{(1 - s - α_{l})}^{2}}{β_{l}^{2}})^{m_{l}} \\ \Rightarrow (by Eq . (14)) \\ α_{l} = \frac{1}{2} \end{matrix}

(19)

Let l run over from 1 to ∞, and repeat the above process, we get

\begin{matrix} \prod_{i = 1}^{\infty} (1 + \frac{{(s - α_{i})}^{2}}{β_{i}^{2}})^{m_{i}} = \prod_{i = 1}^{\infty} (1 + \frac{{(1 - s - α_{i})}^{2}}{β_{i}^{2}})^{m_{i}} \\ \Rightarrow \\ (1 + \frac{{(s - α_{i})}^{2}}{β_{i}^{2}})^{m_{i}} = (1 + \frac{{(1 - s - α_{i})}^{2}}{β_{i}^{2}})^{m_{i}} \\ \Rightarrow \\ α_{i} = \frac{1}{2}, i = 1, 2, 3, \dots, \infty \end{matrix}

(20)

Also, we have the following obvious fact

\begin{matrix} α_{i} = \frac{1}{2}, i = 1, 2, 3, \dots, \infty \\ \Rightarrow \\ (1 + \frac{{(s - α_{i})}^{2}}{β_{i}^{2}})^{m_{i}} = (1 + \frac{{(1 - s - α_{i})}^{2}}{β_{i}^{2}})^{m_{i}} \\ \Rightarrow \\ \prod_{i = 1}^{\infty} (1 + \frac{{(s - α_{i})}^{2}}{β_{i}^{2}})^{m_{i}} = \prod_{i = 1}^{\infty} (1 + \frac{{(1 - s - α_{i})}^{2}}{β_{i}^{2}})^{m_{i}} \end{matrix}

(21)

Further, limiting the imaginary parts

β_{i}

of zeros to

0 < | β_{1} | < | β_{2} | < | β_{3} | < \dots

in order to keep the real multiplicities of zeros unchanged, we finally get

\begin{matrix} \prod_{i = 1}^{\infty} (1 + \frac{{(s - α_{i})}^{2}}{β_{i}^{2}})^{m_{i}} = \prod_{i = 1}^{\infty} (1 + \frac{{(1 - s - α_{i})}^{2}}{β_{i}^{2}})^{m_{i}} \\ \Leftrightarrow \\ \{\begin{matrix} (1 + \frac{{(s - α_{i})}^{2}}{β_{i}^{2}})^{m_{i}} = (1 + \frac{{(1 - s - α_{i})}^{2}}{β_{i}^{2}})^{m_{i}} \\ 0 < | β_{1} | < | β_{2} | < | β_{3} | < \dots \\ i = 1, 2, 3, \dots, \infty \end{matrix} \\ \Leftrightarrow \\ \{\begin{matrix} α_{i} = \frac{1}{2} \\ 0 < | β_{1} | < | β_{2} | < | β_{3} | < \dots \\ i = 1, 2, 3, \dots, \infty \end{matrix} \end{matrix}

i.e.,

f (s) = f (1 - s) \Leftrightarrow \{\begin{matrix} α_{i} = \frac{1}{2} \\ 0 < | β_{1} | < | β_{2} | < | β_{3} | < \dots \\ i = 1, 2, 3, \dots, \infty \end{matrix}

That completes the proof of Lemma 3.

To support the proof of Lemma 3, we need the following classical results (Lemma 4 and Lemma 5) in Polynomial Algebra over Fields, with extension to infinite product (Lemma 6 and Lemma 7).

Lemma 4: Let F be a field,

m (x), g_{1} (x), . . ., g_{n} (x) \in F [x], n \geq 2

. If

m (x)

is irreducible (prime) and divides the product

g_{1} (x) \dots g_{n} (x)

, then

m (x)

divides one of the polynomials

g_{1} (x), . . ., g_{n} (x)

Lemma 5: Let F be a field,

f (x), m (x) \in F [x]

. If

m (x)

is irreducible and

f (x)

is any polynomial, then either

m (x)

divides

f (x)

g c d (m, f) = 1

, (gcd: greatest common divisor).

Lemma 6: Let F be a field,

m (x), g_{1} (x), . . ., g_{\infty} (x) \in F [x]

. If

m (x)

is irreducible and divides the product

g_{1} (x) \dots g_{\infty} (x)

, then

m (x)

divides one of the polynomials

g_{1} (x), . . ., g_{\infty} (x)

Lemma 7: Let F be a field,

p_{1} (x), . . ., p_{\infty} (x), q (x), m (x) \in F [x], p (x) = p_{1} (x) \dots p_{\infty} (x)

. If

m (x)

is irreducible and divides the product

p (x) q (x)

, but

m (x)

and

p (x)

are relatively prime, then

m (x)

divides

q (x)

Remark:

F [x]

is defined as the set of all polynomials in x over F:

F [x] = {\sum_{i = 0}^{\infty} a_{i} x^{i} | a_{i} \in F, a_{i} \neq 0 for all but a finite number of i}

The set

F [x]

equipped with the operations + and · is the polynomial ring in x over the field F. In this paper, F is specified as the field R of real numbers.

Remark: The contents of Lemma 4 and Lemma 5 can be found in many textbooks of Linear Algebra or Advanced Algebra. Then we need only give the proofs of Lemma 6 and Lemma 7.

Proof of Lemma 6: The proof is conducted by Transfinite Induction.

Let

P (γ)

be the statement of Lemma 4, i.e.

“

m (x), g_{1} (x), . . ., g_{n} (x) \in F [x], n \geq 2

. If

m (x)

is irreducible and divides the product

g_{1} (x) \dots g_{n} (x)

, then

m (x)

divides one of the polynomials

g_{1} (x), . . ., g_{n} (x)

” with n replaced by

γ

, where

γ \in A

A = N ⋃ {ω}

with the ordering that

n < ω

for all natural numbers n,

ω

is the smallest limit ordinal other than 0.

Lemma 4 actually can be proved by Mathematical Induction, which includes the Base Case:

P (2)

and the Successor Case:

P (n) \Rightarrow P (n + 1)

P (γ) \Rightarrow P (γ + 1)

, of this proof.

Next we prove the Limit Case:

P (γ < λ) \Rightarrow P (λ)

λ

is any limit ordinal other than 0. For convenience, we first prove

P (γ < ω) \Rightarrow P (ω)

For the sake of contradiction, assume that

P (γ < ω) ⇏ P (ω)

. Then, considering

m (x)

is irreducible with the property stated in Lemma 5, we have

m (x) | g_{1} (x) \dots g_{γ} (x) \Rightarrow m (x) | g_{1} (x) \dots g_{γ} \dots g_{ω} (x) \Rightarrow g c d (m (x), g_{i} (x)) = 1, i \in N \cup {ω} \Rightarrow g c d (m (x), g_{i} (x)) = 1, i \in N

, which contradicts

P (γ < ω) : m (x) | g_{1} (x) \dots g_{γ} (x) \Rightarrow m (x)

divides one of the polynomials

g_{1} (x), . . ., g_{γ} (x)

Thus, we know that the assumption

P (γ < ω) ⇏ P (ω)

does not hold.

Then

P (γ < ω) \Rightarrow P (ω)

is true.

Since

λ \geq ω

, then we obviously have

m (x) | g_{1} (x) \dots g_{γ} (x) \Rightarrow m (x) | g_{1} (x) \dots g_{γ} \dots g_{λ} (x) \Rightarrow g c d (m (x), g_{i} (x)) = 1, i \in N \cup {ω, \dots, λ} \Rightarrow g c d (m (x), g_{i} (x)) = 1, i \in N

, which contradicts

P (γ < λ) : m (x) | g_{1} (x) \dots g_{γ} (x) \Rightarrow m (x)

divides one of the polynomials

g_{1} (x), . . ., g_{γ} (x)

Then the Limit Case:

P (γ < λ) \Rightarrow P (λ)

is true.

That completes the proof of Lemma 6.

Proof of Lemma 7: If

m (x)

is irreducible and divides the product

p (x) q (x) = p_{1} (x) \dots p_{\infty} q (x)

, then, according to Lemma 6,

m (x)

divides one of the polynomials

p_{1} (x), . . ., p_{\infty} (x), q (x)

. Further, if

m (x)

and

p (x)

are relatively prime, then

m (x)

does not divides any factor

p_{i} (x), i = 1, \dots, \infty

p (x)

(otherwise

m (x)

divides

p (x)

, which contradicts the condition “

m (x)

and

p (x)

are relatively prime”). Thus,

m (x)

must divides

q (x)

That completes the proof of Lemma 7.

3. A Proof of the RH

This section is planned to present a proof of the Riemann Hypothesis. We first prove that Statement 2 of the RH is true, and then by Lemma 2, Statement 1 of the RH is also true. To be brief, to prove the Riemann Hypothesis, it suffices to show that

α_{i} = \frac{1}{2}, i = 1, 2, 3, \dots, \infty

in the new expression of

ξ (s)

as shown in Eq.(22).

Proof of the RH: The details are delivered in three steps as follows.

Step 1:

It is well-known that all the zeros of

ξ (s)

always come in complex conjugate pairs. Then by pairing

ρ_{i} = α_{i} + j β_{i}

and

{\bar{ρ}}_{i} = α_{i} - j β_{i}

in the Hadamard product as shown in Eq.(10), we have

\begin{matrix} ξ (s) & = ξ (0) \prod_{ρ} (1 - \frac{s}{ρ}) = ξ (0) \prod_{i = 1}^{\infty} (1 - \frac{s}{ρ_{i}}) (1 - \frac{s}{{\bar{ρ}}_{i}}) \\ = ξ (0) \prod_{i = 1}^{\infty} (1 - \frac{s}{α_{i} + j β_{i}}) (1 - \frac{s}{α_{i} - j β_{i}}) = ξ (0) \prod_{i = 1}^{\infty} (\frac{β_{i}^{2}}{α_{i}^{2} + β_{i}^{2}} + \frac{{(s - α_{i})}^{2}}{α_{i}^{2} + β_{i}^{2}}) \end{matrix}

(22)

where

ξ (0) = \frac{1}{2}, 0 < α_{i} < 1, β_{i} \neq 0

The absolute convergence of the infinite product in Eq.(22) in the form

ξ (s) = ξ (0) \prod_{i = 1}^{\infty} (1 - \frac{s}{ρ_{i}}) (1 - \frac{s}{{\bar{ρ}}_{i}}) = ξ (0) \prod_{i = 1}^{\infty} (1 - \frac{s (2 α_{i} - s)}{| ρ_{i} |^{2}})

(23)

depends on the convergence of infinite series

\sum_{i = 1}^{\infty} \frac{1}{| ρ_{i} |^{2}}

, which is an obvious fact according to Theorem 2 in Section 2, Chapter IV of Ref.[23].

Further, considering the absolute convergence of

ξ (s) = ξ (0) \prod_{i = 1}^{\infty} (1 - \frac{s (2 α_{i} - s)}{| ρ_{i} |^{2}}) = ξ (0) \prod_{i = 1}^{\infty} (\frac{β_{i}^{2}}{α_{i}^{2} + β_{i}^{2}} + \frac{{(s - α_{i})}^{2}}{α_{i}^{2} + β_{i}^{2}})

(24)

we have the following new expression of

ξ (s)

by putting all the

ρ_{i}

related multiple factors (zeros) together in the above Eq.(24)

ξ (s) = ξ (0) \prod_{i = 1}^{\infty} (\frac{β_{i}^{2}}{α_{i}^{2} + β_{i}^{2}} + \frac{{(s - α_{i})}^{2}}{α_{i}^{2} + β_{i}^{2}})^{m_{i}}

(25)

where

m_{i} \geq 1

is the real multiplicity of

ρ_{i}

i = 1, 2, 3, \dots, \infty

Step 2: Replacing s with

1 - s

in Eq.(25), we obtain the infinite product expression of

ξ (1 - s)

, i.e.,

ξ (1 - s) = ξ (0) \prod_{i = 1}^{\infty} {(\frac{β_{i}^{2}}{α_{i}^{2} + β_{i}^{2}} + \frac{{(1 - s - α_{i})}^{2}}{α_{i}^{2} + β_{i}^{2}})}^{m_{i}}

(26)

Step 3: According to the functional equation

ξ (s) = ξ (1 - s)

, and considering Eq.(25) and Eq.(26), we have

ξ (0) \prod_{i = 1}^{\infty} {(\frac{β_{i}^{2}}{α_{i}^{2} + β_{i}^{2}} + \frac{{(s - α_{i})}^{2}}{α_{i}^{2} + β_{i}^{2}})}^{m_{i}} = ξ (0) \prod_{i = 1}^{\infty} {(\frac{β_{i}^{2}}{α_{i}^{2} + β_{i}^{2}} + \frac{{(1 - s - α_{i})}^{2}}{α_{i}^{2} + β_{i}^{2}})}^{m_{i}}

(27)

which is equivalent to

\prod_{i = 1}^{\infty} {(1 + \frac{{(s - α_{i})}^{2}}{β_{i}^{2}})}^{m_{i}} = \prod_{i = 1}^{\infty} {(1 + \frac{{(1 - s - α_{i})}^{2}}{β_{i}^{2}})}^{m_{i}}

(28)

where

β_{i}

are in order of increasing

| β_{i} |

, i.e.,

0 < | β_{1} | \leq | β_{2} | \leq | β_{3} | \leq \dots

To check the absolute convergence of both sides of Eq.(28), it suffices to make a comparison with Eq.(23) without considering multiple zeros in Eq. (28), i.e., to make a comparison between

\prod_{i = 1}^{\infty} (1 + \frac{{(s - α_{i})}^{2}}{β_{i}^{2}})

and

ξ (0) \prod_{i = 1}^{\infty} (1 - \frac{s (2 α_{i} - s)}{| ρ_{i} |^{2}})

. It is well-known that the absolute convergence of

ξ (0) \prod_{i = 1}^{\infty} (1 - \frac{s (2 α_{i} - s)}{| ρ_{i} |^{2}})

depends on the convergence of infinite series

\sum_{i = 1}^{\infty} \frac{1}{| ρ_{i} |^{2}}

(already proved in Step 1); the absolute convergence of

\prod_{i = 1}^{\infty} (1 + \frac{{(s - α_{i})}^{2}}{β_{i}^{2}})

depends on the convergence of infinite series

\sum_{i = 1}^{\infty} \frac{1}{β_{i}^{2}}

, which is also an obvious fact because

0 < α_{i} < 1, | ρ_{i} | \to \infty, | β_{i} | \to \infty, as i \to \infty, {lim}_{i \to \infty} \frac{β_{i}^{2}}{| ρ_{i} |^{2}} = {lim}_{i \to \infty} \frac{β_{i}^{2}}{α_{i}^{2} + β_{i}^{2}} = 1

, that means

\sum_{i = 1}^{\infty} \frac{1}{β_{i}^{2}}

and

\sum_{i = 1}^{\infty} \frac{1}{| ρ_{i} |^{2}}

have the same convergence.

Then, according to Lemma 3, Eq.(28) is equivalent to

α_{i} = \frac{1}{2}; 0 < | β_{1} | < | β_{2} | < | β_{3} | < \dots; i = 1, 2, 3, \dots, \infty

(29)

Thus, we conclude that all the zeros of the completed zeta function

ξ (s)

have real part equal to

\frac{1}{2}

, i.e., Statement 2 of the RH is true. According to Lemma 2, Statement 1 of the RH is also true, i.e., all the non-trivial zeros of the Riemann zeta function

ζ (s)

have real part equal to

\frac{1}{2}

That completes the proof of the RH.

4. Retrospection and Discussion

On the simultaneous zeros of $ξ (s)$

According to Lemma 1, there are two pairs of complex zeros of

ζ (s)

simultaneously, i.e.,

ρ = α + j β, \bar{ρ} = α - j β, 1 - ρ = 1 - α - j β, 1 - \bar{ρ} = 1 - α + j β

. With the proof of the RH, these 2 pairs of zeros are actually only one pair, because

ρ = 1 - \bar{ρ} = \frac{1}{2} + j β, \bar{ρ} = 1 - ρ = \frac{1}{2} - j β

. Thus Lemma 1 could be modified more precisely as follows.

Lemma 1*: Non-trivial zeroes of

ζ (s)

, noted as

ρ = α + j β

, have the following properties

1): The number of non-trivial zeroes is infinity;
2): $β \neq 0$ ;
3): $0 < α < 1$ ;
4): $ρ = 1 - \bar{ρ}, \bar{ρ} = 1 - ρ$ are all non-trivial zeroes.

On the paring of zeros of $ξ (s)$

Hadamard pointed out that to ensure the absolute convergence of the Hadamard product, i.e.,

ξ (s) = ξ (0) \prod_{ρ} (1 - \frac{s}{ρ})

ρ

and

1 - ρ

are paired. In Section 3, the author proved that

ρ

and

\bar{ρ}

can also be paired to ensure the absolute convergence of the Hadamard product. And that the paring of conjugate zeros, i.e.,

ρ

and

\bar{ρ}

, is the right way to express the most essential characteristic of

ξ (s)

as (infinite) polynomial with real coefficients, whereas

1 - ρ

and

1 - \bar{ρ}

are just another pair of conjugate zeros given by

ξ (s) = ξ (1 - s)

5. Conclusion

This paper presents a proof of the RH based on a new expression of

ξ (s)

, i.e.,

ξ (s) = ξ (0) \prod_{i = 1}^{\infty} (\frac{β_{i}^{2}}{α_{i}^{2} + β_{i}^{2}} + \frac{{(s - α_{i})}^{2}}{α_{i}^{2} + β_{i}^{2}})^{m_{i}}

where

ξ (0) = \frac{1}{2}, ρ_{i} = α_{i} + j β_{i}

and

{\bar{ρ}}_{i} = α_{i} - j β_{i}

are the complex conjugate zeros of

ξ (s)

0 < α_{i} < 1

and

β_{i} \neq 0

are real numbers,

0 < | β_{1} | \leq | β_{2} | \leq | β_{3} | \leq \dots

m_{i} \geq 1

is the real multiplicity of

ρ_{i}

The proof is conducted according to the divisibility implied in the (infinite) polynomial equation

ξ (s) = ξ (1 - s)

. The first key-point is the paring of conjugate zeros

ρ

and

\bar{ρ}

to get the new expression of

ξ (s)

. The second key-point is the use of “real multiplicity” of a zero of

ξ (s)

. Obviously, the real multiplicity of a zero of

ξ (s)

is an objective existence, unique, and unchangeable. As a result, the functional equation

ξ (s) = ξ (1 - s)

finally leads to

α_{i} = \frac{1}{2}; 0 < | β_{1} | < | β_{2} | < | β_{3} | < \dots; i = 1, 2, 3, \dots, \infty

Acknowledgments

The author would like to gratefully acknowledge the help received from Dr. Victor Ignatov (Independent Researcher), Prof. Mark Kisin (Harvard University), Prof. Yingmin Jia (Beihang University), Prof. Tianguang Chu (Peking University), Prof. Guangda Hu (Shanghai University), Prof. Jiwei Liu (University of Science and Technology Beijing), and Dr. Shangwu Wang (Beijing 99view Technology Limited, my classmate in Tsinghua University), while preparing this article. The author is also grateful to the editors and referees of PNAS for their constructive comments and suggestions. Finally, with this manuscript, the author pays tribute to Bernhard Riemann and other predecessor mathematicians. They are the shining stars in the sky of human civilization. This manuscript has no associated data.

References

Riemann B. (1859), Über die Anzahl der Primzahlen unter einer gegebenen Grösse. Monatsberichte der Deutschen Akademie der Wissenschaften zu Berlin, 2: 671-680.
Bombieri E. (2000), Problems of the millennium: The Riemann Hypothesis, CLAY.
Peter Sarnak (2004), Problems of the Millennium: The Riemann Hypothesis, CLAY.
Hadamard J. (1896), Sur la distribution des zeros de la fonction ζ(s) et ses conseequences arithmetiques, Bulletin de la Societe Mathematique de France, 14: 199-220, Reprinted in (Borwein et al. 2008). [CrossRef]
de la Vallee-Poussin Ch. J. (1896), Recherches analytiques sur la theorie des nombers premiers, Ann. Soc. Sci. Bruxelles, 20: 183-256.
Hardy G. H. (1914), Sur les Zeros de la Fonction ζ(s) de Riemann, C. R. Acad. Sci. Paris, 158: 1012-1014, JFM 45.0716.04 Reprinted in (Borwein et al. 2008).
Hardy G. H., Littlewood J. E. (1921), The zeros of Riemann’s zeta-function on the critical line, Math. Z., 10 (3-4): 283-317.
Tom M. Apostol (1998), Introduction to Analytic Number Theory, New York: Springer.
C. D. Pan, C. B. Pan (2016), Basic Analytic Number Theory (in Chinese), 2nd Edition, Harbin Institute of Technology Press.
Reyes E. O. (2004), The Riemann zeta function, Master Thesis of California State University, San Bernardino, Theses Digitization Project. 2648. https: //scholarworks.lib.csusb.edu /etd-project/2648.
A. Selberg (1942), On the zeros of the zeta-function of Riemann, Der Kong. Norske Vidensk. Selsk. Forhand. 15: 59-62; also, Collected Papers, Springer- Verlag, Berlin - Heidelberg - New York 1989, Vol. I, 156-159.
N. Levinson (1974), More than one-third of the zeros of the Riemann zeta function are on $σ = \frac{1}{2}$ , Adv. Math. 13: 383-436.
S. Lou and Q. Yao (1981), A lower bound for zeros of Riemann’s zeta function on the line $σ = \frac{1}{2}$ , Acta Mathematica Sinica (in chinese), 24: 390-400.
J. B. Conrey (1989), More than two fifths of the zeros of the Riemann zeta function are on the critical line, J. reine angew. Math. 399: 1-26. [CrossRef]
H. M. Bui, J. B. Conrey and M. P. Young (2011), More than 41% of the zeros of the zeta function are on the critical line, http://arxiv.org/abs/1002.4127v2.
Feng S. (2012), Zeros of the Riemann zeta function on the critical line, Journal of Number Theory, 132(4): 511-542.
Wu X. (2019), The twisted mean square and critical zeros of Dirichlet L-functions. Mathematische Zeitschrift, 293: 825-865. [CrossRef]
Siegel, C. L. (1932), Über Riemanns Nachlaß zur analytischen Zahlentheorie, Quellen Studien zur Geschichte der Math. Astron. Und Phys. Abt. B: Studien 2: 45-80, Reprinted in Gesammelte Abhandlungen, Vol. 1. Berlin: Springer-Verlag, 1966.
Gram, J. P. (1903), Note sur les zéros de la fonction ζ(s) de Riemann, Acta Mathematica, 27: 289-304. [CrossRef]
Titchmarsh E. C. (1935), The Zeros of the Riemann Zeta-Function, Proceedings of the Royal Society of London. Series A, Mathematical and Physical Sciences, The Royal Society, 151 (873): 234-255. [CrossRef]
Titchmarsh E. C. (1936), The Zeros of the Riemann Zeta-Function, Proceedings of the Royal Society of London. Series A, Mathematical and Physical Sciences, The Royal Society, 157 (891): 261-263.
Hadamard J. (1893), Étude sur les propriétés des fonctions entières et en particulier d’une fonction considérée par Riemann. Journal de mathématiques pures et appliquées, 9: 171-216.
Karatsuba A. A., Nathanson M. B. (1993), Basic Analytic Number Theory, Springer, Berlin, Heidelberg.

Figure 1. Illustration of the multiple zeros of

ξ (s)

Figure 1. Illustration of the multiple zeros of

ξ (s)

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content.

© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).

Copyright: This open access article is published under a Creative Commons CC BY 4.0 license, which permit the free download, distribution, and reuse, provided that the author and preprint are cited in any reuse.

Alerts

MDPI Initiatives

Important Links

Choose an area of interest and we will send you notifications of new preprints at your preferred frequency.

Disclaimer

A Proof of the Riemann Hypothesis Based on a New Expression of the Completed Zeta Function

Abstract

Keywords:

Subject:

1. Introduction

2. Lemmas

3. A Proof of the RH

4. Retrospection and Discussion

5. Conclusion

Acknowledgments

References

MDPI Initiatives

Important Links

Subscribe