Article
Version 3
Preserved in Portico This version is not peer-reviewed
Nonlinear Generalisation of Quantum Mechanics
Version 1
: Received: 23 August 2021 / Approved: 27 August 2021 / Online: 27 August 2021 (14:03:12 CEST)
Version 2 : Received: 12 October 2021 / Approved: 14 October 2021 / Online: 14 October 2021 (15:11:22 CEST)
Version 3 : Received: 30 June 2022 / Approved: 1 July 2022 / Online: 1 July 2022 (10:49:24 CEST)
Version 2 : Received: 12 October 2021 / Approved: 14 October 2021 / Online: 14 October 2021 (15:11:22 CEST)
Version 3 : Received: 30 June 2022 / Approved: 1 July 2022 / Online: 1 July 2022 (10:49:24 CEST)
How to cite: Jamali, A. Nonlinear Generalisation of Quantum Mechanics. Preprints 2021, 2021080525. https://doi.org/10.20944/preprints202108.0525.v3 Jamali, A. Nonlinear Generalisation of Quantum Mechanics. Preprints 2021, 2021080525. https://doi.org/10.20944/preprints202108.0525.v3
Abstract
According to our current understanding of quantum mechanics, a `measurement' violates unitarity. In other words as the act of measurement `resets' the probabilities described by the Schrödinger equation, in the very `moment' of the collapse of the wavefunction, conservation of probability does not hold. To make progress in our understanding of the measurement problem it is thus expected that one must encompass in a single equation both otherwise mutually-exclusive processes of measurement and unitary evolution. In this light, turning to the continuity equation, we realize the possibility that if we allow for existence of sources and sinks in the `fluid of probability' we get closer to such a theory by arriving at nonlinear generalizations of Schrödinger and Klein-Gordon equations. The generalized equations derived are then shown to satisfy all conditions that are expected from a proper generalization: simplification to their linear counterparts by a well-defined dynamical condition, and Galilean and Lorentz invariance.
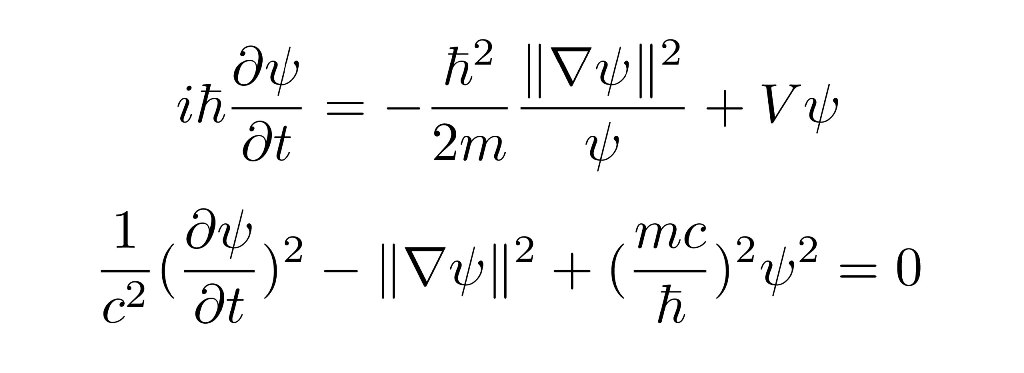
Keywords
quantum mechanics; foundations of quantum mechanics; nonlinear equation; nonlinear quantum mechanics; nonlinear schrödinger equation
Subject
Physical Sciences, Particle and Field Physics
Copyright: This is an open access article distributed under the Creative Commons Attribution License which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.
Comments (1)
We encourage comments and feedback from a broad range of readers. See criteria for comments and our Diversity statement.
Leave a public commentSend a private comment to the author(s)
* All users must log in before leaving a comment
Commenter: Alireza Jamali
Commenter's Conflict of Interests: Author
as a result
* Abstract rewritten.
* The reference to the preprint of Renou et al. is changed to their publiaction in Nature.
* Some typos and syntax corrected.