PreprintArticleVersion 1Preserved in Portico This version is not peer-reviewed
A Topological Approach to Shrinking Higher Dimensions of Space to Observable Space-Time: Can the Dimensional Anisotropy of Space Satisfy Mach’s Principle?
Version 1
: Received: 1 November 2021 / Approved: 5 November 2021 / Online: 5 November 2021 (10:58:49 CET)
How to cite:
Deli, E.; Peters, J.F. A Topological Approach to Shrinking Higher Dimensions of Space to Observable Space-Time: Can the Dimensional Anisotropy of Space Satisfy Mach’s Principle?. Preprints2021, 2021110115. https://doi.org/10.20944/preprints202111.0115.v1
Deli, E.; Peters, J.F. A Topological Approach to Shrinking Higher Dimensions of Space to Observable Space-Time: Can the Dimensional Anisotropy of Space Satisfy Mach’s Principle?. Preprints 2021, 2021110115. https://doi.org/10.20944/preprints202111.0115.v1
Deli, E.; Peters, J.F. A Topological Approach to Shrinking Higher Dimensions of Space to Observable Space-Time: Can the Dimensional Anisotropy of Space Satisfy Mach’s Principle?. Preprints2021, 2021110115. https://doi.org/10.20944/preprints202111.0115.v1
APA Style
Deli, E., & Peters, J.F. (2021). A Topological Approach to Shrinking Higher Dimensions of Space to Observable Space-Time: Can the Dimensional Anisotropy of Space Satisfy Mach’s Principle?. Preprints. https://doi.org/10.20944/preprints202111.0115.v1
Chicago/Turabian Style
Deli, E. and James F. Peters. 2021 "A Topological Approach to Shrinking Higher Dimensions of Space to Observable Space-Time: Can the Dimensional Anisotropy of Space Satisfy Mach’s Principle?" Preprints. https://doi.org/10.20944/preprints202111.0115.v1
Abstract
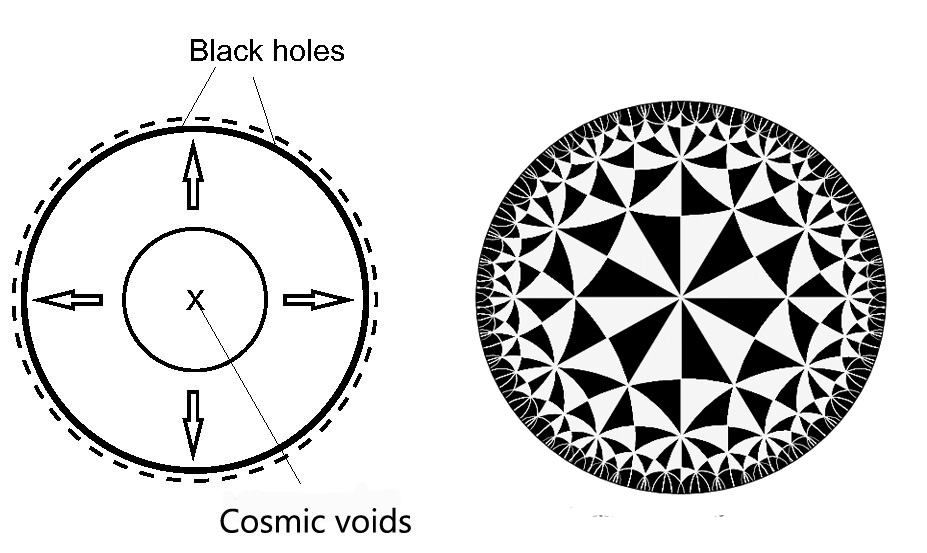
We create a model universe by equipping a topological surface (system) with compact dimensions insulated by an information blocking horizon. The insulated compact WF can produce entanglement independent of distance. Interaction between the system and the WF changes the curvature of the first and the quantum state (frequency) of the second in an interconnected relationship. Thus, the field curvature measures the evolution of the particle WF as time. Positive field curvature creates pressure, whereas negative field curvature generates a vacuum, satisfying the Borsuk-Ulam Theorem and the Page and Wootters mechanism of static time. The accumulation of pressure or vacuum generates poles with contrasting dimensionalities, two-dimensional black hole horizons (time infinite), and four-dimensional cosmic voids (time zero). The orthogonality of the field and the compact WF give rise to global self-regulation that fine-tunes the cosmic parameters and can promote fractal topology. The four-dimensional vacuum in cosmic voids can produce an accelerating expansion without dark energy. When gravity effects are eliminated, we find a new, so far unexplored, order-increasing side of entropy. The verifiable and elegant hypothesis satisfies Mach's principle.
Copyright:
This is an open access article distributed under the Creative Commons Attribution License which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.
