Prerpints.org logo
Preprint
Article

Euclidean Relativity Solves the Hubble Constant Tension

Altmetrics

Downloads

10730

Views

11128

Comments

1

This version is not peer-reviewed

Submitted:

21 May 2024

Posted:

22 May 2024

Read the latest preprint version here

Alerts
Abstract
Special relativity (SR) and general relativity (GR) describe nature in “subjective concepts” (concepts of an observer). Examples of such concepts are space and time—or waves and particles. Despite the Lorentz covariance of SR, the general covariance of GR, and coordinate-free formulations of SR/GR, the perspective of each observer is egocentric. Even if we consider all these egocentric perspectives, we will not craft a “holistic view” of nature (a view taken from all possible perspectives at the same instant in time) because there is no absolute time in SR/GR. Here I show: Euclidean relativity (ER) describes nature in “objective concepts” (concepts that are immanent in all objects). Space and time are replaced by “pure distance”. Waves and particles are replaced by “pure energy”. Each object’s proper space d1, d2, d3 and its proper time τ span an absolute 4D Euclidean spacetime (ES), where d1, d2, d3 and d4 = cτ are pure distances. The invariant parameter in ER is absolute, cosmic time t. An observer’s reality is created by projecting ES orthogonally to his proper space and to his proper time. ER compares to a “heliocentric model 2.0”, in which the sun is the center of our solar system but not of our galaxy. That model provides a holistic view from “beyond” (outside of) our galaxy. Likewise, ER provides a holistic view from beyond space. The “master reality” ES (described by ER) is beyond each observer’s reality (described by SR/GR). ER solves 15 mysteries geometrically, such as the Hubble constant tension and entanglement. Field equations are not required.
Keywords: 
Subject: Physical Sciences  -   Theoretical Physics
This paper introduces holistic thinking to physics. There are two approaches to describing nature: in “subjective concepts” (concepts of an observer) or else in “objective concepts” (concepts that are immanent in all objects). Only the second approach provides a “holistic view of nature” (a view taken from all possible perspectives at the same instant in time). Special relativity (SR) and general relativity (GR) take the first approach (Einstein, 1905b; Einstein, 1916). Euclidean relativity (ER) takes the second approach. Several top journals in physics rejected my theory. Often, I was told that manuscripts are not considered if they challenge SR/GR. This is not how science works! Many hints tell us that SR and GR cannot be the full story. Here is the message of my paper: Subjectively, we live in a curved, non-Euclidean spacetime. Objectively, we live in a flat, Euclidean spacetime.
Seven pieces of advice: (1) Do not take SR/GR as the ultimate truth. Correct predictions do not prove SR/GR. ER predicts the same relativistic effects as SR/GR. (2) Do not think that all theories in physics must be field theories. Previous reviewers did so. ER describes a master reality that is beyond all fields. Physical fields come into play in an observer’s reality only. (3) Be patient and be fair. One paper cannot cover all of physics. SR and GR have been tested for 100+ years. ER deserves the same chance. (4) Do not reject ER on some knee-jerk reaction. A rejection requires solid arguments that disprove ER. Why not cherish the beauty of ER? (5) Do not be prejudiced against a theory that solves many mysteries. New concepts often do so. (6) Appreciate illustrations. As a geometric theory, ER complies with the stringency of math. (7) Consider that you may be biased. Some concepts of today’s physics are obsolete in ER. As an expert in such a concept, you may feel offended. If your concepts do not fit to ER, you may want to consider seeing our world through different eyes (Niemz, 2020).
To sum it all up: SR/GR remain valid in an observer’s reality, but they do not provide a holistic view of nature. Only ER provides a holistic view, which is required for the solution of the Hubble constant tension and other mysteries. I apologize for my many preprint versions, but I received almost no support. The final version is all that is needed. Earlier versions show how I got there. It was tricky to figure out why SR/GR work so well despite an issue. Section 2 is about this issue. Section 3 describes ER. Section 4 recovers the Lorentz factor and gravitational time dilation. In Section 5, ER solves 15 mysteries of physics.

1. Introduction

Today’s concepts of space and time were coined by Albert Einstein. In SR, he merges them into a flat spacetime described by an indefinite distance function. SR is often presented in Minkowski space time because it illustrates the invariance of the spacetime interval very well (Minkowski, 1910). Predicting the lifetime of muons (Rossi & Hall, 1941) is an example that supports SR. In GR, curved spacetime is described by a pseudo-Riemannian metric. Predicting the deflection of starlight (Dyson et al., 1920) and the high accuracy of GPS (Ashby, 2003) are examples that support GR. Quantum field theory (Ryder, 1985) unifies classical field theory, SR, and quantum mechanics (QM) but not GR.
Two postulates of ER: (1) All energy moves through 4D Euclidean spacetime (ES) at the speed of light. (2) The laws of physics have the same form in each “observer’s reality”, which is created by projecting ES orthogonally to his proper space and to his proper time. To improve readability, all of my observers are male. To make up for it, nature is female. My first postulate is stronger than the second postulate of SR: The speed of light c is absolute and universal. My second postulate refers to realities rather than to inertial frames. Moreover, I introduce objective concepts. Space and time are replaced by “pure distance”. Waves and particles are replaced by “pure energy”.
Newburgh and Phipps (1969) pioneered ER. Montanus (1991) described an absolute Euclidean spacetime with a “preferred frame of reference” (a pure time interval is a pure time interval for all observers). Montanus (2023) claims: Without the preferred frame, we would face the twin paradox, non-contact collisions, and a “character paradox” (confusion of photons, particles, antiparticles). I will show that the preferred frame is obsolete. Whatever is proper time for me, it may be proper space for you. There is no twin paradox. Non-contact collisions and the character paradox turn out to be reasonable. Montanus (2001) also tried to formulate kinematic equations in ER using the Lagrange formalism. Montanus (2023) even tried to formulate Maxwell’s equations in ER but wondered about a wrong sign. He overlooked that the SO(4) symmetry of ES is incompatible with waves.
Almeida (2001) investigated geodesics in ES. Gersten (2003) showed that the Lorentz transformation is an SO(4) rotation in a “mixed space” (see Section 3). van Linden (2023) runs a website about various ER models. Physicists are still opposed to ER because dark energy and non-locality make cosmology and QM work, waves are excluded, and paradoxes may turn up if ER is not interpreted correctly. This paper marks a turning point: I disclose an issue in SR/GR. I justify the exclusion of waves. I avoid paradoxes by projecting ES.
It is instructive to contrast Newton’s physics, Einstein’s physics, and ER. In Newton’s physics, all energy moves through 3D Euclidean space as a function of independent time. There is no speed limit for matter. In Einstein’s physics, all energy moves through 4D non-Euclidean spacetime. The speed of matter is v 3 D < c . In ER, all energy moves through ES. The 4D speed of all energy is u 4 D = c . Newton’s physics (Newton, 1687) influenced Kant’s philosophy (Kant, 1781). Will ER reform both physics and philosophy?

2. Disclosing an Issue in Special and General Relativity

In SR (Einstein, 1905b), there are two concepts of time: coordinate time t  and proper time τ . The fourth coordinate in SR is t . In § 1 of SR, Einstein provides an instruction on how to synchronize two clocks at P and Q. At “P time” t P , a light pulse is sent from P to Q. At “Q time” t Q , it is reflected. At “P time” t P * , it is back at P. The clocks synchronize if
t Q − t P = t P * − t Q .
In § 3 of SR, Einstein derives the Lorentz transformation. The coordinates x 1 ,   x 2 ,   x 3 ,   t of an event in a system K are transformed to the coordinates x 1 ' ,   x 2 ' ,   x 3 ' ,   t ' in K’ by
x 1 ' = γ ( x 1 − v 3 D t )   ,   x 2 ' = x 2   ,   x 3 ' = x 3 ,
t ' = γ ( t − v 3 D x 1 / c 2 ) ,
where K’ moves relative to K in x 1 at the constant speed v 3 D and γ = ( 1 − v 3 D 2 / c 2 ) − 0.5 is the Lorentz factor. Mathematically, Equations (1) and (2a–b) are correct for observers in K. There are similar equations for observers in K’. Physically, SR and GR are not wrong, but they have what I call an “issue”. Despite the Lorentz covariance of SR, the general covariance of GR, and coordinate-free formulations of SR/GR, the perspective of each observer is egocentric. Even if we consider all these egocentric perspectives, we will not craft a “holistic view” of nature (I repeat this important definition: a view taken from all possible perspectives at the same instant in time) because there is no absolute time in SR/GR. In ER, there is absolute, cosmic time (see Section 3). Since absolute time is missing in SR/GR, observers in these theories will not always agree on what is past and what is future. Physics has paid an enormous price for dismissing the concept of absolute time: As I will show in Section 5, ER solves 15 fundamental mysteries. Thus, the issue in SR/GR is real.
The issue in SR/GR compares to the issue in the geocentric model: In either case, there is no holistic view. The perspective of each observer is egocentric or geocentric. In the old days, it was natural to believe that all celestial bodies would revolve around Earth. A few astronomers wondered about the retrograde loops of planets and claimed: Earth revolves around the sun. In modern times, engineers improved the precision of rulers and clocks. Eventually, it was natural to believe that it would be fine to describe nature as accurately as possible but from one or more egocentric perspectives. The human brain is very smart, but unfortunately it often deems itself the center/measure of everything.
The analogy of SR/GR to the geocentric model is stunningly close: (1) It holds despite all covariances. After a transformation in SR/GR (or after appointing another “Earth” as the “center of the Universe”), the perspective is still egocentric (or else geocentric). (2) ER compares to a “heliocentric model 2.0”, in which the sun is the center of our solar system but not of our galaxy. That model provides a holistic view from “beyond” (outside of) our galaxy. Likewise, ER provides a holistic view from beyond space. (3) Both the geocentric model and SR/GR miss the big picture. The retrograde loops of planets are obsolete but only from the holistic view provided by the heliocentric model. Likewise, dark energy and non-locality are obsolete but only from the holistic view provided by ER. (4) The heliocentric model was not taken seriously in the old days. ER is not yet taken seriously nowadays. Have physicists not learned from history? Does history repeat itself?

3. The Physics of Euclidean Relativity

The indefinite distance function in SR (Einstein, 1905b) is usually written as
c 2 d τ 2 = c 2 d t 2 − d x 1 2 − d x 2 2 − d x 3 2 ,
where d τ is an infinitesimal distance in proper time τ , while d t and d x i ( i = 1 ,   2 ,   3 ) are infinitesimal distances in coordinate spacetime x 1 ,   x 2 ,   x 3 ,   t . This spacetime is construed because coordinate space x 1 ,   x 2 ,   x 3 and coordinate time t are subjective concepts: They are construed by an observer and not immanent in rulers/clocks. Rulers measure proper distance. Clocks measure proper time. I introduce ER by defining its Euclidean metric
c 2 d t 2 = d d 1 2 + d d 2 2 + d d 3 2 + d d 4 2
where d d i = d x i ( i = 1 , 2 , 3 ) and d d 4 = c d τ are infinitesimal distances in 4D Euclidean spacetime d 1 , d 2 , d 3 , d 4 (ES). In ER, the roles of t and τ are switched: The fourth coordinate is an object’s proper time τ . The invariant line element is cosmic time t . The metric tensor is the identity matrix. Because of this matrix, ER is much simpler than GR. I retain the symbol t because we associate it with time. I prefer the indices 1–4 over 0–3 to stress the symmetry. Each object’s proper space d 1 , d 2 , d 3 and its proper time τ span ES, where d 1 , d 2 , d 3 and d 4 = c τ are pure distances. This spacetime is natural because all d μ ( μ = 1 , 2 , 3 , 4 ) are objective concepts: They are immanent in rulers/clocks. We must not confuse Equation (4) with a Wick rotation (Wick, 1954), where time is imaginary and τ is kept invariant.
Each object is free to label the axes of ES. It labels the axis of its current motion at the speed c as d 4 . Because of length contraction, this axis is not observed by itself but experienced as proper time τ . Some other object may move in d 4 ' at the speed c . It experiences d 4 ' as τ ' . Thus, there is a relative 4D vector “flow of proper time” τ .
τ = d 4 / c   ,   τ ' = d 4 ' / c
τ = d 4 u / c 2   ,   τ ' = d 4 ' u ' / c 2 ,
where u is the 4D velocity of an object in ES. For all objects, there is u μ = d d μ / d t , where t is cosmic time. Thus, Equation (4) is equivalent to my first postulate.
u 1 2 + u 2 2 + u 3 2 + u 4 2 = c 2
My second postulate revises the principle of relativity and defines an observer’s reality. It is created by projecting ES orthogonally to his proper space and to his proper time. Since coordinate time t in Equation (3) is not equal to cosmic time t in Equation (4), there is no continuous transition between SR and ER. In SR, an object is described by the four coordinates x 1 ( τ ) ,   x 2 ( τ ) ,   x 3 ( τ ) , t ( τ ) , where proper time τ is the parameter and t is coordinate time. In ER, an object is described by the four coordinates d 1 ( t ) ,   d 2 ( t ) ,   d 3 ( t ) ,   d 4 ( t ) , where cosmic time t is the parameter and d 4 relates to τ according to Equation (5).
It is instructive to contrast three concepts of time. Coordinate time t is a subjective measure of time: It is equal to τ = | τ | for the observer only. Proper time τ is an objective measure of time: Clocks measure τ independently of observers. Finally, cosmic time t is the total distance covered in ES (length of a geodesic) divided by c . By taking cosmic time as the parameter, all observers agree on what is past and what is future. Since cosmic time is absolute, there is no twin paradox in ER. Twins are the same age in cosmic time. However, ER also seems to have an issue (see Section 6 why it is not an issue): Only in proper coordinates can we access ES, but the proper coordinates of other objects cannot be measured. ER is not a physical problem that we could solve using a Lagrangian or Hamiltonian. ER is an innovative, geometric description of nature based on a Euclidean metric.
Let us compare SR with ER. We consider two identical clocks “r” (red clock) and “b” (blue clock). In SR, “r” shall be “at rest”: It moves only in the c t axis at x 1 = 0 . Clock “b” starts at x 1 = 0 , but it moves in the x 1 axis at a constant speed of v 3 D = 0.6   c . Figure 1 left shows the instant when either clock moved 1.0 s in the coordinate time of “r”. Clock “b” moved 0.6 Ls (light seconds) in x 1 and 0.8 Ls in c t ' . Thus, clock “b” displays “0.8”. In ER, no clock is at rest: Figure 1 right shows the instant when either clock moved 1.0 s in its proper time. Both clocks display “1.0”. Clock “b” moved 0.6 Ls in d 1 and 0.8 Ls in d 4 .
We now assume that an observer R (or B) is moving with the clock “r” (or else “b”). In SR and only from the perspective of R (Figure 1 left), “b” is at c t ' = 0.8   L s when “r” is at c t = 1.0   L s . Thus, “b” is slow with respect to “r” in t ' (of B). In ER and independent of observers (Figure 1 right), “b” is at d 4 = 0.8   L s when “r” is at d 4 = 1.0   L s . Thus, “b” is slow with respect to “r” in d 4 (of R). In SR and ER, “b” is slow with respect to “r”, but time dilation occurs in different axes. Experiments do not disclose the axis in which “b” is slow.
But why does ER provide a holistic view? Well, ES is independent of observers and thus absolute. This is why I call ES the “master reality”. Only the projections from ES are relative. The absolute nature of ES shows up in the rotational symmetry of all ES diagrams: Figure 1 right works for R and for B at once. A second Minkowski diagram is required for B, in which the axes x 1 ' and c t ' are orthogonal. The absoluteness also shows up in Equation (4): All four dimensions are interchangeable. Space and time are replaced by the pure distance d μ ( μ = 1 ,   2 ,   3 ,   4 ). Only observers experience distance as spatial or temporal.
Gersten (2003) demonstrated that the Lorentz transformation is an SO(4) rotation in a mixed space x 1 ,   x 2 ,   x 3 ,   c t ' , where c t ' is the only primed coordinate. I will not repeat the derivation. I consider it my task to turn ER into an accepted theory by revealing its power. However, I wish to point out that this pointless mixed space is another hint that the issue in SR is real. A Lorentz transformation rotates mixed x 1 ,   x 2 ,   x 3 ,   c t ' to x 1 ' ,   x 2 ' ,   x 3 ' ,   c t . In ER, unmixed d 1 ' ,   d 2 ' ,   d 3 ' ,   d 4 ' rotate with respect to d 1 ,   d 2 ,   d 3 ,   d 4 (see Section 4).
There is also a big difference in the synchronization of clocks: In SR, each observer is able to synchronize a uniformly moving clock to his clock (same value of c t in Figure 1 left). If he does, the two clocks are not synchronized from the perspective of the moving clock. In ER, clocks with the same 4D vector τ are always synchronized, while clocks with different τ and τ ' are never synchronized (different values of d 4 in Figure 1 right).

4. Geometric Effects in 4D Euclidean Spacetime

We consider two identical rockets “r” (red rocket) and “b” (blue rocket). Let observer R (or B) be in the rear end of rocket “r” (or else “b”). We use 3D space and proper space as synonyms. The 3D space of R (or B) is spanned by d 1 ,   d 2 ,   d 3 (or else d 1 ' ,   d 2 ' ,   d 3 ' ). The proper time of R (or B) relates to d 4 (or else d 4 ' ). Both rockets started at a point P and move relative to each other at the constant speed v 3 D . We are free to label the axis of motion in 3D space. We label it as d 1 (or d 1 ' ). The ES diagrams in Figure 2 must fulfill my two postulates and the initial condition (same point P). We achieve this by rotating the red and the blue frame with respect to each other. Figure 2 bottom shows the projection to the 3D space of R (or B). We draw 2D rockets but are aware that their width is in d 2 ,   d 3 (or d 2 ' ,   d 3 ' ).
We now verify: (1) The fact that the red and the blue frame are rotated with respect to each other causes length contraction. (2) The fact that proper time flows in different 4D directions for R and for B causes time dilation. Let L i , j be the length of rocket i for observer j . In a first step, we project the blue rocket in Figure 2 top left to the d 1 axis.
sin 2 ⁡ φ + cos 2 ⁡ φ = ( L b , R / L b , B ) 2 + ( v 3 D / c ) 2 = 1
L b , R   =   γ − 1   L b , B ( l e n g t h c o n t r a c t i o n ) ,
where γ = ( 1 − v 3 D 2 / c 2 ) − 0.5 is the same Lorentz factor as in SR. For R, rocket “b” contracts by the factor γ − 1 . Which distances will R observe in his d 4 axis? We mentally continue the rotation of “b” in Figure 2 top left until it points vertically down and serves as R’s ruler in the d 4 axis. In the projection to the 3D space of R, this ruler contracts to zero: The d 4 axis disappears for R because of length contraction at the speed c .
In a second step, we project the blue rocket in Figure 2 top left to the d 4 axis.
sin 2 ⁡ φ + cos 2 ⁡ φ = ( d 4 , B / d 4 , B ' ) 2 + ( v 3 D / c ) 2 = 1
d 4 , B = γ − 1 d 4 , B '
where d 4 , B (or d 4 , B ' ) is the distance that B moved in d 4 (or else d 4 ' ). With d 4 , B ' = d 4 , R (R and B cover the same distance in ES but in different directions), we calculate
d 4 , R   =   γ   d 4 , B ( t i m e d i l a t i o n ) ,
where d 4 , R is the distance that R moved in d 4 . Equations (9) and (12) tell us: SR works so well because γ is recovered if we project ES to d 1 and to d 4 . This is not a surprise. Weyl (1928) showed that the Lorentz group is generated by 4D rotations.
To understand how an acceleration manifests itself in ES, we return to our two clocks “r” and “b”. We assume that “r” and Earth move in the d 4 axis of “r” at the speed c and that “b” accelerates in the d 1 axis of “r” toward Earth (Figure 3). Because of Equation (7), the speed u 1 , b of “b” in d 1 increases at the expense of its speed u 4 , b in d 4 .
Gravitational waves (Abbott et al., 2016) support the idea of GR that gravitation is a feature of spacetime. In ER, the SO(4) symmetry of ES is incompatible with waves. This is not an issue if we associate waves always with an observer’s reality. Like classical physics, I consider gravitation a force in an observer’s reality (not in ES) that has not yet been unified with the other forces of physics. I can imagine that the unification will succeed if force, too, is replaced by an objective concept. Up next, I derive gravitational time dilation in ER. Initially, our clocks “r” and “b” are very far away from Earth. Eventually, “b” falls freely toward Earth in the d 1 axis of “r” (Figure 3). The kinetic energy of “b” in d 1 is
1 2 m u 1 , b 2 = G M m / r
where m is the mass of “b”, G is the gravitational constant, M is the mass of Earth, and r is the distance of clock “b” to Earth’s center. By applying Equation (7), we obtain
u 4 , b 2 = c 2 − u 1 , b 2 = c 2 − 2 G M / r
With u 4 , b = d d 4 , b / d t (“b” moves in the d 4 axis at the speed u 4 , b ) and c = d d 4 , r / d t (“r” moves in the d 4 axis at the speed c ), we calculate
d d 4 , b 2 = ( c 2 − 2 G M / r ) ( d d 4 , r / c ) 2
d d 4 , r   =   γ g r   d d 4 , b ( g r a v i t a t i o n a l t i m e d i l a t i o n ) ,
where γ g r = ( 1 − 2 G M / ( r c 2 ) ) − 0.5 is the same dilation factor as in GR. Equation (16) tells us: GR works so well because γ g r is recovered if we project ES to d 4 .
Summary of time dilation: In SR, a uniformly moving clock “b” is slow with respect to a clock “r” in the proper time of “b”. In GR, an accelerating clock “b” or a clock “b” in a stronger gravitational field is slow with respect to a clock “r” in the proper time of “b”. In ER, a clock “b” is slow with respect to a clock “r” in the proper time of “r” (!) whenever the d 4 ' axis of “b” is rotated with respect to the d 4 axis of “r”. This happens if “b” moves relative to “r” (uniformly or non-uniformly) or if “b” is in a stronger gravitational field (a higher value of γ g r ). Since both γ and γ g r are recovered, the Hafele–Keating experiment (1972) also supports ER. Thus, GPS satellites work in ER just as well as in GR.
Three instructive problems demonstrate how to draw and how to read ES diagrams correctly (Figure 4). Problem 1: In billiards, the blue ball is hit toward the red ball. In ES, both balls move at the speed c . We assume that the red ball moves in its d 4 axis. As the blue ball covers distance in d 1 , its speed in d 4 must be less than c . How can the two balls ever collide if their d 4 values do not match? Problem 2: Some rocket moves along a guide wire. In ES, rocket and wire move at the speed c . We assume that the wire moves in its d 4 axis. As the rocket covers distance in d 1 , its speed in d 4 must be less than c . Doesn’t the wire escape from the rocket? Problem 3: Earth orbits the sun. In ES, they both move at the speed c . We assume that the sun moves in its d 4 axis. As Earth covers distance in d 1 and d 2 , its speed in d 4 must be less than c . Doesn’t the sun escape from the orbital plane?
The questions in the last paragraph seem to disclose geometric paradoxes in ER. The fallacy lies in the assumption that all four dimensions would be spatial. In ES, each object covers the longest distance in its d 4 axis and less distance in all other directions. We solve all problems by projecting ES to the 3D space of the object that moves in d 4 at the speed c . In its 3D space, it is at rest. We see the solutions in the ES diagrams, too, if we read them correctly: For instance, the two balls “r” and “b” in the left ES diagram of Figure 4 collide if d i , r = d i , b ( i = 1 ,   2 ,   3 ) and if the same proper time (!) has elapsed for both balls ( d 4 , r = d 4 , b ' ). Thus, the collision in the 3D space of “r” does not show up as a collision in the ES diagram. This fact is reasonable because only three out of four dimensions are deemed spatial.

5. Solving 15 Fundamental Mysteries of Physics

We recall: (1) An observer’s reality is created by projecting ES orthogonally to his proper space and to his proper time. (2) There is a relative 4D vector τ . (3) Cosmic time t is the correct parameter for a holistic view. In Sects. 5.1 through 5.15, ER solves 15 fundamental mysteries and declares five concepts of today’s physics obsolete.

5.1. Solving the Mystery of Time

Proper time τ is what clocks measure ( d 4 divided by c ). Cosmic time t is the total distance covered in ES divided by c . For each observer, cosmic time and proper time run the same. Only for him does his clock also measure cosmic time. Any other clock (moving or in a stronger gravitational field) is slow with respect to his clock in his proper time.

5.2. Solving the Mystery of Time’s Arrow

Time’s arrow is a synonym for “time moving only forward”. The arrow emerges from the fact that covered distance ( d 4 or total distance) cannot decrease but only increase.

5.3. Solving the Mystery of the Factor c 2 in the Energy Term m c 2

In SR, if forces are absent, the total energy E of an object is given by
E = γ m c 2 = E k i n , 3 D + m c 2
where E k i n , 3 D is its kinetic energy in an observer’s 3D space and m c 2 is its energy at rest. SR does not tell us why there is a factor c 2 in the energy of objects that in SR do not move at the speed c . ER provides the missing clue: The object is never at rest, but it moves in its d 4 ' axis. From the object’s perspective, E k i n , 3 D is zero and m c 2 is its kinetic energy in d 4 ' . The factor c 2 is a hint that it moves through ES at the speed c . In SR, there is also
E 2 = p 2 c 2 = p 3 D 2 c 2 + m 2 c 4
where p is the total momentum of an object and p 3 D is its momentum in an observer’s 3D space. Again, ER is eye-opening: From the object’s perspective, p 3 D is zero and m c is its momentum in d 4 ' . The factor c is a hint that it moves through ES at the speed c .

5.4. Solving the Mystery of Length Contraction and Time Dilation

In SR, length contraction and time dilation can be derived from the Lorentz transformation, but their physical cause remains in the dark. ER discloses that length contraction and time dilation stem from projecting ES to the axes d 1 and d 4 of an observer.

5.5. Solving the Mystery of Gravitational Time Dilation

In GR, gravitational time dilation is a feature of spacetime. ER discloses that gravitational time dilation stems from projecting ES to the d 4 axis of an observer. Equation (7) tells us: If an object accelerates in his proper space, it automatically decelerates in his proper time. Further research is required to understand other gravitational effects in ER.

5.6. Solving the Mystery of the Cosmic Microwave Background

In Sects. 5.6 through 5.11, I outline an “ER-based model of cosmology”. Distances are like numbers. In particular, they are not inflating/expanding. For some reason, there was a Big Bang. In the inflationary Lambda-CDM model based on GR, the Big Bang occurred “everywhere” because space inflated from a singularity. In the ER-based model, the Big Bang can be localized: It injected a huge amount of energy into ES all at once at an origin O, the only natural reference point. The Big Bang occurred at the cosmic time t = 0 . It was a singularity in terms of providing energy and radial momentum. Initially, all this energy receded radially from O at the speed c . Because of forces and spontaneous effects, some energy departed from its radial motion while maintaining the speed c . Today, all energy is confined to a 4D hypersphere. A lot of energy is confined to its expanding 3D hypersurface. Only three dimensions of the 4D hypersphere are deemed spatial.
Shortly after the Big Bang, energy was highly concentrated in ES. In the projection to any 3D space, a very hot and dense plasma was created. While the plasma was expanding, it cooled down. Cosmic recombination radiation (CRR) was emitted that we still observe as cosmic microwave background (CMB) today (Penzias & Wilson, 1965). At temperatures of 3,000 K, hydrogen atoms formed. The universe became increasingly transparent for the CRR. In the Lambda-CDM model, this stage was reached about 380,000 years “after” the Big Bang. In the ER-based model, these are 380,000 light years “away from” the Big Bang. The number needs to be recalculated if there was no cosmic inflation.
In the ES diagrams shown in Figure 5, Earth moves vertically at the speed c . The ER-based model must be able to answer these questions: (1) Why do we still observe the CMB today? (2) Why is the CMB nearly isotropic? (3) Why is the temperature of the CMB very low? Here are some possible answers: (1) The CRR has been scattered multiple times in d 1 ,   d 2 ,   d 3 . Some of the scattered CRR reaches an observer on Earth as CMB (in the projection to his 3D space) after having covered the same distance in d 1 ,   d 2 ,   d 3 as Earth in d 4 . The cross section for scattering is low, but the initial fluence of the CRR was high. (2) The CMB is nearly isotropic because the CRR was created and scattered equally in d 1 , d 2 , d 3 . (3) The temperature of the CMB is very low because the plasma particles had a very high recession speed v 3 D (see Section 5.7) shortly after the Big Bang.

5.7. Solving the Mystery of the Hubble–Lemaître law

According to my first postulate, all celestial bodies move through ES at the speed c . Let v 3 D be the 3D speed at which a galaxy G recedes from Earth in 3D space. Figure 5 left tells us: At the cosmic time t (the time elapsed since the Big Bang), v 3 D relates to the 3D distance D of G to Earth as c relates to the radius r of the 4D hypersphere.
v 3 D = D c / r = H t D
where H t = c / r = 1 / t is the Hubble parameter. If we observe G today at the cosmic time t = t 0 , the recession speed v 3 D and c remain unchanged. Thus, Equation (19) turns into
v 3 D = D 0 c / r 0 = H 0 D 0
where H 0 = c / r 0 = 1 / t 0 is the Hubble constant, D 0 = D   r 0 / r is today’s 3D distance of G to Earth, and r 0 is today’s radius of the 4D hypersphere. Equation (20) is the Hubble–Lemaître law (Hubble, 1929; Lemaître, 1927): The farther a galaxy is, the faster it recedes from Earth. Cosmologists are aware of the parameter H t . They are not yet aware of the 4D Euclidean geometry shown in Figure 5 and of the D 0 in Equation (20). Only ER tells us that Equations (19) and (20) stem from a simple geometry and that we must consider D 0 in Equation (20) rather than D .

5.8. Solving the Mystery of the Flat Universe

For each observer, ES is projected orthogonally to his proper space and to his proper time. Thus, he experiences two seemingly discrete structures: flat 3D space and time.

5.9. Solving the Mystery of Cosmic Inflation

Most cosmologists believe that an inflation of space shortly after the Big Bang (Linde, 1990; Guth, 1997) would explain the isotropic CMB, the flatness of the universe, and large-scale structures (inflated from quantum fluctuations). I just showed that ER explains the first two effects. ER also explains the third effect if the impacts of the quantum fluctuations have been expanding at the speed c . In ER, cosmic inflation is an obsolete concept.

5.10. Solving the Mystery of the Hubble Constant Tension

Up next, I explain why the published values of H 0 do not match (also known as the “Hubble constant tension”). I compare CMB measurements (Planck space telescope) with calibrated distance ladder measurements (Hubble space telescope). According to team A (Aghanim et al., 2020), there is H 0 = 67.66 ± 0.42   k m / s / M p c . According to team B (Riess et al., 2018), there is H 0 = 73.52 ± 1.62   k m / s / M p c . Team B made efforts to minimize the error margins in the distance measurements, but assuming a wrong cause of the redshifts gives rise to a systematic error in team B’s calculation of H 0 .
Let us assume that team A’s value of H 0 is correct. We simulate the supernova of a star S that occurred at a distance of D = 400   M p c from Earth (Figure 5 right). The recession speed v 3 D of S is calculated from measured redshifts. The redshift parameter z = Δ λ / λ tells us how each wavelength λ of the supernova’s light is either passively stretched by an expanding space (team B)—or else redshifted by the Doppler effect of actively receding objects (ER-based model). The supernova occurred at the cosmic time t (arc called “past”), but we observe the supernova at the cosmic time t 0 (arc called “present”). Thus, all redshift data stem from a cosmic time t < t 0 when there was r < r 0 and H t > H 0 . While the supernova’s light moved the distance D in the d 1 axis, Earth moved the same distance D but in the d 4 axis (same speed, my first postulate). There is
1 / H t = r / c = ( r 0 − D ) / c = 1 / H 0 − D / c
For a very short distance of D = 400   k p c , Equation (21) tells us that H t deviates from H 0 by only 0.009 percent. However, when plotting v 3 D versus D for distances from 0 Mpc to 500 Mpc in steps of 25 Mpc (red points in Figure 6), the slope of a straight-line fit through the origin is roughly 10 percent greater than H 0 . Since team B calculates H 0 from similar but mirrored plots (magnitude versus z ), its value of H 0 is roughly 10 percent too high. This solves the Hubble constant tension. Team B’s value is not correct because, according to Equation (20), we must plot v 3 D versus D 0 (blue points in Figure 6) to get a straight line.
Since we are not able to measure D 0 (observable magnitudes relate to D rather than to D 0 ), the easiest way to fix the calculation of team B is to rewrite Equation (20) as
v 3 D , 0 = D c / r 0 = H 0 D
where v 3 D , 0 is today’s 3D speed of another star S 0 (Figure 5 right) that happens to be at the same distance D today at which the supernova of star S occurred. I kindly ask team B to recalculate H 0 after converting all v 3 D to v 3 D , 0 . Equation (21) tells us how to do so.
H t = H 0 c / ( c − H 0 D ) = H 0 / ( 1 − v 3 D , 0 / c )
v 3 D , 0 = v 3 D / ( 1 + v 3 D / c )
By applying Equation (24), all red points in Figure 6 drop down to the points marked in blue. Of course, team B is well aware that the supernova’s light was emitted in the past, but all that counts in the Lambda-CDM model is the timespan during which the light is moving to Earth. Along the way, each wavelength is continuously stretched by expanding space. The parameter z increases during the journey. In the ER-based model, all that counts is the moment when the supernova occurred. Each wavelength is initially redshifted by the Doppler effect. The parameter z remains constant during the journey. It is tied up when the supernova occurs. Space is not expanding. A 3D hypersurface made up of energy (!) is expanding in ES. In ER, expanding space is an obsolete concept.

5.11. Solving the Mystery of an Accelerating Expansion of Space

Team B can fix the systematic error in its calculation of H 0 by converting all v 3 D to v 3 D , 0 according to Equation (24). I now reveal another systematic error, but it is inherent in the Lambda-CDM model. It stems from assuming an accelerating expansion of space and can be fixed only by replacing this model with the ER-based model—unless we postulate dark energy. Perlmutter et al. (1998) and Riess et al. (1998) advocate an accelerating expansion because the calculated recession speeds deviate from Equation (20) and the deviations increase with distance. An acceleration would stretch each wavelength even further.
In ER, these deviations are much easier to understand: The older the redshift data are, the more H t deviates from H 0 , and the more v 3 D deviates from v 3 D , 0 . If another star S 0 (Figure 5 right) happens to be at the same distance of D = 400   M p c today at which the supernova of star S occurred, Equation (24) tells us that S 0 recedes more slowly (27,064 km/s) from Earth than S (29,750 km/s). As long as cosmologists are not aware of the 4D Euclidean geometry, they attribute the deviations from Equation (20) to an accelerating expansion of space caused by dark energy. But dark energy has never been observed. It is a stopgap for an effect that the Lambda-CDM model cannot explain.
For D > 500   M p c , the red points in Figure 6 run away from the straight line. The Hubble constant tension and dark energy are solved with the same clue: In Equation (20), we must not confuse D 0 with D . Because of Equation (19) and H t = c / ( r 0 − D ) , the recession speed v 3 D is not proportional to D but to D / ( r 0 − D ) . The illusion of an accelerating expansion stems from confusing D 0 with D (see Figure 6). Any expansion of space—uniform or else accelerating—is only virtual. There is no accelerating expansion of space even if a Nobel Prize in Physics was given “for the discovery of the accelerating expansion of the Universe through observations of distant supernovae” (The Nobel Foundation, 2011). There are two misconceptions in these words of praise: (1) In the Lambda-CDM model, Universe implies space, but space is not expanding. (2) All but the nearest galaxies recede from Earth, but they do so uniformly. There is no acceleration. In ER, dark energy is an obsolete concept.
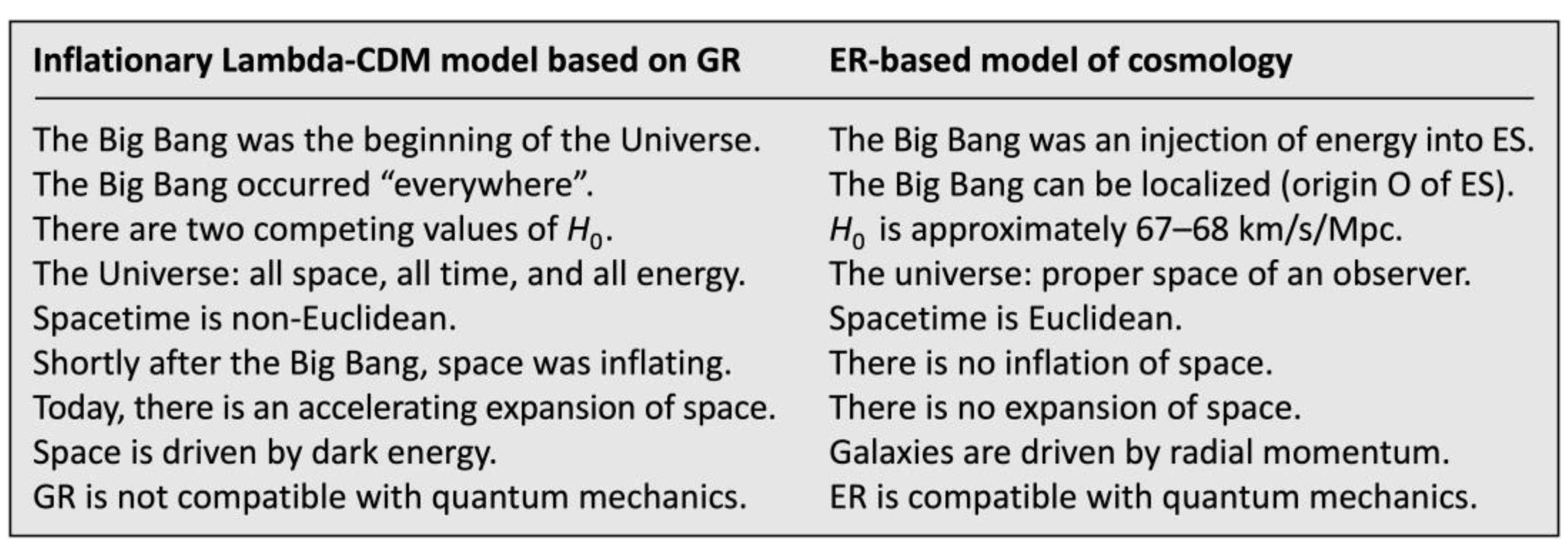
Radial momentum provided by the Big Bang drives all galaxies away from the origin O of ES. They are driven by themselves rather than by dark energy. Table 1 compares two models of cosmology. Be aware that “Universe” (Lambda-CDM model) is not the same as “universe” (ER-based model). Proper space and thus the universe are relative! In the next sections, ER turns out to be compatible with QM. Since quantum gravity is meant to make GR compatible with QM, I conclude: In ER, quantum gravity is an obsolete concept.

5.12. Solving the Mystery of the Wave–Particle Duality

The wave–particle duality was first discussed by Bohr and Heisenberg (Heisenberg, 1969) and has bothered physicists ever since. Electromagnetic waves are oscillations of an electromagnetic field, which propagate through an observer’s 3D space at the speed c . In some experiments, objects behave like waves. In other experiments, the very same objects behave like particles (also known as the “wave–particle duality”). In today’s physics, one object cannot be wave and particle at once because the energy of a wave is distributed in space, while the energy of a particle is always localized in space.
We now solve the duality by replacing waves and particles with the objective concept “pure energy”. To illustrate pure energy (Figure 7), I coin a new word: “wavematter”. In an observer’s reality (external view), a wavematter appears as a wave packet or as a particle. As a wave, it propagates in his x 1 axis at the speed c and it oscillates in his axes x 2 and x 3 (electromagnetic field). The wave propagates and oscillates as a function of coordinate time because we talk about an observer’s reality. In its own reality (internal view), the axis of the wavematter’s 4D motion disappears because of length contraction at the speed c . It deems itself particle at rest. Be aware that “wavematter” is not just another word for the duality. It is an objective concept of energy that finally takes the internal view of photons into account. In today’s physics, there is no internal view of a photon.
Like space and time, waves and particles are subjective concepts: What I deem wave, deems itself particle at rest. Albert Einstein (1905c) taught that energy is equivalent to mass. It is the same equivalence that also causes the wave–particle duality and that inspired me to coin the word “wavematter”. Since each wavematter moves at the speed c , the axis of its 4D motion disappears for itself. From its perspective (that is, in its own reality), all of its energy “condenses” to what we call “mass” in today’s physics.
In a double-slit experiment, wavematters pass through a double-slit and produce an interference pattern on a screen. An observer deems them wave packets as long as he does not track through which slit each wavematter is passing. Here the external view applies. The photoelectric effect is different. Of course, one can externally witness how one photon releases an electron from a metal surface. However, the physical effect—do I have enough energy to release an electron?—is all up to the photon. Only if the photon energy exceeds the binding energy of an electron is this electron released. Here the photon’s internal view is the decisive view. This is why the photon behaves like a particle.
The duality is also observed in matter, such as electrons (Jönsson, 1961). An electron is a wavematter, too. If the electron is not tracked, it behaves like a wave. If the electron is tracked, it behaves like a particle. Since an observer automatically tracks objects that are slow in his 3D space, he deems all slow objects—and thus all macroscopic objects—matter rather than waves. To improve readability, I do not draw wavematters in my ES diagrams. I draw what they are deemed by observers: clocks, rockets, celestial bodies, etc.

5.13. Solving the Mystery of Entanglement

The term “entanglement” was coined by Schrödinger (1935) in his comment on the Einstein–Podolsky–Rosen paradox (Einstein et al., 1935). These three authors argued that QM would not provide a complete description of reality. Schrödinger’s word creation did not solve the paradox but demonstrates our difficulties in comprehending QM. Bell (1964) showed that local hidden-variable theories are not compatible with QM. In experiments (Freedman & Clauser, 1972; Aspect et al., 1982; Bouwmeester et al., 1997), entanglement violates locality. Ever since, entanglement has been considered a non-local effect.
Up next, we untangle entanglement without the concept of non-locality. All we need is ER: The objective concept “pure distance” makes non-locality obsolete. Figure 8 illustrates two wavematters that were created at once at a point P. They move away from each other in opposite directions ± d 4 ' at the speed c . It turns out that the wavematters are automatically entangled. For an observer moving in any direction other than ± d 4 ' (external view), the two wavematters are spatially separated. The observer cannot understand how these wavematters are able to communicate with each other in no time.
For each wavematter (internal view), the ± d 4 ' axis disappears because of length contraction at the speed c . In their common (!) proper space spanned by d 1 ' ,   d 2 ' ,   d 3 ' , either of them is at the same position as its twin. From the internal view, the twins have never been separated, but their proper time flows in opposite 4D directions. The twins communicate with each other in no time because they are spatially united in their proper space. There is a “spooky action at a distance” from the external view only. Entanglement occurs because the proper spaces of an observer and of an observed object may be different. This is possible only if we can interchange all four dimensions as in “pure distance”. ER also explains the entanglement of electrons or atoms. For an observer, they move at a speed v 3 D < c . In their ± d 4 ' axis, they move at the speed c . Any measurement tilts the axis of 4D motion of one twin and destroys the entanglement. In ER, non-locality is an obsolete concept.

5.14. Solving the Mystery of Spontaneous Effects

In spontaneous emission, a photon is emitted by an excited atom. Prior to the emission, the photon energy moves with the atom. After the emission, this energy moves by itself. Today’s physics cannot explain how this energy is boosted to the speed c in no time. In ES, both atom and photon move at the speed c . Thus, there is no need to boost any energy to the speed c . All it takes is energy whose 4D motion at the speed c flips spontaneously into an observer’s 3D space. In absorption, a photon is spontaneously absorbed by an atom. Today’s physics cannot explain how the photon energy is slowed down to the speed of an atom in no time. In ES, both photon and atom move at the speed c . Thus, there is no need to slow down any energy. There are similar arguments for pair production and annihilation. Spontaneity is another clue that all energy moves through ES at the speed c .

5.15. Solving the Mystery of the Baryon Asymmetry

In the Lambda-CDM model, almost all matter was created shortly after the Big Bang. Only then was the temperature high enough to enable pair production. However, baryons and antibaryons should have annihilated each other again because the energy density was also very high. Since we observe more baryons than antibaryons today (also known as the “baryon asymmetry”), it is assumed that more baryons were created shortly after the Big Bang (Canetti et al., 2012). However, pair production should create baryons and antibaryons equally. Right here, the ER-based model scores again: Since each wavematter deems itself particle at rest, the Big Bang injected a huge number of particles into ES. The baryon asymmetry was caused by the Big Bang and is not affected by pair production.
But why do wavematters not deem themselves antiparticles at rest? Well, antiparticles are not the opposite of particles but particles with the opposite electric charge. There is a reasonable character paradox: What I deem antiparticle, deems itself particle. Antiparticles only seem to flow backward in time because proper time flows in opposite 4D directions for any two wavematters created in pair production. ER tells us that these two wavematters are automatically entangled. This gives us an opportunity to falsify ER.

6. Conclusions

ER solves mysteries that have not been solved in 100+ years—or else that have been solved but with concepts that are obsolete in ER: cosmic inflation, expanding space, dark energy, quantum gravity, non-locality. Today’s physics needs these concepts to make cosmology and QM work, but Occam’s razor shaves them off. Thus, physics would be well advised to accept ER. This implies: (1) We limit the scope of SR/GR to an observer’s reality. (2) We internalize that the master reality ES (described by ER) is beyond each observer’s reality (described by SR/GR). (3) In cosmology and QM, we dismiss all obsolete concepts and we build only on objective concepts, such as pure distance and pure energy.
SR/GR are considered two of the greatest achievements of physics because they have been confirmed many times over. I showed that SR/GR do not provide a holistic view, and I can imagine that this constraint is causing the stagnation in today’s physics. Physics got stuck in its own concepts. ER solves 15 mysteries of physics geometrically—without field equations. This tells us that there is a lot more to uncover beyond SR/GR. I consider it very unlikely that 15 solutions in various (!) areas of physics are nothing but 15 coincidences. Only in ES does Mother Nature disclose her secrets. If we think of each observer’s reality as an oversized stage, the key to understanding nature is beyond the stage.
It was a wise decision to award Albert Einstein the Nobel Prize for his theory of the photoelectric effect (Einstein, 1905a) and not for SR/GR. ER penetrates to a deeper level. Einstein—one of the most brilliant physicists ever—failed to realize that the fundamental metric chosen by Mother Nature is Euclidean. Einstein sacrificed absolute space and time. I sacrifice the absolute nature of waves and particles, but I restore absolute, cosmic time. For the first time ever, mankind understands the nature of time: Cosmic time is the total distance covered in ES divided by c . The human brain is able to imagine that we move through ES at the speed c . With that said, conflicts of mankind become all so small.
Is ER a physical or a metaphysical theory? This is a very good question because only in proper coordinates can we access ES, but the proper coordinates of other objects cannot be measured. Physics is the science of describing the universe and its interior. Our primary source of knowledge is observing, but—if we limit physics to observing—even cosmology and QM would be metaphysical. They are built on assumptions that go beyond observing. Observing is always wedded to egocentric perspectives that may give rise to mysteries. ER solves the Hubble constant tension and entanglement by describing nature in her own, objective, natural concepts (pure distance, pure energy) rather than in the concepts of an observer. Since ER helps us understand what we observe, it is a physical theory.
Final remarks: (1) In this paper, I only touched on gravitation. Once again, I kindly ask you to be patient and fair. We should not reject ER because gravitation is still an issue. GR seems to solve gravitation, but GR is not compatible with QM—unless we formulate quantum gravity or the like. (2) Einstein derived SR from a few measurement instructions. We cannot derive ER the same way because the proper coordinates of other objects cannot be measured. This is why I introduced ER by defining its metric in Equation (4). (3) Absolute, cosmic time brings speculations about time travel to an end. Is there any other theory that solves time’s arrow, the Hubble constant tension, and entanglement as beautifully as ER? (4) To cherish the beauty of ER, we must learn to work with it. We must not ask in physics: Why is our reality a projection or a probability function? Dark energy and non-locality are far more speculative than projections. (5) It looks like Plato’s Allegory of the Cave is correct: Mankind experiences projections that are blurred—because of QM.
It is not by chance that the author of this paper is an experimental physicist. It seems to me that SR and GR are not suspicious to theorists. Several prominent theorists told me that ER would be nonsense. I laid the groundwork for ER and showed how powerful it is. Paradoxes are only virtual. The true pillars of physics are ER and QM. Together, they describe the very large and the very small. Requesting a holistic view of nature is what I consider the most innovative part of this paper. A holistic view should outperform the egocentric views of single observers. I demonstrated that it does. Now everyone is welcome to solve even more mysteries in ER. May ER get the broad acceptance that it deserves!

Funding

No funds, grants, or other support was received.

Acknowledgements

I would like to thank Siegfried W. Stein for his contributions to Section 5.10 and for the Figs. 2 and 5 (partly). After several unsuccessful submissions, he eventually decided to withdraw his co-authorship. I also thank Matthias Bartelmann, Dirk Rischke, Jürgen Struckmeier, and Andreas Wipf for some valuable comments. In particular, I thank all reviewers and editors for the precious time that they spent on my manuscript.

Comments

It takes open-minded, courageous editors and reviewers to evaluate a theory that comes with a paradigm shift. Whoever adheres to established concepts is paralyzing the scientific progress. I did not surrender when my paper was rejected by several journals. Interestingly, I was never given any solid arguments. Rather, I was asked to try a different journal. Were the editors dazzled by the success of SR/GR? Did they underestimate the benefits of ER? Even friends refused to support me. However, each setback inspired me to work out the benefits of ER even better. Finally, I succeeded in disclosing an issue in SR/GR and in formulating a new theory that is even more general than GR. These comments shall encourage young scientists to stand up for promising ideas, but be aware that opposing the mainstream is exhausting. Here are some statements that I received from top journals: “Unscholarly research.” “Fake science.” “Too simple to be true.” Well, just as the retrograde loops are obsolete in the heliocentric model, so is the calculus of GR obsolete in ER. The editor-in-chief of a top journal replied: “Publishing is for experts only.” arXiv suspended my submission privileges. Simple and true are not mutually exclusive. Beauty is when they go hand in hand.

Conflict of Interest

The author has no competing interests to declare.

Ethical Approval

not applicable.

References

  1. Abbott, B. P.; et al. (2016). Observation of gravitational waves from a binary black hole merger. Physical Review Letters, 116(6), 061102. [CrossRef]
  2. Aghanim, N.; et al. (2020). Planck 2018 results. VI. Cosmological parameters. Astronomy & Astrophysics, 641, A6. [CrossRef]
  3. Almeida, J. B. (2001). An alternative to Minkowski space-time. arXiv:gr-qc/0104029. [CrossRef]
  4. Ashby, N. (2003). Relativity in the global positioning system. Living Reviews in Relativity, 6(1), 1–42. [CrossRef]
  5. Aspect, A., Dalibard, J., & Roger, G. (1982). Experimental test of Bell’s inequalities using time-varying analyzers. Physical Review Letters, 49(25), 1804–1807. [CrossRef]
  6. Bell, J. S. (1964). On the Einstein Podolsky Rosen paradox. Physics, 1(3), 195–200. [CrossRef]
  7. Bouwmeester, D.; et al. (1997). Experimental quantum teleportation. Nature, 390, 575–579. [CrossRef]
  8. Canetti, L., Drewes, M., & Shaposhnikov, M. (2012). Matter and antimatter in the universe. New Journal of Physics, 14, 095012. [CrossRef]
  9. Dyson, F. W., Eddington, A. S., & Davidson, C. (1920). A determination of the deflection of light by the sun’s gravitational field, from observations made at the total eclipse of May 29, 1919. Philosophical Transactions of the Royal Society A, 220, 291–333. [CrossRef]
  10. Einstein, A. (1905a). Über einen die Erzeugung und Verwandlung des Lichtes betreffenden heuristischen Gesichtspunkt. Annalen der Physik, 322(6), 132–148. [CrossRef]
  11. Einstein, A. (1905b). Zur Elektrodynamik bewegter Körper. Annalen der Physik, 322(10), 891–921. [CrossRef]
  12. Einstein, A. (1905c). Ist die Trägheit eines Körpers von seinem Energieinhalt abhängig? Annalen der Physik, 323(13), 639–641. [CrossRef]
  13. Einstein, A. (1916). Die Grundlage der allgemeinen Relativitätstheorie. Annalen der Physik, 354(7), 769–822. [CrossRef]
  14. Einstein, A., Podolsky, B., & Rosen, N. (1935). Can quantum-mechanical description of physical reality be considered complete? Physical Review, 47(10), 777–780. [CrossRef]
  15. Freedman, S. J., & Clauser, J. F. (1972). Experimental test of local hidden-variable theories. Physical Review Letters, 28(14), 938–941. [CrossRef]
  16. Gersten, A. (2003). Euclidean special relativity. Foundations of Physics, 33(8), 1237–1251. [CrossRef]
  17. Guth, A. H. (1997). The inflationary universe. Perseus Books.
  18. Hafele, J. C., & Keating, R. E. (1972). Around-the-world atomic clocks: Predicted relativistic time gains. Science, 177, 166–168. [CrossRef]
  19. Heisenberg, W. (1969). Der Teil und das Ganze. Piper.
  20. Hubble, E. (1929). A relation between distance and radial velocity among extra-galactic nebulae. Proceedings of the National Academy of Sciences of the United States of America, 15(3), 168–173. [CrossRef]
  21. Jönsson, C. (1961). Elektroneninterferenzen an mehreren künstlich hergestellten Feinspalten. Zeitschrift für Physik, 161, 454–474. [CrossRef]
  22. Kant, I. (1781). Kritik der reinen Vernunft. Hartknoch.
  23. Lemaître, G. (1927). Un univers homogène de masse constante et de rayon croissant, rendant compte de la vitesse radiale des nébuleuses extra-galactiques. Annales de la Société Scientifique de Bruxelles A, 47, 49–59.
  24. Linde, A. (1990). Inflation and quantum cosmology. Academic Press.
  25. Minkowski, H. (1910). Die Grundgleichungen für die elektromagnetischen Vorgänge in bewegten Körpern. Mathematische Annalen, 68, 472–525. [CrossRef]
  26. Montanus, J. M. C. (1991). Special relativity in an absolute Euclidean space-time. Physics Essays, 4(3), 350–356.
  27. Montanus, J. M. C. (2001). Proper-time formulation of relativistic dynamics. Foundations of Physics, 31(9), 1357–1400. [CrossRef]
  28. Montanus, H. (2023, September 23). Proper Time as Fourth Coordinate. ISBN 978-90-829889-4-9. Retrieved May 1, 2024, from https://greenbluemath.nl/proper-time-as-fourth-coordinate/.
  29. Newburgh, R. G., & Phipps Jr., T. E. (1969). A space–proper time formulation of relativistic geometry. Physical Sciences Research Papers (United States Air Force), no. 401.
  30. Newton, I. (1687). Philosophiae naturalis principia mathematica. Joseph Streater.
  31. Niemz, M. H. (2020). Seeing our world through different eyes. Wipf and Stock. Original German version: Niemz, M. H. (2020). Die Welt mit anderen Augen sehen. Gütersloher Verlagshaus.
  32. Penzias, A. A., & Wilson, R. W. (1965). A measurement of excess antenna temperature at 4080 Mc/s. The Astrophysical Journal, 142, 419–421. https://doi.org/10.1086/148307. [CrossRef]
  33. Perlmutter, S.; et al. (1998). Measurements of Ω and Λ from 42 high-redshift supernovae. arXiv:astro-ph/9812133. [CrossRef]
  34. Riess, A. G.; et al. (1998). Observational evidence from supernovae for an accelerating universe and a cosmological constant. The Astronomical Journal, 116(3), 1009–1038. [CrossRef]
  35. Riess, A. G.; et al. (2018). Milky Way Cepheid standards for measuring cosmic distances and application to Gaia DR2. The Astrophysical Journal, 861(2), 126. [CrossRef]
  36. Rossi, B., & Hall, D. B. (1941). Variation of the rate of decay of mesotrons with momentum. Physical Review, 59(3), 223–228. [CrossRef]
  37. Ryder, L. H. (1985). Quantum field theory. Cambridge University Press.
  38. Schrödinger, E. (1935). Die gegenwärtige Situation in der Quantenmechanik. Naturwissenschaften, 23, 807–812. [CrossRef]
  39. The Nobel Foundation (2011). The Nobel Prize in Physics 2011. Retrieved May 1, 2024, from https://www.nobelprize.org/prizes/physics/2011/summary/.
  40. van Linden, R. (2023). Euclidean relativity. Retrieved May 1, 2024, from https://euclideanrelativity.com.
  41. Weyl, H. (1928). Gruppentheorie und Quantenmechanik. Hirzel.
  42. Wick, G. C. (1954). Properties of Bethe-Salpeter wave functions. Physical Review, 96(4), 1124–1134. [CrossRef]
Preprints 107114 g001
Figure 1. Minkowski diagram and ES diagram of two identical clocks “r” (red) and “b” (blue). Left: In SR, “b” is slow with respect to “r” in t ' . Coordinate time is relative (“b” is not at the same positions in c t and c t ' ). Right: In ER, “b” is slow with respect to “r” in d 4 . Cosmic time is absolute (“r” is in d 4 at the same position as “b” in d 4 ' ). Only the ES diagram is rotationally symmetric.
Figure 1. Minkowski diagram and ES diagram of two identical clocks “r” (red) and “b” (blue). Left: In SR, “b” is slow with respect to “r” in t ' . Coordinate time is relative (“b” is not at the same positions in c t and c t ' ). Right: In ER, “b” is slow with respect to “r” in d 4 . Cosmic time is absolute (“r” is in d 4 at the same position as “b” in d 4 ' ). Only the ES diagram is rotationally symmetric.
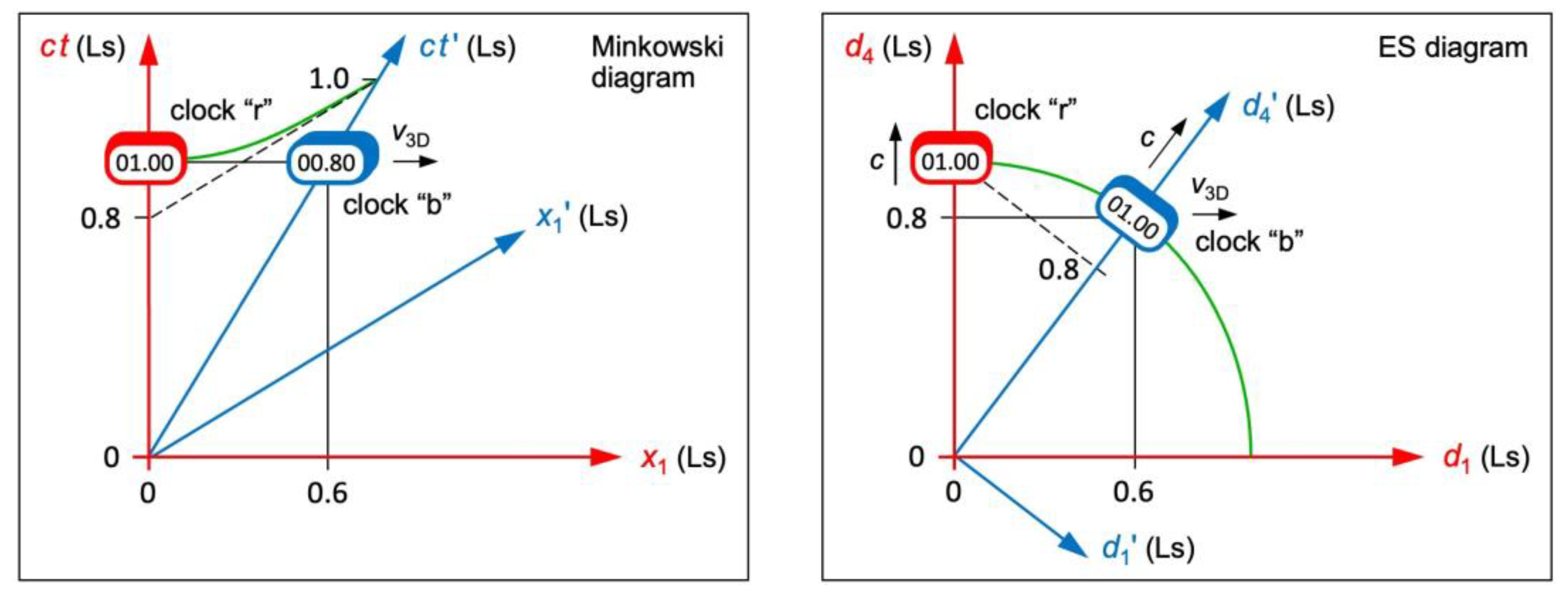
Preprints 107114 g001
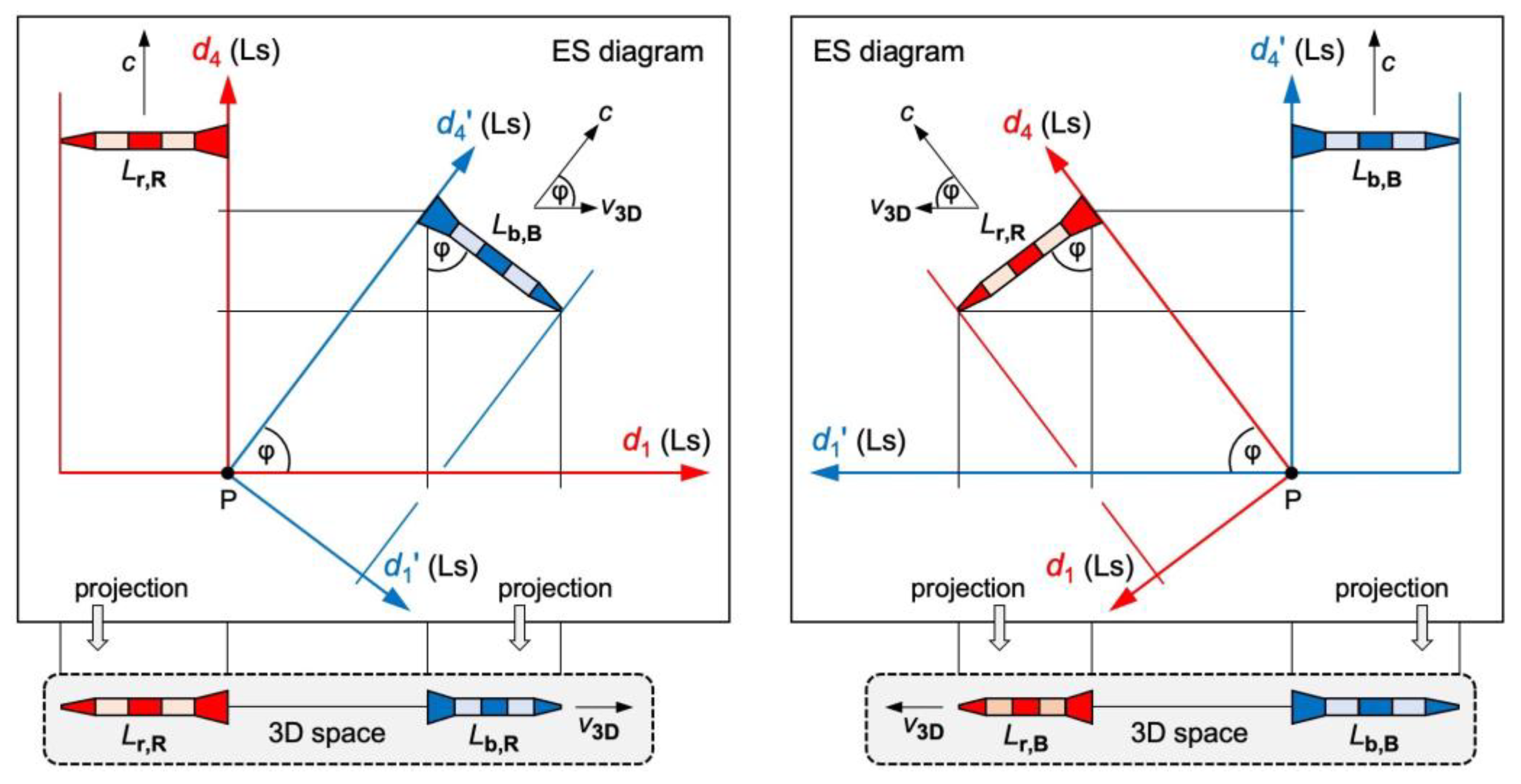
Preprints 107114 g002
Figure 2. ES diagrams and 3D projections of two rockets “r” (red) and “b” (blue). Top: Both rockets move in different 4D directions at the speed c . Bottom left: Projection to the 3D space of R. Rocket “b” contracts to L b , R . Bottom right: Projection to the 3D space of B. Rocket “r” contracts to L r , B .
Figure 2. ES diagrams and 3D projections of two rockets “r” (red) and “b” (blue). Top: Both rockets move in different 4D directions at the speed c . Bottom left: Projection to the 3D space of R. Rocket “b” contracts to L b , R . Bottom right: Projection to the 3D space of B. Rocket “r” contracts to L r , B .
Preprints 107114 g002
Preprints 107114 g003
Figure 3. ES diagram of two identical clocks “r” (red) and “b” (blue). Clock “r” and Earth move in the d 4 axis of “r” at the speed c . Clock “b” accelerates in the d 1 axis of “r” toward Earth.
Figure 3. ES diagram of two identical clocks “r” (red) and “b” (blue). Clock “r” and Earth move in the d 4 axis of “r” at the speed c . Clock “b” accelerates in the d 1 axis of “r” toward Earth.
Preprints 107114 g003
Preprints 107114 g004
Figure 4. Solving three instructive problems in ER. Each snapshot shows one instant in cosmic time. Left: The blue ball “b” is hit toward the red ball “r”. In the projection, the two balls collide. Center: Some rocket moves along a guide wire. In the projection, the wire does not escape from the rocket. Right: Earth orbits the sun. In the projection, the sun does not escape from the orbital plane.
Figure 4. Solving three instructive problems in ER. Each snapshot shows one instant in cosmic time. Left: The blue ball “b” is hit toward the red ball “r”. In the projection, the two balls collide. Center: Some rocket moves along a guide wire. In the projection, the wire does not escape from the rocket. Right: Earth orbits the sun. In the projection, the sun does not escape from the orbital plane.
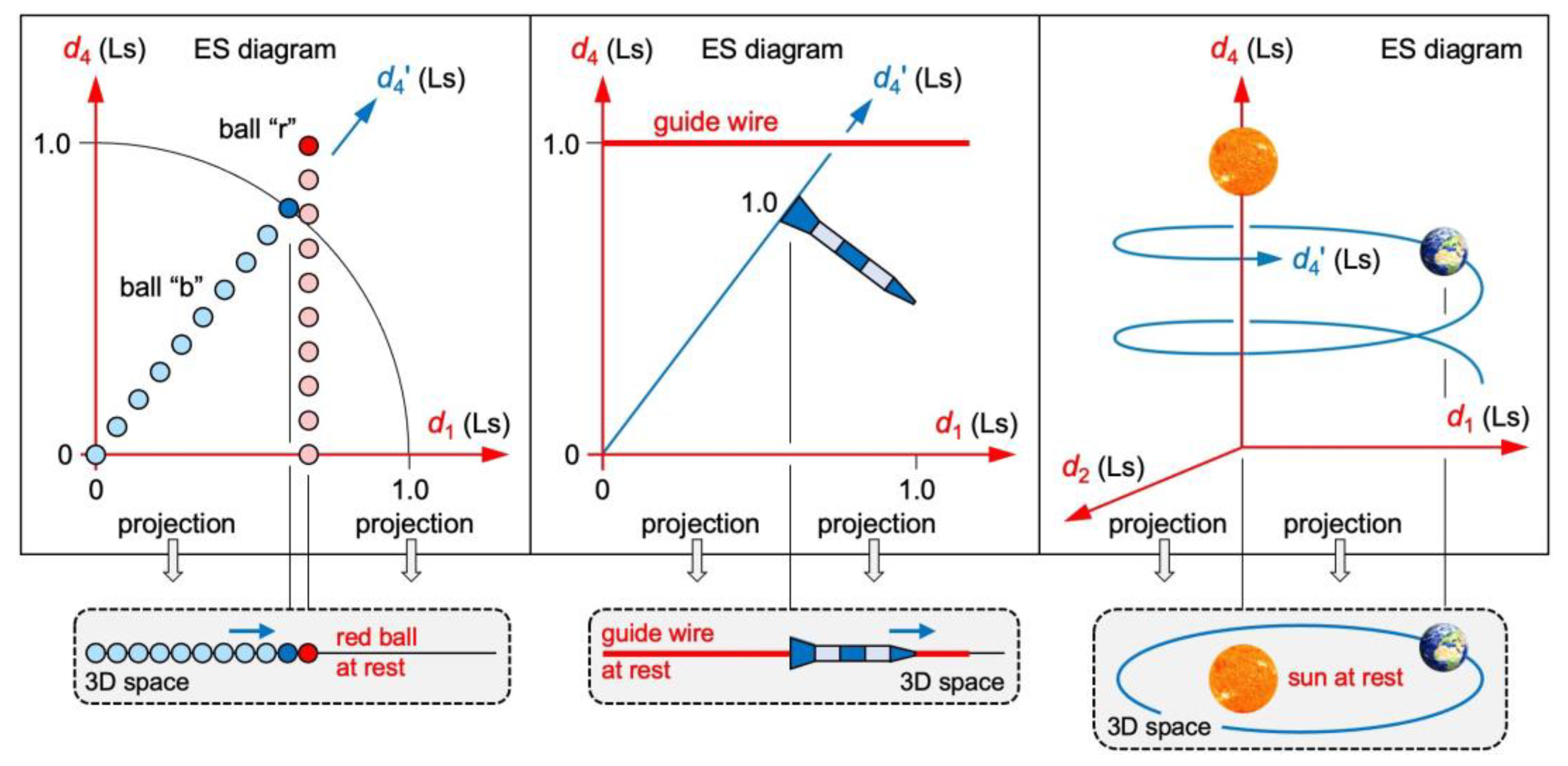
Preprints 107114 g004
Preprints 107114 g005
Figure 5. Solving the mysteries 5.6, 5.7, 5.10, and 5.11. The circular arcs are part of an expanding 3D hypersurface. Left: The galaxy G recedes from Earth at the 3D speed v 3 D . Right: The supernova of a star S occurred at a distance of D = 400   M p c from Earth. If another star S 0 happens to be at the same distance D today, S 0 recedes more slowly from Earth than S .
Figure 5. Solving the mysteries 5.6, 5.7, 5.10, and 5.11. The circular arcs are part of an expanding 3D hypersurface. Left: The galaxy G recedes from Earth at the 3D speed v 3 D . Right: The supernova of a star S occurred at a distance of D = 400   M p c from Earth. If another star S 0 happens to be at the same distance D today, S 0 recedes more slowly from Earth than S .
Preprints 107114 g005
Preprints 107114 g006
Figure 6. Hubble diagram of simulated supernovae at distances up to 1250 Mpc. The horizontal axis is D or else D 0 . Only Equation (20) yields a straight line. Equation (19) does not because H t is not a constant.
Figure 6. Hubble diagram of simulated supernovae at distances up to 1250 Mpc. The horizontal axis is D or else D 0 . Only Equation (20) yields a straight line. Equation (19) does not because H t is not a constant.
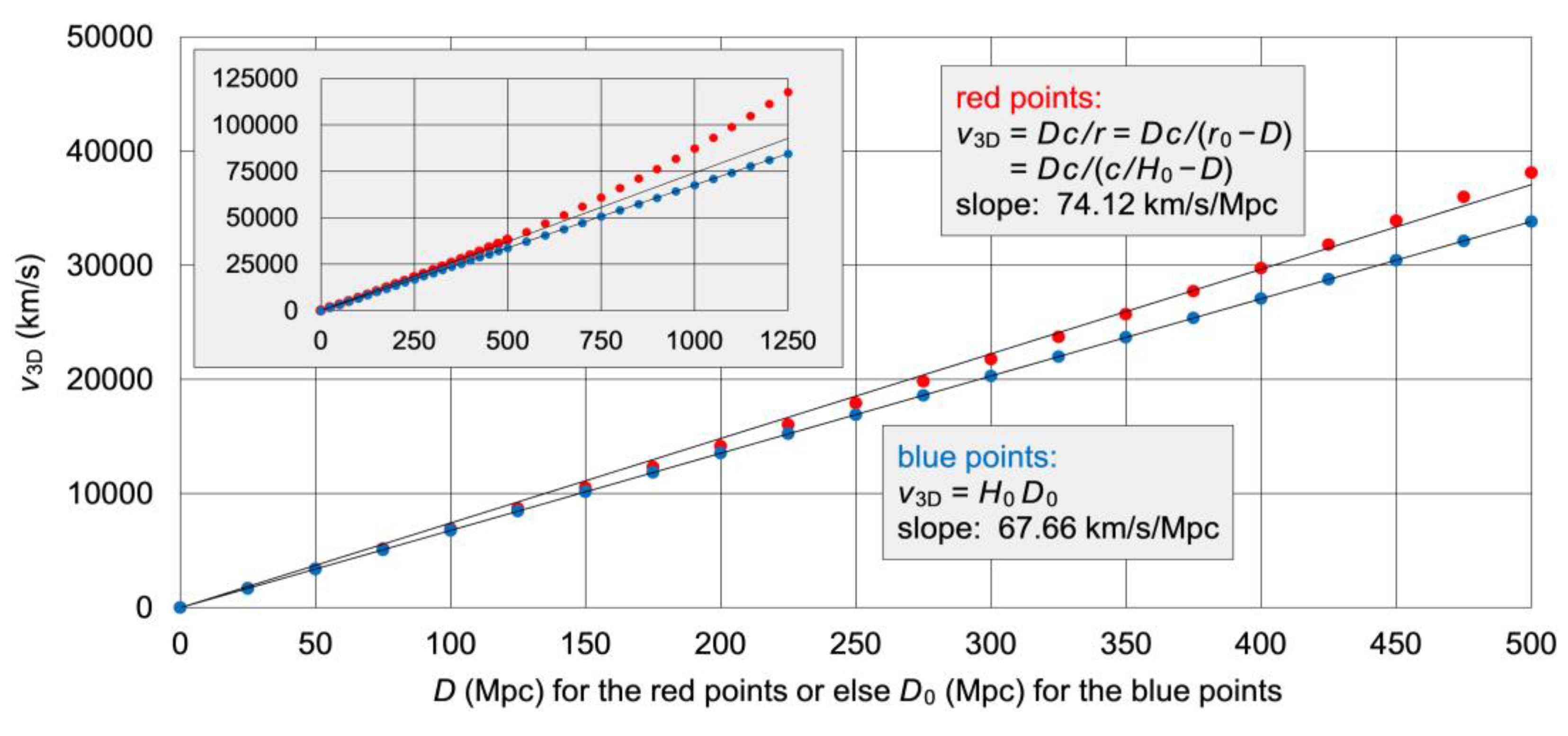
Preprints 107114 g006
Preprints 107114 g007
Figure 7. Illustration of a wavematter. In an observer’s reality (external view), a wavematter appears as a wave packet or as a particle. As a wave (shown here), it propagates and oscillates as a function of coordinate time. In its own reality (internal view), the axis of the wavematter’s 4D motion disappears because of length contraction at the speed c . It deems itself particle at rest.
Figure 7. Illustration of a wavematter. In an observer’s reality (external view), a wavematter appears as a wave packet or as a particle. As a wave (shown here), it propagates and oscillates as a function of coordinate time. In its own reality (internal view), the axis of the wavematter’s 4D motion disappears because of length contraction at the speed c . It deems itself particle at rest.
Preprints 107114 g007
Preprints 107114 g008
Figure 8. Two wavematters moving in ± d 4 ' at the speed c are spatially separated for an observer who moves in any direction other than ± d 4 ' (external view). For each wavematter (internal view), the ± d 4 ' axis disappears. In their common proper space, both wavematters are spatially united.
Figure 8. Two wavematters moving in ± d 4 ' at the speed c are spatially separated for an observer who moves in any direction other than ± d 4 ' (external view). For each wavematter (internal view), the ± d 4 ' axis disappears. In their common proper space, both wavematters are spatially united.
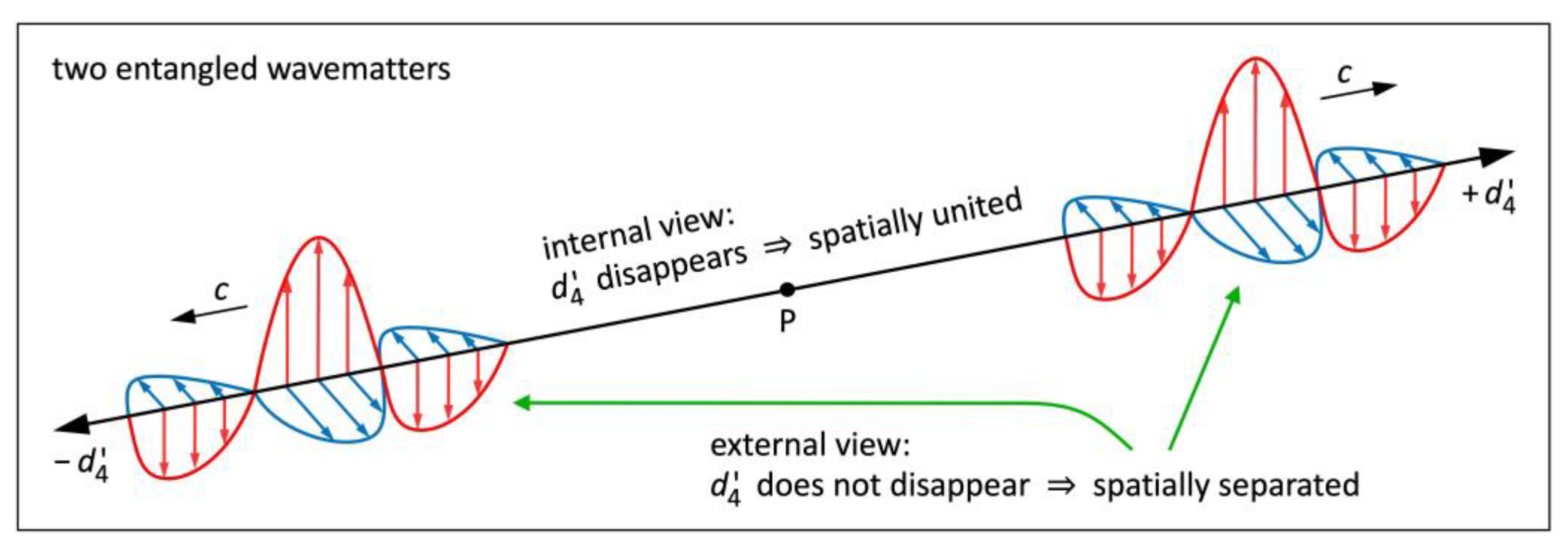
Preprints 107114 g008
Table 1. Comparing two different models of cosmology.
Table 1. Comparing two different models of cosmology.
Preprints 107114 i001
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content.
Copyright: This open access article is published under a Creative Commons CC BY 4.0 license, which permit the free download, distribution, and reuse, provided that the author and preprint are cited in any reuse.
Prerpints.org logo

Preprints.org is a free preprint server supported by MDPI in Basel, Switzerland.

facebook logotwitter logolinkedin logo
MDPI Initiatives
Important Links
Subscribe

Disclaimer

Terms of Use

Privacy Policy

© 2024 MDPI (Basel, Switzerland) unless otherwise stated