Preprint
Article
Dirac Fermion of a Monopole Pair (MP) Model
This version is not peer-reviewed.
Submitted:
17 September 2024
Posted:
18 September 2024
Read the latest preprint version here
Abstract
The electron of superposition states of spin-charge, ±1/2 in probabilistic distribution about a nucleus of an atom is described by non-relativistic Schrödinger wave equation. The equation relates to conservation of energy into space-time and is pertinent to quantum mechanics (QM). Transformation of the electron to Dirac fermion of a complex four-component spinor is by Dirac belt trick and this pursued by relativistic quantum field theory (QFT). How to combine both QM and QFT into 4D space-time at singularity remains lacking without the development of a proper theory of quantum gravity. In this study, how both QM and QFT are integrated within a proposed MP model of hydrogen type of 4D space-time on a geometry perspective is examined. The electron transformation to Dirac fermion appears consistent with Dirac belt trick while sustaining superposition of spin-charge and wave-particle duality. Such a model appears dynamic and its relatable to some basic aspects of both QM and QFT like Dirac theory, wave function collapse, quantized Hamiltonian, non-relativistic wave function, Weyl spinor, Lorentz transformation and electroweak symmetry breaking mechanism. These are succinctly plotted to be explored further into more depth the conventional way in both experiments and theoretical applications.
Keywords:
Dirac fermion
; Quantum mechanics
; Quantum field theory
; Dirac belt-trick
; 4D space-time
Applications of Photonics, Laser, Plasma and Radiation Physics
1. Introduction
Based on QM, the electron of half-integer spin of superposition and its shift in space of probability distribution about the nucleus of an atom is described by Schrödinger wave equation [1]. Its wave-particle duality is depended on the instrumental set-up [2,3] and the electron absorbs and emits energy in quantized form, of infinitesimal steps. By light-matter coupling for observational purposes, any differences to photon’s spin, charge and mass-energy equivalence by, provide the inherent properties of particles such as electron and this is termed causality [4,5]. From this characterization, the effect of the electron as excitation of electron field permeating space based on QFT can be instantaneous over a distance for correlating fields of spin, ±1/2 after splitting or alternatively translated by a pair of photons. However, the idea of wavy form of electron permeating space without interactions and this somehow collapses to a point at observation still remains an abstract concept [6]. Despite such setback, the preferred quest is to make non-relativistic equations become relativistic as all quantum observations is depended on light-matter interactions and their measurements.
Beginning with Klein-Gordon equation [7], the energy and momentum operators of Schrödinger equation,
are adapted in the expression,
Equation (1) is fundamental to the conservation of energy in space-time within the atom and it cannot be derived by QFT. Equation (2) incorporates special relativity, for mass-energy equivalence, is the del operator in 3D space, ℏ is reduced Planck constant and is an imaginary number, . Only one component is considered in Equation (2) and it does not take into account the negative energy contribution from antimatter. In contrast, the Hamiltonian operator, of Dirac equation [8] for a free particle is,
The has four-components of fields, with vectors of momentum, and gamma matrices, α, β represent Pauli matrices and unitarity. The concept is akin to, e+ e– ⟶ 2γ, where the electron annihilates with its antimatter to produce two gamma rays. Antimatter existence is readily observed in both Stern-Gerlach experiment and positron from cosmic rays. The relationship between matter and antimatter at the subatomic level is described by charge conjugation (C), parity inversion (P) and time reversal (T) symmetry. Charge conjugation reverses the charge without changing the direction of the spin vector or momentum of the particle. Only time reversal accounts for changes in the spin direction. Parity is discreet space-time symmetry offered by spatial coordinates and these are invariant under inversion when the charge is reversed. Based on QFT, numerous literatures explore these parameters in order to explain the anomaly of the dominance of matter over antimatter and space-time quantization. The electron dominance over its conjugate pair is explained by 360° rotation, where a positron is generated. Another 360° rotation for a total of 720° rotation and the electron is restored to its original state. The process offers the helical property of the electron as a fermion of fractional spin and is described by so-called Dirac belt trick [9] in an attempt to capture the CPT symmetry. Other related descriptions include Balinese cup trick [10] or Dirac scissors problem [11]. The notion of space-time at singularity, where the electron translates to positron and vice versa from a 4D perspective is not well defined. Quantum gravity at singularity is analogous to discrete space-time on geometry basis, where CPT symmetry becomes prominent for particles assuming its own antimatter like the electron. Conversely, by mass-energy equivalence, quantum gravity is defined to be the quantized state of gravitational field and it requires the existence of graviton, a spin 2 particle. The particle is yet to be positively identified in both high energy physics experiments and gravitational waves emanating from astronomical sources.
In this study, how Dirac fermion is interpreted within a MP model of hydrogen atom type into 4D space-time on a geometry basis is examined. Its interpretation is somewhat equivalent to Klein bottle topology of 2D manifold visualized in 4D [12], where the electron spin vector varies within the manifold without a reference point-boundary akin to a sphere. The 2D manifold of the proposed MP model is assigned to Bohr orbit (BO) of topological torus linked to a pair of light-cones and this levitates between two hemispheres of an elliptical MP field mimicking Dirac string of a little magnet. The BO dissects the MP field linearly and its emergence is promoted by the electron in orbit tangential to it with half spin assigned to a light-cone of a hemisphere. The electron of a charged particle assumes time reversal orbit and this is imposed on clockwise precession of the MP field that rotates and twists the BOs of solenoidal type into n-dimensions by levitation towards maximum twists at the vertices to allow for electron-positron transition by Dirac belt trick. In this way, a spherical model is generated but unlike Klein bottle topology, the electron as a physical entity provides the reference point-boundary tangential to the sphere. Such a model is dynamic and how it becomes relevant to both QM and QFT are described and this has the potential to open up new research paths by conventional way in both experiments and theoretical applications.
2. Dirac Fermion within the Confinement of a MP Model
Additional details on the conceptualization path of the model from electron wave-diffraction is offered elsewhere [13]. In this section, the transformation of an electron of hydrogen atom type to a fermion by Dirac process within a spherical MP model of 4D space-time is presented (Figure 1a–d). First, both Dirac belt trick and CPT symmetry are demonstrated for the electron-positron transition process. Second, the model is consolidated by examining its relevance to the following; quantum mechanics and Dirac notations; visualization of 2D manifolds into 4D space-time; and center of mass reference frame with its dynamics before plotting some of the implications to both QM and QFT for future pursuits.
2a. Unveiling Both Dirac Belt Trick and CPT Symmetry
The electron orbit of time reversal in discrete continuum form of sinusoidal wave is defined by Planck radiation, h. In forward time, the orbit is transformed into an elliptical shape of a MP pair field somewhat mimicking Dirac string (Figure 1a). With clockwise precession, the torque or right- handedness exerted on the MP field shifts the electron of spin up from positions 0 to 4 to assume 360° rotation. Time reversal orbit against clockwise precession allows for maximum twist at the point-boundary or vertex of the MP field at position 4. The electron then flips to spin down mimicking a positron to begin the unfolding process and emits radiation by, . The positron is short-lived from possible repulsion of the proton and by another 360° rotation from positions 5 to 8, the electron is restored to its original state. The electron-positron transition is attained within a hemisphere of the MP field that is interchangeable with the other hemisphere and this sustains CPT symmetry. These intuitions are analogous to Dirac belt trick at 720° rotation with positions 0 to 3 and its transposition at positions 4 to 8 assigned to Dirac four-component spinor, at spherical lightspeed. The conjugate pairs of positions, 1, 3 and 5,7 cancels out the charges to form a topological torus of BO. These levitates into n-dimensions of energy levels by disturbance and are linked to the pair of light-cones of Minkowski space-time (Figure 1b). Clockwise precession by spherical lightspeed is balanced out by the electron’s time reversal orbit in accordance with Newton’s first law to generate an inertia reference frame, λ (Figure 1a) under the conditions,
where the trace function, Tr is the sum of all elements within the model.
2b. Quantum Mechanics and Dirac Notations
The transformation of the electron to a Dirac fermion can accommodate some basic concepts in quantum mechanics and QFT (Figure 1c,d). These are outlined below in bullet points based on ref. [14].
- The electron of wave-particle duality obeys de Broglie relationship, with h assigned to its sinusoidal orbit and mv to BO. It is defined by the wave function, and its orbit of time reversal adheres to the Schrödinger equation, and is fundamental aspect of the MP model (Figure 1c) in accordance with Equation (1) (see also Appendix A for further explanations). Electron spin-charge states of superposition from electron-positron transition is linked to BO defined by with its inner product, . Conjugate charges at positions, 1, 3 and 5 and 7 cancels each other out at spherical lightspeed to form close loops of BO at an energy n-level, where the electron is stabilized by levitation between the hemispheres of the MP field to generate only either spin up or spin down in accordance with Pauli exclusion principle. At 360° rotation, an electron of spin up is produced and at 720° rotation, a positron of spin down is formed. The loops of BOs mimic topological torus (Figure 1d) and by disturbance these become differential manifolds into n-levels or n-dimensions with levitation assumed between the hemispheres of the MP field. In this manner, the electron forms a weak isospin of spin up and spin down into n-dimensions, whereas the z-axis resembles nuclear isospin.
- Both radial and angular wave functions are applicable to the electron, . The radial part, is attributed to the principal quantum number, n and angular momentum, l of a light-cone with respect to r (Figure 1c). The angular part, in degenerate states, with respect to the z-axis is assigned to the BO defined by both and (Figure 1d).
- The BO is defined by a constant structure, ɑ and its orthogonal (perpendicular) to z-axis by linearization (Figure 1d). Its link to electron-positron pair is, for continuous derivation of MP field precession and is relevant to Fourier transform (see also Appendix B). Linear translation for the n-levels along z-axis can relate to the sum of expansion coefficients, , where the electron’s position offers an expectant value, with its probability as, .
- The shift in the electron’s position of hermitian conjugates by Dirac process, P(0→8) = assumes Hamiltonian space with by precession. The complete spherical rotation towards the point-boundary for the polarization states, 0, 1 assumes U(1) symmetry and incorporates Euler’s formula, + 1 = 0 in real space (Figure 1c).
- Singularity at Planck’s length is assigned to the point-boundary at position 0 and this promotes radiation of the type, from the electron-positron transition. Somehow it sustains the principal axis of the MP field as z-axis or nuclear isospin in asymmetry and promotes ±h at spherical lightspeed. The point towards the center is linked to a light-cone that levitates between two hemispheres similar to Weyl spinor (e.g., Figure 1b). Its accessibility is restricted both by levitation and electron orbit at positions 2 and 6.
2c. Visualization of 2D Manifolds into 4D Space-Time
Compact Lie group emerges from BO of topological torus in degeneracy and its levitation into n-dimensions is solenoid, between two hemispheres of the MP field of E to form differential smooth manifolds (Figure 1d). Toward the vertices of the MP field at positions 0 and 4 (Figure 1c), the solenoid is reduced to singularity, and the electron is transformed to a monopole. The monopole is not transferrable by linearization for the MP field of Dirac string resembling a magnet. The electron in orbit and its transition to positron polarizes the MP field to generate an electric dipole moment of quantized electric charge, into n-dimensions, whereas B quantized at the vertices.
Rotation matrices of the type, and for the spherical model is attributed to the MP field by precession with any shift in z-axis mimicking nuclear isospin being trivial, (Figure 1d). The rotation matrix, is assigned to BO into n-dimensions. The matrices are relevant to describe both integer and half-integer spins such as, 0, 1/2 and 1 towards complete rotation at position 0 of spherical point-boundary. Some of these features are explored in here based on refs. [15,16], while further demonstration is offered in Figure 2A of Appendix B.
The chirality of the electron is described by,
where g is electron’s position as subset of the space tangential to the manifolds and G represents the Lie group. For the conjugate positions pairs, 1, 3 and 5, 7 of BO (Figure 1d), Equation (5) validates the operations,
and
where is spin matrix for both spin ±1/2 (Figure 2A). The form, due to electron-positron (±) transition and radiation loss, tangential to the manifolds. For linear transformation along z-axis in 1D space, the BOs are isomorphic with respect to the particle position, (Figure 1d and Figure 2A). By intermittent precession, the inner product of r is a scalar and relates to the boundary of the MP field in the form,
where rotation of both vectors preserve the lengths and relative angles (e.g., Figure 1c). By assigning rotation matrix, R to Equation (7), its transposition is,
where the identity matrix, by reduction (Figure 2A). The orthogonal relationship of BO to clockwise precession along z-axis at 90° for all rotations suggests, . The SO(3) group rotation for integer spin 1 in 3D space is,
Equation (9) can also be pursued for integer spin 0 and higher spin particles. When rotating as 2 x 2 Pauli vector for SU(2) symmetry with respect to a light-cone of half-integer spin (Figure 1d), Equation (9) translates to the form,
where and are Pauli spinors of rank 1 to rank 1/2 tensor relevant for Dirac matrices (Figure 2A). By orthogonal geometry, the column is attributed θ at n-levels along z-axis and the row to BO defined by ϕ in degeneracy. For ladder operators at n-dimension along z-axis, SU(2) is irreducible for the shift in θ and ϕ such as, . Translation of SU(2) by accentuating precession at high energy like, matrices is reduced to the upright MP field position (e.g., Figure 1b). For the particle’s position, when y = 0, z = x is a real number. At x = 0, becomes an imaginary number. The BO linked to the electron’s position can be assigned to SO(2) group in 2D such as,
where, incorporates Dirac process at 720° rotation (e.g., Figure 1a). Similar relationships can be forged for with respect to the BO along x-y plane with respect to Equation (9) in the form,
Substitution of Equation (12) with can relate to polarization states, -1, 1 and 0 at the vertices of the MP field (Figure 1c) from the electron-positron transition such as,
The matrices described by Equation (13) is relevant to Figure 2A. These explanations demonstrate how manifolds of BOs are relevant to compact Lie group and how these levitate within the pair of interchangeable hemispheres of the spherical MP model of 4D space-time.
2d. Center of Mass Reference Frame and Its Dynamics
The center of mass (COM) is assigned to position 0 at the point-boundary or vertex of the elliptical MP field and is attributed to electron-positron transition. Some of its dynamics are described here. First, the COM is analogous to zero-point energy (ZPE) of the hydrogen atom of ultraviolet range of a harmonic oscillator. The oscillator can translate to a hemisphere of the MP field of Dirac string of time invariant and this can be stretched out towards COM of ZPE with its baseline assumed at positions 2 and 6 of superposition states from the electron-positron transition. Second, the electron’s position in orbit is quantized into BOs of n-dimensions and this levitates by stretching into Minkowski space-time (Figure 1b). In this case, the COM is compared to instanton magnetic monopoles by precession of the MP field into Euclidean space-time. This is generated from reduction of the topology of solenoid of n-dimensions towards the spherical boundary (Figure 1d). Third, the COM of ZPE generates instantons from electron-positron transition as a consequence of twisting and unfolding by Dirac process. This is accessible to quantum tunneling with precession of the MP field at spherical lightspeed. Fourth, envelop solitons are applicable to positions 2 and 6 of the electron orbit at the baseline of the pair of hemispheres of the MP field. Stochastic behavior at these positions by electron-positron transition forms a chaotic atomic system of the hydrogen atom. Fifth, these positions offer fundamental graininess to classical limit of quantum mechanics by correspondence principle. The inertia frame, λ (Equation (4)) is transformed to relativistic Campton’s atomic wavelength, for the MP model of hydrogen atom. Dissection of λ into n-dimensions of BOs as n-energy levels is analogous to both the line spectrum and vibrational spectrum of ionized hydrogen molecule. The envelop solitons then form rotational energy levels to the n-levels. Sixth, signals from the solitons of chaotic system either from electron-positron transition or its particle-hole symmetry by ejection of the electron is expected to conceal the point of singularity of the pair of light-cones (Figure 1b). Based on the correspondence principle at high energy, infinite n-dimensions are set to one dimension, i.e., linearly with natural units, . By conservation, coupling of ionized MP models with ejection of the electron is expected to induce envelop solitons at positions 2 and 6. This can restrict direct observations of quarks and generate the property asymptotic freedom. Conversely, where the chaotic system converges with the quantum critical region provided by the light-cones towards the baseline of the hemisphere (e.g., Figure 1a), this becomes important to the pursuit of quantum critical point such as in condensed matter physics for an array of atoms in one-dimensional line. Seventh, qubits 0, 1 and hypercharge –1 are assumed respectively at positions 0, 8 and 4 (Figure 1a,c). These are relevant to classical computing, whereas for quantum computing, the accessibility to the nucleons mimicking the MP model is restricted by the envelop solitons of the chaotic system at positions 2 and 6. Eight, COM is relevant to Coulomb’s law of electric force, between two charged bodies if these equate to electron-positron pair in a vacuum. The radius, r is assigned to the principal axis of the MP field (Figure 1a) and it links COM with the nucleus akin to nuclear isospin. The inertia frame of the model by precession at a constant velocity accommodates centripetal force. Finally, the electron of subatomic particle in orbit upholds the uncertainty principle of undefined position and momentum, whereas its relativistic analog of energy and time is applicable to linear light paths tangential to the spherical MP model. Similarly, Campton’s wavelength defines the atomic MP model at spherical lightspeed.
3. Some Basic Components of both QM and QFT
Further exposition of the helical property of the MP model to induce Dirac belt-trick is offered in Figure 2a–f. The CPT symmetry is sustained for the electron-positron transition at the point- boundary, where COM is assumed. The electron’s time reversal orbit of a MP field mimics Dirac string, and it is subjected to both twisting and unfolding process by clockwise precession. Cancellation of charges at conjugate positions 1, 3 and 5, 7 allows for the emergence of BO of topological torus (Figure 1d) and this accommodates either spin up or spin down states by levitation of the pair of light-cones between interchangeable hemispheres of the MP field into Minkowski space-time (Figure 1b). How all these become compatible with some basic aspects of both QM and QFT are succinctly plotted in bullet points based on refs. [17,18,19]. This is done to pave the path for further researches into more depth the conventional way by applying the model as an intuitive guide.
- ⇒
- Dirac theory and helical property. The fermion field is defined by the famous Dirac equation of the generic form,where are gamma matrices. The exponentials of the matrices, are attributed to the electron’s position by clockwise precession acting on its time reversal orbit. For example, is assigned to the vertex of the MP field and by electron-positron transition at position 0, it sustains z-axis as arrow of time in asymmetry. Thus, arrow of time for a pair of vertices for spin up and spin down incorporates time reversal symmetry. The variables of Dirac matrices are assumed by the electron shift in its positions (Figure 2a–f). Orthogonal projections of the space-time variables, are confined to a hemisphere and assigned to a light-cone to generate spin-charge of the electron (e.g., Figure 1c). These descriptions uphold CPT symmetry and are indirectly incorporated into the famous Dirac equation,where c acts on the coefficients A, B and C and transforms them to and . The exponentials of are denoted i for off-diagonal Pauli matrices for the light-cone (Figure 1d) and is defined by,and zero exponential, is,The qubits 0 and 1 are assigned to the COM at positions 0 and 8, and hypercharge –1 to position 4 (e.g., Figure 1a). Such a notion can become relevant to classical computing, whereas quantum computing for nucleons mimicking the MP model is subjected to quantum chaos of butterfly effect assigned to envelop solitons at either position 2 or 6 encasing quantum critical point of the light-cone (see also subsection 2d). is assigned to oscillations from on-shell momentum of the BOs into n-dimensions (Figure 1d) for anticommutation relationship, of chiral symmetry (Figure 2c,d). The associated vector gauge invariance for the electron-positron transition exhibits the following relationships,andThe exponential factor, iθ refers to the position, i of the electron of a complex number and θ, is its angular momentum (e.g., Figure 1c). The unitary rotations of right-handedness (R) or positive helicity and left-handedness (L) or negative helicity are applicable to the electron transformation to Dirac fermion (e.g., Figure 2A in Appendix B). The process is confined to a hemisphere and this equates to spin-1/2 property of a complex spinor. Two successive rotations of the electron in orbit by clockwise precession of the MP field is identified by . The chirality or vector axial current at the point-boundary is assigned to polarized states, ±1 of the model (Figure 1c). The helical symmetry from projections operators or nuclear isospin of z-axis acting on the spinors (Figure 2e) is,andwhere is likened to thermal radiation of a black body. The usual properties of projection operators are: L + R = 1; RL = LR = 0; L2 = L and R2 = R (e.g., Figure 2a–d) consistent with CPT symmetry.
- ⇒
- Wave function collapse. Dirac fermion or spinor is denoted ψ(x) in 3D Euclidean space and it is superimposed onto the MP model of 4D space-time, ψ(x,t) by clockwise precession (Figure 3a). The latter resembles Minkowski space-time and consists of a light- cone dissected by z-axis as arrow of time into asymmetry (Figure 1b). The former includes both positive and negative curvatures of non-Euclidean space (e.g., Figure 2a,b) normalized to straight paths of Euclidean space (Figure 2c,d). These are of non-abelian Lie group (see subsection 2c) imposed on the surface of the spherical MP model somewhat mimicking Poincaré sphere. The Dirac four-component spinor, is attributed to positions 0 to 3 of conjugate pairs in 3D space. Convergence of positions 1 and 3 at either position 0 or 2 is relevant to the equivalence principle based on general relativity for Euclidean geometry (Figure 3a). The quantum aspect of de Sitter space by geodetic clockwise precession is balanced out by anti-de Sitter form of the electron transition in its orbit of time reversal due to gravity. For the irreducible spinor represented by the MP model, gravity is assigned to a gyroscopic geometry. Any light paths tangential to BOs of n-energy levels interacting with the electron is expected to mimic Fourier transform along z-axis of the MP field as time axis in asymmetry and this is equivalent to wave function collapse (Figure 3b). Constraining the electron’s position offers the uncertainty principle with on-shell momentum of BO susceptible to levitation between the two hemispheres of the MP field. The generated wave amplitudes of BOs can relate to a typical hydrogen emission spectrum (Figure 3c), where wave function collapse of probabilistic distribution by Born’s rule,, is relatable to the hemispheres.
- ⇒
- Quantized Hamiltonian. Two ansatzes adapted from Equation (14) are given by,andwhere outward projection of electron spin at positions 5, 7 is represented by and inward projection at positions 1, 3 by (e.g., Figure 2c,d). By linear transformation, the hermitian plane wave solutions form the basis for Fourier components in 3D space (Figure 1d and Figure 3b). Decomposition of quantized Hamiltonian ensues as,where the constant, is attributed to the dissection of BOs along z-axis. Its conjugate form is by,The coefficients and are ladder operators for u-type spinor and and for v-type spinor at n-dimensions of BOs mimicking topological torus with levitation assumed between the hemispheres of the MP model (e.g., Figure 1d). These are related to Dirac spinors of two spin states, ±1/2 with and as their antiparticles. Dirac Hamiltonian of one-particle quantum mechanics relevant to the MP model of hydrogen atom type is,The quantity in the bracket is provided in Equation (3). By parity transformation, the observable and holographic oscillators are canonically conjugates (e.g., Figure 2c,d). The associated momentum is,With z-axis of the MP field assuming time axis in asymmetry (Figure 1c), V-A currents are projected in either x or y directions in 3D space comparable to Fourier transform (e.g., Figure 3b). These assume the relationships,and its matrix form,where α and β denote the spinor components of . Equations (23a) refers to unitarity of the model and Equation (23b) is assumed by the electron-positron transition about the manifolds of BOs in 3D space (Figure 1d). The independent of time in 3D space obeys the uncertainty principle with respect to the electron’s position, p and momentum, q, as conjugate operators (Figure 1c). The commutation relationship of p and q is,Equation (24) incorporates both matter and antimatter and their translation to linear time (Figure 3b). The electron as a physical entity generates a positive-frequency such as,Equation (25) could explain the dominance of matter (electron) over antimatter based on the conceptualization process of Dirac fermion (e.g., Figure 2a,b).
- ⇒
-
Non-relativistic wave function. Observation by light-matter interaction allows for the emergence of the model from the point-boundary at Planck length. Subsequent energy shells of BOs at the n-levels by excitation accommodates complex fermions, ±1/2, ±3/2, ±5/2 and so forth (Figure 4a). The orbitals of 3D are defined by total angular momentum, and this incorporates both orbital angular momentum, l and spin angular momentum, s (Figure 4b). These are aligned with Schrödinger wave function (e.g., Figure 1c).The reader is also referred to . Within a hemisphere, the model is transformed to a classical oscillator. By clockwise precession, a holographic oscillator from the other hemisphere of the MP field remains hidden. One oscillator levitates about the other (Figure 4b) and both are not simultaneously accessible to observation by Fourier transform (e.g., Figure 3b). Levitation of on-shell momentum of BO into n-levels between the hemispheres of the MP model can be pursued for Fermi-Dirac statistics if these equate to fermion type particles. The point-boundary at the vertex of MP field at position 0 of COM is assigned to ZPE and both vertices constrain vacuum energy with its perturbation by precession. The splitting (Figure 4a) applies to Landé interval rule for the electron of weak isospin and this can somehow relate to lamb shift based on the subshells of BOs into n-dimensions by levitation between the hemispheres of the spherical model (e.g., Figure 1d). Such a scenario is similar to how vibrational spectra of a harmonic oscillator for diatoms like hydrogen molecule incorporates rotational energy levels (Figure 4a). The difference of the classical oscillator to the quantum scale is the application of Schrödinger wave equation (e.g., Figure 1c).
- ⇒
- Weyl spinor. The light-cone within a hemisphere accommodates both matter and antimatter by parity transformation (Figure 4a,b). It is described in the form,Equation (26) corresponds to spin up fermion, a spin down fermion, a spin up antifermion and a spin down antifermion (e.g., Figure 2c,d). By forming its own antimatter, Dirac fermion somewhat resembles Majorana fermions. It is difficult to observe them simultaneously due to wave function collapse restricted to light-particle interaction into linear time (e.g., Figure 3b). Non-relativistic Weyl spinor of a pair of light-cones in 4D by levitation between the hemispheres of the precessing MP field of clockwise direction is relevant to the fundamental Schrödinger wave equation (Figure 1c and Figure 1A in Appendix A). These are defined by reduction of Equation (26) to a bispinor in the form,where are Weyl spinors of chirality with respect to the electron position in orbit. By parity operation, x → x’ = (t, ‒ x), qubits 1 and −1 are generated at the vertices of the MP field (e.g., Figure 1c). Depending on the reference point-boundary of the BO (Figure 1d), the exchanges of left- and right-handed Weyl spinor assumed the process,Conversion of Weyl spinors to Dirac bispinor, are of transposition state (e.g., Figure 2c,d)). The two-component spinor, = 1 are normalized at the point-boundary at position 0 of the spherical model (Figure 1a).
- ⇒
- Lorentz transformation. The Hermitian pair, of Dirac fermion based on Equation (27) undergo Lorentz boost and translate the BOs into n-levels (Figure 1d) of the form,The corresponding Lorentz scalar applicable to scattering from on-shell momentum tangential to the BOs (Figure 1d) is,Equation (30) is referenced to z-axis of the MP field as time axis and is relevant to Fourier transform into linear time. By identical calculation to Equation (29), the Weyl spinor is,Both Weyl spinor of a light-cone (Figure 4a) and Majorana fermion are indistinguishable from Dirac spinor for light-matter interaction confined to position 0 (e.g., Figure 1a).
- ⇒
- Electroweak symmetry breaking mechanism. If the electron as a physical entity is ejected by ionization, a particle-hole is generated at the positions 0 to 8 by assuming conservation of the MP model (Figure 1a). The electron or its particle-hole is of chiral symmetry for the model, where CPT symmetry is described by charge conjugation from electron-positron pairing, parity inversion for electron-positron transition within interchangeable pair of hemispheres (Figure 2c,d), and time reversal to electron orbit of the elliptical MP field. When the MP field is subjected to clockwise precession at spherical lightspeed, the electron is transformed to Dirac fermion by Dirac belt trick (Figure 1a). At high energy, the infinite n-dimensions of conjugate positions, 1, 3 and 5, 7 for BO of topological torus (Figure 1d) and their levitation between two hemispheres of the MP field (Figure 4a,b) is reduced linearly along positions 2 and 6. This coincides with the correspondence principle for non-dimensional system, with the natural units set, . The emergence of COM at the vertices of the MP field from the separation of the pair of hemispheres somehow can relate Higgs-like boson, . The COM is incorporated in the first term of the Higgs field scalar quantity, . The second term is applicable to positions 1, 3 or 5, 7 and both are relevant to the emergence of bosons for the measureable quantity, about horizontal x-axis of the Mexican hat potential. The y-axis consists of imaginary potential, and the emergence of the z-axis of the MP field as time axis in asymmetry for neutral current can be assigned to boson. These descriptions are compatible to Feynman diagrams for the coupling of ionized MP models mimicking proton-proton collisions (Figure 5a,b). The neutrino types (e.g., ) of helical property would mimic the electron-positron transition of flipped hypercharge by Dirac belt trick and are attributed to trivial shift in z-axis, by precession (Figure 1d) towards positions 1 and 3 linked to bosons. From on-shell momentum (), particles acquire energy, or such as for massive photons by Einstein mass-energy equivalence of the form,Based on Equation (32), light and particle-hole coupling from the emergence of the MP field amplitudes from non-dimensional linear setting at at high energy is expected to translate positions 1 and 3 to mimic bosons. These are massive and about 80 times the mass of proton. The particle-hole shift to positions 2 and 6 forms envelope solitons and restricts observation of quarks at the center by allowing the development of asymptotic freedom. Levitation of BOs into n-dimensions is linked to the pair of light-cones between the two hemispheres of the MP field. These can translate to leptons such as tau, muon and their antimatter. Tangential light paths along z-axis can relate to boson of neutral current and COM at position 0 to . In this way, beta decays of to and or similarly, to and can be accounted by MP models coupling. How bosons are linked to the conversion of neutron to proton such as for beta decay is not covered here but it is posited here that the quark model could resemble the MP model, and both are linked by the z-axis as arrow of time in asymmetry akin to nuclear isospin. These explanations appear consistent to lack of supersymmetry observed in high energy experiments and the dilemma associated with baryon asymmetry. Such a prospect is explored further in a subsequent study by the author.
4. Conclusion
The electron and its transformation to Dirac fermion of a complex four-component spinor is pursued within many facets of both QM and QFT based on experimental outcomes from light-matter interactions. However, without the development of a proper theory of quantum gravity, how both QM and QFT are combined at the point of singularity remains an abstract concept. Here, in this study, the integration of both QM and QFT at an atomic level is considered from a geometry perspective based on a proposed MP model of hydrogen type of 4D space-time. The electron transformation to Dirac fermion is shown to be consistent with Dirac belt trick while sustaining superposition of spin-charge and wave-particle duality. Such a model appears dynamic and it is relatable to some basic features of both QM and QFT like Dirac theory, wave function collapse, quantized Hamiltonian, non-relativistic wave function, Weyl spinor, Lorentz transformation and electroweak symmetry breaking mechanism. These are succinctly plotted to be explored further into more depth the conventional way in both experiments and theoretical applications.
Data Availability Statement
The modeling data attempted for the current study are available from the corresponding author upon reasonable request.
Conflicts of Interest
The author declares no competing financial interests.
Appendix A. Mathematical Property of Schrödinger Wave Equation
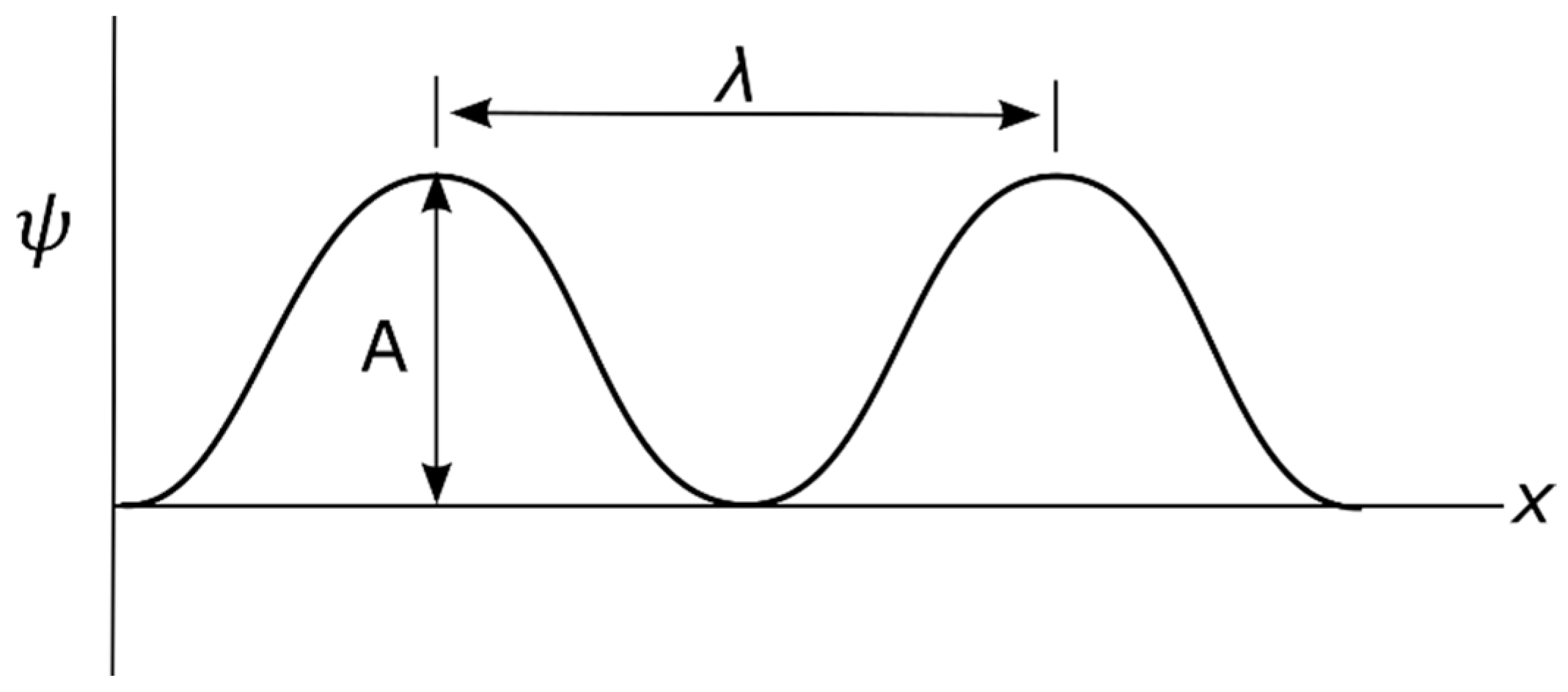
The Schrödinger equation cannot be derived similar to Newton’s law of gravity. Its mathematical property can be plotted [21] beginning with the normalized sine wave function,
where A is the amplitude shown in Figure 1A. Its second derivation is,
Velocity, can be calculated from total energy in the form,
where V is potential energy and kinetic energy is, . Taking the squared function of de Broglie relationship becomes,
By substitution from Equation (3A), Equation (4A(i)) can be rewritten as,
By substitution into Equation (2A), this becomes,
An alternative form of Equation (5A(i)) is,
By first derivation of space-time due to clockwise precession of the MP model (Figure 1c), Equation (5A(ii)) is given by,
In this way, the model offers physical meaning to Schrödinger wave equation as an eigenvalue of similar to Newton’s law of gravity with the electron of isospin represented by, i or 2m.
Figure 1A.
Translation of a sine wave function along x-axis.
Appendix B. Basis of Vectors, Matrices, Tensors and Fourier Transform
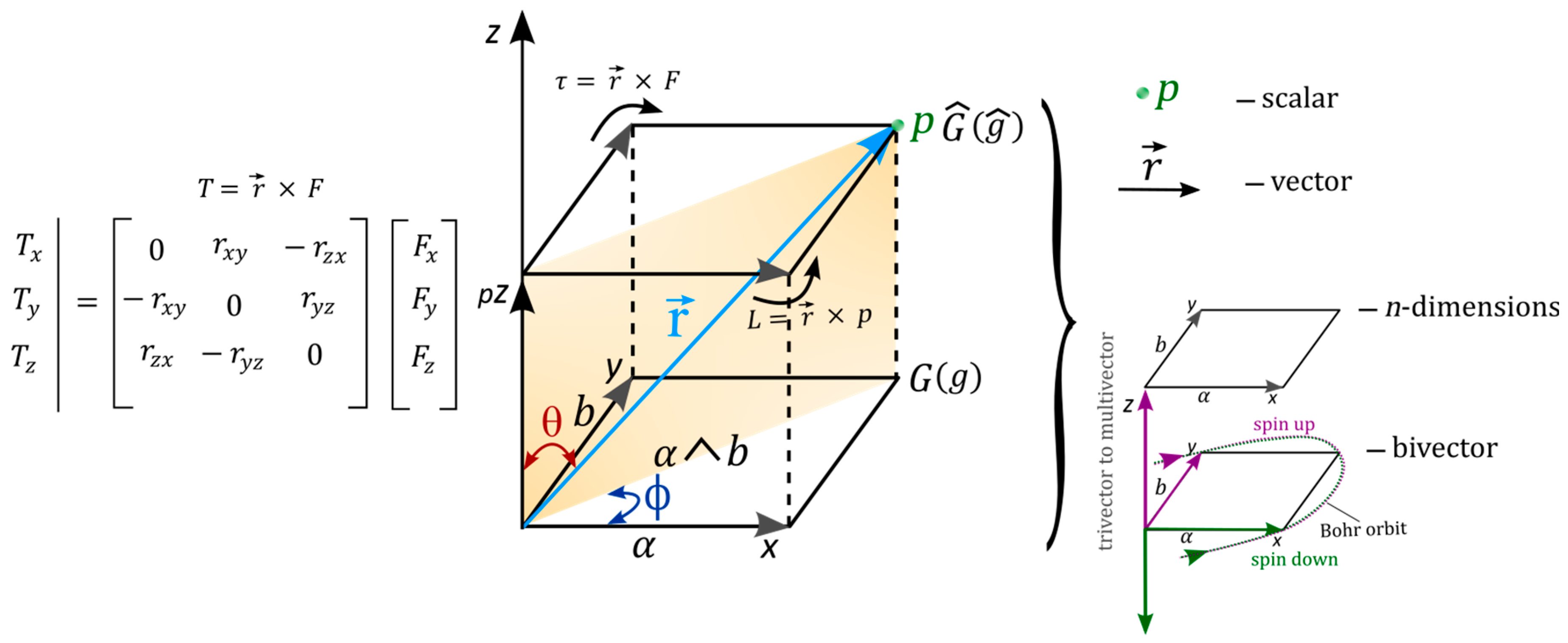
The physicality of the complexity of Dirac four-component spinor can somehow relate to the geometric MP model. The BOs provide the basis for vector to multivector by levitation into n-dimensions or n-energy levels between a pair of hemispheres of the MP field along the z-axis (Figure 2A). By rotation, the BOs generate both spin up and spin down in correspondence to the principal axis of the MP field as time axis in asymmetry. The manifold of BOs can be assigned to a box (Figure 2A). The rotation by is a four 3-fold axis in 3D and its matrices are defined by Cartesian coordinates, x, y and z. These are related to shift in both and as double cover of SU(2) for vector to multivectors assumed within the BOs. They are applicable to the Lie group ladder operators, and of 3D space and 4D space-time. By linearization akin to Fourier transform (e.g., Figure 3b), the boxes of isomorphism consisting of BOs into n-dimensions by levitation between two hemispheres of the MP field and these are translated along the z-axis as time axis in asymmetry (Figure 2A). The BO of bivector field is related to of integers modulo p of prime. The shift along z-axis of isospin from vertices of the MP field of Dirac string is commutative (Figure 2A) and any changes by precession is trivial. The MP model of irreducible representation, is given by [18],
where is composed of algebraic structure (, and ) for vectorization, V into p-dimensional space defined by (Figure 2A). The two operators, and acting on V are
and
where T is identified by the rotational matrices, (Figure 2A) and is the shift in frequency in space along z-axis (e.g., Figure 3b). These intuitive demonstrations somehow provide a geometry perspective to the MP model with its relevance to the Lie group and QFT in terms of Dirac field theory elaborated in the article (see subsection 2c and section 3).
Figure 2A.
The basis of vectors to multivectors, matrices, tensors and Fourier transform. The vectors are aligned with either spin up or spin down by rotation of the BO. Translation along z-axis of nuclear isospin provides trivectors to multivector of BOs into n-dimensions by levitation between two hemispheres of the MP field. Clockwise precession of the MP field becomes unidirectional at spherical lightspeed. The matrices are related to tensors along z-axis by Fourier transform for the commutation, to (see text for details). The is a four 3-fold rotational axis (shaded orange plane) in 3D of the box and is applicable to Fourier transform (e.g., Figure 3b). It mimics a 4-gradient Dirac operator, for vectors to multivector into n-dimensions of the box (see also red rectangle in Figure 1c). Rotation into forward time, is by clockwise precession and this insinuates spin up with the unfolding process for spin down by, . The spin rotation matrices applicable to Clifford algebra are shown to the left. The half-integer spins of SU(2) group provide double cover (bivector) with shift in both and for BOs of topological torus (Figure 1d) into n-dimensions by levitation between two interchangeable hemispheres of the MP field. This is relevant to the Lie group ladder operators, and . Some key features of the dimensional box are expounded to the right.
Figure 2A.
The basis of vectors to multivectors, matrices, tensors and Fourier transform. The vectors are aligned with either spin up or spin down by rotation of the BO. Translation along z-axis of nuclear isospin provides trivectors to multivector of BOs into n-dimensions by levitation between two hemispheres of the MP field. Clockwise precession of the MP field becomes unidirectional at spherical lightspeed. The matrices are related to tensors along z-axis by Fourier transform for the commutation, to (see text for details). The is a four 3-fold rotational axis (shaded orange plane) in 3D of the box and is applicable to Fourier transform (e.g., Figure 3b). It mimics a 4-gradient Dirac operator, for vectors to multivector into n-dimensions of the box (see also red rectangle in Figure 1c). Rotation into forward time, is by clockwise precession and this insinuates spin up with the unfolding process for spin down by, . The spin rotation matrices applicable to Clifford algebra are shown to the left. The half-integer spins of SU(2) group provide double cover (bivector) with shift in both and for BOs of topological torus (Figure 1d) into n-dimensions by levitation between two interchangeable hemispheres of the MP field. This is relevant to the Lie group ladder operators, and . Some key features of the dimensional box are expounded to the right.
Appendix C. Vector Modelling of the MP Model
Schrödinger wave equation forms the basis of quantum mechanics and offers satisfactory explanations to account for probability distribution or orbitals of subatomic particles like electrons in the atom. However, quantum mechanics cannot account for the combination of orbital angular momenta, spin angular momenta and magnetic moments of valence electrons observed in atomic spectra. Russell-Saunders orbital-spin (L-S) coupling ensues by Clebsch-Gordon series such as for the eigenvalue of total angular momentum, , and this incorporates eigenvalues of both orbital angular momentum, l and spin angular momentum, s.
Figure 3A.
Vectors of orbital angular momentum. (a) Vectors L and S precess about this resultant J. In other words, any precession affecting L and S in the form, is reduced to the MP field of magnetic property such as Dirac string along z-axis. The shaded area of bivector from Figure 2A is extended to the point-boundary of the MP model (see also Figure 4a). (b) Vector J of the MP field precesses in correspondence to applied external magnetic field B. Both images are adapted from ref. [22].
Figure 3A.
Vectors of orbital angular momentum. (a) Vectors L and S precess about this resultant J. In other words, any precession affecting L and S in the form, is reduced to the MP field of magnetic property such as Dirac string along z-axis. The shaded area of bivector from Figure 2A is extended to the point-boundary of the MP model (see also Figure 4a). (b) Vector J of the MP field precesses in correspondence to applied external magnetic field B. Both images are adapted from ref. [22].
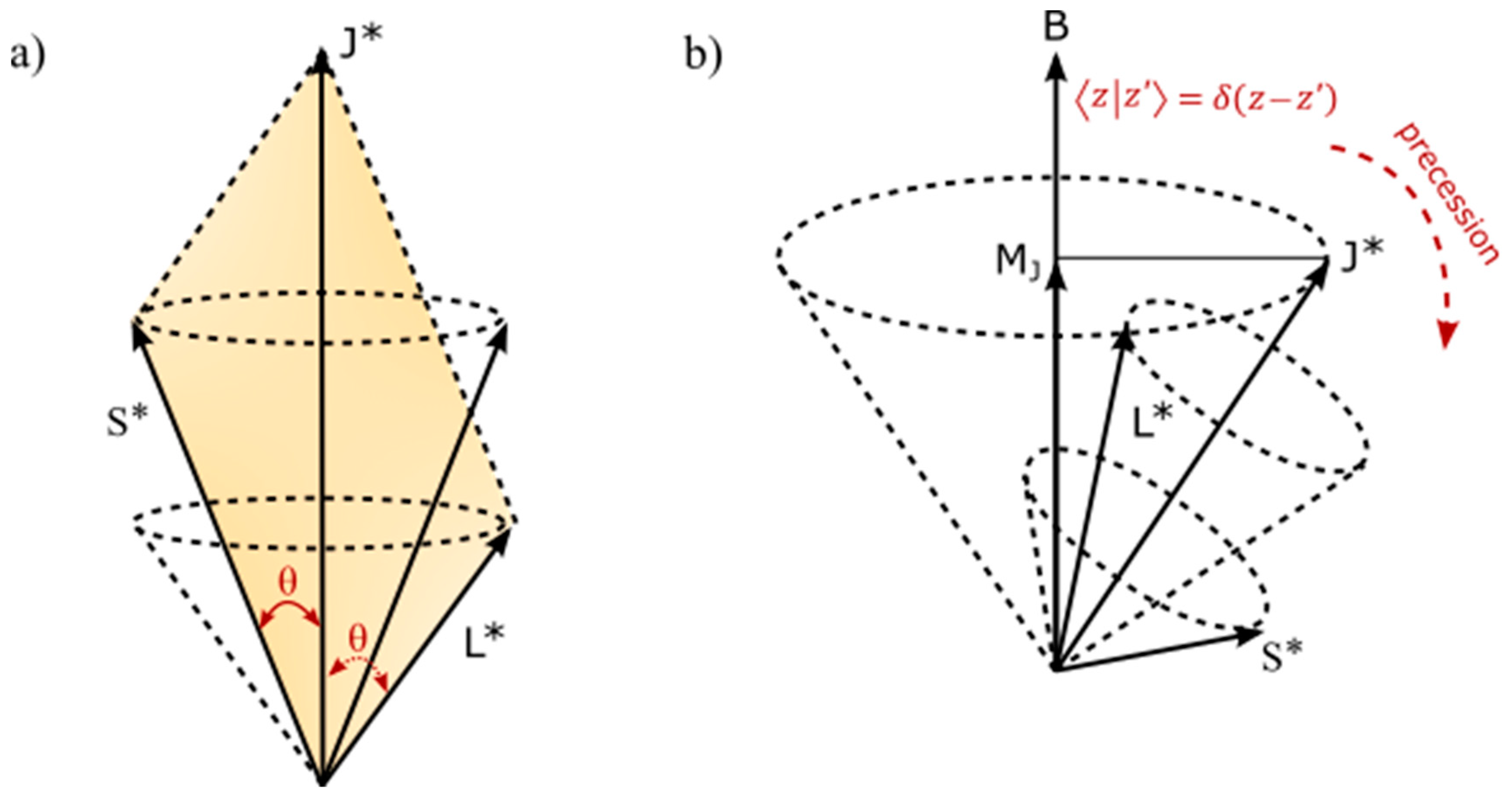
From the emergence of the oscillator at the point-boundary (Figure 4a), levitation of BOs into n-dimensions by levitation between two hemispheres of the MP field can cater for both the resultant orbital angular momentum, L and resultant spin angular momentum, S, where spin-orbit interaction is related to constant shift in the electron position. Complete rotation towards the point-boundary by Dirac process is twice, 2π and the positron-electron transition is related to h. The lone valence electron of the MP model akin to hydrogen atom by precession takes the form,
where i is equal to the subshells (Figure 4a). For example, n = 2 is split into s and p orbitals with each one accommodating spin 1/2 and from electron-positron transition of Dirac process at spherical lightspeed (Figure 1a), this equates to ±1/2 spin in accordance with Pauli exclusion principle. The total angular momentum, , is and at n = 2, l = 1. The former is assumed from the summation of spin, 1/2 + 1/2 + 1/2 from combined s and p subshells of + = , when both orbital and spin angular momenta are aligned in the same direction (Figure 4b). It also provides the magnitude of S. The latter of low energy from = in the form, 1/2 + 1/2 − 1/2 and is assigned to p orbital by cancelling out 1s orbital. This does not consider the orientation such as of the p subshells into n-dimensions by levitation between two hemispheres of the MP field (e.g., Figure 4a). The corresponding L from the combination of in a complete loop of BO at n-dimension is,
where the values of 0, and are generated at n = 0 to n = 2. Their projection with respect to z-axis for the irreducible MP field is,
Equation (8A(iii)) is applicable to the light-cone and the same is projected for such as,
where can take (2l + 1) values in both equations as eigenfunciton of . Both S and L combine to generate J in the form,
Distinction of L and S are provided in Figure 4b and the orientations by precession are applicable to . These explanations can possibly account for odd fermion spin types such as, noted for Zeeman effect (e.g., Figure 4a) and also lamb shift from the electron-positron transition in discreet form (e.g., Figure 3c). In this case, the electron is of weak isospin and z-axis is applicable to nuclear isospin. Both are linked to BOs of topological torus (Figure 1d) into n-dimensions of two hemispheres of the MP field by levitation and are subjected to twisting and unfolding process by Dirac belt trick (Figure 1a,b).
References
- Nelson, E. Derivation of the Schrödinger equation from Newtonian mechanics. Phys. Rev. 150(4), 1079 (1966). [CrossRef]
- Li, Z. Y. Elementary analysis of interferometers for wave—particle duality test and the prospect of going beyond the complementarity principle. Chin. Phys. B 23(11), 110309 (2014). [CrossRef]
- Rabinowitz, M. Examination of wave-particle duality via two-slit interference. Mod. Phys. Lett. B 9(13), 763-789 (1995). [CrossRef]
- Pawłowski, M. et al. Information causality as a physical principle. Nature 461(7267), 1101-1104 (2009). [CrossRef]
- Henson, J. Comparing causality principles. Stud. Hist. Philos M. P, 36(3), 519-543 (2005). [CrossRef]
- Rovelli, C. Space is blue and birds fly through it. Philos. Trans. Royal Soc. Proc. Math. Phys. Eng. 376(2123), 20170312 (2018). [CrossRef]
- Sun, H. Solutions of nonrelativistic Schrödinger equation from relativistic Klein–Gordon equation. Phys. Lett. A 374(2), 116-122 (2009). [CrossRef]
- Alvarez-Gaumé, L. & Vazquez-Mozo, M. A. Introductory lectures on quantum field theory. arXiv preprint hep-th/0510040 (2005).
- Weiss, L. S. et al. Controlled creation of a singular spinor vortex by circumventing the Dirac belt trick. Nat. Commun. 10(1), 1-8 (2019). [CrossRef]
- Silagadze, Z. K. Mirror objects in the solar system?. arXiv preprint astro-ph/0110161 (2001).
- Rieflin, E. Some mechanisms related to Dirac’s strings. Am. J. Phys. 47(4), 379-380 (1979).
- Oshima, S., Kanemaki, S. & Fujita, T. Problems of Real Scalar Klein-Gordon Field. arXiv preprint hep-th/0512156 (2005).
- Yuguru, S. P. Unconventional reconciliation path for quantum mechanics and general relativity. IET Quant. Comm. 3(2), 99–111 (2022). [CrossRef]
- Jaffe. R. L. Supplementary notes on Dirac notation, quantum states, etc. https://web.mit.edu/8.05/handouts/jaffe1.pdf (September, 2007).
- Eigen, C. Spinors for beginners. https://www.youtube.com/@eigenchris (November, 2012).
- Zhelobenko, D. P. Compact Lie groups and their representations. J. Amer. Math. Soc. 40, 26-49 (1973).
- Alvarez-Gaumé, L. & Vazquez-Mozo, M. A. Introductory lectures on quantum field theory. arXiv preprint hep-th/0510040 (2005).
- Burdman, G. Quantum field theory I_Lectures. http://fma.if.usp.br/~burdman (October, 2023).
- Peskin, M. E. & Schroeder, D. V. An introduction to quantum field theory. Addison-Wesley, Massachusetts, USA (1995). pp 13–25, 40–62. [CrossRef]
- https://en.wikipedia.org/wiki/Spinor (updated February 2024).
- Das, I. et al. An introduction to physical chemistry. New Age International (P) Limited, New Delhi, India (2005) 2nd Ed. pp 16-20.
- Singh, R. B. Introduction to modern physics. New Age International (P) Limited, New Delhi, India (2009) 2nd Ed. Vol. 1, pp 420-425.
Figure 1.
The MP model [13]. (a) In flat space, a spinning electron (green dot) in orbit of sinusoidal form (green curve) is of time reversal. It is normalized to an elliptical MP field (black area) of a magnetic field, B mimicking Dirac string. By clockwise precession (black arrows), an electric field, E of inertia frame, λ is generated. The shift in the electron’s position from positions 0 to 4 at 360° rotation against clockwise precession offers maximum twist. At position 4, the electron flips to a positron to begin the unfolding process from positions 5 to 8 for another 360° rotation to restore the electron to its original state analogous to Dirac belt trick. For the total of 720° rotation, a dipole moment (±) is generated for the spherical model with CPT symmetry sustained for the electron. (b) The BOs are defined by conjugate numbered pairs, 1,3 and 5,7, where cancellation of charges allows for the emergence of angular momentum (purple dotted lines) of spin ±1/2 (navy colored light-cones) with vectors aligned to the vertices of the MP field at positions 0 and 4. Levitation of BOs into n-dimensions is linked to a pair of light-cones that is exchangeable between the hemispheres of the MP field of Minkowski space-time. These are projected in degeneracy towards the center and by contraction to the vertices of the spherical model for Euclidean space-time. (c) The electron orbit is tangential to smooth manifold of BO intersecting the MP field and is relevant to both quantum mechanics and Lie group. The precession stages, , at spherical lightspeed is polarized by the electron-positron transition to generate qubits, 0 and ±1 at the vertices of the MP field. The polar coordinates (r, θ, Φ) are attributed to Schrödinger wave function with respect to the electron’s position in space. (d) Topological torus emerges from BO defined by (white loops) and its dissection perpendicularly by (yellow circles) from intermittent clockwise precession stages of the MP field. Any light-matter interactions are tangential to the electron’s position at the base point of BOs and it is solenoidal by levitation into n-dimensions between the two hemispheres. Linearization is assumed along the principal axis of the MP field as time axis in asymmetry. Singularity at the center for the light-cone is constrained by electron’s shift at positions, 2 and 6 along horizontal x-y plane of an irreducible spinor field (insert centered image). The embedded terms and equations are explained in the text.
Figure 1.
The MP model [13]. (a) In flat space, a spinning electron (green dot) in orbit of sinusoidal form (green curve) is of time reversal. It is normalized to an elliptical MP field (black area) of a magnetic field, B mimicking Dirac string. By clockwise precession (black arrows), an electric field, E of inertia frame, λ is generated. The shift in the electron’s position from positions 0 to 4 at 360° rotation against clockwise precession offers maximum twist. At position 4, the electron flips to a positron to begin the unfolding process from positions 5 to 8 for another 360° rotation to restore the electron to its original state analogous to Dirac belt trick. For the total of 720° rotation, a dipole moment (±) is generated for the spherical model with CPT symmetry sustained for the electron. (b) The BOs are defined by conjugate numbered pairs, 1,3 and 5,7, where cancellation of charges allows for the emergence of angular momentum (purple dotted lines) of spin ±1/2 (navy colored light-cones) with vectors aligned to the vertices of the MP field at positions 0 and 4. Levitation of BOs into n-dimensions is linked to a pair of light-cones that is exchangeable between the hemispheres of the MP field of Minkowski space-time. These are projected in degeneracy towards the center and by contraction to the vertices of the spherical model for Euclidean space-time. (c) The electron orbit is tangential to smooth manifold of BO intersecting the MP field and is relevant to both quantum mechanics and Lie group. The precession stages, , at spherical lightspeed is polarized by the electron-positron transition to generate qubits, 0 and ±1 at the vertices of the MP field. The polar coordinates (r, θ, Φ) are attributed to Schrödinger wave function with respect to the electron’s position in space. (d) Topological torus emerges from BO defined by (white loops) and its dissection perpendicularly by (yellow circles) from intermittent clockwise precession stages of the MP field. Any light-matter interactions are tangential to the electron’s position at the base point of BOs and it is solenoidal by levitation into n-dimensions between the two hemispheres. Linearization is assumed along the principal axis of the MP field as time axis in asymmetry. Singularity at the center for the light-cone is constrained by electron’s shift at positions, 2 and 6 along horizontal x-y plane of an irreducible spinor field (insert centered image). The embedded terms and equations are explained in the text.
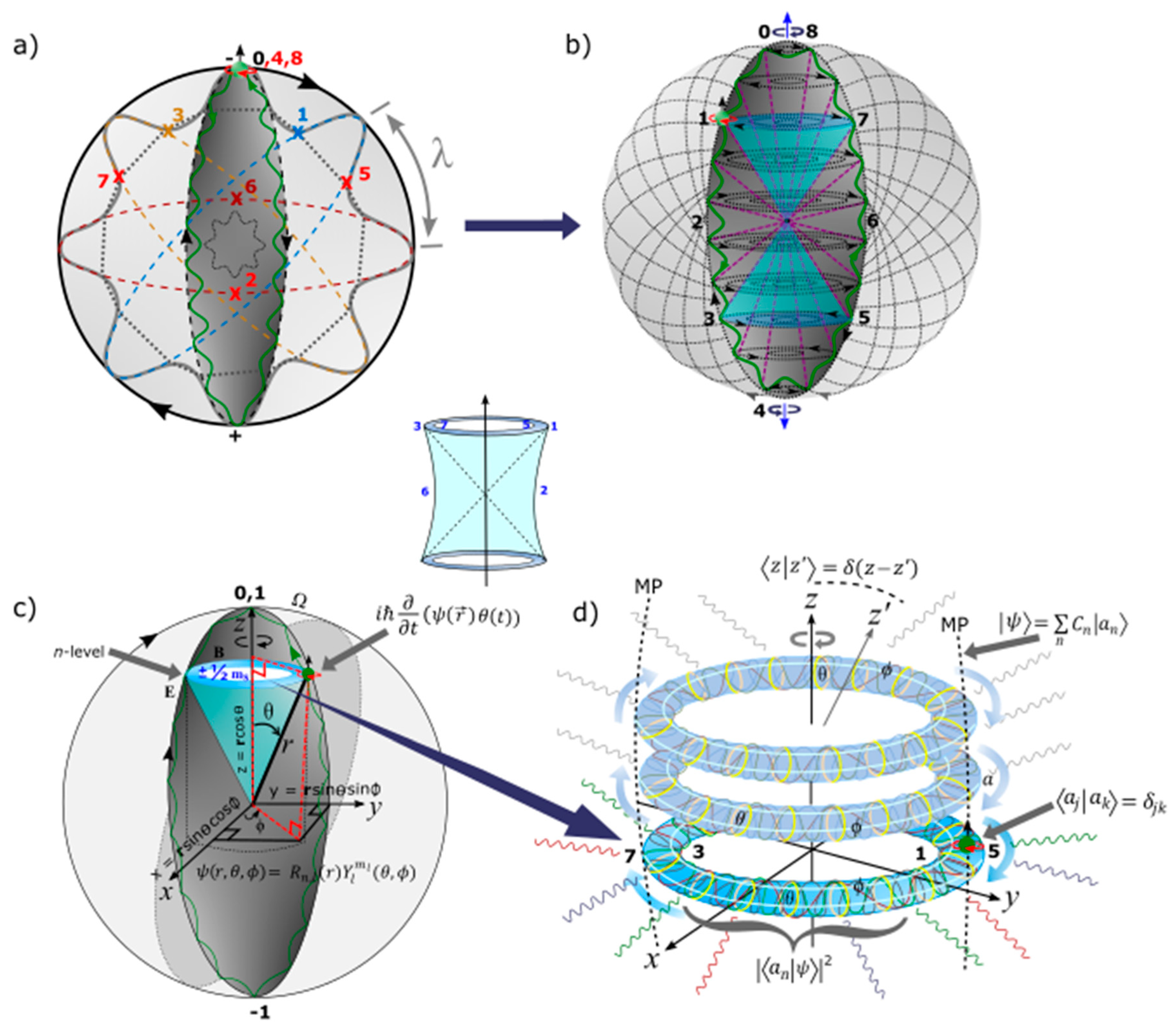
Figure 2.
Exposition of Dirac belt trick. (a) An irreducible spinor field of close loop within a hemisphere is generated at spherical lightspeed with the electron’s (green dot) shift in position of time reversal against clockwise precession of the MP model (Figure 1a). The position of the particle on a straight path (colored lines) is referenced to the MP field of elliptical shape (centered image). (b) Maximum twist is attained at position 4 as detectable energy and the unfolding process by another 360° rotation for a total of 720° rotation restores the electron at position 8 or 0 akin to Dirac belt trick. (c) Precession normalizes the loop to generate an electron of positive helicity or right-handedness. Spin up vector at the point-boundary correlates with the direction of precession. (d) At position 4, the electron flips to a positron of negative helicity or left-handedness. The spin down vector is in opposite direction to the direction of precession to begin the unfolding process. Transposition is attained at 720° rotation, with is expansion coefficients, is unit matrices and is electron-positron transition. (e) Irreducible spinor field at spherical lightspeed. Cancelation of charges at conjugate positions, 1, 3 and 5, 7 from on-shell momentum offers close loops of BOs into 3D of discrete form to stabilize the electron to only generate either spin up or spin down states respectively at position 0 and 4. The point of singularity at the center is evaded by shift in the electron orbit. The slight tilt at position 4 compared to position 0 is attributed to energy loss from the electron-positron transition in the form, . (f) Polarization of the model either horizontally or vertically with respect to the electron-positron pair, ±i at position 0 by 720° rotation generates qubits 0, 1 and hypercharge of –1 respectively at positions 0, 8 and 4. These are relevant to classical computing and accessibility to quantum computing for the nucleons are restricted by the chaotic system of envelop solitons at positions 2 and 6 (see also subsection 2d). Image (e) adapted from ref. [20].
Figure 2.
Exposition of Dirac belt trick. (a) An irreducible spinor field of close loop within a hemisphere is generated at spherical lightspeed with the electron’s (green dot) shift in position of time reversal against clockwise precession of the MP model (Figure 1a). The position of the particle on a straight path (colored lines) is referenced to the MP field of elliptical shape (centered image). (b) Maximum twist is attained at position 4 as detectable energy and the unfolding process by another 360° rotation for a total of 720° rotation restores the electron at position 8 or 0 akin to Dirac belt trick. (c) Precession normalizes the loop to generate an electron of positive helicity or right-handedness. Spin up vector at the point-boundary correlates with the direction of precession. (d) At position 4, the electron flips to a positron of negative helicity or left-handedness. The spin down vector is in opposite direction to the direction of precession to begin the unfolding process. Transposition is attained at 720° rotation, with is expansion coefficients, is unit matrices and is electron-positron transition. (e) Irreducible spinor field at spherical lightspeed. Cancelation of charges at conjugate positions, 1, 3 and 5, 7 from on-shell momentum offers close loops of BOs into 3D of discrete form to stabilize the electron to only generate either spin up or spin down states respectively at position 0 and 4. The point of singularity at the center is evaded by shift in the electron orbit. The slight tilt at position 4 compared to position 0 is attributed to energy loss from the electron-positron transition in the form, . (f) Polarization of the model either horizontally or vertically with respect to the electron-positron pair, ±i at position 0 by 720° rotation generates qubits 0, 1 and hypercharge of –1 respectively at positions 0, 8 and 4. These are relevant to classical computing and accessibility to quantum computing for the nucleons are restricted by the chaotic system of envelop solitons at positions 2 and 6 (see also subsection 2d). Image (e) adapted from ref. [20].
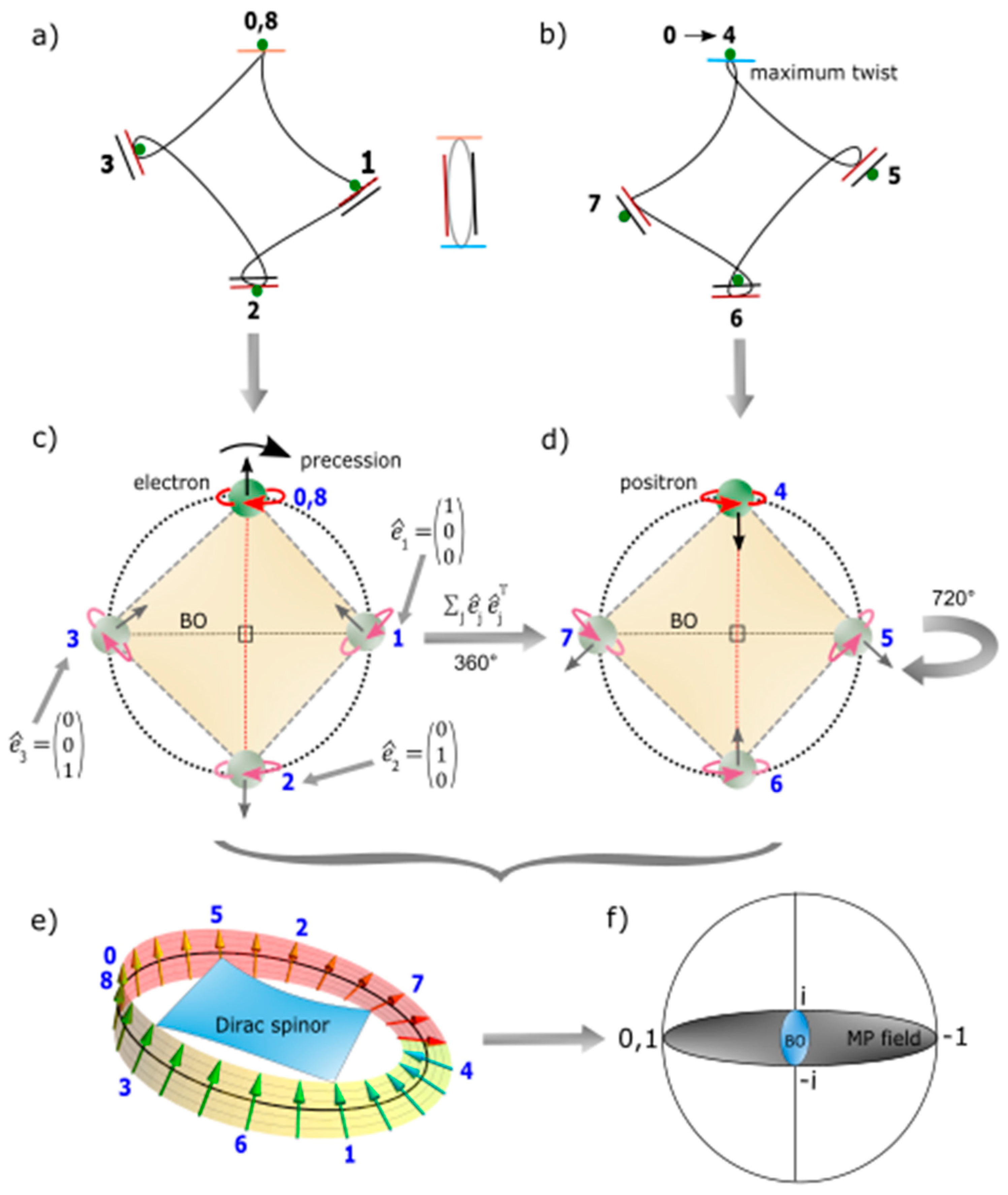
Figure 3.
Wave function collapse. (a) Irreducible Dirac spinor is defined by vector space superimposed on Poincaré sphere. It consists of both Euclidean (straight paths) and non-Euclidean (negative and positive curves) spaces. Clockwise precession by geodesic motion induces a circle at 360° rotation to the negative curves at positions 0 to 3 (e.g., Figure 2c). The polar coordinates (r, θ, Φ) are linked to a light-cone (navy colored). The inward position 6 is projected outward and vice versa for position 2. (b) By on-shell momentum of BO with respect to the electron position in transition is applicable to Fourier transform (blue wavy curve) into linear time. Positions 2 and 6 constrains the reach of singularity. Zooming in towards real particle emergence at position 0 presents both Heisenberg uncertainty principle (black wavy curve) and superposition state. (c) The particle shifts in position and levitation of BOs into n-dimensions between a pair of hemispheres (Figure 1d) can somehow translate to a typical hydrogen emission spectrum. The amplitudes by Fourier transform are shown by white spectral lines and the uncertainty principle with respect to the particle property by colored spectral lines. .
Figure 3.
Wave function collapse. (a) Irreducible Dirac spinor is defined by vector space superimposed on Poincaré sphere. It consists of both Euclidean (straight paths) and non-Euclidean (negative and positive curves) spaces. Clockwise precession by geodesic motion induces a circle at 360° rotation to the negative curves at positions 0 to 3 (e.g., Figure 2c). The polar coordinates (r, θ, Φ) are linked to a light-cone (navy colored). The inward position 6 is projected outward and vice versa for position 2. (b) By on-shell momentum of BO with respect to the electron position in transition is applicable to Fourier transform (blue wavy curve) into linear time. Positions 2 and 6 constrains the reach of singularity. Zooming in towards real particle emergence at position 0 presents both Heisenberg uncertainty principle (black wavy curve) and superposition state. (c) The particle shifts in position and levitation of BOs into n-dimensions between a pair of hemispheres (Figure 1d) can somehow translate to a typical hydrogen emission spectrum. The amplitudes by Fourier transform are shown by white spectral lines and the uncertainty principle with respect to the particle property by colored spectral lines. .
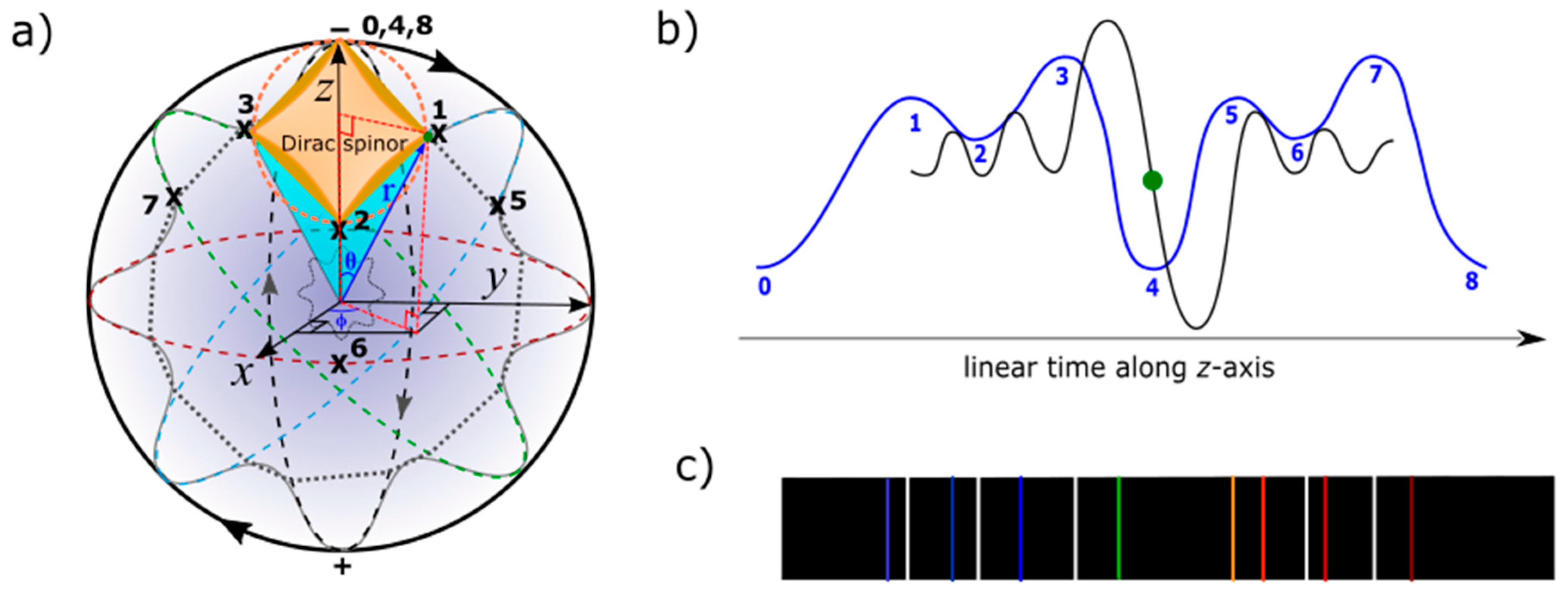
Figure 4.
Light-MP model coupling. (a) To an external observer, the topological point-boundary provides the origin for the emergence of the oscillator (maroon light-cones). Total angular momentum, is minimal with S and L in opposite directions. Levitation of BOs into n-dimensions from n to k for the pair of interchangeable hemispheres coalesces at the point-boundary of COM assigned to electron-positron transition at position 0. The BOs in degeneracy, Φi (see also Figure 1d) can accommodate Fermi-Dirac statistics (green wavy curve) and possibly Fock space for non-relativistic many-particle systems if multielectron are assigned to multiple MP fields. The observable oscillator is partitioned at an infinite boundary towards the center of the MP field and is equivalent to atomic wave of linear time. The blue light-cone is from the perspective of the observer at the center and is relevant to Schrödinger wave function (e.g., Figure 1c) with subshells assigned to spectroscopic notations at n-dimensions. (b) The emergence of quantized magnetic moment, from the point-boundary (maroon light-cones) levitates about the internal frame of the model (blue light-cones). Parity transformation for the conjugate pairs is confined to a hemisphere (e.g., Figure 2a,b) and it is related to z-axis of nuclear isospin. Minimal scatterings (green wavy curves) are applicable to light-MP model interactions along the BOs for the eigenfunction, .
Figure 4.
Light-MP model coupling. (a) To an external observer, the topological point-boundary provides the origin for the emergence of the oscillator (maroon light-cones). Total angular momentum, is minimal with S and L in opposite directions. Levitation of BOs into n-dimensions from n to k for the pair of interchangeable hemispheres coalesces at the point-boundary of COM assigned to electron-positron transition at position 0. The BOs in degeneracy, Φi (see also Figure 1d) can accommodate Fermi-Dirac statistics (green wavy curve) and possibly Fock space for non-relativistic many-particle systems if multielectron are assigned to multiple MP fields. The observable oscillator is partitioned at an infinite boundary towards the center of the MP field and is equivalent to atomic wave of linear time. The blue light-cone is from the perspective of the observer at the center and is relevant to Schrödinger wave function (e.g., Figure 1c) with subshells assigned to spectroscopic notations at n-dimensions. (b) The emergence of quantized magnetic moment, from the point-boundary (maroon light-cones) levitates about the internal frame of the model (blue light-cones). Parity transformation for the conjugate pairs is confined to a hemisphere (e.g., Figure 2a,b) and it is related to z-axis of nuclear isospin. Minimal scatterings (green wavy curves) are applicable to light-MP model interactions along the BOs for the eigenfunction, .

Figure 5.
Feynman diagrams of MP models coupling. (a) Two electrons (green dots) or their particle-hole symmetries at the vertices of the MP models may undergo either repulsion or attraction when approaching each other. (b) Ejection of the electron or positron would induce weak particle-hole isospin. The COM of ZPE at the vertices can account for both boson and fermion types such as neutrinos and antineutrinos of helical property mimicking the electron-positron pair (e.g., Figure 2c,d).
Figure 5.
Feynman diagrams of MP models coupling. (a) Two electrons (green dots) or their particle-hole symmetries at the vertices of the MP models may undergo either repulsion or attraction when approaching each other. (b) Ejection of the electron or positron would induce weak particle-hole isospin. The COM of ZPE at the vertices can account for both boson and fermion types such as neutrinos and antineutrinos of helical property mimicking the electron-positron pair (e.g., Figure 2c,d).

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Copyright: This open access article is published under a Creative Commons CC BY 4.0 license, which permit the free download, distribution, and reuse, provided that the author and preprint are cited in any reuse.
Downloads
885
Views
309
Comments
0
Subscription
Notify me about updates to this article or when a peer-reviewed version is published.
MDPI Initiatives
Important Links
© 2025 MDPI (Basel, Switzerland) unless otherwise stated





