Preprint
Article
Neutrosophic 1-Failed SuperHyperForcing in the SuperHyperFunction To Use Neutrosophic SuperHyperGraphs on Cancer’s Neutrosophic Recognition And Beyond
Altmetrics
Downloads
134
Views
30
Comments
0
This version is not peer-reviewed
Submitted:
01 January 2023
Posted:
04 January 2023
You are already at the latest version
Alerts
Abstract
In this research, new setting is introduced for new SuperHyperNotion, namely, 11 Neutrosophic 1-failed SuperHyperForcing. Two different types of SuperHyperDefinitions 12 are debut for them but the research goes further and the SuperHyperNotion, 13 SuperHyperUniform, and SuperHyperClass based on that are well-defined and 14 well-reviewed. The literature review is implemented in the whole of this research. For 15 shining the elegancy and the significancy of this research, the comparison between this 16 SuperHyperNotion with other SuperHyperNotions and fundamental 17 SuperHyperNumbers are featured. The definitions are followed by the examples and the 18 instances thus the clarifications are driven with different tools. The applications are 19 figured out to make sense about the theoretical aspect of this ongoing research. The 20 “Cancer’s Neutrosophic Recognition” are the under research to figure out the challenges 21 make sense about ongoing and upcoming research. The special case is up. The cells are 22 viewed in the deemed ways. There are different types of them. Some of them are 23 individuals and some of them are well-modeled by the group of cells. These types are all 24 officially called “SuperHyperVertex” but the relations amid them all officially called 25 “SuperHyperEdge”. The frameworks “SuperHyperGraph” and “neutrosophic 26 SuperHyperGraph” are chosen and elected to research about “Cancer’s Neutrosophic 27 Recognition”. Thus these complex and dense SuperHyperModels open up some avenues 28 to research on theoretical segments and “Cancer’s Neutrosophic Recognition”. Some 29 avenues are posed to pursue this research. It’s also officially collected in the form of 30 some questions and some problems. Assume a SuperHyperGraph. Then a “1-failed 31 SuperHyperForcing” Z(NSHG) for a neutrosophic SuperHyperGraph NSHG : (V,E) 32 is the maximum cardinality of a SuperHyperSet S of black SuperHyperVertices 33 (whereas SuperHyperVertices in V (G) \ S are colored white) such that V (G) isn’t 34 turned black after finitely many applications of “the color-change rule”: a white 35 SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white 36 SuperHyperNeighbor of a black SuperHyperVertex. The additional condition is referred 37 by “1-” about the usage of any black SuperHyperVertex only once to act on white 38 SuperHyperVertex to be black SuperHyperVertex; a “neutrosophic 1-failed 39 SuperHyperForcing” Zn(NSHG) for a neutrosophic SuperHyperGraph NSHG : (V,E) 40 is the maximum neutrosophic cardinality of a SuperHyperSet S of black 41 SuperHyperVertices (whereas SuperHyperVertices in V (G) \ S are colored white) such 42 that V (G) isn’t turned black after finitely many applications of “the color-change rule”: 43 a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only 44 1/128 white SuperHyperNeighbor of a black SuperHyperVertex. The additional condition is 45 referred by “1-” about the usage of any black SuperHyperVertex only once to act on 46 white SuperHyperVertex to be black SuperHyperVertex. Assume a SuperHyperGraph. 47 Then an “δ−1-failed SuperHyperForcing” is a maximal 1-failed SuperHyperForcing of 48 SuperHyperVertices with maximum cardinality such that either of the following 49 expressions hold for the (neutrosophic) cardinalities of SuperHyperNeighbors of s ∈ S : 50 |S ∩N(s)| > |S ∩(V \N(s))|+δ, |S ∩N(s)| < |S ∩(V \N(s))|+δ. The first Expression, 51 holds if S is an “δ−SuperHyperOffensive”. And the second Expression, holds if S is an 52 “δ−SuperHyperDefensive”; a“neutrosophic δ−1-failed SuperHyperForcing” is a maximal 53 neutrosophic 1-failed SuperHyperForcing of SuperHyperVertices with maximum 54 neutrosophic cardinality such that either of the following expressions hold for the 55 neutrosophic cardinalities of SuperHyperNeighbors of s ∈ S : |S ∩ N(s)|neutrosophic > 56 |S ∩ (V \ N (s))|neutrosophic + δ, |S ∩ N (s)|neutrosophic < |S ∩ (V \ N (s))|neutrosophic + δ. 57 The first Expression, holds if S is a “neutrosophic δ−SuperHyperOffensive”. And the 58 second Expression, holds if S is a “neutrosophic δ−SuperHyperDefensive”. It’s useful to 59 define “neutrosophic” version of 1-failed SuperHyperForcing. Since there’s more ways to 60 get type-results to make 1-failed SuperHyperForcing more understandable. For the sake 61 of having neutrosophic 1-failed SuperHyperForcing, there’s a need to “redefine” the 62 notion of “1-failed SuperHyperForcing”. The SuperHyperVertices and the 63 SuperHyperEdges are assigned by the labels from the letters of the alphabets. In this 64 procedure, there’s the usage of the position of labels to assign to the values. Assume a 65 1-failed SuperHyperForcing. It’s redefined neutrosophic 1-failed SuperHyperForcing if 66 the mentioned Table holds, concerning, “The Values of Vertices, SuperVertices, Edges, 67 HyperEdges, and SuperHyperEdges Belong to The Neutrosophic SuperHyperGraph” 68 with the key points, “The Values of The Vertices & The Number of Position in 69 Alphabet”, “The Values of The SuperVertices&The maximum Values of Its Vertices”, 70 “The Values of The Edges&The maximum Values of Its Vertices”, “The Values of The 71 HyperEdges&The maximum Values of Its Vertices”, “The Values of The 72 SuperHyperEdges&The maximum Values of Its Endpoints”. To get structural examples 73 and instances, I’m going to introduce the next SuperHyperClass of SuperHyperGraph 74 based on 1-failed SuperHyperForcing. It’s the main. It’ll be disciplinary to have the 75 foundation of previous definition in the kind of SuperHyperClass. If there’s a need to 76 have all SuperHyperConnectivities until the 1-failed SuperHyperForcing, then it’s 77 officially called “1-failed SuperHyperForcing” but otherwise, it isn’t 1-failed 78 SuperHyperForcing. There are some instances about the clarifications for the main 79 definition titled “1-failed SuperHyperForcing”. These two examples get more scrutiny 80 and discernment since there are characterized in the disciplinary ways of the 81 SuperHyperClass based on 1-failed SuperHyperForcing. For the sake of having 82 neutrosophic 1-failed SuperHyperForcing, there’s a need to “redefine” the notion of 83 “neutrosophic 1-failed SuperHyperForcing” and “neutrosophic 1-failed 84 SuperHyperForcing”. The SuperHyperVertices and the SuperHyperEdges are assigned 85 by the labels from the letters of the alphabets. In this procedure, there’s the usage of 86 the position of labels to assign to the values. Assume a neutrosophic SuperHyperGraph. 87 It’s redefined “neutrosophic SuperHyperGraph” if the intended Table holds. And 88 1-failed SuperHyperForcing are redefined “neutrosophic 1-failed SuperHyperForcing” if 89 the intended Table holds. It’s useful to define “neutrosophic” version of 90 SuperHyperClasses. Since there’s more ways to get neutrosophic type-results to make 91 neutrosophic 1-failed SuperHyperForcing more understandable. Assume a neutrosophic 92 SuperHyperGraph. There are some neutrosophic SuperHyperClasses if the intended 93 Table holds. Thus SuperHyperPath, SuperHyperCycle, SuperHyperStar, 94 SuperHyperBipartite, SuperHyperMultiPartite, and SuperHyperWheel, are 95 “neutrosophic SuperHyperPath”, “neutrosophic SuperHyperCycle”, “neutrosophic 96 SuperHyperStar”, “neutrosophic SuperHyperBipartite”, “neutrosophic 97 2/128 SuperHyperMultiPartite”, and “neutrosophic SuperHyperWheel” if the intended Table 98 holds. A SuperHyperGraph has “neutrosophic 1-failed SuperHyperForcing” where it’s 99 the strongest [the maximum neutrosophic value from all 1-failed SuperHyperForcing 100 amid the maximum value amid all SuperHyperVertices from a 1-failed 101 SuperHyperForcing.] 1-failed SuperHyperForcing. A graph is SuperHyperUniform if it’s 102 SuperHyperGraph and the number of elements of SuperHyperEdges are the same. 103 Assume a neutrosophic SuperHyperGraph. There are some SuperHyperClasses as 104 follows. It’s SuperHyperPath if it’s only one SuperVertex as intersection amid two given 105 SuperHyperEdges with two exceptions; it’s SuperHyperCycle if it’s only one 106 SuperVertex as intersection amid two given SuperHyperEdges; it’s SuperHyperStar it’s 107 only one SuperVertex as intersection amid all SuperHyperEdges; it’s 108 SuperHyperBipartite it’s only one SuperVertex as intersection amid two given 109 SuperHyperEdges and these SuperVertices, forming two separate sets, has no 110 SuperHyperEdge in common; it’s SuperHyperMultiPartite it’s only one SuperVertex as 111 intersection amid two given SuperHyperEdges and these SuperVertices, forming multi 112 separate sets, has no SuperHyperEdge in common; it’s SuperHyperWheel if it’s only one 113 SuperVertex as intersection amid two given SuperHyperEdges and one SuperVertex has 114 one SuperHyperEdge with any common SuperVertex. The SuperHyperModel proposes 115 the specific designs and the specific architectures. The SuperHyperModel is officially 116 called “SuperHyperGraph” and “Neutrosophic SuperHyperGraph”. In this 117 SuperHyperModel, The “specific” cells and “specific group” of cells are 118 SuperHyperModeled as “SuperHyperVertices” and the common and intended properties 119 between “specific” cells and “specific group” of cells are SuperHyperModeled as 120 “SuperHyperEdges”. Sometimes, it’s useful to have some degrees of determinacy, 121 indeterminacy, and neutrality to have more precise SuperHyperModel which in this case 122 the SuperHyperModel is called “neutrosophic”. In the future research, the foundation 123 will be based on the “Cancer’s Neutrosophic Recognition” and the results and the 124 definitions will be introduced in redeemed ways. The neutrosophic recognition of the 125 cancer in the long-term function. The specific region has been assigned by the model 126 [it’s called SuperHyperGraph] and the long cycle of the move from the cancer is 127 identified by this research. Sometimes the move of the cancer hasn’t be easily identified 128 since there are some determinacy, indeterminacy and neutrality about the moves and 129 the effects of the cancer on that region; this event leads us to choose another model [it’s 130 said to be neutrosophic SuperHyperGraph] to have convenient perception on what’s 131 happened and what’s done. There are some specific models, which are well-known and 132 they’ve got the names, and some SuperHyperGeneral SuperHyperModels. The moves 133 and the traces of the cancer on the complex tracks and between complicated groups of 134 cells could be fantasized by a neutrosophic SuperHyperPath(-/SuperHyperCycle, 135 SuperHyperStar, SuperHyperBipartite, SuperHyperMultipartite, SuperHyperWheel). 136 The aim is to find either the longest 1-failed SuperHyperForcing or the strongest 137 1-failed SuperHyperForcing in those neutrosophic SuperHyperModels. For the longest 138 1-failed SuperHyperForcing, called 1-failed SuperHyperForcing, and the strongest 139 SuperHyperCycle, called neutrosophic 1-failed SuperHyperForcing, some general results 140 are introduced. Beyond that in SuperHyperStar, all possible SuperHyperPaths have 141 only two SuperHyperEdges but it’s not enough since it’s essential to have at least three 142 SuperHyperEdges to form any style of a SuperHyperCycle. There isn’t any formation of 143 any SuperHyperCycle but literarily, it’s the deformation of any SuperHyperCycle. It, 144 literarily, deforms and it doesn’t form. A basic familiarity with SuperHyperGraph 145 theory and neutrosophic SuperHyperGraph theory are proposed.
Keywords:
Subject: Computer Science and Mathematics - Computer Vision and Graphics
AMS Subject Classification: 05C17, 05C22, 05E45
1. Background
There are some researches covering the topic of this research. In what follows, there are some discussion and literature reviews about them.
First article is titled “properties of SuperHyperGraph and neutrosophic SuperHyperGraph” in Ref. [1] by Henry Garrett (2022). It’s first step toward the research on neutrosophic SuperHyperGraphs. This research article is published on the journal “Neutrosophic Sets and Systems” in issue 49 and the pages 531-561. In this research article, different types of notions like dominating, resolving, coloring, Eulerian(Hamiltonian) neutrosophic path, n-Eulerian(Hamiltonian) neutrosophic path, zero forcing number, zero forcing neutrosophic- number, independent number, independent neutrosophic-number, clique number, clique neutrosophic-number, matching number, matching neutrosophic-number, girth, neutrosophic girth, 1-zero-forcing number, 1-zero- forcing neutrosophic-number, failed 1-zero-forcing number, failed 1-zero-forcing neutrosophic-number, global- offensive alliance, t-offensive alliance, t-defensive alliance, t-powerful alliance, and global-powerful alliance are defined in SuperHyperGraph and neutrosophic SuperHyperGraph. Some Classes of SuperHyperGraph and Neutrosophic SuperHyperGraph are cases of research. Some results are applied in family of SuperHyperGraph and neutrosophic SuperHyperGraph. Thus this research article has concentrated on the vast notions and introducing the majority of notions.
The seminal paper and groundbreaking article is titled “neutrosophic co-degree and neutrosophic degree alongside chromatic numbers in the setting of some classes related to neutrosophic hypergraphs” in Ref. [2] by Henry Garrett (2022). In this research article, a novel approach is implemented on SuperHyperGraph and neutrosophic SuperHyperGraph based on general forms without using neutrosophic classes of neutrosophic SuperHyperGraph. It’s published in prestigious and fancy journal is entitled “Journal of Current Trends in Computer Science Research (JCTCSR)” with abbreviation “J Curr Trends Comp Sci Res” in volume 1 and issue 1 with pages 06-14. The research article studies deeply with choosing neutrosophic hypergraphs instead of neutrosophic SuperHyperGraph. It’s the breakthrough toward independent results based on initial background.
In some articles are titled “(Neutrosophic) SuperHyperModeling of Cancer’s Recognitions Featuring (Neutrosophic) SuperHyperDefensive SuperHyperAlliances” in Ref. [3] by Henry Garrett (2022), “(Neutrosophic) SuperHyperAlliances With SuperHyperDefensive and SuperHyperOffensive Type-SuperHyperSet On (Neutrosophic) SuperHyperGraph With (Neutrosophic) SuperHyperModeling of Cancer’s Recognitions And Related (Neutrosophic) SuperHyperClasses” in Ref. [4] by Henry Garrett (2022), “SuperHyperGirth on SuperHyperGraph and Neutrosophic SuperHyperGraph With SuperHyperModeling of Cancer’s Recognitions” in Ref. [5] by Henry Garrett (2022), “Some SuperHyperDegrees and Co-SuperHyperDegrees on Neutrosophic SuperHyperGraphs and SuperHyperGraphs Alongside Applications in Cancer’s Treatments” in Ref. [6] by Henry Garrett (2022), “SuperHyperDominating and SuperHyperResolving on Neutrosophic SuperHyperGraphs And Their Directions in Game Theory and Neutrosophic SuperHyperClasses” in Ref. [7] by Henry Garrett (2022), “Basic Notions on (Neutrosophic) SuperHyperForcing And (Neutrosophic) SuperHyperModeling in Cancer’s Recognitions And (Neutrosophic) SuperHyperGraphs” in Ref. [8] by Henry Garrett (2022), “Basic Neutrosophic Notions Concerning SuperHyperDominating and Neutrosophic SuperHyperResolving in SuperHyperGraph” in Ref. [9] by Henry Garrett (2022), “Initial Material of Neutrosophic Preliminaries to Study Some Neutrosophic Notions Based on Neutrosophic SuperHyperEdge (NSHE) in Neutrosophic SuperHyperGraph (NSHG)” in Ref. [10] by Henry Garrett (2022), there are some endeavors to formalize the basic SuperHyperNotions about neutrosophic SuperHyperGraph and SuperHyperGraph.
Some studies and researches about neutrosophic graphs, are proposed as book in Ref. [11] by Henry Garrett (2022) which is indexed by Google Scholar and has more than 2347 readers in Scribd. It’s titled “Beyond Neutrosophic Graphs” and published by Ohio: E-publishing: Educational Publisher 1091 West 1st Ave Grandview Heights, Ohio 43212 United State. This research book covers different types of notions and settings in neutrosophic graph theory and neutrosophic SuperHyperGraph theory.
Also, some studies and researches about neutrosophic graphs, are proposed as book in Ref. [12] by Henry Garrett (2022) which is indexed by Google Scholar and has more than 3048 readers in Scribd. It’s titled “Neutrosophic Duality” and published by Florida: GLOBAL KNOWLEDGE - Publishing House 848 Brickell Ave Ste 950 Miami, Florida 33131 United States. This research book presents different types of notions SuperHyperResolving and SuperHyperDominating in the setting of duality in neutrosophic graph theory and neutrosophic SuperHyperGraph theory. This research book has scrutiny on the complement of the intended set and the intended set, simultaneously. It’s smart to consider a set but acting on its complement that what’s done in this research book which is popular in the terms of high readers in Scribd.
1.1. Motivation and Contributions
In this research, there are some ideas in the featured frameworks of motivations. I try to bring the motivations in the narrative ways. Some cells have been faced with some attacks from the situation which is caused by the cancer’s attacks. In this case, there are some embedded analysis on the ongoing situations which in that, the cells could be labelled as some groups and some groups or individuals have excessive labels which all are raised from the behaviors to overcome the cancer’s attacks. In the embedded situations, the individuals of cells and the groups of cells could be considered as “new groups”. Thus it motivates us to find the proper SuperHyperModels for getting more proper analysis on this messy story. I’ve found the SuperHyperModels which are officially called “SuperHyperGraphs” and “Neutrosophic SuperHyperGraphs”. In this SuperHyperModel, the cells and the groups of cells are defined as “SuperHyperVertices” and the relations between the individuals of cells and the groups of cells are defined as “SuperHyperEdges”. Thus it’s another motivation for us to do research on this SuperHyperModel based on the “Cancer’s Neutrosophic Recognition”. Sometimes, the situations get worst. The situation is passed from the certainty and precise style. Thus it’s the beyond them. There are three descriptions, namely, the degrees of determinacy, indeterminacy and neutrality, for any object based on vague forms, namely, incomplete data, imprecise data, and uncertain analysis. The latter model could be considered on the previous SuperHyperModel. It’s SuperHyperModel. It’s SuperHyperGraph but it’s officially called “Neutrosophic SuperHyperGraphs”. The cancer is the disease but the model is going to figure out what’s going on this phenomenon. The special case of this disease is considered and as the consequences of the model, some parameters are used. The cells are under attack of this disease but the moves of the cancer in the special region are the matter of mind. The neutrosophic recognition of the cancer could help to find some treatments for this disease. The SuperHyperGraph and neutrosophic SuperHyperGraph are the SuperHyperModels on the “Cancer’s Neutrosophic Recognition” and both bases are the background of this research. Sometimes the cancer has been happened on the region, full of cells, groups of cells and embedded styles. In this segment, the SuperHyperModel proposes some SuperHyperNotions based on the connectivities of the moves of the cancer in the forms of alliances’ styles with the formation of the design and the architecture are formally called “1-failed SuperHyperForcing” in the themes of jargons and buzzwords. The prefix “SuperHyper” refers to the theme of the embedded styles to figure out the background for the SuperHyperNotions. The neutrosophic recognition of the cancer in the long-term function. The specific region has been assigned by the model [it’s called SuperHyperGraph] and the long cycle of the move from the cancer is identified by this research. Sometimes the move of the cancer hasn’t be easily identified since there are some determinacy, indeterminacy and neutrality about the moves and the effects of the cancer on that region; this event leads us to choose another model [it’s said to be neutrosophic SuperHyperGraph] to have convenient perception on what’s happened and what’s done. There are some specific models, which are well-known and they’ve got the names, and some general models. The moves and the traces of the cancer on the complex tracks and between complicated groups of cells could be fantasized by a neutrosophic SuperHyperPath(-/SuperHyperCycle, SuperHyperStar, SuperHyperBipartite, SuperHyperMultipartite, SuperHyperWheel). The aim is to find either the optimal 1-failed SuperHyperForcing or the neutrosophic 1-failed SuperHyperForcing in those neutrosophic SuperHyperModels. Some general results are introduced. Beyond that in SuperHyperStar, all possible SuperHyperPaths have only two SuperHyperEdges but it’s not enough since it’s essential to have at least three SuperHyperEdges to form any style of a SuperHyperCycle. There isn’t any formation of any SuperHyperCycle but literarily, it’s the deformation of any SuperHyperCycle. It, literarily, deforms and it doesn’t form.
Question 1.
How to define the SuperHyperNotions and to do research on them to find the “ amount of 1-failed SuperHyperForcing” of either individual of cells or the groups of cells based on the fixed cell or the fixed group of cells, extensively, the “amount of 1-failed SuperHyperForcing” based on the fixed groups of cells or the fixed groups of group of cells?
Question 2.
What are the best descriptions for the “Cancer’s Neutrosophic Recognition” in terms of these messy and dense SuperHyperModels where embedded notions are illustrated?
It’s motivation to find notions to use in this dense model is titled “SuperHyperGraphs”. Thus it motivates us to define different types of “1-failed SuperHyperForcing” and “neutrosophic 1-failed SuperHyperForcing” on “SuperHyperGraph” and “Neutrosophic SuperHyperGraph”. Then the research has taken more motivations to define SuperHyperClasses and to find some connections amid this SuperHyperNotion with other SuperHyperNotions. It motivates us to get some instances and examples to make clarifications about the framework of this research. The general results and some results about some connections are some avenues to make key point of this research, “Cancer’s Neutrosophic Recognition”, more understandable and more clear.
The framework of this research is as follows. In the beginning, I introduce basic definitions to clarify about preliminaries. In the subsection “Preliminaries”, initial definitions about SuperHyperGraphs and neutrosophic SuperHyperGraph are deeply-introduced and in-depth-discussed. The elementary concepts are clarified and illustrated completely and sometimes review literature are applied to make sense about what’s going to figure out about the upcoming sections. The main definitions and their clarifications alongside some results about new notions, 1-failed SuperHyperForcing and neutrosophic 1-failed SuperHyperForcing, are figured out in sections “1-failed SuperHyperForcing” and “Neutrosophic 1-failed SuperHyperForcing”. In the sense of tackling on getting results and in order to make sense about continuing the research, the ideas of SuperHyperUniform and Neutrosophic SuperHyperUniform are introduced and as their consequences, corresponded SuperHyperClasses are figured out to debut what’s done in this section, titled “Results on SuperHyperClasses” and “Results on Neutrosophic SuperHyperClasses”. As going back to origin of the notions, there are some smart steps toward the common notions to extend the new notions in new frameworks, SuperHyperGraph and Neutrosophic SuperHyperGraph, in the sections “Results on SuperHyperClasses” and “Results on Neutrosophic SuperHyperClasses”. The starter research about the general SuperHyperRelations and as concluding and closing section of theoretical research are contained in the section “General Results”. Some general SuperHyperRelations are fundamental and they are well-known as fundamental SuperHyperNotions as elicited and discussed in the sections, “General Results”, “1-failed SuperHyperForcing”, “Neutrosophic 1-failed SuperHyperForcing”, “Results on SuperHyperClasses” and “Results on Neutrosophic SuperHyperClasses”. There are curious questions about what’s done about the SuperHyperNotions to make sense about excellency of this research and going to figure out the word “best” as the description and adjective for this research as presented in section, “1-failed SuperHyperForcing”. The keyword of this research debut in the section “Applications in Cancer’s Neutrosophic Recognition” with two cases and subsections “Case 1: The Initial Steps Toward SuperHyperBipartite as SuperHyperModel” and “Case 2: The Increasing Steps Toward SuperHyperMultipartite as SuperHyperModel”. In the section, “Open Problems”, there are some scrutiny and discernment on what’s done and what’s happened in this research in the terms of “questions” and “problems” to make sense to figure out this research in featured style. The advantages and the limitations of this research alongside about what’s done in this research to make sense and to get sense about what’s figured out are included in the section, “Conclusion and Closing Remarks”.
1.2. Preliminaries
In this subsection, the basic material which is used in this research, is presented. Also, the new ideas and their clarifications are elicited.
Definition 3
(Neutrosophic Set). (Ref.[14],Definition 2.1,p.87).
Let X be a space of points (objects) with generic elements in X denoted by then the neutrosophic set A (NS A) is an object having the form
where the functions define respectively the a truth-membership function, an indeterminacy-membership function, and a falsity-membership function of the element to the set A with the condition
The functions and are real standard or nonstandard subsets of
Definition 4
(Single Valued Neutrosophic Set). (Ref. [17],Definition 6,p.2).
Let X be a space of points (objects) with generic elements in X denoted by A single valued neutrosophic set A (SVNS A) is characterized by truth-membership function an indeterminacy-membership function and a falsity-membership function For each point x in A SVNS A can be written as
Definition 5.
The degree of truth-membership, indeterminacy-membership and falsity-membership of the subset of the single valued neutrosophic set :
Definition 6.
The support of of the single valued neutrosophic set :
Definition 7
(Neutrosophic SuperHyperGraph (NSHG)). (Ref.[16],Definition 3,p.291).
Assume is a given set. A neutrosophic SuperHyperGraph (NSHG) S is an ordered pair where
- (i)
- a finite set of finite single valued neutrosophic subsets of
- (ii)
- (iii)
- a finite set of finite single valued neutrosophic subsets of
- (iv)
- (v)
- (vi)
- (vii)
- (viii)
- (ix)
- and the following conditions hold:where
Here the neutrosophic SuperHyperEdges (NSHE) and the neutrosophic SuperHyperVertices (NSHV) are single valued neutrosophic sets. and denote the degree of truth-membership, the degree of indeterminacy-membership and the degree of falsity-membership the neutrosophic SuperHyperVertex (NSHV) to the neutrosophic SuperHyperVertex (NSHV) and denote the degree of truth-membership, the degree of indeterminacy-membership and the degree of falsity-membership of the neutrosophic SuperHyperEdge (NSHE) to the neutrosophic SuperHyperEdge (NSHE) Thus, the th element of the incidence matrix of neutrosophic SuperHyperGraph (NSHG) are of the form , the sets V and E are crisp sets.
Definition 8
Assume a neutrosophic SuperHyperGraph (NSHG) S is an ordered pair The neutrosophic SuperHyperEdges (NSHE) and the neutrosophic SuperHyperVertices (NSHV) of neutrosophic SuperHyperGraph (NSHG) could be characterized as follow-up items.
- If then is called vertex;
- if then is called SuperVertex;
- if for all s are incident in and then is called edge;
- if for all s are incident in and then is called HyperEdge;
- if there’s a is incident in such that and then is called SuperEdge;
- if there’s a is incident in such that and then is called SuperHyperEdge.
If we choose different types of binary operations, then we could get hugely diverse types of general forms of neutrosophic SuperHyperGraph (NSHG).
Definition 9
(t-norm). (Ref.[15], Definition 5.1.1, pp.82-83).
A binary operation is a t-norm if it satisfies the following for :
- If and then
Definition 10.
The degree of truth-membership, indeterminacy-membership and falsity-membership of the subset of the single valued neutrosophic set (with respect to t-norm ):
Definition 11.
The support of of the single valued neutrosophic set :
Definition 12.
(General Forms of Neutrosophic SuperHyperGraph (NSHG)).
Assume is a given set. A neutrosophic SuperHyperGraph (NSHG) S is an ordered pair where
- a finite set of finite single valued neutrosophic subsets of
- a finite set of finite single valued neutrosophic subsets of
Here the neutrosophic SuperHyperEdges (NSHE) and the neutrosophic SuperHyperVertices (NSHV) are single valued neutrosophic sets. and denote the degree of truth-membership, the degree of indeterminacy-membership and the degree of falsity-membership the neutrosophic SuperHyperVertex (NSHV) to the neutrosophic SuperHyperVertex (NSHV) and denote the degree of truth-membership, the degree of indeterminacy-membership and the degree of falsity-membership of the neutrosophic SuperHyperEdge (NSHE) to the neutrosophic SuperHyperEdge (NSHE) Thus, the th element of the incidence matrix of neutrosophic SuperHyperGraph (NSHG) are of the form , the sets V and E are crisp sets.
Definition 13
Assume a neutrosophic SuperHyperGraph (NSHG) S is an ordered pair The neutrosophic SuperHyperEdges (NSHE) and the neutrosophic SuperHyperVertices (NSHV) of neutrosophic SuperHyperGraph (NSHG) could be characterized as follow-up items.
- If then is called vertex;
- if then is called SuperVertex;
- if for all s are incident in and then is called edge;
- if for all s are incident in and then is called HyperEdge;
- if there’s a is incident in such that and then is called SuperEdge;
- if there’s a is incident in such that and then is called SuperHyperEdge.
This SuperHyperModel is too messy and too dense. Thus there’s a need to have some restrictions and conditions on SuperHyperGraph. The special case of this SuperHyperGraph makes the patterns and regularities.
Definition 14.
A graph is SuperHyperUniform if it’s SuperHyperGraph and the number of elements of SuperHyperEdges are the same.
To get more visions on 1-failed SuperHyperForcing, the some SuperHyperClasses are introduced. It makes to have 1-failed SuperHyperForcing more understandable.
Definition 15.
Assume a neutrosophic SuperHyperGraph. There are some SuperHyperClasses as follows.
- (i).
- It’s SuperHyperPath if it’s only one SuperVertex as intersection amid two given SuperHyperEdges with two exceptions;
- (ii).
- it’s SuperHyperCycle if it’s only one SuperVertex as intersection amid two given SuperHyperEdges;
- (iii).
- it’s SuperHyperStar it’s only one SuperVertex as intersection amid all SuperHyperEdges;
- (iv).
- it’s SuperHyperBipartite it’s only one SuperVertex as intersection amid two given SuperHyperEdges and these SuperVertices, forming two separate sets, has no SuperHyperEdge in common;
- (v).
- it’s SuperHyperMultiPartite it’s only one SuperVertex as intersection amid two given SuperHyperEdges and these SuperVertices, forming multi separate sets, has no SuperHyperEdge in common;
- (vi).
- it’s SuperHyperWheel if it’s only one SuperVertex as intersection amid two given SuperHyperEdges and one SuperVertex has one SuperHyperEdge with any common SuperVertex.
Definition 16.
Let an ordered pair be a neutrosophic SuperHyperGraph (NSHG) Then a sequence of neutrosophic SuperHyperVertices (NSHV) and neutrosophic SuperHyperEdges (NSHE)
is called a neutrosophic SuperHyperPath (NSHP) from neutrosophic SuperHyperVertex (NSHV) to neutrosophic SuperHyperVertex (NSHV) if either of following conditions hold:
- there’s a vertex such that
- there’s a SuperVertex such that
- there’s a vertex such that
- there’s a SuperVertex such that
- there are a vertex and a vertex such that
- there are a vertex and a SuperVertex such that
- there are a SuperVertex and a vertex such that
- there are a SuperVertex and a SuperVertex such that
Definition 17.
(Characterization of the Neutrosophic SuperHyperPaths).
Assume a neutrosophic SuperHyperGraph (NSHG) S is an ordered pair A neutrosophic SuperHyperPath (NSHP) from neutrosophic SuperHyperVertex (NSHV) to neutrosophic SuperHyperVertex (NSHV) is sequence of neutrosophic SuperHyperVertices (NSHV) and neutrosophic SuperHyperEdges (NSHE)
could be characterized as follow-up items.
- If for all then NSHP is called path;
- if for all and there’s then NSHP is called SuperPath;
- if for all then NSHP is called HyperPath;
- if there are then NSHP is called SuperHyperPath.
Definition 18.
((neutrosophic) 1-failed SuperHyperForcing).
Assume a SuperHyperGraph. Then
- a 1-failed SuperHyperForcing for a neutrosophic SuperHyperGraph is the maximum cardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. The additional condition is referred by “1-” about the usage of any black SuperHyperVertex only once to act on white SuperHyperVertex to be black SuperHyperVertex;
- a neutrosophic 1-failed SuperHyperForcing for a neutrosophic SuperHyperGraph is the maximum neutrosophic cardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. The additional condition is referred by “1-” about the usage of any black SuperHyperVertex only once to act on white SuperHyperVertex to be black SuperHyperVertex.
Definition 19.
((neutrosophic) 1-failed SuperHyperForcing).
Assume a SuperHyperGraph. Then
-
a 1-failed SuperHyperForcing is a maximal 1-failed SuperHyperForcing of SuperHyperVertices with maximum cardinality such that either of the following expressions hold for the (neutrosophic) cardinalities of SuperHyperNeighbors ofThe Expression (1.1), holds if S is an SuperHyperOffensive. And the Expression (1.2), holds if S is an SuperHyperDefensive;
-
a neutrosophic 1-failed SuperHyperForcing is a maximal neutrosophic 1-failed SuperHyperForcing of SuperHyperVertices with maximum neutrosophic cardinality such that either of the following expressions hold for the neutrosophic cardinalities of SuperHyperNeighbors ofThe Expression (1.3), holds if S is a neutrosophic SuperHyperOffensive. And the Expression (1.4), holds if S is a neutrosophic SuperHyperDefensive.
For the sake of having neutrosophic 1-failed SuperHyperForcing, there’s a need to “redefine” the notion of “neutrosophic SuperHyperGraph”. The SuperHyperVertices and the SuperHyperEdges are assigned by the labels from the letters of the alphabets. In this procedure, there’s the usage of the position of labels to assign to the values.
Definition 20.
Assume a neutrosophic SuperHyperGraph. It’s redefined neutrosophic SuperHyperGraph if the Table (1) holds.
Table 1.
The Values of Vertices, SuperVertices, Edges, HyperEdges, and SuperHyperEdges Belong to The Neutrosophic SuperHyperGraph Mentioned in the Definition (20)
Table 1.
The Values of Vertices, SuperVertices, Edges, HyperEdges, and SuperHyperEdges Belong to The Neutrosophic SuperHyperGraph Mentioned in the Definition (20)
| The Values of The Vertices | The Number of Position in Alphabet |
| The Values of The SuperVertices | The maximum Values of Its Vertices |
| The Values of The Edges | The maximum Values of Its Vertices |
| The Values of The HyperEdges | The maximum Values of Its Vertices |
| The Values of The SuperHyperEdges | The maximum Values of Its Endpoints |
It’s useful to define “neutrosophic” version of SuperHyperClasses. Since there’s more ways to get neutrosophic type-results to make neutrosophic 1-failed SuperHyperForcing more understandable.
Definition 21.
Assume a neutrosophic SuperHyperGraph. There are some neutrosophic SuperHyperClasses if the Table (2) holds. Thus SuperHyperPath, SuperHyperCycle, SuperHyperStar, SuperHyperBipartite, SuperHyperMultiPartite, and SuperHyperWheel, are neutrosophic SuperHyperPath, neutrosophic SuperHyperCycle, neutrosophic SuperHyperStar, neutrosophic SuperHyperBipartite, neutrosophic SuperHyperMultiPartite, and neutrosophic SuperHyperWheel if the Table (2) holds.
Table 2.
The Values of Vertices, SuperVertices, Edges, HyperEdges, and SuperHyperEdges Belong to The Neutrosophic SuperHyperGraph, Mentioned in the Definition (21)
Table 2.
The Values of Vertices, SuperVertices, Edges, HyperEdges, and SuperHyperEdges Belong to The Neutrosophic SuperHyperGraph, Mentioned in the Definition (21)
| The Values of The Vertices | The Number of Position in Alphabet |
| The Values of The SuperVertices | The maximum Values of Its Vertices |
| The Values of The Edges | The maximum Values of Its Vertices |
| The Values of The HyperEdges | The maximum Values of Its Vertices |
| The Values of The SuperHyperEdges | The maximum Values of Its Endpoints |
It’s useful to define “neutrosophic” version of 1-failed SuperHyperForcing. Since there’s more ways to get type-results to make 1-failed SuperHyperForcing more understandable.
For the sake of having neutrosophic 1-failed SuperHyperForcing, there’s a need to “redefine” the notion of “1-failed SuperHyperForcing”. The SuperHyperVertices and the SuperHyperEdges are assigned by the labels from the letters of the alphabets. In this procedure, there’s the usage of the position of labels to assign to the values.
Definition 22.
Assume a 1-failed SuperHyperForcing. It’s redefined neutrosophic 1-failed SuperHyperForcing if the Table (3) holds.
Table 3.
The Values of Vertices, SuperVertices, Edges, HyperEdges, and SuperHyperEdges Belong to The Neutrosophic SuperHyperGraph Mentioned in the Definition (22)
Table 3.
The Values of Vertices, SuperVertices, Edges, HyperEdges, and SuperHyperEdges Belong to The Neutrosophic SuperHyperGraph Mentioned in the Definition (22)
| The Values of The Vertices | The Number of Position in Alphabet |
| The Values of The SuperVertices | The maximum Values of Its Vertices |
| The Values of The Edges | The maximum Values of Its Vertices |
| The Values of The HyperEdges | The maximum Values of Its Vertices |
| The Values of The SuperHyperEdges | The maximum Values of Its Endpoints |
2. Neutrosophic 1-Failed SuperHyperForcing
Example 23.
Assume the neutrosophic SuperHyperGraphs in the Figures (1), (2), (3), (4), (5), (6), (7), (8), (9), (10), (11), (12), (13), (14), (15), (16), (17), (18), (19), and (20).
-
On the Figure (1), the neutrosophic SuperHyperNotion, namely, neutrosophic 1-failed SuperHyperForcing, is up. and are some empty neutrosophic SuperHyperEdges but is a loop neutrosophic SuperHyperEdge and is an neutrosophic SuperHyperEdge. Thus in the terms of neutrosophic SuperHyperNeighbor, there’s only one neutrosophic SuperHyperEdge, namely, The neutrosophic SuperHyperVertex, is isolated means that there’s no neutrosophic SuperHyperEdge has it as an endpoint. Thus neutrosophic SuperHyperVertex, is contained in every given neutrosophic 1-failed SuperHyperForcing. All the following neutrosophic SuperHyperSets of neutrosophic SuperHyperVertices are the simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing.The neutrosophic SuperHyperSets of neutrosophic SuperHyperVertices, are the simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing. The neutrosophic SuperHyperSets of the neutrosophic SuperHyperVertices, are the maximum neutrosophic cardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex. There’re only two neutrosophic SuperHyperVertices outside the intended neutrosophic SuperHyperSet. Thus the non-obvious neutrosophic 1-failed SuperHyperForcing aren’t up. The obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing is a neutrosophic SuperHyperSet excludes only two neutrosophic SuperHyperVertices are titled to neutrosophic SuperHyperNeighbors in a connected neutrosophic SuperHyperNotion SuperHyperGraph But the neutrosophic SuperHyperSets of neutrosophic SuperHyperVertices, don’t have more than two neutrosophic SuperHyperVertices outside the intended neutrosophic SuperHyperSet. Thus the non-obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing aren’t up. To sum them up, the neutrosophic SuperHyperSets of neutrosophic SuperHyperVertices, aren’t the non-obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing. Since the neutrosophic SuperHyperSets of the neutrosophic SuperHyperVertices, are the neutrosophic SuperHyperSet Ss of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex and they are neutrosophic 1-failed SuperHyperForcing. Since it’sthe maximum neutrosophic cardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex. There aren’t only more than two neutrosophic SuperHyperVertices outside the intended neutrosophic SuperHyperSets, Thus the non-obvious neutrosophic 1-failed SuperHyperForcing, aren’t up. The obvious simple type-neutrosophic SuperHyperSets of the neutrosophic 1-failed SuperHyperForcing, are a neutrosophic SuperHyperSets, doesn’t exclude only more than two neutrosophic SuperHyperVertices in a connected neutrosophic SuperHyperNotion SuperHyperGraph It’s interesting to mention that the only obvious simple type-neutrosophic SuperHyperSets of the neutrosophic 1-failed SuperHyperForcing amid those obvious simple type-neutrosophic SuperHyperSets of the neutrosophic 1-failed SuperHyperForcing, is only
-
On the Figure (2), the neutrosophic SuperHyperNotion, namely, neutrosophic 1-failed SuperHyperForcing, is up. and are some empty neutrosophic SuperHyperEdges but is an neutrosophic SuperHyperEdge. Thus in the terms of neutrosophic SuperHyperNeighbor, there’s only one neutrosophic SuperHyperEdge, namely, The neutrosophic SuperHyperVertex, is isolated means that there’s no neutrosophic SuperHyperEdge has it as an endpoint. Thus neutrosophic SuperHyperVertex, is contained in every given neutrosophic 1-failed SuperHyperForcing. All the following neutrosophic SuperHyperSets of neutrosophic SuperHyperVertices are the simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing.The neutrosophic SuperHyperSets of neutrosophic SuperHyperVertices, are the simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing. The neutrosophic SuperHyperSets of the neutrosophic SuperHyperVertices, are the maximum neutrosophic cardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex. There’re only two neutrosophic SuperHyperVertices outside the intended neutrosophic SuperHyperSet. Thus the non-obvious neutrosophic 1-failed SuperHyperForcing aren’t up. The obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing is a neutrosophic SuperHyperSet excludes only two neutrosophic SuperHyperVertices are titled to neutrosophic SuperHyperNeighbors in a connected neutrosophic SuperHyperNotion SuperHyperGraph But the neutrosophic SuperHyperSets of neutrosophic SuperHyperVertices, don’t have more than two neutrosophic SuperHyperVertices outside the intended neutrosophic SuperHyperSet. Thus the non-obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing aren’t up. To sum them up, the neutrosophic SuperHyperSets of neutrosophic SuperHyperVertices, aren’t the non-obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing. Since the neutrosophic SuperHyperSets of the neutrosophic SuperHyperVertices, are the neutrosophic SuperHyperSet Ss of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex and they are neutrosophic 1-failed SuperHyperForcing. Since it’s the maximum neutrosophic cardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex. There aren’t only more than two neutrosophic SuperHyperVertices outside the intended neutrosophic SuperHyperSets, Thus the non-obvious neutrosophic 1-failed SuperHyperForcing, aren’t up. The obvious simple type-neutrosophic SuperHyperSets of the neutrosophic 1-failed SuperHyperForcing, are a neutrosophic SuperHyperSets, doesn’t exclude only more than two neutrosophic SuperHyperVertices in a connected neutrosophic SuperHyperNotion SuperHyperGraph It’s interesting to mention that the only obvious simple type-neutrosophic SuperHyperSets of the neutrosophic 1-failed SuperHyperForcing amid those obvious simple type-neutrosophic SuperHyperSets of the neutrosophic 1-failed SuperHyperForcing, is only
- On the Figure (3), the neutrosophic SuperHyperNotion, namely, neutrosophic 1-failed SuperHyperForcing, is up. and are some empty neutrosophic SuperHyperEdges but is an neutrosophic SuperHyperEdge. Thus in the terms of neutrosophic SuperHyperNeighbor, there’s only one neutrosophic SuperHyperEdge, namely, The neutrosophic SuperHyperSets of neutrosophic SuperHyperVertices, are the simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing. The neutrosophic SuperHyperSets of the neutrosophic SuperHyperVertices, are the maximum neutrosophic cardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex. There’re only two neutrosophic SuperHyperVertices outside the intended neutrosophic SuperHyperSet. Thus the non-obvious neutrosophic 1-failed SuperHyperForcing aren’t up. The obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing is a neutrosophic SuperHyperSet excludes only two neutrosophic SuperHyperVertices are titled to neutrosophic SuperHyperNeighbors in a connected neutrosophic SuperHyperNotion SuperHyperGraph But the neutrosophic SuperHyperSets of neutrosophic SuperHyperVertices, don’t have more than two neutrosophic SuperHyperVertices outside the intended neutrosophic SuperHyperSet. Thus the non-obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing aren’t up. To sum them up, the neutrosophic SuperHyperSets of neutrosophic SuperHyperVertices, aren’t the non-obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing. Since the neutrosophic SuperHyperSets of the neutrosophic SuperHyperVertices, are the neutrosophic SuperHyperSet Ss of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex and they are neutrosophic 1-failed SuperHyperForcing. Since they’ve the maximum neutrosophic cardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex. There aren’t only more than two neutrosophic SuperHyperVertices outside the intended neutrosophic SuperHyperSets, Thus the non-obvious neutrosophic 1-failed SuperHyperForcing, aren’t up. The obvious simple type-neutrosophic SuperHyperSets of the neutrosophic 1-failed SuperHyperForcing, are the neutrosophic SuperHyperSets, don’t exclude only more than two neutrosophic SuperHyperVertices in a connected neutrosophic SuperHyperNotion SuperHyperGraph It’s interesting to mention that the only obvious simple type-neutrosophic SuperHyperSets of the neutrosophic 1-failed SuperHyperForcing amid those obvious simple type-neutrosophic SuperHyperSets of the neutrosophic 1-failed SuperHyperForcing, is only
- On the Figure (4), the neutrosophic SuperHyperNotion, namely, an neutrosophic 1-failed SuperHyperForcing, is up. There’s no empty neutrosophic SuperHyperEdge but are a loop neutrosophic SuperHyperEdge on and there are some neutrosophic SuperHyperEdges, namely, on alongside on and on The neutrosophic SuperHyperSet of neutrosophic SuperHyperVertices, is the simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing. The neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices, is the maximum neutrosophic cardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex. There’re only two neutrosophic SuperHyperVertices outside the intended neutrosophic SuperHyperSet. Thus the non-obvious neutrosophic 1-failed SuperHyperForcing isn’t up. The obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing is a neutrosophic SuperHyperSet excludes only two neutrosophic SuperHyperVertices are titled to neutrosophic SuperHyperNeighbors in a connected neutrosophic SuperHyperNotion SuperHyperGraph But the neutrosophic SuperHyperSet of neutrosophic SuperHyperVertices, doesn’t have more than two neutrosophic SuperHyperVertices outside the intended neutrosophic SuperHyperSet. Thus the non-obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing isn’t up. To sum them up, the neutrosophic SuperHyperSet of neutrosophic SuperHyperVertices, isn’t the non-obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing. Since the neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices, is the neutrosophic SuperHyperSet Ss of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex and they are neutrosophic 1-failed SuperHyperForcing. Since it’s the maximum neutrosophic cardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex. There aren’t only more than two neutrosophic SuperHyperVertices outside the intended neutrosophic SuperHyperSet, Thus the non-obvious neutrosophic 1-failed SuperHyperForcing, isn’t up. The obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing, is a neutrosophic SuperHyperSet, doesn’t exclude only more than two neutrosophic SuperHyperVertices in a connected neutrosophic SuperHyperNotion SuperHyperGraph
-
On the Figure (5), the neutrosophic SuperHyperNotion, namely, SuperHyperForcing, is up. There’s neither empty neutrosophic SuperHyperEdge nor loop neutrosophic SuperHyperEdge. The neutrosophic SuperHyperSet of neutrosophic SuperHyperVertices,is the simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing. The neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices,is the maximum neutrosophic cardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex. There’re only two neutrosophic SuperHyperVertices outside the intended neutrosophic SuperHyperSet. Thus the non-obvious neutrosophic 1-failed SuperHyperForcing isn’t up. The obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing is a neutrosophic SuperHyperSet excludes only two neutrosophic SuperHyperVertices are titled to neutrosophic SuperHyperNeighbors in a connected neutrosophic SuperHyperNotion SuperHyperGraph But the neutrosophic SuperHyperSet of neutrosophic SuperHyperVertices,doesn’t have more than two neutrosophic SuperHyperVertices outside the intended neutrosophic SuperHyperSet. Thus the non-obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing isn’t up. To sum them up, the neutrosophic SuperHyperSet of neutrosophic SuperHyperVertices,isn’t the non-obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing. Since the neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices,is the neutrosophic SuperHyperSet Ss of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex and they are neutrosophic 1-failed SuperHyperForcing. Since it’s the maximum neutrosophic cardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex. There aren’t only more than two neutrosophic SuperHyperVertices outside the intended neutrosophic SuperHyperSet,Thus the non-obvious neutrosophic 1-failed SuperHyperForcing,isn’t up. The obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing,is a neutrosophic SuperHyperSet,doesn’t exclude only more than two neutrosophic SuperHyperVertices in a connected neutrosophic SuperHyperNotion SuperHyperGraph is mentioned as the SuperHyperModel in the Figure (5).
-
On the Figure (6), the neutrosophic SuperHyperNotion, namely, neutrosophic 1-failed SuperHyperForcing, is up. There’s neither empty neutrosophic SuperHyperEdge nor loop neutrosophic SuperHyperEdge. The neutrosophic SuperHyperSet of neutrosophic SuperHyperVertices,is the simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing. The neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices,is the maximum neutrosophic cardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex. There’re only two neutrosophic SuperHyperVertices outside the intended neutrosophic SuperHyperSet. Thus the non-obvious neutrosophic 1-failed SuperHyperForcing isn’t up. The obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing is a neutrosophic SuperHyperSet excludes only two neutrosophic SuperHyperVertices are titled to neutrosophic SuperHyperNeighbors in a connected neutrosophic SuperHyperNotion SuperHyperGraph But the neutrosophic SuperHyperSet of neutrosophic SuperHyperVertices,doesn’t have more than two neutrosophic SuperHyperVertices outside the intended neutrosophic SuperHyperSet. Thus the non-obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing isn’t up. To sum them up, the neutrosophic SuperHyperSet of neutrosophic SuperHyperVertices,isn’t the non-obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing. Since the neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices,is the neutrosophic SuperHyperSet Ss of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex and they are neutrosophic 1-failed SuperHyperForcing. Since it’s the maximum neutrosophic cardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex. There aren’t only more than two neutrosophic SuperHyperVertices outside the intended neutrosophic SuperHyperSet,Thus the non-obvious neutrosophic 1-failed SuperHyperForcing,isn’t up. The obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing,is a neutrosophic SuperHyperSet,doesn’t exclude only more than two neutrosophic SuperHyperVertices in a connected neutrosophic SuperHyperNotion SuperHyperGraph with a illustrated SuperHyperModeling of the Figure (6).
-
On the Figure (7), the neutrosophic SuperHyperNotion, namely, neutrosophic 1-failed SuperHyperForcing, is up. There’s neither empty neutrosophic SuperHyperEdge nor loop neutrosophic SuperHyperEdge. The neutrosophic SuperHyperSet of neutrosophic SuperHyperVertices,is the simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing. The neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices,is the maximum neutrosophic cardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex. There’re only two neutrosophic SuperHyperVertices outside the intended neutrosophic SuperHyperSet. Thus the non-obvious neutrosophic 1-failed SuperHyperForcing isn’t up. The obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing is a neutrosophic SuperHyperSet excludes only two neutrosophic SuperHyperVertices are titled to neutrosophic SuperHyperNeighbors in a connected neutrosophic SuperHyperNotion SuperHyperGraph But the neutrosophic SuperHyperSet of neutrosophic SuperHyperVertices,doesn’t have more than two neutrosophic SuperHyperVertices outside the intended neutrosophic SuperHyperSet. Thus the non-obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing isn’t up. To sum them up, the neutrosophic SuperHyperSet of neutrosophic SuperHyperVertices,isn’t the non-obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing. Since the neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices,is the neutrosophic SuperHyperSet Ss of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex and they are neutrosophic 1-failed SuperHyperForcing. Since it’s the maximum neutrosophic cardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex. There aren’t only more than two neutrosophic SuperHyperVertices outside the intended neutrosophic SuperHyperSet,Thus the non-obvious neutrosophic 1-failed SuperHyperForcing,isn’t up. The obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing,is a neutrosophic SuperHyperSet,doesn’t exclude only more than two neutrosophic SuperHyperVertices in a connected neutrosophic SuperHyperNotion SuperHyperGraph of depicted SuperHyperModel as the Figure (7).
-
On the Figure (8), the neutrosophic SuperHyperNotion, namely, neutrosophic 1-failed SuperHyperForcing, is up. There’s neither empty neutrosophic SuperHyperEdge nor loop neutrosophic SuperHyperEdge. The neutrosophic SuperHyperSet of neutrosophic SuperHyperVertices,is the simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing. The neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices,is the maximum neutrosophic cardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex. There’re only two neutrosophic SuperHyperVertices outside the intended neutrosophic SuperHyperSet. Thus the non-obvious neutrosophic 1-failed SuperHyperForcing isn’t up. The obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing is a neutrosophic SuperHyperSet excludes only two neutrosophic SuperHyperVertices are titled to neutrosophic SuperHyperNeighbors in a connected neutrosophic SuperHyperNotion SuperHyperGraph But the neutrosophic SuperHyperSet of neutrosophic SuperHyperVertices,doesn’t have more than two neutrosophic SuperHyperVertices outside the intended neutrosophic SuperHyperSet. Thus the non-obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing isn’t up. To sum them up, the neutrosophic SuperHyperSet of neutrosophic SuperHyperVertices,isn’t the non-obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing. Since the neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices,is the neutrosophic SuperHyperSet Ss of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex and they are neutrosophic 1-failed SuperHyperForcing. Since it’s the maximum neutrosophic cardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex. There aren’t only more than two neutrosophic SuperHyperVertices outside the intended neutrosophic SuperHyperSet,Thus the non-obvious neutrosophic 1-failed SuperHyperForcing,isn’t up. The obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing,is a neutrosophic SuperHyperSet,doesn’t exclude only more than two neutrosophic SuperHyperVertices in a connected neutrosophic SuperHyperNotion SuperHyperGraph of dense SuperHyperModel as the Figure (8).
-
On the Figure (9), the neutrosophic SuperHyperNotion, namely, neutrosophic 1-failed SuperHyperForcing, is up. There’s neither empty neutrosophic SuperHyperEdge nor loop neutrosophic SuperHyperEdge. The neutrosophic SuperHyperSet of neutrosophic SuperHyperVertices,is the simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing. The neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices,is the maximum neutrosophic cardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex. There’re only two neutrosophic SuperHyperVertices outside the intended neutrosophic SuperHyperSet. Thus the non-obvious neutrosophic 1-failed SuperHyperForcing isn’t up. The obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing is a neutrosophic SuperHyperSet excludes only two neutrosophic SuperHyperVertices are titled to neutrosophic SuperHyperNeighbors in a connected neutrosophic SuperHyperNotion SuperHyperGraph But the neutrosophic SuperHyperSet of neutrosophic SuperHyperVertices,doesn’t have more than two neutrosophic SuperHyperVertices outside the intended neutrosophic SuperHyperSet. Thus the non-obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing isn’t up. To sum them up, the neutrosophic SuperHyperSet of neutrosophic SuperHyperVertices,isn’t the non-obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing. Since the neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices,is the neutrosophic SuperHyperSet Ss of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex and they are neutrosophic 1-failed SuperHyperForcing. Since it’s the maximum neutrosophic cardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex. There aren’t only more than two neutrosophic SuperHyperVertices outside the intended neutrosophic SuperHyperSet,Thus the non-obvious neutrosophic 1-failed SuperHyperForcing,isn’t up. The obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing,is a neutrosophic SuperHyperSet,doesn’t exclude only more than two neutrosophic SuperHyperVertices in a connected neutrosophic SuperHyperNotion SuperHyperGraph with a messy SuperHyperModeling of the Figure (9).
-
On the Figure (10), the neutrosophic SuperHyperNotion, namely, neutrosophic 1-failed SuperHyperForcing, is up. There’s neither empty neutrosophic SuperHyperEdge nor loop neutrosophic SuperHyperEdge. The neutrosophic SuperHyperSet of neutrosophic SuperHyperVertices,is the simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing. The neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices,is the maximum neutrosophic cardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex. There’re only two neutrosophic SuperHyperVertices outside the intended neutrosophic SuperHyperSet. Thus the non-obvious neutrosophic 1-failed SuperHyperForcing isn’t up. The obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing is a neutrosophic SuperHyperSet excludes only two neutrosophic SuperHyperVertices are titled to neutrosophic SuperHyperNeighbors in a connected neutrosophic SuperHyperNotion SuperHyperGraph But the neutrosophic SuperHyperSet of neutrosophic SuperHyperVertices,doesn’t have more than two neutrosophic SuperHyperVertices outside the intended neutrosophic SuperHyperSet. Thus the non-obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing isn’t up. To sum them up, the neutrosophic SuperHyperSet of neutrosophic SuperHyperVertices,isn’t the non-obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing. Since the neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices,is the neutrosophic SuperHyperSet Ss of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex and they are neutrosophic 1-failed SuperHyperForcing. Since it’s the maximum neutrosophic cardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex. There aren’t only more than two neutrosophic SuperHyperVertices outside the intended neutrosophic SuperHyperSet,Thus the non-obvious neutrosophic 1-failed SuperHyperForcing,isn’t up. The obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing,is a neutrosophic SuperHyperSet,doesn’t exclude only more than two neutrosophic SuperHyperVertices in a connected neutrosophic SuperHyperNotion SuperHyperGraph of highly-embedding-connected SuperHyperModel as the Figure (10).
- On the Figure (11), the neutrosophic SuperHyperNotion, namely, neutrosophic 1-failed SuperHyperForcing, is up. There’s neither empty neutrosophic SuperHyperEdge nor loop neutrosophic SuperHyperEdge. The neutrosophic SuperHyperSet of neutrosophic SuperHyperVertices, is the simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing. The neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices, is the maximum neutrosophic cardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex. There’re only two neutrosophic SuperHyperVertices outside the intended neutrosophic SuperHyperSet. Thus the non-obvious neutrosophic 1-failed SuperHyperForcing isn’t up. The obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing is a neutrosophic SuperHyperSet excludes only two neutrosophic SuperHyperVertices are titled to neutrosophic SuperHyperNeighbors in a connected neutrosophic SuperHyperNotion SuperHyperGraph But the neutrosophic SuperHyperSet of neutrosophic SuperHyperVertices, doesn’t have more than two neutrosophic SuperHyperVertices outside the intended neutrosophic SuperHyperSet. Thus the non-obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing isn’t up. To sum them up, the neutrosophic SuperHyperSet of neutrosophic SuperHyperVertices, isn’t the non-obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing. Since the neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices, is the neutrosophic SuperHyperSet Ss of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex and they are neutrosophic 1-failed SuperHyperForcing. Since it’sthe maximum neutrosophic cardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex. There aren’t only more than two neutrosophic SuperHyperVertices outside the intended neutrosophic SuperHyperSet, Thus the non-obvious neutrosophic 1-failed SuperHyperForcing, isn’t up. The obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing, is a neutrosophic SuperHyperSet, doesn’t exclude only more than two neutrosophic SuperHyperVertices in a connected neutrosophic SuperHyperNotion SuperHyperGraph
- On the Figure (12), the neutrosophic SuperHyperNotion, namely, neutrosophic 1-failed SuperHyperForcing, is up. There’s neither empty neutrosophic SuperHyperEdge nor loop neutrosophic SuperHyperEdge. The neutrosophic SuperHyperSet of neutrosophic SuperHyperVertices, is the simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing. The neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices, is the maximum neutrosophic cardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex. There’re only two neutrosophic SuperHyperVertices outside the intended neutrosophic SuperHyperSet. Thus the non-obvious neutrosophic 1-failed SuperHyperForcing isn’t up. The obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing is a neutrosophic SuperHyperSet excludes only two neutrosophic SuperHyperVertices are titled to neutrosophic SuperHyperNeighbors in a connected neutrosophic SuperHyperNotion SuperHyperGraph But the neutrosophic SuperHyperSet of neutrosophic SuperHyperVertices, doesn’t have more than two neutrosophic SuperHyperVertices outside the intended neutrosophic SuperHyperSet. Thus the non-obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing isn’t up. To sum them up, the neutrosophic SuperHyperSet of neutrosophic SuperHyperVertices, isn’t the non-obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing. Since the neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices, is the neutrosophic SuperHyperSet Ss of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex and they are neutrosophic 1-failed SuperHyperForcing. Since it’s the maximum neutrosophic cardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex. There aren’t only more than two neutrosophic SuperHyperVertices outside the intended neutrosophic SuperHyperSet, Thus the non-obvious neutrosophic 1-failed SuperHyperForcing, isn’t up. The obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing, is a neutrosophic SuperHyperSet, doesn’t exclude only more than two neutrosophic SuperHyperVertices in a connected neutrosophic SuperHyperNotion SuperHyperGraph in highly-multiple-connected-style SuperHyperModel On the Figure (12).
- On the Figure (13), the neutrosophic SuperHyperNotion, namely, neutrosophic 1-failed SuperHyperForcing, is up. There’s neither empty neutrosophic SuperHyperEdge nor loop neutrosophic SuperHyperEdge. The neutrosophic SuperHyperSet of neutrosophic SuperHyperVertices, is the simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing. The neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices, is the maximum neutrosophic cardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex. There’re only two neutrosophic SuperHyperVertices outside the intended neutrosophic SuperHyperSet. Thus the non-obvious neutrosophic 1-failed SuperHyperForcing isn’t up. The obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing is a neutrosophic SuperHyperSet excludes only two neutrosophic SuperHyperVertices are titled to neutrosophic SuperHyperNeighbors in a connected neutrosophic SuperHyperNotion SuperHyperGraph But the neutrosophic SuperHyperSet of neutrosophic SuperHyperVertices, doesn’t have more than two neutrosophic SuperHyperVertices outside the intended neutrosophic SuperHyperSet. Thus the non-obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing isn’t up. To sum them up, the neutrosophic SuperHyperSet of neutrosophic SuperHyperVertices, isn’t the non-obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing. Since the neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices, is the neutrosophic SuperHyperSet Ss of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex and they are neutrosophic 1-failed SuperHyperForcing. Since it’s the maximum neutrosophic cardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex. There aren’t only more than two neutrosophic SuperHyperVertices outside the intended neutrosophic SuperHyperSet, Thus the non-obvious neutrosophic 1-failed SuperHyperForcing, isn’t up. The obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing, is a neutrosophic SuperHyperSet, doesn’t exclude only more than two neutrosophic SuperHyperVertices in a connected neutrosophic SuperHyperNotion SuperHyperGraph
- On the Figure (14), the neutrosophic SuperHyperNotion, namely, neutrosophic 1-failed SuperHyperForcing, is up. There’s neither empty neutrosophic SuperHyperEdge nor loop neutrosophic SuperHyperEdge. The neutrosophic SuperHyperSet of neutrosophic SuperHyperVertices, is the simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing. The neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices, is the maximum neutrosophic cardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex. There’re only two neutrosophic SuperHyperVertices outside the intended neutrosophic SuperHyperSet. Thus the non-obvious neutrosophic 1-failed SuperHyperForcing isn’t up. The obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing is a neutrosophic SuperHyperSet excludes only two neutrosophic SuperHyperVertices are titled to neutrosophic SuperHyperNeighbors in a connected neutrosophic SuperHyperNotion SuperHyperGraph But the neutrosophic SuperHyperSet of neutrosophic SuperHyperVertices, doesn’t have more than two neutrosophic SuperHyperVertices outside the intended neutrosophic SuperHyperSet. Thus the non-obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing isn’t up. To sum them up, the neutrosophic SuperHyperSet of neutrosophic SuperHyperVertices, isn’t the non-obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing. Since the neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices, is the neutrosophic SuperHyperSet Ss of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex and they are neutrosophic 1-failed SuperHyperForcing. Since it’s the maximum neutrosophic cardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex. There aren’t only more than two neutrosophic SuperHyperVertices outside the intended neutrosophic SuperHyperSet, Thus the non-obvious neutrosophic 1-failed SuperHyperForcing, isn’t up. The obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing, is a neutrosophic SuperHyperSet, doesn’t exclude only more than two neutrosophic SuperHyperVertices in a connected neutrosophic SuperHyperNotion SuperHyperGraph
- On the Figure (15), the neutrosophic SuperHyperNotion, namely, neutrosophic 1-failed SuperHyperForcing, is up. There’s neither empty neutrosophic SuperHyperEdge nor loop neutrosophic SuperHyperEdge. The neutrosophic SuperHyperSet of neutrosophic SuperHyperVertices, is the simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing. The neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices, is the maximum neutrosophic cardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex. There’re only two neutrosophic SuperHyperVertices outside the intended neutrosophic SuperHyperSet. Thus the non-obvious neutrosophic 1-failed SuperHyperForcing isn’t up. The obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing is a neutrosophic SuperHyperSet excludes only two neutrosophic SuperHyperVertices are titled to neutrosophic SuperHyperNeighbors in a connected neutrosophic SuperHyperNotion SuperHyperGraph But the neutrosophic SuperHyperSet of neutrosophic SuperHyperVertices, doesn’t have more than two neutrosophic SuperHyperVertices outside the intended neutrosophic SuperHyperSet. Thus the non-obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing isn’t up. To sum them up, the neutrosophic SuperHyperSet of neutrosophic SuperHyperVertices, isn’t the non-obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing. Since the neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices, is the neutrosophic SuperHyperSet Ss of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex and they are neutrosophic 1-failed SuperHyperForcing. Since it’s the maximum neutrosophic cardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex. There aren’t only more than two neutrosophic SuperHyperVertices outside the intended neutrosophic SuperHyperSet, Thus the non-obvious neutrosophic 1-failed SuperHyperForcing, isn’t up. The obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing, is a neutrosophic SuperHyperSet, doesn’t exclude only more than two neutrosophic SuperHyperVertices in a connected neutrosophic SuperHyperNotion SuperHyperGraph as Linearly-Connected SuperHyperModel On the Figure (15).
-
On the Figure (16), the neutrosophic SuperHyperNotion, namely, neutrosophic 1-failed SuperHyperForcing, is up. There’s neither empty neutrosophic SuperHyperEdge nor loop neutrosophic SuperHyperEdge. The neutrosophic SuperHyperSet of neutrosophic SuperHyperVertices,is the simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing. The neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices,is the maximum neutrosophic cardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex. There’re only two neutrosophic SuperHyperVertices outside the intended neutrosophic SuperHyperSet. Thus the non-obvious neutrosophic 1-failed SuperHyperForcing isn’t up. The obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing is a neutrosophic SuperHyperSet excludes only two neutrosophic SuperHyperVertices are titled to neutrosophic SuperHyperNeighbors in a connected neutrosophic SuperHyperNotion SuperHyperGraph But the neutrosophic SuperHyperSet of neutrosophic SuperHyperVertices,doesn’t have more than two neutrosophic SuperHyperVertices outside the intended neutrosophic SuperHyperSet. Thus the non-obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing isn’t up. To sum them up, the neutrosophic SuperHyperSet of neutrosophic SuperHyperVertices,isn’t the non-obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing. Since the neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices,is the neutrosophic SuperHyperSet Ss of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex and they are neutrosophic 1-failed SuperHyperForcing. Since it’s the maximum neutrosophic cardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex. There aren’t only more than two neutrosophic SuperHyperVertices outside the intended neutrosophic SuperHyperSet,Thus the non-obvious neutrosophic 1-failed SuperHyperForcing,isn’t up. The obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing,is a neutrosophic SuperHyperSet,doesn’t exclude only more than two neutrosophic SuperHyperVertices in a connected neutrosophic SuperHyperNotion SuperHyperGraph
-
On the Figure (17), the neutrosophic SuperHyperNotion, namely, neutrosophic 1-failed SuperHyperForcing, is up. There’s neither empty neutrosophic SuperHyperEdge nor loop neutrosophic SuperHyperEdge. The neutrosophic SuperHyperSet of neutrosophic SuperHyperVertices,is the simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing. The neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices,is the maximum neutrosophic cardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex. There’re only two neutrosophic SuperHyperVertices outside the intended neutrosophic SuperHyperSet. Thus the non-obvious neutrosophic 1-failed SuperHyperForcing isn’t up. The obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing is a neutrosophic SuperHyperSet excludes only two neutrosophic SuperHyperVertices are titled to neutrosophic SuperHyperNeighbors in a connected neutrosophic SuperHyperNotion SuperHyperGraph But the neutrosophic SuperHyperSet of neutrosophic SuperHyperVertices,doesn’t have more than two neutrosophic SuperHyperVertices outside the intended neutrosophic SuperHyperSet. Thus the non-obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing isn’t up. To sum them up, the neutrosophic SuperHyperSet of neutrosophic SuperHyperVertices,isn’t the non-obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing. Since the neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices,is the neutrosophic SuperHyperSet Ss of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex and they are neutrosophic 1-failed SuperHyperForcing. Since it’s the maximum neutrosophic cardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex. There aren’t only more than two neutrosophic SuperHyperVertices outside the intended neutrosophic SuperHyperSet,Thus the non-obvious neutrosophic 1-failed SuperHyperForcing,isn’t up. The obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing,is a neutrosophic SuperHyperSet,doesn’t exclude only more than two neutrosophic SuperHyperVertices in a connected neutrosophic SuperHyperNotion SuperHyperGraph as Lnearly-over-packed SuperHyperModel is featured On the Figure (17).
- On the Figure (18), the neutrosophic SuperHyperNotion, namely, neutrosophic 1-failed SuperHyperForcing, is up. There’s neither empty neutrosophic SuperHyperEdge nor loop neutrosophic SuperHyperEdge. The neutrosophic SuperHyperSet of neutrosophic SuperHyperVertices, is the simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing. The neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices, is the maximum neutrosophic cardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex. There’re only two neutrosophic SuperHyperVertices outside the intended neutrosophic SuperHyperSet. Thus the non-obvious neutrosophic 1-failed SuperHyperForcing isn’t up. The obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing is a neutrosophic SuperHyperSet excludes only two neutrosophic SuperHyperVertices are titled to neutrosophic SuperHyperNeighbors in a connected neutrosophic SuperHyperNotion SuperHyperGraph But the neutrosophic SuperHyperSet of neutrosophic SuperHyperVertices, doesn’t have more than two neutrosophic SuperHyperVertices outside the intended neutrosophic SuperHyperSet. Thus the non-obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing isn’t up. To sum them up, the neutrosophic SuperHyperSet of neutrosophic SuperHyperVertices, isn’t the non-obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing. Since the neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices, is the neutrosophic SuperHyperSet Ss of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex and they are neutrosophic 1-failed SuperHyperForcing. Since it’s the maximum neutrosophic cardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex. There aren’t only more than two neutrosophic SuperHyperVertices outside the intended neutrosophic SuperHyperSet, Thus the non-obvious neutrosophic 1-failed SuperHyperForcing, isn’t up. The obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing, is a neutrosophic SuperHyperSet, doesn’t exclude only more than two neutrosophic SuperHyperVertices in a connected neutrosophic SuperHyperNotion SuperHyperGraph
-
On the Figure (19), the neutrosophic SuperHyperNotion, namely, neutrosophic 1-failed SuperHyperForcing, is up. There’s neither empty neutrosophic SuperHyperEdge nor loop neutrosophic SuperHyperEdge. The neutrosophic SuperHyperSet of neutrosophic SuperHyperVertices,is the simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing. The neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices,is the maximum neutrosophic cardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex. There’re only two neutrosophic SuperHyperVertices outside the intended neutrosophic SuperHyperSet. Thus the non-obvious neutrosophic 1-failed SuperHyperForcing isn’t up. The obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing is a neutrosophic SuperHyperSet excludes only two neutrosophic SuperHyperVertices are titled to neutrosophic SuperHyperNeighbors in a connected neutrosophic SuperHyperNotion SuperHyperGraph But the neutrosophic SuperHyperSet of neutrosophic SuperHyperVertices,doesn’t have more than two neutrosophic SuperHyperVertices outside the intended neutrosophic SuperHyperSet. Thus the non-obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing isn’t up. To sum them up, the neutrosophic SuperHyperSet of neutrosophic SuperHyperVertices,isn’t the non-obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing. Since the neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices,is the neutrosophic SuperHyperSet Ss of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex and they are neutrosophic 1-failed SuperHyperForcing. Since it’s the maximum neutrosophic cardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex. There aren’t only more than two neutrosophic SuperHyperVertices outside the intended neutrosophic SuperHyperSet,Thus the non-obvious neutrosophic 1-failed SuperHyperForcing,isn’t up. The obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing,is a neutrosophic SuperHyperSet,doesn’t exclude only more than two neutrosophic SuperHyperVertices in a connected neutrosophic SuperHyperNotion SuperHyperGraph
-
On the Figure (20), the neutrosophic SuperHyperNotion, namely, neutrosophic 1-failed SuperHyperForcing, is up. There’s neither empty neutrosophic SuperHyperEdge nor loop neutrosophic SuperHyperEdge. The neutrosophic SuperHyperSet of neutrosophic SuperHyperVertices,is the simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing. The neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices,is the maximum neutrosophic cardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex. There’re only two neutrosophic SuperHyperVertices outside the intended neutrosophic SuperHyperSet. Thus the non-obvious neutrosophic 1-failed SuperHyperForcing isn’t up. The obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing is a neutrosophic SuperHyperSet excludes only two neutrosophic SuperHyperVertices are titled to neutrosophic SuperHyperNeighbors in a connected neutrosophic SuperHyperNotion SuperHyperGraph But the neutrosophic SuperHyperSet of neutrosophic SuperHyperVertices,doesn’t have more than two neutrosophic SuperHyperVertices outside the intended neutrosophic SuperHyperSet. Thus the non-obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing isn’t up. To sum them up, the neutrosophic SuperHyperSet of neutrosophic SuperHyperVertices,isn’t the non-obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing. Since the neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices,is the neutrosophic SuperHyperSet Ss of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex and they are neutrosophic 1-failed SuperHyperForcing. Since it’s the maximum neutrosophic cardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex. There aren’t only more than two neutrosophic SuperHyperVertices outside the intended neutrosophic SuperHyperSet,Thus the non-obvious neutrosophic 1-failed SuperHyperForcing,isn’t up. The obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing,is a neutrosophic SuperHyperSet,doesn’t exclude only more than two neutrosophic SuperHyperVertices in a connected neutrosophic SuperHyperNotion SuperHyperGraph
Figure 1.
The neutrosophic SuperHyperGraphs Associated to the Notions of neutrosophic 1-failed SuperHyperForcing in the Examples () and (23).
Figure 1.
The neutrosophic SuperHyperGraphs Associated to the Notions of neutrosophic 1-failed SuperHyperForcing in the Examples () and (23).
Figure 2.
The neutrosophic SuperHyperGraphs Associated to the Notions of neutrosophic 1-failed SuperHyperForcing in the Examples () and (23).
Figure 2.
The neutrosophic SuperHyperGraphs Associated to the Notions of neutrosophic 1-failed SuperHyperForcing in the Examples () and (23).
Figure 3.
The neutrosophic SuperHyperGraphs Associated to the Notions of neutrosophic 1-failed SuperHyperForcing in the Examples () and (23).
Figure 3.
The neutrosophic SuperHyperGraphs Associated to the Notions of neutrosophic 1-failed SuperHyperForcing in the Examples () and (23).
Figure 4.
The neutrosophic SuperHyperGraphs Associated to the Notions of neutrosophic 1-failed SuperHyperForcing in the Examples () and (23).
Figure 4.
The neutrosophic SuperHyperGraphs Associated to the Notions of neutrosophic 1-failed SuperHyperForcing in the Examples () and (23).
Figure 5.
The neutrosophic SuperHyperGraphs Associated to the Notions of neutrosophic 1-failed SuperHyperForcing in the Examples () and (23).
Figure 5.
The neutrosophic SuperHyperGraphs Associated to the Notions of neutrosophic 1-failed SuperHyperForcing in the Examples () and (23).
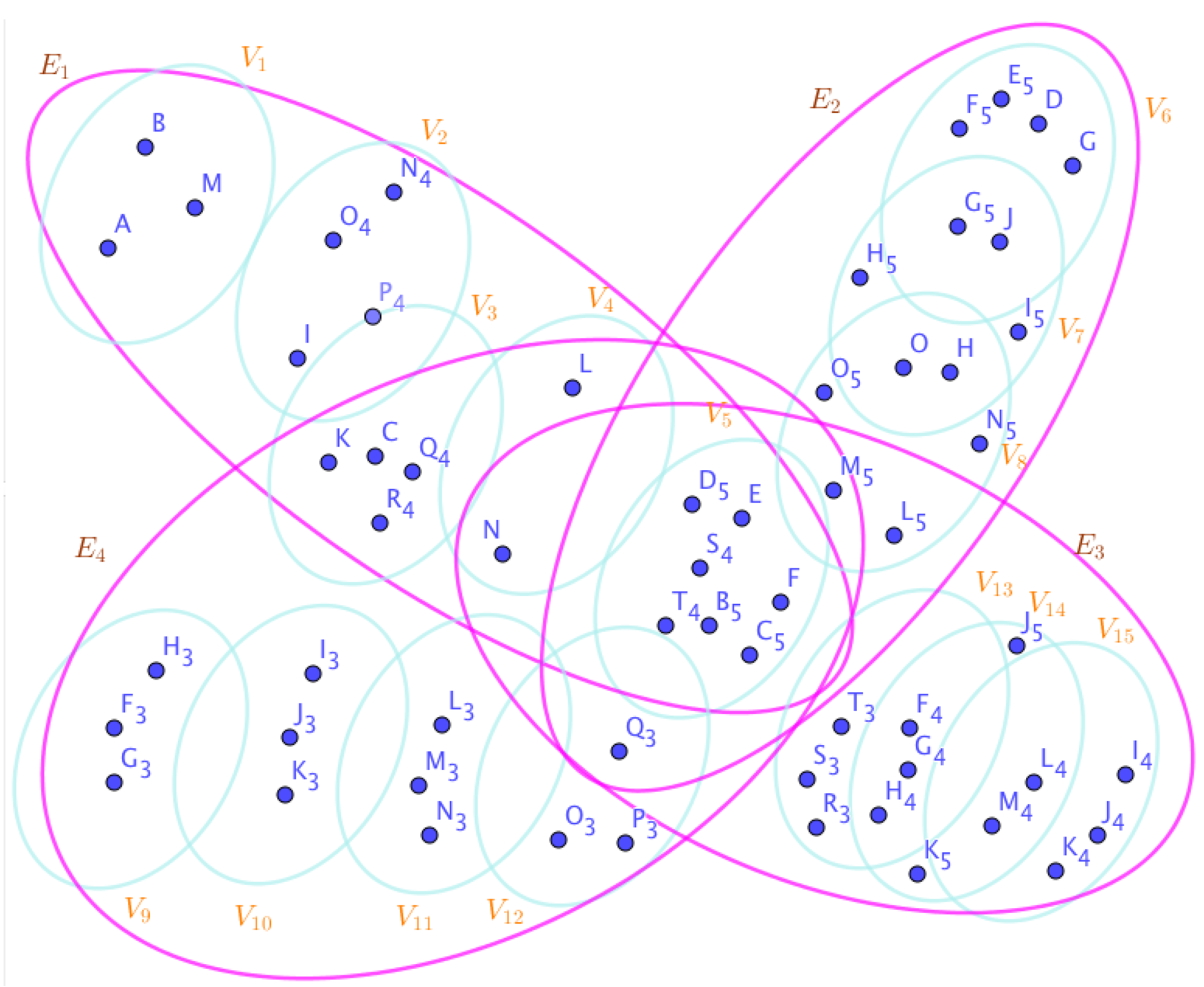
Figure 6.
The neutrosophic SuperHyperGraphs Associated to the Notions of neutrosophic 1-failed SuperHyperForcing in the Examples () and (23).
Figure 6.
The neutrosophic SuperHyperGraphs Associated to the Notions of neutrosophic 1-failed SuperHyperForcing in the Examples () and (23).

Figure 7.
The neutrosophic SuperHyperGraphs Associated to the Notions of neutrosophic 1-failed SuperHyperForcing in the Examples () and (23).
Figure 7.
The neutrosophic SuperHyperGraphs Associated to the Notions of neutrosophic 1-failed SuperHyperForcing in the Examples () and (23).
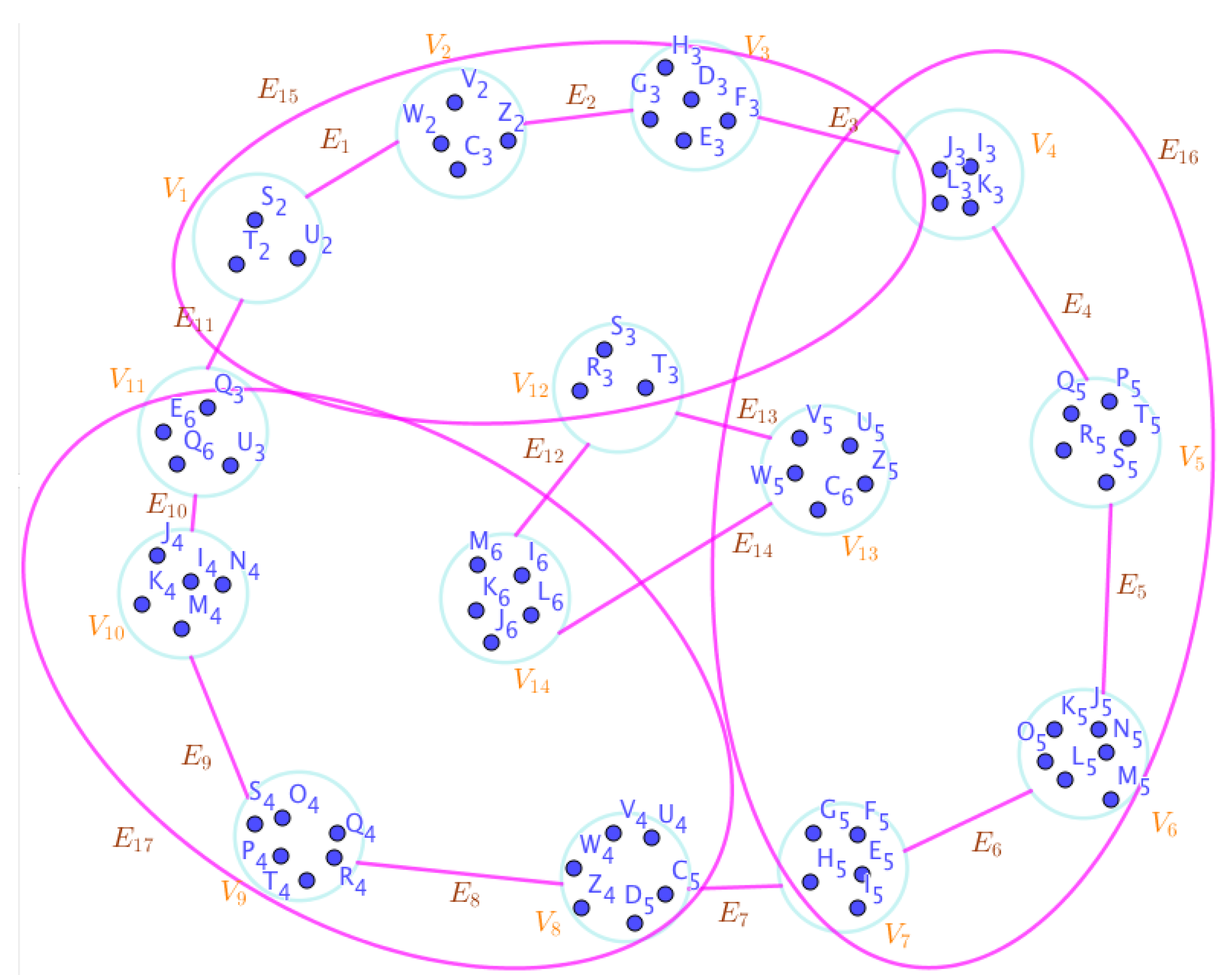
Figure 8.
The neutrosophic SuperHyperGraphs Associated to the Notions of neutrosophic 1-failed SuperHyperForcing in the Examples () and (23).
Figure 8.
The neutrosophic SuperHyperGraphs Associated to the Notions of neutrosophic 1-failed SuperHyperForcing in the Examples () and (23).

Figure 9.
The neutrosophic SuperHyperGraphs Associated to the Notions of neutrosophic 1-failed SuperHyperForcing in the Examples () and (23).
Figure 9.
The neutrosophic SuperHyperGraphs Associated to the Notions of neutrosophic 1-failed SuperHyperForcing in the Examples () and (23).
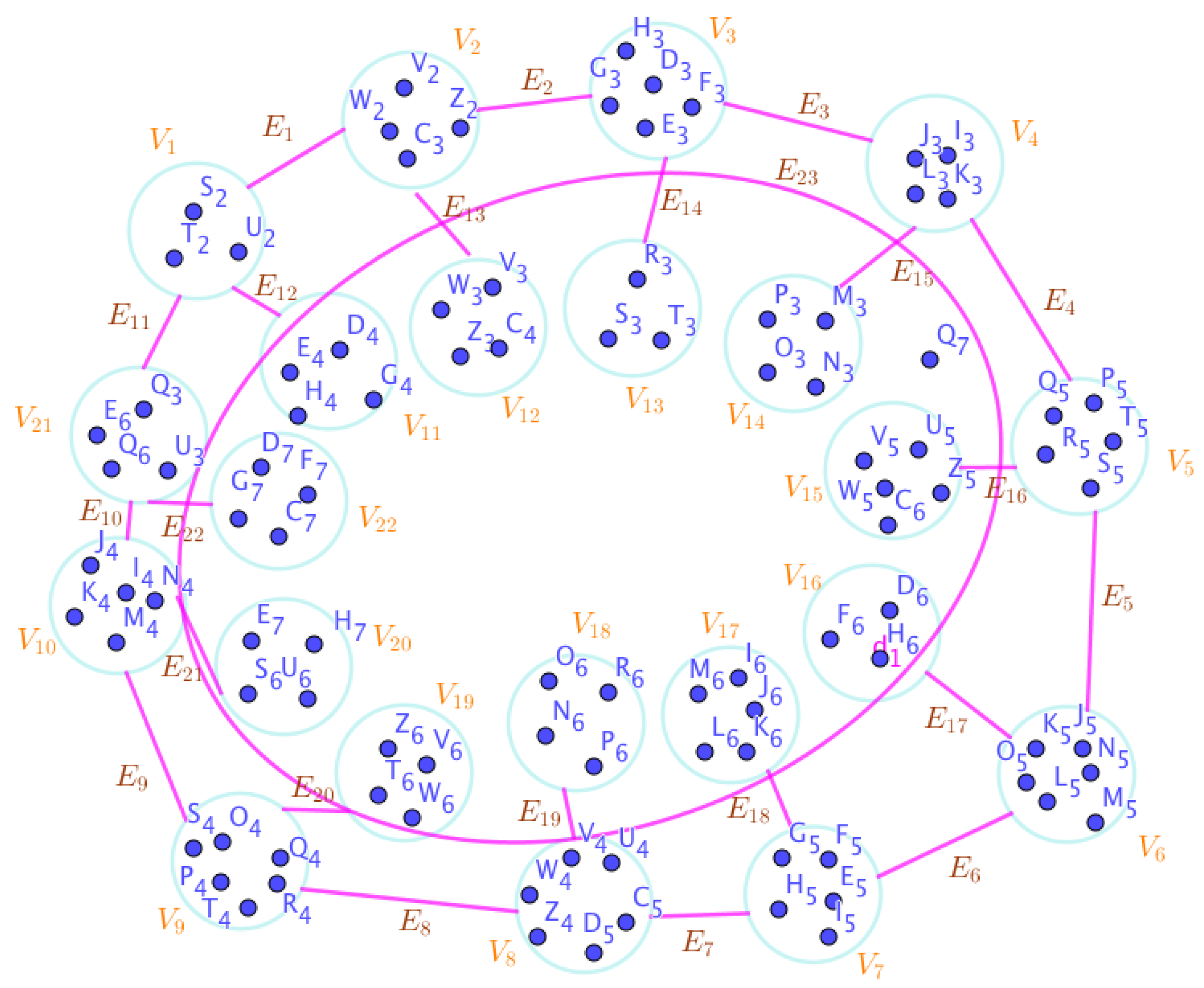
Figure 10.
The neutrosophic SuperHyperGraphs Associated to the Notions of neutrosophic 1-failed SuperHyperForcing in the Examples () and (23).
Figure 10.
The neutrosophic SuperHyperGraphs Associated to the Notions of neutrosophic 1-failed SuperHyperForcing in the Examples () and (23).
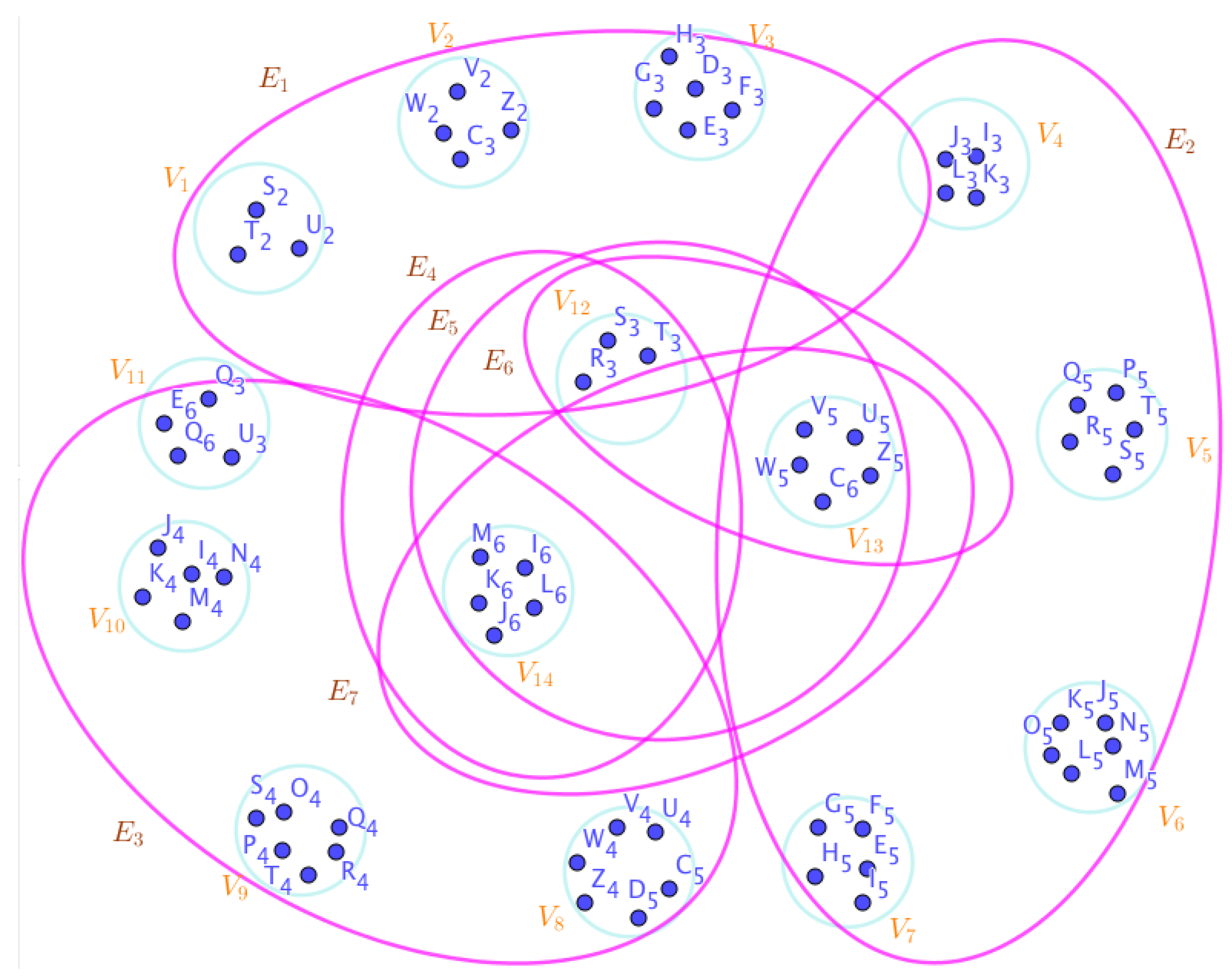
Figure 11.
The neutrosophic SuperHyperGraphs Associated to the Notions of neutrosophic 1-failed SuperHyperForcing in the Examples () and (23).
Figure 11.
The neutrosophic SuperHyperGraphs Associated to the Notions of neutrosophic 1-failed SuperHyperForcing in the Examples () and (23).
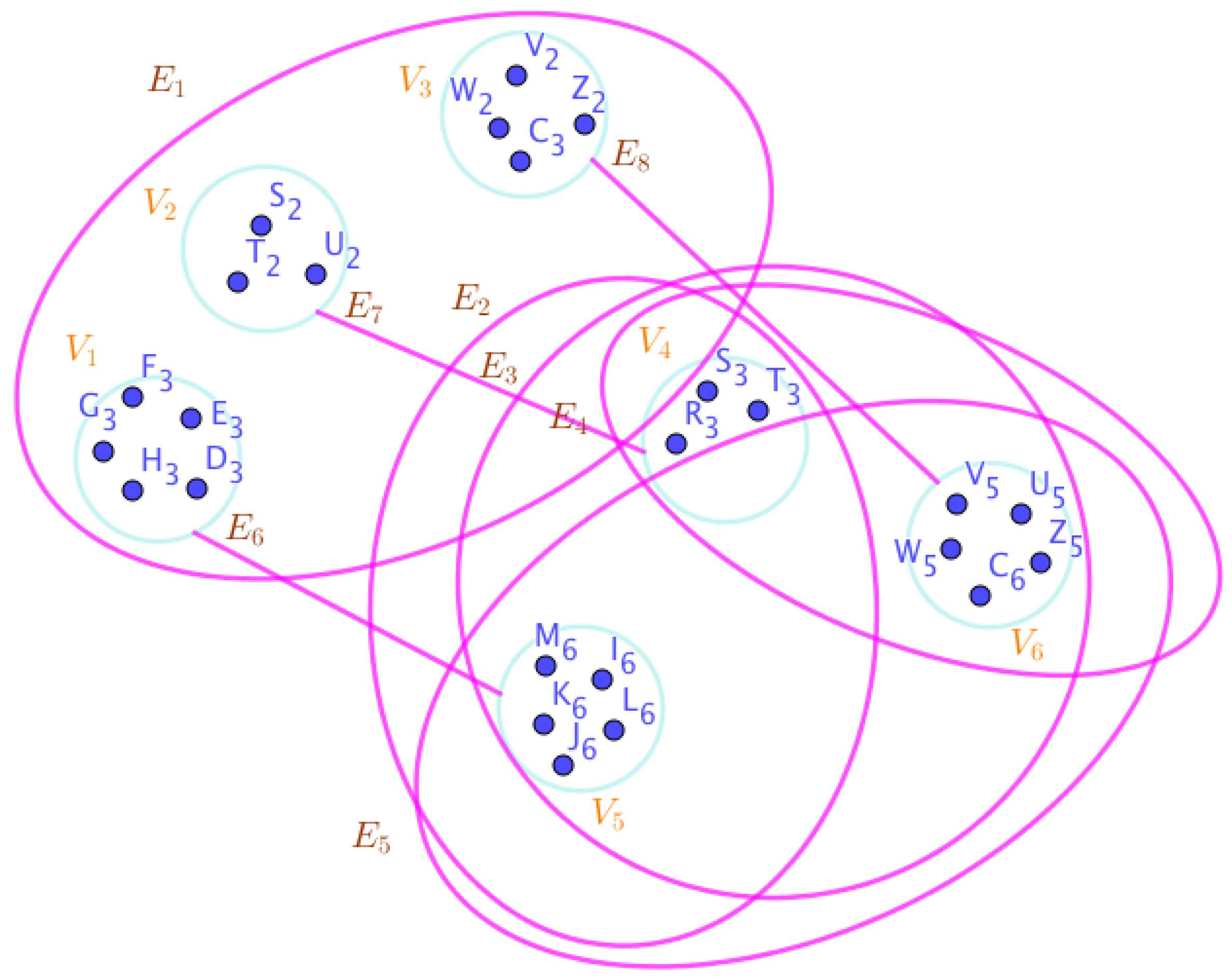
Figure 12.
The neutrosophic SuperHyperGraphs Associated to the Notions of neutrosophic 1-failed SuperHyperForcing in the Examples () and (23).
Figure 12.
The neutrosophic SuperHyperGraphs Associated to the Notions of neutrosophic 1-failed SuperHyperForcing in the Examples () and (23).

Figure 13.
The neutrosophic SuperHyperGraphs Associated to the Notions of neutrosophic 1-failed SuperHyperForcing in the Examples () and (23).
Figure 13.
The neutrosophic SuperHyperGraphs Associated to the Notions of neutrosophic 1-failed SuperHyperForcing in the Examples () and (23).
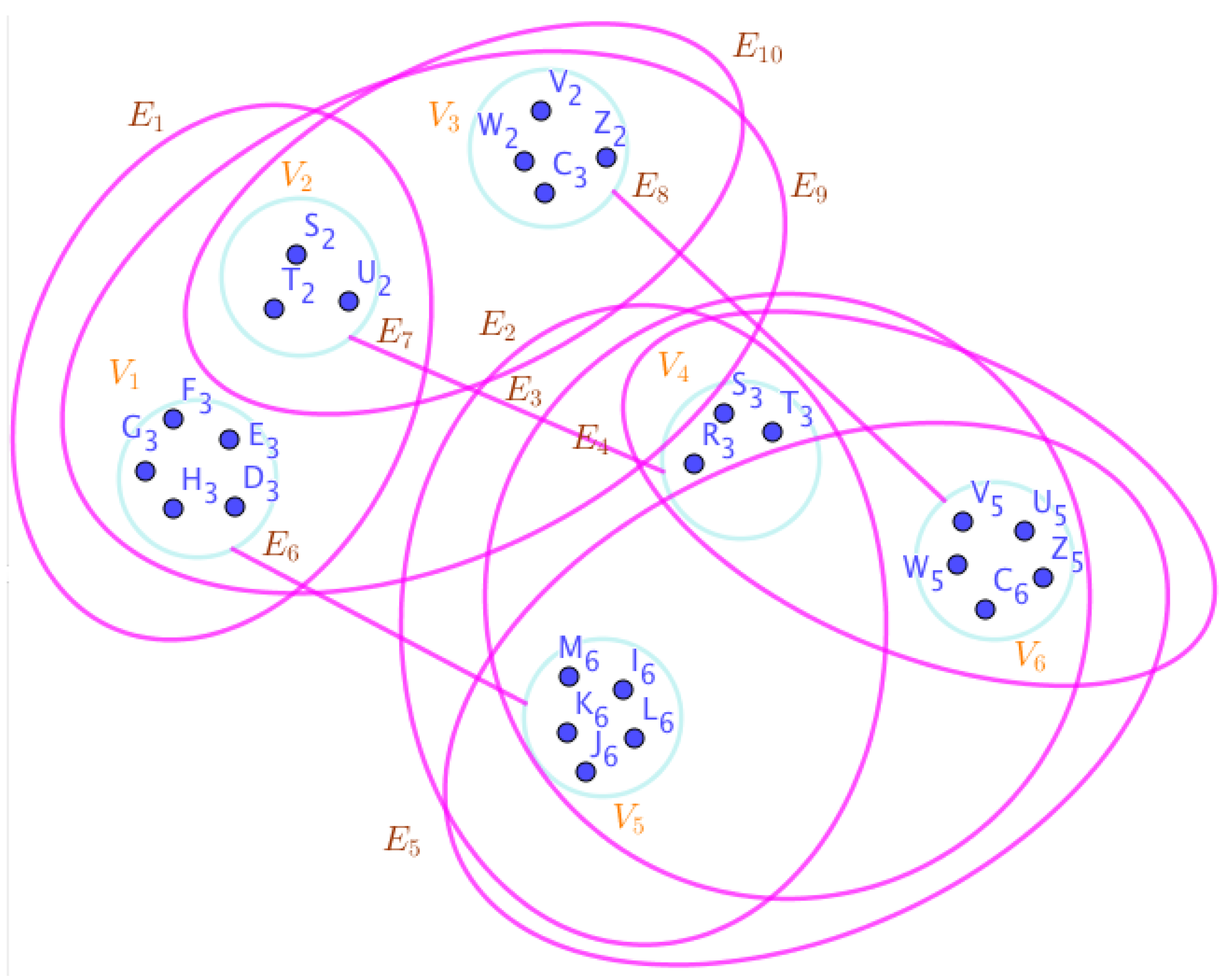
Figure 14.
The neutrosophic SuperHyperGraphs Associated to the Notions of neutrosophic 1-failed SuperHyperForcing in the Examples () and (23).
Figure 14.
The neutrosophic SuperHyperGraphs Associated to the Notions of neutrosophic 1-failed SuperHyperForcing in the Examples () and (23).
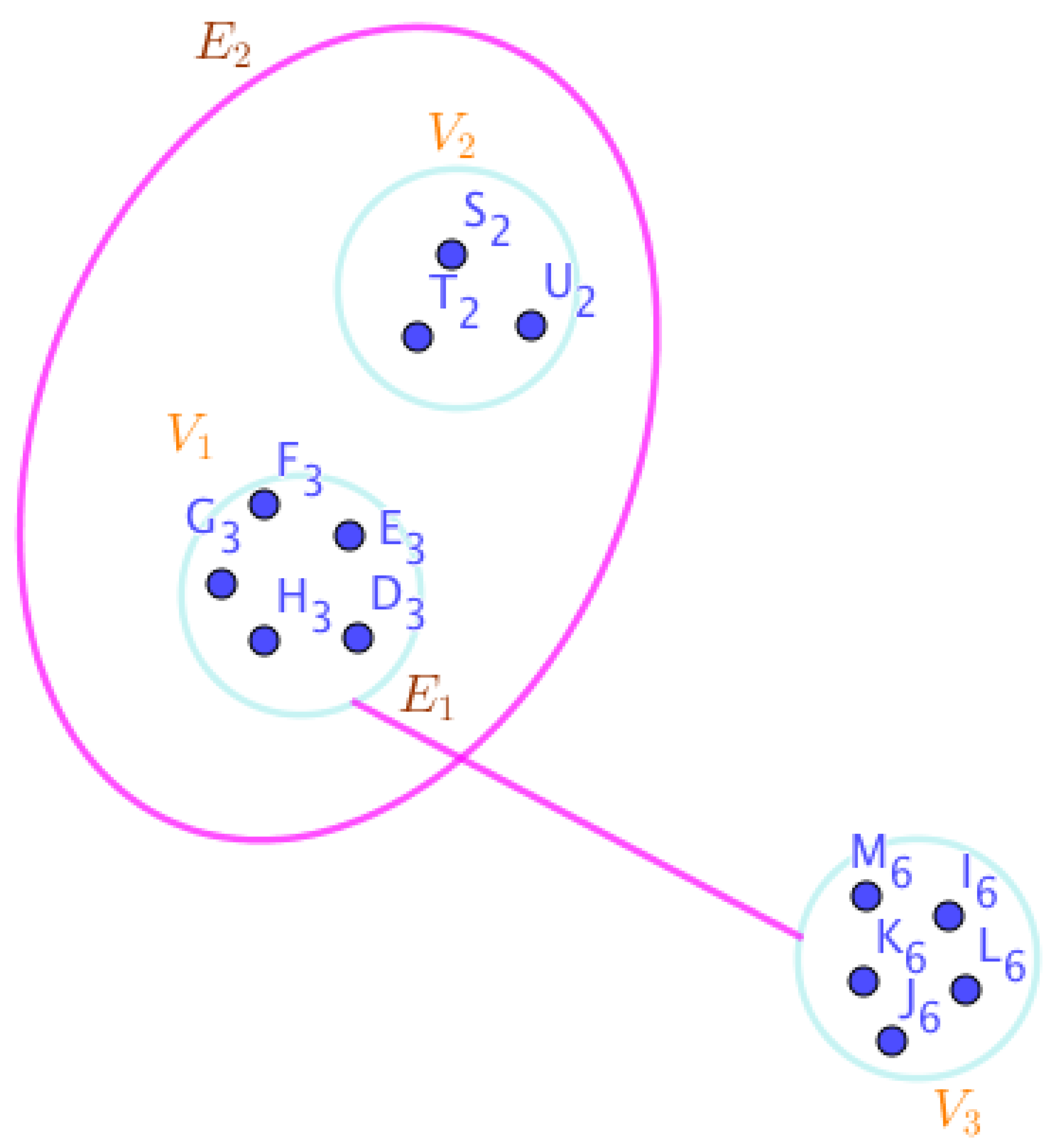
Figure 15.
The neutrosophic SuperHyperGraphs Associated to the Notions of neutrosophic 1-failed SuperHyperForcing in the Examples () and (23).
Figure 15.
The neutrosophic SuperHyperGraphs Associated to the Notions of neutrosophic 1-failed SuperHyperForcing in the Examples () and (23).

Figure 16.
The neutrosophic SuperHyperGraphs Associated to the Notions of neutrosophic 1-failed SuperHyperForcing in the Examples () and (23).
Figure 16.
The neutrosophic SuperHyperGraphs Associated to the Notions of neutrosophic 1-failed SuperHyperForcing in the Examples () and (23).
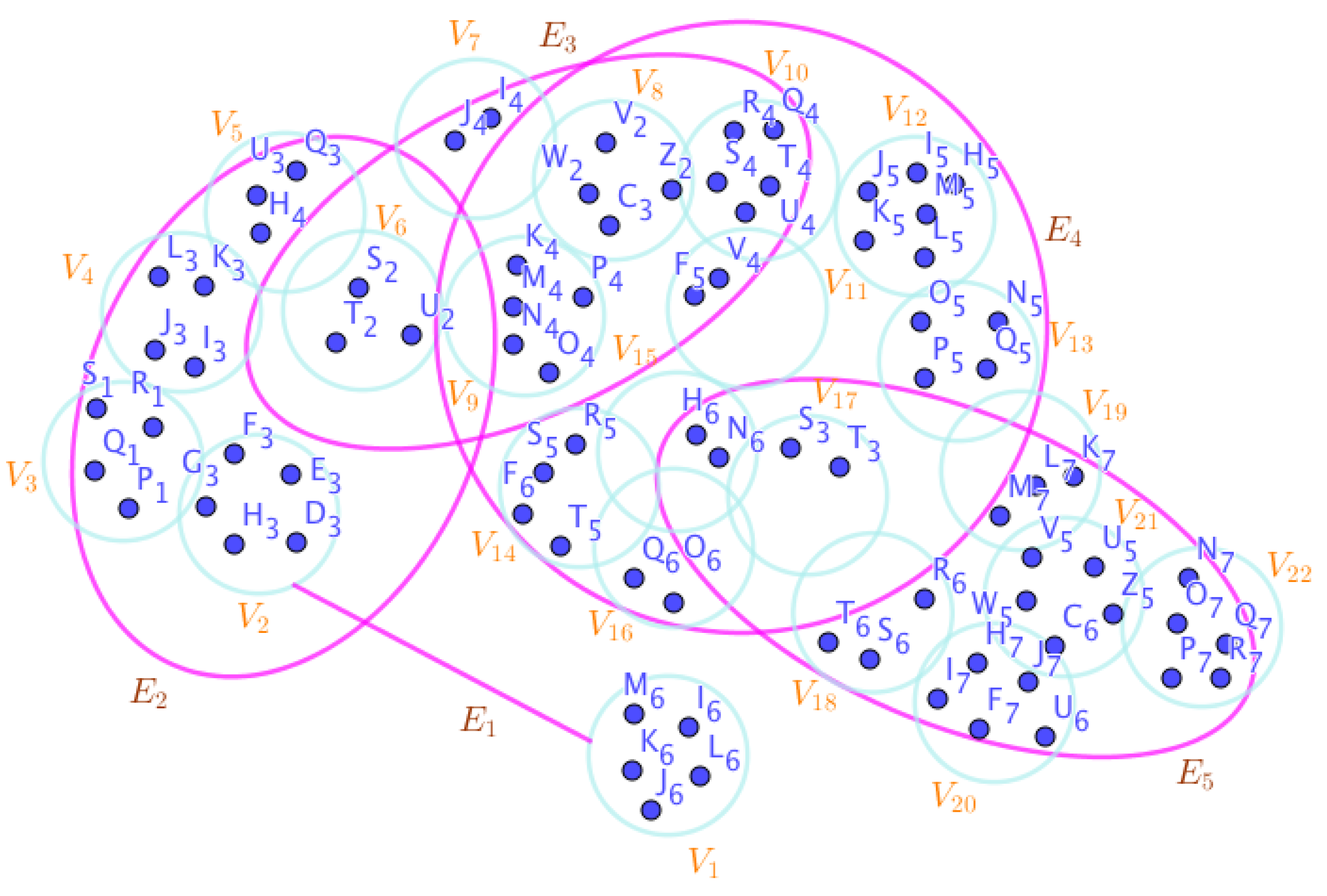
Figure 17.
The neutrosophic SuperHyperGraphs Associated to the Notions of neutrosophic 1-failed SuperHyperForcing in the Examples () and (23).
Figure 17.
The neutrosophic SuperHyperGraphs Associated to the Notions of neutrosophic 1-failed SuperHyperForcing in the Examples () and (23).

Figure 18.
The neutrosophic SuperHyperGraphs Associated to the Notions of neutrosophic 1-failed SuperHyperForcing in the Examples () and (23).
Figure 18.
The neutrosophic SuperHyperGraphs Associated to the Notions of neutrosophic 1-failed SuperHyperForcing in the Examples () and (23).

Figure 19.
The neutrosophic SuperHyperGraphs Associated to the Notions of neutrosophic 1-failed SuperHyperForcing in the Examples () and (23).
Figure 19.
The neutrosophic SuperHyperGraphs Associated to the Notions of neutrosophic 1-failed SuperHyperForcing in the Examples () and (23).
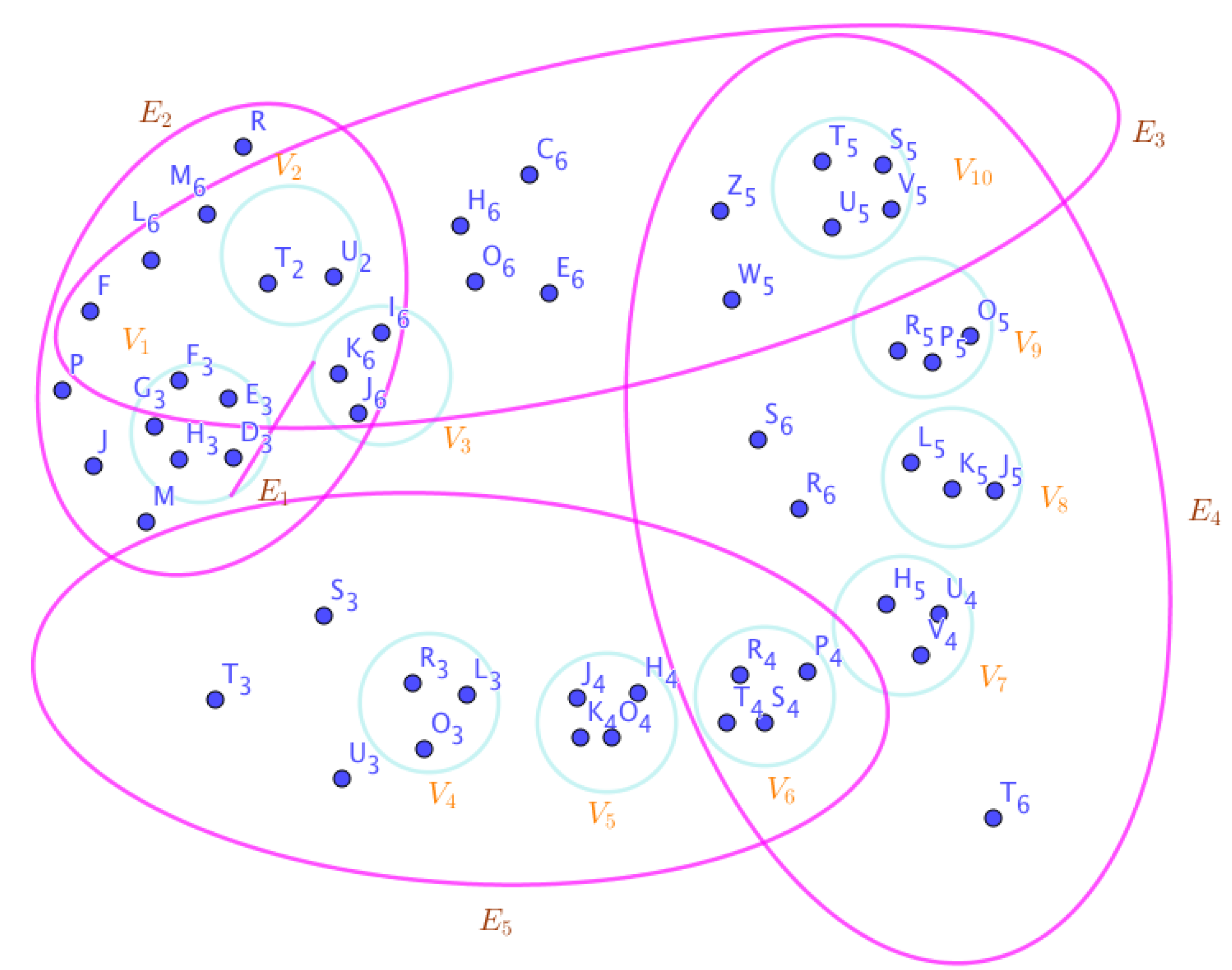
Figure 20.
The neutrosophic SuperHyperGraphs Associated to the Notions of neutrosophic 1-failed SuperHyperForcing in the Examples () and (23).
Figure 20.
The neutrosophic SuperHyperGraphs Associated to the Notions of neutrosophic 1-failed SuperHyperForcing in the Examples () and (23).

Proposition 24.
Assume a connected neutrosophic SuperHyperNotion SuperHyperGraph Then in the worst case, literally, is an neutrosophic 1-failed SuperHyperForcing. In other words, the most neutrosophic cardinality, the upper sharp bound for neutrosophic cardinality, of neutrosophic 1-failed SuperHyperForcing is the neutrosophic cardinality of
Proof.
Assume a connected neutrosophic SuperHyperNotion SuperHyperGraph The neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices is a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex but it isn’t an neutrosophic 1-failed SuperHyperForcing. Since it doesn’t have the maximum neutrosophic cardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex. The neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices is the maximum neutrosophic cardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) but it isn’t an neutrosophic 1-failed SuperHyperForcing. Since it doesn’t do the procedure such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex [there’s at least one white without any white neutrosophic SuperHyperNeighbor outside implying there’s, by the connectedness of the connected neutrosophic SuperHyperNotion SuperHyperGraph a neutrosophic SuperHyperVertex, titled its neutrosophic SuperHyperNeighbor, to the neutrosophic SuperHyperSet S does the “the color-change rule”.]. There’re only two neutrosophic SuperHyperVertices outside the intended neutrosophic SuperHyperSet, Thus the obvious neutrosophic 1-failed SuperHyperForcing, is up. The obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing, is a neutrosophic SuperHyperSet, excludes only two neutrosophic SuperHyperVertices are titled in a connected neutrosophic neutrosophic SuperHyperNeighbors neutrosophic SuperHyperGraph Since the neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices is the maximum neutrosophic cardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex. □
Proposition 25.
Assume a connected neutrosophic SuperHyperNotion SuperHyperGraph Then the extreme number of neutrosophic 1-failed SuperHyperForcing has, the most neutrosophic cardinality, the upper sharp bound for neutrosophic cardinality, is the extreme neutrosophic cardinality of if there’s an neutrosophic 1-failed SuperHyperForcing with the most neutrosophic cardinality, the upper sharp bound for neutrosophic cardinality.
Proof.
Assume a connected neutrosophic SuperHyperNotion SuperHyperGraph Consider there’s an neutrosophic 1-failed SuperHyperForcing with the most neutrosophic cardinality, the upper sharp bound for neutrosophic cardinality. The neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices is a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex but it isn’t an neutrosophic 1-failed SuperHyperForcing. Since it doesn’t have the maximum neutrosophic cardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex. The neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices is the maximum neutrosophic cardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) but it isn’t an neutrosophic 1-failed SuperHyperForcing. Since it doesn’t do the procedure such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex [there’s at least one white without any white neutrosophic SuperHyperNeighbor outside implying there’s, by the connectedness of the connected neutrosophic SuperHyperNotion SuperHyperGraph a neutrosophic SuperHyperVertex, titled its neutrosophic SuperHyperNeighbor, to the neutrosophic SuperHyperSet S does the “the color-change rule”.]. There’re only two neutrosophic SuperHyperVertices outside the intended neutrosophic SuperHyperSet, Thus the obvious neutrosophic 1-failed SuperHyperForcing, is up. The obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing, is a neutrosophic SuperHyperSet, excludes only two neutrosophic SuperHyperVertices are titled in a connected neutrosophic neutrosophic SuperHyperNeighbors neutrosophic SuperHyperGraph Since the neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices is the maximum neutrosophic cardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex. It implies that extreme number of neutrosophic 1-failed SuperHyperForcing has, the most neutrosophic cardinality, the upper sharp bound for neutrosophic cardinality, is Thus it induces that the extreme number of neutrosophic 1-failed SuperHyperForcing has, the most neutrosophic cardinality, the upper sharp bound for neutrosophic cardinality, is the extreme neutrosophic cardinality of if there’s an neutrosophic 1-failed SuperHyperForcing with the most neutrosophic cardinality, the upper sharp bound for neutrosophic cardinality. □
Proposition 26.
Assume a connected neutrosophic SuperHyperNotion SuperHyperGraph If a neutrosophic SuperHyperEdge has z neutrosophic SuperHyperVertices, then number of those neutrosophic SuperHyperVertices from that neutrosophic SuperHyperEdge belong to any neutrosophic 1-failed SuperHyperForcing.
Proof.
Assume a connected neutrosophic SuperHyperNotion SuperHyperGraph Let a neutrosophic SuperHyperEdge has z neutrosophic SuperHyperVertices. Consider number of those neutrosophic SuperHyperVertices from that neutrosophic SuperHyperEdge belong to any given neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices. Consider there’s an neutrosophic 1-failed SuperHyperForcing with the most neutrosophic cardinality, the upper sharp bound for neutrosophic cardinality. The neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices is a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex but it isn’t an neutrosophic 1-failed SuperHyperForcing. Since it doesn’t have the maximum neutrosophic cardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex. The neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices is the maximum neutrosophic cardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) but it isn’t an neutrosophic 1-failed SuperHyperForcing. Since it doesn’t do the procedure such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex [there’s at least one white without any white neutrosophic SuperHyperNeighbor outside implying there’s, by the connectedness of the connected neutrosophic SuperHyperNotion SuperHyperGraph a neutrosophic SuperHyperVertex, titled its neutrosophic SuperHyperNeighbor, to the neutrosophic SuperHyperSet S does the “the color-change rule”.]. There’re only two neutrosophic SuperHyperVertices outside the intended neutrosophic SuperHyperSet, Thus the obvious neutrosophic 1-failed SuperHyperForcing, is up. The obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing, is a neutrosophic SuperHyperSet, excludes only two neutrosophic SuperHyperVertices are titled in a connected neutrosophic neutrosophic SuperHyperNeighbors neutrosophic SuperHyperGraph Since the neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices is the maximum neutrosophic cardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex. It implies that extreme number of neutrosophic 1-failed SuperHyperForcing has, the most neutrosophic cardinality, the upper sharp bound for neutrosophic cardinality, is Thus it induces that the extreme number of neutrosophic 1-failed SuperHyperForcing has, the most neutrosophic cardinality, the upper sharp bound for neutrosophic cardinality, is the extreme neutrosophic cardinality of if there’s an neutrosophic 1-failed SuperHyperForcing with the most neutrosophic cardinality, the upper sharp bound for neutrosophic cardinality. Thus all the following neutrosophic SuperHyperSets of neutrosophic SuperHyperVertices are the simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing. It’s the contradiction to the neutrosophic SuperHyperSet either or is an neutrosophic 1-failed SuperHyperForcing. Thus any given neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices contains the number of those neutrosophic SuperHyperVertices from that neutrosophic SuperHyperEdge with z neutrosophic SuperHyperVertices less than isn’t an neutrosophic 1-failed SuperHyperForcing. Thus if a neutrosophic SuperHyperEdge has z neutrosophic SuperHyperVertices, then number of those neutrosophic SuperHyperVertices from that neutrosophic SuperHyperEdge belong to any neutrosophic 1-failed SuperHyperForcing. □
Proposition 27.
Assume a connected neutrosophic SuperHyperNotion SuperHyperGraph There’s a neutrosophic SuperHyperEdge has only two distinct neutrosophic SuperHyperVertices outside of an neutrosophic 1-failed SuperHyperForcing. In other words, there’s an unique neutrosophic SuperHyperEdge has only two distinct white neutrosophic SuperHyperVertices.
Proof.
Assume a connected neutrosophic SuperHyperNotion SuperHyperGraph Let a neutrosophic SuperHyperEdge has some neutrosophic SuperHyperVertices. Consider some numbers of those neutrosophic SuperHyperVertices from that neutrosophic SuperHyperEdge excluding three distinct neutrosophic SuperHyperVertices, belong to any given neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices. Consider there’s an neutrosophic 1-failed SuperHyperForcing with the most neutrosophic cardinality, the upper sharp bound for neutrosophic cardinality. The neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices is a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex but it isn’t an neutrosophic 1-failed SuperHyperForcing. Since it doesn’t have the maximum neutrosophic cardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex. The neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices is the maximum neutrosophic cardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) but it isn’t an neutrosophic 1-failed SuperHyperForcing. Since it doesn’t do the procedure such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex [there’s at least one white without any white neutrosophic SuperHyperNeighbor outside implying there’s, by the connectedness of the connected neutrosophic SuperHyperNotion SuperHyperGraph a neutrosophic SuperHyperVertex, titled its neutrosophic SuperHyperNeighbor, to the neutrosophic SuperHyperSet S does the “the color-change rule”.]. There’re only two neutrosophic SuperHyperVertices outside the intended neutrosophic SuperHyperSet, Thus the obvious neutrosophic 1-failed SuperHyperForcing, is up. The obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing, is a neutrosophic SuperHyperSet, excludes only two neutrosophic SuperHyperVertices are titled in a connected neutrosophic neutrosophic SuperHyperNeighbors neutrosophic SuperHyperGraph Since the neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices is the maximum neutrosophic cardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex. It implies that extreme number of neutrosophic 1-failed SuperHyperForcing has, the most neutrosophic cardinality, the upper sharp bound for neutrosophic cardinality, is Thus it induces that the extreme number of neutrosophic 1-failed SuperHyperForcing has, the most neutrosophic cardinality, the upper sharp bound for neutrosophic cardinality, is the extreme neutrosophic cardinality of if there’s an neutrosophic 1-failed SuperHyperForcing with the most neutrosophic cardinality, the upper sharp bound for neutrosophic cardinality. Thus if a neutrosophic SuperHyperEdge has some neutrosophic SuperHyperVertices, then, with excluding two distinct neutrosophic SuperHyperVertices, the all number of those neutrosophic SuperHyperVertices from that neutrosophic SuperHyperEdge belong to any neutrosophic 1-failed SuperHyperForcing. Thus, in a connected neutrosophic SuperHyperNotion SuperHyperGraph there’s a neutrosophic SuperHyperEdge has only two distinct neutrosophic SuperHyperVertices outside of neutrosophic 1-failed SuperHyperForcing. In other words, there’s a neutrosophic SuperHyperEdge has only two distinct white neutrosophic SuperHyperVertices which are neutrosophic SuperHyperNeighbors. □
Proposition 28.
Assume a connected neutrosophic SuperHyperNotion SuperHyperGraph The all exterior neutrosophic SuperHyperVertices belong to any neutrosophic 1-failed SuperHyperForcing if there’s one of them such that there are only two interior neutrosophic SuperHyperVertices are mutually neutrosophic SuperHyperNeighbors.
Proof.
Assume a connected neutrosophic SuperHyperNotion SuperHyperGraph Let a neutrosophic SuperHyperEdge has some neutrosophic SuperHyperVertices. Consider some numbers of those neutrosophic SuperHyperVertices from that neutrosophic SuperHyperEdge excluding three distinct neutrosophic SuperHyperVertices, belong to any given neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices. Consider there’s an neutrosophic 1-failed SuperHyperForcing with the most neutrosophic cardinality, the upper sharp bound for neutrosophic cardinality. The neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices is a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex but it isn’t an neutrosophic 1-failed SuperHyperForcing. Since it doesn’t have the maximum neutrosophic cardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex. The neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices is the maximum neutrosophic cardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) but it isn’t an neutrosophic 1-failed SuperHyperForcing. Since it doesn’t do the procedure such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex [there’s at least one white without any white neutrosophic SuperHyperNeighbor outside implying there’s, by the connectedness of the connected neutrosophic SuperHyperNotion SuperHyperGraph a neutrosophic SuperHyperVertex, titled its neutrosophic SuperHyperNeighbor, to the neutrosophic SuperHyperSet S does the “the color-change rule”.]. There’re only two neutrosophic SuperHyperVertices outside the intended neutrosophic SuperHyperSet, Thus the obvious neutrosophic 1-failed SuperHyperForcing, is up. The obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing, is a neutrosophic SuperHyperSet, excludes only two neutrosophic SuperHyperVertices are titled in a connected neutrosophic neutrosophic SuperHyperNeighbors neutrosophic SuperHyperGraph Since the neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices is the maximum neutrosophic cardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex. It implies that extreme number of neutrosophic 1-failed SuperHyperForcing has, the most neutrosophic cardinality, the upper sharp bound for neutrosophic cardinality, is Thus it induces that the extreme number of neutrosophic 1-failed SuperHyperForcing has, the most neutrosophic cardinality, the upper sharp bound for neutrosophic cardinality, is the extreme neutrosophic cardinality of if there’s an neutrosophic 1-failed SuperHyperForcing with the most neutrosophic cardinality, the upper sharp bound for neutrosophic cardinality. Thus if a neutrosophic SuperHyperEdge has some neutrosophic SuperHyperVertices, then, with excluding two distinct neutrosophic SuperHyperVertices, the all number of those neutrosophic SuperHyperVertices from that neutrosophic SuperHyperEdge belong to any neutrosophic 1-failed SuperHyperForcing. Thus, in a connected neutrosophic SuperHyperNotion SuperHyperGraph there’s a neutrosophic SuperHyperEdge has only two distinct neutrosophic SuperHyperVertices outside of neutrosophic 1-failed SuperHyperForcing. In other words, here’s a neutrosophic SuperHyperEdge has only two distinct white neutrosophic SuperHyperVertices. In a connected neutrosophic SuperHyperNotion SuperHyperGraph the all exterior neutrosophic SuperHyperVertices belong to any neutrosophic 1-failed SuperHyperForcing if there’s one of them such that there are only two interior neutrosophic SuperHyperVertices are mutually neutrosophic SuperHyperNeighbors. □
Proposition 29.
Assume a connected neutrosophic SuperHyperNotion SuperHyperGraph The any neutrosophic 1-failed SuperHyperForcing only contains all interior neutrosophic SuperHyperVertices and all exterior neutrosophic SuperHyperVertices where there’s any of them has two neutrosophic SuperHyperNeighbors out.
Proof.
Assume a connected neutrosophic SuperHyperNotion SuperHyperGraph Let a neutrosophic SuperHyperEdge has some neutrosophic SuperHyperVertices. Consider some numbers of those neutrosophic SuperHyperVertices from that neutrosophic SuperHyperEdge excluding three distinct neutrosophic SuperHyperVertices, belong to any given neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices. Consider there’s an neutrosophic 1-failed SuperHyperForcing with the most neutrosophic cardinality, the upper sharp bound for neutrosophic cardinality. The neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices is a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex but it isn’t an neutrosophic 1-failed SuperHyperForcing. Since it doesn’t have the maximum neutrosophic cardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex. The neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices is the maximum neutrosophic cardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) but it isn’t an neutrosophic 1-failed SuperHyperForcing. Since it doesn’t do the procedure such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex [there’s at least one white without any white neutrosophic SuperHyperNeighbor outside implying there’s, by the connectedness of the connected neutrosophic SuperHyperNotion SuperHyperGraph a neutrosophic SuperHyperVertex, titled its neutrosophic SuperHyperNeighbor, to the neutrosophic SuperHyperSet S does the “the color-change rule”.]. There’re only two neutrosophic SuperHyperVertices outside the intended neutrosophic SuperHyperSet, Thus the obvious neutrosophic 1-failed SuperHyperForcing, is up. The obvious simple type-neutrosophic SuperHyperSet of the neutrosophic 1-failed SuperHyperForcing, is a neutrosophic SuperHyperSet, excludes only two neutrosophic SuperHyperVertices are titled in a connected neutrosophic neutrosophic SuperHyperNeighbors neutrosophic SuperHyperGraph Since the neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices is the maximum neutrosophic cardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex. It implies that extreme number of neutrosophic 1-failed SuperHyperForcing has, the most neutrosophic cardinality, the upper sharp bound for neutrosophic cardinality, is Thus it induces that the extreme number of neutrosophic 1-failed SuperHyperForcing has, the most neutrosophic cardinality, the upper sharp bound for neutrosophic cardinality, is the extreme neutrosophic cardinality of if there’s an neutrosophic 1-failed SuperHyperForcing with the most neutrosophic cardinality, the upper sharp bound for neutrosophic cardinality. Thus if a neutrosophic SuperHyperEdge has some neutrosophic SuperHyperVertices, then, with excluding two distinct neutrosophic SuperHyperVertices, the all number of those neutrosophic SuperHyperVertices from that neutrosophic SuperHyperEdge belong to any neutrosophic 1-failed SuperHyperForcing. Thus, in a connected neutrosophic SuperHyperNotion SuperHyperGraph there’s a neutrosophic SuperHyperEdge has only two distinct neutrosophic SuperHyperVertices outside of neutrosophic 1-failed SuperHyperForcing. In other words, here’s a neutrosophic SuperHyperEdge has only two distinct white neutrosophic SuperHyperVertices. In a connected neutrosophic SuperHyperNotion SuperHyperGraph the all exterior neutrosophic SuperHyperVertices belong to any neutrosophic 1-failed SuperHyperForcing if there’s one of them such that there are only two interior neutrosophic SuperHyperVertices are mutually neutrosophic SuperHyperNeighbors. Thus in a connected neutrosophic SuperHyperNotion SuperHyperGraph any neutrosophic 1-failed SuperHyperForcing only contains all interior neutrosophic SuperHyperVertices and all exterior neutrosophic SuperHyperVertices where there’s any of them has two neutrosophic SuperHyperNeighbors out. □
Remark 30.
The words “neutrosophic 1-failed SuperHyperForcing” and “neutrosophic SuperHyperDominating” refer to the maximum type-style and the minimum type-style. In other words, they refer to both the maximum[minimum] number and the neutrosophic SuperHyperSet with the maximum[minimum] neutrosophic cardinality.
Proposition 31.
Assume a connected neutrosophic SuperHyperNotion SuperHyperGraph An neutrosophic 1-failed SuperHyperForcing contains the neutrosophic SuperHyperDominating.
Proof.
Assume a connected neutrosophic SuperHyperNotion SuperHyperGraph By applying the Proposition (29), the results are up. Thus in a connected neutrosophic SuperHyperNotion SuperHyperGraph an neutrosophic 1-failed SuperHyperForcing contains the neutrosophic SuperHyperDominating. □
3. Results on Neutrosophic SuperHyperClasses
Proposition 32.
Assume a connected neutrosophic SuperHyperPath Then an 1-failed neutrosophic SuperHyperForcing-style with the maximum neutrosophic cardinality is a neutrosophic SuperHyperSet of the exterior neutrosophic SuperHyperVertices.
Proposition 33.
Assume a connected neutrosophic SuperHyperPath Then an 1-failed neutrosophic SuperHyperForcing is a neutrosophic SuperHyperSet of the exterior neutrosophic SuperHyperVertices and the interior neutrosophic SuperHyperVertices with only two exceptions in the form of interior neutrosophic SuperHyperVertices from the same neutrosophic SuperHyperEdge. An 1-failed neutrosophic SuperHyperForcing has the neutrosophic number of all the neutrosophic SuperHyperVertices minus two. Thus,
Where is the unary operation on the SuperHyperVertices of the SuperHyperGraph to assign the determinacy, the indeterminacy and the neutrality, for respectively.
Proof.
Assume a connected neutrosophic SuperHyperPath Let a neutrosophic SuperHyperEdge has some neutrosophic SuperHyperVertices. Consider some neutrosophic numbers of those neutrosophic SuperHyperVertices from that neutrosophic SuperHyperEdge excluding three distinct neutrosophic SuperHyperVertices, belong to any given neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices. Consider there’s an 1-failed neutrosophic SuperHyperForcing with the most neutrosophic cardinality, the upper sharp bound for neutrosophic cardinality. The neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices is a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex but it isn’t an 1-failed neutrosophic SuperHyperForcing. Since it doesn’t have the maximum neutrosophic cardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex. The neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices is the maximum neutrosophic cardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) but it isn’t an 1-failed neutrosophic SuperHyperForcing. Since it doesn’t do the procedure such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex [there’s at least one white without any white neutrosophic SuperHyperNeighbor outside implying there’s, by the connectedness of the connected neutrosophic neutrosophic SuperHyperGraph a neutrosophic SuperHyperVertex, titled its neutrosophic SuperHyperNeighbor, to the neutrosophic SuperHyperSet S does the “the color-change rule”.]. There’re only two neutrosophic SuperHyperVertices outside the intended neutrosophic SuperHyperSet, Thus the obvious 1-failed neutrosophic SuperHyperForcing, is up. The obvious simple type-neutrosophic SuperHyperSet of the 1-failed neutrosophic SuperHyperForcing, is a neutrosophic SuperHyperSet, excludes only two neutrosophic SuperHyperVertices are titled in a connected neutrosophic neutrosophic SuperHyperNeighbors neutrosophic SuperHyperGraph Since the neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices is the maximum neutrosophic cardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex. It implies that neutrosophic number of 1-failed neutrosophic SuperHyperForcing has, the most neutrosophic cardinality, the upper sharp bound for neutrosophic cardinality, is Thus it induces that the neutrosophic number of 1-failed neutrosophic SuperHyperForcing has, the most neutrosophic cardinality, the upper sharp bound for neutrosophic cardinality, is the neutrosophic cardinality of if there’s an 1-failed neutrosophic SuperHyperForcing with the most neutrosophic cardinality, the upper sharp bound for neutrosophic cardinality. Thus if a neutrosophic SuperHyperEdge has some neutrosophic SuperHyperVertices, then, with excluding two distinct neutrosophic SuperHyperVertices, the all neutrosophic number of those neutrosophic SuperHyperVertices from that neutrosophic SuperHyperEdge belong to any 1-failed neutrosophic SuperHyperForcing. Thus, in a connected neutrosophic neutrosophic SuperHyperGraph there’s a neutrosophic SuperHyperEdge has only two distinct neutrosophic SuperHyperVertices outside of 1-failed neutrosophic SuperHyperForcing. In other words, here’s a neutrosophic SuperHyperEdge has only two distinct white neutrosophic SuperHyperVertices. In a connected neutrosophic neutrosophic SuperHyperGraph the all exterior neutrosophic SuperHyperVertices belong to any 1-failed neutrosophic SuperHyperForcing if there’s one of them such that there are only two interior neutrosophic SuperHyperVertices are mutually neutrosophic SuperHyperNeighbors. Then an 1-failed neutrosophic SuperHyperForcing is a neutrosophic SuperHyperSet of the exterior neutrosophic SuperHyperVertices and the interior neutrosophic SuperHyperVertices with only two exceptions in the form of interior neutrosophic SuperHyperVertices from the same neutrosophic SuperHyperEdge. An 1-failed neutrosophic SuperHyperForcing has the neutrosophic number of all the neutrosophic SuperHyperVertices minus two. Thus,
where is the unary operation on the SuperHyperVertices of the SuperHyperGraph to assign the determinacy, the indeterminacy and the neutrality, for respectively. □
Example 34.
In the Figure (21), the connected neutrosophic SuperHyperPath is highlighted and featured.
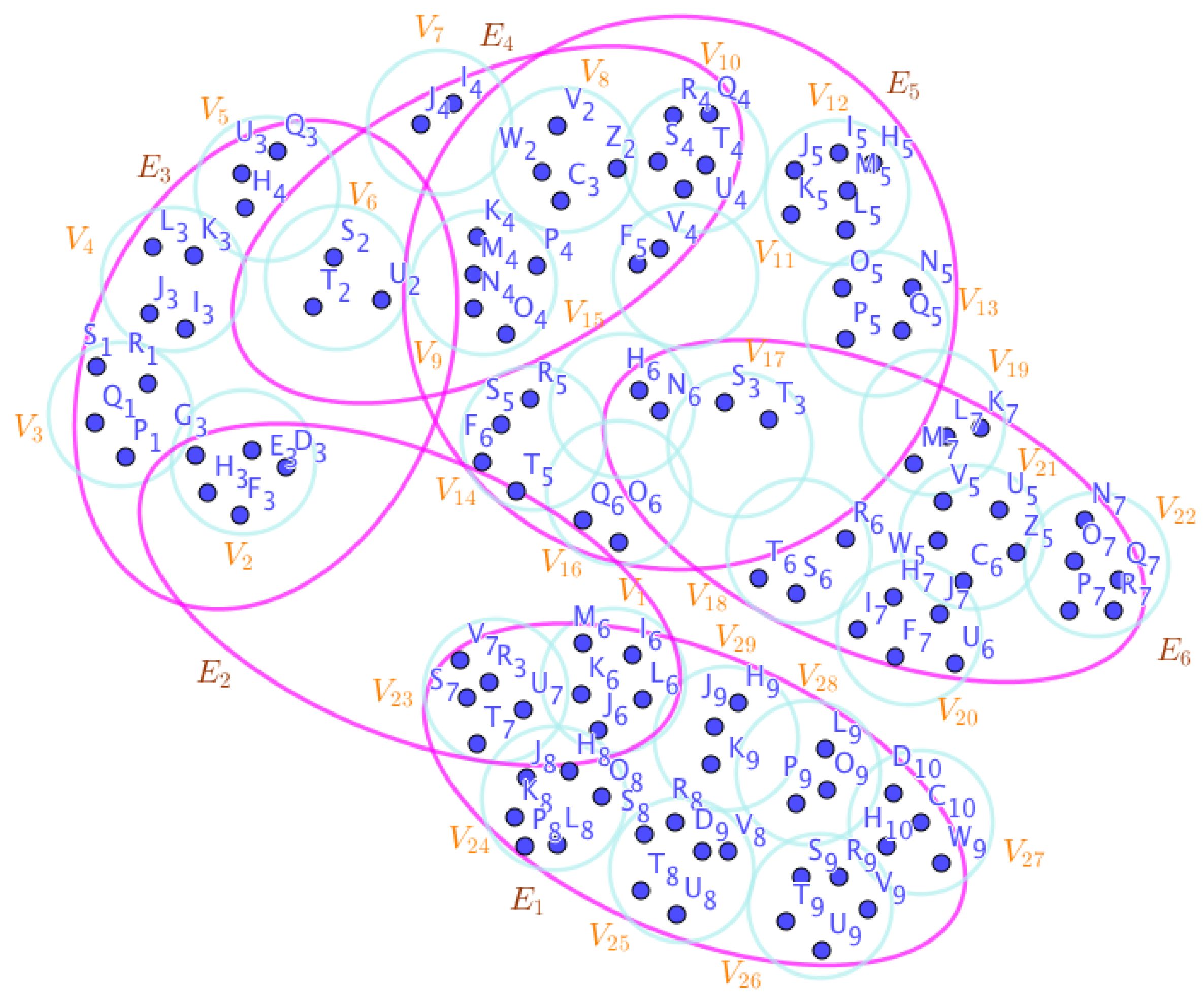
Figure 21.
A neutrosophic SuperHyperPath Associated to the Notions of 1-failed neutrosophic SuperHyperForcing in the Example (34).
Figure 21.
A neutrosophic SuperHyperPath Associated to the Notions of 1-failed neutrosophic SuperHyperForcing in the Example (34).

By using the Figure (21) and the Table (4), the neutrosophic SuperHyperPath is obtained.
The neutrosophic SuperHyperSet,
of the neutrosophic SuperHyperVertices of the connected neutrosophic SuperHyperPath in the neutrosophic SuperHyperModel (21), is the 1-failed neutrosophic SuperHyperForcing.
Table 4.
The Values of Vertices, SuperVertices, Edges, HyperEdges, and SuperHyperEdges Belong to The Neutrosophic SuperHyperPath Mentioned in the Example (34)
Table 4.
The Values of Vertices, SuperVertices, Edges, HyperEdges, and SuperHyperEdges Belong to The Neutrosophic SuperHyperPath Mentioned in the Example (34)
| The Values of The Vertices | The Number of Position in Alphabet |
| The Values of The SuperVertices | The maximum Values of Its Vertices |
| The Values of The Edges | The maximum Values of Its Vertices |
| The Values of The HyperEdges | The maximum Values of Its Vertices |
| The Values of The SuperHyperEdges | The maximum Values of Its Endpoints |
Proposition 35.
Assume a connected neutrosophic SuperHyperCycle Then an 1-failed neutrosophic SuperHyperForcing is a neutrosophic SuperHyperSet of the exterior neutrosophic SuperHyperVertices and the interior neutrosophic SuperHyperVertices with only two exceptions in the form of interior neutrosophic SuperHyperVertices from the same neutrosophic SuperHyperEdge. An 1-failed neutrosophic SuperHyperForcing has the neutrosophic number of all the neutrosophic SuperHyperVertices minus on the 2 neutrosophic numbers excerpt the same exterior neutrosophic SuperHyperPart. Thus,
Where is the unary operation on the SuperHyperVertices of the SuperHyperGraph to assign the determinacy, the indeterminacy and the neutrality, for respectively.
Proof.
Assume a connected neutrosophic SuperHyperCycle Let a neutrosophic SuperHyperEdge has some neutrosophic SuperHyperVertices. Consider some neutrosophic numbers of those neutrosophic SuperHyperVertices from that neutrosophic SuperHyperEdge excluding three distinct neutrosophic SuperHyperVertices, belong to any given neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices. Consider there’s an 1-failed neutrosophic SuperHyperForcing with the most neutrosophic cardinality, the upper sharp bound for neutrosophic cardinality. The neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices is a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex but it isn’t an 1-failed neutrosophic SuperHyperForcing. Since it doesn’t have the maximum neutrosophic cardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex. The neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices is the maximum neutrosophic cardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) but it isn’t an 1-failed neutrosophic SuperHyperForcing. Since it doesn’t do the procedure such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex [there’s at least one white without any white neutrosophic SuperHyperNeighbor outside implying there’s, by the connectedness of the connected neutrosophic neutrosophic SuperHyperGraph a neutrosophic SuperHyperVertex, titled its neutrosophic SuperHyperNeighbor, to the neutrosophic SuperHyperSet S does the “the color-change rule”.]. There’re only two neutrosophic SuperHyperVertices outside the intended neutrosophic SuperHyperSet, Thus the obvious 1-failed neutrosophic SuperHyperForcing, is up. The obvious simple type-neutrosophic SuperHyperSet of the 1-failed neutrosophic SuperHyperForcing, is a neutrosophic SuperHyperSet, excludes only two neutrosophic SuperHyperVertices are titled in a connected neutrosophic neutrosophic SuperHyperNeighbors neutrosophic SuperHyperGraph Since the neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices is the maximum neutrosophic cardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex. It implies that neutrosophic number of 1-failed neutrosophic SuperHyperForcing has, the most neutrosophic cardinality, the upper sharp bound for neutrosophic cardinality, is Thus it induces that the neutrosophic number of 1-failed neutrosophic SuperHyperForcing has, the most neutrosophic cardinality, the upper sharp bound for neutrosophic cardinality, is the neutrosophic cardinality of if there’s an 1-failed neutrosophic SuperHyperForcing with the most neutrosophic cardinality, the upper sharp bound for neutrosophic cardinality. Thus if a neutrosophic SuperHyperEdge has some neutrosophic SuperHyperVertices, then, with excluding two distinct neutrosophic SuperHyperVertices, the all neutrosophic number of those neutrosophic SuperHyperVertices from that neutrosophic SuperHyperEdge belong to any 1-failed neutrosophic SuperHyperForcing. Thus, in a connected neutrosophic neutrosophic SuperHyperGraph there’s a neutrosophic SuperHyperEdge has only two distinct neutrosophic SuperHyperVertices outside of 1-failed neutrosophic SuperHyperForcing. In other words, here’s a neutrosophic SuperHyperEdge has only two distinct white neutrosophic SuperHyperVertices. In a connected neutrosophic neutrosophic SuperHyperGraph the all exterior neutrosophic SuperHyperVertices belong to any 1-failed neutrosophic SuperHyperForcing if there’s one of them such that there are only two interior neutrosophic SuperHyperVertices are mutually neutrosophic SuperHyperNeighbors. Then an 1-failed neutrosophic SuperHyperForcing is a neutrosophic SuperHyperSet of the exterior neutrosophic SuperHyperVertices and the interior neutrosophic SuperHyperVertices with only two exceptions in the form of interior neutrosophic SuperHyperVertices from the same neutrosophic SuperHyperEdge. An 1-failed neutrosophic SuperHyperForcing has the neutrosophic number of all the neutrosophic SuperHyperVertices minus on the 2 neutrosophic numbers excerpt the same exterior neutrosophic SuperHyperPart. Thus,
Where is the unary operation on the SuperHyperVertices of the SuperHyperGraph to assign the determinacy, the indeterminacy and the neutrality, for respectively. □
Example 36.
In the Figure (22), the connected neutrosophic SuperHyperCycle is highlighted and featured.
By using the Figure (22) and the Table ((5)), the neutrosophic SuperHyperCycle is obtained.
The obtained neutrosophic SuperHyperSet, by the Algorithm in previous result, of the neutrosophic SuperHyperVertices of the connected neutrosophic SuperHyperCycle in the neutrosophic SuperHyperModel (22), is the 1-failed neutrosophic SuperHyperForcing.
Table 5.
The Values of Vertices, SuperVertices, Edges, HyperEdges, and SuperHyperEdges Belong to The Neutrosophic SuperHyperCycle Mentioned in the Example (36)
Table 5.
The Values of Vertices, SuperVertices, Edges, HyperEdges, and SuperHyperEdges Belong to The Neutrosophic SuperHyperCycle Mentioned in the Example (36)
| The Values of The Vertices | The Number of Position in Alphabet |
| The Values of The SuperVertices | The maximum Values of Its Vertices |
| The Values of The Edges | The maximum Values of Its Vertices |
| The Values of The HyperEdges | The maximum Values of Its Vertices |
| The Values of The SuperHyperEdges | The maximum Values of Its Endpoints |
Proposition 37.
Assume a connected neutrosophic SuperHyperStar Then an 1-failed neutrosophic SuperHyperForcing is a neutrosophic SuperHyperSet of the exterior neutrosophic SuperHyperVertices and the interior neutrosophic SuperHyperVertices, excluding the neutrosophic SuperHyperCenter, with only one exception in the form of interior neutrosophic SuperHyperVertices from any given neutrosophic SuperHyperEdge. An 1-failed neutrosophic SuperHyperForcing has the neutrosophic number of the neutrosophic cardinality of the second neutrosophic SuperHyperPart minus one. Thus,
Where is the unary operation on the SuperHyperVertices of the SuperHyperGraph to assign the determinacy, the indeterminacy and the neutrality, for respectively.
Proof.
Assume a connected neutrosophic SuperHyperStar Let a neutrosophic SuperHyperEdge has some neutrosophic SuperHyperVertices. Consider some neutrosophic numbers of those neutrosophic SuperHyperVertices from that neutrosophic SuperHyperEdge excluding three distinct neutrosophic SuperHyperVertices, belong to any given neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices. Consider there’s an 1-failed neutrosophic SuperHyperForcing with the most neutrosophic cardinality, the upper sharp bound for neutrosophic cardinality. The neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices is a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex but it isn’t an 1-failed neutrosophic SuperHyperForcing. Since it doesn’t have the maximum neutrosophic cardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex. The neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices is the maximum neutrosophic cardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) but it isn’t an 1-failed neutrosophic SuperHyperForcing. Since it doesn’t do the procedure such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex [there’s at least one white without any white neutrosophic SuperHyperNeighbor outside implying there’s, by the connectedness of the connected neutrosophic neutrosophic SuperHyperGraph a neutrosophic SuperHyperVertex, titled its neutrosophic SuperHyperNeighbor, to the neutrosophic SuperHyperSet S does the “the color-change rule”.]. There’re only two neutrosophic SuperHyperVertices outside the intended neutrosophic SuperHyperSet, Thus the obvious 1-failed neutrosophic SuperHyperForcing, is up. The obvious simple type-neutrosophic SuperHyperSet of the 1-failed neutrosophic SuperHyperForcing, is a neutrosophic SuperHyperSet, excludes only two neutrosophic SuperHyperVertices are titled in a connected neutrosophic neutrosophic SuperHyperNeighbors neutrosophic SuperHyperGraph Since the neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices is the maximum neutrosophic cardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex. It implies that neutrosophic number of 1-failed neutrosophic SuperHyperForcing has, the most neutrosophic cardinality, the upper sharp bound for neutrosophic cardinality, is Thus it induces that the neutrosophic number of 1-failed neutrosophic SuperHyperForcing has, the most neutrosophic cardinality, the upper sharp bound for neutrosophic cardinality, is the neutrosophic cardinality of if there’s an 1-failed neutrosophic SuperHyperForcing with the most neutrosophic cardinality, the upper sharp bound for neutrosophic cardinality. Thus if a neutrosophic SuperHyperEdge has some neutrosophic SuperHyperVertices, then, with excluding two distinct neutrosophic SuperHyperVertices, the all neutrosophic number of those neutrosophic SuperHyperVertices from that neutrosophic SuperHyperEdge belong to any 1-failed neutrosophic SuperHyperForcing. Thus, in a connected neutrosophic neutrosophic SuperHyperGraph there’s a neutrosophic SuperHyperEdge has only two distinct neutrosophic SuperHyperVertices outside of 1-failed neutrosophic SuperHyperForcing. In other words, here’s a neutrosophic SuperHyperEdge has only two distinct white neutrosophic SuperHyperVertices. In a connected neutrosophic neutrosophic SuperHyperGraph the all exterior neutrosophic SuperHyperVertices belong to any 1-failed neutrosophic SuperHyperForcing if there’s one of them such that there are only two interior neutrosophic SuperHyperVertices are mutually neutrosophic SuperHyperNeighbors. Then an 1-failed neutrosophic SuperHyperForcing is a neutrosophic SuperHyperSet of the exterior neutrosophic SuperHyperVertices and the interior neutrosophic SuperHyperVertices, excluding the neutrosophic SuperHyperCenter, with only one exception in the form of interior neutrosophic SuperHyperVertices from any given neutrosophic SuperHyperEdge. An 1-failed neutrosophic SuperHyperForcing has the neutrosophic number of the neutrosophic cardinality of the second neutrosophic SuperHyperPart minus one. Thus,
Where is the unary operation on the SuperHyperVertices of the SuperHyperGraph to assign the determinacy, the indeterminacy and the neutrality, for respectively. □
Example 38.
In the Figure (23), the connected neutrosophic SuperHyperStar is highlighted and featured.
By using the Figure (23) and the Table (6), the neutrosophic SuperHyperStar is obtained.
The obtained neutrosophic SuperHyperSet, by the Algorithm in previous result, of the neutrosophic SuperHyperVertices of the connected neutrosophic SuperHyperStar in the neutrosophic SuperHyperModel (23), is the 1-failed neutrosophic SuperHyperForcing.
Table 6.
The Values of Vertices, SuperVertices, Edges, HyperEdges, and SuperHyperEdges Belong to The Neutrosophic SuperHyperStar Mentioned in the Example (38)
Table 6.
The Values of Vertices, SuperVertices, Edges, HyperEdges, and SuperHyperEdges Belong to The Neutrosophic SuperHyperStar Mentioned in the Example (38)
| The Values of The Vertices | The Number of Position in Alphabet |
| The Values of The SuperVertices | The maximum Values of Its Vertices |
| The Values of The Edges | The maximum Values of Its Vertices |
| The Values of The HyperEdges | The maximum Values of Its Vertices |
| The Values of The SuperHyperEdges | The maximum Values of Its Endpoints |
Proposition 39.
Assume a connected neutrosophic SuperHyperBipartite Then an 1-failed neutrosophic SuperHyperForcing is a neutrosophic SuperHyperSet of the exterior neutrosophic SuperHyperVertices and the interior neutrosophic SuperHyperVertices with only two exceptions in the form of interior neutrosophic SuperHyperVertices from same neutrosophic SuperHyperEdge. An 1-failed neutrosophic SuperHyperForcing has the neutrosophic number of the neutrosophic cardinality of the first neutrosophic SuperHyperPart minus one plus the second neutrosophic SuperHyperPart minus one. Thus,
Where is the unary operation on the SuperHyperVertices of the SuperHyperGraph to assign the determinacy, the indeterminacy and the neutrality, for respectively.
Proof.
Assume a connected neutrosophic SuperHyperBipartite Let a neutrosophic SuperHyperEdge has some neutrosophic SuperHyperVertices. Consider some neutrosophic numbers of those neutrosophic SuperHyperVertices from that neutrosophic SuperHyperEdge excluding three distinct neutrosophic SuperHyperVertices, belong to any given neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices. Consider there’s an 1-failed neutrosophic SuperHyperForcing with the most neutrosophic cardinality, the upper sharp bound for neutrosophic cardinality. The neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices is a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex but it isn’t an 1-failed neutrosophic SuperHyperForcing. Since it doesn’t have the maximum neutrosophic cardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex. The neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices is the maximum neutrosophic cardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) but it isn’t an 1-failed neutrosophic SuperHyperForcing. Since it doesn’t do the procedure such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex [there’s at least one white without any white neutrosophic SuperHyperNeighbor outside implying there’s, by the connectedness of the connected neutrosophic neutrosophic SuperHyperGraph a neutrosophic SuperHyperVertex, titled its neutrosophic SuperHyperNeighbor, to the neutrosophic SuperHyperSet S does the “the color-change rule”.]. There’re only two neutrosophic SuperHyperVertices outside the intended neutrosophic SuperHyperSet, Thus the obvious 1-failed neutrosophic SuperHyperForcing, is up. The obvious simple type-neutrosophic SuperHyperSet of the 1-failed neutrosophic SuperHyperForcing, is a neutrosophic SuperHyperSet, excludes only two neutrosophic SuperHyperVertices are titled in a connected neutrosophic neutrosophic SuperHyperNeighbors neutrosophic SuperHyperGraph Since the neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices is the maximum neutrosophic cardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex. It implies that neutrosophic number of 1-failed neutrosophic SuperHyperForcing has, the most neutrosophic cardinality, the upper sharp bound for neutrosophic cardinality, is Thus it induces that the neutrosophic number of 1-failed neutrosophic SuperHyperForcing has, the most neutrosophic cardinality, the upper sharp bound for neutrosophic cardinality, is the neutrosophic cardinality of if there’s an 1-failed neutrosophic SuperHyperForcing with the most neutrosophic cardinality, the upper sharp bound for neutrosophic cardinality. Thus if a neutrosophic SuperHyperEdge has some neutrosophic SuperHyperVertices, then, with excluding two distinct neutrosophic SuperHyperVertices, the all neutrosophic number of those neutrosophic SuperHyperVertices from that neutrosophic SuperHyperEdge belong to any 1-failed neutrosophic SuperHyperForcing. Thus, in a connected neutrosophic neutrosophic SuperHyperGraph there’s a neutrosophic SuperHyperEdge has only two distinct neutrosophic SuperHyperVertices outside of 1-failed neutrosophic SuperHyperForcing. In other words, here’s a neutrosophic SuperHyperEdge has only two distinct white neutrosophic SuperHyperVertices. In a connected neutrosophic neutrosophic SuperHyperGraph the all exterior neutrosophic SuperHyperVertices belong to any 1-failed neutrosophic SuperHyperForcing if there’s one of them such that there are only two interior neutrosophic SuperHyperVertices are mutually neutrosophic SuperHyperNeighbors. Then an 1-failed neutrosophic SuperHyperForcing is a neutrosophic SuperHyperSet of the exterior neutrosophic SuperHyperVertices and the interior neutrosophic SuperHyperVertices with only two exceptions in the form of interior neutrosophic SuperHyperVertices from same neutrosophic SuperHyperEdge. An 1-failed neutrosophic SuperHyperForcing has the neutrosophic number of the neutrosophic cardinality of the first neutrosophic SuperHyperPart minus one plus the second neutrosophic SuperHyperPart minus one. Thus,
Where is the unary operation on the SuperHyperVertices of the SuperHyperGraph to assign the determinacy, the indeterminacy and the neutrality, for respectively. □
Example 40.
In the Figure (24), the connected neutrosophic SuperHyperBipartite is highlighted and featured.

Figure 24.
A neutrosophic SuperHyperBipartite Associated to the Notions of 1-failed neutrosophic SuperHyperForcing in the Example (40).
Figure 24.
A neutrosophic SuperHyperBipartite Associated to the Notions of 1-failed neutrosophic SuperHyperForcing in the Example (40).

By using the Figure (24) and the Table (7), the neutrosophic SuperHyperBipartite is obtained.
The obtained neutrosophic SuperHyperSet, by the Algorithm in previous result, of the neutrosophic SuperHyperVertices of the connected neutrosophic SuperHyperBipartite in the neutrosophic SuperHyperModel (24), is the 1-failed neutrosophic SuperHyperForcing.
Table 7.
The Values of Vertices, SuperVertices, Edges, HyperEdges, and SuperHyperEdges Belong to The Neutrosophic SuperHyperBipartite Mentioned in the Example (40)
Table 7.
The Values of Vertices, SuperVertices, Edges, HyperEdges, and SuperHyperEdges Belong to The Neutrosophic SuperHyperBipartite Mentioned in the Example (40)
| The Values of The Vertices | The Number of Position in Alphabet |
| The Values of The SuperVertices | The maximum Values of Its Vertices |
| The Values of The Edges | The maximum Values of Its Vertices |
| The Values of The HyperEdges | The maximum Values of Its Vertices |
| The Values of The SuperHyperEdges | The maximum Values of Its Endpoints |
Proposition 41.
Assume a connected neutrosophic SuperHyperMultipartite Then an 1-failed neutrosophic SuperHyperForcing is a neutrosophic SuperHyperSet of the exterior neutrosophic SuperHyperVertices and the interior neutrosophic SuperHyperVertices with only one exception in the form of interior neutrosophic SuperHyperVertices from a neutrosophic SuperHyperPart and only one exception in the form of interior neutrosophic SuperHyperVertices from another neutrosophic SuperHyperPart. An 1-failed neutrosophic SuperHyperForcing has the neutrosophic number of all the summation on the neutrosophic cardinality of the all neutrosophic SuperHyperParts minus two excerpt distinct neutrosophic SuperHyperParts. Thus,
where is the unary operation on the SuperHyperVertices of the SuperHyperGraph to assign the determinacy, the indeterminacy and the neutrality, for respectively.
Proof.
Assume a connected neutrosophic SuperHyperMultipartite Let a neutrosophic SuperHyperEdge has some neutrosophic SuperHyperVertices. Consider some neutrosophic numbers of those neutrosophic SuperHyperVertices from that neutrosophic SuperHyperEdge excluding three distinct neutrosophic SuperHyperVertices, belong to any given neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices. Consider there’s an 1-failed neutrosophic SuperHyperForcing with the most neutrosophic cardinality, the upper sharp bound for neutrosophic cardinality. The neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices is a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex but it isn’t an 1-failed neutrosophic SuperHyperForcing. Since it doesn’t have the maximum neutrosophic cardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex. The neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices is the maximum neutrosophic cardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) but it isn’t an 1-failed neutrosophic SuperHyperForcing. Since it doesn’t do the procedure such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex [there’s at least one white without any white neutrosophic SuperHyperNeighbor outside implying there’s, by the connectedness of the connected neutrosophic neutrosophic SuperHyperGraph a neutrosophic SuperHyperVertex, titled its neutrosophic SuperHyperNeighbor, to the neutrosophic SuperHyperSet S does the “the color-change rule”.]. There’re only two neutrosophic SuperHyperVertices outside the intended neutrosophic SuperHyperSet, Thus the obvious 1-failed neutrosophic SuperHyperForcing, is up. The obvious simple type-neutrosophic SuperHyperSet of the 1-failed neutrosophic SuperHyperForcing, is a neutrosophic SuperHyperSet, excludes only two neutrosophic SuperHyperVertices are titled in a connected neutrosophic neutrosophic SuperHyperNeighbors neutrosophic SuperHyperGraph Since the neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices is the maximum neutrosophic cardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex. It implies that neutrosophic number of 1-failed neutrosophic SuperHyperForcing has, the most neutrosophic cardinality, the upper sharp bound for neutrosophic cardinality, is Thus it induces that the neutrosophic number of 1-failed neutrosophic SuperHyperForcing has, the most neutrosophic cardinality, the upper sharp bound for neutrosophic cardinality, is the neutrosophic cardinality of if there’s an 1-failed neutrosophic SuperHyperForcing with the most neutrosophic cardinality, the upper sharp bound for neutrosophic cardinality. Thus if a neutrosophic SuperHyperEdge has some neutrosophic SuperHyperVertices, then, with excluding two distinct neutrosophic SuperHyperVertices, the all neutrosophic number of those neutrosophic SuperHyperVertices from that neutrosophic SuperHyperEdge belong to any 1-failed neutrosophic SuperHyperForcing. Thus, in a connected neutrosophic neutrosophic SuperHyperGraph there’s a neutrosophic SuperHyperEdge has only two distinct neutrosophic SuperHyperVertices outside of 1-failed neutrosophic SuperHyperForcing. In other words, here’s a neutrosophic SuperHyperEdge has only two distinct white neutrosophic SuperHyperVertices. In a connected neutrosophic neutrosophic SuperHyperGraph the all exterior neutrosophic SuperHyperVertices belong to any 1-failed neutrosophic SuperHyperForcing if there’s one of them such that there are only two interior neutrosophic SuperHyperVertices are mutually neutrosophic SuperHyperNeighbors. Then an 1-failed neutrosophic SuperHyperForcing is a neutrosophic SuperHyperSet of the exterior neutrosophic SuperHyperVertices and the interior neutrosophic SuperHyperVertices with only one exception in the form of interior neutrosophic SuperHyperVertices from a neutrosophic SuperHyperPart and only one exception in the form of interior neutrosophic SuperHyperVertices from another neutrosophic SuperHyperPart. An 1-failed neutrosophic SuperHyperForcing has the neutrosophic number of all the summation on the neutrosophic cardinality of the all neutrosophic SuperHyperParts minus two excerpt distinct neutrosophic SuperHyperParts. Thus,
where is the unary operation on the SuperHyperVertices of the SuperHyperGraph to assign the determinacy, the indeterminacy and the neutrality, for respectively. □
Example 42.
In the Figure (25), the connected neutrosophic SuperHyperMultipartite is highlighted and featured.
By using the Figure (25) and the Table (8), the neutrosophic SuperHyperMultipartite is obtained.
The obtained neutrosophic SuperHyperSet, by the Algorithm in previous result, of the neutrosophic SuperHyperVertices of the connected neutrosophic SuperHyperMultipartite in the neutrosophic SuperHyperModel (25), is the 1-failed neutrosophic SuperHyperForcing.
Table 8.
The Values of Vertices, SuperVertices, Edges, HyperEdges, and SuperHyperEdges Belong to The Neutrosophic SuperHyperMultipartite Mentioned in the Example (42)
Table 8.
The Values of Vertices, SuperVertices, Edges, HyperEdges, and SuperHyperEdges Belong to The Neutrosophic SuperHyperMultipartite Mentioned in the Example (42)
| The Values of The Vertices | The Number of Position in Alphabet |
| The Values of The SuperVertices | The maximum Values of Its Vertices |
| The Values of The Edges | The maximum Values of Its Vertices |
| The Values of The HyperEdges | The maximum Values of Its Vertices |
| The Values of The SuperHyperEdges | The maximum Values of Its Endpoints |
Proposition 43.
Assume a connected neutrosophic SuperHyperWheel Then an 1-failed neutrosophic SuperHyperForcing is a neutrosophic SuperHyperSet of the exterior neutrosophic SuperHyperVertices and the interior neutrosophic SuperHyperVertices, excluding the neutrosophic SuperHyperCenter, with only one exception in the form of interior neutrosophic SuperHyperVertices from any given neutrosophic SuperHyperEdge. An 1-failed neutrosophic SuperHyperForcing has the neutrosophic number of all the neutrosophic number of all the neutrosophic SuperHyperEdges minus two neutrosophic numbers excerpt two neutrosophic SuperHyperNeighbors. Thus,
Where is the unary operation on the SuperHyperVertices of the SuperHyperGraph to assign the determinacy, the indeterminacy and the neutrality, for respectively.
Proof.
Assume a connected neutrosophic SuperHyperWheel Let a neutrosophic SuperHyperEdge has some neutrosophic SuperHyperVertices. Consider some neutrosophic numbers of those neutrosophic SuperHyperVertices from that neutrosophic SuperHyperEdge excluding three distinct neutrosophic SuperHyperVertices, belong to any given neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices. Consider there’s an 1-failed neutrosophic SuperHyperForcing with the most neutrosophic cardinality, the upper sharp bound for neutrosophic cardinality. The neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices is a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex but it isn’t an 1-failed neutrosophic SuperHyperForcing. Since it doesn’t have the maximum neutrosophic cardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex. The neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices is the maximum neutrosophic cardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) but it isn’t an 1-failed neutrosophic SuperHyperForcing. Since it doesn’t do the procedure such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex [there’s at least one white without any white neutrosophic SuperHyperNeighbor outside implying there’s, by the connectedness of the connected neutrosophic neutrosophic SuperHyperGraph a neutrosophic SuperHyperVertex, titled its neutrosophic SuperHyperNeighbor, to the neutrosophic SuperHyperSet S does the “the color-change rule”.]. There’re only two neutrosophic SuperHyperVertices outside the intended neutrosophic SuperHyperSet, Thus the obvious 1-failed neutrosophic SuperHyperForcing, is up. The obvious simple type-neutrosophic SuperHyperSet of the 1-failed neutrosophic SuperHyperForcing, is a neutrosophic SuperHyperSet, excludes only two neutrosophic SuperHyperVertices are titled in a connected neutrosophic neutrosophic SuperHyperNeighbors neutrosophic SuperHyperGraph Since the neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices is the maximum neutrosophic cardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that isn’t turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex with the additional condition is referred by “1-” about the usage of any black neutrosophic SuperHyperVertex only once to act on white neutrosophic SuperHyperVertex to be black neutrosophic SuperHyperVertex. It implies that neutrosophic number of 1-failed neutrosophic SuperHyperForcing has, the most neutrosophic cardinality, the upper sharp bound for neutrosophic cardinality, is Thus it induces that the neutrosophic number of 1-failed neutrosophic SuperHyperForcing has, the most neutrosophic cardinality, the upper sharp bound for neutrosophic cardinality, is the neutrosophic cardinality of if there’s an 1-failed neutrosophic SuperHyperForcing with the most neutrosophic cardinality, the upper sharp bound for neutrosophic cardinality. Thus if a neutrosophic SuperHyperEdge has some neutrosophic SuperHyperVertices, then, with excluding two distinct neutrosophic SuperHyperVertices, the all neutrosophic number of those neutrosophic SuperHyperVertices from that neutrosophic SuperHyperEdge belong to any 1-failed neutrosophic SuperHyperForcing. Thus, in a connected neutrosophic neutrosophic SuperHyperGraph there’s a neutrosophic SuperHyperEdge has only two distinct neutrosophic SuperHyperVertices outside of 1-failed neutrosophic SuperHyperForcing. In other words, here’s a neutrosophic SuperHyperEdge has only two distinct white neutrosophic SuperHyperVertices. In a connected neutrosophic neutrosophic SuperHyperGraph the all exterior neutrosophic SuperHyperVertices belong to any 1-failed neutrosophic SuperHyperForcing if there’s one of them such that there are only two interior neutrosophic SuperHyperVertices are mutually neutrosophic SuperHyperNeighbors. Then an 1-failed neutrosophic SuperHyperForcing is a neutrosophic SuperHyperSet of the exterior neutrosophic SuperHyperVertices and the interior neutrosophic SuperHyperVertices, excluding the neutrosophic SuperHyperCenter, with only one exception in the form of interior neutrosophic SuperHyperVertices from any given neutrosophic SuperHyperEdge. An 1-failed neutrosophic SuperHyperForcing has the neutrosophic number of all the neutrosophic number of all the neutrosophic SuperHyperEdges minus two neutrosophic numbers excerpt two neutrosophic SuperHyperNeighbors. Thus,
where is the unary operation on the SuperHyperVertices of the SuperHyperGraph to assign the determinacy, the indeterminacy and the neutrality, for respectively. □
Example 44.
In the Figure (26), the connected neutrosophic SuperHyperWheel is highlighted and featured.
By using the Figure (26) and the Table (9), the neutrosophic SuperHyperWheel is obtained.
The obtained neutrosophic SuperHyperSet, by the Algorithm in previous result, of the neutrosophic SuperHyperVertices of the connected neutrosophic SuperHyperWheel in the neutrosophic SuperHyperModel (26), is the 1-failed neutrosophic SuperHyperForcing.
Table 9.
The Values of Vertices, SuperVertices, Edges, HyperEdges, and SuperHyperEdges Belong to The Neutrosophic SuperHyperWheel Mentioned in the Example (44).
Table 9.
The Values of Vertices, SuperVertices, Edges, HyperEdges, and SuperHyperEdges Belong to The Neutrosophic SuperHyperWheel Mentioned in the Example (44).
| The Values of The Vertices | The Number of Position in Alphabet |
| The Values of The SuperVertices | The maximum Values of Its Vertices |
| The Values of The Edges | The maximum Values of Its Vertices |
| The Values of The HyperEdges | The maximum Values of Its Vertices |
| The Values of The SuperHyperEdges | The maximum Values of Its Endpoints |
4. General Results
For the 1-failed neutrosophic SuperHyperForcing, and the neutrosophic 1-failed neutrosophic SuperHyperForcing, some general results are introduced.
Remark 45.
Let remind that the neutrosophic 1-failed neutrosophic SuperHyperForcing is “redefined” on the positions of the alphabets.
Corollary 46.
Assume 1-failed neutrosophic SuperHyperForcing. Then
where is the unary operation on the neutrosophic SuperHyperVertices of the neutrosophic SuperHyperGraph to assign the determinacy, the indeterminacy and the neutrality, for respectively.
Corollary 47.
Assume a neutrosophic SuperHyperGraph on the same identical letter of the alphabet. Then the notion of neutrosophic 1-failed neutrosophic SuperHyperForcing and 1-failed neutrosophic SuperHyperForcing coincide.
Corollary 48.
Assume a neutrosophic SuperHyperGraph on the same identical letter of the alphabet. Then a consecutive sequence of the neutrosophic SuperHyperVertices is a neutrosophic 1-failed neutrosophic SuperHyperForcing if and only if it’s an 1-failed neutrosophic SuperHyperForcing.
Corollary 49.
Assume a neutrosophic SuperHyperGraph on the same identical letter of the alphabet. Then a consecutive sequence of the neutrosophic SuperHyperVertices is a strongest neutrosophic SuperHyperCycle if and only if it’s a longest neutrosophic SuperHyperCycle.
Corollary 50.
Assume neutrosophic SuperHyperClasses of a neutrosophic SuperHyperGraph on the same identical letter of the alphabet. Then its neutrosophic 1-failed neutrosophic SuperHyperForcing is its 1-failed neutrosophic SuperHyperForcing and reversely.
Corollary 51.
Assume a neutrosophic SuperHyperPath(-/neutrosophic SuperHyperCycle, neutrosophic SuperHyperStar, neutrosophic SuperHyperBipartite, neutrosophic SuperHyperMultipartite, neutrosophic SuperHyperWheel) on the same identical letter of the alphabet. Then its neutrosophic 1-failed neutrosophic SuperHyperForcing is its 1-failed neutrosophic SuperHyperForcing and reversely.
Corollary 52.
Assume a neutrosophic SuperHyperGraph. Then its neutrosophic 1-failed neutrosophic SuperHyperForcing isn’t well-defined if and only if its 1-failed neutrosophic SuperHyperForcing isn’t well-defined.
Corollary 53.
Assume neutrosophic SuperHyperClasses of a neutrosophic SuperHyperGraph. Then its neutrosophic 1-failed neutrosophic SuperHyperForcing isn’t well-defined if and only if its 1-failed neutrosophic SuperHyperForcing isn’t well-defined.
Corollary 54.
Assume a neutrosophic SuperHyperPath(-/neutrosophic SuperHyperCycle, neutrosophic SuperHyperStar, neutrosophic SuperHyperBipartite, neutrosophic SuperHyperMultipartite, neutrosophic SuperHyperWheel). Then its neutrosophic 1-failed neutrosophic SuperHyperForcing isn’t well-defined if and only if its 1-failed neutrosophic SuperHyperForcing isn’t well-defined.
Corollary 55.
Assume a neutrosophic SuperHyperGraph. Then its neutrosophic 1-failed neutrosophic SuperHyperForcing is well-defined if and only if its 1-failed neutrosophic SuperHyperForcing is well-defined.
Corollary 56.
Assume neutrosophic SuperHyperClasses of a neutrosophic SuperHyperGraph. Then its neutrosophic 1-failed neutrosophic SuperHyperForcing is well-defined if and only if its 1-failed neutrosophic SuperHyperForcing is well-defined.
Corollary 57.
Assume a neutrosophic SuperHyperPath(-/neutrosophic SuperHyperCycle, neutrosophic SuperHyperStar, neutrosophic SuperHyperBipartite, neutrosophic SuperHyperMultipartite, neutrosophic SuperHyperWheel). Then its neutrosophic 1-failed neutrosophic SuperHyperForcing is well-defined if and only if its 1-failed neutrosophic SuperHyperForcing is well-defined.
Proposition 58.
Let be a neutrosophic SuperHyperGraph. Then V is
- the dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- the strong dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- the connected dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- the δ-dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- the strong δ-dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- the connected δ-dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing.
Proof.
Suppose is a neutrosophic SuperHyperGraph. Consider All neutrosophic SuperHyperMembers of V have at least one neutrosophic SuperHyperNeighbor inside the neutrosophic SuperHyperSet more than neutrosophic SuperHyperNeighbor out of neutrosophic SuperHyperSet. Thus,
V is the dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing since the following statements are equivalent.
V is the strong dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing since the following statements are equivalent.
V is the connected dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing since the following statements are equivalent.
V is the -dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing since the following statements are equivalent.
V is the strong -dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing since the following statements are equivalent.
V is connected -dual 1-failed neutrosophic SuperHyperForcing since the following statements are equivalent.
□
Proposition 59.
Let be a neutrosophic SuperHyperGraph. Then ∅ is
- the neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- the strong neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- the connected defensive neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- the δ-neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- the strong δ-neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- the connected δ-neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing.
Proof.
Suppose is a neutrosophic SuperHyperGraph. Consider All neutrosophic SuperHyperMembers of ∅ have no neutrosophic SuperHyperNeighbor inside the neutrosophic SuperHyperSet less than neutrosophic SuperHyperNeighbor out of neutrosophic SuperHyperSet. Thus,
∅ is the neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing since the following statements are equivalent.
∅ is the strong neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing since the following statements are equivalent.
∅ is the connected neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing since the following statements are equivalent.
∅ is the -neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing since the following statements are equivalent.
∅ is the strong -neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing since the following statements are equivalent.
∅ is the connected -neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing since the following statements are equivalent.
□
Proposition 60.
Let be a neutrosophic SuperHyperGraph. Then an independent neutrosophic SuperHyperSet is
- the neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- the strong neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- the connected neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- the δ-neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- the strong δ-neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- the connected δ-neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing.
Proof.
Suppose is a neutrosophic SuperHyperGraph. Consider All neutrosophic SuperHyperMembers of S have no neutrosophic SuperHyperNeighbor inside the neutrosophic SuperHyperSet less than neutrosophic SuperHyperNeighbor out of neutrosophic SuperHyperSet. Thus,
An independent neutrosophic SuperHyperSet is the neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing since the following statements are equivalent.
An independent neutrosophic SuperHyperSet is the strong neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing since the following statements are equivalent.
An independent neutrosophic SuperHyperSet is the connected neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing since the following statements are equivalent.
An independent neutrosophic SuperHyperSet is the -neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing since the following statements are equivalent.
An independent neutrosophic SuperHyperSet is the strong -neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing since the following statements are equivalent.
An independent neutrosophic SuperHyperSet is the connected -neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing since the following statements are equivalent.
□
Proposition 61.
Let be a neutrosophic SuperHyperUniform neutrosophic SuperHyperGraph which is a neutrosophic SuperHyperCycle/neutrosophic SuperHyperPath. Then V is a maximal
- neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- strong neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- connected neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- -neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- strong -neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- connected -neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
Where the exterior neutrosophic SuperHyperVertices and the interior neutrosophic SuperHyperVertices coincide.
Proof.
Suppose is a neutrosophic SuperHyperGraph which is a neutrosophic SuperHyperUniform neutrosophic SuperHyperCycle/neutrosophic SuperHyperPath.
Consider one segment is out of S which is neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. This segment has neutrosophic SuperHyperNeighbors in S, i.e, Suppose such that By it’s the exterior neutrosophic SuperHyperVertices and the interior neutrosophic SuperHyperVertices coincide and it’s neutrosophic SuperHyperUniform neutrosophic SuperHyperCycle, Thus
Thus it’s contradiction. It implies every isn’t neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing in a given neutrosophic SuperHyperUniform neutrosophic SuperHyperCycle.
Consider one segment, with two segments related to the neutrosophic SuperHyperLeaves as exceptions, is out of S which is neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. This segment has neutrosophic SuperHyperNeighbors in S, i.e, Suppose such that By it’s the exterior neutrosophic SuperHyperVertices and the interior neutrosophic SuperHyperVertices coincide and it’s neutrosophic SuperHyperUniform neutrosophic SuperHyperPath, Thus
Thus it’s contradiction. It implies every isn’t neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing in a given neutrosophic SuperHyperUniform neutrosophic SuperHyperPath.
are obvious by
By is maximal and it’s a neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. Thus it’s -neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing.
are obvious by □
Proposition 62.
Let be a neutrosophic SuperHyperGraph which is a neutrosophic SuperHyperUniform neutrosophic SuperHyperWheel. Then V is a maximal
- dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- strong dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- connected dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- -dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- strong -dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- connected -dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
Where the exterior neutrosophic SuperHyperVertices and the interior neutrosophic SuperHyperVertices coincide.
Proof.
Suppose is a neutrosophic SuperHyperUniform neutrosophic SuperHyperGraph which is a neutrosophic SuperHyperWheel.
Consider one segment is out of S which is neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. This segment has neutrosophic SuperHyperNeighbors in S, i.e, Suppose such that By it’s the exterior neutrosophic SuperHyperVertices and the interior neutrosophic SuperHyperVertices coincide and it’s neutrosophic SuperHyperUniform neutrosophic SuperHyperWheel, Thus
Thus it’s contradiction. It implies every is neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing in a given neutrosophic SuperHyperUniform neutrosophic SuperHyperWheel.
are obvious by
By is maximal and it is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. Thus it’s a dual -neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing.
are obvious by □
Proposition 63.
Let be a neutrosophic SuperHyperUniform neutrosophic SuperHyperGraph which is a neutrosophic SuperHyperCycle/neutrosophic SuperHyperPath. Then the number of
- the 1-failed neutrosophic SuperHyperForcing;
- the 1-failed neutrosophic SuperHyperForcing;
- the connected 1-failed neutrosophic SuperHyperForcing;
- the -1-failed neutrosophic SuperHyperForcing;
- the strong -1-failed neutrosophic SuperHyperForcing;
- the connected -1-failed neutrosophic SuperHyperForcing.
is one and it’s only
Where the exterior neutrosophic SuperHyperVertices and the interior neutrosophic SuperHyperVertices coincide.
Proof.
Suppose is a neutrosophic SuperHyperGraph which is a neutrosophic SuperHyperUniform neutrosophic SuperHyperCycle/neutrosophic SuperHyperPath.
Consider one segment is out of S which is neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. This segment has neutrosophic SuperHyperNeighbors in S, i.e, Suppose such that By it’s the exterior neutrosophic SuperHyperVertices and the interior neutrosophic SuperHyperVertices coincide and it’s neutrosophic SuperHyperUniform neutrosophic SuperHyperCycle, Thus
Thus it’s contradiction. It implies every isn’t neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing in a given neutrosophic SuperHyperUniform neutrosophic SuperHyperCycle.
Consider one segment, with two segments related to the neutrosophic SuperHyperLeaves as exceptions, is out of S which is neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. This segment has neutrosophic SuperHyperNeighbors in S, i.e, Suppose such that By it’s the exterior neutrosophic SuperHyperVertices and the interior neutrosophic SuperHyperVertices coincide and it’s neutrosophic SuperHyperUniform neutrosophic SuperHyperPath, Thus
Thus it’s contradiction. It implies every isn’t neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing in a given neutrosophic SuperHyperUniform neutrosophic SuperHyperPath.
are obvious by
By is maximal and it’s a neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. Thus it’s -neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing.
are obvious by □
Proposition 64.
Let be a neutrosophic SuperHyperUniform neutrosophic SuperHyperGraph which is a neutrosophic SuperHyperWheel. Then the number of
- the dual 1-failed neutrosophic SuperHyperForcing;
- the dual 1-failed neutrosophic SuperHyperForcing;
- the dual connected 1-failed neutrosophic SuperHyperForcing;
- the dual -1-failed neutrosophic SuperHyperForcing;
- the strong dual -1-failed neutrosophic SuperHyperForcing;
- the connected dual -1-failed neutrosophic SuperHyperForcing.
is one and it’s only
Where the exterior neutrosophic SuperHyperVertices and the interior neutrosophic SuperHyperVertices coincide.
Proof.
Suppose is a neutrosophic SuperHyperUniform neutrosophic SuperHyperGraph which is a neutrosophic SuperHyperWheel.
Consider one segment is out of S which is neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. This segment has neutrosophic SuperHyperNeighbors in S, i.e, Suppose such that By it’s the exterior neutrosophic SuperHyperVertices and the interior neutrosophic SuperHyperVertices coincide and it’s neutrosophic SuperHyperUniform neutrosophic SuperHyperWheel, Thus
Thus it’s contradiction. It implies every isn’t a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing in a given neutrosophic SuperHyperUniform neutrosophic SuperHyperWheel.
are obvious by
By is maximal and it’s a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. Thus it isn’t an -neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing.
are obvious by □
Proposition 65.
Let be a neutrosophic SuperHyperUniform neutrosophic SuperHyperGraph which is a neutrosophic SuperHyperStar/neutrosophic SuperHyperComplete neutrosophic SuperHyperBipartite/neutrosophic SuperHyperComplete neutrosophic SuperHyperMultipartite. Then a neutrosophic SuperHyperSet contains [the neutrosophic SuperHyperCenter and] the half of multiplying r with the number of all the neutrosophic SuperHyperEdges plus one of all the neutrosophic SuperHyperVertices is a
- dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- strong dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- connected dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- -dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- strong -dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- connected -dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing.
Proof. Consider n half neutrosophic SuperHyperVertices are in S which is neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. A neutrosophic SuperHyperVertex has either or one neutrosophic SuperHyperNeighbors in If the neutrosophic SuperHyperVertex is non-neutrosophic SuperHyperCenter, then
If the neutrosophic SuperHyperVertex is neutrosophic SuperHyperCenter, then
Thus it’s proved. It implies every S is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing in a given neutrosophic SuperHyperStar.
Consider n half neutrosophic SuperHyperVertices are in S which is neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. A neutrosophic SuperHyperVertex has at most neutrosophic SuperHyperNeighbors in
Thus it’s proved. It implies every S is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing in a given neutrosophic SuperHyperComplete neutrosophic SuperHyperBipartite which isn’t a neutrosophic SuperHyperStar.
Consider n half neutrosophic SuperHyperVertices are in S which is neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing and they’re chosen from different neutrosophic SuperHyperParts, equally or almost equally as possible. A neutrosophic SuperHyperVertex has at most neutrosophic SuperHyperNeighbors in
Thus it’s proved. It implies every S is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing in a given neutrosophic SuperHyperComplete neutrosophic SuperHyperMultipartite which is neither a neutrosophic SuperHyperStar nor neutrosophic SuperHyperComplete neutrosophic SuperHyperBipartite.
are obvious by
By is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. Thus it’s -dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing.
are obvious by □
Proposition 66.
Let be a neutrosophic SuperHyperUniform neutrosophic SuperHyperGraph which is a neutrosophic SuperHyperStar/neutrosophic SuperHyperComplete neutrosophic SuperHyperBipartite/neutrosophic SuperHyperComplete neutrosophic SuperHyperMultipartite. Then a neutrosophic SuperHyperSet contains the half of multiplying r with the number of all the neutrosophic SuperHyperEdges plus one of all the neutrosophic SuperHyperVertices in the biggest neutrosophic SuperHyperPart is a
- neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- strong neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- connected neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- δ-neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- strong δ-neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- connected δ-neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing.
Proof. Consider the half of multiplying r with the number of all the neutrosophic SuperHyperEdges plus one of all the neutrosophic SuperHyperVertices in the biggest neutrosophic SuperHyperPart are in S which is neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. A neutrosophic SuperHyperVertex has either or zero neutrosophic SuperHyperNeighbors in If the neutrosophic SuperHyperVertex is in then
Thus it’s proved. It implies every S is a neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing in a given neutrosophic SuperHyperStar.
Consider the half of multiplying r with the number of all the neutrosophic SuperHyperEdges plus one of all the neutrosophic SuperHyperVertices in the biggest neutrosophic SuperHyperPart are in S which is neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. A neutrosophic SuperHyperVertex has no neutrosophic SuperHyperNeighbor in
Thus it’s proved. It implies every S is a neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing in a given neutrosophic SuperHyperComplete neutrosophic SuperHyperBipartite which isn’t a neutrosophic SuperHyperStar.
Consider the half of multiplying r with the number of all the neutrosophic SuperHyperEdges plus one of all the neutrosophic SuperHyperVertices in the biggest neutrosophic SuperHyperPart are in S which is neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. A neutrosophic SuperHyperVertex has no neutrosophic SuperHyperNeighbor in
Thus it’s proved. It implies every S is a neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing in a given neutrosophic SuperHyperComplete neutrosophic SuperHyperMultipartite which is neither a neutrosophic SuperHyperStar nor neutrosophic SuperHyperComplete neutrosophic SuperHyperBipartite.
are obvious by
By S is a neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. Thus it’s an -neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing.
are obvious by □
Proposition 67.
Let be a neutrosophic SuperHyperUniform neutrosophic SuperHyperGraph which is a neutrosophic SuperHyperStar/neutrosophic SuperHyperComplete neutrosophic SuperHyperBipartite/neutrosophic SuperHyperComplete neutrosophic SuperHyperMultipartite. Then Then the number of
- dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- strong dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- connected dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- -dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- strong -dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- connected -dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing.
is one and it’s only a neutrosophic SuperHyperSet contains [the neutrosophic SuperHyperCenter and] the half of multiplying r with the number of all the neutrosophic SuperHyperEdges plus one of all the neutrosophic SuperHyperVertices. Where the exterior neutrosophic SuperHyperVertices and the interior neutrosophic SuperHyperVertices coincide.
Proof. Consider n half neutrosophic SuperHyperVertices are in S which is neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. A neutrosophic SuperHyperVertex has either or one neutrosophic SuperHyperNeighbors in If the neutrosophic SuperHyperVertex is non-neutrosophic SuperHyperCenter, then
If the neutrosophic SuperHyperVertex is neutrosophic SuperHyperCenter, then
Thus it’s proved. It implies every S is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing in a given neutrosophic SuperHyperStar.
Consider n half neutrosophic SuperHyperVertices are in S which is neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. A neutrosophic SuperHyperVertex has at most neutrosophic SuperHyperNeighbors in
Thus it’s proved. It implies every S is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing in a given neutrosophic SuperHyperComplete neutrosophic SuperHyperBipartite which isn’t a neutrosophic SuperHyperStar.
Consider n half neutrosophic SuperHyperVertices are in S which is neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing and they’re chosen from different neutrosophic SuperHyperParts, equally or almost equally as possible. A neutrosophic SuperHyperVertex has at most neutrosophic SuperHyperNeighbors in
Thus it’s proved. It implies every S is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing in a given neutrosophic SuperHyperComplete neutrosophic SuperHyperMultipartite which is neither a neutrosophic SuperHyperStar nor neutrosophic SuperHyperComplete neutrosophic SuperHyperBipartite.
are obvious by
By is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. Thus it’s -dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing.
are obvious by □
Proposition 68.
Let be a neutrosophic SuperHyperGraph. The number of connected component is if there’s a neutrosophic SuperHyperSet which is a dual
- neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- strong neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- connected neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- 1-failed neutrosophic SuperHyperForcing;
- strong 1-neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- connected 1-neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing.
Proof. Consider some neutrosophic SuperHyperVertices are out of S which is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. These neutrosophic SuperHyperVertex-type have some neutrosophic SuperHyperNeighbors in S but no neutrosophic SuperHyperNeighbor out of Thus
Thus it’s proved. It implies every S is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing and number of connected component is
are obvious by
By S is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. Thus it’s a dual 1-neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing.
are obvious by □
Proposition 69.
Let be a neutrosophic SuperHyperGraph. Then the number is at most and the neutrosophic number is at most
Proof.
Suppose is a neutrosophic SuperHyperGraph. Consider All neutrosophic SuperHyperMembers of V have at least one neutrosophic SuperHyperNeighbor inside the neutrosophic SuperHyperSet more than neutrosophic SuperHyperNeighbor out of neutrosophic SuperHyperSet. Thus,
V is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing since the following statements are equivalent.
V is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing since the following statements are equivalent.
V is connected a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing since the following statements are equivalent.
V is a dual -neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing since the following statements are equivalent.
V is a dual strong -neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing since the following statements are equivalent.
V is a dual connected -neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing since the following statements are equivalent.
Thus V is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing and V is the biggest neutrosophic SuperHyperSet in Then the number is at most and the neutrosophic number is at most □
Proposition 70.
Let be a neutrosophic SuperHyperGraph which is neutrosophic SuperHyperComplete. The number is and the neutrosophic number is in the setting of dual
- neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- strong neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- connected neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- -neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- strong -neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- connected -neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing.
Proof. Consider n half neutrosophic SuperHyperVertices are out of S which is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. A neutrosophic SuperHyperVertex has n half neutrosophic SuperHyperNeighbors in
Thus it’s proved. It implies every S is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing in a given neutrosophic SuperHyperComplete neutrosophic SuperHyperGraph. Thus the number is and the neutrosophic number is in the setting of a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing.
Consider n half neutrosophic SuperHyperVertices are out of S which is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. A neutrosophic SuperHyperVertex has n half neutrosophic SuperHyperNeighbors in
Thus it’s proved. It implies every S is a dual strong neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing in a given neutrosophic SuperHyperComplete neutrosophic SuperHyperGraph. Thus the number is and the neutrosophic number is in the setting of a dual strong neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing.
Consider n half neutrosophic SuperHyperVertices are out of S which is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. A neutrosophic SuperHyperVertex has n half neutrosophic SuperHyperNeighbors in
Thus it’s proved. It implies every S is a dual connected neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing in a given neutrosophic SuperHyperComplete neutrosophic SuperHyperGraph. Thus the number is and the neutrosophic number is in the setting of a dual connected neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing.
Consider n half neutrosophic SuperHyperVertices are out of S which is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. A neutrosophic SuperHyperVertex has n half neutrosophic SuperHyperNeighbors in
Thus it’s proved. It implies every S is a dual -neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing in a given neutrosophic SuperHyperComplete neutrosophic SuperHyperGraph. Thus the number is and the neutrosophic number is in the setting of a dual -neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing.
Consider n half neutrosophic SuperHyperVertices are out of S which is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. A neutrosophic SuperHyperVertex has n half neutrosophic SuperHyperNeighbors in
Thus it’s proved. It implies every S is a dual strong -neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing in a given neutrosophic SuperHyperComplete neutrosophic SuperHyperGraph. Thus the number is and the neutrosophic number is in the setting of a dual strong -neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing.
Consider n half neutrosophic SuperHyperVertices are out of S which is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. A neutrosophic SuperHyperVertex has n half neutrosophic SuperHyperNeighbors in
Thus it’s proved. It implies every S is a dual connected -neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing in a given neutrosophic SuperHyperComplete neutrosophic SuperHyperGraph. Thus the number is and the neutrosophic number is in the setting of a dual connected -neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. □
Proposition 71.
Let be a neutrosophic SuperHyperGraph which is The number is 0 and the neutrosophic number is for an independent neutrosophic SuperHyperSet in the setting of dual
- neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- strong neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- connected neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- 0-neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- strong 0-neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- connected 0-neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing.
Proof.
Suppose is a neutrosophic SuperHyperGraph. Consider All neutrosophic SuperHyperMembers of ∅ have no neutrosophic SuperHyperNeighbor inside the neutrosophic SuperHyperSet less than neutrosophic SuperHyperNeighbor out of neutrosophic SuperHyperSet. Thus,
∅ is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing since the following statements are equivalent.
The number is 0 and the neutrosophic number is for an independent neutrosophic SuperHyperSet in the setting of a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing.
∅ is a dual strong neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing since the following statements are equivalent.
The number is 0 and the neutrosophic number is for an independent neutrosophic SuperHyperSet in the setting of a dual strong neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing.
∅ is a dual connected neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing since the following statements are equivalent.
The number is 0 and the neutrosophic number is for an independent neutrosophic SuperHyperSet in the setting of a dual connected neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing.
∅ is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing since the following statements are equivalent.
The number is 0 and the neutrosophic number is for an independent neutrosophic SuperHyperSet in the setting of a dual 0-neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing.
∅ is a dual strong 0-neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing since the following statements are equivalent.
The number is 0 and the neutrosophic number is for an independent neutrosophic SuperHyperSet in the setting of a dual strong 0-neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing.
∅ is a dual connected neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing since the following statements are equivalent.
The number is 0 and the neutrosophic number is for an independent neutrosophic SuperHyperSet in the setting of a dual connected 0-offensive neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. □
Proposition 72.
Let be a neutrosophic SuperHyperGraph which is neutrosophic SuperHyperComplete. Then there’s no independent neutrosophic SuperHyperSet.
Proposition 73.
Let be a neutrosophic SuperHyperGraph which is neutrosophic SuperHyperCycle/neutrosophic SuperHyperPath/neutrosophic SuperHyperWheel. The number is and the neutrosophic number is in the setting of a dual
- neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- strong neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- connected neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- -neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- strong -neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- connected -neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing.
Proof.
Suppose is a neutrosophic SuperHyperGraph which is neutrosophic SuperHyperCycle/neutrosophic SuperHyperPath/neutrosophic SuperHyperWheel.
Consider one neutrosophic SuperHyperVertex is out of S which is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. This neutrosophic SuperHyperVertex has one neutrosophic SuperHyperNeighbor in S, i.e, suppose such that By it’s neutrosophic SuperHyperCycle, Thus
Thus it’s contradiction. It implies every isn’t a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing in a given neutrosophic SuperHyperCycle.
Consider one neutrosophic SuperHyperVertex is out of S which is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. This neutrosophic SuperHyperVertex has one neutrosophic SuperHyperNeighbor in S, i.e, Suppose such that By it’s neutrosophic SuperHyperPath, Thus
Thus it’s contradiction. It implies every isn’t a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing in a given neutrosophic SuperHyperPath.
Consider one neutrosophic SuperHyperVertex is out of S which is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. This neutrosophic SuperHyperVertex has one neutrosophic SuperHyperNeighbor in S, i.e, Suppose such that By it’s neutrosophic SuperHyperWheel, Thus
Thus it’s contradiction. It implies every isn’t a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing in a given neutrosophic SuperHyperWheel.
are obvious by
By V is maximal and it’s a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. Thus it’s a dual -neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing.
are obvious by
Thus the number is and the neutrosophic number is in the setting of all types of a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. □
Proposition 74.
Let be a neutrosophic SuperHyperGraph which is neutrosophic SuperHyperStar/complete neutrosophic SuperHyperBipartite/complete neutrosophic SuperHyperMultiPartite. The number is and the neutrosophic number is in the setting of a dual
- neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- strong neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- connected neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- -neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- strong -neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- connected -neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing.
Proof. Consider n half neutrosophic SuperHyperVertices are in S which is neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. A neutrosophic SuperHyperVertex has at most n half neutrosophic SuperHyperNeighbors in If the neutrosophic SuperHyperVertex is the non-neutrosophic SuperHyperCenter, then
If the neutrosophic SuperHyperVertex is the neutrosophic SuperHyperCenter, then
Thus it’s proved. It implies every S is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing in a given neutrosophic SuperHyperStar.
Consider n half neutrosophic SuperHyperVertices are in S which is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing.
Thus it’s proved. It implies every S is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing in a given complete neutrosophic SuperHyperBipartite which isn’t a neutrosophic SuperHyperStar.
Consider n half neutrosophic SuperHyperVertices are in S which is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing and they are chosen from different neutrosophic SuperHyperParts, equally or almost equally as possible. A neutrosophic SuperHyperVertex in S has half neutrosophic SuperHyperNeighbors in
Thus it’s proved. It implies every S is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing in a given complete neutrosophic SuperHyperMultipartite which is neither a neutrosophic SuperHyperStar nor complete neutrosophic SuperHyperBipartite.
are obvious by
By is maximal and it’s a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. Thus it’s a dual -neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing.
are obvious by
Thus the number is and the neutrosophic number is in the setting of all dual 1-failed neutrosophic SuperHyperForcing. □
Proposition 75.
Let be a neutrosophic SuperHyperFamily of the neutrosophic SuperHyperGraphs which are from one-type neutrosophic SuperHyperClass which the result is obtained for the individuals. Then the results also hold for the neutrosophic SuperHyperFamily of these specific neutrosophic SuperHyperClasses of the neutrosophic SuperHyperGraphs.
Proof.
There are neither neutrosophic SuperHyperConditions nor neutrosophic SuperHyperRestrictions on the neutrosophic SuperHyperVertices. Thus the neutrosophic SuperHyperResults on individuals, are extended to the neutrosophic SuperHyperResults on neutrosophic SuperHyperFamily, □
Proposition 76.
Let be a strong neutrosophic SuperHyperGraph. If S is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing, then such that
Proof. Suppose is a strong neutrosophic SuperHyperGraph. Consider Since S is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing,
Suppose is a strong neutrosophic SuperHyperGraph. Consider Since S is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing,
□
Proposition 77.
Let be a strong neutrosophic SuperHyperGraph. If S is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing, then
- S is neutrosophic SuperHyperDominating set;
- there’s such that is neutrosophic SuperHyperChromatic number.
Proof. Suppose is a strong neutrosophic SuperHyperGraph. Consider Since S is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing, either
or
It implies S is neutrosophic SuperHyperDominating neutrosophic SuperHyperSet.
Suppose is a strong neutrosophic SuperHyperGraph. Consider Since S is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing, either
or
Thus every neutrosophic SuperHyperVertex has at least one neutrosophic SuperHyperNeighbor in The only case is about the relation amid neutrosophic SuperHyperVertices in S in the terms of neutrosophic SuperHyperNeighbors. It implies there’s such that is neutrosophic SuperHyperChromatic number. □
Proposition 78.
Let be a strong neutrosophic SuperHyperGraph. Then
Proof. Suppose is a strong neutrosophic SuperHyperGraph. Let
It implies V is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. For all neutrosophic SuperHyperSets of neutrosophic SuperHyperVertices Thus for all neutrosophic SuperHyperSets of neutrosophic SuperHyperVertices It implies for all neutrosophic SuperHyperSets of neutrosophic SuperHyperVertices So for all neutrosophic SuperHyperSets of neutrosophic SuperHyperVertices
Suppose is a strong neutrosophic SuperHyperGraph. Let
It implies V is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. For all neutrosophic SuperHyperSets of neutrosophic SuperHyperVertices Thus for all neutrosophic SuperHyperSets of neutrosophic SuperHyperVertices It implies for all neutrosophic SuperHyperSets of neutrosophic SuperHyperVertices So for all neutrosophic SuperHyperSets of neutrosophic SuperHyperVertices □
Proposition 79.
Let be a strong neutrosophic SuperHyperGraph which is connected. Then
Proof. Suppose is a strong neutrosophic SuperHyperGraph. Let where x is arbitrary and
It implies is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. For all neutrosophic SuperHyperSets of neutrosophic SuperHyperVertices Thus for all neutrosophic SuperHyperSets of neutrosophic SuperHyperVertices It implies for all neutrosophic SuperHyperSets of neutrosophic SuperHyperVertices So for all neutrosophic SuperHyperSets of neutrosophic SuperHyperVertices
Suppose is a strong neutrosophic SuperHyperGraph. Let where x is arbitrary and
It implies is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. For all neutrosophic SuperHyperSets of neutrosophic SuperHyperVertices Thus for all neutrosophic SuperHyperSets of neutrosophic SuperHyperVertices It implies for all neutrosophic SuperHyperSets of neutrosophic SuperHyperVertices So for all neutrosophic SuperHyperSets of neutrosophic SuperHyperVertices □
Proposition 80.
Let be an odd neutrosophic SuperHyperPath. Then
- the neutrosophic SuperHyperSet is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- and corresponded neutrosophic SuperHyperSet is ;
- the neutrosophic SuperHyperSets and are only a dual 1-failed neutrosophic SuperHyperForcing.
Proof. Suppose is an odd neutrosophic SuperHyperPath. Let where for all and
It implies is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. If where then
So where isn’t a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. It induces is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing.
and are trivial.
By is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. Thus it’s enough to show that is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. Suppose is an odd neutrosophic SuperHyperPath. Let where for all and
It implies is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. If where then
So where isn’t a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. It induces is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. □
Proposition 81.
Let be an even neutrosophic SuperHyperPath. Then
- the set is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- and corresponded neutrosophic SuperHyperSets are and
- the neutrosophic SuperHyperSets and are only dual 1-failed neutrosophic SuperHyperForcing.
Proof. Suppose is an even neutrosophic SuperHyperPath. Let where for all and
It implies is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. If where then
So where isn’t a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. It induces is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing.
and are trivial.
By is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. Thus it’s enough to show that is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. Suppose is an even neutrosophic SuperHyperPath. Let where for all and
It implies is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. If where then
So where isn’t a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. It induces is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. □
Proposition 82.
Let be an even neutrosophic SuperHyperCycle. Then
- the neutrosophic SuperHyperSet is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- and corresponded neutrosophic SuperHyperSets are and
- the neutrosophic SuperHyperSets and are only dual 1-failed neutrosophic SuperHyperForcing.
Proof. Suppose is an even neutrosophic SuperHyperCycle. Let where for all and
It implies is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. If where then
So where isn’t a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. It induces is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing.
and are trivial.
By is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. Thus it’s enough to show that is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. Suppose is an even neutrosophic SuperHyperCycle. Let where for all and
It implies is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. If where then
So where isn’t a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. It induces is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. □
Proposition 83.
Let be an odd neutrosophic SuperHyperCycle. Then
- the neutrosophic SuperHyperSet is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- and corresponded neutrosophic SuperHyperSet is ;
- the neutrosophic SuperHyperSets and are only dual 1-failed neutrosophic SuperHyperForcing.
Proof. Suppose is an odd neutrosophic SuperHyperCycle. Let where for all and
It implies is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. If where then
So where isn’t a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. It induces is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing.
and are trivial.
By is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. Thus it’s enough to show that is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. Suppose is an odd neutrosophic SuperHyperCycle. Let where for all and
It implies is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. If where then
So where isn’t a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. It induces is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. □
Proposition 84.
Let be neutrosophic SuperHyperStar. Then
- the neutrosophic SuperHyperSet is a dual maximal 1-failed neutrosophic SuperHyperForcing;
- the neutrosophic SuperHyperSets and are only dual 1-failed neutrosophic SuperHyperForcing.
Proof. Suppose is a neutrosophic SuperHyperStar.
It implies is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. If then
So isn’t a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. It induces is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing.
and are trivial.
By is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. Thus it’s enough to show that is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. Suppose is a neutrosophic SuperHyperStar. Let
It implies is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. □
Proposition 85.
Let be neutrosophic SuperHyperWheel. Then
- the neutrosophic SuperHyperSet is a dual maximal neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- the neutrosophic SuperHyperSet is only a dual maximal neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing.
Proof. Suppose is a neutrosophic SuperHyperWheel. Let There are either
or
It implies is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. If where then There are either
or
So where isn’t a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. It induces is a dual maximal neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing.
and are obvious. □
Proposition 86.
Let be an odd neutrosophic SuperHyperComplete. Then
- the neutrosophic SuperHyperSet is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- the neutrosophic SuperHyperSet is only a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing.
Proof. Suppose is an odd neutrosophic SuperHyperComplete. Let Thus
It implies is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. If where then
So where isn’t a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. It induces is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing.
and are obvious. □
Proposition 87.
Let be an even neutrosophic SuperHyperComplete. Then
- the neutrosophic SuperHyperSet is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- the neutrosophic SuperHyperSet is only a dual maximal neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing.
Proof. Suppose is an even neutrosophic SuperHyperComplete. Let Thus
It implies is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. If where then
So where isn’t a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. It induces is a dual maximal neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing.
and are obvious. □
Proposition 88.
Let be a m-neutrosophic SuperHyperFamily of neutrosophic SuperHyperStars with common neutrosophic SuperHyperVertex neutrosophic SuperHyperSet. Then
- the neutrosophic SuperHyperSet is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing for
- for
- for
- the neutrosophic SuperHyperSets and are only dual 1-failed neutrosophic SuperHyperForcing for
Proof. Suppose is a neutrosophic SuperHyperStar.
It implies is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing for If then
So isn’t a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing for It induces is a dual maximal neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing for
and are trivial.
By is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing for Thus it’s enough to show that is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing for Suppose is a neutrosophic SuperHyperStar. Let
It implies is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing for □
Proposition 89.
Let be an m-neutrosophic SuperHyperFamily of odd neutrosophic SuperHyperComplete neutrosophic SuperHyperGraphs with common neutrosophic SuperHyperVertex neutrosophic SuperHyperSet. Then
- the neutrosophic SuperHyperSet is a dual maximal neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing for
- for
- for
- the neutrosophic SuperHyperSets are only a dual maximal 1-failed neutrosophic SuperHyperForcing for
Proof. Suppose is odd neutrosophic SuperHyperComplete. Let Thus
It implies is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing for If where then
So where isn’t a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing for It induces is a dual maximal neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing for
and are obvious. □
Proposition 90.
Let be a m-neutrosophic SuperHyperFamily of even neutrosophic SuperHyperComplete neutrosophic SuperHyperGraphs with common neutrosophic SuperHyperVertex neutrosophic SuperHyperSet. Then
- the neutrosophic SuperHyperSet is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing for
- for
- for
- the neutrosophic SuperHyperSets are only dual maximal 1-failed neutrosophic SuperHyperForcing for
Proof. Suppose is even neutrosophic SuperHyperComplete. Let Thus
It implies is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing for If where then
So where isn’t a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing for It induces is a dual maximal neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing for
and are obvious. □
Proposition 91.
Let be a strong neutrosophic SuperHyperGraph. Then following statements hold;
- if and a neutrosophic SuperHyperSet S of neutrosophic SuperHyperVertices is an t-neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing, then S is an s-neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- if and a neutrosophic SuperHyperSet S of neutrosophic SuperHyperVertices is a dual t-neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing, then S is a dual s-neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing.
Proof. Suppose is a strong neutrosophic SuperHyperGraph. Consider a neutrosophic SuperHyperSet S of neutrosophic SuperHyperVertices is an t-neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. Then
Thus S is an s-neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing.
Suppose is a strong neutrosophic SuperHyperGraph. Consider a neutrosophic SuperHyperSet S of neutrosophic SuperHyperVertices is a dual t-neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. Then
Thus S is a dual s-neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. □
Proposition 92.
Let be a strong neutrosophic SuperHyperGraph. Then following statements hold;
- if and a neutrosophic SuperHyperSet S of neutrosophic SuperHyperVertices is an t-neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing, then S is an s-neutrosophic SuperHyperPowerful 1-failed neutrosophic SuperHyperForcing;
- if and a neutrosophic SuperHyperSet S of neutrosophic SuperHyperVertices is a dual t-neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing, then S is a dual s-neutrosophic SuperHyperPowerful 1-failed neutrosophic SuperHyperForcing.
Proof. Suppose is a strong neutrosophic SuperHyperGraph. Consider a neutrosophic SuperHyperSet S of neutrosophic SuperHyperVertices is an t-neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. Then
Thus S is an neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. By S is an neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing and S is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing, S is an s-neutrosophic SuperHyperPowerful 1-failed neutrosophic SuperHyperForcing.
Suppose is a strong neutrosophic SuperHyperGraph. Consider a neutrosophic SuperHyperSet S of neutrosophic SuperHyperVertices is a dual t-neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. Then
Thus S is an neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. By S is an neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing and S is a dual neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing, S is an neutrosophic SuperHyperPowerful 1-failed neutrosophic SuperHyperForcing. □
Proposition 93.
Let be a[an] [r-]neutrosophic SuperHyperUniform-strong-neutrosophic SuperHyperGraph. Then following statements hold;
- if then is an 2-neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- if then is a dual 2-neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- if then is an r-neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- if then is a dual r-neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing.
Proof. Suppose is a[an] [r-]neutrosophic SuperHyperUniform-strong-neutrosophic SuperHyperGraph. Then
Thus S is an 2-neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing.
Suppose is a[an] [r-]neutrosophic SuperHyperUniform-strong-neutrosophic SuperHyperGraph. Then
Thus S is a dual 2-neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing.
Suppose is a[an] [r-]neutrosophic SuperHyperUniform-strong-neutrosophic SuperHyperGraph. Then
Thus S is an r-neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing.
Suppose is a[an] [r-]neutrosophic SuperHyperUniform-strong-neutrosophic SuperHyperGraph. Then
Thus S is a dual r-neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. □
Proposition 94.
Let is a[an] [r-]neutrosophic SuperHyperUniform-strong-neutrosophic SuperHyperGraph. Then following statements hold;
- if is an 2-neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- if is a dual 2-neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- if is an r-neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- if is a dual r-neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing.
Proof. Suppose is a[an] [r-]neutrosophic SuperHyperUniform-strong-neutrosophic SuperHyperGraph. Then
Suppose is a[an] [r-]neutrosophic SuperHyperUniform-strong-neutrosophic SuperHyperGraph and a dual 2-neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. Then
Suppose is a[an] [r-]neutrosophic SuperHyperUniform-strong-neutrosophic SuperHyperGraph and an r-neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing.
Suppose is a[an] [r-]neutrosophic SuperHyperUniform-strong-neutrosophic SuperHyperGraph and a dual r-neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. Then
□
Proposition 95.
Let is a[an] [r-]neutrosophic SuperHyperUniform-strong-neutrosophic SuperHyperGraph which is a neutrosophic SuperHyperComplete. Then following statements hold;
- if is an 2-neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- if is a dual 2-neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- if is an -neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- if is a dual -neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing.
Proof. Suppose is a[an] [r-]neutrosophic SuperHyperUniform-strong-neutrosophic SuperHyperGraph and an 2- neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. Then
Suppose is a[an] [r-]neutrosophic SuperHyperUniform-strong-neutrosophic SuperHyperGraph and a dual 2-neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. Then
Suppose is a[an] [r-]neutrosophic SuperHyperUniform-strong-neutrosophic SuperHyperGraph and an ()-neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing.
Suppose is a[an] [r-]neutrosophic SuperHyperUniform-strong-neutrosophic SuperHyperGraph and a dual r-neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. Then
□
Proposition 96.
Let is a[an] [r-]neutrosophic SuperHyperUniform-strong-neutrosophic SuperHyperGraph which is a neutrosophic SuperHyperComplete. Then following statements hold;
- if then is an 2-neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- if then is a dual 2-neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- if then is -neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- if then is a dual -neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing.
Proof. Suppose is a[an] [r-]neutrosophic SuperHyperUniform-strong-neutrosophic SuperHyperGraph which is a neutrosophic SuperHyperComplete. Then
Thus S is an 2-neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing.
Suppose is a[an] [r-]neutrosophic SuperHyperUniform-strong-neutrosophic SuperHyperGraph which is a neutrosophic SuperHyperComplete. Then
Thus S is a dual 2-neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing.
Suppose is a[an] [r-]neutrosophic SuperHyperUniform-strong-neutrosophic SuperHyperGraph which is a neutrosophic SuperHyperComplete. Then
Thus S is an ()-neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing.
Suppose is a[an] [r-]neutrosophic SuperHyperUniform-strong-neutrosophic SuperHyperGraph which is a neutrosophic SuperHyperComplete. Then
Thus S is a dual ()-neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. □
Proposition 97.
Let is a[an] [r-]neutrosophic SuperHyperUniform-strong-neutrosophic SuperHyperGraph which is neutrosophic SuperHyperCycle. Then following statements hold;
- if is an 2-neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- if is a dual 2-neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- if is an 2-neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- if is a dual 2-neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing.
Proof. Suppose is a[an] [r-]neutrosophic SuperHyperUniform-strong-neutrosophic SuperHyperGraph and S is an 2-neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. Then
Suppose is a[an] [r-]neutrosophic SuperHyperUniform-strong-neutrosophic SuperHyperGraph and S is a dual 2-neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. Then
Suppose is a[an] [r-]neutrosophic SuperHyperUniform-strong-neutrosophic SuperHyperGraph and S is an 2-neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing.
Suppose is a[an] [r-]neutrosophic SuperHyperUniform-strong-neutrosophic SuperHyperGraph and S is a dual r-neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. Then
□
Proposition 98.
Let is a[an] [r-]neutrosophic SuperHyperUniform-strong-neutrosophic SuperHyperGraph which is neutrosophic SuperHyperCycle. Then following statements hold;
- if then is an 2-neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- if then is a dual 2-neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- if then is an 2-neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing;
- if then is a dual 2-neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing.
Proof. Suppose is a[an] [r-]neutrosophic SuperHyperUniform-strong-neutrosophic SuperHyperGraph which is neutrosophic SuperHyperCycle. Then
Thus S is an 2-neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing.
Suppose is a[an] [r-]neutrosophic SuperHyperUniform-strong-neutrosophic SuperHyperGraph which is neutrosophic SuperHyperCycle. Then
Thus S is a dual 2-neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing.
Suppose is a[an] [r-]neutrosophic SuperHyperUniform-strong-neutrosophic SuperHyperGraph which is neutrosophic SuperHyperCycle. Then
Thus S is an 2-neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing.
Suppose is a[an] [r-]neutrosophic SuperHyperUniform-strong-neutrosophic SuperHyperGraph which is neutrosophic SuperHyperCycle. Then
Thus S is a dual 2-neutrosophic SuperHyperDefensive 1-failed neutrosophic SuperHyperForcing. □
5. Applications in Cancer’s Neutrosophic Recognition
The cancer is the disease but the model is going to figure out what’s going on this phenomenon. The special case of this disease is considered and as the consequences of the model, some parameters are used. The cells are under attack of this disease but the moves of the cancer in the special region are the matter of mind. The neutrosophic recognition of the cancer could help to find some treatments for this disease.
In the following, some steps are devised on this disease.
- Step
- 1. (Definition) The neutrosophic recognition of the cancer in the long-term function.
- Step
- 2. (Issue) The specific region has been assigned by the model [it’s called neutrosophic SuperHyperGraph] and the long cycle of the move from the cancer is identified by this research. Sometimes the move of the cancer hasn’t be easily identified since there are some determinacy, indeterminacy and neutrality about the moves and the effects of the cancer on that region; this event leads us to choose another model [it’s said to be neutrosophic SuperHyperGraph] to have convenient perception on what’s happened and what’s done.
- Step
- 3. (Model) There are some specific models, which are well-known and they’ve got the names, and some general models. The moves and the traces of the cancer on the complex tracks and between complicated groups of cells could be fantasized by a neutrosophic SuperHyperPath(-/neutrosophic SuperHyperCycle, neutrosophic SuperHyperStar, neutrosophic SuperHyperBipartite, neutrosophic SuperHyperMultipartite, neutrosophic SuperHyperWheel). The aim is to find either the 1-failed neutrosophic SuperHyperForcing or the neutrosophic 1-failed neutrosophic SuperHyperForcing in those neutrosophic SuperHyperModels.
5.1. Case 1: The Initial Steps Toward neutrosophic SuperHyperBipartite as neutrosophic SuperHyperModel
- Step
-
4. (Solution) In the Figure (27), the neutrosophic SuperHyperBipartite is highlighted and featured.By using the Figure (27) and the Table (10), the neutrosophic SuperHyperBipartite is obtained.
Table 10.
The Values of Vertices, SuperVertices, Edges, HyperEdges, and neutrosophic SuperHyperEdges Belong to The neutrosophic SuperHyperBipartite
Table 10.
The Values of Vertices, SuperVertices, Edges, HyperEdges, and neutrosophic SuperHyperEdges Belong to The neutrosophic SuperHyperBipartite
| The Values of The Vertices | The Number of Position in Alphabet |
| The Values of The SuperVertices | The maximum Values of Its Vertices |
| The Values of The Edges | The maximum Values of Its Vertices |
| The Values of The HyperEdges | The maximum Values of Its Vertices |
| The Values of The neutrosophic SuperHyperEdges | The maximum Values of Its Endpoints |
5.2. Case 2: The Increasing Steps Toward Neutrosophic SuperHyperMultipartite as Neutrosophic SuperHyperModel
- Step
- 4. (Solution) In the Figure (28), the neutrosophic SuperHyperMultipartite is highlighted and featured.Figure 28. A neutrosophic SuperHyperMultipartite Associated to the Notions of 1-failed neutrosophic SuperHyperForcing.Figure 28. A neutrosophic SuperHyperMultipartite Associated to the Notions of 1-failed neutrosophic SuperHyperForcing.
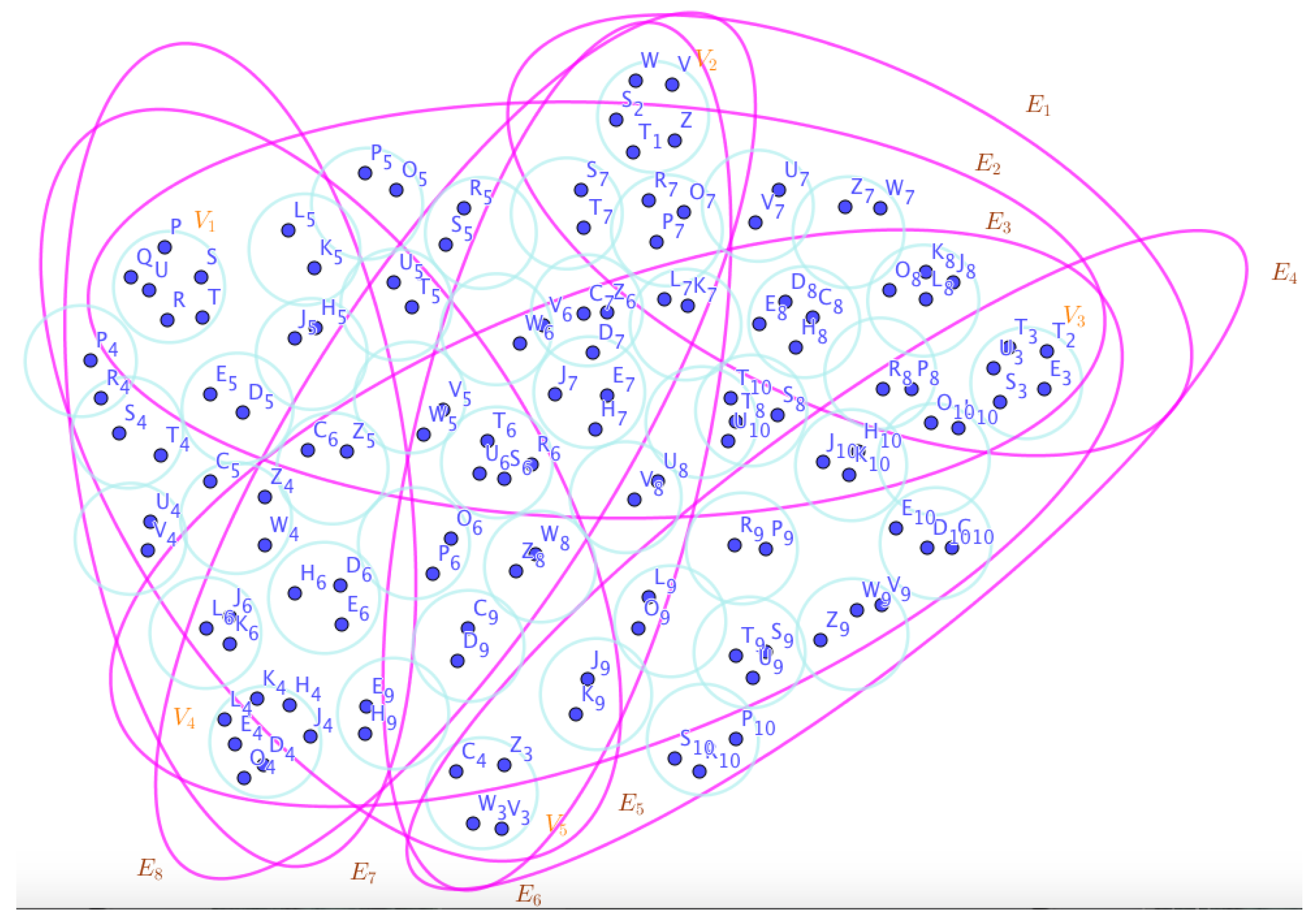
By using the Figure (28) and the Table (11), the neutrosophic SuperHyperMultipartite is obtained.
Table 11.
The Values of Vertices, SuperVertices, Edges, HyperEdges, and neutrosophic SuperHyperEdges Belong to The neutrosophic SuperHyperMultipartite
Table 11.
The Values of Vertices, SuperVertices, Edges, HyperEdges, and neutrosophic SuperHyperEdges Belong to The neutrosophic SuperHyperMultipartite
| The Values of The Vertices | The Number of Position in Alphabet |
| The Values of The SuperVertices | The maximum Values of Its Vertices |
| The Values of The Edges | The maximum Values of Its Vertices |
| The Values of The HyperEdges | The maximum Values of Its Vertices |
| The Values of The neutrosophic SuperHyperEdges | The maximum Values of Its Endpoints |
6. Open Problems
In what follows, some “problems” and some “questions” are proposed.
The 1-failed neutrosophic SuperHyperForcing and the neutrosophic 1-failed neutrosophic SuperHyperForcing are defined on a real-world application, titled “Cancer’s Neutrosophic Recognition”.
Question 99.
Which the else neutrosophic SuperHyperModels could be defined based on Cancer’s neutrosophic recognition?
Question 100.
Are there some neutrosophic SuperHyperNotions related to 1-failed neutrosophic SuperHyperForcing and the neutrosophic 1-failed neutrosophic SuperHyperForcing?
Question 101.
Are there some Algorithms to be defined on the neutrosophic SuperHyperModels to compute them?
Question 102.
Which the neutrosophic SuperHyperNotions are related to beyond the 1-failed neutrosophic SuperHyperForcing and the neutrosophic 1-failed neutrosophic SuperHyperForcing?
Question 103.
The 1-failed neutrosophic SuperHyperForcing and the neutrosophic 1-failed neutrosophic SuperHyperForcing do a neutrosophic SuperHyperModel for the Cancer’s neutrosophic recognition and they’re based on 1-failed neutrosophic SuperHyperForcing, are there else?
Question 1004.
Which the fundamental neutrosophic SuperHyperNumbers are related to these neutrosophic SuperHyperNumbers types-results?
Problem 105.
What’s the independent research based on Cancer’s neutrosophic recognition concerning the multiple types of neutrosophic SuperHyperNotions?
7. Conclusions and Closing Remarks
In this section, concluding remarks and closing remarks are represented. The drawbacks of this research are illustrated. Some benefits and some advantages of this research are highlighted.
This research uses some approaches to make neutrosophic SuperHyperGraphs more understandable. In this endeavor, two neutrosophic SuperHyperNotions are defined on the 1-failed neutrosophic SuperHyperForcing. For that sake in the second definition, the main definition of the neutrosophic SuperHyperGraph is redefined on the position of the alphabets. Based on the new definition for the neutrosophic SuperHyperGraph, the new neutrosophic SuperHyperNotion, neutrosophic 1-failed neutrosophic SuperHyperForcing, finds the convenient background to implement some results based on that. Some neutrosophic SuperHyperClasses and some neutrosophic SuperHyperClasses are the cases of this research on the modeling of the regions where are under the attacks of the cancer to recognize this disease as it’s mentioned on the title “Cancer’s Neutrosophic Recognition”. To formalize the instances on the neutrosophic SuperHyperNotion, 1-failed neutrosophic SuperHyperForcing, the new neutrosophic SuperHyperClasses and neutrosophic SuperHyperClasses, are introduced. Some general results are gathered in the section on the 1-failed neutrosophic SuperHyperForcing and the neutrosophic 1-failed neutrosophic SuperHyperForcing. The clarifications, instances and literature reviews have taken the whole way through. In this research, the literature reviews have fulfilled the lines containing the notions and the results. The neutrosophic SuperHyperGraph and neutrosophic SuperHyperGraph are the neutrosophic SuperHyperModels on the “Cancer’s Neutrosophic Recognition” and both bases are the background of this research. Sometimes the cancer has been happened on the region, full of cells, groups of cells and embedded styles. In this segment, the neutrosophic SuperHyperModel proposes some neutrosophic SuperHyperNotions based on the connectivities of the moves of the cancer in the longest and strongest styles with the formation of the design and the architecture are formally called “1-failed neutrosophic SuperHyperForcing” in the themes of jargons and buzzwords. The prefix “neutrosophic SuperHyper” refers to the theme of the embedded styles to figure out the background for the neutrosophic SuperHyperNotions.
In the Table (12), some limitations and advantages of this research are pointed out.
Table 12.
A Brief Overview about Advantages and Limitations of this Research
| Advantages | Limitations |
| 1.Redefining neutrosophic SuperHyperGraph | 1.General Results |
| 2.1-failed neutrosophic SuperHyperForcing | |
| 3.Neutrosophic 1-failed neutrosophic SuperHyperForcing | 2.Other neutrosophic SuperHyperNumbers |
| 4.Modeling of Cancer’s Neutrosophic Recognition | |
| 5.neutrosophic SuperHyperClasses | 3.neutrosophic SuperHyperFamilies |
References
- Henry Garrett, “Properties of SuperHyperGraph and Neutrosophic SuperHyperGraph”, Neutrosophic Sets and Systems 49 (2022) 531-561 (doi: 10.5281/zenodo.6456413). (http://fs.unm.edu/NSS/NeutrosophicSuperHyperGraph34.pdf). (https://digitalrepository.unm.edu/nss_journal/vol49/iss1/34). 2022. [CrossRef]
- Henry Garrett, “Neutrosophic Co-degree and Neutrosophic Degree alongside Chromatic Numbers in the Setting of Some Classes Related to Neutrosophic Hypergraphs”, J Curr Trends Comp Sci Res 1(1) (2022) 06-14.
- Henry Garrett, “(Neutrosophic) SuperHyperModeling of Cancer’s Recognitions Featuring (Neutrosophic) SuperHyperDefensive SuperHyperAlliances”, Preprints. 2022; 2022120549. [CrossRef]
- Henry Garrett, “(Neutrosophic) SuperHyperAlliances With SuperHyperDefensive and SuperHyperOffensive Type-SuperHyperSet On (Neutrosophic) SuperHyperGraph With (Neutrosophic) SuperHyperModeling of Cancer’s Recognitions And Related (Neutrosophic) SuperHyperClasses”, Preprints. 2022; 2022120540. [CrossRef]
- enry Garrett, “SuperHyperGirth on SuperHyperGraph and Neutrosophic SuperHyperGraph With SuperHyperModeling of Cancer’s Recognitions”, Preprints. 2022; 2022120500. [CrossRef]
- Henry Garrett, “Some SuperHyperDegrees and Co-SuperHyperDegrees on Neutrosophic SuperHyperGraphs and SuperHyperGraphs Alongside Applications in Cancer’s Treatments”, Preprints. 2022; 2022120324. [CrossRef]
- Henry Garrett, “SuperHyperDominating and SuperHyperResolving on Neutrosophic SuperHyperGraphs And Their Directions in Game Theory and Neutrosophic SuperHyperClasses”, Preprints. 2022; 2022110576. [CrossRef]
- Henry Garrett, “Basic Notions on (Neutrosophic) SuperHyperForcing And (Neutrosophic) SuperHyperModeling in Cancer’s Recognitions And (Neutrosophic) SuperHyperGraphs”, ResearchGate. 2022. [CrossRef]
- Henry Garrett, “Basic Neutrosophic Notions Concerning SuperHyperDominating and Neutrosophic SuperHyperResolving in SuperHyperGraph”, ResearchGate. 2022. [CrossRef]
- Henry Garrett, “Initial Material of Neutrosophic Preliminaries to Study Some Neutrosophic Notions Based on Neutrosophic SuperHyperEdge (NSHE) in Neutrosophic SuperHyperGraph (NSHG)”, ResearchGate. 2022. [CrossRef]
- Henry Garrett, (2022). “Beyond Neutrosophic Graphs”, Ohio: E-publishing: Educational Publisher 1091 West 1st Ave Grandview Heights, Ohio 43212 United States. ISBN: 979-1-59973-725-6 (http://fs.unm.edu/BeyondNeutrosophicGraphs.pdf).
- Henry Garrett, (2022). “Neutrosophic Duality”, Florida: GLOBAL KNOWLEDGE - Publishing House 848 Brickell Ave Ste 950 Miami, Florida 33131 United States. ISBN: 978-1-59973-743-0 (http://fs.unm.edu/NeutrosophicDuality.pdf).
- F. Smarandache, “Extension of HyperGraph to n-SuperHyperGraph and to Plithogenic n-SuperHyperGraph, and Extension of HyperAlgebra to n-ary (Classical-/Neutro-/Anti-) HyperAlgebra”, Neutrosophic Sets and Systems 33 (2020) 290-296. [CrossRef]
- M. Akram et al., “Single-valued neutrosophic Hypergraphs”, TWMS J. App. Eng. Math. 8 (1) (2018) 122-135. [CrossRef]
- S. Broumi et al., “Single-valued neutrosophic graphs”, Journal of New Theory 10 (2016) 86-101. [CrossRef]
- H. Wang et al., “Single-valued neutrosophic sets”, Multispace and Multistructure 4 (2010) 410-413.
- H.T. Nguyen and E.A. Walker, “A First course in fuzzy logic”, CRC Press, 2006.
Figure 22.
A neutrosophic SuperHyperCycle Associated to the Notions of 1-failed neutrosophic SuperHyperForcing in the Example (36).
Figure 22.
A neutrosophic SuperHyperCycle Associated to the Notions of 1-failed neutrosophic SuperHyperForcing in the Example (36).
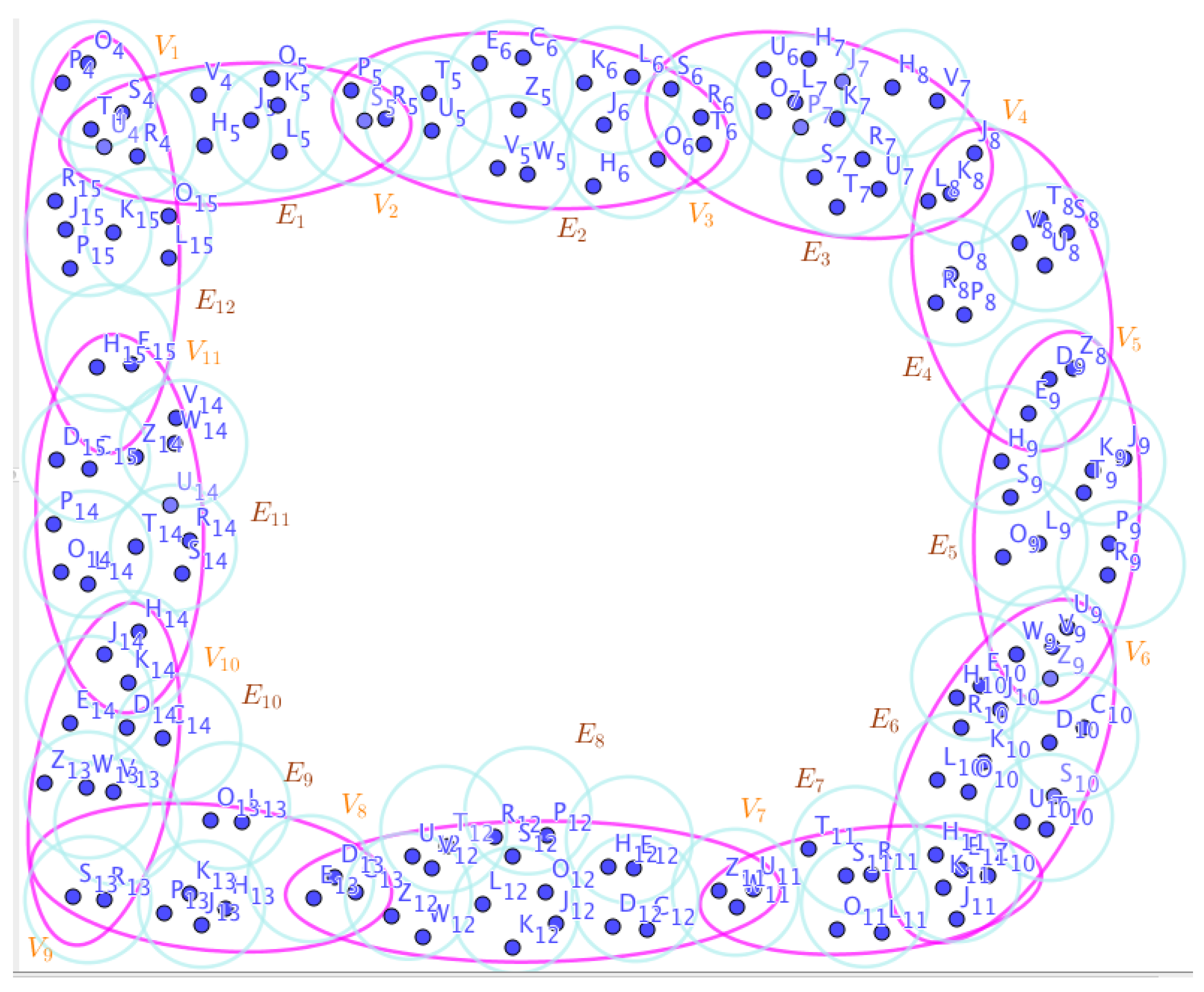
Figure 23.
A neutrosophic SuperHyperStar Associated to the Notions of 1-failed neutrosophic SuperHyperForcing in the Example (38).
Figure 23.
A neutrosophic SuperHyperStar Associated to the Notions of 1-failed neutrosophic SuperHyperForcing in the Example (38).

Figure 25.
A neutrosophic SuperHyperMultipartite Associated to the Notions of 1-failed neutrosophic SuperHyperForcing in the Example (42).
Figure 25.
A neutrosophic SuperHyperMultipartite Associated to the Notions of 1-failed neutrosophic SuperHyperForcing in the Example (42).

Figure 26.
A neutrosophic SuperHyperWheel Associated to the Notions of 1-failed neutrosophic SuperHyperForcing in the Example (44).
Figure 26.
A neutrosophic SuperHyperWheel Associated to the Notions of 1-failed neutrosophic SuperHyperForcing in the Example (44).

Figure 27.
A neutrosophic SuperHyperBipartite Associated to the Notions of 1-failed neutrosophic SuperHyperForcing.
Figure 27.
A neutrosophic SuperHyperBipartite Associated to the Notions of 1-failed neutrosophic SuperHyperForcing.

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Copyright: This open access article is published under a Creative Commons CC BY 4.0 license, which permit the free download, distribution, and reuse, provided that the author and preprint are cited in any reuse.
MDPI Initiatives
Important Links
© 2024 MDPI (Basel, Switzerland) unless otherwise stated






