Preprint
Article
Basic Notions on (Neutrosophic) SuperHyperForcing And (Neutrosophic) SuperHyperModeling in Cancer’s Recognitions And (Neutrosophic) SuperHyperGraphs
Altmetrics
Downloads
121
Views
28
Comments
0
This version is not peer-reviewed
Submitted:
30 December 2022
Posted:
05 January 2023
You are already at the latest version
Alerts
Abstract
In this research, assume a SuperHyperGraph. Then a “SuperHyperForcing” Z(NSHG) for a neutrosophic SuperHyperGraph NSHG : (V,E) is the minimum cardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in V (G) \ S are colored white) such that V (G) is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex; a “neutrosophic SuperHyperForcing” Zn(NSHG) for a neutrosophic SuperHyperGraph NSHG : (V,E) is the minimum neutrosophic cardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in V (G) \ S are colored white) such that V (G) is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. Assume a SuperHyperGraph. Then a “SuperHyperForcing” Z(NSHG) for a neutrosophic SuperHyperGraph NSHG : (V,E) is the minimum cardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in V (G) \ S are colored white) such that V (G) is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex; a “neutrosophic SuperHyperForcing” Zn(NSHG) for a neutrosophic SuperHyperGraph NSHG : (V,E) is the minimum neutrosophic cardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in V (G) \ S are colored white) such that V (G) is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. Assume a SuperHyperGraph. Then an “δ−SuperHyperForcing” is a minimal SuperHyperForcing of SuperHyperVertices with minimum cardinality such that either of the following expressions hold for the (neutrosophic) cardinalities of SuperHyperNeighbors of s ∈ S : |S∩N(s)|>|S∩(V \N(s))|+δ, |S∩N(s)|<|S∩(V \N(s))|+δ.Thefirst Expression, holds if S is an “δ−SuperHyperOffensive”. And the second Expression, holds if S is an “δ−SuperHyperDefensive”; a“neutrosophic δ−SuperHyperForcing” is a minimal neutrosophic SuperHyperForcing of SuperHyperVertices with minimum neutrosophic cardinality such that either of the following expressions hold for the neutrosophic cardinalities of SuperHyperNeighbors of s ∈ S : |S ∩ N(s)|neutrosophic > |S ∩ (V \ N (s))|neutrosophic + δ, |S ∩ N (s)|neutrosophic < |S ∩ (V \ N (s))|neutrosophic + δ. The first Expression, holds if S is a “neutrosophic δ−SuperHyperOffensive”. And the second Expression, holds if S is a “neutrosophic δ−SuperHyperDefensive”. It’s useful to define “neutrosophic” version of SuperHyperForcing. Since there’s more ways to get type-results to make SuperHyperForcing more understandable. For the sake of having neutrosophic SuperHyperForcing, there’s a need to “redefine” the notion of “SuperHyperForcing”. The SuperHyperVertices and the SuperHyperEdges are assigned by the labels from the letters of the alphabets. In this procedure, there’s the usage of the position of labels to assign to the values. A basic familiarity with SuperHyperGraph theory and neutrosophic SuperHyperGraph theory are proposed.
Keywords:
Subject: Computer Science and Mathematics - Computer Vision and Graphics
MSC: 05C17, 05C22, 05E45
1. Background
2. SuperHyperForcing
Definition 1.
((neutrosophic) SuperHyperForcing).
Assume a SuperHyperGraph. Then
- a SuperHyperForcing for a neutrosophic SuperHyperGraph is the minimum cardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex;
- a neutrosophic SuperHyperForcing for a neutrosophic SuperHyperGraph is the minimum neutrosophic cardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex.
Definition 2.
((neutrosophic) SuperHyperForcing).
Assume a SuperHyperGraph. Then
-
an SuperHyperForcing is a minimal SuperHyperForcing of SuperHyperVertices with minimum cardinality such that either of the following expressions hold for the (neutrosophic) cardinalities of SuperHyperNeighbors ofThe Expression (1), holds if S is an SuperHyperOffensive. And the Expression (6), holds if S is an SuperHyperDefensive;
-
a neutrosophic SuperHyperForcing is a minimal neutrosophic SuperHyperForcing of SuperHyperVertices with minimum neutrosophic cardinality such that either of the following expressions hold for the neutrosophic cardinalities of SuperHyperNeighbors ofThe Expression (7), holds if S is a neutrosophicSuperHyperOffensive. And the Expression (8), holds if S is a neutrosophicSuperHyperDefensive.
Example 1.
Assume the SuperHyperGraphs in the Figure 1, Figure 2, Figure 3, Figure 4, Figure 5, Figure 6, Figure 7, Figure 8, Figure 9, Figure 10, Figure 11, Figure 12, Figure 13, Figure 14, Figure 15, Figure 16, Figure 17, Figure 18, Figure 19, and Figure 20.
-
On the Figure 1, the SuperHyperNotion, namely, SuperHyperForcing, is up. and are some empty SuperHyperEdges but is a loop SuperHyperEdge and is an SuperHyperEdge. Thus in the terms of SuperHyperNeighbor, there’s only one SuperHyperEdge, namely, The SuperHyperVertex, is isolated means that there’s no SuperHyperEdge has it as an endpoint. Thus SuperHyperVertex, is contained in every given SuperHyperForcing. All the following SuperHyperSets of SuperHyperVertices are the simple type-SuperHyperSet of the SuperHyperForcing.The SuperHyperSet of the SuperHyperVertices, is a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex but it isn’t a SuperHyperForcing. Since it doesn’t have the minimum cardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. There’s only one SuperHyperVertex outside the intended SuperHyperSet. Thus the obvious SuperHyperForcing is up. The obvious simple type-SuperHyperSet of the SuperHyperForcing is a SuperHyperSet excludes only one SuperHyperVertex in a connected neutrosophic SuperHyperGraph
-
On the Figure 2, the SuperHyperNotion, namely, SuperHyperForcing, is up. and are some empty SuperHyperEdges but is an SuperHyperEdge. Thus in the terms of SuperHyperNeighbor, there’s only one SuperHyperEdge, namely, The SuperHyperVertex, is isolated means that there’s no SuperHyperEdge has it as an endpoint. Thus SuperHyperVertex, is contained in every given SuperHyperForcing. All the following SuperHyperSets of SuperHyperVertices are the simple type-SuperHyperSet of the SuperHyperForcing.The SuperHyperSet of the SuperHyperVertices, is a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex but it isn’t a SuperHyperForcing. Since it doesn’t have the minimum cardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. There’s only one SuperHyperVertex outside the intended SuperHyperSet. Thus the obvious SuperHyperForcing is up. The obvious simple type-SuperHyperSet of the SuperHyperForcing is a SuperHyperSet excludes only one SuperHyperVertex in a connected neutrosophic SuperHyperGraph
-
On the Figure 3, the SuperHyperNotion, namely, SuperHyperForcing, is up. and are some empty SuperHyperEdges but is an SuperHyperEdge. Thus in the terms of SuperHyperNeighbor, there’s only one SuperHyperEdge, namely, All the following SuperHyperSets of SuperHyperVertices are the simple type-SuperHyperSet of the SuperHyperForcing.The SuperHyperSet of the SuperHyperVertices, is a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex but it isn’t a SuperHyperForcing. Since it doesn’t have the minimum cardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. There’s only one SuperHyperVertex outside the intended SuperHyperSet. Thus the obvious SuperHyperForcing is up. The obvious simple type-SuperHyperSet of the SuperHyperForcing is a SuperHyperSet excludes only one SuperHyperVertex in a connected neutrosophic SuperHyperGraph Thus all the following SuperHyperSets of SuperHyperVertices are the simple type-SuperHyperSet of the SuperHyperForcing.since the SuperHyperSets of the SuperHyperVertices, are the SuperHyperSets Ss of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex and they are SuperHyperForcing. Since they’vethe minimum cardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. There’s only one SuperHyperVertex outside the intended SuperHyperSet, Thus the obvious SuperHyperForcing, is up. The obvious simple type-SuperHyperSet of the SuperHyperForcing, is a SuperHyperSet, excludes only one SuperHyperVertex in a connected neutrosophic SuperHyperGraph
- On the Figure 4, the SuperHyperNotion, namely, SuperHyperForcing, is up. There’s no empty SuperHyperEdge but are a loop SuperHyperEdge on and there are some SuperHyperEdges, namely, on alongside on and on The SuperHyperSet of SuperHyperVertices, is the simple type-SuperHyperSet of the SuperHyperForcing. The SuperHyperSet of the SuperHyperVertices, is the minimum cardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. In There’s not only one SuperHyperVertex outside the intended SuperHyperSet. Thus the non-obvious SuperHyperForcing is up. The obvious simple type-SuperHyperSet of the SuperHyperForcing is a SuperHyperSet excludes only one SuperHyperVertex in a connected neutrosophic SuperHyperGraph But the SuperHyperSet of SuperHyperVertices, has more than one SuperHyperVertex outside the intended SuperHyperSet. Thus the non-obvious simple type-SuperHyperSet of the SuperHyperForcing is up. To sum them up, the SuperHyperSet of SuperHyperVertices, is the non-obvious simple type-SuperHyperSet of the SuperHyperForcing. Since the SuperHyperSet of the SuperHyperVertices, is the SuperHyperSet Ss of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex and they are SuperHyperForcing. Since it’s the minimum cardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. There’s only more than one SuperHyperVertex outside the intended SuperHyperSet, Thus the non-obvious SuperHyperForcing, is up. The non-obvious simple type-SuperHyperSet of the SuperHyperForcing, is a SuperHyperSet, excludes only more than one SuperHyperVertex in a connected neutrosophic SuperHyperGraph
- On the Figure 5, the SuperHyperNotion, namely, SuperHyperForcing, is up. There’s neither empty SuperHyperEdge nor loop SuperHyperEdge. The SuperHyperSet of SuperHyperVertices, is the simple type-SuperHyperSet of the SuperHyperForcing. The SuperHyperSet of the SuperHyperVertices, is the minimum cardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. In There’s not only one SuperHyperVertex outside the intended SuperHyperSet. Thus the non-obvious SuperHyperForcing is up. The obvious simple type-SuperHyperSet of the SuperHyperForcing is a SuperHyperSet excludes only one SuperHyperVertex in a connected neutrosophic SuperHyperGraph But the SuperHyperSet of SuperHyperVertices, has more than one SuperHyperVertex outside the intended SuperHyperSet. Thus the non-obvious simple type-SuperHyperSet of the SuperHyperForcing is up. To sum them up, the SuperHyperSet of SuperHyperVertices, is the non-obvious simple type-SuperHyperSet of the SuperHyperForcing. Since the SuperHyperSet of the SuperHyperVertices, is the SuperHyperSet Ss of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex and they are SuperHyperForcing. Since it’sthe minimum cardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. There’s only more than one SuperHyperVertex outside the intended SuperHyperSet, Thus the non-obvious SuperHyperForcing, is up. The non-obvious simple type-SuperHyperSet of the SuperHyperForcing, is a SuperHyperSet, excludes only more than one SuperHyperVertex in a connected neutrosophic SuperHyperGraph
- On the Figure 6, the SuperHyperNotion, namely, SuperHyperForcing, is up. There’s neither empty SuperHyperEdge nor loop SuperHyperEdge. The SuperHyperSet of SuperHyperVertices, is the simple type-SuperHyperSet of the SuperHyperForcing. The SuperHyperSet of the SuperHyperVertices, is the minimum cardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. In There’s not only one SuperHyperVertex outside the intended SuperHyperSet. Thus the non-obvious SuperHyperForcing is up. The obvious simple type-SuperHyperSet of the SuperHyperForcing is a SuperHyperSet excludes only one SuperHyperVertex in a connected neutrosophic SuperHyperGraph But the SuperHyperSet of SuperHyperVertices, has more than one SuperHyperVertex outside the intended SuperHyperSet. Thus the non-obvious simple type-SuperHyperSet of the SuperHyperForcing is up. To sum them up, the SuperHyperSet of SuperHyperVertices, is the non-obvious simple type-SuperHyperSet of the SuperHyperForcing. Since the SuperHyperSet of the SuperHyperVertices, is the SuperHyperSet Ss of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex and they are SuperHyperForcing. Since it’sthe minimum cardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. There’s only more than one SuperHyperVertex outside the intended SuperHyperSet, Thus the non-obvious SuperHyperForcing, is up. The non-obvious simple type-SuperHyperSet of the SuperHyperForcing, is a SuperHyperSet, excludes only more than one SuperHyperVertex in a connected neutrosophic SuperHyperGraph
- On the Figure 7, the SuperHyperNotion, namely, SuperHyperForcing, is up. There’s neither empty SuperHyperEdge nor loop SuperHyperEdge. The SuperHyperSet of SuperHyperVertices, is the simple type-SuperHyperSet of the SuperHyperForcing. The SuperHyperSet of the SuperHyperVertices, is the minimum cardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. In There’s not only one SuperHyperVertex outside the intended SuperHyperSet. Thus the non-obvious SuperHyperForcing is up. The obvious simple type-SuperHyperSet of the SuperHyperForcing is a SuperHyperSet excludes only one SuperHyperVertex in a connected neutrosophic SuperHyperGraph But the SuperHyperSet of SuperHyperVertices, has more than one SuperHyperVertex outside the intended SuperHyperSet. Thus the non-obvious simple type-SuperHyperSet of the SuperHyperForcing is up. To sum them up, the SuperHyperSet of SuperHyperVertices, is the non-obvious simple type-SuperHyperSet of the SuperHyperForcing. Since the SuperHyperSet of the SuperHyperVertices, is the SuperHyperSet Ss of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex and they are SuperHyperForcing. Since it’sthe minimum cardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. There’s only more than one SuperHyperVertex outside the intended SuperHyperSet, Thus the non-obvious SuperHyperForcing, is up. The non-obvious simple type-SuperHyperSet of the SuperHyperForcing, is a SuperHyperSet, excludes only more than one SuperHyperVertex in a connected neutrosophic SuperHyperGraph
- On the Figure 8, the SuperHyperNotion, namely, SuperHyperForcing, is up. There’s neither empty SuperHyperEdge nor loop SuperHyperEdge. The SuperHyperSet of SuperHyperVertices, is the simple type-SuperHyperSet of the SuperHyperForcing. The SuperHyperSet of the SuperHyperVertices, is the minimum cardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. In There’s not only one SuperHyperVertex outside the intended SuperHyperSet. Thus the non-obvious SuperHyperForcing is up. The obvious simple type-SuperHyperSet of the SuperHyperForcing is a SuperHyperSet excludes only one SuperHyperVertex in a connected neutrosophic SuperHyperGraph But the SuperHyperSet of SuperHyperVertices, has more than one SuperHyperVertex outside the intended SuperHyperSet. Thus the non-obvious simple type-SuperHyperSet of the SuperHyperForcing is up. To sum them up, the SuperHyperSet of SuperHyperVertices, is the non-obvious simple type-SuperHyperSet of the SuperHyperForcing. Since the SuperHyperSet of the SuperHyperVertices, is the SuperHyperSet Ss of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex and they are SuperHyperForcing. Since it’sthe minimum cardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. There’s only more than one SuperHyperVertex outside the intended SuperHyperSet, Thus the non-obvious SuperHyperForcing, is up. The non-obvious simple type-SuperHyperSet of the SuperHyperForcing, is a SuperHyperSet, excludes only more than one SuperHyperVertex in a connected neutrosophic SuperHyperGraph
- On the Figure 9, the SuperHyperNotion, namely, SuperHyperForcing, is up. There’s neither empty SuperHyperEdge nor loop SuperHyperEdge. The SuperHyperSet of SuperHyperVertices, is the simple type-SuperHyperSet of the SuperHyperForcing. The SuperHyperSet of the SuperHyperVertices, is the minimum cardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. In There’s not only one SuperHyperVertex outside the intended SuperHyperSet. Thus the non-obvious SuperHyperForcing is up. The obvious simple type-SuperHyperSet of the SuperHyperForcing is a SuperHyperSet excludes only one SuperHyperVertex in a connected neutrosophic SuperHyperGraph But the SuperHyperSet of SuperHyperVertices, has more than one SuperHyperVertex outside the intended SuperHyperSet. Thus the non-obvious simple type-SuperHyperSet of the SuperHyperForcing is up. To sum them up, the SuperHyperSet of SuperHyperVertices, is the non-obvious simple type-SuperHyperSet of the SuperHyperForcing. Since the SuperHyperSet of the SuperHyperVertices, is the SuperHyperSet Ss of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex and they are SuperHyperForcing. Since it’sthe minimum cardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. There’s only more than one SuperHyperVertex outside the intended SuperHyperSet, Thus the non-obvious SuperHyperForcing, is up. The non-obvious simple type-SuperHyperSet of the SuperHyperForcing, is a SuperHyperSet, excludes only more than one SuperHyperVertex in a connected neutrosophic SuperHyperGraph
- On the Figure 10, the SuperHyperNotion, namely, SuperHyperForcing, is up. There’s neither empty SuperHyperEdge nor loop SuperHyperEdge. The SuperHyperSet of SuperHyperVertices, is the simple type-SuperHyperSet of the SuperHyperForcing. The SuperHyperSet of the SuperHyperVertices, is the minimum cardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. In There’s not only one SuperHyperVertex outside the intended SuperHyperSet. Thus the non-obvious SuperHyperForcing is up. The obvious simple type-SuperHyperSet of the SuperHyperForcing is a SuperHyperSet excludes only one SuperHyperVertex in a connected neutrosophic SuperHyperGraph But the SuperHyperSet of SuperHyperVertices, has more than one SuperHyperVertex outside the intended SuperHyperSet. Thus the non-obvious simple type-SuperHyperSet of the SuperHyperForcing is up. To sum them up, the SuperHyperSet of SuperHyperVertices, is the non-obvious simple type-SuperHyperSet of the SuperHyperForcing. Since the SuperHyperSet of the SuperHyperVertices, is the SuperHyperSet Ss of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex and they are SuperHyperForcing. Since it’sthe minimum cardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. There’s only more than one SuperHyperVertex outside the intended SuperHyperSet, Thus the non-obvious SuperHyperForcing, is up. The non-obvious simple type-SuperHyperSet of the SuperHyperForcing, is a SuperHyperSet, excludes only more than one SuperHyperVertex in a connected neutrosophic SuperHyperGraph
- On the Figure 11, the SuperHyperNotion, namely, SuperHyperForcing, is up. There’s neither empty SuperHyperEdge nor loop SuperHyperEdge. The SuperHyperSet of SuperHyperVertices, is the simple type-SuperHyperSet of the SuperHyperForcing. The SuperHyperSet of the SuperHyperVertices, is the minimum cardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. In There’s not only one SuperHyperVertex outside the intended SuperHyperSet. Thus the non-obvious SuperHyperForcing is up. The obvious simple type-SuperHyperSet of the SuperHyperForcing is a SuperHyperSet excludes only one SuperHyperVertex in a connected neutrosophic SuperHyperGraph But the SuperHyperSet of SuperHyperVertices, has more than one SuperHyperVertex outside the intended SuperHyperSet. Thus the non-obvious simple type-SuperHyperSet of the SuperHyperForcing is up. To sum them up, the SuperHyperSet of SuperHyperVertices, is the non-obvious simple type-SuperHyperSet of the SuperHyperForcing. Since the SuperHyperSet of the SuperHyperVertices, is the SuperHyperSet Ss of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex and they are SuperHyperForcing. Since it’sthe minimum cardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. There’s only more than one SuperHyperVertex outside the intended SuperHyperSet, Thus the non-obvious SuperHyperForcing, is up. The non-obvious simple type-SuperHyperSet of the SuperHyperForcing, is a SuperHyperSet, excludes only more than one SuperHyperVertex in a connected neutrosophic SuperHyperGraph
- On the Figure 12, the SuperHyperNotion, namely, SuperHyperForcing, is up. There’s neither empty SuperHyperEdge nor loop SuperHyperEdge. The SuperHyperSet of SuperHyperVertices, is the simple type-SuperHyperSet of the SuperHyperForcing. The SuperHyperSet of the SuperHyperVertices, is the minimum cardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. In There’s not only one SuperHyperVertex outside the intended SuperHyperSet. Thus the non-obvious SuperHyperForcing is up. The obvious simple type-SuperHyperSet of the SuperHyperForcing is a SuperHyperSet excludes only one SuperHyperVertex in a connected neutrosophic SuperHyperGraph But the SuperHyperSet of SuperHyperVertices, has more than one SuperHyperVertex outside the intended SuperHyperSet. Thus the non-obvious simple type-SuperHyperSet of the SuperHyperForcing is up. To sum them up, the SuperHyperSet of SuperHyperVertices, is the non-obvious simple type-SuperHyperSet of the SuperHyperForcing. Since the SuperHyperSet of the SuperHyperVertices, is the SuperHyperSet Ss of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex and they are SuperHyperForcing. Since it’sthe minimum cardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. There’s only more than one SuperHyperVertex outside the intended SuperHyperSet, Thus the non-obvious SuperHyperForcing, is up. The non-obvious simple type-SuperHyperSet of the SuperHyperForcing, is a SuperHyperSet, excludes only more than one SuperHyperVertex in a connected neutrosophic SuperHyperGraph
- On the Figure 13, the SuperHyperNotion, namely, SuperHyperForcing, is up. There’s neither empty SuperHyperEdge nor loop SuperHyperEdge. The SuperHyperSet of SuperHyperVertices, is the simple type-SuperHyperSet of the SuperHyperForcing. The SuperHyperSet of the SuperHyperVertices, is the minimum cardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. In There’s not only one SuperHyperVertex outside the intended SuperHyperSet. Thus the non-obvious SuperHyperForcing is up. The obvious simple type-SuperHyperSet of the SuperHyperForcing is a SuperHyperSet excludes only one SuperHyperVertex in a connected neutrosophic SuperHyperGraph But the SuperHyperSet of SuperHyperVertices, has more than one SuperHyperVertex outside the intended SuperHyperSet. Thus the non-obvious simple type-SuperHyperSet of the SuperHyperForcing is up. To sum them up, the SuperHyperSet of SuperHyperVertices, is the non-obvious simple type-SuperHyperSet of the SuperHyperForcing. Since the SuperHyperSet of the SuperHyperVertices, is the SuperHyperSet Ss of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex and they are SuperHyperForcing. Since it’sthe minimum cardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. There’s only more than one SuperHyperVertex outside the intended SuperHyperSet, Thus the non-obvious SuperHyperForcing, is up. The non-obvious simple type-SuperHyperSet of the SuperHyperForcing, is a SuperHyperSet, excludes only more than one SuperHyperVertex in a connected neutrosophic SuperHyperGraph
- On the Figure 14, the SuperHyperNotion, namely, SuperHyperForcing, is up. There’s neither empty SuperHyperEdge nor loop SuperHyperEdge. The SuperHyperSet of SuperHyperVertices, is the simple type-SuperHyperSet of the SuperHyperForcing. The SuperHyperSet of the SuperHyperVertices, is the minimum cardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. In There’s not only one SuperHyperVertex outside the intended SuperHyperSet. Thus the non-obvious SuperHyperForcing is up. The obvious simple type-SuperHyperSet of the SuperHyperForcing is a SuperHyperSet excludes only one SuperHyperVertex in a connected neutrosophic SuperHyperGraph But the SuperHyperSet of SuperHyperVertices, has more than one SuperHyperVertex outside the intended SuperHyperSet. Thus the non-obvious simple type-SuperHyperSet of the SuperHyperForcing is up. To sum them up, the SuperHyperSet of SuperHyperVertices, is the non-obvious simple type-SuperHyperSet of the SuperHyperForcing. Since the SuperHyperSet of the SuperHyperVertices, is the SuperHyperSet Ss of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex and they are SuperHyperForcing. Since it’sthe minimum cardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. There’s only more than one SuperHyperVertex outside the intended SuperHyperSet, Thus the non-obvious SuperHyperForcing, is up. The non-obvious simple type-SuperHyperSet of the SuperHyperForcing, is a SuperHyperSet, excludes only more than one SuperHyperVertex in a connected neutrosophic SuperHyperGraph
- On the Figure 15, the SuperHyperNotion, namely, SuperHyperForcing, is up. There’s neither empty SuperHyperEdge nor loop SuperHyperEdge. The SuperHyperSet of SuperHyperVertices, is the simple type-SuperHyperSet of the SuperHyperForcing. The SuperHyperSet of the SuperHyperVertices, is the minimum cardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. In There’s not only one SuperHyperVertex outside the intended SuperHyperSet. Thus the non-obvious SuperHyperForcing is up. The obvious simple type-SuperHyperSet of the SuperHyperForcing is a SuperHyperSet excludes only one SuperHyperVertex in a connected neutrosophic SuperHyperGraph But the SuperHyperSet of SuperHyperVertices, has more than one SuperHyperVertex outside the intended SuperHyperSet. Thus the non-obvious simple type-SuperHyperSet of the SuperHyperForcing is up. To sum them up, the SuperHyperSet of SuperHyperVertices, is the non-obvious simple type-SuperHyperSet of the SuperHyperForcing. Since the SuperHyperSet of the SuperHyperVertices, is the SuperHyperSet Ss of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex and they are SuperHyperForcing. Since it’sthe minimum cardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. There’s only more than one SuperHyperVertex outside the intended SuperHyperSet, Thus the non-obvious SuperHyperForcing, is up. The non-obvious simple type-SuperHyperSet of the SuperHyperForcing, is a SuperHyperSet, excludes only more than one SuperHyperVertex in a connected neutrosophic SuperHyperGraph
-
On the Figure 16, the SuperHyperNotion, namely, SuperHyperForcing, is up. There’s neither empty SuperHyperEdge nor loop SuperHyperEdge. The SuperHyperSet of SuperHyperVertices,is the simple type-SuperHyperSet of the SuperHyperForcing. The SuperHyperSet of the SuperHyperVertices,is the minimum cardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. In There’s not only one SuperHyperVertex outside the intended SuperHyperSet. Thus the non-obvious SuperHyperForcing is up. The obvious simple type-SuperHyperSet of the SuperHyperForcing is a SuperHyperSet excludes only one SuperHyperVertex in a connected neutrosophic SuperHyperGraph But the SuperHyperSet of SuperHyperVertices,has more than one SuperHyperVertex outside the intended SuperHyperSet. Thus the non-obvious simple type-SuperHyperSet of the SuperHyperForcing is up. To sum them up, the SuperHyperSet of SuperHyperVertices,is the non-obvious simple type-SuperHyperSet of the SuperHyperForcing. Since the SuperHyperSet of the SuperHyperVertices,is the SuperHyperSet Ss of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex and they are SuperHyperForcing. Since it’sthe minimum cardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. There’s only more than one SuperHyperVertex outside the intended SuperHyperSet,Thus the non-obvious SuperHyperForcing,is up. The non-obvious simple type-SuperHyperSet of the SuperHyperForcing,is a SuperHyperSet,excludes only more than one SuperHyperVertex in a connected neutrosophic SuperHyperGraph
-
On the Figure 17, the SuperHyperNotion, namely, SuperHyperForcing, is up. There’s neither empty SuperHyperEdge nor loop SuperHyperEdge. The SuperHyperSet of SuperHyperVertices,is the simple type-SuperHyperSet of the SuperHyperForcing. The SuperHyperSet of the SuperHyperVertices,is the minimum cardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. In There’s not only one SuperHyperVertex outside the intended SuperHyperSet. Thus the non-obvious SuperHyperForcing is up. The obvious simple type-SuperHyperSet of the SuperHyperForcing is a SuperHyperSet excludes only one SuperHyperVertex in a connected neutrosophic SuperHyperGraph But the SuperHyperSet of SuperHyperVertices,has more than one SuperHyperVertex outside the intended SuperHyperSet. Thus the non-obvious simple type-SuperHyperSet of the SuperHyperForcing is up. To sum them up, the SuperHyperSet of SuperHyperVertices,is the non-obvious simple type-SuperHyperSet of the SuperHyperForcing. Since the SuperHyperSet of the SuperHyperVertices,is the SuperHyperSet Ss of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex and they are SuperHyperForcing. Since it’sthe minimum cardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. There’s only more than one SuperHyperVertex outside the intended SuperHyperSet,Thus the non-obvious SuperHyperForcing,is up. The non-obvious simple type-SuperHyperSet of the SuperHyperForcing,is a SuperHyperSet,excludes only more than one SuperHyperVertex in a connected neutrosophic SuperHyperGraph
- On the Figure 18, the SuperHyperNotion, namely, SuperHyperForcing, is up. There’s neither empty SuperHyperEdge nor loop SuperHyperEdge. The SuperHyperSet of SuperHyperVertices, is the simple type-SuperHyperSet of the SuperHyperForcing. The SuperHyperSet of the SuperHyperVertices, is the minimum cardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. In There’s not only one SuperHyperVertex outside the intended SuperHyperSet. Thus the non-obvious SuperHyperForcing is up. The obvious simple type-SuperHyperSet of the SuperHyperForcing is a SuperHyperSet excludes only one SuperHyperVertex in a connected neutrosophic SuperHyperGraph But the SuperHyperSet of SuperHyperVertices, has more than one SuperHyperVertex outside the intended SuperHyperSet. Thus the non-obvious simple type-SuperHyperSet of the SuperHyperForcing is up. To sum them up, the SuperHyperSet of SuperHyperVertices, is the non-obvious simple type-SuperHyperSet of the SuperHyperForcing. Since the SuperHyperSet of the SuperHyperVertices, is the SuperHyperSet Ss of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex and they are SuperHyperForcing. Since it’sthe minimum cardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. There’s only more than one SuperHyperVertex outside the intended SuperHyperSet, Thus the non-obvious SuperHyperForcing, is up. The non-obvious simple type-SuperHyperSet of the SuperHyperForcing, is a SuperHyperSet, excludes only more than one SuperHyperVertex in a connected neutrosophic SuperHyperGraph
-
On the Figure 19, the SuperHyperNotion, namely, SuperHyperForcing, is up. There’s neither empty SuperHyperEdge nor loop SuperHyperEdge. The SuperHyperSet of SuperHyperVertices,is the simple type-SuperHyperSet of the SuperHyperForcing. The SuperHyperSet of the SuperHyperVertices,is the minimum cardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. In There’s not only one SuperHyperVertex outside the intended SuperHyperSet. Thus the non-obvious SuperHyperForcing is up. The obvious simple type-SuperHyperSet of the SuperHyperForcing is a SuperHyperSet excludes only one SuperHyperVertex in a connected neutrosophic SuperHyperGraph But the SuperHyperSet of SuperHyperVertices,has more than one SuperHyperVertex outside the intended SuperHyperSet. Thus the non-obvious simple type-SuperHyperSet of the SuperHyperForcing is up. To sum them up, the SuperHyperSet of SuperHyperVertices,is the non-obvious simple type-SuperHyperSet of the SuperHyperForcing. Since the SuperHyperSet of the SuperHyperVertices,is the SuperHyperSet Ss of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex and they are SuperHyperForcing. Since it’sthe minimum cardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. There’s only more than one SuperHyperVertex outside the intended SuperHyperSet,Thus the non-obvious SuperHyperForcing,is up. The non-obvious simple type-SuperHyperSet of the SuperHyperForcing,is a SuperHyperSet,excludes only more than one SuperHyperVertex in a connected neutrosophic SuperHyperGraph
-
On the Figure 20, the SuperHyperNotion, namely, SuperHyperForcing, is up. There’s neither empty SuperHyperEdge nor loop SuperHyperEdge. The SuperHyperSet of SuperHyperVertices,is the simple type-SuperHyperSet of the SuperHyperForcing. The SuperHyperSet of the SuperHyperVertices,is the minimum cardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. In There’s not only one SuperHyperVertex outside the intended SuperHyperSet. Thus the non-obvious SuperHyperForcing is up. The obvious simple type-SuperHyperSet of the SuperHyperForcing is a SuperHyperSet excludes only one SuperHyperVertex in a connected neutrosophic SuperHyperGraph But the SuperHyperSet of SuperHyperVertices,has more than one SuperHyperVertex outside the intended SuperHyperSet. Thus the non-obvious simple type-SuperHyperSet of the SuperHyperForcing is up. To sum them up, the SuperHyperSet of SuperHyperVertices,is the non-obvious simple type-SuperHyperSet of the SuperHyperForcing. Since the SuperHyperSet of the SuperHyperVertices,is the SuperHyperSet Ss of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex and they are SuperHyperForcing. Since it’sthe minimum cardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. There’s only more than one SuperHyperVertex outside the intended SuperHyperSet,Thus the non-obvious SuperHyperForcing,is up. The non-obvious simple type-SuperHyperSet of the SuperHyperForcing,is a SuperHyperSet,excludes only more than one SuperHyperVertex in a connected neutrosophic SuperHyperGraph
Figure 1.
The SuperHyperGraphs Associated to the Notions of SuperHyperForcing in the Examples (1) and (2).
Figure 1.
The SuperHyperGraphs Associated to the Notions of SuperHyperForcing in the Examples (1) and (2).

Figure 2.
The SuperHyperGraphs Associated to the Notions of SuperHyperForcing in the Examples (1) and (2).
Figure 2.
The SuperHyperGraphs Associated to the Notions of SuperHyperForcing in the Examples (1) and (2).

Figure 3.
The SuperHyperGraphs Associated to the Notions of SuperHyperForcing in the Examples (1) and (2).
Figure 3.
The SuperHyperGraphs Associated to the Notions of SuperHyperForcing in the Examples (1) and (2).

Figure 4.
The SuperHyperGraphs Associated to the Notions of SuperHyperForcing in the Examples (1) and (2).
Figure 4.
The SuperHyperGraphs Associated to the Notions of SuperHyperForcing in the Examples (1) and (2).
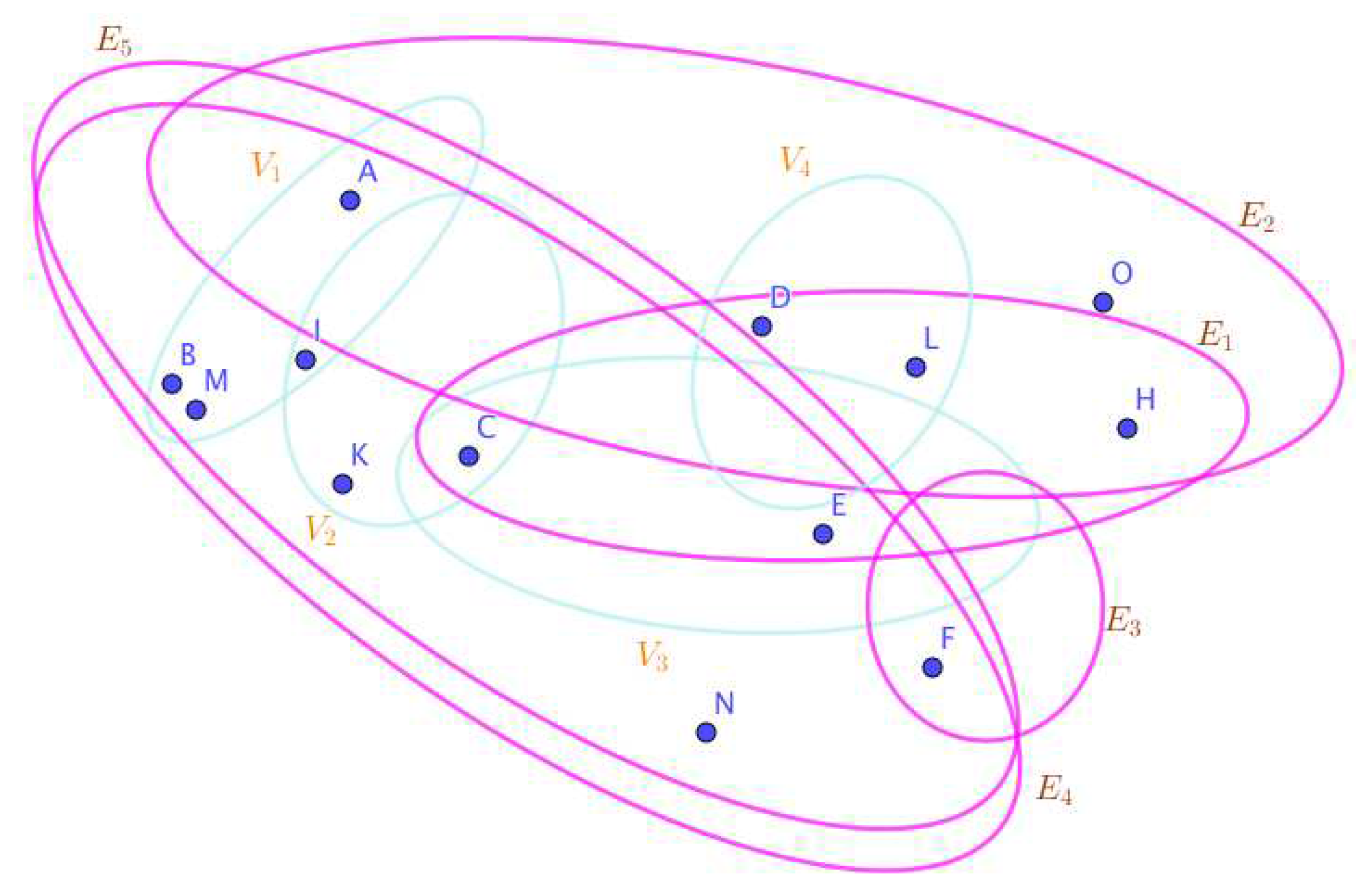
Figure 5.
The SuperHyperGraphs Associated to the Notions of SuperHyperForcing in the Examples (1) and (2).
Figure 5.
The SuperHyperGraphs Associated to the Notions of SuperHyperForcing in the Examples (1) and (2).
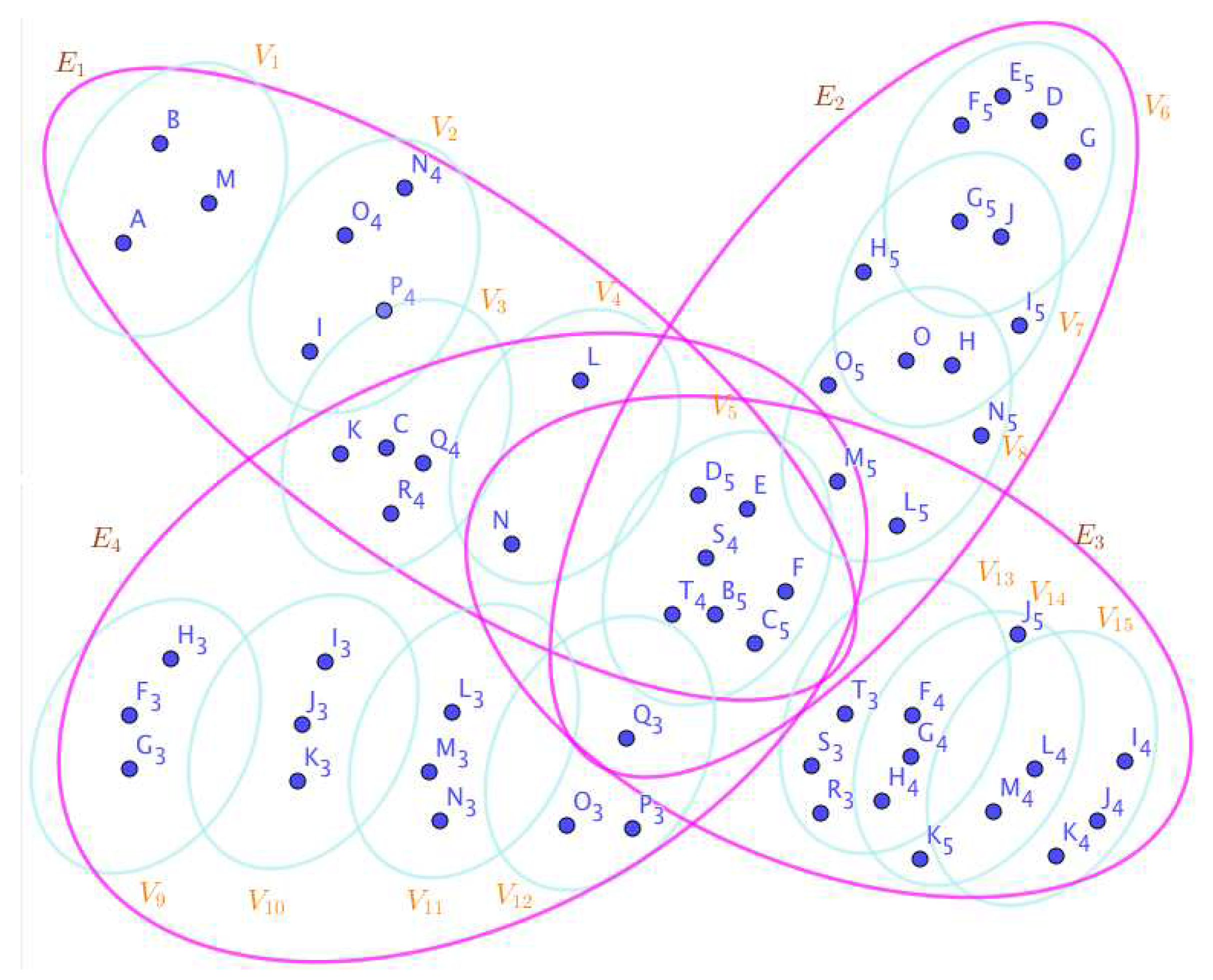
Figure 6.
The SuperHyperGraphs Associated to the Notions of SuperHyperForcing in the Examples (1) and (2).
Figure 6.
The SuperHyperGraphs Associated to the Notions of SuperHyperForcing in the Examples (1) and (2).
Figure 7.
The SuperHyperGraphs Associated to the Notions of SuperHyperForcing in the Examples (1) and (2).
Figure 7.
The SuperHyperGraphs Associated to the Notions of SuperHyperForcing in the Examples (1) and (2).

Figure 8.
The SuperHyperGraphs Associated to the Notions of SuperHyperForcing in the Examples (1) and (2).
Figure 8.
The SuperHyperGraphs Associated to the Notions of SuperHyperForcing in the Examples (1) and (2).

Figure 9.
The SuperHyperGraphs Associated to the Notions of SuperHyperForcing in the Examples (1) and (2).
Figure 9.
The SuperHyperGraphs Associated to the Notions of SuperHyperForcing in the Examples (1) and (2).

Figure 10.
The SuperHyperGraphs Associated to the Notions of SuperHyperForcing in the Examples (1) and (2).
Figure 10.
The SuperHyperGraphs Associated to the Notions of SuperHyperForcing in the Examples (1) and (2).

Figure 11.
The SuperHyperGraphs Associated to the Notions of SuperHyperForcing in the Examples (1) and (2).
Figure 11.
The SuperHyperGraphs Associated to the Notions of SuperHyperForcing in the Examples (1) and (2).
Figure 12.
The SuperHyperGraphs Associated to the Notions of SuperHyperForcing in the Examples (1) and (2).
Figure 12.
The SuperHyperGraphs Associated to the Notions of SuperHyperForcing in the Examples (1) and (2).
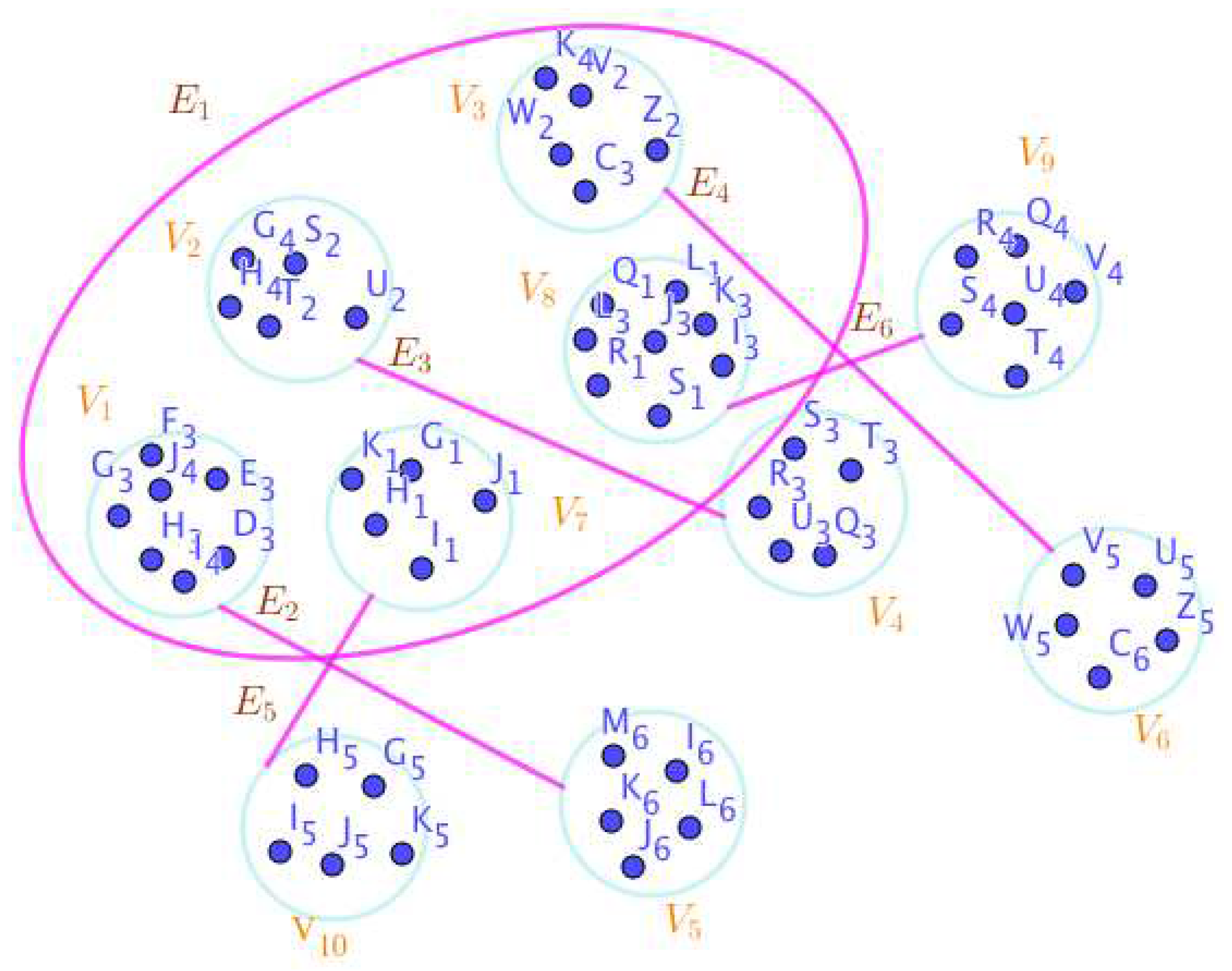
Figure 13.
The SuperHyperGraphs Associated to the Notions of SuperHyperForcing in the Examples (1) and (2).
Figure 13.
The SuperHyperGraphs Associated to the Notions of SuperHyperForcing in the Examples (1) and (2).

Figure 14.
The SuperHyperGraphs Associated to the Notions of SuperHyperForcing in the Examples (1) and (2).
Figure 14.
The SuperHyperGraphs Associated to the Notions of SuperHyperForcing in the Examples (1) and (2).
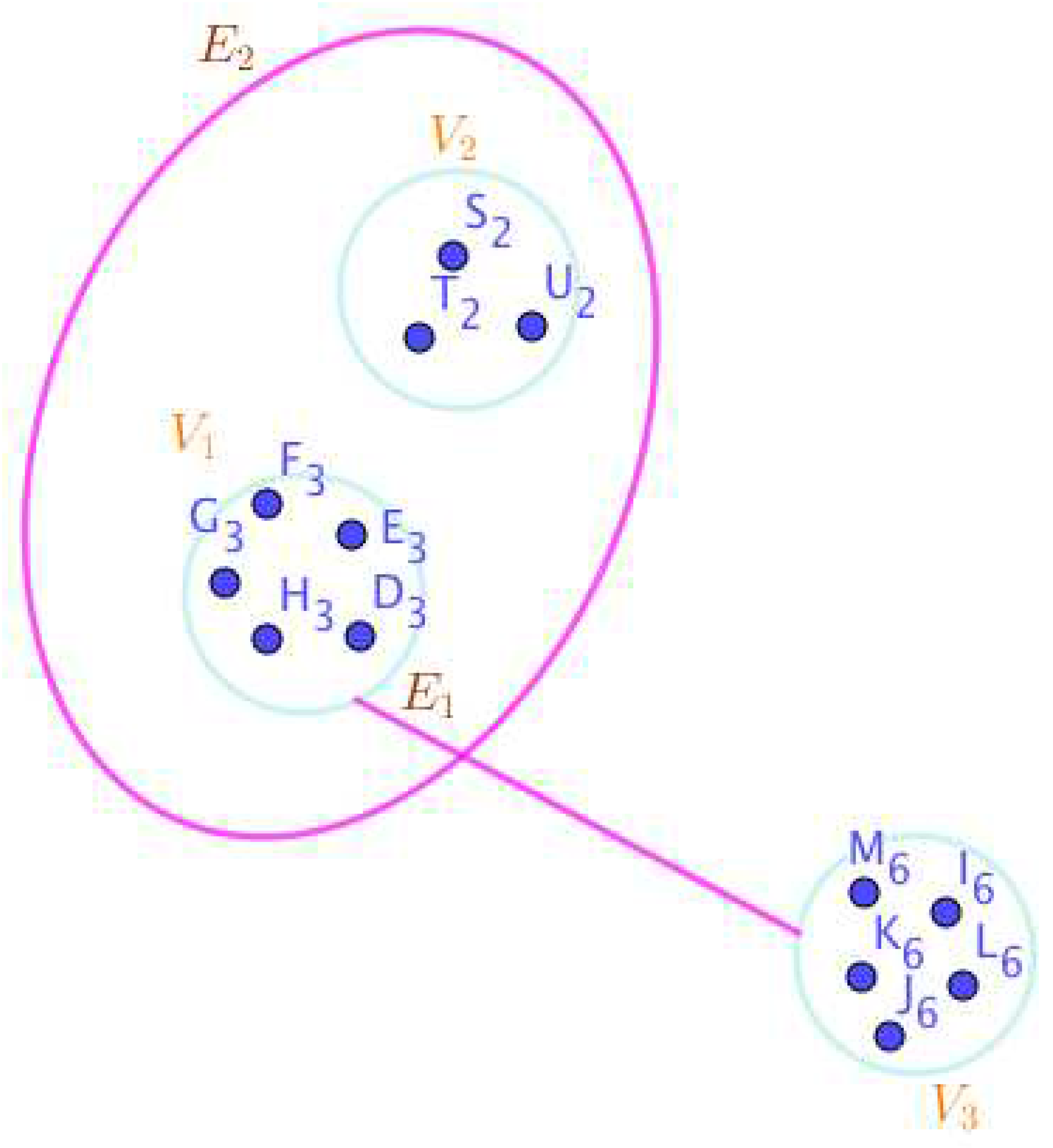
Figure 15.
The SuperHyperGraphs Associated to the Notions of SuperHyperForcing in the Examples (1) and (2).
Figure 15.
The SuperHyperGraphs Associated to the Notions of SuperHyperForcing in the Examples (1) and (2).

Figure 16.
The SuperHyperGraphs Associated to the Notions of SuperHyperForcing in the Examples (1) and (2).
Figure 16.
The SuperHyperGraphs Associated to the Notions of SuperHyperForcing in the Examples (1) and (2).
Figure 17.
The SuperHyperGraphs Associated to the Notions of SuperHyperForcing in the Examples (1) and (2).
Figure 17.
The SuperHyperGraphs Associated to the Notions of SuperHyperForcing in the Examples (1) and (2).

Figure 18.
The SuperHyperGraphs Associated to the Notions of SuperHyperForcing in the Examples (1) and (2).
Figure 18.
The SuperHyperGraphs Associated to the Notions of SuperHyperForcing in the Examples (1) and (2).

Figure 19.
The SuperHyperGraphs Associated to the Notions of SuperHyperForcing in the Examples (1) and (2).
Figure 19.
The SuperHyperGraphs Associated to the Notions of SuperHyperForcing in the Examples (1) and (2).

Figure 20.
The SuperHyperGraphs Associated to the Notions of SuperHyperForcing in the Examples (1) and (2).
Figure 20.
The SuperHyperGraphs Associated to the Notions of SuperHyperForcing in the Examples (1) and (2).

Proposition 1.
Assume a connected neutrosophic SuperHyperGraph Then in the worst case, literally, is a SuperHyperForcing. In other words, the most cardinality, the upper sharp bound for cardinality, of SuperHyperForcing is the cardinality of
Proof.
Assume a connected neutrosophic SuperHyperGraph The SuperHyperSet of the SuperHyperVertices V is a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex but it isn’t a SuperHyperForcing. Since it doesn’t have the minimum cardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. There’s only one SuperHyperVertex outside the intended SuperHyperSet, Thus the obvious SuperHyperForcing, is up. The obvious simple type-SuperHyperSet of the SuperHyperForcing, is a SuperHyperSet, excludes only one SuperHyperVertex in a connected neutrosophic SuperHyperGraph □
Proposition 2.
Assume a connected neutrosophic SuperHyperGraph Then the extreme number of SuperHyperForcing has, the most cardinality, the upper sharp bound for cardinality, is the extreme cardinality of V if there’s a SuperHyperForcing with the most cardinality, the upper sharp bound for cardinality.
Proof.
Assume a connected neutrosophic SuperHyperGraph Consider there’s a SuperHyperForcing with the most cardinality, the upper sharp bound for cardinality. The SuperHyperSet of the SuperHyperVertices V is a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex but it isn’t a SuperHyperForcing. Since it doesn’t have the minimum cardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. There’s only one SuperHyperVertex outside the intended SuperHyperSet, Thus the obvious SuperHyperForcing, is up. The obvious simple type-SuperHyperSet of the SuperHyperForcing, is a SuperHyperSet, excludes only one SuperHyperVertex in a connected neutrosophic SuperHyperGraph It implies that extreme number of SuperHyperForcing has, the most cardinality, the upper sharp bound for cardinality, is choose Thus it induces that the extreme number of SuperHyperForcing has, the most cardinality, the upper sharp bound for cardinality, is the extreme cardinality of V if there’s a SuperHyperForcing with the most cardinality, the upper sharp bound for cardinality. □
Proposition 3.
Assume a connected neutrosophic SuperHyperGraph If a SuperHyperEdge has z SuperHyperVertices, then number of those SuperHyperVertices from that SuperHyperEdge belong to any SuperHyperForcing.
Proof.
Assume a connected neutrosophic SuperHyperGraph Let a SuperHyperEdge has z SuperHyperVertices. Consider number of those SuperHyperVertices from that SuperHyperEdge belong to any given SuperHyperSet of the SuperHyperVertices. The SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex but there are two white SuperHyperNeighbors outside implying there’s no SuperHyperVertex to the SuperHyperSet S does the “the color-change rule”. So it doesn’t have the minimum cardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. There’s only one SuperHyperVertex outside the intended SuperHyperSet. Thus the obvious SuperHyperForcing is up. The obvious simple type-SuperHyperSet of the SuperHyperForcing is a SuperHyperSet excludes only one SuperHyperVertex in a connected neutrosophic SuperHyperGraph Thus all the following SuperHyperSets of SuperHyperVertices are the simple type-SuperHyperSet of the SuperHyperForcing. It’s the contradiction to the SuperHyperSet S is a SuperHyperForcing. Thus any given SuperHyperSet of the SuperHyperVertices contains the number of those SuperHyperVertices from that SuperHyperEdge with z SuperHyperVertices less than isn’t a SuperHyperForcing. Thus if a SuperHyperEdge has z SuperHyperVertices, then number of those SuperHyperVertices from that SuperHyperEdge belong to any SuperHyperForcing. □
Proposition 4.
Assume a connected neutrosophic SuperHyperGraph Every SuperHyperEdge has only one unique SuperHyperVertex outside of SuperHyperForcing. In other words, every SuperHyperEdge has only one unique white SuperHyperVertex.
Proof.
Assume a connected neutrosophic SuperHyperGraph Let a SuperHyperEdge has some SuperHyperVertices. Consider some numbers of those SuperHyperVertices from that SuperHyperEdge excluding two unique SuperHyperVertices, belong to any given SuperHyperSet of the SuperHyperVertices. The SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex but there are two white SuperHyperNeighbors outside implying there’s no SuperHyperVertex to the SuperHyperSet S does the “the color-change rule”. So it doesn’t have the minimum cardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. There’s only one SuperHyperVertex outside the intended SuperHyperSet. Thus the obvious SuperHyperForcing is up. The obvious simple type-SuperHyperSet of the SuperHyperForcing is a SuperHyperSet excludes only one SuperHyperVertex in a connected neutrosophic SuperHyperGraph Thus all the following SuperHyperSets of SuperHyperVertices are the simple type-SuperHyperSet of the SuperHyperForcing. It’s the contradiction to the SuperHyperSet S is a SuperHyperForcing. Thus any given SuperHyperSet of the SuperHyperVertices contains the number of those SuperHyperVertices from that SuperHyperEdge with some SuperHyperVertices less than excluding one unique SuperHyperVertex, isn’t a SuperHyperForcing. Thus if a SuperHyperEdge has some SuperHyperVertices, then, with excluding one unique SuperHyperVertex, the all number of those SuperHyperVertices from that SuperHyperEdge belong to any SuperHyperForcing. Thus, in a connected neutrosophic SuperHyperGraph every SuperHyperEdge has only one unique SuperHyperVertex outside of SuperHyperForcing. In other words, every SuperHyperEdge has only one unique white SuperHyperVertex. □
Proposition 5.
Assume a connected neutrosophic SuperHyperGraph The all exterior SuperHyperVertices belong to any SuperHyperForcing if for any of them, there’s only one interior SuperHyperVertex is a SuperHyperNeighbor to any of them.
Proof.
Assume a connected neutrosophic SuperHyperGraph Let a SuperHyperEdge has some SuperHyperVertices. Consider some numbers of those SuperHyperVertices from that SuperHyperEdge excluding two unique SuperHyperVertices, belong to any given SuperHyperSet of the SuperHyperVertices. The SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex but there are two white SuperHyperNeighbors outside implying there’s no SuperHyperVertex to the SuperHyperSet S does the “the color-change rule”. So it doesn’t have the minimum cardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. Consider some numbers of those SuperHyperVertices from that SuperHyperEdge, without any exclusion on some SuperHyperVertices, belong to any given SuperHyperSet of the SuperHyperVertices. The SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex but it implies it doesn’t have the minimum cardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. There’s only one SuperHyperVertex outside the intended SuperHyperSet. Thus the obvious SuperHyperForcing is up. The obvious simple type-SuperHyperSet of the SuperHyperForcing is a SuperHyperSet excludes only one SuperHyperVertex in a connected neutrosophic SuperHyperGraph Thus all the following SuperHyperSets of SuperHyperVertices are the simple type-SuperHyperSet of the SuperHyperForcing. It’s the contradiction to the SuperHyperSet S is a SuperHyperForcing. Thus any given SuperHyperSet of the SuperHyperVertices contains the number of those SuperHyperVertices from that SuperHyperEdge with some SuperHyperVertices less than excluding one unique SuperHyperVertex, isn’t a SuperHyperForcing. Thus if a SuperHyperEdge has some SuperHyperVertices, then, with excluding one unique SuperHyperVertex, the all number of those SuperHyperVertices from that SuperHyperEdge belong to any SuperHyperForcing. Thus, in a connected neutrosophic SuperHyperGraph every SuperHyperEdge has only one unique SuperHyperVertex outside of SuperHyperForcing. In other words, every SuperHyperEdge has only one unique white SuperHyperVertex. In a connected neutrosophic SuperHyperGraph the all exterior SuperHyperVertices belong to any SuperHyperForcing if for any of them, there’s only one interior SuperHyperVertex is a SuperHyperNeighbor to any of them. □
Proposition 6.
Assume a connected neutrosophic SuperHyperGraph The any SuperHyperForcing only contains all interior SuperHyperVertices and all exterior SuperHyperVertices where any of them has one SuperHyperNeighbor out.
Proof.
Assume a connected neutrosophic SuperHyperGraph Let a SuperHyperEdge has some SuperHyperVertices. Consider some numbers of those SuperHyperVertices from that SuperHyperEdge excluding two unique SuperHyperVertices, belong to any given SuperHyperSet of the SuperHyperVertices. The SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex but there are two white SuperHyperNeighbors outside implying there’s no SuperHyperVertex to the SuperHyperSet S does the “the color-change rule”. So it doesn’t have the minimum cardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. Consider some numbers of those SuperHyperVertices from that SuperHyperEdge, without any exclusion on some SuperHyperVertices, belong to any given SuperHyperSet of the SuperHyperVertices. The SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex but it implies it doesn’t have the minimum cardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. There’s only one SuperHyperVertex outside the intended SuperHyperSet. Thus the obvious SuperHyperForcing is up. The obvious simple type-SuperHyperSet of the SuperHyperForcing is a SuperHyperSet excludes only one SuperHyperVertex in a connected neutrosophic SuperHyperGraph Thus all the following SuperHyperSets of SuperHyperVertices are the simple type-SuperHyperSet of the SuperHyperForcing. It’s the contradiction to the SuperHyperSet S is a SuperHyperForcing. Thus any given SuperHyperSet of the SuperHyperVertices contains the number of those SuperHyperVertices from that SuperHyperEdge with some SuperHyperVertices less than excluding one unique SuperHyperVertex, isn’t a SuperHyperForcing. Thus if a SuperHyperEdge has some SuperHyperVertices, then, with excluding one unique SuperHyperVertex, the all number of those SuperHyperVertices from that SuperHyperEdge belong to any SuperHyperForcing. Thus, in a connected neutrosophic SuperHyperGraph every SuperHyperEdge has only one unique SuperHyperVertex outside of SuperHyperForcing. In other words, every SuperHyperEdge has only one unique white SuperHyperVertex. In a connected neutrosophic SuperHyperGraph the any SuperHyperForcing only contains all interior SuperHyperVertices and all exterior SuperHyperVertices where any of them has one SuperHyperNeighbor out. □
Remark 1.
The words “SuperHyperForcing” and “SuperHyperDominating” refer to the minimum type-style. In other words, they refer to both the minimum number and the SuperHyperSet with the minimum cardinality.
Proposition 7.
Assume a connected neutrosophic SuperHyperGraph A complement of SuperHyperForcing is the SuperHyperDominating.
Proof.
Assume a connected neutrosophic SuperHyperGraph By applying the Proposition (6), the results are up. Thus in a connected neutrosophic SuperHyperGraph a complement of SuperHyperForcing is the SuperHyperDominating. □
3. Neutrosophic SuperHyperForcing
For the sake of having neutrosophic SuperHyperForcing, there’s a need to “redefine” the notion of “neutrosophic SuperHyperGraph”. The SuperHyperVertices and the SuperHyperEdges are assigned by the labels from the letters of the alphabets. In this procedure, there’s the usage of the position of labels to assign to the values.
Definition 3.
Assume a neutrosophic SuperHyperGraph. It’s redefined neutrosophic SuperHyperGraph if the Table 1 holds.
It’s useful to define “neutrosophic” version of SuperHyperClasses. Since there’s more ways to get neutrosophic type-results to make neutrosophic SuperHyperForcing more understandable.
Definition 4.
Assume a neutrosophic SuperHyperGraph. There are some neutrosophic SuperHyperClasses if the Table 2 holds. Thus SuperHyperPath, SuperHyperCycle, SuperHyperStar, SuperHyperBipartite, SuperHyperMultiPartite, and SuperHyperWheel, are neutrosophic SuperHyperPath, neutrosophic SuperHyperCycle, neutrosophic SuperHyperStar, neutrosophic SuperHyperBipartite, neutrosophic SuperHyperMultiPartite, and neutrosophic SuperHyperWheel if the Table 2 holds.
It’s useful to define “neutrosophic” version of SuperHyperForcing. Since there’s more ways to get type-results to make SuperHyperForcing more understandable.
For the sake of having neutrosophic SuperHyperForcing, there’s a need to “redefine” the notion of “SuperHyperForcing”. The SuperHyperVertices and the SuperHyperEdges are assigned by the labels from the letters of the alphabets. In this procedure, there’s the usage of the position of labels to assign to the values.
Definition 5.
Definition 6.
((neutrosophic) SuperHyperForcing).
Assume a SuperHyperGraph. Then
- a SuperHyperForcing for a neutrosophic SuperHyperGraph is the minimum cardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex;
- a neutrosophic SuperHyperForcing for a neutrosophic SuperHyperGraph is the minimum neutrosophic cardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex.
Definition 7.
((neutrosophic) SuperHyperForcing).
Assume a SuperHyperGraph. Then
-
an SuperHyperForcing is a minimal SuperHyperForcing of SuperHyperVertices with minimum cardinality such that either of the following expressions hold for the (neutrosophic) cardinalities of SuperHyperNeighbors ofThe Expression (5), holds if S is an SuperHyperOffensive. And the Expression (6), holds if S is an SuperHyperDefensive;
-
a neutrosophic SuperHyperForcing is a minimal neutrosophic SuperHyperForcing of SuperHyperVertices with minimum neutrosophic cardinality such that either of the following expressions hold for the neutrosophic cardinalities of SuperHyperNeighbors ofThe Expression (7), holds if S is a neutrosophicSuperHyperOffensive. And the Expression (8), holds if S is a neutrosophicSuperHyperDefensive.
Example 2.
Assume the neutrosophic SuperHyperGraphs in the Figure 1, Figure 2, Figure 3, Figure 4, Figure 5, Figure 6, Figure 7, Figure 8, Figure 9, Figure 10, Figure 11, Figure 12, Figure 13, Figure 14, Figure 15, Figure 16, Figure 17, Figure 18, Figure 19, and Figure 20.
-
On the Figure 1, the neutrosophic SuperHyperNotion, namely, neutrosophic SuperHyperForcing, is up. is the neutrosophic type-SuperHyperSet of the neutrosophic SuperHyperForcing. The details are followed by the upcoming statements. and are some empty SuperHyperEdges but is a loop SuperHyperEdge and is an SuperHyperEdge. Thus in the terms of SuperHyperNeighbor, there’s only one SuperHyperEdge, namely, The SuperHyperVertex, is isolated means that there’s no SuperHyperEdge has it as an endpoint. Thus SuperHyperVertex, is contained in every given neutrosophic SuperHyperForcing. All the following SuperHyperSets of SuperHyperVertices are the simple type-SuperHyperSet of the neutrosophic SuperHyperForcing.The SuperHyperSet of the SuperHyperVertices, is a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex but it isn’t a neutrosophic SuperHyperForcing. Since it doesn’t have the minimum neutrosophic SuperHyperCardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. There’s only one SuperHyperVertex outside the intended SuperHyperSet. Thus the obvious neutrosophic SuperHyperForcing is up. The obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing is a SuperHyperSet excludes only one SuperHyperVertex in a connected neutrosophic SuperHyperGraph
-
On the Figure 2, the neutrosophic SuperHyperNotion, namely, neutrosophic SuperHyperForcing, is up. is the neutrosophic type-SuperHyperSet of the neutrosophic SuperHyperForcing. The details are followed by the upcoming statements. and are some empty SuperHyperEdges but is an SuperHyperEdge. Thus in the terms of SuperHyperNeighbor, there’s only one SuperHyperEdge, namely, The SuperHyperVertex, is isolated means that there’s no SuperHyperEdge has it as an endpoint. Thus SuperHyperVertex, is contained in every given neutrosophic SuperHyperForcing. All the following SuperHyperSets of SuperHyperVertices are the simple type-SuperHyperSet of the neutrosophic SuperHyperForcing.The SuperHyperSet of the SuperHyperVertices, is a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex but it isn’t a neutrosophic SuperHyperForcing. Since it doesn’t have the minimum neutrosophic SuperHyperCardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. There’s only one SuperHyperVertex outside the intended SuperHyperSet. Thus the obvious neutrosophic SuperHyperForcing is up. The obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing is a SuperHyperSet excludes only one SuperHyperVertex in a connected neutrosophic SuperHyperGraph
-
On the Figure 3, the neutrosophic SuperHyperNotion, namely, neutrosophic SuperHyperForcing, is up. is the neutrosophic type-SuperHyperSet of the neutrosophic SuperHyperForcing. The details are followed by the upcoming statements. and are some empty SuperHyperEdges but is an SuperHyperEdge. Thus in the terms of SuperHyperNeighbor, there’s only one SuperHyperEdge, namely, All the following SuperHyperSets of SuperHyperVertices are the simple type-SuperHyperSet of the neutrosophic SuperHyperForcing.The SuperHyperSet of the SuperHyperVertices, is a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex but it isn’t a neutrosophic SuperHyperForcing. Since it doesn’t have the minimum neutrosophic SuperHyperCardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. There’s only one SuperHyperVertex outside the intended SuperHyperSet. Thus the obvious neutrosophic SuperHyperForcing is up. The obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing is a SuperHyperSet excludes only one SuperHyperVertex in a connected neutrosophic SuperHyperGraph Thus all the following SuperHyperSets of SuperHyperVertices are the simple type-SuperHyperSet of the neutrosophic SuperHyperForcing.since the SuperHyperSets of the SuperHyperVertices, are the SuperHyperSets Ss of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex and they are neutrosophic SuperHyperForcing. Since they’vethe minimum neutrosophic SuperHyperCardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. There’s only one SuperHyperVertex outside the intended SuperHyperSet, Thus the obvious neutrosophic SuperHyperForcing, is up. The obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing, is a SuperHyperSet, excludes only one SuperHyperVertex in a connected neutrosophic SuperHyperGraph
- On the Figure 4, the neutrosophic SuperHyperNotion, namely, neutrosophic SuperHyperForcing, is up. is the neutrosophic type-SuperHyperSet of the neutrosophic SuperHyperForcing. The details are followed by the upcoming statements. There’s no empty SuperHyperEdge but are a loop SuperHyperEdge on and there are some SuperHyperEdges, namely, on alongside on and on The SuperHyperSet of SuperHyperVertices, is the simple type-SuperHyperSet of the neutrosophic SuperHyperForcing. The SuperHyperSet of the SuperHyperVertices, is the minimum neutrosophic SuperHyperCardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. In There’s not only one SuperHyperVertex outside the intended SuperHyperSet. Thus the non-obvious neutrosophic SuperHyperForcing is up. The obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing is a SuperHyperSet excludes only one SuperHyperVertex in a connected neutrosophic SuperHyperGraph But the SuperHyperSet of SuperHyperVertices, has more than one SuperHyperVertex outside the intended SuperHyperSet. Thus the non-obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing is up. To sum them up, the SuperHyperSet of SuperHyperVertices, is the non-obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing. Since the SuperHyperSet of the SuperHyperVertices, is the SuperHyperSet Ss of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex and they are neutrosophic SuperHyperForcing. Since it’sthe minimum neutrosophic SuperHyperCardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. There’s only more than one SuperHyperVertex outside the intended SuperHyperSet, Thus the non-obvious neutrosophic SuperHyperForcing, is up. The non-obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing, is a SuperHyperSet, excludes only more than one SuperHyperVertex in a connected neutrosophic SuperHyperGraph
-
On the Figure 5, the neutrosophic SuperHyperNotion, namely, neutrosophic SuperHyperForcing, is up.is the neutrosophic type-SuperHyperSet of the neutrosophic SuperHyperForcing. The details are followed by the upcoming statements. There’s neither empty SuperHyperEdge nor loop SuperHyperEdge. The SuperHyperSet of SuperHyperVertices, is the simple type-SuperHyperSet of the neutrosophic SuperHyperForcing. The SuperHyperSet of the SuperHyperVertices, is the minimum neutrosophic SuperHyperCardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. In There’s not only one SuperHyperVertex outside the intended SuperHyperSet. Thus the non-obvious neutrosophic SuperHyperForcing is up. The obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing is a SuperHyperSet excludes only one SuperHyperVertex in a connected neutrosophic SuperHyperGraph But the SuperHyperSet of SuperHyperVertices, has more than one SuperHyperVertex outside the intended SuperHyperSet. Thus the non-obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing is up. To sum them up, the SuperHyperSet of SuperHyperVertices, is the non-obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing. Since the SuperHyperSet of the SuperHyperVertices, is the SuperHyperSet Ss of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex and they are neutrosophic SuperHyperForcing. Since it’sthe minimum neutrosophic SuperHyperCardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. There’s only more than one SuperHyperVertex outside the intended SuperHyperSet, Thus the non-obvious neutrosophic SuperHyperForcing, is up. The non-obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing, is a SuperHyperSet, excludes only more than one SuperHyperVertex in a connected neutrosophic SuperHyperGraph
-
On the Figure 6, the neutrosophic SuperHyperNotion, namely, neutrosophic SuperHyperForcing, is up.is the neutrosophic type-SuperHyperSet of the neutrosophic SuperHyperForcing. The details are followed by the upcoming statements. There’s neither empty SuperHyperEdge nor loop SuperHyperEdge. The SuperHyperSet of SuperHyperVertices, is the simple type-SuperHyperSet of the neutrosophic SuperHyperForcing. The SuperHyperSet of the SuperHyperVertices, is the minimum neutrosophic SuperHyperCardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. In There’s not only one SuperHyperVertex outside the intended SuperHyperSet. Thus the non-obvious neutrosophic SuperHyperForcing is up. The obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing is a SuperHyperSet excludes only one SuperHyperVertex in a connected neutrosophic SuperHyperGraph But the SuperHyperSet of SuperHyperVertices, has more than one SuperHyperVertex outside the intended SuperHyperSet. Thus the non-obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing is up. To sum them up, the SuperHyperSet of SuperHyperVertices, is the non-obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing. Since the SuperHyperSet of the SuperHyperVertices, is the SuperHyperSet Ss of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex and they are neutrosophic SuperHyperForcing. Since it’sthe minimum neutrosophic SuperHyperCardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. There’s only more than one SuperHyperVertex outside the intended SuperHyperSet, Thus the non-obvious neutrosophic SuperHyperForcing, is up. The non-obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing, is a SuperHyperSet, excludes only more than one SuperHyperVertex in a connected neutrosophic SuperHyperGraph
-
On the Figure 7, the neutrosophic SuperHyperNotion, namely, neutrosophic SuperHyperForcing, is up.is the neutrosophic type-SuperHyperSet of the neutrosophic SuperHyperForcing. The details are followed by the upcoming statements. There’s neither empty SuperHyperEdge nor loop SuperHyperEdge. The SuperHyperSet of SuperHyperVertices, is the simple type-SuperHyperSet of the neutrosophic SuperHyperForcing. The SuperHyperSet of the SuperHyperVertices, is the minimum neutrosophic SuperHyperCardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. In There’s not only one SuperHyperVertex outside the intended SuperHyperSet. Thus the non-obvious neutrosophic SuperHyperForcing is up. The obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing is a SuperHyperSet excludes only one SuperHyperVertex in a connected neutrosophic SuperHyperGraph But the SuperHyperSet of SuperHyperVertices, has more than one SuperHyperVertex outside the intended SuperHyperSet. Thus the non-obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing is up. To sum them up, the SuperHyperSet of SuperHyperVertices, is the non-obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing. Since the SuperHyperSet of the SuperHyperVertices, is the SuperHyperSet Ss of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex and they are neutrosophic SuperHyperForcing. Since it’sthe minimum neutrosophic SuperHyperCardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. There’s only more than one SuperHyperVertex outside the intended SuperHyperSet, Thus the non-obvious neutrosophic SuperHyperForcing, is up. The non-obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing, is a SuperHyperSet, excludes only more than one SuperHyperVertex in a connected neutrosophic SuperHyperGraph
-
On the Figure 8, the neutrosophic SuperHyperNotion, namely, neutrosophic SuperHyperForcing, is up.is the neutrosophic type-SuperHyperSet of the neutrosophic SuperHyperForcing. The details are followed by the upcoming statements. There’s neither empty SuperHyperEdge nor loop SuperHyperEdge. The SuperHyperSet of SuperHyperVertices, is the simple type-SuperHyperSet of the neutrosophic SuperHyperForcing. The SuperHyperSet of the SuperHyperVertices, is the minimum neutrosophic SuperHyperCardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. In There’s not only one SuperHyperVertex outside the intended SuperHyperSet. Thus the non-obvious neutrosophic SuperHyperForcing is up. The obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing is a SuperHyperSet excludes only one SuperHyperVertex in a connected neutrosophic SuperHyperGraph But the SuperHyperSet of SuperHyperVertices, has more than one SuperHyperVertex outside the intended SuperHyperSet. Thus the non-obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing is up. To sum them up, the SuperHyperSet of SuperHyperVertices, is the non-obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing. Since the SuperHyperSet of the SuperHyperVertices, is the SuperHyperSet Ss of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex and they are neutrosophic SuperHyperForcing. Since it’sthe minimum neutrosophic SuperHyperCardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. There’s only more than one SuperHyperVertex outside the intended SuperHyperSet, Thus the non-obvious neutrosophic SuperHyperForcing, is up. The non-obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing, is a SuperHyperSet, excludes only more than one SuperHyperVertex in a connected neutrosophic SuperHyperGraph
-
On the Figure 9, the neutrosophic SuperHyperNotion, namely, neutrosophic SuperHyperForcing, is up.is the neutrosophic type-SuperHyperSet of the neutrosophic SuperHyperForcing. The details are followed by the upcoming statements. There’s neither empty SuperHyperEdge nor loop SuperHyperEdge. The SuperHyperSet of SuperHyperVertices, is the simple type-SuperHyperSet of the neutrosophic SuperHyperForcing. The SuperHyperSet of the SuperHyperVertices, is the minimum neutrosophic SuperHyperCardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. In There’s not only one SuperHyperVertex outside the intended SuperHyperSet. Thus the non-obvious neutrosophic SuperHyperForcing is up. The obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing is a SuperHyperSet excludes only one SuperHyperVertex in a connected neutrosophic SuperHyperGraph But the SuperHyperSet of SuperHyperVertices, has more than one SuperHyperVertex outside the intended SuperHyperSet. Thus the non-obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing is up. To sum them up, the SuperHyperSet of SuperHyperVertices, is the non-obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing. Since the SuperHyperSet of the SuperHyperVertices, is the SuperHyperSet Ss of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex and they are neutrosophic SuperHyperForcing. Since it’sthe minimum neutrosophic SuperHyperCardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. There’s only more than one SuperHyperVertex outside the intended SuperHyperSet, Thus the non-obvious neutrosophic SuperHyperForcing, is up. The non-obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing, is a SuperHyperSet, excludes only more than one SuperHyperVertex in a connected neutrosophic SuperHyperGraph
- On the Figure 10, the neutrosophic SuperHyperNotion, namely, neutrosophic SuperHyperForcing, is up. is the neutrosophic type-SuperHyperSet of the neutrosophic SuperHyperForcing. The details are followed by the upcoming statements. There’s neither empty SuperHyperEdge nor loop SuperHyperEdge. The SuperHyperSet of SuperHyperVertices, is the simple type-SuperHyperSet of the neutrosophic SuperHyperForcing. The SuperHyperSet of the SuperHyperVertices, is the minimum neutrosophic SuperHyperCardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. In There’s not only one SuperHyperVertex outside the intended SuperHyperSet. Thus the non-obvious neutrosophic SuperHyperForcing is up. The obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing is a SuperHyperSet excludes only one SuperHyperVertex in a connected neutrosophic SuperHyperGraph But the SuperHyperSet of SuperHyperVertices, has more than one SuperHyperVertex outside the intended SuperHyperSet. Thus the non-obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing is up. To sum them up, the SuperHyperSet of SuperHyperVertices, is the non-obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing. Since the SuperHyperSet of the SuperHyperVertices, is the SuperHyperSet Ss of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex and they are neutrosophic SuperHyperForcing. Since it’sthe minimum neutrosophic SuperHyperCardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. There’s only more than one SuperHyperVertex outside the intended SuperHyperSet, Thus the non-obvious neutrosophic SuperHyperForcing, is up. The non-obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing, is a SuperHyperSet, excludes only more than one SuperHyperVertex in a connected neutrosophic SuperHyperGraph
- On the Figure 11, the neutrosophic SuperHyperNotion, namely, neutrosophic SuperHyperForcing, is up. is the neutrosophic type-SuperHyperSet of the neutrosophic SuperHyperForcing. The details are followed by the upcoming statements. There’s neither empty SuperHyperEdge nor loop SuperHyperEdge. The SuperHyperSet of SuperHyperVertices, is the simple type-SuperHyperSet of the neutrosophic SuperHyperForcing. The SuperHyperSet of the SuperHyperVertices, is the minimum neutrosophic SuperHyperCardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. In There’s not only one SuperHyperVertex outside the intended SuperHyperSet. Thus the non-obvious neutrosophic SuperHyperForcing is up. The obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing is a SuperHyperSet excludes only one SuperHyperVertex in a connected neutrosophic SuperHyperGraph But the SuperHyperSet of SuperHyperVertices, has more than one SuperHyperVertex outside the intended SuperHyperSet. Thus the non-obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing is up. To sum them up, the SuperHyperSet of SuperHyperVertices, is the non-obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing. Since the SuperHyperSet of the SuperHyperVertices, is the SuperHyperSet Ss of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex and they are neutrosophic SuperHyperForcing. Since it’sthe minimum neutrosophic SuperHyperCardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. There’s only more than one SuperHyperVertex outside the intended SuperHyperSet, Thus the non-obvious neutrosophic SuperHyperForcing, is up. The non-obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing, is a SuperHyperSet, excludes only more than one SuperHyperVertex in a connected neutrosophic SuperHyperGraph
-
On the Figure 12, the neutrosophic SuperHyperNotion, namely, neutrosophic SuperHyperForcing, is up.is the neutrosophic type-SuperHyperSet of the neutrosophic SuperHyperForcing. The details are followed by the upcoming statements. is the neutrosophic type-SuperHyperSet of the neutrosophic SuperHyperForcing. The details are followed by the upcoming statements. There’s neither empty SuperHyperEdge nor loop SuperHyperEdge. The SuperHyperSet of SuperHyperVertices, is the simple type-SuperHyperSet of the neutrosophic SuperHyperForcing. The SuperHyperSet of the SuperHyperVertices, is the minimum neutrosophic SuperHyperCardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. In There’s not only one SuperHyperVertex outside the intended SuperHyperSet. Thus the non-obvious neutrosophic SuperHyperForcing is up. The obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing is a SuperHyperSet excludes only one SuperHyperVertex in a connected neutrosophic SuperHyperGraph But the SuperHyperSet of SuperHyperVertices, has more than one SuperHyperVertex outside the intended SuperHyperSet. Thus the non-obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing is up. To sum them up, the SuperHyperSet of SuperHyperVertices, is the non-obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing. Since the SuperHyperSet of the SuperHyperVertices, is the SuperHyperSet Ss of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex and they are neutrosophic SuperHyperForcing. Since it’sthe minimum neutrosophic SuperHyperCardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. There’s only more than one SuperHyperVertex outside the intended SuperHyperSet, Thus the non-obvious neutrosophic SuperHyperForcing, is up. The non-obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing, is a SuperHyperSet, excludes only more than one SuperHyperVertex in a connected neutrosophic SuperHyperGraph
- On the Figure 13, the neutrosophic SuperHyperNotion, namely, neutrosophic SuperHyperForcing, is up. is the neutrosophic type-SuperHyperSet of the neutrosophic SuperHyperForcing. The details are followed by the upcoming statements. There’s neither empty SuperHyperEdge nor loop SuperHyperEdge. The SuperHyperSet of SuperHyperVertices, is the simple type-SuperHyperSet of the neutrosophic SuperHyperForcing. The SuperHyperSet of the SuperHyperVertices, is the minimum neutrosophic SuperHyperCardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. In There’s not only one SuperHyperVertex outside the intended SuperHyperSet. Thus the non-obvious neutrosophic SuperHyperForcing is up. The obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing is a SuperHyperSet excludes only one SuperHyperVertex in a connected neutrosophic SuperHyperGraph But the SuperHyperSet of SuperHyperVertices, has more than one SuperHyperVertex outside the intended SuperHyperSet. Thus the non-obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing is up. To sum them up, the SuperHyperSet of SuperHyperVertices, is the non-obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing. Since the SuperHyperSet of the SuperHyperVertices, is the SuperHyperSet Ss of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex and they are neutrosophic SuperHyperForcing. Since it’sthe minimum neutrosophic SuperHyperCardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. There’s only more than one SuperHyperVertex outside the intended SuperHyperSet, Thus the non-obvious neutrosophic SuperHyperForcing, is up. The non-obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing, is a SuperHyperSet, excludes only more than one SuperHyperVertex in a connected neutrosophic SuperHyperGraph
- On the Figure 14, the neutrosophic SuperHyperNotion, namely, neutrosophic SuperHyperForcing, is up. is the neutrosophic type-SuperHyperSet of the neutrosophic SuperHyperForcing. The details are followed by the upcoming statements. There’s neither empty SuperHyperEdge nor loop SuperHyperEdge. The SuperHyperSet of SuperHyperVertices, is the simple type-SuperHyperSet of the neutrosophic SuperHyperForcing. The SuperHyperSet of the SuperHyperVertices, is the minimum neutrosophic SuperHyperCardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. In There’s not only one SuperHyperVertex outside the intended SuperHyperSet. Thus the non-obvious neutrosophic SuperHyperForcing is up. The obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing is a SuperHyperSet excludes only one SuperHyperVertex in a connected neutrosophic SuperHyperGraph But the SuperHyperSet of SuperHyperVertices, has more than one SuperHyperVertex outside the intended SuperHyperSet. Thus the non-obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing is up. To sum them up, the SuperHyperSet of SuperHyperVertices, is the non-obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing. Since the SuperHyperSet of the SuperHyperVertices, is the SuperHyperSet Ss of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex and they are neutrosophic SuperHyperForcing. Since it’sthe minimum neutrosophic SuperHyperCardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. There’s only more than one SuperHyperVertex outside the intended SuperHyperSet, Thus the non-obvious neutrosophic SuperHyperForcing, is up. The non-obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing, is a SuperHyperSet, excludes only more than one SuperHyperVertex in a connected neutrosophic SuperHyperGraph
- On the Figure 15, the neutrosophic SuperHyperNotion, namely, neutrosophic SuperHyperForcing, is up. is the neutrosophic type-SuperHyperSet of the neutrosophic SuperHyperForcing. The details are followed by the upcoming statements. There’s neither empty SuperHyperEdge nor loop SuperHyperEdge. The SuperHyperSet of SuperHyperVertices, is the simple type-SuperHyperSet of the neutrosophic SuperHyperForcing. The SuperHyperSet of the SuperHyperVertices, is the minimum neutrosophic SuperHyperCardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. In There’s not only one SuperHyperVertex outside the intended SuperHyperSet. Thus the non-obvious neutrosophic SuperHyperForcing is up. The obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing is a SuperHyperSet excludes only one SuperHyperVertex in a connected neutrosophic SuperHyperGraph But the SuperHyperSet of SuperHyperVertices, has more than one SuperHyperVertex outside the intended SuperHyperSet. Thus the non-obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing is up. To sum them up, the SuperHyperSet of SuperHyperVertices, is the non-obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing. Since the SuperHyperSet of the SuperHyperVertices, is the SuperHyperSet Ss of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex and they are neutrosophic SuperHyperForcing. Since it’sthe minimum neutrosophic SuperHyperCardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. There’s only more than one SuperHyperVertex outside the intended SuperHyperSet, Thus the non-obvious neutrosophic SuperHyperForcing, is up. The non-obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing, is a SuperHyperSet, excludes only more than one SuperHyperVertex in a connected neutrosophic SuperHyperGraph
-
On the Figure 16, the neutrosophic SuperHyperNotion, namely, neutrosophic SuperHyperForcing, is up.is the neutrosophic type-SuperHyperSet of the neutrosophic SuperHyperForcing. The details are followed by the upcoming statements. There’s neither empty SuperHyperEdge nor loop SuperHyperEdge. The SuperHyperSet of SuperHyperVertices,is the simple type-SuperHyperSet of the neutrosophic SuperHyperForcing. The SuperHyperSet of the SuperHyperVertices,is the minimum neutrosophic SuperHyperCardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. In There’s not only one SuperHyperVertex outside the intended SuperHyperSet. Thus the non-obvious neutrosophic SuperHyperForcing is up. The obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing is a SuperHyperSet excludes only one SuperHyperVertex in a connected neutrosophic SuperHyperGraph But the SuperHyperSet of SuperHyperVertices,has more than one SuperHyperVertex outside the intended SuperHyperSet. Thus the non-obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing is up. To sum them up, the SuperHyperSet of SuperHyperVertices,is the non-obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing. Since the SuperHyperSet of the SuperHyperVertices,is the SuperHyperSet Ss of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex and they are neutrosophic SuperHyperForcing. Since it’sthe minimum neutrosophic SuperHyperCardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. There’s only more than one SuperHyperVertex outside the intended SuperHyperSet,Thus the non-obvious neutrosophic SuperHyperForcing,is up. The non-obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing,is a SuperHyperSet,excludes only more than one SuperHyperVertex in a connected neutrosophic SuperHyperGraph
-
On the Figure 17, the neutrosophic SuperHyperNotion, namely, neutrosophic SuperHyperForcing, is up.is the neutrosophic type-SuperHyperSet of the neutrosophic SuperHyperForcing. The details are followed by the upcoming statements. There’s neither empty SuperHyperEdge nor loop SuperHyperEdge. The SuperHyperSet of SuperHyperVertices,is the simple type-SuperHyperSet of the neutrosophic SuperHyperForcing. The SuperHyperSet of the SuperHyperVertices,is the minimum neutrosophic SuperHyperCardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. In There’s not only one SuperHyperVertex outside the intended SuperHyperSet. Thus the non-obvious neutrosophic SuperHyperForcing is up. The obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing is a SuperHyperSet excludes only one SuperHyperVertex in a connected neutrosophic SuperHyperGraph But the SuperHyperSet of SuperHyperVertices,has more than one SuperHyperVertex outside the intended SuperHyperSet. Thus the non-obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing is up. To sum them up, the SuperHyperSet of SuperHyperVertices,is the non-obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing. Since the SuperHyperSet of the SuperHyperVertices,is the SuperHyperSet Ss of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex and they are neutrosophic SuperHyperForcing. Since it’sthe minimum neutrosophic SuperHyperCardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. There’s only more than one SuperHyperVertex outside the intended SuperHyperSet,Thus the non-obvious neutrosophic SuperHyperForcing,is up. The non-obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing,is a SuperHyperSet,excludes only more than one SuperHyperVertex in a connected neutrosophic SuperHyperGraph
-
On the Figure 18, the neutrosophic SuperHyperNotion, namely, neutrosophic SuperHyperForcing, is up.is the neutrosophic type-SuperHyperSet of the neutrosophic SuperHyperForcing. The details are followed by the upcoming statements. There’s neither empty SuperHyperEdge nor loop SuperHyperEdge. The SuperHyperSet of SuperHyperVertices, is the simple type-SuperHyperSet of the neutrosophic SuperHyperForcing. The SuperHyperSet of the SuperHyperVertices, is the minimum neutrosophic SuperHyperCardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. In There’s not only one SuperHyperVertex outside the intended SuperHyperSet. Thus the non-obvious neutrosophic SuperHyperForcing is up. The obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing is a SuperHyperSet excludes only one SuperHyperVertex in a connected neutrosophic SuperHyperGraph But the SuperHyperSet of SuperHyperVertices, has more than one SuperHyperVertex outside the intended SuperHyperSet. Thus the non-obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing is up. To sum them up, the SuperHyperSet of SuperHyperVertices, is the non-obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing. Since the SuperHyperSet of the SuperHyperVertices, is the SuperHyperSet Ss of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex and they are neutrosophic SuperHyperForcing. Since it’sthe minimum neutrosophic SuperHyperCardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. There’s only more than one SuperHyperVertex outside the intended SuperHyperSet, Thus the non-obvious neutrosophic SuperHyperForcing, is up. The non-obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing, is a SuperHyperSet, excludes only more than one SuperHyperVertex in a connected neutrosophic SuperHyperGraph
-
On the Figure 19, the neutrosophic SuperHyperNotion, namely, neutrosophic SuperHyperForcing, is up.is the neutrosophic type-SuperHyperSet of the neutrosophic SuperHyperForcing. The details are followed by the upcoming statements.There’s neither empty SuperHyperEdge nor loop SuperHyperEdge. The SuperHyperSet of SuperHyperVertices,is the simple type-SuperHyperSet of the neutrosophic SuperHyperForcing. The SuperHyperSet of the SuperHyperVertices,is the minimum neutrosophic SuperHyperCardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. In There’s not only one SuperHyperVertex outside the intended SuperHyperSet. Thus the non-obvious neutrosophic SuperHyperForcing is up. The obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing is a SuperHyperSet excludes only one SuperHyperVertex in a connected neutrosophic SuperHyperGraph But the SuperHyperSet of SuperHyperVertices,has more than one SuperHyperVertex outside the intended SuperHyperSet. Thus the non-obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing is up. To sum them up, the SuperHyperSet of SuperHyperVertices,is the non-obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing. Since the SuperHyperSet of the SuperHyperVertices,is the SuperHyperSet Ss of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex and they are neutrosophic SuperHyperForcing. Since it’sthe minimum neutrosophic SuperHyperCardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. There’s only more than one SuperHyperVertex outside the intended SuperHyperSet,Thus the non-obvious neutrosophic SuperHyperForcing,is up. The non-obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing,is a SuperHyperSet,excludes only more than one SuperHyperVertex in a connected neutrosophic SuperHyperGraph
-
On the Figure 20, the neutrosophic SuperHyperNotion, namely, neutrosophic SuperHyperForcing, is up.is the neutrosophic type-SuperHyperSet of the neutrosophic SuperHyperForcing. The details are followed by the upcoming statements. There’s neither empty SuperHyperEdge nor loop SuperHyperEdge. The SuperHyperSet of SuperHyperVertices,is the simple type-SuperHyperSet of the neutrosophic SuperHyperForcing. The SuperHyperSet of the SuperHyperVertices,is the minimum neutrosophic SuperHyperCardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. In There’s not only one SuperHyperVertex outside the intended SuperHyperSet. Thus the non-obvious neutrosophic SuperHyperForcing is up. The obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing is a SuperHyperSet excludes only one SuperHyperVertex in a connected neutrosophic SuperHyperGraph But the SuperHyperSet of SuperHyperVertices,has more than one SuperHyperVertex outside the intended SuperHyperSet. Thus the non-obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing is up. To sum them up, the SuperHyperSet of SuperHyperVertices,is the non-obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing. Since the SuperHyperSet of the SuperHyperVertices,is the SuperHyperSet Ss of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex and they are neutrosophic SuperHyperForcing. Since it’sthe minimum neutrosophic SuperHyperCardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. There’s only more than one SuperHyperVertex outside the intended SuperHyperSet,Thus the non-obvious neutrosophic SuperHyperForcing,is up. The non-obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing,is a SuperHyperSet,excludes only more than one SuperHyperVertex in a connected neutrosophic SuperHyperGraph
Proposition 8.
Assume a connected neutrosophic SuperHyperGraph Then in the worst case, literally, is a neutrosophic SuperHyperForcing. In other words, the most neutrosophic SuperHyperCardinality, the upper sharp bound for neutrosophic SuperHyperCardinality, of neutrosophic SuperHyperForcing is the neutrosophic SuperHyperCardinality of
Proof.
Assume a connected neutrosophic SuperHyperGraph The SuperHyperSet of the SuperHyperVertices V is a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex but it isn’t a neutrosophic SuperHyperForcing. Since it doesn’t have the minimum neutrosophic SuperHyperCardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. There’s only one SuperHyperVertex outside the intended SuperHyperSet, Thus the obvious neutrosophic SuperHyperForcing, is up. The obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing, is a SuperHyperSet, excludes only one SuperHyperVertex in a connected neutrosophic SuperHyperGraph □
Proposition 9.
Assume a connected neutrosophic SuperHyperGraph Then the extreme number of neutrosophic SuperHyperForcing has, the most neutrosophic SuperHyperCardinality, the upper sharp bound for neutrosophic SuperHyperCardinality, is the extreme neutrosophic SuperHyperCardinality of V if there’s a neutrosophic SuperHyperForcing with the most neutrosophic SuperHyperCardinality, the upper sharp bound for neutrosophic SuperHyperCardinality.
Proof.
Assume a connected neutrosophic SuperHyperGraph Consider there’s a neutrosophic SuperHyperForcing with the most neutrosophic SuperHyperCardinality, the upper sharp bound for neutrosophic SuperHyperCardinality. The SuperHyperSet of the SuperHyperVertices V is a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex but it isn’t a neutrosophic SuperHyperForcing. Since it doesn’t have the minimum neutrosophic SuperHyperCardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. There’s only one SuperHyperVertex outside the intended SuperHyperSet, Thus the obvious neutrosophic SuperHyperForcing, is up. The obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing, is a SuperHyperSet, excludes only one SuperHyperVertex in a connected neutrosophic SuperHyperGraph It implies that extreme number of neutrosophic SuperHyperForcing has, the most neutrosophic SuperHyperCardinality, the upper sharp bound for neutrosophic SuperHyperCardinality, is choose Thus it induces that the extreme number of neutrosophic SuperHyperForcing has, the most neutrosophic SuperHyperCardinality, the upper sharp bound for neutrosophic SuperHyperCardinality, is the extreme neutrosophic SuperHyperCardinality of V if there’s a neutrosophic SuperHyperForcing with the most neutrosophic SuperHyperCardinality, the upper sharp bound for neutrosophic SuperHyperCardinality. □
Proposition 10.
Assume a connected neutrosophic SuperHyperGraph If a SuperHyperEdge has z SuperHyperVertices, then number of those SuperHyperVertices from that SuperHyperEdge belong to any neutrosophic SuperHyperForcing.
Proof.
Assume a connected neutrosophic SuperHyperGraph Let a SuperHyperEdge has z SuperHyperVertices. Consider number of those SuperHyperVertices from that SuperHyperEdge belong to any given SuperHyperSet of the SuperHyperVertices. The SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex but there are two white SuperHyperNeighbors outside implying there’s no SuperHyperVertex to the SuperHyperSet S does the “the color-change rule”. So it doesn’t have the minimum neutrosophic SuperHyperCardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. There’s only one SuperHyperVertex outside the intended SuperHyperSet. Thus the obvious neutrosophic SuperHyperForcing is up. The obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing is a SuperHyperSet excludes only one SuperHyperVertex in a connected neutrosophic SuperHyperGraph Thus all the following SuperHyperSets of SuperHyperVertices are the simple type-SuperHyperSet of the neutrosophic SuperHyperForcing. It’s the contradiction to the SuperHyperSet S is a neutrosophic SuperHyperForcing. Thus any given SuperHyperSet of the SuperHyperVertices contains the number of those SuperHyperVertices from that SuperHyperEdge with z SuperHyperVertices less than isn’t a neutrosophic SuperHyperForcing. Thus if a SuperHyperEdge has z SuperHyperVertices, then number of those SuperHyperVertices from that SuperHyperEdge belong to any neutrosophic SuperHyperForcing. □
Proposition 11.
Assume a connected neutrosophic SuperHyperGraph Every SuperHyperEdge has only one unique SuperHyperVertex outside of neutrosophic SuperHyperForcing. In other words, every SuperHyperEdge has only one unique white SuperHyperVertex.
Proof.
Assume a connected neutrosophic SuperHyperGraph Let a SuperHyperEdge has some SuperHyperVertices. Consider some numbers of those SuperHyperVertices from that SuperHyperEdge excluding two unique SuperHyperVertices, belong to any given SuperHyperSet of the SuperHyperVertices. The SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex but there are two white SuperHyperNeighbors outside implying there’s no SuperHyperVertex to the SuperHyperSet S does the “the color-change rule”. So it doesn’t have the minimum neutrosophic SuperHyperCardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. There’s only one SuperHyperVertex outside the intended SuperHyperSet. Thus the obvious neutrosophic SuperHyperForcing is up. The obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing is a SuperHyperSet excludes only one SuperHyperVertex in a connected neutrosophic SuperHyperGraph Thus all the following SuperHyperSets of SuperHyperVertices are the simple type-SuperHyperSet of the neutrosophic SuperHyperForcing. It’s the contradiction to the SuperHyperSet S is a neutrosophic SuperHyperForcing. Thus any given SuperHyperSet of the SuperHyperVertices contains the number of those SuperHyperVertices from that SuperHyperEdge with some SuperHyperVertices less than excluding one unique SuperHyperVertex, isn’t a neutrosophic SuperHyperForcing. Thus if a SuperHyperEdge has some SuperHyperVertices, then, with excluding one unique SuperHyperVertex, the all number of those SuperHyperVertices from that SuperHyperEdge belong to any neutrosophic SuperHyperForcing. Thus, in a connected neutrosophic SuperHyperGraph every SuperHyperEdge has only one unique SuperHyperVertex outside of neutrosophic SuperHyperForcing. In other words, every SuperHyperEdge has only one unique white SuperHyperVertex. □
Proposition 12.
Assume a connected neutrosophic SuperHyperGraph The all exterior SuperHyperVertices belong to any neutrosophic SuperHyperForcing if for any of them, there’s only one interior SuperHyperVertex is a SuperHyperNeighbor to any of them.
Proof.
Assume a connected neutrosophic SuperHyperGraph Let a SuperHyperEdge has some SuperHyperVertices. Consider some numbers of those SuperHyperVertices from that SuperHyperEdge excluding two unique SuperHyperVertices, belong to any given SuperHyperSet of the SuperHyperVertices. The SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex but there are two white SuperHyperNeighbors outside implying there’s no SuperHyperVertex to the SuperHyperSet S does the “the color-change rule”. So it doesn’t have the minimum neutrosophic SuperHyperCardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. Consider some numbers of those SuperHyperVertices from that SuperHyperEdge, without any exclusion on some SuperHyperVertices, belong to any given SuperHyperSet of the SuperHyperVertices. The SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex but it implies it doesn’t have the minimum neutrosophic SuperHyperCardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. There’s only one SuperHyperVertex outside the intended SuperHyperSet. Thus the obvious neutrosophic SuperHyperForcing is up. The obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing is a SuperHyperSet excludes only one SuperHyperVertex in a connected neutrosophic SuperHyperGraph Thus all the following SuperHyperSets of SuperHyperVertices are the simple type-SuperHyperSet of the neutrosophic SuperHyperForcing. It’s the contradiction to the SuperHyperSet S is a neutrosophic SuperHyperForcing. Thus any given SuperHyperSet of the SuperHyperVertices contains the number of those SuperHyperVertices from that SuperHyperEdge with some SuperHyperVertices less than excluding one unique SuperHyperVertex, isn’t a neutrosophic SuperHyperForcing. Thus if a SuperHyperEdge has some SuperHyperVertices, then, with excluding one unique SuperHyperVertex, the all number of those SuperHyperVertices from that SuperHyperEdge belong to any neutrosophic SuperHyperForcing. Thus, in a connected neutrosophic SuperHyperGraph every SuperHyperEdge has only one unique SuperHyperVertex outside of neutrosophic SuperHyperForcing. In other words, every SuperHyperEdge has only one unique white SuperHyperVertex. In a connected neutrosophic SuperHyperGraph the all exterior SuperHyperVertices belong to any neutrosophic SuperHyperForcing if for any of them, there’s only one interior SuperHyperVertex is a SuperHyperNeighbor to any of them. □
Proposition 13.
Assume a connected neutrosophic SuperHyperGraph The any neutrosophic SuperHyperForcing only contains all interior SuperHyperVertices and all exterior SuperHyperVertices where any of them has one SuperHyperNeighbor out.
Proof.
Assume a connected neutrosophic SuperHyperGraph Let a SuperHyperEdge has some SuperHyperVertices. Consider some numbers of those SuperHyperVertices from that SuperHyperEdge excluding two unique SuperHyperVertices, belong to any given SuperHyperSet of the SuperHyperVertices. The SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex but there are two white SuperHyperNeighbors outside implying there’s no SuperHyperVertex to the SuperHyperSet S does the “the color-change rule”. So it doesn’t have the minimum neutrosophic SuperHyperCardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. Consider some numbers of those SuperHyperVertices from that SuperHyperEdge, without any exclusion on some SuperHyperVertices, belong to any given SuperHyperSet of the SuperHyperVertices. The SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex but it implies it doesn’t have the minimum neutrosophic SuperHyperCardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. There’s only one SuperHyperVertex outside the intended SuperHyperSet. Thus the obvious neutrosophic SuperHyperForcing is up. The obvious simple type-SuperHyperSet of the neutrosophic SuperHyperForcing is a SuperHyperSet excludes only one SuperHyperVertex in a connected neutrosophic SuperHyperGraph Thus all the following SuperHyperSets of SuperHyperVertices are the simple type-SuperHyperSet of the neutrosophic SuperHyperForcing. It’s the contradiction to the SuperHyperSet S is a neutrosophic SuperHyperForcing. Thus any given SuperHyperSet of the SuperHyperVertices contains the number of those SuperHyperVertices from that SuperHyperEdge with some SuperHyperVertices less than excluding one unique SuperHyperVertex, isn’t a neutrosophic SuperHyperForcing. Thus if a SuperHyperEdge has some SuperHyperVertices, then, with excluding one unique SuperHyperVertex, the all number of those SuperHyperVertices from that SuperHyperEdge belong to any neutrosophic SuperHyperForcing. Thus, in a connected neutrosophic SuperHyperGraph every SuperHyperEdge has only one unique SuperHyperVertex outside of neutrosophic SuperHyperForcing. In other words, every SuperHyperEdge has only one unique white SuperHyperVertex. In a connected neutrosophic SuperHyperGraph the any neutrosophic SuperHyperForcing only contains all interior SuperHyperVertices and all exterior SuperHyperVertices where any of them has one SuperHyperNeighbor out. □
Remark 2.
The words “neutrosophic SuperHyperForcing” and “SuperHyperDominating” refer to the minimum type-style. In other words, they refer to both the minimum number and the SuperHyperSet with the minimum neutrosophic SuperHyperCardinality.
Proposition 14.
Assume a connected neutrosophic SuperHyperGraph A complement of neutrosophic SuperHyperForcing is the SuperHyperDominating.
Proof.
Assume a connected neutrosophic SuperHyperGraph By applying the Proposition (13), the results are up. Thus in a connected neutrosophic SuperHyperGraph a complement of neutrosophic SuperHyperForcing is the SuperHyperDominating. □
4. Results on SuperHyperClasses
Proposition 15.
Assume a connected SuperHyperPath Then a SuperHyperForcing-style with the maximum SuperHyperCardinality is a SuperHyperSet of the exterior SuperHyperVertices.
Proposition 16.
Assume a connected SuperHyperPath Then a SuperHyperForcing is a SuperHyperSet of the exterior SuperHyperVertices and the interior SuperHyperVertices with only one exception in the form of interior SuperHyperVertices from any given SuperHyperEdge. A SuperHyperForcing has the number of all the SuperHyperVertices minus on the number of exterior SuperHyperParts plus one.
Proof.
Assume a connected SuperHyperPath Let a SuperHyperEdge has some SuperHyperVertices. Consider some numbers of those SuperHyperVertices from that SuperHyperEdge excluding two unique SuperHyperVertices, belong to any given SuperHyperSet of the SuperHyperVertices. The SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex but there are two white SuperHyperNeighbors outside implying there’s no SuperHyperVertex to the SuperHyperSet S does the “the color-change rule”. So it doesn’t have the minimum SuperHyperCardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. Consider some numbers of those SuperHyperVertices from that SuperHyperEdge, without any exclusion on some SuperHyperVertices, belong to any given SuperHyperSet of the SuperHyperVertices. The SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex but it implies it doesn’t have the minimum SuperHyperCardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. There’s only one SuperHyperVertex outside the intended SuperHyperSet. Thus the obvious SuperHyperForcing is up. The obvious simple type-SuperHyperSet of the SuperHyperForcing is a SuperHyperSet excludes only one SuperHyperVertex in a connected SuperHyperPath Thus all the following SuperHyperSets of SuperHyperVertices are the simple type-SuperHyperSet of the SuperHyperForcing. It’s the contradiction to the SuperHyperSet S is a SuperHyperForcing. Thus any given SuperHyperSet of the SuperHyperVertices contains the number of those SuperHyperVertices from that SuperHyperEdge with some SuperHyperVertices less than excluding one unique SuperHyperVertex, isn’t a SuperHyperForcing. Thus if a SuperHyperEdge has some SuperHyperVertices, then, with excluding one unique SuperHyperVertex, the all number of those SuperHyperVertices from that SuperHyperEdge belong to any SuperHyperForcing. Thus, in a connected SuperHyperPath every SuperHyperEdge has only one unique SuperHyperVertex outside of SuperHyperForcing. In other words, every SuperHyperEdge has only one unique white SuperHyperVertex. In a connected SuperHyperPath the any SuperHyperForcing only contains all interior SuperHyperVertices and all exterior SuperHyperVertices where any of them has one SuperHyperNeighbor out. Then a SuperHyperForcing is a SuperHyperSet of the exterior SuperHyperVertices and the interior SuperHyperVertices with only one exception in the form of interior SuperHyperVertices from any given SuperHyperEdge. A SuperHyperForcing has the number of all the SuperHyperVertices minus on the number of exterior SuperHyperParts plus one. □
Example 3.
In the Figure 21, the connected SuperHyperPath is highlighted and featured. The SuperHyperSet, of the SuperHyperVertices of the connected SuperHyperPath in the SuperHyperModel (21), is the SuperHyperForcing.
Proposition 17.
Assume a connected SuperHyperCycle Then a SuperHyperForcing is a SuperHyperSet of the exterior SuperHyperVertices and the interior SuperHyperVertices with only one exception in the form of interior SuperHyperVertices from any given SuperHyperEdge. A SuperHyperForcing has the number of all the SuperHyperVertices minus on the number of exterior SuperHyperParts.
Proof.
Assume a connected SuperHyperCycle Let a SuperHyperEdge has some SuperHyperVertices. Consider some numbers of those SuperHyperVertices from that SuperHyperEdge excluding two unique SuperHyperVertices, belong to any given SuperHyperSet of the SuperHyperVertices. The SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex but there are two white SuperHyperNeighbors outside implying there’s no SuperHyperVertex to the SuperHyperSet S does the “the color-change rule”. So it doesn’t have the minimum SuperHyperCardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. Consider some numbers of those SuperHyperVertices from that SuperHyperEdge, without any exclusion on some SuperHyperVertices, belong to any given SuperHyperSet of the SuperHyperVertices. The SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex but it implies it doesn’t have the minimum SuperHyperCardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. There’s only one SuperHyperVertex outside the intended SuperHyperSet. Thus the obvious SuperHyperForcing is up. The obvious simple type-SuperHyperSet of the SuperHyperForcing is a SuperHyperSet excludes only one SuperHyperVertex in a connected SuperHyperCycle Thus all the following SuperHyperSets of SuperHyperVertices are the simple type-SuperHyperSet of the SuperHyperForcing. It’s the contradiction to the SuperHyperSet S is a SuperHyperForcing. Thus any given SuperHyperSet of the SuperHyperVertices contains the number of those SuperHyperVertices from that SuperHyperEdge with some SuperHyperVertices less than excluding one unique SuperHyperVertex, isn’t a SuperHyperForcing. Thus if a SuperHyperEdge has some SuperHyperVertices, then, with excluding one unique SuperHyperVertex, the all number of those SuperHyperVertices from that SuperHyperEdge belong to any SuperHyperForcing. Thus, in a connected SuperHyperCycle every SuperHyperEdge has only one unique SuperHyperVertex outside of SuperHyperForcing. In other words, every SuperHyperEdge has only one unique white SuperHyperVertex. In a connected SuperHyperCycle the any SuperHyperForcing only contains all interior SuperHyperVertices and all exterior SuperHyperVertices where any of them has one SuperHyperNeighbor out. Then a SuperHyperForcing is a SuperHyperSet of the exterior SuperHyperVertices and the interior SuperHyperVertices with only one exception in the form of interior SuperHyperVertices from any given SuperHyperEdge. A SuperHyperForcing has the number of all the SuperHyperVertices minus on the number of exterior SuperHyperParts. □
Example 4.
In the Figure 22, the connected SuperHyperCycle is highlighted and featured. The obtained SuperHyperSet, by the Algorithm in previous result, of the SuperHyperVertices of the connected SuperHyperCycle in the SuperHyperModel (22), is the SuperHyperForcing.
Proposition 18.
Assume a connected SuperHyperStar Then a SuperHyperForcing is a SuperHyperSet of the exterior SuperHyperVertices and the interior SuperHyperVertices with only one exception in the form of interior SuperHyperVertices from any given SuperHyperEdge. A SuperHyperForcing has the number of all the SuperHyperVertices minus on the cardinality of the second SuperHyperPart plus one.
Proof.
Assume a connected SuperHyperStar Let a SuperHyperEdge has some SuperHyperVertices. Consider some numbers of those SuperHyperVertices from that SuperHyperEdge excluding two unique SuperHyperVertices, belong to any given SuperHyperSet of the SuperHyperVertices. The SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex but there are two white SuperHyperNeighbors outside implying there’s no SuperHyperVertex to the SuperHyperSet S does the “the color-change rule”. So it doesn’t have the minimum SuperHyperCardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. Consider some numbers of those SuperHyperVertices from that SuperHyperEdge, without any exclusion on some SuperHyperVertices, belong to any given SuperHyperSet of the SuperHyperVertices. The SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex but it implies it doesn’t have the minimum SuperHyperCardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. There’s only one SuperHyperVertex outside the intended SuperHyperSet. Thus the obvious SuperHyperForcing is up. The obvious simple type-SuperHyperSet of the SuperHyperForcing is a SuperHyperSet excludes only one SuperHyperVertex in a connected SuperHyperStar Thus all the following SuperHyperSets of SuperHyperVertices are the simple type-SuperHyperSet of the SuperHyperForcing. It’s the contradiction to the SuperHyperSet S is a SuperHyperForcing. Thus any given SuperHyperSet of the SuperHyperVertices contains the number of those SuperHyperVertices from that SuperHyperEdge with some SuperHyperVertices less than excluding one unique SuperHyperVertex, isn’t a SuperHyperForcing. Thus if a SuperHyperEdge has some SuperHyperVertices, then, with excluding one unique SuperHyperVertex, the all number of those SuperHyperVertices from that SuperHyperEdge belong to any SuperHyperForcing. Thus, in a connected SuperHyperStar every SuperHyperEdge has only one unique SuperHyperVertex outside of SuperHyperForcing. In other words, every SuperHyperEdge has only one unique white SuperHyperVertex. In a connected SuperHyperStar the any SuperHyperForcing only contains all interior SuperHyperVertices and all exterior SuperHyperVertices where any of them has one SuperHyperNeighbor out. Then a SuperHyperForcing is a SuperHyperSet of the exterior SuperHyperVertices and the interior SuperHyperVertices with only one exception in the form of interior SuperHyperVertices from any given SuperHyperEdge. A SuperHyperForcing has the number of all the SuperHyperVertices minus on the cardinality of the second SuperHyperPart plus one. □
Example 5.
In the Figure 23, the connected SuperHyperStar is highlighted and featured. The obtained SuperHyperSet, by the Algorithm in previous result, of the SuperHyperVertices of the connected SuperHyperStar in the SuperHyperModel (23), is the SuperHyperForcing.
Proposition 19.
Assume a connected SuperHyperBipartite Then a SuperHyperForcing is a SuperHyperSet of the exterior SuperHyperVertices and the interior SuperHyperVertices with only one exception in the form of interior SuperHyperVertices from any given SuperHyperEdge. A SuperHyperForcing has the number of all the SuperHyperVertices minus on the cardinality of the first SuperHyperPart plus the second SuperHyperPart.
Proof.
Assume a connected SuperHyperBipartite Let a SuperHyperEdge has some SuperHyperVertices. Consider some numbers of those SuperHyperVertices from that SuperHyperEdge excluding two unique SuperHyperVertices, belong to any given SuperHyperSet of the SuperHyperVertices. The SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex but there are two white SuperHyperNeighbors outside implying there’s no SuperHyperVertex to the SuperHyperSet S does the “the color-change rule”. So it doesn’t have the minimum SuperHyperCardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. Consider some numbers of those SuperHyperVertices from that SuperHyperEdge, without any exclusion on some SuperHyperVertices, belong to any given SuperHyperSet of the SuperHyperVertices. The SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex but it implies it doesn’t have the minimum SuperHyperCardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. There’s only one SuperHyperVertex outside the intended SuperHyperSet. Thus the obvious SuperHyperForcing is up. The obvious simple type-SuperHyperSet of the SuperHyperForcing is a SuperHyperSet excludes only one SuperHyperVertex in a connected SuperHyperBipartite Thus all the following SuperHyperSets of SuperHyperVertices are the simple type-SuperHyperSet of the SuperHyperForcing. It’s the contradiction to the SuperHyperSet S is a SuperHyperForcing. Thus any given SuperHyperSet of the SuperHyperVertices contains the number of those SuperHyperVertices from that SuperHyperEdge with some SuperHyperVertices less than excluding one unique SuperHyperVertex, isn’t a SuperHyperForcing. Thus if a SuperHyperEdge has some SuperHyperVertices, then, with excluding one unique SuperHyperVertex, the all number of those SuperHyperVertices from that SuperHyperEdge belong to any SuperHyperForcing. Thus, in a connected SuperHyperBipartite every SuperHyperEdge has only one unique SuperHyperVertex outside of SuperHyperForcing. In other words, every SuperHyperEdge has only one unique white SuperHyperVertex. In a connected SuperHyperBipartite the any SuperHyperForcing only contains all interior SuperHyperVertices and all exterior SuperHyperVertices where any of them has one SuperHyperNeighbor out. Then a SuperHyperForcing is a SuperHyperSet of the exterior SuperHyperVertices and the interior SuperHyperVertices with only one exception in the form of interior SuperHyperVertices from any given SuperHyperEdge. A SuperHyperForcing has the number of all the SuperHyperVertices minus on the cardinality of the first SuperHyperPart plus the second SuperHyperPart. □
Example 6.
In the Figure 24, the connected SuperHyperBipartite is highlighted and featured. The obtained SuperHyperSet, by the Algorithm in previous result, of the SuperHyperVertices of the connected SuperHyperBipartite in the SuperHyperModel (24), is the SuperHyperForcing.
Proposition 20.
Assume a connected SuperHyperMultipartite Then a SuperHyperForcing is a SuperHyperSet of the exterior SuperHyperVertices and the interior SuperHyperVertices with only one exception in the form of interior SuperHyperVertices from any given SuperHyperEdge. A SuperHyperForcing has the number of all the SuperHyperVertices minus the number of all the the summation on the cardinality of the SuperHyperParts.
Proof.
Assume a connected SuperHyperMultipartite Let a SuperHyperEdge has some SuperHyperVertices. Consider some numbers of those SuperHyperVertices from that SuperHyperEdge excluding two unique SuperHyperVertices, belong to any given SuperHyperSet of the SuperHyperVertices. The SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex but there are two white SuperHyperNeighbors outside implying there’s no SuperHyperVertex to the SuperHyperSet S does the “the color-change rule”. So it doesn’t have the minimum SuperHyperCardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. Consider some numbers of those SuperHyperVertices from that SuperHyperEdge, without any exclusion on some SuperHyperVertices, belong to any given SuperHyperSet of the SuperHyperVertices. The SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex but it implies it doesn’t have the minimum SuperHyperCardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. There’s only one SuperHyperVertex outside the intended SuperHyperSet. Thus the obvious SuperHyperForcing is up. The obvious simple type-SuperHyperSet of the SuperHyperForcing is a SuperHyperSet excludes only one SuperHyperVertex in a connected SuperHyperMultipartite Thus all the following SuperHyperSets of SuperHyperVertices are the simple type-SuperHyperSet of the SuperHyperForcing. It’s the contradiction to the SuperHyperSet S is a SuperHyperForcing. Thus any given SuperHyperSet of the SuperHyperVertices contains the number of those SuperHyperVertices from that SuperHyperEdge with some SuperHyperVertices less than excluding one unique SuperHyperVertex, isn’t a SuperHyperForcing. Thus if a SuperHyperEdge has some SuperHyperVertices, then, with excluding one unique SuperHyperVertex, the all number of those SuperHyperVertices from that SuperHyperEdge belong to any SuperHyperForcing. Thus, in a connected SuperHyperMultipartite every SuperHyperEdge has only one unique SuperHyperVertex outside of SuperHyperForcing. In other words, every SuperHyperEdge has only one unique white SuperHyperVertex. In a connected SuperHyperMultipartite the any SuperHyperForcing only contains all interior SuperHyperVertices and all exterior SuperHyperVertices where any of them has one SuperHyperNeighbor out. Then a SuperHyperForcing is a SuperHyperSet of the exterior SuperHyperVertices and the interior SuperHyperVertices with only one exception in the form of interior SuperHyperVertices from any given SuperHyperEdge. A SuperHyperForcing has the number of all the SuperHyperVertices minus the number of all the the summation on the cardinality of the SuperHyperParts. □
Example 7.
In the Figure 25, the connected SuperHyperMultipartite is highlighted and featured. The obtained SuperHyperSet, by the Algorithm in previous result, of the SuperHyperVertices of the connected SuperHyperMultipartite in the SuperHyperModel (25), is the SuperHyperForcing.
Proposition 21.
Assume a connected SuperHyperWheel Then a SuperHyperForcing is a SuperHyperSet of the exterior SuperHyperVertices and the interior SuperHyperVertices with only one exception in the form of interior SuperHyperVertices from any given SuperHyperEdge. A SuperHyperForcing has the number of all the SuperHyperVertices minus the number of all the SuperHyperEdges.
Proof.
Assume a connected SuperHyperWheel Let a SuperHyperEdge has some SuperHyperVertices. Consider some numbers of those SuperHyperVertices from that SuperHyperEdge excluding two unique SuperHyperVertices, belong to any given SuperHyperSet of the SuperHyperVertices. The SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex but there are two white SuperHyperNeighbors outside implying there’s no SuperHyperVertex to the SuperHyperSet S does the “the color-change rule”. So it doesn’t have the minimum SuperHyperCardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. Consider some numbers of those SuperHyperVertices from that SuperHyperEdge, without any exclusion on some SuperHyperVertices, belong to any given SuperHyperSet of the SuperHyperVertices. The SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex but it implies it doesn’t have the minimum SuperHyperCardinality of a SuperHyperSet S of black SuperHyperVertices (whereas SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white SuperHyperVertex is converted to a black SuperHyperVertex if it is the only white SuperHyperNeighbor of a black SuperHyperVertex. There’s only one SuperHyperVertex outside the intended SuperHyperSet. Thus the obvious SuperHyperForcing is up. The obvious simple type-SuperHyperSet of the SuperHyperForcing is a SuperHyperSet excludes only one SuperHyperVertex in a connected SuperHyperWheel Thus all the following SuperHyperSets of SuperHyperVertices are the simple type-SuperHyperSet of the SuperHyperForcing. It’s the contradiction to the SuperHyperSet S is a SuperHyperForcing. Thus any given SuperHyperSet of the SuperHyperVertices contains the number of those SuperHyperVertices from that SuperHyperEdge with some SuperHyperVertices less than excluding one unique SuperHyperVertex, isn’t a SuperHyperForcing. Thus if a SuperHyperEdge has some SuperHyperVertices, then, with excluding one unique SuperHyperVertex, the all number of those SuperHyperVertices from that SuperHyperEdge belong to any SuperHyperForcing. Thus, in a connected SuperHyperWheel every SuperHyperEdge has only one unique SuperHyperVertex outside of SuperHyperForcing. In other words, every SuperHyperEdge has only one unique white SuperHyperVertex. In a connected SuperHyperWheel the any SuperHyperForcing only contains all interior SuperHyperVertices and all exterior SuperHyperVertices where any of them has one SuperHyperNeighbor out. Then a SuperHyperForcing is a SuperHyperSet of the exterior SuperHyperVertices and the interior SuperHyperVertices with only one exception in the form of interior SuperHyperVertices from any given SuperHyperEdge. A SuperHyperForcing has the number of all the SuperHyperVertices minus the number of all the SuperHyperEdges. □
Example 8.
In the Figure 26, the connected SuperHyperWheel is highlighted and featured. The obtained SuperHyperSet, by the Algorithm in previous result, of the SuperHyperVertices of the connected SuperHyperWheel in the SuperHyperModel (26), is the SuperHyperForcing.
5. Results on Neutrosophic SuperHyperClasses
Proposition 22.
Assume a connected neutrosophic SuperHyperPath Then a neutrosophic SuperHyperForcing is a neutrosophic SuperHyperSet of the exterior SuperHyperVertices and the interior SuperHyperVertices with only one exception in the form of interior SuperHyperVertices from any given SuperHyperEdge with the minimum cardinality. A SuperHyperForcing has the minimum neutrosophic cardinality on all SuperHyperForcing has the number of all the SuperHyperVertices minus on the number of exterior SuperHyperParts plus one. Thus,
Where is the unary operation on the SuperHyperVertices of the SuperHyperGraph to assign the determinacy, the indeterminacy and the neutrality, for respectively.
Proof.
Assume a connected neutrosophic SuperHyperPath Let a neutrosophic SuperHyperEdge has some neutrosophic SuperHyperVertices. Consider some extreme numbers of those neutrosophic SuperHyperVertices from that neutrosophic SuperHyperEdge excluding two unique neutrosophic SuperHyperVertices, belong to any given neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices. The neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex but there are two white neutrosophic SuperHyperNeighbors outside implying there’s no neutrosophic SuperHyperVertex to the neutrosophic SuperHyperSet S does the “the color-change rule”. So it doesn’t have the minimum neutrosophic SuperHyperCardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex. Consider some extreme numbers of those neutrosophic SuperHyperVertices from that neutrosophic SuperHyperEdge, without any exclusion on some neutrosophic SuperHyperVertices, belong to any given neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices. The neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex but it implies it doesn’t have the minimum neutrosophic SuperHyperCardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex. There’s only one neutrosophic SuperHyperVertex outside the intended neutrosophic SuperHyperSet. Thus the obvious neutrosophic SuperHyperForcing is up. The obvious simple type-neutrosophic SuperHyperSet of the neutrosophic SuperHyperForcing is a neutrosophic SuperHyperSet excludes only one neutrosophic SuperHyperVertex in a connected neutrosophic SuperHyperPath Thus all the following neutrosophic SuperHyperSets of neutrosophic SuperHyperVertices are the simple neutrosophic type-SuperHyperSet of the neutrosophic SuperHyperForcing. It’s the contradiction to the neutrosophic SuperHyperSet S is a neutrosophic SuperHyperForcing. Thus any given neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices contains the number of those neutrosophic SuperHyperVertices from that neutrosophic SuperHyperEdge with some neutrosophic SuperHyperVertices less than excluding one unique neutrosophic SuperHyperVertex, isn’t a neutrosophic SuperHyperForcing. Thus if a neutrosophic SuperHyperEdge has some neutrosophic SuperHyperVertices, then, with excluding one unique neutrosophic SuperHyperVertex, the all number of those neutrosophic SuperHyperVertices from that neutrosophic SuperHyperEdge belong to any neutrosophic SuperHyperForcing. Thus, in a connected neutrosophic SuperHyperPath every neutrosophic SuperHyperEdge has only one unique neutrosophic SuperHyperVertex outside of neutrosophic SuperHyperForcing. In other words, every neutrosophic SuperHyperEdge has only one unique white neutrosophic SuperHyperVertex. In a connected neutrosophic SuperHyperPath the any neutrosophic SuperHyperForcing only contains all interior neutrosophic SuperHyperVertices and all exterior neutrosophic SuperHyperVertices where any of them has one neutrosophic SuperHyperNeighbor out. Then a neutrosophic SuperHyperForcing is a neutrosophic SuperHyperSet of the exterior neutrosophic SuperHyperVertices and the interior neutrosophic SuperHyperVertices with only one exception in the form of interior neutrosophic SuperHyperVertices from any given neutrosophic SuperHyperEdge with the minimum neutrosophic cardinality. A neutrosophic SuperHyperForcing has the minimum neutrosophic cardinality on all neutrosophic SuperHyperForcing has the number of all the neutrosophic SuperHyperVertices minus on the number of exterior neutrosophic SuperHyperParts plus one. Thus,
Where is the unary operation on the SuperHyperVertices of the SuperHyperGraph to assign the determinacy, the indeterminacy and the neutrality, for respectively. □
Example 9.
In the Figure 27, the connected neutrosophic SuperHyperPath is highlighted and featured.
The neutrosophic SuperHyperSet, of the neutrosophic SuperHyperVertices of the connected neutrosophic SuperHyperPath in the neutrosophic SuperHyperModel (27), is the neutrosophic SuperHyperForcing.
Proposition 23.
Assume a connected neutrosophic SuperHyperCycle Then a neutrosophic SuperHyperForcing is a neutrosophic SuperHyperSet of the exterior neutrosophic SuperHyperVertices and the interior neutrosophic SuperHyperVertices with only one exception in the form of interior neutrosophic SuperHyperVertices from any given neutrosophic SuperHyperEdge with the minimum neutrosophic cardinality. A neutrosophic SuperHyperForcing has the minimum neutrosophic cardinality on all neutrosophic SuperHyperForcing has the number of all the neutrosophic SuperHyperVertices minus on the number of exterior neutrosophic SuperHyperParts plus one. Thus,
Where is the unary operation on the SuperHyperVertices of the SuperHyperGraph to assign the determinacy, the indeterminacy and the neutrality, for respectively.
Proof.
Assume a connected neutrosophic SuperHyperCycle Let a neutrosophic SuperHyperEdge has some neutrosophic SuperHyperVertices. Consider some extreme numbers of those neutrosophic SuperHyperVertices from that neutrosophic SuperHyperEdge excluding two unique neutrosophic SuperHyperVertices, belong to any given neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices. The neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex but there are two white neutrosophic SuperHyperNeighbors outside implying there’s no neutrosophic SuperHyperVertex to the neutrosophic SuperHyperSet S does the “the color-change rule”. So it doesn’t have the minimum neutrosophic SuperHyperCardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex. Consider some extreme numbers of those neutrosophic SuperHyperVertices from that neutrosophic SuperHyperEdge, without any exclusion on some neutrosophic SuperHyperVertices, belong to any given neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices. The neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex but it implies it doesn’t have the minimum neutrosophic SuperHyperCardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex. There’s only one neutrosophic SuperHyperVertex outside the intended neutrosophic SuperHyperSet. Thus the obvious neutrosophic SuperHyperForcing is up. The obvious simple type-neutrosophic SuperHyperSet of the neutrosophic SuperHyperForcing is a neutrosophic SuperHyperSet excludes only one neutrosophic SuperHyperVertex in a connected neutrosophic SuperHyperCycle Thus all the following neutrosophic SuperHyperSets of neutrosophic SuperHyperVertices are the simple neutrosophic type-SuperHyperSet of the neutrosophic SuperHyperForcing. It’s the contradiction to the neutrosophic SuperHyperSet S is a neutrosophic SuperHyperForcing. Thus any given neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices contains the number of those neutrosophic SuperHyperVertices from that neutrosophic SuperHyperEdge with some neutrosophic SuperHyperVertices less than excluding one unique neutrosophic SuperHyperVertex, isn’t a neutrosophic SuperHyperForcing. Thus if a neutrosophic SuperHyperEdge has some neutrosophic SuperHyperVertices, then, with excluding one unique neutrosophic SuperHyperVertex, the all number of those neutrosophic SuperHyperVertices from that neutrosophic SuperHyperEdge belong to any neutrosophic SuperHyperForcing. Thus, in a connected neutrosophic SuperHyperCycle every neutrosophic SuperHyperEdge has only one unique neutrosophic SuperHyperVertex outside of neutrosophic SuperHyperForcing. In other words, every neutrosophic SuperHyperEdge has only one unique white neutrosophic SuperHyperVertex. In a connected neutrosophic SuperHyperCycle the any neutrosophic SuperHyperForcing only contains all interior neutrosophic SuperHyperVertices and all exterior neutrosophic SuperHyperVertices where any of them has one neutrosophic SuperHyperNeighbor out. Then a neutrosophic SuperHyperForcing is a neutrosophic SuperHyperSet of the exterior neutrosophic SuperHyperVertices and the interior neutrosophic SuperHyperVertices with only one exception in the form of interior neutrosophic SuperHyperVertices from any given neutrosophic SuperHyperEdge with the minimum neutrosophic cardinality. A neutrosophic SuperHyperForcing has the minimum neutrosophic cardinality on all neutrosophic SuperHyperForcing has the number of all the neutrosophic SuperHyperVertices minus on the number of exterior neutrosophic SuperHyperParts plus one. Thus,
Where is the unary operation on the SuperHyperVertices of the SuperHyperGraph to assign the determinacy, the indeterminacy and the neutrality, for respectively. □
Example 10.
In the Figure 28, the SuperHyperCycle is highlighted and featured.
The obtained neutrosophic SuperHyperSet, by the Algorithm in previous result, of the neutrosophic SuperHyperVertices of the connected neutrosophic SuperHyperCycle in the neutrosophic SuperHyperModel (22), is the neutrosophic SuperHyperForcing.
Proposition 24.
Assume a connected neutrosophic SuperHyperStar Then a neutrosophic SuperHyperForcing is a neutrosophic SuperHyperSet of the exterior neutrosophic SuperHyperVertices and the interior neutrosophic SuperHyperVertices with only one exception in the form of interior neutrosophic SuperHyperVertices from any given neutrosophic SuperHyperEdge with the minimum neutrosophic cardinality. A neutrosophic SuperHyperForcing has the minimum neutrosophic cardinality on all neutrosophic SuperHyperForcing has the number of all the neutrosophic SuperHyperVertices minus on the neutrosophic cardinality of the second neutrosophic SuperHyperPart plus one. Thus,
Where is the unary operation on the SuperHyperVertices of the SuperHyperGraph to assign the determinacy, the indeterminacy and the neutrality, for respectively.
Proof.
Assume a connected neutrosophic SuperHyperStar Let a neutrosophic SuperHyperEdge has some neutrosophic SuperHyperVertices. Consider some extreme numbers of those neutrosophic SuperHyperVertices from that neutrosophic SuperHyperEdge excluding two unique neutrosophic SuperHyperVertices, belong to any given neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices. The neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex but there are two white neutrosophic SuperHyperNeighbors outside implying there’s no neutrosophic SuperHyperVertex to the neutrosophic SuperHyperSet S does the “the color-change rule”. So it doesn’t have the minimum neutrosophic SuperHyperCardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex. Consider some extreme numbers of those neutrosophic SuperHyperVertices from that neutrosophic SuperHyperEdge, without any exclusion on some neutrosophic SuperHyperVertices, belong to any given neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices. The neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex but it implies it doesn’t have the minimum neutrosophic SuperHyperCardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex. There’s only one neutrosophic SuperHyperVertex outside the intended neutrosophic SuperHyperSet. Thus the obvious neutrosophic SuperHyperForcing is up. The obvious simple type-neutrosophic SuperHyperSet of the neutrosophic SuperHyperForcing is a neutrosophic SuperHyperSet excludes only one neutrosophic SuperHyperVertex in a connected neutrosophic SuperHyperStar Thus all the following neutrosophic SuperHyperSets of neutrosophic SuperHyperVertices are the simple neutrosophic type-SuperHyperSet of the neutrosophic SuperHyperForcing. It’s the contradiction to the neutrosophic SuperHyperSet S is a neutrosophic SuperHyperForcing. Thus any given neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices contains the number of those neutrosophic SuperHyperVertices from that neutrosophic SuperHyperEdge with some neutrosophic SuperHyperVertices less than excluding one unique neutrosophic SuperHyperVertex, isn’t a neutrosophic SuperHyperForcing. Thus if a neutrosophic SuperHyperEdge has some neutrosophic SuperHyperVertices, then, with excluding one unique neutrosophic SuperHyperVertex, the all number of those neutrosophic SuperHyperVertices from that neutrosophic SuperHyperEdge belong to any neutrosophic SuperHyperForcing. Thus, in a connected neutrosophic SuperHyperStar every neutrosophic SuperHyperEdge has only one unique neutrosophic SuperHyperVertex outside of neutrosophic SuperHyperForcing. In other words, every neutrosophic SuperHyperEdge has only one unique white neutrosophic SuperHyperVertex. In a connected neutrosophic SuperHyperStar the any neutrosophic SuperHyperForcing only contains all interior neutrosophic SuperHyperVertices and all exterior neutrosophic SuperHyperVertices where any of them has one neutrosophic SuperHyperNeighbor out. Then a neutrosophic SuperHyperForcing is a neutrosophic SuperHyperSet of the exterior neutrosophic SuperHyperVertices and the interior neutrosophic SuperHyperVertices with only one exception in the form of interior neutrosophic SuperHyperVertices from any given neutrosophic SuperHyperEdge with the minimum neutrosophic cardinality. A neutrosophic SuperHyperForcing has the minimum neutrosophic cardinality on all neutrosophic SuperHyperForcing has the number of all the neutrosophic SuperHyperVertices minus on the neutrosophic cardinality of the second neutrosophic SuperHyperPart plus one. Thus,
Where is the unary operation on the SuperHyperVertices of the SuperHyperGraph to assign the determinacy, the indeterminacy and the neutrality, for respectively. □
Example 11.
In the Figure 29, the SuperHyperStar is highlighted and featured.
The obtained neutrosophic SuperHyperSet, by the Algorithm in previous result, of the neutrosophic SuperHyperVertices of the connected neutrosophic SuperHyperStar in the neutrosophic SuperHyperModel (29), is the neutrosophic SuperHyperForcing.
Proposition 25.
Assume a connected neutrosophic SuperHyperBipartite Then a neutrosophic SuperHyperForcing is a neutrosophic SuperHyperSet of the exterior neutrosophic SuperHyperVertices and the interior neutrosophic SuperHyperVertices with only one exception in the form of interior neutrosophic SuperHyperVertices from any given neutrosophic SuperHyperEdge with the minimum neutrosophic cardinality. A neutrosophic SuperHyperForcing has the minimum neutrosophic cardinality on all neutrosophic SuperHyperForcing has the number of all the neutrosophic SuperHyperVertices minus on the neutrosophic cardinality of the first neutrosophic SuperHyperPart plus the second neutrosophic SuperHyperPart. Thus,
Where is the unary operation on the SuperHyperVertices of the SuperHyperGraph to assign the determinacy, the indeterminacy and the neutrality, for respectively.
Proof.
Assume a connected neutrosophic SuperHyperBipartite Let a neutrosophic SuperHyperEdge has some neutrosophic SuperHyperVertices. Consider some extreme numbers of those neutrosophic SuperHyperVertices from that neutrosophic SuperHyperEdge excluding two unique neutrosophic SuperHyperVertices, belong to any given neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices. The neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex but there are two white neutrosophic SuperHyperNeighbors outside implying there’s no neutrosophic SuperHyperVertex to the neutrosophic SuperHyperSet S does the “the color-change rule”. So it doesn’t have the minimum neutrosophic SuperHyperCardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex. Consider some extreme numbers of those neutrosophic SuperHyperVertices from that neutrosophic SuperHyperEdge, without any exclusion on some neutrosophic SuperHyperVertices, belong to any given neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices. The neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex but it implies it doesn’t have the minimum neutrosophic SuperHyperCardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex. There’s only one neutrosophic SuperHyperVertex outside the intended neutrosophic SuperHyperSet. Thus the obvious neutrosophic SuperHyperForcing is up. The obvious simple type-neutrosophic SuperHyperSet of the neutrosophic SuperHyperForcing is a neutrosophic SuperHyperSet excludes only one neutrosophic SuperHyperVertex in a connected neutrosophic SuperHyperBipartite Thus all the following neutrosophic SuperHyperSets of neutrosophic SuperHyperVertices are the simple neutrosophic type-SuperHyperSet of the neutrosophic SuperHyperForcing. It’s the contradiction to the neutrosophic SuperHyperSet S is a neutrosophic SuperHyperForcing. Thus any given neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices contains the number of those neutrosophic SuperHyperVertices from that neutrosophic SuperHyperEdge with some neutrosophic SuperHyperVertices less than excluding one unique neutrosophic SuperHyperVertex, isn’t a neutrosophic SuperHyperForcing. Thus if a neutrosophic SuperHyperEdge has some neutrosophic SuperHyperVertices, then, with excluding one unique neutrosophic SuperHyperVertex, the all number of those neutrosophic SuperHyperVertices from that neutrosophic SuperHyperEdge belong to any neutrosophic SuperHyperForcing. Thus, in a connected neutrosophic SuperHyperBipartite every neutrosophic SuperHyperEdge has only one unique neutrosophic SuperHyperVertex outside of neutrosophic SuperHyperForcing. In other words, every neutrosophic SuperHyperEdge has only one unique white neutrosophic SuperHyperVertex. In a connected neutrosophic SuperHyperBipartite the any neutrosophic SuperHyperForcing only contains all interior neutrosophic SuperHyperVertices and all exterior neutrosophic SuperHyperVertices where any of them has one neutrosophic SuperHyperNeighbor out. Then a neutrosophic SuperHyperForcing is a neutrosophic SuperHyperSet of the exterior neutrosophic SuperHyperVertices and the interior neutrosophic SuperHyperVertices with only one exception in the form of interior neutrosophic SuperHyperVertices from any given neutrosophic SuperHyperEdge with the minimum neutrosophic cardinality. A neutrosophic SuperHyperForcing has the minimum neutrosophic cardinality on all neutrosophic SuperHyperForcing has the number of all the neutrosophic SuperHyperVertices minus on the neutrosophic cardinality of the first neutrosophic SuperHyperPart plus the second neutrosophic SuperHyperPart. Thus,
Where is the unary operation on the SuperHyperVertices of the SuperHyperGraph to assign the determinacy, the indeterminacy and the neutrality, for respectively. □
Example 12.
In Figure 30, the SuperHyperBipartite is highlighted and featured.
The obtained neutrosophic SuperHyperSet, by the Algorithm in previous result, of the neutrosophic SuperHyperVertices of the connected neutrosophic SuperHyperBipartite in the neutrosophic SuperHyperModel (30), is the neutrosophic SuperHyperForcing.
Proposition 26.
Assume a connected neutrosophic SuperHyperMultipartite Then a neutrosophic SuperHyperForcing is a neutrosophic SuperHyperSet of the exterior neutrosophic SuperHyperVertices and the interior neutrosophic SuperHyperVertices with only one exception in the form of interior neutrosophic SuperHyperVertices from any given neutrosophic SuperHyperEdge with the minimum neutrosophic cardinality. A neutrosophic SuperHyperForcing has the minimum neutrosophic cardinality on all neutrosophic SuperHyperForcing has the number of all the neutrosophic SuperHyperVertices minus on the neutrosophic number of the summation on the neutrosophic cardinality of the neutrosophic SuperHyperParts. Thus,
Where is the unary operation on the SuperHyperVertices of the SuperHyperGraph to assign the determinacy, the indeterminacy and the neutrality, for respectively.
Proof.
Assume a connected neutrosophic SuperHyperMultipartite Let a neutrosophic SuperHyperEdge has some neutrosophic SuperHyperVertices. Consider some extreme numbers of those neutrosophic SuperHyperVertices from that neutrosophic SuperHyperEdge excluding two unique neutrosophic SuperHyperVertices, belong to any given neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices. The neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex but there are two white neutrosophic SuperHyperNeighbors outside implying there’s no neutrosophic SuperHyperVertex to the neutrosophic SuperHyperSet S does the “the color-change rule”. So it doesn’t have the minimum neutrosophic SuperHyperCardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex. Consider some extreme numbers of those neutrosophic SuperHyperVertices from that neutrosophic SuperHyperEdge, without any exclusion on some neutrosophic SuperHyperVertices, belong to any given neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices. The neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex but it implies it doesn’t have the minimum neutrosophic SuperHyperCardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex. There’s only one neutrosophic SuperHyperVertex outside the intended neutrosophic SuperHyperSet. Thus the obvious neutrosophic SuperHyperForcing is up. The obvious simple type-neutrosophic SuperHyperSet of the neutrosophic SuperHyperForcing is a neutrosophic SuperHyperSet excludes only one neutrosophic SuperHyperVertex in a connected neutrosophic SuperHyperMultipartite Thus all the following neutrosophic SuperHyperSets of neutrosophic SuperHyperVertices are the simple neutrosophic type-SuperHyperSet of the neutrosophic SuperHyperForcing. It’s the contradiction to the neutrosophic SuperHyperSet S is a neutrosophic SuperHyperForcing. Thus any given neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices contains the number of those neutrosophic SuperHyperVertices from that neutrosophic SuperHyperEdge with some neutrosophic SuperHyperVertices less than excluding one unique neutrosophic SuperHyperVertex, isn’t a neutrosophic SuperHyperForcing. Thus if a neutrosophic SuperHyperEdge has some neutrosophic SuperHyperVertices, then, with excluding one unique neutrosophic SuperHyperVertex, the all number of those neutrosophic SuperHyperVertices from that neutrosophic SuperHyperEdge belong to any neutrosophic SuperHyperForcing. Thus, in a connected neutrosophic SuperHyperMultipartite every neutrosophic SuperHyperEdge has only one unique neutrosophic SuperHyperVertex outside of neutrosophic SuperHyperForcing. In other words, every neutrosophic SuperHyperEdge has only one unique white neutrosophic SuperHyperVertex. In a connected neutrosophic SuperHyperMultipartite the any neutrosophic SuperHyperForcing only contains all interior neutrosophic SuperHyperVertices and all exterior neutrosophic SuperHyperVertices where any of them has one neutrosophic SuperHyperNeighbor out. Then a neutrosophic SuperHyperForcing is a neutrosophic SuperHyperSet of the exterior neutrosophic SuperHyperVertices and the interior neutrosophic SuperHyperVertices with only one exception in the form of interior neutrosophic SuperHyperVertices from any given neutrosophic SuperHyperEdge with the minimum neutrosophic cardinality. A neutrosophic SuperHyperForcing has the minimum neutrosophic cardinality on all neutrosophic SuperHyperForcing has the number of all the neutrosophic SuperHyperVertices minus on the neutrosophic number of the summation on the neutrosophic cardinality of the neutrosophic SuperHyperParts. Thus,
Where is the unary operation on the SuperHyperVertices of the SuperHyperGraph to assign the determinacy, the indeterminacy and the neutrality, for respectively. □
Example 13.
In Figure 31, the SuperHyperMultipartite is highlighted and featured.
The obtained neutrosophic SuperHyperSet, by the Algorithm in previous result, of the neutrosophic SuperHyperVertices of the connected neutrosophic SuperHyperMultipartite in the neutrosophic SuperHyperModel (31), is the neutrosophic SuperHyperForcing.
Proposition 27.
Assume a connected neutrosophic SuperHyperWheel Then a neutrosophic SuperHyperForcing is a neutrosophic SuperHyperSet of the exterior neutrosophic SuperHyperVertices and the interior neutrosophic SuperHyperVertices with only one exception in the form of interior neutrosophic SuperHyperVertices from any given neutrosophic SuperHyperEdge with the minimum neutrosophic cardinality. A neutrosophic SuperHyperForcing has the minimum neutrosophic cardinality on all neutrosophic SuperHyperForcing has the number of all the neutrosophic SuperHyperVertices minus the number of all the neutrosophic SuperHyperEdges. Thus,
Where is the unary operation on the SuperHyperVertices of the SuperHyperGraph to assign the determinacy, the indeterminacy and the neutrality, for respectively.
Proof.
Assume a connected neutrosophic SuperHyperWheel Let a neutrosophic SuperHyperEdge has some neutrosophic SuperHyperVertices. Consider some extreme numbers of those neutrosophic SuperHyperVertices from that neutrosophic SuperHyperEdge excluding two unique neutrosophic SuperHyperVertices, belong to any given neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices. The neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex but there are two white neutrosophic SuperHyperNeighbors outside implying there’s no neutrosophic SuperHyperVertex to the neutrosophic SuperHyperSet S does the “the color-change rule”. So it doesn’t have the minimum neutrosophic SuperHyperCardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex. Consider some extreme numbers of those neutrosophic SuperHyperVertices from that neutrosophic SuperHyperEdge, without any exclusion on some neutrosophic SuperHyperVertices, belong to any given neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices. The neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex but it implies it doesn’t have the minimum neutrosophic SuperHyperCardinality of a neutrosophic SuperHyperSet S of black neutrosophic SuperHyperVertices (whereas neutrosophic SuperHyperVertices in are colored white) such that is turned black after finitely many applications of “the color-change rule”: a white neutrosophic SuperHyperVertex is converted to a black neutrosophic SuperHyperVertex if it is the only white neutrosophic SuperHyperNeighbor of a black neutrosophic SuperHyperVertex. There’s only one neutrosophic SuperHyperVertex outside the intended neutrosophic SuperHyperSet. Thus the obvious neutrosophic SuperHyperForcing is up. The obvious simple type-neutrosophic SuperHyperSet of the neutrosophic SuperHyperForcing is a neutrosophic SuperHyperSet excludes only one neutrosophic SuperHyperVertex in a connected neutrosophic SuperHyperWheel Thus all the following neutrosophic SuperHyperSets of neutrosophic SuperHyperVertices are the simple neutrosophic type-SuperHyperSet of the neutrosophic SuperHyperForcing. It’s the contradiction to the neutrosophic SuperHyperSet S is a neutrosophic SuperHyperForcing. Thus any given neutrosophic SuperHyperSet of the neutrosophic SuperHyperVertices contains the number of those neutrosophic SuperHyperVertices from that neutrosophic SuperHyperEdge with some neutrosophic SuperHyperVertices less than excluding one unique neutrosophic SuperHyperVertex, isn’t a neutrosophic SuperHyperForcing. Thus if a neutrosophic SuperHyperEdge has some neutrosophic SuperHyperVertices, then, with excluding one unique neutrosophic SuperHyperVertex, the all number of those neutrosophic SuperHyperVertices from that neutrosophic SuperHyperEdge belong to any neutrosophic SuperHyperForcing. Thus, in a connected neutrosophic SuperHyperWheel every neutrosophic SuperHyperEdge has only one unique neutrosophic SuperHyperVertex outside of neutrosophic SuperHyperForcing. In other words, every neutrosophic SuperHyperEdge has only one unique white neutrosophic SuperHyperVertex. In a connected neutrosophic SuperHyperWheel the any neutrosophic SuperHyperForcing only contains all interior neutrosophic SuperHyperVertices and all exterior neutrosophic SuperHyperVertices where any of them has one neutrosophic SuperHyperNeighbor out. Then a neutrosophic SuperHyperForcing is a neutrosophic SuperHyperSet of the exterior neutrosophic SuperHyperVertices and the interior neutrosophic SuperHyperVertices with only one exception in the form of interior neutrosophic SuperHyperVertices from any given neutrosophic SuperHyperEdge with the minimum neutrosophic cardinality. A neutrosophic SuperHyperForcing has the minimum neutrosophic cardinality on all neutrosophic SuperHyperForcing has the number of all the neutrosophic SuperHyperVertices minus the number of all the neutrosophic SuperHyperEdges. Thus,
Where is the unary operation on the SuperHyperVertices of the SuperHyperGraph to assign the determinacy, the indeterminacy and the neutrality, for respectively. □
Example 14.
In the Figure 32, the SuperHyperWheel is highlighted and featured.
The obtained neutrosophic SuperHyperSet, by the Algorithm in previous result, of the neutrosophic SuperHyperVertices of the connected neutrosophic SuperHyperWheel in the neutrosophic SuperHyperModel (32), is the neutrosophic SuperHyperForcing.
References
- Henry Garrett, “Properties of SuperHyperGraph and Neutrosophic SuperHyperGraph”, Neutrosophic Sets and Systems 49 (2022) 531-561 (doi: 10.5281/zenodo.6456413). (http://fs.unm.edu/NSS/ NeutrosophicSuperHyperGraph34.pdf). (https://digitalrepository.unm.edu/nss_journal/vol49/iss1/34). [CrossRef]
- Henry Garrett, “Neutrosophic Co-degree and Neutrosophic Degree alongside Chromatic Numbers in the Setting of Some Classes Related to Neutrosophic Hypergraphs”, J Curr Trends Comp Sci Res 1(1) (2022) 06-14.Henry Garrett, “”, Preprints 2022, 2022120549. [CrossRef]
- Henry Garrett, “(Neutrosophic) SuperHyperModeling of Cancer’s Recognitions Featuring (Neutrosophic) SuperHyperDefensive SuperHyperAlliances”, Preprints 2022, 2022120549. [CrossRef]
- Henry Garrett, “(Neutrosophic) SuperHyperAlliances With SuperHyperDefensive and SuperHyperOffensive Type-SuperHyperSet On (Neutrosophic) SuperHyperGraph With (Neutrosophic) SuperHyperModeling of Cancer’s Recognitions And Related (Neutrosophic) SuperHyperClasses”, Preprints 2022, 2022120540. [CrossRef]
- Henry Garrett, “SuperHyperGirth on SuperHyperGraph and Neutrosophic SuperHyperGraph With SuperHyperModeling of Cancer’s Recognitions”, Preprints 2022, 2022120500. [CrossRef]
- Henry Garrett, “Some SuperHyperDegrees and Co-SuperHyperDegrees on Neutrosophic SuperHyperGraphs and SuperHyperGraphs Alongside Applications in Cancer’s Treatments”, Preprints 2022, 2022120324. [CrossRef]
- Henry Garrett, “SuperHyperDominating and SuperHyperResolving on Neutrosophic SuperHyperGraphs And Their Directions in Game Theory and Neutrosophic SuperHyperClasses”, Preprints 2022, 2022110576. [CrossRef]
- Henry Garrett, “Basic Neutrosophic Notions Concerning SuperHyperDominating and Neutrosophic SuperHyperResolving in SuperHyperGraph”, ResearchGate 2022. [CrossRef]
- Henry Garrett, “Initial Material of Neutrosophic Preliminaries to Study Some Neutrosophic Notions Based on Neutrosophic SuperHyperEdge (NSHE) in Neutrosophic SuperHyperGraph (NSHG)”, ResearchGate 2022. [CrossRef]
- Henry Garrett, (2022). “Beyond Neutrosophic Graphs”, Ohio: E-publishing: Educational Publisher 1091 West 1st Ave Grandview Heights, Ohio 43212 United States. ISBN: 979-1-59973-725-6 (http://fs.unm.edu/BeyondNeutrosophicGraphs.pdf).
- Henry Garrett, (2022). “Neutrosophic Duality”, Florida: GLOBAL KNOWLEDGE - Publishing House 848 Brickell Ave Ste 950 Miami, Florida 33131 United States. ISBN: 978-1-59973-743-0 (http://fs.unm.edu/NeutrosophicDuality.pdf).
- F. Smarandache, “Extension of HyperGraph to n-SuperHyperGraph and to Plithogenic n-SuperHyperGraph, and Extension of HyperAlgebra to n-ary (Classical-/Neutro-/Anti-) HyperAlgebra”, Neutrosophic Sets and Systems 33 (2020) 290-296. [CrossRef]
- M. Akram et al., “Single-valued neutrosophic Hypergraphs”, TWMS J. App. Eng. Math. 8 (1) (2018) 122-135.
- S. Broumi et al., “Single-valued neutrosophic graphs”, Journal of New Theory 10 (2016) 86-101.
- H. Wang et al., “Single-valued neutrosophic sets”, Multispace and Multistructure 4 (2010) 410-413.
- H.T. Nguyen and E.A. Walker, “A First course in fuzzy logic”, CRC Press, 2006.
Figure 21.
A SuperHyperPath Associated to the Notions of SuperHyperForcing in the Example (3).
Figure 22.
A SuperHyperCycle Associated to the Notions of SuperHyperForcing in the Example (4).
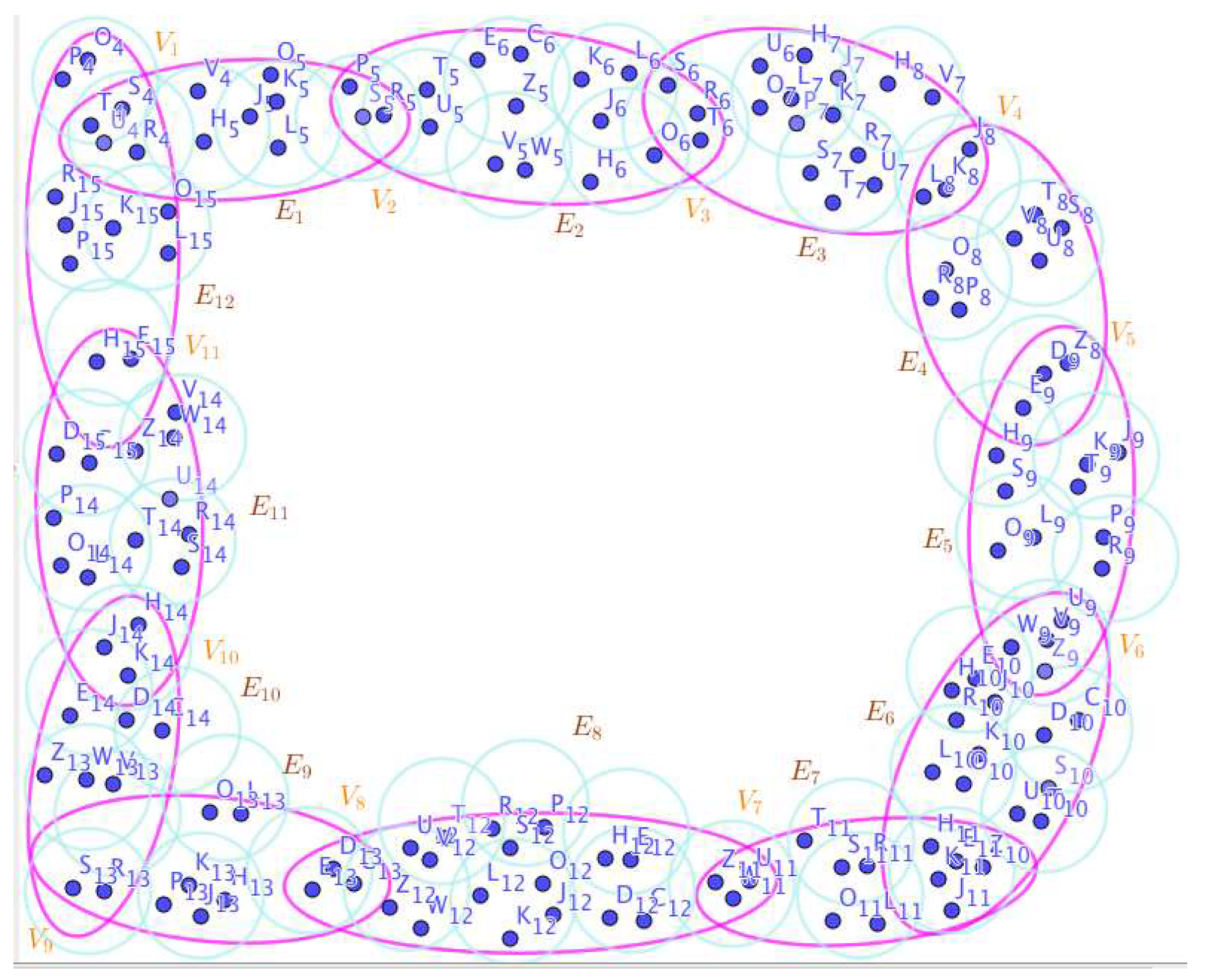
Figure 23.
A SuperHyperStar Associated to the Notions of SuperHyperForcing in the Example (5).

Figure 24.
A SuperHyperBipartite Associated to the Notions of SuperHyperForcing in the Example (6).

Figure 25.
A SuperHyperMultipartite Associated to the Notions of SuperHyperForcing in the Example (7).
Figure 25.
A SuperHyperMultipartite Associated to the Notions of SuperHyperForcing in the Example (7).

Figure 26.
A SuperHyperWheel Associated to the Notions of SuperHyperForcing in the Example (8).

Figure 27.
A Neutrosophic SuperHyperPath Associated to the Notions of SuperHyperForcing in the Example (9).
Figure 27.
A Neutrosophic SuperHyperPath Associated to the Notions of SuperHyperForcing in the Example (9).

Figure 28.
A Neutrosophic SuperHyperCycle Associated to the Notions of SuperHyperForcing in the Example (10).
Figure 28.
A Neutrosophic SuperHyperCycle Associated to the Notions of SuperHyperForcing in the Example (10).
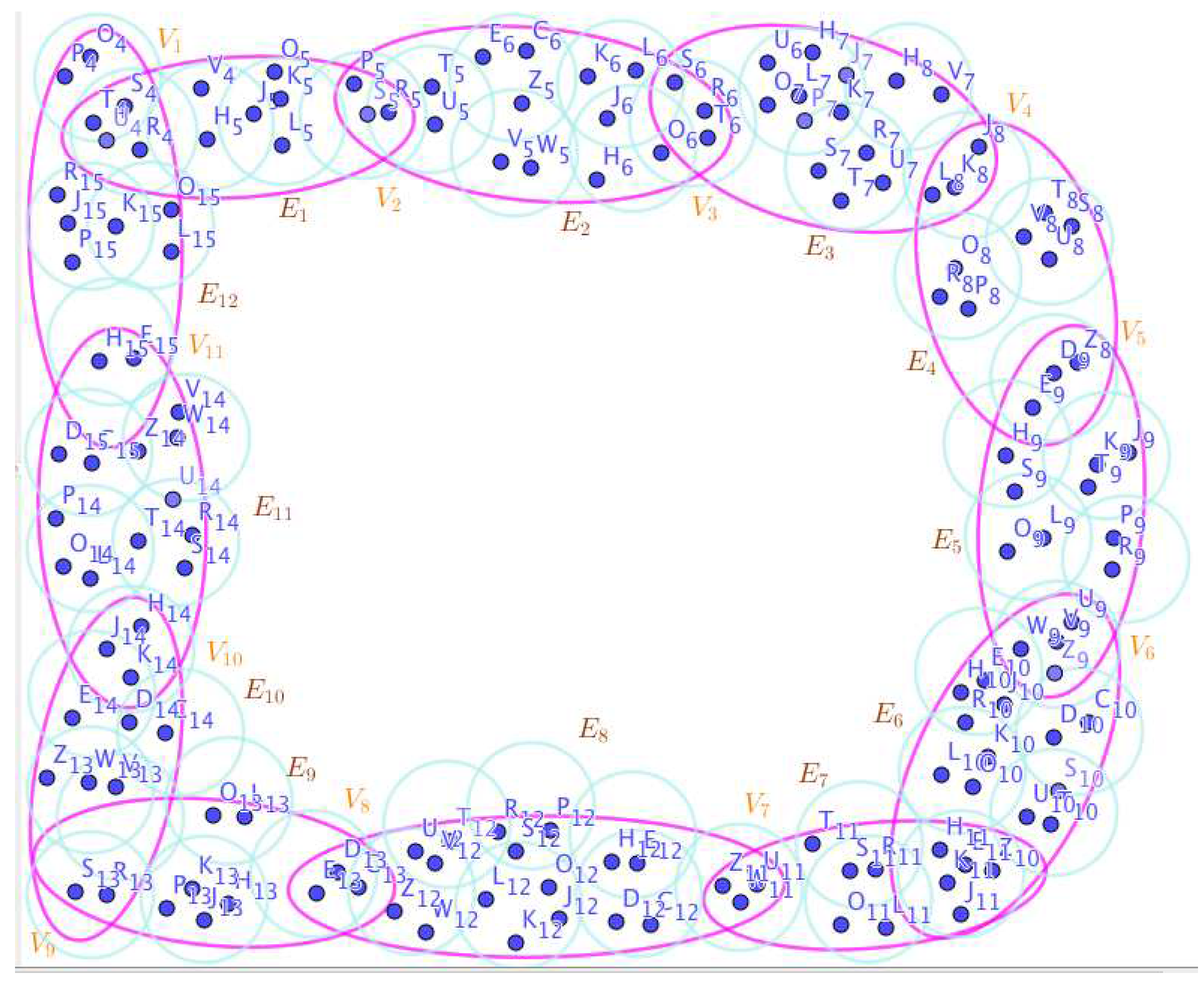
Figure 29.
A Neutrosophic SuperHyperStar Associated to the Notions of SuperHyperForcing in the Example (11).
Figure 29.
A Neutrosophic SuperHyperStar Associated to the Notions of SuperHyperForcing in the Example (11).

Figure 30.
A Neutrosophic SuperHyperBipartite Associated to the Notions of SuperHyperForcing in the Example (12).
Figure 30.
A Neutrosophic SuperHyperBipartite Associated to the Notions of SuperHyperForcing in the Example (12).

Figure 31.
A Neutrosophic SuperHyperMultipartite Associated to the Notions of SuperHyperForcing in the Example (13).
Figure 31.
A Neutrosophic SuperHyperMultipartite Associated to the Notions of SuperHyperForcing in the Example (13).

Figure 32.
A Neutrosophic SuperHyperWheel Associated to the Notions of SuperHyperForcing in the Example (14).
Figure 32.
A Neutrosophic SuperHyperWheel Associated to the Notions of SuperHyperForcing in the Example (14).

Table 1.
The Values of Vertices, SuperVertices, Edges, HyperEdges, and SuperHyperEdges Belong to The Neutrosophic SuperHyperGraph Mentioned in the Definition (5).
Table 1.
The Values of Vertices, SuperVertices, Edges, HyperEdges, and SuperHyperEdges Belong to The Neutrosophic SuperHyperGraph Mentioned in the Definition (5).
| The Values of The Vertices | The Number of Position in Alphabet |
|---|---|
| The Values of The SuperVertices | The Minimum Values of Its Vertices |
| The Values of The Edges | The Minimum Values of Its Vertices |
| The Values of The HyperEdges | The Minimum Values of Its Vertices |
| The Values of The SuperHyperEdges | The Minimum Values of Its Endpoints |
Table 2.
The Values of Vertices, SuperVertices, Edges, HyperEdges, and SuperHyperEdges Belong to The Neutrosophic SuperHyperGraph, Mentioned in the Definition (4).
Table 2.
The Values of Vertices, SuperVertices, Edges, HyperEdges, and SuperHyperEdges Belong to The Neutrosophic SuperHyperGraph, Mentioned in the Definition (4).
| The Values of The Vertices | The Number of Position in Alphabet |
|---|---|
| The Values of The SuperVertices | The Minimum Values of Its Vertices |
| The Values of The Edges | The Minimum Values of Its Vertices |
| The Values of The HyperEdges | The Minimum Values of Its Vertices |
| The Values of The SuperHyperEdges | The Minimum Values of Its Endpoints |
Table 3.
The Values of Vertices, SuperVertices, Edges, HyperEdges, and SuperHyperEdges Belong to The Neutrosophic SuperHyperGraph Mentioned in the Definition (5).
Table 3.
The Values of Vertices, SuperVertices, Edges, HyperEdges, and SuperHyperEdges Belong to The Neutrosophic SuperHyperGraph Mentioned in the Definition (5).
| The Values of The Vertices | The Number of Position in Alphabet |
|---|---|
| The Values of The SuperVertices | The Minimum Values of Its Vertices |
| The Values of The Edges | The Minimum Values of Its Vertices |
| The Values of The HyperEdges | The Minimum Values of Its Vertices |
| The Values of The SuperHyperEdges | The Minimum Values of Its Endpoints |
Table 4.
The Values of Vertices, SuperVertices, Edges, HyperEdges, and SuperHyperEdges Belong to The Neutrosophic SuperHyperPath Mentioned in the Example (9).
Table 4.
The Values of Vertices, SuperVertices, Edges, HyperEdges, and SuperHyperEdges Belong to The Neutrosophic SuperHyperPath Mentioned in the Example (9).
| The Values of The Vertices | The Number of Position in Alphabet |
|---|---|
| The Values of The SuperVertices | The Minimum Values of Its Vertices |
| The Values of The Edges | The Minimum Values of Its Vertices |
| The Values of The HyperEdges | The Minimum Values of Its Vertices |
| The Values of The SuperHyperEdges | The Minimum Values of Its Endpoints |
Table 5.
The Values of Vertices, SuperVertices, Edges, HyperEdges, and SuperHyperEdges Belong to The Neutrosophic SuperHyperCycle Mentioned in the Example (10).
Table 5.
The Values of Vertices, SuperVertices, Edges, HyperEdges, and SuperHyperEdges Belong to The Neutrosophic SuperHyperCycle Mentioned in the Example (10).
| The Values of The Vertices | The Number of Position in Alphabet |
|---|---|
| The Values of The SuperVertices | The Minimum Values of Its Vertices |
| The Values of The Edges | The Minimum Values of Its Vertices |
| The Values of The HyperEdges | The Minimum Values of Its Vertices |
| The Values of The SuperHyperEdges | The Minimum Values of Its Endpoints |
Table 6.
The Values of Vertices, SuperVertices, Edges, HyperEdges, and SuperHyperEdges Belong to The Neutrosophic SuperHyperStar Mentioned in the Example (11).
Table 6.
The Values of Vertices, SuperVertices, Edges, HyperEdges, and SuperHyperEdges Belong to The Neutrosophic SuperHyperStar Mentioned in the Example (11).
| The Values of The Vertices | The Number of Position in Alphabet |
|---|---|
| The Values of The SuperVertices | The Minimum Values of Its Vertices |
| The Values of The Edges | The Minimum Values of Its Vertices |
| The Values of The HyperEdges | The Minimum Values of Its Vertices |
| The Values of The SuperHyperEdges | The Minimum Values of Its Endpoints |
Table 7.
The Values of Vertices, SuperVertices, Edges, HyperEdges, and SuperHyperEdges Belong to The Neutrosophic SuperHyperBipartite Mentioned in the Example (12).
Table 7.
The Values of Vertices, SuperVertices, Edges, HyperEdges, and SuperHyperEdges Belong to The Neutrosophic SuperHyperBipartite Mentioned in the Example (12).
| The Values of The Vertices | The Number of Position in Alphabet |
|---|---|
| The Values of The SuperVertices | The Minimum Values of Its Vertices |
| The Values of The Edges | The Minimum Values of Its Vertices |
| The Values of The HyperEdges | The Minimum Values of Its Vertices |
| The Values of The SuperHyperEdges | The Minimum Values of Its Endpoints |
Table 8.
The Values of Vertices, SuperVertices, Edges, HyperEdges, and SuperHyperEdges Belong to The Neutrosophic SuperHyperMultipartite Mentioned in the Example (13).
Table 8.
The Values of Vertices, SuperVertices, Edges, HyperEdges, and SuperHyperEdges Belong to The Neutrosophic SuperHyperMultipartite Mentioned in the Example (13).
| The Values of The Vertices | The Number of Position in Alphabet |
|---|---|
| The Values of The SuperVertices | The Minimum Values of Its Vertices |
| The Values of The Edges | The Minimum Values of Its Vertices |
| The Values of The HyperEdges | The Minimum Values of Its Vertices |
| The Values of The SuperHyperEdges | The Minimum Values of Its Endpoints |
Table 9.
The Values of Vertices, SuperVertices, Edges, HyperEdges, and SuperHyperEdges Belong to The Neutrosophic SuperHyperWheel Mentioned in the Example (14).
Table 9.
The Values of Vertices, SuperVertices, Edges, HyperEdges, and SuperHyperEdges Belong to The Neutrosophic SuperHyperWheel Mentioned in the Example (14).
| The Values of The Vertices | The Number of Position in Alphabet |
|---|---|
| The Values of The SuperVertices | The Minimum Values of Its Vertices |
| The Values of The Edges | The Minimum Values of Its Vertices |
| The Values of The HyperEdges | The Minimum Values of Its Vertices |
| The Values of The SuperHyperEdges | The Minimum Values of Its Endpoints |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Copyright: This open access article is published under a Creative Commons CC BY 4.0 license, which permit the free download, distribution, and reuse, provided that the author and preprint are cited in any reuse.
MDPI Initiatives
Important Links
© 2024 MDPI (Basel, Switzerland) unless otherwise stated






