Introduction

1. Model of the universe

1.6. Force acting on a test mass

1.6.1. Case I : Force acting on a test particle (object) placed on the surface of observer (Earth)

Figure 2. Representation of virtual-graviton flux lines passing through an object (test particle) placed on the surface of Earth (observer).

Figure 2. Representation of virtual-graviton flux lines passing through an object (test particle) placed on the surface of Earth (observer).

The following assumptions are made for an object resting on the surface of the Earth:

• The size of the object is negligible compared to the Earth.
• The virtual-graviton flux generated by the object is negligible compared to the flux generated by the Earth.
• The flux lines are collimated at the object.

The vacuum energy density at the object is obtained by integrating Eq. (11) and taking the energy density at infinity, i.e., ${\zeta }^{*}$ as the datum.

$${\zeta }_t=\frac{{\left(\zeta \sout{V}\mathrm{\Gamma }\right)}_o}{4\pi D{m}_g}\frac{M_o}{r_{ot}}$$

(13)

The influence of the test particle on the energy density is negligible compared to the Earth. Therefore, the energy density inside the test particle is assumed to be given by Eq. (13).

Although the test particle is at rest on the Earth, the vacuum is not. The equilibrium between the test particle and the vacuum is governed by ${\mathrm{\Gamma }}_t$. The variation of flux over the volume of the test particle is also ignored.

The test particle interacts with the vacuum through graviton exchange. Coupling with the virtual-graviton flux is also the only pathway through which the Earth affects the test particle, if the physical contact is ignored. Therefore, as before, the vacuum energy flux, energy density gradient, and pressure gradient are calculated for the test particle as

$${\mathbf{J}}_{\mathbf{t}}=\frac{{\left(\zeta \sout{V}\mathrm{\Gamma }\right)}_o}{4\pi D{m}_g}\frac{{\left(\sout{V}\mathrm{\Gamma }\right)}_t}{4\pi m_g}\frac{M_o{M}_t}{r_{ot}{x}_t^2}$$

(14)

$$\nabla {\zeta }_t=-\frac{{\left(\zeta \sout{V}\mathrm{\Gamma }\right)}_o}{4\pi D{m}_g}\frac{{\left(\sout{V}\mathrm{\Gamma }\right)}_t}{4\pi D{m}_g}\frac{M_o{M}_t}{r_{ot}{x}_t^2}$$

(15)

$$\nabla {\mathbf{P}}_{\mathbf{t}}=-\frac{{\left(\zeta \sout{V}\mathrm{\Gamma }\right)}_o}{4\pi D{m}_g}\frac{{\left(\sout{V}\mathrm{\Gamma }\right)}_t}{4\pi D{m}_g}\frac{M_o{M}_t}{r_{ot}{x}_t^2}$$

(16)

In this case, $x_t$ is measured radially outward from the center of the test particle.

The net pressure gradient at any point is space is $\nabla \mathbf{P}=\nabla {\mathbf{P}}_{\mathbf{o}}+\nabla {\mathbf{P}}_{\mathbf{t}}$. The combined action of the Earth and test particle accelerates the vacuum super-fluid. But, as pointed out earlier, the influence of the test particle on the vacuum is ignored compared to the Earth. Therefore, the vacuum super-fluid is approximated as accelerated under the influence of the Earth alone. Thus, the pressure at the test particle is obtained by integrating the net pressure gradient with the Earth as the source. The pressure at infinity is taken as the datum, so the pressure at the test particle is

$$\mathbf{P}=-\frac{{\left(\zeta \sout{V}\mathrm{\Gamma }\right)}_o}{4\pi D{m}_g}\frac{M_o}{r_{ot}}-\frac{{\left(\zeta \sout{V}\mathrm{\Gamma }\right)}_o}{4\pi D{m}_g}\frac{{\left(\sout{V}\mathrm{\Gamma }\right)}_t}{4\pi D{m}_g}\frac{M_o{M}_t}{r_{ot}^2}$$

(17)

The velocity of the virtual-graviton super-fluid at the test particle is calculated using Bernoulli’s principle.

$$v_{\zeta }^2=\frac{2\mathbf{P}}{\rho }$$

(18)

Here, $\rho$ is the density of vacuum super-fluid and is taken constant at every point in space. The velocity of the vacuum at infinity is taken as zero.

The momentum carried by a virtual-graviton near the test particle is $m_g(v_{\zeta }+v_g)$. As before, $v_g$ is oriented in random directions with equal probabilities. Therefore, the average value of $v_g$ equals zero, i.e., the average momentum carried by a virtual-graviton is $m_g{v}_{\zeta }$. Similarly, the outgoing observer-graviton carries a momentum $m_g{v}_{\zeta }$. But the outgoing observer-graviton is ejected in a random direction with equal probability. Therefore, the net momentum carried by the outgoing observer-graviton is also zero. It is concluded that the average momentum transferred to the observer in interaction with one virtual-graviton is $m_g{v}_{\zeta }$.

The force transferred on the test particle is

$$F=\frac{dp}{dt}$$

(19)

Here, $p=m_g{v}_{\zeta }$. From Eq. (6), the equilibrium rate is not the same for the observer and the test particle. Equation (19) gives the force acting on the test particle, in an ideal case when ${\mathrm{\Gamma }}_t=1$. To calculate the force on the test particle, in its own time frame, Eq. (7) is used:

$$\begin{array}{cc}\hfill F& ={\mathrm{\Gamma }}_t\frac{dp}{d{t}_t}\hfill \end{array}$$

(20)

$$\begin{array}{cc}\hfill & =\dot{m_g}{v}_{\zeta }{\mathrm{\Gamma }}_t\hfill \end{array}$$

(21)

$$\begin{array}{cc}\hfill & =\rho v_{\zeta }^2{\mathrm{\Gamma }}_t{A}_t\hfill \end{array}$$

(22)

Here, $\dot{m_g}$ is the graviton mass flow rate at test particle, and $A_t$ is the cross-sectional area of test particle normal to graviton flow.

Substitute the value of $v_{\zeta }$ from Eq. (18) in Eq. (22) to obtain the expression of force

$$F=-2{\mathrm{\Gamma }}_t{A}_t\left\{\frac{{\left(\zeta \sout{V}\mathrm{\Gamma }\right)}_o}{4\pi D{m}_g}\frac{M_o}{r_{ot}}+\frac{{\left(\zeta \sout{V}\mathrm{\Gamma }\right)}_o}{4\pi D{m}_g}\frac{{\left(\sout{V}\mathrm{\Gamma }\right)}_t}{4\pi D{m}_g}\frac{M_o{M}_t}{r_{ot}^2}\right\}$$

(23)

The first term in the parenthesis of Eq. (23) has no coupling with the test particle. It represents the uncoupled graviton flux that can couple with another test particle, as will be shown in subsequent sections. As such, this term will be ignored from the expression of force.1

The final expression of force between observer and the test particle is

$$F=-G^b\frac{M_o{M}_t}{r_{ot}^2}{\mathrm{\Gamma }}_t^2$$

(24)

Here, $G^b=2\frac{{\left(\zeta \sout{V}\mathrm{\Gamma }\right)}_o}{4\pi D{m}_g}\frac{{\left(\sout{V}A\right)}_t}{4\pi D{m}_g}$.

1.6.2. Case II: Force acting on a planet orbiting a star

Figure 3. (a) Representation of virtual-graviton flux lines between the planet (test particle) and star (observer) (b) Approximation of flux lines passing through the planet and converging at some point between the planet and the star.

Figure 3. (a) Representation of virtual-graviton flux lines between the planet (test particle) and star (observer) (b) Approximation of flux lines passing through the planet and converging at some point between the planet and the star.

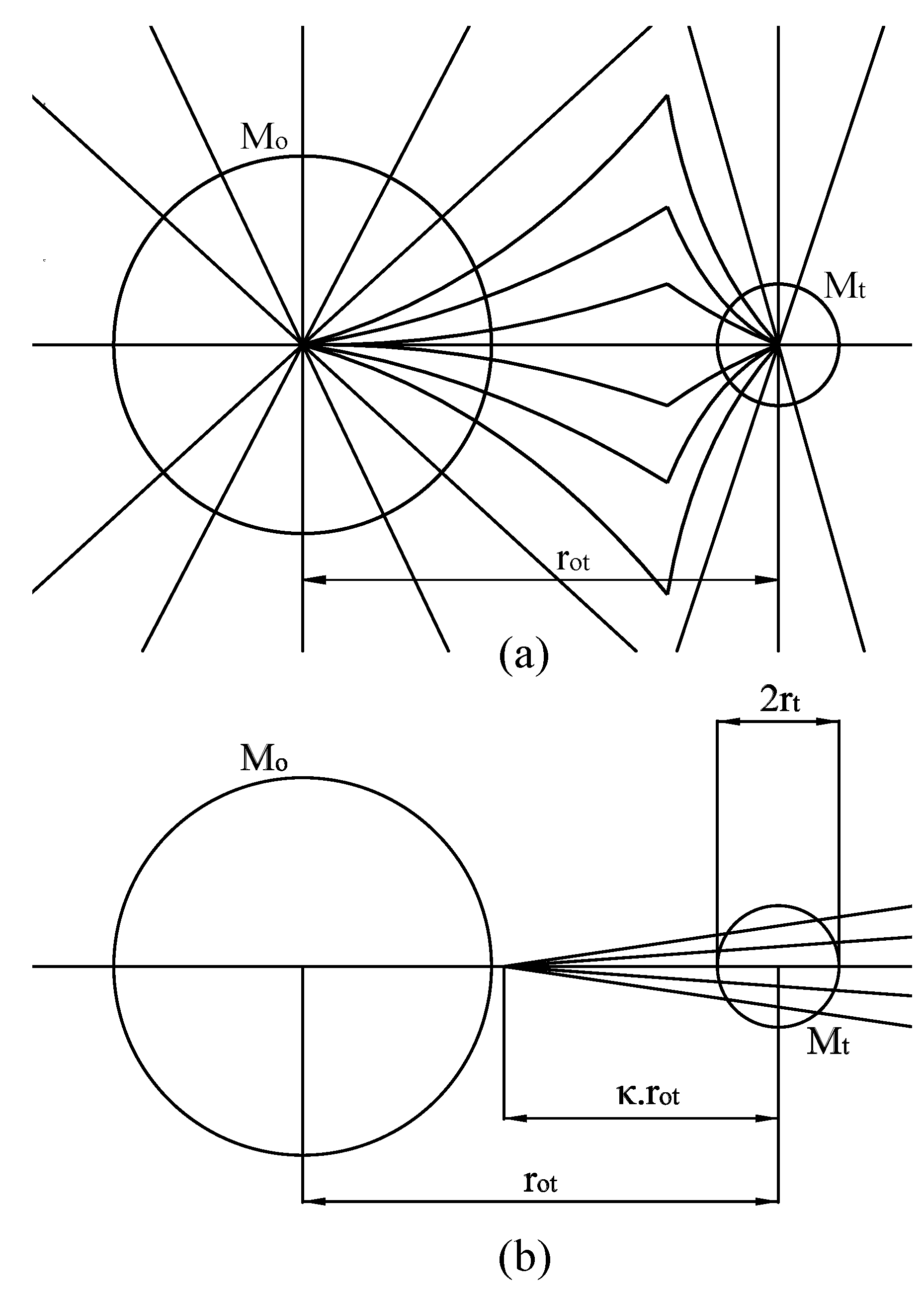
The following assumptions are made:

• The energy density at the location of the planet is determined by the energy gradient set by the star.
• The flux generated by the star is greater than the planet.
• $r_{ot},\kappa r_{ot}\gg r_t$.

Since the flux generated by the planet is not ignored, the net flux at any point inside the planet is a vector sum given by ${\mathbf{J}}_{\mathbf{o}}+{\mathbf{J}}_{\mathbf{t}}$. Therefore, th virtual-gravitons’ velocity is a vector along the net flux direction. Thus, the flux lines are not collimated about $r_{ot}$, which makes the force calculation difficult.

An approximation is made based on the following argument. In the previous case, the flux of the test particle is ignored in favor of the Earth, and flux lines converge on Earth’s center. In an extreme case where the flux generated by the Earth is ignored in favor of the test particle, the situation will reverse, and flux lines will converge at the center of the test particle. Therefore, in between the two extremes, the flux lines converge at some point between the Earth and the test particle..

In the present case, where the star’s flux is greater than the planet, the flux lines may be assumed to converge at a distance $\kappa r_{ot}$ from the planet. Since the planet’s size is much smaller than the distance $\kappa r_{ot}$, it is further assumed that flux lines are collimated at the planet. Following the above argument, the force between the planet and the sun is similar to the Eq. (24):

$$F=-G^b{\kappa }^{*}\frac{M_o{M}_t}{r_{ot}^2}{\mathrm{\Gamma }}_t^2$$

(25)

Where ${\kappa }^{*}=\frac{1}{{\kappa }^2}$.

2. Implications

3. Concluding remarks

References

1. Isham, C.J. Prima facie questions in quantum gravity. In Canonical gravity: From classical to quantum; Springer, 1994; pp. 1–21.
2. Kiefer, C. Conceptual problems in quantum gravity and quantum cosmology. International Scholarly Research Notices 2013, 2013. [Google Scholar] [CrossRef]
3. Rovelli, C. Notes for a brief history of quantum gravity. arXiv preprint gr-qc/0006061 2000. [Google Scholar]
4. Carlip, S. Is quantum gravity necessary? Classical and Quantum Gravity 2008, 25, 154010. [Google Scholar] [CrossRef]
5. Jefimenko, O.D. On the experimental proofs of relativistic length contraction and time dilation. Zeitschrift für Naturforschung A 1998, 53, 977–982. [Google Scholar] [CrossRef]
6. Sastry, G. Is length contraction really paradoxical? American Journal of Physics 1987, 55, 943–946. [Google Scholar] [CrossRef]
7. Lorentz, H.A. Electromagnetic phenomena in a system moving with any velocity smaller than that of light. In Collected Papers; Springer, 1937; pp. 172–197.
8. Hecht, E. Einstein never approved of relativistic mass. The Physics Teacher 2009, 47, 336–341. [Google Scholar] [CrossRef]
9. Okun, L.B. The concept of mass. Physics today 1989, 42, 31–36. [Google Scholar] [CrossRef]
10. Adler, C.G. Does mass really depend on velocity, dad? American Journal of Physics 1987, 55, 739–743. [Google Scholar] [CrossRef]
11. Rubin, V.C.; Ford Jr, W.K.; Thonnard, N.; Burstein, D. Rotational properties of 23 SB galaxies. The Astrophysical Journal 1982, 261, 439–456. [Google Scholar] [CrossRef]
12. Bosma, A. 21-cm line studies of spiral galaxies. II. The distribution and kinematics of neutral hydrogen in spiral galaxies of various morphological types. The Astronomical Journal 1981, 86, 1825–1846. [Google Scholar] [CrossRef]
13. Morgan, E. Estimating the vacuum energy density–an overview of possible scenarios. Jozef Stefan Institute 2005. [Google Scholar]
14. Moreva, E.; Brida, G.; Gramegna, M.; Giovannetti, V.; Maccone, L.; Genovese, M. Time from quantum entanglement: an experimental illustration. Physical Review A 2014, 89, 052122. [Google Scholar] [CrossRef]
15. Lee, J.W.; Kim, H.C.; Lee, J. Gravity as a quantum entanglement force. Journal of the Korean Physical Society 2015, 66, 1025–1030. [Google Scholar] [CrossRef]
16. Krisnanda, T.; Tham, G.Y.; Paternostro, M.; Paterek, T. Observable quantum entanglement due to gravity. npj Quantum Information 2020, 6, 1–6. [Google Scholar] [CrossRef]
17. Sanders, R.H.; McGaugh, S.S. Modified Newtonian dynamics as an alternative to dark matter. Annual Review of Astronomy and Astrophysics 2002, 40, 263–317. [Google Scholar] [CrossRef]
18. Barukčić, I. Newton’s gravitational constant big g is not a constant. Journal of Modern Physics 2016, 7, 510–522. [Google Scholar] [CrossRef]
19. Rothleitner, C.; Schlamminger, S. Invited review article: Measurements of the Newtonian constant of gravitation, G. Review of Scientific Instruments 2017, 88, 111101. [Google Scholar] [CrossRef] [PubMed]
20. Quinn, T.; Speake, C. The Newtonian constant of gravitation—a constant too difficult to measure? An introduction, 2014.
21. Wu, J.; Li, Q.; Liu, J.; Xue, C.; Yang, S.; Shao, C.; Tu, L.; Hu, Z.; Luo, J. Progress in precise measurements of the gravitational constant. Annalen der Physik 2019, 531, 1900013. [Google Scholar] [CrossRef]
22. Mohr, P.J.; Taylor, B.N.; Newell, D. The fundamental physical constants. Physics Today 2007, 60, 52–55. [Google Scholar] [CrossRef]
23. Gershteyn, M.L.; Gershteyn, L.I.; Gershteyn, A.; Karagioz, O.V. Experimental evidence that the gravitational constant varies with orientation. arXiv preprint physics/0202058 2002. [Google Scholar]
24. Will, C.M. Relativistic gravity in the solar system. II. Anisotropy in the Newtonian gravitational constant. The Astrophysical Journal 1971, 169, 141. [Google Scholar] [CrossRef]
25. Iorio, L. Observational constraints on spatial anisotropy of G from orbital motions. Classical and Quantum Gravity 2011, 28, 225027. [Google Scholar] [CrossRef]
26. Dirac, P.A.M. Is there an Aether? Nature 1951, 168, 906–907. [Google Scholar] [CrossRef]
27. Dirac, P. Is there an Æther? Nature 1952, 169, 702–702. [Google Scholar] [CrossRef]
28. Sinha, K.; Sivaram, C.; Sudarshan, E. Aether as a superfluid state of particle-antiparticle pairs. Foundations of Physics 1976, 6, 65–70. [Google Scholar] [CrossRef]
29. Sinha, K.; Sivaram, C.; Sudarshan, E. The superfluid vacuum state, time-varying cosmological constant, and nonsingular cosmological models. Foundations of Physics 1976, 6, 717–726. [Google Scholar] [CrossRef]
30. Sinha, K.; Sudarshan, E. The superfluid as a source of all interactions. Foundations of Physics 1978, 8, 823–831. [Google Scholar] [CrossRef]
31. Urban, M.; Couchot, F.; Sarazin, X.; Djannati-Atai, A. The quantum vacuum as the origin of the speed of light. The European Physical Journal D 2013, 67, 58. [Google Scholar] [CrossRef]
32. Trippe, S. A simplified treatment of gravitational interaction on galactic scales. arXiv preprint arXiv:1211.4692 2012. [Google Scholar] [CrossRef]
33. Platscher, M.; Smirnov, J.; Meyer, S.; Bartelmann, M. Long range effects in gravity theories with Vainshtein screening. Journal of Cosmology and Astroparticle Physics 2018, 2018, 009. [Google Scholar] [CrossRef]
34. Ali, A.F.; Das, S. Cosmology from quantum potential. Physics letters B 2015, 741, 276–279. [Google Scholar]
35. Pushing gravity : new perspectives on Le Sage’s theory of gravitation / edited by Matthew R. Edwards.; Apeiron: Montreal, 2002.
36. Múnera, H.A. A Le Sagian atomic-type model for propagation and generation of gravity. In Should the Laws of Gravitation be Reconsidered?–The Scientific Legacy of Maurice Allais; 2011; pp. 385–422. [Google Scholar]
37. Maxwell, J.C. Atom. Encyclopaedia Britannica 1875, 9, 46–47. [Google Scholar]
38. Poincaré, H.; Maitland, F. Science and method; Courier Corporation, 2003.
39. Zamyatnin, Y.S.; Konovalov, V.Y. Probability of nuclear fisson and effective fisson cross sections: Datasheet from Landolt-Börnstein - Group I Elementary Particles, Nuclei and Atoms · Volume 16A1: “Low Energy Neutrons and their Interaction with Nuclei and Matter. Part 1” in SpringerMaterials. 2000. [Google Scholar] [CrossRef]
40. Anderson, E. Problem of time in quantum gravity. Annalen der Physik 2012, 524, 757–786. [Google Scholar] [CrossRef]
41. Isham, C.J. Canonical quantum gravity and the problem of time. In Integrable systems, quantum groups, and quantum field theories; Springer, 1993; pp. 157–287.
42. Anderson, E. Problem of time in quantum gravity. Annalen der Physik 2012, 524, 757–786. [Google Scholar] [CrossRef]
43. Zeh, H.D.; Page, D.N. The physical basis of the direction of time, 1990.
44. Lebowitz, J.L.; others. Boltzmann’s entropy and time’s arrow. Physics today 1993, 46, 32–32. [Google Scholar] [CrossRef]
45. Gold, T. The arrow of time. American Journal of Physics 1962, 30, 403–410. [Google Scholar] [CrossRef]
46. Celotti, A.; Miller, J.C.; Sciama, D.W. Astrophysical evidence for the existence of black holes. Classical and Quantum Gravity 1999, 16, A3. [Google Scholar] [CrossRef]
47. Weinberg, S. The cosmological constant problem. Reviews of modern physics 1989, 61, 1. [Google Scholar] [CrossRef]