Prerpints.org logo
Preprint
Article

On the Thermodynamics of Matter

Altmetrics

Downloads

295

Views

154

Comments

0

This version is not peer-reviewed

Submitted:

23 February 2023

Posted:

24 February 2023

Read the latest preprint version here

Alerts
Abstract
The holographic principle states that the information about the volume of space is stored on its boundary. Assuming this holds, we can explain the aspects of gravity. It strongly seems that ”information” is a more fundamental entity than the structure of spacetime as used as basis in almost every gravity theory. In this paper, we present this connection between gravity and information. This naturally explains the four classical tests of gravity namely the gravitational redshift, the perihelion precession, the bending of light and the gravitational time delay. The spacetime need to be extended to 5D to explain gravity in this context.
Keywords: 
Subject: Physical Sciences  -   Astronomy and Astrophysics

1. Introduction

Our first encounter with gravity came from the realization of Newton of gravity as an attractive force between every two objects in the universe, mathematically described by the universal law of gravitation. Despite being experienced by us in daily life, gravity remains the most mysterious force of all. Einstein realized gravity not as a fundamental force but as a very abstract phenomenon arising from the curvature of spacetime induced by matter and encoded in the famous Einstein’s field equation. General Relativity(GR) as we call the Einstein’s theory is a metric theory that beautifully explains every phenomenon observed at large scales to date. However, the theory is incompatible with another cornerstone and highly successful theory of Quantum Mechanics. Moreover being a metric theory, it admits certain unphysical solutions such as closed timelike curves and spacetime singularity. It also remains difficult to explain dark matter and dark energy within the framework of GR. The first link between thermodynamics and gravity came from the black hole physics where there is an apparent connection between horizon area and the entropy of the black hole. Hawking[1] first showed that the area of the horizon(A) of a black hole is a non-decreasing function of time.
d A d t ≥ 0
Bekenstein[2,3,4] took this further and asserted the equivalence of the horizon area with the thermodynamic parameter, entropy(S) as
S = γ A
where γ is a constant. The claim got a robust description when Hawking[5] derived the temperature of the black hole, thus making the relation between the area and entropy clear. Later, in 1995, Jacobson[6] derived the Einstein’s equation from the proportionality of entropy and horizon area together with the relation d Q = T d S connecting heat, entropy, and temperature. There are also closely related follow up articles[7,8,9,10,11]. Another work relating thermodynamics and gravity are due to Padmanabhan[12,13,14,15,16,17,18,19,20,21,22,23] and his collaborators[24,25,26,27,28]. These results suggest that gravity may be explained as an emergent phenomenon and has a thermodynamic or entropic origin. Recently, Verlinde[29] proposed Newtonian gravity as a physical entropic force, caused by changes in the information associated with the positions of material bodies, although, this description of Newtonian gravity as a physical entropic force has been technically questioned too[30,31,32,33]. Our goal here is different, we are making no connection with the background spacetime metric g μ ν and are thus not set to derive Einstein’s gravity as Jacobson and Padmanabhan did because we do not think that Einstein’s approach of describing gravity as background spacetime curvature is the only and ultimate reality. We are also not justifying or falsifying the claim of Verlinde of Newtonian gravity as a physical entropic force. Since we are not using the Einstein’s approach of gravity as spacetime curvature, we explicitly show then, how can we explain the gravitational redshift, the modified equation of motion for both massive and massless particles and the accelerated expansion of the universe. This way of describing gravity as a non-metric theory naturally does away with the inherent unphysical problems of a metric theory such as closed timelike curves and spacetime singularity. Keeping these things in mind, we begin our discussion by understanding this new connection between gravity and "information" in the system. In section 3 we explain how the thermodynamic nature of our system changes the total energy and this leads to the extension of the spacetime to 5 D . In section 4 we find the solution for a point mass in our theory. In section 5 we review the Quantum Focusing Conjecture(QFC)[34] which conjectures that the quantum expansion Θ , where Θ is given by
Θ = θ + 4 G ℏ A S o u t ′
( θ is the classical expansion and A is the width of null congruence along its generator), cannot increase along any congruence, which is valid for quantum states too
d Θ d λ ≤ 0
where λ is an affine parameter. We conclude the paper by showing using the QFC how the second derivative of the radius of a null boundary which respects the null energy condition(NEC) cannot decrease
R ¨ ( t ) ≥ 0
This can be applied to a black hole’s event horizon and we can get an additional constraint other than the Hawking area theorem.

2. Some Terminology

Preprints 69072 g001
Figure 1. Our system consists of a source mass M and a "boundary" which is a data storing surface such as a holographic screen. T is the temperature of the bounding surface arising from the evenly distribution of the total energy E of the mass m as N bits of information as it approaches the holographic boundary.
Figure 1. Our system consists of a source mass M and a "boundary" which is a data storing surface such as a holographic screen. T is the temperature of the bounding surface arising from the evenly distribution of the total energy E of the mass m as N bits of information as it approaches the holographic boundary.
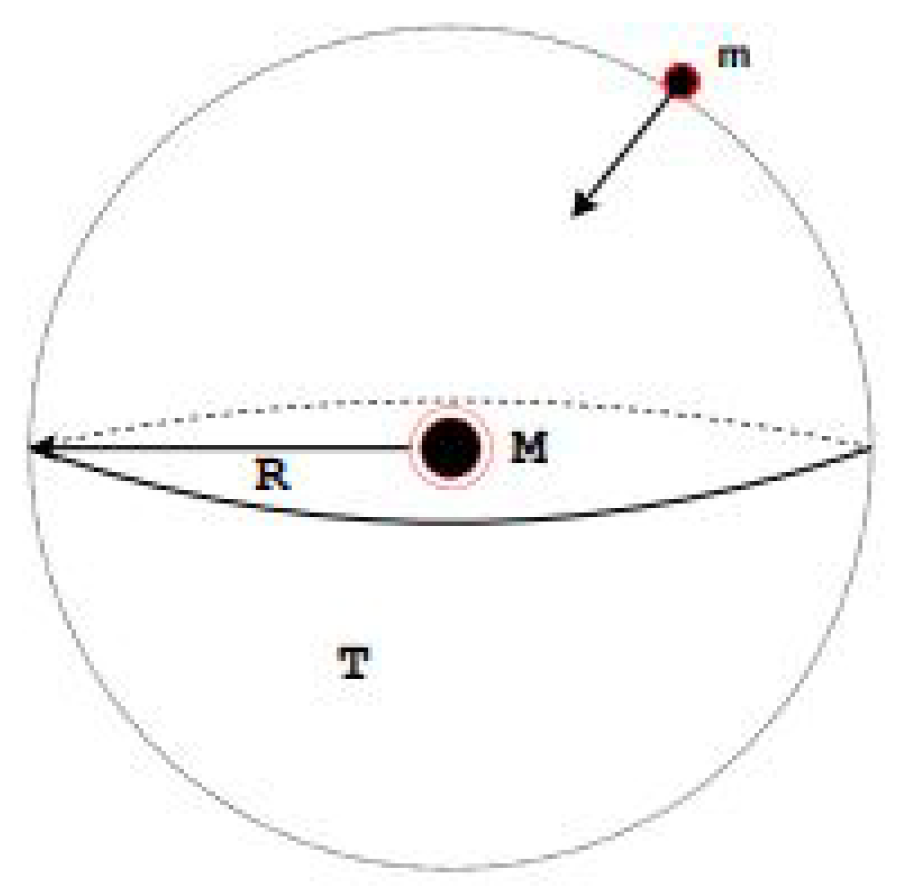
Preprints 69072 g001
Consider the system consisting of a source mass M and a boundary. The "boundary" here is a data storing surface such as a holographic screen. The Bekenstein bound[35], gives the bound on entropy associated with a mass M as1
S ≤ 2 π R M ℏ
where R is the size of the system and ℏ is the reduced Planck’s constant. The "disorder capacity" of a mass M is defined as the maximum entropy associated with it as
C D = 2 π R M ℏ
1In the rest of the paper we set G = c = k B = 1
The "information capacity" C I is defined as the maximum entropy of the system. This entropy is assumed to follow the holographic principle[36,37] such that the information about the volume of space is stored on the boundary. Thus we assume that the total degrees of freedom is given by
N = A l p 2
where A is the surface area of the boundary and l p is the Planck length. The holographic principle has strong pieces of evidence from the AdS/CFT correspondence[38] and black hole physics[5,3]. Thus,
C I = N = A l p 2
The "disorder" D is defined by2
D = C D C I
2see [39]
The temperature T of the boundary is found by assuming that the total energy of the object is stored on the boundary as evenly bits of information N as it comes near the boundary thus we can use the equipartition rule of thermodynamics
p 0 = 1 2 N T
where p 0 is the energy of the object near the boundary.

3. Effect on Total Energy

Due to the thermodynamic nature of our system, the total energy changes by the internal energy(U) and the fluctuation energy as we show now. The internal energy is given by
U = T S
where T is the temperature of the boundary and S is given by C D . To find the degree of fluctuation in our system we expand the disorder D about its equilibrium position x 0 μ
D = D 0 + ∂ D ∂ x μ | 0 d x μ + 1 2 ∂ 2 D ∂ x μ ∂ x ν | 0 d x μ d x ν + . . . . .
Since x 0 μ is the equilibrium point for D, we have
∂ D ∂ x μ | 0 = 0
Thus, in second order we have:
Δ D = D − D 0 = 1 2 ∂ 2 D ∂ x μ ∂ x ν | 0 d x μ d x ν
The hessian h μ ν which captures the degree of "fluctuation" in our thermodynamic system is given as
h μ ν = ∂ μ ∂ ν D
Another important dimensionless quantity(which can be used to define the "length" of fluctuation) is defined as
h ˜ μ ν = A π h μ ν
where A is the surface area of the boundary. d l 2 is the length squared of fluctuation given by
d l 2 = 1 2 h ˜ μ ν d x μ d x ν
We thus define the fluctuation energy as
d l d τ 2 = 1 2 h ˜ μ ν d x μ ˙ d x ν ˙
This thermodynamic expansion of the total energy comes at the expense of extending the 4 D spacetime to 5 D spacetime such that the non-zero metric elements are given by
g 00 = 1 , g 11 = g 22 = g 33 = g 04 = g 40 = − 1
The fifth element being defined as
p 4 = U + d l d τ

4. Static Mass and Spherically Symmetric Solution

The total degrees of freedom on the boundary is
N = A l p 2 = 4 π r 2 l p 2
Thus
U = M p 0 r
for h ˜ μ ν , only 11 component survives. Thus
h ˜ 11 = 4 M r
Hence the fluctuation energy in our thermodynamic system is
d l d τ 2 = 2 M r r ˙ 2
Thus
d l d τ = 2 M r r ˙
So we get
d x 4 = M r d t + 2 M r d r
The spacetime interval is given as
d s 2 = g μ ν d x μ d x ν
where g μ ν is the fixed 5 D background spacetime. Hence in the spherical coordinates we get the spacetime interval as
d s 2 = d t 2 − d r 2 − r 2 ( d θ 2 + s i n 2 θ ϕ 2 ) − 2 M r d t 2 − 2 2 M r d t d r
Eq.(29) is the Gullstrand-Painleve metric and a coordinate transformation converts it to the well-known Schwarzschild metric given as
d s 2 = 1 − 2 M r d t 2 − 1 − 2 M r − 1 d r 2 − r 2 ( d θ 2 + s i n 2 θ d ϕ 2 )

5. Quantum Focusing Conjecture

Using the Quantum Focusing Conjecture(QFC)[34], we can show that the second derivative of the radius of a null boundary which respects the null energy condition(NEC) cannot is non-negative. In this section we first review the formulation of QFC.

5.1. Generalised Entropy for Cauchy-splitting surfaces

Generalised entropy was originally defined in [40] in asymptotically flat space as the area A of all black hole horizons, plus the entropy of matter systems outside the black holes:
S g e n = S o u t + A 4 G ℏ + c o u n t e r t e r m s
A rigorous definition of S o u t can be given as the von Neumann entropy of the quantum state of the exterior of the horizon:
S o u t = − t r ρ o u t l n ρ o u t
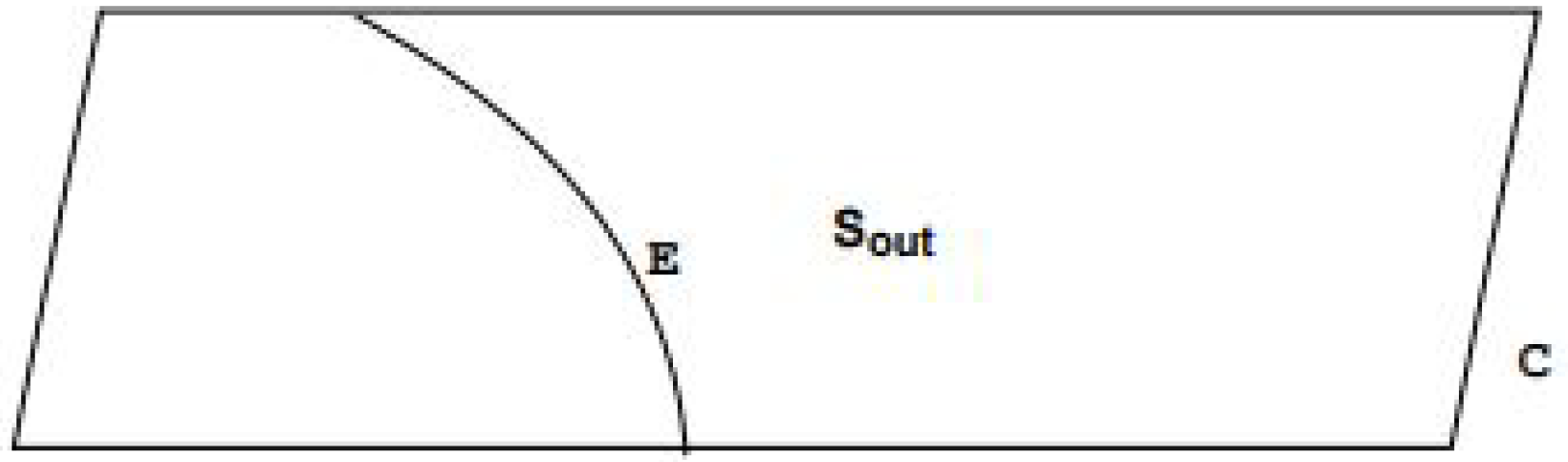Preprints 69072 g002
Figure 2. A Cauchy surface C is divided into two parts by a surface E. S o u t is defined as entropy restricted to one side of splitting surface E.
Figure 2. A Cauchy surface C is divided into two parts by a surface E. S o u t is defined as entropy restricted to one side of splitting surface E.
Preprints 69072 g002
The GSL was introduced to keep the second law of thermodynamics intact when matter entropy is lost in a black hole. Bekenstein conjectured that GSL [40] survives: the area increase of the black hole will compensate for the lost matter entropy, so that the generalized entropy will not decrease. The notion of generalised entropy can be extended beyond the context of causal horizons [34]. Let σ be a spacelike codimension-2 surface that splits a Cauchy surface Σ into two portions. By picking any one of the two sides of σ arbitrarily, we can define an entropy restricted to one side of σ as S o u t .

5.2. Quantum Focusing Conjecture

It conjectures [34] that the quantum expansion Θ , where Θ is given by:
Θ = θ + 4 G ℏ A S o u t ′
( θ is the classical expansion and A is the width of null congruence along its generator), cannot increase along any congruence, which is valid for quantum states too:
d Θ d λ ≤ 0
where λ is an affine parameter. The evolution of the expansion θ along congruence is determined by the Raychaudhuri equation:
d θ d λ = − θ 2 2 − σ a b σ a b − R a b k a k b :
where R a b is the Ricci tensor, σ a b is the shear and k a is the (null) tangent vector to the congruence. This gives QFC as:
0 ≥ Θ ′ = θ ′ + 4 G ℏ A ( S o u t ′ ′ − S o u t ′ θ ) = − 1 2 θ 2 − ζ 2 − 8 π G 〈 T k k 〉 + 4 G ℏ A ( S o u t ′ ′ − S o u t ′ θ )
ζ is shear. The special choice of congruence for θ = ζ = 0 , gives the Quantum Null Energy Condition (QNEC):
〈 T k k 〉 ≥ ℏ 2 π A S o u t ′ ′
The entropy S o u t which refers to the entropy on a spacelike Cauchy surface can be alternatively seen as the entropy of the state restricted to the part of null surface N [34]. The stress tensor T a b and entanglement entropy S o u t is related to relative entropy S ( ρ | | ρ o ) on a null surface by[34]
2 π A ℏ T k k − S o u t ′ ′ = S ′ ′ ( ρ | | ρ o )
where T k k = T a b k a k b , k a is a null vector, i.e, it satisfies g μ ν k μ k ν = 0 . ρ is an arbitrary state and ρ o is the vacuum state.

6. Accelerated Expansion of a Null Surface

Let a null surface N enclose a region of space which respects the null energy condition(NEC) given by
T k k ≥ 0
Eq. (38) holds for this surface. Assuming N to be spherical, let at an initial time its radius be R. On applying NEC, Eq. (38) becomes an inequality and we get,
S ′ ′ ( ρ | | ρ o ) ≥ − S o u t ′ ′
The relationship between relative entropy and Bekenstein bound is given by [41]
S ( ρ | | ρ o ) = K v − S v
where K v is localized energy and S v ≡ S o u t − S o u t ( 0 ) is a localized entropy. Here S o u t ( 0 ) is the vacuum entropy. Eq. (40) can be written explicitly in the form
d 2 S ( ρ | | ρ o ) d λ 2 ≥ − d 2 S o u t d λ 2
where λ is an affine parameter. Thus, we can write
d 2 K v d λ 2 − d 2 S o u t d λ 2 + d 2 S o u t ( 0 ) d λ 2 ≥ − d 2 S o u t d λ 2
Since the vacuum entropy S o u t ( 0 ) is independent of affine parameter λ it gives:
d 2 S o u t ( 0 ) d λ 2 = 0
, thus from Eq. (43), we get
d 2 K v d λ 2 ≥ 0
Now we equate the classical expression for K v . Using K v ≡ b E R [41], where b is a constant and E is the total energy of the system, we get
d 2 b E R d λ 2 ≥ 0
and since the total energy E of the system is constant, thus choosing the affine parameter λ as time, we finally get
R ¨ ≥ 0
Thus the second derivative of the radius of a null boundary which respects the NEC cannot decrease. Applying this to a black hole’s event horizon we get an additional constraint than the previously known Hawking area theorem.
d A d t ≥ 0
and
d 2 R d t 2 ≥ 0
where R is the radius and A is the surface area of the boundary.

7. Conclusion

This approach of describing gravity as information leads to a very important question that whether or not gravity is a fundamental force as popularly seen. We think the way gravity affects time strongly suggests that gravity can not be a force in the usual sense. Of course, we have to make an additional assumption that the degrees of freedom follows the holographic principle and scales as the area rather than the volume of space but this assumption is very robust in itself looking at black hole physics and gauge/gravity duality. The other important result we showed is that, if we assume a null surface which encloses matter that respects the NEC then the second derivative of its radius is non-negative. This can be applied to a black hole’s event horizon.

Conflict of Interest

The author declares no conflict of interest.

References

  1. Hawking, S.W. Gravitational radiation from colliding black holes. Physical Review Letters 1971, 26, 1344. [Google Scholar] [CrossRef]
  2. Bekenstein, J.D. Black holes and the second law. Lett. Nuovo Cim. 1972, 4, 737–740. [Google Scholar] [CrossRef]
  3. Bekenstein, J.D. Black Holes and Entropy. Phys. Rev. D 1973, 7, 2333–2346. [Google Scholar] [CrossRef]
  4. Bekenstein, J.D. Generalized second law of thermodynamics in black-hole physics. Physical Review D 1974, 9, 3292. [Google Scholar] [CrossRef]
  5. Hawking, S.W. Particle creation by black holes. Communications in Mathematical Physics 1975, 43, 199–220. [Google Scholar] [CrossRef]
  6. Jacobson. Thermodynamics of spacetime: The Einstein equation of state. Physical review letters 1995, 75, 1260–1263. [Google Scholar] [CrossRef] [PubMed]
  7. Jacobson, T.; Parentani, R. Horizon entropy. Foundations of Physics 2003, 33, 323–348. [Google Scholar] [CrossRef]
  8. Ted Jacobson. On the nature of black hole entropy. In AIP Conference Proceedings, volume 493, pages 85–97. American Institute of Physics, 1999.
  9. Eling, C.; Guedens, R.; Jacobson, T. Nonequilibrium thermodynamics of spacetime. Physical Review Letters 2006, 96, 121301. [Google Scholar] [CrossRef]
  10. Eling, C. Hydrodynamics of spacetime and vacuum viscosity. Journal of High Energy Physics 2008, 2008, 048. [Google Scholar] [CrossRef]
  11. Chirco, G.; Liberati, S. Nonequilibrium thermodynamics of spacetime: The role of gravitational dissipation. Physical Review D 2010, 81, 024016. [Google Scholar] [CrossRef]
  12. Padmanabhan, T. is gravity an intrinsically quantum phenomenon? dynamics of gravity from the entropy of space–time and the principle of equivalence. Modern Physics Letters A 2002, 17, 1147–1158. [Google Scholar] [CrossRef]
  13. Padmanabhan, T. Gravity from spacetime thermodynamics. Astrophysics and space science 2003, 285, 407–417. [Google Scholar] [CrossRef]
  14. Padmanabhan, T. Entropy of static spacetimes and microscopic density of states. Classical and Quantum Gravity 2004, 21, 4485. [Google Scholar] [CrossRef]
  15. Padmanabhan, T. Gravity and the thermodynamics of horizons. Physics Reports 2005, 406, 49–125. [Google Scholar] [CrossRef]
  16. Padmanabhan, T. Holographic gravity and the surface term in the Einstein-Hilbert action. Brazilian Journal of Physics 2005, 35, 362–372. [Google Scholar] [CrossRef]
  17. Padmanabhan, T. A new perspective on gravity and dynamics of space–time. International Journal of Modern Physics D 2005, 14, 2263–2269. [Google Scholar] [CrossRef]
  18. Padmanabhan, T. Gravity: A new holographic perspective. International Journal of Modern Physics D 2006, 15, 1659–1675. [Google Scholar] [CrossRef]
  19. Padmanabhan, T. Gravity as an emergent phenomenon. International Journal of Modern Physics D 2008, 17, 591–596. [Google Scholar] [CrossRef]
  20. Padmanabhan, T. Entropy density of spacetime and thermodynamic interpretation of field equations of gravity in any diffeomorphism invariant theory. arXiv preprint 2009, arXiv:0903.1254. [Google Scholar]
  21. Padmanabhan, T. Thermodynamical aspects of gravity: new insights. Reports on Progress in Physics 2010, 73, 046901. [Google Scholar] [CrossRef]
  22. Thanu Padmanabhan. Lessons from classical gravity about the quantum structure of spacetime. In Journal of Physics: Conference Series, volume 306, page 012001. IOP Publishing, 2011.
  23. Padmanabhan, T. Gravity and is thermodynamics. Current Science 2015, 2236–2242. [Google Scholar] [CrossRef]
  24. Paranjape, A.; Sarkar, S.; Padmanabhan, T. Thermodynamic route to field equations in Lanczos-Lovelock gravity. Physical Review D 2006, 74, 104015. [Google Scholar] [CrossRef]
  25. Mukhopadhyay, A.; Padmanabhan, T. Holography of gravitational action functionals. Physical Review D 2006, 74, 124023. [Google Scholar] [CrossRef]
  26. Kothawala, D.; Sarkar, S.; Padmanabhan, T. Einstein’s equations as a thermodynamic identity: The cases of stationary axisymmetric horizons and evolving spherically symmetric horizons. Physics Letters B 2007, 652, 338–342. [Google Scholar] [CrossRef]
  27. Kothawala, D.; Padmanabhan, T. Thermodynamic structure of Lanczos-Lovelock field equations from near-horizon symmetries. Physical Review D 2009, 79, 104020. [Google Scholar] [CrossRef]
  28. Kolekar, S.; Padmanabhan, T. Holography in action. Physical Review D 2010, 82, 024036. [Google Scholar] [CrossRef]
  29. Verlinde, E. On the origin of gravity and the laws of Newton. Journal of High Energy Physics 2011, 2011, 1–27. [Google Scholar] [CrossRef]
  30. Chaichian, M.; Oksanen, M.; Tureanu, A. On gravity as an entropic force. Physics Letters B 2011, 702, 419–421. [Google Scholar] [CrossRef]
  31. Gao, S. Is gravity an entropic force? Entropy 2011, 13, 936–948. [Google Scholar] [CrossRef]
  32. Kobakhidze, A. Gravity is not an entropic force. Physical Review D 2011, 83, 021502. [Google Scholar] [CrossRef]
  33. Kobakhidze, A. Once more: gravity is not an entropic force. arXiv preprint 2011, arXiv:1108.4161. [Google Scholar]
  34. Bousso, R.; Fisher, Z.; Leichenauer, S.; Wall, A.C. Quantum focusing conjecture. Physical Review D 2016, 93, 064044. [Google Scholar] [CrossRef]
  35. Bekenstein, J.D. Universal upper bound on the entropy-to-energy ratio for bounded systems. Phys. Rev. D 1981, 23, 287–298. [Google Scholar] [CrossRef]
  36. Hooft, G. Dimensional reduction in quantum gravity. arXiv preprint 1993, arXiv:gr-qc/9310026. [Google Scholar]
  37. Susskind, L. The world as a hologram. Journal of Mathematical Physics 1995, 36, 6377–6396. [Google Scholar] [CrossRef]
  38. Maldacena, J. The large-N limit of superconformal field theories and supergravity. International journal of theoretical physics 1999, 38, 1113–1133. [Google Scholar] [CrossRef]
  39. Landsberg, P. Can entropy and “order” increase together? Physics Letters A 1984, 102, 171–173. [Google Scholar] [CrossRef]
  40. Bekenstein, J.D. Black holes and the second law. Lettere al Nuovo Cimento (1971-1985) 2019, 4, 737–740. [Google Scholar] [CrossRef]
  41. Casini, H. Relative entropy and the Bekenstein bound. Classical and Quantum Gravity 2008, 25, 205021. [Google Scholar] [CrossRef]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content.
Copyright: This open access article is published under a Creative Commons CC BY 4.0 license, which permit the free download, distribution, and reuse, provided that the author and preprint are cited in any reuse.
Prerpints.org logo

Preprints.org is a free preprint server supported by MDPI in Basel, Switzerland.

facebook logotwitter logolinkedin logo
MDPI Initiatives
Important Links
Subscribe

Disclaimer

Terms of Use

Privacy Policy

© 2024 MDPI (Basel, Switzerland) unless otherwise stated