Prerpints.org logo
Preprint
Review

Corneal Biomechanics and Glaucoma

Altmetrics

Downloads

203

Views

88

Comments

0

A peer-reviewed article of this preprint also exists.

Submitted:

16 February 2023

Posted:

01 March 2023

You are already at the latest version

Alerts
Abstract
Biomechanics is a branch of biophysics that deals with mechanics applied to biology. The biomechanics of the cornea plays a significant role in managing patients with glaucoma. While evidence suggests a higher risk of glaucoma in patients with thin and stiffer corneas, it also affects the measurement of intraocular pressure (IOP). We reviewed the pertinent literature to help the understanding of corneal biomechanics and how it can help optimize clinical and surgical treatments and a better approach to diagnosing and managing patients with glaucoma.
Keywords: 
Subject: Medicine and Pharmacology  -   Ophthalmology

1. Introduction

Biomechanics is a branch of biophysics that deals with mechanics applied to biology for the human or animal bodies. While biomechanics is especially concerned with the muscles and the skeleton, it is also used for referring to the functioning of any other part of a body, such as a cornea [1]. The analysis of the corneal biomechanics has helped clinicians detect early or mild corneal ectasias [2,3,4], which can be further enhanced by integrating tomographic data obtained with the Pentacam (Oculus GmbH; Wetzlar, Germany) [3].
Corneal biomechanics also plays a significant role in managing patients with glaucoma [5]. First, evidence suggests a higher risk of glaucoma in patients with thin and stiffer corneas [6,7,8]. Second, corneal biomechanics affects and is affected by intraocular pressure (IOP) [9,10,11]. Therefore, one of the significant challenges of contemporary ophthalmology is understanding the independent role of corneal biomechanical properties and IOP on the ocular response to mechanical stimuli to ensure accurate measurements and proper monitoring of glaucoma patients [1].

2. The Ocular Response Analyzer

The first device that allowed the assessment of in vivo biomechanical properties was the ocular response analyzer (ORA; Reichert Ophthalmic Instruments, Inc., Buffalo, NY, USA), introduced in 2005 by David Luce (Figure 1) [12]. The ORA is a modified non-contact tonometer (NCT) designed to provide a more accurate measurement of the IOP than the Goldmann applanation tonometer (GAT) by compensating for corneal biomechanics. It produces a fast air jet that deforms the corneal curvature and records each moment of deformation. As the air pulse starts, the cornea moves inwardly, up to the first stage of applanation. At this point, the first IOP measurement is taken (P1). After a brief state of concavity, the air pulse ends. The cornea moves back to its initial position while passing through the second stage of applanation, where the system provides a second IOP measurement (P2). The difference between P1-P2 is considered corneal hysteresis (CH) (Figure 2) [13]. The CH corresponds to a dissipation of energy during the loading and unloading phases representing the viscoelastic characteristics of the cornea and sclera. It has no direct correspondence to corneal stiffness [14]. Studies have shown that CH is a dynamic parameter affected directly by the IOP with an inverse correlation. An increase in IOP decreases CH and vice versa. The CH is a parameter of the entire globe, not exclusively of the cornea. The whole eye globe responds when a loading force is applied to the cornea. As a consequence, it dissipates energy [15]. Studies have shown that CH will decrease the stiffening sclera, confirming that CH is not a local corneal parameter [16].
Other parameters generated by the ORA software are the corneal resistance factor (CRF), the compensated intraocular pressure (IOPcc), and the Goldmann correlated IOP (IOPg). The CRF is a theoretical measure of the elastic properties of the cornea, calculated with the formula a [P1–0.7P2] + d where a and d are calibration and regression constants to maximize correlation with the central corneal thickness (CCT) [17]. The IOPcc is less influenced by corneal structure properties, particularly CCT, than IOP measured by conventional GAT [18]. In a systematic review, Zhang and collaborators compared ORA and GAT in post-refractive surgery eyes. They showed that the IOPcc is closer to the true IOP in eyes that underwent corneal procedures [19]. Lastly, the IOPg is the mean of the applanation pressures and is given by the formula IOPg= (P1-P2)/2.
IOP is a constant force (loading) per unit area under the globe, playing an essential role in the biomechanical response [9,10,11]. The IOP is the most impactor predictor of deformation amplitude (DA) under an air jet load, followed by stiffness and thickness [20]. A greater IOP on a weaker cornea can produce a stiffer response than a lower IOP on a stronger cornea. The cornea and sclera have a non-linear stiffening response under the increase of IOP. Stress is a force per unit of cross-sectional area in a loaded stretched tissue. Strain is a non-dimensional deformation or percent stretch when the tissue is pulled [21]. The tangent elastic moduli are related to stiffness and are given by the stress-strain slope at a determined strain value. The slope has a non-linear behavior: as the load increases, the slope increases too [21]. The stress distribution in the cornea can be quantified using the Hoop stress formula, σ=P•R/2t, where σ is the stress, P is the IOP, R is the radius of curvature, and t is the corneal thickness. We can conclude with this equation that a thinner and cornea flatter cornea is associated with higher stress [21].
The scleral material biochemical properties also contribute to the observed corneal response in air-puff-induced deformation [20,22]. The biomechanical resistance of the sclera occurs after the aqueous displacement during the corneal recovery on the second applanation in jet air commercial tonometry. Studies have shown that the stiffer the sclera, the greater the resistance to aqueous motion, which could be wrongly interpreted as a stiffer corneal deformation [23].

3. The Corvis ST Dynamic Scheimpflug Analyzer

The Corvis ST (Oculus, Wetzlar, Germany) is also a non-contact tonometer system with a collimated air pulse and a consistent pressure profile (Figure 3). The device uses an ultra-high-speed (UHS) Scheimpflug camera to acquire 4,300 frames per second, covering 8.5 mm horizontally of a single slit, which allows a dynamical evaluation of the corneal deformation [24].
Although similar to the ORA, in which an air jet deforms the cornea, the Corvis ST uses a fixed pressure from the air jet. The cornea bends inwards to the first applanation, and then to the point that the highest concavity (HC) is achieved (Figure 4). Afterward, the cornea recovers in the outward direction and undergoes a second applanation before returning to its natural position. Advanced algorithms identify the cornea's anterior and posterior limits, and the IOP is measured on the first corneal applanation moment. Once the measurement is performed, the device provides a set of corneal deformation parameters based on the dynamic inspection of the corneal response, including analysis of those parameters that are extracted at the highest concavity point [14,24].
Whole-eye movement (WEM) is the resistance of other tissues after the cornea reaches its deformation limits and the air pressure increases. The orbital soft tissue and structure limit the WEM. The dynamic corneal response (DCR) parameters associated with the loading phase are elastic in nature [21]. One of these parameters is the point of highest concavity, the time point of the most significant resistance to aqueous movement. The stiffness parameter at the highest concavity (SP-HC), which is the moment of capture of the scleral response, is the load at first applanation (Air pressure-IOP) divided by displacement from first applanation (A1) to highest concavity [25]. Deformation ratio 2 mm (DA ratio) and integrated inverse radius (IIR) are DCR parameters related to the shape of the cornea during deformation, independent of IOP and associated with CCT. Other elastic DCR parameters associated with corneal stiffness are stress-strain index (SSI) and stiffness at the first applanation point (SP-A1) [21]. All the elastic parameters like SSI, SP-A1, and SP-HC are calculated with different algorithms, and their interpretation must be considered as different forms of stiffness. Decreasing the DA ratio, peak distance, and IIR are related to greater resistance to change in the shape of the cornea and stiffness increase. A list of all deformation parameters provided by the Corvis ST is presented in Table 1.
The Corvis ST calculates the IOP value based on the first applanation time pressure [24]. The biomechanical-compensated IOP (bIOP), available in the Vinciguerra Screening Report (Figure 5), is an IOP parameter corrected through a finite element method, using deformation data beyond CCT and age, including the deformation response [26]. For the development of the bIOP algorithm, the analysis considered eyes with different variations of IOP (10- 30mmHg), CCT (445-645 microns), and age (30- 90 years old). In each case, the corneal deformation response was predicted and used to estimate the Corvis IOP. The final analysis led to an algorithm relating the real IOP as a function of the Corvis IOP, CCT, and age. Subsequently, this algorithm of predictions of the corrected IOP was applied to a clinical data set involving a large number of normal eyes to investigate the association with corneal stiffness parameters, age, and CCT. Results demonstrated that the uncorrected IOP has a strong correlation with CCT and a waek correlation with age, whereas applying the algorithm to IOP measurements resulted in an IOP less correlated with both CCT and age. The Vinciguerra screen enabled the calculation of indexes, including Ambrósio Relational Thickness over the horizontal meridian (ARTh) and Corvis Biomechanical Index (CBI), which helps to discriminate between keratoconic and normal healthy cases [27].
More recently, Ambrósio and coworkers applied artificial intelligence to combine biomechanical and tomographic data and developed the Tomographic Biomechanical Index (TBI). This index demonstrated high sensitivity to diagnose mild or subclinical ectasia in very asymmetric ectasia with normal tomographic maps (VAE-NT) cases (Figure 6) [28,29].
Ahmed et al. introduced a new intelligent algorithm of material stiffness to assess the biomechanical properties of the human cornea in vivo, the Stress-Strain Index (SSI). While the SSI showed no significant correlation with CCT and IOP, this index was significantly correlated with age [30]. Another study showed a possible association between Corvis ST (CSV) measurements and CH. Measurements of CST, ORA, axial length, average corneal curvature, CCT, and IOP with GAT were performed in patients with primary OAG and eyes from normal subjects. Parameters including DA (corneal softness), SP A1 (corneal stiffness), and Inverse Radius (integrated area under the curve of the inverse concave radius) were significantly correlated with CH. However, CST parameters were significant but weakly or moderately related to ORA-measured CH [31].

4. Hysteresis and Glaucoma

Although it is still debatable whether a stiffer globe contributes to glaucoma or rather it is a consequence of the disease [21], there is growing evidence supporting an association between stiffer corneas and OAG and a higher risk of glaucoma in patients with thin and stiffer corneas [6,7,8,32]. Congdon et al. investigated the association of CH with visual perimeter damage and glaucoma progression risk [33]. Another study suggested that CH and CRF, associated with CCT, could be considered risk factors for glaucoma [34]. Suzanna et al. have found that lower hysteresis was associated with the risk of developing OAG, even when controlling for IOP, CCT, field status, age, and medical glaucoma therapy. Each one mmHg reduction in CH was associated with a risk of 21 % converting to glaucoma in patients with ocular hypertension [35]. The CH increases with surgeries, medical treatment, and lasers [36,37,38]. Patients with low CH respond better to IOP reduction treatments [36].
Unlike CCT, the CH is a dynamic parameter that changes with age, surgeries, prostaglandin treatment, and IOP [21]. There is a positive relationship between CCT and CH but a negative relationship between IOP and CH [39]. Thick corneas dissipate better energy than thin corneas, and eyes with higher pressure tend to dissipate less energy. The age-related thinning of the cornea happens slowly over time, which explains why it causes a lower impact on the CH than the acute variations of IOP [34,40,41]. Also important to note that an increase in CCT caused by corneal edema is associated with a lower CH, stressing the importance of considering all parameters and not only CCT [42].
The sclera also plays a central role in the biomechanical effects of the eye, being an anatomic link between the cornea and lamina cribrosa [43]. Many studies are trying to investigate the central role of the sclera in glaucoma and its relationship with lamina cribrosa [9,44]. It is unclear if scleral stiffness protects against the IOP peaks, maintaining the integrity of the optic head disc and the LC, or could be the cause of glaucoma [11,45].
Two randomized clinical trials, the Collaborative Initial Glaucoma Treatment Study and the Advanced Glaucoma Intervention Study have suggested that progressive visual field loss in OAG is associated with high IOP variations [46,47]. These studies indicate that transient high variations on IOP may expand the scleral channel, increasing strain in lamina cribrosa and causing axon damage. The biomechanical capacity of biomechanics properties of the entire eye, including the optic disc and the connective tissue within the scleral channel to dissipate energy and preserve RGC axons, may play an essential role in explaining different responses to IOP variations [44,48]. Age and CH are related to LC displacement of the lamina cribosa, and the ability to dampen IOP fluctuations could protect eyes with glaucoma from further damage [9]. Considering that CH is the ability of the cornea and the entire eye to dampen energy, a lower CH has been associated with visual field loss in OAG and normal tension glaucoma [49,50]. The CH is indeed the most important predictor compared to CRF, CCT, Goldmann-correlated IOP, corneal-compensated IOP, and refractive error [49].
Interestingly, other studies did not show any differences in corneal mechanics between glaucoma or healthy controls and some even suggest that glaucoma patients in fact have more deformable corneas [51,52,53]. A plausible explication is using incorrect Corvis ST parameters to evaluate corneal stiffness. Another reason for these differences may be a selection bias of patients in prostaglandin treatment, which leads to the consequent lowering of the stiffness of the entire globe. The bind of analogs of prostaglandins to F-receptors located at the ciliary body, trabecular meshwork, episclera, sclera, and cornea likely explains the increase of the CH [54,55,56]. This mechanism activates the F-receptors increasing the extracellular matrix expansion and reducing the collagen [57]. The remodeling of these structures decreases the resistance of aqueous flow at the uveoscleral outflow pathway and possibly increases the capacity of the entire globe to dissipate energy [58,59]. The fact that change in corneal compensated IOP from PGA therapy persist for 6 weeks after the cessation of therapy raises the question whether the PGA-induced structural alterations are reversible [56].
The stiffness parameter at highest concavity (SP-HC) is a new parameter that evaluates scleral stiffness, although its relationship with the risk of glaucoma is still unclear. Vinciguerra et al. showed that a low SP-HC is associated with advanced visual field defects in OAG. However, a limitation of the study is that a substantial number of glaucoma patients included in the analysis were treated with analogs of prostaglandins, which are known to decrease corneal stiffness [48,60]. Other researchers also studied patients under therapy for glaucoma and did not find an association between SP-HC and the risk of glaucoma [7]. For that reason, further studies are needed to clarify the role of SP-HC in OAG, glaucoma suspects, and healthy patients, and its relationship with CH and other conditions that change ocular rigidity, like aging and race. Studies have suggested that ORA waveform parameters related to the shape of the second peak are associated with scleral stiffness. This new approach may help us evaluate and understand in vivo the association between scleral stiffness and the risk of glaucoma [61].

5. Conclusions

Understanding corneal biomechanics can help diagnose and evaluate the prognosis of glaucoma. It will also allow the optimization of clinical and surgical treatments and better manage the procedures that mechanically interact or interfere with the eye. This includes a better approach to diagnosing and managing patients with glaucoma, keratoconus risk profiling, refractive surgery planning, and optimization of different collagen crosslinking treatment protocols [62,63].

Author Contributions

All authors participated in the conceptualization, methodology, resources; data curation, writing—original draft preparation, writing—review and editing, and have read and agreed to the published version of the manuscript.

Funding

This research received no external funding.

Institutional Review Board Statement

Ethical review and approval were not applicable since the study was a review of the literature not involving humans or animals.

Informed Consent Statement

Not applicable.

Data Availability Statement

Not applicable.

Conflicts of Interest

Dr. Ambrósio is a consultant to Oculus (Wetzlar, Germany). The other authors declare no conflict of interest.

References

  1. Liu J, Roberts CJ. Influence of corneal biomechanical properties on intraocular pressure measurement: quantitative analysis. J Cataract Refract Surg 2005;31(1):146-55. [CrossRef]
  2. Fontes BM, Ambrosio R, Jr., Jardim D, Velarde GC, Nose W. Corneal biomechanical metrics and anterior segment parameters in mild keratoconus. Ophthalmology 2010;117(4):673-9. [CrossRef]
  3. Luz A, Fontes BM, Lopes B, Ramos I, Schor P, Ambrosio R, Jr. ORA waveform-derived biomechanical parameters to distinguish normal from keratoconic eyes. Arq Bras Oftalmol 2013;76(2):111-7. [CrossRef]
  4. Ventura BV, Machado AP, Ambrosio R, Jr., et al. Analysis of waveform-derived ORA parameters in early forms of keratoconus and normal corneas. J Refract Surg 2013;29(9):637-43. [CrossRef]
  5. Silva JASd, Silva RSd, Ambrósio Jr R. Relevância da biomecânica da córnea no glaucoma. Revista Brasileira de Oftalmologia 2012;71.
  6. Catania F, Morenghi E, Rosetta P, Paolo V, Vinciguerra R. Corneal Biomechanics Assessment with Ultra High Speed Scheimpflug Camera in Primary Open Angle Glaucoma Compared with Healthy Subjects: A meta-analysis of the Literature. Curr Eye Res 2023;48(2):161-171. [CrossRef]
  7. Qassim A, Mullany S, Abedi F, et al. Corneal Stiffness Parameters Are Predictive of Structural and Functional Progression in Glaucoma Suspect Eyes. Ophthalmology 2021;128(7):993-1004. [CrossRef]
  8. Salvetat ML, Zeppieri M, Tosoni C, Felletti M, Grasso L, Brusini P. Corneal Deformation Parameters Provided by the Corvis-ST Pachy-Tonometer in Healthy Subjects and Glaucoma Patients. J Glaucoma 2015;24(8):568-74. [CrossRef]
  9. Lanzagorta-Aresti A, Perez-Lopez M, Palacios-Pozo E, Davo-Cabrera J. Relationship between corneal hysteresis and lamina cribrosa displacement after medical reduction of intraocular pressure. Br J Ophthalmol 2017;101(3):290-294. (In eng). [CrossRef]
  10. Zeimer RC, Ogura Y. The relation between glaucomatous damage and optic nerve head mechanical compliance. Arch Ophthalmol 1989;107(8):1232-4. (In eng). [CrossRef]
  11. Kimball EC, Nguyen C, Steinhart MR, et al. Experimental scleral cross-linking increases glaucoma damage in a mouse model. Exp Eye Res 2014;128:129-40. (In eng). [CrossRef]
  12. Luce, DA. Determining in vivo biomechanical properties of the cornea with an ocular response analyzer. J Cataract Refract Surg 2005;31(1):156-62. [CrossRef]
  13. Brandt, JD. Central corneal thickness, tonometry, and glaucoma risk--a guide for the perplexed. Can J Ophthalmol 2007;42(4):562-6. (https://www.ncbi.nlm.nih.gov/pubmed/17641698).
  14. Roberts, CJ. Concepts and misconceptions in corneal biomechanics. J Cataract Refract Surg 2014;40(6):862-9. [CrossRef]
  15. Roberts, CJ. Corneal hysteresis and beyond: Does it involve the sclera? Journal of Cataract & Refractive Surgery 2021;47(4):427-429. [CrossRef]
  16. Taroni L, Bernabei F, Pellegrini M, et al. Corneal Biomechanical Response Alteration After Scleral Buckling Surgery for Rhegmatogenous Retinal Detachment. Am J Ophthalmol 2020;217:49-54. [CrossRef]
  17. Kotecha, A. What biomechanical properties of the cornea are relevant for the clinician? Surv Ophthalmol 2007;52 Suppl 2:S109-14. [CrossRef]
  18. Hager A, Schroeder B, Sadeghi M, Grossherr M, Wiegand W. [The influence of corneal hysteresis and corneal resistance factor on the measurement of intraocular pressure]. Ophthalmologe 2007;104(6):484-9. [CrossRef]
  19. Zhang H, Sun Z, Li L, Sun R, Zhang H. Comparison of intraocular pressure measured by ocular response analyzer and Goldmann applanation tonometer after corneal refractive surgery: a systematic review and meta-analysis. BMC Ophthalmol 2020;20(1):23. [CrossRef]
  20. Nguyen BA, Roberts CJ, Reilly MA. Biomechanical Impact of the Sclera on Corneal Deformation Response to an Air-Puff: A Finite-Element Study. Front Bioeng Biotechnol 2018;6:210. (In eng). [CrossRef]
  21. Yuhas PT, Roberts CJ. Clinical Ocular Biomechanics: Where Are We after 20 Years of Progress? Curr Eye Res 2023;48(2):89-104. [CrossRef]
  22. Nguyen BA, Reilly MA, Roberts CJ. Biomechanical contribution of the sclera to dynamic corneal response in air-puff induced deformation in human donor eyes. Exp Eye Res 2020;191:107904. (In eng). [CrossRef]
  23. Metzler KM, Mahmoud AM, Liu J, Roberts CJ. Deformation response of paired donor corneas to an air puff: intact whole globe versus mounted corneoscleral rim. J Cataract Refract Surg 2014;40(6):888-96. [CrossRef]
  24. Ambrósio Jr RR, I. Luz, A. Faria, F. C. Andreas, S. Krug, M. Belin, M. W. Roberts, C. J. Dynamic ultra-high speed Scheimpflug imaging for assessing corneal biomechanical properties. Rev Bras Oftalmol 2013;72(2). [CrossRef]
  25. Roberts CJ, Mahmoud AM, Bons JP, et al. Introduction of Two Novel Stiffness Parameters and Interpretation of Air Puff-Induced Biomechanical Deformation Parameters With a Dynamic Scheimpflug Analyzer. J Refract Surg 2017;33(4):266-273. [CrossRef]
  26. Joda AA, Shervin MM, Kook D, Elsheikh A. Development and validation of a correction equation for Corvis tonometry. Comput Methods Biomech Biomed Engin 2016;19(9):943-53. [CrossRef]
  27. Vinciguerra R, Ambrosio R, Jr., Elsheikh A, et al. Detection of Keratoconus With a New Biomechanical Index. J Refract Surg 2016;32(12):803-810. [CrossRef]
  28. Ambrosio R, Jr. , Lopes BT, Faria-Correia F, et al. Integration of Scheimpflug-Based Corneal Tomography and Biomechanical Assessments for Enhancing Ectasia Detection. J Refract Surg 2017;33(7):434-443. [CrossRef]
  29. Sedaghat MR, Momeni-Moghaddam H, Ambrosio R, Jr., et al. Long-term Evaluation of Corneal Biomechanical Properties After Corneal Cross-linking for Keratoconus: A 4-Year Longitudinal Study. J Refract Surg 2018;34(12):849-856. [CrossRef]
  30. Eliasy A, Chen KJ, Vinciguerra R, et al. Determination of Corneal Biomechanical Behavior in-vivo for Healthy Eyes Using CorVis ST Tonometry: Stress-Strain Index. Front Bioeng Biotechnol 2019;7:105. [CrossRef]
  31. Fujishiro T, Matsuura M, Fujino Y, et al. The Relationship Between Corvis ST Tonometry Parameters and Ocular Response Analyzer Corneal Hysteresis. J Glaucoma 2020;29(6):479-484. [CrossRef]
  32. Wong BJ, Moghimi S, Zangwill LM, et al. Relationship of Corneal Hysteresis and Anterior Lamina Cribrosa Displacement in Glaucoma. Am J Ophthalmol 2020;212:134-143. [CrossRef]
  33. Congdon NG, Broman AT, Bandeen-Roche K, Grover D, Quigley HA. Central corneal thickness and corneal hysteresis associated with glaucoma damage. Am J Ophthalmol 2006;141(5):868-75. [CrossRef]
  34. De Moraes CV, Hill V, Tello C, Liebmann JM, Ritch R. Lower corneal hysteresis is associated with more rapid glaucomatous visual field progression. J Glaucoma 2012;21(4):209-13. [CrossRef]
  35. Susanna CN, Diniz-Filho A, Daga FB, et al. A Prospective Longitudinal Study to Investigate Corneal Hysteresis as a Risk Factor for Predicting Development of Glaucoma. Am J Ophthalmol 2018;187:148-152. [CrossRef]
  36. Agarwal DR, Ehrlich JR, Shimmyo M, Radcliffe NM. The relationship between corneal hysteresis and the magnitude of intraocular pressure reduction with topical prostaglandin therapy. Br J Ophthalmol 2012;96(2):254-7. [CrossRef]
  37. Hirneiss C, Sekura K, Brandlhuber U, Kampik A, Kernt M. Corneal biomechanics predict the outcome of selective laser trabeculoplasty in medically uncontrolled glaucoma. Graefes Arch Clin Exp Ophthalmol 2013;251(10):2383-8. [CrossRef]
  38. Sun L, Shen M, Wang J, et al. Recovery of corneal hysteresis after reduction of intraocular pressure in chronic primary angle-closure glaucoma. Am J Ophthalmol 2009;147(6):1061-6, 1066 e1-2. [CrossRef]
  39. Kotecha A, Elsheikh A, Roberts CR, Zhu H, Garway-Heath DF. Corneal thickness- and age-related biomechanical properties of the cornea measured with the ocular response analyzer. Invest Ophthalmol Vis Sci 2006;47(12):5337-47. [CrossRef]
  40. Brandt JD, Gordon MO, Beiser JA, et al. Changes in central corneal thickness over time: the ocular hypertension treatment study. Ophthalmology 2008;115(9):1550-6, 1556 e1. [CrossRef]
  41. Sullivan-Mee M, Katiyar S, Pensyl D, Halverson KD, Qualls C. Relative importance of factors affecting corneal hysteresis measurement. Optom Vis Sci 2012;89(5):E803-11. [CrossRef]
  42. Silva JASd, Silva RSd, Jr RA. Relevância da biomecânica da córnea no glaucoma. Revista Brasileira de Oftalmologia;73(1):37-39. [CrossRef]
  43. Sigal IA, Flanagan JG, Ethier CR. Factors influencing optic nerve head biomechanics. Invest Ophthalmol Vis Sci 2005;46(11):4189-99. [CrossRef]
  44. Sigal IA, Yang H, Roberts MD, et al. IOP-induced lamina cribrosa deformation and scleral canal expansion: independent or related? Invest Ophthalmol Vis Sci 2011;52(12):9023-32. [CrossRef]
  45. Coudrillier B, Campbell IC, Read AT, et al. Effects of Peripapillary Scleral Stiffening on the Deformation of the Lamina Cribrosa. Invest Ophthalmol Vis Sci 2016;57(6):2666-77. [CrossRef]
  46. Musch DC, Gillespie BW, Niziol LM, Lichter PR, Varma R, Group CS. Intraocular pressure control and long-term visual field loss in the Collaborative Initial Glaucoma Treatment Study. Ophthalmology 2011;118(9):1766-73. [CrossRef]
  47. Nouri-Mahdavi K, Hoffman D, Coleman AL, et al. Predictive factors for glaucomatous visual field progression in the Advanced Glaucoma Intervention Study. Ophthalmology 2004;111(9):1627-35. [CrossRef]
  48. Vinciguerra R, Rehman S, Vallabh NA, et al. Corneal biomechanics and biomechanically corrected intraocular pressure in primary open-angle glaucoma, ocular hypertension and controls. Br J Ophthalmol 2020;104(1):121-126. [CrossRef]
  49. Anand A, De Moraes CG, Teng CC, Tello C, Liebmann JM, Ritch R. Corneal hysteresis and visual field asymmetry in open angle glaucoma. Invest Ophthalmol Vis Sci 2010;51(12):6514-8. [CrossRef]
  50. Helmy H, Leila M, Zaki AA. Corneal biomechanics in asymmetrical normal-tension glaucoma. Clin Ophthalmol 2016;10:503-10. [CrossRef]
  51. Miki A, Yasukura Y, Weinreb RN, et al. Dynamic Scheimpflug Ocular Biomechanical Parameters in Untreated Primary Open Angle Glaucoma Eyes. Invest Ophthalmol Vis Sci 2020;61(4):19. [CrossRef]
  52. Pradhan ZS, Deshmukh S, Dixit S, et al. A comparison of the corneal biomechanics in pseudoexfoliation glaucoma, primary open-angle glaucoma and healthy controls using Corvis ST. PLoS One 2020;15(10):e0241296. [CrossRef]
  53. Silva N, Ferreira A, Baptista PM, et al. Corneal Biomechanics for Ocular Hypertension, Primary Open-Angle Glaucoma, and Amyloidotic Glaucoma: A Comparative Study by Corvis ST. Clin Ophthalmol 2022;16:71-83. [CrossRef]
  54. Schlotzer-Schrehardt U, Zenkel M, Nusing RM. Expression and localization of FP and EP prostanoid receptor subtypes in human ocular tissues. Invest Ophthalmol Vis Sci 2002;43(5):1475-87. (https://www.ncbi.nlm.nih.gov/pubmed/11980863).
  55. Sharif NA, Kelly CR, Crider JY, Williams GW, Xu SX. Ocular hypotensive FP prostaglandin (PG) analogs: PG receptor subtype binding affinities and selectivities, and agonist potencies at FP and other PG receptors in cultured cells. J Ocul Pharmacol Ther 2003;19(6):501-15. [CrossRef]
  56. Shen SR, Fleming GP, Jain SG, Roberts CJ. A Review of Corneal Biomechanics and Scleral Stiffness in Topical Prostaglandin Analog Therapy for Glaucoma. Curr Eye Res 2023;48(2):172-181. [CrossRef]
  57. Weinreb RN, Toris CB, Gabelt BT, Lindsey JD, Kaufman PL. Effects of prostaglandins on the aqueous humor outflow pathways. Surv Ophthalmol 2002;47 Suppl 1:S53-64. [CrossRef]
  58. Kim JW, Lindsey JD, Wang N, Weinreb RN. Increased human scleral permeability with prostaglandin exposure. Invest Ophthalmol Vis Sci 2001;42(7):1514-21. (https://www.ncbi.nlm.nih.gov/pubmed/11381055).
  59. Lindsey JD, Crowston JG, Tran A, Morris C, Weinreb RN. Direct matrix metalloproteinase enhancement of transscleral permeability. Invest Ophthalmol Vis Sci 2007;48(2):752-5. [CrossRef]
  60. Scott JA, Roberts CJ, Mahmoud AM, Jain SG. Evaluating the Relationship of Intraocular Pressure and Anterior Chamber Volume With Use of Prostaglandin Analogues. J Glaucoma 2021;30(5):421-427. [CrossRef]
  61. Aoki S, Murata H, Matsuura M, et al. The Relationship between the Waveform Parameters from the Ocular Response Analyzer and the Progression of Glaucoma. Ophthalmol Glaucoma 2018;1(2):123-131. [CrossRef]
  62. Faria-Correia F, Ramos I, Valbon B, Luz A, Roberts CJ, Ambrosio R, Jr. Scheimpflug-based tomography and biomechanical assessment in pressure-induced stromal keratopathy. J Refract Surg 2013;29(5):356-8. [CrossRef]
  63. Goldich Y, Marcovich AL, Barkana Y, et al. Clinical and corneal biomechanical changes after collagen cross-linking with riboflavin and UV irradiation in patients with progressive keratoconus: results after 2 years of follow-up. Cornea 2012;31(6):609-14. [CrossRef]
Preprints 68751 g001
Figure 1. Ocular Response Analyzer tonometer (Image from Reichert Ophthalmic Instruments, Inc., Buffalo, NY, USA).
Figure 1. Ocular Response Analyzer tonometer (Image from Reichert Ophthalmic Instruments, Inc., Buffalo, NY, USA).
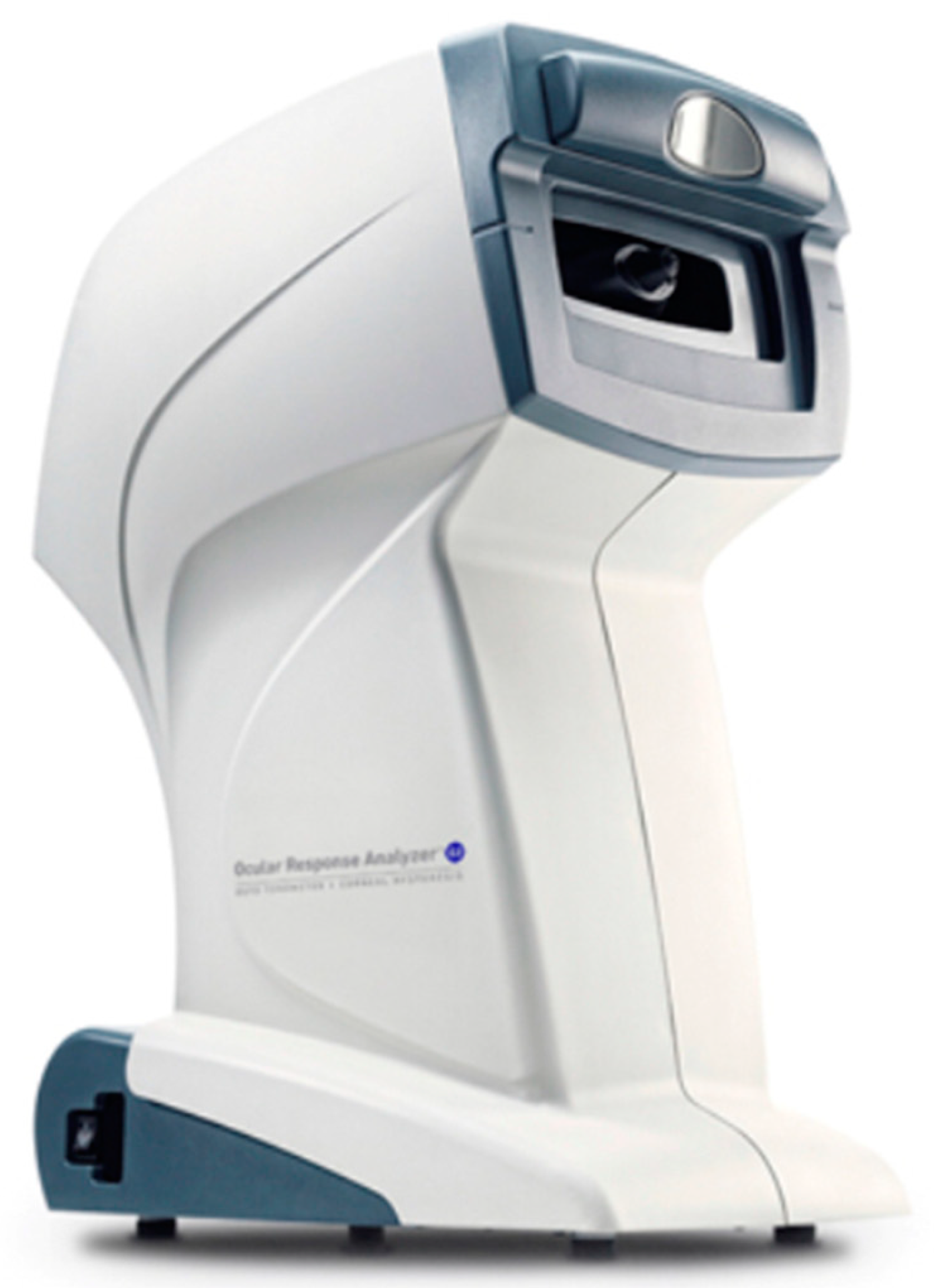
Preprints 68751 g001
Preprints 68751 g002
Figure 2. ORA measurements show the air pulse deforming the cornea. The parameters generated are corneal Hysteresis (CH) and corneal resistance factor (CRF). Image from Kaushik S, et al. Pandav SS. Ocular Response Analyzer. J Curr Glaucoma Pract. 2012 Jan-Apr;6(1):17-19.
Figure 2. ORA measurements show the air pulse deforming the cornea. The parameters generated are corneal Hysteresis (CH) and corneal resistance factor (CRF). Image from Kaushik S, et al. Pandav SS. Ocular Response Analyzer. J Curr Glaucoma Pract. 2012 Jan-Apr;6(1):17-19.
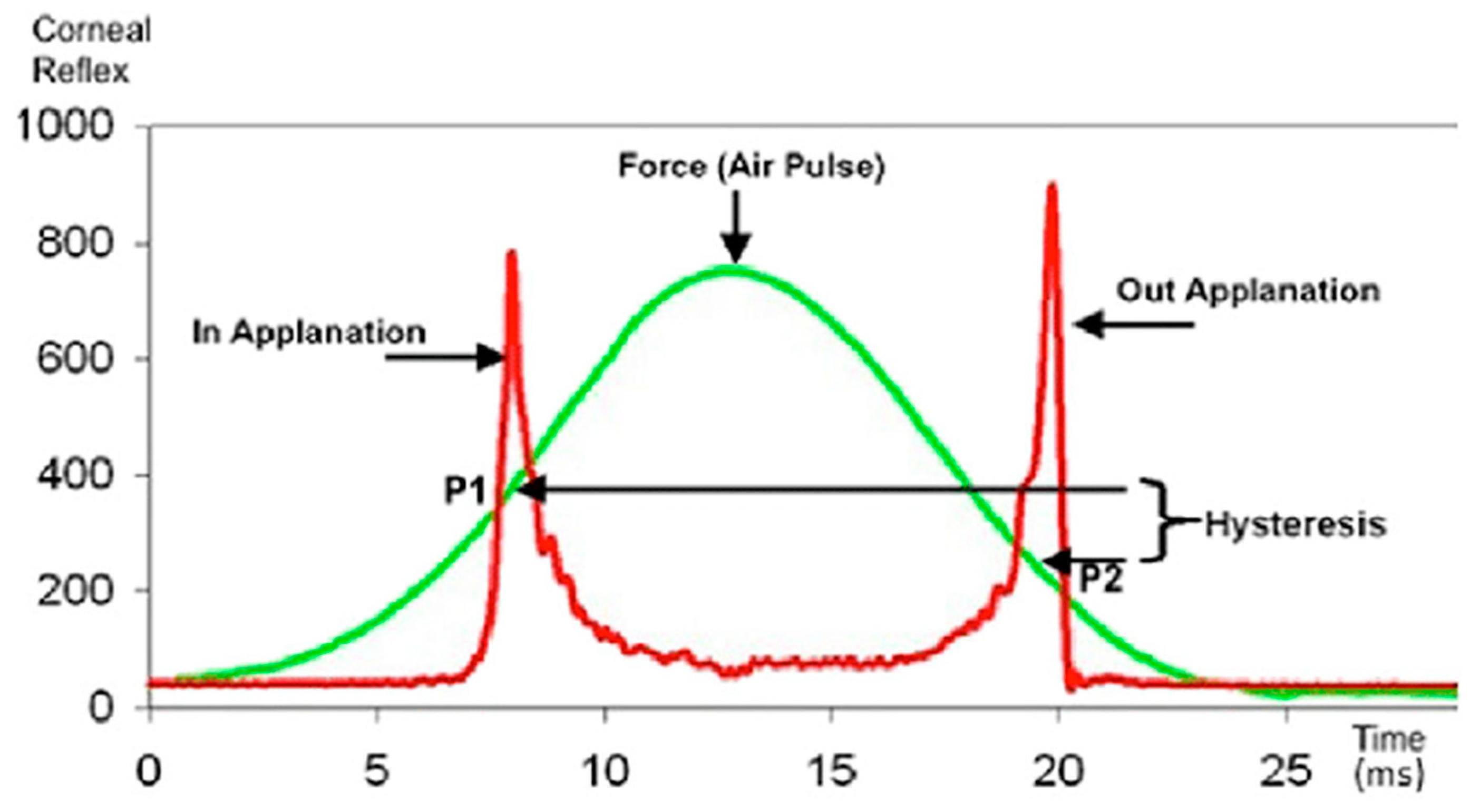
Preprints 68751 g002
Preprints 68751 g003
Figure 3. Oculus Corvis ST (Wetzlar,Germany).
Figure 3. Oculus Corvis ST (Wetzlar,Germany).
Preprints 68751 g003
Preprints 68751 g004
Figure 4. Dynamic ultra-high-speed Scheimpflug imaging for assessing corneal biomechanical response and properties. The highest point concavity (HC) is shown in the image’s lower right corner. Personal archive.
Figure 4. Dynamic ultra-high-speed Scheimpflug imaging for assessing corneal biomechanical response and properties. The highest point concavity (HC) is shown in the image’s lower right corner. Personal archive.
Preprints 68751 g004
Preprints 68751 g005
Figure 5. The Vinciguerra screening report shows the adjusted biomechanically intraocular pressure (bIOP), the Ambrósio Relational Thickness over the horizontal meridian(ARTh), and The Corvis Biomechanical Index(CBI). Personal archive.
Figure 5. The Vinciguerra screening report shows the adjusted biomechanically intraocular pressure (bIOP), the Ambrósio Relational Thickness over the horizontal meridian(ARTh), and The Corvis Biomechanical Index(CBI). Personal archive.
Preprints 68751 g005
Preprints 68751 g006
Figure 6. The ARV (Ambrosio, Roberts, and Vinciguerra) biomechanical and tomographic assessment shows the Tomographic Biomechanical Index (TBI) and the Corvis Biomechanical Index (CBI). Personal archive.
Figure 6. The ARV (Ambrosio, Roberts, and Vinciguerra) biomechanical and tomographic assessment shows the Tomographic Biomechanical Index (TBI) and the Corvis Biomechanical Index (CBI). Personal archive.
Preprints 68751 g006
Table 1. Corneal deformation parameters provided by the Corvis ST.
Table 1. Corneal deformation parameters provided by the Corvis ST.
Corvis ST parameter Definition
1st Applanation The first applanation of the cornea during the air puff (in ms). The length of the applanation at this moment appears in parenthesis (in mm).
Highest Concavity The instant that the cornea assumes its maximum concavity during the air puff (in ms). The length of the distance between the two peaks of the cornea at this moment appears in parenthesis (in mm).
2nd Applanation The second applanation of the cornea during the air puff (in milliseconds). The length of the applanation at this moment appears in parenthesis (in mm).
Maximum Deformation The amount (in mm) of the maximum cornea deformation during the air puff
Wing Distance The length of the distance between the two peaks of the cornea at this instant (in mm)
Maximum Velocity (in) The maximum velocity during the ingoing phase (in m/s)
Maximum Velocity The maximum velocity during the outgoing phase (in m/s)
Curvature Radius Normal The cornea in its natural state radius of curvature (in mm)
Curvature Radius HC The cornea radius of curvature at the time of maximum concavity during the air puff (in mm)
Cornea Thickness Measurement of the corneal thickness (in mm)
IOP Measurement of the intraocular pressure (in mmHg)
bIOP Biomechanically-corrected IOP
DA ratio Max (Deformation amplitude ratiomax 2mm) Ratio between the deformation amplitude at the apex and the average deformation amplitude measured at 2 mm from the center
ARth (Ambrósio’s relational thickness to the horizontal profile) Describes thickness profile in the temporal-nasal direction and defined as corneal thickness thinnest to pachymetric progression
SP-A1(Stiffness parameter at A1) Describes corneal stiffness as defined by resultant pressure (Pr) divided by deflection amplitude at A1
SP-HC Corneal stiffness at the highest concavity point
TBI (Tomographic biomechanical index) Index that combined tomographic and biomechanical data for keratoconus detection
BGF (Biomechanical Glaucoma factor) Independent risk indicator for normal tension glaucoma
SSI (Stress-strain index) Index that indicates the position of the stress-strain curves. Less dependent on corneal thickness and IOP.
CBI (Corvis biomechanical index Overall biomechanical index for keratoconus detection
Whole eye movement (WEM) The entire globe's movement after the cornea passes its limits during the jet air pulse resisted by the orbital structures.
Deformation Amplitude (DA) The movement of the corneal deformation from apex to highest concavity
Deflection amplitude DeflA The difference between The DA and the WEM
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content.
Copyright: This open access article is published under a Creative Commons CC BY 4.0 license, which permit the free download, distribution, and reuse, provided that the author and preprint are cited in any reuse.
Prerpints.org logo

Preprints.org is a free preprint server supported by MDPI in Basel, Switzerland.

facebook logotwitter logolinkedin logo
MDPI Initiatives
Important Links
Subscribe

Disclaimer

Terms of Use

Privacy Policy

© 2024 MDPI (Basel, Switzerland) unless otherwise stated