Application of Active Disturbance Rejection in a Bearingless Machine with Split Winding

Preprint

Article

Application of Active Disturbance Rejection in a Bearingless Machine with Split Winding

Altmetrics

Downloads

136

Views

Comments

Rodrigo de Andrade Teixeira,Werbet Luiz Almeida da Silva,Adson Emanuel Santos Amaral,Walter Martins Rodrigues,

Andres Ortiz Salazar

Elmer Rolando Llanos Villarreal^*

Rodrigo de Andrade Teixeira,Werbet Luiz Almeida da Silva,Adson Emanuel Santos Amaral,Walter Martins Rodrigues,

Andres Ortiz Salazar

Elmer Rolando Llanos Villarreal^*

This version is not peer-reviewed

Submitted:

28 February 2023

Posted:

02 March 2023

You are already at the latest version

Alerts

Abstract

This paper has the objective of implementing the radial rotor position control of a three-phase bearingless induction machine with split winding and optimized drive structure, using the ADRC technique. Because it is a multivariable, nonlinear, time-varying system with coupled variables, it is necessary to use advanced control strategies in order for the system to operate efficiently and with good dynamic performance. The ADRC controller considers the total disturbance composed of unmodeled dynamics, nonlinearities, uncertainties, and load variations, as a new system state, to be estimated in real-time through an extended state observer. In this mode, disturbances are compensated in real-time, eliminating regime errors and with good response to disturbances in general.

Keywords:

Subject: Engineering - Electrical and Electronic Engineering

1. Introduction

Magnetically suspended induction machines meet a specific industry demand for less noisy motors that can reach higher speeds and do not cause product contamination in situations where the machine directly handles the process. The induction motor is a multivariable, nonlinear system [1] that has a strong coupling between variables. When used in bearingless systems, the degree of control complexity is even greater, especially when placed in situations with shaft load application. In these cases, linear controllers may not achieve a sufficiently fast and stable response for adequate suspension and control of the other system parameters. The emergence of microprocessor devices and power electronics has provided conditions for the application of advanced control theories such as predictive controllers, sliding mode controllers, neural and artificial network-based controllers, Fuzzy controllers, and others [2]. In recent years, the ADRC Control technique has been used for the control of various types of systems. ADRC Control is a controller originally based on nonlinear functions, composed of three main blocks: Tracking Differentiator (TD), Extended State Observer (ESO), and a block corresponding to the control law that has received different contributions over the years. This work investigated the application of ADRC controllers to the position control of a split-winding induction machine, aiming to understand the limitations of applying this type of controller to the motor-bearing system and to explore the benefits that a technique with active disturbance rejection can bring to radial position control. In [3], [4] investigates the application of the Active Disturbance Rejection Control (ADRC) technique on the stabilization and control of the rotor radial position of a bearingless induction machine with split winding.

2. Split Winding Bearingless Motor

The first split-winding bearing motor was proposed by Salazar & Stephan [5]. They built a 4-pole two-phase motor that used the motor’s torque coils for radial force generation. In this topology, only one of the phases was responsible for radial position control and both phases were used for torque generation. The two-phase motor proposed by [5] was the starting point for the work of [6], who proposed a three-phase motor in which the coils of each phase were divided into two. In this topology, the three phases were used in the generation of radial force and torque. In [7] Paiva’s work, the speed vector control of the three-phase motor-mancal was done using neural networks for rotor flux estimation. In this work, the performance of the machine operating with a conventional state estimator and with a neural state estimator was compared. Valci in [8] analyzed the feasibility of using a conventional induction machine as a split-winding bearingless motor. He performed position and current control of the machine, however, he observed that radial position control was not satisfactory for speeds below rated. In [9] studied the replacement of PID controllers for radial position control with controllers based on Fuzzy logic. Noting the strong nonlinear and parameter-varying characteristics of induction motor bearings, he analyzed the contribution of Fuzzy controllers on transient and permanent regime performance. In order to reduce the number of equipment needed for machine control in [10] optimized the structure of the motor bearing by proposing a new way to connect the coils in the machine stator. In [10] investigated the spatial distribution of the machine’s coils and realized that it was possible to generate the radial position control components using only two phases. In this topology, the coils of one of the machine phases are connected directly to the center point of the inverter’s DC bus. In this way, only two phases are controlled and the drive structure is optimized.

In this paper, the ADRC control technique is applied with two different structures to the control of a bearingless induction machine, the first structure uses linear estimation while the second uses nonlinear estimation. The results obtained show the dynamic behavior of the system output and states for the two versions.

2.1. Operation Principle

In the bearingless split winding induction machine, the electric currents in the stator and rotor coils generate magnetic fields that interact with each other. As a result of this interaction, forces arise that act on the rotor promoting its rotation and radial positioning. In the stator of the three-phase bearing motor, there are three divided groups of windings, each phase being displaced by

120^{o}

relative to the others. With this distribution, it is possible to control the radial positioning of the rotor in all directions from the composition of the radial forces generated. Figure 1 shows the position of the windings relative to the X and Y axes.

In the optimization proposal by [10] the coil connection arrangement was modified to the structure shown in Figure 2. This modification reduced the number of control and drive components for the currents in each phase.

Considering the currents

I_{b}

and

I_{c}

are given respectively by:

\begin{matrix} I_{b} = I_{m} c o s (ω t - \frac{2 π}{3}) \\ I_{c} = I_{m} c o s (ω t + \frac{2 π}{3}) \end{matrix}

(1)

The sum of these currents, for the circuit in Figure 2 results in:

\begin{matrix} I_{b} + I_{c} = I_{m} c o s (ω t) \end{matrix}

(2)

Since

I_{b} + I_{c} = - I_{a}

, it can be seen that for the modification performed by [10], the characteristics of the coil currents of phase A have been preserved.

To illustrate the behavior of the currents per phase in the split-wound three-phase induction machine driven by a 4-arm inverter. Figure 3 shows the waveforms of the currents in each phase for the situation where the rotor is centered on the air gap. When the rotor is centered the modulation components are zero, therefore the phase currents have the same modulus

I_{a} = I_{b} = I_{c}

When unbalance occurs in one of the modulation components or disturbances that tend to move the rotor radially, the phase currents are altered by the control system in order to generate radial forces that bring the rotor back to the center of the stator.

Figure 4 shows the behavior of the currents during radial force generation to the right. To move the rotor shaft in the indicated direction, the control system must increase the currents of coils

B_{1}

and

C_{2}

, and reduce the currents of coils

B_{2}

and

C_{1}

3. ADRC Control

Modern control theory is focused on the time domain and can be applied to systems with multiple inputs and outputs, linear and nonlinear systems, and time-varying and time-invariant systems. One of the difficulties of modern state feedback control systems is that some variables are not accessible by measurement. In this sense, the concept of state observer becomes very useful to reconstruct non-measurable state variables from measurable variables. The Active Disturbance Rejection Control technique uses state observers to estimate in real-time the disturbances involved in the controlled process and then uses this information to obtain a good disturbance rejection capability [11]. In [12] ADRC control was proposed as a two-degree-of-freedom controller, with an inner loop for state estimation and disturbance rejection and an outer loop for controlling the process output dynamics. This structure is implemented based on three blocks, a reference generator, responsible for generating a smoothed version of the input signal and its derivatives, an estimator, responsible for estimating the process states and all the dynamics that differentiate it from a simple integrator system, and the control law, which subtracts from the original control the dynamics estimated by the observer. Figure 5 presents the general idea of the operating principle of an ADRC controller.

Considering a second-order process with representation in the state of states given by

\begin{matrix} \ddot{y} & = & - a \dot{y} - b y + w + b u \end{matrix}

(3)

y is the output; u is the input; w represents an external disturbance; a and b are system parameters. An approximate value of the disturbance b is the information needed for the ADRC implementation. Considering that there is no measurement available it is possible to approximate its value according to (3)

\begin{matrix} \ddot{y} = - a \dot{y} - b y + w + (b - b_{0}) u + b_{0} u = f + b_{0} u \end{matrix}

(4)

The term f in expression (4) is called the generalized disturbance and its value is given by

f = - a \dot{y} - b y + w + (b - b_{0}) u

. The generalized disturbance is capable of encompassing a wide range of uncertainties and effects, such as: combined external and time-varying disturbances, unmodeled internal dynamics, nonlinearities, and internal parameter variations.

Assuming

x_{1} = y

and

x_{2} = {\dot{x}}_{1}

, the second-order system of equation (4) can be represented in state space by:

\begin{matrix} {\dot{x}}_{1} & = & x_{2} \\ {\dot{x}}_{2} & = & f + b_{0} u \\ y & = & x_{1} \end{matrix}

(5)

If the generalized disturbance f can be estimated, its effect can be removed from the dynamics of the controlled process using (6).

\begin{matrix} u & = & \frac{u_{0} - f}{b_{0}} \end{matrix}

(6)

The ADRC control of the process represented in (4) is shown in Figure 6.

The generalized disturbance is capable of encompassing a wide range of uncertainties and effects, such as: external and time-varying disturbances, unmodeled internal dynamics, nonlinearities, and internal parameter variations. ADRC groups these uncertainties and effects and uses this information to remove the effects of this information from the process control.

In [13] a linear structure for ADRC applied to second-order systems was proposed. The block diagram in Figure 7 shows the application of linear ADRC to a second-order

G_{p}

system.

The output y is controlled by a LADRC from the application of input r. The states estimated by the linear extended state observer (LESO), are given by

z_{1}, z_{2}

, and

z_{3}

. The parameter

b_{0}

is a tuning parameter. The external disturbances are represented by: d (input disturbance) and n (measurement noise).

Keeping fixed the linear ADRC structure proposed in [13] this paper analyzed the application of two extended state observer structures. The first structure uses a linear observer with saturator at the output as shown in Figure 8. This structure mitigates the effects arising from the high error values between the estimated states and their actual values. The second structure uses nonlinear extended state observer as shown in Figure 9. This structure makes it possible to observe the influence of adding the nonlinearities on the dynamic performance of the system.

4. Description of the System

4.1. System Overview

Figure 10 provides an overview of the bench. The equipment has been grouped into the following blocks: monitoring and control, drive, and bearingless machine.

The bearingless motor block consists of the bearingless electric induction machine on which the tests were performed. The drive block comprises the entire infrastructure of electronic components and equipment responsible for providing the power supply at the appropriate levels to control the machine variables. The monitoring and control block consists of the electronic circuits, processors, and monitoring devices that were necessary to implement the system’s control and supervision routines. The variable

i_{a b x y}

represents the four-phase electrical connections that allow the drive block to supply controlled electrical currents to the bearing motor. The figure also indicates the reading of these

i_{a b x y}

currents performed by the monitoring and control block. The variable

Δ_{x y}

represents the signals corresponding to the radial positions of the x and y axes of the bearing motor read by the monitoring and control block. Finally, the orange arrow named PWM indicates the signals sent by the monitoring and control block to the drive block.

Figure 11 shows a real image of the experimental bench. This structure is located in the Actuation and Control Systems Laboratory in the Computer and Automation Engineering Department at UFRN.

4.2. Split-Winding Bearingless Machine

The prototype used works vertically with the goal of simplifying radial position control. The machine’s rotor is supported in the axial position by a mechanical bearing that is also responsible for fixing the machine’s lower end. Figure 12 shows the prototype.

The electrical data of the machine is detailed in Table 1.

4.3. Sensors

Two types of sensors are used on the bench: displacement sensors and current sensors. The displacement sensors detect the variation of the radial position of the machine shaft and the current sensors detect the phase currents reaching the machine.

4.4. DSP TMS320F28335

The DSP

T M S 320 F 28335

is a digital signal controller that has characteristics of a conventional processor because it contains in its structure circuits capable of executing programs stored in memories and also has additional circuits for digital signal processing, which includes hardware with special architecture and instructions for mathematical calculations and are able to perform real-time operations. In addition, the

T M S 320 F 28335

has in its structure memory and programmable input and output components, which makes the

T M S 320 F 28335

also a microcontroller.

4.5. Control Algorithm Implementation

The block diagram in Figure 13 shows the blocks needed for the practical experiments with the system. With the exception of the inverter block, all other blocks were implemented in C language in the DSP programming interface.

The general idea of the control algorithm is the existence of two cascaded control loops: the current control loop, with fast dynamic response and with the objective of making the currents in the controlled phases follow the specified reference; and the position control loop, which has slower dynamics than the current control loop and operates in a more external way, generating the references for the current control loop. The position control loop aims to maintain the radial position at the reference values

Y_{r e f}

and

X_{r e f}

, for this, the position control signals are added to the magnetization currents

I_{m}

to generate the necessary currents for the positioning of the rotor radial. The bias current block is responsible for generating via software the magnetizing currents of the machine. This block implements equations 1 and its arguments are the angular velocity and the peak value of the magnetizing currents. In the control algorithm, there are two ADRC blocks, one for each radial positioning axis of the machine. These blocks implement all the blocks required in the structure of the ADRC technique. These blocks receive the error calculated from subtracting the radial positions of the rotor by its references

Y_{r e f}

and

X_{r e f}

and perform the necessary operations to generate the correction signal

Δ_{i} y

and

Δ_{i} x

. The rotational transformation block converts the control signals calculated in the x-y positioning referential to b-c corresponding to the spatial alignment of the controlled motor-mani fold coils. The function of the modulation block is to perform the composition of the rotation currents

I_{b}

and

I_{c}

with the control signals

Δ_{i} b

and

Δ_{i c}

and thus generate the references

I_{b 1}^{*}, I_{b 2}^{*}, I_{c 1}^{*}

and

I_{c 2}^{*}

. Finally, the current control block implements four digital PI controllers, which monitor the machine currents and generate the PWM control signals for driving the inverters.

5. Experiments and Results

Two versions of ADRC controllers have been implemented in the

T M S 320 F 28335

DSP. The first one used the linear form proposed by Gao (2003) for the extended observer and the PD controller. The other version used fal-type nonlinearities in the observer structure. The results in three dimensions for the two implemented controllers can be seen in Figure 14a and 14b.

Figure 14a shows the behavior of positions X and Y in steady state for the first version of the controller. A mean error of

- 1.17 %

with a standard deviation of

0.03

mm was obtained for the X axis. As for the Y-axis, an average error of

0.76 %

with a standard deviation of

0.05

mm was obtained. Figure 14b shows the behavior of the X and Y positions in a steady state for the version with nonlinearities. The mean error for the X-axis was

- 0.09 %

with a standard deviation of

0.07

mm. For the Y axis, the mean error was

- 0.05 %

with a standard deviation also of

0.05

mm. The results indicate the effect of adding non-linearity on the observer’s structure. It was possible to improve the regime error with the non-linear versions, but the variation around the reference became more evident, indicating an increase in the aggressiveness of the controller response.

Figure 15a,b shows the behavior of the radial position signals when there are step-type reference variations in the X-axis only, keeping the Y- axis reference fixed, and Figure 15c,d when step-type variations occur in Y, keeping the X-axis reference is fixed.

The results in Figure 15 show that step variations in one of the axes do not result in a significant influence on the dynamic behavior of the opposite axis. Variations in the axes were also performed for the controller with a non-linear observer. The results also indicate that there is a decoupling in the dynamic behavior of the radial position between the two axes.

The same test was performed to the controller with nonlinear observer. The results are showed in Figure 16.

The addition of a radial load to the machine shaft in operation is an important analysis since this load is seen by the position controllers as an external disturbance in the form of a step. Therefore, it is a simple way to impose a perturbation and analyze the robustness of the controller in situations like this. For this analysis, an apparatus that allowed the addition of a radial load aligned with the X-axis was used. Figure 17 illustrates how the device was used to add the radial disturbance.

Figure 15. Response of radial position control under step setpoint changes for Linear ADRC.

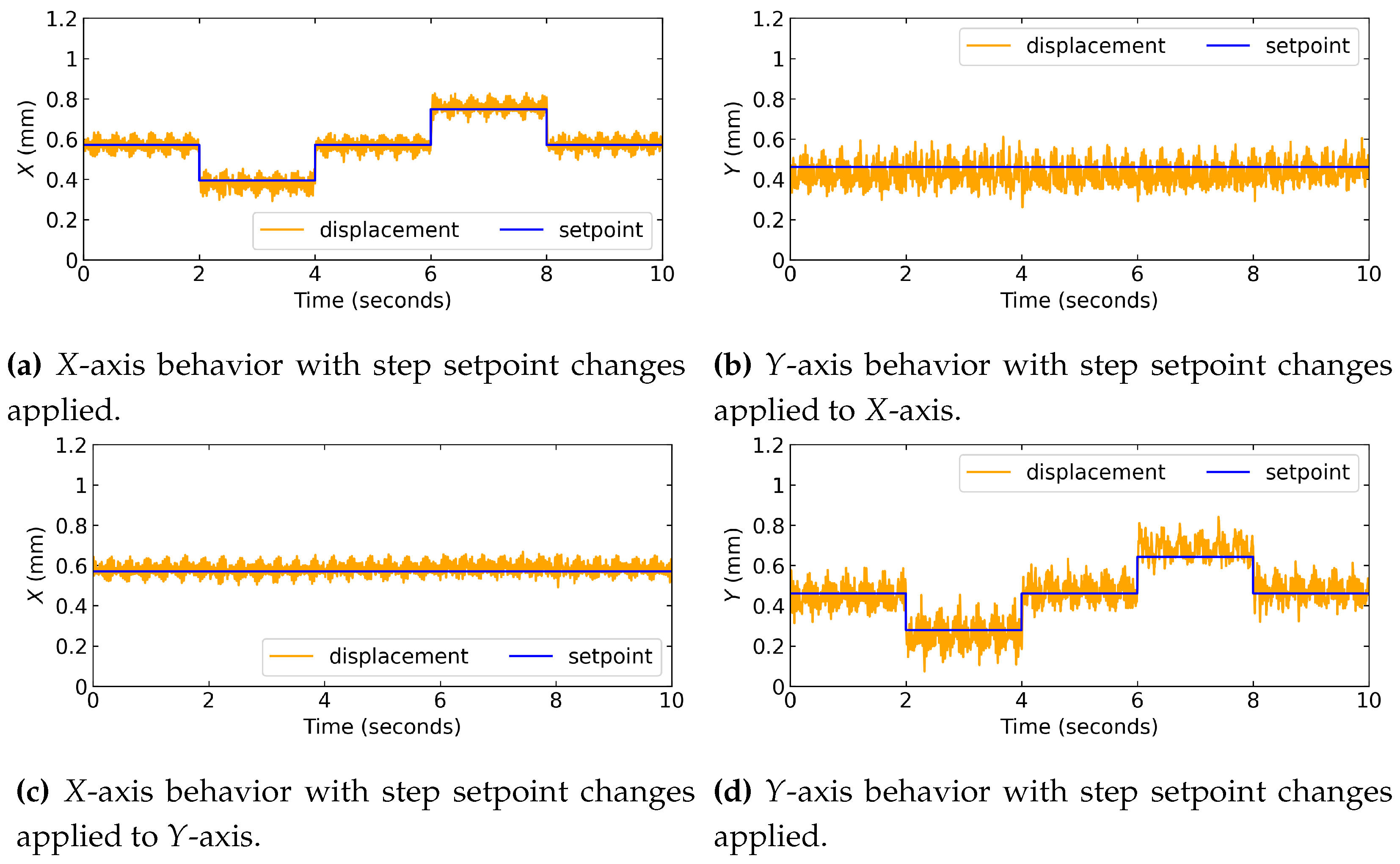
Figure 17. Illustrative image of the machine subjected to load addition.

For each controller, the

z_{1}

z_{2}

, and

z_{3}

states of the X and Y axes were collected in a time interval that allowed detecting the exact instant of addition of the radial load to the machine axis. Figure 18 and Figure 19 show the behavior of the states of the X and Y axes for the four implemented controllers. In each graph, a green dashed vertical line was inserted to indicate the instant the application of 150 grams of radial load aligned to the X-axis. This load was inserted so that the radial effort was applied in the positive direction of the axis. Figure 18a,b show the state behavior of the linear controller. Visually, it is possible to state that there was a certain degree of rejection of the radial disturbance, but it is evident that the regime error of the X-axis increased after the moment of application of the load. The Y-axis remained stable. It is not possible to perceive variations in the

z_{3}

state on the scale used because the linear controller was implemented with saturators at the observers’ output in order to avoid the Peak phenomenon.

Figure 16. Response of radial position control under step setpoint changes for Nonlinear ADRC.

Figure 18. Estimated states behavior for Linear ADRC under radial load application.

Through the analysis of the

z_{1}

states of Figure 19a,b it is possible to conclude that the ADRC controller with NESO was less influenced by the application of the radial load in the steady state since qualitatively the response was very similar for the intervals before and after the addition of the disturbance. The behavior of the states indicated an aggressive reaction of the controller in the transient regime, resulting in high values of

z_{2}

during this region of the collection. The change in the mean value observed for the

z_{3}

state indicates that this variable was able to identify the addition of the external disturbance. the good rejection of the observed perturbation may be a result of this. Quantitatively, the results of Figure 18a,b and Figure 19a,b can be summarized according to Table 2. Table 2 shows the average values in a steady state of the observed states.

Figure 19. Estimated states behavior for Nolinear ADRC under radial load application.

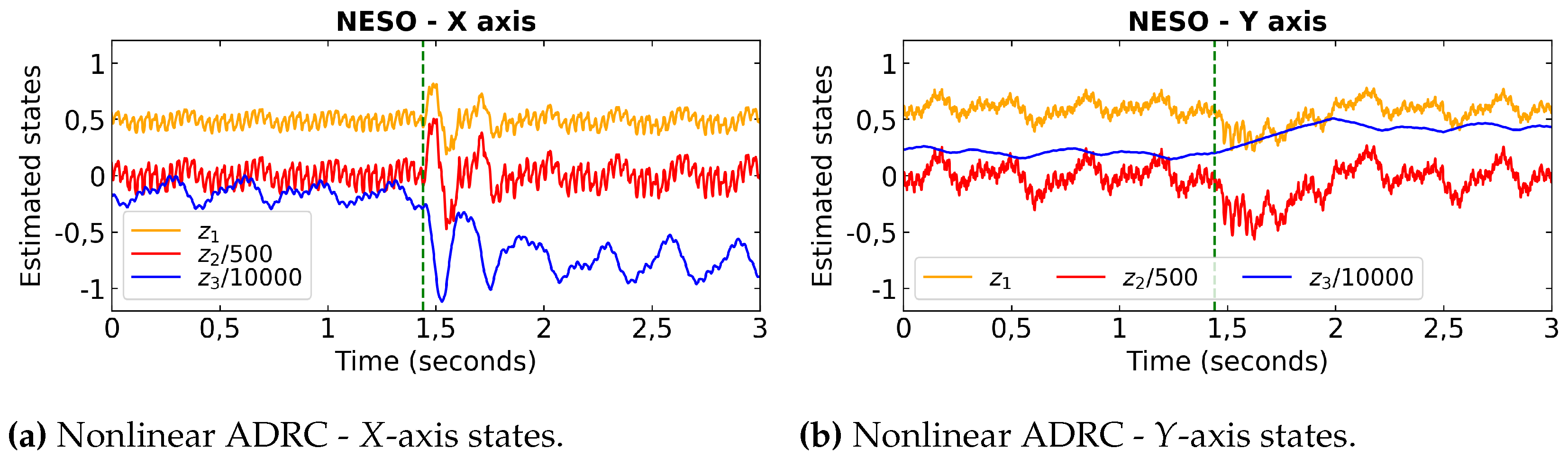
The value of the

z_{1}

state for the linear controller indicates that there was a displacement of the mean value of the output after applying the load. This is a limitation of the linear observer as its states need to read limited in amplitude to avoid peak phenomenon and guarantee stability. As indicated by the value of

z_{3}

after applying the load, it was blocked in the imposed saturation. The saturation of state outputs impairs the ability to reject disturbances. The mean value of the

z_{1}

state of the non-linear indicates that it was able to reject the load addition. Furthermore, the significant change in the value of

z_{3}

shows that it was able to identify the disturbance.

The value of

z_{3}

in Table 2 becomes more negative after adding the load because the control loop error is given by:

\begin{matrix} E r r o r = p o s - r e f \end{matrix}

(7)

that is, when the radial position is displaced from the reference value in the sense of increasing the error value, the control signal must increase in order to restore the equilibrium position close to the reference. Knowing that the control signal is calculated by:

\begin{matrix} u & = & \frac{u_{0} - z_{3}}{b_{0}} \end{matrix}

(8)

it means that the more negative the value of

z_{3}

, the greater the values of the control signals and the greater the control effort in the sense of rejecting the action of the disturbance in the system output. The more positive the displacement value is in relation to the reference, the greater the control signal must be.

Finally, to analyze the relationship between the value of

z_{3}

and the intensity of the radial disturbance applied to the motor shaft and also to verify to what extent the system could reject the radial load, masses of 32 grams were added one by one in the application support of the load. This test was performed with the NESO-based controller only. In the first step, the radial load support was aligned on the X-axis and then on the Y-axis. For each added mass, the regime errors of the X and Y positions were calculated and the

z_{3}

states were collected. The results are shown in Figure 20a,b and Figure 21a,b. Figure 20a,b show the results for the radial load bearing aligned with the X-axis.

Figure 20a shows that the regime error remained close to zero for loads of up to 224 grams. When the radial load increased to 256 grams, the position control in the X-axis became unstable but remained stable on the Y-axis. Figure 20b shows the estimation of generalized disturbances for the X and Y axes increase in load and the

z_{3}

state of the X-axis. This variable becomes more negative as the load increases, as expected. Figure 20b also shows that the load added in the X direction also influenced the

z_{3}

state of the Y axis. This indicates that the load support was not perfectly aligned with the desired axis, or that the X and Y sensors could not have been perfectly spaced 90 degrees apart. Figure 21a and 21b show the same analysis, this time for the radial load added in the positive direction of Y. The Y axis showed a lower radial disturbance rejection capacity since it only managed to keep the regime error close to zero up to 160 grams of load. The Y-axis control became unstable for the 192-gram load, while the X-axis remained stable for all analyzed loads. Figure 21b indicates that there is also an approximately linear relationship between the value of

z_{3}

and the value of the added charge. Likewise, there was a smaller scale detection of radial disturbance by the

z_{3}

state of the X-axis. In this test, the influence on the cross-axis was greater. The results of the load curve for both axes showed that there is a linear relationship between the perturbation and the

z_{3}

state. This relationship is fundamental to the success of the strategy and indicates that the extended state observers are capable of detecting external disturbances without the need for physical sensors installed in the process.

6. Conclusions

In this paper, the application of the ADRC active disturbance rejection technique to a bearingless induction machine was analyzed. During the laboratory tests, two structures for the extended state estimator were used, a linear structure and a nonlinear structure. The results for step reference variation, for both versions, show good dynamic decoupling between the radial displacements in the X and Y axes. The test with load imposition showed that both observers were able to detect the generalized disturbance, however, only the nonlinear observer was able to maintain the same average system output value before and after load application. The results obtained show the success of the ADRC technique in the active estimation and rejection of disturbances in the radial positioning process of a split rotor bearingless machine. The results also show the higher active rejection capability of the technique applied with a nonlinear observer since with this type of observer disturbances of this nature are estimated and compensated.

Author Contributions

R. A. T., W. A. L. S. and A. O. S. conceived and designed the study; R. A. T. and W. A. L. S., methodology ; R. A. T. and W. A. L. S. performed the simulations and experiments; E. R. L. V., W. M. R. and A. O. S. reviewed the manuscript and provided valuable suggestions; E. R. L. V., R. A. T., W. A. L. S and W. M. R. wrote the paper; supervision, A. O. S. and W. M. R.

Funding

This research was funded in part by the Coordenação de Aperfeiçoamento de Pessoal de Nível Superior—Brasil (CAPES)—Finance Code 001 and Conselho Nacional de Desenvolvimento Científico e Tecnológico (CNPq).

Conflicts of Interest

The authors declare no conflicts of interest.

Abbreviations

The following abbreviations are used in this manuscript:

ADRC	Active Disturbance Rejection Control
ESO	Extended State Observer
DSP	Digital Signal Processing
LADRC	Linear Active Disturbance Rejection Control
PD	Proportional-Derivative
PI	Proportional-Integral
PID	Proportional-Integral-Derivative
PWM	Pulse Width Modulation
TD	Tracking Differentiator

References

Yang, Z.; Jia, J.; Sun, X.; Xu, T. An enhanced linear adrc strategy for a bearingless induction motor. IEEE Transactions on Transportation Electrification 2021, 8(1), 1255–1266. [Google Scholar] [CrossRef]
Yang, Z.; Ji, J.; Sun, X.; Zhu, H.; Q. Zhao, Q. Active Disturbance Rejection Control for Bearingless Induction Motor Based on Hyperbolic Tangent Tracking Differentiator. IEEE Journal of Emerging and Selected Topics in Power Electronics 2020, 8(3), 2623–2633. [Google Scholar] [CrossRef]
Silva, W. L. A.; Salazar, A. O.; Cabral Neto, R. J. N.; Villarreal, E. R. L. Radial Position Control of a Bearingless Machine with Active Disturbance Rejection Control. 2020 IEEE XXVII International Conference on Electronics, Electrical Engineering and Computing (INTERCON), Lima, Perú, 2020, pp. 1–5.
Silva, W. L. A.; Salazar, A. O.; Vieira, P. V. F.; Jácome, M. C.; Villarreal, E. R. L. Radial Position Control of a Bearingless Machine with Active Disturbance Rejection Control Fuzzy an approach. 2021 IEEE International Conference on Automation/XXIV Congress of the Chilean Association of Automatic Control (ICA-ACCA), Santiago Chile, Chile, 2021, pp.1–5.
Salazar, A. O.; Stephan, R. M. A bearingless method for induction machines. IEEE Transactions on Magnetics 1993, 29(6), 2965–2967. [Google Scholar] [CrossRef]
Ferreira, J. M. S.; Salazar, A. O. Máquina de Indução Sem Mancais: Modelo e Acionamento. Eletrônica de Potência. 2007, 12(3), 2509–2521.
De Paiva, J. A.; Salazar, A. O.; Maitelli, A. L. Review of Control Strategies and Model Estimation Techiniques Applied to Bearingless Induction Machine with Divided Winding. The 1st Brazilian Workshop on Magnetic Bearings,Rio de Janeiro: COPPE UFRJ. 2013, 1, 01–06. [Google Scholar]
Victor, V. F. ; Quintaes, F. O. ; Lopes, J. S. B. ; L. D. Santos Junior, L. D. ; Lock, A. S. ; Salazar, A. O. Analysis and Study of a Bearingless AC Motor Type Divided Winding, Based on a Conventional Squirrel Cage Induction Motor. IEEE Transactions on Magnetics. 2012, 48(11), 3571–3574.
Nunes, E. A. D. F.; Salazar, A. O.; Villarreal, E. R. L.; Souza, F. E. C.; Dos Santos Júnior, L. P.; Lopes, J. S. B.; Luque, J. C. C. Proposal of a fuzzy controller for radial position in a bearingless induction motor. IEEE Access. 2019, 7, 114808–114816. [Google Scholar] [CrossRef]
Carvalho Souza, F. E.; Silva, W.; Ortiz Salazar, A.; Paiva, J.; Moura, D.; Villarreal, E.R.L. A Novel Driving Scheme for Three-Phase Bearingless Induction Machine with Split Winding. Energies. 2021, 14(4930), 1–15. [Google Scholar] [CrossRef]
Zhu, L.; Zhang, G.; Jing, R.; Bi, G.; Xiang, R.; Wang, G.; Xu, D. Nonlinear active disturbance rejection control strategy for permanent magnet synchronous motor drives. IEEE Transactions on Energy Conversion. 2022, 37(3), 2119–2129. [Google Scholar] [CrossRef]
Chen, W. H.; Yang, J.; Guo, L.; Li, S. Disturbance-Observer-Based Control and Related Methods—An Overview. IEEE Transactions on Industrial Electronics. 2015, 63(2), 1083–1095. [Google Scholar] [CrossRef]
Gao, Zhiqiang (2003), Scaling and bandwidth-parameterization based controller tuning. Proceedings of the 2003 American Control Conference, 2003. Vol. 6, pp. 4989–4996.

Figure 1. Spatial arrangement of the stator windings of a bearingless induction motor with split winding.

Figure 2. Connection of the coils for two-phase position control.

Figure 3. Stator of bearingless induction motor with split winding.

Figure 4. Stator of bearingless induction motor with split winding subjected to right-side radial force.

Figure 5. General idea of the ADRC controller.

Figure 6. ADRC block diagram.

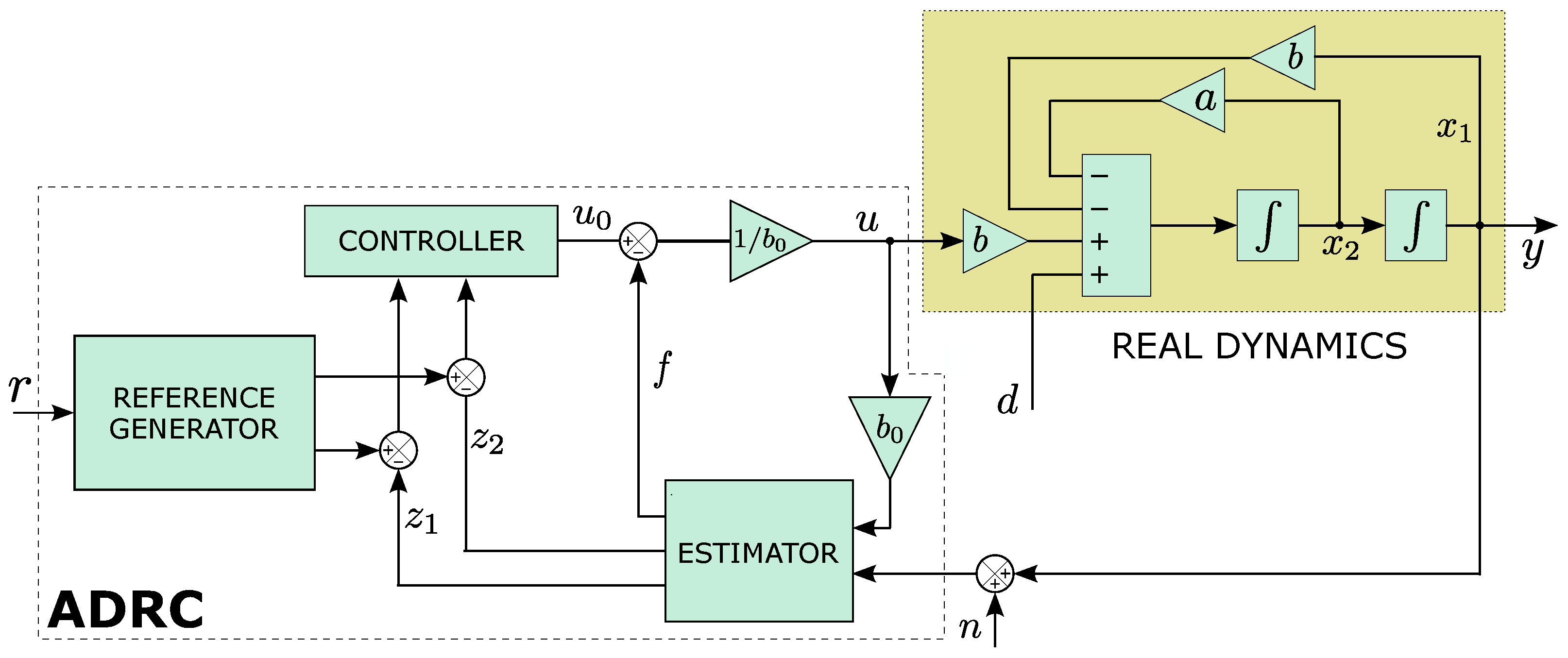
Figure 7. LADRC block diagram.

Figure 8. Linear observer.

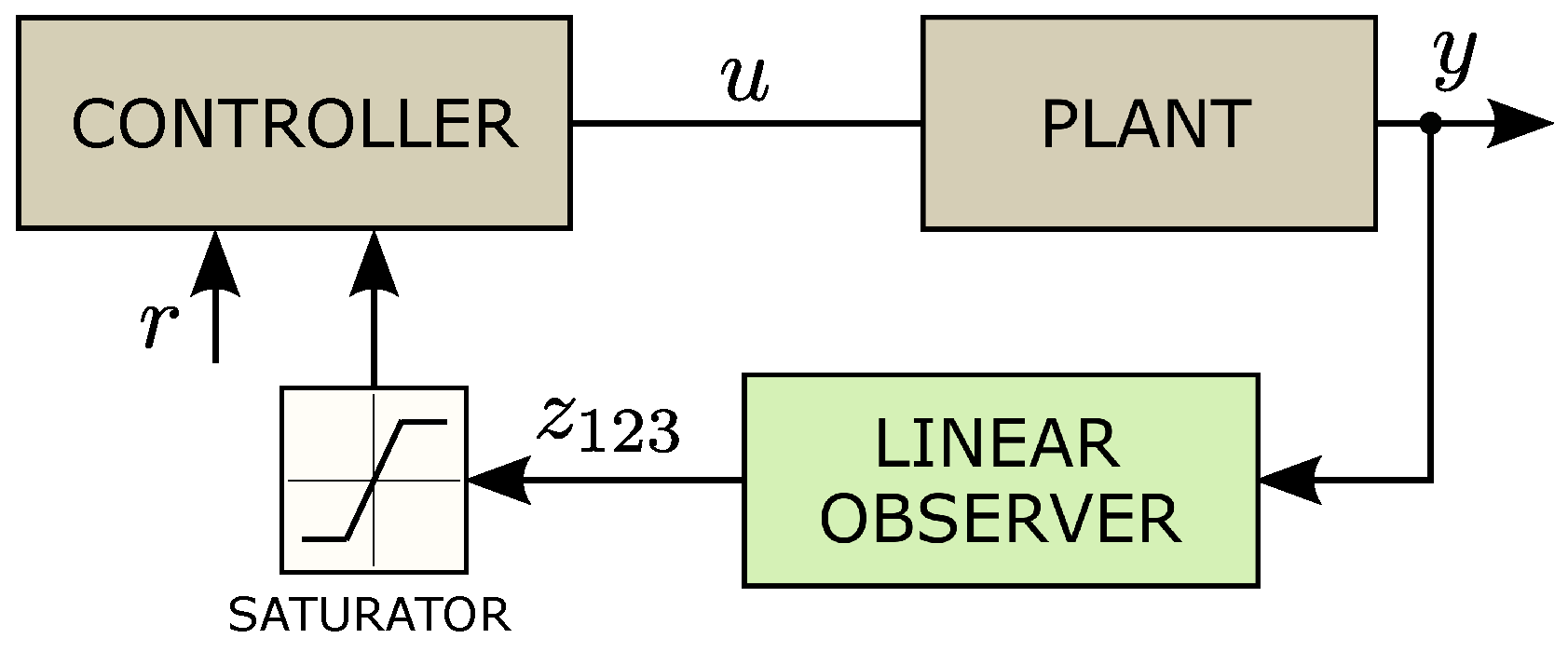
Figure 9. Nonlinear observer.

Figure 10. Overview of the components of the experimental bench.

Figure 11. Experimental bench.

Figure 12. Split-winding bearing machine prototype.

Figure 13. Control System diagram.

Figure 14. Steady state response of radial position control for the Linear and Nonlinear controllers in 3D.

Figure 20. Generalized disturbance estimation and mean steady state error for X and Y axes to differente values of radial load aligned with the X-axis.

Figure 21. Generalized disturbance estimation and mean steady state error for X and Y axes to different values of radial load aligned with the Y-axis.

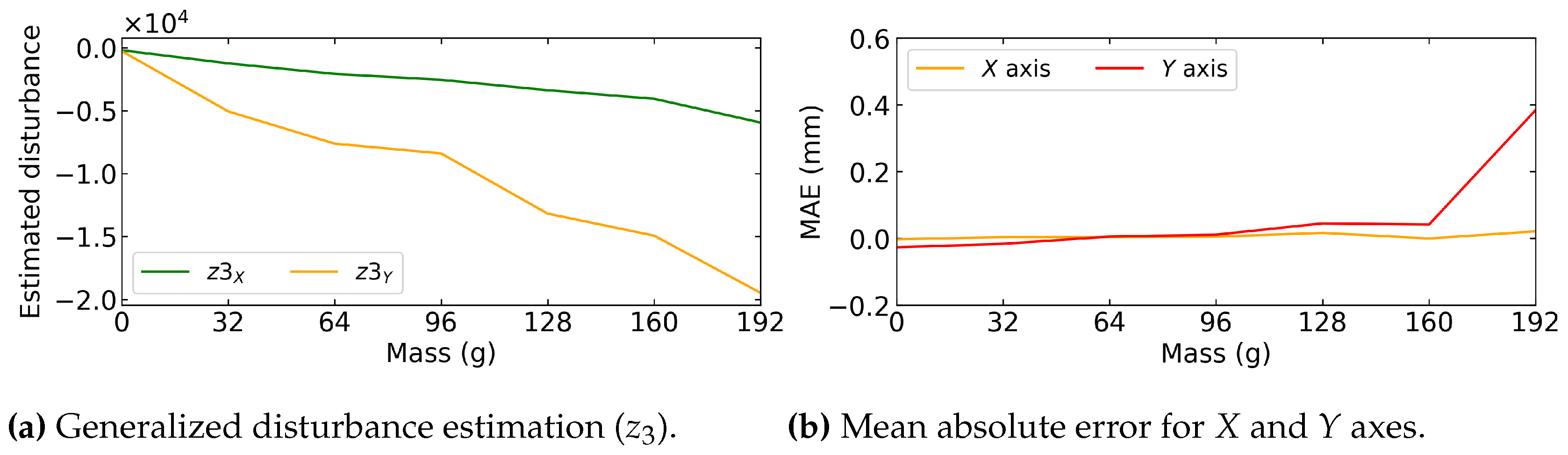
Table 1. Machine parameters.

Parameters	Values
Frequency	60 Hz
Poles number	4
Supply voltage	$220 / 380$ V
Current	$3.02 / 1.75$ A
No-load current	$1.9$ A
No-load power	160 W
Stator resistance per phase	$0.63$ Ohm

Table 2. Mean values of the states estimated by the Linear and Nonlinear ESO before and after the radial load application.

Controller	State $z_{1}$		State $z_{2}$		State $z_{3}$
Load application	before	after	before	after	before	after
Linear ESO	$0.57$	$0.64$	$- 0.32$	$- 0.33$	$1.64$	$- 7$
Nonlinear ESO	$0.48$	$0.48$	$- 1.34$	$- 2.6$	$- 1522$	$- 7909$

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content.

© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).

Copyright: This open access article is published under a Creative Commons CC BY 4.0 license, which permit the free download, distribution, and reuse, provided that the author and preprint are cited in any reuse.

MDPI Initiatives

Important Links

Choose an area of interest and we will send you notifications of new preprints at your preferred frequency.

Disclaimer

Application of Active Disturbance Rejection in a Bearingless Machine with Split Winding

Abstract

1. Introduction

2. Split Winding Bearingless Motor

2.1. Operation Principle

3. ADRC Control

4. Description of the System

4.1. System Overview

4.2. Split-Winding Bearingless Machine

4.3. Sensors

4.4. DSP TMS320F28335

4.5. Control Algorithm Implementation

5. Experiments and Results

6. Conclusions

Author Contributions

Funding

Conflicts of Interest

Abbreviations

References

MDPI Initiatives

Important Links

Subscribe