Prerpints.org logo
Preprint
Article

High Efficiency and High Stability for SHG in a Nd : YVO4 Laser with a KTP Intracavity and Q-switching through Harmonic Modulation

Altmetrics

Downloads

151

Views

30

Comments

0

A peer-reviewed article of this preprint also exists.

Submitted:

11 March 2023

Posted:

20 March 2023

You are already at the latest version

Alerts
Abstract
In this paper, the stabilization and high efficiency of an unstable Second Harmonic Generation (SHG) of a Nd : YVO4 laser with a KTP intracavity is carried out by using a passive Q-switching crystal and a parametric modulation method (harmonic modulation) is shown. The harmonic modulation was defined as um(t) = Amsin(2π fmt), with modulation amplitude as Am(Vpp), and modulation frequency as fm(Hz), applied to the pumping of the Nd : YVO4 -KTP laser to control the amplitude and frequency of the emission, thus stabilizing the dynamic states of this laser. The promising application of this green light source is materialized when such light is necessary for high-density optics, such as in the treatment materials industry or in some aesthetic applications.
Keywords: 
Subject: Biology and Life Sciences  -   Forestry

1. Introduction

Currently, one of the most widely used solid-state lasers in industrial applications, such as materials processing, cutting and welding, is the N d : Y V O 4 laser (yttrium orthovanadate crystal doped with neodymium) [1]. The N d : Y V O 4 is used due to its high absorption coefficient and its wide section of stimulated emission; in addition, it has a better performance as compared to a N d : Y A G ( Y 2 A l 5 O 12 aluminum oxide and itrium oxide doped with neodymium Nd+3) laser. However, thermal effects (thermal lens) are more significant in the N d : Y V O 4 ; so, it is necessary to monitor the glass temperature to keep it low [2,3,4,5,6,7]. Lasers doped with N d + 4 , such as Nd:YAG and N d : Y V O 4 , usually emit radiation at a wavelength of 1064 nm, supported by a K T i O P O 4 crystal (potassium titanyl phosphate crystal or KTP). Inside the laser cavity, it is possible to double the frequency by converting the infrared light of 1064 nm into green light of 532 nm; this phenomenon is called Second Harmonic Generation (SHG) [8]. Due to the nonlinear coupling between modes in the SHG, the crystal leads to irregular fluctuations in the laser output, which is amplified by a Q factor of the laser cavity due to the presence of the amplifying laser [9], also known as the green problem. This irregular behavior has been studied in detail and attributed to the destabilization of the relaxation of the oscillations, which has always been present in this type of lasers due to the nonlinear coupling of the longitudinal modes [10,11,12,13,14,15,16,17,18,19].
There are several approaches in order to stabilize the green light generated by SHG in a solid-state laser. One of them is the use of external modulation, where low emission power is achieved by using nonlinear waves for SHG. This solution provides high conversion efficiency, and high power can be achieved by using flat waves [20]. In a N d : Y V O 4 laser, placement of the KTP crystal is fundamental to obtain green emission by folding the frequency. When the crystal is placed inside the cavity green radiation is emitted at high power, since the energy density of the radiation is higher inside the optical cavity. The green light intensity produced by the KTP crystal is proportional to the square of the fundamental wavelength intensity, but tends to be unstable. This instability is caused by the competition of modes in the cavity; if the KTP is placed outside the cavity, it is also possible to generate the second harmonic, and the emission in this case is stable, but with low power [21].
An approach to suppressing unwanted fluctuations is to control the injection current of the pump diode by means of electronic feedback. In 1992 [10], Rajarshi Roy et al. succeeded in stabilizing the output waveforms of the laser using a modification of the chaos control method proposed by Ott-Grebogy-Yorke (OGY) ([22]); this control technique is known as Occasional Proportional Feedback (OPF), and in other systems, such as CO2 lasers, the method has been used to stabilize unstable periodic orbits [23]. A parametric modulation technique has been implemented by adding a sinusoidal signal to the system parameters in lasers made of erbium-doped fibers [24,25] to induce multistability and to stabilize the laser. The harmonic modulation technique consists in adding a periodic signal to the input of the system; in this case, it is a sinusoidal periodic signal to an accessible parameter of the laser system, to obtain a modulated parameter.
There is a method to increase the output power of a laser by modulating losses. This method is known as Q-switching, and it is divided into two techniques: active and passive Q-switching. In active Q-switching, losses are modulated by active elements, such as acousto-optic or electro-optic modulators; in passive Q-switching, losses are automatically modulated by a saturable absorber, and the pulse is generated when the energy stored in the medium reaches the appropiate level [26]. Normally, the average power of lasers in Q-switch operation is lower than the optical power of a continuous wave laser and the instabilities are reduced by placing a Q-switch crystal inside the cavity [27,28,29,30,31].
In this paper, an experimental study of a N d : Y V O 4 laser with a KTP crystal intracavity emitting at 1064 nm is shown. In such case, the KTP crystal produces a laser emission at 532 nm, which tends to be unstable and gives rise to the so-called “green problem” treated in recent years [10,11]. By applying controlled external modulation and using a passive Q-switching, it is possible to stabilize the emission and obtain a high conversion efficiency. The emission of the laser is analyzed based on the response in therms of optical power, optical spectra, frequency spectra and time series by using the local maxima and inter-spike-interval (ISI). This work focuses on stabilizing the green emission in frequency and pulse amplitude by adding harmonic modulation to the pumping current, where this modulation is applied by changing the amplitude and frequency. In Section 2 the experimental setup of a N d : Y V O 4 laser with a KTP crystal intracavity for second harmonic generation with external modulation is presented. Then, in Section 3, some results on stabilizing the frequency and amplitude of the SHG of the N d : Y V O 4 laser are shown. Finally, in Section 4, the results obtained and the conclusions are presented.

2. Preliminaries

There are a few methods to generate green light, but the best known is probably second harmonic generation. The green problem arises when the cavity generates not only the second harmonic, but also other frequencies due to the competition of longitudinal modes, which produce chaotic behavior[9]. The generation of those frequencies, as well as the generation of the second harmonic, are related to the polarization of the medium. This polarization occurs when an electric field is applied to the material as follows:
P → = ϵ 0 χ E → ,
where ϵ 0 is the permitivity in the free space and χ is the linear susceptibility. If the electric field is small, the approximation is linear, but if the electric field is large, the best approximation is nonlinear; then, we can expand the equation of polarization in power series as:
P → = ϵ 0 χ E → + ϵ 0 χ ( 2 ) E → 2 + ϵ 0 χ ( 3 ) E → 3 + ⋯ + ϵ 0 χ ( n ) E → n , P → = P → ( 1 ) + P → ( 2 ) + P → ( 3 ) + ⋯ + P → ( n ) ,
note that χ ( 2 ) , χ ( 3 ) , ⋯, χ ( n ) are the nonlinear susceptibilities of second, third and n t h –order, respectively, and P ( n ) is the polarization of order n. Next, suppose an electromagnetic wave
E → = E 1 e − ω 1 t + E 2 e − ω 2 t + c . c . ,
where ω 1 and ω 2 are frequencies, E 1 and E 2 are the involved amplitudes of the electromagnetic wave, while c . c . represents a complex conjugate term. Substituting in the polarization of second order of Equation (2), the next equation is obtained:
P → ( 2 ) = ϵ 0 χ ( 2 ) ( E 1 e − i ω 1 t + E 2 e − i ω 2 t + c . c . ) 2 , P → ( 2 ) = ϵ 0 χ ( 2 ) ( E 1 e − i ω 1 t + E 2 e − i ω 2 t + E 1 * e i ω 1 t + E 2 * e i ω 2 t ) 2 , P → ( 2 ) = 2 ϵ 0 χ ( 2 ) ( E 1 E 1 * + E 2 E 2 * ) + ϵ 0 χ ( 2 ) ( E 1 E 1 * e − i 2 ω 1 t + E 2 E 2 * e − i 2 ω 2 t ) + 2 ϵ 0 χ ( 2 ) E 1 * E 2 e − i ( ω 1 + ω 2 ) t + 2 ϵ 0 χ ( 2 ) E 1 E 2 * e − i ( ω 1 − ω 2 ) t .
By analyzing equation (4), we can identify terms with different frequencies corresponding to the 4 non-linear effects of second order: Optical Rectification (OR), Second Harmonic Generation (SHG), Generation of Frequency Sum (GFS) and Generation of Difference Frequency (GDF):
P → O R ( 2 ) = 2 ϵ 0 χ ( 2 ) ( E 1 E 1 * + E 2 E 2 * ) ,
P → S H G ( 2 ) = ϵ 0 χ ( 2 ) ( E 1 E 1 * e − i 2 ω 1 t + E 2 E 2 * e − i 2 ω 2 t ) ,
P → G F S ( 2 ) = 2 ϵ 0 χ ( 2 ) E 1 * E 2 e − i ( ω 1 + ω 2 ) t ,
P → G D F ( 2 ) = 2 ϵ 0 χ ( 2 ) E 1 E 2 * e − i ( ω 1 − ω 2 ) t .
In the cavity of a laser, it is possible to generate other longitudinal modes, creating more frequency combinations and making the behavior more unstable [32]. The first time this phenomenon was observed in a laser was in 1961, when it was reported that in addition to the laser’s natural wavelength (694.3 nm), a second harmonic (347.2 nm) had been generated [8]. With this finding the study of nonlinear optics and the use of SHG in different areas of science began [33,34].

3. Experimental Setup

In Figure 1, the scheme of the experimental arrangement of an N d : Y V O 4 laser with a KTP crystal intracavity for second harmonic generation is shown. As can be seen, the arrangement is the simplest in order to get the easiest way to generate the SHG. This experiment was carried out with a laser diode pumping at 808 nm (LD, Moduligth ML2020), a current source (Bk-Precision 1696) and a lens (L) with focal length f = 5 mm, which is used to focus the beam onto the N d : Y V O 4 . The cavity consist of the N d : Y V O 4 crystal and a KTP intracavity glass, which have special coatings that work as reflecting surfaces at the wavelengths used (Figure 1), and at the output there are two beam splitters: BS1 (BS010), designed for wavelengths from 400 to 700 nm, and BS2 (BS011), is designed for wavelengths of 700 to 1100 nm. The resulting beam of BS1 is filtered by a bandpass filter (FL532-10, FG) at 532 nm, and the BS2 beam is filtered by a bandpass filter (FL1064-10, IRF) at 1064 nm. Such filtering affects photo-detectors PD1 and PD2, respectively, which convert the optical signal to an electrical one to be analyzed by the oscilloscope (DPO7104); the output of PD1 is also analyzed by a frequency spectrum analyzer (FSA Aeroflex). The unfiltered beam is introduced into an optical fiber to be analyzed in an optical spectrum analyzer (OSA MS9030A).
By carrying out the implementation of the experimental setup shown in Figure 1, it will be possible to know the unstable behavior that characterizes this type of laser and its output as a second harmonic. With this, we can analyze the original response of the N d : Y V O 4 -KTP laser which will serve as a starting point before applying the harmonic modulation A m s i n ( 2 π f m t ) to the system.
In order to analyze the emission of the N d : Y V O 4 -KTP laser with external modulation in the experimental setup shown in Figure 1, a sinusoidal modulation signal given by an arbitrary signal generator (AFG) is added to the pump current. With the AFG device, the pump current I m is given as
I m = I 0 + u m ( t ) ,
where I 0 is the initial current parameter, and u m ( t ) = A m s i n ( 2 π f m t ) is an external harmonic modulation, with A m being the modulation amplitude (Vpp) and f m (Hz) the modulation frequency. The control signal, with a suitable gain A m and a frequency close to the desired limit cycle, must be able to eliminate coexisting states.
Using the same arrangement shown in Figure 1, it is possible to separate the green (532 nm) and infrared (1064 nm) emissions through the use of beam splitters (BS1 and BS2) at the output of the laser; then, we characterize the behavior of the laser when applying the modulation. The acquisition of data can be done through the use of the oscilloscope (temporal series), the optical spectrum analyzer (optical spectra), and the second harmonic (532 nm); the frequency spectrum can also be obtained, using the frequency spectra.
In order to select the best performance from the pump laser diode (LD), the voltage and current of the source were fixed as V = 7 V and I = 2.1 A, respectively, and modulation in frequency and amplitude was applied as 10 kHz < f m < 90 kHz in increments of 1 kHz, while amplitude was increased in steps of 1 Vpp within a range of 2 Vpp < A m < 20 Vpp. Figure 2a–e) shows the emission of LD at 808 nm for f m = 60 kHz and A m variable, and Figure 3a–e) shows the emission of the laser diode with A m = 15 Vpp and variable f m .
In Figure 4, we can observe that the peak-peak voltage (Vpp) of the emission increases by increasing f m or A m , but the intensity of the emission does not have the same amplitude to A m , and only a percentage is observed; this relationship between the amplitude of the modulation and the amplitude of the emission is shown in Figure 5. The peak-to-peak voltage of the LD emission was calculated by varying modulation amplitude (Figure 5a) and modulation frequency (Figure 5b).

4. Results of the Stabilization in Frequency and Amplitude of SHG of the Nd : YVO 4

The stability of the intensity of the emission of the second harmonic in N d : Y V O 4 -KTP lasers is known to be normally irregular; so, in this experiment, it was worked to stabilize the intensity of the emission and also to achieve greater conversion efficiency. The wavelength of the second harmonic (532nm) with respect to the emission at the fundamental wavelength (1064 nm). Passive Q-switching has been used to obtain an emission intensity with less stability, but they may present greater conversion efficiency of the laser power. It will be shown that with the harmonic modulation u m ( t ) = A m sin ( 2 π f m t ) , there is an emission intensity that has stability and greater conversion efficiency. In this section, the modulation parameters for amplitude ( A m ) and frequency ( f m ) are defined, for which the emission of the second harmonic has greater stability.
In order to know the behavior of the output power as a function of the pumping power, from the experimental arrangement shown in Figure 1, a sweep of 0 to 4.5 W of optical pumping power was made, and the results can be seen in Figure 6, which shows the Nd:YVO4 laser output, as a continuous wave and in Q-Switching mode at 1064 nm, as well as the power output as a continuous wave and in Q-Switching mode at 532 nm.
The intracavity conversion efficiency was found by relate the optical power at 532 nm to the optical power at 1064 nm. In Table 1, we can see that if the laser array was in the Q-switching mode, efficiency was 40 % , higher than the results in previous works [30], whereas if the laser array was not in Q-switching mode, efficiency was only 12 % .
Figure 7. shows the optical spectra amplitude at wavelength range 350 nm < λ < 1300 nm of the laser emission N d : Y V O 4 , with a modulation amplitude range 4 Vpp < A m < 20 Vpp to know the behavior of current output when modulating the diode current. The characteristic wavelengths of 808 nm of the pump laser diode, 1064 nm. of the emission of N d : Y V O 4 and 532 nm. of the second harmonic when using the KTP are observed. The wavelength of 350 nm corresponds to the frequency sums phenomenon of the fundamental wavelengths 1064 nm and second harmonic (532 nm).
Using the experimental arrangement for the laser configuration shown in Figure 1, it was found that the pulse frequency without modulation is F Q ≂ 57 kHz; this is observed in Figure 8, where the parameters of the modulation signal were kept constant with voltage V = 7 V and current I = 2.1 A.
Using the experimental arrangement for the laser configuration shown in Figure 9, the frequency spectra of the N d : Y V O 4 laser emission were obtained by varying the modulation frequency 1 kHz < f m < 100 kHz while using a range of amplitude variation modulation of 4 Vpp < A m < 20 Vpp.
Figure 10 is a map in which the black areas represent the minimums of the standard deviation: Figure 10a) represents the standard deviation of the local maxima, Figure 10b) represents the standard deviation of the interspike-interval (ISI), and Figure 10c) represents the values of the parameters for which there are minimums of standard deviation of the ISI and of the local maxima of the emission at 532 nm.

5. Conclusions

The implementation of an intracavity N d : Y V O 4 —KTP laser with passive Q—switching ( C r 4 + : Y A G ) was presented, using a harmonic modulation technique to stabilize the emission in amplitude and frequency of the laser. With respect to the optical power, it was found that the conversion efficiency between the fundamental wavelength 1064 nm and the wavelength of 532 nm, in pulsed emission (crystal for Q-switching) and using a saturable absorber ( C r 4 + : Y A G ) intracavity, resulted in 40 % conversion efficiency. Analyzing the time series of the laser emission when modulating signal u m ( t ) = A m sin ( 2 π f m t ) is added to the pumping, the frequency looking is achieved at the dominant frequency range 45 k H z < f m < 60 k H z , for the amplitude 10 V p p < A m < 20 V p p ; under this conditions the laser emission is stabilized in amplitude and frequency. The F Q relaxation frequency of the Nd: YVO4 laser was found using a modulation amplitude of 14 V p p < A m < 16 V p p and a modulation frequency f m = F Q = 57 KHz. The best result appeared with the modulation parameters A m = 15 V p p and f m = 57 k H z , with which it was possible to stabilize the emission laser; those data were obtained from the calculation of the standard deviation of the local maxima and the ISI map. It can be said that in order to stabilize the emission of the N d : Y V O 4 -KTP intracavity laser with passive Q-switching, a modulation frequency that is approximately equal to the oscillation frequency of the laser ( f m = F Q ), must be sought a harmonic resonance between the modulation frequency and the relaxation frequency of the laser, thus obtaining a stable emission of high efficiency.

Author Contributions

S.M.A.D.: Writing—Original Draft, Writing—Review & Editing, Methodology, Software, Validation, Data Curation, Visualization. J.H.G.L.: Writing—Review & Editing, Resources, Project administration. R.J.R.: Supervision, Funding acquisition, Writing—Review & Editing, Resources. V.A.: Writing—Review & Editing, Resources. J.L.E.M.: Writing—Review & Editing, Visualization, Conceptualization. G.H.C.: Writing—Original Draft, Writing - Review & Editing, Methodology, Software, Validation, Data Curation, Visualization, Project administration.

Funding

This project was supported by CONACYT under project number 320597.

Acknowledgments

A.D.S.M. acknowledges support from National Council of Science and Technology -CONACyT-, Mexico, CONACYT. J.L.E.M. thanks CONACYT for financial support (CVU-706850, project: A1-S-26123, and project: 320597). R.J.R. thanks CONACYT for financial support, project No. 320597.

Conflicts of Interest

The authors certify that they have NO affiliations with or involvement in any organization or entity with any financial interest (such as honoraria; educational grants; participation in speakers’ bureaus; membership, employment, consultancies, stock ownership, or other equity interest; and expert testimony or patent-licensing arrangements), or non-financial interest (such as personal or professional relationships, affiliations, knowledge or beliefs) in the subject matter or materials discussed in this manuscript.

References

  1. Eichhorn, M. Laser physics: from principles to practical work in the lab; Springer Science & Business Media, 2014.
  2. Zhang, Y.; Zhao, S.; Li, D.; Yang, K.; Li, G.; Zhang, G.; Cheng, K. Diode-pumped doubly Q-switched mode-locked YVO4/Nd: YVO4/KTP green laser with AO and GaAs saturable absorber. Optical Materials 2011, 33, 303–307. [Google Scholar] [CrossRef]
  3. Mukhopadhyay, P.; Alsous, M.; Ranganathan, K.; Sharma, S.; Gupta, P.; George, J.; Nathan, T. Analysis of laser-diode end-pumped intracavity frequency-doubled, passively Q-switched and mode-locked Nd: YVO4 laser. Applied Physics B 2004, 79, 713–720. [Google Scholar] [CrossRef]
  4. Liu, J.; Yang, J.; Fan, X.; Wang, G. Symmetric and short green-pulse generation by doubly Q-switched Nd: YVO4 laser. Laser Physics 2010, 20, 222–225. [Google Scholar] [CrossRef]
  5. Wang, X.; Li, M. Continuous-wave passively mode-locked Nd: YVO4/KTP green laser with a semiconductor saturable absorber mirror. Laser physics 2010, 20, 733–736. [Google Scholar] [CrossRef]
  6. Zhuo, Z.; Li, T.; Li, X.; Yang, H. Investigation of Nd: YVO4/YVO4 composite crystal and its laser performance pumped by a fiber coupled diode laser. Optics communications 2007, 274, 176–181. [Google Scholar] [CrossRef]
  7. Fields, R.; Birnbaum, M.; Fincher, C. Highly efficient Nd: YVO4 diode-laser end-pumped laser. Applied physics letters 1987, 51, 1885–1886. [Google Scholar] [CrossRef]
  8. Franken, P.; Hill, A.E.; Peters, C.e.; Weinreich, G. Generation of optical harmonics. Physical Review Letters 1961, 7, 118. [Google Scholar] [CrossRef]
  9. McDonagh, L.; Wallenstein, R. Low-noise 62 W CW intracavity-doubled TEM 00 Nd: YVO 4 green laser pumped at 888 nm. Optics letters 2007, 32, 802–804. [Google Scholar] [CrossRef] [PubMed]
  10. Roy, R.; Murphy Jr, T.; Maier, T.; Gills, Z.; Hunt, E. Dynamical control of a chaotic laser: Experimental stabilization of a globally coupled system. Physical Review Letters 1992, 68, 1259. [Google Scholar] [CrossRef]
  11. Campos-Mejía, A.; Pisarchik, A.; Pinto-Robledo, V.; Sevilla-Escoboza, R.; Jaimes-Reátegui, R.; Huerta-Cuellar, G.; Vera-Avila, V. Synchronization of infrared and green components in a loss-modulated dual-cavity Nd: YAG laser with second harmonic generation. The European Physical Journal Special Topics 2014, 223, 2799–2806. [Google Scholar] [CrossRef]
  12. Pyragas, K.; Lange, F.; Letz, T.; Parisi, J.; Kittel, A. Dynamics and control of a multimode laser: Reduction of space-dependent rate equations to a low-dimensional system. Physical Review E 2000, 63, 016204. [Google Scholar] [CrossRef]
  13. Le Garrec, B.; Raze, G.; Thro, P.; Gilbert, M. High-average-power diode-array-pumped frequency-doubled YAG laser. Optics letters 1996, 21, 1990–1992. [Google Scholar] [CrossRef] [PubMed]
  14. Konno, S.; Kojima, T.; Fujikawa, S.; Yasui, K. High-brightness 138-W green laser based on an intracavity-frequency-doubled diode-side-pumped Q-switched Nd: YAG laser. Optics letters 2000, 25, 105–107. [Google Scholar] [CrossRef]
  15. Wang, H.; Huang, W.; Zhou, Z.; Cao, H. Experimental study of a high power and high efficiency CW diode-side-pumped Nd: YAG laser. Optics & Laser Technology 2004, 36, 69–73. [Google Scholar] [CrossRef]
  16. Lee, S.; Kim, Y.G.; Cha, B.H.; Kim, Y.K. A diode-pumped linear intracavity frequency doubled Nd: YAG rod laser with 40ns pulse width and 73W green output power. Optics & Laser Technology 2004, 36, 265–271. [Google Scholar] [CrossRef]
  17. Zhuang, F.; Zheng, Y.; Huang, C.; Wei, Y.; Zhu, H.; Zhang, G. Efficient and compact intracavity-frequency-doubled YVO4/Nd: YVO4/KTP laser through analysis of the interaction length. Optics communications 2010, 283, 3324–3327. [Google Scholar] [CrossRef]
  18. Zhang, Y.; Zhao, X.; Zhang, H.; Meng, L.; Li, L.; Li, X.; Wang, P.; Yao, J. High-power continuous wave green beam generation by use of simple linear cavity with side-pumped module. Optics communications 2010, 283, 5161–5164. [Google Scholar] [CrossRef]
  19. Liu, C.; Roy, R.; Abarbanel, H.D.; Gills, Z.; Nunes, K. Influence of noise on chaotic laser dynamics. Physical Review E 1997, 55, 6483. [Google Scholar] [CrossRef]
  20. Jensen, O.B.; Andersen, P.E.; Sumpf, B.; Hasler, K.H.; Erbert, G.; Petersen, P.M. 1.5 W green light generation by single-pass second harmonic generation of a single-frequency tapered diode laser. Optics Express 2009, 17, 6532–6539. [Google Scholar] [CrossRef]
  21. Hitz, B. Generating High Green Power Without the Green Problem. Photonics Spectra 2007, 41, 92–93. [Google Scholar]
  22. Ott, E.; Grebogi, C.; Yorke, J.A. Controlling chaos. Physical review letters 1990, 64, 1196. [Google Scholar] [CrossRef] [PubMed]
  23. Pisarchik, A.; Corbalán, R.; Chizhevsky, V.; Vilaseca, R.; Kuntsevich, B. Dynamic stabilization of unstable periodic orbits in a CO 2 laser by slow modulation of cavity detuning. International Journal of Bifurcation and Chaos 1998, 8, 1783–1789. [Google Scholar] [CrossRef]
  24. Pisarchik, A.N.; Kir’yanov, A.V.; Barmenkov, Y.O.; Jaimes-Reátegui, R. Dynamics of an erbium-doped fiber laser with pump modulation: theory and experiment. JOSA B 2005, 22, 2107–2114. [Google Scholar] [CrossRef]
  25. Sevilla-Escoboza, R.; Pisarchik, A.N.; Jaimes-Reátegui, R.; Huerta-Cuellar, G. Selective monostability in multi-stable systems. Proceedings of the Royal Society A: Mathematical, Physical and Engineering Sciences 2015, 471, 20150005. [Google Scholar] [CrossRef]
  26. Khanin, Y.I. Fundamentals of Laser Dynamics; Cambridge International Science Publishing, 2008.
  27. Kee, H.H.; Lee, G.; Newson, T.P. Narrow linewidth CW and Q-switched erbium-doped fibre loop laser. Electronics Letters 1998, 34, 1318–1319. [Google Scholar] [CrossRef]
  28. Yang, X.Q.; Wang, H.X.; He, J.L.; Zhang, B.T.; Huang, H.T. A compact passively Q-switched intra-cavity frequency doubled Nd: YAG/Cr4+: YAG composite crystal green laser. Laser physics 2009, 19, 1964. [Google Scholar] [CrossRef]
  29. Tsai, T.Y.; Fang, Y.C. A saturable absorber Q-switched all-fiber ring laser. Optics express 2009, 17, 1429–1434. [Google Scholar] [CrossRef] [PubMed]
  30. Matía-Hernando, P.; Guerra, J.M.; Weigand, R. An Nd: YLF laser Q-switched by a monolayer-graphene saturable-absorber mirror. Laser Physics 2013, 23, 025003. [Google Scholar] [CrossRef]
  31. Degnan, J.J. Theory of the optimally coupled Q-switched laser. IEEE Journal of Quantum Electronics 1989, 25, 214–220. [Google Scholar] [CrossRef]
  32. Boyd, R.W. The nonlinear optical susceptibility. Nonlinear optics 2008, 3, 1–67. [Google Scholar]
  33. Alam, M.; Voravutinon, N.; Warycha, M.; Whiting, D.; Nodzenski, M.; Yoo, S.; West, D.P.; Veledar, E.; Poon, E. Comparative effectiveness of nonpurpuragenic 595-nm pulsed dye laser and microsecond 1064-nm neodymium: yttrium-aluminum-garnet laser for treatment of diffuse facial erythema: a double-blind randomized controlled trial. Journal of the American Academy of Dermatology 2013, 69, 438–443. [Google Scholar] [CrossRef] [PubMed]
  34. Shen, Y. Surface properties probed by second-harmonic and sum-frequency generation. Nature 1989, 337, 519–525. [Google Scholar] [CrossRef]
Preprints 69673 g001
Figure 1. Schematic arrangement of the N d : Y V O 4 -KTP laser with a KTP crystal intracavity for second harmonic generation and modulated by an external signal.
Figure 1. Schematic arrangement of the N d : Y V O 4 -KTP laser with a KTP crystal intracavity for second harmonic generation and modulated by an external signal.
Preprints 69673 g001
Preprints 69673 g002
Figure 2. Pump laser diode emission by varying the harmonic modulation amplitude for a fixed frequency f m = 60 k H z and modulation amplitude A m : (a) 4 V, (b) 8 V, (c)12 V, (d) 16V, (e) 20 V.
Figure 2. Pump laser diode emission by varying the harmonic modulation amplitude for a fixed frequency f m = 60 k H z and modulation amplitude A m : (a) 4 V, (b) 8 V, (c)12 V, (d) 16V, (e) 20 V.
Preprints 69673 g002
Preprints 69673 g003
Figure 3. Laser diode emission by varying the harmonic modulation frequency and with fixed amplitude A m =15 V and modulation frequency f m : (a) 15 kHz, (b) 30 kHz, (c) 45 kHz, (d) 60 kHz, (e) 75 kHz.
Figure 3. Laser diode emission by varying the harmonic modulation frequency and with fixed amplitude A m =15 V and modulation frequency f m : (a) 15 kHz, (b) 30 kHz, (c) 45 kHz, (d) 60 kHz, (e) 75 kHz.
Preprints 69673 g003
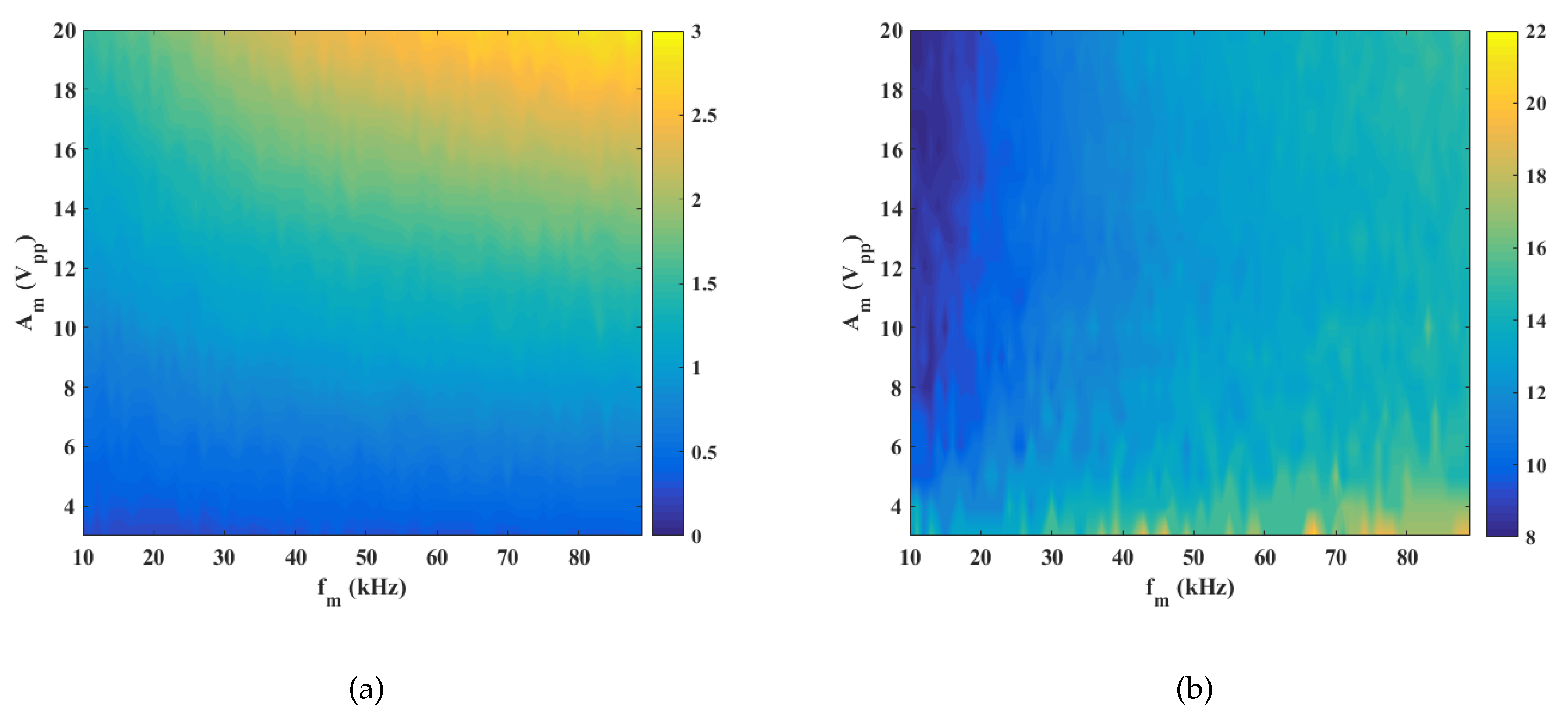
Preprints 69673 g004
Figure 4. (a) Peak-to-peak voltage map of the electric current of the DL emission when modulated with signal u m ( t ) = A m sin ( 2 π f m t ) . (b) Percentage relationship of the V pp that presents the electrical intensity of the emission of the DL with respect to the modulation amplitude A m ( V p p ) .
Figure 4. (a) Peak-to-peak voltage map of the electric current of the DL emission when modulated with signal u m ( t ) = A m sin ( 2 π f m t ) . (b) Percentage relationship of the V pp that presents the electrical intensity of the emission of the DL with respect to the modulation amplitude A m ( V p p ) .
Preprints 69673 g004
Preprints 69673 g005
Figure 5. Curves of V p p of the emission of the LD varying (a) amplitude ( A m ) y (b) frequency ( f m ).
Figure 5. Curves of V p p of the emission of the LD varying (a) amplitude ( A m ) y (b) frequency ( f m ).
Preprints 69673 g005
Preprints 69673 g006
Figure 6. Power of the laser with KTP intra-cavity.
Figure 6. Power of the laser with KTP intra-cavity.
Preprints 69673 g006
Preprints 69673 g007
Figure 7. Surface of optical spectra.
Figure 7. Surface of optical spectra.
Preprints 69673 g007
Preprints 69673 g008
Figure 8. (a) Time series of the 1064 nm wavelength emission, (b) Time series of the wavelength radiation of 532 nm. (c) Spectrum frequency of the emission of 532 nm.
Figure 8. (a) Time series of the 1064 nm wavelength emission, (b) Time series of the wavelength radiation of 532 nm. (c) Spectrum frequency of the emission of 532 nm.
Preprints 69673 g008
Preprints 69673 g009
Figure 9. Frequency spectrum maps obtained by varying harmonic modulation frequency 1 kHz < f m < 100 kHz and modulation amplitude: (a) A m = 4 Vpp, (b) A m = 8 Vpp, (c) A m = 12 Vpp, (d) A m = 16 Vpp, (e) A m = 20 Vpp.
Figure 9. Frequency spectrum maps obtained by varying harmonic modulation frequency 1 kHz < f m < 100 kHz and modulation amplitude: (a) A m = 4 Vpp, (b) A m = 8 Vpp, (c) A m = 12 Vpp, (d) A m = 16 Vpp, (e) A m = 20 Vpp.
Preprints 69673 g009
Preprints 69673 g010
Figure 10. Stability zones for different amplitudes A m and frequencies f m .
Figure 10. Stability zones for different amplitudes A m and frequencies f m .
Preprints 69673 g010
Table 1. Optical efficiency conversion with intra-cavity configuration at V = 7 V and I = 2.1 A.
Table 1. Optical efficiency conversion with intra-cavity configuration at V = 7 V and I = 2.1 A.
Q-Switch Efficiency ( % )
Intracavity X 12.7
√ 40.0
Optical efficiency conversion with intra-cavity configuration at V = 7 V and I = 2.1 A.
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content.
Copyright: This open access article is published under a Creative Commons CC BY 4.0 license, which permit the free download, distribution, and reuse, provided that the author and preprint are cited in any reuse.
Prerpints.org logo

Preprints.org is a free preprint server supported by MDPI in Basel, Switzerland.

facebook logotwitter logolinkedin logo
MDPI Initiatives
Important Links
Subscribe

Disclaimer

Terms of Use

Privacy Policy

© 2024 MDPI (Basel, Switzerland) unless otherwise stated