Topological Vortexes, Asymptotic Freedom and Multifractals

Preprint

Article

Topological Vortexes, Asymptotic Freedom and Multifractals

Altmetrics

Downloads

353

Views

109

Comments

A peer-reviewed article of this preprint also exists.

Supplementary Material

supplementary.zip (13.54MB )

Alexander Migdal^*

This version is not peer-reviewed

Submitted:

21 March 2023

Posted:

22 March 2023

Read the latest preprint version here

Alerts

Abstract

We study the Kelvinons: monopole ring solutions to the Euler equations, regularized as the Burgers vortex in the viscous core. There is finite anomalous dissipation in the inviscid limit. However, in the anomalous Hamiltonian, some terms are growing as logarithms of Reynolds number; these terms come from the core of the Burgers vortex. In our theory, the turbulent multifractal phenomenon is similar to asymptotic freedom in QCD, with these logarithmic terms summed up by an RG equation. The small effective coupling does not imply small velocity; on the contrary, velocity is large compared to its fluctuations, which opens the way for a quantitative theory. In the leading order in the perturbation theory in this effective coupling constant, we compute running multifractal dimensions for high moments of velocity circulation, in good agreement with the data for quantum Turbulence and available data for classical Turbulence. The logarithmic dependence of fractal dimensions on the loop size comes from the running coupling in anomalous dimensions. This slow logarithmic drift of fractal dimensions would be barely observable at Reynolds numbers achievable at modern DNS.

Keywords:

Subject: Physical Sciences - Theoretical Physics

1. Introduction

In 1948 Burgers discovered his vortex solution to the Navier-Stokes equations [1]. This exact stationary solution with cylindrical geometry had an anomalous dissipation (a finite dissipation at vanishing viscosity).

It should have been a breakthrough in the theory of Turbulence. Instead, a phenomenological K41 theory by Kolmogorov and Obukhov [2] dominated the turbulence studies for the next 80 years, while the Burgers’ discovery was almost forgotten.

While qualitatively describing some important turbulence features, the K41 scaling laws led the microscopic theory to a dead end.

The main question: how is the inviscid Navier-Stokes theory different from the Euler theory was unanswered, nor were the other nascent questions like what is the microscopic mechanism of the spontaneous stochasticity?

In the last few years, there has been some progress in understanding the role of the singular topological solutions of the Euler equations, with Burgers solutions resolving singularities. This progress was inspired by the geometric approach to Turbulence initiated in the 80-ties and 90-ties [3,4,5,6], leading to the area law prediction [5].

This prediction was verified recently in DNS [7,8], which triggered a flux of new studies in the geometric theory of Turbulence.

This work was recently revised and summarized in a review paper [9].

Judging by the first responses to this review, this theory is too radical to swallow for the turbulent community. Such a theory needs to be explained and advanced further.

There are many subtleties to be discussed, some minor corrections to be made, and some questions to be answered. Also, there are more data to compare with the Kelvinon theory and the Loop equation solutions.

2. Trying to Bend the Burgers Cylindrical Vortex into a Torus

Let us start with the Burgers vortex solution of the Navier-Stokes equation:

\begin{matrix} \vec{v} = \{- a x - g (r) y, - b y + g (r) x, c z\}; \end{matrix}

(1a)

\begin{matrix} \vec{ω} = \{0, 0, \frac{c Γ_{B} e^{- \frac{c r^{2}}{4 ν}}}{8 π ν}\}; \end{matrix}

(1b)

\begin{matrix} g (r) = \frac{(1 - e^{- \frac{c r^{2}}{4 ν}}) Γ_{B}}{2 π r^{2}}; \end{matrix}

(1c)

\begin{matrix} r = \sqrt{x^{2} + y^{2}}; \end{matrix}

(1d)

\begin{matrix} a = b = c / 2; \end{matrix}

(1e)

This solution describes an infinite cylinder with vorticity decaying as a Gaussian with the width

\begin{matrix} w = \sqrt{2 ν / c}; \end{matrix}

(2)

This constant c represents the strain

\hat{S}

in the direction of the symmetry axis.

\begin{matrix} c = \vec{t} \cdot \hat{S} \cdot \vec{t}; \end{matrix}

(3)

\begin{matrix} \hat{t} = {0, 0, 1}; \end{matrix}

(4)

\begin{matrix} \hat{S} = ∥ S_{α β} ∥ = \frac{\partial_{β} v_{α} + \partial_{α} v_{β}}{2} = diag (- a, - b, c) \end{matrix}

(5)

The parameter

Γ_{B}

represents the circulation in the closed loop surrounding this axis far from its center. By the Stokes theorem, this circulation reduces to an area integral over the surface bounded by this loop, which tends to

Γ_{B}

in the limit when the radius is much larger than the width w of the vorticity core.

This relation can be directly verified by integrating Burgers velocity around the contour and neglecting exponential terms in

g (r)

It is also straightforward to compute anomalous dissipation

\begin{matrix} E = ν \int d^{3} r {\vec{ω}}^{2} = \frac{L c Γ_{B}^{2}}{8 π} \end{matrix}

(6)

where

L \to \infty

is the length of the cylinder.

Let us try to compactify the Burgers vortex by bending a cylinder into the torus. Consider a circular vortex line with a radius R much larger than the thickness w of the viscous core.

Locally, at the distances from the core

r \sim w

, the torus is equivalent to the cylinder, up to higher order terms in

w / R

At larger distances, the vorticity is equivalent to the delta function in the cross-section plane, and velocity is purely potential. It adds up from a linear term and a singular term

\begin{matrix} \vec{ω} (\vec{r}) \to Γ_{B} \vec{t} δ (x) δ (y); \end{matrix}

(7)

\begin{matrix} \vec{v} (\vec{r}) \to (- a x, - b y, c z) + \frac{Γ_{B}}{2 π} \frac{(- y, x, 0)}{x^{2} + y^{2}}; \end{matrix}

(8)

An important property of the Burgers solution is that the strain tensor (5) is constant and has no singularities in the inviscid limit, unlike the rotational part of velocity. This constant strain represents the local value of the nonsingular Euler strain outside the vortex core, continued inside the tube..

The invariant formula for the inviscid limit of vorticity would be the line integral (with l being the length of the line)

\begin{matrix} \vec{ω} (\vec{r}) = \oint_{C} d \vec{C} (l) Γ_{B} (l) δ^{3} (\vec{r} - \vec{C} (l)); \end{matrix}

(9)

\begin{matrix} | {\vec{C}}^{'} (l) | = 1 \end{matrix}

(10)

When the point

\vec{r}

approaches some point

\vec{C} (l_{0})

at the loop, the loop integration cancels the delta function, and one recovers the Burgers delta function in the

x y

plane.

\begin{matrix} \vec{ω} (\vec{r}) \to {\vec{C}}^{'} (l_{0}) Γ_{B} (l_{0}) δ (x) δ (y); \end{matrix}

(11)

\begin{matrix} {\vec{C}}^{'} (l_{0}) = (0, 0, 1); \end{matrix}

(12)

In the linear vicinity of a point

\vec{C} (l_{0})

at this loop, we use the Burgers solution with

z = l - l_{0}

to find the derivatives

\begin{matrix} \partial_{l} Γ_{B} = 0; \end{matrix}

(13a)

\begin{matrix} \partial_{l} \vec{v} (\vec{C} (l)) = \hat{S} (\vec{C} (l)) \cdot {\vec{C}}^{'} (l); \end{matrix}

(13b)

The velocity field here is taken at the loop C, which is a center of the Burgers core.

There is an important boundary condition for the strain tensor at the loop

\begin{matrix} \hat{S} (\vec{C} (l)) \cdot {\vec{C}}^{'} (l) = c (l) {\vec{C}}^{'} (l); \end{matrix}

(14)

In general, the eigenvalue

c (l)

depends on the point l at the loop, as the strain could be a function of coordinates and the loop changes direction. This eigenvalue must be positive.

This boundary condition is an analog of the CVS conditions [9] (confined vortex surface) relating the vortex sheet shape and the boundary value of the strain. In the case of the vortex sheet, this relation imposed restrictions on a surface shape, as the Euler strain did not have any free parameters to adjust.

In the case of the loop, these restrictions can be treated as extra boundary conditions on the more complex Euler flow outside the tube, as we shall see in the next Sections.

These restrictions are quite strong. In the case of a curved loop we are looking for, the constant strain cannot have the loop tangent vector

{\vec{C}}^{'} (l)

as its eigenvector.

Unlike the Burgers formulas, these relations (13) are parametric invariant and do not depend on the coordinate frame. This invariance makes them correct generalizations of Burger’s solution to an arbitrary smooth loop.

These equations can be readily integrated1

\begin{matrix} Γ_{B} (l) = c o n s t; \end{matrix}

(15)

\begin{matrix} \vec{v} (\vec{r} \in C) = \int_{C}^{\vec{r}} \hat{S} ({\vec{r}}^{'}) \cdot d {\vec{r}}^{'} \end{matrix}

(16)

We found a parametric invariant vector integral of the strain along the loop. Another way to derive this formula is as follows:

\begin{matrix} \vec{v} (\vec{r} \in C) = \int_{C}^{\vec{r}} d {\vec{r}}^{'} \cdot \vec{\nabla} \otimes \vec{v} ({\vec{r}}^{'}) = \\ \int_{C}^{\vec{r}} d {\vec{r}}^{'} \cdot \hat{S} ({\vec{r}}^{'}) + \int_{C}^{\vec{r}} d {\vec{r}}^{'} \times \vec{ω} ({\vec{r}}^{'}) \end{matrix}

(17)

The second term here vanishes because the vorticity at the loop

\vec{ω} ({\vec{r}}^{'})

is aligned with its tangent vector

d {\vec{r}}^{'}

From this relation, by integrating by parts, one can derive the following exact relation for the circulation over the large loop C

\begin{matrix} Γ_{C} = \oint_{C} d r_{α} v_{α} = - \oint_{C} r_{α} d v_{α} = - \oint_{C} r_{α} S_{α β} d r_{β} \end{matrix}

(18)

In virtue of the eigenvalue equation, this is also equivalent to

\begin{matrix} Γ_{C} = - \oint_{C} d l {\vec{C}}^{'} (l) \cdot \vec{C} (l) c (l) \end{matrix}

(19)

The generalization of Burger’s anomalous dissipation is also straightforward [9]:

\begin{matrix} E = \frac{Γ_{B}^{2}}{8 π} \oint_{C} d l {\vec{C}}^{'} (l) \cdot \hat{S} \cdot {\vec{C}}^{'} (l) = \frac{Γ_{B}^{2}}{8 π} \oint_{C} d l c (l) \end{matrix}

(20)

The problem we are facing is related to the strain. As we have seen, it cannot be a constant tensor (its highest eigenvector must be equal to the local direction of the loop everywhere).

The generic Euler (singular) velocity field, corresponding to the above singular vorticity line, reads

\begin{matrix} \vec{v} (\vec{r}) \overset{?}{=} \vec{\nabla} Φ - \frac{Γ_{B}}{4 π} \vec{\nabla} \times \oint_{C} \frac{d {\vec{r}}^{'}}{|\vec{r} - {\vec{r}}^{'}|} \end{matrix}

(21)

The harmonic potential

Φ (\vec{r})

must be such that the strain at the loop

\begin{matrix} S_{α β} (\vec{C} (l)) \overset{?}{=} \partial_{α} \partial_{β} Φ (\vec{C} (l)) \end{matrix}

(22)

has the local tangent vector

{\vec{C}}^{'} (l)

as its main eigenvector at every point on the loop.

This main eigenvalue c must be positive. Two lower eigenvalues

- a, - b

do not have to be equal, as it was revealed by a further study of the Burgers vortex. As was shown by Moffat et. al. [10], the vortex solution for a general non-axisymmetric strain tends to the symmetric Burgers solution in the turbulent limit

| Γ_{B} | ≫ ν

We are only interested in that limit, so we can skip the requirement of equal lower eigenvalues.

So, is this it? A weak Euler flow regularized by a Burger vortex core? Not so fast.

This solution would not be valid for an arbitrary smooth loop C, because, in general, the eigenvalue condition will not hold.

We are unaware of any theorems that would prove the existence of the harmonic potential with the prescribed main eigenvector of its Hessian

S_{α β}

on a closed loop C in space. Apparently, such a harmonic potential does not exist for an arbitrary (or even smooth) loop.

3. Matching Principle and Anomalies in the Euler Hamiltonian

The stationary Euler solution we are looking for must minimize the Hamiltonian

\begin{matrix} H = \int_{R^{3}} \frac{{\vec{v}}^{2}}{2} \end{matrix}

(23)

In our case of the singular velocity field, we have to split this energy integral into two parts: inside and outside a thin tube

T

surrounding the loop C. The radius R of a local cross-section of the boundary

\partial T

of this tube must be much larger than Burger’s thickness w but much smaller than the local curvature radius

R_{C}

of the loop C.

Under these conditions, the inside of the tube is described by a cylindrical Burgers vortex, while the outside is some Euler flow in remaining space

G = R^{3} ∖ T

. This remaining space has the topology of the full torus, the same as

T

. We understand the space

R^{3}

is compactified as a sphere

S^{3}

by including the infinity.

At the surface of the tube and its vicinity, for the whole range

w ≪ R ≪ R_{C}

, there must be a match of the inside Burgers flow up to higher order corrections in

w / R

with the (yet unspecified) Euler flow up to

R / R_{C}

corrections.

This is the matching principle, which we suggested first for the vortex sheets, and then for the vortex lines (see [9] and references to earlier work within).

Thus, the Euler Hamiltonian can be written as the sum of two terms

\begin{matrix} H = \int_{G} \frac{{\vec{v}}_{E}^{2}}{2} + \int_{T} \frac{{\vec{v}}_{B}^{2}}{2} \end{matrix}

(24)

The last term is an anomaly; it was calculated in [9]. Up to negligible power corrections in

R / R_{C}, w / R

\begin{matrix} \int_{T} \frac{{\vec{v}}_{B}^{2}}{2} = \frac{Γ_{B}^{2}}{8 π} \oint_{C} d l (γ + log \frac{c (l) R^{2}}{8 ν}); \end{matrix}

(25)

\begin{matrix} c (l) = {\vec{C}}^{'} (l) \cdot \hat{S} (\vec{C} (l)) \cdot {\vec{C}}^{'} (l) \end{matrix}

(26)

The term with

log R

\begin{matrix} \frac{Γ_{B}^{2}}{4 π} \oint_{C} d l log R \end{matrix}

(27)

is canceled by a similar term coming from the Euler field. This cancellation is a consequence of the matching conditions, as discussed in [9]. Here are these calculations. Taking the derivative in R, we find the contribution of the surface

\partial T

\begin{matrix} {\vec{v}}_{E} (\vec{r}) \to \frac{Γ_{B}}{2 π} \frac{(- y, x, 0)}{x^{2} + y^{2}}; \end{matrix}

(28)

\begin{matrix} \partial_{R} \int_{G} \frac{{\vec{v}}_{E}^{2}}{2} = - \int_{\partial T} \frac{{\vec{v}}_{E}^{2}}{2} \to - \frac{Γ_{B}^{2}}{8 π^{2}} \int_{C} d l \frac{2 π}{R} = - \frac{Γ_{B}^{2}}{4 π} \oint_{C} d l \frac{1}{R} \end{matrix}

(29)

which adds up to zero with the derivative of the above

log R

term. Therefore, the sum of these two terms in the Hamiltonian does not depend on R ( up to neglected power corrections).

As a result of this independence, we can take a limit

R \to 0

in the regularized Euler part and finally find the anomalous Hamiltonian

\begin{matrix} H = H_{E} + \frac{Γ_{B}^{2}}{8 π} \oint_{C} d l (γ + log \frac{{c (l) | C |}^{2}}{8 ν}); \end{matrix}

(30a)

\begin{matrix} H_{E} = lim_{R \to 0} (\int_{G} \frac{{\vec{v}}_{E}^{2}}{2} + \frac{Γ_{B}^{2}}{8 π} | C | log \frac{R^{2}}{{| C |}^{2}}); \end{matrix}

(30b)

This Hamiltonian has the common flaw of all Euler flows (except topological ones): it continuously scales down to zero

{\vec{v}}_{E} = 0, Γ_{B} = 0

by changing the scale of the velocity field.

Therefore, the zero velocity provides the absolute minimum of the Hamiltonian unless there are some topological restrictions on a flow, preventing continuous scaling to zero within a topological class.

4. Topological Euler Flow

The way to build topological solutions to the Euler equations was discovered in the eighties [11,12]. These are spherical Clebsch or Faddeev variables, as we suggested in [9]. These variables are elements of 2-sphere geometrically, 3D rigid rotators in the Hamiltonian dynamics, or the

S^{2}

sigma model in the statistical field theory language.

The vorticity is locally parametrized as

\begin{matrix} \vec{ω} = \frac{1}{2} Z e_{a b c} S_{a} \vec{\nabla} S_{b} \times \vec{\nabla} S_{c}; \end{matrix}

(31)

\begin{matrix} S_{1}^{2} + S_{2}^{2} + S_{3}^{2} = 1; \end{matrix}

(32)

where Z is some parameter with the dimension of viscosity, staying finite when

ν \to 0

One could use various coordinates on the sphere. In particular, there are canonical coordinates

\begin{matrix} ϕ_{1} = Z S_{3}; \end{matrix}

(33)

\begin{matrix} ϕ_{2} = arg (S_{1} + ı S_{2}); \end{matrix}

(34)

With these coordinates, the vorticity becomes parametrized as with the ordinary Clebsch variables

\begin{matrix} \vec{ω} = \vec{\nabla} ϕ_{2} \times \vec{\nabla} ϕ_{1} \end{matrix}

(35)

except these two variables

ϕ_{1}, ϕ_{2}

vary on a rectangle

- Z \leq ϕ_{1} \leq Z, - π \leq ϕ_{2} \leq π

rather than the whole plane

R_{2}

The Euler equations are then equivalent to passive convection of the Clebsch field by the velocity field (modulo gauge transformations, as we argue in [9]):

\begin{matrix} \partial_{t} ϕ_{a} = - \vec{v} \cdot \vec{\nabla} ϕ_{a} \end{matrix}

(36)

\begin{matrix} \vec{v} = {(ϕ_{2} \vec{\nabla} ϕ_{1})}^{⊥}; \end{matrix}

(37)

Here

V^{⊥}

denotes projection to the transverse direction in Fourier space, or:

V_{α}^{⊥} (r) = V_{α} (r) + \partial_{α} \partial_{β} \int d^{3} r^{'} \frac{V_{β} (r^{'})}{4 π | r - r^{'} |}

(38)

As we can see from this representation of velocity, it has a gap

\begin{matrix} Δ \vec{v} (S) = 2 π n \vec{\nabla} ϕ_{1} (S) \end{matrix}

(39)

at the surface where the phase

ϕ_{2}

has the gap

Δ ϕ_{2} = 2 π n

This surface is bounded by a singular line C where the angular velocity in the transverse plane diverges as

1 / r

, preserving a finite circulation for an infinitesimal dual loop

δ C

encircling C (Figure 1).

The velocity gap must vanish at the edge of the surface, which requires the boundary condition

\begin{matrix} \vec{\nabla} S_{3} (C) = 0; \end{matrix}

(40)

The velocity itself must be directed along the edge, i.e., parallel to the tangent vector

{\vec{C}}^{'} (l)

of the loop

\begin{matrix} \vec{v} (\vec{C} (l)) ‖ {\vec{C}}^{'} (l) \end{matrix}

(41)

This tube cross-section’s boundary

δ C

is mapped on the loop

γ

S^{2}

, covered n times. Topologically, a circle is mapped on a circle with homotopy

π_{1} (S_{1}) = Z

The circulation

\oint_{δ C} v_{α} d r_{α}

is related to the vorticity flux through the tube’s cross-section, which equates it to the Burgers parameter

Γ_{B}

The circulation around a loop on a 2-sphere equals the area of one of two complementary spherical caps

Ω_{\pm}; \partial Ω_{\pm} = γ

, depending upon the orientation of

γ

, i.e., the sign of the winding number n (see Figure 2).

In our coordinates, this loop

γ

is some horizontal circle

S_{3} = cos λ = const

\begin{matrix} Γ_{B} = \oint_{δ C} v_{α} d r_{α} = \int_{Ω_{\pm}} d ϕ_{2} \land d ϕ_{1} = 2 π n Z (1 - sign n cos λ); \end{matrix}

(42)

The boundary value of

S_{3} (C) = cos (λ)

remains as a constant parameter of our Clebsch field, to be determined later from the minimization of the Hamiltonian.

There is, of course, a possibility to get zero circulation (and, therefore, zero velocity, in case

Z = 0

cos λ = sign n

Let us now turn to the circulation

Γ_{C}

around the original loop. In the same way, as with the Burgers circulation

Γ_{B}

, this circulation can be written as a vorticity flux through some Stokes surface bounded by the loop and passing through the Euler region.

A small part of this surface will pass through the Burgers tube. As the vorticity inside the Burgers tube is directed towards its axis, we can choose this surface to pass this tube in the local tangent plane to the loop. The normal to this local tangent plane

\vec{n} ‖ {\vec{C}}^{'} (l) \times \vec{C} " (l)

is orthogonal to the direction of the vorticity

\vec{ω} ‖ {\vec{C}}^{'} (l)

Therefore, the flux is determined solely by the vorticity in the Euler region, which means that the singular-line solution (21) with potential flow outside the loop does not provide a finite circulation

Γ_{C}

This requirement is the ultimate reason for the topological solution.

There is no contradiction at this level with the Clebsch field, but there is an interesting relation based on the Stokes theorem.

Let us compute the Euler flux through the discontinuity surface on the upper side

S_{C}^{+}

. There is only a tangent discontinuity of velocity at the surface

S_{C} ∖ C

, coming from the delta function in vorticity. The boundary values are

\begin{matrix} \vec{ω} \to 2 π n δ (z) \vec{σ} \times \vec{\nabla} ϕ_{1}; \end{matrix}

(43)

\begin{matrix} {\vec{v}}^{+} - {\vec{v}}^{-} = 2 π n \vec{\nabla} ϕ_{1}; \end{matrix}

(44)

\begin{matrix} S_{3}^{+} = S_{3}^{-}; \end{matrix}

(45)

\begin{matrix} ϕ_{2}^{+} = ϕ_{2}^{-} - 2 π n; \end{matrix}

(46)

\begin{matrix} ω_{n}^{+} = ω_{n}^{-} = - \vec{σ} \cdot \vec{\nabla} ϕ_{2}^{\pm} \times \vec{\nabla} ϕ_{1}^{\pm} \end{matrix}

(47)

Here

\vec{σ}

is the local normal vector to the surface, and z is the normal coordinate.

The flux through the surface is

\begin{matrix} Γ_{C} = \int_{S_{C}^{+}} d \vec{σ} \cdot {\vec{ω}}^{+} = \int_{Ω_{\pm}} d ϕ_{1} \land d ϕ_{2} = 2 π m Z (1 - sign m cos λ); \end{matrix}

(48)

where

m \in Z

is a winding number for

ϕ_{2}

around the loop C.

These two winding numbers

n, m

are consistent with the boundary condition [9] at the surface

\partial T

of the infinitesimal tube

T

\begin{matrix} ϕ_{2} (\vec{C} (l) + \vec{ξ}) = m α + n β; \end{matrix}

(49a)

\begin{matrix} \vec{ξ} = ϵ (\vec{n} cos β + \vec{σ} sin β); \end{matrix}

(49b)

\begin{matrix} \vec{n} = \frac{{\vec{C}}^{''}}{| {\vec{C}}^{''} |}; \end{matrix}

(49c)

\begin{matrix} \vec{σ} = {\vec{C}}^{'} \times \vec{n}; \end{matrix}

(49d)

\begin{matrix} α = \frac{2 π l}{| C |}; \end{matrix}

(49e)

\begin{matrix} | C | = \oint_{C} d l; \end{matrix}

(49f)

Here,

ϵ \to 0

is the radius of the tube.

Let us briefly discuss the topology of the Kelvinon. The equation (49a) implements the mapping of a torus on a circle with homotopy

π_{1} (T^{2}) ≅ Z \times Z

, which corresponds to a pair of integer winding numbers

n, m \in Z

What about mapping the 3D space by the Clebsch field

S_{a} (\vec{r})

The Hopf mapping of the compactified 3-space to a 2-sphere,

S^{3} \mapsto S^{2}

, was already implemented by a spherical Clebsch field in [11,12], but our Kelvinon is different.

The Kelvinon implements a mapping of the compactified 3-space

G ≅ S^{3}

without a monopole ring

T ≅ T^{3}

onto the spherical cap

Ω_{\pm} ≅ D^{2}

rather than the full sphere

S^{2}

Topologically,

\begin{matrix} (G ≅ S^{3} ∖ T^{3} ≅ T^{3}) \mapsto D^{2}; \end{matrix}

(50)

As we have seen, this mapping is described by two winding numbers. Depending upon the signs of these winding numbers, the Clebsch field maps physical space on one of the two complementary caps on a sphere separated by a circle

S_{3} = cos λ

The Appendix presents a family of smooth Clebsch fields with desired properties, including winding numbers

n, m

and a decrease of vorticity at infinity. We do not compute velocity for these examples, as it would require a solution of a nontrivial Neumann problem on a minimal surface bounded by an arbitrary smooth loop C.

The vorticity is shown as a vector flow in 3D in Figure 3,Figure 4,Figure 5. The Mathematica^®code producing these plots is published on Wolfram Cloud [13].

Comparing the expression (48) to Burger’s contribution to the line integral of velocity at the center of the core of the tube, we get a self-consistency relation between parameters of the Euler flow

\oint_{C} r_{α} S_{α β} d r_{β} = - 2 π m Z (1 - sign m cos λ);

(51)

This relation being linear, the normalization factor Z can be canceled so that this is a restriction on a Faddeev vector field

S_{a} (\vec{r})

. The eigenvalue equation (14) also restricts the field

S_{a} (\vec{r})

, leaving the normalization factor arbitrary.

As suggested in [9], this factor will be determined from the energy balance between the incoming energy flow and anomalous dissipation.

There is some advance we have recently made in this part of the Kelvinon theory; we present it in the next Section.

5. Energy Balance Revisited

For every stationary solution of the Navier-Stokes equation, the time derivative of any functional of the velocity field, including the Euler Hamiltonian, must vanish.

Our matching principle suggests using this Navier-Stokes relation to fix the remaining free parameter Z of the Euler flow in the Clebsch variables.

The energy dissipation is localized in the Burgers vortex core. We know it in an inviscid limit as a functional of the local Euler strain at the loop (20). At fixed Clebsch field

S_{a} (\vec{r})

, this dissipation is proportional to

Z^{3}

(two powers of Z from circulation

Γ_{B}

and one from the strain.)

The matching energy pumping into this region can be written in many forms. In the review paper [9], we used the energy flux through the boundary of the volume V, which flux we then estimated as

ϵ V

There is a more direct approach, using notorious external random forces

\vec{f} (\vec{r})

, which we take as a random uniform vector inside this volume.

This point needs clarification. Usual forces are also a function of time, correlated by a

δ (t - t^{'}) f (\vec{r} - {\vec{r}}^{'})

with some slow function of space distance

\vec{r} - {\vec{r}}^{'}

. Then the time averaging is assumed, leading to some expressions for the equal time velocity correlations.

In our approach, we replace time averages with ensemble averages. We have an ensemble of stationary flows, each with its uniform force

\vec{f}

drawn from a Gaussian distribution. Our force is a space-independent Gaussian random vector with delta variance

\begin{matrix} 〈f_{α} f_{β}〉 = σ δ_{α β} \end{matrix}

(52)

This ensemble averaging is equivalent to time averaging with delta-correlated Gaussian force (such force is a different sample of a Gaussian vector at different times).

The energy pumping created by such a force inside a volume V before Gaussian averaging is simply the work made by this force over the net momentum of the fluid inside this volume

\begin{matrix} E = \int_{V} δ \vec{v} \cdot \vec{f}; \end{matrix}

(53)

This perturbed velocity

δ \vec{v}

, in turn, depends on the force through the Navier-Stokes equation. We shall assume this relation to be linear, and later we justify that assumption in the inviscid limit utilizing asymptotic freedom.

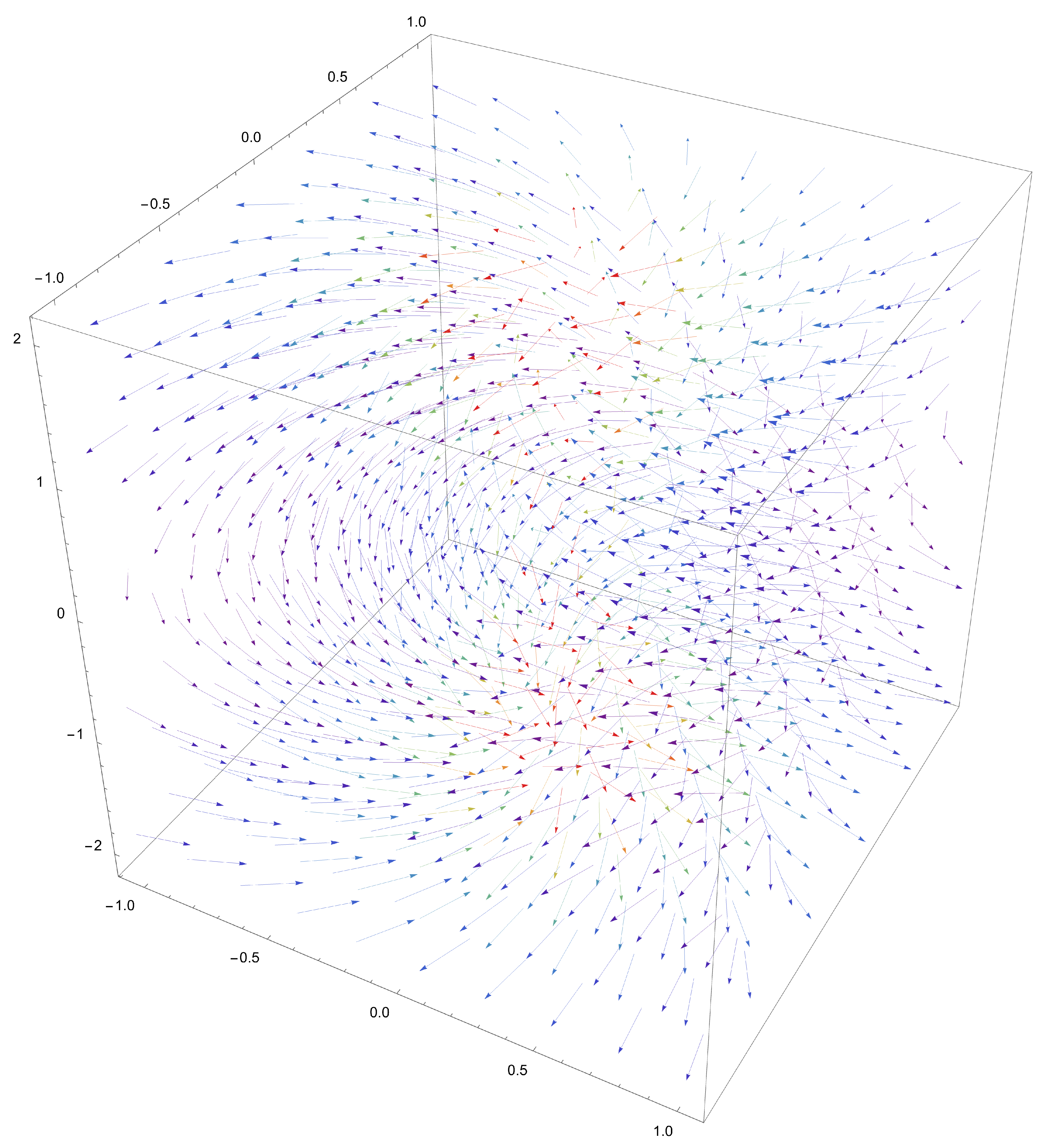
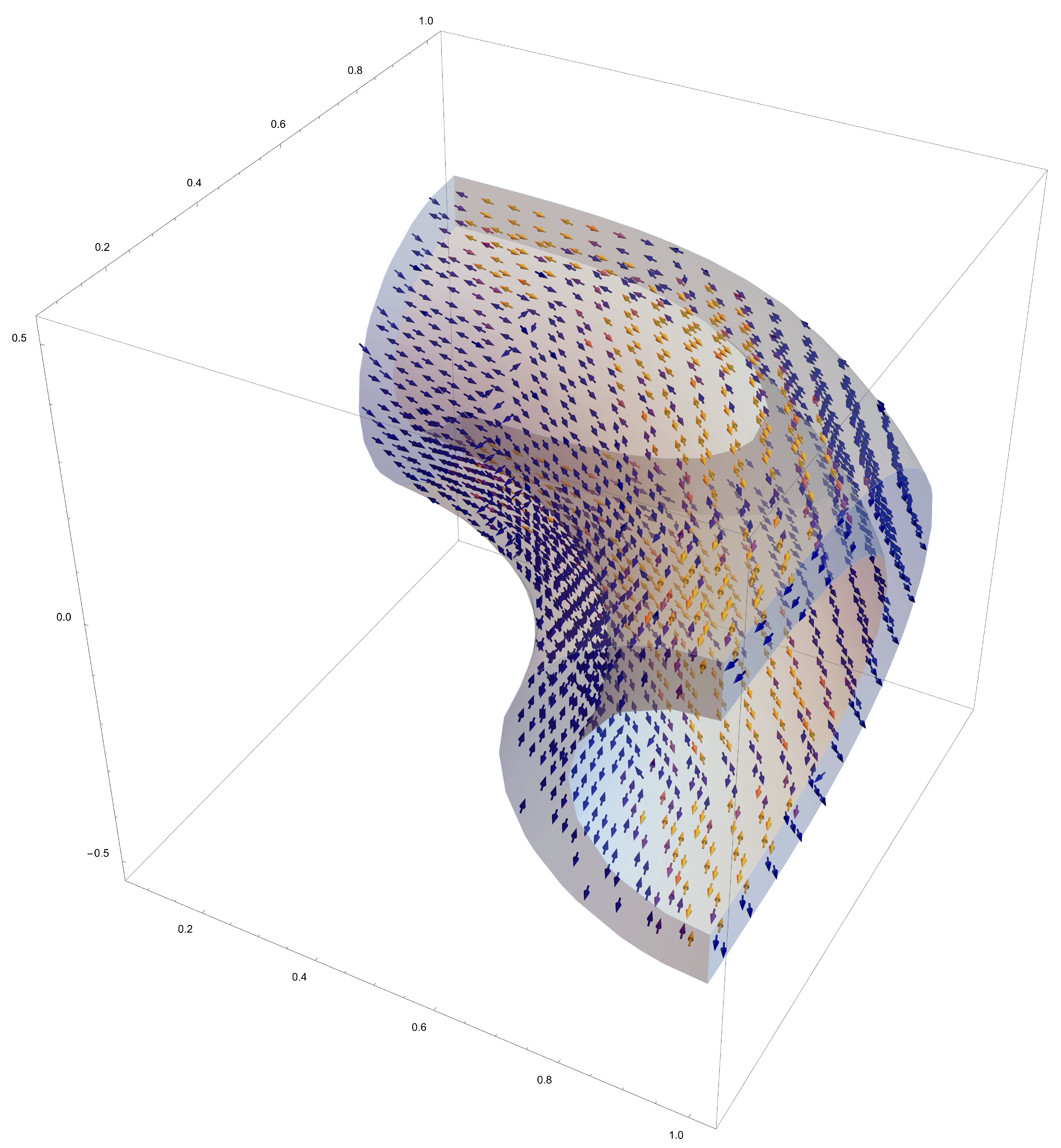
Figure 3. The stream plot of the 3D vorticity field from the topological example of the Kelvinon field is in Appendix.

Figure 4. A toroidal layer far from the core of the stream plot of the 3D vorticity field from the topological example of the Kelvinon field in the Appendix.

Figure 5. A toroidal layer close to the core of the stream plot of the 3D vorticity field from the topological example of the Kelvinon field in the Appendix.

In the linear approximation, we have to solve linearized Euler equations for

δ \vec{v}, δ p

\begin{matrix} \hat{G} \cdot δ \vec{v} + \vec{\nabla} δ p = \vec{f}; \end{matrix}

(54a)

\begin{matrix} {\hat{G}}_{α β} = (\partial_{β} v_{α}) \hat{I} + δ_{α β} v_{γ} \partial_{γ} \end{matrix}

(54b)

\begin{matrix} {\vec{\nabla}}^{2} δ p = - \vec{\nabla} \cdot \hat{G} \cdot δ \vec{v}; \end{matrix}

(54c)

Symbolically, we can write the result

\begin{matrix} E = \vec{f} \cdot \hat{Q} \cdot \vec{f}; \end{matrix}

(55)

\begin{matrix} \hat{Q} = \int_{V} \int_{V} d^{3} \vec{r} d^{3} {\vec{r}}^{'} {(\hat{G} - \frac{1}{{\vec{\nabla}}^{2}} \vec{\nabla} \otimes \vec{\nabla} \cdot \hat{G})}^{- 1} (\vec{r}, {\vec{r}}^{'}) \end{matrix}

(56)

Let us count the factors of Z here, assuming

\vec{f}

to be

O (1)

. As we have shown in [9] (see also the next Section), in the inviscid limit, there is asymptotic freedom: Z grows as a power of the logarithm of the effective Reynolds number

\begin{matrix} Z \sim {(log Rey)}^{\frac{1}{3}} \to \infty; \end{matrix}

(57)

\begin{matrix} Rey = \frac{{〈c〉}_{C} {| C |}^{2}}{ν} \end{matrix}

(58)

Then, we have

\hat{G} \sim Z, \hat{Q} \sim 1 / Z

in the (55).

This makes the correction

δ \vec{v} \sim 1 / Z

much smaller than

\vec{v} \sim Z

δ \vec{v} / \vec{v} \sim Z^{- 2} \sim {(log Rey)}^{- \frac{2}{3}}

(59)

Now we have justified linear approximation for the perturbation of the Euler equation by an external pumping force.

In general, asymptotic freedom in Turbulence makes the fluctuations of the velocity field around Kelvinon go to zero as a power of the logarithm of the Reynolds number.

The same thing happens in QCD with fluctuations of the gluon field around the instanton: these fluctuations logarithmically die out compared to the instanton field.

As the background velocity fluctuation

{\vec{v}}_{0} = {〈δ \vec{v}〉}_{V}

is a linear function of

\vec{f}

, the Gaussian distribution of

\vec{f}

is equivalent to the Gaussian distribution of

{\vec{v}}_{0}

, which was considered in [9]. The difference is that we now have a microscopic equation (54), which allows us to compute this background velocity field once the base Kelvinon flow is known.

Let us turn back to the energy balance. Naturally, only the total energy of the Navier-Stokes flow is stationary. There are some contributions

E_{d}^{'}, E_{p}^{'}

from the remainder of the fluid both to the energy pumping

E_{p}

and to the energy dissipation

E_{d}

in the energy balance equations

\begin{matrix} E_{p}^{'} + \vec{f} \cdot \hat{Q} \cdot \vec{f} - σ tr \tilde{Q} = E_{d}^{'} + \frac{Γ_{B}^{2}}{8 π} \oint_{C} d l c (l); \end{matrix}

(60)

(61)

where we subtracted the mean over the random forces from the

\tilde{Q}

term to satisfy the mean energy balance. The outside volume energy flow components

E_{d}^{'}, E_{p}^{'}

are treated as constants rather than random numbers (self-averaging of the random forces acting in the remaining infinite volume.)

We have to factor our Z and solve the resulting equation (with variables

\tilde{X}

corresponding to X with

Z = 1

)

\begin{matrix} Z^{3} A = B + \frac{1}{Z} (\vec{f} \cdot \tilde{Q} \cdot \vec{f} - σ tr \tilde{Q}); \end{matrix}

(62a)

\begin{matrix} A = \frac{{\tilde{Γ}}_{B}^{2}}{8 π} \oint_{C} d l \tilde{c} (l); \end{matrix}

(62b)

\begin{matrix} B = E_{p}^{'} - E_{d}^{'}; \end{matrix}

(62c)

The unknown parameter B here can be estimated as excessive energy pumped into our volume to be dissipated inside the singular vorticity tube. This parameter is proportional to the missing volume in each of the energy flows

E_{d}^{'}, E_{p}^{'}

\begin{matrix} B \approx ϵ V [C] \propto {ϵ | C |}^{3} \end{matrix}

(63)

where

ϵ

is a Kolmogorov energy flow per unit volume and

V [C]

is the volume occupied by our soliton around the singular loop C.

The last estimate

V \propto {| C |}^{3}

implies that the loop has only one scale, which can be taken as its length C.

There are two scales for a large, almost flat loop with small normal deviations: the minimal area

| S [C] |

and the width

Δ

of vorticity field distribution around this surface. In this case

V [C] \sim | S [C] | | Δ |

We postpone the discussion of large flat loops to the next section.

The solution of this quartic equation at small A equals to

\begin{matrix} Z \to {(\frac{B}{A})}^{\frac{1}{3}} + \vec{f} \cdot \hat{M} \cdot \vec{f} - σ tr \hat{M}; \end{matrix}

(64a)

\begin{matrix} \hat{M} = \frac{\tilde{Q}}{3 B}; \end{matrix}

(64b)

\begin{matrix} A \sim \frac{1}{log Rey} \to 0 \end{matrix}

(64c)

6. Asymptotic Freedom Revisited

Now we can revisit and extend the analysis of [9] of asymptotic freedom (logarithmic decrease of running coupling constant of the Kelvinon theory with the local Reynolds number).

Let us elaborate on the relation (62b). Using the basic formula (42), we find

\begin{matrix} A = g \oint_{C} d l \tilde{c} (l); \end{matrix}

(65a)

\begin{matrix} g = \frac{π n^{2} {(1 - sign n cos λ)}^{2}}{2}; \end{matrix}

(65b)

\begin{matrix} S_{3} (\vec{C} (l)) = cos λ; \forall l; \end{matrix}

(65c)

\begin{matrix} {\tilde{S}}_{α β} (\vec{C} (l)) C_{β}^{'} (l) = \tilde{c} (l) C_{α}^{'} (l); \end{matrix}

(65d)

\begin{matrix} \oint r_{α} d r_{β} {\tilde{S}}_{α β} = - 2 π m (1 - sign m cos λ); \end{matrix}

(65e)

\begin{matrix} {\tilde{S}}_{α β} (\vec{r}) = \frac{\partial_{α} \tilde{v_{β}} + \partial_{β} \tilde{v_{α}}}{2}; \end{matrix}

(65f)

\begin{matrix} \tilde{v_{α}} = {(ϕ_{2} \partial_{α} S_{3})}_{⊥}; \end{matrix}

(65g)

The variables with tilde

\tilde{S}, \tilde{v}, \dots

correspond to the normalized flow, with

Z \Rightarrow 1

Factoring out the

Z -

dependence in the anomalous Euler Hamiltonian (30), we find

\begin{matrix} H = Z^{2} (\tilde{H} + g | C | log \frac{Z}{ν}); \end{matrix}

(66a)

\begin{matrix} \tilde{H} = g \oint_{C} d l (γ + log \frac{\tilde{c} (l) {| C |}^{2}}{8}) + lim_{R \to 0} (\int_{P} \frac{{\tilde{v}}_{E}^{2}}{2} + g | C | log \frac{R^{2}}{{| C |}^{2}}); \end{matrix}

(66b)

\begin{matrix} Z = {(\frac{ϵ V [C]}{\oint_{C} d l \tilde{c} (l)})}^{\frac{1}{3}} g^{- \frac{1}{3}} \end{matrix}

(66c)

We note that this factor g depends on only one dynamical variable: the boundary value

S_{3} (C) = cos λ

. The Clebsch fields

ϕ_{2}, S_{3}

outside the loop C satisfy the passive advection equations (36). The boundary value of the strain

\tilde{S}

satisfies the boundary conditions (65).

The parameter g must minimize the Hamiltonian, with fixed loop C and fixed Kolmogorov constant

ϵ

Varying the Hamiltonian (66) with respect to g, we find the transcendental equation for

g, Z

\begin{matrix} g = \frac{g_{0}}{- 1 + log \frac{Z}{ν}}; \end{matrix}

(67)

\begin{matrix} g_{0} = \frac{2 \tilde{H}}{| C |}; \end{matrix}

(68)

\begin{matrix} (- 1 + log \frac{Z}{ν}) Z^{3} = ϵ R^{4} [C]; \end{matrix}

(69)

\begin{matrix} R^{4} [C] = \frac{g_{0} V [C]}{\oint_{C} d l \tilde{c} (l)} \end{matrix}

(70)

This

R [C]

is a functional of the loop C. By dimensional counting, it scales as

| C |

\begin{matrix} R [C] = | C | f [\frac{C}{| C |}] \end{matrix}

(71)

This estimate assumes that there are no other scales in the solution. As mentioned above, for the smooth, almost flat loop C, there may be an effective scale in the normal direction to the minimal surface.

Neglecting such a case (it will be considered in the next Section), we can take

R = R [C]

as a definition of the loop scale and write down the "renormalization group" equation for effective coupling

g_{R} = \frac{g}{g_{0}}

\begin{matrix} \frac{d g_{R}}{d log R} = β (g_{R}); \end{matrix}

(72)

\begin{matrix} β (g_{R}) = - \frac{4 g_{R}^{2}}{3 + g_{R}}; \end{matrix}

(73)

This beta function corresponds to the transcendental equation for

g_{R}

\begin{matrix} \frac{3}{g_{R}} - log g_{R} = - 3 + 4 log (\frac{ϵ^{\frac{1}{4}} R}{ν^{\frac{3}{4}}}) \end{matrix}

(74)

The beta function and the solution for

g_{R} (log R)

are plotted in Figure 6. There are no roots of this beta function; it monotonously goes to

- \infty

The roots of the beta function would correspond to the fixed point of RG, leading to scaling laws with anomalous dimensions. That would justify the traditional multifractal scaling laws. However, in our theory, the beta function is calculable in explicit form and does not have a root.

Therefore, we have asymptotic freedom instead of the usual multifractal scaling laws. As discussed below, the difference is hard to observe in DNS and even harder in real experiments on Earth.

Now, with asymptotic freedom, we can justify the linearization of the Euler equation in the presence of fixed external random force: this is the leading term of expansion in our running coupling constant.

In the same leading approximation, the Z factor grows as a power of

log Rey

\begin{matrix} Z \to {(\frac{ϵ}{3} R^{4} log (\frac{ϵ R^{4}}{ν^{3}}))}^{\frac{1}{3}} \end{matrix}

(75)

7. Multifractals

For a purely historic reason, the multifractals are assumed to be pure scaling laws for the moments of the velocity differences, or circulation, in our case, as a function of the scale R.

Parisi and Frisch [14] borrowed this idea from Conformal Field Theory, where the moments of similar structure functions are described by scaling laws with anomalous dimensions.

However, this was only half of the story, and the other half was somehow dropped.

In the conformal field theory of critical phenomena in statistical mechanics, the anomalous dimensions are universal functions of the normal dimension of the fluctuating variable (power of circulation in our case).

At the same time, in asymptotically free theories, like QCD, the anomalous dimensions depend on the so-called running coupling constant, which tends to zero inversely proportional to the logarithm of scale.

So, the multifractal as a critical phenomenon has some precedent in statistical and quantum field theory. However, it is a dynamic question of whether the effective coupling is a universal number (a simple root of the beta function at finite coupling constant in the case of conformal theory) or it is running to zero like in asymptotically free theories (the double root of the beta function at zero coupling constant).

We can now answer that question for the Kelvinon theory in favor of asymptotic freedom[9].

Our asymptotic freedom does not correspond to small velocity. On the contrary, velocity is large while its fluctuations are small; the same happens with the gluon field around instanton in QCD.

The unexpected phenomenon in Turbulence is that the powers of the Reynolds number do not appear in our expansion. In the inviscid limit, the positive powers of viscosity become negligible, and the convergence to this limit slows down to the inverse powers of the logarithm of the viscosity.

This expansion in the zeroth approximation involves the nontrivial Kelvinon field

S_{a} (\vec{r}) \in S^{2}

, mapping the physical space (with added infinity and removed loop C) onto the spherical cap

Ω_{\pm} \in S^{2}

There are two possibilities for the orientation

sign n = \pm 1

, corresponding to an upper or lower cap on a sphere. These two possibilities are equivalent as they correspond to the reflection

S_{3} \Rightarrow - S_{3}

. In the asymptotically free limit, the boundary value

S_{3} (C) = cos λ

tends to the North or South pole, depending upon the sign of n.

\begin{matrix} cos λ = sign n + O ({(log Rey)}^{- \frac{1}{3}}) \end{matrix}

(76)

This asymptotic formula will be compatible with our self-consistency relation (51) for the circulation

Γ_{C}

only if

sign m = - sign n

. Then both sides of this equation are

O (1)

when the running coupling constant

g_{R}

goes to zero.

\begin{matrix} \oint_{C} r_{α} {\tilde{S}}_{α β} d r_{β} = - 2 π m (1 + sign n cos λ) \to - 4 π m; \end{matrix}

(77)

In this case, with the

\vec{f}

correction in the linear approximation:

\begin{matrix} Γ_{C} \to 4 π m Z \to 4 π m ϵ^{\frac{1}{3}} R^{\frac{4}{3}} g_{R}^{- \frac{1}{3}} + 4 π m (\vec{f} \cdot \hat{M} \cdot \vec{f} - σ tr \hat{M}) \end{matrix}

(78a)

This expression for the velocity circulation has the same structure as the one in [9]

\begin{matrix} Γ_{C} = τ + \vec{ξ} \cdot \hat{q} \cdot \vec{ξ}; \end{matrix}

(79a)

\begin{matrix} \vec{ξ} \sim N (0, 1); \end{matrix}

(79b)

\begin{matrix} τ = 4 π m ϵ^{\frac{1}{3}} R^{\frac{4}{3}} g_{R}^{- \frac{1}{3}} - 4 π m σ tr \hat{M}; \end{matrix}

(79c)

\begin{matrix} \hat{q} = 4 π m σ \hat{M}; \end{matrix}

(79d)

The circulation shift

τ

shows the logarithmic growth with the scale R through the running coupling

g_{R}^{- \frac{1}{3}} \sim {(log R)}^{\frac{1}{3}}

, but the parabolic term

\vec{ξ} \cdot \hat{q} \cdot ξ

does not depend on the running coupling constant.

According to [9], this corresponds to the circulation PDF

\begin{matrix} W (Γ) \propto \frac{1}{\sqrt{| Γ - τ |}} exp (- \frac{| Γ - τ |}{q_{0}}) \end{matrix}

(80)

where

q_{0} > 0

is the leading eigenvalue of the

3 \times 3

matrix

\hat{q}

The effective fractal dimension for higher moments, corresponding to the saddle point in the integral for the moments

\begin{matrix} 〈Γ^{p}〉 \propto \int d Γ Γ^{p} W (Γ) \to saddle point; \end{matrix}

(81)

\begin{matrix} λ (p, log R) \equiv \frac{\partial log 〈Γ^{p}〉}{\partial log R} \to p \frac{\partial log q_{0}}{\partial log R} + (1 + \frac{1}{2 p}) \frac{\partial (τ / q_{0})}{\partial log R}; if p > p_{c}; \end{matrix}

(82)

If there is no internal scale in a Kelvinon, the parameter R is proportional to the size of the loop

| C |

, and the area inside the loop scales as

R^{2}

. In that region, we have a K41 formula for

λ

, up to higher corrections in asymptotically free coupling constant

g_{R}

\begin{matrix} λ (p, log R) \to p \frac{\partial log τ}{\partial log R} \to \frac{4 p}{3} if p < p_{c}; \end{matrix}

(83)

As it was shown in [9] from an alternative dynamical theory (loop equations), the asymptotic dependence of the circulation PDF in the true inertial range

| Γ | ≫ ν, ϵ^{\frac{1}{3}} {| R |}^{\frac{4}{3}} ≫ ν

must tend to the scaling law

Γ \sim \sqrt{| S_{m i n} [C] |}

, where

| S_{m i n} [C] |

is the minimal area of the surface bounded by C.

In our context, this means that

R^{\frac{4}{3}}

is to be replaced by

\sqrt{| S_{m i n} |}

As for the next correction in

λ (p, log \sqrt{| S_{m i n} |})

at large p, it depends upon the dimensionless ratio

τ / q_{0}

Asymptotic freedom tells us this ratio goes to infinity as

g_{R}^{- \frac{1}{3}}

. Differentiating that and using the RG equation, we find

\begin{matrix} \frac{τ}{q_{0}} \propto g_{R}^{- \frac{1}{3}}; \end{matrix}

(84)

\begin{matrix} \frac{\partial (τ / q_{0})}{\partial log R} \to g_{R}^{\frac{2}{3}} (const + O (g_{R})) \end{matrix}

(85)

Thus, our prediction for the asymptotic behavior of the running index

λ (p, log \sqrt{| S_{m i n} |})

\begin{matrix} λ (p > p_{c}, log \sqrt{| S_{m i n} |}) \to p + const (1 + \frac{1}{2 p}) g_{R}^{\frac{2}{3}}; \end{matrix}

(86)

(87)

where

g_{R}

is related to

log \sqrt{| S_{m i n} |}

by a transcendental equation (74).

We do not have enough data to compare this formula for varying

log \sqrt{| S_{m i n} |}

. However, we have the data obtained for the fractal dimension of classical [7,8] and quantum [15] circulation in turbulent flows.

These authors used a square loop C with a variable side a and fitted their data to constant values of

λ (p)

for each p over the inertial range of

log a

, neglecting possible systematic deviations from that fit.

In the future, it would be interesting to fit that data by our formula with the running coupling constant

g_{R} (log a)

Here are the DNS data from these papers, fitted by our formula with constant

g_{R}

Figure 7, Figure 8. The more detailed analysis of underlying data may reveal this slow dependence of

log a

We are not trying to undermine the important numerical work [7,8,15] and successful phenomenological models [16,17] explaining these data by approximating the chain of the Hopf equation for velocity moments.

The phenomenological theory cannot distinguish between the constant fractal dimensions and those running with logarithms of scale; this is a task for a microscopic theory.

The microscopic theory so far says nothing about the transient region

p \sim 5

where both asymptotic laws break. Only the phenomenological theory [16,17] accurately describes the fractal dimensions (for velocity differences only !) in the whole domain of small and large p.

8. Conclusions

Here is what we added to the previous results published in the review paper [9].

We clarified the topology of the Kelvinon. Its boundary value $ϕ_{2} (\partial T)$ at the surface of the infinitesimal tube $T$ surrounding the singular line C maps a torus on a circle, which mapping is described by two integer winding numbers related to velocity circulations around two cycles of the torus.
The 3D field $S_{a} (\vec{r})$ maps the compactified 3-space without the infinitesimal tube $T$ onto one of the two caps on a sphere $S^{2}$ separated by the circle $γ : S_{3} = const$ .
We modified the energy balance analysis of [9] using conventional random forces and expanding the energy pumping into the Kelvinon in series in the running coupling constant $g_{R} \sim 1 / log R$ . This approach gives us a microscopic definition and corrects the $log R$ dependence of the phenomenological parameters in the circulation PDF tails [9].
We studied the vorticity field in the topological family of Kelvinons and presented 3D stream plots of vorticity Figure 3,Figure 4,Figure 5.
We found the self-consistency conditions for the Kelvinon field (51), (14) from the matching conditions with the Burgers vortex, overlooked in the [9].
Using these conditions, we removed the ambiguity in relative signs of the winding numbers $n, m$ : they must have opposite signs.
We computed and compared predictions of the Kelvinon theory for the fractal dimension $λ (p, log r)$ in the leading perturbation expansion in $1 / log r$ with the DNS data (Figure 7, Figure 8) with a good fit except for the transient region $4 < p < 7$ .

In conclusion, we suggested a microscopic quantitative approach to the turbulence problem, and we presented some predictions for the multifractal indexes, modified by powers of the logarithm of the scale.

Here is what still needs to be elaborated and clarified.

The self-consistency conditions (51), (14) need to be investigated further. Presumably, the boundary values of the Clebsch field on each side of the discontinuity surface provide the set of free parameters needed to satisfy these self-consistency conditions.
The notion of the region occupied by Kelvinon needs to be clarified and defined unambiguously. With correct definition, observable results should not depend upon the shape of the boundary of this region, and its volume should be a well-defined functional of the loop C.
Higher correction in perturbation expansion in the running coupling constant $g_{R}$ need to be computed; fractal dimensions should become universal functions of the logarithm of scale without any phenomenological parameters to fit the DNS data.

Data Availability Statement

Data sharing does not apply to this article, as no new data were created or analyzed in this study.

Acknowledgments

I benefited from discussions of this theory with my friends and colleagues: Konstantin Khanin, Camillo De Lellis, Nader Masmoudi, Sasha Polyakov, Karim Shariff, Katepalli Sreenivasan, Grigory Volovik, Pavel Wiegmann and Victor Yakhot. I am also grateful to Kartik Iyer and Juan Ignacio Polanco for providing me with some data from their DNS of classical and quantum Turbulence. This research was supported by a Simons Foundation award ID 686282 at NYU Abu Dhabi.

Appendix Topological family of the Kelvinon fields

Let us present an explicit example of the Clebsch field with the required topology, which could serve as initial data for the Hamiltonian minimization by relaxation.

We introduce a surface of the minimal area bounded by our loop C

\begin{matrix} S_{m i n} (C) = \underset{S : \partial S = C}{arg min} \int_{S} d S \end{matrix}

(A1)

For every point

\vec{r} \in R_{3}

, there is the nearest point

{\vec{r}}_{1}

at the minimal surface

S_{m i n} (C)

\begin{matrix} {\vec{r}}_{1} = \underset{{\vec{r}}^{'} \in S_{m i n} (C)}{arg min} {(\vec{r} - {\vec{r}}^{'})}^{2}; \end{matrix}

(A2)

For this point

{\vec{r}}_{1}

at the surface there is also a nearest point

{\vec{r}}_{0}

at its edge C, minimizing the geodesic distance

d (a, b)

along the surface from

{\vec{r}}_{1}

to the edge

\begin{matrix} s_{0} = \underset{s}{arg min} d ({\vec{r}}_{1}, \vec{C} (s)); \end{matrix}

(A3)

\begin{matrix} {\vec{r}}_{0} = \vec{C} (s_{0}); \end{matrix}

(A4)

Let us also introduce the local frame with vectors

\vec{t} (s), \vec{n} (s), \vec{σ} (s)

at the loop:

\begin{matrix} {\vec{C}}^{'} {(s)}^{2} = 1; \end{matrix}

(A5a)

\begin{matrix} \vec{t} (s) = {\vec{C}}^{'} (s); \end{matrix}

(A5b)

\begin{matrix} \vec{n} (s) = \frac{{\vec{C}}^{''} (s)}{| {\vec{C}}^{''} (s) |} \end{matrix}

(A5c)

\begin{matrix} \vec{σ} (s) = \vec{t} (s) \times \vec{n} (s); \end{matrix}

(A5d)

Our field is then defined as follows:

\begin{matrix} α = \frac{2 π s_{0}}{\oint | d \vec{C} |}; \end{matrix}

(A6a)

\begin{matrix} β = arg ((\vec{r} - {\vec{r}}_{0}) \cdot (\vec{n} (s_{0}) + ı \vec{σ} (s_{0}))); \end{matrix}

(A6b)

\begin{matrix} ρ = \sqrt{{(\vec{r} - {\vec{r}}_{1})}^{2} + d {({\vec{r}}_{1}, {\vec{r}}_{0})}^{2}}; \end{matrix}

(A6c)

\begin{matrix} θ = f (ρ^{2}); \end{matrix}

(A6d)

\begin{matrix} f (0) = λ; f (\infty) = π; f^{'} (ρ^{2}) > 0; \end{matrix}

(A6e)

\begin{matrix} ϕ = m α + n β; \end{matrix}

(A6f)

\begin{matrix} \vec{S} = (sin θ cos ϕ, sin θ sin ϕ, cos θ); \end{matrix}

(A6g)

In the variational solution for the Kelvinon flow, one may use this Anzatz and optimize the smooth monotonous function

f (ρ^{2})

to reach the minimum of the Euler Hamiltonian at fixed winding numbers

n, m

In this example, the Clebsch field maps the physical space

G = R^{3} ∖ T

onto the disk on a 2-sphere

Ω_{+} : S_{3} < cos λ

. With the function

f (ρ^{2})

monotonously decreasing from

f (0) = λ

f (\infty) = 0

, this Kelvinon would map the physical space to the complementary region

Ω_{-}

When the point

\vec{r}

approaches the nearest point

{\vec{r}}_{1}

at the minimal surface, the difference

\vec{η} = \vec{r} - {\vec{r}}_{1}

is normal to this surface.

When the point

{\vec{r}}_{1}

approaches the nearest point

{\vec{r}}_{0}

at the edge C of the surface, the geodesic becomes a straight line in

R_{3}

, tangent to the surface and

ρ

becomes Euclidean distance to the loop

\begin{matrix} d ({\vec{r}}_{1}, {\vec{r}}_{0}) \to | {\vec{r}}_{1} - {\vec{r}}_{0} |; \end{matrix}

(A7)

\begin{matrix} ρ^{2} \to | \vec{r} - {\vec{r}}_{1} |^{2} + | {\vec{r}}_{1} - {\vec{r}}_{0} |^{2} = {| \vec{r} - {\vec{r}}_{0} |}^{2} \end{matrix}

(A8)

Note that all variables

s_{0}, {\vec{r}}_{0}, {\vec{r}}_{1}, α, β, ρ, θ, ϕ

depend on

\vec{r} \in R_{3}

through the minimization of the distance to the surface and the loop. By construction,

ρ = | \vec{r} - {\vec{r}}_{0} |

away from the surface, when

{\vec{r}}_{1} = {\vec{r}}_{0}, d ({\vec{r}}_{1}, {\vec{r}}_{0}) = 0

The Euler angles

θ, ϕ

for the Clebsch field take the boundary values at the loop :

\begin{matrix} ϕ (\vec{r} \to C) \to m α + n β; \end{matrix}

(A9)

\begin{matrix} θ (\vec{r} \to C) = λ + O ({(\vec{r} - C)}^{2}); \end{matrix}

(A10)

and

θ (\infty) = 0 or π

Assuming the decay

f (ρ^{2}) \to f (\infty) + const / ρ^{2}

, one may estimate the decay rate of

\vec{\nabla} cos θ \sim 1 / | \vec{r} |^{3}, \vec{\nabla} ϕ \sim 1 / | \vec{r} |

, which corresponds to vorticity decaying as

1 / | \vec{r} |^{4}

. This decay is sufficient for the convergence of the enstrophy integral at infinity.

The Biot-Savart integral for velocity corresponding to such vorticity would decay as

1 / {\vec{r}}^{2}

or faster, sufficient for convergence of the Euler Hamiltonian at infinity.

Let us move

\vec{r}

along the normal from the surface at

{\vec{r}}_{1}

. Our parametrization of

θ

does not change in the first order in normal shift

\vec{η} = \vec{r} - {\vec{r}}_{1}

, as

ρ^{2}

has only quadratic terms in

\vec{η}

We conclude that the normal derivative of the Clebsch field

ϕ_{1} = Z (1 + cos θ)

vanishes

\begin{matrix} \partial_{n} ϕ_{1} = 0; \end{matrix}

(A11)

We requested vanishing normal velocity at the discontinuity surface for this surface to be stationary.

In terms of the Clebsch parametrization, the normal velocity would vanish provided

\begin{matrix} \partial_{n} Φ = {(\vec{\nabla} \times Ψ)}_{n}; \vec{r} \in S_{C} ∖ C \end{matrix}

(A12)

As for the angular field

ϕ

in (A6), its normal derivative does not vanish in the general case. The angle

α

does not change when the point

\vec{r}

moves in normal direction

\vec{N} ({\vec{r}}_{1})

from the surface projection

{\vec{r}}_{1}

by infinitesimal shift

\vec{η} = ϵ \vec{N} ({\vec{r}}_{1})

. However, another angle

β

changes in the linear order in

ϵ

\begin{matrix} \vec{N} ({\vec{r}}_{1}) \cdot (\vec{n} (s_{0}) + ı \vec{σ} (s_{0})) \neq 0 \end{matrix}

(A13)

Notes

1	A rookie mistake would be to forget the l dependence of the tangent vector ${\vec{C}}^{'} (l)$ and the parameter c and write a non-periodic formula $\vec{v} \overset{?}{=} c {\vec{C}}^{'} (l) l$ for the velocity field.

References

Burgers, J. A Mathematical Model Illustrating the Theory of Turbulence. In Advances in Applied Mechanics; Von Mises, R.; Von Kármán, T., Eds.; Elsevier, 1948; Vol. 1, pp. 171 – 199. [CrossRef]
Kolmogorov, A. The local structure of turbulence in incompressible viscous fluid for very large Reynolds numbers. C. R. Acad. Sci. URSS 1941, 30(4), 301–305.
Migdal, A.A. Random Surfaces and Turbulence. Proceedings of the International Workshop on Plasma Theory and Nonlinear and Turbulent Processes in Physics, Kiev, April 1987; Bar’yakhtar, V.G., Ed. World Scientific, 1988, p. 460.
Agishtein, M.E.; Migdal, A.A. Dynamics of vortex surfaces in three dimensions: Theory and simulations. Physica D: Nonlinear Phenomena 1989, 40, 91 – 118. [CrossRef]
Migdal, A. Loop Equation and Area Law in Turbulence. In Quantum Field Theory and String Theory; Baulieu, L.; Dotsenko, V.; Kazakov, V.; Windey, P., Eds.; Springer US, 1995; pp. 193–231. [CrossRef]
Migdal, A.A. Turbulence as Statistics of Vortex Cells. The First Landau Institute Summer School, 1993: Selected Proceedings; Mineev, V., Ed. Gordon and Breach, 1993, pp. 178–204, [arXiv:hep-th/hep-th/9306152v2].
Iyer, K.P.; Sreenivasan, K.R.; Yeung, P.K. Circulation in High Reynolds Number Isotropic Turbulence is a Bifractal. Phys. Rev. X 2019, 9, 041006. [CrossRef]
Iyer, K.P.; Bharadwaj, S.S.; Sreenivasan, K.R. The area rule for circulation in three-dimensional turbulence. Proceedings of the National Academy of Sciences of the United States of America 2021, 118, e2114679118. [CrossRef]
Migdal, A. Statistical Equilibrium of Circulating Fluids. Physics Reports 2023, 1011C, 1–117, [arXiv:physics.flu-dyn/2209.12312]. [CrossRef]
Moffatt, H.K.; Kida, S.; Ohkitani, K. Stretched vortices – the sinews of turbulence; large-Reynolds-number asymptotics. Journal of Fluid Mechanics 1994, 259, 241–264. [CrossRef]
Kuznetsov, E.; Mikhailov, A. On the topological meaning of canonical Clebsch variables. Physics Letters A 1980, 77, 37 – 38. [CrossRef]
Levich, E. The Hamiltonian formulation of the Euler equation and subsequent constraints on the properties of randomly stirred fluids. Physics Letters A 1981, 86, 165–168.
Migdal, A. Vector Plot of Kelvinon Vorticity. https://www.wolframcloud.com/obj/sasha.migdal/Published/VectorPlotClebsch.nb, 2023.
Parisi, G.; Frisch, U. On the singularity structure of fully developed turbulence Turbulence and Predictability. Geophysical Fluid Dynamics: Proc. Intl School of Physics E. Fermi; M Ghil, R.B.; Parisi, G., Eds. Amsterdam: North-Holland, 1985, pp. 84–88.
Müller, N.P.; Polanco, J.I.; Krstulovic, G. Intermittency of Velocity Circulation in Quantum Turbulence. Phys. Rev. X 2021, 11, 011053. [CrossRef]
Sreenivasan, K.R.; Yakhot, V. Dynamics of three-dimensional turbulence from Navier-Stokes equations. Phys. Rev. Fluids 2021, 6, 104604. [CrossRef]
Sreenivasan, K.R.; Yakhot, V. The saturation of exponents and the asymptotic fourth state of turbulence, 2022. [CrossRef]

Figure 1. The dual loop (red) encircling the monopole ring (blue). The Burgers vortex resolves the singular vortex line.

Figure 2. The regions at

Ω_{\pm} \in S^{2}

, with opposite orientations of the boundary loop

\partial Ω_{\pm} = γ

. The areas

| Ω_{\pm} |

add up to

4 π

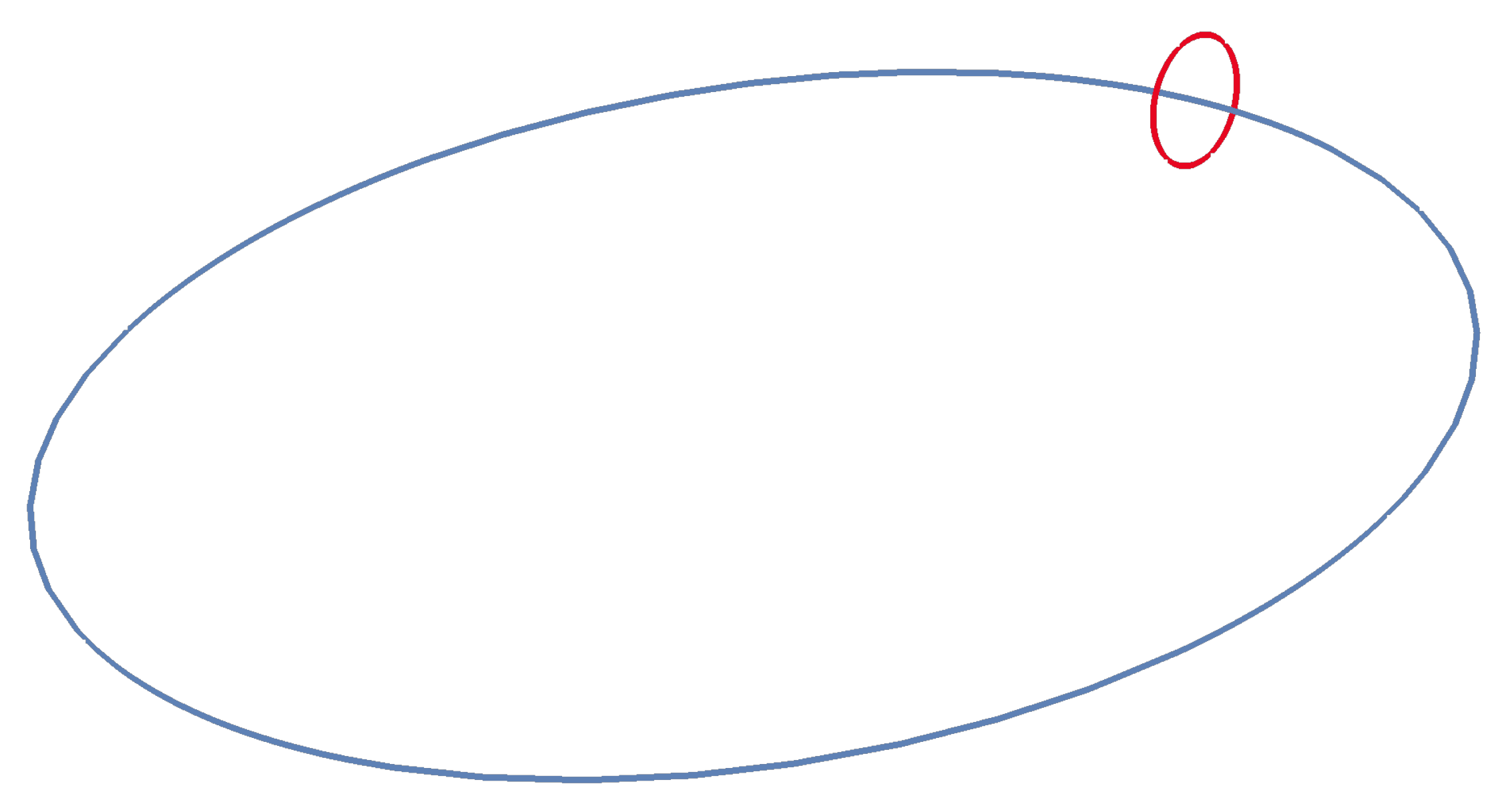
Figure 2. The regions at

Ω_{\pm} \in S^{2}

, with opposite orientations of the boundary loop

\partial Ω_{\pm} = γ

. The areas

| Ω_{\pm} |

add up to

4 π

Figure 6. The beta function

β (g)

and the running coupling constant

g_{R} (log R)

Figure 6. The beta function

β (g)

and the running coupling constant

g_{R} (log R)

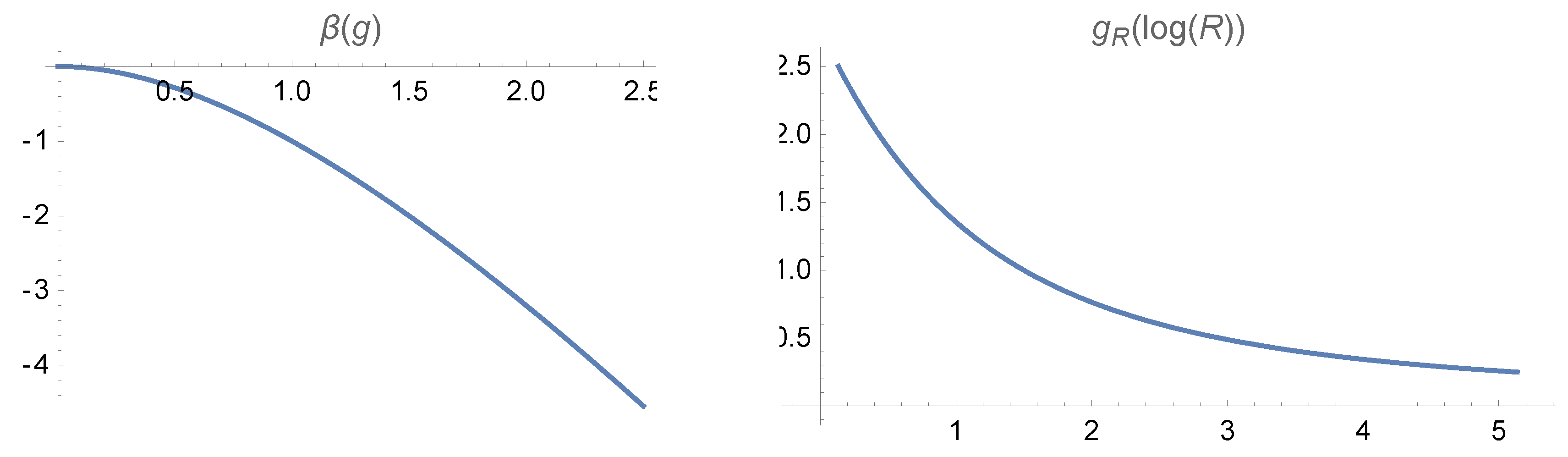
Figure 7. DNS data [8] for classical circulation fractal dimensions

λ (p)

fitted against our asymptotic formula (86) with

const g_{R}^{\frac{2}{3}} = 1.359

(blue) and K41 line

\frac{4 p}{3}

(green).

Figure 7. DNS data [8] for classical circulation fractal dimensions

λ (p)

fitted against our asymptotic formula (86) with

const g_{R}^{\frac{2}{3}} = 1.359

(blue) and K41 line

\frac{4 p}{3}

(green).

Figure 8. DNS data [15] for quantum circulation fractal dimensions

λ (p)

fitted against our asymptotic formula (86) with

const g_{R}^{\frac{2}{3}} = 1.442

(blue) and K41 line

\frac{4 p}{3}

(green).

Figure 8. DNS data [15] for quantum circulation fractal dimensions

λ (p)

fitted against our asymptotic formula (86) with

const g_{R}^{\frac{2}{3}} = 1.442

(blue) and K41 line

\frac{4 p}{3}

(green).

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content.

© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).

Copyright: This open access article is published under a Creative Commons CC BY 4.0 license, which permit the free download, distribution, and reuse, provided that the author and preprint are cited in any reuse.

MDPI Initiatives

Important Links

Choose an area of interest and we will send you notifications of new preprints at your preferred frequency.

Disclaimer

Topological Vortexes, Asymptotic Freedom and Multifractals

Abstract

1. Introduction

2. Trying to Bend the Burgers Cylindrical Vortex into a Torus

3. Matching Principle and Anomalies in the Euler Hamiltonian

4. Topological Euler Flow

5. Energy Balance Revisited

6. Asymptotic Freedom Revisited

7. Multifractals

8. Conclusions

Data Availability Statement

Acknowledgments

Appendix Topological family of the Kelvinon fields

Notes

References

MDPI Initiatives

Important Links

Subscribe