4-Component Spinors for SL(4,C) and Four Types of Transformations

Preprint

Article

4-Component Spinors for SL(4,C) and Four Types of Transformations

Altmetrics

Downloads

241

Views

206

Comments

Mehmet Ali Kuntman^*

This version is not peer-reviewed

Submitted:

09 April 2023

Posted:

11 April 2023

Read the latest preprint version here

Alerts

Abstract

We define a spinor-Minkowski metric for SL(4,C). It is not a trivial generalization of the SL(2,C) metric and it involves the Minkowski metric. We define 4x4 version of the Pauli matrices and their 4-component generalized eigenvectors. The generalized eigenvectors can be regarded as 4-component spinors and they can be grouped into four categories. Each category transforms in its own way. The outer products of pairwise combinations of 4-component spinors can be associated with 4-vectors.

Keywords:

Subject: Physical Sciences - Theoretical Physics

1. Introduction

Let

L_{A}

be an element of

S L (2, C)

. In an exponential form with parameters

θ

and

η

L_{A} = e x p (- \frac{i}{2} (\vec{θ} \cdot \vec{σ} + i \vec{η} \cdot \vec{σ}))

(1)

\vec{σ}

is the Pauli vector with

σ_{1} = σ_{x}, σ_{2} = σ_{y}, σ_{3} = σ_{z}

. The subscript

_{A}

is introduced in order to distinguish the other forms of L that will be introduced subsequently.

We rewrite

L_{A}

and its complex conjugate in the following compact forms:

L_{A} = e x p (- \frac{i}{2} {\vec{π}}_{A} \cdot \vec{σ}), L_{A}^{*} = e x p (\frac{i}{2} {\vec{π}}_{A}^{*} \cdot {\vec{σ}}^{*})

(2)

{(π_{A})}_{i} = θ_{i} + i η_{i}

and

^{*}

denotes complex conjugation.

L_{A}

corresponds to the Lorentz transformation with

θ_{i}

and

η_{i}

being the rotation and boost parameters, respectively.

It is well known that the complex version of the

4 \times 4

Lorentz transformation matrix can be written as a matrix direct product of

L_{A}

and

L_{A}^{*}

λ = L_{A} \otimes L_{A}^{*}

(3)

In order to obtain the familiar real matrix form of the Lorentz transformation it is enough to change the basis:

Λ = A (L_{A} \otimes L_{A}^{*}) A^{- 1}

(4)

where

A = \frac{1}{\sqrt{2}} (\begin{matrix} 1 & 0 & 0 & 1 \\ 0 & 1 & 1 & 0 \\ 0 & i & - i & 0 \\ 1 & 0 & 0 & - 1 \end{matrix}), A^{- 1} = A^{†} = \frac{1}{\sqrt{2}} (\begin{matrix} 1 & 0 & 0 & 1 \\ 0 & 1 & - i & 0 \\ 0 & 1 & i & 0 \\ 1 & 0 & 0 & - 1 \end{matrix})

(5)

Now, it is straightforward to show that

S O (3, 1)

can be written as a commutative product of

S L (4, C)

and

S L {(4, C)}^{*}

by simply rewriting Eq.(4) in a factorized form:

Λ = [A (L_{A} \otimes I) A^{- 1}] [A (I \otimes L_{A}^{*}) A^{- 1}] = Z_{A} Z_{A}^{*} = Z_{A}^{*} Z_{A},

(6)

Z_{A} = A (L_{A} \otimes I) A^{- 1}, Z_{A}^{*} = A (I \otimes L_{A}^{*}) A^{- 1} .

(7)

Z_{A}

and

Z_{A}^{*}

are the

4 \times 4

versions of

L_{A}

and

L_{A}^{*}

matrices. They can be expressed in terms of

Σ_{i}

matrices:

Z_{A} = e x p (- \frac{i}{2} {\vec{π}}_{A} \cdot \vec{Σ}), Z_{A}^{*} = e x p (\frac{i}{2} {\vec{π}}_{A}^{*} \cdot {\vec{Σ}}^{*}) .

(8)

\vec{Σ} = (Σ_{1}, Σ_{2}, Σ_{3})

and

Σ_{i}

are

4 \times 4

versions of Pauli matrices:

Σ_{1} = (\begin{matrix} 0 & 1 & 0 & 0 \\ 1 & 0 & 0 & 0 \\ 0 & 0 & 0 & - i \\ 0 & 0 & i & 0 \end{matrix}), Σ_{2} = (\begin{matrix} 0 & 0 & 1 & 0 \\ 0 & 0 & 0 & i \\ 1 & 0 & 0 & 0 \\ 0 & - i & 0 & 0 \end{matrix}), Σ_{3} = (\begin{matrix} 0 & 0 & 0 & 1 \\ 0 & 0 & - i & 0 \\ 0 & i & 0 & 0 \\ 1 & 0 & 0 & 0 \end{matrix})

(9)

These are traceless Hermitian matrices and they satisfy the same commutation relations as

σ_{i}

matrices

[\frac{1}{2} Σ_{i}, \frac{1}{2} Σ_{j}] = \frac{i}{2} ϵ^{i j k} Σ_{k} .

(10)

By definition,

Σ_{μ} = A (σ_{μ} \otimes I) A^{- 1}

(μ = 0, 1, 2, 3)

Σ_{0}

is the

4 \times 4

identity.

Σ_{μ}

basis do not form a complete set for

4 \times 4

matrices, but the set of

Σ_{μ} Σ_{ν}^{*}

does.

From the Eq.(7),

Z_{A}

can be found in terms of the elements of

L_{A}

Z_{A} = (\begin{matrix} α_{0} & α_{1} & α_{2} & α_{3} \\ α_{1} & α_{0} & - i α_{3} & i α_{2} \\ α_{2} & i α_{3} & α_{0} & - i α_{1} \\ α_{3} & - i α_{2} & i α_{1} & α_{0} \end{matrix})

(11)

where

α_{0} = \frac{1}{2} (L_{11} + L_{22})

α_{1} = \frac{1}{2} (L_{12} + L_{21})

α_{2} = \frac{i}{2} (L_{12} - L_{21})

, and

α_{3} = \frac{1}{2} (L_{11} - L_{22})

. Hence,

L_{A}

can be written in terms of

α_{μ}

L_{A} = (\begin{matrix} α_{0} + α_{3} & α_{1} - i α_{2} \\ α_{1} + i α_{2} & α_{0} - α_{3} \end{matrix})

(12)

We can write

L_{A}

and

Z_{A}

in terms of

σ_{μ}

and

Σ_{μ}

matrices:

L_{A} = α_{0} σ_{0} + α_{1} σ_{1} + α_{2} σ_{2} + α_{3} σ_{3}

(13)

Z_{A} = α_{0} Σ_{0} + α_{1} Σ_{1} + α_{2} Σ_{2} + α_{3} Σ_{3}

(14)

Or, simply

L_{A} = {(+ + + +)}_{σ} .

(15)

Z_{A} = {(+ + + +)}_{Σ} .

(16)

We also define the spinor metric g for

S L (4, C)

that corresponds to the spinor metric

ϵ

S L (2, C)

g = g^{μ ν} = i η Σ_{2}^{*} = (\begin{matrix} 0 & 0 & i & 0 \\ 0 & 0 & 0 & - 1 \\ - i & 0 & 0 & 0 \\ 0 & 1 & 0 & 0 \end{matrix}); g^{- 1} = g_{μ ν} = g^{†} .

(17)

η

is the mostly minus Minkowski metric1.

Z_{A}

preserves the Minkowski metric:

Z_{A}^{T} η Z_{A} = η

(18)

Since

η

is real,

Z_{A}^{T} η Z_{A} = η

directly entails

Λ^{T} η Λ = η

. In an analogy with

ϵ σ_{i} ϵ^{- 1} = - σ_{i}^{*}

, we have the following very useful relation:

g Σ_{i} g^{- 1} = - Σ_{i}^{*}

(19)

In this note we will show that there are eight generalized eigenvectors of

Σ_{3}^{*}

matrix that can be interpreted as 4-component covariant spinors. The generalized eigenvectors can be pairwise grouped into four categories. The first pair transforms in the usual way, but the other three transform in different ways.

In the following we will study the first and the second pairs in detail, and we will introduce the remaining two in the subsequent sections.

2. The First and the Second Pairs and Their Transformation Properties

Let

L_{A} = e x p (- \frac{i}{2} {\vec{π}}_{A} \cdot \vec{σ})

be the

(\frac{1}{2}, 0)

representation of the Lorentz group that acts on the 2-component left-chiral spinor

ξ_{_{L}}

ξ_{_{L}} \to ξ_{_{L}}^{'} = L_{A} ξ_{_{L}} .

(20)

where

L_{A} = (\begin{matrix} L_{11} & L_{12} \\ L_{21} & L_{22} \end{matrix})

(21)

In terms of the components

u, v

ξ_{_{L}}

u \to u^{'} = L_{11} u + L_{12} v, v \to v^{'} = L_{21} u + L_{22} v .

(22)

Let us call this transformation scheme

T_{A}

Let

{\dot{L}}_{A} = e x p (- \frac{i}{2} {\vec{π}}_{A}^{*} \cdot \vec{σ})

be the dotted version corresponding to the

(0, \frac{1}{2})

representation of the Lorentz group.

{\dot{L}}_{A} = {(L_{A}^{- 1})}^{†}

. Let

ξ_{_{R}}

be the 2-component right-chiral spinor.

ξ_{_{R}} = ϵ ξ_{_{L}}^{*}

, where

ϵ = ϵ^{a b} = (\begin{matrix} 0 & 1 \\ - 1 & 0 \end{matrix}), ϵ^{- 1} = ϵ_{a b} = ϵ^{†} .

(23)

ξ_{_{R}}

transforms as

ξ_{_{R}} \to ξ_{_{R}}^{'} = {\dot{L}}_{A} ξ_{_{R}}

(24)

In terms of the components

u, v

, Eq.(24) is equivalent to the scheme

T_{A}

given in Eq.(22).

What happens when

L_{A}

acts on

ϵ ξ_{_{L}}

? In this case, in terms of the components

u \to u^{'} = L_{22} u - L_{21} v, v \to v^{'} = - L_{12} u + L_{11} v

(25)

Let us call this transformation scheme

T_{B}

. We can write

T_{B}

in a matrix form:

(\begin{matrix} u \\ v \end{matrix}) \to (\begin{matrix} u^{'} \\ v^{'} \end{matrix}) = (\begin{matrix} L_{22} & - L_{21} \\ - L_{12} & L_{11} \end{matrix}) (\begin{matrix} u \\ v \end{matrix})

(26)

Let us name this transformation matrix as

L_{B}

. Note that,

L_{B} = {({\dot{L}}_{A})}^{*}

, and Eq.(26) is nothing but the transformation of

ξ_{_{L}}

under the action of

L_{B}

, which is a type

T_{B}

transformation.

Now, let

Z_{A} = e x p (- \frac{i}{2} {\vec{π}}_{A} \cdot \vec{Σ})

be the

(\frac{1}{2}, 0)

representation of

S L (4, C)

that acts on the first pair of the 4-component undotted covariant spinors:

χ_{_{(1)}} \to Z_{A} χ_{_{(1)}}, χ_{_{(2)}} \to Z_{A} χ_{_{(2)}}

(27)

where

χ_{_{(1)}}

and

χ_{_{(2)}}

are the generalized eigenvectors of

Σ_{3}^{*}

χ_{_{(1)}} = \frac{1}{\sqrt{2}} (\begin{matrix} u \\ v \\ - i v \\ u \end{matrix}), χ_{_{(2)}} = \frac{1}{\sqrt{2}} (\begin{matrix} - v \\ - u \\ - i u \\ v \end{matrix})

(28)

Indices in the parentheses are simply labels for 4-component spinors.

Now consider the second pair of the generalized eigenvectors of

Σ_{3}^{*}

χ_{_{(3)}} = \frac{1}{\sqrt{2}} (\begin{matrix} - v \\ u \\ - i u \\ - v \end{matrix}), χ_{_{(4)}} = \frac{1}{\sqrt{2}} (\begin{matrix} u \\ - v \\ - i v \\ - u \end{matrix})

(29)

Transformation scheme of

χ_{_{(3)}}

and

χ_{_{(4)}}

is different from that of

χ_{_{(1)}}

and

χ_{_{(2)}}

. Under the action of

Z_{A}

χ_{_{(1)}}

and

χ_{_{(2)}}

transform according to the scheme

T_{A}

, but

χ_{_{(3)}}

and

χ_{_{(4)}}

transform according to the scheme

T_{B}

. However, we may think in an alternative way: Suppose that

χ_{_{(3)}}

and

χ_{_{(3)}}

are different kind of objects with different transformation properties, such that another transformation matrix,

Z_{B}

, acts on them and under the action of

Z_{B}

they transform according to the scheme

T_{A}

χ_{_{(3)}} \to Z_{B} χ_{_{(3)}}, χ_{_{(4)}} \to Z_{B} χ_{_{(4)}},

(30)

By definition

Z_{B} = A (L_{B} \otimes I) A^{- 1}

Z_{B} = (\begin{matrix} α_{0} & - α_{1} & α_{2} & - α_{3} \\ - α_{1} & α_{0} & i α_{3} & i α_{2} \\ α_{2} & - i α_{3} & α_{0} & i α_{1} \\ - α_{3} & - i α_{2} & - i α_{1} & α_{0} \end{matrix}) = α_{0} Σ_{0} - α_{1} Σ_{1} + α_{2} Σ_{2} - α_{3} Σ_{3} .

(31)

Or, simply

Z_{B} = {(+ - + -)}_{Σ}

(32)

Now let

{\dot{Z}}_{A} = e x p (- \frac{i}{2} {\vec{π}}_{A}^{*} \cdot \vec{Σ})

be the

(0, \frac{1}{2})

representation.

{\dot{Z}}_{A} = {(Z_{A}^{- 1})}^{†}

. We regard the generalized eigenvectors of

Σ_{3}

as 4-component undotted contravariant spinors and we define the first pair as follows:

χ^{(1)} = \frac{1}{\sqrt{2}} (\begin{matrix} v \\ - u \\ - i u \\ v \end{matrix}), χ^{(2)} = \frac{1}{\sqrt{2}} (\begin{matrix} u \\ - v \\ i v \\ - u \end{matrix})

(33)

Under the action of

{\dot{Z}}_{A}

, dotted versions of

χ^{^{(1)}}

and

χ^{^{(1)}}

transform according to the scheme

T_{A}

{\dot{χ}}^{^{(1)}} \to {\dot{Z}}_{A} {\dot{χ}}^{^{(1)}}, {\dot{χ}}^{^{(2)}} \to {\dot{Z}}_{A} {\dot{χ}}^{^{(2)}}

(34)

The second pair of the generalized eigenvectors of

Σ_{3}

is defined as

χ^{(3)} = \frac{1}{\sqrt{2}} (\begin{matrix} u \\ v \\ i v \\ u \end{matrix}), χ^{(4)} = \frac{1}{\sqrt{2}} (\begin{matrix} v \\ u \\ - i u \\ - v \end{matrix})

(35)

Under the action of

{\dot{Z}}_{A}

, the dotted versions of

χ^{^{(3)}}

and

χ^{^{(4)}}

transform according to the scheme

T_{B}

. But, they transform according to the scheme

T_{A}

under the action of

{\dot{Z}}_{B}

{\dot{χ}}^{^{(3)}} \to {\dot{Z}}_{B} {\dot{χ}}^{^{(3)}}, {\dot{χ}}^{^{(4)}} \to {\dot{Z}}_{B} {\dot{χ}}^{^{(4)}}

(36)

where

{\dot{Z}}_{B} = {(Z_{B}^{- 1})}^{†}

by definition.

χ^{^{(a)}}

is related to

χ_{_{(a)}}

by the

S L (4, C)

metric,

χ^{(a)} = g χ_{_{(a)}}

, and its dotted version is defined as 3.

{\dot{χ}}^{(a)} = {(g χ_{_{(a)}})}^{*} .

(37)

We write various forms of Z and L matrices in compact notation to manifest the parallelism between them:

L_{A} = {(+ + + +)}_{σ}, L_{B} = {(+ - + -)}_{σ}, {\dot{L}}_{A} = {(+ - - -)}_{σ}^{*}, {\dot{L}}_{B} = {(+ + - +)}_{σ}^{*} .

(38)

Z_{A} = {(+ + + +)}_{Σ}, Z_{B} = {(+ - + -)}_{Σ}, {\dot{Z}}_{A} = {(+ - - -)}_{Σ}^{*}, {\dot{Z}}_{B} = {(+ + - +)}_{Σ}^{*} .

(39)

3. Outer Products of 4-Component Spinors and Null 4-Vectors

Let us define the outer product

W_{_{L}} = ξ_{_{L}} ξ_{_{L}}^{†}

which transforms as

W_{_{L}} \to W_{_{L}}^{'} = (L_{A} ξ_{_{L}}) {(L_{A} ξ_{_{L}})}^{†} = L_{A} W_{_{L}} L_{A}^{†}

(40)

This is a type

T_{A}

transformation. Determinant of

W_{_{L}}

is zero, hence

W_{_{L}}

can be associated with a null 4-vector through the substitutions,

t = \frac{1}{2} (u \dot{u} + v \dot{v}), x = \frac{1}{2} (u \dot{v} + v \dot{u}), y = \frac{i}{2} (u \dot{v} - v \dot{u}), z = \frac{1}{2} (u \dot{u} - v \dot{v})

W_{_{L}} = (\begin{matrix} t + z & x - i y \\ x + i y & t - z \end{matrix})

(41)

We also define the outer product

W_{_{R}} = ξ_{_{R}} ξ_{_{R}}^{†}

which transforms as

W_{_{R}} \to W_{_{R}}^{'} = ({\dot{L}}_{A} ξ_{_{R}}) {({\dot{L}}_{A} ξ_{_{R}})}^{†} = {\dot{L}}_{A} W_{_{R}} {\dot{L}}_{A}^{†}

(42)

This is also a type

T_{A}

transformation. Determinant of

W_{_{R}}

is zero and

W_{_{R}}

can be associated with a null 4-vector:

W_{_{R}} = (\begin{matrix} t - z & - x + i y \\ - x - i y & t + z \end{matrix})

(43)

Note that

W_{_{R}}

can be obtained from

W_{_{L}}

by parity inversion.

There are outer product forms of 4-component spinors that can be associated with null 4-vectors.

W_{_{(11)}} = χ_{_{(1)}} χ_{_{(1)}}^{†}

and

W_{_{(22)}} = χ_{_{(2)}} χ_{_{(2)}}^{†}

transform in a similar way with

W_{_{L}}

W_{_{(11)}} = (\begin{matrix} u \dot{u} & u \dot{v} & i u \dot{v} & u \dot{u} \\ v \dot{u} & v \dot{v} & i v \dot{v} & v \dot{u} \\ - i v \dot{u} & - i v \dot{v} & v \dot{v} & - i v \dot{u} \\ u \dot{u} & u \dot{v} & i u \dot{v} & u \dot{u} \end{matrix}), W_{_{(22)}} = (\begin{matrix} v \dot{v} & v \dot{u} & - i v \dot{u} & - v \dot{v} \\ u \dot{v} & u \dot{u} & - i u \dot{u} & - u \dot{v} \\ i u \dot{v} & i u \dot{u} & u \dot{u} & - i u \dot{v} \\ - v \dot{v} & - v \dot{u} & i v \dot{u} & v \dot{v} \end{matrix})

(44)

For

a = 1

and

a = 2

W_{_{(a a)}}

transform according to the scheme

T_{A}

W_{_{(a a)}} \to W_{_{(a a)}}^{'} = (Z_{A} χ_{_{(a)}}) {(Z_{A} χ_{_{(a)}})}^{†} = Z_{A} W_{_{(a a)}} Z_{A}^{†} .

(45)

This equation is equivalent to the Eq.(40), and it is the main motivation behind the interpretation of

χ_{_{(a)}}

as 4-component spinors for

S L (4, C)

{\dot{W}}^{^{(11)}} = {\dot{χ}}^{^{(1)}} {\dot{χ}}^{^{(1) †}}

and

{\dot{W}}^{^{(22)}} = {\dot{χ}}^{^{(2)}} {\dot{χ}}^{^{(2) †}}

transform in a similar way with

W_{_{R}}

{\dot{W}}^{^{(11)}} = (\begin{matrix} v \dot{v} & - u \dot{v} & - i u \dot{v} & v \dot{v} \\ - v \dot{u} & u \dot{u} & i u \dot{u} & - v \dot{u} \\ i v \dot{u} & - i u \dot{u} & u \dot{u} & i v \dot{u} \\ v \dot{v} & - u \dot{v} & - i u \dot{v} & v \dot{v} \end{matrix}), {\dot{W}}^{^{(22)}} = (\begin{matrix} u \dot{u} & - v \dot{u} & i v \dot{u} & - u \dot{u} \\ - u \dot{v} & v \dot{v} & - i v \dot{v} & u \dot{v} \\ - i u \dot{v} & i v \dot{v} & v \dot{v} & i u \dot{v} \\ - u \dot{u} & v \dot{u} & - i v \dot{u} & u \dot{u} \end{matrix})

(46)

For

a = 1

and

a = 2

{\dot{W}}^{^{(a a)}}

transform according to the scheme

T_{A}

{\dot{W}}^{^{(a a)}} \to {\dot{Z}}_{A} {\dot{W}}^{^{(a a)}} {\dot{Z}}_{A}^{†}

(47)

W_{_{(a a)}}

and

{\dot{W}}^{^{(a a)}}

are Hermitian and zero determinant matrices, hence they correspond to null 4-vectors.

We also have outer products of 4-component spinors of the other kind. For

a = 3

and

a = 4

W_{_{(a a)}}

and

{\dot{W}}^{^{(a a)}}

transform according to the scheme

T_{A}

under the action of

Z_{B}

and

{\dot{Z}}_{B}

W_{_{(a a)}} \to Z_{B} W_{_{(a a)}} Z_{B}^{†}, {\dot{W}}^{^{(a a)}} \to {\dot{Z}}_{B} {\dot{W}}^{^{(a a)}} {\dot{Z}}_{B}^{†}

(48)

These are also Hermitian and zero determinant matrices and they correspond to null 4-vectors.

4. Quaternion Forms and 4-Vectors

In general, we can treat

t, x, y

and z as variables that do not depend on u and v. Then, we can associate the following matrices

X_{_{L}}

and

X_{_{R}}

with 4-vectors, which are not necessarily null:

W_{_{L}} \to X_{_{L}} = (\begin{matrix} t + z & x - i y \\ x + i y & t - z \end{matrix}) = t σ_{0} + x σ_{1} + y σ_{2} + z σ_{3} = {(+ + + +)}_{σ}

(49)

W_{_{R}} \to X_{_{R}} = (\begin{matrix} t - z & - x + i y \\ - x - i y & t + z \end{matrix}) = t σ_{0} - x σ_{1} - y σ_{2} - z σ_{3} = {(+ - - -)}_{σ}

(50)

det

X_{_{L}} =

det

X_{_{R}} = t^{2} - x^{2} - y^{2} - z^{2}

and in general not zero.

X_{_{L}}

and

X_{_{R}}

transform as

X_{_{L}} \to X_{_{L}}^{'} = L_{A} X_{_{L}} L_{A}^{†}, X_{_{R}} \to X_{_{R}}^{'} = {\dot{L}}_{A} X_{_{R}} {\dot{L}}_{A}^{†}

(51)

These are matrix representations of quaternions, because

- i σ_{1}, - i σ_{2}, - i σ_{3}

matrices have the same properties as the Hamilton’s quaternion basis,

i, j, k

X_{_{L}} = t σ_{0} + i x (- i σ_{1}) + i y (- i σ_{2}) + i z (- i σ_{3}) = t 1 + i x i + i y j + i z k .

(52)

Similarly,

X_{_{R}} = t 1 - i x i - i y j - i z k .

(53)

In order to make the analogy with

S L (4, C)

we consider the following two column objects that are pairwise combinations of 4-component spinors:

χ_{_{A}} = \frac{1}{\sqrt{2}} (\begin{matrix} u & - v \\ v & - u \\ - i v & - i u \\ u & v \end{matrix}), χ_{_{B}} = \frac{1}{\sqrt{2}} (\begin{matrix} - v & u \\ u & - v \\ - i u & - i v \\ - v & - u \end{matrix}), χ^{^{A}} = \frac{1}{\sqrt{2}} (\begin{matrix} v & u \\ - u & - v \\ - i u & i v \\ v & - u \end{matrix}), χ^{^{B}} = \frac{1}{\sqrt{2}} (\begin{matrix} u & v \\ v & u \\ i v & - i u \\ u & - v \end{matrix})

(54)

where

χ_{_{A}} = (χ_{_{(1)}}, χ_{_{(2)}})

χ_{_{B}} = (χ_{_{(3)}}, χ_{_{(4)}})

χ^{^{A}} = (χ^{^{(1)}}, χ^{^{(2)}})

χ^{^{B}} = (χ^{^{(3)}}, χ^{^{(4)}})

We define an outer product of 4-component spinor pair in the form

W_{_{A}} = χ_{_{A}} χ_{_{A}}^{†}

, which is formally a quaternion:

W_{_{A}} = \frac{1}{2} (\begin{matrix} u \dot{u} + v \dot{v} & u \dot{v} + v \dot{u} & i u \dot{v} - i v \dot{u} & u \dot{u} - v \dot{v} \\ v \dot{u} + u \dot{v} & v \dot{v} + u \dot{u} & i v \dot{v} - i u \dot{u} & v \dot{u} - u \dot{v} \\ - i v \dot{u} + i u \dot{v} & - i v \dot{v} + i u \dot{u} & v \dot{v} + u \dot{u} & - i v \dot{u} - i u \dot{v} \\ u \dot{u} - v \dot{v} & u \dot{v} - v \dot{u} & i u \dot{v} + i v \dot{u} & u \dot{u} + v \dot{v} \end{matrix})

(55)

W_{_{A}}

can be written as a sum of two basic forms:

W_{_{A}} = W_{_{(11)}} + W_{_{(22)}}

. In its present form det

W_{_{A}} = 0

and

W_{_{A}}

corresponds to a null 4-vector, but we can associate

W_{_{A}}

with an arbitrary 4-vector in terms of the variables

t, x, y

and z:

W_{_{A}} \to Q_{_{A}} = (\begin{matrix} t & x & y & z \\ x & t & - i z & i y \\ y & i z & t & - i x \\ z & - i y & i x & t \end{matrix}) = t Σ_{0} + x Σ_{1} + y Σ_{2} + z Σ_{3} .

(56)

Q_{_{A}} = {(+ + + +)}_{Σ}

and it is the

4 \times 4

version of

X_{_{L}}

Q_{_{A}} = A (X_{_{L}} \otimes I) A^{- 1}

(57)

Similarly, we define

{\dot{W}}^{^{A}}

{\dot{W}}^{^{A}} = {\dot{χ}}^{^{A}} {\dot{χ}}^{^{A †}} = {\dot{W}}^{^{(11)}} + {\dot{W}}^{^{(22)}} = \frac{1}{2} (\begin{matrix} \dot{v} v + \dot{u} u & - \dot{v} u - \dot{u} v & - i \dot{v} u + i \dot{u} v & \dot{v} v - \dot{u} u \\ - \dot{u} v - \dot{v} u & \dot{u} u + \dot{v} v & i \dot{u} u - i \dot{v} v & - \dot{u} v + \dot{v} u \\ i \dot{u} v - i \dot{v} u & - i \dot{u} u + i \dot{v} v & \dot{u} u + \dot{v} v & i \dot{u} v + i \dot{v} u \\ \dot{v} v - \dot{u} u & - \dot{v} u + \dot{u} v & - i \dot{v} u - i \dot{u} v & \dot{v} v + \dot{u} u \end{matrix})

(58)

In terms of the variables

t, x, y

and z:

{\dot{W}}^{^{A}} \to {\dot{Q}}^{^{A}} = (\begin{matrix} t & - x & - y & - z \\ - x & t & i z & - i y \\ - y & - i z & t & i x \\ - z & i y & - i x & t \end{matrix}) = t Σ_{0} - x Σ_{1} - y Σ_{2} - z Σ_{3} .

(59)

{\dot{Q}}^{^{A}} = {(+ - - -)}_{Σ}

and it is the

4 \times 4

version of

X_{_{R}}

{\dot{Q}}^{^{A}} = A (X_{_{R}} \otimes I) A^{- 1} .

(60)

{\dot{Q}}^{^{A}}

can be obtained from

Q_{_{A}}

by parity inversion and they transform as

Q_{_{A}} \to Z_{A} Q_{_{A}} Z_{A}^{†}, {\dot{Q}}^{^{A}} \to {\dot{Z}}_{A} {\dot{Q}}^{^{A}} {\dot{Z}}_{A}^{†}

(61)

These are type

T_{A}

transformations, hence these forms correspond to 4-vectors.

The outer product

W_{_{B}} = χ_{_{B}} χ_{_{B}}^{†}

is also a quaternion:

W_{_{B}} = \frac{1}{2} (\begin{matrix} v \dot{v} + u \dot{u} & - v \dot{u} - u \dot{v} & - i v \dot{u} + i u \dot{v} & v \dot{v} - u \dot{u} \\ - u \dot{v} - v \dot{u} & u \dot{u} + v \dot{v} & i u \dot{u} - i v \dot{v} & - u \dot{v} + v \dot{u} \\ i u \dot{v} - i v \dot{u} & - i u \dot{u} + i v \dot{v} & u \dot{u} + v \dot{v} & i u \dot{v} + i v \dot{u} \\ v \dot{v} - u \dot{u} & - v \dot{u} + u \dot{v} & - i v \dot{u} - i u \dot{v} & v \dot{v} + u \dot{u} \end{matrix})

(62)

In terms of variables

t, x, y

and z:

W_{_{B}} \to Q_{_{B}} = (\begin{matrix} t & - x & y & - z \\ - x & t & i z & i y \\ y & - i z & t & i x \\ - z & - i y & - i x & t \end{matrix}) = t Σ_{0} - x Σ_{1} + y Σ_{2} - z Σ_{3} .

(63)

Q_{_{B}} = {(+ - + -)}_{Σ}

We also write

{\dot{W}}^{^{B}}

{\dot{W}}^{^{B}} = {\dot{χ}}^{^{B}} {\dot{χ}}^{^{B †}} = \frac{1}{2} (\begin{matrix} \dot{u} u + \dot{v} v & \dot{u} v + \dot{v} u & i \dot{u} v - i \dot{v} u & \dot{u} u - \dot{v} v \\ \dot{v} u + \dot{u} v & \dot{v} v + \dot{u} u & i \dot{v} v - i \dot{u} u & \dot{v} u - \dot{u} v \\ - i \dot{v} u + i \dot{u} v & - i \dot{v} v + i \dot{u} u & \dot{v} v + \dot{u} u & - i \dot{v} u - i \dot{u} v \\ \dot{u} u - \dot{v} v & \dot{u} v - \dot{v} u & i \dot{u} v + i \dot{v} u & \dot{u} u + \dot{v} v \end{matrix})

(64)

{\dot{W}}^{^{B}} \to {\dot{Q}}^{^{B}} = (\begin{matrix} t & x & - y & z \\ x & t & - i z & - i y \\ - y & i z & t & - i x \\ z & i y & i x & t \end{matrix}) = t Σ_{0} + x Σ_{1} - y Σ_{2} + z Σ_{3} .

(65)

{\dot{Q}}^{^{B}} = {(+ + - +)}_{Σ}

and it can be obtained from

Q_{_{B}}

by parity inversion.

Q_{_{B}}

and

{\dot{Q}}^{^{B}}

transform with

Z_{B}

and

{\dot{Z}}_{B}

Q_{_{B}} \to Z_{B} Q_{_{B}} Z_{B}^{†}, {\dot{Q}}^{^{B}} \to {\dot{Z}}_{B} {\dot{Q}}^{^{B}} {\dot{Z}}_{B}^{†}

(66)

These transformations obey the scheme

T_{A}

also, hence they correspond to 4-vectors.

With the compact notation we can show a very nice symmetry: The form of the transformation matrix matches the form of the transformed object. For example,

Z_{A} = {(+ + + +)}_{Σ}

acts on the form

Q_{A} = {(+ + + +)}_{Σ}

Z_{B} = {(+ - + -)}_{Σ}

acts on the form

Q_{B} = {(+ - + -)}_{Σ}

{\dot{Z}}_{A} = {(+ - - -)}_{Σ}^{*}

acts on the form

{\dot{Q}}^{A} = {(+ - - -)}_{Σ}

, and

{\dot{Z}}_{B} = {(+ + - +)}_{Σ}^{*}

acts on the form

{\dot{Q}}^{B} = {(+ + - +)}_{Σ}

5. Two More Pairs of Spinors

There are four eigenvectors of

Σ_{3}^{*}

that constitute a complete orthonormal set of basis:

e_{1} = (\begin{matrix} 1 \\ 0 \\ 0 \\ 1 \end{matrix}), e_{2} = (\begin{matrix} 1 \\ 0 \\ 0 \\ - 1 \end{matrix}), e_{3} = (\begin{matrix} 0 \\ 1 \\ - i \\ 0 \end{matrix}), e_{4} = (\begin{matrix} 0 \\ 1 \\ i \\ 0 \end{matrix}) .

(67)

e_{1}

and

e_{3}

correspond to

+ 1

eigenvalue and

e_{2}

and

e_{4}

correspond to

- 1

eigenvalue. We obtain eight generalized eigenvectors by combining the basis corresponding to the same eigenvalue. For example, we can obtain the four generalized eigenvectors that we have previously studied as follows:

χ_{_{(1)}} = u e_{1} + v e_{3}, χ_{_{(2)}} = - v e_{2} - u e_{4},

(68)

χ_{_{(3)}} = - v e_{1} + u e_{3}, χ_{_{(4)}} = u e_{2} - v e_{4},

(69)

We can obtain four more generalized eigenvectors of

Σ_{3}^{*}

by changing the sign or swapping u and v:

χ_{_{(5)}} = - u e_{1} + v e_{3}, χ_{_{(6)}} = v e_{2} - u e_{4},

(70)

χ_{_{(7)}} = v e_{1} + u e_{3}, χ_{_{(8)}} = u e_{2} + v e_{4},

(71)

Totally we get eight undotted covariant spinors:

χ_{_{(1)}} = (\begin{matrix} u \\ v \\ - i v \\ u \end{matrix}), χ_{_{(2)}} = (\begin{matrix} - v \\ - u \\ - i u \\ v \end{matrix}), χ_{_{(3)}} = (\begin{matrix} - v \\ u \\ - i u \\ - v \end{matrix}), χ_{_{(4)}} = (\begin{matrix} u \\ - v \\ - i v \\ - u \end{matrix}),

(72)

χ_{_{(5)}} = (\begin{matrix} - u \\ v \\ - i v \\ - u \end{matrix}), χ_{_{(6)}} = (\begin{matrix} v \\ - u \\ - i u \\ - v \end{matrix}), χ_{_{(7)}} = (\begin{matrix} v \\ u \\ - i u \\ v \end{matrix}), χ_{_{(8)}} = (\begin{matrix} u \\ v \\ i v \\ - u \end{matrix}) .

(73)

We can group

χ_{_{(a)}}

(

a = 1, 2, \dots 8

) pairwise:

P_{_{A}} = {χ_{_{(1)}}, χ_{_{(2)}}}, P_{_{B}} = {χ_{_{(3)}}, χ_{_{(4)}}}, P_{_{C}} = {χ_{_{(5)}}, χ_{_{(6)}}}, P_{_{D}} = {χ_{_{(7)}}, χ_{_{(8)}}},

(74)

We already know that

P_{A}

transforms with

Z_{A}

and

P_{B}

transforms with

Z_{B}

. Following the same procedure that we have applied in the previous sections we can show that

P_{C}

and

P_{D}

transform with

Z_{C}

and

Z_{D}

respectively:

Z_{C} = A (L_{C} \otimes I) A^{- 1}, Z_{D} = A (L_{D} \otimes I) A^{- 1},

(75)

where

L_{C} = (\begin{matrix} L_{11} & - L_{12} \\ - L_{21} & L_{22} \end{matrix}) = (\begin{matrix} α_{0} + α_{3} & - α_{1} + i α_{2} \\ - α_{1} - i α_{2} & α_{0} - α_{3} \end{matrix}) = {(+ - - +)}_{σ}

(76)

L_{D} = (\begin{matrix} L_{22} & L_{21} \\ L_{12} & L_{11} \end{matrix}) = (\begin{matrix} α_{0} - α_{3} & α_{1} + i α_{2} \\ α_{1} - i α_{2} & α_{0} + α_{3} \end{matrix}) = {(+ + - -)}_{σ}

(77)

{\dot{L}}_{C} = {(+ + + -)}_{σ}^{*} = L_{D}^{*}

(78)

{\dot{L}}_{D} = {(+ - + +)}_{σ}^{*} = L_{C}^{*}

(79)

Z_{C} = (\begin{matrix} α_{0} & - α_{1} & - α_{2} & α_{3} \\ - α_{1} & α_{0} & - i α_{3} & - i α_{2} \\ - α_{2} & i α_{3} & α_{0} & i α_{1} \\ α_{3} & i α_{2} & - i α_{1} & α_{0} \end{matrix}) = {(+ - - +)}_{Σ}

(80)

Z_{D} = (\begin{matrix} α_{0} & α_{1} & - α_{2} & - α_{3} \\ α_{1} & α_{0} & i α_{3} & - i α_{2} \\ - α_{2} & - i α_{3} & α_{0} & - i α_{1} \\ - α_{3} & i α_{2} & i α_{1} & α_{0} \end{matrix}) = {(+ + - -)}_{Σ}

(81)

There are also the dotted versions:

{\dot{Z}}_{C} = (\begin{matrix} α_{0}^{*} & α_{1}^{*} & α_{2}^{*} & - α_{3}^{*} \\ α_{1}^{*} & α_{0}^{*} & i α_{3}^{*} & i α_{2}^{*} \\ α_{2}^{*} & - i α_{3}^{*} & α_{0}^{*} & - i α_{1}^{*} \\ - α_{3}^{*} & - i α_{2}^{*} & i α_{1}^{*} & α_{0}^{*} \end{matrix}) = {(+ + + -)}_{Σ}^{*}

(82)

{\dot{Z}}_{D} = (\begin{matrix} α_{0}^{*} & - α_{1}^{*} & α_{2}^{*} & α_{3}^{*} \\ - α_{1}^{*} & α_{0}^{*} & - i α_{3}^{*} & i α_{2}^{*} \\ α_{2}^{*} & i α_{3}^{*} & α_{0}^{*} & i α_{1}^{*} \\ α_{3}^{*} & - i α_{2}^{*} & - i α_{1}^{*} & α_{0}^{*} \end{matrix}) = {(+ - + +)}_{Σ}^{*}

(83)

We also define the contravariat spinors

χ^{^{(a)}} = g χ_{_{(a)}}

(

a = 1, 2, \dots 8

) that correspond to the generalized eigenvectors of

Σ_{3}

χ^{^{(1)}} = (\begin{matrix} v \\ - u \\ - i u \\ v \end{matrix}), χ^{^{(2)}} = (\begin{matrix} u \\ - v \\ i v \\ - u \end{matrix}), χ^{^{(3)}} = (\begin{matrix} u \\ v \\ i v \\ u \end{matrix}), χ^{^{(4)}} = (\begin{matrix} v \\ u \\ - i u \\ - v \end{matrix}),

(84)

χ^{^{(5)}} = (\begin{matrix} v \\ u \\ i u \\ v \end{matrix}), χ^{^{(6)}} = (\begin{matrix} u \\ v \\ - i v \\ - u \end{matrix}), χ^{^{(7)}} = (\begin{matrix} u \\ - v \\ - i v \\ u \end{matrix}), χ^{^{(8)}} = (\begin{matrix} - v \\ u \\ - i u \\ v \end{matrix}) .

(85)

We group them pairwise:

P^{^{A}} = {χ^{^{(1)}}, χ^{^{(2)}}}, P^{^{B}} = {χ^{^{(3)}}, χ^{^{(4)}}}, P^{^{C}} = {χ^{^{(5)}}, χ^{^{(6)}}}, P^{^{D}} = {χ^{^{(7)}}, χ^{^{(8)}}}

(86)

Each pair of the dotted contravariant spinors transform with the associated dotted Z matrix.

We define four two-column covariant objects:

χ_{_{A}} = (χ_{_{(1)}}, χ_{_{(2)}}), χ_{_{B}} = (χ_{_{(3)}}, χ_{_{(4)}}), χ_{_{C}} = (χ_{_{(5)}}, χ_{_{(6)}}), χ_{_{D}} = (χ_{_{(7)}}, χ_{_{(8)}})

(87)

And we define the corresponding two-column contravariant objects

χ^{^{A}} = (χ^{^{(1)}}, χ^{^{(2)}}), χ^{^{B}} = (χ^{^{(3)}}, χ^{^{(4)}}), χ^{^{C}} = (χ^{^{(5)}}, χ^{^{(6)}}), χ^{^{D}} = (χ^{^{(7)}}, χ^{^{(8)}})

(88)

Finally, we construct eight outer products that lead to the following quaternions:

χ_{_{A}} χ_{_{A}}^{†} \to Q_{_{A}} = {(+ + + +)}_{Σ}, {\dot{χ}}^{^{A}} {\dot{χ}}^{^{A †}} \to {\dot{Q}}^{^{A}} = {(+ - - -)}_{Σ} .

(89)

χ_{_{B}} χ_{_{B}}^{†} \to Q_{_{B}} = {(+ - + -)}_{Σ}, {\dot{χ}}^{^{B}} {\dot{χ}}^{^{B †}} \to {\dot{Q}}^{^{B}} = {(+ + - +)}_{Σ} .

(90)

χ_{_{C}} χ_{_{C}}^{†} \to Q_{_{C}} = {(+ - - +)}_{Σ}, {\dot{χ}}^{^{C}} {\dot{χ}}^{^{C †}} \to {\dot{Q}}^{^{C}} = {(+ + + -)}_{Σ} .

(91)

χ_{_{D}} χ_{_{D}}^{†} \to Q_{_{D}} = {(+ + - -)}_{Σ}, {\dot{χ}}^{^{D}} {\dot{χ}}^{^{D †}} \to {\dot{Q}}^{^{D}} = {(+ - + +)}_{Σ} .

(92)

Each form transforms in its own way with the matching Z or

\dot{Z}

matrix.

6. Complex Conjugated Forms

Let us write the complex conjugates of the quaternion forms:

Q_{_{A}} = {(+ + + +)}_{Σ} \overset{c . c .}{\to} {\dot{Q}}_{_{A}} = {(+ + + +)}_{Σ^{*}}

(93)

Q_{_{B}} = {(+ - + -)}_{Σ} \overset{c . c .}{\to} {\dot{Q}}_{_{B}} = {(+ - + -)}_{Σ^{*}}

(94)

Q_{_{C}} = {(+ - - +)}_{Σ} \overset{c . c .}{\to} {\dot{Q}}_{_{C}} = {(+ - - +)}_{Σ^{*}}

(95)

Q_{_{D}} = {(+ + - -)}_{Σ} \overset{c . c .}{\to} {\dot{Q}}_{_{D}} = {(+ + - -)}_{Σ^{*}}

(96)

{\dot{Q}}^{^{A}} = {(+ - - -)}_{Σ} \overset{c . c .}{\to} Q^{^{A}} = {(+ - - -)}_{Σ^{*}}

(97)

{\dot{Q}}^{^{B}} = {(+ + - +)}_{Σ} \overset{c . c .}{\to} Q^{^{B}} = {(+ + - +)}_{Σ^{*}}

(98)

{\dot{Q}}^{^{C}} = {(+ + + -)}_{Σ} \overset{c . c .}{\to} Q^{^{C}} = {(+ + + -)}_{Σ^{*}}

(99)

{\dot{Q}}^{^{D}} = {(+ - + +)}_{Σ} \overset{c . c .}{\to} Q^{^{D}} = {(+ - + +)}_{Σ^{*}}

(100)

Conjugate forms reside in the dual space that spanned by

Σ_{μ}^{*}

. They don’t have any counterpart in

S L (2, C)

. Dotted lower indexed and undotted upper indexed forms transform with

Z_{\dots}^{*}

or with

{({\dot{Z}}_{\dots})}^{*}

matrices respectively and all transformations obey the scheme

T_{A}

4-vector scalar product can be defined by using the dual forms in two equivalent ways. Let

Q

and

P

be two 4-vectors and let

Q_{_{X}}

and

P_{_{X}}

be the corresponding quaternions (

X = A, B, C, D

) :

Q \cdot P = \frac{1}{4} Tr (Q_{_{X}}^{T} P^{^{X}})

(101)

Or, noting that

P^{^{X}} = g P_{_{X}} g^{- 1}

, we can write also as:

Q \cdot P = \frac{1}{4} (Q_{μ \dot{ν}} P^{μ \dot{ν}})

(102)

where now indices refer to the components and the summation convention is implied.

In general, in order to get something real we have to use both

Σ

and

Σ^{*}

. As an example,

Λ = Z Z^{*} = Z^{*} Z

, is the real Lorentz transformation matrix.

7. Four Types of Transformations for SL(2, C)

We can suggest a similar formalism for

S L (2, C)

. Let

ξ_{_{A}}, ξ_{_{B}}, ξ_{_{C}}

and

ξ_{_{D}}

be covariant spinors:

ξ_{_{A}} = (\begin{matrix} u \\ v \end{matrix}), ξ_{_{B}} = (\begin{matrix} v \\ - u \end{matrix}), ξ_{_{C}} = (\begin{matrix} u \\ - v \end{matrix}), ξ_{_{D}} = (\begin{matrix} v \\ u \end{matrix})

(103)

and let

ξ^{^{A}}, ξ^{^{B}}, ξ^{^{C}}

and

ξ^{^{D}}

be contravariant spinors:

ξ^{^{A}} = (\begin{matrix} v \\ - u \end{matrix}), ξ^{^{B}} = (\begin{matrix} - u \\ - v \end{matrix}), ξ^{^{C}} = (\begin{matrix} - v \\ - u \end{matrix}), ξ_{_{D}} = (\begin{matrix} u \\ - v \end{matrix})

(104)

where

ξ^{^{A}} = ξ_{_{B}}

ξ^{^{B}} = - ξ_{_{A}}

ξ^{^{C}} = - ξ_{_{D}}

and

ξ^{^{D}} = ξ_{_{C}}

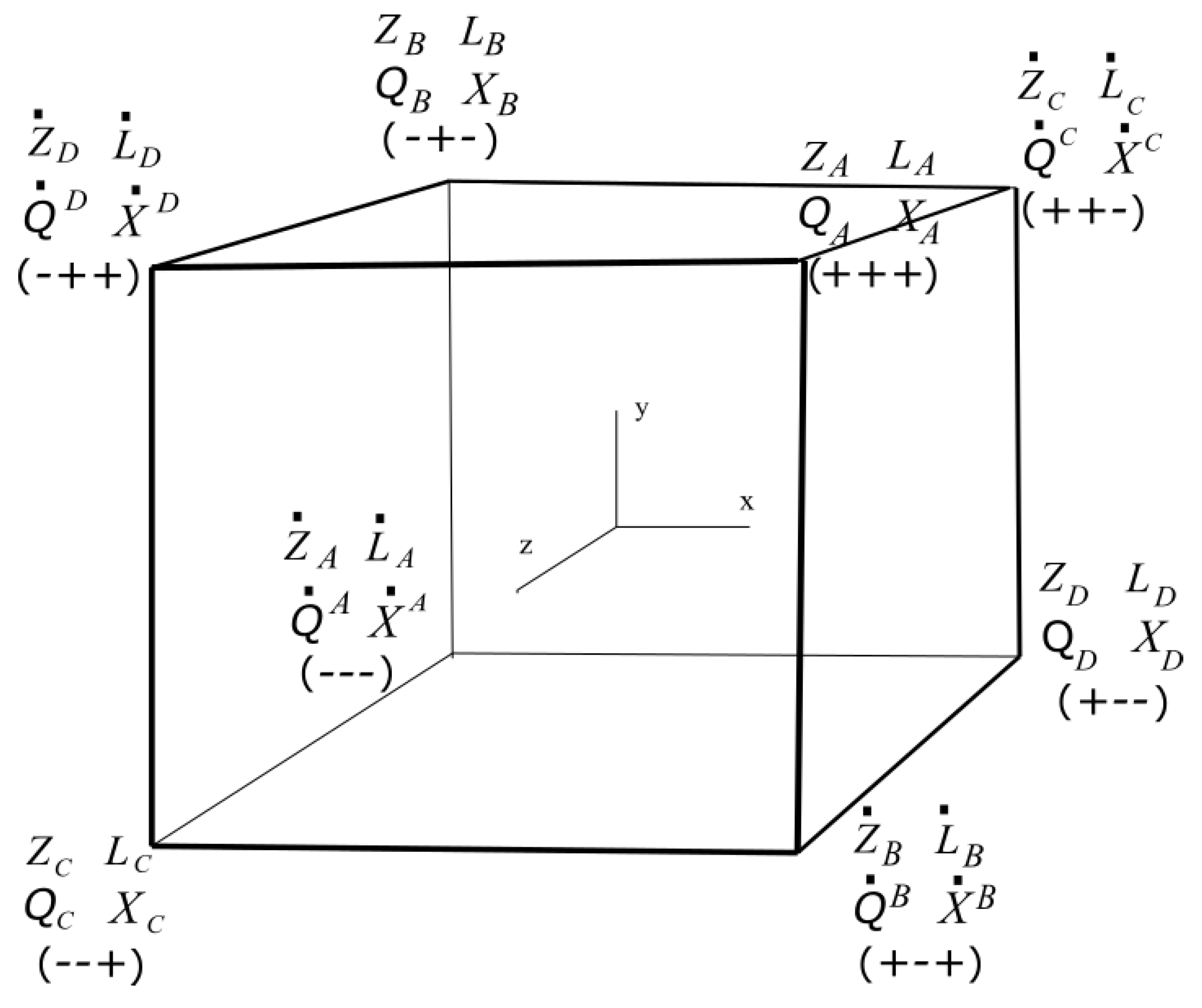
. This proliferation is necessary for the symmetry in Figure 1.

We have the following transformation properties:

ξ_{_{A}} \to L_{A} ξ_{_{A}}, ξ_{_{B}} \to L_{B} ξ_{_{B}}, ξ_{_{C}} \to L_{C} ξ_{_{C}}, ξ_{_{D}} \to L_{D} ξ_{_{D}}

(105)

{\dot{ξ}}^{^{A}} \to {\dot{L}}_{A} ξ^{^{A}}, {\dot{ξ}}^{^{B}} \to {\dot{L}}_{B} ξ^{^{B}}, {\dot{ξ}}^{^{C}} \to {\dot{L}}_{C} ξ^{^{C}}, {\dot{ξ}}^{^{D}} \to {\dot{L}}_{D} ξ^{^{D}},

(106)

{\dot{L}}_{A} = L_{B}^{*}, {\dot{L}}_{B} = L_{A}^{*}, {\dot{L}}_{C} = L_{D}^{*}, {\dot{L}}_{D} = L_{C}^{*}

(107)

All transformations obey the scheme

T_{A}

We have the following outer products:

ξ_{_{A}} ξ_{_{A}}^{†} \to X_{_{A}} = {(+ + + +)}_{σ}, ξ_{_{B}} ξ_{_{B}}^{†} \to X_{_{B}} = {(+ - + -)}_{σ}

(108)

ξ_{_{C}} ξ_{_{C}}^{†} \to X_{_{C}} = {(+ - - +)}_{σ}, ξ_{_{D}} ξ_{_{D}}^{†} \to X_{_{D}} = {(+ + - -)}_{σ}

(109)

{\dot{ξ}}^{^{A}} {\dot{ξ}}^{^{A †}} \to {\dot{X}}^{^{A}} = {(+ - - -)}_{σ}, {\dot{ξ}}^{^{B}} {\dot{ξ}}^{^{B †}} \to {\dot{X}}^{^{B}} = {(+ + - +)}_{σ},

(110)

{\dot{ξ}}^{^{C}} {\dot{ξ}}^{^{C †}} \to {\dot{X}}^{^{C}} = {(+ + + -)}_{σ}, {\dot{ξ}}^{^{D}} {\dot{ξ}}^{^{D †}} \to {\dot{X}}^{^{D}} = {(+ - + +)}_{σ},

(111)

It is worth noting that complex conjugating these forms does not yield anything new.

Appendix A

Appendix A.1. Various Forms of Z and L Matrices

Let us begin with the exponential form

Z_{A} = e^{R}

, where

R = - \frac{i}{2} \vec{π} \cdot \vec{Σ}

π_{i} = θ_{i} + i η_{i}

. Let

ϕ

be the complex angle defined as

ϕ = \frac{1}{2} \sqrt{π_{1}^{2} + π_{2}^{2} + π_{3}^{2}}

. Using the property

R^{2} = - ϕ^{2} I

Z_{A} = cos ϕ I - \frac{i sin ϕ}{2 ϕ} \vec{π} \cdot \vec{Σ} = (\begin{matrix} α_{0} & α_{1} & α_{2} & α_{3} \\ α_{1} & α_{0} & - i α_{3} & i α_{2} \\ α_{2} & i α_{3} & α_{0} & - i α_{1} \\ α_{3} & - i α_{2} & i α_{1} & α_{0} \end{matrix})

(A1)

where

α_{0} = cos ϕ, α_{1} = - \frac{i sin ϕ}{2 ϕ} π_{1}, α_{2} = - \frac{i sin ϕ}{2 ϕ} π_{2}, α_{3} = - \frac{i sin ϕ}{2 ϕ} π_{3} .

(A2)

Or in a compact form

Z_{A} = α_{0} Σ_{0} + α_{1} Σ_{1} + α_{2} Σ_{2} + α_{3} Σ_{3} = {(+ + + +)}_{Σ} .

(A3)

It is easy to show that

Z_{A}^{- 1} = α_{0} Σ_{0} - α_{1} Σ_{1} - α_{2} Σ_{2} - α_{3} Σ_{3} = {(+ - - -)}_{Σ} .

(A4)

and

Z_{A}^{†} = α_{0}^{*} Σ_{0} + α_{1}^{*} Σ_{1} + α_{2}^{*} Σ_{2} + α_{3}^{*} Σ_{3} = {(+ + + +)}_{Σ}^{*}

(A5)

where complex conjugation is applied only to

α_{μ}

The corresponding

L_{A}

L_{A} = (\begin{matrix} α_{0} + α_{3} & α_{1} - i α_{2} \\ α_{1} + i α_{2} & α_{0} - α_{3} \end{matrix})

(A6)

In terms of the Pauli matrices:

L_{A} = α_{0} σ_{0} + α_{1} σ_{1} + α_{2} σ_{2} + α_{3} σ_{3} = {(+ + + +)}_{σ} .

(A7)

In order to write

Z_{B}

we first find

L_{B} = {({\dot{L}}_{A})}^{*}

, where

{\dot{L}}_{A} = {(L_{A}^{- 1})}^{†} = (\begin{matrix} α_{0}^{*} - α_{3}^{*} & - α_{1}^{*} + i α_{2}^{*} \\ - α_{1}^{*} - i α_{2}^{*} & α_{0}^{*} + α_{3}^{*} \end{matrix}) = {(+ - - -)}_{σ}^{*} .

(A8)

From the definition

Z_{B} = A (L_{B} \otimes I) A^{- 1}

Z_{B} = (\begin{matrix} α_{0} & - α_{1} & α_{2} & - α_{3} \\ - α_{1} & α_{0} & i α_{3} & i α_{2} \\ α_{2} & - i α_{3} & α_{0} & i α_{1} \\ - α_{3} & - i α_{2} & - i α_{1} & α_{0} \end{matrix}) = α_{0} Σ_{0} - α_{1} Σ_{1} + α_{2} Σ_{2} - α_{3} Σ_{3} .

(A9)

Or, simply

Z_{B} = {(+ - + -)}_{Σ}

(A10)

We write various forms of Z and L matrices in compact forms:

L_{A} = {(+ + + +)}_{σ}, L_{B} = {(+ - + -)}_{σ}, {\dot{L}}_{A} = {(+ - - -)}_{σ}^{*}, {\dot{L}}_{B} = {(+ + - +)}_{σ}^{*} .

(A11)

Z_{A} = {(+ + + +)}_{Σ}, Z_{B} = {(+ - + -)}_{Σ}, {\dot{Z}}_{A} = {(+ - - -)}_{Σ}^{*}, {\dot{Z}}_{B} = {(+ + - +)}_{Σ}^{*} .

(A12)

Although, all types of Z and L matrices are in the same form, there is a very important difference between them. Because of the particular property of the Pauli matrices,

σ_{1}^{*} = σ_{1}, σ_{3}^{*} = σ_{3}

, but

σ_{2}^{*} = - σ_{2}

, we have the following relations:

L_{B} = {\dot{L}}_{A}^{*}, {\dot{L}}_{B} = L_{A}^{*} .

(A13)

For example,

{\dot{L}}_{A} = {(+ - - -)}_{σ}^{*} \to {\dot{L}}_{A}^{*} = {(+ - - -)}_{σ^{*}} = {(+ - + -)}_{σ} = L_{B} .

(A14)

On the other hand we do not have a similar property with

Σ

matrices, hence

Z_{B} \neq {\dot{Z}}_{A}^{*}, {\dot{Z}}_{B} \neq Z_{A}^{*} .

(A15)

The structural difference between

S L (2, C)

and

S L (4, C)

becomes more apparent when we write the matrices in exponential forms. In order to do this we have to define two types of

\vec{π}

{\vec{π}}_{A} = (π_{1}, π_{2}, π_{3})

and

{\vec{π}}_{B} = (- π_{1}, π_{2}, - π_{3})

L_{A} = e x p (- \frac{i}{2} {\vec{π}}_{A} \cdot \vec{σ}), L_{B} = e x p (- \frac{i}{2} {\vec{π}}_{B} \cdot \vec{σ}), {\dot{L}}_{A} = e x p (- \frac{i}{2} {\vec{π}}_{A}^{*} \cdot \vec{σ}), {\dot{L}}_{B} = e x p (- \frac{i}{2} {\vec{π}}_{B}^{*} \cdot \vec{σ}) .

(A16)

Z_{A} = e x p (- \frac{i}{2} {\vec{π}}_{A} \cdot \vec{Σ}), Z_{B} = e x p (- \frac{i}{2} {\vec{π}}_{B} \cdot \vec{Σ}), {\dot{Z}}_{A} = e x p (- \frac{i}{2} {\vec{π}}_{A}^{*} \cdot \vec{Σ}), {\dot{Z}}_{B} = e x p (- \frac{i}{2} {\vec{π}}_{B}^{*} \cdot \vec{Σ}) .

(A17)

Due to the properties,

- {\vec{π}}_{B} \cdot \vec{σ} = {\vec{π}}_{A} \cdot {\vec{σ}}^{*}

and

- {\vec{π}}_{B} \cdot {\vec{σ}}^{*} = {\vec{π}}_{A} \cdot \vec{σ}

, the relations in Eq.(A13) hold. But we do not have similar relations with the

Σ

matrices.

Appendix A.2. Two Types of Lorentz Transformations

Let

ξ_{_{A}}

and

χ_{_{A}}

be 2- and 4-component spinors that transform according to the scheme

T_{A}

, and let

V_{_{A}}

be a 4-vector that can be associated with the outer products

ξ_{_{A}} ξ_{_{A}}^{†}

and

χ_{_{A}} χ_{_{A}}^{†}

V_{_{A}}

transforms as

V_{_{A}} \to V_{_{A}}^{'} = Λ_{_{A}} V_{_{A}},

(A18)

Λ_{_{A}}

is the usual

4 \times 4

real Lorentz transformation matrix that corresponds to the scheme

T_{A}

Λ_{A} = A (L_{A} \otimes L_{A}^{*}) A^{- 1}

(A19)

where

L_{A} = L

. The corresponding

S L (4, C)

elements are

Z_{A} = A (L_{A} \otimes I) A^{- 1}, Z_{A}^{*} = A (I \otimes L_{A}^{*}) A^{- 1}

(A20)

Λ_{A} = Z_{A} Z_{A}^{*} = Z_{A}^{*} Z_{A} .

(A21)

Let

χ_{_{B}}

be a 4-component spinor that transforms according to the scheme

T_{B}

, and let

V_{_{B}}

be a 4-vector associated with the outer product

χ_{_{B}} χ_{_{B}}^{†}

V_{_{B}}

transforms as

V_{_{B}} \to V_{_{B}}^{'} = Λ_{_{B}} V_{_{B}},

(A22)

where

Λ_{_{B}}

is the

4 \times 4

real Lorentz transformation matrix that corresponds to the scheme

T_{B}

Λ_{B} = A (L_{B} \otimes L_{B}^{*}) A^{- 1}

(A23)

. where

L_{B} = {\dot{L}}_{A}^{*}

by definition. The corresponding

S L (4, C)

elements are

Z_{B} = A (L_{B} \otimes I) A^{- 1}, Z_{B}^{*} = A (I \otimes L_{B}^{*}) A^{- 1} .

(A24)

Λ_{B} = Z_{B} Z_{B}^{*} = Z_{B}^{*} Z_{B} .

(A25)

In this case, we don’t have a counterpart of

χ_{_{B}}

in the formalism of

S L (2, C)

Λ_{A}

and

Λ_{B}

preserves the Minkowski norm due to the relations

Λ_{A}^{T} η Λ_{A} = η

and

Λ_{B}^{T} η Λ_{B} = η

. Since

η

is real, these relations follow from the following more fundamental relations:

Z_{A}^{T} η Z_{A} = η, Z_{B}^{T} η Z_{B} = η .

(A26)

Appendix A.3. The Other Way Around

Suppose that, for

a = 1

a = 2

, the eigenvectors of

Σ_{3}^{*}

and

Σ_{3}

transform as follows:

χ_{_{(a)}} \to χ_{_{(a)}}^{'} = Z_{A} χ_{_{(a)}}, χ^{(a)} \to χ^{{(a)}^{'}} = {\dot{Z}}_{A}^{*} χ^{(a)}

(A27)

We know that these transformations obey the scheme

T_{A}

Suppose that, for

a = 3

a = 4

, the eigenvectors of

Σ_{3}^{*}

and

Σ_{3}

are mathematical objects that have different properties than the eigenvectors with indices

a = 1

a = 2

. Possibly, they describe physical objects of different kind which transform as

χ_{_{(a)}} \to χ_{_{(a)}}^{'} = Z_{B} χ_{_{(a)}}, χ^{(a)} \to χ^{{(a)}^{'}} = {\dot{Z}}_{B}^{*} χ^{(a)}

(A28)

In this case, these transformations will also obey the scheme

T_{A}

, and accordingly all null and non-null 4-vectors that correspond to the outer product forms and associated quaternion forms will transform in the usual way, i.e., they transform with

Λ_{A}

References

Han, D.; Kim, Y.S.; Noz, M.E. Polarization optics and bilinear representation of the Lorentz group. Physics Letters A 1996, 219, 26–21. [Google Scholar] [CrossRef]
K. N. Srinivasa Rao, Linear Algebra and Group Theory for Physicists, Second Edition, Hindustan Book Agency, New Delhi, India.
Sudha and, A.V. Gopala Rao, "Polarization Elements: A Group Theoretical Study".
D. Han, Y. S. D. Han, Y. S. Kim, Marilyn E. arXiv:physics/9703032V1[physics.optics] 28 Mar 1997.
Kuntman, E.; Kuntman, M.A.; Arteaga, O. Vector and matrix states for Mueller matrices of nondepolarizing optical media. J. Opt. Soc. Am. A 2017, 34, 80–86. [Google Scholar] [CrossRef] [PubMed]
Kuntman, E.; Kuntman, M.A.; Canillas, A.; Arteaga, O. Quaternion algebra for Stokes–Mueller formalism. J. Opt. Soc. Am. A 2019, 36, 492–497. [Google Scholar] [CrossRef] [PubMed]
Azzam, R.M.A. Propagation of partially polarized light through anisotropic media with or without depolarization: A differential 4 × 4 matrix calculus. J. Opt. Soc. Am. 1978, 68, 1756. [Google Scholar] [CrossRef]
Barakat, R. Exponential versions of the Jones and Mueller–Jones polarization matrices. J. Opt. Soc. Am. A 1996, 13, 1. [Google Scholar] [CrossRef]
Cloude, S.R. Group theory and polarization algebra. Optik 1986, 75, 26–36. [Google Scholar]
Hamilton, W.R. On a new species of imaginary quantities connected with the theory of quaternions. Proceedings of the Royal Irish Academy 1844, 2, 424–434. [Google Scholar]
Başkal, Sibel and Kim, Young S and Noz, Marilyn E, "Physics of the Lorentz Group," Morgan and Claypool Publishers (2015).
A.A. Bogush, V.M. A.A. Bogush, V.M. Red’kov, "On unique parametrization of the linear group GL(4.C) and its subgroups by using the Dirac matrix algebra basis," arXiv:hep-th/0607054V1 (2006).

1	We can define the spinor metric for $S L (4, C)$ as $i η Σ_{1}^{}$ or $i η Σ_{3}^{}$ if we like. These metrics also have the same properties of g.
2	We may use the generalized eigenvectors of $Σ_{1}$ or $Σ_{2}$ matrices as well, but, in that case, we have to employ the other forms of the spinor metric.
3	The upper dot on a spinorial object simply means complex conjugation: ${\dot{χ}}^{^{(a)}} = {(χ^{^{(a)}})}^{}$ . But, the upper dot on an element of $S L (2, C)$ or $S L (4, C)$ has a particular meaning. ${\dot{L}}_{A} = e x p (- \frac{i}{2} {\vec{π}}_{A}^{} \cdot \vec{σ}) \neq L_{A}^{}$ . Similarly, ${\dot{Z}}_{A} = e x p (- \frac{i}{2} {\vec{π}}_{A}^{} \cdot \vec{Σ}) \neq Z_{A}^{*}$ .

Figure 1. Reflections and inversions.

Σ^{*}

space is not shown.

Figure 1. Reflections and inversions.

Σ^{*}

space is not shown.

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content.

© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).

Copyright: This open access article is published under a Creative Commons CC BY 4.0 license, which permit the free download, distribution, and reuse, provided that the author and preprint are cited in any reuse.

MDPI Initiatives

Important Links

Choose an area of interest and we will send you notifications of new preprints at your preferred frequency.

Disclaimer

4-Component Spinors for SL(4,C) and Four Types of Transformations

Abstract

1. Introduction

2. The First and the Second Pairs and Their Transformation Properties

3. Outer Products of 4-Component Spinors and Null 4-Vectors

4. Quaternion Forms and 4-Vectors

5. Two More Pairs of Spinors

6. Complex Conjugated Forms

7. Four Types of Transformations for SL(2, C)

Appendix A

Appendix A.1. Various Forms of Z and L Matrices

Appendix A.2. Two Types of Lorentz Transformations

Appendix A.3. The Other Way Around

References

MDPI Initiatives

Important Links

Subscribe