By definition, a two-qubit system is a quantum system consisting of a pair of two-level quantum elements [1]. For example, a quantum system of two spins is one. In this case, the basic quantum state of an arbitrary two-qubit system will be written uniformly as:

Based on this, the general quantum state of a two-qubit system can be written in the following form: where λ_k, k = 1, 4 are the so-called complex amplitudes that satisfy the well- known normalization condition:

Next, let C be the complex plane. Then it is obvious that the four –vector of complex amplitudes Λ = (λ₁, λ₂, λ₃, λ₄) ∈ C⁴ – is an element of the four- dimensional vector (complex) space. As [2] is known, changes in the states of a quantum system are studied based on the analysis of changes in the values of the corresponding complex amplitudes –λ_k, k = 1, 4, over time.

Speaking differently, any change in the state of a quantum system is a conse- quence of changes in the phase space of events C⁴. In the language of mathemat- ics, this means that the original four-dimensional complex space C⁴ undergoes a linear non-degenerate transformation with the help of some unitary matrix ([2]) of the form:

Any unitary matrix of the form (4) is called a two-qubit quantum gate. If such a gate is known, then we can say that the quantum system goes from one state Λ = (λ₁, λ₂, λ₃, λ₄) to another state Ω = (ω₁, ω₂, ω₃, ω₄) like this:

It is clear that the larger the set of q(6)uantum gates at our disposal, the more we know about the various states of a quantum system. A set of gates is said to be universal if any unitary transformation can be approximated with any given accuracy by a finite sequence of gates from this set. The essential problem here is that gates of the form (4) are not permutation matrices, that is, in the general case for two gates A, B we get:

In this case, nothing can be said about which state of the quantum system was the previous and which was the next. Moreover, finding a gate (unitary matrix) of the form (4) in itself is still an unsolved, most difficult problem in matrix algebra. Nevertheless, in this article we pose the problem of extracting a commutative (Abelian) gate group from the entire set of unitary matrices of the form (4). If this problem is successfully solved, we will find and describe the continuum set of two-qubit quantum gates. Moreover, all valves will be per- mutable. This is a step of fundamental importance for solving applied problems of quantum informatics. We note that in what follows we will essentially rely on the mathematical methods of four-dimensional analysis, first described in the monograph [2

Let Z = (z₁, z₂, z₃, z₄), an arbitrary element of the event space C⁴. Then (see [2]), we can find a bijection of the following form:

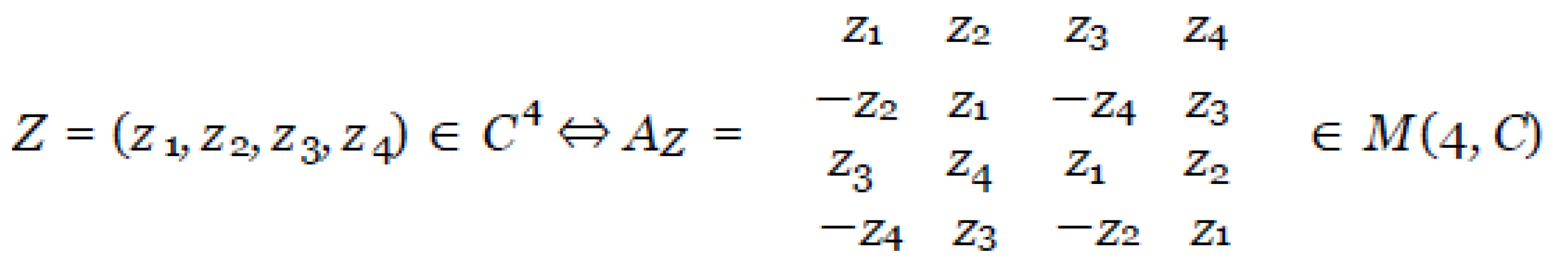
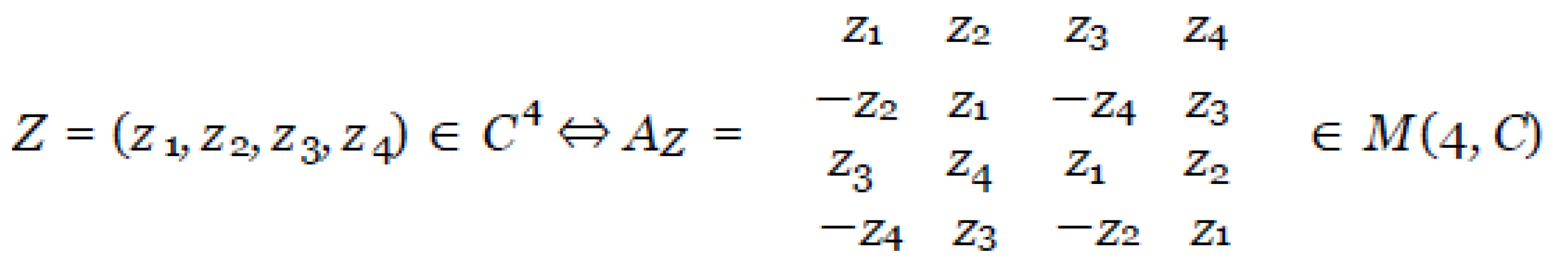
It is easy to show that the set of four-dimensional complex numbers C⁴ and the set of complex matrices M (4, C) are linear spaces over the field of complex num- bers. It is very important that the set M (4, C) consists entirely of permutation matrices, that is, the operation of matrix multiplication in it is commutative. This is easily verified.

Further, the key point is that there is an isomorphism between these sets, as linear spaces over the field of complex numbers. This follows automatically from the correspondence of the form:

The presence of isomorphism shows that any movement in the space of four- dimensional complex numbers C⁴ corresponds to a similar movement in the matrix sets M (4, C) and vice versa. And this means that we must look for gates (unitary matrices) precisely in the class of matrices M (4, C). This is the key finding of our research. The description of a two-qubit quantum system, a priori, is given not by any matrices of the form (4), but by unitary matrices of the form A_Z ∈ M (4, C) from the formula (7). The method for finding unitary (orthogonal) matrices of just this type is described in detail in the monograph [2].

The main result of this paper can be formulated as a theorem.

where the matrix elements are given by the formulas:

We see that the formulas (9) include four arbitrary angles α, β, γ, δ. If we take α = β = γ = δ = 0 in them, then we get an identical gate of the form:

All other gates (an infinite set) are also obtained by substituting the specific values of these four, free parameters. Theorem 1 is proved simply, based on the definition of a unitary matrix. Indeed, if A_ven is a unitary matrix, A^∗ is a complex conjugate matrix, then by definition we have: where E is the (4 × 4) identity matrix. The resulting system of nonlinear algebraic equations is solved explicitly. The general solution of this system has the form (9).

So, using the formula (9), we found an uncountable set of all possible gates. This means that it is now possible to measure all possible states of a quantum system. For the sake of clarity, we will indicate a number of simple gates: for example, there is a shift gate

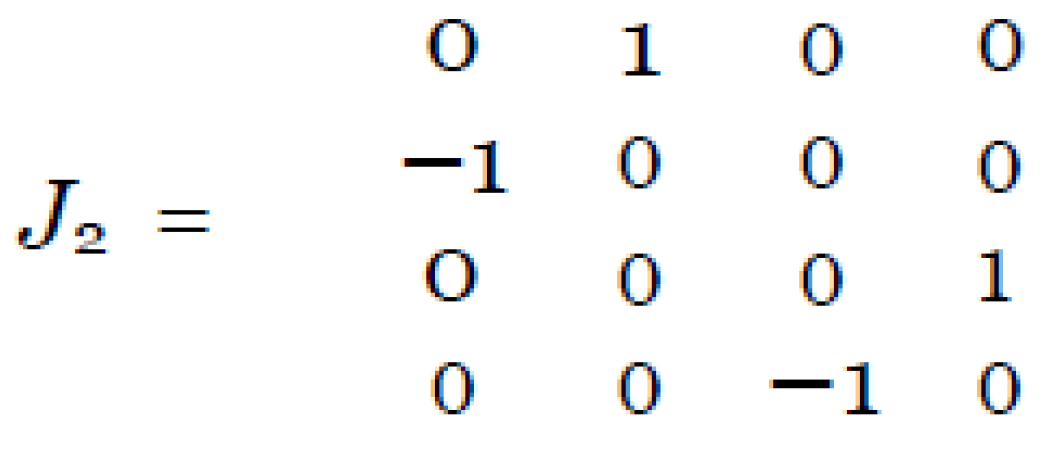
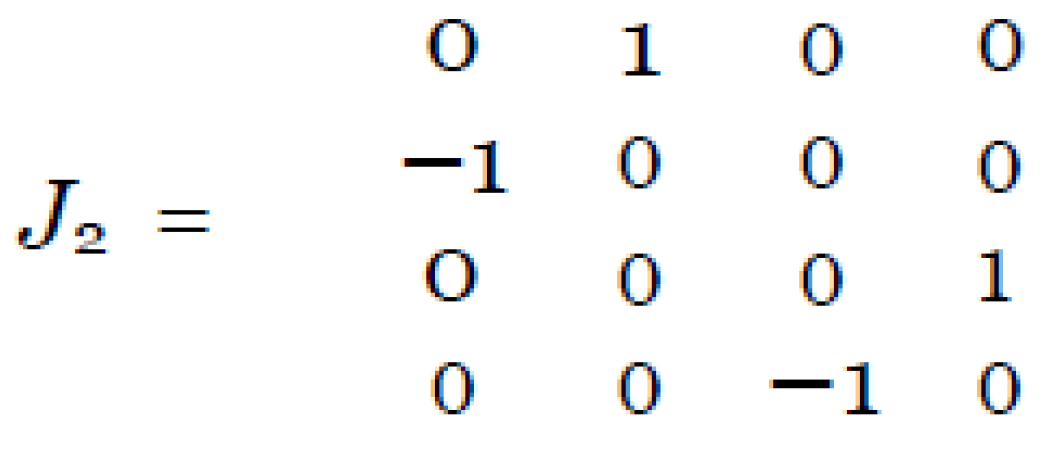
Further, it can be shown that all gates with real elements have the following form:

Similarly, all gates with purely imaginary elements have a similar form:

Since the set of gates form a commutative group, all possible products of these gates are also gates. Of fundamental importance is the fact that we have succeeded in describing the entire continuum set of two-qubit quantum gates, which has not been possible so far