Preprint
Review
Self-Starting Soliton-Comb Regimes in χ(2) Microresonators
Altmetrics
Downloads
186
Views
70
Comments
0
A peer-reviewed article of this preprint also exists.
supplementary.pdf (509.20KB )
This version is not peer-reviewed
Submitted:
17 April 2023
Posted:
17 April 2023
You are already at the latest version
Alerts
Abstract
Discovery of stable and broad frequency combs in monochromatically pumped high-Q optical Kerr microresonators caused by generation of temporal solitons can be regarded as one of the major breakthroughs in nonlinear optics during the last two decades. Transfer of the soliton-comb concept to χ(2) microresonators promises lowering of the pump power, new operation regimes, and entering new spectral ranges; scientifically, it is of a big challenge. Here we represent an overview of stable and accessible soliton-comb regimes in monochromatically pumped χ(2) microresonators discovered during the last several years. The main stress is made on lithium niobate based resonators. The overview pretends to be rather simple, complete, and comprehensive: It incorporates the main factors affecting the soliton-comb generation, such as the choice of the pumping scheme (pumping to the first or second harmonic), the choice of phase matching scheme (natural or artificial), the effects of temporal walk-off and dispersion coefficients, and also the influence of frequency detunings and Q-factors. Most of discovered nonlinear regimes are self-starting – they can be accessed from noise upon a not very abrupt increase of the pump power. The soliton-comb generation scenarios are not universal – they can be realized only under proper combinations of the above mentioned factors. We indicate what kind of restrictions on the experimental conditions have to be imposed to get the soliton-comb generation.
Keywords:
Subject: Physical Sciences - Optics and Photonics
1. Introduction
Generation of broad frequency combs, i.e. of a large sequences of equidistant coherent light lines, in monochromatically pumped high-Q Kerr microresonators [1,2,3,4,5] can be regarded as one of the main discoveries in nonlinear optics during the last two decades. Remarkably, the spectral positions of these lines do not generally coincide with (are not close to) the discrete resonator modes because of dispersion of the latter. This is why interpretation of the combs as a sequential parametric excitation of new resonator modes is rather fruitless. The right physical picture of the frequency comb in question is proven to be generation of a spatially narrow soliton propagating along the resonator rim with a constant velocity [5]. Fourier expansion of the light field employing its periodicity over the circumference ensures equidistance of the light lines with the frequency spacing , where R is the major resonator radius. Thus, the comb and soliton are two physical terms expressing different properties of the same nonlinear light state. Most probably, the soliton nature of broad frequency combs has no alternative.
The soliton-comb concept is nowadays generally accepted for resonators. It is applicable not only to glass based Kerr microresonators, but also to resonators made of crystals [5,6,7,8,9,10]. One can speak of the corresponding comb technology. Amount of publications on the combs can be estimated as . Note that the dissipative solitons in question are essentially different from (and more complicated then) the so-called conservative solitons associated typically with the nonlinear Schrödinger equation [11,12]. The dissipative solitons balance not only the dispersion broadening and nonlinear compression, but also the gain owing to external pumping and internal resonator losses.
Transfer of the soliton-comb concept to the microresonators, possessing the lower second-order nonlinearity, promises further lowering of the pump power, entering new spectral ranges, and new attractive operation regimes, see also below. This transfer cannot be straightforward because of specific and important features inherent in the nonlinearity [13]. In contrast to the case, elementary nonlinear processes involve not four but three light quanta. These processes are the thresholdless generation of the second harmonic (or sum frequencies) and the inverse threshold process of parametric oscillation. The comb generation can be viewed as a cascade of these processes. Running of them requires employment of the phase matching (PM) for the first harmonic (FH) and second harmonic (SH) resonator modes. The PM conditions in microresonators can in turn be either natural, relevant to certain light wavelengths, or artificial, using the so-called radial poling [14,15,16,17,18], see also below.
As soon as mutually coupled FH and SH modes are distinguished, we must deal with duality of the solitons and combs – each of them must consist of FH and SH components, see also Figure 1. The presence of sub-combs in the FH and SH frequency ranges can definitely be regarded as a positive feature. Also, two different pumping schemes (pumping into FH or SH resonator modes) have to be envisaged. These FH and SH pumping cases are substantially different. The presence of temporal walk-off between the FH and SH modes, which can be viewed as the difference of the corresponding group velocities, is also an important feature of the case. The effect of the walk-off on the soliton-comb generation is generally negative – it has to be minimized when possible. Furthermore, the presence of two dispersion coefficients, relevant to the FH and SH spectral ranges, has to be taken into account. Generally, the case provides much more room to the search for soliton-comb regimes as compared to the case. Conservative solitons in media, that can be regarded as a basis for dissipative cavity solitons, are reviewed in [19].
Axially symmetric microresonators are wide spread [17,18,20,21,22,23]. Their linear properties are largely common with those of the resonators [17,24,25,26]. Each resonator can be characterized by a major radius R, see Figure 1. The modes (whispering gallery modes) are localized near the resonator rim; the shape of the rim can be different. Each mode can be labeled by an integer azimuth number m and, additionally, two transverse modal numbers. All modal functions are proportional to , where is the azimuth angle. While exact analytical solutions exist only for the spherical case, approximate methods to calculate the modal frequencies, functions, and nonlinear interactions are well developed [24,27,28,29,30,31]. This is also relevant to the methods for coupling light into and out of resonator. Owing to the presence of a coupler (tapered fiber, prism, etc.), the inverse total (loaded) quality factor consists of internal and coupling contributions.
The most common lithium niobate (LN) based resonators are discs with the major radius mm, the minor radius , and quality factors [17,18]. The modes can be viewed as quasi-plane waves with frequencies , discrete wavenumbers , and an effective refractive index n depended on the modal numbers, R, and r. For mm, n is close to the bulk index ; the difference characterizes the effects of geometric dispersion. In the optical range, the azimuth number is . The frequency distance between neighboring modes with is much larger than the line width. As LN is a uniaxial optical material, modes with ordinary (o) and extraordinary (e) polarizations have to be distinguished.
Investigations of the SH generation and parametric oscillation in microresonators are also known [17,18]. In LN resonators, the PM condition for the SH generation, , can be fulfilled naturally at the FH wavelength nm with the use of o- and e-polarized FH and SH modes, respectively [14]. The parametric oscillation for this natural (birefringent) PM corresponds to the SH wavelength nm. Here and later subscripts 1 and 2 refer to the FH and SH ranges respectively. Employment of the radial poling of LN resonators [15,16,17] enables one to fulfill the quasi-PM conditions at practically any wavelength on demand via a controllable breaking the axial symmetry. It is also necessary to mention that, because of very small resonator line widths, fine tuning means (like the temperature or geometric tuning) have to be used to control the linear and nonlinear phenomena [17,18,25,32].
Both and combs originate from parametric instabilities inside the resonators. This is why continuous-wave monochromatic pump is able to generate temporal solitons. The threshold pump power for the instability is largely controlled by the relevant modal Q factors. Roughly speaking, in the and cases is proportional to and , respectively. For , the nonlinearity is typically dominating, and belongs to the W range [17,18].
Initial experimental attempts to get combs dealt with bulk PPLN systems [33,34,35,36] that are quite specific in physics. While some tens of comb lines were observed, neither solitons nor broad comb spectra were reported. Recently, first experimental efforts to generate the combs in microresonators have been made [37,38,39], no soliton-comb regimes are observed so far to the best of our knowledge. Furthermore, we can claim that this failure is in agreement with our theoretical results on the necessary conditions for realization of such regimes. Therefore, we see the main tasks of this mini-review in (i) determination of the conditions for the soliton-comb generation and () in predictions of the main properties of the soliton-comb states. Also, we will indicate where and why the necessary conditions were violated in the known experiments. Lastly, we will compare our findings with the known early predictions for the soliton-comb states [40,41,42,43]. As far as possible, the cases considered will be applied to experimentally realizable and important situations.
A considerable part of the literature on nonlinear phenomena in microresonators, see, e.g., [4,17,20,39], extensively employs the quantum-mechanical terminology and notation deeply within the classical domain, where applicability of the classical Maxwell equations is beyond any doubt. Being well developed and attracting, the quantum-mechanical formalism looks here out of place and confusing. We claim, that it can easily be replaced by the classical Hamiltonian formalism [44,45]. Keeping all merits of the quantum formalism, it is fully adequate to the situation.
2. Basic Equations, Parameters, and Definitions
2.1. Periodic and Antiperiodic States
Let first the pump frequency be close to a modal frequency and the FH-SH phase matching be natural, i.e. the azimuth symmetry takes place. Then, two variants have to be considered, namely pumping into a FH and into a SH mode. In the FH pumping case, the PM condition can be satisfied with a high accuracy only for a single azimuth number . It is implied that the frequency detunings and are much smaller in the values than the intermodal distance . The light electric field can be represented as
where and are slowly varying (and generally vectorial) FH and SH amplitudes (envelopes). Obviously, these amplitudes are -periodic in , and the SH azimuth number is even.
In the SH pumping case, the situation is different, as illustrated by Figure 2. The above PM condition can be fulfilled only if the azimuth number of the pumped mode is even (subfigure a). If is odd (subfigure b), two FH modes (with even and odd azimuth numbers) must be excited. Here, the simplest PM conditions are , . Let us use (regardless of parity of ) the ansatz
for the light field. Then, the first exponential factor is periodic for even and antiperiodic for odd . As the true light field is periodic, we must accept that the FH amplitude is antiperiodic for odd , i.e. . The SH amplitude is still periodic, . A dual FH-SH state with antiperiodic amplitude and periodic amplitude is named the antiperiodic state [46,47]. The antiperiodic sates (solutions) are topologically different from the conventional periodic states. No temporal evolution can make conversion between these two states.
We stress that the antiperiodic states are relevant to pumping into an odd SH mode. Otherwise, we have a periodic dual state, such that and .
Using above the vectorial notation, we wanted to stress the generality of the periodic and antiperiodic light states for the nonlinearity. While the FH and SH resonator modes can be different in polarization, one can always deal with scalar FH and SH amplitudes denoted in what follows as F and S.
2.2. Equations for the Modal Amplitudes
Now, we write down nonlinear equations for the complex modal amplitudes and relevant to the FH and SH frequency domains. It is assumed, as in all known theoretical studies, that the modal polarization and the transverse modal numbers stay the same within each of these domains. We start from the phase matching case relevant to an even azimuth number in the absence of radial poling (full azimuth symmetry). This takes place for the FH pumping, and is also applicable to the SH pumping into an even mode, see Figure 2a. It is useful to count the FH and SH frequencies from and . In accordance with Equations (1, 2), , in the FH pumping case, while , in the case of SH pumping. Furthermore, we introduce instead of and more convenient FH and SH modal numbers and . With these preliminaries, we have
Here the dots indicate differentiation in time, , , are modal decay rates, and are the nonlinear coupling coefficient and the internal pump amplitudes, and are Kronecker deltas. For the FH and SH pumping, we have and , respectively. The modal amplitudes and are defined such that and are the modal energies. This definition corresponds to the classical Hamiltonian formalism [44]. The dimensionless quantities and are nothing but the number of modal FH and SH light quanta in the classical limit. The presence of a factor of 2 in set (3) reflects the Hamiltonian nature of the nonlinear interaction – the total energy is conserving for and .
The coefficients and can be determined in a quite general manner via modeling of the modes and their coupling in and out of the resonator [29,31]:
where d is the relevant component of the conventional susceptibility tensor, is the effective interaction cross-section, is the pump power, is the coupling contribution to , and is a representative refraction index. Further details can be found in Sect. 1 (S1) of the Supplementary Materials.
The rate coefficients and can be expressed by the group velocities and dispersion coefficients if we use the expansion [48] with near the points and :
Parameters v and are expressed by the refractive index : and , where the prime indicates differentiation in . Knowledge of the dependences , with or without the geometric dispersion, enables one to calculate and as functions of and . Further details can be found in S2 of supplementary materials. Expressions for detunings depend on the pumping scheme. For the FH pumping, and . In the SH pumping case, and .
Consider now the remaining case of SH pumping into a mode with odd azimuth number , when two FH modes with even and odd azimuth numbers enter the phase matching condition, see also Figure 2b. The SH modes can be numerated again with integer . The only way to numerate symmetrically the FH modes is to introduce semi-integer number , such that . The form of sets (3) and (5) remains the same, and the only difference is in the expression for detuning : .
2.3. The Impact of Radial Poling
The radial poling enables one to adjust the phase matching to practically any desirable spectral point with no effect on the linear properties. In polar materials, like LN, the largest components of the quadratic susceptibility tensor, and , change sign under inversion of the spontaneous polarization. The periodic radial poling results in periodic sign changes of any of these components, as illustrated schematically by Figure 3a.
Let it be d with the absolute value . The periodic sign-changing function can be represented by the Fourier series
If is the number of poling periods, the nonzero components correspond to . In the case of ± symmetric radial domain structure ( duty cycle ratio), which is the most suitable for quasi-phase matching, only the Fourier harmonics with odd ratios are nonzero. For these harmonics , see also Figure 3b. The reduction factor decreases with increasing , but remains comparable with 1 for . Typically , and the neighboring peaks are well separated from each other.
Employment of the largest first Fourier harmonics of for quasi-phase matching corresponds to the PM condition for the SH generation. The sign "plus" is relevant to the most typical case of decreasing wavelength dependence of the refraction index [49]. We see that the SH azimuth number is even for even alternation number and odd for odd number . In the second case, SH pumping leads to the excitation of periodic states. In the case of odd combination , the above PM condition cannot be fulfilled. However, we can fulfill the PM condition leading to the excitation of the antiperiodic states. Thus, the quasi-PM via the radial poling results in a controllable shift of the FH azimuth number . Set (3) for the FH and SH amplitudes stays unchanged.
Note that microresonators made of commercial PPLN wafers [20] are not appropriate for the spectral adjustment in question. The spectrum includes here many significant peaks in addition to leading to unwanted interference of many cascading processes.
The possibility to adjust the phase matching to any spectral range raises the question about specific goals and properties of the radial poling. Figure 4 shows the dependences and for e-polarized modes in a LN based resonator with mm. The effects of geometric dispersion are very weak here. Zero walk-off point corresponds to nm, where the dispersion coefficients and are opposite in signs. Vicinity of this point, as will be shown below, is especially attractive for the soliton-comb generation. The number of periods , necessary to achieve quasi-PM at this point can be estimated as 284. Further details can be found in S3 of supplementary materials.
2.4. Fundamental Representation
The primary set of ordinary differential Equation (3) is convenient for numerical simulations. However, many fundamental features of the soliton-comb states become more evident if we transform it to a set of partial differential equations. This can be done in terms of the FH and SH amplitudes and defined by the Fourier expansions
For integer values of , this leads automatically to the periodic states, while for integer l and semi-integer j we arrive at a antiperiodic state.
Using Equations (3), (5), and (7) and introducing the rim coordinate , one can make sure that the periodic and antiperiodic states obey the same set of nonlinear equations for any phase matching scheme [46,47]:
where . At perfect resonant pumping and phase matching, we have . All parameters entering set (8) are known or controllable in experiment. For the solitons, set (8) plays the role similar to the role of the nonlinear Lugiato–Lefever equation for the soliton-comb states [4,5]. However, these two cases are fundamentally different.
Consider some important properties and useful transformations of set (8):
- –
- This nonlinear set admits dual steady-state solutions , circulating with a common velocity without shape changes. Solitons belong indeed to this class of solutions. Velocity has to be determined simultaneously with the shape of the envelopes.
- –
- Set (8) is written for a static coordinate frame. It is practical to rewrite it for a coordinate frame moving with velocity . To do this, it is sufficient to drop the term in the first equation and replace by in the second one. It is assumed from now on.
- –
- In a dual steady state propagating with a constant velocity , the modal amplitudes and oscillate in time as and . This elementary property is useful to control the establishment of dual steady states in numerical simulations, see Section 1.
- –
- Attempts to rewrite set (8) for the antiperiodic states using the replacements lead to restoration of the periodicity but also to an explicit z-dependence of the right-hand sides (non-autonomous system). This in inappropriate.
The structure of Equation (8) gives a hint about normalization of parameters relevant to the linear properties of the system. Such a normalization enables one to decrease the number of variable parameters. In what follows, we will use the following normalized detunings, walk-off, and dispersion parameters , , and :
As a rule, the actual values of detunings are restricted by the inequalities . The actual values of and substantially depend on the group velocity difference , the dispersion coefficients , and the decay rates .
For LN resonators, typical values of the decay rates are , such that . On contrary, we have typically . Exception from this rule is possible in a close vicinity of zero walk-off point nm. The decay rates and are typically not strongly different, such that assumption can be used sometimes to simplify modeling.
Generally speaking, the values of F, S, and also need some normalization. It is, however, different in the FH and SH pumping cases. We will provide the relevant information when necessary.
One more important property of set (8) is the presence of spatially uniform steady-state background solutions , [50]. In steady state, and . Obviously, such a dual background is possible only for the periodic states. The dual background is closely related both to the properties of solitons and to the thresholds of instabilities leading to soliton-comb formation. Omitting all derivatives in (8), one can easily come to a closed algebraic equation for that is, however, different for the FH and SH pumping cases. We have and for the FH and SH pumping cases, respectively. Dependence of on the pump amplitudes is presented graphically in Figure 5.
In both pumping cases, dependences are controlled by the phase , such that multi-valued solutions exist for sufficiently small .
Importantly, the dual background with always exists in the FH pumping case, see Figure 5a. At the SH pumping, the situation is different. For sufficiently small values of , there is a single background state, such that and , see Figure 5b. This difference has dramatic consequences for the dual comb-soliton states, see Section 4,Section 5,Section 6. Note also that Figure 5 gives a clear hint about normalization of the amplitudes and pump amplitudes in the FH and SH pumping cases.
Lastly, we mention two limiting cases for set (8).
- –
- In the limit , (no gain, no modal decay) we proceed to the conservative case reviewed in [19]. A number of exact soliton solutions are known here, but they are far from the subject of our study.
- –
- In the the dissipativeless driven limit at and , one can also obtain exact soliton solutions [51]. It turns out, however, that they all are unstable, i.e. cannot be realized.
3. Instability Thresholds
As known, generation of second harmonic is thresholdless – it corresponds just to forced oscillation caused by the nonlinearity. This thresholdless process becomes merely more efficient in the presence of FH-SH phase matching. On contrary, excitation of FH harmonics in the presence of SH modes is always a coherent threshold process. This explains a big difference in the properties of parametric excitation for the FH and SH pumping schemes in microresonators [50].
The simplest and most efficient is the SH pumping scheme. At sufficiently small pump powers (small values of at ), we deal simply with the linear excitation of the pumped mode . Its steady-state amplitude is ; detuning characterizes just the distance to the linear resonance. To see the effect of on the FH harmonics, we consider the first of Equation (3) with and . The quantities and with an arbitrary j obey obviously two linear differential equations. Can they have a nonzero steady-state solution? Using the zero determinant solvability condition for linear algebraic equations and Equation (9) for the normalized parameters, we obtain the threshold condition in the form [46,47,50]
where is the normalized pump amplitude. The same threshold equation can be obtained using a more general approach: Namely, one can set to determine the complex characteristic exponent from an algebraic solvability condition. Then, one can make sure that for , while for . Thus, small amplitudes decay below the threshold and grow exponentially above it. This fully justifies the use of the threshold EquatiEon (10).
Consider in some detail the threshold properties given by Equation (10). Remarkably, it includes neither the walk-off parameter nor the SH dispersion coefficient . The threshold equation refers to the FH pairs j and . The value of j can be integer or semi-integer depending on the type of solution in question. The thresholds are generally different for different . However, the minimal in values of are the same for all . The value of minimizing the threshold for a pair is ; it is substantially different for different . Using this property, one can excite selectively any pair on demand. The absolute threshold minimum, can be achieved indeed at , i.e. at the exactly resonant excitation of the pumped SH mode. It is clear now that parameter can be expressed by the pump power as .
Now we turn to the FH pumping scheme. It is relevant not only to the comb generation issue, but also to the SH excitation. Complexity of this scheme, compared to the previous one, has fairly simple reasons. The parametric instability requires sufficiently large values of the SH amplitude . They can be achieved, in turn, only for sufficiently large values of the amplitude of the pumped mode . Keeping this fact in mind, we consider what happens if we admit the presence of small perturbations and , such that and . Substituting these expressions in Equation (8) with , we see that the SH perturbation becomes linearly linked to the FH perturbation via the term , which was absent earlier. As the result, we must deal with a quartet of perturbations , , , instead of the former parametric pair . The characteristic equation for becomes a cumbersome four-degree algebraic equation including all linear parameters , , and [50]. The threshold properties become complicated, they cannot be covered by simple relations similar to Equation (10).
Nevertheless, a general overview and illustrations of the threshold properties, which do not pretend to be complete, are possible. We introduce first the normalized pump amplitude , this normalization is different from that relevant to the SH pumping. The presence of parameter , ranging from 0 to very large values, in the threshold equation, raises question about the effect of walk-off. It turns out that the lowest threshold value of at zero walk-off () corresponds to , i.e. to an internal instability of the dual background [50]. For detunings , this value is minimal, . With increase of , some quartets with become quickly the easiest to excite. However, the optimal values of depend significantly on the dispersion coefficients. This is illustrated by Figure 6 obtained with the use of numerical methods.
SubFigure 6a is plotted for and representative dispersion parameters relevant to the quasi-PM in LN. Within a broad range of , the lowest threshold corresponds to harmonics with very large values of . SubFigure 6b corresponds to the natural PM, where the dispersion coefficients are negative and relatively large in the absolute values. The effect of is strongly different here and it strongly depends on . The reason is in competition of the walk-off and dispersion terms in the rate coefficient entering Equation (3) for S. Anyhow, selective excitation of modes with is practically impossible. The effects of nonzero detunings can also be investigated numerically. They also do not facilitate selective excitation of harmonics with small values of .
4. Numerical Methods
We simulated predominantly set (3) in the coordinate frame moving with velocity using the fourth-order Runge-Kutta method. Initially, for verification purposes, we solved also set (8) of partial differential equations using the step-split Fourier method. The results were practically equivalent. The number of Fourier harmonics taken into account (and the mesh points in ) ranged from 32 to 1024 within each of FH and SH frequency domains. Accuracy of the calculations was controlled by changing the time step and the number of harmonics. Different values of the variable input dimensionless parameters of the system () were used. With harmonics calculated, one can investigate temporal evolution of the spatial profiles and comb spectra . Also, it is possible to verify establishment of steady states moving with a constant velocity and, if applicable, determine this velocity .
Three main problems were considered numerically:
– We verified numerically the analytical results relevant to the instability thresholds. To do so, we used initial conditions with very small random complex values of and to see the initial exponential growth or decay of for different values of . Coincidence of the analytical and numerical results with a high accuracy was always achieved.
– We verified stability of the dual soliton solutions obtained analytically in [46,51] for the known limiting cases. As initial conditions, we used Fourier transforms of the relevant analytical expressions for and . Numerical analysis has shown that all the analytical solutions are unstable: Temporal evolution leads inevitably to irregular spatial profiles and comb spectra.
– We tried to generate stable steady-state soliton-comb states starting from very small random complex amplitudes and . As a rule, evolution of our nonlinear systems well above the threshold leads ultimately, after a stage of exponential growth, to highly irregular saturated behavior. For an abrupt switching the pump on, rough features of this irregular behavior depend also on the choice of initial conditions. Nevertheless, for many well defined choices of the input parameters, proper pumping scheme, and not very abrupt switching of the pump on, temporal evolution leads reliably and uniquely to multi-parametric families of comb-soliton states. Below we describe the corresponding adiabatic procedure of growing of soliton-comb states as applied (for definiteness) to the SH pumping case.
Let FH harmonics possess the lowest threshold. For , we have then the steady state with and zero other harmonics. Switching to slightly above , we obtain a steady state including and only few relatively small other essential FH and SH harmonics. After that, the steady-state harmonics are used as new initial conditions at a slightly larger value of . This procedure is repeated then many times to come to a steady state well above the threshold. The number of FH and SH harmonics taken into account has to be chosen properly. The final state is insensitive to the choice of initial random amplitudes. The states achieved in this way can be qualified as self-starting steady states; their stability is doubtless. Let us stress that achievement of the soliton-comb states with the adiabatic procedure is not guaranteed. However, in many cases it takes place.
Restriction on the rise time of the pump, , necessary to achieve the steady state is found to be not very obligating. It is sufficient to fulfill the inequality , which corresponds typically to s. Note also, that soliton-comb states can be achieved often in a few steps of step-wise increase of the pump power.
Establishment of soliton-comb steady states and the corresponding velocity can be quantified with a high accuracy using a correlation method. We use the fact that Fourier harmonic ( or ) known in the coordinate frame moving with velocity can be recalculated in the frame moving with an arbitrary velocity through multiplication by . Let us introduce the dimensionless error parameter
where refers to a coordinate frame moving with velocity , t is the calculation time and is a variable time shift. Obviously, turns to zero only when we deal with the steady state and, simultaneously, . The error parameter calculated for modestly large evolution times, , and minimized over shows extremely small values () caused by the numerical noise, see also below. For smaller t, i.e., during the transient stage, it is larger by many orders of magnitude. We have thus a numerical tool to control the establishment of steady states and determine precisely velocity .
Figure 7 shows three examples of temporal evolution of relevant to our adiabatic procedure applied to the antiperiodic case at s−1, , and parameters , , representative for a close vicinity of the zero walk-off point in LN based resonators. The harmonics in question are . The normalized pump amplitude takes sequentially 17 values above the threshold: , , …, and 30. SubFigure 7a,b,c exhibit evolution of after taking the first two and the last values at , , and . The most general feature this evolution is always the same: It is an almost exponential decrease of from initial values of the order of 1 to extremely low values . Note also some quantitative details. The longest evolution, subFigure 7a, starts from modal noise. It takes about for the harmonics to grow exponentially and reach the saturation. The corresponding evolution time can be identified with the rise time of near-threshold optical parametric oscillation. The further evolution runs, which start from regular distributions of the FH and SH harmonics achieved during the previous runs, occur substantially faster. Our choice of 17 steps of evolution to reach the state with is largely arbitrary. In many cases, the same final state can be achieved in a few steps.
In the next sections we will see in details the spatial and spectral properties of the dual states grown with the use of our adiabatic procedure.
5. Soliton-Comb States for SH Pumping
Below we report the results on soliton-comb generation obtained with our adiabatic procedure in the SH pumping case for LN resonators [47,52]. The -dependences of , , , and and the spectra and are of our prime interest. Also, we are interested in dependences of the soliton-comb characteristics on the pump amplitude , the walk-off parameter , and other variable parameters of the system. Generally, the soliton position in the resonator depends on the choice of initial random amplitudes. Since the azimuth angle can be counted from any point, we can chose its zero point as convenient.
We start our report with the case of quasi-PM via the radial poling. Here, the pump wavelength can take practically any value. In particular, it can be made arbitrary close to the zero walk-off point nm. This case is especially important. For sufficiently small values of , the group velocity difference is given by in accordance with Figure 4a. Correspondingly, for nm and typical values of R and the walk-off parameter ranges from 0 to large negative and positive values, while the dispersion coefficients stay practically constant and opposite in signs, , .
5.1. Antiperiodic solutions near nm
Figure 8 corresponds to an antiperiodic steady state moving with velocity . It is relevant to zero walk-off (, ), , zero detunings (), mm, and decay rates , s−1 (, ). The total number of harmonics taken into account is 1024. SubFigure 8a,b show the central parts of the normalized FH and SH intensity profiles – far from the center the intensities stay constants. It is evident that we deal with a narrow dual dark-bright soliton. Far from the soliton core, the normalized values of and ( and 1) nicely correspond to the dual background considered in Section 2.
At the first sight, this feature is not consistent with the absence of the dual background for the antiperiodic states. The point is that the amplitude tends to the opposite left and right constant values far from the center, and these values can be found from Equation (8). The facts that (i) the intensity profiles are even in and (ii) the FH intensity turns exactly to zero at the soliton center are exclusively due to our zero walk-off assumption.
SubFigure 8c,d exhibit -dependences of the phases and . While an overall change of across the resonator is general for the antiperiodic states, the jump at is typical only for . More specifically, the line on the complex plane crosses the zero point at a finite angle because of the symmetry leading to an abrupt jump of the phase. For , the function is smooth. As concerned the SH phase , it shows only smooth (but large) deviations from zero near the soliton center, see Figure 8d.
The FH and SH comb spectra are shown in subFigure 8e,f. They are symmetric in j and l and very broad. The normalizations of F and S are slightly different and specific for the SH pumping case. On the other hand, they provide the maximum values of and of the order of 1. The shapes of the FH and SH spectra are notably different. The central peak in Figure 8f) is due to the pump. To quantify the comb spectra, we define the number of significant FH and SH lines ( and ) whose normalized intensities are above the cut-off level . This characteristic is conditional and rather useful; the lines of this strength are typically well above the light noise. In our case and corresponding to well developed dual combs.
With increasing , the dual soliton is progressively narrowing and the comb spectra are getting broader. No deterioration of the soliton-comb states was seen up to the maximal investigated values . Despite an apparent simplicity of the soliton profiles at , no analytical solutions for them is known so far.
It is not difficult to estimate the numbers of light quanta and in the FH and SH modes for the data of Figure 8e,f. Using Equation (4) for and setting m2, we obtain that they are roughly of the order of for . Thus, we are deeply within the classical range. This is valid also for the subsequent illustrations. At the same time, far tails of the comb spectra can experience quantum effects.
Next, we consider the effects of nonzero walk-off. Nonzero values of influence neither the thresholds nor the outcome of the adiabatic procedure – the system still evolves to a single-soliton steady state. The most evident effects are (i) nonzero soliton velocity, , and (ii) asymmetry of the soliton profiles and the comb spectra. The larger , the stronger is this asymmetry. This is illustrated by Figure 9. Compared to Figure 8 we have changed from 0 to nm, this corresponds to . Asymmetry of the soliton profiles and comb spectra is already evident. Furthermore, the soliton profiles acquire pronounced tails. The value of is now far from zero, and is about two times smaller than earlier. At the same time, the background values of the FH and SH intensities are the same, and the total number of the comb lines is even slightly larger than it was at . Changing sign of results in the mirror reflection of the graphs of Figure 9 about the central vertical line and in changing sign of the soliton velocity .
To gain new insights, we consider color maps of velocity parameter and of the total comb line number on the plane for a few representative combinations of the decay rates and , see Figure 10. Three first subfigures of the upper row represent the maps of for the combinations (a1), , (b1), and (c1) with s−1. Subfigures a2, b2, and c2 of the lower row represent the corresponding maps of the total comb line number N. Subfigures d1 and d2 give additionally cross-sections and at for our combinations of and .
As evident from the maps, both and N grow monotonously with increasing . As concerned dependences of these parameters on (or ), they are decreasing only outside a vertical central strip, whose width depends on . The decrease of and persists for nm. In short, large values of the walk-off parameter, are harmful for the soliton-comb generation in question.
The range of small (or ), illustrated in some details in the subfigures d1 and d2, is worthy of attention. The dependences and at large values of are not always continuous. This means that small variations of can lead to notably different results when adiabatically increasing . Continuous changes of and at in Figure 10 take place only for . The optimum value of and the corresponding largest peak values of N take place for . In accordance with our definition of they correspond to the largest (and the pump power). Anyhow, the increase of N via optimization of is not very large. Note lastly that the wavelength range of 20 nm relevant to Figure 10 strongly exceeds the intermodal wavelength distance of nm, so that the indicated spectral features are not available for the standard experimental fine tuning means.
Now we turn to the effects of the frequency detunings. These effects are of two kinds. One can consider the effects of on initiation of soliton-comb states via an adiabatic increase of . These strong and important effects are considered in Section 5.2. Alternatively, we can vary slowly upon reaching the above considered soliton states at large values of . This issue, which is largely (but not only) about the area of existence of developed soliton-comb states, is considered below.
We have extended our adiabatic procedure for slow independent changes of and . In fact, two similar scanning procedures were used. Within the first one, we determined initially the soliton velocity and the comb line number N by increasing and decreasing at . After that, starting from a point , we increased and decreased detuning . The achieved steady-state values of the amplitudes and were used as new initial conditions in each step of changing or . Within the second procedure, the scanning order was inverted: initially we varied at , and after that increased and decreased starting from points . We have verified whether the functions and depend on the scanning order. Also, we have made sure that each steady state on the plane is still relevant to a single-soliton antiperiodic state.
Consider first the case zero walk-off, . In our coordinate frame moving with velocity , the positive and negative propagation directions are equivalent, such that the soliton velocity difference is expected to be zero. Surprizingly, we have found that this is not always the case. Figure 11a represents color map of for , , and s−1 (as in Figure 8). The uniformly dark-blue colored lower and upper parts, which are separated from the rest by two slanted borders (bifurcation lines), correspond to within an accuracy of MHz. Between these borders we have a smooth distribution whose scale is comparable with that of Figure 10. This distribution practically does not depend on the scanning order. But what is the sign of ? We have found that it randomly depends on fine particularities of our calculation procedure, such as the presence of very weak remnant
velocity perturbations. In essence, we have a clear example of the spontaneous symmetry breaking, when the state with a high symmetry () becomes unstable against the excitation of one of two equivalent states of lower symmetry with . One of the most known examples of such a symmetry breaking is the ferroelectric second-order transition below the Curie temperature.
Let us now take a look at the corresponding color map of N, Figure 11b. The distribution is rather smooth. The lower bifurcation line of Figure 11a) is barely seen here, while the upper line is pronounced. Remarkably, increase of at is favorable for the comb in spite of the increasing instability threshold, see Equation (10). The characteristic scale in is here about 1, which strongly exceeds the scale relevant to the changes in . This assertion is general for ; it is justified by plotting maps of for different values of and different combinations of and . In short, we have a vast family of single-soliton antiperiodic states continuously depending on the input parameters.
Our attempts to generate periodic soliton-comb solutions at failed. The reason and the possibilities to get new solutions are indicated in Section 5.2.
5.2. Selective Generation of Periodic and Antiperiodic Milti-Soliton States
The idea for generation of periodic and antiperiodic multi-soliton states roots in the threshold conditions of Section 3 and a simple observation. The point is that the FH profile of Figure 8 with a sharp minimum emerges with increasing as a continuous (without bifurcations) development of the near-threshold profile corresponding to the lowest threshold for FH harmonics with . One can suggest thus that the spatial structure of developed solitons can be determined by the threshold conditions.
To develop this idea, we consider the excitation diagrams of Figure 12.
Diagrams a) and b) correspond to the antiperiodic and periodic cases. The cyan circles on the horizontal axis separate the regions where the lowest thresholds correspond to different values of the FH harmonic number . Within a broad range in diagram a), , including the zero point, an adiabatic increase of is expected to lead to single-soliton antiperiodic states. This is in line with the above considered results. For , we can expect three-soliton antiperiodic states, etc.
The periodic case, diagram b), is expected to be different. In the range , including the zero point, the lowest threshold corresponds to , i.e. to the generation of the dual background. As this background is stable, no periodic solitons are expected. However, in the range , where the lowest threshold corresponds to , two-soliton periodic state is expected. Similarly, we can hope to generate four-soliton periodic states for . As concerned detuning , it plays a passive role by increasing non-selectively all thresholds according to Equation (10).
Now we consider the results of the corresponding numerical experiments on selective growing of multi-soliton states [53]. Figure 13 shows the periodic two-soliton state grown via adiabatic increase of for , s−1, , and . Remarkably, the phase shows two opposite -steps when crossing each of two narrow soliton areas. Thus, our periodic two-soliton solution consists of two equally spaced mutually repelling antiperiodic solitons. This structure emerges every time spontaneously without imposing the antiperiodic conditions. Velocity of the state is . The FH and SH comb spectra, shown in Figure 13c,f, are symmetric and structurally similar to those of Figure 8e,f. Because of -periodicity in , the comb line spacing is , it is doubled compared to the single-soliton case. Correspondingly, the number of significant comb lines (above ) is about two times smaller than it would be in the single-soliton antiperiodic case at .
Changing sequentially to and , which corresponds to the lowest threshold for and 3, we have grown periodic four- and six-soliton states. They all consist of pairs of the same antiperiodic solitons. The comb line spacing increases with accordingly. Further increase of does not lead, however, to new multi-soliton states. Temporal development tends to break such states into the former two-, four-, and six-soliton solutions. This feature is not related to insufficiently small time step and/or insufficiently large number of harmonics taken into account. Also, we mention the case relevant to the lowest threshold for . Our adiabatic procedure leads here, as expected, to a dual background steady state .
Generalization to the case of nonzero walk-off () and different decay rates and is rather trivial. We still have multi-soliton periodic solutions consisting of pairs of the former antiperiodic solitons moving with velocity .
Now we switch to multi-soliton antiperiodic states relevant to semi-integer values of j, see Figure 12a. Figure 14 shows representatively the FH and SH intensity profiles for the antiperiodic three-soliton state grown for and . The other input parameters are the same as in Figure 13.
Each of three equally spaced solitons has a familiar structure with oscillating tails. Owing to a relatively large negative value of , the tails are strongly pronounced and the spatial asymmetry is inverted as compared to Figure 9. The soliton velocity difference cm/s. Interestingly, antiperiodic five- and seven-soliton states can be easily grown for and in spite of strong tail overlaps. This contrasts with multi-soliton periodic states.
5.3. Natural PM: The Absence of Solitons
The natural phase matching, that can be realized in LN based resonators at nm, is of special interest. Avoiding complicated procedure of the radial poling and availability of tunable laser sources in this range make it very attractive. Furthermore, it was indicated [54] that the value of the walk-off parameter can be substantially decreased for sufficiently small microresonators ( mm) using the effects of geometric dispersion. This implies the use of proper Sellmeier equations for [49,55] and relations for the modal frequencies including the size corrections [29], see S2 of supplementary materials for details. It turns out, however, that the main problem with the use of natural PM for the soliton-comb generation is the dispersion issue [56]. In contrast to the above considered cases, the FH and SH dispersion parameters are both negative: and of cm2/s.
Aiming to see the role of the signs of the dispersion parameters, we conducted first some test numerical experiments. For s−1, , , the absolute values , and four combinations of the signs of , we have launched our adiabatic procedure for growing of the antiperiodic soliton states. In two cases with , we got the same known single-soliton solution, see Figure 8. In two other cases with we obtained no soliton states, and the comb spectra remained undeveloped. Additionally, we varied the values of input parameters , , and and switched from the antiperiodic to periodic case. No soliton solutions were found as well. The sign of the product is thus crucial for the soliton formation.
6. Solutions for FH pumping
The case of FH pumping is important for experiment, because it corresponds to the conditions of SH generation, including the tuning issues. At the first sight, switching to the FH pumping case is trivial – it is sufficient to set and in sets (3) and (8). The normalized pump amplitude is here , and only the periodic states are allowed. While the threshold conditions are more complicated now, they can be evaluated numerically for any set of the input parameters. With these preliminaries, we consider the most important cases [56].
We start from vicinity of the zero walk-off point nm. The value of the walk-off parameter is controlled here by the wavelength difference , and the dispersion coefficients can be treated as constants. Setting () and brings us to the situation where the lowest instability threshold, , corresponds to , i.e. to auto-oscillations of the dual background [50]. Figure 15 illustrates what happens with increasing when applying our adiabatic procedure at s−1.
Close enough to the threshold we do see auto-oscillations of and , see Figure 15a. The oscillation amplitude and period increases and decreases with increasing , respectively, while harmonics with remain very small. For , these spatial harmonics become notable. Their amplitudes grow gradually, and the number of significant comb lines reaches for with the comb line distance . The corresponding normalized FH and SH intensity profiles are shown in Figure 15b by lines 1 and 2. Further increase of results, however, in a sharp transition to an irregular spatial behavior, see subFigure 15c,d. This contrasts strongly with the case of SH pumping. Variations of the input parameters and do not change this unfavorable scenario.
With increase of , the threshold values with quickly decrease and become lower than the internal instability threshold. This is illustrated by Figure 6a relevant to our input parameters. For , the modes with possess the lowest thresholds. Application of our adiabatic procedure to the case , results, e.g., in the excitation of -periodic dual state (with six intensity peaks) near the threshold. With increase of , it transforms via a bifurcation to a periodic state (at ). For the system shows again an irregular spatial behavior. The number of significant comb lines N does not exceed 25 for . Employment of nonzero detunings does not help much.
For , the threshold values approach 2 from above, and selective excitation of harmonics with becomes possible. However, the thresholds for are only slightly higher. Consider representatively the case , , and relevant to the lowest threshold for . Applying the adiabatic procedure, we have a -periodic dual state near the threshold. For and 18 it transforms sequentially via bifurcations to a - and -periodic states. Correspondingly, the comb line distance increases from 2 to 4 and, lastly, to 8. Figure 16a,b show complicated steady-state -periodic asymmetric FH,SH intensity profiles for , while Figure 16c,d exhibit the corresponding comb spectra.
While the steady state found shows a regular spatial behavior, it cannot be regarded either a soliton state or a pronounced comb state.
For , the above scenario of decreasing spatial period and increasing the comb line distance with increasing remains the same. At , we have a periodic dual state with a modest the comb line number . Suppression of SH harmonics with and asymmetry of the intensity profiles and comb spectra are strongly pronounced.
Lastly, we touch the case of natural PM at nm, when small and/or modest values of are available owing to the geometric dispersion, see S2 of supplementary materials for details. The values of the quality factors are expected to be substantially larger than they are at 2698 nm [57] leading to larger absolute values of the dispersion coefficients . As representative values, we chose mm, , and , which corresponds to . The relevant dependences are presented in Figure 6b. While they differ from the threshold dependences of Figure 6a, one important feature is the same: It is impossible to excite selectively the modes with for .
A large number of our numerical experiments, conducted at different values of and , show persistently the same scenario: Increasing above the threshold leads (via bifurcations) to dual states with smaller and smaller spatial periods (larger and larger comb line spacing) and, ultimately, to an irregular spatial behavior. The larger , the longer is the interval of with the regular behavior. On the other hand, the number of significant comb lines N within this interval remains modest; it does not exceed 15. No signs of soliton-comb states were detected.
7. Discussion
Experimental and theoretical studies of dissipative soliton-comb states is a vast, perspective, and almost unexplored research area. It is strongly different from the corresponding Kerr soliton domain, which is, in turn, much broader the studies of conservative Kerr solitons. The nonlinearity is fundamentally different from Kerr’s one resulting in a number of specific qualitative features including duality of the nonlinear states and the necessity to employ the phase matching and to minimize the temporal walk-off. The number of important variable parameters of the system is significantly larger than it is in the Kerr case. Deficit of analytical tools forces researches to rely on numerical methods.
Combining analytical and numerical tools and approaches, we have predicted an unprecedently vast family of dual soliton-comb steady states. Using the term "vast", we mean a continuous dependence of the parameters of the state on all input parameters – the pump power, the modal quality factors, the frequency detunings, and the walk-off. The found states are self-starting, i.e., easily accessible via nor very abrupt switching the pump on and changing the detunings. The soliton-comb states are divided into two topological classes – periodic and antiperiodic depending on the parity of the azimuth number of the pumped SH mode. The steady state solutions can be single- and multi-soliton ones. All our soliton-comb states are dissipative, they cannot be reduced to (obtained from) the known conservative soliton solutions.
Remarkably, the predicted self-starting soliton-comb states are allowed only for the SH pumping. They require opposite signs of the FH and SH dispersion coefficients. This condition is fulfilled near the zero walk-off point nm in LN based resonators. However, the phase matching at this point requires a precise radial poling. Being available, it represents a serious technical problem. For the natural phase matching in LN resonators, the necessary condition is not fulfilled, and soliton-comb states seem to be forbidden. Despite strong efforts, no soliton-comb solutions were found for the FH pumping. This contradicts initial expectations about similarity of the FH and SH pumping cases but has profound reasons behind.
Both intuitive considerations and numerical modeling indicate that substantial differences in the FH-SH group velocities , which are typical of resonators, are unfavorable for the soliton-comb generation. Quantitatively, this means that the combination has to be not much larger than 1. This condition is not generally fulfilled in non-structured high-Q resonators. Minimization of the walk-off parameter can be accomplished via the radial poling. Thus, there is one more physical reason to employ the radial poling for the comb generation.
Despite our massive failed efforts to find self-starting soliton-comb steady states for the FH pumping, we cannot claim that all sensible combinations of the input parameters (, , , , and ) are exhausted. Also, we cannot claim that the initial assumption of the same transverse structure of the modes within each of FH and SH frequency domains is fully justified. This problem remains still open for both and resonators. It is likely that suppression of the excitation of unwanted transverse modes takes further efforts on the mode management.
A distinctive feature of our approach is employment of a simple classical equivalent of the Hamiltonian quantum formalism of [4,5,17] and many other papers. While quantum electrodynamics aspects of microresonators are important [17], the comb regimes belong indeed to the classical range, where the use of quantum language is redundant. As we have shown, the actual numbers of modal light quanta in the comb spectra are very large.
The effects of nonlinearity in microresonators is one more issue to consider. The possibility to neglect the nonlinearity is largely controlled by the modal quality factors. Let us consider for simplicity the SH pumping case. The pump power thresholds and for the parametric instability caused by the and nonlinearities are roughly proportional to and , such that for the effects of Kerr nonlinearity are expected to be negligible. For modest quality factors, , which are typical for ring waveguides, the situation can be more complicated. An interplay between the and nonlinear processes becomes possible [42].
The above considered soliton-comb steady states in microresonators are not the first predicted ones and known in the literature. To the best of our knowledge, the other known stable dissipative soliton states [40,41,42,43] belong to the periodic ones. Furthermore, they are not self-starting, i.e., not easily accessible. The single-soliton numerical solutions of [40] are relevant to the FH pumping at the zero walk-off point. Both bright-bright and dark-dark locally stable solitons were exhibited. Similar numerical soliton solutions were found in [41,42]. Additionally, quasi-solitons at very large detunings, , were predicted. Remarkably, the soliton-comb states of [40] are allowed for the same and opposite signs of the dispersion coefficients. Generation of flat-top solitons via an additional spatial modulation of the pump amplitude was found numerically in the SH pumping case for opposite signs of the dispersion coefficients [43]. A considerable amount of soliton related models was proposed for bulk parametric oscillators. They are beyond the scope of this review.
The first experimental attempts to get combs in high-Q LN based microresonators dealt with the FH pumping, the natural phase matching, and large walk-off [37,38]. Neither solitons no broad comb spectra similar to the Kerr soliton spectra, were observed. This is in agreement with our theoretical results on the necessary conditions for realization of such regimes. The authors of [39] claim for discovery of Pockels soliton microcomb in an aluminium nitride microring resonator at SH pumping. The parameters used correspond to modest quality factors , a large walk-off parameter , and the threshold power only slightly below . The observed broad FH comb spectrum was practically symmetric, which is the fingerprint of the absence of dominating contribution of the nonlinearity. The observed and numerically modeled combs are due to the Kerr nonlinearity. The role of quadratic nonlinearity is mostly in initiation of the parametric instability. Thus, net soliton-comb states are still waiting for experimental discovery and exploration.
8. Conclusions
A vast family of stable soliton-comb steady states is accessible in microresonators via not very abrupt switching the monochromatic pump on. The necessary conditions for the soliton-comb generation are (i) pumping into a SH resonator mode and () opposite signs of the FH and SH dispersion coefficients. Also, minimization of the group velocity (free spectral range) difference for the phase matched FH and SH modes is highly desirable. For LN based resonators, the latter can be accomplished simultaneously with () using proper radial poling of the resonator. Pumping into FH resonator modes is not favorable for realization of the soliton-comb states. Employment of high-Q resonators () ensures low generation thresholds (within the W range) and negligibly small influence of the Kerr nonlinearity.
Supplementary Materials
The following supporting information can be downloaded at the website of this paper posted on Preprints.org, Figure S1: Geometry of the problem; Figure S2: Wavelength dependences; Figure S3: Wavelength dependences; Figure S4: Wavelength dependences; Figure S5: Schematic of a periodically poled microresonator; Figure S6: Rim poling period.
Author Contributions
investigation, B.S. and E.P.; data curation, S.S.; writing, B.S. All authors have read and agreed to the published version of the manuscript.
Funding
The work of S. S. was funded by the Russian Science Foundation (Grant No. 17-72-30006-P).
Institutional Review Board Statement
Not applicable.
Informed Consent Statement
Not applicable.
Data Availability Statement
Not applicable.
Conflicts of Interest
The authors declare no conflict of interest.
References
- Del’Haye, P.; Schliesser, A.; Arcizet, O.; Wilken, T.; Holzwarth, R; Kippenberg, T. J. Optical frequency comb generation from a monolithic microresonator. Nature 2007, 450, 1214–1217. [Google Scholar] [CrossRef]
- Del’Haye, P.; Herr, T.; Gavartin, E.; Gorodetsky, M.L.; Holzwarth, R; Kippenberg, T. J. Octave spanning tunable frequency comb from a microresonator. Phys. Rev. Lett. 2011, 107, 063901. [Google Scholar] [CrossRef]
- Kippenberg, T.J.; Holzwarth, R.; Diddams, S.A. Microresonator-based optical frequency combs. Science 2011, 332, 555–559. [Google Scholar] [CrossRef]
- Herr, T.; Brasch, V.; Jost, J.D.; Wang, C.Y.; Kondratiev, N.M.; Gorodetsky, M.L.; Kippenberg, T.J. Temporal solitons in optical microresonators. Nature Photonics 2014, 8, 145–152. [Google Scholar] [CrossRef]
- Kippenberg, T.J.; Gaeta, A.L.; Lipson, M.; Gorodetsky, M.L. Dissipative Kerr solitons in optical microresonators. Science 2018, 361, 567–572. [Google Scholar] [CrossRef]
- Suh, M.G.; Vahala, K. Gigahertz-repetition-rate soliton microcombs. Optica 2018, 5, 65–66. [Google Scholar] [CrossRef]
- Gaeta, A.L.; Lipson, M.; Kippenberg, T.J. Photonic-chip-based frequency combs. Nature Photonics 2019, 13, 158–169. [Google Scholar] [CrossRef]
- Chang, L.; Xie, W.; et al. Shu, H.; Yang, Q; Shen, B.; Boes, A.; Peters, J.D; Jin, W.; Xiang, C.; Liu, S.; Moille, G.; Yu, S.; Wang, X.; Srinivasan, K.; Papp, S.B.; Vahala K.; Bowers, J.E.. Ultra-efficient frequency comb generation in AlGaAs-on-insulator microresonators. Nature Communications 2020, 11, 1331. [CrossRef] [PubMed]
- Fujii, S.; Tanaka, S.; Ohtsuka, T.; Kogure, S.; Wada, K.; Kumazaki, H.; Tasaka, S.; Hashimoto, Y.; Kobayashi, Y.; Araki, T.; Furusawa, K.; Sekine, N.; Kawanishi, S.; Tanabe, T. Dissipative Kerr soliton microcombs for FEC-free optical communications over 100 channels. Opt. Express 2022, 30, 1351–1364. [Google Scholar] [CrossRef] [PubMed]
- Fujii, S.; Wada, K.; Sugano, R.; Kumazaki, H.; Kogure, S.; Kato, Y.K.; Tanabe, T. Versatile tuning of Kerr soliton microcombs in crystalline microresonators. Communications Physics 2023, 6, 1–7. [Google Scholar] [CrossRef]
- Akhmediev, N.; Ankiewicz, A. Dissipative Solitons, Lecture Notes in Physics; Springer-Verlag: Berlin, Germany, 2005. [Google Scholar]
- Grelu, P.; Akhmediev, N. Dissipative solitons for mode-locked lasers. Nature Photonics 2012, 6, 84–92. [Google Scholar] [CrossRef]
- Boyd, R.W. Nonlinear Optics; Academic Press: New York, USA, 2008. [Google Scholar]
- Fürst, J.U.; Strekalov, D.V.; Elser, D.; Lassen, M.; Andersen, U.L.; Marquardt, C.; Leuchs, G. Naturally phase-matched second-harmonic generation in a whispering-gallery-mode resonator. Phys. Rev. Lett. 2010, 104, 153901. [Google Scholar] [CrossRef]
- Beckmann, T.; Linnenbank, H.; Steigerwald, H.; Sturman, B.; Haertle, D.; Buse, K.; Breunig, I. Highly tunable low-threshold optical parametric oscillation in radially poled whispering gallery resonators. Phys. Rev. Lett. 2011, 106, 143903. [Google Scholar] [CrossRef]
- Mohageg, M.; Strekalov, D.V.; Savchenkov, A.A.; Matsko, A.B.; Ilchenko, V.S.; Maleki, L. Calligraphic poling of lithium niobate. Opt. Express 2005, 13, 3408–3419. [Google Scholar] [CrossRef]
- Strekalov, D.; Marquardt, Ch.; Matsko, A.B.; Schwefel, H.G.L.; Leuchs, G. Nonlinear and quantum optics with whispering gallery resonators. J. Opt. 2016, 18, 123002. [Google Scholar] [CrossRef]
- Breunig, I. Three-wave mixing in whispering gallery resonators. Laser and Photonics Reviews 2016, 10, 569–587. [Google Scholar] [CrossRef]
- Buryak, A.V.; Trapani, P. Di; Skryabin, D.V.; Trillod, S. Optical solitons due to quadratic nonlinearities: from basic physics to futuristic applications. Phys. Rep. 2002, 370, 63–235. [Google Scholar] [CrossRef]
- Ilchenko, V.S.; Savchenkov, A.A.; Matsko, A.B.; Maleki, L. Nonlinear optics and crystalline whispering gallery mode cavities. Phys. Rev. Lett. 2004, 92, 043903–043907. [Google Scholar] [CrossRef] [PubMed]
- Jia, Y.; Hanka, K.; Zawilski, K.T.; Schunemann, P.G.; Buse, K.; Breunig, I. Continuous-wave whispering-gallery optical parametric oscillator based on CdSiP2. Opt. Express 2018, 26, 10833–10841. [Google Scholar] [CrossRef] [PubMed]
- Guo, X.; Zou, C.-L.; Tang, H.X. Second-harmonic generation in aluminum nitride microrings with 2500%/W conversion efficiency. Optica 2016, 3, 1126–1131. [Google Scholar] [CrossRef]
- Kuo, P.S.; Bravo-Abad, J.; Solomon, G.S. Second-harmonic generation using 4¯-quasi-phasematching in a GaAs whispering-gallery-mode microcavity. Nature Communications 2013, 5, 3109. [Google Scholar] [CrossRef] [PubMed]
- Gorodetsky, M.L. High-Q Optical Microresonators; Fizmatgiz: Moscow, Russia, in Russian, 2011. [Google Scholar]
- Matsko, A.B.; Ilchenko, V.S. Optical resonators with whispering-gallery modes – Part I: Basics. IEEE J. of Selected Topics in Quantum Electronics 2006, 12, 3–14. [Google Scholar] [CrossRef]
- Maleki, L.; Ilchenko, V.S.; Savchenkov, A.A.; Matsko, A.B. Crystalline whispering gallery mode resonators in optics and photonics. In Practical Applications of Microresonators in Optics and Photonics; Matsko, A.B., Ed.; CRC Press: Boca Raton, USA, 2019. [Google Scholar]
- Gorodetsky, M.L.; Demchenko, Yu.A. Accurate analytical estimates of eigenfrequencies and dispersion in whispering-gallery spheroidal resonators. Proceedings of SPIE 2012, 8236, 823623. [Google Scholar]
- Demchenko, Yu.A.; Gorodetsky, M.L. Analytical estimates of eigenfrequencies, dispersion, and field distribution in whispering gallery resonators. J. Opt. Soc. Am. B 2013, 30, 3056–3063. [Google Scholar] [CrossRef]
- Breunig, I.; Sturman, B.; Sedlmeir, F.; Schwefel, H.G.L.; Buse, K. Whispering gallery modes at the rim of an axisymmetric optical resonator: Analytical versus numerical description and comparison with experiment. Opt. Express 2013, 21, 30683–30692. [Google Scholar] [CrossRef] [PubMed]
- Sturman, B.; Podivilov, E.; Werner, C.S.; Breunig, I. Vectorial perturbation theory for axisymmetric whispering gallery resonators. Phys. Rev. A 2019, 99, 013810. [Google Scholar] [CrossRef]
- Sturman, B.; Breunig, I. Generic description of second-order nonlinear phenomena in whispering-gallery resonators. J. Opt. Soc. Am. B 2011, 28, 2465–2471. [Google Scholar] [CrossRef]
- Werner, Ch.S.; Yoshiki, W.; Herr, S.J.; Breunig, I.; Buse, K. Geometric tuning: spectroscopy using whispering-gallery resonator frequency-synthesizers. Optica 2017, 4, 1205–1208. [Google Scholar] [CrossRef]
- Ricciardi, I.; Mosca, S.; Parisi, M.; Maddaloni, P.; Santamaria, L.; De Natale, P.; De Rosa, M. Frequency comb generation in quadratic nonlinear media. Phys. Rev. A 2015, 91, 063839. [Google Scholar] [CrossRef]
- Leo, F.; Hansson, T.; Ricciardi, I.; De Rosa, M.; Coen, S.; Wabnitz, S.; Erkintalo, M. Walk-off-induced modulation instability, temporal pattern formation, and frequency comb generation in cavity-enhanced second-harmonic generation. Phys. Rev. Lett. 2016, 116, 033901. [Google Scholar] [CrossRef]
- Mosca, S.; Ricciardi, I.; Parisi, M.; Maddaloni, P.; Santamaria, L.; De Natale, P.; De Rosa, M. Direct generation of optical frequency combs in χ(2) nonlinear cavities. Nanophotonics 2016, 5, 316–331. [Google Scholar] [CrossRef]
- Mosca, S.; Parisi, M.; Ricciardi, I.; Leo, F.; Hansson, T.; Erkintalo, M.; Maddaloni, P.; De Natale, P.; Wabnitz, S.; De Rosa, M. Modulation instability induced frequency comb generation in a continuously pumped optical parametric oscillator. Phys. Rev. Lett. 2018, 121, 093903. [Google Scholar] [CrossRef] [PubMed]
- Szabados, J.; Puzyrev, D.N.; Minet, Y.; Reis, L.; Buse, K.; Villois, A.; Skryabin, D.V.; Breunig, I. Frequency comb generation via cascaded second-order nonlinearities in microresonators. Phys. Rev. Lett. 2020, 124, 203902. [Google Scholar] [CrossRef] [PubMed]
- Szabados, J.; Sturman, B.; Breunig, I. Frequency comb generation threshold via second-harmonic excitation in χ(2) optical microresonators. APL Photonics 2020, 5, 116102. [Google Scholar] [CrossRef]
- Bruch, A.W.; Liu, X.; Gong, Z.; Surya, J.B.; Li, M.; Zou, C.-L.; Tang, H.X. Pockels soliton microcomb. Nature Photonics 2021, 15, 21–27. [Google Scholar] [CrossRef]
- Hannson, T.; Parra-Rivas, P.; Bernard, M.; Leo, F.; Gelens, L.; Wabnitz, S. Quadratic soliton combs in doubly resonant second-harmonic generation. Opt. Lett. 2018, 43, 6033–6036. [Google Scholar] [CrossRef]
- Villois, A.; Skryabin, D. Soliton and quasi-soliton frequency combs due to second harmonic generation in microresonators. Opt. Express 2019, 27, 7098–7107. [Google Scholar] [CrossRef]
- Villois, A.; Kondratiev, N.; Breunig, I.; Puzyrev, D.N.; Skryabin, D.V. Frequency combs in a microring optical parametric oscillator. Opt. Lett. 2019, 44, 4443–4446. [Google Scholar] [CrossRef]
- Lobanov, V.E. Two-color flat-top solitons in microresonator-based optical parametric oscillators. Phys. Rev. A 2020, 102, 013518. [Google Scholar] [CrossRef]
- Landau, L.D.; Lifshitz, E.M. Mechanics, 3rd ed.; Elsevier Ltd.: Amsterdam, Netherlands, 1976. [Google Scholar]
- Zakharov, V.E.; Kuznetsov, E.A. Hamiltonian formalism for nonlinear waves. Physics - Uspekhi 1997, 40, 1087–1116. [Google Scholar] [CrossRef]
- Podivilov, E.; Smirnov, S.; Breunig, I.; Sturman, B. Nonlinear solutions for χ(2) frequency combs in optical microresonators. Phys. Rev. A 2020, 101, 023815. [Google Scholar] [CrossRef]
- Smirnov, S.; Sturman, B.; Podivilov, E.; Breunig, I. Walk-off controlled self-starting frequency combs in χ(2) optical microresonators. Opt. Express 2020, 28, 18006–18017. [Google Scholar] [CrossRef]
- The use of this expansion is justified, for the FH and SH spectra must be relatively narrow (|j|,|l|≪∼104) within our envelope approximation.
- Nikogosyan, D.N. Nonlinear Optical Crystals: A Complete Survey; Springer: New York, USA, 2005. [Google Scholar]
- Sturman, B.; Podivilov, E.; Szabados, J.; Breunig, I. Dual backgrounds and their stability during frequency comb and second harmonic generation in χ(2) microresonators. J. Opt. Soc. Am. B 2022, 39, 378–387. [Google Scholar] [CrossRef]
- Podivilov, E.; Sturman, B.; Breunig, I. Frequency comb solutions for driven χ(2) optical microresonators. J. Opt. Soc. Am. B 2020, 37, 3316–3324. [Google Scholar] [CrossRef]
- Smirnov, S.; Andryushkov, V.; Podivilov, E.; Sturman, B.; Breunig, I. Walk-off controlled self-starting frequency combs in χ(2) optical microresonators. Opt. Express 2021, 29, 27434–27449. [Google Scholar] [CrossRef] [PubMed]
- Podivilov, E.; Smirnov, S.; Sturman, B. Adiabatic growing, multistability, and control of soliton-comb states in χ(2) microresonators for pumping into second-harmonic modes. JETP Letters 2022, 115, 553–559. [Google Scholar] [CrossRef]
- Breunig, I. , Freiburg University, Germany, Personal communication, 2020.
- Umemura, N.; Matsuda, D. Thermo-optic dispersion formula for the ordinary wave in 5 mol % MgO doped LiNbO3 and its application to temperature insensitive second-harmonic generation. Opt. Commun. 2016, 367, 167–173. [Google Scholar] [CrossRef]
- Smirnov, S.; Podivilov, E.; Sturman, B. Effects of dispersion and pumping scheme on soliton-comb generation in χ(2) microresonators. J. Opt. Soc. Am. B 2023, 40, 515–522. [Google Scholar] [CrossRef]
- Leidinger, M.; Fieberg, S.; Waasem, N.; Kühnemann, F.; Buse, K.; Breunig, I. Comparative study on three highly sensitive absorption measurement techniques characterizing lithium niobate over its entire transparent spectral range. Opt. Express 2015, 23, 21690–21705. [Google Scholar] [CrossRef] [PubMed]
Figure 1.
Schematic of comb generation; R and are the major resonator radius and the azimuth angle. The output comb spectra are due to a dual FH-SH soliton propagating along the resonator rim with velocity . The red spots indicate localization of the resonator modes near the rim.
Figure 1.
Schematic of comb generation; R and are the major resonator radius and the azimuth angle. The output comb spectra are due to a dual FH-SH soliton propagating along the resonator rim with velocity . The red spots indicate localization of the resonator modes near the rim.
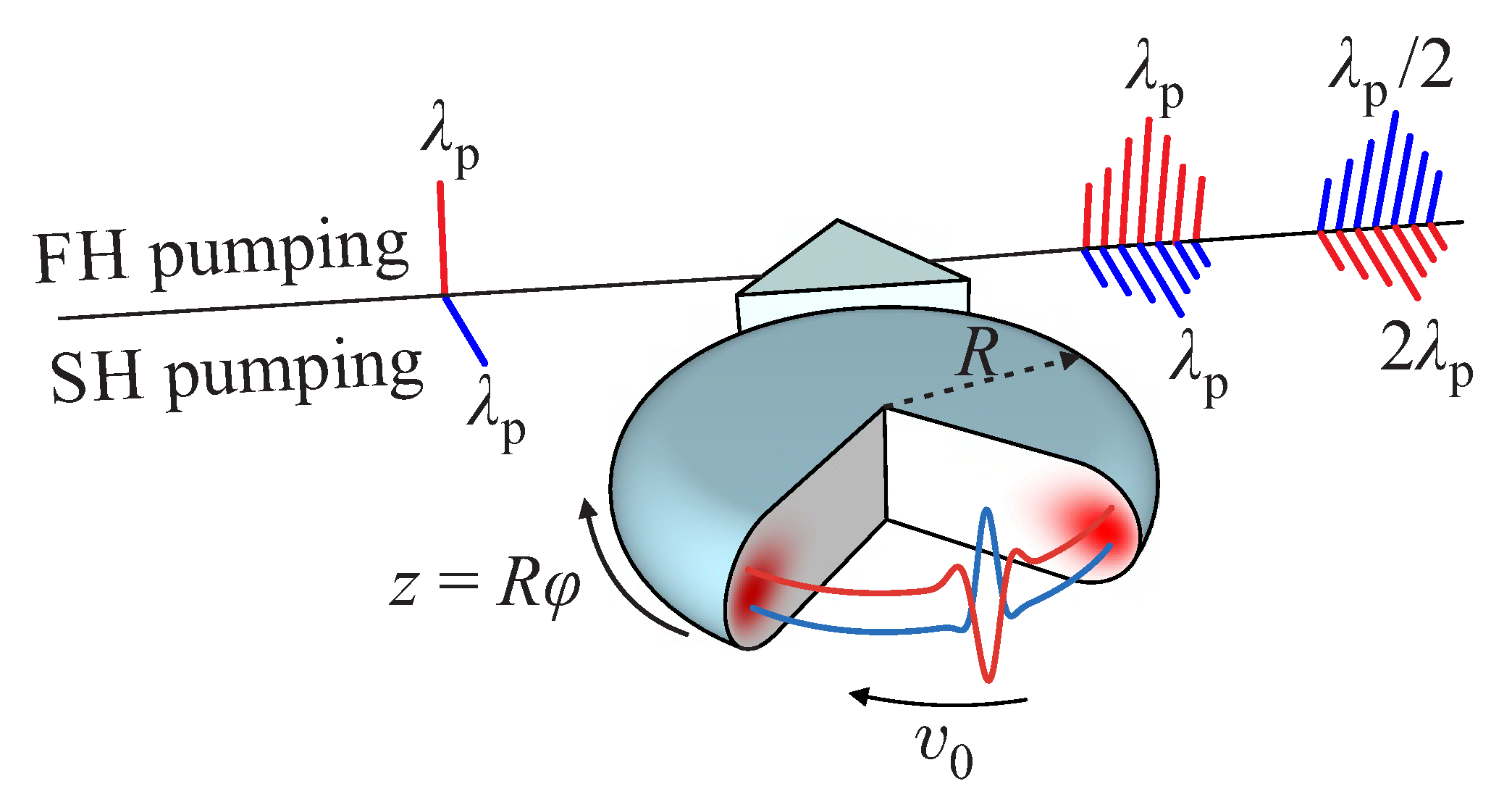
Figure 2.
Excitation of periodic (a) and antiperiodic (b) states for SH-pumping into even and odd resonator modes. The primary FH azimuth number is integer in (a) and semi-integer in (b). Side harmonics arise automatically in (b) above the threshold.
Figure 2.
Excitation of periodic (a) and antiperiodic (b) states for SH-pumping into even and odd resonator modes. The primary FH azimuth number is integer in (a) and semi-integer in (b). Side harmonics arise automatically in (b) above the threshold.

Figure 3.
(a) Illustration of the azimuth dependence of the susceptibility coefficient for a perfect symmetric radial domain pattern with . (b) First three harmonics of the corresponding Fourier spectrum with , , and .
Figure 3.
(a) Illustration of the azimuth dependence of the susceptibility coefficient for a perfect symmetric radial domain pattern with . (b) First three harmonics of the corresponding Fourier spectrum with , , and .
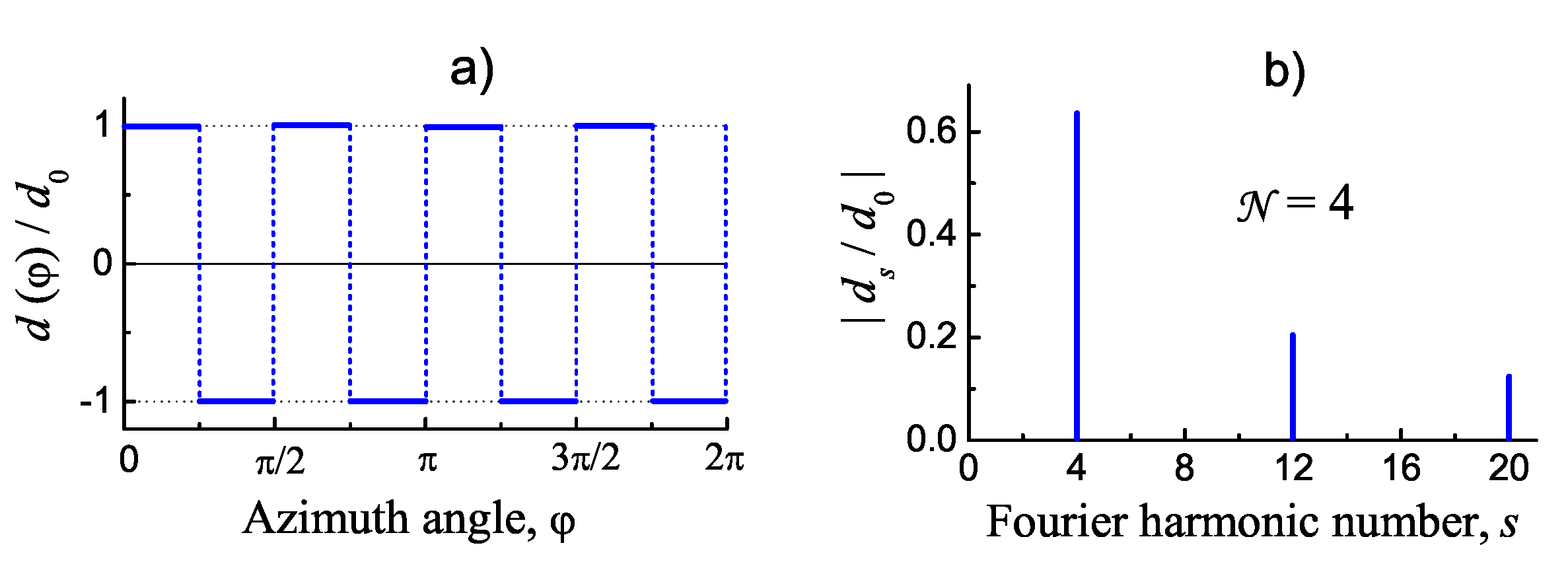
Figure 4.
Dependences (a) and (b) for an LN based resonator with mm and . The dotted and solid lines refer to the bulk index and the effective index n incorporating the effects of geometric dispersion. The vertical line m (m) corresponds to zero walk-off point .
Figure 4.
Dependences (a) and (b) for an LN based resonator with mm and . The dotted and solid lines refer to the bulk index and the effective index n incorporating the effects of geometric dispersion. The vertical line m (m) corresponds to zero walk-off point .
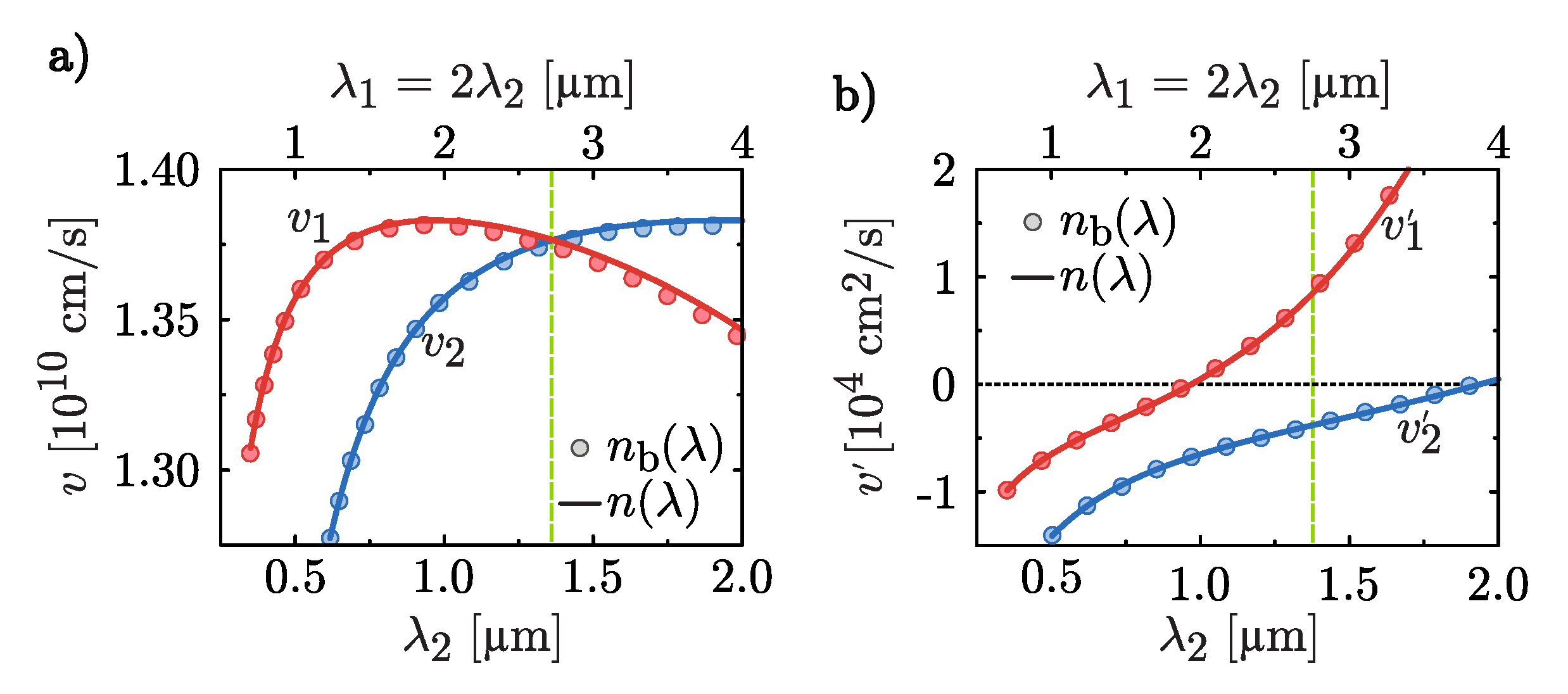
Figure 5.
Normalized dependences of on the pump strength parameters for the cases of FH (a) and SH (b) pumping. The curves are relevant to different values of the phase . Zero detuning case, , corresponds to . The horizontal red line in (b) is related to the single background state, , .
Figure 5.
Normalized dependences of on the pump strength parameters for the cases of FH (a) and SH (b) pumping. The curves are relevant to different values of the phase . Zero detuning case, , corresponds to . The horizontal red line in (b) is related to the single background state, , .
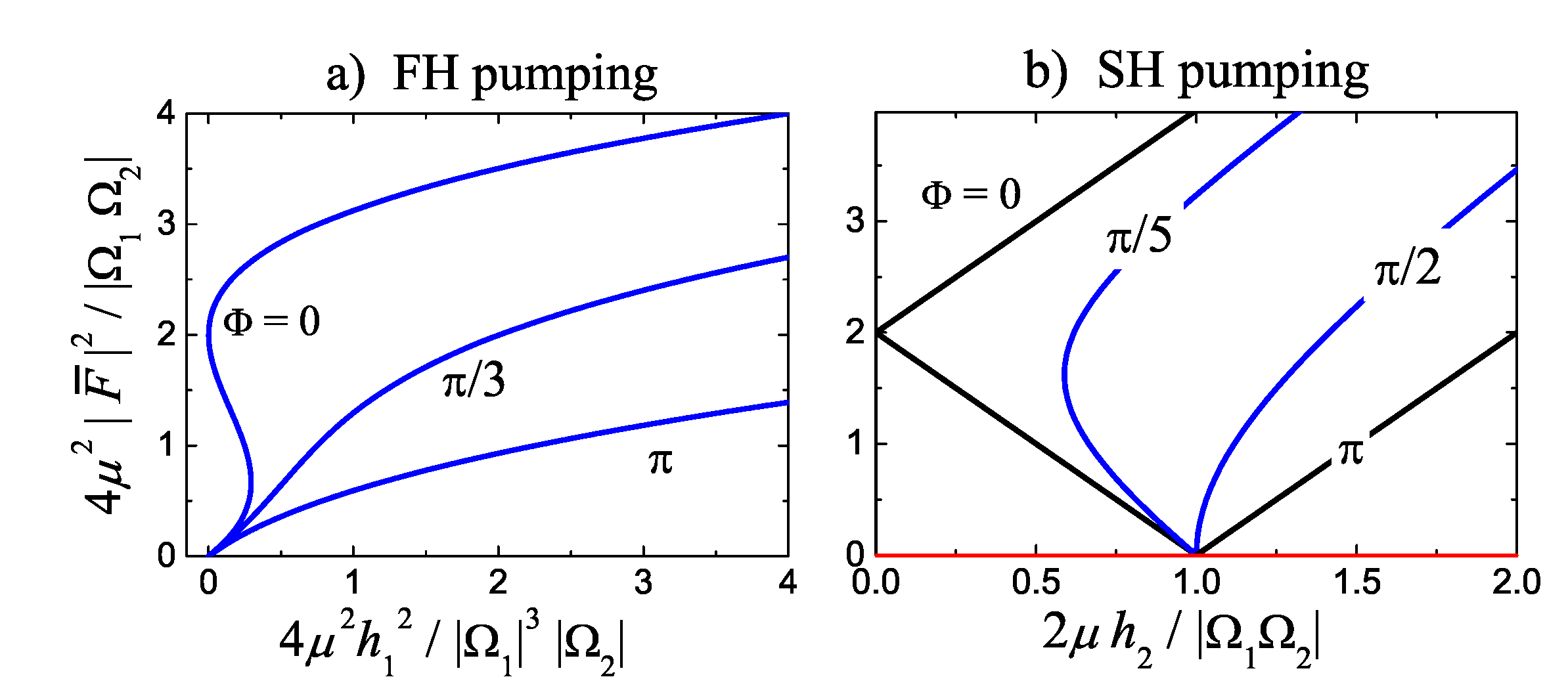
Figure 6.
Threshold dependences for FH pumping at . (a) nm, , and of . (b) nm, , of . Two horizontal lines and 2 indicate the internal instability threshold and the limiting value .
Figure 6.
Threshold dependences for FH pumping at . (a) nm, , and of . (b) nm, , of . Two horizontal lines and 2 indicate the internal instability threshold and the limiting value .
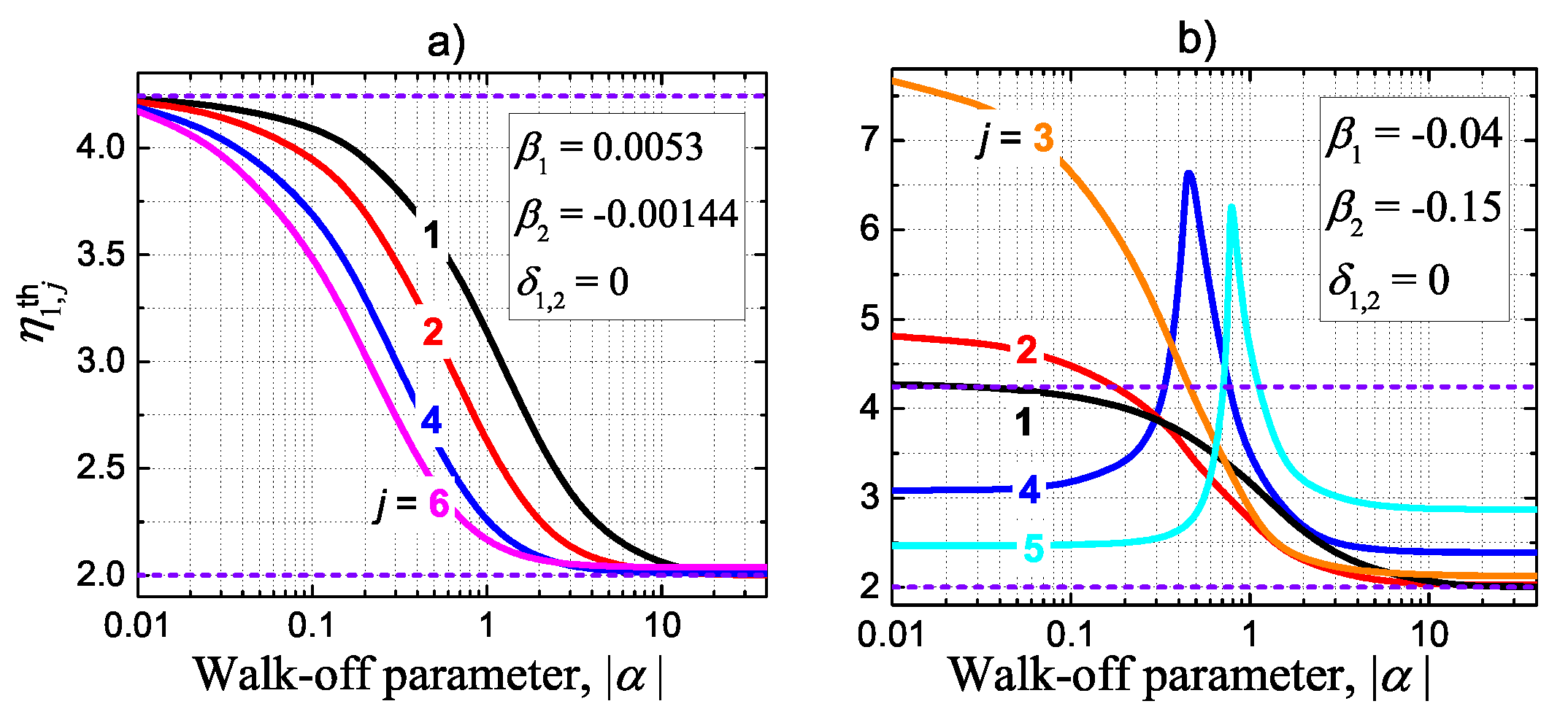
Figure 7.
Three representative evolution runs for the discrepancy parameter relevant to the FH harmonics and . The states under study are antiperiodic. The input dimensionless parameters are , , , s−1, and . The time step is . The evolution run relevant to (a) starts at from noise and ends up at . The evolution runs (b,c) start from the previous steady-state values of the FH amplitudes.
Figure 7.
Three representative evolution runs for the discrepancy parameter relevant to the FH harmonics and . The states under study are antiperiodic. The input dimensionless parameters are , , , s−1, and . The time step is . The evolution run relevant to (a) starts at from noise and ends up at . The evolution runs (b,c) start from the previous steady-state values of the FH amplitudes.
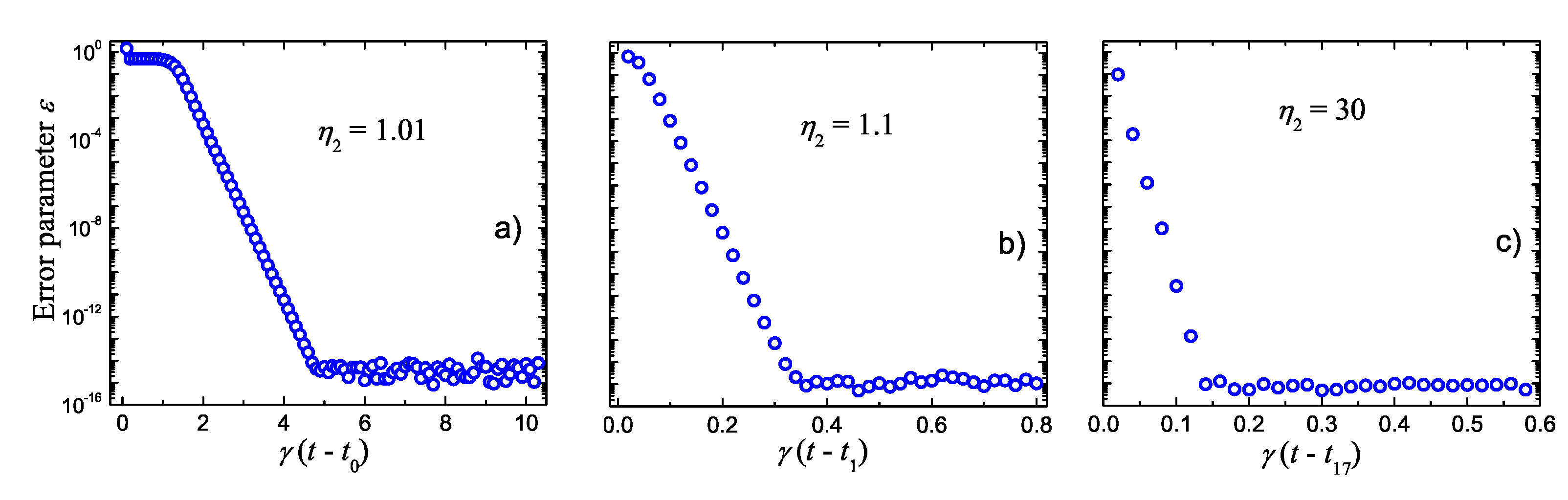
Figure 8.
Properties of the antiperiodic steady state grown adiabatically for , mm, , and ; 1024 FH and SH harmonics are taken into account. The upper and lower rows refer to the FH and SH characteristics, respectively. They show the normalized intensity profiles (a and d), the phase profiles (b and e), and the comb spectra (c and f). The horizontal dashed lines indicate the cut-off level of . The number of FH and SH comb lines above is 122 and 162.
Figure 8.
Properties of the antiperiodic steady state grown adiabatically for , mm, , and ; 1024 FH and SH harmonics are taken into account. The upper and lower rows refer to the FH and SH characteristics, respectively. They show the normalized intensity profiles (a and d), the phase profiles (b and e), and the comb spectra (c and f). The horizontal dashed lines indicate the cut-off level of . The number of FH and SH comb lines above is 122 and 162.
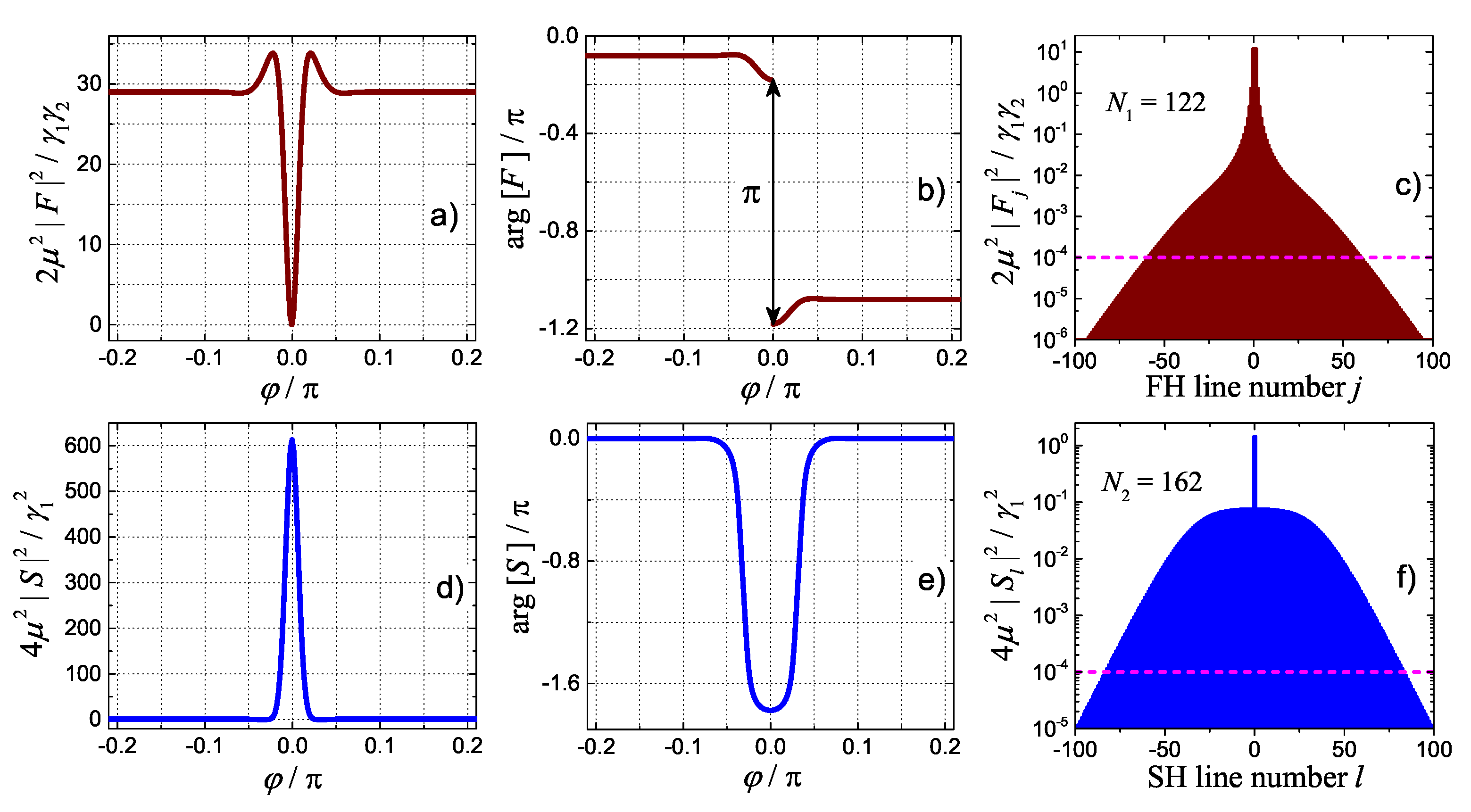
Figure 9.
The effect of walk-off on the soliton-comb properties. The only difference in the input parameters as compared to Figure 8 is nm corresponding to the walk-off parameter .
Figure 9.
The effect of walk-off on the soliton-comb properties. The only difference in the input parameters as compared to Figure 8 is nm corresponding to the walk-off parameter .
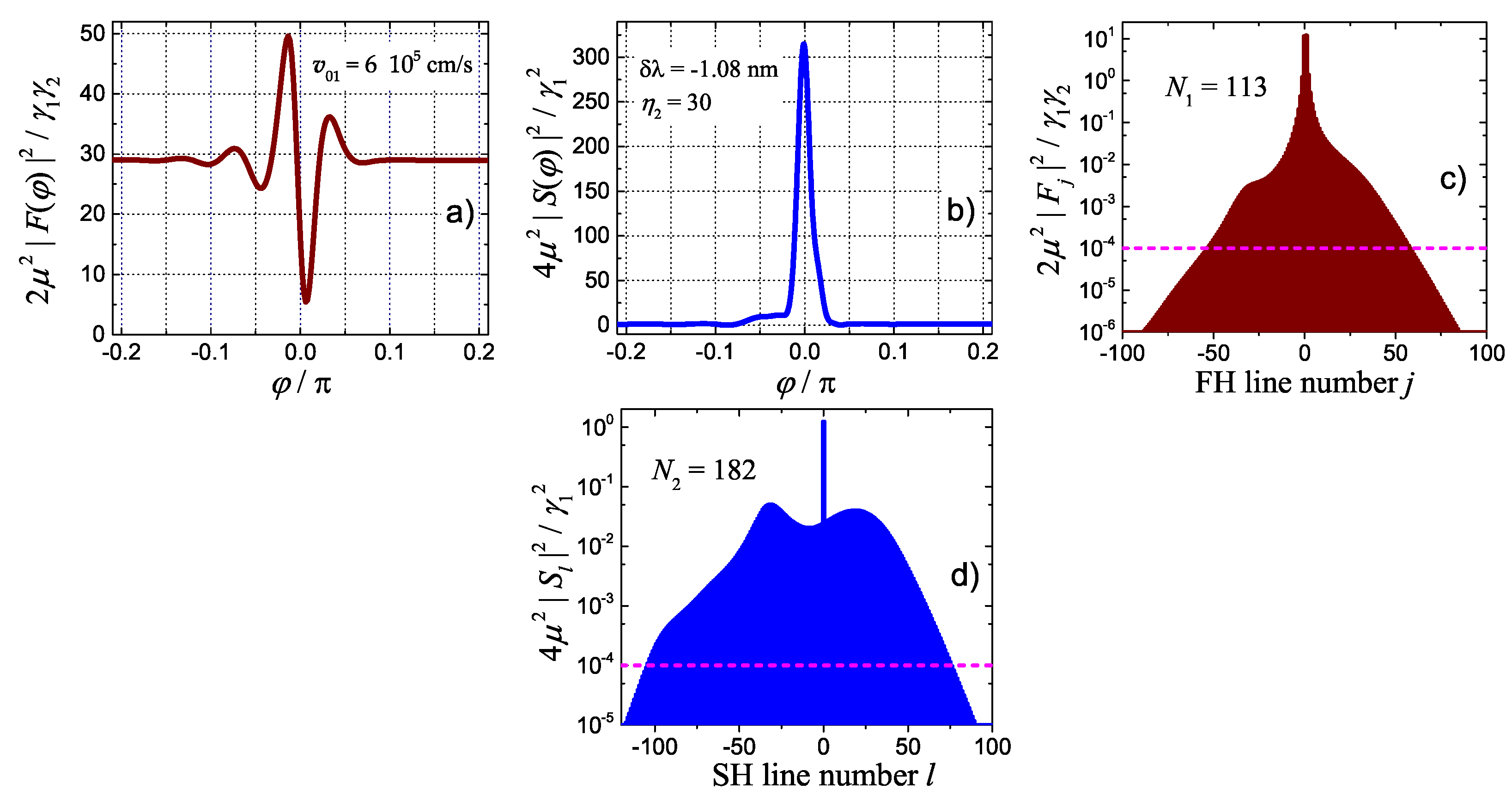
Figure 10.
Subfigures a1 to c1: Color maps of velocity parameter [MHz] on the plane. Subfigures a2 to c2: The same for the total comb line number . The first, second, and third columns refer to , ; , and with s−1. Each map is obtained by stepwise increase of starting from the value of 2 and incorporates the data of calculation variants with up to 1024 Fourier harmonics taken into account. The insets in a1 to c1 indicate the signs of . Subfigures d1 and d2 give the crosssections and for relevant to the maps a1 to c1 and a2 to c2, respectively. Note different scales of in d1 and d2.
Figure 10.
Subfigures a1 to c1: Color maps of velocity parameter [MHz] on the plane. Subfigures a2 to c2: The same for the total comb line number . The first, second, and third columns refer to , ; , and with s−1. Each map is obtained by stepwise increase of starting from the value of 2 and incorporates the data of calculation variants with up to 1024 Fourier harmonics taken into account. The insets in a1 to c1 indicate the signs of . Subfigures d1 and d2 give the crosssections and for relevant to the maps a1 to c1 and a2 to c2, respectively. Note different scales of in d1 and d2.
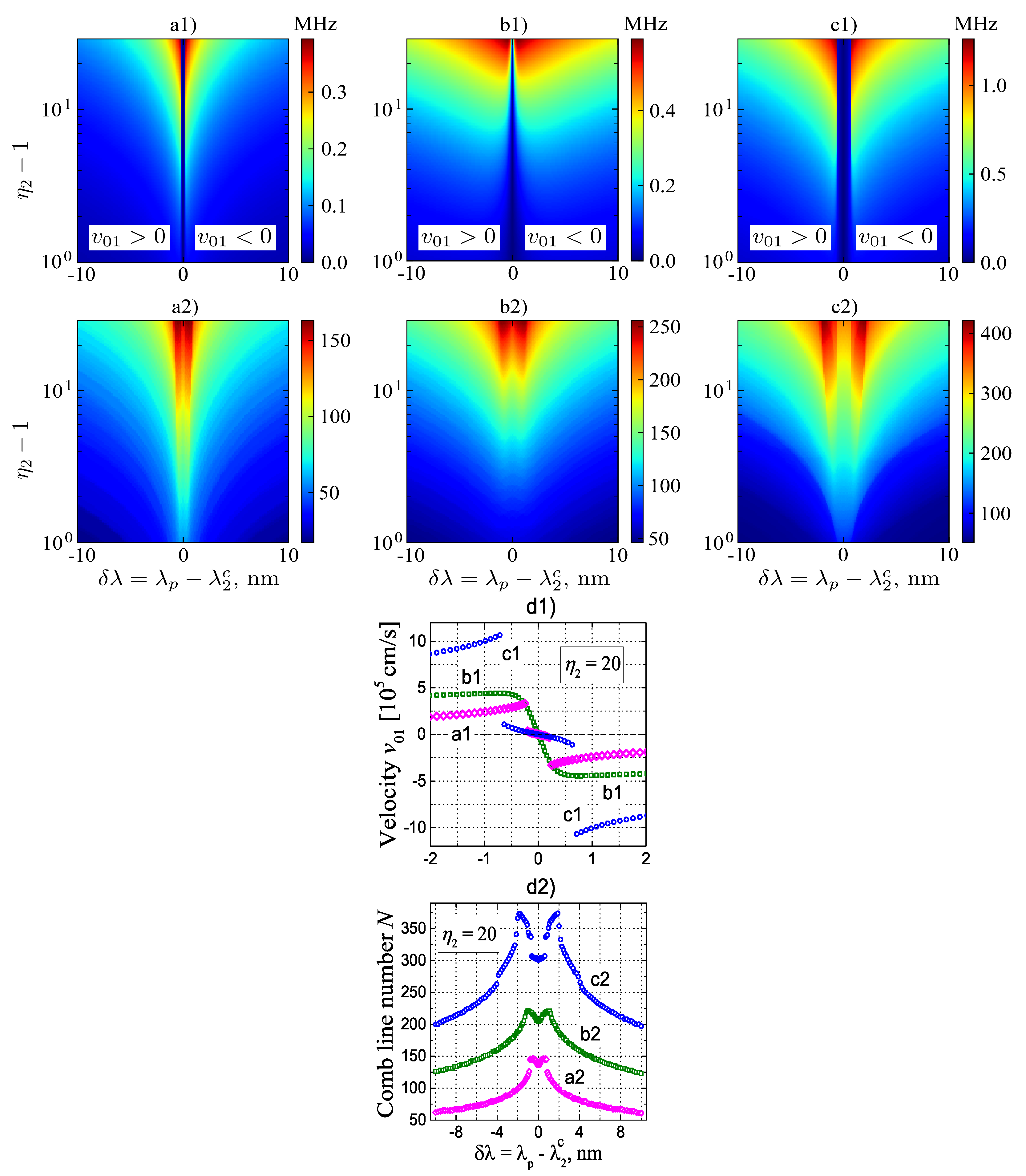
Figure 11.
Dependences of (a) and N (b) on and for , , and s−1. Each map incorporates the data of calculation variants. Within the dark-blue colored parts in a), .
Figure 11.
Dependences of (a) and N (b) on and for , , and s−1. Each map incorporates the data of calculation variants. Within the dark-blue colored parts in a), .
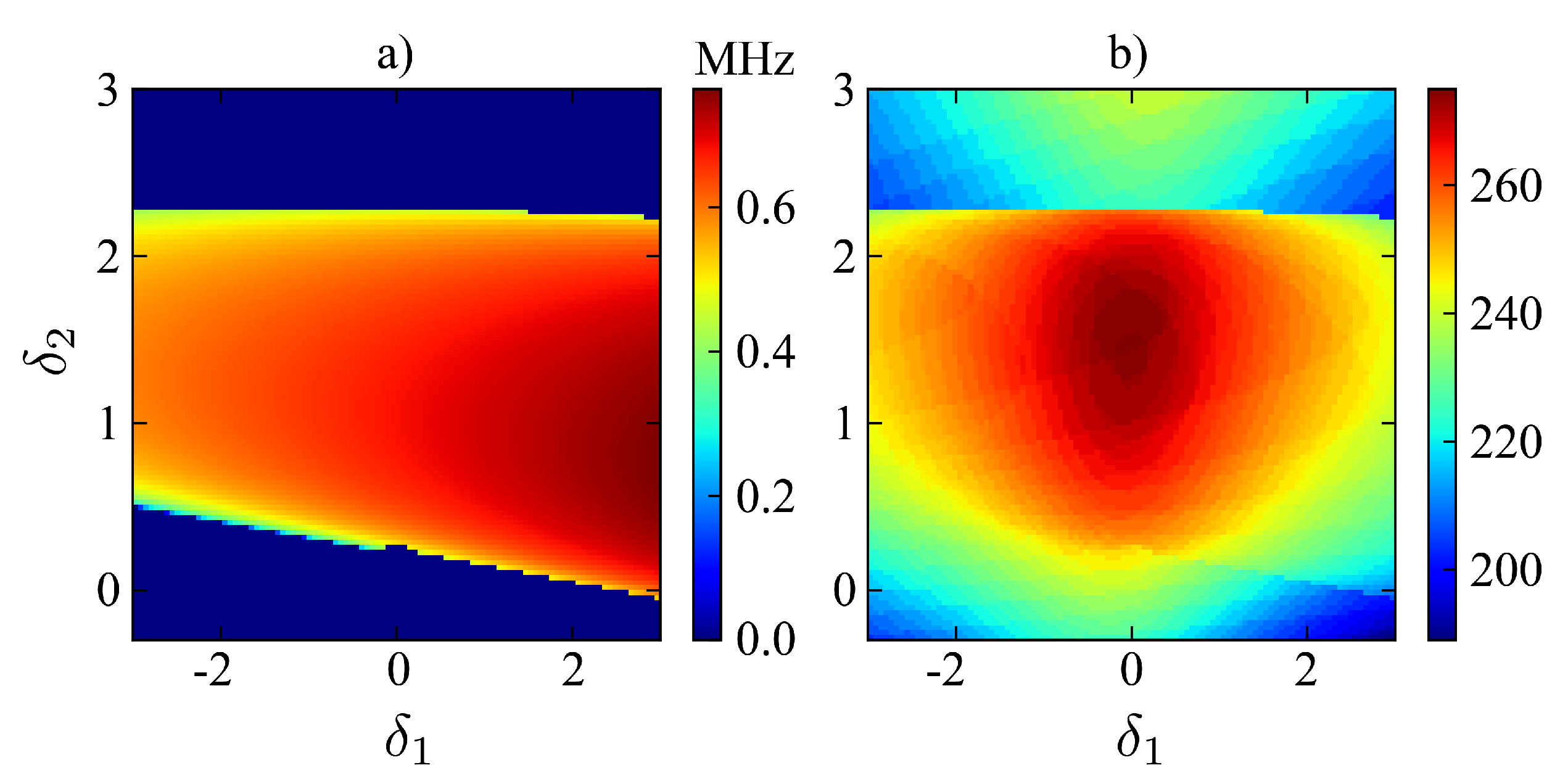
Figure 12.
Diagrams of growing of antiperiodic (a) and periodic (b) multi-soliton states by (i) adiabatic increase of and (ii) the subsequent slow change of . The cyan circles indicate the borders of the allowed ranges for initial values of . The lowest thresholds occur at . For , stable states grown via the adiabatic two-step (i-ii) procedure exist in a broad range of exciding the shown one.
Figure 12.
Diagrams of growing of antiperiodic (a) and periodic (b) multi-soliton states by (i) adiabatic increase of and (ii) the subsequent slow change of . The cyan circles indicate the borders of the allowed ranges for initial values of . The lowest thresholds occur at . For , stable states grown via the adiabatic two-step (i-ii) procedure exist in a broad range of exciding the shown one.
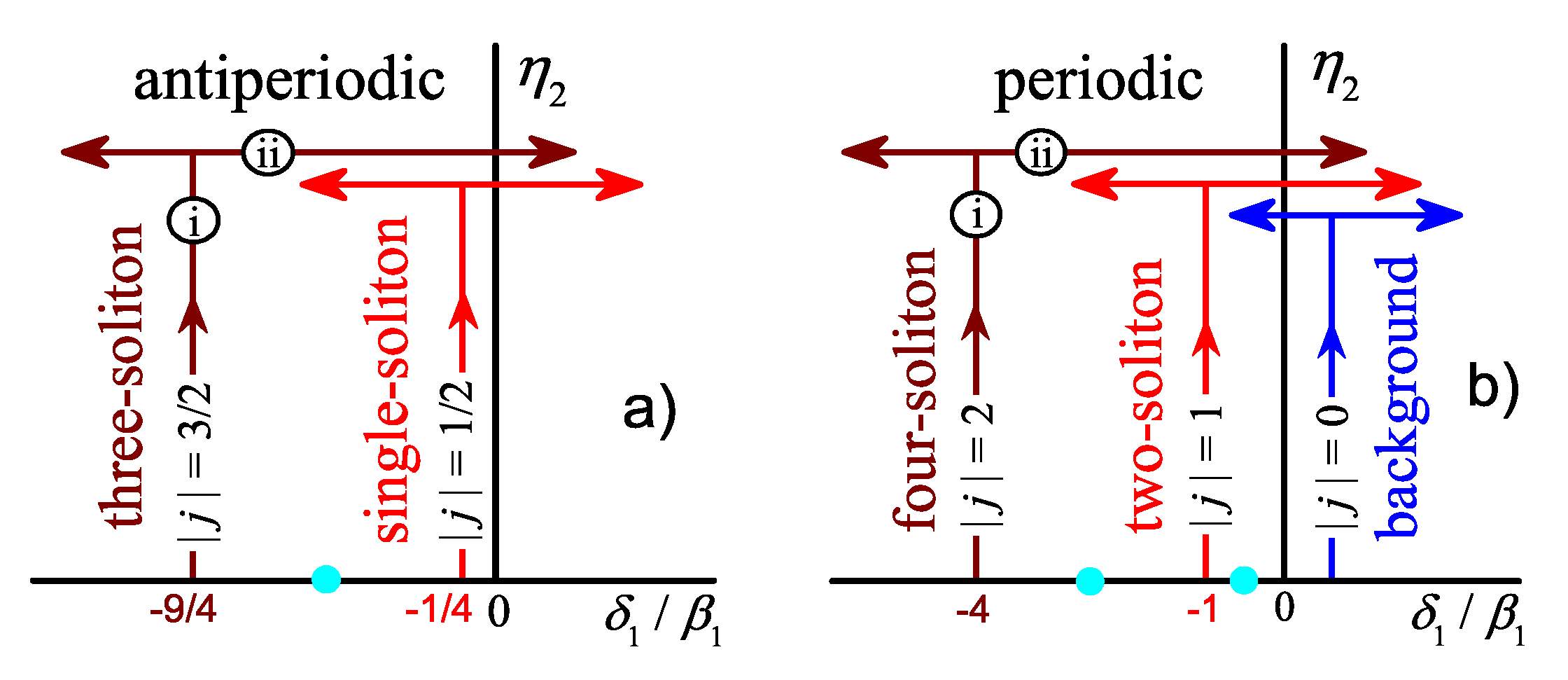
Figure 13.
Periodic adiabatically grown two-soliton state for , , , , and s−1. The upper and bottom rows refer to the FH and SH characteristics, respectively. They show the normalized intensity profiles (a and d), the phase profiles (b and e), and the comb spectra (c and f).
Figure 13.
Periodic adiabatically grown two-soliton state for , , , , and s−1. The upper and bottom rows refer to the FH and SH characteristics, respectively. They show the normalized intensity profiles (a and d), the phase profiles (b and e), and the comb spectra (c and f).
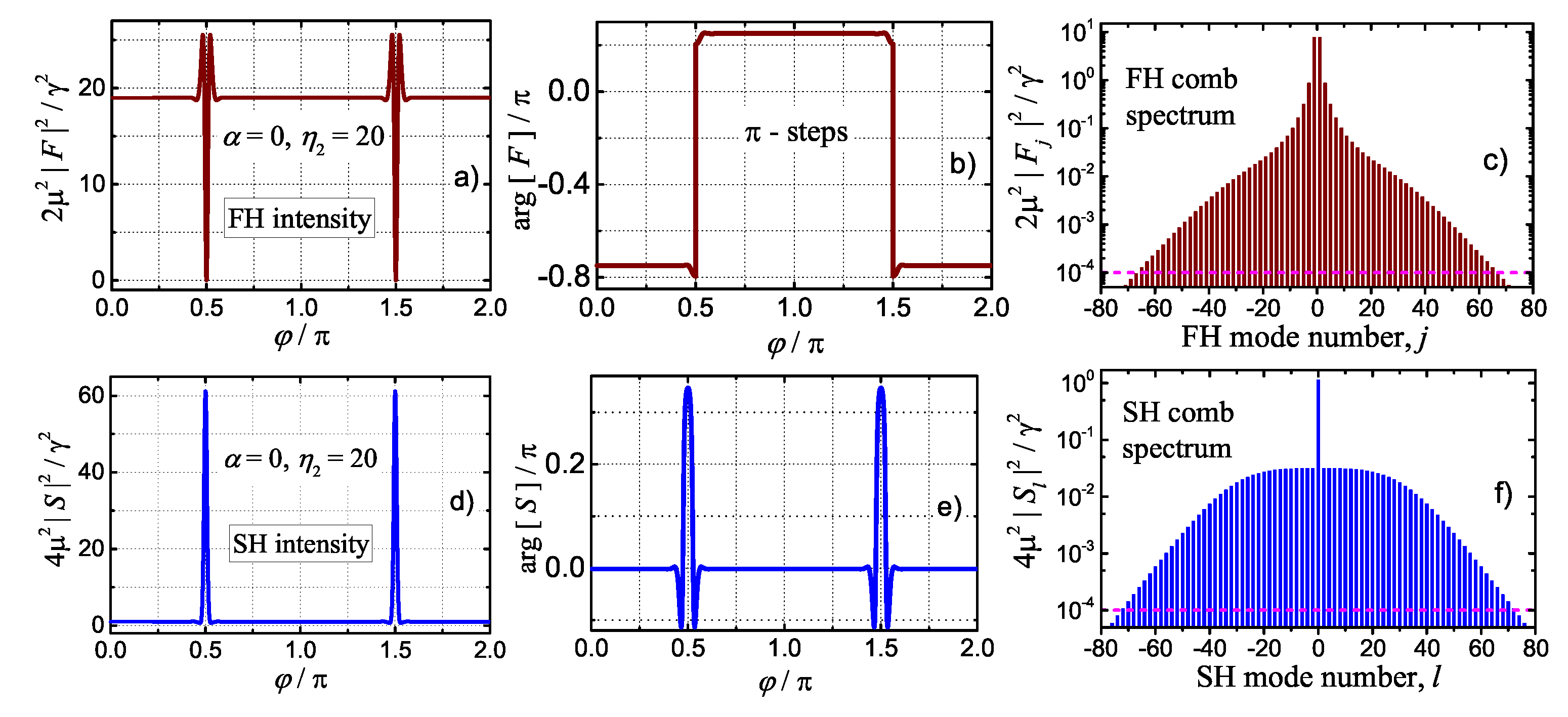
Figure 14.
Antiperiodic adiabatically grown three-soliton state for ( nm) and . The other parameters correspond to Figure 13. Subfigures (a and b) correspond to the normalized FH and SH intensity profiles.
Figure 14.
Antiperiodic adiabatically grown three-soliton state for ( nm) and . The other parameters correspond to Figure 13. Subfigures (a and b) correspond to the normalized FH and SH intensity profiles.
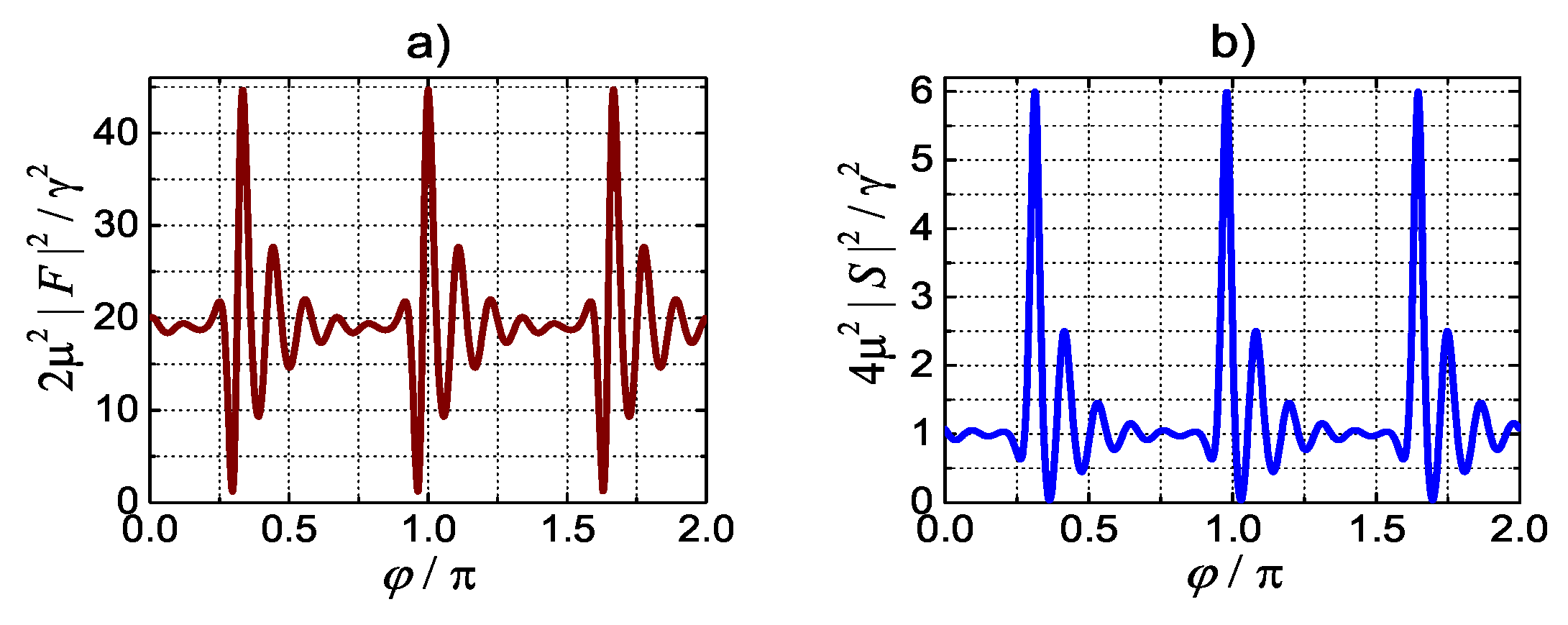
Figure 15.
FH pumping at and . a): A fragment of steady-state auto-oscillations at ; the spatial harmonics with are negligible here. b): FH and SH intensity profiles (lines 1 and 2) at . c) and d): FH and SH intensity profiles at .
Figure 15.
FH pumping at and . a): A fragment of steady-state auto-oscillations at ; the spatial harmonics with are negligible here. b): FH and SH intensity profiles (lines 1 and 2) at . c) and d): FH and SH intensity profiles at .

Figure 16.
FH pumping at 2698 nm. a) and b): Periodic FH and SH intensity profiles for , , , and . c) and d): The corresponding FH and SH comb spectra. The number of significant comb lines (higher than ) is , and the comb line distance .
Figure 16.
FH pumping at 2698 nm. a) and b): Periodic FH and SH intensity profiles for , , , and . c) and d): The corresponding FH and SH comb spectra. The number of significant comb lines (higher than ) is , and the comb line distance .

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Copyright: This open access article is published under a Creative Commons CC BY 4.0 license, which permit the free download, distribution, and reuse, provided that the author and preprint are cited in any reuse.
MDPI Initiatives
Important Links
© 2024 MDPI (Basel, Switzerland) unless otherwise stated




