Preprint
Article
Developing the Teacher Self-efficacy Scale in the Field of Mathematics Learning Disability
Altmetrics
Downloads
165
Views
59
Comments
0
This version is not peer-reviewed
Submitted:
30 May 2023
Posted:
31 May 2023
You are already at the latest version
Alerts
Abstract
In the study, it was aimed to develop a valid and reliable scale in order to determine the self-efficacy perceptions of classroom teachers in the field of mathematics learning difficulties. In the development process of the scale, the basic resources and the scale development studies in the literature were examined. First, a pool of substances was created. Content validity was ensured by taking the opinions of 21 expert participants in the 24 question areas determined in the item pool. Content validity index was used in content validity. In this analysis, two questions were removed from the scale. Preliminary application was made with 44 participating teachers who are experts in their fields and exploratory factor analysis was started. For exploratory factor analysis, 359 people consisting of classroom teachers in Sakarya province were studied. In the explanatory factor analysis, 5 items were removed from the scale by looking at the factor load. The factor loadings for the 17 items in the scale vary between 0.508 and 0.976. Among the comparative fit indices obtained as a result of confirmatory factor analysis, RMSEA=0.067 and Cmin/DF=1.568 were calculated. The Cronbach alpha reliability coefficient of the measurements is 0.970. The data obtained were analyzed with statistical methods using SPSS 25, AMOS 24 and Microsoft Excel programs. The validity and reliability criteria of the scale developed within the scope of the study were met.
Keywords:
Subject: Social Sciences - Education
1. Introduction
Individuals may differ for various reasons and in terms of their characteristics. These differences can be seen in one or more features (Özyürek, 2006). If individuals differ significantly from their peers in terms of their characteristics and competencies and need special education, they are called individuals who require special education (Official Gazette, 2018). One of the groups in the classification of individuals requiring special education is learning difficulties. Samuel Kirk was the first person to use the term learning disability in 1962. In 1975, learning disability was accepted as a special education category for the first time (Bender, 2014). The first studies on special learning disabilities started with the research conducted by two doctors at the same time towards the end of the 1800s (Arabacı, 2018). When the research is examined, it is seen that the studies are mostly focused on neurological, psychological and educational areas.
Learning Disability is classified as reading disorder (dyslexia), written expression disorder (dysgraphia), and mathematics learning disability (dyscalculia) (Balcı, 2015; Smith, 2004; Lerner, 1989).
Students who have difficulty learning mathematics in Turkey continue their education as full-time inclusive students in general education classes within the scope of special education. In addition, these students also benefit from supportive education classroom services for mathematics lessons (Girli, 2015). It is known that classroom teachers work in these institutions. The teacher is the person who interacts with the student, designs and implements the program, uses tools, measures and evaluates. Teachers have a very important function in determining, creating and evaluating their behaviors for the student (Gözütok, 1991; Deniz and Hakan, 2021). Teachers can create academic advances in the student with their work (Exley, 2003)
For this reason, teachers’ self-efficacy beliefs play a decisive role in their feelings, thoughts and behaviors about teaching (Demirel, 1993). On the other hand, teachers’ self-efficacy beliefs towards teaching also affect their success in classroom management (Gibson, S., & Dembo, 1984; Saklofske et al., 1988).
In addition, in studies investigating the relationship between teaching self-efficacy beliefs and student achievement (Allinder, 1995, Ross, 1994), it is seen that there is a high correlation and teachers with strong self-efficacy beliefs about teaching have more desire to use methods that will make their students more successful.
This makes self-efficacy belief an important concept for the teaching profession. Bandura (1993, 1997) states that teachers’ abilities and self-efficacy beliefs are effective in the formation of learning environments that will ensure the development of students’ cognitive and academic competencies.
When the literature is examined, it is seen that the studies conducted to measure the competence of teachers started with Gibson and Dembo (1984). It can be said that the most accepted scale in the field of teacher competence is the scale developed by Gibson and Dembo in 1984. Researchers developed a 30-item scale consisting of two dimensions: personal teaching competence and general teaching competence.
It is seen that research on self-efficacy beliefs focuses on pre-school teachers, classroom teachers, different branch teachers (Saracaloğlu and Yenice, 2009; Üstüner, et al., 2009) and teacher candidates.
In the study in which Tschannen-Moran and Woolfolk Hoy (2001) developed a scale called "Teachers’ Self-efficacy Beliefs", they stated that teacher competence belief has an important role in planning the teaching process of teachers (such as selected methods and techniques, student-oriented goal setting), classroom management and classroom communication skills.
In Turkey, there are measurement tools recently adapted to measure teachers’ self-efficacy belief. Diken (2004) adapted the "Teacher Competence Scale" developed by Gibson and Dembo (1984) and revised by Guskey and Passaro (1994) into Turkish. Çapa, Çakıroğlu and Sarıkaya (2005) adapted the Teacher Self-Efficacy Scale developed by Tschannen-Moran and Hoy (2001) into Turkish.
The "Teacher Self-Efficacy Perception Scale" developed by Schwarzer et al. (1999) based on Bandura’s theory was adapted to Turkish by Dilmaç and Izgar (2008) to be used by prospective teachers (Dilmaç and Izgar, 2008).
Aydın (2008) developed the "Self-Efficacy Beliefs Scale for Environmental Education" in order to measure the self-efficacy beliefs of teacher candidates and classroom teachers towards environmental education. In the study conducted by Aksoy and Diken (2009), "Guidance Teacher Special Education Self-Efficacy Scale" was developed to measure the self-efficacy beliefs of counselors regarding psychological counseling and guidance in special education.
Korkut et al. (2016) developed a five-factor acceptable scale in their studies to develop a teacher competence scale for students with learning disabilities. When the studies on teacher competence in different branches in the literature are examined, it is seen that Davran (2006) developed a four-factor Teacher Competence Scale and Dursun and Saracaloğlu (2017) developed a five-factor Information Technology Teacher Competence Scale.
In addition to these scales, there are also scales developed to measure teachers’ self-efficacy in certain areas (Akkoyunlu, Orhan and Umay, 2005; Bıkmaz, 2002; Ekici, 2005).
When the literature on Turkey is examined, it is not possible to say that this research accumulation is sufficient even if an increase in the studies conducted on this subject in recent years is noticeable. On the other hand, it is observed that there are significant deficiencies in the measurement tools that can be used to measure self-efficacy beliefs in the field. (Bıkmaz, 2004).
Measurements of teachers’ self-efficacy specific to the field of mathematics learning disability allow them to understand and predict their behaviors more accurately. In addition, the data to be obtained from the studies on teachers’ self-efficacy in teaching will also provide information on the measures to be taken in the teaching processes, especially in order to increase the academic success of students with mathematics learning difficulties in these subjects. In Turkey, it is known that there is a need for a measurement tool with proven validity and reliability in this regard. These reasons made it necessary to develop a valid and reliable scale in order to determine teachers’ self-efficacy perceptions of mathematics learning difficulties.
2. Method
2.1. Research Model
In the study, since it was aimed to develop a valid and reliable scale to determine teachers’ self-efficacy perceptions in the field of mathematics learning difficulties, the research was built on the screening model. The screening model tries to define the event, individuals and objects that are included in the research under their own conditions and as they are (Karasar, 2018). Through the model, the data on teachers’ self-efficacy perceptions in the field of mathematics learning difficulties were analyzed holistically through the measurement tool developed with the contribution of qualitative methods.
2.2. Population and Sample of the Research
The population of the research consists of classroom teachers working in Sakarya provinces. 329 classroom teachers working in Sakarya province participated in the study. The number of items in the scale developed in the research is 24. In this case, the calculated number of samples should be at least 120. In this context, 329 classroom teachers participated in the research. Therefore, in the development of the scale, more than 5 times the number of items were reached by classroom teachers. When we look at the scale development studies in the literature, Nunnally (1978) stated that the sample size should be taken as five times the number of items. According to DeVellis (2014:157), for each item in the scale, participants should be between 5 and 10 times.
70% of the teachers participating in the study are female and 30% are male. 48.8% of the participants participated in the scale development process face-to-face and 51.2% participated remotely ON THE WEB. 16.3% of the participating teachers have professional seniority of 5 years or less, 20% have professional seniority of 6-10 years, 12.5% have professional seniority of 11-15 years, 22.5% have professional seniority of 16-20 years, and 28.7% have professional seniority of 21 years or more. 1.2% of the participants have an associate degree, 88.8% have a bachelor’s degree and 10% have a master’s degree or higher education. 55% of the participating teachers received special education and 45% did not receive special education. 18.8% of the participating teachers received training on math learning disability and 81.2% did not receive training on math learning disability.
2.3. Data Analysis
Kaiser-Meyer Olkin (KMO) coefficient applied to determine whether the data and sample were suitable for factor analysis was 0.902 and the Bartlett test result (Chi Square 2286.609; p<0.001) was found to be significant. The measurement result found shows that the sampling adequacy is very good (Büyüköztürk, 2012). Explanatory factor analysis (EFA) was performed to examine the factor structures in which the main components of the scale were distributed and to see the dimensions. Confirmatory factor analysis (CFA) was performed to test the model that the scale is two-dimensional. Finally, the Cronbach Alpha Coefficient (Tezbaşaran, 1997) was calculated to determine the reliability of the scale. The data obtained were analyzed with SPSS Statistics 25 Package Program, AMOS 24 and Microsoft Excel Pivot programs.
3. FINDINGS AND COMMENTARY
3.1. Scale Development Studies of the Research
Scale development studies consist of five stages at the theory level. These are; literature review and item pooling, identifying field experts, receiving expert opinions and renewing the item pool, calculating the content validity rates of the revised item pool through the Lawshe technique and creating the original form of the scale (Lawshe, 1975:567; McGartland et al., 2003; Yurdugül, 2005). In this study, the following stages specified by many researchers were followed in order to develop the measurement tool for classroom teachers’ self-efficacy perceptions regarding mathematics learning difficulties (Karasar, 2018).
3.1.1. Substance Pool Process of the Research
The process of creating an item pool started with a large literature search. Domestic and international studies that measure the perception of self-efficacy regarding mathematics learning difficulties have been encountered very rarely, and mostly topics related to general self-efficacy perceptions for courses in schools have been studied. As a result of all these processes, scale items were created to measure the self-efficacy perceptions of classroom teachers about mathematics learning difficulties. In the process of creating the item pool, 3 classroom teachers, 3 mathematics teachers, 2 special education teachers, 1 psychologist working in the Guidance Research Center and 1 director of the Guidance Research Center, a total of 10 people who have previously worked in the field of mathematics learning difficulties or have master’s/doctorate subjects in this field, contributed. Participants were asked to write items related to self-efficacy perception related to mathematics learning disability. In line with the answers received from the participants, scale items were prepared by taking expert opinions from 1 academician in the field of mathematics learning difficulties, 1 in the field of special education, 1 in the field of scale development, and a total of 3 academicians. According to expert opinions in the scale, similar substances, inappropriate for the content and repeated substances were removed from the pool. As a result, 24 precursors were identified. A 5-point Likert type was prepared for the developed scale. Accordingly, the items in the scale were as follows: "Never (1 point) ", "Little (2 points)", "Medium (3 points) ", "Good (4 points)", "Always (5 points)".
3.1.2. Expert Opinion and Scope Validity Rates of the Research
While creating the item pool, the content validity of the items developed at the same time was tested. In this context, experts in their field were used to test. For this, the distribution of field experts formed from academicians and practitioners in the field is shown in Table 1 below.
A total of 24 items were created during the item pool process. The content validity test of these 24 items was performed. 21 field experts were studied on September 2022 (Table 1). Field experts consisted of two groups. The first group is a group of academicians consisting of 1 professor, 2 associate professors, 3 research assistants and a total of 8 people. In the selection of academicians, the criterion of publishing at least one scientific study was taken in mathematics learning difficulty and scale development research. The second group is a group of practitioners consisting of 5 classroom teachers, 4 counselors and psychological counselors, 2 Director/Assistant Director of the Guidance Research Center (RAM), 2 teachers receiving RAM, and a total of 13 people. Participants in the second study group were selected from among those who were trained in learning disability or math learning disability and experienced learning disability in their study areas. The study was conducted face-to-face. According to Lawshe (1975:567), the experts who received the suitability of item structures should be evaluated as "necessary", "corrected" and "unnecessary"; DeVellis (2014:100) stated that the expert group formed in scale development evaluated the originality and comprehensibility of the items. In light of these approaches, expert opinions on the scale items developed to determine the self-efficacy of the teachers participating in the study and content validity rates are given in Table 2 below.
Each item of the content validity rate (CVA) is calculated by the content validity index (CVA) formula (Ne: Required; N: Total number of experts; α=0.05) (Lawshe, 1975:567). According to the findings in Table 2, it can be said that the scale and its sub-items are statistically significant since the overall score of the scale is "KGO>0.703" (p<0.05). In addition, according to the Lawshe technique, the minimum value of 0.42, which corresponds to 21 field experts, was taken and the items 21 and 22, which are smaller than this value, were removed from the scale (CVI21=-0.24<0.42; CVI22=0.05<0.42). Based on all these findings, it was determined that the overall scale developed was statistically significant.
3.1.3. Preliminary Application Study of the Research
Pre-application is a very important stage in which the validity and reliability of the developed scale are questioned based on observational data. At this stage, care should be taken to ensure that the sample group to be pre-applied is compatible with the target audience of the research (Mertens, 1998; Büyüköztürk, 2012:143). In the scale development study, a preliminary application study was conducted with 44 teachers in November 2022 with the face-to-face interview technique in Sakarya province after expert opinions and content validity. There are two criteria in the selection of teachers. The first criterion is the presence of students with mathematics learning difficulties in their classes. The second criterion is that he/she has received in-service training on mathematics learning difficulties or has done postgraduate studies in this field. Accordingly, it was observed that teachers answered the developed scale questions in an average of 6 minutes and 41 seconds. They stated that the questions were understood and there was no expression that would challenge the misunderstanding. These results show that the analysis phase can be started without any question changes in the developed draft scale.
3.1.4. Accuracy Control of the Scale in the Study
In order not to affect the validity and reliability analysis results in the scale study, the averages, standard deviations and frequency distributions were investigated and the accuracy was checked. Material errors that may arise from reasons such as leaving questions unanswered, making mistakes in the entry of data, etc. have been checked. As a result of the controls, it was observed that there was no missing data, incorrect data and incorrect data entry-coding.
In the scale study, various parameters were examined to determine whether the data showed normal distribution. In this context, the skewness and kurtosis values and Kolmogorov-Smirnov values of the data were examined. Since our sample size was more than 30 individuals in the study, the Kolmogorov-Smirnov value was taken for the normality criterion. As seen in the findings in Table 5, the skewness and kurtosis values of the variables were in the range of ±2 (George and Mallery: 2010). In addition, as a result of the Kolmogorov-Smirnov test, the variables showed a significance value of less than 5% (p<0.05). According to these results, it was accepted that the data showed a normal distribution.
3.1.5. Construct Validity and Reliability in the Scale
Exploratory factor analysis was performed for the construct validity of the scale. Confirmatory factor analysis was tested according to the structures of the scale obtained with exploratory factor analysis results. In addition to these, the general validity of the scale was tested by conducting a criterion-based validity study with similarity and differentiation validity. In scale development studies, the validity test precedes the reliability test. The reason for this is that while validity is more about accuracy, reliability is more about stability and consistency (Alpar, 2012:412). Reliability is the consistency in measurement repetition or measurement repetition during measurement (Altunışık et al., 2010:122). Reliability is used for the consistency of the items that make up the scale among themselves.
3.1.6. Exploratory Factor Analyses of the Scale
Exploratory Factor Analysis (EFA) was performed to determine the construct validity of the scale and to reveal the factor structure. For this, basic components and direct oblique rotation methods were used. The reason for this is that the method of basic components is the most frequently and easily used method in practice, and the direct oblique rotation method is used when it is considered that there is a relationship between factors (Büyüköztürk, 2012:124-126).
First of all, the Kaiser-Meyer-Olkin (KMO) sample adequacy value was found to be 0.949 and the sample size was found to be sufficient for EFA. It is an index that compares the size of the partial coefficients with the size of the correlation coefficients observed. If the KMO index is less than 0.50, it is unacceptable, it is classified as weak between 0.50-0.59, moderate between 0.60-0.69, good between 0.70-0.79, very good between 0.80-0.89, and excellent between 0.90-1.00 (Büyüköztürk, 2012; Çokluk et al., 2012). The lowest KMO values calculated for each item were found to be 0.902 and confirmed that the sample was sufficient. However, Bartlett’s sphericity relationship test is another index data showing the appropriateness of explanatory factor analysis (Çokluk et al., 2012:208; Güvenç, 2022:71). As a result of the Barlett test, χ2= 2286.609 ~ df = 136, p=0.000<0.001, and this finding showed that the correlation between the items was large enough for EFA.
As seen in Table 4, the first of the horse dimensions consists of 11 items (items 1, 2, 3, 4, 5, 6, 7, 8, 9, 11 and 13) and the second consists of 6 items (items 14, 15, 16, 17, 18, 19). Tabachnick and Fidell (2007:490) stated that an item can be loaded on more than one item. In such cases, considering the difference between the scale items loaded on more than one factor, he states that it would be appropriate to exclude these items from the analysis if the difference takes a value of 0.10 or less. Accordingly, items 10 and 12 were attributed to more than one factor in the scale, and the difference between the factors on which these items were attributed was found to be less than 0.10. These two items were excluded from the analysis. In addition, Büyüköztürk (2012:136) stated that there may be items that do not give a factor load in any dimension. In such cases, he said, it would be appropriate to exclude the relevant items from the analysis. Accordingly, items 20, 21 and 22 in the scale were not attributed to any factor and these three items were excluded from the analysis.
The lowest item loads were determined as 0.508. Therefore, since factor loads of 0.40 and above are considered ideal (Field, 2009:666), it has been evaluated that the factors contribute significantly to the items. As a result of EFA, it was determined that the scale consisting of 17 items had a 2-dimensional (factor) structure and this factor explained 71.34% of the total variance. In addition, the factors were named as "Personal Teaching Competence" and "Instructional Support Competence", respectively.
3.1.7. Confirmatory Factor Analyses of the Scale
The factor structure of the statements in the scale developed in the study was determined by exploratory factor analysis. Confirmatory factor analysis (CFA) will be performed at this stage in scale development. Thus, the structural accuracy of the scale can be tested. CFA is a type of structural equation model that determines the relationship between the hidden variables observed in the scale. In scale studies, the adaptation of the scale appears to be an important situation. For this reason, fit indices are used to measure the suitability of the research model with confirmatory factor analysis. Goodness of fit is the overlap value between the covariance matrix estimated in the developed scale and the matrix observed by the research (Raykov and Marcoulides, 2006:27). In the study, RMSEA (Kaya, 2014:36), Cmin/df (Tabachnick and Fidell, 2007:488), GFI (Çapık, 2014: 200), CFI (Elderlyoğlu, 2017: 81), NFI (Kaya, 2014: 36) and TLI (Gürbüz and Şahin, 2016:338) values were taken as criteria for the scale development goodness of fit test.
Table 5 summarizes the acceptable and perfectly acceptable fit indices of goodness of fit criteria values:.
Table 5.
Goodness of Fit Metrics and Reference Values.
| Fit Indices | CFA Results | CFA Results | Conclusion | Acceptable fit indices | Excellent fit indices |
|---|---|---|---|---|---|
| CMIN/DF | 1,904 | 1,568 | Excellent | 2 ≤ Cmin/DF ≤ 5 | 0 ≤ Cmin/DF ≤ 2 |
| GFI | 0.877 | 0.909 | acceptable? | 0,90 ≤ GFI ≤ 0,95 | 0,95 ≤ GFI ≤ 1,00 |
| CFI | 0.947 | 0,975 | Excellent | 0,90 ≤ CFI ≤ 0,95 | 0,95 ≤ CFI ≤ 1,00 |
| NFI | 0.904 | 0,934 | acceptable? | 0,90 ≤ GFI ≤ 0,95 | 0,95 ≤ GFI ≤ 1,00 |
| TLI | 0,936 | 0,966 | Excellent | 0,90 ≤ TLI ≤ 1,00 | 0,95 ≤ TLI ≤ 1,00 |
| RMSEA | 0,092 | 0.067 | acceptable? | 0.05 ≤ RMSEA ≤ 0.08 | 0,00 ≤ RMSEA ≤ 0,05 |
| χ2=158.4; df=101; | |||||
Source: Schermelleh-Engel, Moosbrugger, & Müller, (2003).
According to the data in Table 5, the goodness of fit values of the confirmatory factor analysis are seen in the evaluation scale for managers’ performance program applications. Modifications were made to reach good fit values in confirmatory factor analysis. In this context, modifications were made between the error terms e1 and e2 and e1 and e3 in the items of the personal teaching competence factor, and between the error terms e12 and e16 in the items of the educational support competence factor. As a result of the modification analysis made from here, it was seen that the goodness of fit values of the model; GFI, NFI and RMSA values have acceptable good fit values, and Cmin/df, CFI and TLI and values have excellent acceptable good fit values (Schermelleh-Engel, Moosbrugger and Müller, 2003).
The CFA and goodness of fit test values of the criteria used to test the structural accuracy of the scale developed in the study are given in Figure 1 below (A: Personal teaching competence factor; B: Educational support competence factor).
In Figure 1C, Teacher Self-Efficacy Scale Confirmatory Factor Analysis in the Field of Learning Difficulty in Mathematics.
Figure 1 shows the confirmatory factor analysis and path graph of the teacher self-efficacy scale in the field of mathematics learning disability. Factor loadings of the confirmatory factor analysis of the teacher self-efficacy scale in the field of mathematics learning disability were found to be between 0.68 and 0.90 for the personal teaching competence factor. Here, the factor load of the first question of the Personal teaching competence factor is 0.68. The expected value is at least 0.70 or close to the factor load. However, considering other factor loads, 0.68 is considered to be negligible and acceptable (Schermelleh-Engel, Moosbrugger and Müller, 2003). It was determined that it varied between 0.80 and 0.88 for the educational support adequacy factor. In addition, the significance values of the regression weights obtained as a result of confirmatory factor analysis were less than 0.05. According to these results, we can say that the items are loaded correctly to the factors.
3.2. Reliability Analyses of the Scale
According to Bollen (1989), reliability is consistency in measurement. In other words, it is the determination of measurement under different conditions to achieve the same results (Bollen, 1989). In reliability analysis; Cronbach’s Alpha method is used as the main method. If the Cronbach Alpha coefficient values of the scale items are between 0.80 and 1.00, it can be said that the scale is at a high reliability level. If the coefficient values are between 0.60 and 0.80, it can be said that the scale is quite reliable. Again, if the coefficient values are between 0.40 and 0.60, the reliability level of the scale is considered low. Finally, if the coefficient values are between 0.00 and 0.40, it is considered that the scale is not a reliable scale (Alpar, 2012).
Table 6 shows the factor correlation values of the teacher self-efficacy scale in the field of mathematics learning disability. Accordingly, it was found that there was a positive and highly significant relationship between the personal teaching competence factor and the educational support competence factor.
Reliability analyses of the scale and its sub-factors are given in Table 7. Accordingly, it is seen that the scale is at a very high reliable level (Cronbach’s Alpha=0.970). Considering the values obtained as a result of the reliability analysis of the sub-dimensions of the scale, the Personal teaching competence dimension (Cronbach’s Alpha=0.961) and the Teaching support competence dimension (Cronbach’s Alpha=0.938), it can be said that it is at a very high level of reliability. Therefore, it can be said that the scale developed in the study has a high level of reliability in general.
Table 8 shows the reliability total statistical table data of the scale. According to the table, when we remove any item, the Cronbach’s Alpha value of the scale, which is 0.970, does not exceed any item. Therefore, it was accepted that the reliability of the scale was high in general.
4. Conclusion and Recommendations
In this study, a valid and reliable scale that can be used to determine teachers’ self-efficacy in the field of mathematics learning difficulties was developed. The scale consists of a total of 17 items answered in five-point Likert type. The scale also measures teachers’ self-efficacy in learning mathematics and the dimensions of personal teaching competence and instructional support competence, which are two sub-factors. The Cronbach’s Alpha coefficient of the scale was 0.88. The high score to be obtained from the scale indicates that teachers’ self-efficacy belief in mathematics learning difficulties is high.
The scale developed in this study can be used in research on mathematical learning difficulties. The self-efficacy of students with learning disabilities can be increased by determining the self-efficacy of teachers with learning disabilities. In addition, it can be determined in which ways teachers can provide supportive education to students with mathematics learning difficulties. Studies can be planned to improve teachers’ self-efficacy.
References
- Akkoyunlu, B. , Orhan, F., & Umay, A. (2005). Computer teaching self-efficacy scale for computer teachers. Hacettepe University Journal of Education Faculty, 29, 1-8.
- Allinder. R. M. (1995). An examination of the relativity between teacher effectiveness and curriculum based measurement and student achievement. Remedial & Special Education. 27.141-152.
- Alpar, R. (2012). Applied Statistics and Validity-Reliability with Examples from Sports Health and Educational Sciences, Ankara: Detay Publishing.
- Altunışık, R. , Coşkun, R., Bayraktaroğlu, S. and Yıldırım, E. (2010). |||UNTRANSLATED_CONTENT_START|||Sosyal Bilimlerde Araştırma Yöntemleri, SPSS Uygulamalı. |||UNTRANSLATED_CONTENT_END|||Sakarya: Sakarya Yayıncılık.
- Arabacı, Ö. (2018). Examination of the Knowledge Levels and Opinions of Classroom Teacher Candidates on Special Learning Difficulties, Unpublished Master’s Thesis. Gaziantep University, Gaziantep.
- Aydın, N. (2008). The effect of classroom level, seniority and value orientations on the self-efficacy beliefs of classroom teacher candidates and teachers towards environmental education. (Published master thesis) Aydın: Adnan Menderes University Institute of Social Sciences.
- Balcı, E. (2019). Teachers’ opinions about dyslexia and the problems they face. Aegean Education Journal, 20(1), 162-179.
- Bandura, A. (1993). Perceived self-efficacy in cognitive development and functioning. Educational psychologist, 28(2), 117-148.
- Bandura, A. (1997). |||UNTRANSLATED_CONTENT_START|||Self-efficacy: The exercise of control. |||UNTRANSLATED_CONTENT_END|||New York: Freeman.
- Bender, W. N. (2014). Individuals with learning disabilities and their education. In H. Sarı (Ed.), Changing definitions of special learning disability (M.Yılmaz, Trans.) (pp. 1-37). Ankara: Nobel.
- Bıkmaz, F. H. (2002). Self-efficacy belief scale in science teaching. Educational Sciences and Practice, 1(2), 197-210.
- Bıkmaz, F.H. (2004). Öz yeterlik inançları. In Raven, Y. and Deryakulu, D. (Ed.), Individual differences in education (pp. 289-315). Ankara: Nobel Yayın Dağıtım.
- Büyüköztürk, Ş. (2012) Scientific Research Methods. Ankara: Pegem Akademi Yayınları.
- Çapa, Y. , Çakırogğlu, J. and Sarıkaya, H. (2005). The development and validation of a turkish version of the teachers’ sense of efficacy scale. Education and Science, 30 (137), 74-81.
- Çapık, C. (2014). Use of confirmatory factor analysis in validity and reliability studies. Anadolu Journal of Nursing and Health Sciences, 17(3), 196-205.
- Davran, E. (2006). The Effect of Teaching Practice in Primary Education Institutions on Teacher Candidates’ Acquisition of Teaching Competencies (Van province example). Yüzüncü Yıl University Institute of Social Sciences, Master’s Thesis. Van.
- Deniz, S. , & Hakan, S. (2021). Development of the teacher competence scale in the field of special learning disability. Trakya University Journal of Social Sciences, 23(1), 1-20. 1.
- DeVellis, R. F. (2014). Scale development theories and applications. (3. Edition). Nobel Yayıncılık.
- Diken, İ.H. (2004). Turkish adaptation of the teaching competence scale, validity and reliability study. Journal of Educational Research, 16, 102-112.
- Dilmaç, B. and Izgar, H. (2008). Examination of self-efficacy perceptions and epistemological beliefs of prospective administrator teachers. Web: (Download Date: 10.02.2023). Available online: http://www.sosyalbil.selcuk.edu.tr/sos_mak/articles/2008/20/HIZGAR-DILMA C.PDF.
- Dursun, F. and Saracaloğlu, A. S. (2017). Evaluation of Information Technology Teacher Competencies. |||UNTRANSLATED_CONTENT_START|||Turkish Studies International Periodical for the Languages, Literature and History of Turkish or Turkic, 12 (23), 89-120.|||UNTRANSLATED_CONTENT_END|||.
- Ekici, G. (2005). Validity and reliability of the biology self-efficacy scale. Hacettepe University Journal of Education Faculty, 29, 85-94.
- Exley, S. (2003). The effectiveness of teaching strategies for students with dyslexia based on their preferred learning styles. British Journal of Special Education, 30(4), 213-220. [CrossRef]
- George, D. , & Mallery, P. (2016). SPSS For Windows, Step By Step: A Simple Guide And Reference. Boston, Ma: Allynand Bacon.
- Gibson, S. & Dembo, M. H. (1984). Teacher efficacy: A construct validation. |||UNTRANSLATED_CONTENT_START|||Journal of Educational Psychology, 76(4), 569–582. |||UNTRANSLATED_CONTENT_END|||.
- Girli, A. (2015). Education of children with learning disabilities. S. S. Yıldırım-Doğru, (Ed.), Learning Disabilities (p. 227-232). The Educational Book.
- Gözütok, D. (1991). Evaluation of professional behaviors of teachers and students according to their perceptions. Ankara University Journal of Faculty of Educational Sciences, 24(2), 405-409.
- Guskey, T. R. , & Passaro, P. D. (1994). Teacher effectiveness: A study of construct dimensions. American Educational Research Journal, 31, 627-643.
- Gürbüz, S. & Şahin, F. (2016), Research Methods in Social Sciences; Philosophy-Method-Analysis (2. Ed.). Ankara: Seçkin Yayıncılık.
- Güvenç, H. (2022). Factors affecting satisfaction with the purchased housing. Unpublished PhD Thesis, Hasan Kalyoncu University Graduate Education Institute, Gaziantep.
- Karasar, N. (2018). Scientific research method (Thirty-third edition). |||UNTRANSLATED_CONTENT_START|||Ankara: Nobel Yayıncılık. |||UNTRANSLATED_CONTENT_END|||.
- Kaya, Ş. (2014), Structural equation modeling: the relationship between dizziness, anxiety and exaggerating bodily sensations. Unpublished PhD Thesis, Uludağ University, Bursa.???
- Korkut, A. , Keskin, I., & Can, S. (2016). Developing a scale of teacher competencies for students with learning disabilities. Journal of Amasya University Faculty of Education, 5(1), 133-155.
- Lawshe, C. H. (1975). Quantitative aproach to content validity. FARUK ERSAN EVYAPAN be given Personnel Phychology, 28(4), 563-575.
- Lerner, J. W. (1989). Educational interventions in learning disabilities. Journal of the American Academy of Child & Adolescent Psychiatry, 28(3), 326-331.
- McGartland, R. D. , Berg-Weger, M., Tebb, S., Lee, E. S., & Rauch, S. (2003). “Objectifying content validity: Conducting a content validity study in social work research”. Social Work Research, 27(2), 94 – 104.
- Mertens, D. (1998). Research methods in education and psycohology. New York: SAGE Pub.
- Nunnally, J. C. (1978). An overview of psychological measurement. Clinical diagnosis of mental disorders: A handbook, 97-146.
- Özyürek, M. (2006). Changing attitudes towards people with disabilities. Ankara: Kök Yayıncılık.
- Raykov, T. & Marcoulides, G. A. (2006). A First Course in Structural Equation Modeling. Lawrence (2nd Ed.). Mahwah, New Jersey: Lawrence Erlbaum.
- Official Gazette (2018). Special Education Services Regulation.
- Saklofske, D. H. , Michayluk, J. O., & Randhawa, B. S. (1988). Teachers’ effectiveness and teaching behaviors. Psychological reports, 63(2), 407-414.
- Saracaloğlu, A. S. and Yenice, N. (2009). Examination of science and classroom teachers’ self-efficacy beliefs in terms of some variables. Theory and Practice in Education. 5. (2). 244-260.
- Schwarzer, R. , Schmitz, G. S., & Daytner, G. T. (1999). Teacher Self-Efficacy Scale. Directory of Survey Instruments.
- Smith, T. E. (2005). IDEA 2004: Another round in the reauthorization process. Remedial and Special Education, 26(6), 314-319.
- Tabachnick, B. G. & Fidell, L. S. (2007). Using Multivariate Statistics (5th ed.). New York: Allyn and Bacon.
- Tezbaşaran, A. A. (1997). Likert-type scale development guide. Turkish Psychologists Association (TPD).
- Tschannen-Moran, M. , & Hoy, A. W. (2001). Teacher competence: Catching a difficult structure. Teaching and teacher education, 17 (7), 783-805.
- Üstüner, M. , Demirtaş, H., Cömert, M., & Özer, N. (2009). Secondary school teachers’ self-efficacy beliefs. Mehmet Akif Ersoy University Journal of Education Faculty, 9(17), 1-16.
- Elderly, M. M. (2017). Factor analysis and validity in the social sciences: using exploratory and confirmatory factor analyses. Istanbul University Journal of Business Administration Faculty, 46(Special Issue), 74-85.
- Yurdagül, H. (2005). Using content validity indices for content validity in scale development studies. XIV. National Educational Sciences Congress, 1,771-774.
Figure 1.
Caption.
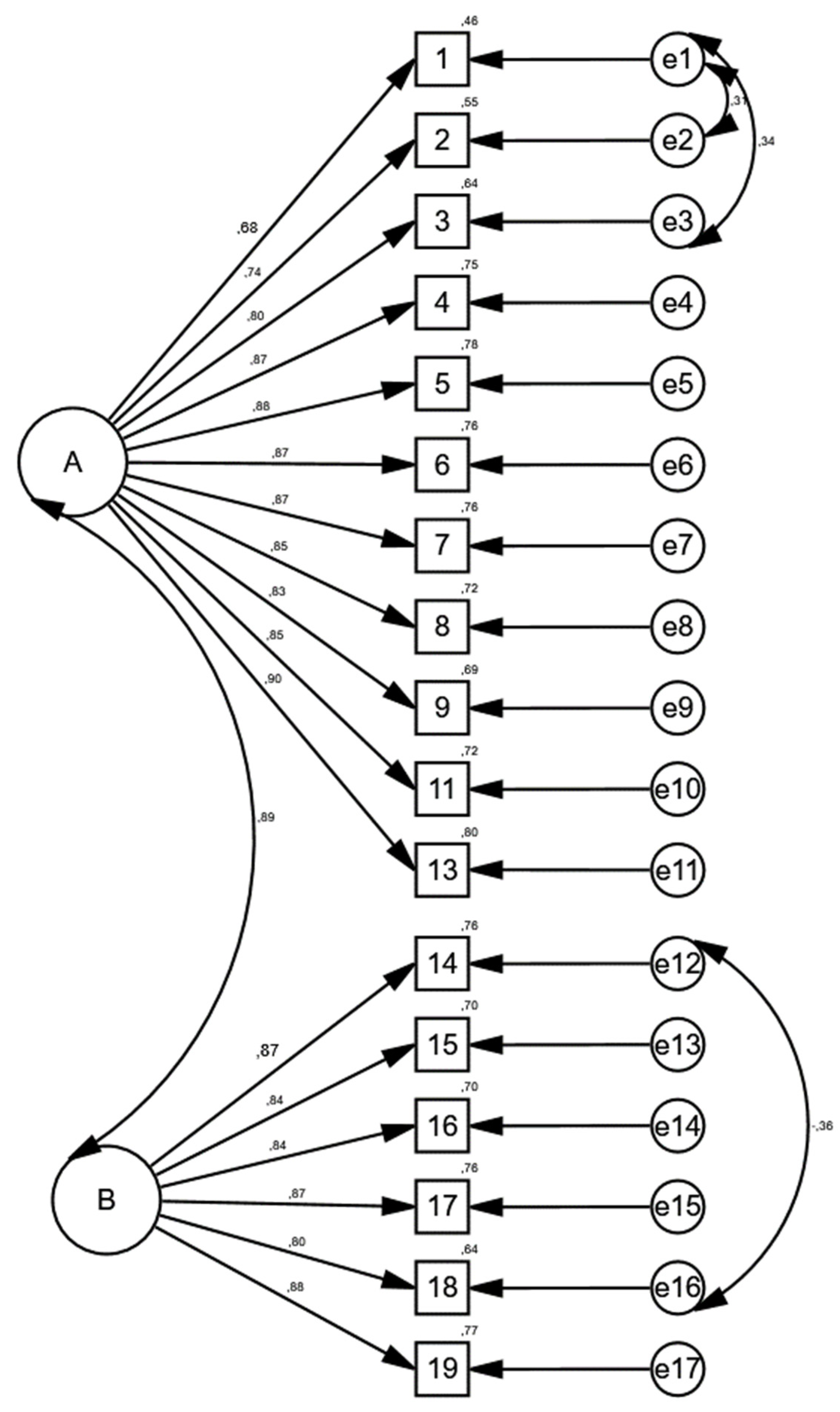
Table 1.
Distribution of Item Pool Area Experts.
| Distribution of Field Experts | ||||
|---|---|---|---|---|
| Academicians | ||||
| Professor | Assoc. Dr. | Dr. Prof. Dr. | Research Assistant | Total |
| 1 | 2 | 2 | 3 | 8 |
| Performers | ||||
| Classroom Teacher | Guidance and Psychological Counselor | RAM Manager/Vice Manager | Special education teacher | Total |
| 5 | 4 | 2 | 2 | 13 |
| Grand Total | 21 | |||
Table 2.
Mathematics Learning Difficulty Area Classroom Teacher Self-Efficacy Scale.
| Mathematics Learning Difficulty Area Classroom Teacher Self-Efficacy Scale | Unnecessary | Necessary but must be corrected | Required | Scope Validity Rate | |
| Substances | |||||
| 1 | I can notice the symptoms that exist in students with math learning difficulties. | 17 | 2 | 2 | 0.62 |
| 2 | I am knowledgeable about the characteristics of students with Math Learning Difficulty. | 16 | 2 | 3 | 0.52 |
| 3 | I am competent in making program-based evaluations for students with Math Learning Difficulty. | 17 | 2 | 2 | 0.62 |
| 4 | I can plan teaching for students with Math Learning Difficulties | 19 | 0 | 2 | 0.81 |
| 5 | I can identify methods and techniques for students diagnosed with Math Learning Difficulty. | 18 | 1 | 2 | 0.71 |
| 6 | I can make adaptations in methods and techniques for students with Math Learning Difficulty. | 18 | 2 | 1 | 0.71 |
| 7 | I am competent in what learning strategies are for students with Math Learning Difficulties. | 19 | 1 | 1 | 0.81 |
| 8 | I am qualified to improve the math skills of students with Math Learning Difficulty. | 16 | 2 | 3 | 0.52 |
| 9 | I am competent in editing the content for the student diagnosed with Math Learning Disability. | 15 | 4 | 1 | 0,43 |
| 10 | I am competent in planning counting and four operations activities for students with Math Learning Difficulty. | 19 | 1 | 1 | 0.81 |
| 11 | I can use special teaching strategies that are suitable for students with Math Learning Difficulty. | 18 | 0 | 3 | 0.71 |
| 12 | I am qualified to benefit from technology for students with Math Learning Difficulty. | 19 | 1 | 1 | 0.81 |
| 13 | My knowledge of adapting teaching is sufficient for students with Math Learning Difficulty. | 17 | 3 | 1 | 0.62 |
| 14 | I can arrange appropriate classroom environments for students with Math Learning Difficulties. | 17 | 4 | 0 | 0.62 |
| 15 | I can guide the families of students with Math Learning Difficulty. | 20 | 0 | 1 | 0.90 |
| 16 | I am competent in cooperating with other teachers for the student diagnosed with Math Learning Disability. | 20 | 1 | 0 | 0.90 |
| 17 | I can help my students with Math Learning Difficulty solve numeracy problems. | 17 | 2 | 2 | 0.62 |
| 18 | I am competent in the process evaluation used to improve the success of students diagnosed with Math Learning Disability in the course. | 18 | 1 | 2 | 0.71 |
| 19 | I can prepare different materials for students with mathematics learning difficulties. | 16 | 1 | 4 | 0.52 |
| 20 | I am competent in developing the problem-solving skills of my students with Math Learning Difficulty. | 17 | 3 | 1 | 0.62 |
| 21 | I understand students with Math Learning Difficulties | 8 | 9 | 4 | -0.24 |
| 22 | I know the difficulties of my students with Math Learning Difficulty | 11 | 5 | 9 | 0,05 |
| 23 | I do not have enough information about the characteristics of students diagnosed with Math Learning Difficulty. | 19 | 1 | 1 | 0.81 |
| 24 | I do not have enough knowledge to organize the program content for students diagnosed with Math Learning Disability. | 20 | 0 | 1 | 0.90 |
| Mean/average | 0.703 (70%) | ||||
Table 3.
Normality Test Table.
| Skewness | Kurtosis | Kolmogorov-Smirnov | |||
|---|---|---|---|---|---|
| Statistical | df | Sig. | |||
| I can notice the symptoms that exist in students with math learning difficulties. | 0,225 | ,851 | 0,170 | 329 | 0.000 |
| I am knowledgeable about the characteristics of students with Math Learning Difficulty. | ,358 | 761 | 183 | 329 | 0.000 |
| I am competent in making program-based evaluations for students with Math Learning Difficulty. | 0,404 | .697 | 0,187 | 329 | 0.000 |
| I can plan the teaching related to students with Math Learning Difficulty. | 0,532 | .442* | 0.212 | 329 | 0.000 |
| I can identify methods and techniques for students diagnosed with Math Learning Difficulty. | 0.441 | -.557 | 0,187 | 329 | 0.000 |
| I can make adaptations in methods and techniques for students with Math Learning Difficulty. | ,561 | 385 | 0.209 | 329 | 0.000 |
| I am competent in what learning strategies are for students with Math Learning Difficulties. | 0,706 | 0,077 | 0.211 | 329 | 0.000 |
| I am qualified to improve the math skills of students with Math Learning Difficulty. | 0.597 | -.251 | 0.223 | 329 | 0.000 |
| I am competent in editing the content for the student diagnosed with Math Learning Disability. | 0,641 | ,460 | 0,203 | 329 | 0.000 |
| I am competent in planning counting and four operations activities for students with Math Learning Difficulty. | 0,543 | 409 | 0.223 | 329 | 0.000 |
| I can use special teaching strategies that are suitable for students with Math Learning Difficulty. | 0.845 | -0,060 | 0.231 | 329 | 0.000 |
| I am qualified to benefit from technology for students with Math Learning Difficulty. | ,544 | ,379 | 0.213 | 329 | 0.000 |
| My knowledge of adapting teaching is sufficient for students with Math Learning Difficulty. | -,707 | 176 | 0,250 | 329 | 0.000 |
| I can arrange appropriate classroom environments for students with Math Learning Difficulties. | .583 | 181 | 0,225 | 329 | 0.000 |
| I can guide the families of students with Math Learning Difficulty. | .434 | .735 | .208 | 329 | 0.000 |
| I am competent in cooperating with other teachers for the student diagnosed with Math Learning Disability. | 0.341 | .895 | .184 | 329 | 0.000 |
| I can help my students with Math Learning Difficulty solve numeracy problems. | 0,378 | .554 | 0.180 | 329 | 0.000 |
| I am competent in the process evaluation used to improve the success of students diagnosed with Math Learning Disability in the course. | 0.185 | .907 | -0.172 | 329 | 0.000 |
| I can prepare different materials for students with mathematics learning difficulties. | 0.430 | -,707 | 0,193 | 329 | 0.000 |
| I am competent in developing the problem-solving skills of my students with Math Learning Difficulty. | 0.743 | -.352 | 0.213 | 329 | 0.000 |
| I do not have enough information about the characteristics of students diagnosed with Math Learning Difficulty. | 0.334 | 726- | 0.276 | 329 | 0.000 |
| I do not have enough knowledge to organize the program content for students diagnosed with Math Learning Disability. | 0.756 | 0.820 | 0.271 | 329 | 0.000 |
Table 4.
Teacher Self-Efficacy Scale Factor Analysis Findings in the Field of Learning Difficulty in Mathematics.
Table 4.
Teacher Self-Efficacy Scale Factor Analysis Findings in the Field of Learning Difficulty in Mathematics.
| Substances | Factor 1 | Factor 2 |
|---|---|---|
| Personal Teaching Qualification 4 | .976 | |
| Personal Teaching Qualification 6 | .941 | |
| Personal Teaching Qualification 5 | .940 | |
| Personal Teaching Competence 7 | ,884 | |
| Personal Teaching Qualification 2 | .746 | |
| Personal Teaching Competence 3 | .735 | |
| Personal Teaching Competence 9 | .735 | |
| Personal Teaching Qualification 13 | .630 | |
| Personal Teaching Qualification 11 | .628 | |
| Personal Teaching Competence 1 | 620 | |
| Personal Teaching Competence 8 | .553 | |
| Instructional Support Competence 19 | .894 | |
| Instructional Support Competence 18 | ,891 | |
| Instructional Support Competence 16 | .761 | |
| Instructional Support Competence 17 | .759 | |
| Instructional Support Competence 15 | -.718 | |
| Instructional Support Competence 14 | .508 | |
| Eigenvalue | 8.388 | 4,531 |
| Variance Explained | 66,875 | 4.460 |
| Total Variance Explained | 71,335 | |
Table 6.
Scale Internal Consistency Factor Correlation Matrix.
| *Factor Correlation Matrix | ||
| Factor | Personal teaching qualification | Instructional support competence |
| Personal teaching qualification | 1.000 | |
| Instructional support competence | .841 | 1.000 |
**. Correlation is significant at the 0.01 level (2-tailed).
Table 7.
Reliability Analysis Table of the Scale.
| Reliability | Dimensions | Number of Items | Cronbach’s Alpha |
|---|---|---|---|
| Overall internal consistency | Scale | 17 | .970 |
| Internal consistencies of Sub-Factors | Personal teaching qualification | 11 | 0,961 |
| Instructional support competence | 6 | 0.938 |
Table 8.
Table of Reliability Total Statistics of the Scale.
| Matter | Scale Mean if Item Deleted | Scale Variance if Item Deleted | Corrected Item-Total Correlation (CITC) | Cronbach’s Alpha if Item Deleted | |
| 1 | 36.3333 | 210,630 | .683 | .970 | |
| 2 | 36.6744 | 211,659 | .740 | 969 | |
| 3 | 36.7054 | 210,131 | ,799 | 969 | |
| 4 | 36.7907 | 209,354 | .821 | .968 | |
| 5 | 36.6822 | 208,609 | 840 | .968 | |
| 6 | 36.7984 | 210,350 | .821 | .968 | |
| 7 | 36.8527 | 210,345 | -.832 | .968 | |
| 8 | 36.7364 | 209,555 | -.832 | .968 | |
| 9 | 36.7287 | 207,449 | .813 | .968 | |
| 10 | 36.8295 | 207.908 | .816 | .968 | |
| 11 | 36.7674 | 207,883 | ,879 | .968 | |
| 12 | 36.6667 | 209,005 | .843 | .968 | |
| 13 | 36.5504 | 208,171 | ,787 | 969 | |
| 14 | 36.4884 | 207,267 | .783 | 969 | |
| 15 | 36.5891 | 208,713 | .823 | .968 | |
| 16 | 36.3643 | 209,671 | .711 | .970 | |
| 17 | 36.5736 | 207,450 | .812 | .968 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Copyright: This open access article is published under a Creative Commons CC BY 4.0 license, which permit the free download, distribution, and reuse, provided that the author and preprint are cited in any reuse.
MDPI Initiatives
Important Links
© 2024 MDPI (Basel, Switzerland) unless otherwise stated






