Preprint
Article
Nucleon-Pair Shell Model with Symmetry Broken Basis
Altmetrics
Downloads
132
Views
27
Comments
0
A peer-reviewed article of this preprint also exists.
This version is not peer-reviewed
Submitted:
06 June 2023
Posted:
12 June 2023
You are already at the latest version
Alerts
Abstract
The nucleon-pair shell model (NPSM) with broken symmetry basis is studied. The results demonstrate the validity of NPSM with symmetry broken basis, which leads to a significant reduction in the dimensionality of the multi-pair configuration space. We have observed that the axial-deformed basis provides a satisfactory description of the low-lying spectrum, even without the need for the projection procedure. In the case of the triaxial-deformed basis, we have investigated the variation after angular momentum projection, indicating the potential for NPSM to find exact solutions in the half-closed system.
Keywords:
Subject: Physical Sciences - Nuclear and High Energy Physics
1. Introduction
Atomic nuclei are self-bounded quantum many-body systems. Modeling many-body problem is one of the crucial challenging problems in theoretical nuclear physics. There has been much progress in this topic: the Green’s function Monte Carlo Method [1,2], the full configuration interaction (FCI) approaches, so-called no-core shell model (NCSM) [3], and the symmetry-adapted no-core shell model [4], etc. The methods mentioned above are limited to the cases for light nuclei. The coupled-cluster method (CC) [5] has been applied to both light nuclei and medium mass nuclei around closed shell. The mean-field theory can be applied to the whole nuclear chat [6], but the mean-field approximation may have lost the key many-body correlation between nucleons. The valence space shell model (SM) play an important role in describing and understanding nuclear structure [7,8,9,10,11,12,13]. However, the full-fledged SM studies are still out of reach for the heavy mass nuclei because of the huge number of configurations [14]. There are many models worked on rotational symmetry broken basis, and the angular momenta are restored by projection technique, such as the projected Hartree-Fock [15,16] including variation after projection [15,17], the Hartree Fock-Bogoliubov [15,18,19,20] and projected relativistic mean-field calculations [21], the Monte Carlo shell model [22,23], the projected shell model [24,25,26], the projected configuration interaction [27] and projected generator coordinate methods [28,29].
In 1993, a new technique, known as generalized wick theory, has been proposed to calculate the commutators for coupled operators and fermion clusters by Chen et al.[30,31]. Based on this new technique, a nucleon pair shell model (NPSM) has been constructed [32], in which the building blocks of the configuration space are nucleon-pairs. Due to the success of the interacting boson model (IBM)[33], model space of NPSM was truncated to the pair subspace, then the model is developed into the so-called SD-pair shell model (SDPSM). It was shown that the collectivity of the low-lying states can be described very well in the pair subspace [34,35,36,37]. It is also found that within the framework of M-schemed NPSM, the CPU time required in calculating the matrix elements can be reduced a lot [38,39].
At a more fundamental level, the truncation of the NPSM should be studied carefully. Up to now, no one has proved the convergency of the truncation. To compensate for such problem, the basis should be constructed by more kinds of collective pairs, and the pair structure coefficients should also be determined by a variation procedure [40,41,42,43]. But the dimensionality of the many-pair model space increases rapidly with the number of collective pairs, and hindering its further study. In the NPSM, the nucleon-pairs always have good angular momentum J and the third component M, and the many-body basis keeps the rotational invariance. Therefore, both the total and intermediate angular momentum J or third component M, depending on J-scheme or M-scheme is adopted, should be good quantum numbers. To work with a complete subspace, a group of multi-pair basis should be considered, so we introduce the symmetry broken pairs in the NPSM in this paper, and some preliminary results of half-closed nucleus are given.
2. The Model
In this paper, as a preliminary report, we discuss the half-closed nuclei only. The general form of the shell model Hamiltonian is adopted as
where stands for a oscillator single particle orbit (nlj), is the single-particle creation operator, is the single-particle annihilation operator, is the single-particle energy, the symbol × represent angular momentum coupling, which is given
where is Clebsch-Gordan (CG) coefficient.
2.1. NPSM Framework
The building blocks of the NPSM are collective pairs with good angular momentum J and third component M, designated as , which is built from many non-collective pairs ,
Then the multi-pair basis can be constructed in both M-scheme [38,39] and J-scheme [32,36], respectively,
and
When certain collective pairs are considered, a set of multi-pair basis are constructed, and those bases are dependent on each pair structure coefficients. The matrix elements of Hamiltonian can be carried out by using the commutators between collective pairs [32,36,38,39]. Then the Hamiltonian can be diagonalized in such subspace, and the sum of few lowest energies E can be minimized
Taking into consideration of the broken rotational symmetry of pairs, the collective pairs with good angular momentum are mixed. First, we would like to introduce the axial-deformed pairs, which are given by summing the pairs with the same quantum number M and different angular momentum J,
where the axial-deformed pairs keep the third component of angular momentum as a good quantum number. A set of multi-pair basis with fixed total third component M are constructed, after the collective pairs are introduced,
The Hamiltonian can be diagonalized in a subspace with fixed total third component M.
One can also introduce the triaxial-deformed pairs,
where both the angular momentum J and third component M are mixed to break the rotational invariance. The multi-pair basis is given,
Since all rotational symmetry are broken, only one basis exists. In current work, the triaxial-deformed pairs are chosen to be different, where the pair structure coefficients are independent for the different triaxial-deformed pairs.
2.2. Restore Rotational Symmetry
The rotational symmetry can be restored by the standard projection process via quadrature over orthogonal functions. The advantage of the rotation of a state with good total angular momentum J and third component does not mix total angular momentum J, but does mix the third component [15].
where is a Wigner D matrix, is the rotation operator over the Euler angles
where and are the generators of rotations about the z and y axes, respectively. The Wigner D functions are the matrix elements of the rotation operator in a basis with good angular momentum quantum numbers, and the Wigner D functions compose of a complete orthogonal set,
To carry out angular momentum projection, the collective pairs should be rotated. For the collective pairs with definite angular momentum J and M, the rotation is
For triaxial-deformed pairs case, the rotation of pairs are given as
Any rotational symmetry broken basis is a mixture of states with good angular momenta
where index i are used to distinguish components with same quantum number J but different third component . Applying the rotation operator to the basis,
where indicate the quantum number in initial state, and denote the third component in rotated state. The norm matrix elements are projected out by the standard equation,
The Hamiltonian matrix elements are given
Then the generalized eigenvalue problems for every J are solved, where the solutions are labeled by r,
In this work, we only perform variation after projection on triaxial-deformed basis.
3. Numerical Results
3.1. Axial-Deformed Basis
Usually the collective pairs with lowest angular momenta J, such as S (J = 0), D (J =2) and G (J = 4), are considered. For the low-lying states, these collective pairs should be dominant. The dimension of multi-pair basis increases dramatically when more types of collective pairs are included. Originally those many-pair basis are non-normal and non-orthogonal, the Hamiltonian matrix elements should be transformed to normal orthogonal basis. To this end, one have to diagonalize the norm matrix, which are very painful for large configuration space. On the contrary, the dimension of configuration space will be reduced when the rotation symmetry is broken. As shown in Figure 1, the dimension increases slowly for the case with axial-deformed basis, where the angular momentum J of collective pairs are mixed but third component M does not.
As a preliminary report, the low-lying spectrum of 138Sn are studied for axial-deformed basis. We assume that the nucleus 132Sn is a closed core and the 82-126 shell( 0h9/2, 1f7/2, 1f5/2, 2p3/2, 2p1/2, and 0i13/2) is chosen as the valence neutrons shell.
The two-body matrix elements of the effective interaction are derived from the CD-Bonn NN potential [44]. The strong short-range repulsion term is renormalized by integrating out the high-momentum components above a certain cutoff momentum [45]. A smooth potential , keeping the physics of bare nucleon-nucleon interaction up to , is constructed, and can be used in calculations of shell model effective interactions. In this work, is fixed as 2.2 fm−1. Then the shell model effective interaction, with the Coulomb force for protons, is carried out within the framework of -box folded-diagram expansion [46,47,48]. In this paper, the -box is calculated up to the second order in .
Our configuration are constructed by SDG collective pairs. Although the angular momentum J of each collective pairs is mixed, the third component M is a good quantum number and the total M of each multi-pair basis is also a good quantum number. The effective Hamiltonian is diagonalized in this subspace and the pair structure coefficients are determined by searching the minimum energy.
The lowest energy levels in each axial-deformed subspace with different third component M in the NPSM are shown in Figure 2, where the shell model calculations are carried out using KSHELL code [49]. It is seen that the results got from the NPSM are close to the SM. The shift of level from the NPSM to SM is 0.059 MeV, and the shift of is 0.095 MeV. Those results suggest the low-lying levels of 138Sn can be described in the SDG pair subspace. This result suggested that the NPSM can reproduce the spectrum very well with the axial-deformed basis.
3.2. Triaxial-Deformed Basis
The NPSM was also studied with the triaxial-deformed basis. There are two ways to recover the rotational symmetry: Variation before projection (VBP) and variation after projection (VAP). Here the VAP is used. The low-lying levels of 20O nucleus is studied with the USDA interaction [50]. Only one basis is used for each level in our variation procedure. The results given in Figure 3 show that the NPSM are in consistent with those got in the SM. For example, the first level from SM is MeV, the one from NPSM is also MeV, the difference between the SM and NPSM is close to the limit of machine precision.
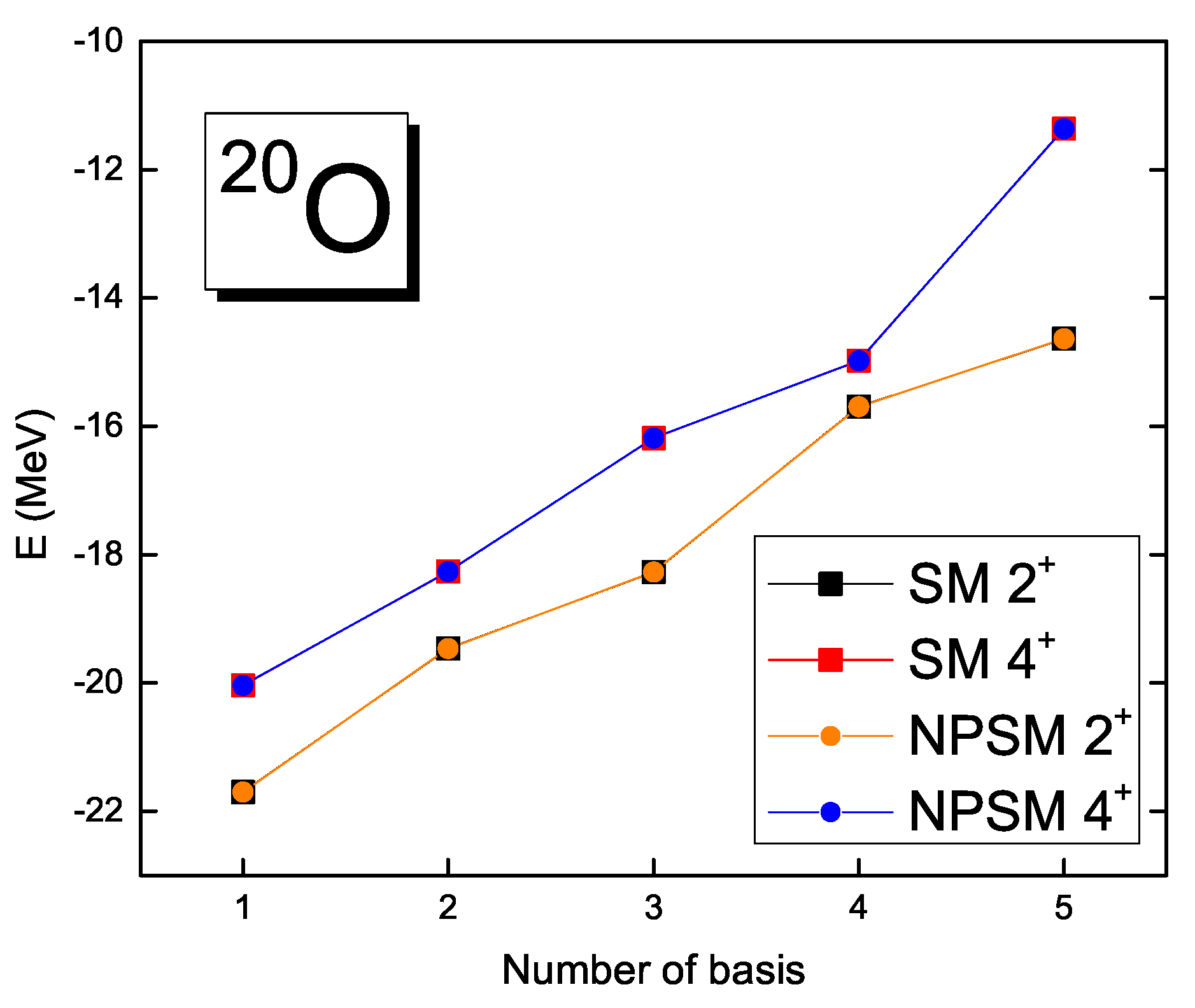
To generate more levels with same angular momenta J, more basis should be used in configuration space. For example, if we want to obtain the second states, the first level should be calculated first, which can be described by one basis, after determining the parameters for the first basis, the second basis are considered in the model space, then the parameters of the second basis are fixed by a variation procedure.
As shown in Figure 4, the lowest 5 levels with J=2 and J=4 are calculated in the SM and NPSM with triaxial-deformed basis. We found that the difference between the NPSM and SM is negligible within the machine precision limit. Those results suggest that if the VAP is adopted, there may be no significant difference between the NPSM and SM.
4. Summary and Discussion
In this paper, the validity of the symmetry broken basis in the NPSM was studied. It was found that the NPSM with axial-deformed basis can reproduce the low-lying spectrum of the half-closed nucleus very well. If the triaxial-deformed basis and variation after projection were used for half-closed nucleus, the difference between the NPSM and SM is negligible. These results may suggest that the axial-deformed basis and triaxial-deformed basis may provide a satisfied description for low-lying spectrum. The case for the proton-neutron coupled open system will be studied in our next work.
Acknowledgments
This work was supported by the Natural Science Foundation of China (11475091, 11875171, 11875158, 11675071,12275141), China Postdoctoral Science Foundation (2020M680849), Special Foundation for theoretical physics Research Program of China (12047534), Natural Science Foundation of Tianjin(20JCYBJC01510), the U.S. National Science Foundation (OIA-1738287 and PHY-1913728), the U.S. Department of Energy (DE-SC0005248), and the LSU-LNNU joint research program (9961). The work was carried out at National Supercomputer Center in Tianjin, and the calculations were performed on TianHe-1(A).
References
- Carlson, J.; Schiavilla, R. Structure and dynamics of few-nucleon systems. Rev. Mod. Phys. 1998, 70, 743–841. [CrossRef]
- Pieper, S.C.; Wiringa, R.B. QUANTUM MONTE CARLO CALCULATIONS OF LIGHT NUCLEI. Annu. Rev. Nucl. Part. Sci. 2001, 51, 53–90. [CrossRef]
- Barrett, B.R.; Navratil, P.; Vary, J.P. Ab initio no core shell model. Prog. Part. Nucl. Phys. 2012, 69. [CrossRef]
- Dytrych, T.; Launey, K.D.; Draayer, J.P.; Maris, P.; Vary, J.P.; Saule, E.; Catalyurek, U.; Sosonkina, M.; Langr, D.; Caprio, M.A. Collective Modes in Light Nuclei from First Principles. Phys. Rev. Lett. 2013, 111, 252501. [CrossRef]
- Hagen, G.; Papenbrock, T.; Hjorth-Jensen, M.; Dean, D.J. Coupled-cluster computations of atomic nuclei. Rep. Prog. Phys. 2014, 77, 096302. [CrossRef]
- Bender, M.; Heenen, P.H.; Reinhard, P.G. Self-consistent mean-field models for nuclear structure. Rev. Mod. Phys. 2003, 75, 121–180. [CrossRef]
- Roth, R. Importance truncation for large-scale configuration interaction approaches. Phys. Rev. C 2009, 79, 064324. [CrossRef]
- Bertulani, C. Nuclear Physics in a Nutshell (Princeton university press, Princeton ) 2007, p. 119.
- Talmi, I.; R. Barrett, B. Simple Models of Complex Nuclei: The Shell Model and Interacting Boson Model. Phys. Today 1994, 47, 102–104.
- D. Lawson, R. Theory of the Nuclear Shell Model (Oxford University Press, New York) 1980.
- Otsuka, T.; Honma, M.; Mizusaki, T.; Shimizu, N.; Utsuno, Y. Monte Carlo shell model for atomic nuclei. Prog. Part. Nucl. Phys. 2001, 47, 319–400. [CrossRef]
- Caurier, E.; Martinez-Pinedo, G.; Nowacki, F.; Poves, A.; Zuker, A.P. The shell model as a unified view of nuclear structure. Rev. Mod. Phys. 2005, 77, 427–488. [CrossRef]
- Koonin, S.E.; Dean, D.J.; Langanke, K. Shell model Monte Carlo methods. Phys. Rep. 1996, 278, 1–77. [CrossRef]
- Iachello, F.; Talmi, I. Shell-model foundations of the interacting boson model. Rev. Mod. Phys. 1987, 59, 339–361. [CrossRef]
- Ring, P.; Schuck, P.; Strayer, M.R. The Nuclear Many-Body Problem; 2004.
- Gunye, M.R.; Warke, C.S. Projected Hartree-Fock Spectra of 2s − 1d-Shell Nuclei. Phys. Rev. 1967, 156, 1087–1093. [CrossRef]
- Rodríguez, T.R.; Egido, J.L.; Robledo, L.M.; Rodríguez-Guzmán, R. Quality of the restricted variation after projection method with angular momentum projection. Phys. Rev. C 2005, 71, 044313. [CrossRef]
- Hara, K.; Hayashi, A.; Ring, P. Exact angular momentum projection of cranked Hartree-Fock-Bogoliubov wave functions. Nucl. Phys. A 1982, 385, 14–28. [CrossRef]
- Enami, K.; Tanabe, K.; Yoshinaga, N. Microscopic description of high-spin states: Quantum-number projections of the cranked Hartree-Fock-Bogoliubov self-consistent solution. Phys. Rev. C 1999, 59, 135–153. [CrossRef]
- Sheikh, J.A.; Ring, P. Symmetry-projected Hartree–Fock–Bogoliubov equations. Nucl. Phys. A 2000, 665, 71–91. [CrossRef]
- Yao, J.M.; Meng, J.; Ring, P.; Arteaga, D.P. Three-dimensional angular momentum projection in relativistic mean-field theory. Phys. Rev. C 2009, 79, 044312. [CrossRef]
- Honma, M.; Mizusaki, T.; Otsuka, T. Nuclear Shell Model by the Quantum Monte Carlo Diagonalization Method. Phys. Rev. Lett. 1996, 77, 3315–3318. [CrossRef]
- Abe, T.; Maris, P.; Otsuka, T.; Shimizu, N.; Tsunoda, Y.; Utsuno, Y.; Vary, J.P.; Yoshida, T. Recent development of Monte Carlo shell model and its application to no-core calculations. J. Physics: Conf. Ser. 2013, 454, 012066. [CrossRef]
- HARA, K.; SUN, Y. PROJECTED SHELL MODEL AND HIGH-SPIN SPECTROSCOPY. Int. J. Mod. Phys. E 1995, 04, 637–785, [https://doi.org/10.1142/S0218301395000250]. [CrossRef]
- Sun, Y.; Hara, K. Fortran code of the Projected Shell Model: Feasible shell model calculations for heavy nuclei. Comput. Phys. Commun. 1997, 104, 245–258. [CrossRef]
- Sheikh, J.A.; Hara, K. Triaxial Projected Shell Model Approach. Phys. Rev. Lett. 1999, 82, 3968–3971. [CrossRef]
- Gao, Z.C.; Horoi, M. Angular momentum projected configuration interaction with realistic Hamiltonians. Phys. Rev. C 2009, 79, 014311. [CrossRef]
- Rodríguez-Guzmán, R.; Egido, J.L.; Robledo, L.M. Quadrupole collectivity in N≈28 nuclei with the angular momentum projected generator coordinate method. Phys. Rev. C 2002, 65, 024304. [CrossRef]
- Yao, J.M.; Meng, J.; Ring, P.; Vretenar, D. Configuration mixing of angular-momentum-projected triaxial relativistic mean-field wave functions. Phys. Rev. C 2010, 81, 044311. [CrossRef]
- Chen, J.Q. The Wick theorem for coupled fermion clusters. Nucl. Phys. A 1993, 562, 218 – 240. [CrossRef]
- Jin-Quan, C.; Bing-Qing, C.; Klein, A. Factorization of commutators: The Wick theorem for coupled operators. Nucl. Phys. A 1993, 554, 61 – 76. [CrossRef]
- Chen, J.Q. Nucleon-pair shell model: Formalism and special cases. Nucl. Phys. A 1997, 626, 686 – 714. [CrossRef]
- A, A.; Iachello, F. The Interacting Boson Model; Cambridge University Press, 2001; pp. 139–200.
- Luo, Y.A.; Chen, J.Q.; Draayer, J. Nucleon-pair shell model calculations of the even Ceven Xe and Ba nuclei. Nucl. Phys. A 2000, 669, 101 – 118. [CrossRef]
- Zhao, Y.M.; Yoshinaga, N.; Yamaji, S.; Arima, A. Validity of the SD-pair truncation of the shell model. Phys. Rev. C 2000, 62, 014316. [CrossRef]
- Zhao, Y.; Arima, A. Phys. Rep. 2014, 545, 1 – 45.
- Zhao, Y.M.; Yoshinaga, N.; Yamaji, S.; Arima, A. Validity of the SD-pair truncation of the shell model. Phys. Rev. C 2000, 62, 014316. [CrossRef]
- He, B.C.; Li, L.; Luo, Y.A.; Zhang, Y.; Pan, F.; Draayer, J.P. Nucleon pair shell model in M scheme. Phys. Rev. C 2020, 102, 024304. [CrossRef]
- Lei, Y.; Lu, Y.; Zhao, Y.M. Nucleon-pair approximation with uncoupled representation * 2021. 45, 054103. [CrossRef]
- Fu, G.J.; Lei, Y.; Zhao, Y.M.; Pittel, S.; Arima, A. Nucleon-pair approximation of the shell model with isospin symmetry. Phys. Rev. C 2013, 87, 044310. [CrossRef]
- Fu, G.J.; Shen, J.J.; Zhao, Y.M.; Arima, A. Spin-aligned isoscalar pair correlation in 96Cd, 94Ag, and 92Pd. Phys. Rev. C 2013, 87, 044312. [CrossRef]
- Cheng, Y.Y.; Zhao, Y.M.; Arima, A. Nucleon-pair approximation with particle-hole excitations. Phys. Rev. C 2018, 97, 024303. [CrossRef]
- Meng, X.f.; Wang, F.r.; Luo, Y.a.; Pan, F.; Draayer, J.P. SD-pair shell model study for 126Xe and 128Ba. Phys. Rev. C 2008, 77, 047304. [CrossRef]
- Machleidt, R. High-precision, charge-dependent Bonn nucleon-nucleon potential. Phys. Rev. C 2001, 63, 024001. [CrossRef]
- Bogner, S.; Kuo, T.T.S.; Coraggio, L.; Covello, A.; Itaco, N. Low momentum nucleon-nucleon potential and shell model effective interactions. Phys. Rev. C 2002, 65, 051301. [CrossRef]
- Hjorth-Jensen, M.; Kuo, T.T.; Osnes, E. Realistic effective interactions for nuclear systems. Phys. Rep. 1995, 261, 125–270. [CrossRef]
- Coraggio, L.; Covello, A.; Gargano, A.; Itaco, N.; Kuo, T. Shell-model calculations and realistic effective interactions. Prog. Part. Nucl. Phys. 2009, 62, 135–182. [CrossRef]
- Coraggio, L.; Covello, A.; Gargano, A.; Itaco, N.; Kuo, T. Effective shell-model hamiltonians from realistic nucleon–nucleon potentials within a perturbative approach. Ann. Phys. 2012, 327, 2125–2151. [CrossRef]
- Shimizu, N.; Mizusaki, T.; Utsuno, Y.; Tsunoda, Y. Thick-restart block Lanczos method for large-scale shell-model calculations. Comput. Phys. Commun. 2019, 244, 372–384. [CrossRef]
- Brown, B.A.; Richter, W.A. New “USD” Hamiltonians for the sd shell. Phys. Rev. C 2006, 74, 034315. [CrossRef]
Figure 1.
The dimension of configuration space for 3 identical pairs against the types of collective pairs are presented. The spherical basis in M-scheme and the axial-deformed basis are shown. The collective pair S, D, G, I, K and M are the pairs of angular momentum J = 0, 2, 4, 6, 8 and 10, respectively.
Figure 1.
The dimension of configuration space for 3 identical pairs against the types of collective pairs are presented. The spherical basis in M-scheme and the axial-deformed basis are shown. The collective pair S, D, G, I, K and M are the pairs of angular momentum J = 0, 2, 4, 6, 8 and 10, respectively.
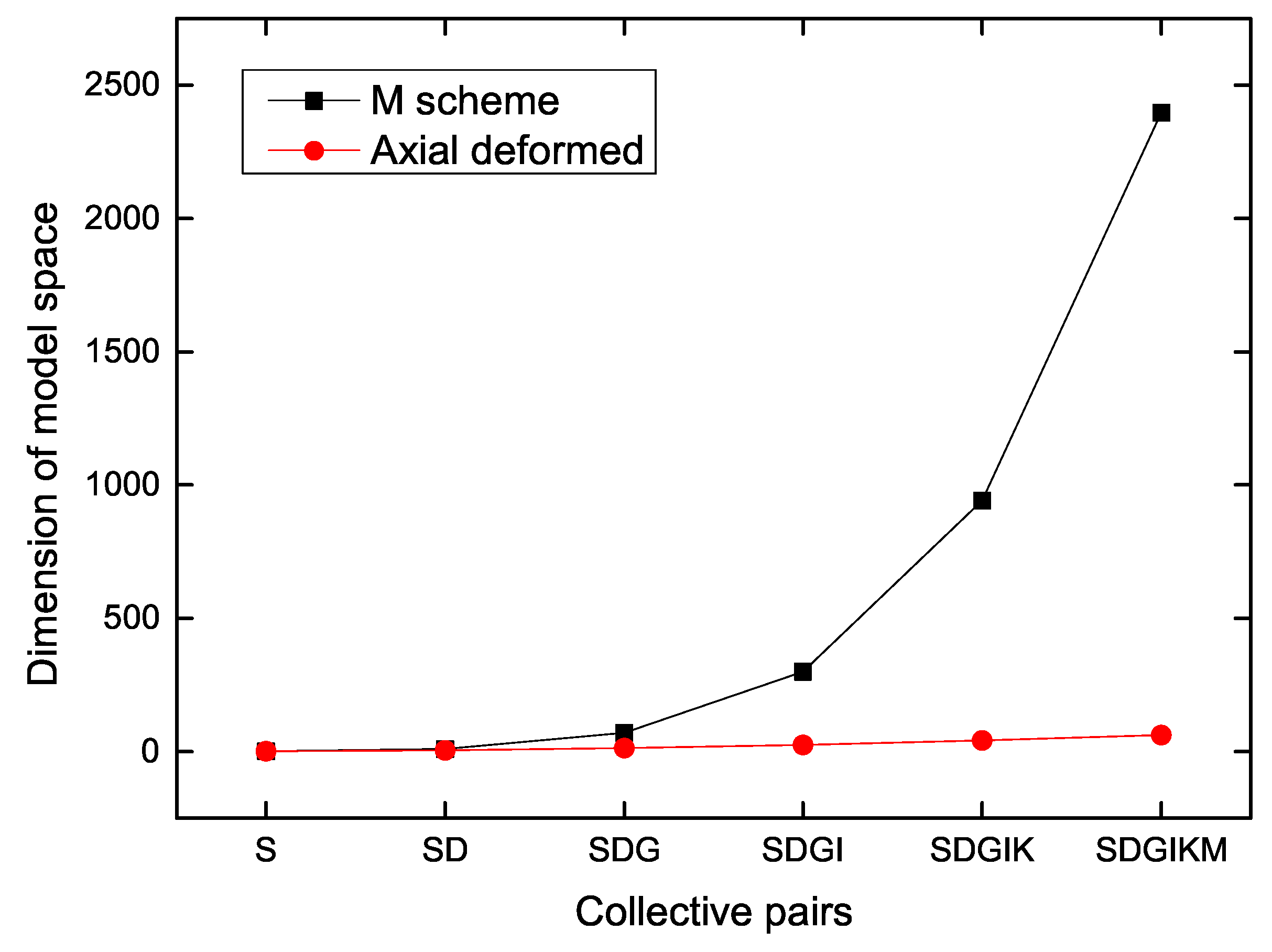
Figure 2.
The low-lying spectrum of 138Sn are studied in the shell model and NPSM with axial-deformed basis.
Figure 2.
The low-lying spectrum of 138Sn are studied in the shell model and NPSM with axial-deformed basis.
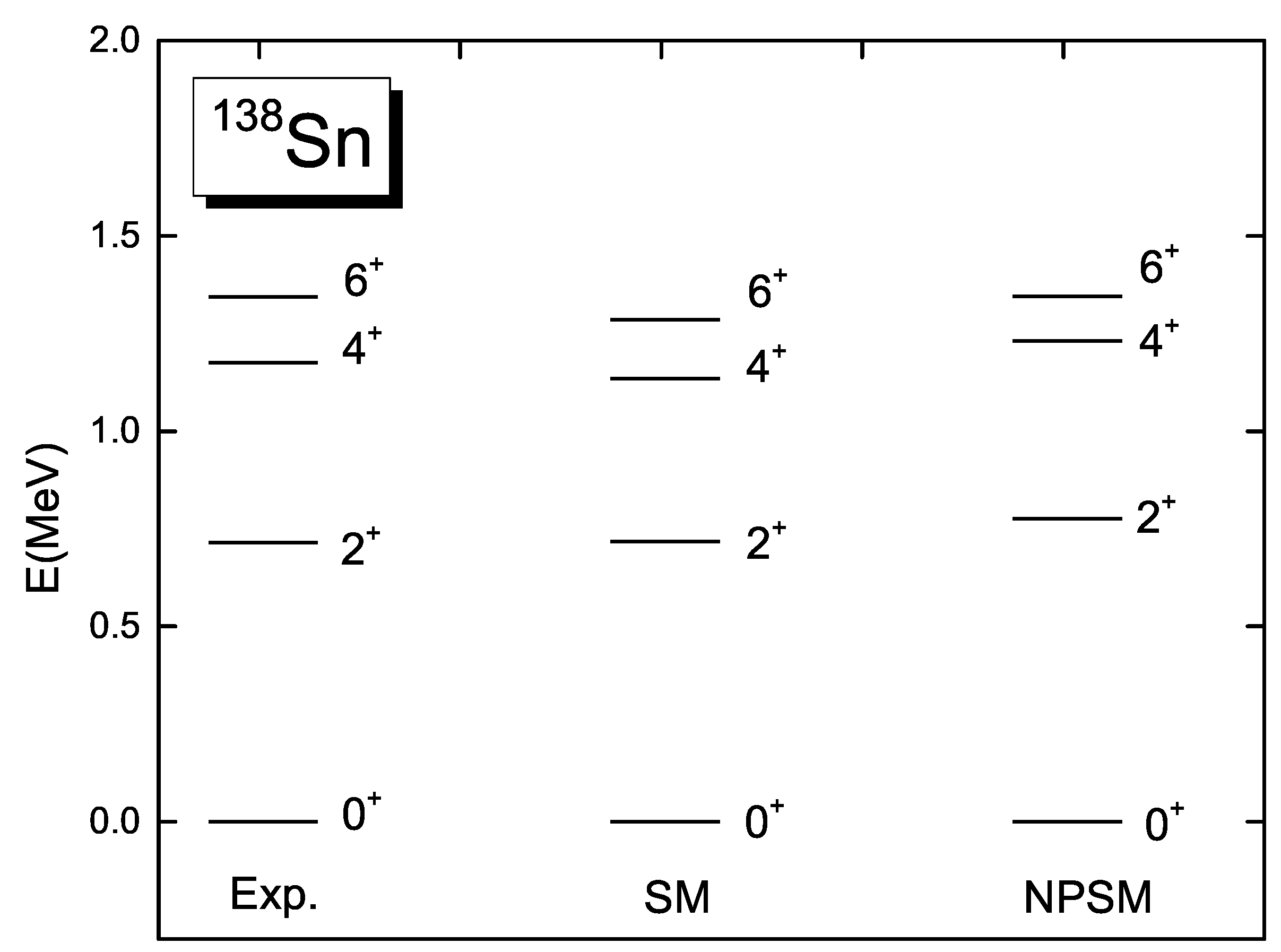
Figure 3.
The low-lying spectrum of 20O are studied in the shell model and NPSM with triaxial-deformed basis.
Figure 3.
The low-lying spectrum of 20O are studied in the shell model and NPSM with triaxial-deformed basis.
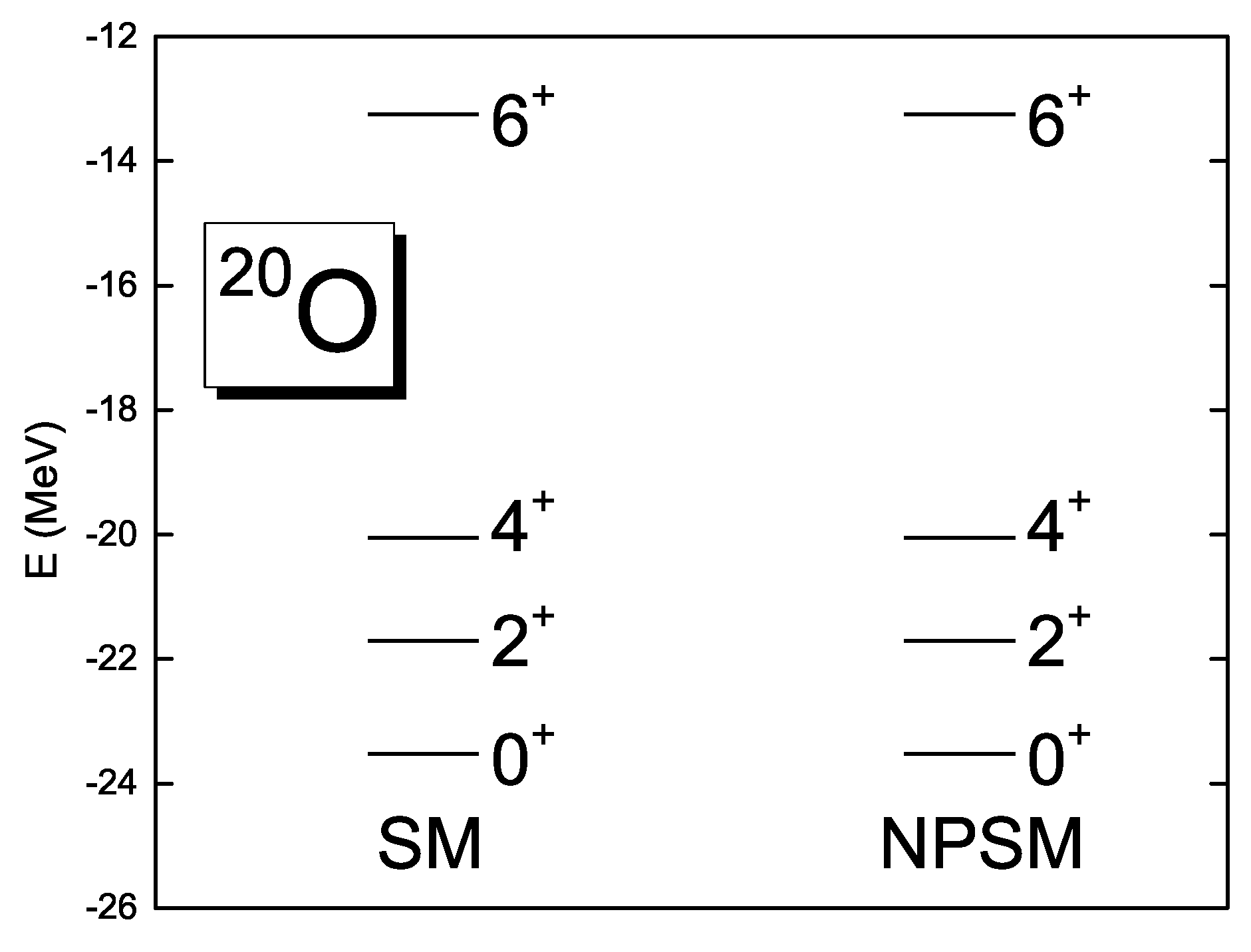
Figure 4.
The levels with J=2 and J=4 of 20O are calculated in the SM and NPSM with triaxial-deformed basis.
Figure 4.
The levels with J=2 and J=4 of 20O are calculated in the SM and NPSM with triaxial-deformed basis.
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Copyright: This open access article is published under a Creative Commons CC BY 4.0 license, which permit the free download, distribution, and reuse, provided that the author and preprint are cited in any reuse.
MDPI Initiatives
Important Links
© 2024 MDPI (Basel, Switzerland) unless otherwise stated





