Prerpints.org logo
Preprint
Article

Synergistic Effects of LSPR, SPP, and Waveguide Plasmonic Resonance on the Same Material: A Promising Hypothesis to Enhanced Organic Solar Cells Efficiency

Altmetrics

Downloads

171

Views

42

Comments

0

A peer-reviewed article of this preprint also exists.

Submitted:

27 June 2023

Posted:

28 June 2023

You are already at the latest version

Alerts
Abstract
In this work we explore the utilization of plasmonic resonance (PR) in silver nanowires to enhance the performance of organic solar cells. Plasmonic resonance is a phenomenon in which nanoscale conductive materials exhibit oscillation of conduction electrons, resulting in the creation of an electric field. Enhancing light absorption is crucial for improving organic solar cell efficiency and incorporating metallic nanostructures to induce surface plasmon resonance (SPR) shows promise in achieving this goal. We discuss the two key mechanisms of plasmonic effects: far-field scattering and near-field resonance modes. Far-field scattering extends the optical path of incident light, while near-field plasmonic effects involve localized surface plasmon resonance (LSPR) and plasmonic cavity modes, enhancing absorption by strengthening electric fields near the nanostructures. Silver nanowires are the focus of this study, and finite-difference time-domain (FDTD) simulation software is used to investigate their plasmonic resonance behavior in a ZnO/Silver nanowires/ZnO (ZAZ) electrode structure. The simulations reveal the dominance of LSPR in this configuration, with intense electric fields inside the nanowire and propagation into the surrounding medium, offering opportunities for enhanced light absorption in the organic solar cell's active layer.
Keywords: 
Subject: Physical Sciences  -   Optics and Photonics
University of Limoges, Centre National de la Recherche Scientifique, XLIM, UMR 7252, F-87000 Limoges, France; issoufou.ibrahim@etu.unilim.fr (I.I.Z.); bruno.lucas@unilim.fr (B.L.); sylvain.vedraine@unilim.fr (S.V.)

1. Introduction

The interaction of light with certain nanoscale conductive materials can induce a well-known phenomenon in the literature referred to a plasmonic resonance (PR). At a specific wavelength for each material with enough available electron, an electric field is created due to the oscillation of conduction electrons around the atoms of the material. The collective oscillation mode of these electrons is called surface plasmons, and the nanomaterials that support surface plasmons are referred to as plasmonic materials [1,2]. This phenomenon is widely recognized in the fields of physics, chemistry, and materials science due to its diverse range of potential applications, including optical sensing, light generation, solar cells, biomedicine, and electronics. With its growing popularity, SPR has become a significant research topic aimed at understanding its properties and exploring its potential applications. The discovery of the SPR phenomenon dates back over a century to Wood [3]. Wood observed anomalous reflected structures when polarized light was projected onto a metalized diffraction grating [3]. Lord Rayleigh [4] attempted to elucidate the phenomenon a few years later (in 1907). The physical interpretation was not resolved until 1968 by Otto [5]. Also in 1968, Kretschmann and Raether [6] described the excitation of surface plasmons (SP) using a specific optical setup. The specificity of this excitation is the presence of a plasmon at the interface of the material with its surrounding media, conversely to the volume plasmon resonance located only inside the material.
In organic photovoltaic (OPV) devices, the relatively low mobility of charge carriers (around 10-4 cm2.V-1.s-1) and the short diffusion length in most organic semiconductor materials [7] necessitate the use of thin active layers, typically around 100 nm or less, to enhance charge diffusion and extraction [8]. However, this thin active layer leads to poor absorption of incident light, resulting in significant energy loss of photons that could otherwise be converted into electrical energy. Extensive research has therefore been conducted to improve light absorption in OPVs. Multiple studies have demonstrated that surface plasmon resonance (SPR) can be introduced in OPVs through the incorporation of metallic nanostructures, without varying the thickness of the active layer. This introduction of SPR amplifies light collection in the active layer, thereby enhancing photovoltaic performance [9,10,11,12,13,14]. In fact, the absorption of the active layer is directly proportional to the square of the electric field, as described by the following equation [15]:
Q z , λ = α λ n i n 0 E z 2 E 0 2
Here, Q(z, λ) represents the absorption as a function of the position (z) within the device stack, wavelength (λ), α(λ) denotes the absorption coefficient, ni and n0 are the refractive indexes of the i layer and the surrounding medium, and E(z) and E0 represent the electric fields at position z and the incident electric field, respectively.
This equation establishes a direct relationship between the absorption of the active layer and the electric field resulting from surface plasmon resonance. However, it should be noted that the propagation of this electric field is limited in terms of distance, as further discussed in this investigation. By enhancing absorption, the generation of electrons can be improved, consequently enhancing the external quantum efficiency. Alternatively, achieving similar absorption with a thinner active layer can mitigate the challenges associated with charge diffusion length commonly observed in organic solar cells. Furthermore, this effect can be exploited in organic solar cells with a thick active layer for better efficiency [13].

1.1. Far-field plasmonic effects: scattering

Far-field scattering results in the optical path of incident light being lengthened, which can enhance photon absorption. The size of metal nanoparticles that best matches the far-field scattering effect is generally greater than 30 nm [16].
For plasmonic nanostructures embedded in layers at the interface of transparent electrodes (i.e. HTL (hole transport layer) in conventional-type solar cells or ETL (electron transport layer) in inverted-type cells), forward scattering is required. The geometry and interface of plasmonic nanostructures can be optimized to minimize surface reflection. In addition, the optical paths of incident sunlight in the absorbing medium and their interaction time are thus increased, resulting in improved absorption efficiency of the active layer [17].
The direction of scattering can be adjusted by controlling the size and geometry of the plasmonic nanostructures. Starting with nanoparticles around 50 nm in diameter, photons are scattered forwards and backwards in comparable quantities [18]. As particle size increases, more radiation is scattered backwards.
Nanoparticles (NPs) whose size is below the above-mentioned intermediate range exhibit low scattering, which induces insufficient optical absorption in the active layer linked to this component. In addition, the conductivity of the device is negatively affected as more charge-trapping sites are formed at the interfaces of the NPs due to their large surface-to-volume ratio. For plasmonic nanostructures integrated outside the active layer, these losses cancel out the possible advantages of small NPs (e.g. ~20 nm), which can accentuate the coupling of the plasmonic field close to the active layer [19].

1.2. Near-field plasmonic effects

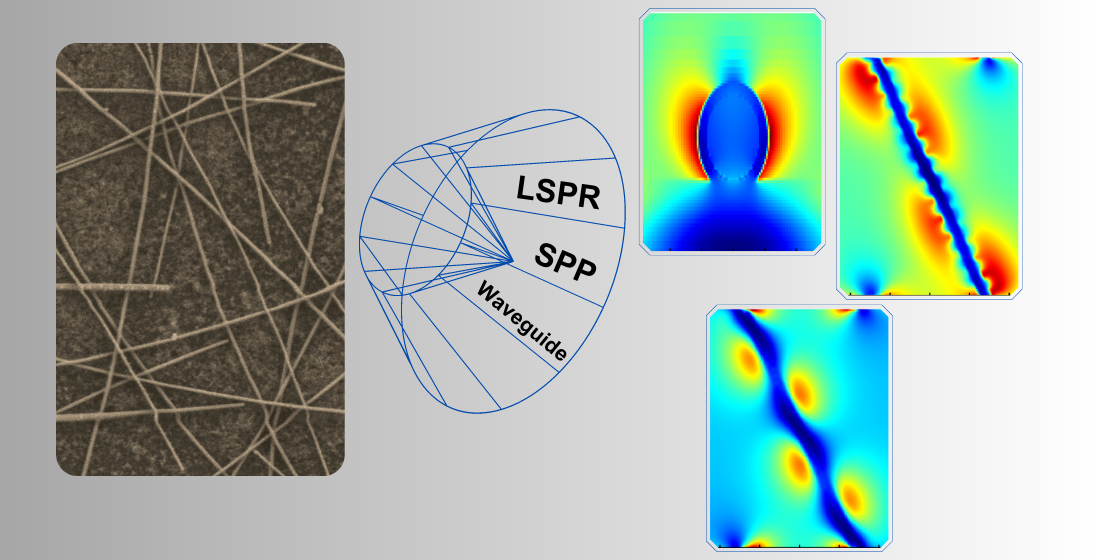
There are four modes of plasmonic resonance (Figure 1), which can be classified into two groups: propagative and non-propagative modes.

1.2.1. Non-propagating modes

Localised Surface Plasmon Resonance (LSPR)
When a surface plasmon is confined to an NP of a size smaller than the wavelength of the incident light, the free electrons of the NP participate in the collective oscillation, which is called localized surface plasmon resonance (LSPR, Figure 1a). The LSP has two important effects. Firstly, the electric fields near the particle surface are strongly strengthened, this strengthening being greatest at the surface and decreasing rapidly with distance [20]. Secondly, the particle's optical radiation exhibits a maximum at the plasmon's resonance frequency. The resonance frequency depends on the geometry of the metal nanostructures. Consequently, appropriate adaptation of nanoparticle size and morphology is an effective approach to controlling the resulting plasmon electric field radiation to meet specific demands. For noble metal nanoparticles, resonance typically occurs in the visible and near-infrared wavelength region [24,25]. LSPR often occurs when the surfaces of plasmonic nanostructures are textured [26].
NPs smaller than 30 nm act as sub-wavelength antennas during LSPR excitation. In such cases, the absorption enhancement comes mainly from the LSPR effect rather than the scattering effect [27]. The near field plasmon induced in the active layer increases the absorption cross-section and thus effectively enhances photon absorption.
Plasmonic cavity mode
When specific metal-dielectric configurations are built, the spectral wavelength ranges for absorption enhancement can be extended further due to plasmonic coupling modes. For example, a metal-dielectric-metal (MDM) structure is obtained when the active layer is sandwiched between the metal electrode and the metal NPs layer. By appropriately selecting NP geometries, surface plasmon stationary waves can be generated due to constructive interference in the finite cavity, reducing light leakage from surface plasmon generation in the lateral gaps between NPs. Thus, the plasmon-cavity mode is excited in the MDM and a strong electromagnetic field is confined to the light-absorbing layer. Interestingly, the field enhancement from the plasmon-cavity mode (Figure 1b) is independent of polarization or incident light angles [28].

1.2.2. Propagating modes

Surface plasmon polariton (SPP)
Unlike LSPR, surface plasmon polariton (SPP, also known as SPR [29]) often occurs on or near a planar metal (Figure 1c). The SPP is an electromagnetic wave at infrared or visible frequencies that propagates undulatory along the metal-dielectric interface [30,31,32]. The charge movements of the SPP involve contributions from both the metal and the dielectric. Perpendicular to the interface, the spatial confinement of SPPs lies in a sub-wavelength scale range, resulting in a more intense electromagnetic field. The amplitude of SPP waves decreases exponentially with increasing distance in each medium from the interface. An SPP can propagate along the interface for 10-100 µm until its energy is consumed by absorption or scattering [33,34]. This increase in optical path length with lateral propagation significantly enhances absorption.
The photonic waveguide mode
In addition to the SPP mode, a further mode type can be excited at planar metal/dielectric interfaces during incident radiation, which also contributes to absorption enhancement. It's called the "waveguide mode" because of its similarity to the propagation model in dielectric waveguides. Along its lateral propagation, part of the waveguide mode can be absorbed by organic semiconductors, in which excitons are generated [35,36].
Excitation of the waveguide mode is independent of the polarization of the incident irradiation. This means that waveguide modes can be generated irrespective of whether the polarization direction of the excitation is parallel or orthogonal to the directions of the metal nanostructures [37].
Given the architecture of the device and the structure of the metal nanostructures to be used, some modes will be much more favored than others. In our case, we are using metal nanowires that have a cylindrical shape with a diameter of 35 nm and a length of 25 µm based on our previous investigation showing an experimental increase of the efficiency of an organic solar cells despite its thick active layer [13]. Later in this article, we'll look at the modes that are predominant in this type of structure.
In this work, we will first explore the concept of plasmonic resonance, followed by the study of plasmonic resonance in silver nanowires using Finite-Difference Time Domain (FDTD) simulation software, in which a ZnO/AgNWs/ZnO (ZAZ) electrode model will be constructed with the different materials used. We will observe the spatial distribution of the electric field as a function of wavelength and the type of plasmonic resonance.

2. Methods

The ZAZ electrode was modeled according to the structure described in Figure 2a We modelized a nanowire made with silver and surrounded with an interface layer of a solar cells made with ZnO. We modeled the substrate with silica glass (SiO2). The optical indexes used to model ZnO, and silver nanowires (AgNWs) were taken from references [38] and [39] respectively. Figure 2b–d show the real (n) and imaginary (k) refractive indexes of SiO2, ZnO and AgNWs respectively. SiO2 is characterized by an almost constant real optical index equal to 1.46 and zero imaginary part in the visible spectrum. All the materials used in this configuration have been chosen to ensure the photons pass through to reach the active layer which can be further place instead or Air, onto the ZnO surrounding layer. As a result, they are all transparent to visible light except for AgNWs, which individually reflect visible light, but when deposited to form a ZAZ electrode, they exhibit high transmission [13].
All our simulations are performed in three dimensions. The simulation area is delimited by PML (Perfect Matched Layer) boundary conditions along the z-axis, Periodic along the x-axis and Bloch along the y-axis. The spatial mesh size is 0.25 nm minimum, and the time mesh size is 3.36×10-18 s. The surrounding medium is air, and the illumination source consists of two polychromatic plane waves polarized at 90° to each other, the aim being to obtain unpolarized illumination. Various monitors were introduced into the simulation to observe the transmission and spatial distribution of the electric field around the AgNWs.

3. Result and discussion

3.1. ZAZ electrode modeling with one silver nanowire

In this section, we investigate plasmon resonance around a single silver nanowire in order to observe the behavior of the electric field as a function of the wavelength. The diameter of the silver nanowires is 35 nm, which corresponds approximately to the diameter of the AgNWs used experimentally [13]. The lower layer of ZnO nanoparticles (20 nm) is located below the AgNW and the upper layer is modeled by the ring around the AgNW with a diameter greater than 45 nm (Figure 3a).
Figure 3a shows the absorption of the ZAZ electrode, with an absorption peak at around 347 nm (typical resonance of silver nanosphere); the resulting electric field is shown in Figure 3b. The localized field inside the AgNW is intense due to the absorption of the nanowire at the chosen wavelength. A high electric field intensity is noted at the top of AgNW/ZnO interface, given the difference in permittivity between the two materials, but a low intensity on the edges. Its radiation extends over a length of around 30 nm from the ZnO interface and spreads out into the air. The ZnO layer seems to favor plasmon propagation in air, as the electric field is stronger there than in ZnO. This effect is particularly interesting and will enable us to take advantage of this surface plasmon in the active layer that will fill this space.
The LSPR is the privileged plasmonic resonance mode in this configuration. A multi-wavelengths investigation (Figure 4) represent the electric field distributed around the cross-section of the silver nanowire. At 302 nm, the electric field takes the form of two lobes positioned on either side of the silver nanowire directed towards the air, under the influence of the substrate. These lobes show a strong presence of scattering compared with plasmon resonance. This radiation is very similar to that observed with a metal sphere, as demonstrated in several research studies [41]. Although this electric field is localized around the AgNW, its amplitude is very low at this wavelength. At 316 nm, the electric field reaches greater distances vertically, but its intensity is still very low. Part of the electric field starts to be absorbed by the AgNW.
At 347 nm, corresponding to the AgNW absorption peak, a significant portion of the electric field remains confined within the AgNW, with a high concentration at the AgNW/ZnO interface, as mentioned in the paragraph above. Absorption inside the nanowires is limited after 387 nm which make possible for the most part of the light to be absorbed. Radiation is almost uniform around the AgNW, with intensities low but higher than those observed before the absorption wavelength. There is a strong localization of the field, which means a high proportion of plasmonic resonance.
Beyond 347 nm, we see a significant increase in the electric field up to 608 nm from which lobes due the scattering tends to be majority. At 416 nm, we find the maximum electric field intensity (311 V2.m-2). The electric field distribution changes shape as a function of wavelength. This is due to the interaction of light with silver being strongly related to wavelength. For wavelengths between 347 and 387 nm, the field distribution is almost uniform around the AgNWs. However, for wavelengths above 387 nm, we note an increasingly localized distribution towards the lower section of the AgNW. High-intensity hotspots at the AgNW/ZnO interface reflect very intense local confinement of the electric field, at certain wavelengths.
All these electric field exaltation phenomena contribute to increasing the absorption of the active layer of an organic solar cell over a wide wavelength spectrum, as long as the field is present beyond the ZnO.
We have just seen the behavior of the electric field on the cross-section of an AgNW and the associated plasmonic resonance mode. We will now investigate the distribution of the electric field over the longitudinal section of the AgNW.
Figure 5 shows the longitudinal distribution of the electric field. Below the absorption wavelength, the electric field intensity is very low, as we noticed with the LSPR mode. At the absorption peak (347 nm), the electric field is confined solely to the AgNW and its surroundings.
In the following section, we develop a hypothesis to explain the interpretation of the electric field distribution along the silver nanowire.
At 450 nm, we observe a wave propagating along the AgNW with high intensity. We assume that this is the waveguide mode. This mode appears at the metal-dielectric interface (Figure 1d) and is noted in our structures with cylindrical geometry. This also explains why we observe a strong confinement of the electric field in the lower section of the AgNWs when we observe its cross-section (Figure 7f). At 520 nm, this mode continues to propagate with a different frequency and a weaker but uniformly distributed intensity. We obtain the same behavior for the wavelength at 550 nm.
From 630 nm onwards, a second oscillation superposed to the first oscillation of the electric field appears around the AgNW, with smaller wavelengths superposed on the waveguide mode. This is an SPP mode (Figure 5), as the waveform (visible at 637 nm) corresponds to the propagation of the SPP mode as illustrated in Figure 1c Furthermore, the AgNWs are coated and in contact with the ZnO, which can be likened to the Otto configuration, as discussed by Liqun Sun group [42], enabling the attenuated total reflection (ATR) condition to optically excite the SPP mode in very restricted positions. Since the propagation and localized modes (waveguide, LSPR and SPP) are superposed, it is complex to distinguish the single contribution of each mode. However, we noted a positive impact of these modes, as areas of low electric field intensity, when the waveguide mode is propagating alone, present a high intensity when the SPP mode is present.
The waveguide mode continues to propagate up to 800 nm, with increasing wavelengths as it moves away from the absorption peak.
Figure 6 shows the behavior of the electric field as a function of the distance between the AgNW/ZnO interface and the monitor. By varying the position of the monitor up to 40 nm, we notice a certain increase in the electric field, which reaches its maximum value at around 20 nm above the interface. This seems to be in line with our hypothesis concerning the propagation of SPP and waveguide modes, since, in accordance with the Otto configuration, the distance between the dielectric and the metal is a few tens of nanometers; in our case, this distance is 20 nm.
To sum up, we have demonstrated that for a silver nanowire, several plasmonic resonance modes can take place. Each of these modes can have its own peak in electric field intensity, with the LSPR mode peaking at 416 nm and the waveguide mode at 450 nm.

3.2. Modeling a ZAZ electrode with multiple silver nanowires

The aim of this numerical study is to propose a numerical model of the ZAZ electrode that approximates that of the experimental one, in order to demonstrate plasmon resonance. To this end, we have used a script to generate a semi-random array of ZnO-coated silver nanowires.
The numerical model structure closest to the filling factor of an experimental ZAZ electrode is that with five AgNWs. The electric field is maximum at 454 nm, close to the peak of the waveguide mode.
Preprints 77836 g007
Figure 7. Normalized sqared electric field distribution for a wavelength of 454 nm and a ZAZ electrode composed of: (a) one AgNW; (b) two AgNWs; (c) three AgNWs; (d) four AgNWs; (e) 5 AgNWs.; (f) Normalized squared electric field distribution on the cross-section of an AgNW. The wavelength at which the field is observed is 454 nm, with the monitor at 10 nm above the AgNWs.
Figure 7. Normalized sqared electric field distribution for a wavelength of 454 nm and a ZAZ electrode composed of: (a) one AgNW; (b) two AgNWs; (c) three AgNWs; (d) four AgNWs; (e) 5 AgNWs.; (f) Normalized squared electric field distribution on the cross-section of an AgNW. The wavelength at which the field is observed is 454 nm, with the monitor at 10 nm above the AgNWs.
Preprints 77836 g007
The two modes of plasmon propagation (SPP and waveguide) are also present for several AgNWs through the ondulation of the electric field along the wires. For example, in Figure 7b we show both propagation modes, with the slightly vertical nanowire showing waveguide mode propagation and the slightly horizontal nanowire showing the superposition of both propagation modes. As we saw before, the waveguide mode is independent of polarization and angle of incidence, which is one of the reasons why we can see it on AgNWs regardless of wavelength.
In Figure 7c,d. and e., the electric field propagation around the silver nanowires differs at each AgNW/AgNW intersection. At some intersections, we see areas of low electric intensity, while at others, we see areas of very high intensity. This is due to the interference of electrical waves from plasmon resonance. At intersections with low electric field intensity, interference with waves from different AgNWs is destructive, whereas at intersections with high electric field intensity, interference is constructive.
Figure 7f illustrates the electric field distribution associated with the LSPR mode. A high electric field intensity is located on either side of the AgNW, as discussed above. This mode is clearly perceptible at the end of the AgNWs, where a strong confinement of the electric field is observed (Figure 7d,e).
The simultaneous existence of these different modes of plasmon propagation is very interesting for organic solar cells, as it would allow us to increase their absorption without increasing the thickness of the active layer.

4. Conclusion

In this work, we discussed the concept of plasmon resonance. There are four main modes: LSPR, SPP, waveguide and photonic cavity. These modes can be classified as propagative and non-propagative. SPP and waveguide are classified as propagative modes, while LSPR and photonic cavity are classified as non-propagative modes. Considering the ZAZ electrode configuration, the photonic cavity mode cannot be excited due to the geometry of the AgNWs used.
To observe the electric field distribution resulting from plasmon resonance, we used the FDTD method. This enabled us to visualize the spatial distribution of the electric field across and along the cross-section of an AgNW. Excitation of the LSPR mode is evident, with significant confinement of the electric field around the cross-section of the AgNW and mainly located outside the ZnO layer. The last present a role of spacer. The distribution of the electric field along the AgNWs revealed the presence of different waves at certain wavelengths. We hypothesized that a superposition of the SPP and waveguide modes is the cause of the electric field fluctuations observed at certain wavelengths.
All these mechanisms related to plasmon resonance are of major interest for integration into organic solar cells to enhance their photovoltaic performance.

References

  1. G. V. Naik, V. M. Shalaev, et A. Boltasseva, « Alternative Plasmonic Materials: Beyond Gold and Silver », Advanced Materials, vol. 25, no 24, p. 3264-3294, 2013. [CrossRef]
  2. A. Boltasseva et H. A. Atwater, « Materials science. Low-loss plasmonic metamaterials », Science, vol. 331, no 6015, p. 290-291, janv. 2011. [CrossRef]
  3. R. W. Wood, « XLII. On a remarkable case of uneven distribution of light in a diffraction grating spectrum », The London, Edinburgh, and Dublin Philosophical Magazine and Journal of Science, vol. 4, no 21, p. 396-402, sept. 1902. [CrossRef]
  4. Lord Rayleigh, « III. Note on the remarkable case of diffraction spectra described by Prof. Wood », The London, Edinburgh, and Dublin Philosophical Magazine and Journal of Science, vol. 14, no 79, p. 60-65, juill. 1907. [CrossRef]
  5. A. Otto, « Excitation of nonradiative surface plasma waves in silver by the method of frustrated total reflection », Z. Physik, vol. 216, no 4, p. 398-410, août 1968. [CrossRef]
  6. E. KRETSCHMANN et H. RAETHER, « Radiative Decay of Non Radiative Surface Plasmons Excited by Light », Z. Naturforsch., A: Astrophys., Phys. Phys. Chem., vol. 23, no 2135-2136, nov. 1968. [CrossRef]
  7. A. C. Mayer, S. R. Scully, B. E. Hardin, M. W. Rowell, et M. D. McGehee, « Polymer-based solar cells », Materials Today, vol. 10, no 11, p. 28-33, nov. 2007. [CrossRef]
  8. S. M. Falke et al., « Coherent ultrafast charge transfer in an organic photovoltaic blend », Science, vol. 344, no 6187, p. 1001-1005, mai 2014. [CrossRef]
  9. S. Vedraine et al., « Intrinsic absorption of plasmonic structures for organic solar cells », Solar Energy Materials and Solar Cells, vol. 95, p. S57-S64, mai 2011. [CrossRef]
  10. K. N’Konou et al., « Impact of Ag@SiO2 core-shell nanoparticles on the photoelectric current of plasmonic inverted organic solar cells », Synthetic Metals, vol. 239, p. 22-28, mai 2018. [CrossRef]
  11. H. I. Park et al., « High Performance Organic Photovoltaics with Plasmonic-Coupled Metal Nanoparticle Clusters », ACS Nano, vol. 8, no 10, p. 10305-10312, oct. 2014. [CrossRef]
  12. D. H. Wang et al., « Enhancement of Donor-Acceptor Polymer Bulk Heterojunction Solar Cell Power Conversion Efficiencies by Addition of Au Nanoparticles », Angew. Chem. Int. Ed., vol. 50, no 24, p. 5519-5523, juin 2011. [CrossRef]
  13. I. Ibrahim Zamkoye, J. Bouclé, N. Leclerc, B. Lucas, et S. Vedraine, « Silver Nanowire Electrodes Integrated in Organic Solar Cells with Thick Active Layer Based on a Low-Cost Donor Polymer », Solar RRL, vol. 7, no 2, p. 2200756, 2023. [CrossRef]
  14. M. Chalh, S. Vedraine, B. Lucas, et B. Ratier, « Plasmonic Ag nanowire network embedded in zinc oxide nanoparticles for inverted organic solar cells electrode », Solar Energy Materials and Solar Cells, vol. 152, p. 34-41, août 2016. [CrossRef]
  15. F. Monestier et al., « Modeling the short-circuit current density of polymer solar cells based on P3HT:PCBM blend », Solar Energy Materials and Solar Cells, vol. 91, no 5, p. 405-410, mars 2007. [CrossRef]
  16. S.-W. Baek, J. Noh, C.-H. Lee, B. Kim, M.-K. Seo, et J.-Y. Lee, « Plasmonic Forward Scattering Effect in Organic Solar Cells: A Powerful Optical Engineering Method », Sci Rep, vol. 3, no 1, p. 1726, déc. 2013. [CrossRef]
  17. M. Salvador et al., « Electron Accumulation on Metal Nanoparticles in Plasmon-Enhanced Organic Solar Cells », ACS Nano, vol. 6, no 11, p. 10024-10032, nov. 2012. [CrossRef]
  18. S. Mokkapati, F. J. Beck, R. de Waele, A. Polman, et K. R. Catchpole, « Resonant nano-antennas for light trapping in plasmonic solar cells », J. Phys. D: Appl. Phys., vol. 44, no 18, p. 185101, avr. 2011. [CrossRef]
  19. Q. Gan, F. J. Bartoli, et Z. H. Kafafi, « Plasmonic-Enhanced Organic Photovoltaics: Breaking the 10% Efficiency Barrier », Advanced Materials, vol. 25, no 17, p. 2385-2396, 2013. [CrossRef]
  20. J.-F. Masson, « Portable and field-deployed surface plasmon resonance and plasmonic sensors », Analyst, vol. 145, no 11, p. 3776-3800, juin 2020. [CrossRef]
  21. K. Jung et al., « Plasmonic Organic Solar Cells Employing Nanobump Assembly via Aerosol-Derived Nanoparticles », ACS Nano, vol. 8, no 3, p. 2590-2601, mars 2014. [CrossRef]
  22. G. Das et al., « Plasmonic nanostructures for the ultrasensitive detection of biomolecules », vol. 39, p. 547-586, nov. 2016. [CrossRef]
  23. « The Plasmonic Insulator-Metal-Insulator as a Two-level System - SPP7 Abstract Submission Form ». https://program.eventact.com/Agenda/Lecture/90825?code=1638046 (consulté le 24 juin 2023).
  24. B. Sharma, R. R. Frontiera, A.-I. Henry, E. Ringe, et R. P. Van Duyne, « SERS: Materials, applications, and the future », Materials Today, vol. 15, no 1, p. 16-25, janv. 2012. [CrossRef]
  25. E. L. Lim, C. C. Yap, M. A. Mat Teridi, C. H. Teh, A. R. bin Mohd Yusoff, et M. H. Hj Jumali, « A review of recent plasmonic nanoparticles incorporated P3HT: PCBM organic thin film solar cells », Organic Electronics, vol. 36, p. 12-28, sept. 2016. [CrossRef]
  26. Y. H. Jang, Y. J. Jang, S. Kim, L. N. Quan, K. Chung, et D. H. Kim, « Plasmonic Solar Cells: From Rational Design to Mechanism Overview », Chem. Rev., vol. 116, no 24, p. 14982-15034, déc. 2016. [CrossRef]
  27. A. J. Morfa, K. L. Rowlen, T. H. Reilly, M. J. Romero, et J. van de Lagemaat, « Plasmon-enhanced solar energy conversion in organic bulk heterojunction photovoltaics », Appl. Phys. Lett., vol. 92, no 1, p. 013504, janv. 2008. [CrossRef]
  28. A. Moreau et al., « Controlled-reflectance surfaces with film-coupled colloidal nanoantennas », Nature, vol. 492, no 7427, Art. no 7427, déc. 2012. [CrossRef]
  29. H. Kallmann et M. Pope, « Photovoltaic Effect in Organic Crystals », J. Chem. Phys., vol. 30, no 2, p. 585-586, févr. 1959. [CrossRef]
  30. H. Raether, « Surface Plasmons on Smooth and Rough Surfaces and on Gratings », Springer Tracts in Modern Physics, vol. 111, janv. 1988. [CrossRef]
  31. X. H. Li, W. E. I. Sha, W. C. H. Choy, D. D. S. Fung, et F. X. Xie, « Efficient Inverted Polymer Solar Cells with Directly Patterned Active Layer and Silver Back Grating », J. Phys. Chem. C, vol. 116, no 12, p. 7200-7206, mars 2012. [CrossRef]
  32. L. Zhou et al., « Light Extraction of Trapped Optical Modes in Polymer Light-Emitting Diodes with Nanoimprinted Double-Pattern Gratings », ACS Appl. Mater. Interfaces, vol. 6, no 20, p. 18139-18146, oct. 2014. [CrossRef]
  33. I. Suárez et al., « Propagation length enhancement of surface plasmon polaritons in gold nano-/micro-waveguides by the interference with photonic modes in the surrounding active dielectrics », Nanophotonics, vol. 6, no 5, p. 1109-1120, sept. 2017. [CrossRef]
  34. T. Iqbal, « Propagation length of surface plasmon polaritons excited by a 1D plasmonic grating », Current Applied Physics, vol. 15, no 11, p. 1445-1452, nov. 2015. [CrossRef]
  35. S. Ahn, D. Rourke, et W. Park, « Plasmonic nanostructures for organic photovoltaic devices », J. Opt., vol. 18, no 3, p. 033001, févr. 2016. [CrossRef]
  36. J. B. Lassiter et al., « Plasmonic Waveguide Modes of Film-Coupled Metallic Nanocubes », Nano Lett., vol. 13, no 12, p. 5866-5872, déc. 2013. [CrossRef]
  37. C.-H. Chou et F.-C. Chen, « Plasmonic nanostructures for light trapping in organic photovoltaic devices », Nanoscale, vol. 6, no 15, p. 8444-8458, juill. 2014. [CrossRef]
  38. Q. H. Li, D. Zhu, W. Liu, Y. Liu, et X. C. Ma, « Optical properties of Al-doped ZnO thin films by ellipsometry », Applied Surface Science, vol. 254, no 10, p. 2922-2926, mars 2008. [CrossRef]
  39. E. D. Palik, Handbook of Optical Constants of Solids, vol. 3, 5 vol. Academic Press, 1998.
  40. M. Chalh, « Elaboration, caractérisation et modélisation optique d’électrodes transparentes intégrant des nanofils d’Ag pour des applications solaires », p. 223.
  41. J.-F. Li, Y.-J. Zhang, S.-Y. Ding, R. Panneerselvam, et Z.-Q. Tian, « Core–Shell Nanoparticle-Enhanced Raman Spectroscopy », Chem. Rev., vol. 117, no 7, p. 5002-5069, avr. 2017. [CrossRef]
  42. L. Sun, J. Wang, E. Zhang, et Q. Tian, « Study on the Mechanism of Super-Resolution Near-Field Structure High-Density Optical Data Storage », Jpn. J. Appl. Phys., vol. 42, no 8R, p. 5113, août 2003. [CrossRef]
Preprints 77836 g001
Figure 1. (a) representation of the surface plasmon localized on the nanoparticles [20], (b) Plasmon cavity [21]; (c) Schematic diagrams illustrating a surface plasmon polariton (SPP) [22]; (d). Plasmon waveguide [23].
Figure 1. (a) representation of the surface plasmon localized on the nanoparticles [20], (b) Plasmon cavity [21]; (c) Schematic diagrams illustrating a surface plasmon polariton (SPP) [22]; (d). Plasmon waveguide [23].
Preprints 77836 g001
Preprints 77836 g002
Figure 2. (a) Structure of the numerical model of the ZAZ electrode and boundary conditions; optical indexes of: (b) SiO2; (c) ZnO; (d) AgNWs [40].
Figure 2. (a) Structure of the numerical model of the ZAZ electrode and boundary conditions; optical indexes of: (b) SiO2; (c) ZnO; (d) AgNWs [40].
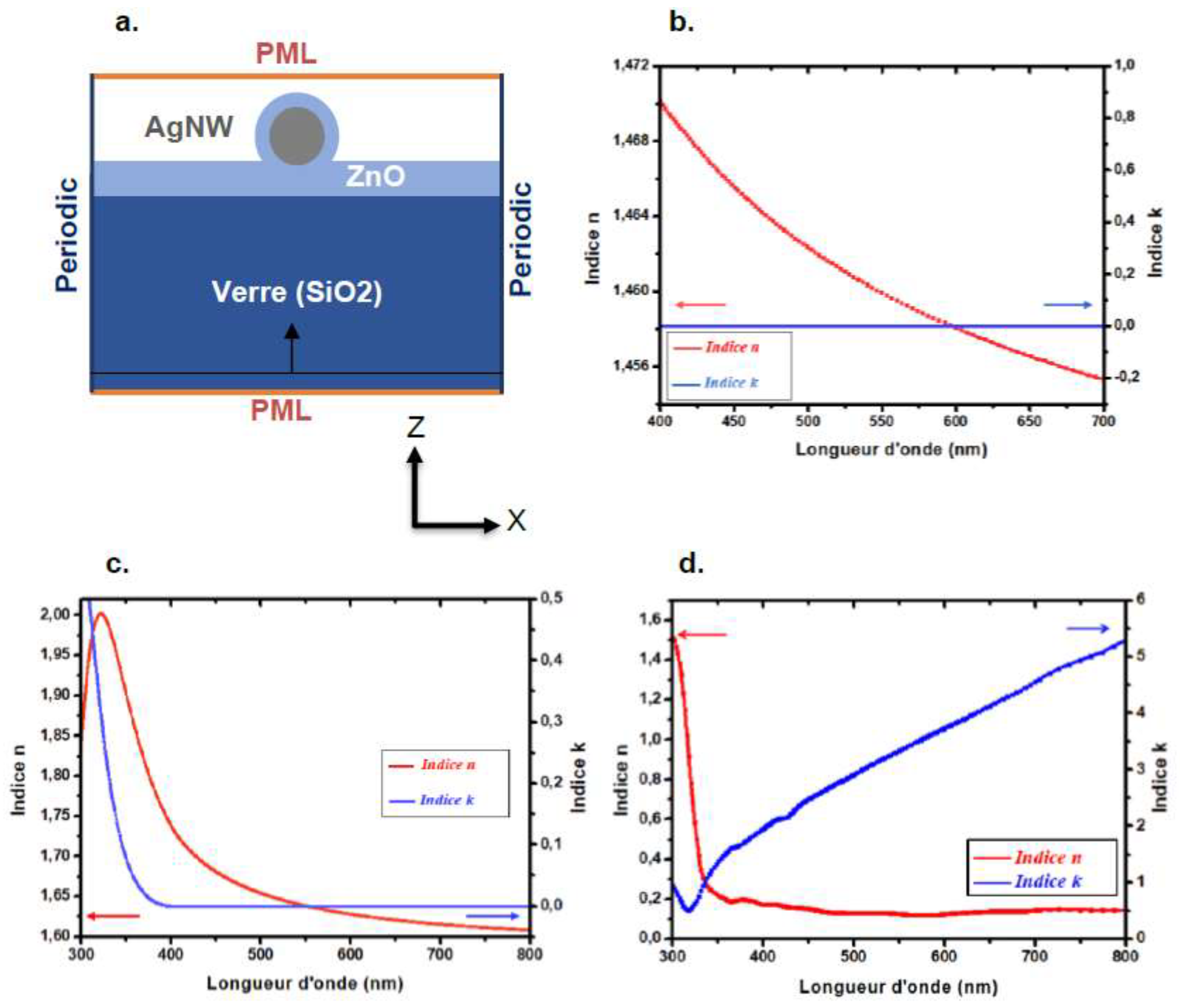
Preprints 77836 g002
Preprints 77836 g003
Figure 3. (a) Absorption of the modeled ZAZ electrode; (b) Spatial distribution of the squared modulus of the electric field around AgNW at peak absorption wavelength (347 nm).
Figure 3. (a) Absorption of the modeled ZAZ electrode; (b) Spatial distribution of the squared modulus of the electric field around AgNW at peak absorption wavelength (347 nm).
Preprints 77836 g003
Preprints 77836 g004
Figure 4. Spatial distribution of the normalized squared electric field on a section of silver nanowire at different wavelengths.
Figure 4. Spatial distribution of the normalized squared electric field on a section of silver nanowire at different wavelengths.
Preprints 77836 g004
Preprints 77836 g005
Figure 5. Longitudinal distribution of the normalized squared electric field 10 nm above the silver nanowire at different wavelengths. The polarization of the 2 plane-wave sources is shown in the bottom left of the first figure. It provides a better understanding of the "diagonal" resultant representing the direction of the observed exaltations.
Figure 5. Longitudinal distribution of the normalized squared electric field 10 nm above the silver nanowire at different wavelengths. The polarization of the 2 plane-wave sources is shown in the bottom left of the first figure. It provides a better understanding of the "diagonal" resultant representing the direction of the observed exaltations.
Preprints 77836 g005
Preprints 77836 g006
Figure 6. Variation of the electric field squared as a function of distance d from the observation point to the AgNW/ZnO interface (d=0). The observation point is located at the center of the AgNWs, by vertical translation, we can move it up or down, thus varying the distance d. the monitoring wavelength is between 400 and 470 nm, depending on the location of the observation point.
Figure 6. Variation of the electric field squared as a function of distance d from the observation point to the AgNW/ZnO interface (d=0). The observation point is located at the center of the AgNWs, by vertical translation, we can move it up or down, thus varying the distance d. the monitoring wavelength is between 400 and 470 nm, depending on the location of the observation point.
Preprints 77836 g006
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content.
Copyright: This open access article is published under a Creative Commons CC BY 4.0 license, which permit the free download, distribution, and reuse, provided that the author and preprint are cited in any reuse.
Prerpints.org logo

Preprints.org is a free preprint server supported by MDPI in Basel, Switzerland.

facebook logotwitter logolinkedin logo
MDPI Initiatives
Important Links
Subscribe

Disclaimer

Terms of Use

Privacy Policy

© 2024 MDPI (Basel, Switzerland) unless otherwise stated