Preprint
Review
Leakage Currents Measurements of Surge Arresters
Altmetrics
Downloads
684
Views
22
Comments
0
A peer-reviewed article of this preprint also exists.
This version is not peer-reviewed
Submitted:
29 June 2023
Posted:
29 June 2023
You are already at the latest version
Alerts
Abstract
The paper presents the methods of assessing the technical condition of varistor surge arresters used in laboratory tests and in operation - performed without disconnecting the arresters from the network. The analysis of diagnostic methods was supplemented with the results of measurements of the leakage current of arresters coming directly from production and used in the power industry. Among the available methods of evaluating the technical condition of arresters, mainly indicator solutions (temperature, operation counter) and using the measurement of selected parameters of the leakage current are used. In the latter, the method of determining the resistive component of the leakage current, determined on the basis of the analysis of voltage and current waveforms, or only the arrester current, has become widespread. In this type of measurements, current clamps are used in operation, and additionally in voltage measurements from voltage transformers, where you have to take into account the fundamental, additional sources of errors discussed in the article. These errors and dispersion resulting from the production technology may fundamentally hinder the proper assessment of the technical condition, hence it is so important to properly recognize the listed basic sources of measurement uncertainty. In addition, the analysis should take into account three factors related to external conditions - temperature, voltage applied to the arrester and the content of higher harmonics in the supply voltage for which appropriate methods have been provided to determine the active component of the leakage current for reference conditions.
Keywords:
Subject: Engineering - Electrical and Electronic Engineering
1. Introduction
High voltage surge arresters have an important role in the power system, especially in protecting the insulation of power lines and transformers against lightning and switching overvoltages. Their appropriate selection makes it possible to obtain proper insulation coordination between the electrical strength of the protected insulation and the level of protection clearly defined by the protective characteristics of surge arresters. Periodical inspection of the technical condition of the arrester allows to control the maintenance of the insulation coordination margin and thus ensure the correct operation of the transformer in the conditions of voltage exposure. Information on the condition of surge arresters - similarly to other elements of the energy system - should be obtained already at the level of acceptance measurements, so as to determine the operationally acceptable reference levels of the arrester's quality indicators and the planned method of earlier liquidation of elements characterized by poor technical condition. This approach allows for higher operational reliability of the energy system, in which emergency shutdowns of the network are undesirable phenomena due to the problems of maintaining the continuity of electricity supply to consumers.
The basic element of the arrester are varistors made on the basis of zinc oxide ZnO (semiconductor) with many appropriate admixtures (mainly bismuth), which, as a consequence of the multi-phase structure, are characterized by non-linear voltage-current characteristics.
For the voltage-current characteristics of the varistor in the initial range, it is observed at voltages forcing the flow of small currents (e.g. up to 1 mA, (Figure 1, point (I1, U1)), with the resistance value of the varistor in the order of gigaohms. On the other hand, for a small change in the voltage U in the area of the so-called breakdown (Figure 1, point (I2, U2)), the current I reaches values of the order of kiloamperes, which is usually described by the power relationship (1):
where k – material dependent constant and α – nonlinearity coefficient. At higher voltages, due to the reduction of barriers between the grains, the current conduction character of the varistor is typically resistive with a resistance of a few ohms [3].
U = kIα
Laboratory tests show that in ZnO ceramics, in the case of high-frequency waveforms, of the order of 1 MHz, typical for lightning overvoltages, there is a maximum dielectric loss factor. An increase in the temperature of the arrester strongly favors the increase of conductive properties. An increase in leakage and polarization currents is then observed. In addition, aging with current surges causes a shift to the right of the voltage-current characteristics, which is physically associated with a change in the grain size distribution in the varistor volume - especially as a result of negative polarity surges. This phenomenon is related to the influence of temperature on the conduction mechanisms, especially in smaller varistors (e.g. low voltage), where relatively small exposures, due to weaker absorption of current surges, can cause strong heating of the varistor and change its U(I) characteristic, which shifts to right towards larger currents [1, 3].
At the level of LV and MV grids, the commercial power industry usually operates the surge arresters until they fail, and any checks usually force the voltage to be switched off, the surge arrester disconnected from the grid system, measurements carried out using external voltage sources, a decision to replace or reconnect the apparatus to the grid. From the point of view of lightning and surge protection, it is periodically checked according to the internal operating instructions: in the scope of visual inspection - condition of insulators (cracks, scratches, traces of surface discharges), corrosion condition of fittings and connecting wires, damage or too high mechanical stress, number of surge arresters, value of leakage current, condition of earthing connections - condition of the conductor, continuity, corrosion, protective coatings. The result is an inspection card on which the condition of the device is entered - good, medium (within the scheduled date) and bad (immediate repair).
As part of a specialist or ad hoc review, specific lists of measurement activities can be proposed. This applies in particular to the area of power substations in the HV area, where power utilities have introduced their own inspection procedures consisting in testing surge arresters disconnected from the network or assessed online. Then, in order to assess surge arresters, the permissible levels of leakage current or reference voltage are given. For example, the manufacturer of the Tridelta arrester type SBK-II 96/10.2 expects a reference voltage level in the range of 101.6 - 106.3 kV when inducing a leakage current of a maximum value of 5 mA. In HV grid systems, the shutdown of the voltage is usually limited and in this case the activities are usually in the form of continuous measurements monitoring the technical condition of the surge arresters especially in places providing overvoltage protection for distribution systems at the transformer/switching station (T/S_S).
Recommendations for the diagnostics of MV and HV spark-free surge arresters are provided in Appendix D to the standard [2] containing guidelines for their use and selection. The standard applies to surge arresters that will be operated in three-phase systems with a nominal voltage above 1 kV. The supplement is a review of methods widely used in the power engineering of the facility, unfortunately based on a literature review from the years up to and including 1993, and proposes the use of simple technical solutions consisting in the introduction of:
- damage indicators that do not disconnect the arrester from the network, but only indicate the technical condition by indicating the amplitude and time of current flow or the temperature of the varistors;
- devices signaling the state of partial or complete destruction of the varistor element (e.g. disconnectors);
- special devices measuring the number and/or amplitude of current and/or voltage surges;
- series spark gaps in solutions requiring disconnection from the network or remaining in the network system;
- temperature analyzers;
- measurements of harmonic leakage current or active power losses.
Indicator devices are a component of a complete arrester or its additional element connected in series and are divided into fault indicators, disconnectors and operation counters. Damage indicator, in case of exceeding the current amplitude or the duration of a certain critical current value, only indicates this fact, but without automatic disconnection of the arrester from the network. The disconnector, in turn, is designed to isolate the arrester from the network system at the time of its failure. Typically, an explosive element (solutions for MV) is used for this purpose, triggered by the flow of a short-circuit current of a certain amplitude and duration. The disadvantage of the applied solution is the fact that after the arrester is disconnected, there is no overvoltage protection in the power network section until it is replaced.
Another way to determine the degree of degradation of the surge arrester is to use a trip counter triggered by a discharge current exceeding a certain amplitude. In the case of multiple discharges with times between discharges of less than 50 ms, due to the design of the counting system, not all discharges may be counted. In some designs, a sufficiently long follow current flow is required for the meter to work, which may cause difficulties in counting short discharge currents.
An interesting method of analyzing the state of the surge arrester is to use a thermal imaging camera. Intensive heating of the varistor structure causes a local increase in the temperature of the insulating housing, indicating problems with the varistor or water penetration into the housing and local increase in surface currents in the varistor stack.
However, from the methods proposed by the standard [2], the methods based on the determination of the leakage current and its resistive component gained special importance in the online diagnostics of HV arresters, and in the off-line diagnostics of MV and LV arresters. The standard specifies the level of the capacitive component in the range of 0.2 to 3 mA, depending on the capacitance of the arrester, which is typically 60 ÷ 150 pF per kV of the rated voltage, referred to the area of 1 cm2 of the varistor element. The diagram given in the standard gives a typical U/Ur characteristic indicating a leakage current of about 1 mA at a voltage of 0.6 Ur. Doubling the active component usually causes a slight increase by approx. 10% of the leakage current, which means that the main diagnostic effort goes towards the development of methods analyzing the active component of the leakage current in which the third harmonic of this current has a significant share in the active current (from 10 to 40 %).
In most cases, in the power industry, there are methods that ensure constant monitoring of the leakage current of limiters, unfortunately in the presence of frequent external disturbances. The standard for calculating the active component proposes various methods of leakage current analysis (a method of using a voltage signal as a reference, a method of compensating the capacitive component using a voltage signal, a method of compensation without using a voltage signal, a method of compensation using the analysis of currents in three phases and harmonics analysis using the following methods: harmonic, third harmonic with harmonic compensation in mains voltage, first order harmonic analysis). Table 5 in [2] indicates that in professional practice the methods of analysis of harmonics of the leakage current are generally used, the origins of which date back to the 1980s.
An undoubted advantage of the harmonic analysis method is the ability to measure the state of the arrester without disconnecting it from the network. Due to the significant error resulting from the content of the third harmonic in the supply voltage, even within the range of 100% ÷ 350%, in practice the method of third harmonic analysis with compensation with the signal related to the third harmonic of the limiter's capacitive current has become the most popular. In practice, the method of measuring the leakage current based on the determination of the active component or the power of losses isolated on the arrester is most often used. Both measurements on the surge arrester connected to the network (operational) or disconnected from the network (laboratory with DC or AC voltage) are used. In order to enable the measurement of the leakage current, a special insulated earthing clamp is installed between the arrester and the earthing, to which a measuring device is connected periodically (periodic diagnostics) or permanently (monitoring with recording the leakage current value on the memory card or in the supervisory system).
Currently, papers on new numerically advanced methods of determining the active component are still being published. A review of existing older and innovative ideas is included in publications [8, 9]. The work [8] lists innovative extensions created after establishing the content of Appendix D of the standard. These include works [17-22], which analyze various techniques for analyzing the current signal - both only the total leakage current and additionally with a separate resistive component, allowing to obtain the amplitude and shift angles in the current signal for the components - total and/or resistive [17-20], as well as leading to the determination of the shape and similarity of current signals recorded in a synchronous manner [21, 22]. On the other hand, in the work [9], a number of improved classical methods have been compiled that allow to obtain its resistive component from the current signal, specifying the limitations of their use, of which the presence of higher harmonics in the supply voltage was indicated in the first place.
New methods of determining the resistive component and their practical applications leading to online diagnostics of limiters are still described in the literature [11, 12, 23-31]. For example, an interesting solution is the online method of measuring the resistive component proposed in [11], which uses a remote non-simultaneous method of measuring the resistive current (RNS). RNS remotely and non-simultaneously measures all types of resistive current parameters using remote non-simultaneous phase difference measurement methods and specific harmonic analysis algorithms. The results of the simulation and application of the proposed method showed sufficient accuracy, also in the conditions of frequency deviations and the presence of harmonic components in the supply voltage, which makes it possible to effectively use it in online diagnostics.
Great opportunities are offered by the machine learning method, which, by determining patterns of clearly defined damage, offers ways to effectively determine [13, 20, 21]:
- surface conductivity;
- deposition of metallic impurities.
For example, paper [13] describes the results of the analysis of surge arresters from operation with a rated voltage of 11 kV (continuous operating voltage 9 kV, rated discharge current 5 kA), which were artificially soiled on the surface using the SLM method described in the IEC 60507 standard. This method uses a spray application of a mixture of NaCl and kaolin dissolved in distilled water. Using different compositions of the spray mixture, different conductivities of the outer layer were obtained after the process of appropriate drying in a thermal chamber. The correctness of the applied layer in terms of its uniformity was confirmed by using machine learning to detect dirt obtained at different concentrations of conductive material (5 levels) and relative humidity levels of 40% and 70%. During aging, an increase in the 3rd harmonic of the leakage current and a decrease in HR, defined as the ratio of the fundamental harmonic to the 3rd harmonic of the leakage current, was observed.
Measurements taken in the field are used to determine the lifetime of limiters in software and used in planning repairs. An example is the analysis [14] of the MOSA test results leading to the creation of a model combining ANFIS and the SVR model used at a later stage to determine the remaining life of use. A time series consisting of the leakage current and the value of the third harmonic component obtained from field measurements was used to teach and validate the implemented models. Forecasting models were qualitatively and quantitatively assessed using inspection graphics and analysis of adopted metrics for four different time horizons, respectively. In methods of this type, it is important to determine the correct criterion indicating the critical level of the exploited ZnO ceramics, for which physical and chemical tests are necessary, also taking into account the statistical differences between the output parameters of new arresters, and then of elements subjected to aging in complex exposure conditions.
An example of test results obtained by the authors for two MV and LV oxide surge arresters is presented in Figure 2 and Figure 3, respectively. The dispersion of the obtained values of the active component of the leakage current indicates the extreme variation in production quality and the related difficulties in unambiguous conclusions eliminating the surge arresters from operation. In principle, this type of work should be carried out on specific control samples each time in power plants when a batch of surge arresters is put into operation. This applies in particular to very poor quality arresters, an example of which is shown in Figure 3. The data collected in relation to individual technical solutions and manufacturers could be used as reference data for the analysis of operational results only in the case of observing the stability of the measurement results of leakage currents and reduced voltages. As can be seen from Figure 3, for LV arresters, this kind of preliminary testing should be crucial even in the context of the decision to put the delivered batch of products into operation.
In some countries, power utilities use methods for HV surge arresters that measure the following parameters of the leakage current: peak and average value, and the content of harmonic components. On the basis of the determined values, the coefficients p1, p2 and p3 are calculated using the formulas (2-4):
which should be included in the range p1∈(0,5; 0,76), p2∈(0,01; 0,06), p3∈(0,01; 0,09) [32].
If the permissible values are exceeded, it is recommended to disconnect from the mains voltage and measure the leakage current at DC voltage. In order to check the effectiveness of the above-mentioned method at deformed mains voltage, when there are high distortions in the leakage current, additional tests can be performed to determine the suitability of the above-mentioned method in extreme operating conditions (rain, dirt).
2. An example of the leakage current measurement for HV surge arrester
During the tests, measurements of the leakage current of HV surge arresters were carried out using two voltage sources with different content of higher harmonics. The measurements concerned complete, operated overhead surge arresters with a continuous operating voltage of Uc = 77 kV and a rated discharge current of 10 kA.
The tests were carried out using 50 Hz test sets with a voltage of up to 150 kV and 300 kV (Figure 4) characterized by a significant distortion of the voltage curve (THD > 10%). The voltage was measured using a WMUT 3 meter connected to a capacitive divider, and the current was determined on the basis of voltage measurement on a non-inductive resistor. Both given values were recorded with the Tektronix TDS 5034B oscilloscope in order to perform the necessary calculations to determine the active component of the leakage current. The oscilloscope's input channels recorded 10,000 samples in 40 ms, from which the current and voltage harmonics were then calculated.
In order to determine the technical condition of six arresters, the leakage current was measured and its active component was determined at the continuous operating voltage Uc = 77 kV and rated Ur = 96 kV. In addition, in accordance with the manufacturer's data, the reference voltage of the arrester was checked by forcing the maximum value of the leakage current at the level of 5 mA, which, according to the manufacturer's guidelines, should be within the range of 101.6 ÷ 106.3 kV. In addition, for both test assemblies, characteristic values were determined in accordance with the methodology proposed by [32]:
- peak value;
- average value;
- harmonic content,
and then calculated values of p1, p2, p3 employing formulas 2 - 4.
The measurements were aimed at checking the correctness of the measurement of the leakage current with different content of higher harmonics in the supply voltage and to determine the impact of higher voltage harmonics on the obtained test results. A comparison of the test results of the limiter with a dry surface and during the influence of artificial rain was also made.
A summary of the measurement results on two test assemblies with a significant and different content of higher voltage harmonics is shown in Table 1. Even with a significant content of higher harmonics, the p1 indicators of the technical condition of the arrester are correct. On the other hand, the remaining p2 and p3 indices concerning higher harmonics clearly exceed the acceptable levels given in [32].
Large distortions of the voltage curve cause a strong increase in current harmonics of higher orders, which causes a significant increase in the value of the leakage current, including its active component. Calculation of the actual value of the active component of the leakage current additionally requires knowledge of the frequency characteristics of the arrester, which allows for the compensation of dominant harmonic components. The given example of leakage current measurement indicates the need to introduce advanced numerical analyzes that will enable determination of the active component in reference conditions.
The technical condition of the tested 6 surge arresters is similar. Figure 5 shows the level of the leakage current and its active component measured with the test unit B for the unit with a THDU of 20%. With a high content of the 3rd harmonic in the supply voltage, for individual arresters, the active component of the leakage current, with a small dispersion of the measured values, maintains a relatively low value up to and including the continuous operating voltage. For the tested limiters, a change in the I(U) characteristic is observed after exceeding the voltage Uc, which is related to the increase in the resistive component in the leakage current (Figure 6 and Figure 7). In addition, the rms values of the voltage at the leakage current amplitude of 5 mA are in accordance with the requirements of the arrester manufacturer, which suggests their correct technical condition.
The comparison of the measurement results of the arrester with a dry and wet surface shows several times higher values of the active component of the leakage current in conditions of artificial rain (Figure 7).
3. Errors and their correction in determining the active component of the leakage current
In order to correctly determine the active component of the leakage current, it is easiest to calculate its value using the appropriate measurement procedures only on the basis of the leakage current oscillogram. For this purpose, various algorithms are used, among which the shift method [12, 27] and orthogonal vectors [33] should be mentioned. In operational applications, the current of the arrester is measured using current clamps with specific frequency characteristics that allow for proper calibration of the measuring systems and determination of measurement errors. It should be remembered that for diagnostic purposes, small currents of the order of several tens - several hundred μA are measured, usually in interference conditions, e.g. in the case of HV line surge arresters, when we are dealing with corona and relatively high electric field strength near the ground [34]. The measurements carried out by the author analyze the following technical problems that occur when measuring leakage current:
- angular and amplitude errors of commercial current clamps used to measure leakage current in surge arresters;
- angular and amplitude errors of voltage transformers used in MV lines;
- measurement uncertainties related to the characteristic disturbances in the current signal related to the properties of the ZnO arrester and the influence of temperature, voltage amplitude and shape, described in more detail later in this work.
3.1. Metrological properties of current clamps
In order to correctly determine the leakage current of the arrester and its components using the clamp method, it is necessary to determine the amplitude and phase errors of the measuring transducers used in the frequency range of at least 5 kHz.
The selected frequency range results from the content of higher voltage harmonics typical for power systems, the permissible level of which results from the regulations and the standard [35] related to the issue of electricity quality. As a standard, the THD level in the supply voltage is up to 8% in LV and MV networks for voltages not exceeding 36 kV. The harmonic distortion factor THD and the voltage harmonic percentage level are given by relations (5) and (6).
The permissible levels of harmonic components up to the 25th order specified in the standard are the same (Figure 8), and the highest values are observed for the 3rd, 5th and 7th harmonics, which should not exceed 5%, 6% and 5%, respectively . The levels of current harmonics recorded during electricity quality measurements are much higher and, depending on the distortion and short-circuit power of the supply voltage source and the type of non-linear receiver, the THD factor in the load current may reach much higher values, even in the order of several dozen percent.
The scaling of the clamps with a sensitivity of 1 μA used in the measurements was performed for frequencies in the range up to 20 kHz in the system shown in Figure 9. Before scaling, a routine calibration of the oscilloscope's measurement inputs was carried out and AC coupling was set in all its channels. In addition, the lack of phase shifts between the inputs was checked. The combination of the calibration system allows you to immediately assess certain metrological properties of the ALCL type current clamps used. The documentation provided by the manufacturer indicates that the clamps obtain an output voltage signal depending on the input resistance of the oscilloscope input, which is 1 MΩ as standard in the device used.
According to the characteristics provided by the manufacturer, a significant sensitivity of the clamps was obtained, but unfortunately with incorrect transmission of higher harmonics of the measured signal. Therefore, the input of the oscilloscope was loaded with various resistors, looking for the optimal value, i.e. one at which a sufficient signal was still obtained to measure the current at least at the level of 10 μA, and additionally, the correct signal transfer at higher frequencies. Finally, the value of the parallel resistance was set at 100 kΩ, which allowed to maintain the shape of the current curve up to the frequency of 10 kHz, at the expense of an acceptable reduction of the recorded voltage proportional to the current flowing in the cable. In addition, the central position of the wire in the clamps should be maintained in order to obtain repeatable test results.
Due to the fact that the leakage currents of the surge arrester increase approximately proportionally to the frequency, it may turn out that with a significant content of high order harmonics, the calibration factor specified only for the fundamental frequency will cause significant errors in the measurement of the leakage current, overstating the final result. In addition, using the algorithms for determining the active component of the leakage current, the correction time shifts introduced in the clamp measurements will cause additional errors.
Figure 10 shows the recorded voltage signals from the calibrated clamps (oscilloscope channel 2) and the voltage drop across the non-inductive series resistance in the circuit (oscilloscope channel 3) with a sinusoidal signal with a frequency of 50 Hz set on the power generator. The voltage signal from the current clamp clearly precedes the reference signal coming from the non-inductive resistor. For the waveform defined in this way, the amplitude calibration factor can be determined as the current in the circuit related to the output voltage signal of the clamps, and the phase calibration factor as the phase shift between the voltage waveforms coming from the current clamps and the voltage drop across the non-inductive resistor. The waveforms of the above-mentioned calibration coefficients significantly depend on the frequency, which is shown in Figure 11 and Figure 12, respectively.
The frequency response shown in Figure 12 shows that the voltage signal produced by the clamps up to 1 kHz leads the actual current in the circuit and then lags behind. On the other hand, the characteristic of transferring the current values from Figure 11 by the clamps is strongly non-linear with a minimum for the frequency of 2 kHz. Both operation at low frequencies and then for higher current frequencies above 5 kHz causes a reduction in the voltage signal, and thus the need to introduce higher coefficients to be converted into the correct output value corresponding to the actual current in the circuit. The quite complicated characteristics of the clamps show that the conversion of the signal recorded with the above method in technical measurements requires initially decomposition of the signal into higher harmonics, conversion of the component signals using appropriate amplitude and phase correction factors, and then assembling the signal into a real one, which can, for example, be implemented programmatically in the Labview software.
It should be noted that in relation to the direct method of measuring the voltage drop on the reference resistor with an oscilloscope, other methods give several percent errors resulting from the data processing algorithm in analog-digital converters (Figure 13 and Figure 14). It seems that with the nature of the voltage signal in the clamps, the best results can be obtained by averaging the samples obtained in subsequent periods and using calculations in accordance with the principles of theoretical electrical engineering.
The results of leakage current measurements using the current inputs of the PK1 and PK2 multimeters, shown in Figure 14, indicate small errors in relation to the method treated as a standard one. However, the use of commercial PK-C current clamps may cause large errors when working with signals with frequencies exceeding 2 kHz (Figure 13).
3.2. Metrological properties of current clamps
Similar problems as described in point 3.1 occur when voltage transformers are used for oscillography or voltage measurement in power systems also on surge arresters in order to more accurately calculate the active component. Voltage transformers in power measurement systems ensure the adjustment of the voltage on the secondary side to the standardized requirements of measuring devices, e.g. analog and digital voltmeters or voltage coils of power meters, or actuators in protection systems. In the case of using unearthed voltage transformers, additional galvanic separation is obtained between the high and low voltage circuits, because all parts of the primary winding are isolated from the earth in accordance with the rated insulation level of the device.
The accuracy of the voltage or current transformation of the transformer is determined by its class, which gives the maximum voltage and angular errors only in the range of the rated frequency of the transformer [36, 37]. In order to determine the expected errors in the transformation of frequencies higher than the rated one, measurements of the ratio and the phase shift of the signal from the secondary to the primary side as a function of the frequency of the voltage applied to the primary side of the transformer with a ratio of 15000/100 V/V and class 0.5 were performed. A power generator was used, which produced a voltage of up to 300 V and frequencies in the range of 10 Hz ÷ 20 kHz. Channel 1 of the oscilloscope records the voltage waveform on the primary side uMN(t) through a voltage probe with a ratio of 1:100, and channel 2 directly the voltage umn(t) of the secondary winding (Figure 15). The indicated measurements were made at frequencies of 0.05; 0.1; 0.2; 0.5; 1, 2, 4, 6, 8, 10, 12, 14, 16, 18 and 20 kHz, for which the relations kU(f) and ϕ(f) were plotted, shown in Figure 16.
On the basis of the performed measurements, it was found that the input signal attenuation occurred at a frequency of about 8 kHz, and for the frequency of 10 kHz and (16 ÷ 18) kHz, an increase in the ratio resulting from resonance phenomena was found. At the given frequencies, there are strong voltage distortions and a phase shift between the primary and secondary voltages of the transformer. In the given analyzed case, in the frequency range up to 1250 Hz, which is important in mapping the course of typical voltages in power grids, no significant errors were found.
3.3. Correction factors in leakage current measurements
Leakage current measurements, seemingly simple to carry out, require taking into account a number of correction factors - the influence of temperature - kT, voltage - kU and harmonic content (frequency) in order to convert the leakage current to a reference level determined, for example, by the temperature of 293 K, voltage amplitude Uc only with harmonic frequency primary. In principle, the measurement of leakage current should be abandoned in difficult weather conditions, when in the presence of dew, soot, atmospheric precipitation, the level of leakage current due to significant surface leakage may lead to incorrect conclusions (Figure 7).
3.3.1. Voltage and temperature correction factors in leakage current analysis
In order to obtain correct diagnostic conclusions from the measurements of the leakage current of the ZnO arresters, a number of analyzes were performed to determine the impact of the above factors by defining the correction factors - temperature kT and voltage kU. These coefficients are necessary to calculate the leakage current of the arrester measured in any voltage and temperature conditions determined by temperature and effective voltage, taking into account the content of higher harmonics. The leakage current is recalculated to the reference level set by the measurer in order to unambiguously compare the results of measurements carried out over a longer time horizon, when changes in both analyzed quantities clearly occur in operating conditions. The kT and kU coefficients should be determined for a given type of arrester after leakage current measurements carried out in various temperature and voltage conditions [2, 38]. Figure 17 shows examples of kU coefficients for LV arresters with operating voltage Uc=275 V and rated discharge current 20 kA with time parameters 8/20 μs/μs. In addition, the data of the manufacturer of the LCM500 device used in the diagnostics of medium and high voltage limiters, tabulated in [38], has been added. Reference levels U/Ur=0.7 and Tw=293 K are proposed here.
The correction factors for LV arresters obtained in own measurements are similar to those for medium voltage surge arresters [38] only for the condition U/Ur>0.7. At the supply voltages, much greater discrepancies were obtained, which may be related to the very small values of leakage currents and additional errors in their measurement.
An increase in temperature causes a decrease in the permanent operating voltage in a manner dependent on the chemical composition of the varistor ceramics [39, 41]. With a significant content of iron and aluminum oxides, these changes can be even several times for the selected temperature [42]. Common temperature coefficients K defined by the formula (7) in [43] are given at the level of (0.5 ÷ 1)∙10-3/K:
where:
- UTw(1 mA) – voltage at Tw and current 1 mA;
- UT0(1 mA) - voltage at T0 and current 1 mA;
- Tw – temperature of surge arrester;
- T0 – temperature at reference level equal 293 K.
For most surge arresters in proper technical condition, the voltage at the forced leakage current with a temperature increase of 20 K decreases by about 1% [43]. Increased heat release in the varistor structure affects the voltage at its terminals and reduces the permissible levels of current and energy surges as a function of temperature.
Changes in the conductivity of the varistor structure γ measured at the reference voltage U1 mA, i.e. also its temperature coefficients, occur according to different band characteristics [40, 41]. It turns out that the influence of the chemical composition on the γ(1/Tw) characteristic is a complex model containing several partial conductivities in a series-parallel system. However, regardless of the chemical composition of the varistor, the function describing the conductivity of the varistor from its leakage current is correctly approximated by the power function γ = aIb with constants a and b [41]
Another method, more often used in practice, is to determine the value of the leakage current I(Uc) at the voltage of continuous operation of the arrester Uc, and then to calculate the temperature coefficients kT, which are directly converted to the reference temperature, e.g. 293 K according to the equation (8):
In order to determine the correction factors, ZnO varistors from two manufacturers, A and B, were used. In one case, the tests concerned 8 surge arresters with a continuous operating voltage of 275 V from manufacturer A. In the second case, varistors (10 pieces of each type) from manufacturer B were tested with a voltage of 0.28 kV, 0.5 kV, 1 kV and 3 kV. The surge arresters were stored in the thermal chamber for 5 hours until the temperature distribution in the varistor structure was normalized, and then the current-voltage characteristics were measured in the system with the correctly measured current, forcing a direct or alternating voltage coming in the second case from a sinusoidal voltage generator with a low content of higher harmonics.
The correction factors were determined in two operating states - at direct voltage (manufacturer A and B) on the basis of standard current-voltage characteristics and at alternating voltage (only manufacturer A) by determining the resistive component of the leakage current according to the methodology described in [44].
Figure 18 shows exemplary current-voltage characteristics of the tested surge arresters at the continuous operating voltage Uc = 275 V and the rated discharge current 20 kA, 8/20 μs. Above the DC voltage corresponding to the amplitude of the continuous operating voltage, a slight effect of temperature on the leakage current level is observed.
On the basis of the measured I(U) characteristics, the kT coefficients for direct voltage, shown in Figure 19, were calculated. These coefficients for varistors from manufacturers A and B, marked for similar continuous operating voltage, are significantly different. The determined correction factors, especially at low ambient temperatures, are characterized by a significant dispersion of the current value results.
For surge arresters from manufacturer A, the course of the kT(Tw) coefficient was additionally determined from the measurements of the active component of the leakage current at alternating voltage, which is similar to the data given in [38] for the temperature range (263 ÷ 303) K. For higher temperatures, slightly higher values of the coefficient were observed kT, which may result from the properties of the tested ZnO ceramics (Figure 20).
The data from the publication [45] show that with the identical chemical composition of materials, a change in the temperature profile of the sintering process leads to visible differences in the pre-breakdown characteristics only in the field of tests performed at direct voltage. It follows that the determination of temperature and voltage coefficients should be performed with alternating voltages.
Figure 21 compares the impact of the arrester dimensions related to the continuous operating voltage Uc on the course of the temperature coefficient kT. The increase in the voltage Uc and thus the volume of the varistor significantly reduces the influence of temperature on the leakage current, which is indicated by the flatter kT(Tw) characteristic.
3.3.2. Correction due to higher voltage harmonics
Figure 22 shows the shape of the voltage and current of the arrester, respectively, at the mains voltage lower than the continuous operating voltage U<Uc, when there is a small level of the resistive component, but with a significant distortion of the current waveform due to higher harmonics of the supply voltage.
In order to determine the influence of the harmonic frequency of the voltage on the value of the leakage current for the tested arresters, the results of the I(f) characteristics tests were used, on the basis of which the characteristics of the module z(f) and the displacement angle ф(f) of the arrester impedance for voltages in the range from 150 to 340 V were calculated.
The impedance characteristic of the limiter enables the introduction of a correction for the content of higher harmonics by implementing the following algorithm:
- calculation of the content of higher harmonics uk and ik in the supply voltage and leakage current;
- calculation of the theoretical, higher harmonics of the leakage current itk, based on the characteristics obtained in impedance measurements and the FFT fast Fourier transform algorithm for the supply voltage;
- correction of the content of higher harmonics in the measured leakage current according to the ik-itk action.
The correction of higher harmonics, which allows to obtain the waveform of the corrected leakage current icor(t), can occur for all lines of higher harmonics, even up to the order of 100, or only for those that cause a significant change in the current of a given harmonic. In practice, due to the dominance of the odd harmonics 3rd, 5th, 7th, 9th, 11th, 13th, 15th in the supply voltage, it is sufficient to consider only the listed frequencies (Figure 23 and Figure 24).
Correction of the current waveform occurs up to the frequency of 50 Hz (non-distorted voltage) and allows to compare the leakage current results for the measured surge arresters, even with a strongly distorted voltage. The obtained waveform of icor(t) allows for the correct calculation of parameters important for the assessment of the technical condition of the arrester: leakage current, resistive component on the basis of active power according to [44]. The relative error related to the measurement results at undistorted voltage, in the case of calculating the active component for the corrected leakage current icor(t), does not exceed 5%. The proposed concept of correcting the measurements of surge arresters obtained at distorted voltages, provided that the frequency characteristics obtained from the manufacturer for a given type of arrester are available, makes it possible to compare test results and track changes in the leakage current and its active component. This is important for predicting the aging rate of the arrester during operation.
The proposed method can be used provided that the arrester is supplied with voltage U<Uc and there is no strong distortion of the leakage current associated with the presence of a resistive component greater or comparable to the capacitive current. In the case of U>Uc, the correction is also possible, provided that the impedance characteristic for the arrester with a high value of the active component of the leakage current is available.
3.3.3. Failure to determine the leakage current under non-reference conditions
In order to determine the conversion factor of the measured leakage current value into the actual value, the appropriate correction factors given in point 3.3 should be taken into account. For this purpose, assuming the correct calibration of the current clamps, the following formula should be used:
where coefficients kU, kT, kTHD result from dependencies (10 - 12) determined empirically for a selected type of surge arresters.
IT0(Uc) = kT ∙ IT(Uc)
The kT and kU coefficients can be read directly from the appropriate kT(T) and kU(U) graphs. This type of data is sometimes provided by manufacturers in data sheets. The results of tests contained in publications on the influence of ambient temperature on the level of leakage current indicate different waveforms of the value of leakage current measured at different test voltages for a specific type of arrester. For example, for surge arresters with a rated voltage of 120 kV, a 20% increase in the leakage current was found in relation to the reference temperature of 303 K [46]. The kTHD factor, on the other hand, depends on the content of individual harmonics during the measurement. In order to calculate it, the algorithm given in point 3.3.2 should be used. This coefficient can be estimated approximately by giving the ratio of the RMS values of the 50 Hz fundamental harmonic band to the leakage current of the varistor.
A cursory analysis of the dependencies listed in Figure 25, Figure 27 and Figure 28 allows to directly determine the maximum additional measurement error introduced during, for example, a temperature of 323 K.
During the error analysis, the leakage current of 6 low-voltage surge arresters was first measured for different voltages and temperatures. The obtained results made it possible to determine the correction factors kU and kT for individual surge arresters and the averaged factor, which in the further part of the analysis was used to convert the measurement results obtained at voltage U and temperature T to reference values, in this case 275 V and 293 K. Due to the different the technical condition of the tested arresters and the dispersion of characteristics being a feature of varistor production, errors were calculated for conversion into reference values for individual arresters, which are shown in Figure 26.

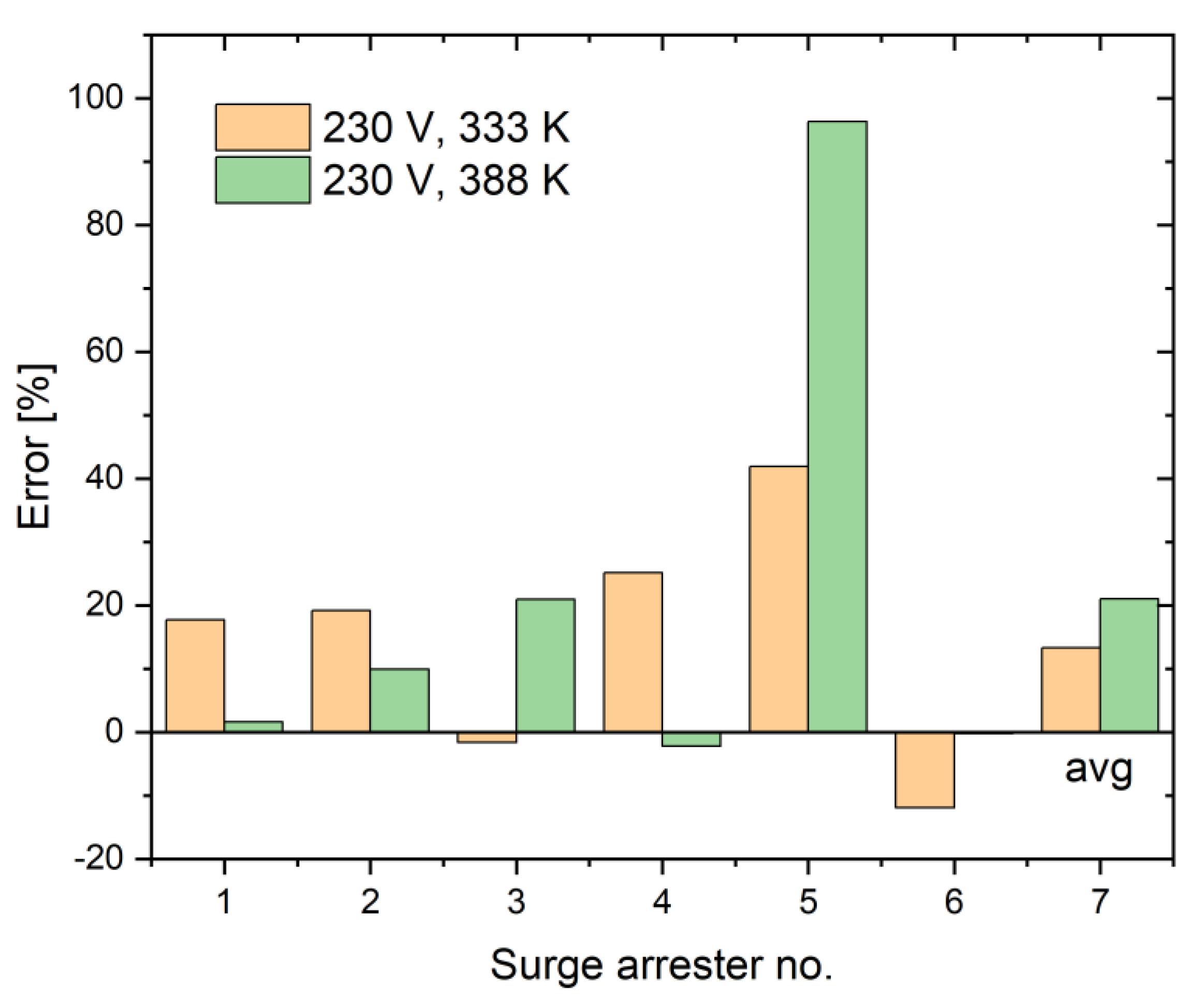
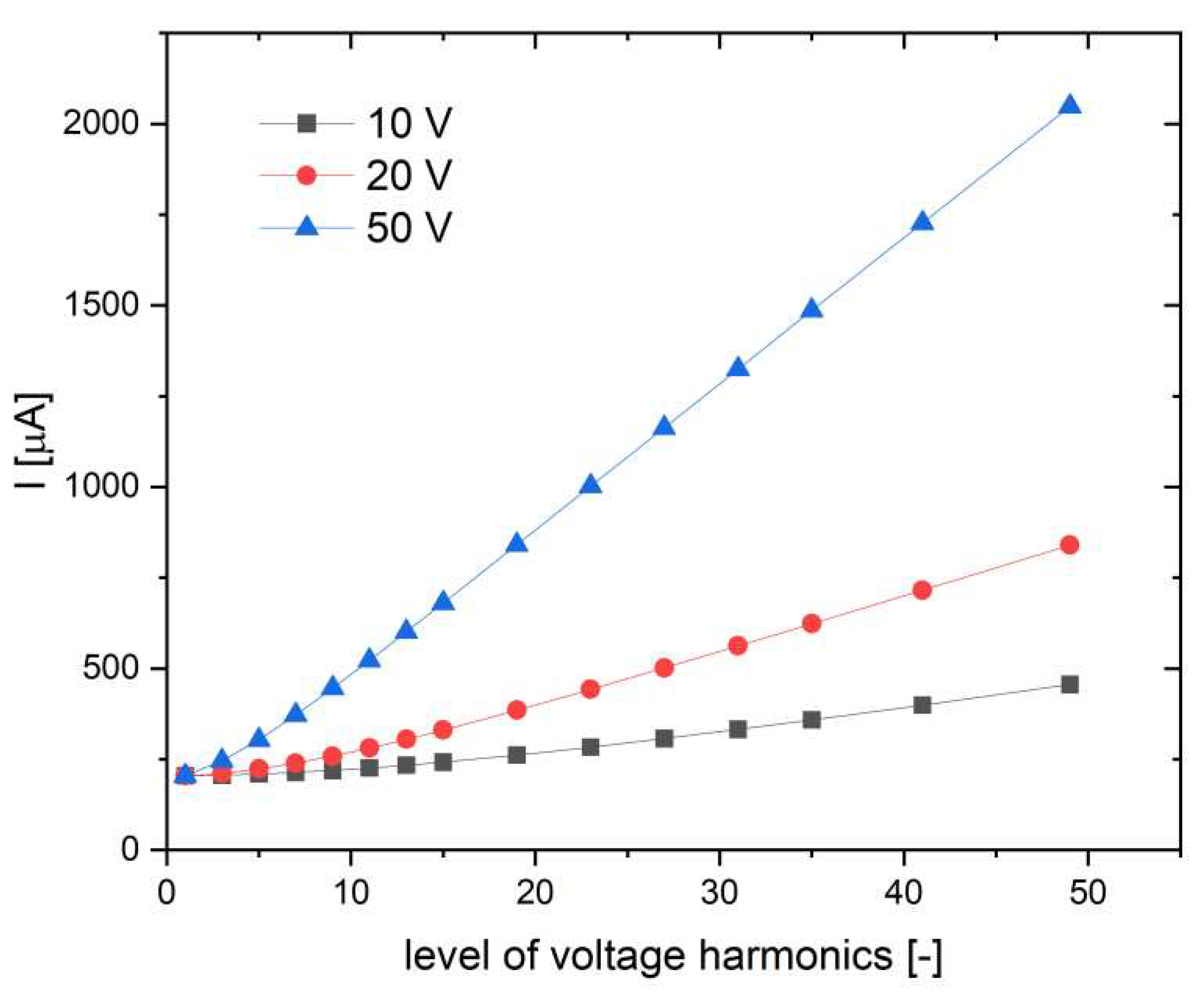

Figure 25.
Comparison of the corrected waveform of the leakage current icor(t) with the current of the fundamental harmonic.
Figure 25.
Comparison of the corrected waveform of the leakage current icor(t) with the current of the fundamental harmonic.

Figure 26.
Errors obtained when using temperature coefficients when converting measurement results to reference values U=275 V and T=293 K.
Figure 26.
Errors obtained when using temperature coefficients when converting measurement results to reference values U=275 V and T=293 K.

Figure 27.
Influence of the level of voltage harmonics on the leakage current of the arrester.

Figure 28.
Influence of voltage harmonics on the active component of the leakage current.

In order to determine the influence of higher voltage harmonics on the value of the leakage current, simulations were performed in the Mathcad program using the averaged impedance characteristics of the surge arresters. On their basis, the values of the leakage currents and the active component were calculated during the operation of the arrester at 230 V, on which only one odd harmonic of the order of 3 to 49 with an amplitude of 10 V (4.3%), 20 V (8.7%) was imposed. and 50V (21.7%). The influence of the phase angle of the superimposed voltage harmonic on the value of the leakage current was also checked.
The results of the numerical analyzes obtained indicate that the leakage current of the arrester strongly depends on the frequency of the higher voltage harmonic. The change of the current for a higher harmonic with an amplitude of 20 V imposed on the base waveform with a frequency of 50 Hz increases by as much as 411% from the 3rd to the 49th component. For higher frequencies of the higher harmonic, a significant capacitive component is introduced, visible in the leakage current of the arrester (Figure 27).
On the other hand, the active component of the leakage current with the same content of higher harmonics at the level of 8.7%, which is exceeding the permissible values in distribution and transmission networks, increases in relation to the 50 Hz component by only 6% (Figure 28).
A simplified correction of the leakage current value may consist in calculating the fast Fourier transform from the waveform of the measured current and calculating the current only from the first harmonic. In addition, having the phase of the electric field strength or directly the supply voltage, you can calculate the phase shift between the current and voltage waveforms for the first harmonic and, on this basis, calculate the active current component in a simplified way. In the case of LV arresters, this procedure gives correct results with an error not exceeding 10%, as shown in Figure 29.
On the basis of the obtained simulation results, in accordance with the guidelines [47], it is possible to abandon the introduction of correction factors for the value of the active component of the leakage current, especially that with the increase in the order of the harmonic in power networks, its amplitude strongly decreases. The permissible contents of voltage harmonics of higher orders from the 26th and above are not normalized. The guidelines of the standard [35] end on the 25th order, for which the content is required at the level of 1.5%, and for even harmonics of the 6th - 24th order it is 0.5%. The total content of higher harmonics in the supply voltage THD cannot be higher than 8%.
4. Conclusions
Based on the analyzes of the effect of voltage distortion on the measurement error of the leakage current and its active component, the following conclusions can be drawn:
- temperature and voltage have a significant influence on the value of the leakage current and its resistive component. Appropriate correction factors kU and kT depend on the chemical composition and production process of varistors and their dimensions;
- during measurements of the leakage current of surge arresters, even at U<Uc, there are strong current distortions, also in the range of higher frequencies;
- the presence of voltage harmonics necessitates the introduction of appropriate correction factors in order to correctly calculate the leakage current at the fundamental frequency of 50 Hz;
- there is no need for some methods [4, 15, 47] to correct the calculations of the resistive component of the leakage current;
- determining the frequency characteristics of surge arresters enables effective correction of leakage currents measured in conditions of distorted voltage;
- the influence of harmonics can be corrected in a simplified way on the basis of the Fourier transform of the current waveform and performing calculations only for the first harmonic of the current.
Author Contributions
Conceptualization and methodology, M.O.; formal analysis and investigation, M.O. and L.S.L;X.X.; resources, X.X.; data curation, M.O..; writing—original draft preparation, M.O.; writing—review and editing, L.S.L. and G.R.; visualization, L.S.L.; supervision, G.R.; All authors have read and agreed to the published version of the manuscript.
Conflicts of Interest
The authors declare no conflict of interest.
References
- Goran Dobric, Zlatan Stojkovic,, Zoran Stojanovic, Experimental verification of monitoring techniques for metal-oxide surge arrester, IET Generation, Transmission & Distribution, 2019. [CrossRef]
- EN IEC 60099-5:2018, Surge arresters - Part 5: Selection and application recommendations.
- P. PAPLIŃSKI, J. WAŃKOWICZ, H. ŚMIETANKA, P. RANACHOWSKI, Z. RANACHOWSKI, S. KUDELA, M. ALEKSIEJUK, COMPARATIVE STUDIES ON DEGRADATION OF VARISTORS SUBJECTED TO OPERATION IN SURGE ARRESTERS AND SURGE ARRESTER COUNTERS, Arch. Metall. Mater. 65 (2020), 1, 367-374. [CrossRef]
- Fernando S. N., Raghuveer M. R., Technique to examine the influence of voltage harmonics on Leakage Current Based MOSA Diagnostic Indicator, 2000 Annual Report Conference on Electrical Insulation and Dielectric Phenomena, 2000 Conference on Electrical Insulation and Dielectric Phenomena, 15-18 October 2000, vol. 2, s. 596 – 599, ISBN 0-7803-6413-9. [CrossRef]
- PN-EN 60099-4:20015, Ograniczniki przepięć - Część 4: Beziskiernikowe ograniczniki przepięć z tlenków metali do sieci prądu przemiennego.
- PN-EN 60099-5:2014-01, Ograniczniki przepięć -- Część 5: Zalecenia wyboru i stosowania.
- Koseda H., Wrocławski M., Przepięcia w sieci 15 kV i 110 kV koncernu Energa, Materiały Międzynarodowej Konferencji Bezpieczeństwo i niezawodność nowoczesnych systemów rozliczeniowo – pomiarowych ze zdalnym odczytem i zdalnym monitoringiem parametrów sieci, Serock 2005.
- Arup Kumar Das and Sovan Dalai, Recent Development in Condition Monitoring Methodologies of MOSA Employing Leakage Current Signal: A Review, IEEE SENSORS JOURNAL, vol. 21, no. 13, pp. 14559-14568, July 1, 2021. [CrossRef]
- Abdullah Munir, Zulkurnain Abdul-Malek, Rai Naveed Arshad, Resistive Leakage Current Based Condition Assessment of Zinc Oxide Surge Arrester: A Review, 13th International Conference on the Properties and Applications of Dielectric Materials (ICPADM 2021.
- Instrukcja Standardy eksploatacji urządzeń elektroenergetycznych w PGE Dystrybucja S.A. Oddział Łódź-Teren.
- Zhongjun Fu, Student Member, IEEE, Jianyu Wang, Arturo Bretas, Senior Member, IEEE, Yun Ou, and Genyuan Zhou, Measurement Method for Resistive Current Components of Metal Oxide Surge Arrester in Service, IEEE TRANSACTIONS ON POWER DELIVERY, VOL. 33, NO. 5, OCTOBER 2018. [CrossRef]
- S. Mohamed Arshad, Asanka.S. Rodrigo, Modified Phase Shifting of Leakage Current to Condition Monitoring of Metal Oxide Surge Arresters in Power System. [CrossRef]
- A.K. Das et al.: Cross Spectrum Aided Surface Condition Assessment of Metal Oxide Surge Arrester Employing Convolutional Neural Network. [CrossRef]
- Vandilson R. N. Barbosa , Student Member, IEEE, George R. S. Lira , Member, IEEE, Marianna B. B. Dias , Student Member and Edson G. Costa , Senior Member, IEEE, Estimation of Metal Oxide Surge Arresters’ Useful Life Based on Time Series Forecasts, IEEE TRANSACTIONS ON POWER DELIVERY, VOL. 37, NO. 2, APRIL 2022, IEEE. [CrossRef]
- J. Lundquist, L. Stenstrom, A. Schej, and B. Hansen, “New method for measurement of the resistive leakage currents of metal-oxide surge arresters in service,” IEEE Trans. Power Del., vol. 5, no. 4, pp. 1811–1822, Oct. 1990. [CrossRef]
- B. Lee and S. Kang, “A new on-line leakage current monitoring system of ZnO surge arresters,” Mater. Sci. Eng., B, vol. 119, no. 1, pp. 13–18, 2005. [CrossRef]
- G. R. S. Lira and E. G. Costa, “MOSA monitoring technique based on analysis of total leakage current,” IEEE Trans. Power Del., vol. 28, no. 2, pp. 1057–1062, Apr. 2013. [CrossRef]
- M. Khodsuz and M. Mirzaie, “Evaluation of ultraviolet ageing, pollution and varistor degradation effects on harmonic contents of surge arrester leakage current,” IET Sci., Meas. Technol., vol. 9, no. 8, pp. 979–986, Nov. 2015. [CrossRef]
- A. Metwally, M. Eladawy, and E. A. Feilat, “Online conditio monitoring of surge arresters based on third-harmonic analysis of leakage current,” IEEE Trans. Dielectr. Electr. Insul., vol. 24, no. 4, pp. 2274–2281, Sep. 2017.
- W. Doorsamy and P. Bokoro, “Condition monitoring of metal-oxide surge arresters using leakage current signal analysis,” in Proc. IEEE Int. Conf. High Voltage Eng. Appl. (ICHVE), Athens, Greece, Sep. 2018, pp. 1–4. [CrossRef]
- A.K. Das, B. Ghosh, S. Dalai, and B. Chatterjee, “Sensing Surface contamination of metal oxide surge arrester through resistive leakage current signal analysis by mathematical morphology,” IEEE Sensors J., vol. 20, no. 16, pp. 9460–9468, Aug. 2020. [CrossRef]
- K. Das, S. Dalai, and B. Chatterjee, “Deep learning-based surface contamination severity prediction of metal oxide surge arrester in power system,” IET Sci., Meas. Technol., pp. 1–9, Feb. 2021. [CrossRef]
- Schei, Diagnostics techniques for surge arresters with main reference to on-line measurement of resistive leakage current of metal-oxide arresters, in International Conference on Large High Voltage Electric Systems (CIGRÉ 2000), 2000, pp. 1-10.
- S. Shirakawa, F. Endo, H. Kitajima, S. Kobayashi, K. Goto, and M. Sakai, "Maintenance of surge arrester by a portable arrester leakage current detector, IEEE Transactions on Power Delivery, vol. 3, no. 3, pp. 998-1003, 1988. [CrossRef]
- A.H. Khavari, A. Munir, and Z. Abdul-Malek, Circuit-based Method for Extracting the Resistive Leakage Current of Metal Oxide Surge Arrester, Bulletin of Electrical Engineering and Informatics, vol. 9, no. 6, 2020. [CrossRef]
- Lundquist, L. Stenstrom, A. Schei, and B. Hansen, New method for measurement of the resistive leakage currents of metal-oxide surge arresters in service, IEEE Transactions on Power Delivery, vol. 5, no. 4, pp. 1811-1822, 1990. [CrossRef]
- Z. Abdul-Malek, Novizon, and Aulia, A new method to extract the resistive component of the metal oxide surge arrester leakage current, in 2008 IEEE 2nd International Power and Energy Conference, 2008, pp. 399-402. [CrossRef]
- M. Khodsuz and M. Mirzaie, An improved time-delay addition method for MOSA resistive leakage current extraction under applied harmonic voltage, Measurement, vol. 77, pp. 327-334, 2016. [CrossRef]
- Y. Han, Z. Li, and H. Zheng, A new method to extract the resistive current of MOA based on least square, in Properties and Applications of Dielectric Materials (ICPADM), 2015 IEEE 11th International Conference on the, 2015, pp. 312-315: IEEE. [CrossRef]
- Y. Han, Z. Li, H. Zheng, and W. Guo, A decomposition method for the total leakage current of MOA based on multiple linear regression, IEEE transactions on power delivery, vol. 31, no. 4, pp. 1422-1428, 2016. [CrossRef]
- G. Dobrić, Z. Stojanović, and Z. Stojković, The application of genetic algorithm in diagnostics of metal-oxide surge arrester, Electric Power Systems Research, vol. 119, pp. 76-82, 2015. [CrossRef]
- Patent PL 194370 B1, Urządzenie do diagnozowania stanu zużycia ograniczników przepięć, zwłaszcza ograniczników znajdujących się w eksploatacji.
- Marek Olesz, Determining the leakage current resistive component by the orthogonal vector method, September 2018, Conference: 2018 34th International Conference on Lightning Protection (ICLP). [CrossRef]
- Euler C. i inni, Inductive current sensor based on nanocrystalline alloys, XIX Imeko World Congress, Fundamental and Applied Metrology, 2009, Portugal, pp. 840 - 843.
- PN – EN 50160: 2010, Parametry napięcia zasilającego w publicznych sieciach elektroenergetycznych. 2: – EN 50160.
- PN-EN 61869-1:2009, Przekładniki – Część 1: Wymagania ogólne.
- PN-EN 61869-3:2011, Przekładniki – Część 3: Wymagania szczegółowe dotyczące prze-kładników napięciowych indukcyjnych.
- Hinrichsen V., Monitoring of High Voltage Metal Oxide Surge Arresters, VI Jornadas In-ternacionales de Aislamiento Eléctrico, Bilbao, 22./23.10.1997, Paper 6.4.
- Larsen V., Lien K., In-Service Testing and Diagnosis of Gapless Metal Oxide Surge Ar-resters, Materiały IX International Symposium on Lightning Protection, 26th-30th No-vember 2007 – Foz do Iguaçu, Brazil.
- Fernandez-Hevia D. i inni: Bulk-grain resistivity and positive temperature coefficient of ZnO-based varistors, Applied Physics Letters vol. 82, no. 2, 13 January 2003, pp. 221 – 214. [CrossRef]
- Li S. i inni, The relation between residual voltage ratio and microstructural parametres of ZnO varistors, Materials Letters 59, 2005, pp. 302 - 307. [CrossRef]
- Sawalha A. et al., Electrical conductivity study in pure and doped ZnO ceramic system. Physica B 2009, vol. 404, pp. 1316-1320. [CrossRef]
- EPCOS, SIOV Metal Oxide Varistors, General technical information, Epcos AG, April 2011. 20 April.
- Olesz M.: Algorytmy obliczania składowej czynnej prądu upływowego ograniczników przepięć, Przegląd Elektrotechniczny 11a, 2012.
- Fujiwara Y., Shibuya Y, Imataki M, Nitta T.: Evaluation of surge degradation of metal oxide surge arrester, IEEE Trans. Power App. PAS 101, 1982, pp. 978-985. [CrossRef]
- Woo C. L. i inni (Zulkurnain Abdul-Maleka, Saeed Vahabi Mashaka), Effect of Ambient Temperature on Leakage Current of Gapless Metal, Jurnal Teknologi, vol. 64, no. 4, 2013, pp. 157–161.
- Xu Z. i inni, A current ortogonality metod to extract resistive leakage current of MOSA, IEEE trans. On Power Delivery, vol. 28, no. 1, January 2013. [CrossRef]
Figure 1.
U(I) characteristics of a new and aged surge arrester.
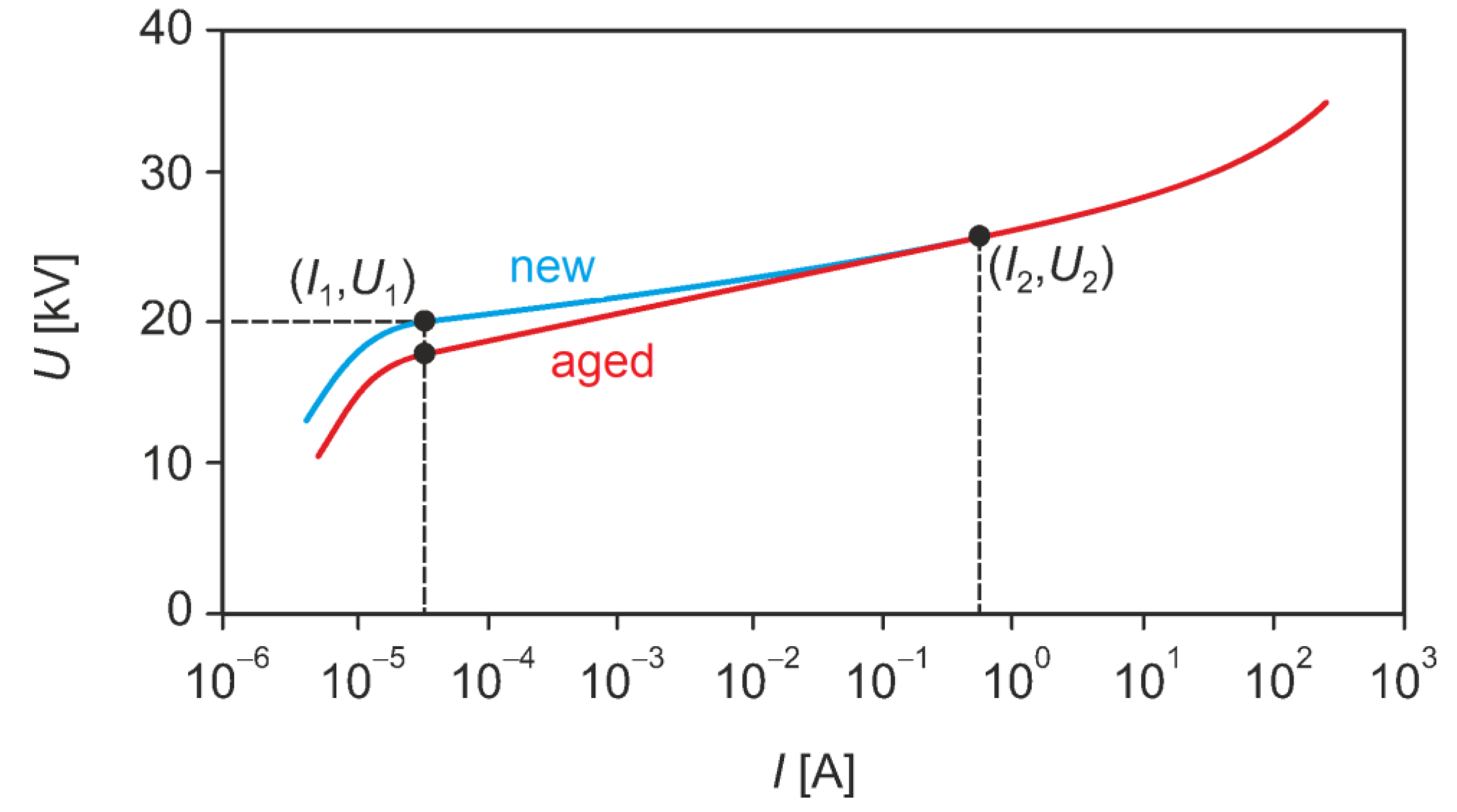
Figure 2.
Differentiation of leakage currents of MV arresters a) new and b) after applying a single current surge, obtained on the basis of testing 10 samples of an arrester with similar rated parameters from manufacturers A, B, C, D.
Figure 2.
Differentiation of leakage currents of MV arresters a) new and b) after applying a single current surge, obtained on the basis of testing 10 samples of an arrester with similar rated parameters from manufacturers A, B, C, D.

Figure 3.
Differentiation of leakage currents of LV arresters a) new and b) after applying a single current surge, obtained on the basis of testing 10 samples of an arrester with similar rated parameters from manufacturers E and F.
Figure 3.
Differentiation of leakage currents of LV arresters a) new and b) after applying a single current surge, obtained on the basis of testing 10 samples of an arrester with similar rated parameters from manufacturers E and F.

Figure 4.
Measuring stand with tested arrester: 1 - high voltage transformer 300 kV, 2 - voltage divider capacitor, 3 - surge arrester.
Figure 4.
Measuring stand with tested arrester: 1 - high voltage transformer 300 kV, 2 - voltage divider capacitor, 3 - surge arrester.

Figure 5.
Current-voltage characteristics for 6 tested arresters in the range of leakage current (average, maximum and minimum values of leakage current are marked) in the conditions of supplying unit B.
Figure 5.
Current-voltage characteristics for 6 tested arresters in the range of leakage current (average, maximum and minimum values of leakage current are marked) in the conditions of supplying unit B.

Figure 6.
The course of the leakage current of the arrester No. 4 measured at the voltage Uc = 77 kV and Ur = 96 kV with the power supply conditions of the test unit A.
Figure 6.
The course of the leakage current of the arrester No. 4 measured at the voltage Uc = 77 kV and Ur = 96 kV with the power supply conditions of the test unit A.
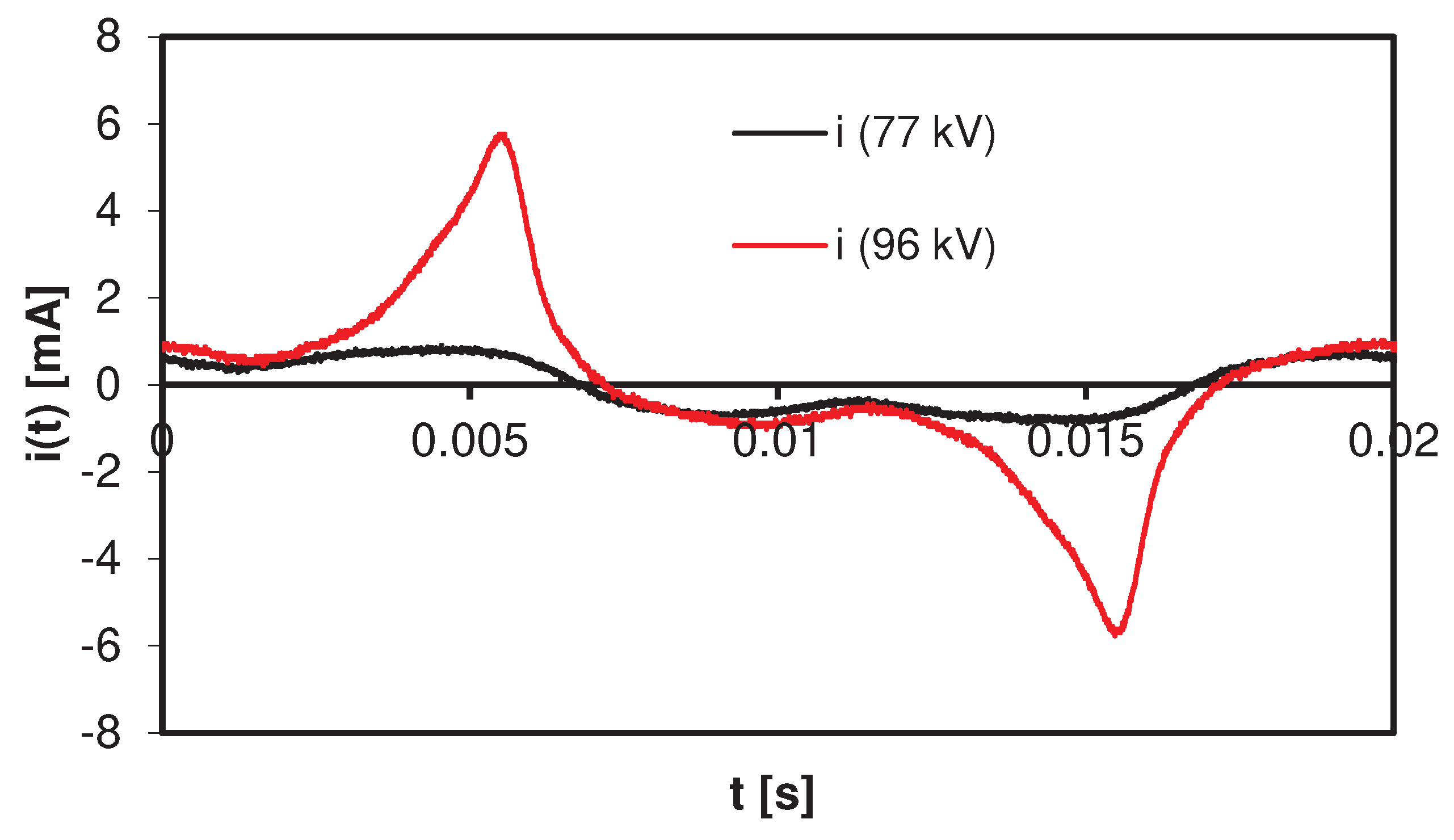
Figure 7.
Influence of the surface condition of the arrester No. 4 on the value of the resistive component of the leakage current.
Figure 7.
Influence of the surface condition of the arrester No. 4 on the value of the resistive component of the leakage current.

Figure 8.
Permissible levels of harmonic components in LV and MV networks [35].
Figure 8.
Permissible levels of harmonic components in LV and MV networks [35].

Figure 9.
Scheme of the measuring stand for current clamp calibration.

Figure 10.
Voltage waveform from current clamp Uclamp and non-inductive resistor UR = 50 Ω
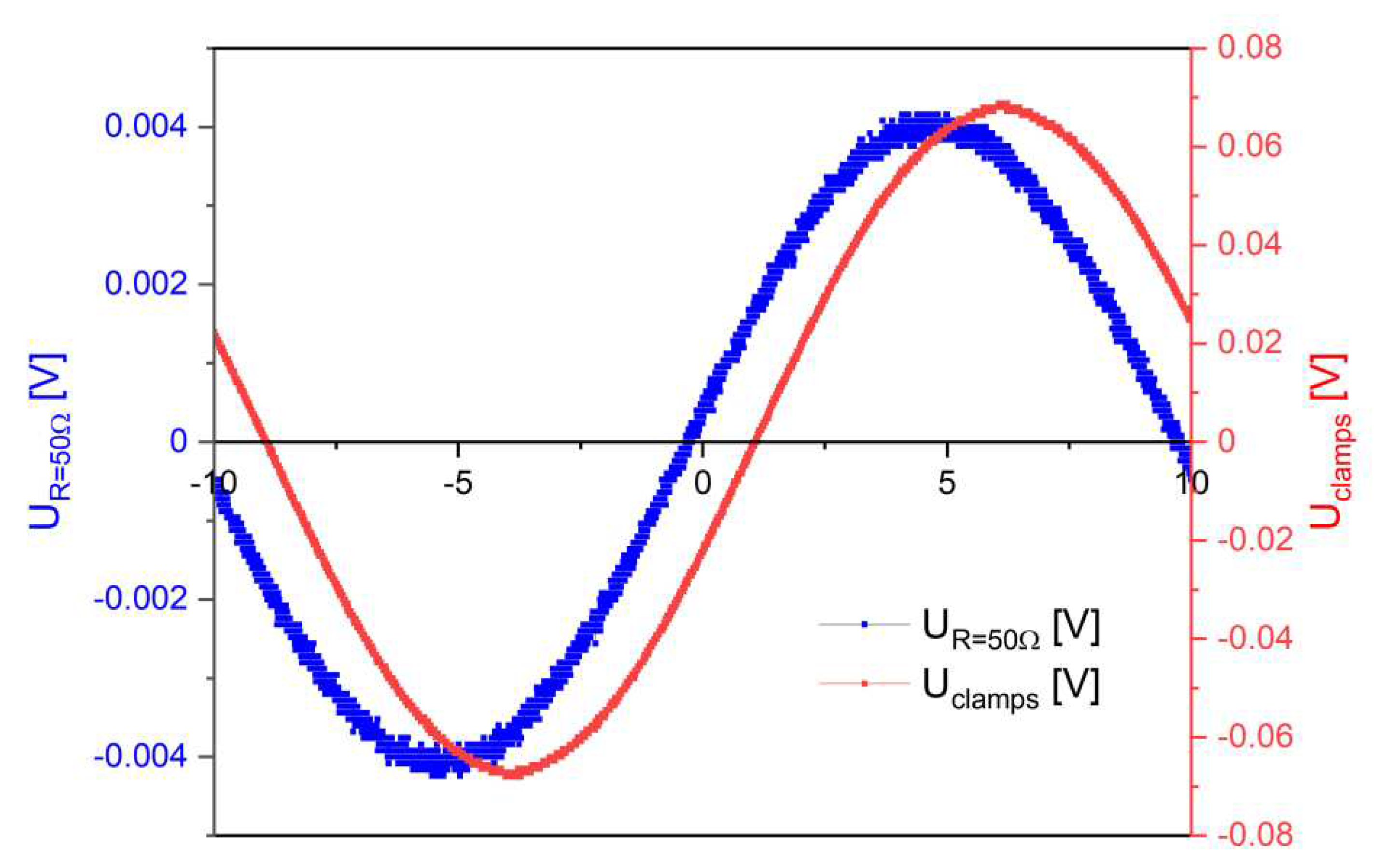
Figure 11.
Amplitude calibration coefficient determined by the quotient of the current in the circuit to the voltage generated at the output of the clamps.
Figure 11.
Amplitude calibration coefficient determined by the quotient of the current in the circuit to the voltage generated at the output of the clamps.

Figure 12.
Phase calibration coefficient determined by the shift of the clamp signal in relation to the real signal.
Figure 12.
Phase calibration coefficient determined by the shift of the clamp signal in relation to the real signal.

Figure 13.
Amplitude error of current measurement for commercial meters in the range up to 1 kHz (PK1, PK2 - commercial instruments with voltage measurement function, PK-C - clamp meter with a sensitivity of 1 μA).
Figure 13.
Amplitude error of current measurement for commercial meters in the range up to 1 kHz (PK1, PK2 - commercial instruments with voltage measurement function, PK-C - clamp meter with a sensitivity of 1 μA).

Figure 14.
Amplitude error of current measurement for commercial meters in the range up to 1 kHz (PK1, PK2 - commercial instruments with voltage measurement function, PK-C - clamp meter with a sensitivity of 1 μA).
Figure 14.
Amplitude error of current measurement for commercial meters in the range up to 1 kHz (PK1, PK2 - commercial instruments with voltage measurement function, PK-C - clamp meter with a sensitivity of 1 μA).

Figure 15.
Measuring system for analysis of voltage transformer frequency characteristics.

Figure 16.
Frequency characteristics of the UMZ20 type transformer, a) voltage ratio, b) phase shift between the voltages of the primary and secondary windings.
Figure 16.
Frequency characteristics of the UMZ20 type transformer, a) voltage ratio, b) phase shift between the voltages of the primary and secondary windings.

Figure 17.
Correction factors [38] taking into account the influence of voltage, IR(MO) means correction factors kU determined in own measurements.
Figure 17.
Correction factors [38] taking into account the influence of voltage, IR(MO) means correction factors kU determined in own measurements.
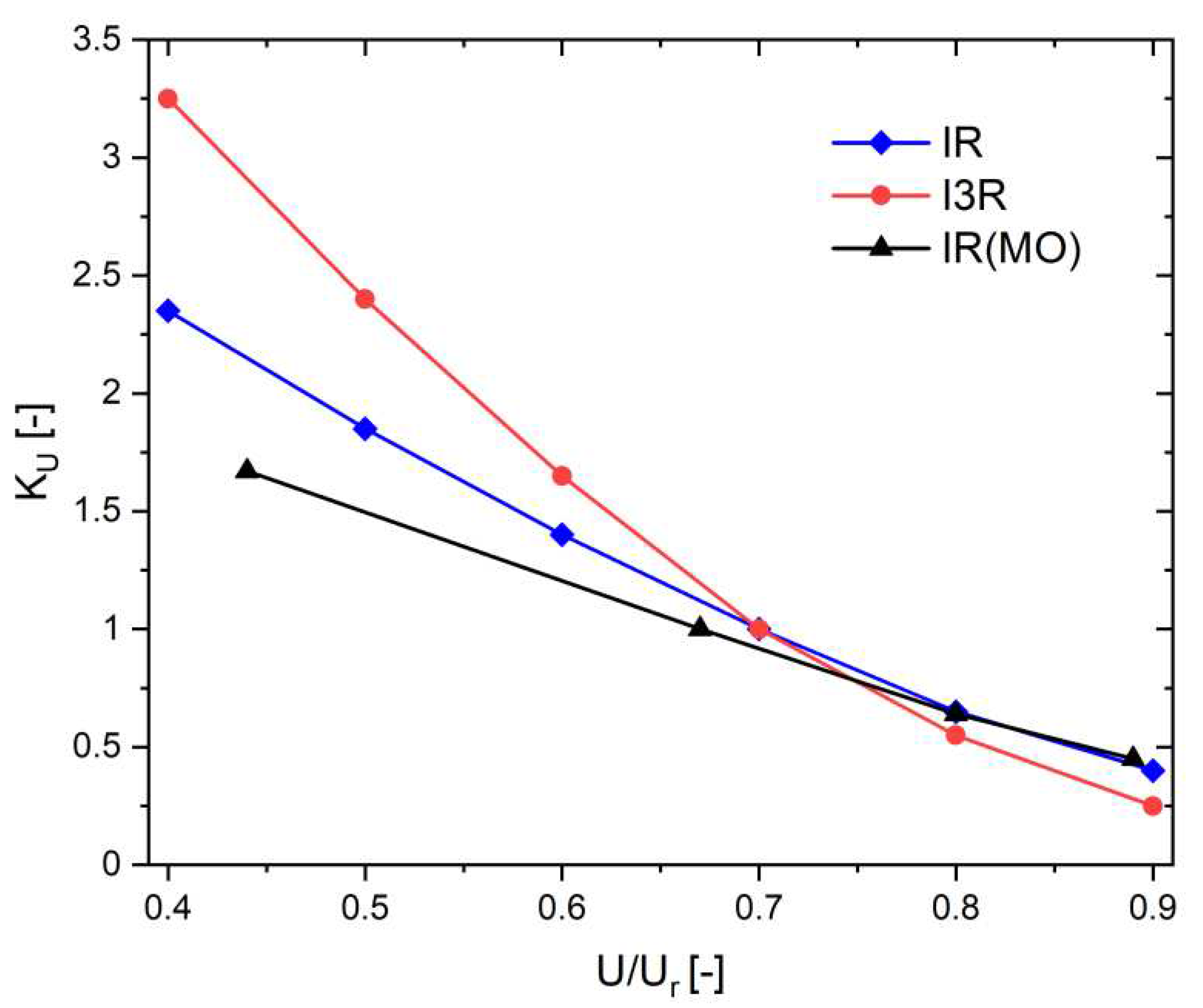
Figure 18.
I(U) characteristics for direct voltage of an exemplary surge arrester from manufacturer A.
Figure 18.
I(U) characteristics for direct voltage of an exemplary surge arrester from manufacturer A.

Figure 19.
Temperature correction factors calculated from measurements at DC voltage to convert leakage current measurements to reference conditions for Tw = 293 K.
Figure 19.
Temperature correction factors calculated from measurements at DC voltage to convert leakage current measurements to reference conditions for Tw = 293 K.

Figure 20.
Comparison of leakage current measurements at alternating voltage, IR, I3R - resistive component and its 3rd harmonic for leakage current according to [2, 39], IR(MO) - active component of leakage current determined in own measurements for the manufacturer A.
Figure 20.
Comparison of leakage current measurements at alternating voltage, IR, I3R - resistive component and its 3rd harmonic for leakage current according to [2, 39], IR(MO) - active component of leakage current determined in own measurements for the manufacturer A.

Figure 21.
Influence of the dimensions of the arrester manufacturer B (the height of the varistor is for 280 V - 3.5 mm, 0.5 kV - 5 mm, 1 kV - 8 mm and for 3 kV - 25 mm) on the value of the kT coefficient.
Figure 21.
Influence of the dimensions of the arrester manufacturer B (the height of the varistor is for 280 V - 3.5 mm, 0.5 kV - 5 mm, 1 kV - 8 mm and for 3 kV - 25 mm) on the value of the kT coefficient.

Figure 22.
Leakage current i(t) of surge arrester no. 8 measured at mains voltage u(t) with rms value of 237.9 V.
Figure 22.
Leakage current i(t) of surge arrester no. 8 measured at mains voltage u(t) with rms value of 237.9 V.

Figure 23.
Recorded values of voltage u(t) and leakage current i(t) at distorted voltage U=237.8 V. The corrected course of the leakage current icor(t) was calculated for odd harmonics from 1 to 15.
Figure 23.
Recorded values of voltage u(t) and leakage current i(t) at distorted voltage U=237.8 V. The corrected course of the leakage current icor(t) was calculated for odd harmonics from 1 to 15.

Figure 24.
Comparison of the corrected waveform of the leakage current icor(t) with the current of the fundamental harmonic 50 Hz i50Hz(t) resulting from the analysis with the FFT algorithm of the real waveform i(t).
Figure 24.
Comparison of the corrected waveform of the leakage current icor(t) with the current of the fundamental harmonic 50 Hz i50Hz(t) resulting from the analysis with the FFT algorithm of the real waveform i(t).

Figure 29.
Error in determining the active component δIR and the capacitive component δIC for individual surge arresters on the basis of taking into account the first harmonic of the leakage current.
Figure 29.
Error in determining the active component δIR and the capacitive component δIC for individual surge arresters on the basis of taking into account the first harmonic of the leakage current.

Table 1.
Results of measurements and calculations of characteristic values of the leakage current for arrester no. 4.
Table 1.
Results of measurements and calculations of characteristic values of the leakage current for arrester no. 4.
| Test stand A | Test stand B | |||
|---|---|---|---|---|
| Leakage current parameter |
U=77 kV THDU 13% |
U =96 kV THDU 11% |
U =77 kV THDU 21% |
U =96 kV THDU 18% |
| Peak value Imax [µA] | 678,8 | 1021,9 | 948,9 | 1328,5 |
| Average value Iavg [µA] | 408,4 | 597,8 | 491,1 | 779,0 |
| Harmonic content Ih [µA] | 171,5 | 308,1 | 415,2 | 510,4 |
| p1 | 0,60 | 0,58 | 0,52 | 0,59 |
| p2 | 0,25 | 0,30 | 0,44 | 0,38 |
| p3 | 0,42 | 0,52 | 0,85 | 0,66 |
| IR [µA] | 43,6 | 247,18 | 172,36 | 408,17 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Copyright: This open access article is published under a Creative Commons CC BY 4.0 license, which permit the free download, distribution, and reuse, provided that the author and preprint are cited in any reuse.
MDPI Initiatives
Important Links
© 2024 MDPI (Basel, Switzerland) unless otherwise stated






