Prerpints.org logo
Preprint
Article

Finite Element Study on Stability in the Femoral Neck and Head Connection to Varying Geometric Parameters with the Relates Implications on the Effect of Wear

Altmetrics

Downloads

134

Views

29

Comments

0

A peer-reviewed article of this preprint also exists.

Submitted:

11 July 2023

Posted:

12 July 2023

You are already at the latest version

Alerts
Abstract
Total Hip Arthroplasty (THA) is a common surgical procedure used to treat hip osteoar-thritis and other joint conditions that cause pain and functional limitation. Traditionally, THA has been most performed in elderly patients, but in recent years there has been an in-crease in hip arthroplasties in young patients. Femoral prosthesis rupture is a rare but sig-nificant complication that can also occur in young patients undergoing total hip arthro-plasty (THA). Some of the factors that can contribute to femoral prosthesis ruptures include abnormal overload, defects in the design lack of geometric fit, type of materials used in the stem and femoral head connection. The aim of this study is to analyze the criticalities in the contact between the femoral head and the stem neck. In particular, the two types of con-tacts will be taken into consideration: proximal and distal and through the finite element method (FEA) the criticalities will be defined. The results show that in the proximal contact the voltages are higher than 500 Mpa. This is due to the fact that there are higher circum-ferential voltages. In addition, to prevent bacterial infiltration or debris from the outside, the distal connection is the recommended one.
Keywords: 
Subject: Engineering  -   Bioengineering

1. Introduction

Total hip arthroplasty (THA) is a standard surgery that helps many patients relieve pain, regain mobility, and improve quality of life. Most modern THA implant designs use a modular conical junction in which the surfaces of the head hole cone and femoral stem cone fit together and, thus, friction-controlled locking is generated. This ensures both axial and rotational stability [1]. The characteristic of modularity has become quite widespread since the 80s as it simplifies the intervention and / or subsequent revisions. The modular components give the surgeon a high degree of freedom in the operating phase with regard to the choice of components, offset, biomaterials constituting the head and acetabular insertion. Thus, it is possible to better adapt the acetabular components, neck and stem of the prosthesis to the patient’s anatomy and hip biomechanics [2]. Modular femoral prostheses offer several advantages over standard prostheses. First of all, they allow the surgeon to customize the prosthesis according to the anatomical characteristics of the patient, ensuring better adaptation and greater stability. In addition, they can be used to address specific clinical conditions, such as hip dysplasia or femoral bone abnormalities. [3] The modulation of these prostheses also allows greater flexibility in treating patients with prosthesis wear or laxity problems, as components can be easily replaced or adjusted to improve the long-term effectiveness of the prosthesis. However, it is important to emphasize that the use of modular femoral prostheses requires specialized skills and knowledge on the part of the surgeon. It is critical that the surgeon is properly trained in the use of such prostheses and has a thorough understanding of the patient’s specific anatomy and needs to ensure proper placement and stability of the prosthesis. In conclusion, modular femoral replacements offer a personalized treatment option for patients who need hip replacement surgery. They allow greater flexibility in adapting the prosthesis to individual needs and can help improve long-term clinical outcomes. Despite this surgical advantage, in recent years attention has focused on mechanically assisted interstitial corrosion caused by agitation and accompanying corrosion resulting from micromovement to the modular connection. It is a process of deterioration of the prosthesis material due to interaction with body fluid, saline solutions and other elements present in the biological environment. Corrosion can lead to several problems, including: Metal ion release: Corrosion can cause metal particles to be released in the surrounding area. These particles can provoke an inflammatory response in surrounding tissue and can potentially damage bone and other tissues. Formation of deposits: Corrosion can lead to the formation of deposits of oxides and other corrosive substances on the surfaces of the prosthesis. [4] These deposits can adversely affect the functioning of the joint and cause irritation or damage to surrounding tissues. Material loss: Corrosion can cause a gradual loss of material from the prosthesis, compromising its structural integrity and reducing its durability. To reduce the risk of corrosion of femoral prostheses, several strategies have been developed:
Use of corrosion-resistant materials: Materials such as titanium alloys or ceramics have been introduced that exhibit greater corrosion resistance than traditional materials such as metal-chrome-cobalt.
Prosthesis design improvement: Improvements have been made to the prosthesis design to reduce areas of contact and friction that can promote corrosion. This includes reducing metal components in direct contact and using protective coatings. Regular monitoring: It is important to regularly monitor patients with femoral dentures for signs of corrosion or other problems. This can be done through X-ray checks, clinical examinations and blood tests to assess any signs of inflammation or elevated levels of metals in the blood. Importantly, corrosion of femoral prostheses is a problem that can vary from patient to patient and depends on several factors, including the type of prosthesis used, the patient’s physical activity and the specific condition of the joint. Proper clinical follow-up and the involvement of an orthopedic specialist are essential to ensure the appropriate assessment and management of patients with femoral prostheses. This concern has recently been highlighted by the high failure rates of large metal-on-metal THAs attributed to adverse local tissue reactions from exposure to corrosion debris [3,4]. The National Joint Registry (NJR) documented 138,0472 hip replacements, of which 90% were initial replacements and 10% were revisions. In addition, 12.5% of implant revisions were performed due to implant joint failure due to wear [3]. In addition, metal ion-related complications, such as inflammatory reactions and pseudotumors, have been linked to the head-neck junction of hip replacements [5,6]. Several aspects influence the operation of the head-to-stem pin, including the angular discrepancy between the head and the stem pin [7,8], the assembly process in surgery [9,10], the geometry of the pin [11,12], the size of the acetabular head [12,13], the combination of articular materials [11,12,14], the roughness of the contact surface [15,16], and possible contamination at the interface [17,18]. The stability of the coupling is achieved by forcing the head on the morse cone of the neck at the time of implantation, therefore, some studies have found that a forcing of 6,000 N was sufficient to ensure some stability.[18]
Theoretically, the contact between the conical hollow of the head and the morse cone of the neck should be extended along the entire length of the coupling. On the other hand, due to machining tolerances, contact does not take place along the entire length of the coupling, but usually in a single limited area Figure 1. Once the semi-angle of the φ 1 morse cone of the neck and the semi-angle of the conical φ 2 slot of the head is defined, we can calculate φ 3 = φ 2 - φ 1 . In the event that φ 2 > φ 1 , there will be limited contact at the apex of the morse cone with a reduction of the surrounding tensions inside the ceramic that constitutes the head [19]. If φ 2 < φ 1 , there will be contact in the lower area of the morse cone. In a load condition such that the vector changes direction with respect to the conicity, both the proximal and distal contact conditions could cause separation at the conical junction and subsequent entry of corrosive fluids into the cone. The ingress of liquids in conjunction with the hypoxic conditions prevailing at the junction would aggravate corrosion. Therefore, a conical junction that does not allow the ingress of fluids can be considered a desirable design feature. Figure 2
Conic geometry itself has evolved, including a reduction in both taper diameter and pin length. Reducing taper length from 20 mm to less than 10 mm resulted in an increase in the range of motion without impact (ROM), although it also reduced the surface area available for conical contact. Figure 3. This change reduces the stability of the taper but increases the relative movement of the coupling cone under load.
The aim of this study is to evaluate, by means of finite element analysis (FEM), the influence of the conical contact situation in terms of differences in taper angle and contact area on the stability of the conical connection of a Titanium alloy stem and Ceramic head for 2 different conditions Figure 4. What we want to understand is whether, as shown in other studies, a distal connection is more stable.

2. Materials and Methods

The model of the head-to-stem pin, a conventional hip prosthesis, and its components used in this study are shown in Figure 5. Subsequently, the finite element model with standard boundary and load conditions is illustrated.
Modelling of Hip Implants
First, a hip prosthesis with smooth head-stem pins was designed. In this experiment, titanium alloy, Ti-6Al-4V, was selected for the stem material, and cobalt chromium alloy, Co-Cr-Mo, was selected for the acetabular head material because they are widely used in the manufacture of hip replacement systems [20,21,22]. Table 1 summarizes the physical characteristics of the material alloys evaluated in this study [23,24]. A 3D model of the hip implant was created using Inventor Autodesk, a 3D modeling software platform.
Autodesk, and a 3D, CAD, CAM modeling software platform were used to build the 3D model in this study. The 2D geometry and 3D CAD model view of the hip implant with 2D geometry and the 3D CAD model view are shown in Figure 6.
In the case of contact between the head and the femoral stem, it is important to consider several aspects in FEA analysis:
Physical contact: It is necessary to define the type of contact between the head and the femoral stem, for example, a complete contact or a partial contact. You must also specify the friction or adhesion properties between the two surfaces. In particular, for this analysis a contact between head and femoral stem with friction equal to 0.3 was considered.[34]
Applied loads: It is important to define the loads and load conditions that simulate the physical activity of the patient. These can include compression loads, bending loads or torsion loads, depending on the specific analysis you want to conduct.
Constraint conditions: It is necessary to establish the constraint conditions of the model, for example by fixing the lower end of the femoral stem to simulate fixation in the bone.
Once all these aspects have been defined, FEA simulations can be performed to evaluate the stresses, deformations, and behavior of the contact between the head and the femoral stem. FEA analysis results can provide useful information about stress distribution, contact stability, and the possibility of micro-movements or potential problems such as voltage concentration.
Boundary and Loading Conditions with the Finite Element Model
In this study, boundary conditions include the fixed support zone of the plant and load conditions to estimate mechanical performance using a finite element computational model. This study follows the ISO-7206-6:2013 standard for building a mechanical fatigue test system for hip head-to-stem pin joints in computational model environments. Walking is the most common activity, while jogging is one of the most physically demanding activities. Walking and jogging loads were used here by subjects aged 50 to 68 years weighing between 70 and 120 kg (AVG 75 kg). In [26], the mechanical characterization of the functional load conditions of hip prostheses was presented. Figure 7 shows the resulting force of walking and jogging,[27] For 10% to 55% of each gait cycle, walking required more strength while this was between 25% and 55% for jogging.
For this work, the FEM analysis was performed with an ANSYS 2023 calculation program. The 3D geometry was created in Inventor and later imported into ANSYS 2023. A contact was assigned between the stem head and the head with friction in which the coefficient of static friction is 0.2 [27,28]. The mesh used to discretize the 3D into small elements to facilitate resolution was 0.5mm. Mesh thickening is a technique used in finite element analysis (FEA) to study the contact between two surfaces more accurately. When analyzing the contact between the head and the femoral stem of a prosthesis, the thickening of the mesh can help to obtain more precise results on the stresses and behavior of the surfaces in contact. In finite element analysis, the mesh represents the discretization of the three-dimensional model into finite elements. A denser mesh is characterized by a greater number of smaller elements, which allows you to better approximate complex geometry and stress variations within the model. In the case of the study of contact between the head and the femoral stem, the thickening of the mesh can be carried out in areas of interest, such as the interface between the two components. By using a denser mesh in these regions, contact behavior can be more accurately captured, including local effects of pressure, stress, and deformation. Mesh thickening can be accomplished by increasing the density of elements along contact surfaces or using localized refinement techniques, such as mesh refinement based on solution error criteria. This can take longer to compute as the number of items in the contact area increases. However, results obtained with a denser mesh can provide greater accuracy in the evaluation of contact and local stresses. Recent research on finite element studies based on hip replacements has used quadratic tetrahedral mesh elements [27,30,31] more than any other form. According to ANSYS [32], the preferred choice for complicated nonlinear geometry are tetrahedral mesh elements. Figure 8.

3. Results

In the following finite element study, as can be seen in (Figures 11,12) which shows the results obtained in the two configurations of proximal and distal contact for a femoral neck head pair in Co-Cr / Ti under a forcing load equal to 4000N, it can be seen that in the distal contact, the stresses on the neck are limited in a small area and vary between 80 and 150 Mpa while inside the hole made on the Co-Cr head voltage values of about 60 Mpa are reached. What is however most stressed to bending as a result of contact in the distal area is the lower part of the head where about 500Mpa are reached, this value could trigger cracks and risks of breakage. In the proximal contact, on the other hand, the circumferential tensions are higher and distributed over the entire surface of the neck because the contact area has decreased as a result of the same load applied, which therefore leads to an overall increase in tensions.
Preprints 79181 g010
Figure 12. FEM analysis results of the prosthesis with the stem head contact in the proximal zone. (1:Stress Von Mises on the upper stem area, 2: Stress Von Mises on the stem neck, 3: Von Mises stress in the conical hole of the head, 4: Section showing the distribution of contact stresses between stem and head).
Figure 12. FEM analysis results of the prosthesis with the stem head contact in the proximal zone. (1:Stress Von Mises on the upper stem area, 2: Stress Von Mises on the stem neck, 3: Von Mises stress in the conical hole of the head, 4: Section showing the distribution of contact stresses between stem and head).
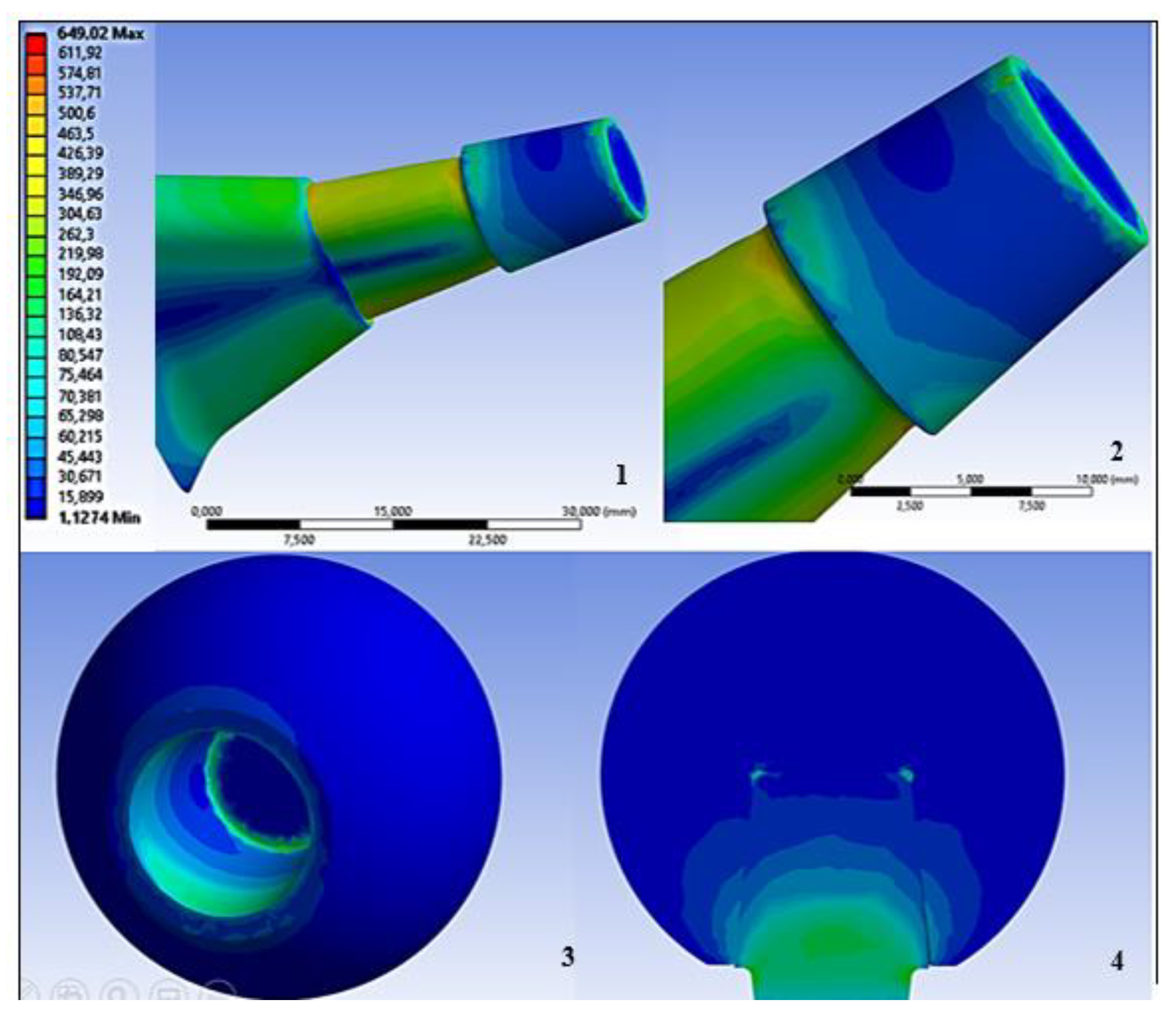
Preprints 79181 g010
We can also observe that distal contact induces less von Mises stress on the stem neck (484 Mpa) than (512 Mpa) if a distal contact is considered.

4. Discussion

In a laboratory study,[30] the stability of the connection was measured through torque-test and impact test. ISO 7206–13:2016. The torque test measures the torque that must be applied from the outside to compromise the stability of the cone morse connection. Figure 13
As can be seen from the experimental results Figure 14, a distal contact allows a higher Torque-off therefore improving the stability.
A numerical study was performed by Fallahnezhad et al. [32,36] with the aim of simulating abrasive wear depending on the contact situation, increased wear for the proximal contact situation was reported. Distal contact revealed less wear. In addition, a distal contact decreases the risk according to which physiological liquids can infiltrate the connection and compromise the repassivation of titanium with consequences on the increase in the corrosion rate. The current study investigated the influence of geometric conic features by FEM finite element analysis considering impact force. The contact state seems to affect stability. The complete contact situation, as can be seen from the result obtained in the laboratory, led to the lowest stability of the conical connection compared to distal and proximal contact situations. With a greater conical angular difference (proximal or distal contact situation), contact occurs over a smaller area leading to locally increased contact pressure. A possible explanation for the increased resistance in the distal contact situation could be that the contact area is distally larger than proximal due to the conical shape of the connection. Another numerical study was conducted by Fallahnezhad et al. [35] with the aim of simulating abrasive wear depending on the contact situation (Fallahnezhad et al.,)[34] They reported increased wear due to the proximal contact situation. Distal contact revealed less wear. For this study, we used the Von Mises results to analyze the connection. The von Mises stress is a parameter used to assess the state of stress within a material or structure. In the case of the connection between the head and the femoral stem of a prosthesis, von Mises stress can be used to assess the state of stress at the interface between the two components. Finite element analysis (FEA) can be used to calculate von Mises stress in the femoral head-stem connection. During the analysis, the three-dimensional model of the prosthesis, including the geometries of the head and stem, is discretized into finite elements and subjected to appropriate loads and constraint conditions. FEA analysis results provide information on von Mises stress at the interface between the head and the femoral stem. This parameter indicates the equivalent stress within the material and can be used to assess the level of stress and the resistance of the interface to deformation or fracture. Proper design, the femoral head-stem connection aims to minimize von Mises stress to ensure even distribution of stresses and reduce the risk of interface failure or impairment. It is important to consider the mechanical properties of the materials used for the head and stem, as well as the shape and size of the interface, in order to optimize the strength and durability of the connection. The von Mises stress assessment can be combined with other critical factors, such as applied load, constraint conditions, material properties and geometric features, to ensure proper design and accurate evaluation of the performance of the femoral head-stem connection in the prosthesis.
An increase in tension in contact between the stem and the femoral head of a prosthesis can be caused by several factors, including:
Geometric mismatch: If the geometry of the head and stem is not perfectly matched, for example due to dimensional or shape differences, a concentrated load or uneven distribution of stress at the point of contact may occur. High applied loads: If the prosthesis is subjected to high physical loads or high-impact activities, there may be an increase in tension in the contact between the stem and the femoral head. Tension concentration: The presence of surface irregularities, defects or discontinuities in the material or contact surface can create tension concentration points, which can lead to increased local voltages. Incompatible materials: The use of materials with different mechanical properties, such as a difference in elastic modulus or coefficient of thermal expansion, can cause an uneven discharge of tensions and an increase in local stresses in the contact. Inadequate lubrication: Inadequate lubrication between the stem and femoral head can increase friction and generate high tensions in the contact. To cope with increased tensions in femoral stem-head contact, several strategies can be adopted, including:
Design optimization: Redesign the geometry of the head and stem to ensure better matching and distribution of tensions in the contact.
Material improvement: Use materials with compatible mechanical properties and high fatigue resistance to reduce the risk of high stresses.
Improved contact surfaces: Implement surface treatments or special coatings to reduce friction and improve contact between the stem and the femoral head.
Proper lubrication: Ensure that there is adequate lubrication between the contact surfaces to reduce friction and tensions in the contact.

5. Conclusions

Breaking the contact between the head and the femoral stem of a prosthesis is a serious complication and can cause the prosthesis to fail. This situation can result from several factors, including: [16,17]
Overload: Applying excessive or unexpected loads on the prosthesis can overcome the resistance of contact between the head and the femoral stem, leading to rupture.
Material defects: If the material used for the head or stem has defects or imperfections, such as cracks or inclusions, this can weaken contact and increase the risk of breakage.
Inadequate design: A non-optimized prosthesis design, such as inappropriate head or stem geometry, can contribute to tension concentration and contact breakage.
Lack of geometric fit: Poor geometric adaptability between the head and the femoral stem can cause an uneven discharge of tension during physical activity, increasing the risk of rupture.
Weakness of the interface: If the interface between the head and the femoral stem has not been properly prepared or if cementation or fixation has not been performed correctly, the interface may be weak and lead to contact breakage.
To mitigate the risk of rupture of head-to-stem femoral contact, appropriate precautions are required during the design, fabrication and implantation phase of the prosthesis. These include:
Optimized design: A carefully thought-out design that takes into account the tensions and forces at play during physical activity can help minimize concentrated stress and improve contact duration.
Material selection: Using materials with high strength and reliability, such as titanium or cobalt-chromium alloys, can improve contact resistance and reduce the risk of breakage.
Quality control: Thorough verification and control of the materials used, as well as the manufacturing and installation processes, are essential to ensure the conformity and quality of the femoral head-stem contact.
Proper fixation procedures: Implementing effective fixation methods, such as cementation or the use of appropriate anchoring systems, can help ensure stable and durable contact between the head and the femoral stem.
A comparative analysis of the tensions exchanged at the femoral prosthesis head neck interface using the FEM method is presented. This investigation is conducted in the event that the head is pressed to the stem with a force of 4000N. [23,24,25,27,32]The results of the study also compared with a study carried out in a laboratory that evaluates the stability of the system using the torque-off test technique indicate in both tests that the distal contact is the one that guarantees greater stability even if the femoral stem is more stressed in flexion than a contact in the proximal area. Furthermore, a study [30] shows that distal contact is the one that guarantees a lower rate of wear and less infiltration of physiological liquids that can compromise the stability of the system triggering corrosion phenomena. The limitation of this study lies in the fact that it is not possible to study the effect of roughness to finite elements as the results are dependent on the type of contact that is simulated numerically, and the size of the mesh used. Finite element analysis (FEA) offers an important methodology for assessing the contact between the head and the femoral stem of a prosthesis. However, it is important to be aware of the possible limitations associated with using this technique in the analysis of femoral head-stem contact. Some of the main limitations include:
Simplified geometry: In FEA analysis, it is often necessary to simplify the complex geometry of the femoral head-stem contact to make the model computationally manageable. This can lead to an approximate representation of the actual geometry, which could affect the accuracy of the results.[29,35] Materials and properties: The choice of materials used in FEA modeling of femoral head-stem contact is crucial. However, achieving the accurate mechanical properties of prosthetic materials can be a challenge, as they can vary depending on the supplier or manufacturing process. Using inaccurate property values can affect the results of the analysis. Contact interface: Modelling the contact interface between the head and the femoral stem requires the assignment of a suitable contact pattern. However, choosing the contact model and associated parameters can be complex and can affect the results of the analysis. In addition, the effect of lubrication or coating effects on the interface may not be fully represented in FEA analysis. Loads and constraints: Assigning loads and constraints in FEA analysis of femoral head-stem contact can be challenging. Accurately determining the loads acting on the hip joint during daily activities can be complex. In addition, applying the correct constraints to simulate anchoring the femoral stem to the bone may require an accurate representation of the bone-stem interface. [36,37,38] Experimental verification: The FEA analysis of the femoral head-stem contact must always be accompanied by an experimental verification. The results of the FEA analysis should be compared with experimental data, such as laboratory tests or clinical trials, to ensure the accuracy and validity of the results. Despite these limitations, FEA analysis remains a valuable tool for understanding and optimizing femoral head-stem contact in prosthetics. It is important to carefully consider these limitations and adopt an integrated approach that includes both FEA analysis and experimental verification to obtain a complete and accurate assessment of the interaction between the head and the femoral stem. Future studies could numerically simulate the physiological environment in terms of Ph coefficient of friction and temperature as well as the effect that the variation of head material has on the exchange of tensions on contact.

Author Contributions

Conceptualization, M.C. and B.T.; methodology, M.C.; software, M.C.; validation, B.T., G.S. formal analysis, M.C. and B.T.; investigation, M.C.; resources, M.C. and B.T.; data curation,M.C.; writing—original draft preparation, M.C. and B.T.; writing—review and editing,M.C, B.T, G.S, D.C..; visualization, B.T, G.S..; supervision, G.S. and B.T.; project administration, G.S. and B.T. All authors have read and agreed to the published version of the manuscript.

Funding

This research received no external funding.

Institutional Review Board Statement

Not applicable.

Informed Consent Statement

Not applicable.

Data Availability Statement

All experimental data to support the findings of this study are available contacting the corresponding author upon request.

Acknowledgments

None.

Conflicts of Interest

The authors declare no conflict of interest.

References

  1. Sharkey, P.F.; Austin, M.S.; Hozack, W. Total hip arthroplasty in the young patient. Instr. Course Lect. 2006, 55, 173–176. [Google Scholar] [PubMed]
  2. Friedman, R.J.; Black, J.; O Galante, J.; Jacobs, J.J.; Skinner, H.B. Current concepts in orthopaedic biomaterials and implant fixation. Instr. Course Lect. 1994, 43. [Google Scholar] [CrossRef]
  3. et al. K Osman et al. _Corrosion at the head-neck interface of current designs of modular femoral components: essential questions and answers relating to corrosion in modular head{neck junctions_. In: The bone & joint journal 98.5 (2016, pp. 579,584). [CrossRef]
  4. Nicola Punzi. _Contact analysis in “hard-on-hard” hip replacements_. University of Pisa, Department of Information Engineering, Master’s Degree in Biomedical Engineering, 2013.
  5. Graichen Bergmann, F Graichen e A Rohlmann. _Hip joint loading during walking and running, measured in two patients_. In: Journal of biomechanics 26.8 (1993),pp. 969,990. [CrossRef]
  6. Bergmann, G.; Deuretzbacher, G.; Heller, M.; Graichen, F.; Rohlmann, A.; Strauss, J.; Duda, G. Hip contact forces and gait patterns from routine activities. J. Biomech. 2001, 34, 859–871. [Google Scholar] [CrossRef]
  7. Bech, N.H.; Haverkamp, D. Impingement around the hip: beyond cam and pincer. EFORT Open Rev. 2018, 3, 30–38. [Google Scholar] [CrossRef] [PubMed]
  8. Vassalou, E.E.; Zibis, A.H.; Klontzas, M.E.; Karantanas, A.H. Imaging of Impingement Syndromes around the Hip Joint. HIP Int. 2017, 27, 317–328. [Google Scholar] [CrossRef]
  9. Achar, S.; Yamanaka, J. Apophysitis and Osteochondrosis: Common Causes of Pain in Growing Bones. Am. Fam. Physician 2019, 99, 610–618. [Google Scholar] [PubMed]
  10. Garcia-Montoya, L.; Gul, H.; Emery, P. Recent advances in ankylosing spondylitis: understanding the disease and management. F1000Research 2018, 7, 1512. [Google Scholar] [CrossRef] [PubMed]
  11. Thomas, G.P.; Brown, M.A. Genetics and genomics of ankylosing spondylitis. Immunol. Rev. 2010, 233, 162–180. [Google Scholar] [CrossRef]
  12. et al. _Hip arthroplasty: comparison between standard access route, side access with mini incision and minimally invasive anterior access_. In: Giornale Italiano di Ortopedia e Traumatologia 32 (2006).
  13. Gianluca Grandi. _Hip lining arthroplasty with coupling metal-metal: 142 consecutive cases at a minimum follow-up of 5 years_. University of the Bologna studies, 2011.
  14. Matteo Sacchetti. _Biomaterials for the realization of hip and knee prostheses University of the Bologna studios, 2017.
  15. Tsikandylakis, G.; Mohaddes, M.; Cnudde, P.; Eskelinen, A.; Kärrholm, J.; Rolfson, O. Head size in primary total hip arthroplasty. EFORT Open Rev. 2018, 3, 225–231. [Google Scholar] [CrossRef]
  16. Isao Matsushita et al. _Activities of daily living after total hip arthroplasty. Is a 32-mm femoral head superior to a 26-mm head for improving daily activities_ In: International orthopaedics 35.1 (2011), pp. 25{29. [CrossRef]
  17. Delay, C.; Putman, S.; Dereudre, G.; Girard, J.; Lancelier-Bariatinsky, V.; Drumez, E.; Migaud, H. Is there any range-of-motion advantage to using bearings larger than 36 mm in primary hip arthroplasty: A case-control study comparing 36-mm and large-diameter heads. Orthop. Traumatol. Surg. Res. 2016, 102, 735–740. [Google Scholar] [CrossRef]
  18. Pijls, B.G.; Meessen, J.M.T.A.; Tucker, K.; Stea, S.; Steenbergen, L.; Fenstad, A.M.; Mäkelä, K.; Stoica, I.C.; Goncharov, M.; Overgaard, S.; et al. MoM total hip replacements in Europe: a NORE report. EFORT Open Rev. 2019, 4, 423–429. [Google Scholar] [CrossRef] [PubMed]
  19. Sevi B Kocagoz et al. _Does taper angle clearance inuence fretting and corrosion damage at the head{stem interface A matched cohort retrieval study_. In: Seminars in arthroplasty. Vol. 24. 4. Elsevier. 2013, pp. 246{254. [CrossRef]
  20. Kao, Y.-Y.J.; Koch, C.N.; Wright, T.M.; Padgett, D.E. Flexural Rigidity, Taper Angle, and Contact Length Affect Fretting of the Femoral Stem Trunnion in Total Hip Arthroplasty. J. Arthroplast. 2016, 31, 254–258. [Google Scholar] [CrossRef] [PubMed]
  21. Goldberg JR, Gilbert JL. Electrochemical response of CoCrMo to high-speed fracture of its metal oxide using an electrochemical scratch test method. J Biomed Mater Res 1997;37(3):421–431. PubMed PMID: 9368147. Epub 1997/11/22. Eng. [CrossRef]
  22. Goldberg JR, Gilbert JL, Jacobs JJ, Bauer TW, Paprosky W, Leurgans S. A multicenter retrieval study of the taper interfaces of modular hip prostheses. Clin Orthop Related Res 2002;401:149– 161. PubMed Epub 2002/08/02. eng. [PubMed]
  23. Gilbert, J.L.; Buckley, C.A.; Jacobs, J.J. In vivo corrosion of modular hip prosthesis components in mixed and similar metal combinations. The effect of crevice, stress, motion, and alloy coupling. J. Biomed. Mater. Res. 1993, 27, 1533–1544. [Google Scholar] [CrossRef] [PubMed]
  24. Lundberg, H.J.; Ha, N.Q.; Hall, D.J.; Urban, R.M.; Levine, B.R.; Pourzal, R. Contact Mechanics and Plastic Deformation at the Local Surface Topography Level After Assembly of Modular Head-Neck Junctions in Modern Total Hip Replacement Devices. 2015, 59–82. [CrossRef]
  25. Ashkanfar, A.; Langton, D.J.; Joyce, T.J. A large taper mismatch is one of the key factors behind high wear rates and failure at the taper junction of total hip replacements: A finite element wear analysis. J. Mech. Behav. Biomed. Mater. 2017, 69, 257–266. [Google Scholar] [CrossRef] [PubMed]
  26. Choroszyński, M.R.; Skrzypek, S.J. Biomaterials for hip implants – important considerations relating to the choice of materials. bams 2017, 13, 133–145. [Google Scholar] [CrossRef]
  27. Maruyama, N.; Kawasaki, H.; Yamamoto, A.; Hiromoto, S.; Imai, H.; Hanawa, T. Friction-Wear Properties of Nickel-Free Co–Cr–Mo Alloy in a Simulated Body Fluid. Mater. Trans. 2005, 46, 1588–1592. [Google Scholar] [CrossRef]
  28. Co-Cr-Mo Alloy in a Simulated Body Fluid. Mater. Trans. 2005, 46, 1588–1592. [CrossRef]
  29. OrthoLoad Loading of the Orthopaedic Implant. Available online: https://orthoload.com/ (accessed on 15 October 2022).
  30. Fallahnezhad, K.; Farhoudi, H.; Oskouei, R.H.; Taylor, M. Influence of geometry and materials on the axial and torsional strength of the head–neck taper junction in modular hip replacements: A finite element study. J. Mech. Behav. Biomed. Mater. 2016, 60, 118–126. [Google Scholar] [CrossRef]
  31. Fallahnezhad, K.; Farhoudi, H.; Oskouei, R.H.; Taylor, M. Influence of geometry and materials on the axial and torsional strength. [CrossRef]
  32. Feyzi, M.; Fallahnezhad, K.; Taylor, M.; Hashemi, R. A review on the finite element simulation of fretting wear and corrosion in the taper junction of hip replacement implants. Comput. Biol. Med. 2020, 130, 104196. [Google Scholar] [CrossRef] [PubMed]
  33. ANSYS Meshing User’s Guide; Ansys: Canonsburg, PA, USA, 2013.
  34. Fallahnezhad, K.; Feyzi, M.; Hashemi, R.; Taylor, M. The Role of the Assembly Force in the Tribocorrosion Behaviour of Hip Implant Head-Neck Junctions: An Adaptive Finite Element Approach. Bioengineering 2022, 9, 629. [Google Scholar] [CrossRef] [PubMed]
  35. Alkhatib, S.E.; Mehboob, H.; Tarlochan, F. Finite Element Analysis of Porous Titanium Alloy Hip Stem to Evaluate the Biomechanical Performance During Walking and Stair Climbing. J. Bionic Eng. 2019, 16, 1103–1115. [Google Scholar] [CrossRef]
  36. Performance DuringWalking and Stair Climbing. J. Bionic Eng. 2019, 16, 1103–1115.
  37. The Fundamentals of FEA Meshing for Structural Analysis; Ansys: Canonsburg, PA, USA, 2021; Volume 2022.
  38. Feyzi, M.; Fallahnezhad, K.; Taylor, M.; Hashemi, R. A review on the finite element simulation of fretting wear and corrosion in the taper junction of hip replacement implants. Comput. Biol. Med. 2020, 130, 104196. [Google Scholar] [CrossRef] [PubMed]
Preprints 79181 g001
Figure 1. Coupling between morse cone and head.
Figure 1. Coupling between morse cone and head.
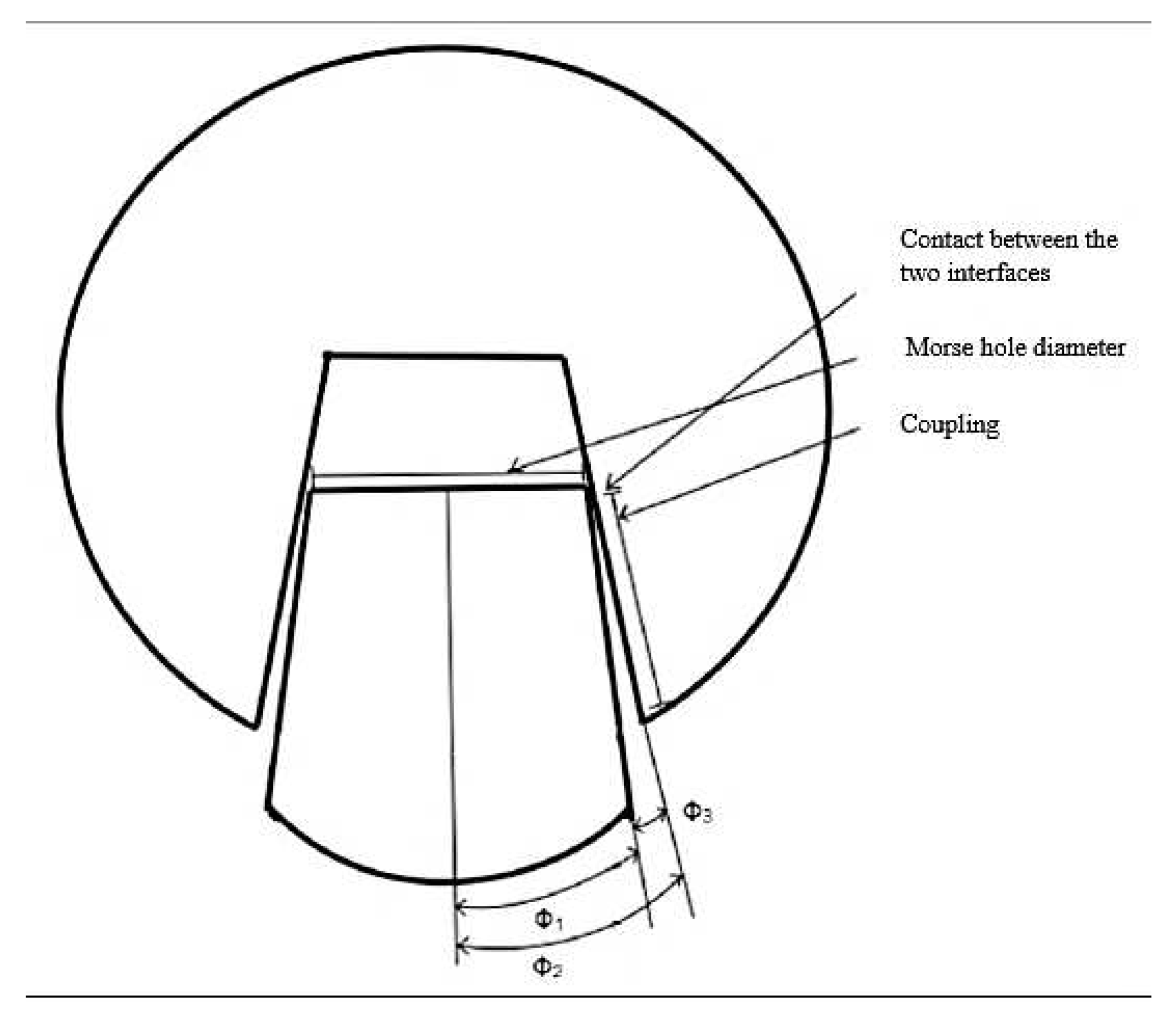
Preprints 79181 g001
Preprints 79181 g002
Figure 2. Geometric parameters of conical contact (a) proximal contact(b), distal contact (c).
Figure 2. Geometric parameters of conical contact (a) proximal contact(b), distal contact (c).
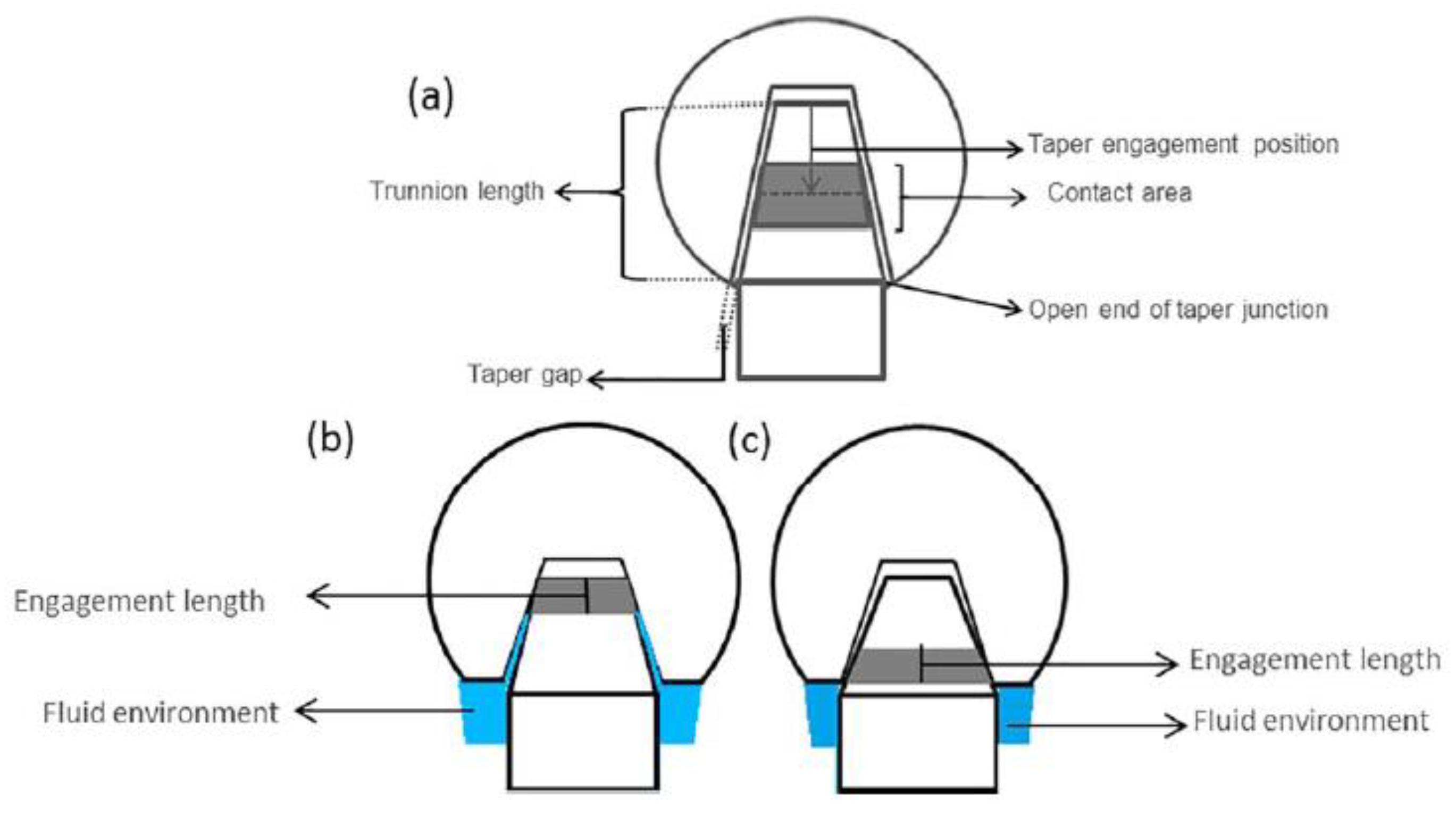
Preprints 79181 g002
Preprints 79181 g003
Figure 3. Increased range of motion for a smaller cone (a) compared to a wider cone (b).
Figure 3. Increased range of motion for a smaller cone (a) compared to a wider cone (b).
Preprints 79181 g003
Preprints 79181 g004
Figure 4. Left model with proximal contact right model with distal contact.
Figure 4. Left model with proximal contact right model with distal contact.
Preprints 79181 g004
Preprints 79181 g005
Figure 5. Components of a femoral prosthesis.
Figure 5. Components of a femoral prosthesis.
Preprints 79181 g005
Preprints 79181 g006
Figure 6. Size of the prosthesis analysed in this study.
Figure 6. Size of the prosthesis analysed in this study.
Preprints 79181 g006
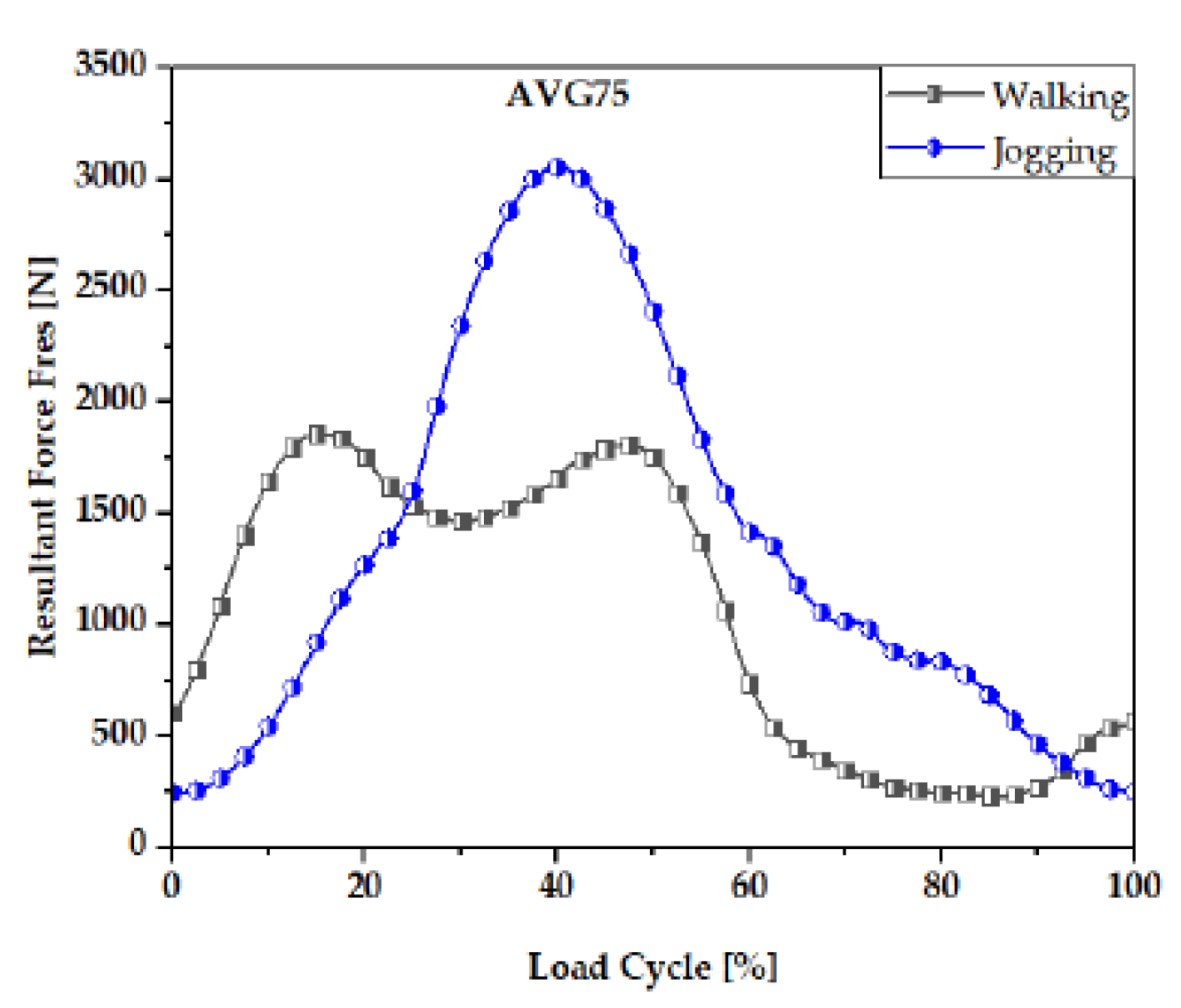
Preprints 79181 g007
Figure 7. Resultant Force, values for walking and jogging loading conditions.
Figure 7. Resultant Force, values for walking and jogging loading conditions.
Preprints 79181 g007
Preprints 79181 g008
Figure 8. On the left application of the load on the head, on the right tetrahedral mesh used.
Figure 8. On the left application of the load on the head, on the right tetrahedral mesh used.
Preprints 79181 g008
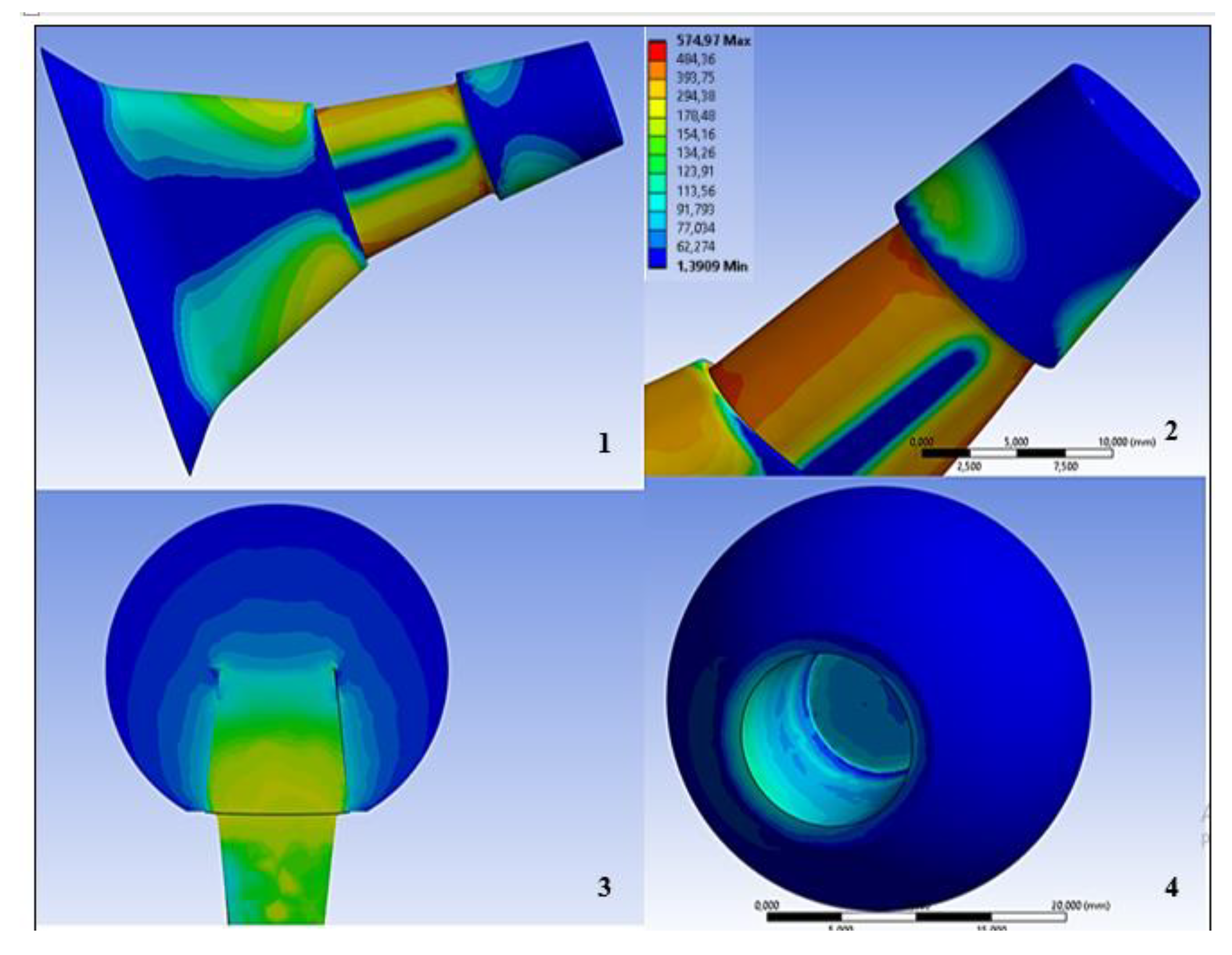
Preprints 79181 g009
Figure 11. Results of FEM analysis of the prosthesis with head and stem contact in the distal area. (1:Stress Von Mises on the upper stem area, 2: Stress Von Mises on the stem neck,3: Section showing the distribution of contact stresses between stem and head, 4: Von Mises stress in the conical hole of the head).
Figure 11. Results of FEM analysis of the prosthesis with head and stem contact in the distal area. (1:Stress Von Mises on the upper stem area, 2: Stress Von Mises on the stem neck,3: Section showing the distribution of contact stresses between stem and head, 4: Von Mises stress in the conical hole of the head).
Preprints 79181 g009
Preprints 79181 g011
Figure 13. Investigation procedure for determining the taper connection strength: starting with the fixation of the implant components (left), then the impaction of the two taper components (middle) and finally the torque-off test (right).
Figure 13. Investigation procedure for determining the taper connection strength: starting with the fixation of the implant components (left), then the impaction of the two taper components (middle) and finally the torque-off test (right).
Preprints 79181 g011
Preprints 79181 g012
Figure 14. Influence of the contact situation on the taper strength when using metal heads.
Figure 14. Influence of the contact situation on the taper strength when using metal heads.
Preprints 79181 g012
Table 1. Mechanical characteristics used for the study. [25].
Table 1. Mechanical characteristics used for the study. [25].
Elastic Modulus (Gpa) Yield Strength (Mpa) Ultimate Strength (Mpa) Density (kg/m3)
Ti6Al4V 110 910 1000 4400
Co-Cr 220 840 1280 8500
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content.
Copyright: This open access article is published under a Creative Commons CC BY 4.0 license, which permit the free download, distribution, and reuse, provided that the author and preprint are cited in any reuse.
Prerpints.org logo

Preprints.org is a free preprint server supported by MDPI in Basel, Switzerland.

facebook logotwitter logolinkedin logo
MDPI Initiatives
Important Links
Subscribe

Disclaimer

Terms of Use

Privacy Policy

© 2024 MDPI (Basel, Switzerland) unless otherwise stated