Preprint
Article
Large-Range Switchable Asymmetric Transmission and Circular Conversion Dichroism in a VO2 Based Metasurface
Altmetrics
Downloads
131
Views
21
Comments
0
A peer-reviewed article of this preprint also exists.
This version is not peer-reviewed
Submitted:
13 July 2023
Posted:
14 July 2023
You are already at the latest version
Alerts
Abstract
Reconfigurable chiral metasurfaces with dynamic polarization manipulation capability are highly required in optical integrated systems. In this paper, we simultaneously realized giant and large-range switchable asymmetric transmission (AT) and circular conversion dichroism (CCD) in a vanadium dioxide (VO2) based metasurface. The AT and CCD of the insulator VO2 based metasurface reach 0.95 and 0.92, respectively. Utilizing the insulator-to-metallic phase transition of VO2, the AT and CCD could be continuously switched to near zero. Furthermore, the physics mechanism of the giant and switchable AT and CCD are analyzed. The proposed metasurface with large-range switchable AT and CCD is promising in the applications of biochemistry detection, chiral imaging and biosensing.
Keywords:
Subject: Physical Sciences - Optics and Photonics
1. Introduction
Chirality is defined as the characteristic for one object cannot be coincided with its mirror image [1], which has broad potential application prospects in chiral coding [2], analytical chemistry [3], chiral biosensing [4] and so on. Chiral effects, circular birefringence (CB) and circular dichroism (CD), are first found in three-dimension spiral structures while interacting with circular polarized light (CPL) [5]. However, these chiral effects are relatively weak in nature materials. Differently, chiral metasurfaces, artificially designed subwavelength optical structures, possess excellent performance in enhancing CB and CD. With the development of chiral metasurfaces, the two-dimension chiral effects of asymmetric transmission (AT) and circular conversion dichroism (CCD) are also obtained in planar symmetry broken structures [6]. Up to now, metasurfaces have been widely used to realize intrinsic [6] and extrinsic chirality [7] by breaking the symmetry of unit structure and oblique incidence, respectively. Unlike the extrinsic chirality at the expense of oblique incidence, intrinsic chirality could be realized in symmetry broken structures under normal incidence. For instance, Ahsan Sarwar Rana and Inki Kim et al. realized both AT (0.58) and CCD (0.55) in an asymmetric planar structure [8]. Similarly, both AT (0.8) and CD (0.88) are realized in a Z-shape germanium metasurface [6]. Although giant 2D chirality has been realized, the chiral effects of these metasurfaces are fixed once the structures are fabricated [9].
To attain dynamic chiral effects, some active materials including 2D materials graphene [10,11] and black phosphorus [7], phase change materials Ge2Sb2Te5 (GST) [12,13] and VO2 [14,15], liquid crystal [16] and ultrathin TiN films [17] are applied in traditional static metasurfaces. Among them, phase-change material VO2 exhibits obvious phase transition behavior under the excitations of thermal [18], electrical [19,20] or optical [19] stimuli. With the phase transition from insulator to metallic state, the conductivity of VO2 will change 4-5 orders of magnitudes. Besides, the lower phase-transition temperature (68 ) of VO2 than that of GST (160 ) [12] shows the advantage of lower energy consumption. Benefit from these advantages, VO2 has applied to construct reconfigurable chiral metasurfaces. Lv et al. designed a bilayer twisted E-shape structure integrated with VO2 and achieved strength-switchable AT and CD (0.6/0.45 ~0) [21]. Meng Liu et al. realized dynamic AT and CCD in a VO2 based split rings structure and the AT/CCD ranges from 0.12/0.75 to near zero [22]. Though the strength-switchable AT and CCD have been realized, the switching ranges of these chiral effects are not broad enough, which will limit the application of chiral metasurfaces.
In this work, the giant and strength-switchable 2D chiral effects of AT and CCD are simultaneously achieved in a VO2 based metasurface in THz region. The metasurface allows the transmission of RCP wave propagating along -z direction (forward) and prevents the propagation of RCP in z direction (backward), exhibiting AT effect as shown in Figure 1 (a). Meanwhile, the giant difference of cross-polarization reflection of LCP and RCP light indicates the existence of giant CCD in Figure 1(b). The maximum values of AT and CCD of the insulator VO2 based metasurface are 0.95 and 0.92 at 0.69 THz. Furthermore, by thermally controlling the phase of VO2, AT/CCD could be dynamically switched from 0.95/0.92 to near zero. The strength and the switching range of AT and CCD are much improved compared with previously reported works. The proposed metasurface with giant and switchable 2D chirality will promote the applications in optical imaging and chiral biosensing etc.
2. Design and simulations
It is widely used to break the structures’ geometric symmetry in the wave propagation direction to generate chirality [23,24]. To break the symmetry of our structure, asymmetric G-shape and J-shape Au resonators are placed on the opposite sides of the VO2 inserted polymer layer as shown in Figure 2. When VO2 is insulator state, the incident CPL could polarization selectively penetrate and realize giant chiral effect. In contrast, the both incident CPL will be blocked when the VO2 phase is changed to metallic state and show very weak chiral effect. Thus, utilizing the thermal tuning the properties of VO2, the chiral effect of the VO2 based reconfigurable metasurface could be modulated dynamically. The structure of the period array based on VO2 with giant chiral effect is shown in Figure 2 (a). The structure marked by dashed frame in Figure 2 (a) is the unit structure. The top layer is the 90° rotated G-shaped Au resonator and the bottom layer is the 180° rotated J-shaped Au resonator as shown in Figure 2(c)(d). After optimization, the thickness of the Au resonators in both top and bottom layers is the same as . The thickness of the separated polymers and inserted VO2 layer are and as labeled in Figure 2 (b).
The optical property of Au substrate could be described by Drude model and the conductivity is set as [21]. The polymer spacer is regarded as lossy dielectric material with the permittivity of 3.5 + 0.00945i [25]. With the temperature change from room temperature to phase-transition temperature , Drude model is used to characterize the permittivity of VO2 in THz region as [18]
where is permittivity at infinite frequency. The permittivity of the VO2 is determined by plasma frequency (,). Thus, the optical properties of VO2 under different phases could be described by conductivities. Specially, with the insulator-to-metallic transition of VO2, the conductivity of VO2 increases from 200 s/m to 2×105 s/m. In simulation, we numerically calculated the transmission and reflection spectra by using finite element method (FEM) in frequency domain in CST Microwave Studio. The periodic boundary conditions are used in x and y directions and open boundary is applied in z direction. Mesh is set as adaptive tetrahedral grids.
3. Giant and switchable asymmetric transmission
The polarization transmission coefficient spectra of CPL propagating forward and backward are numerically calculated in Figure 3(a)(b). The four transmission coefficients of the CPL propagating forward are not coincided with each other. The right-to-left polarization transmission coefficient () and right-to-right one () are higher than the other two coefficients ( and ) in the band of 0.65 - 0.8 THz as shown in Figure 3(a). Specially, the maximum values of RCP transmission coefficients and reach 0.729 and 0.649 at 0.69 THz, while the LCP ones ( and ) approach to 0. Since the change of propagation direction will result in the exchange of two enantiomeric arrangements, the subscripts “+” and “-” representing RCP and LCP will be exchanged, namely ,,, and . The polarization transmission coefficients of CPL propagating in opposite directions are shown in Figure 3(b). The directions propagating forward and backward in transmission coefficients are represented by arrows “→” and “←”, respectively. As expected, the co-polarization transmission coefficients propagating backward are the same as the forward ones . Similarly, the cross-polarization transmission coefficients propagating backward and the forward ones are also the same.
The transmission difference of the CPL propagating forward and backward is described as AT phenomenon [5]. To describe the transmission difference propagating in opposite directions, the transmission of CPL propagating forward and backward are separately calculated in Figure 4(c)(d). The polarization transmissions are derived from polarization transmission coefficients as and . The transmission of RCP () propagating forward are very high and the value of the value at peak is about 0.95 at 0.69 THz, but the ones of LCP maintains low values and the dip value even approach to zero in Figure 3(c). As expected, the transmission of RCP/LCP light propagating forward is equal to the transmission of LCP/RCP propagating backward (,) as the curves shown in Figure 3(d). The transmission difference of RCP light propagating forward and backward in Figure 3(c)(d) means the incident RCP light could transmit our metasurface forward but the RCP light from opposite direction will be blocked.
To estimate the giant AT performance quantitatively, the AT parameters of RCP and LCP light ( and ) are defined by the polarization transmission differences as [26]
As shown in Figure 3(e), the maximum AT parameter of RCP () reaches 0.95 at 0.69 THz. Since the inversion relationship between polarization states and propagation direction, the AT parameters of LCP and RCP light are negative of each other (). The maximum absolute value of AT parameters for LCP is at 0.69 THz with Thus, the metasurface with giant AT parameter allows near perfect transmission of RCP light propagating forward and prevents that of LCP, which is corresponding to the RCP light propagating backward.
By controlling the phase of VO2, the strength of AT effect could be continuously switched. As shown in Figure 3(f), when VO2 is in insulator state (200 S/m), the metasurface exhibits giant AT effect (0.95) at 0.69 THz. But the AT will be disappeared when VO2 is transformed into metallic state (2×105 S/m). The strength of AT could be dynamically switched in the broad switching range from 0.95 to 0.05. The VO2 based chiral metasurface with giant and large-range switchable AT shows great potential in the fields of optical integrated system.
4. Giant and switchable circular conversion dichroism
Not only giant AT effect, the same nanostructure also shows great polarization selectivity for the reflection CPL. The reflection coefficient spectra of the CPL propagating forward and backward are displayed in Figure 4(a)(b). The co-polarized refection coefficients of both CPL propagating in two opposite directions are equal ( and ) at 0.5-0.87 THz. Differently, the cross-polarized reflection coefficients are totally different. In detail, the left-to-right polarization reflection coefficient is much larger than the one of right-to-left one in Figure 4(a). Especially, the is as large as 0.96 at 0.69 THz while approaches to zero at the same frequency, which indicates the giant polarization selectivity of reflected light. In contrast, for the reflected light incident backward, the difference between cross-polarized reflection coefficients is not remarkable as shown in Figure 4(b).
To quantitatively characterize the giant difference of the cross-polarization reflection coefficients, CCD is calculated in Figure 4(c), which is defined by the difference of and as [22]. The curves of and are not coincided in the whole frequency band and the maximum difference occurs at 0.69 THz with the values of and respectively. The maximum of CCD reaches 0.92 at 0.69 THz. The high CCD represents the giant difference between the cross-polarization conversion of incident LCP and RCP light.
The strength of CCD could be dynamically switched in the VO2 based active metasurface. By unitizing the phase-transition of VO2, the strength of CCD of metasurface is continuously controlled from 0.92 to ~0 at 0.69 THz in Figure 4(d). In details, with the conductivity increasing from 200 S/m (insulator VO2) to 2×105 S/m (metallic VO2), the CCD value is gradually decreased in a broad range at 0.69 THz. The broad-range and continuous switching for CCD is a major advance when compared with the previously reported works.
5. The principle of chiral effects
To explore the physic mechanism of AT and CCD, the electric field and surface current distributions of the chiral metasurface at 0.69 THz are given out in Figure 5. As mentioned, the metasurface with insulator VO2 exhibits different polarization responses for RCP and LCP light. The incident RCP light could penetrate metasurface as the homogeneous electric field distributed above and below the metasurface in Figure 5(a). In contrast, the incident LCP light will be reflected which leads to obvious difference of electric filed distributions above and below the metasurface as shown in Figure 5(b). The huge difference in transmission and reflection of RCP and LCP light exhibits remarkable AT and CCD effects.
To explain the mechanism of different responses for RCP and LCP light, the surface current distributions in the top and bottom layers are shown in Figure 5(e)(f)(i)(j). Under the excitation of RCP light, there are enhanced parallel and circular surface currents in the top layer as shown in Figure 5(e). In detail, the parallel surface current points to -y direction and the circular surface current flows clockwise. As known, electric dipole resonance is characterized by parallel surface current flowing from one pole to another pole and magnetic dipole resonance is characterized by circular surface currents [27]. Thus, with incidence of RCP light, the electric current marked by arrows shows the excitations of electric () and magnetic () dipole resonances in the top layer in Figure 5(e). Similarly, electric and magnetic dipole resonances could be simultaneously excited in the bottom layer (Figure 5(i)).
In contrast, with LCP light incidence, the electric and magnetic dipole resonances could also be excited in Figure 5(f)(j), but electric moment pointing in opposite directions as the black arrows marked in the both layers in comparisons (Figure 5(e) and Figure 5(f), Figure 5(i) and Figure 5(j)). Differently, the directions of electric moment is reversed at the same geometric position in the top and bottom layers under LCP excitation compared with RCP excitation. It is worth noting that the intensity of surface current under LCP excitation is generally enhanced than that of RCP excitation. Because the variation of resonance modes and surface current intensity will result in the different responses for RCP and LCP and generate giant AT and CCD in the metasurface with insulator VO2.
By changing the phase of VO2, both the values of AT and CCD could be continuously switched to near zero. When VO2 undergoes insulator-to-metallic transition, the incident CPL could hardly penetrate the metallic VO2 and excites very weak electric field and surface current in the bottom layer as shown in Figure 5(g)(h)(k)(l). The difference of electric and magnetic resonance modes and surface current are unremarkable under the excitations of RCP and LCP light, which results in the weak response difference between RCP and LCP light. Thus, when the VO2 is changed to metallic state, the 2D chiral effect including AT and CCD almost could not be excited in the same nanostructure and achieves large-range dynamic switching.
Here, we compare the chiral performances of our chiral structure with previously reported works in Table 1. The first three works have realized prominent but fixed chiral effects. The latter two works could realize active manipulation for AT and CCD/CD by integrating VO2, but the switching range are not large enough. In contrast, not only the stronger AT (0.95)/CCD (0.92) are obtained but also the larger-range switching for 2D chirality from ~1 to ~0 are realized by controlling the phase states of VO2. The proposed chiral metasurfaces with simultaneous giant AT/CCD and broad range switching capability for chirality are essential for the applications of polarization filtering, polarization optical isolating and so on.
6. Conclusions
In conclusion, we numerically achieved giant and strength-switchable AT and CCD in a VO2 based chiral metasurface by breaking the geometric symmetry of structures in the wave propagation direction. The giant AT parameter (0.95) and CCD (0.92) are realized in THz region, which is much improved compared with the proposed chiral metasurfaces. Furthermore, the strength of the AT and CCD effect are large-range switched from ~ 1 to ~0 by thermally controlling VO2. The physical mechanism of the giant and reconfigurable AT and CCD are analyzed by surface current distributions. The giant chirality originates from the polarization selective excitations of electric and magnetic dipole resonances. The proposed active metasurface with broad switchable range for both AT and CCD will promote the development of integration chiral devices.
Supplementary Materials
The following supporting information can be downloaded at the website of this paper posted on Preprints.org. Figure 1. The dual functions of the proposed VO2, Figure 2. (a) The schematic illustration of the VO2 based chiral metasurface, Figure 3. Giant and switchable AT, Figure 4. Giant and switchable CCD effect. Figure 5. The electric field and surface current distributions at 0.69 THz.
Author Contributions
All authors contributed to the study’s conception and design. Theoretical analysis, structure simulation, date collection were performed by Songliang Zhao, Huan Jiang, Jingyu Wang, Wenchang Zhu and Weiren Zhao. Data processing and graph drawing were carried out Songliang Zhao and Huan Jiang. The first draft of manuscript is written by Songliang Zhao. All the authors read and approved the finial manuscript.
Funding
National Natural Science Foundation of China (12004080); Funding by Science and Technology Projects in Guangzhou (202201010540).
Data Availability
Data underlying the results presented in this paper are not publicly available at this time but may be obtained from the authors upon reasonable request.
Conflicts of Interest
The authors declare no conflict of interest.
References
- Hwang J, Song M H, Park B et al Electro-tunable optical diode based on photonic bandgap liquid-crystal heterojunctions. Nat Mater 2005, 4 383-387.
- Li Z, Jiang C, Wang K et al Active manipulation of Dirac semimetals supported chiral coding metasurfaces for multifunctional applications in terahertz region. Results in Physics 2023, 46.
- Valev V K, Baumberg J J, Sibilia C et al Chirality and chiroptical effects in plasmonic nanostructures: fundamentals, recent progress, and outlook. Adv Mater 2013, 25, 2517–2534. [CrossRef] [PubMed]
- Zhan P, Dutta P K, Wang P et al Reconfigurable Three-Dimensional Gold Nanorod Plasmonic Nanostructures Organized on DNA Origami Tripod. ACS Nano 2017, 11, 1172–1179. [CrossRef]
- L. D. Barron, Molecular light scattering and optical activity (Cambridge University Press, 2009).
- Ma Z, Li Y, Li Y et al All-dielectric planar chiral metasurface with gradient geometric phase. Opt Express 2018, 26, 6067–6078. [CrossRef]
- Zeng Y, Xu J, Xiao W et al Giant 2D-chiroptical response in an achiral metasurface integrated with black phosphorus. Opt Express 2022, 30, 8266–8274. [CrossRef] [PubMed]
- Rana A S, Kim I, Ansari M A et al Planar Achiral Metasurfaces-Induced Anomalous Chiroptical Effect of Optical Spin Isolation. ACS Appl Mater Interfaces 2020, 12, 48899–48909. [CrossRef]
- Shi T, Deng Z L, Geng G et al Planar chiral metasurfaces with maximal and tunable chiroptical response driven by bound states in the continuum. Nat Commun 2022, 13, 4111. [CrossRef]
- Cui Y, Wang X, Jiang H et al High-efficiency and tunable circular dichroism in chiral graphene metasurface. Journal of Physics D: Applied Physics 2021 55.
- Tao X, Qi L, Fu T et al A tunable dual-band asymmetric transmission metasurface with strong circular dichroism in the terahertz communication band. Optics & Laser Technology 2022, 150.
- Jiang H, Peng K Z, Cui Y et al Design and simulation of a GST-based metasurface with strong and switchable circular dichroism. Opt Lett 2022, 47, 1907–1910. [CrossRef]
- Dong G, Qin C, Lv T et al Dynamic chiroptical responses in transmissive metamaterial using phase-change material. Journal of Physics D: Applied Physics 2020, 53.
- Lv T, Li Y, Qin C et al Versatile polarization manipulation in vanadium dioxide-integrated terahertz metamaterial. Opt Express 2022, 30, 5439–5449. [CrossRef]
- Yang J-K, and Jeong H-S Switchable Metasurface with VO2 Thin Film at Visible Light by Changing Temperature. Photonics 2021, 8.
- Baranzadeh F, and Nozhat N High Performance Plasmonic Nano-Biosensor Based on Tunable Ultra-Narrowband Perfect Absorber Utilizing Liquid Crystal. Plasmonics 2020, 16, 253–262.
- Qin F, Chen X, Yi Z et al Ultra-broadband and wide-angle perfect solar absorber based on TiN nanodisk and Ti thin film structure. Solar Energy Materials and Solar Cells 2020, 211.
- Wen Q-Y, Zhang H-W, Yang Q-H et al Terahertz metamaterials with VO2 cut-wires for thermal tunability. Applied Physics Letters 2010, 97.
- Zhang C, Zhou G, Wu J et al Active Control of Terahertz Waves Using Vanadium-Dioxide-Embedded Metamaterials. Physical Review Applied 2019, 11.
- Butakov N A, Knight M W, Lewi T et al Broadband Electrically Tunable Dielectric Resonators Using Metal–Insulator Tranitions. ACS Photonics 2018, 5, 4056–4060. [CrossRef]
- Lv T T, Li Y X, Ma H F et al Hybrid metamaterial switching for manipulating chirality based on VO2 phase transition. Sci Rep 2016, 6 23186.
- Liu M, Plum E, Li H et al Switchable Chiral Mirrors. Advanced Optical Materials 2020, 8.
- Lv T, Chen X, Dong G et al Dual-band dichroic asymmetric transmission of linearly polarized waves in terahertz chiral metamaterial. Nanophotonics 2020, 9, 3235–3242. [CrossRef]
- Luo C, Sang T, Li S et al Stretchable Chiral Metamaterial for Flexible Control of Broadband Asymmetric Transmission. Plasmonics 2022, 18, 29–37.
- Hu F, Wang L, Quan B et al Design of a polarization insensitive multiband terahertz metamaterial absorber. Journal of Physics D: Applied Physics 2013, 46.
- Singh R, Plum E, Menzel C et al Terahertz metamaterial with asymmetric transmission. Physical Review B 2009, 80.
- Jiang H, Zhao W, and Jiang Y All-dielectric circular polarizer with nearly unit transmission efficiency based on cascaded tensor Huygens surface. Opt Express 2016, 24, 17738–17745. [CrossRef]
- Han B, Li S, Li Z et al Asymmetric transmission for dual-circularly and linearly polarized waves based on a chiral metasurface. Opt Express 2021, 29, 19643–19654. [CrossRef] [PubMed]
- Ren Y, Zhou T, Jiang C et al Thermally switching between perfect absorber and asymmetric transmission in vanadium dioxide-assisted metamaterials. Opt Express 2021, 29, 7666–7679. [CrossRef] [PubMed]
Figure 1.
The dual functions of the proposed VO2 based metasurface. (a) AT (b) CCD in reflection mode.
Figure 1.
The dual functions of the proposed VO2 based metasurface. (a) AT (b) CCD in reflection mode.
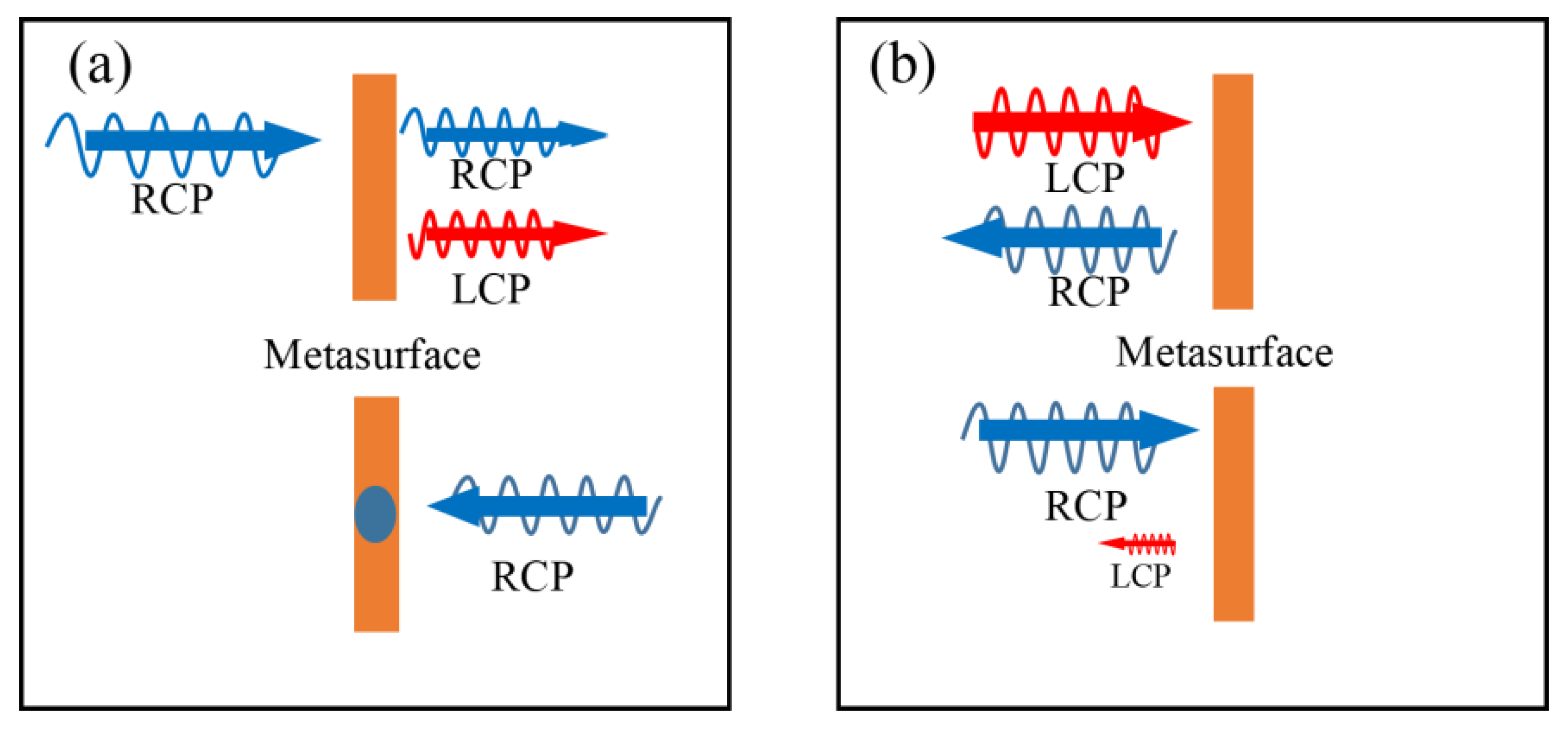
Figure 2.
(a) The schematic illustration of the VO2 based chiral metasurface. The (b) side, (c) top and (d) bottom views of the unit structure. The geometrical dimensions are , , , , , , , , , , .
Figure 2.
(a) The schematic illustration of the VO2 based chiral metasurface. The (b) side, (c) top and (d) bottom views of the unit structure. The geometrical dimensions are , , , , , , , , , , .

Figure 3.
Giant and switchable AT. The transmission coefficient spectra of the CPL propagating (a) forward and (b) backward. The transmission of RCP and LCP propagating (c) forward and (d) backward. (e) AT parameters of RCP and LCP light. (f) Strength-switchable AT.
Figure 3.
Giant and switchable AT. The transmission coefficient spectra of the CPL propagating (a) forward and (b) backward. The transmission of RCP and LCP propagating (c) forward and (d) backward. (e) AT parameters of RCP and LCP light. (f) Strength-switchable AT.

Figure 4.
Giant and switchable CCD effect. Reflection coefficients spectra incident (a) forward and (b) backward. (c) The cross-polarization reflection coefficients and CCD spectra. (d) The strength switchable CCD by tuning the phase of VO2.
Figure 4.
Giant and switchable CCD effect. Reflection coefficients spectra incident (a) forward and (b) backward. (c) The cross-polarization reflection coefficients and CCD spectra. (d) The strength switchable CCD by tuning the phase of VO2.

Figure 5.
The electric field and surface current distributions at 0.69 THz. With (a)(b)(e)(f)(i)(j) insulator and (c)(d)(g)(h)(k)(l) metallic VO2, (a)-(d) the electric fields at the side layers and (e)-(l) surface current at the (e)-(h) top and (i)-(l) bottom layers excited by (a)(e)(i)(c)(g)(k) RCP and (b)(f)(j) (d)(h)(l) LCP light.
Figure 5.
The electric field and surface current distributions at 0.69 THz. With (a)(b)(e)(f)(i)(j) insulator and (c)(d)(g)(h)(k)(l) metallic VO2, (a)-(d) the electric fields at the side layers and (e)-(l) surface current at the (e)-(h) top and (i)-(l) bottom layers excited by (a)(e)(i)(c)(g)(k) RCP and (b)(f)(j) (d)(h)(l) LCP light.

Table 1.
The comparison with reported chiral metasurfaces.
| Nano-structures | Bandwidths | AT | CCD/CD | Switching capability |
Active materials | Years |
|---|---|---|---|---|---|---|
| Nano-fifin design [8] | 500-800 nm | 0.58 | CCD=0.55 | No | / | 2020 |
| Z-shape [28] | 3.0-8.0 GHz | 0.38 | CD=0.75 | No | / | 2021 |
| Twisted S-shaped structure [23] | 0.4-2.0 THz | 0.73 | CD=0.28 | No | / | 2020 |
| Double-layered arcs [29] | 0.8-1.5 µm | 0.15 | CD=0.7 | Yes | VO2 | 2021 |
| Split rings [22] | 0.4-0.8 THz | 0.12 | CCD=0.75 | Yes | VO2 | 2020 |
| “G”- and “J”- shaped hybrid structure | 0.5-1.0 THz | 0.95 | CCD=0.92 | Yes | VO2 | This work |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Copyright: This open access article is published under a Creative Commons CC BY 4.0 license, which permit the free download, distribution, and reuse, provided that the author and preprint are cited in any reuse.
MDPI Initiatives
Important Links
© 2024 MDPI (Basel, Switzerland) unless otherwise stated






