Preprint
Article
The Random Vibrations of the Active Body of the Cultivators
Altmetrics
Downloads
115
Views
44
Comments
0
A peer-reviewed article of this preprint also exists.
This version is not peer-reviewed
Submitted:
20 July 2023
Posted:
21 July 2023
You are already at the latest version
Alerts
Abstract
The article continues the exposition of the results obtained in the research of an agricultural machine for processing soil, designed for research with applications including exploitation. The MCLS complex cultivator was designed for the research of the working processes of the instruments intended for soil processing. The MCLS cultivator is a modulated machine (it can work for three working widths: 1, 2, and 4 m, with tractors of different powers) that is designed to use a wide range of working bodies.
The experimental data obtained with the structure with a working width of 1 m and the results of their processing within the framework of the theory of random vibrations are presented in this article. The experimental results are analysed as random vibrations of the supports of the active body. As a result, the main characteristics of random vibrations are exposed: the distribution function, the average value, the autocorrelation, and the frequency spectrum. These general results regarding random vibrations are used for several critical applications in the design, execution, and exploitation of some subassemblies and assemblies of agricultural machines of this type. The main applications are: estimating the probability of the occurrence of dangerous load peaks, counting and selecting the load peaks that produce fatigue accumulation in the material of the supports of the working bodies, identifying some design deficiencies or defects in the work regime, estimating the effects of vibrations on the quality of soil processing. All the outcomes are comprised of applications in MCLS research and exploitation. The applications pursue well-known objectives of modeling the working processes of agricultural machines: safety at work, increasing the quality of work, optimizing energy consumption, and increasing productivity, all in a broad context to obtain a compromise situation
Keywords:
Subject: Biology and Life Sciences - Agricultural Science and Agronomy
1. Introduction
The phenomena and work processes encountered in agriculture involve, for the most part, important areas of the field of biology (environment, soil, plants, animals, etc.), which is the “living.” According to all the assessments in the literature, the field of life is a field of unpredictability, uncertainty, randomness, and probability. According to [1], “understanding randomness is essential for modern life, as it underpins decisions under uncertainty.”
Random phenomena are treated in physics through theories different from deterministic ones, using the concepts of probability theory and statistics. In [22], it is shown that “in mechanical engineering, random vibration is motion which is non-deterministic, meaning that future behaviour cannot be precisely predicted. The randomness is a characteristic of the excitation or input, not the mode shapes or natural frequencies. Some common examples include an automobile riding on a rough road, wave height on the water, or the load induced on an airplane wing during flight. Structural response to random vibration is usually treated using statistical or probabilistic approaches. Mathematically, random vibration is characterized as an ergodic and stationary process.”
In [43], it is explained that an experiment is random when the answer cannot be precisely and unambiguously predicted, as several answers are possible.
The main ways of approaching random vibrations are, according to [22], A measurement of the acceleration spectral density (ASD) is the usual way to specify random vibration. The root mean square acceleration (Grms) is the square root of the area under the ASD curve in the frequency domain. The Grms value is typically used to express the overall energy of a particular random vibration event and is a statistical value used in mechanical engineering for structural design and analysis purposes”. Also, in [22], it is stated that While the term power spectral density (PSD) is commonly used to specify a random vibration event, ASD is more appropriate when acceleration is being measured and used in structural analysis and testing.” Such specifications and assessments can be found in [23,24,25,26,27,28]. In the case of the study whose results are presented in this article, we worked with sequences of specific deformations measured and recorded, which were then converted into loading forces on the supports of the active working bodies.
As scientific literature shows, work processes in agriculture have random characteristics [5,6,7,8,9,10,11,12,13,14,15]. Much of the specified literature has agro-economic or bio-economic origins, many of which emphasises management. Uncertainties in agricultural work processes are also caused by management system uncertainties [2]. In [3], it is stated that ”problems related to agriculture are, in essence, stochastic because of the uncertain nature of their parameters. Many systems in this sector are affected by the uncertainty caused by factors such as climatic conditions on yield. Uncertainty and imperfect information involved therein challenge decision-making, as decision-makers are led to make decisions before observing the realization of the random factors. Traditional approaches to dealing with agricultural problems do not integrate the risks and uncertaintiesinvolved therein, while it is relevant for efficient managerial decision-making to consider uncertainties and respond to opportunities and threats.” The authors [4] show that ”The second law of thermodynamics states that entropy or randomness in a given system will increase with time”. This is shown in science, where more and more biological processes have been found to be independent” and ”randomness is the fundamental and overarching principle that helps to explain how traits are independently passed from parent to offspring. It is the presence of randomness in all biological systems that this paper aims to highlight.”
In the exploitation of agricultural machines, the study of vibrations is done more and more experimentally. The results are processed using mathematical statistics in the spirit of the theory of random vibrations [30,31,32,33,34,35,36]. The classical theory of vibrations cannot effectively study the complex vibrations of agricultural machines due to objective reasons: the difficulty of estimating model constants, nonlinear behaviours and excitations, most often of a random nature. Apart from these aspects, the influence of games with a functional character, which is impossible to model in a deterministic way, is manifest. Concrete cases are harvesters [30,32], farm tractors in transport or various agricultural works [31,33,34,35,36,44,45,46], as well as cultivators subsoilers [66,67,73].
In the field of agricultural processes that directly involve the soil, the random character of the process is due for the most part to the random properties of the soil. In agricultural machines intended for soil processing, the soil-working body contact excites the analysed structures. The highly random properties of the soil induce the excitation of the process (especially contact forces) to have a deep random character. In general, the dynamic processes produced by the contact between the soil and the working body of agricultural machines intended for soil processing are well included in the category of random vibrations. Sometimes the machines themselves include vibration sources with adjustable, programmable characteristics to achieve a certain type of soil processing. In the latter case, in the experimentally recorded signals, it is natural for the deterministic vibration elements to appear in the spectrum, even if they have been altered to some extent. The random vibrations of agricultural machines intended for soil processing are another example of random vibrations encountered in the technique, apart from those given in [22]. We recall the definition of the agricultural cultivator as given in [71], that is, an agricultural machine that serves to shred and loosen the soil, to destroy weeds from crops of creeping plants, etc. This definition was added because the study material presented in this article has as a main component a working variant of a complex cultivator with variable working width. According to [72], the cultivator can be understood as an agricultural machine used for surface soil work to loosen and destroy weeds without overturning the furrow.
The original aspect of this study is the presentation of a research method based on the theory of random vibrations using the capabilities of a complex modulated cultivator with a variable working width, MCLS. MCLS performance testing is presented simultaneously as a research method for other similar machines or for machines obtained from MCLS by modulating or fitting different active bodies that the structure can use.
Also, the paper tries to show the researchers the benefits produced by the study of vibrations in the experimental framework with the help of the theory of random functions. Most researchers are trained in the scientific spirit of the deterministic theory of vibrations, which is why their first tendency is to look for answers in terms of the deterministic theory. From there, it comes to mathematical modelling and simulation, which are easy to develop on the computer. After the models are run, however, it is found that it is difficult to give answers to the real problems, especially to motivate and validate the hypotheses and mathematical models. Advanced designers in the field of such phenomena have been using the path of experimentation and the theory of random functions for decades. The deterministic approach tries to force physical reality into the model’s pattern while modelling in the experimental framework seeks to take as much as possible from the real conditions and extract a minimum of useful conditions in conception, design, execution, and exploitation. The most difficult problems with this last option are the generalisation (which is low for the time being) and the generalisation costs (which are very high).
2. Material and Methods
Research on the MCLS soil tillage machine (variant with a working width of 1 m) is the subject of this article. The presented results describe the experiments and the statistical processing of the experimental data. The MCLS complex cultivator was initially conceived, designed, and realised to facilitate research in the field of soil processing [16,17]. The machine was designed for three working widths: 4 m and 2 m (with an 80 HP tractor) and 1 m (for a 45 HP tractor). Also, the supporting structure can support the installation of 5-10 types of working bodies to make comparative estimates as complete as possible. After conception, design, and execution, the idea of using the machine in farm operations also appeared. Thus, the machine can be used simultaneously for farm applications and research. Images from the experimental activity with the MCLS machine in all working variants are given in Figure 1, Figure 2, Figure 3 and Figure 4.
The experiments were carried out in Romania, in the Bucharest-Ilfov area, in an experimental plot owned by the INMA Bucharest Institute, which has a transitional temperate continental climate. The morphological characteristics of the soil were: red-yellow clay loam, in a dry state, medium and small angular agglomerate structure, slightly loosened. The soil moisture at the measurement time was between 20 and 35 percent (Delta-T HH2 digital moisture meter), while the average soil compaction level was 45 KPa.
Traction is provided by agricultural tractors New Holland 80 TD and New Holland 50 TD. The working speeds were between 0.78 and 2.15 m/s. The results presented in this article refer to the experiment carried out at a constant speed of 0.789 m/s.
The experiments were carried out on a plot of 500 square meters, marked with benchmarks. For each test, 10 meters were set for the acceleration and 10 meters for the deceleration of the tractor, and 30 meters were set for the evaluation of the cultivator (see Figure 4).
The QuantumX MX1615B data acquisition system was used for the strain gauge measurements, with 32 measurement channels and KFG-6-120-C1-11 N15C2 strain gauge sensors (120 Ω). The strain gauge amplifiers, QuantumX MX1615B, are suitable for precise data acquisition in full-bridge, half-bridge, and quarter-bridge configurations, as well as for strain gauge-based transducers, potentiometers, resistance thermometers (PT100), or normalised voltage (+/- 10V).
The measurement of the forces on each support of the working body was done by the strain gauge methodology, with the procedure including a calibration stage of the indications of the deformation sensors. Although it is not the most precise measurement method, it is convenient and the most accessible, especially considering that only values measured by the same procedure are compared and are, therefore, hypothetically, affected by comparable errors.
The experiments took place in the testing ground of INMA Bucharest, where the soil was classified as reddish-brown from the forest and pre-processed with autumn ploughing (coarse). An image of the location of the experimental polygon appears in Figure 5, with detail, by reporting to the Bucharest-Baneasa airport runway.
2.1. The Subject of Research
Approaching the soil processing phenomenon using an MCLS-type structure involves statistical processing of experimental results (descriptive and inferential statistics, random process theory). Descriptive and partial inferential statistics were discussed in a previous article [42]. In this article, the focus will be on approaching the problem within the theory of random processes. I demonstrated in the article [42] that all the data strings that are generated by the contact between the ground and the working body have a random character and another comparable to the pseudorandom strings generated by special programs or to the strings of prime numbers of the same length.
In order to approach it within the theory of random functions, the phenomenon of ground processing by each organ was considered a phenomenon of random vibrations. The main random component is the excitement of the process. Excitation is generated by the interaction of the working body with the ground. In the range of demands considered, the vibrations can be considered linear-elastic.
In this article, the analysis will be made only for the MCLS variant with a working width of 1 m because in the case of this variant, signals were collected from all the supports of the working bodies throughout the experiment. The analysis carried out on the simple version with a width of 1 m can later be extended to the versions with higher working widths, for which the load on each support cannot yet be measured, and other random parameters also appear (for example, the clearances in the truss with a working width of 4 m).
The indexing of the measurement locations and the signal transmission channels from the deformation sensors to the acquisition board is shown in Figure 5. An image of the MCLS variant in experimental work, on the parcelled track of the polygon, with the complete data acquisition equipment, is shown in Figure 6.
2.1.1. The Formulation of the Problem in Terms of the Theory of Random Vibrations
According to [18], physical processes that cannot be characterised by deterministic functions, i.e., vibrations whose instantaneous values cannot be predicted as functions of time, are called random. The random nature of the vibrations of the investigated structure was demonstrated in [42], where even the degree of randomness was quantified. A relatively simple criterion for experimental recognition of the randomness of a phenomenon that is experimental is formulated, for example, in [18]: if, in several experiments organised under identical controllable conditions, the measured quantities differ only by quantities of the order of errors of measurement, the phenomenon can be considered deterministic; it is reproducible, so its development after the moment of measurement is predictable. Otherwise, the phenomenon is random. Apparently, the criterion seems simple, but in the case of soil processing and, in addition, using non-conventional and insufficiently verified structure load measurement systems (strain gauge methodology), this criterion is difficult to use. Given the findings from [42], we opted for the random vibration variant to describe the working process of the 1 m working width version of the MCLS complex cultivator.
2.1.2. Distribution Functions
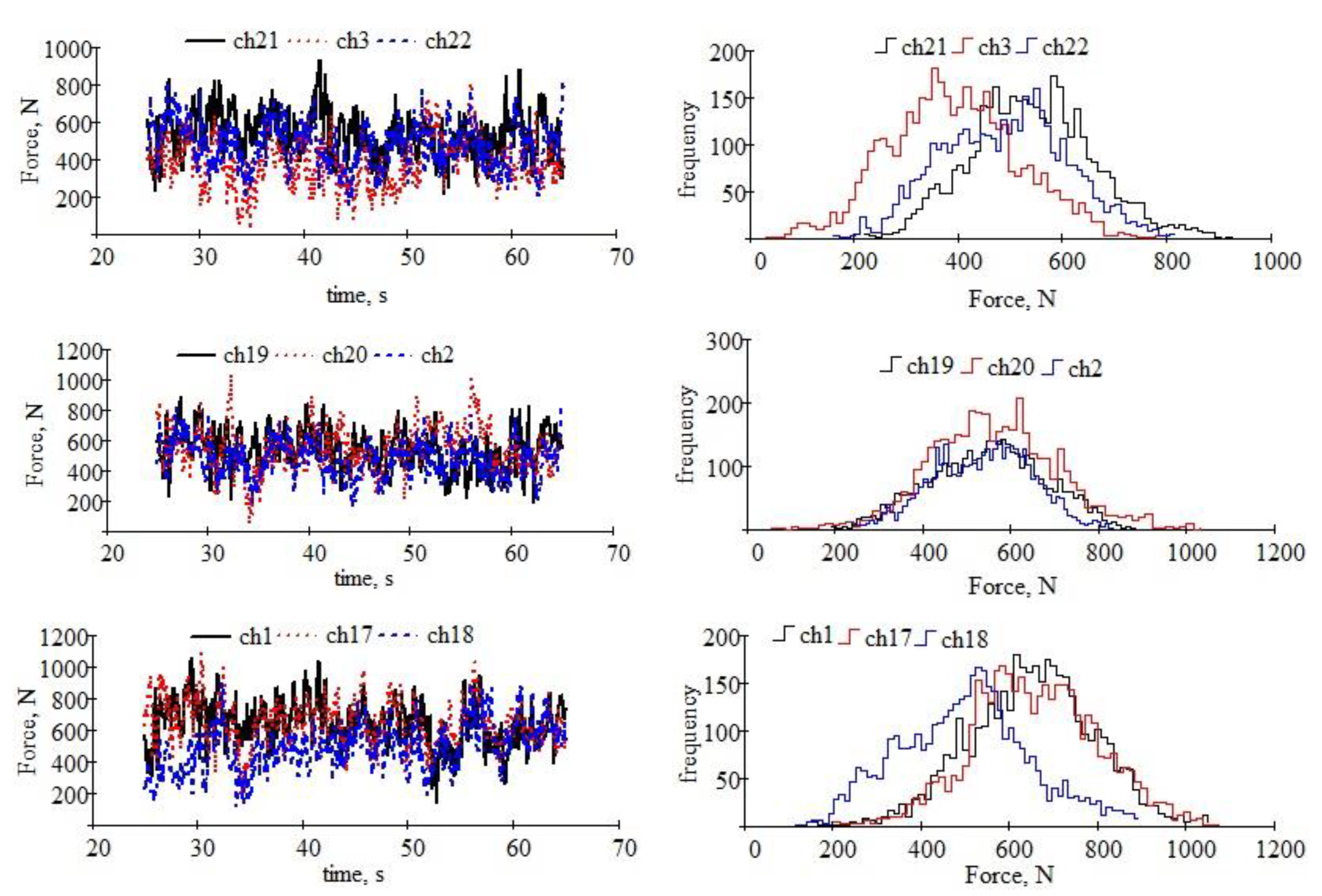
In Figure 8, in the column of graphs on the left, the temporal variations of the forces that load the supports of the twelve working bodies of the version with a working width of 1 m of the MCLS are shown. Also, in Figure 8, the histogram of the frequencies of the force values is represented in the right column. The polygon of frequencies is built using the histograms,. When the number of classes in the histograms becomes very large (theoretically to infinity), the frequency polygon tends to reflect the real distribution density of each force. The frequency histograms are constructed with 64 classes using the formula of Mosteller and Tukey [74]. We took into account the length of the data strings obtained experimentally in calculating the number of classes of the histograms.
The elementary formula (2) was used to calculate frequency histograms.
where:
Article
is the number of observations from the examined random sequence, and is the number of classes in the frequency histogram. Then the probability density histogram was calculated according to formula (5),
where is the series of probability densities. The series of probabilities (or cumulative probabilities) is calculated using the formula (6).
Figure 7.
The time dependence of the load in the supports of the active bodies (left column) and the frequency distribution of the load values (right column). The four rows of figures correspond to the four lines of active organs, starting from the top with the ones closest to the tractor.
Figure 7.
The time dependence of the load in the supports of the active bodies (left column) and the frequency distribution of the load values (right column). The four rows of figures correspond to the four lines of active organs, starting from the top with the ones closest to the tractor.

In Figure 8, the probability density and the probability that the random variable (the series of numerical values over time recorded at each of the twelve measurement locations) will take different values in the experimental range are shown.
Figure 8.
The probability density and the probability of taking certain values from the experimental range for the forces recorded in the twelve measurement locations.
Figure 8.
The probability density and the probability of taking certain values from the experimental range for the forces recorded in the twelve measurement locations.

The probability densities calculated as shown above do not resemble those found in statistics books or in articles in which the experimental results are approximated, by hypothesis, with one of the classical statistical distributions (normal, exponential, Student, Fischer, etc.). Probability distributions differ from the statistical densities with which the experimental curves are usually approximated. Precision is lost by approximating with the classical statistical distributions of the probability densities, and we cannot precisely estimate the local error in relation to the experimental data. For this reason, we preferred, considering our goals, to interpolate the empirical probability densities through cubic spline functions, since we wanted maximum precision and were not interested in using the results in immediate theoretic models. Regarding the probability curves, they have shapes very similar to the classical ones, but for the same reasons, we proceeded with the probabilities by interpolating with spline functions. In Figure 8, the experimental curves interpolated by spline functions are given in each graph for the twelve series of 4000 numerical data points.
The probabilities interpolated with spline functions are used to calculate the probability of the occurrence of a force greater than a fixed force (related to the limit resistance characteristics of the material of the supports of the working body).
The distribution functions of the twelve random variables described by the numerical sequences in Figure 7 are calculated in the same way as the probability densities and probabilities whose graphic representations are given in Figure 8. Therefore, the distribution functions are expressed as interpolations by cubic spline functions, for which the graphic representations are given in Figure 9. The graphic representations of the twelve distribution functions of the sequences of numerical values coming from the measurement points located on the supports of the twelve working bodies are grouped three by one on the graphic, corresponding to the three working bodies in each line, with the counting starting from the back of the tractor.
For complete agreement with the theoretical definition, the interpolated distribution functions are extended with the value zero to the left of the minimum value and with value one to the right of the maximum value of the corresponding random variable.
2.1.3. Characteristic Functions of Random Vibrations
From a theoretical point of view, the first tested properties of random vibrations are stationarity and ergodicity. The definitions of these notions can be found in all the literature dedicated to random vibrations, for example, [18,19,21,22,23,24,25,26,27,28]. In general, the response of structures to random vibrations is studied through experiments, and they have strictly elaborated procedural standards [29].
A synthetic characterisation of random signals is given in [43]. The non-deterministic signal has specific characteristics: mean, dispersion, global average, global dispersion, histogram, power spectral density, etc. The signal can have a certain degree of predictability in its evolution over time. Depending on specific characteristics, the non-deterministic signal can be:
Stationary, if the mean and dispersion do not depend on time but are constant;
Ergodic if the mean per portion does not differ from the global mean;
White noise has a constant power spectral density throughout the frequency band.
Testing the stationarity and ergodicity of the experimental signals (sequences of finite length) cannot be done using the definitions of the mean value and the autocorrelation function of the random variables, for example, in [18]. The definitions in [18] assume processes for crossing the limit after the number of samples. We do not have an infinite number of samples, and if we did, we would be constrained by costs to limit the duration of the experiences as much as possible. For this reason, the calculations are made for a finite number of samples, limited by the number of samples in the entire sequence.
In Figure 10, we see the behaviour of the average value of the random variable given by the registration with the ch4 code (coming from the working body in the first line after the tractor, on the extreme left). The tendency to decrease the amplitude is observed with the increase in the number of samples in the sequence considered for calculating the average value. Therefore, one can only suspect asymptotic behaviour, but such behaviour cannot be stated strictly theoretically.
In Figure 11, the terms below the limit of the definition of the autocorrelation function are calculated for the subsequence of the ch4 signal, with the graphic representation being made only for five values of the gap. There is a tendency to decrease the amplitude of the autocorrelation function with the gap value and a weak asymptotic tendency, which does not allow us to make statements about the stationarity and/or ergodicity of the signal.
In Figure 12, the autocorrelation curves are given for each of the twelve signals collected from the supports of the working bodies of the 1 m working width version of the MCLS. The curves are calculated using the lcorr function of the programme [69]. The calculation can also be done directly by programming the three simple formulas given in [70]. These curves can be considered substitutes in the field of real signals of finite length for the assessment of the stationarity or ergodicity of signals. In Figure 12, it is first observed that all twelve signals tend to asymptote in time towards the value 0. First, this means that the signals are weakly autocorrelated (another argument for their randomness). The fact that they are not constant in time shows that the signals are neither stationary nor ergodic.
2.1.4. Spectral Analysis
The frequency spectrum of the signals collected from the twelve working bodies provides interesting information, especially from identifying possible deterministic components that appear in all the examined sequences, which indicates possible deficiencies of the working regime, abnormal clearance or defects. Deterministic components can also appear in agricultural soil processing machines that produce programmable vibrations, which is not the case for the structure examined in this paper.
Another application of the frequency spectrum of the random sequence is the estimation of the risk of resonant vibrations produced by random components on the elements of the cultivator structure. Components of the excitation that produce resonances are unlikely because of the coincidence of a constant excitation with one of the first natural frequencies of the structure (here we refer only to the support of the working organ, in the general case, the calculation being very voluminous) is difficult to manifest. Even if it happens, the damping from the metal material and the ground in which it moves severely diminishes the resonant effect. Therefore, resonance effects are probably due to the improper functioning of some internal organs or a work speed that, combined with the soil profile, can produce them. However, a comparison between the own spectrum of the supports of the working body and the spectrum of the excitations can be made to investigate the resonance problem at the supports of the working body or some more complex sub-assemblies. Figure 13 shows an example of a study of the distribution of the natural frequencies of the studied structure (here, the supports of the working body) in relation to the excitation spectrum (signals from the supports), obtained using the Fourier transform. In Figure 13, with solid grey bars, the spectra of the twelve supports of the working bodies are drawn, in the window 14–16 Hz range, in which the fundamental frequency of the ‘support falls. The red bar with a circle at the upper end marks the position of the fundamental natural frequency of the supports in the chosen frequency range. The fundamental Eigen frequency and the other Eigen frequencies are calculated and shown in Figure 14 (the first five natural frequencies), but they were not experimentally determined as they should have been when calibrating the structure.
Since we had no data about the operation in the non-linear elastic domain or in the plastic domain or about the damping capacity of the steel from which the supports are built, we worked in the linear elastic domain, which makes the displacement values exaggerated when calculating the natural frequencies. For this reason, we have not given numerical data relative to the deformed shapes corresponding to the five fundamental natural frequencies.
The value of the fundamental natural frequency represented by the red bar with a circle at the upper end in each of the twelve graphs in Figure 13 corresponds to the support or semi-hard fixation of the support (15.151 Hz). Overlaps of the fundamental frequency over local maxima of the excitation spectrum occur for channels ch4, ch23, ch24, ch3, ch20, ch2, and ch1. However, there are no reasons for concern because the peaks in the spectra of the supports have small values and the damping capacity of the material of the supports and especially of the soil in which the working body moves, is high.
In addition, when calculating the spectra of all the twelve signals coming from the twelve measurement locations, it is found that they are all highly correlated, with the minimum value of the correlation of two such numerical sequences being 0.979. The most intensely correlated signals correspond to channel pairs: ch2 and ch17, ch2 and ch1, and ch1 and ch21. At the lowest intensities, we find the correlations between channels ch4 and ch1, respectively, ch4 and ch17.
A better understanding of the distribution of the frequency spectrum of all twelve signals coming from the measurement location of the supports of the working body of the cultivator in relation to the natural (calculated) frequencies of the supports is possible using the graphic from Figure 15 and Figure 16.
In Figure 15, the spectral distribution is plotted for all signals in the range (window) 0–50 Hz, which includes the first three fundamental frequencies. Details in narrow intervals around the first three fundamental frequencies (see Figure 15), are plotted in Figure 16. It is found that, around the first three natural frequencies of the supports of the active organs, there are few frequencies from the signal spectrum, and, in addition, their magnitude is very small (compare the magnitudes of the frequencies in Figure 17 with those of the spectrum components in Figure 15).
2.1.5. RMS and the Average Value
In many studies of random vibrations, RMS (Root Mean Square) is calculated as a measure of amplitude extremes. The frequent use of RMS in the study of vibrations is also due to its direct connection with the energetic content of vibrations and implicitly with their destructive capacity [68]. RMS is related to the vibration amplitude and its average value.
Table 1 gives the RMS values and the average value for each sequence recorded in the examined experiment. It can be seen that the two measures of the force amplitude are close. Moreover, the correlation between the two matrices (that of the RMS and the average values) is very high: 0.999.
3. Results
The main results that will be presented are applications for the design, execution, and operation of agricultural machines such as the MCLS complex cultivator, which is presented in the chapter. 2. The results refer to the calculation of the probability of the occurrence of dangerous peaks, the selection and counting of the peaks of force that produce fatigue accumulation (in the supports of the active organs), the identification of design defects or deficiencies or of the work regime, and effects on the quality of the work.
3.1. The Probability of the Occurrence of Dangerous Loads
To identify and count the dangerous peaks of force in support of the working organ, the characteristic resistance limits of the material from which it is constructed will be used: the bending fatigue limit stress, , the yield stress (plasticisation), , breaking or yielding limit stress, . To be able to solve the proposed problem, the finite element method was used to determine the state of equivalent tension in support of a working body as a result of the application of forces with experimentally determined values. The material used has the following limit characteristics: = 620.422 MPa, =723.826 MPa, modulus of elasticity = 210,000 MPa, Poisson’s ratio = 0.28, mass density = 7700 kg/m3, and the fatigue resistance limit by bending, =380 MPa. The structural model used is described in [16,17]. The occurrence of damage phenomena (irreversible deformations, cracks, or breaks in the material) is decided with the help of the structural model by comparing the values of the equivalent stress (Von Mises) in the structure with the critical limit stresses of the material. In Figure 18, the colour maps of the state of equivalent tension resulting from the application of the average (518 N) and maximum (1086 N) forces to the support of the working body are shown.
It can be observed that the maximum equivalent stress for the load of 518 N, has a value of 428.5 MPa (with a maximum resultant relative displacement of 77 mm), and for the load of 1086 N, it has a value of 942.3 MPa. Therefore, the average load of 518 N does not pose damage problems, and the support works in the field of linear elasticity with a safety coefficient of approximately 1.45. In the case of the maximum stress (1086 N), the equivalent stress in the structure reaches maximum values higher than the breaking limit stress of the material. However, the organ will not yield yet because the core of the bar from which the support is built presents an appreciable area that works in the linear elastic domain. For the maximum value of 1086 N, the yield stress is exceeded on an appreciable area (Figure 17), and even the breaking stress is exceeded on narrow areas in strips near the longer sides of the cross-section. However, the section works in linear elastic mode in the central area, so a failure is not directly recorded. However, in this working regime, high peaks lead to the accumulation of fatigue and possibly premature failure.
Using the results provided by the structural analysis of the support of the working body and the probabilities interpolated with cubic spline functions, and it is possible to calculate the probability of exceeding the limit forces that cause the critical limits of the material to be exceeded: fatigue, plasticisation, and breaking. First, based on the hypothesis of the linear elastic behaviour of the support material of the working body, the graphic representation in Figure 18.
Note: To calculate the equation of the oblique line in Figure 18, we accepted the hypothesis of linear elastic behaviour of the support material upon bending up to the splasticisation limit. Thus, the fatigue limit is included in the elastic bearing. The equation of the oblique line in Figure 18 is:
where the elastic constant is calculated using the result of the structural analysis for the average value (518 N) of the force applied on the twelve sequences of analysed force values: = 0.827 N/Mpa.
The probability that the loading force corresponding to each of the twelve supports of the active organs will exceed the critical values of flow, rupture, and fatigue (characteristics of the material of the supports) is shown in the graph in Figure 19.
3.2. Counting the Peaks That Produce Fatigue
One of the immediate applications of this research is the possibility of counting the “cycles” that produce the fatigue of the support material of the working body. Using the data and the graph in Figure 18, one can find that the “part” of the random sequence of force values that requires the support of the working body can produce the accumulation of fatigue, cracks, and breaks in the material.
A stress sequence filter is the simplest selection method for that part of the stress that produces fatigue accumulation, plastic yielding, or breaks. The expression of such a filter is given by formula (8).
where is the sequence of experimental data in force values, converted into values of the equivalent stress (Von Mises) in the material, according to relation (7).
In Figure 20, the load peaks of the support of the active body from the extreme left of the first line of working bodies (ch4) after the back of the tractor are highlighted. Prominent spikes produce fatigue build-up in the support material.
The wear of the support in 40 seconds will be calculated by structural engineers using the above data. During this time, the vehicle moves approximately 30 meters, covering approximately 30 square meters of land. The result allows the estimation of the life span in time (neglecting the wear caused by the action of water or other environmental factors) in hectares of ploughed land or seasons or years of exploitation. In order to determine the lifetime of the supports of the working bodies, any structural analysis programme that has this facility can be used (for example, [75,76]).
3.3. Identification of Defects, Deficiencies, or Operating Errors
Most often, the study of random vibrations is dedicated to or requires the inclusion of the identification of sources of errors, defects, deficiencies, premature wear, etc., in the operating regime. Measuring the random vibrations of structures and improving or even optimising them based on the measurements has become one of the most used methods in the study of phenomena affected by vibrations [37,38,39,40,41].
The research carried out in the MCLS complex cultivator case highlighted work deficiencies, especially the problem of low-quality control of the working depth. It was shown in [42] that using only two working depth control wheels (located in the front of the MCLS) is insufficient. A pair of wheels would also be necessary at the rear. In addition, the suspension-traction system (connection to the tractor) should also be checked to not raise the structure at the front during work. The identification of this problem was made by analysing the distribution of the intensity of the measured force, which indicated appreciably higher forces on the row of organs at the back (ch1, ch17, ch18, see Figure 6) in relation to those measured on the first row of organs behind the tractor (ch4, ch23 and ch24, see Figure 5). The main reason and validation of the suspicion were found by analysing the images in work [42]. This deficiency partially generates the non-uniformity of the working depth, treated in 3.4.
Another deficiency valid only the case where the MCLS operates in exploitation mode in any of its work variants is unsatisfactory soil fragmentation. According to the analysis in the field and on the photos, it is necessary (the granulometric analysis of the processed soil was not done) for coupling some shredding elements (discs, rollers, etc.) to the back of the structure. In the research regime of the pure effects of the working body, the installation of additional shredding structures is prohibited because it alters the desired effects.
Another category of possible deficiencies, which can be avoided partially from the design stage and through systematic experimentation in working mode, are resonances and interferences, also called knocks. Both phenomena are defined in the literature, for example, [47]. A search for possible resonances in the supports of the working organs is presented in subchapter 2.5 when calculating the frequency spectra for the twelve supports of the working bodies. The literature includes the results of some studies related to the problems of vibrations in agricultural machines [31,32,48,49,50,51,52,53,54,55,56].
3.4. Effects on the Quality of the Working
The vibration of the supports of the cultivator’s working organs involves relative movements horizontally, vertically, and laterally. The vibrations of the cultivator are complex, they are not reduced to the elastic vibrations of the working supports, but there are random rigid vibrations of the supporting structure and the working organs. All these types of vibrations have consequences on the main parameters of the work quality: working depth, working width in the first place, energy consumption, productivity, and comfort of the tractor driver.
In order to give only an ideal picture of the variations of the working depth due to random vibrations, we performed an elementary and ideal calculation on the deformations of the supports of the working bodies in the work process by applying some forces included in the experimental range. Using the finite element method and the model to calculate the first five natural frequencies, we estimated the relative displacements (deformations) in the three directions for the extremity of the support to which the working body is attached. The results are given in Table 2.
The results in Table 2 are slightly exaggerated because the support material is assumed to be perfectly linearly elastic (which is not true). However, they suggest a good value as an order of magnitude for the relative displacements. The dominant overshoots are manifested in the forward and vertical directions on the ground. These values are approximately rigidly related. At the average value of the force from the recordings related to the analysed experiment (500 N), the relative vertical displacement reaches 7 cm, which seriously affects the programmed working depth (10 cm). These situations are not frequent, but they are facilitated by exceeding a working speed limit, deformations of the working surface (unevenness), and errors in the working depth regulation system.
In such conditions, the cultivator cannot be a tool to process the soil with high precision (maximum error of the order of 10% of the theoretical depth) at the working depth. A previously levelled and sufficiently crushed work surface, combined with an adapted work speed, are mandatory conditions for precise work in terms of working depth. A general picture of the quality of the work performed in terms of working depth is shown in Figure 21.
The calculation from this subchapter is based on the graphic representation in Figure 21, which uses the hypothesis of the operation of the supports of the working bodies of the MCLS in a linear-elastic regime without damping, which leads to an overestimation of the relative displacements. Direct measurements regarding the working depth and its monitoring are insufficiently developed in the available experimental technique; therefore, the estimation method described above was used.
An option to improve the accuracy of the working depth is to ballast the load-bearing structure by adding some ballast materials. Increasing the precision of the working depth in this way will lead to higher energy consumption and, possibly, a decrease in productivity.
Also, a problem that must be solved for this machine is finding the maximum speed at which it can work without manifesting intense vertical oscillations throughout the entire structure, which completely compromises the quality of soil processing. The speed limit, which is determined through tests, is dependent on the characteristics of the soil, so an exact speed limit cannot be indicated a priori.
4. Comments
According to [57], most random vibration analyses are intended to realistically characterise the behaviour of structural systems excited by random inputs. The responses of real systems are known only when measured during their actual physical loading (and even then, only approximately). The effects caused by the use of simplifying assumptions in the process of numerical simulation of physical systems are rarely evaluated. Because of the depth of our knowledge in the analysis of linear systems subject to stationary media, we often idealise real systems as linear and the inputs as stationary. Practically, all real systems are nonlinear and random to a small or large extent. Therefore, the characterisation of the response can only be approximate. Such reasoning also directed our attention to the experimental or theoretical-empirical research of the working processes of agricultural machines.
Starting from these realities, we tried to formulate the problem within the strict framework of random vibrations. We tried to use as few simplifying assumptions or assumptions from the theoretical field of linear vibrations as possible. For this, we constructed the characteristic functions of the empirical sequences exactly as they result from the definition (Chapter 2). Probability densities and probabilities are obtained directly using the numerical sequences and interpolating them with spline functions. We did not use probability density modelling with idealised functions (normal distributions, Student, Fischer, etc.), and consequently, the resulting probabilities are also modelled on real data.
Representations of the spectral frequency distributions of the twelve supports of the working bodies (2.5, Figure 16 and Figure 17), can be found in [30], for the chassis of a harvester. The orders of magnitude and the numerical values are also comparable, being characteristic of the working processes of agricultural machines. As values, the forces resulting from the experimental study described in this article are comparable to those found for the working bodies and also for a cultivator by the authors [58]. The obtained frequency spectra are similar to those from [59]. Unfortunately, we could not get accelerometer signals from the experimenter, which was very important. Acceleration recording remains a problem to be solved for MCLS. Some of the forces’ values and the frequency spectra frequencies were also obtained in [42]. In the same register of concerns and spectral values, [32] estimates the effects of frequencies and vertical accelerations on several types of tractors using frequency-acceleration diagrams. I did not have access to such diagrams in this paper and used frequency-load force diagrams on the working body. However, the principle of selecting deficient or dangerous work regimes is the same. The authors [35] use the same principle of spectral selection of the vibration level on the front axle of the Valtra 800L tractor. As an estimator of the vibration level, the authors [35] use RMS (Root Mean Square Amplitude, [60]) for the experimentally recorded acceleration sequences in the version indicated by the authors [35]. The RMS maximums are recorded in the frequency range where the maximum oscillation amplitudes of our force sequences were located. The optimisation of the frame of a precision agricultural machine for sowing vegetables by avoiding resonances, based on the same principles as in this article, is done in [49]. The authors proceed similarly [73] for cultivators with working bodies very similar to those with which the MCLS cultivator was equipped in our experiments. Similarly, the authors [50] proceed with the study of the vibration of rapeseed seeds, additionally using high-speed photography and image recognition. All the frequency spectra (in this case normalised) are used in [55] to carry out experimental investigations with vertical damping for block-modular aggregates. As in this article, the authors [61] use spectral analysis and finite element analysis to predict fatigue accumulation in the arm of an excavator. The use of statistical analysis to estimate fatigue life dates back a long time [62], even for an agricultural cultivator, this being one of the main applications of the study of random vibrations. A similar approach for the testing of agricultural machines in an accelerated regime is proposed by the authors [63], in the manner that we also proposed in chapter 2.1.2. It is emphasised that not only the value of the load matters but also its frequency. The authors proceed similarly [64,65]. A solution to reduce the force of resistance to advance for a cultivator by inducing forced vibrations is presented in [66]. Also, the return to some classical solutions, which had been partially abandoned, appeared after studies of random vibrations [77].
5. Conclusions
C1) The analysis of random vibrations on any of the MCLS complex cultivator’s variants is an opportunity and an obligation that must be mentioned in the work methodology that uses this equipment for research. The experiments carried out with the MCLS variants were also its first research applications. The literature shows that this is the most realistic way of approaching work processes in agriculture, having indisputable advantages over mathematical modelling and simulation. Among the most essential advantages is eliminating some assumptions that are often overly simplistic, lacking real motivation, and, above all, rarely verified, at least in the validation process. Moreover, simulation and mathematical modelling must validate their results experimentally; otherwise, they remain only scientific ballast.
C2) The main results with immediate applications of random vibrations are the improvement of noise and vibration, the determination of dangerous demands for the elements of the structure, the determination of some limit values necessary for the choice of the material of some parts and sub-assemblies in the design, the estimation of the lifetime of the required elements in the regime of accumulation of fatigue, the identification of design or work regime deficiencies, and procedures for improving the quality of soil processing.
C3) The presented results underline the deficiencies that must be resolved to increase the grower’s performance. The recommendations refer to the control of the horizontalisation of the structure during work (with consequences in increasing the quality of soil processing relative to the working depth), the possibilities of ballasting the structure for better control of the working depth, limiting the operating speed, and the requirement of soil processing on some lands with satisfactory flatness.
C4) A vital conclusion drawn from the results obtained is that to avoid malfunctions or damage due to premature fatigue, it is recommended that the “weakest” design elements, the ones that give way first, be among the cheapest or be simple, easy-to-replace, and cheap elements inserted into the subassemblies that will fail first in the event of an overload. We refer in particular to the fact that it is not rational to take into account the maximum loading for the structure (experimentally recorded). However, it is good to impose provisions of introducing safety elements. Thus, important and expensive subassemblies and parts will be protected. In the design of the 1960s and 1970s, for example, there were safety screws on ploughs that, by giving way first, protected the working bodies, their supports, and the load-bearing structure. Later, the abandonment of some of these safety elements produced the failure of load-bearing structures, for example.
C5) Since we failed to validate the intuitive assumption that the working organs in the first line behind the tractor would be the most intensively requested, neither in these investigations nor in those described in [42], we deduce that, at least in the experimental conditions, the distribution of the maximum load is random over the twelve working bodies. An important consequence of this finding is that we cannot schedule a rotation of the working bodies on the load-bearing structure positions to equalise the wear and use the full working capacity of all working bodies. Moreover, a statistically higher load was observed for the working body in the last line of organs in the load-bearing structure. First, only the result of the statistical analysis was validated based on the analysis of the images taken during the experiments. It is shown that certain aspects thatcertain aspects must be fixed in the operation of this machine, which will improve the quality of the work.
C6) The results of the experimental research agree with those in the literature, presenting a significant randomness and revealing average values of the efforts at the level of working bodies expected from a theoretical point of view. The experiments present an original character through a large number of measurement points, through the study of the distribution of their results and the investigation of the links between these measurements.
Obviously, in such a complex problem as soil processing, there will always be future work directions. In the case of the problem whose research is described in this article, there are many open problems, among which are mentioned: the dependence of the descriptive and inferential statistical characteristics on the work speed, the estimation of the soil processing problem with these work organs on all the work variants of the MCLS, solving the problems already listed for all the categories of working bodies that can be mounted on the load-bearing structure of the MCLS, the study of the processing speed limit depending on the quality of the work performed. All these problems, which do not cover even half of the ways to continue the studies, should be carried out on several types of soil at different humidity levels, aiming, if not to optimise, at least to improve agricultural soil processing works. Another interesting problem is the selection of a minimum number of measurements of the soil characteristics, which can be used to fix the starting parameters of the soil processing. Among these measurements, we mention humidity, resistance to penetration, plant residues amount or density, and soil resistance’s quantified characteristics (apart from resistance to penetration).
Before choosing a way to continue, evaluating the possible benefits of each possibility of continuing the research is required. The evaluation creates the conditions for an optimal choice of development paths, considering the material and human resources, especially the enormous costs of experimental research, which in the end necessarily also includes the theoretical one.
Author Contributions
Conceptualisation, P.C.; C.N. and V.M.; methodology, P.C.; C.N. and V.M.; software, P.C. and C.P.; validation, P.C, C.N.; C.P.; R.S.; C.M.I.; O.D.C. and E.A.L.; formal analysis, P.C.; C.N, R.S.; N.V.V.; C.M.I and E.A.L.; investigation, P.C.; C.N.; C.P.; V.M.; N.V.V.; N.U.; M.M.; C.M.I. and O.D.C.; resources, P.C.; R.S.; N.V.V.; N.U. and M.M.; data curation, P.C, C.P.; V.M.; O.D.C. and E.A.L.; writing—original draft preparation, P.C. and C.N.; writing—review and editing, P.C.; V.M. and N.U.; visualisation, N.U.; O.D.C. and E.A.L.; supervision, P.C.; V.M.; N.V.V.; N.U. and M.M.; project administration, P.C.; V.M.; R.S. and C.M.I.; funding acquisition, V.M, N.V.V.; N.U. and M.M. All authors have read and agreed to the published version of the manuscript.
Funding
The APC was funded by University Politehnica of Bucharest, Romania, within the PubArt Program.
Conflicts of Interest
The authors declare no conflict of interest.
References
- Ingram, J. ; Randomness and probability: exploring student ‘teachers’ conceptions, Mathematical Thinking and learning, Taylor and Francis Group, 2022, pp. 1–19. [CrossRef]
- Costake, N. ; Cu privire la conceptul de Management societal informatizat, Revista Romana de Statistica, Trim I/2013 – Supliment, pp. 153–301.
- Kawtar El, K.; Driss, M. ; Stochastic Programming for Agricultural Planning. Journal of Positive School Psychology 2022, 6, 1938–1944. [Google Scholar]
- Raun, W.R.; Dhillon, J.; Aula, L.; Eichoff, E.; Weymeyer, G.; Figueirdeo, B.; Lynch, O.; Nambi, E.; Oyebiyi, F.; Fornah, A. ; Unpredictible Nature of Environment on Nitrogen Supply and Demand. Agronomy Journal 2019, 111, 2633–3403. [Google Scholar] [CrossRef]
- Nyamekye, A.B.; Nyadzi, E.; Dewulf, E.; Werners, S.; Van Slobbe, E.; Biesbroek, R.G.; Termeer, C.J.A.M.; Ludwig, F. ; Forecast probability, lead time and farmer decision-making in rice farming systems in Northern Ghana. Climate Risk Management 2021, 31. [Google Scholar] [CrossRef]
- Hamsa, K.R.; Bellundagi, V. ; Review on Decision-making under Risk and Uncertainty in Agriculture. Economic Affairs 2017, 62, 447–453. [Google Scholar] [CrossRef]
- Buschena, D.E.; Zilberman, D. ; What Do We Know About Decision Making Under Risk and Where Do We Go from Here? Journal of Agricultural and Resource Economics 1994, 19, 425–445. [Google Scholar]
- Kumari, P.L.; Reddy, P.M. ; Decision Making under Uncertainty in Agriculture-A Case Study. International Journal of Current Microbiology and Applied Sciences 2021, 10, 188–194. [Google Scholar]
- Eisele, M.; Troost, Ch. , Berger Th., How Bayesian Are Farmers When Making Climate Adaptation Decisions? A Computer Laboratory Experiment for Parameterising Models of Expectation Formation. Journal of Agricultural Economics 2021, 72, 805–828. [Google Scholar] [CrossRef]
- Magda, D.; Girard, N.; Angeon, V.; Cholez, C.; Raulet_Croset, N.; Sabbadin, R.; Salliou, N.; Barnaud, C.; Monteil, C.; Peyrard, N.; A Plurality of Viewpoints Regarding the Uncertainties of the Agroecological Transition. In: Bergez, JE.; Audouin, E.; Therond, O. (eds) Agroecological Transitions: From Theory to Practice in Local Participatory Design. Springer, Cham., 2019, pp. 99–120. [CrossRef]
- Spash, C.L. ; Strong uncertainity, Ignorance and indeterminacy, Greenhouse Economics: Value and Ethics, London: Routledge, 2002, pp. 120–152.
- Litre, G.; Bursztyn, M. Climatic and socio-economic risks and adaptation strategies among livestock family farmers in the pampa biome. Ambiente&Sociedade 2015, XVIII, 53–79. [Google Scholar]
- Coletta, A.; Gianpietri, E.; Santeramo, F.G.; Severini, S.; Triestini, S. ; A preliminary test on risk and ambiguity attitudes, and time preferences in decisions under uncertainty: towards a better explanation of participation in crop insurance schemes. Bio-Based and Applied Economics 2018, 7, 265–277. [Google Scholar]
- Bunyasiri, I.N. ; Impacts of income uncertainty and diversified agriculture on savings of thai agricultural households. The Business and Management Review 2017, 8, 311–322. [Google Scholar]
- Burgman, M. ; Risks and Decisions for Conservation and Environmental Management, Cambridge University Press, 2005.
- Cardei, P.; Constantin, N.; Sfiru, R.; Muraru, V.; Condruz, P. ; Modular load-bearing structures for the study of interactions between the soil and working parts of agricultural machines. Journal of Physics Conference Series 2021, 1781, 012063. [Google Scholar] [CrossRef]
- Cardei, P.; Nicolae, C.; Sfiru, R.; Muraru, V.; Muraru, S.L. ; Structural analysis of a modulated load-bearing structure designed to investigate the interaction between soil and the working parts of agricultural machines. Journal of Physics Conference Series 2021, 1781, 012064. [Google Scholar] [CrossRef]
- Buzdugan, Gh. , Fetcu, I.; Radeş, M.; Vibratii mecanice, Editura Didactica si Pedagogica, Bucuresti, 1982.
- Tiron, M. ; Analiza preciziei de estimare a functiilor aleatoare, Editura Tehnica, Bucuresti, 1981.
- Iosifescu, M.; Moineagu, C.; Trebici, V.; Ursianu, E. ; Mica Enciclopedie de Statistica, Editura Stiintifica si Enciclopedica, Bucuresti, 1985.
- Kun II, P. ; Fundamentals of Probability and Stochastic Processes with Applications to Communications, Springer, 2018.
- Available online: https://en.wikipedia.org/wiki/Random_vibration.
- Crandall, S.H. ; Random Vibration, New York:MIT Press/Wiley, 1958.
- Crandall, S.H.; Mark, W.D. ; Random Vibration in Mechanical Systems, New Yorlk: Academic Press, 1963.
- Bolotin, V.V. ; Random Vibrations of Elastic Systems, The Hague, The Nederlands: Martinus Nijhoff Publishers, 1994.
- Elishakoff, I. ; Probabilistic Methods in the Theory of Structures: Random Strength of Materials, Random Vibration, and Buckling, World Scientific, Singapore, 2017.
- Elishakoff, I. ; Solution Manual to Accompany Probabilistic Methods in the Theory of Structures: Problems with Complete, Worked Through Solutions, World Scientific, Singapore, 2018.
- Elishakoff, I. ; Dramatic Effect of Cross-Correlations in Random Vibrations of Discrete Systems, Beams, Plates, and Shells, Springer, Nature, Switzerland, 2020.
- Tricker, R.; Tricker, S. ; Environmental Requirements for Electrochemical and Electronic Equipment, 8.4.4.5 Other Standards, 1999. Available online: https://www.sciencedirect.com/topics/earth-and-planetary-sciences/random-vibration.
- Yao, Y.; Li, X.; Yang, Z.; Li, L.; Geng, D.; Huang, P.; Li, Y.; Song, Z. ; Vibration Characteristics of Corn Combine Harvester with the Time-Varying Mass System under Non-Stationary Random Vibration. Agriculture 2022, 12. [Google Scholar] [CrossRef]
- Garciano, L.; Torisu, R, Takeda, J. ; Sakai,K.; Random, quasi-periodic and chaotic vibrations of farm tractors. Journal of the Japanese Society of Agricultural Machinery 2002, 64, 91–98. [Google Scholar]
- Vladut, V.; Biris, S.S.; Petre, A.A.; Voicea, I.; Cujbescu, D.; Ungureanu, N.; Matei Gh, Popa, D. ; Boruz, S.; Constantin, A.M.; Vasile, C.; Comparative sudy of vibrations in apparatus combinations tangential Threshing. Analele Universităţii din Craiova, seria Agricultură – Montanologie – Cadastru (Annals of the University of Craiova - Agriculture, Montanology, Cadastre Series) 2021, 51, 615–627. [Google Scholar] [CrossRef]
- Matache, M.G.; Voicu, Gh. , Epure, M.; Voicea, I.F.; Gageanu, I.; Ghilvacs, M.; Measuring vibrations level during transportation work for electrical tractor. Acta Technica Corviniensis-Bulletin of Engineering 2020, XIII, 27–30. [Google Scholar]
- dos Santos, V.C.; Monteiro, L. De, A.; Macedo, D.; X.; S.; Costa, E.; Whole body vibration in operators using agricultural soil preparation equipment. Ciência Rural, Santa Maria 2019, 49, 1–9. [Google Scholar]
- Villbor, G.; P. ; Santos, F.L.; de Queiroz, D.M.; Guedes, D.M.; Vibration levels on rear and front axles of a tractor in agricultural operations. Acta Scientarium. Technology 2014, 36, 7–14. [Google Scholar] [CrossRef]
- Hosseinpour-Zarnaq, M.; Omid, M.; Biabani-Aghdam, E. ; Fault diagnosis of tractor auxiliary gearbox using vibration analysis and random forest classifier. Information Processing in Agriculture 2022, 9, 60–67. [Google Scholar] [CrossRef]
- Ghazali, M.; H.; Rahiman, W.; Vibration Analysis for Machine Monitoring and Diagnosis: A Systematic Review. Shock and Vibration, Hindawi 2021, 1-25.
- Li, J.; Liu, Y.; Xing, Zh. , Zeng, F.; Rotating machinery anomaly detection using data reconstruction generative adversarial networks with vibration energy analysis. AIP Advances 2022. [Google Scholar]
- Harris, C. ; M.; Crede, C.; E.; Socuri si vibratii, Editura Tehnica, Bucuresti, 1969.
- Miki, D.; Demachi, K. ; Bearing fault diagnosis using weakly supervised long short-term memory. Journal of Nuclear Science and Technology 2020, 57, 1091–1100. [Google Scholar] [CrossRef]
- Ebrahimi, E.; Javadikia, P.; Jalili, M.H.; Astan, N.; Haidari, M.; Bavandpour, M. ;, Intelligent Fault Detection of Retainer Clutch Mechanism of Tractor by ANFIS and Vibration Analysis. Modern Mechanical Engineering, 2013, 3, 17–24. [Google Scholar] [CrossRef]
- Cardei, P.; Constantin, N.; Muraru, V.; Persu, C.; Sfiru, R.; Estimation of the random intensity of the soil tillage draft forces in the supports of the working bodies of a cultivator, preprint RG. 2023. [CrossRef]
- Available online: http://www.mobilindustrial.ro/current_version/online_docs/COMPENDIU/semnale_deterministe_si_ned eterministe__aleatorii_htm.
- Marin C., Vibratiile structurilor mecanice, Editura Impuls, Bucuresti, 2003.
- Xin, J.; Kaikang, Ch. , Jiangtao, J.; Kaixuan Zh., Xinwu, D.; Hao, M.; Intelligent vibration detection and control system of agricultural machinery engine. Measurement 2019, 145, 503–510. [Google Scholar]
- Choi, W.-S.; Pratama, P.S.; Supeno, D.; Woo, J.-H.; Lee E-S. ; Chung, S.-W.; Park Ch.-S.; Dynamic vibration characteristics of electric agricultural vehicle based on finite element method. Globa Journal of Engineering Science and Researches 2017, 4, 27–31. [Google Scholar]
- Iacob, C.; Cheorghita, S. ;I.; Soare, M.; Dragos, L.; Dictionar de mecanica, Editura Stiintifica si Enciclopedica, Bucuresti, 1980.
- Wang, P.; Gao, M.; Sun, Y.; Zhang, H.; Liao, Y.; Xie, Sh. , A vibration-powered self-contained node by profiling mechanism and its application in cleaner agricultural production. Journal of Cleaner Production 2022, 366. [Google Scholar] [CrossRef]
- Wang, J.; Xu, Ch. , Xu, Y.; Wang, J.; Zhou, W.; Wang, Q.; Tang, H.; Resonance Analysis and Vibration Reduction Optimization of Agricultural Machinery Frame—Taking Vegetable Precision Seeder as an Example. Processes 2021, 9, 1–19. [Google Scholar] [CrossRef]
- Zhan, G.; Ma, L.; Zong, W.; Liu, W.; Deng, D.; Lian, G. ; Study on the Vibration Characteristics of Rape Plants Based on High-Speed Photography and Image Recognition. Agriculture 2022, 12, 1–14. [Google Scholar] [CrossRef]
- Sheng, J.; Zeng, Y.; Liu, G.; Liu, R. ; Determination of Weak Knock Characteristics for Two-Stroke Spark Ignition UAV Engines Based on Mallat Decomposition Algorithm. Mathematical Problems in Engineering 2021, 1–11. [Google Scholar] [CrossRef]
- Wang, S.; Lu, B. ; Detecting the weak damped oscillation signal in the agricultural machinery working environment by vibrational resonance in the duffing system. Journal of Mechanical Science and Technology 2022, 36, 5925–5937. [Google Scholar] [CrossRef]
- Jun, L.; Xueping, L.; Zhou, Y.; Tiansheng, H.; Kunpeng, X.; Wei, L. ; Transversal vibration analysis of resonance condition and frequency for orchard chain ropeway system. Transactions of the Chinese Society of Agricultural Engineering 2014, 30, 50–57. [Google Scholar]
- Farrokhi Zanganeh, N.; Shahgholi Gh., Agh, S.; Studying the effect of balancer on engine vibration of MaSSey Ferguson 285 tractor. Acta Technologica Agriculturae 1, Nitra, Slovaca Universitas Agriculturae Nitriae 2021, 14-19. [CrossRef]
- Bulgakov, V.; Kuvachov, V.; Ivanovs, S.; Melnyk, V. ; Experimental investigations in vertical vibration damping of agricultural aggregate of block-modular type, Engineering for Rural Development. Proceedings of the International Scientific Conference (Latvia), Jelgava, No. 20, 2021, pp. 635–642.
- Esehaghbeygi, A.; Abedi, M.; Razavi, J.; Hemmat, A. ; Field evaluation of a vibrating bent-share cultivator. Research in Agricultural Engineering 2020, 66, 123–130. [Google Scholar] [CrossRef]
- Paez, T. L. ; Random Vibrations: Assessment of the State of the Art, Sandia National Laboratories Albuquerque, New Mexica, 1999.
- Abbaspour-Gilandeh, Y.; Fazeli, M.; Roshanianfard, A.; Herandez-Hernandez, J.L.; Penna, A.F.; Herrera-Miranda, I. ; Effect of Different Working and Tool Parameters on Performance of Several Types of Cultivators. Agriculture 2020, 10, 1–19. [Google Scholar] [CrossRef]
- Gong, Y.; Ren, L.; Han, X.; Gao, A.; Jing, Sh. , Feng Ch., Song, Y.; Analysis of Operating Condition for Vibration of a Self-Propelled Monorail Branch Chipper. Agriculture 2023, 13, 1–22. [Google Scholar]
- Available online: https://vru.vibrationresearch.com/glossary/rms-root-mean-square/.
- Zhao, G.; Xiao, J.; Zhou, Q. ; Fatigue Models Based on Real Load Spectra and Corrected S-N Curve for Estimating the Residual Service Life of the Remanufactured Excavator Beam. Metals 2021, 11. [Google Scholar] [CrossRef]
- Harral, B.B. ; The application of a statistical fatigue life prediction method to agricultural equipment. International Journal of Fatigue 1987, 9, 115–118. [Google Scholar] [CrossRef]
- Wen, Ch. , Xie, B.; Li Zh., Yin, Y.; Zhao, X.; Song Zh., Power density-based fatigue load spectrum editing for accelerated durability testing for tractor front axles. Biosystems Engineering 2020, 200, 73–88. [Google Scholar] [CrossRef]
- Yeter, B.; Garbatov, Y.; Soares, C.G. ; Spectral Fatigue Assessment of an Offshore Wind Turbine Structure under Wave and Wind Loading, Developments in Maritime Transportation and Exploitation of Sea ResourcesPublisher: Francis & Taylor Group London, UK Editors: Guedes Soares, C. & Lopez Pena, F.
- Paraforos, D. S.; Griepentrog, H.W. ; Surface profiles acquisition for assessing fatigue life of agricultural machinery in test facilities, The 18th International Conference of the ISTVSAt: Seoul, Korea, 2014.
- Esehaghbeygi, A.; Abedi, M.; Razavi, J.; Hemmat, A. ; Field evaluation of a vibrating dual bent-share cultivator. Research in Aggricultural Engineering 2020, 66, 123–130. [Google Scholar] [CrossRef]
- Badescu, M.; Croitoru, St. , Petcu, A.; Marin, E.; Boruz, S.; Ivan Gh., Vladut, V.; Matache, M.; Cujbescu, D.; Voicea, I.; Construction evolution of deep soil loosening equipment in romania in the country specific soil conditions, Analele Universităţii din Craiova, seria Agricultură – Montanologie – Cadastru (Annals of the University of Craiova - Agriculture, Montanology, Cadastre Series), Vol. XLIV, 2014, pp. 25-34.
- Available online: https://blog.endaq.com/vibration-measurements-vibration-analysis-basics.
- Mathcad 2001. Available online: https://www.mathcad.com/en.
- Available online: https://real-statistics.com/time-series-analysis/stochastic-processes/autocorrelationfunction/.
- Available online: https://dexonline.ro/definitie/cultivator.
- Available online: https://www.xn--dicionar-qxb.com/Cultivator.
- Polushkin, O.A.; Ignatenko, V.I.; Ignatenko, I.V.; Vyalikov, I.L.; Bogdanovich, V.P. ; Dynamic models of cultivator spring tine performance, MATEC Web Conferences 226, 2018, pp. 1–7.
- Doğan, N.; Doğan, I. ; Determination of the number of bins/classes used in histograms and frequency tables: a short bibliography. Journal of Statistical Research 2010, 7, 77–86. [Google Scholar]
- SOLIDWORKS. Available online: https://www.solidworks.com/.
- SOLIDWORKS. Available online: https://help.solidworks.com/2021/english/SolidWorks/cworks/c_Fatigue_Analysis.htm.
- Cardei, P.; Constantin, N.; Gradinaru, V.; Marin, E.; Manea, D.; Matache, M.; Muraru, V.; Muraru, C.; Pirna, I.; Siru, R.; Sorica, C.; Stanciu, L.; Vladut, V. ; Structural analysis and materials focused on mechanics, mechatronics, maintenance and operation of machinery for agriculture and food industry, Editura Terra Nostra, Iasi, 2012, pp. 166-171.
Figure 1.
The MCLS complex cultivator operates in the version with a working width of 4 m.

Figure 2.
MCLS in operation in the 2 m working width variant.

Figure 3.
MCLS in operation in the 1 m working width variant.

Figure 4.
The location and parcelling of the land on which the experiments were carried out with the MCLS machine.
Figure 4.
The location and parcelling of the land on which the experiments were carried out with the MCLS machine.

Figure 5.
Indexing of measurement locations on the structure.
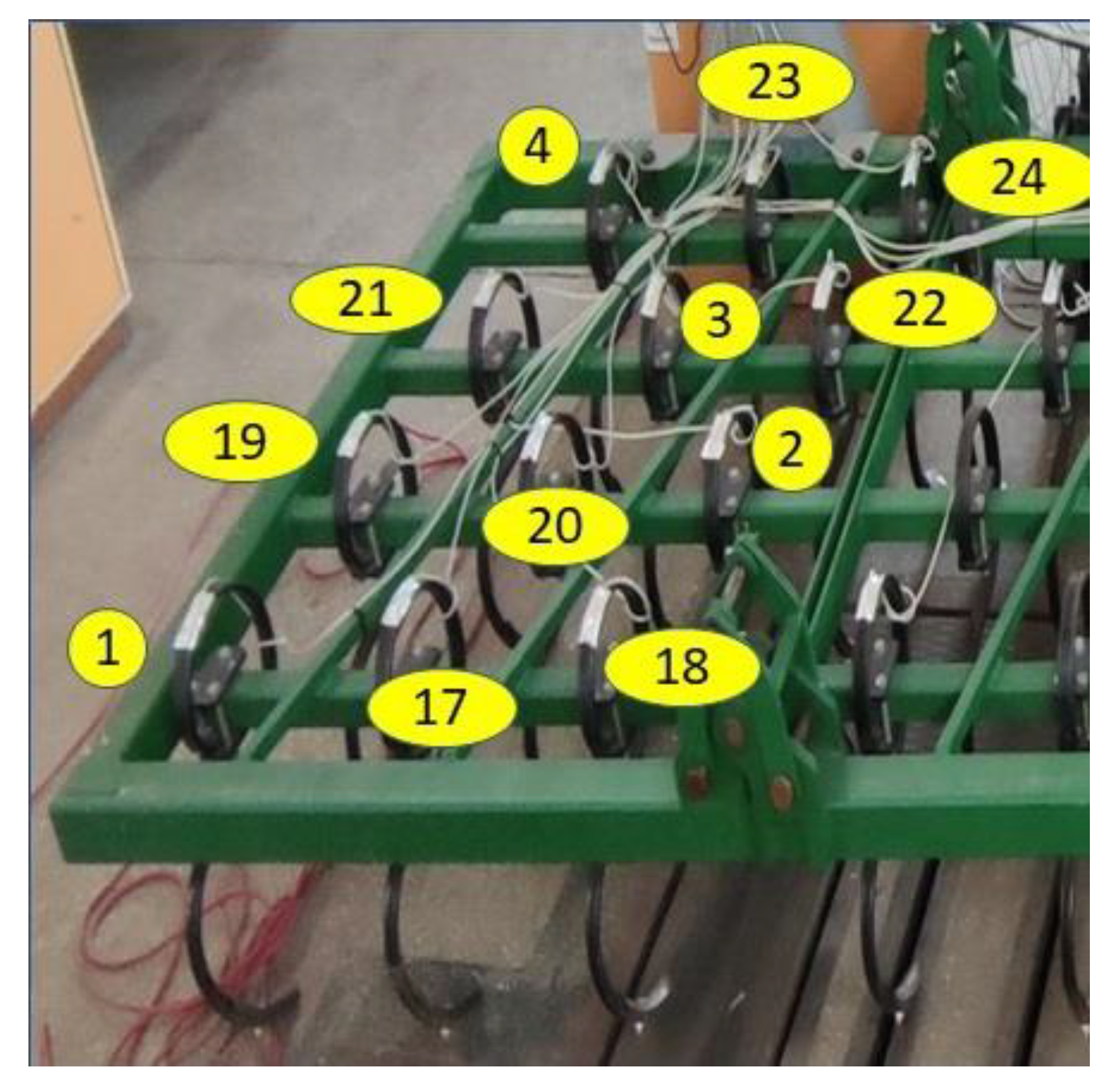
Figure 6.
Variant with a working width of 1 m, in operation with a 45 HP tractor.

Figure 9.
The graphic representation of the distribution functions of the twelve numerical data sequences was obtained by measuring in the locations indicated in Figure 5.
Figure 9.
The graphic representation of the distribution functions of the twelve numerical data sequences was obtained by measuring in the locations indicated in Figure 5.

Figure 10.
The behaviour of average values increases as the number of samples used in their calculation increases.
Figure 10.
The behaviour of average values increases as the number of samples used in their calculation increases.

Figure 11.
Autocorrelations of the ch4 signal, for five offset () values.
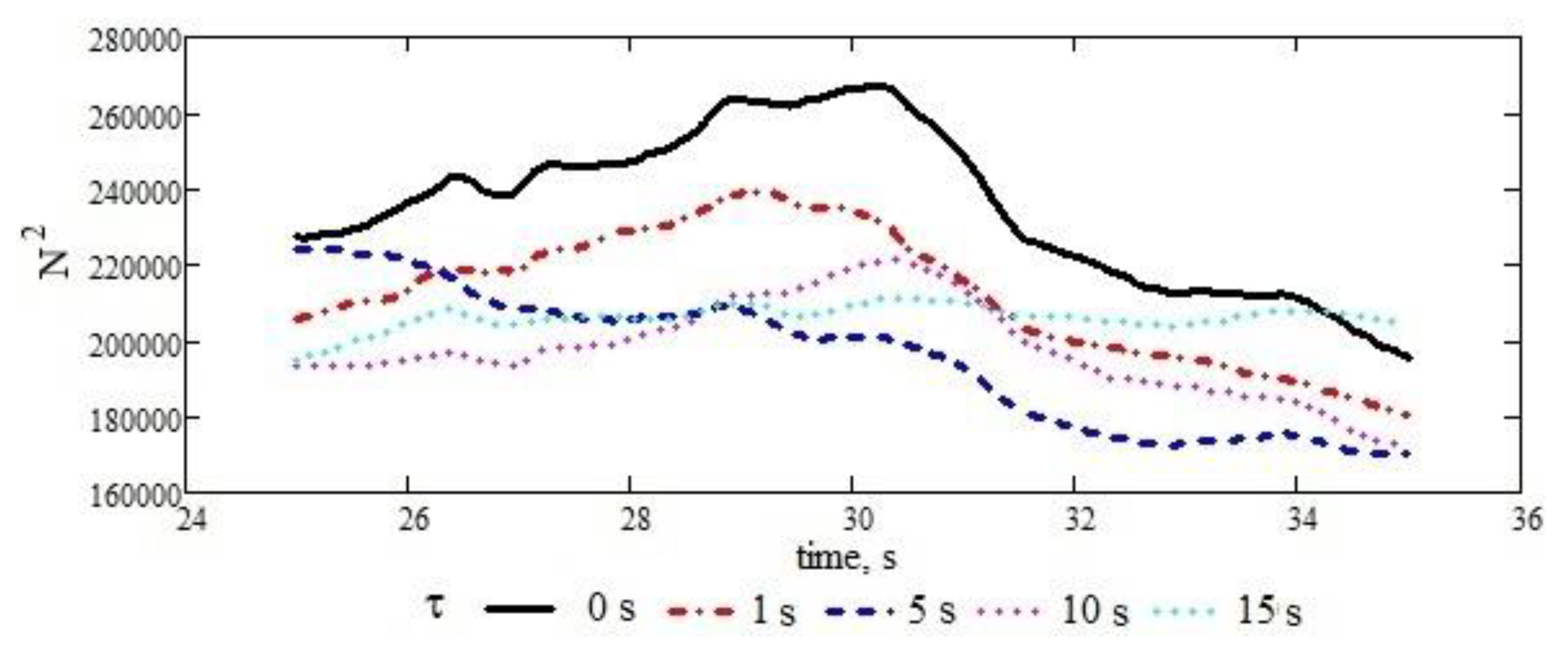
Figure 12.
The autocorrelations of the twelve signals recorded in the experiment, were calculated using [68].
Figure 12.
The autocorrelations of the twelve signals recorded in the experiment, were calculated using [68].
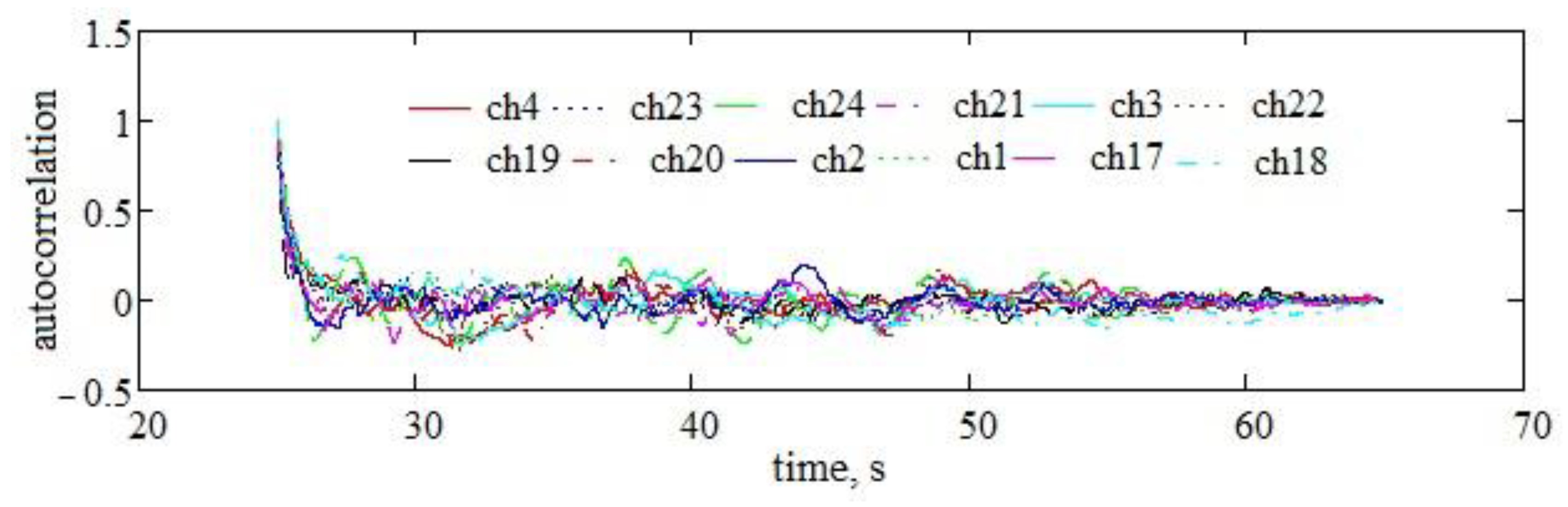
Figure 13.
Representation of the frequency spectrum of the twelve signals from the supports of the working bodies, compared to the fundamental frequency of the support, calculated by the finite element method (represented by the thin red bar with a circle at the upper end).
Figure 13.
Representation of the frequency spectrum of the twelve signals from the supports of the working bodies, compared to the fundamental frequency of the support, calculated by the finite element method (represented by the thin red bar with a circle at the upper end).
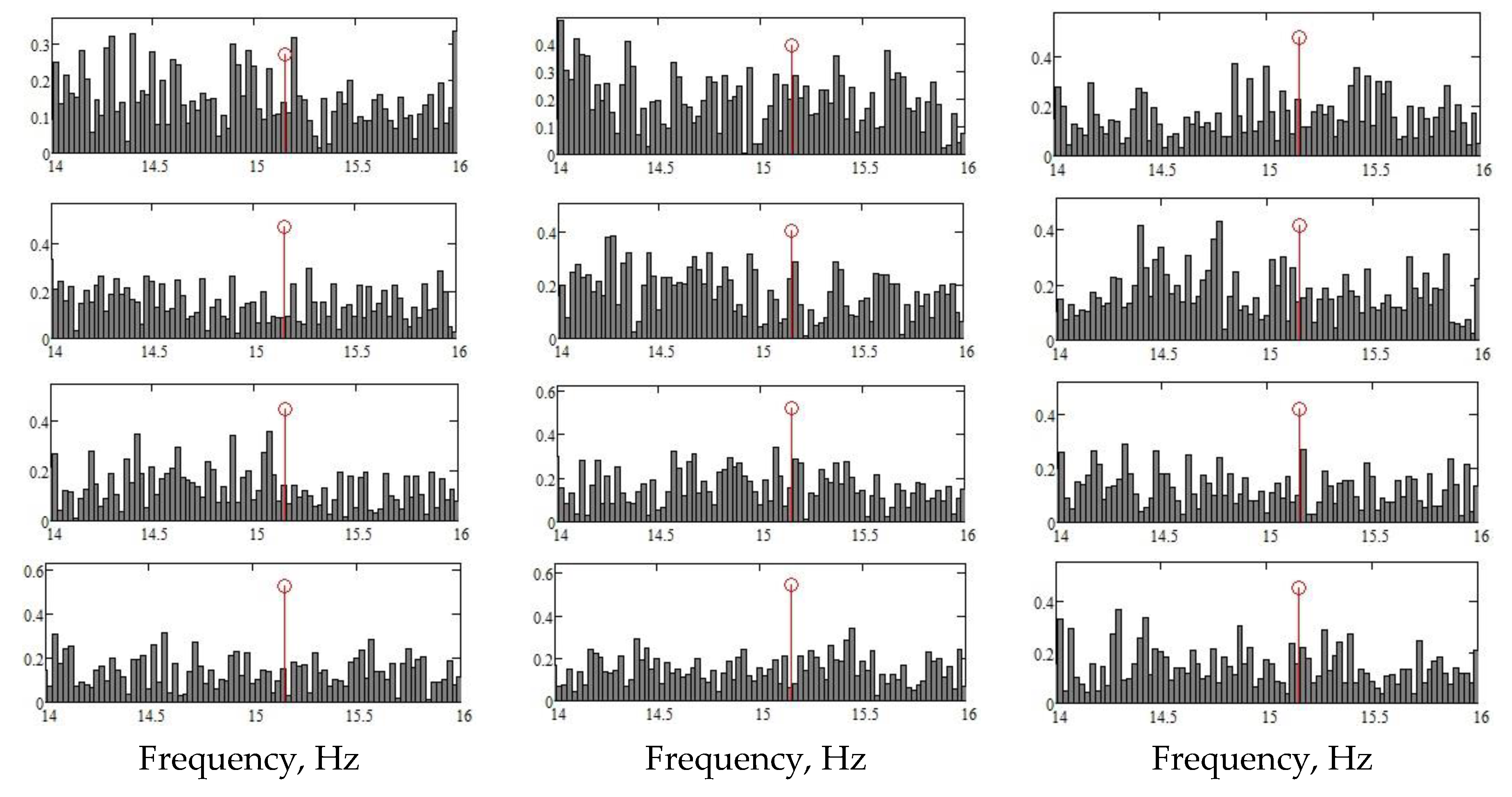
Figure 14.
The first five natural frequencies of the supports of the working bodies of the MCLS, in three variants of fixation on the load-bearing structure and the deformed forms of the structure in the vibration mode correspond to the fundamental frequency.
Figure 14.
The first five natural frequencies of the supports of the working bodies of the MCLS, in three variants of fixation on the load-bearing structure and the deformed forms of the structure in the vibration mode correspond to the fundamental frequency.
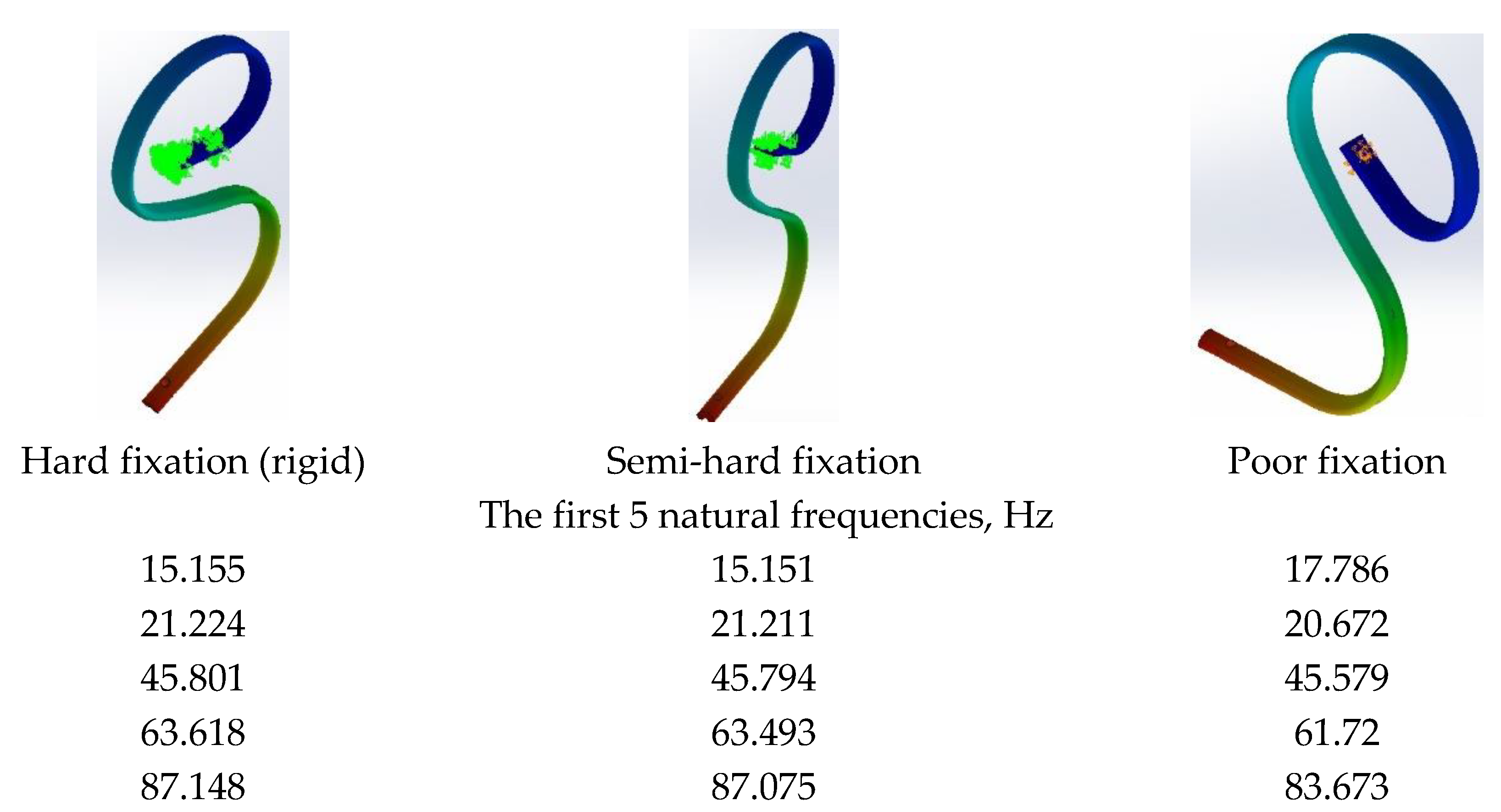
Figure 15.
The frequency spectrum for the twelve signals (in the 0-50 Hz window).

Figure 16.
The frequency spectra of all twelve signals in narrow windows around the first three natural frequencies of the support of the working organ (see Figure 14).
Figure 16.
The frequency spectra of all twelve signals in narrow windows around the first three natural frequencies of the support of the working organ (see Figure 14).

Figure 17.
The von Mises stress map on the section with the maximum load on the support of a working body of the MCLS. The load values were 518 N (left) and 1086 N (right).
Figure 17.
The von Mises stress map on the section with the maximum load on the support of a working body of the MCLS. The load values were 518 N (left) and 1086 N (right).
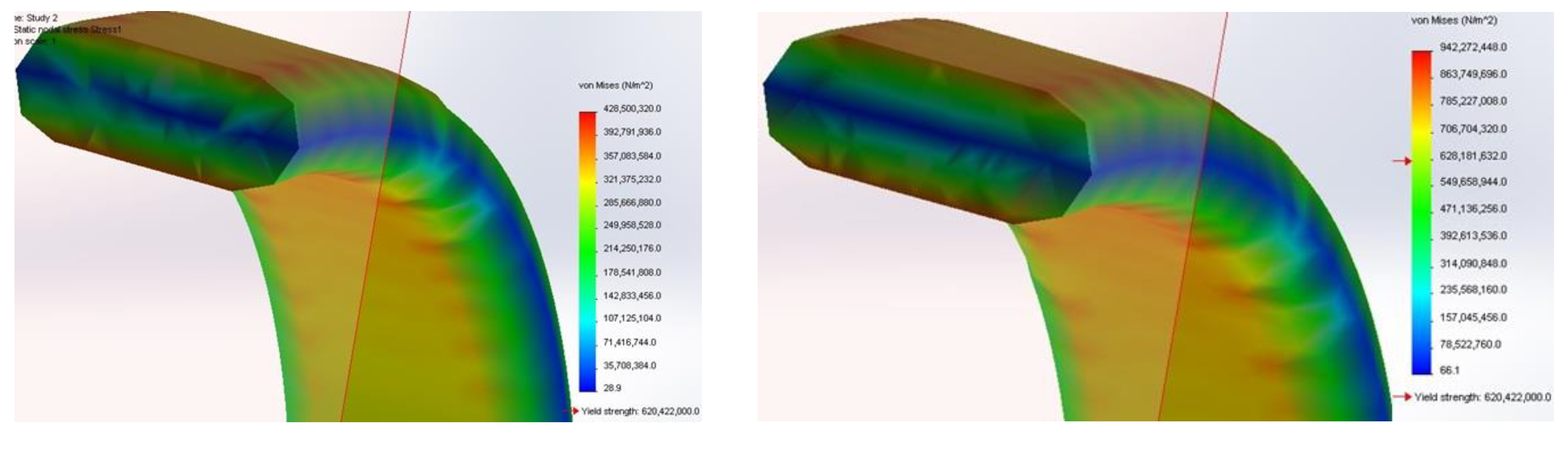
Figure 18.
The variation of the maximum Von Mises stress in the material of the support of the active organs depends on the loading of the organ compared to the critical limits of the material.
Figure 18.
The variation of the maximum Von Mises stress in the material of the support of the active organs depends on the loading of the organ compared to the critical limits of the material.

Figure 19.
The probability that the loading force will exceed the limits of flow, rupture, and fatigue of the material of the support of the working body.
Figure 19.
The probability that the loading force will exceed the limits of flow, rupture, and fatigue of the material of the support of the working body.

Figure 20.
Filtering the sequence of forces that produces the accumulation of fatigue in the signal, corresponds to channel ch4.
Figure 20.
Filtering the sequence of forces that produces the accumulation of fatigue in the signal, corresponds to channel ch4.

Figure 21.
Errors in the working depth are calculated according to the measurements made on each channel.
Figure 21.
Errors in the working depth are calculated according to the measurements made on each channel.

Table 1.
RMS and average values for the numerical sequences corresponding to the twelve measurement points (see Figure 6), organised in the table according to the order of the physical structure.
Table 1.
RMS and average values for the numerical sequences corresponding to the twelve measurement points (see Figure 6), organised in the table according to the order of the physical structure.
| RMS, N | Average Values, N | ||||
|---|---|---|---|---|---|
| 477.145 | 429.029 | 560.354 | 453.124 | 410.584 | 537.523 |
| 549.272 | 405.641 | 498.193 | 536.507 | 385.602 | 483.493 |
| 557.469 | 571.771 | 543.765 | 541.699 | 552.885 | 532.069 |
| 659.646 | 665.703 | 514.895 | 644.269 | 650.431 | 493.188 |
Table 2.
The values of the relative displacements of the tip of the support of the working body, are calculated using a linear-elastic structural model built by the finite element method.
Table 2.
The values of the relative displacements of the tip of the support of the working body, are calculated using a linear-elastic structural model built by the finite element method.
| Force magnitude,N | Relative displacement (deformation), m | ||
|---|---|---|---|
| (lateral) | (forward) | ||
| 100 | 0.001060 | 0.01329 | 0.007152 |
| 200 | 0.003340 | 0.04182 | 0.023520 |
| 500 | 0.009401 | 0.01179 | 0.072160 |
| 1000 | 0.020660 | 0.25910 | 0.190700 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Copyright: This open access article is published under a Creative Commons CC BY 4.0 license, which permit the free download, distribution, and reuse, provided that the author and preprint are cited in any reuse.
MDPI Initiatives
Important Links
© 2024 MDPI (Basel, Switzerland) unless otherwise stated








