Prerpints.org logo
Preprint
Article

Low-Carbon Optimization Design for Low-Temperature Granary Roof Insulation in Different Ecological Grain Storage Zones in China

Altmetrics

Downloads

99

Views

19

Comments

0

A peer-reviewed article of this preprint also exists.

Submitted:

28 July 2023

Posted:

31 July 2023

You are already at the latest version

Alerts
Abstract
The optimization design of buildings is very important the energy consumption, carbon emissions ,and sustainable development of buildings. The low-temperature granary has low grain storage temperature and high energy consumption indexes. The design scheme of roof insulation for low-temperature granary should be determined in actual building design processes by considering economy, carbon emissions, and outdoor climate, comprehensively. In this paper, the low-temperature granary roof insulation for different ecological grain storage zones in China are optimized by using a new low-carbon optimization design method. The low-carbon optimization design method can response to the economical issue, emission reduction issue, and outdoor climate issue, simultaneously. The application results of the optimization design method in ecological grain storage zones in China indicate that outdoor climate has significant impacts on the economic performance and carbon reduction effect of roof insulation. The considering of carbon emission cost can apparently increase economic efficiency of roof insulation. The optimal economic thickness of expanded polystyrene (EPS) in Urumqi, Harbin, Zhengzhou, Changsha, Guiyang and Haikou cities is 0.025 m, 0.037 m, 0.085 m, 0.097 m, 0.072 m and 0.148 m, respectively. The different outdoor climates of seven ecological grain storage areas in China have important influences on the comprehensive economic performances of low-temperature granary roof insulation. The design of low-temperature granary roof insulation in Haikou city has the best economic performances among the seven ecological grain storage zones in China.
Keywords: 
Subject: Engineering  -   Civil Engineering

1. Introduction

Building industry has become one of the most energy-intensive industries in modern society. The product of building materials belongs to high energy-intensive production process. The reason for high energy consumption of buildings is the lack of scientific design and construction of buildings [1]. On the other hand, the lack of energy waste solutions also leads to high building energy consumption. The design and application of insulation in building envelope structures, including roof, floor and exterior walls, is a commonly used energy-saving method for buildings [2]. The low-temperature granary has low grain storage temperature and high energy consumption indexes. Low-temperature granary uses low-temperature storage environments to prevent and control the occurrence of grain loss phenomena because of pests and mold, and ensure the quality of stored grains. The grain storage temperature is below 15℃ in the low-temperature granary, and the large temperature difference between indoor and outdoor air can lead to high energy consumption for low-temperature grain storage [3]. The study of energy-saving and emission reduction techniques for low-temperature granaries has important practical significance and high engineering application value.
Energy-saving and emission reduction methods of buildings are very important to the sustainable development of building, environment, and human society [4, 5]. Zhang et al.[1] analyzed the evolution rules of the three parties’ behavior strategies and evolution stabilization strategies and found the key factors influencing the equilibrium by using the Mitchell’s score-based approach and the system dynamics model. Zhao et al. [2] presented a BIM based low-carbon building design optimization framework. An improved genetic algorithm is utilized to trade off building low-carbon energy saving and daylighting performance. Andrade et al. [6] carried out studies on the carbon reduction potential of ultra-low energy buildings in severely cold regions of China by using an optimization method combining meta-models. Yang et al. [7] investigated seven driving factors of carbon emission evolution, encompassing energy, population, and income, and assessed the historical reduction in CO2 emissions from residential buildings in Henan Province in China by using the Kaya-LMDI decomposition method. Yang et al. [8] examined the value, potential, challenges and improvement path of China’s green hydrogen industry, systematically. Liu et al. [9] investigated the retrofitting of existing residential buildings in China’s hot summer–cold winter climate region to improve energy performance and move towards low-energy building goals. Wei et al. [10] measured the lifecycle carbon emissions of buildings and decomposed the drivers of carbon emissions in the materialization stage and operation stage of building. Optimized design is one of the most efficient ways to reduce energy consumption and carbon emission of buildings. Building design involves economy issue, low-carbon issue, and environmental protection issue, and the optimization design of buildings is a multi-objective optimization problem [11].
Literature review shows that few previous studies consider both the economic performance and carbon reduction effect of building insulation in solving the problem of building insulation design, simultaneously [12, 13]. It is not conducive to achieving the comprehensive optimization of economic performance and carbon reduction effect in building insulation design. So, the comprehensive optimization of economic performance and carbon reduction effect of building insulation is taken into account in this study. In this paper, the low-temperature granary roof insulation for different ecological grain storage zones in China are optimized in terms of carbon reduction by using a new low-carbon optimization design method. The structure of this paper is as follows: Section 2 presents a new low-carbon optimization design method of building roof insulation; Section 3 depicts the application results in the low-temperature granary roof insulation for different ecological grain storage zones in China; Section 4 proposes the results and discussions of this study; Section 5 presents the research conclusions, shortcomings and future research prospects.

2. Materials and Methods

2.1. Accounting for building roof insulation optimization design method

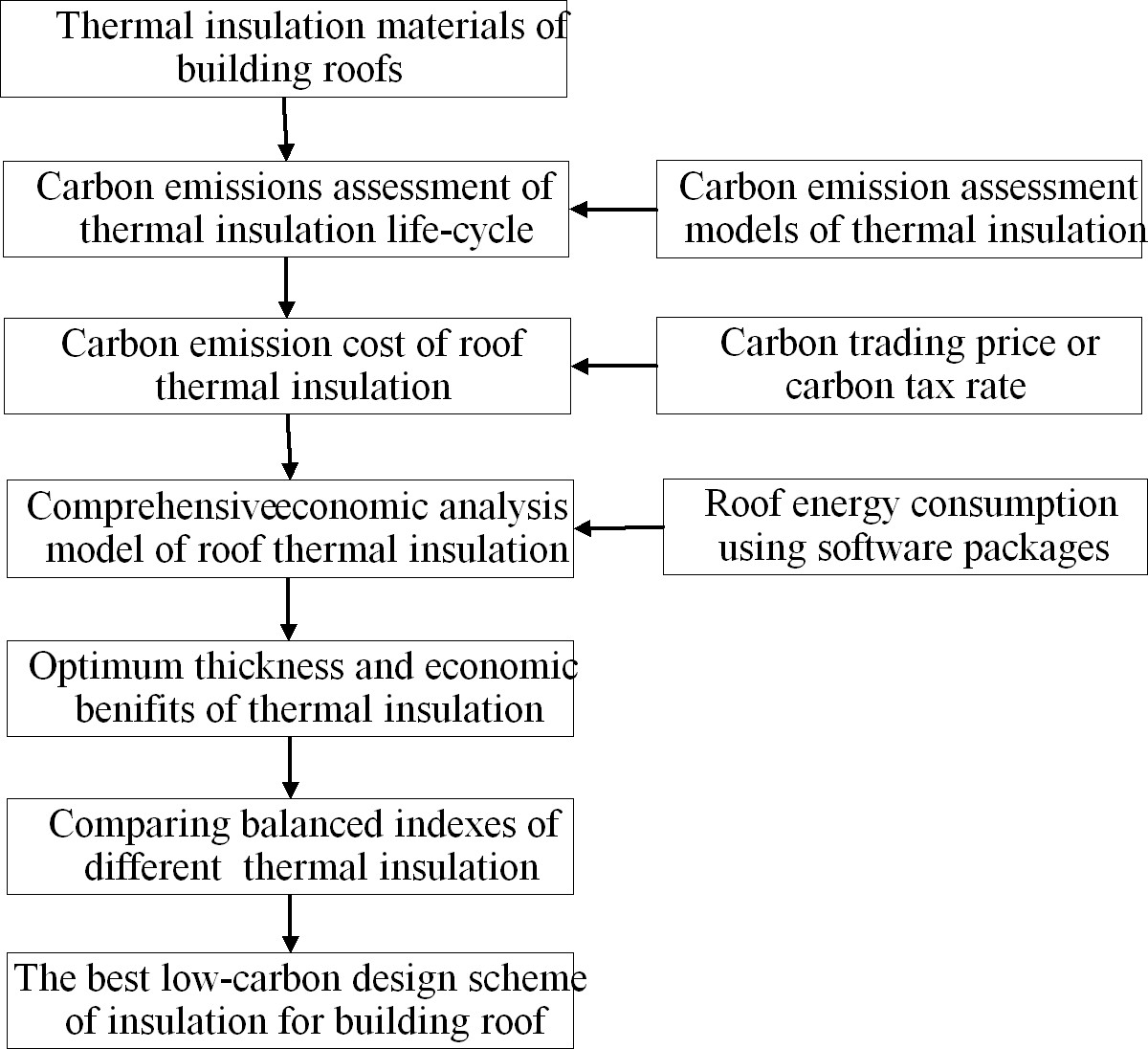
The optimization design of building roof insulation refers to minimizing the sum of roof energy consumption cost, roof construction cost, and carbon reduction effect in the entire life cycle of the roof insulation. In this study, carbon emission of building roof insulation is determined by using the popular carbon emission factor method and process analysis method in the whole life-cycle period. Emission trading scheme (ETS) and carbon tax (CT) are used to establish the relationship between carbon emissions and economic performance of roof insulation [14]. Carbon emission cost of roof insulation can be calculated based on life cycle carbon emissions and carbon tax rate (Or carbon trading price). The traditional P1-P2 economic analysis model for building insulation is modified to consider the carbon emission cost of roof insulation. Balanced index of carbon reduction effect is used to search for the best candidate roof insulation design scheme. The best roof insulation low-carbon design scheme is obtained when the balanced index of carbon reduction effect is the smallest. Figure 1 provides a flowchart for determining the best roof insulation design scheme.

2.2. Carbon emission of low-temperature granary roof insulation

2.2.1. Basic concepts and carbon exchange ways

Carbon emission is the general term or abbreviation for all greenhouse gas (GHG) emissions. According to the United Nations Framework Convention on Climate Change (UNFCCC), GHG includes the following six main categories [15]: 1) CO2; 2) CH4; 3) N2O; 4) HFCs, Example CHF3; 5) SF6, Halogenated ethers and NF3 etc.; 6) PFCs, Example CF4、CnF2n+2. According to the definition of global warming potential (GWP), the overall effect of GHGs is assessed by equivalent values for each type of GHG according to their greenhouse effect corresponding to the mass of CO2 of the same effect, using equivalent conversions. Among them, the equivalent CO2 emissions (CO2e) are often used as a measure of GHG emissions. In addition, other GHGs are usually ignored in general studies due to various considerations such as the specificity of emission sources, the cumulative effect, and the feasibility and validity of data statistics [16].
In the construction processes and the use processes of buildings, there are many carbon exchange ways, which in general can be summarized as follows: 1) Carbon emissions generated during the use of fossil energy; 2) Carbon emissions or bio-carbon sequestration from industrial production such as fossil energy extraction [17]; 3) Carbon emissions from agricultural production such as grain cultivation and nitrogen fertilizer application; 4) Carbon emissions from composting, landfilling or incineration of waste; 5) Indirect carbon reduction through the use of clean energy instead of traditional energy sources; 6) Carbon storage through CO2 capture and storage technology [18].

2.2.2. Determination method of carbon missions for roof insulation

The calculation of carbon emissions of the whole building process is the research focus of the low-carbon building evaluation system. At present, the process analysis method [19] is a common method for carbon emission chemical analysis in the international community. This method is to split the production process according to the process flow, use the product of the measured carbon emission coefficient and the corresponding carbon emission source activity data to express the carbon emission generated by each process, and then calculate the total carbon emission of the whole process according to the carbon emission of each process.
E = ∑ ( ε × Q )
Where, Q and ε are the activity data and emission factors of each process, respectively.
If there is interaction of energy or raw materials in the process flow, it is calculated in a cycle according to Equation (1). Define the technology matrix A ˜ = | a ˜ i j | , where, a ˜ i j is the quantity of the ith product consumed or produced by process j. The relationship between the net production y ˜ and the duration of each process x ˜ is as follows:
A ˜ ⋅ x ˜ = y ˜
Further defining the net output column vector of the product Q ˜ n e t = | Q ˜ n e t , j | and the carbon emission factor row vector ε ˜ = | ε ˜ j | , where ε ˜ j is the carbon emission generated by process j per unit time, and assuming that A ˜ is a non-singular matrix, the total carbon emission can be expressed as:
E = ε ˜ ⋅ A ˜ − 1 ⋅ Q ˜ n e t

2.2.3. Building life cycle assessment

Life Cycle Assessment (LCA) is the only reasonable criterion for selecting materials, equipment and components [20]. This paper divides the system boundary and establishes a quantitative model for the life cycle of roof insulation system based on LCA theory, and describes the greenhouse gas emission reduction policy. Building life cycle can be divided into four stages: part production, construction, building operation and maintenance renewal, and building demolition and disposal [21]. Among them, the production of building parts can be divided into the production process of building materials and equipment. All four stages must use energy, that is, the production process involving energy, while the transportation of building parts and energy transmission and distribution connect these parts to form a whole [22], as shown in Figure 2.
The scope of this paper’s study of the life cycle of roof insulation systems considers the six phases of building production, transportation, construction, operation, demolition and disposal, which can reduce energy consumption and carbon emissions during the use of building equipment (such as cooling, ventilation and lighting) due to the roof insulation [23]. Roof insulation produces almost no greenhouse gas emissions during the operational phase, so carbon emissions during the operational phase are usually ignored, and thus the carbon cost of insulation during the operational phase does not impact the capital investment in roof insulation.

2.2.4. Life cycle carbon emission evaluation model

Life cycle carbon emission of roof insulation ( C t o t ) can be determined through summing carbon emissions in six stages of the entire life cycle for roof insulation.
C t o t = C p r o + C t r a + C c o n + C o p e + C d e m + C d i s
where, C p r o represents carbon emissions from insulation in the production stage, kgCO2e. C t r a represents carbon emissions from insulation in the transportation stage, kgCO2e. C c o n represents carbon emissions from insulation in the construction stage, kgCO2e. C o p e represents carbon emissions from insulation in the operation stage, kgCO2e. C d e m represents carbon emissions from insulation in the demolition stage of insulation, kgCO2e. C d i s represents carbon emissions from insulation in the disposal stage, kgCO2e.
Carbon emissions from insulation in the operation stage can be ignored for almost no greenhouse gas emissions. If building retrofitting occurred, the carbon emissions from building retrofitting can be calculated by using Equation (5). The following is the calculating formula for the carbon emissions in the other five stages of the entire life cycle of roof insulation :
C p r o = ∑ i = 1 m M i ⋅ ( 1 + ε i ) ⋅ F i + C p r o , m a c = ∑ i = 1 l T m a c , i ⋅ F m a c , i
C t r a = ∑ i = 1 n M A i ⋅ D i ⋅ k i ⋅ ψ
C c o n = ∑ i = 1 n T c o n , i ⋅ b i + C c o n , t e m
C d e m = ∑ i = 1 p E d e m , i ⋅ F d e m , i
C d i s = C d i s , t r a + C d i s , r e c
where, C p r o , m a t represents the carbon emissions from raw materials in the production process, kgCO2e. ε i is the loss rate of the ith material in the production process. M i is the ith material consumption, m3. m is the total number of types of insulation. F i is the carbon emission factor of the ith material, kgCO2e/m3. T m a c , i is running time of the ith production equipment, h. F m a c , i is carbon emission factor for the ith production equipment, kgCO2e/h. l is the total number of the concerned equipment in the production process. M A i represents consumption of for ith insulation material, m3. D i represents transportation distance of the ith insulation material, km. k i represents carbon emission factor of the ith insulation material in transportation processes, kgCO2e/km. ψ represents empty return rate of transportation vehicle. C c o n , t e m represents carbon emission of temporary measures at the construction site during the construction phase, kgCO2e. T c o n , i represents running time of the ith construction equipment, h. b i represents carbon emission factor of the ith construction equipment, kgCO2e/h. n represents the number of construction machinery. C d e m represents carbon emissions from insulation in the demolition process, kgCO2e. E d e m , i represents engineering quantity, m2. F d e m , i represents carbon emission factor of theith procedure, kgCO2e/m2. p represents the total number of demolition procedures. C d i s , t r a represents carbon emissions of waste transportation, kgCO2e. C d i s , r e c represents carbon emissions of insulation recycling, kgCO2e.

2.3. Comprehensive economic analysis model for roof insulation

2.3.1. Carbon reduction policies and life-cycle carbon costs

Carbon emissions from insulation in the operation process will be ignored for almost no greenhouse gas emissions. If building retrofitting occurred, the carbon emissions of building retrofitting can be determined by using Equation (5). Carbon emissions in the other five stages of the entire life cycle for roof insulation can be calculated by using the following equations: To combat global warming, many countries have adopted diverse policy measures [24]. There are many classifications of GHG emission reduction policies regarding the building sector, which can be broadly classified into mandatory systems, incentive policies, and GHG pricing-based emission reduction policies. Among them, GHG pricing-based abatement policies are those that aim to reduce GHG emissions by pricing and charging for them. There are two basic forms of GHG pricing-based abatement policies: carbon trading scheme (CTS) and carbon tax (CT). The emission trading scheme is a scheme in which the government sets the total amount of GHG emissions and the sum of emission permits issued for companies or individuals cannot exceed the upper limit of GHG emissions. The government can issue GHG emission permits in the form of free or auction [25]. There are various emission trading mechanisms, such as cap-and-trade, voluntary, and baseline-credit models, and the basic principles of their operation [26] , as shown in Figure 3.
When there is great uncertainty in the marginal cost of GHG emission reduction, the effect of emission trading mechanism to control GHG emissions is better than carbon tax, and the government can accurately control the total amount of GHG emissions within the predicted range by using this mechanism. However, it is more difficult for the government to design carbon emissions trading mechanism compared to carbon tax, and it also requires higher management and transaction costs. If the allocation of GHG emission allowances lacks public transparency or leaves more room for manipulation by powerful capital groups, it may lead to rent-seeking behavior by interested parties [27].
Carbon tax refers to the government setting a tax rate on GHG emissions and using the price of GHG emissions to further drive producers or consumers to determine the total GHG emissions of firms or individuals [24]. The basic principle of carbon tax operation is shown in Figure 4. Garbaccio et al. [25] analyzed the potential impact of carbon tax on China’s economic development based on a general equilibrium model, and the results showed that the government’s carbon tax is beneficial to reduce carbon emissions, while it can promote the long-term growth of China’s economy. Compared to other policy instruments, carbon taxes have lower administrative and transaction costs and can reconfigure the types of taxes while improving the rationality of the taxation mechanism [29]. The fiscal revenue obtained by the government through the carbon tax can be used to implement supporting incentive policies to further influence people’s perceptions and behaviors of emission reduction [30], thus forming a virtuous cycle.
At present, with the existing level of emission reduction technology in China, it is important to use the emission reduction policy for the promotion of low-carbon technologies in buildings. In order to further elaborate the implementation effect of GHG emission reduction tools, the following four coordinate systems are established. Among them, the vertical and horizontal coordinates are the GHG emission intensity and construction cost per unit area, respectively, and the curve indicates the inverse relationship between the horizontal and vertical coordinates, and the circle indicates the overall construction side. As shown in Figure 5 (a), when the government has no intervention, the GHG emission intensity of construction has no the upper limit, which is not conducive to the low carbon development of China’s construction industry.
As shown in Figure 5 (b), when the government introduces mandatory laws and regulations on carbon emission reduction, the GHG emission intensity of construction enterprises is required to not exceed the specified standard. As shown in Figure 5 (c), when the government adopts incentive policies to guide construction enterprises to adopt green and low GHG-emitting materials, the enterprises can put the government subsidies into the construction, which reduces the construction cost of the enterprises correspondingly, and the construction cost curve is thus shifted. However, the incentive policy requires high-cost investment and cannot be widely promoted, so most enterprises still keep their low-cost and high-emission production methods unchanged. As shown in Figure 5 (d), when the government adopts the GHG pricing-based abatement policy tool, the cost of GHG emissions generated by buildings is internalized as a part of the cost of the enterprises. During the implementation of the policy, the government will give a certain amount of GHG quotas to enterprises free of charge, and if the enterprises generate more GHG than the total amount of quotas, they need to buy GHG quotas in the market. Meanwhile, enterprises that produce less than their GHG quotas can sell their excess quotas in the market, thus stimulating their emission reduction behavior and changing the curve of production cost at the same time.
The previous two policy tools are easy to implement but have limitations in terms of emission reduction effects. The GHG pricing-based policy tools are more efficient, do not require higher cost investment from the government, and can be promoted vigorously throughout the society, which is beneficial to promote GHG-emission reduction in the construction industry and the optimization and upgrading of industrial structure.

2.3.2. Carbon emission costs roof thermal insulation

Energy conservation, economic performance, carbon reduction and the other influence factors should be considered in the optimization design of roof insulation. These three influencing factors will interact with each other. Energy conservation due to roof insulation will reduce electricity consumption cost caused by building roofs. Energy conservation due to roof insulation will also reduce carbon emissions because of electricity consumption reeducation. Currently, there is still no quantitative determination methods of the impact of carbon reduction on the economic performance of roof insulation. The new occurrence of carbon control measures: carbon trading [31] and carbon tax [32] provides a potential economic method for quantifying carbon emissions of roof insulation. The relationship between carbon emissions and economic performance of roof insulation can be established by using the carbon trading scheme and carbon tax. The carbon trading market internalizes carbon emissions as a part of enterprise operating costs. So, the carbon emission cost can be included in the economic analysis models of roof insulation. The following is the calculating formula of carbon emission cost:
C = i n s 0.001 S t o t ⋅ q t a x , c a r V
where, C i n s is carbon emission cost of roof insulation, USD/m3. S t o t is the total carbon emissions of roof insulation, tCO2e. q t a x , c a r is carbon emission price, USD/tCO2e. V represents the amount of the consumed roof insulation, m3.

2.3.3. Comprehensive economic analysis model for roof insulation

Carbon emission cost will influence the economic indexes for insulation in low-temperature granary roof. The traditional P1-P2 economic analysis model has been applied in evaluating economic performances of building insulation [33]. In this paper, carbon emission cost will be included in the P1-P2 economic analysis model, which can evaluate economic performances of insulation comprehensively. When the life cycle total investment cost of insulation ( L C T i n ) is the minimum or life cycle saving of insulation ( L C S i n ) is the maximum, the insulation layer thickness is optimal. The improved P1-P2 economic analysis model for insulation are given as follows:
L C T i n = P 1 ⋅ E C c o + P 2 ( G i ⋅ λ + C i n s ⋅ λ + I C )
L C S i n = P 1 ⋅ ( E C s a + E C t ) − P 2 ( G i ⋅ λ + I C )
P 1 = P W F ( L c , r , z ) = { 1 r − z [ 1 − 1 + z 1 + r ] L c , r ≠ z L c 1 + r , r = z
P 2 = D P + ( 1 − D P ) P W F ( N min , 0 , z ) P W F ( N m , 0 , c ) + P 1 ⋅ M − R ( 1 + z ) L c
L p = { ln [ 1 − P 2 ( G i ⋅ λ + C c ) ( r − w ) Δ E c ] ln ( 1 + w 1 + r ) , r ≠ z P 2 ( G i ⋅ λ + C c ) ( z − r ) Δ E c , r = z
where, P 1 is the ratio of the first year’s energy consumption cost to the total energy consumption cost. P 2 is the ratio of total investment to initial investment in roof insulation. E C c o is energy consumption capital investment, USD/m2. E C s a is the decrease of energy consumption capital investment because of roof insulation, USD/m2. E C t is the decrease of carbon emission capital investment because of roof insulation, USD/m2. G i is the price of insulation material, USD/m3. I C is the comprehensive capital investment of insulation, USD/m2. λ is insulation layer thickness, m. P W F is the present worth factor. r is market rate. r =1% [34]. z is the inflation rate. z =5% [35]. L c is the time period of the entire lifecycle, year. D P is the ratio of the down payment to the capital investment. D P = 100 % [36]. M is the proportion of installment payments to total investment in roof insulation. N m is loan period. N min = min ( L c , N m ) . c is the loan interest rate. R is the proportion of resale price to total investment in roof insulation. R = 0 . L p is the discounted payback period, year.

2.4. Balanced index of carbon reduction effect

Assessment indexes of carbon emissions can be used to assess different control measures for carbon emissions of buildings. Conventional index [37], load index [38], and balanced index [39] are three common types of assessment indexes of carbon emissions. In this paper, balanced index of carbon reduction effect can be used to investigated comprehensively the carbon emission factor and the additional capital consumption factor [40]. Balanced index of carbon reduction effect ( B i ) can be calculated by Equation (16). The value of balanced index of carbon reduction effect decreases with the increase of return rate of investment in carbon emission reduction. The best roof insulation low-carbon design scheme is obtained when the balanced index of carbon reduction effect is the smallest.
B i = I r ⋅ E R o I o ⋅ E R r
where, I o is the capital investment without control measures for carbon emissions, USD/m2. E R o is the carbon emission without l control measures for carbon emissions, kgCO2e/m2. I r is the capital investment because of roof insulation, USD/m2. E R r represents the carbon emission reduction for control measures for carbon emissions, kgCO2e/m2.

3. Application of optimization design method in low-temperature granary roof

3.1. Typical cities in China and concerned building

The low-temperature granary is used as an object in this study because its indoor air temperature (Below 15℃) is lower than other building types. China has a wide area and large differences in climate from one place to another [41]. The climate condition is one of the most important external influencing factors that must be considered for low-carbon design of low-temperature granary. The roof insulation optimization design method was applied the low-temperature granary roofs in seven ecological grain storage areas in China. Seven typical cities were selected from the seven ecological grain storage zones in China. These seven typical cities are Xining city (With typical Qinghai-Tibet Plateau grain storage climate characteristics), Urumqi city (With typical Mengxin grain storage climate characteristics), Harbin city (With typical northeast grain storage climate characteristics), Zhengzhou city (With typical north China grain storage climate characteristics), Changsha city (With typical central China grain storage climate characteristics), Guiyang city (With typical southwest grain storage climate characteristics) and Haikou city (With typical south China grain storage climate characteristics).
Typical roof structure of low-temperature granary was used to analyze the economic performances of roof insulation in seven ecological grain storage zones in China. Table 1 shows the performance parameters of roof elements for typical roof structure of low-temperature granary. The indoor air temperature in the low-temperature granary is set as 15℃. The grain storage temperature in low-temperature granary is maintained at 15℃ by using the air-conditioning systems with 2.3 energy efficiency ratio. The life cycle of insulation materials is set as 20 years.

3.2. Life-cycle carbon emissions of roof thermal insulation

COMSOL software and the EnergyPlus software were utilized to forecast the energy consumption of low-temperature granary roofs, respectively. To ensure the accuracy of the prediction results, the simulation results are mutually verified with each other. The total annual cooling load of low-temperature granary roof were obtained through summing the hour-by-hour heat transfer per unit area of the roof. Table 2 shows the roof cooling loads for the seven typical cities. Xining city is a typical representative city of Qinghai-Tibet Plateau grain storage climate characteristics. It should be noted that the number of months with outdoor air average temperature >15°C is 0. Therefore, the low-temperature granary in Xining city do not require mechanical cooling to maintain the lower grain storage temperatures. Xining city will not be involved in the economic performance analysis of granary roof insulation.
Four types of common insulation materials in granary roof were discussed in this study. These four types of common insulation materials were rock wool (RW), extruded polystyrene (XPS), polyurethane (PU), and expanded polystyrene (EPS). Carbon emissions and corresponding carbon emission cost for the above four types of insulation materials were calculated by using the methods presented in Section 2.3, as shown in Table 3. The carbon emission of EPS is 0.451 tCO2e/m3, which is the smallest carbon emission among the four types of insulation materials. Therefore, EPS is used in this paper as the insulation material in low-temperature granary roof in seven ecological grain zones in China. In this paper, electricity price is set as 0.1027 USD/kWh. The carbon trading price is assumed to be 8.29 USD/tCO2e [42], and the electricity price is 0.0083 USD/ kWh.
This section may be divided by subheadings. It should provide a concise and precise description of the experimental results, their interpretation, as well as the experimental conclusions that can be drawn.

4. Results and discussions

4.1. Economic performance analysis of granary roof insulation

Figure 6 shows the calculated results of L C T for EPS in granary roof in the selected six typical cities in China with different insulation thickness. Figure 7 shows the calculated results of L C S for EPS in granary roof in the selected six typical cities in China with different insulation thickness. There is a minimum point of L C T , and this point corresponds to the thickness of the insulation material that is the optimal thickness. When the material thickness is less than or thicker than this economic thickness, the L C T will all increase accordingly. There is a maximum minimum point of L C S , and this point also corresponds to the thickness of the insulation material that is the optimal thickness. When the material thickness is less than or thicker than this economic thickness, the L C S will all decrease accordingly. The calculated minimum values of L C T in Urumqi city, Harbin city, Zhengzhou city, Changsha city, Guiyang city and Haikou city is 13.98 USD/m2, 15.94 USD/m2, 23.59 USD/m2, 25.37 USD/m2, 21.40 USD/m2and 33.27 USD/m2, respectively. Meanwhile, the L C S of Urumqi city, Harbin city, Zhengzhou city, Changsha city, Guiyang city and Haikou city is 4.01 USD/m2, 8.13 USD/m2, 40.63 USD/m2, 49.85 USD/m2, 29.14 USD/m2 and 105.76 USD/m2, respectively. The ranking of economic benefits of six typical cities is Urumqi < Harbin < Guiyang < Zhengzhou < Changsha < Haikou. Therefore, the Haikou city achieves the best economic benefits among the six typical cities, followed by Changsha city, and Harbin city and Urumqi city have smaller economic benefits. Since Harbin city and Urumqi city with their natural climatic conditions can meet the requirements of low-temperature grain storage in most cases, the energy consumption saved by using low-temperature grain storage technology is less compared to other cities, and thus the economic benefits it brings are not significant.

4.2. Roof insulation optimal insulation layer thicknesses

When the carbon emission cost of roof insulation is not considered, the optimal insulation layer thicknesses in low-temperature granary for Urumqi city, Harbin city, Zhengzhou city, Changsha city, Guiyang city and Haikou city is 0.026 m, 0.039 m, 0.088 m, 0.101 m, 0.075 m and 0.152 m, respectively. When the carbon emission cost of roof insulation is considered, the optimal insulation layer thicknesses in low-temperature granary for Urumqi city, Harbin city, Zhengzhou city, Changsha city, Guiyang city and Haikou city is 0.025 m, 0.037 m, 0.085 m, 0.097 m, 0.072 m and 0.148 m, respectively. Therefore, the carbon emission costs because of roof insulation decreased by 3.85 % ~ 5.13 % for the selected six typical cities. After determining the optimal insulation layer thicknesses in low-temperature granary, the payback periods of roof insulation has been determined by using the Equation (15). When the carbon emission cost of roof insulation is not considered, the payback period of roof insulation in low-temperature granary for Urumqi city, Harbin city, Zhengzhou city, Changsha city, Guiyang city and Haikou city is 2.62 years, 2.55 years, 1.95 years, 1.86 years, 2.17 years and 1.45 years, respectively. When the carbon emission cost of roof insulation is considered, the payback period of roof insulation in low-temperature granary for Urumqi city, Harbin city, Zhengzhou city, Changsha city, Guiyang city and Haikou city is 2.58 years, 2.43 years, 1.89 years, 1.79 years, 2.09 years and 1.41 years, respectively. As shown in Figure 8, the carbon emission costs of roof insulation cause the payback periods to be reduced by 1.52 % ~ 4.71 %. The smaller payback period is, the better the economic performance of the insulation material is.

4.3. Effect of carbon emission cost on economic performance of roof insulation

The lifecycle carbon emission cost due to roof insulation will increase the lifecycle total investment of building roof insulation. Considering the carbon emission cost, the increase in L C T value for six typical cities is 0.67 % to 1.69 %, as shown in Figure 9. Figure 10 shows the increase in L C S after considering carbon emission costs. Energy consumption reduction because of insulation will decrease the carbon emissions due to energy consumption. Reduction of carbon emission cost will bring about the increase of L C S for roof insulation. The L C S increases by 1.78 USD/m2, 1.55 USD/m2, 5.22 USD/m2, 5.51 USD/m2, 4.06 USD/m2 and 11.54 USD/m2 for Urumqi city, Harbin city, Zhengzhou city, Changsha city, Guiyang city and Haikou city, respectively. The increase in L C S after considering carbon emission cost is 12.25 %~24.06 %. It is worth noting that the increase of L C S (12.25 % ~ 24.06 %) is much higher than the increase of L C T (0.67 % ~ 1.69 %). Therefore, considering carbon emission cost can significantly improve the economic benefits of roof insulation in low-temperature granary.

4.4. Calculated balanced indexes for different ecological grain storage zones

The balanced indexes of roof insulation in low-temperature granary were calculated by using Equation (16). Figure 11 shows the balanced indexes of roof insulation with and without considering carbon emission costs for the six typical cities. For each city, the balanced index of roof insulation after considering carbon emission cost is smaller than that without considering carbon emission cost. When the carbon emission cost is considered, the variation value of the balanced indexes of roof insulation for the six typical cities are 0.066 ~ 0.108. The priority order of the balanced indexes of roof insulation for six typical cities is: Haikou city > Changsha city > Zhengzhou city > Guiyang city > Harbin city > Urumqi city. The economic benefit of roof insulation in low-temperature granary for Haikou city is the greatest among the six typical cities in China.

5. Conclusion

Although carbon trading mechanism, carbon tax and the other carbon reduction policy and measures are beneficial to urban environmental protection and building sustainable development, studies on the low-carbon optimization design of buildings is still very insufficient. In this paper, the low-temperature granary roof insulation for different ecological grain storage zones in China are optimized in terms of carbon reduction. The following conclusions were obtained.
1) A novel low-carbon design optimization method of roof insulation is very useful to determine the best insulation design scheme in low-temperature granary roof.
2) The P1-P2 economic analysis model is modified to cope with the carbon emissions of roof insulation. The carbon emission cost has been found to have a certain influences on the economic performance of low-temperature granary roof insulation.
3) The outdoor climates of different ecological grain storage zones in China have significant impacts on the economic performance and carbon reduction effect of low-temperature granary roof insulation.
4) The priority order of the balanced indexes of roof insulation for six typical cities is: Haikou city > Changsha city > Zhengzhou city > Guiyang city > Harbin city > Urumqi city. The economic benefit of roof insulation in low-temperature granary for Haikou city is the greatest among the six typical cities in China.
This paper only takes the roof insulation materials in buildings as the research object, and investigates the low-carbon design optimization of roof insulation. In the future, the research scope can be extended to the low-carbon design optimization of the whole building. Although carbon trading mechanism, carbon tax and the other carbon reduction policy and measures are very helpful to reduce carbon emissions in buildings, more low-carbon economic analysis method and more insulation material types should be studied to achieve the goal of building energy conservation and emission reduction.

Author Contributions

Dinan Li: conceptualization, methodology, investigation. Yuge Huang: writing - review & editing, data curation, conceptualization. Chengzhou Guo: supervision, formal analysis. Haitao Wang: writing-original draft. Lu Huang: validation. Jianwei: validation.

Funding

The research work presented in this paper is financially supported by Science and Technology Planning Project of Henan Province of China (No.232102320225), the Open Project Program of Henan Key Laboratory of Grain and Oil Storage Facility & Safety (NO. 2022KF05), Cultivation Programme for Young Backbone Teachers of Henan University of Technology (No. 21420069), and Innovative Funds Plan of Henan University of Technology (No. 2020ZKCJ05, 2020ZKCJ022).

Institutional Review Board Statement

The study did not require ethical approval.

Informed Consent Statement

Informed consent was obtained from all subjects involved in the study.

Data Availability Statement

Not applicable.

Conflicts of Interest

The authors declare no conflict of interest.

References

  1. Zhang, Z.; Song, J.; Wang, W. Study on the behavior strategy of the subject of low-carbon retrofit of residential buildings based on tripartite evolutionary game. Sustainability 2023, 15, 7629. [Google Scholar] [CrossRef]
  2. Zhang, Z.; Wang, W.; Song, J.; Wang, Z.; Wang, W. Multi-objective optimization of ultra-low energy consumption buildings in severely cold regions considering life cycle performance. Sustainability 2022, 14, 16440. [Google Scholar] [CrossRef]
  3. Carcassi, O.B.; Minotti, P.; Habert, G.; Paoletti, I.; Claude, S.; Pittau, F. Carbon footprint assessment of a novel bio-based composite for building insulation. Sustainability 2022, 14, 1384. [Google Scholar] [CrossRef]
  4. Luo, T.; Tan, Y.T.; Langston, C.; Xue, X.L. Mapping the knowledge roadmap of low carbon building: Ascientometric analysis. Energy Build. 2019, 194, 163–176. [Google Scholar] [CrossRef]
  5. Zhang, Y.; Qin, M.; Lv, M.; Li, Y. Data-based analysis of environmental attractiveness towards low-carbon development in seaside cities. Buildings 2022, 12, 2197. [Google Scholar] [CrossRef]
  6. Andrade, I.; Land, J.; Gallardo, P.; Krumdieck, S. Application of the InTIME methodology for the transition of office buildings to low carbon—a case study. Sustainability 2022, 14, 12053. [Google Scholar] [CrossRef]
  7. Yang, X.; Sima, Y.; Lv, Y.; Li, M. Research on influencing factors of residential building carbon emissions and carbon peak: a case of Henan province in China. Sustainability 2023, 15, 10243. [Google Scholar] [CrossRef]
  8. Yang, L.; Wang, S.; Zhang, Z.; Lin, K.; Zheng, M. Current Development Status, Policy Support and Promotion Path of China’s Green Hydrogen Industries under the Target of Carbon Emission Peaking and Carbon Neutrality. Sustainability 2023, 15, 10118. [Google Scholar] [CrossRef]
  9. Liu, C.; Sharples, S.; Mohammadpourkarbasi, H. A review of building energy retrofit measures, passive design strategies and building regulation for the low carbon development of existing dwellings in the hot summer–cold winter region of China. Energies 2023, 16, 4115. [Google Scholar] [CrossRef]
  10. Wei, J.; Shi, W.; Ran, J.; Pu, J.; Li, J.; Wang, K. Exploring the Driving Factors and Their Spatial Effects on Carbon Emissions in the Building Sector. Energies 2023, 16, 3094. [Google Scholar] [CrossRef]
  11. Xiang, J.; Liu, H.; Li, X.; Jones, P.; Perisoglou, E. Multi - objective optimization of ultra-low energy housing in hot summer cold winter climate zone of China based on a probabilistic behavioral model. Buildings 2023, 13, 1172. [Google Scholar] [CrossRef]
  12. Wang, Z.L.; Zhang, S.Y.; Qiu, L.M. A new low-carbon design method based on multi-agent interactive reinforcement learning. Proc IMechE Part C: J. Mechanical. Engineering. Science 2019, 233, 539–553. [Google Scholar] [CrossRef]
  13. Han, Y.M.; Li, J.Z.; Lou, X.Y.; Fan, C.Y.; Geng, Z.Q. Energy saving of buildings for reducing carbon dioxide emissions using novel dendrite net integrated adaptive mean square gradient. Appl. Energy 2021, 309, 118409. [Google Scholar] [CrossRef]
  14. Ren, S.D.; Gui, F.Z.; Zhao, Y.W.; Zhan, M.; Wang, W.L.; Zhou, J.Q. An Extenics-based scheduled configuration methodology for low-carbon product design in consideration of contradictory problem solving. Sustainability 2021, 13, 5859. [Google Scholar] [CrossRef]
  15. Su, S.; Wang, Q.; Han, L.X.; Hong, J.Q.; Liu, Z.W. BIM-DLCA: An integrated dynamic environmental impact assessment model for buildings. Build. Environ. 2020, 183, 107218. [Google Scholar] [CrossRef]
  16. Cang, Y.J.; Luo, Z.X.; Yang, L.; Han, B. A new method for calculating the embodied carbon emissions from buildings in schematic design: Taking “building element” as basic unit. Build. Environ. 2020, 185, 107306. [Google Scholar] [CrossRef]
  17. Peng, J.; Li, W.Q.; Li, Y.; Xie, Y.M.; Xu, Z.L. Innovative product design method for low-carbon footprint based on multi-layer carbon footprint information. J. Clean. Prod. 2019, 228, 729–745. [Google Scholar] [CrossRef]
  18. Wang, Z.L.; Zhang, S.Y.; Qiu, L.M. A new low-carbon design method based on multi-agent interactive reinforcement learning. Proc IMechE Part C: J. Mechanical. Engineering. Science 2019, 233, 539–553. [Google Scholar] [CrossRef]
  19. Ylmen, P.; Mjornell, K.; Berlin, J.; Arfvidsson, J. Approach to manage parameter and choice uncertainty in life cycle optimisation of building design: Case study of optimal insulation thickness. Build. Environ. 2021, 191, 107544. [Google Scholar] [CrossRef]
  20. Mohsin, M.; Rasheed, A.K.; Sun, H.P. Developing low carbon economies: an aggregated composite index based on carbon emissions. Sustain. Energy Techn. 2019, 35, 365–374. [Google Scholar] [CrossRef]
  21. Mohsin, M.; Rasheed, A.K.; Sun, H.P. Developing low carbon economies: an aggregated composite index based on carbon emissions. Sustain. Energy Techn. 2019, 35, 365–374. [Google Scholar] [CrossRef]
  22. Habibi, S.; Valladares, O.P.; Peña, D.M. Sustainability performance by ten representative intelligent Façade technologies: A systematic review. Sustain. Energy Techn. 2022, 52(A), 102001. [Google Scholar] [CrossRef]
  23. Rosti, B.; Omidvar, A.; Monghasemi, N. Optimal insulation thickness of common classic and modern exterior walls in different climate zones of Iran. J. Build. Eng. 2020, 27, 100954. [Google Scholar] [CrossRef]
  24. Khastar, M.; Aslani, A.; Nejati, M.; Bekhrad, K.; Naaranoja, M. Evaluation of the carbon tax effects on the structure of Finnish industries: A computable general equilibrium analysis. Sustain. Energy Techn. 2020, 37, 100611. [Google Scholar] [CrossRef]
  25. Timilsina, G.R. Carbon tax under the Clean Development Mechanism: a unique approach for reducing greenhouse gas emissions in developing countries. Climat. Pol. 2009, 9, 139–154. [Google Scholar] [CrossRef]
  26. Jia, Z.; Lin, B. Rethinking the choice of carbon tax and carbon trading in China. Technolog. Forecast. Soc. 2020, 159, 120187. [Google Scholar] [CrossRef]
  27. Quan, B.A.I.; Shan, H.U.; Li, J.G. Interpretation of IPCC AR6 on buildings. Adv. Clim. Chang. Res. 2022, 18, 557. Available online: http://www.Climatechange.cn/EN/Y2022/V18/I5/557.
  28. Garbaccio, R.F.; Ho, M.S.; Jorgenson, D.W. Controlling carbon emissions in China. Environ. Dev. Econ. 1999, 4, 493–518. [Google Scholar] [CrossRef]
  29. Li, D.Z.; Chen, H.X.; Hui, E.M.; Zhang, J.B.; Li, Q.M. A methodology for estimating the life-cycle carbon efficiency of a residential building. Build Environ. 2013, 59, 448–455. [Google Scholar] [CrossRef]
  30. Zhang, X.C.; Wang, F.L. Life-cycle assessment and control measures for carbon emissions of typical buildings in China. Build. Environ. 2015, 86, 89–97. [Google Scholar] [CrossRef]
  31. Liu, J.Y.; Zhang, Y.J. Has carbon emissions trading system promoted non-fossil energy development in China. Appl. Energ. 2021, 302, 117613. [Google Scholar] [CrossRef]
  32. Lou, Y.L.; Yang, Y.Z.; Ye, Y.Y.; He, C.; Zuo, W.D. The economic impacts of carbon emission trading scheme on building retrofits: A case study with U. S. medium office buildings. Build. Environ. 2022, 221, 109311. [Google Scholar] [CrossRef]
  33. Duffie, J.A.; Beckman, W.A. Solar energy thermal processes. Physics Today 1976, 29(4), 62–67. [Google Scholar] [CrossRef]
  34. FRED economic Data, Interest rates, Discount rate for China. Available online: https:// fred. stlouisfed. org/ series/ INTDSRCNM193N (accessed on 21 July 2020).
  35. Trading Economics, China inflation rate, 2020. Available online: https://tradingeconomics. com/ china/ inflation - cpi (accessed on 21 July 2020).
  36. Yu, J.H.; Yang, C.Z.; Tian, L.W.; Liao, D. A study on optimum insulation thicknesses of external walls in hot summer and cold winter zone of China. Appl. Energ. 2009, 86(11), 2520–2529. [Google Scholar] [CrossRef]
  37. Li, D.Z.; Chen, H.X.; Hui, E.C.M.; Zhang, J.B.; Li, Q.M. A methodology for estimating the life-cycle carbon efficiency of a residential building. Build Environ 2013, 59, 448–455. [Google Scholar] [CrossRef]
  38. Zhang, X.C.; Wang, F.L. Life-cycle assessment and control measures for carbon emissions of typical buildings in China. Build Environ 2015, 86, 89–97 https://doi org/101016/jbuildenv201501003. [Google Scholar] [CrossRef]
  39. Ramesh, T.; Prakash, R.; Shukla, K.K. Life cycle energy analysis of buildings: an overview. Energy Build 2010, 42(10), 1592–1600. [Google Scholar] [CrossRef]
  40. Zhao, S.; Zhu, Y.; Lou, P.; Hu, Y.; Xu, C.; Chen, Y. Optimization model of substation building envelope–renewable energy utilization based on life-cycle minimum carbon emissions. Buildings 2023, 13, 1602. [Google Scholar] [CrossRef]
  41. Wang, Q.; Guo, W.; Xu, X.; Deng, R.; Ding, X.; Chen, T. Analysis of carbon emission reduction paths for the production of prefabricated building components based on evolutionary game theory. Buildings 2023, 13, 1557. [Google Scholar] [CrossRef]
  42. Jia, Z.; Lin, B. Rethinking the choice of carbon tax and carbon trading in China. Technolog. Forecast. Soc. 2020, 159, 120187. [Google Scholar] [CrossRef]
Preprints 80856 g001
Figure 1. Flowchart for determining the best roof insulation design scheme.
Figure 1. Flowchart for determining the best roof insulation design scheme.
Preprints 80856 g001
Preprints 80856 g002
Figure 2. Building life cycle.
Figure 2. Building life cycle.
Preprints 80856 g002
Preprints 80856 g003
Figure 3. The basic principle of emissions trading mechanism operation.
Figure 3. The basic principle of emissions trading mechanism operation.
Preprints 80856 g003
Preprints 80856 g004
Figure 4. The basic principle of carbon tax operation.
Figure 4. The basic principle of carbon tax operation.
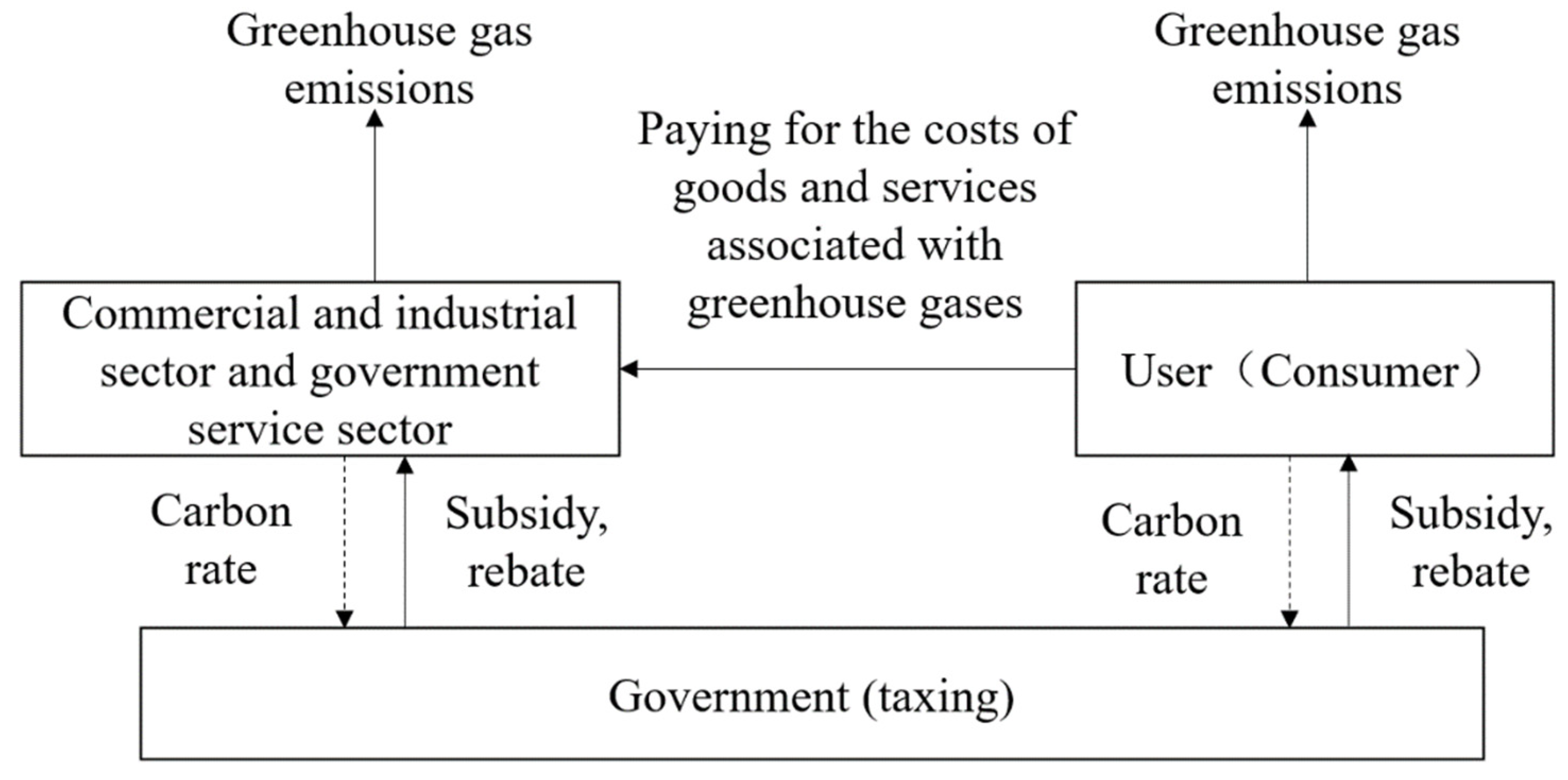
Preprints 80856 g004
Preprints 80856 g005
Figure 5. Feasibility and implementation effectiveness of GHG abatement tools.
Figure 5. Feasibility and implementation effectiveness of GHG abatement tools.
Preprints 80856 g005
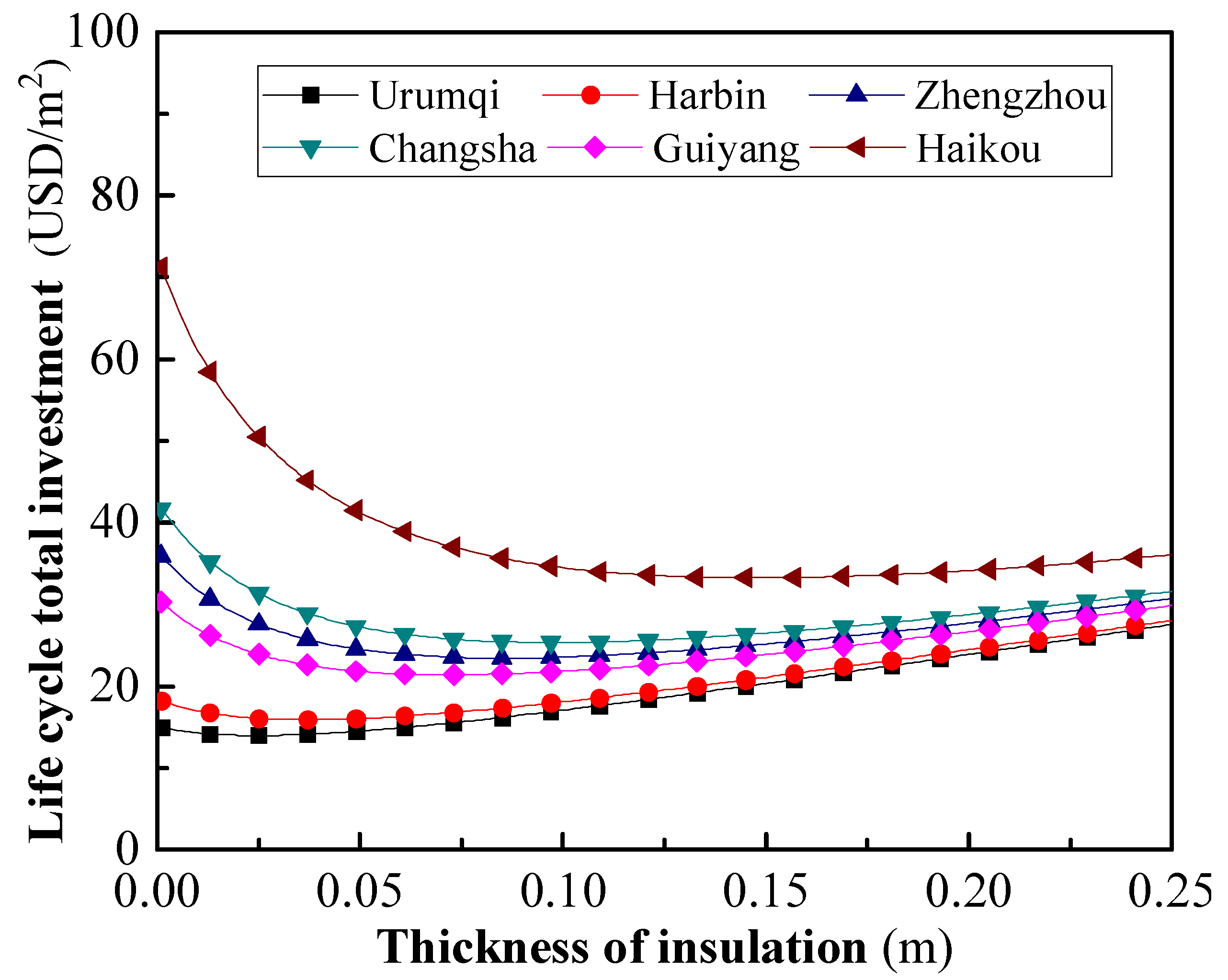
Preprints 80856 g006
Figure 6. Life cycle total investment of granary roof insulation.
Figure 6. Life cycle total investment of granary roof insulation.
Preprints 80856 g006
Preprints 80856 g007
Figure 7. Life cycle net present value of granary roof insulation.
Figure 7. Life cycle net present value of granary roof insulation.
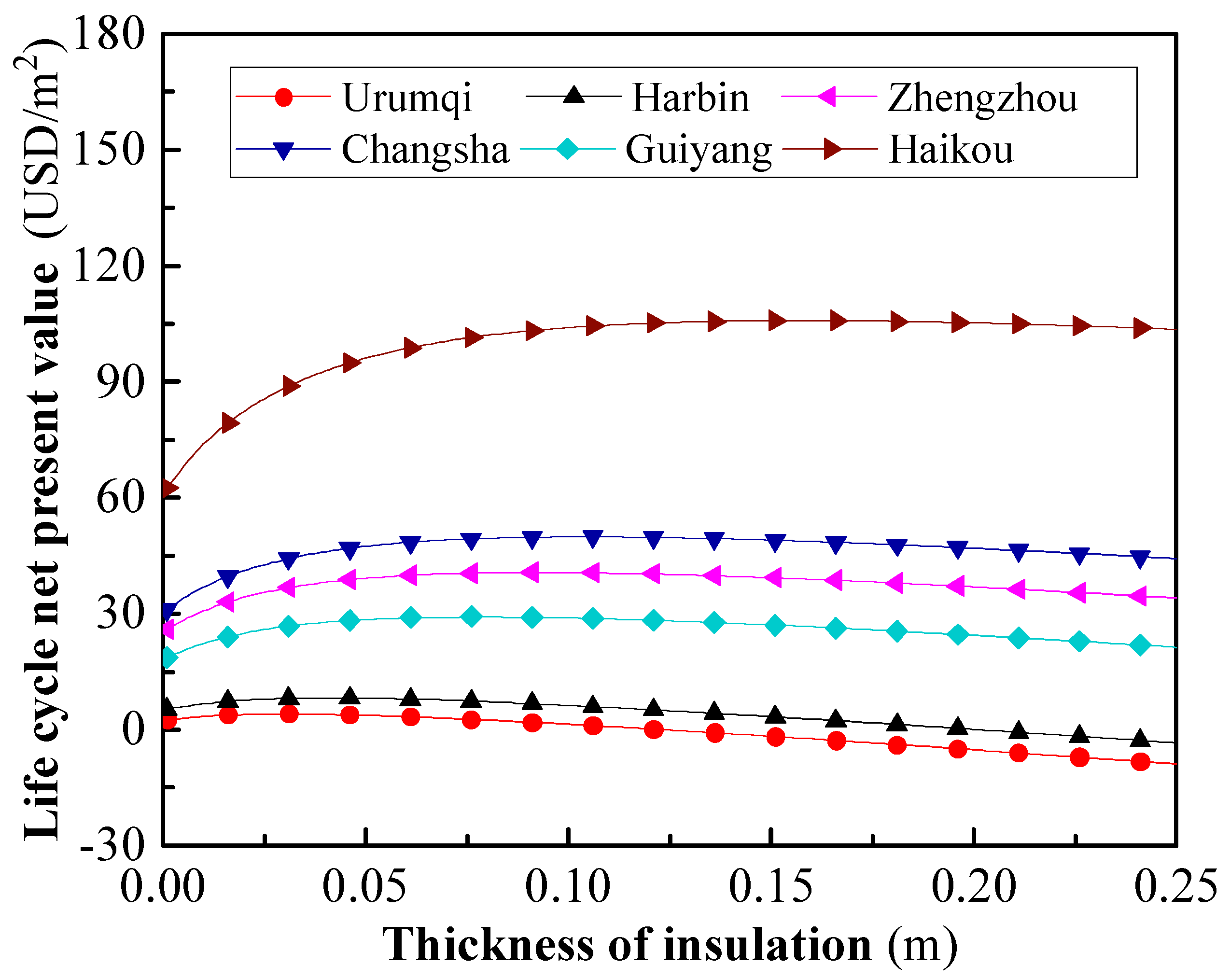
Preprints 80856 g007
Preprints 80856 g008
Figure 8. Payback periods without and with considering carbon emission cost.
Figure 8. Payback periods without and with considering carbon emission cost.
Preprints 80856 g008
Preprints 80856 g009
Figure 9. Increased LCT of roof insulation due to carbon emissions cost.
Figure 9. Increased LCT of roof insulation due to carbon emissions cost.
Preprints 80856 g009
Preprints 80856 g010
Figure 10. Increased LCS of roof insulation due to carbon emission cost.
Figure 10. Increased LCS of roof insulation due to carbon emission cost.
Preprints 80856 g010
Preprints 80856 g011
Figure 11. Balanced indexes without and with considering of carbon emission cost.
Figure 11. Balanced indexes without and with considering of carbon emission cost.
Preprints 80856 g011
Table 1. Performance parameters of roof elements.
Table 1. Performance parameters of roof elements.
Layers Material name Thermal conductivity (W/m·K) Density (kg/m3) Specific heat capacity(J/kg·K) Thickness (mm)
1 Waterproof layer 0.23 900 1620 5
2 Cement mortar 0.93 1800 1050 20
3 EPS 0.039 25 1380 30
4 Cement mortar 0.93 1800 1050 20
5 Cement slag 0.26 900 920 100
6 Reinforced concrete 1.74 2500 920 120
7 Cement mortar 0.93 1800 1050 25
Table 2. Roof cooling load.
Table 2. Roof cooling load.
Typical city Number of days for average temperature > 5℃ Cooling load without insulation (MW/m2） Cooling load With insulation (MW/m2）
Xining 0-30 - -
Urumqi 112-194 211.514328 64.965168
Harbin 55-122 186.863112 58.068504
Zhengzhou 143-192 496.436294 149.47992
Changsha 121-253 518.710788 158.03532
Guiyang 173-224 399.005784 122.57575
Haikou 289-352 1017.59494 312.38114
Table 3. Carbon emission cost of roof insulation.
Table 3. Carbon emission cost of roof insulation.
Insulation type Insulation stage Carbon emissions（tCO2e/m3） Carbon emission cost
（USD/m3）
EPS Production 4.5E-01 3.73E+00
Transportation 2.1E-05 1.73E-04
Construction 5.6E-04 4.60E-03
Demolition 5.0E-04 4.11E-03
Disposal stage 7.0E-05 5.75E-04
Total 4.51E-01 3.74E+00
XPS Production 6.3E-01 5.22E+00
Transportation 2.9E-05 2.39E-04
Construction 5.6E-04 4.6E-03
Demolition 5.0E-04 4.11E-03
Disposal stage 9.7E-05 7.98E-04
Total 6.31E-01 5.23E+00
PU Production 7.5E-01 6.22E+00
Transportation 2.9E-05 2.39E-04
Construction 5.6E-04 4.6E-03
Demolition 5.0E-04 4.11E-03
Disposal stage 8.3E-05 6.83E-04
Total 7.51E-01 6.23E+00
RW Production 5.5E-01 4.56E+00
Transportation 4.8E-05 3.94E-04
Construction 4.6E-04 3.78E-03
Demolition 4.1E-04 3.38E-03
Disposal stage 1.6E-05 1.31E-04
Total 5.51E-01 4.57E+00
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content.
Copyright: This open access article is published under a Creative Commons CC BY 4.0 license, which permit the free download, distribution, and reuse, provided that the author and preprint are cited in any reuse.
Prerpints.org logo

Preprints.org is a free preprint server supported by MDPI in Basel, Switzerland.

facebook logotwitter logolinkedin logo
MDPI Initiatives
Important Links
Subscribe

Disclaimer

Terms of Use

Privacy Policy

© 2024 MDPI (Basel, Switzerland) unless otherwise stated