Preprint
Article
Geometrized Vacuum Physics. Part III. Curved Vacuum Area
This version is not peer-reviewed.
Submitted:
11 August 2023
Posted:
14 August 2023
Read the latest preprint version here
Abstract
This article is the third part of a scientific project under the general title "Geometrized vacuum physics based on the Algebra of Signatures". In the first two papers [1,2], the ideal (i.e., non-curved and immobile) local region of vacuum was studied and the foundations of the Algebra of Signatures were laid. This article considers the possibilities of describing the curved and moving state of the same vacuum region on the basis of the mathematical apparatus of the Algebra of Signatures. The reasons for the multilateral consideration of vacuum and twisting of intra-vacuum processes into spiral bundles are disclosed. The 4-tensor is introduced for two-sided and 16-sided consideration of the curvature of the local vacuum region. On the basis of kinematic models, the following assumptions were made: about the inert properties of vacuum layers; about the possibility of displacement of vacuum layers relative to each other at a speed significantly exceeding the speed of light; about the possibility of "rupture" of the local region of vacuum. The proposed kinematic models of the movement of vacuum layers can be a theoretical basis for the development of "zero" (i.e., vacuum) technologies.
Keywords:
Subject:
Physical Sciences - Theoretical Physics1. Background and Introduction
This work is the third of a series of articles under the title "Geometric vacuum physics". In the previous two articles [1,2], the foundations of the Algebra of Stignatures and the Algebra of Signatures were presented, which were obtained on the basis of the study of an ideal (i.e., non-curved and immobile) region of the vacuum.
This article considers the possibility of expanding the capabilities of the Algebra of Signatures to describe the curvature of the same region of the vacuum.
In §1 of the article [1], it was shown that as a result of probing an ideal region of vacuum with light rays with a wavelength λm,n (from the subrange Δλ = 10m ÷ 10n cm) from three mutually perpendicular directions makes it possible to obtain a light cubic lattice (see Figure 1 of the article [1]). Such a lattice illuminated from the void was called the λm,n-vacuum (or 3Dm,n-landscape).
It was also shown in [1] that if we similarly probe the area of ideal vacuum with light rays of other wavelengths, we will obtain an infinite number of λm,n-vacuums (i.e., 3Dm,n-landscapes with an edge length cubic cell εm,n ~ 100λm,n) which nested into each other like nesting dolls (see Figure 1, or Figure 2 in [1]).
As already noted in §2 of the article [1], if the area of vacuum under study is not curved, then all λm,n-vacuums will be represented as ideal cubic 3Dm,n-lattices, which differ from each other only by the length of the edge of cubic cell εm,n ~ 102λm,n (see Figure 1).
However, if the region of vacuum under study is curved, then all λm,n-vacuums will differ somewhat from each other due to the fact that light rays (i.e., eikonals) with different wavelengths have different thicknesses (i.e., circular cross section of the eikonal, see Figure 2, or Figure 3 in [1]), which leads to averaging of vacuum curvature within the beam thickness.
In this case, each λm,n-vacuum (i.e., the light 3Dm,n-landscape) will be unique. That is, each λm,n-vacuum is only one 3-dimensional "slice" of the curved vacuum region. For a complete description of a curved region of vacuum, it is necessary to have an infinite set of curved λm,n-vacuums nested in each other (see Figure 3 or Figure 4 in [1]).
Thus, the local volume of a curved area of vacuum is an infinitely complex system consisting of an infinite number of λm,n-vacuums nested in each other.
However, the situation is simplified by the fact that in the entire studied range of electromagnetic wave lengths, all λm,n-vacuums obey the same physical and geometric laws. Therefore, the method of describing one curved area of the λk,r-vacuum is automatically extended to all other λm,n-vacuums.
The mathematical apparatus of the Algebra of Stignatures is developed below, designed to study the local volume of only one curved λm,n-vacuum. But this mathematical apparatus is suitable for studying not only all λm,n-vacuums, but also any other deformed continuous media in which wave disturbances propagate at a constant speed.
Below, we develop the mathematical apparatus of the Algebra of Stignatures, designed to study the local volume of only one curved λm,n-vacuum. But this mathematical apparatus is suitable for studying not only all λm,n-vacuums, but also any other deformed continuous media in which wave disturbances propagate at a constant speed.
2. Materials and Method
2.1. Curved area of λm,n-vacuum
Let’s consider a curved area of vacuum. If the wavelength λm,n of test monochromatic light rays is much smaller than the dimensions of the vacuum curvature, then in this area the cubic cell of the λm,n-vacuum (i.e., the cubic cell of the 3Dm,n-landscape, which limited by these rays) will be curved (see Figure 4a).
Figure 4.
a) Curved cubic cell of the λm,n-vacuum; b) One of the corners of a curved λm,n-vacuum cubic cell.
Figure 4.
a) Curved cubic cell of the λm,n-vacuum; b) One of the corners of a curved λm,n-vacuum cubic cell.

We consider one of the eight vertices of the curved cube of the λm,n-vacuum (see Figure 4a). Let’s replace the distorted edges emerging from this vertex with distorted axes of the curvilinear coordinate system x′0(а), x′1(а), x′2(а), x3′(а) (see Figure 4b). We denote the same edges of the original, ideal cube by the pseudo-Cartesian coordinate system x0(а), x1(а), x2(а), x3(а).
In the area of the vertex of the angle under consideration (see Figure 4b), its distortions can be decomposed into two components: 1) changing the lengths (compression or expansion) of the axes x′0(а), x′1(а), x′2(а), x3′(а) while maintaining right angles between these axes; 2) deviations of the angles between the axes x′0(а), x′1(а), x′2(а), x′3(а) from right angles (i.e., 90o) while preserving their lengths.
Let's consider these distortions separately.
1) Let only the lengths of the axes x′0(а), x′1(а), x′2(а), x3′(а) change near the vertex during the curvature, then these axes can be expressed through the axes of the original ideal x0(а), x1(а), x2(а), x3(а) using the corresponding coordinate transformations [3]:
where
is the Jacobian of the transformation, or the components of the elongation tensor.
x′0(а) = α00(а)x0(а) + α01(а)x1(а) + α02(а)x2(а) + α03(а)x3(а);
x′1(а) = α10(а)x0(а) + α11(а)x1(а) + α12(а)x2(а) + α13(а)x3(а);
x′2(а) = α20(а)x0(а) + α21(а)x1(а) + α22(а)x2(а) + α23(а)x3(а);
x′3 (а) = α30(а)x0(а) + α31(а)x1(а) + α32(а)x2(а) + α33(а)x3(а),
x′1(а) = α10(а)x0(а) + α11(а)x1(а) + α12(а)x2(а) + α13(а)x3(а);
x′2(а) = α20(а)x0(а) + α21(а)x1(а) + α22(а)x2(а) + α23(а)x3(а);
x′3 (а) = α30(а)x0(а) + α31(а)x1(а) + α32(а)x2(а) + α33(а)x3(а),
αij(a) =dx′i(a)/dxj(a)
2) Now let the distortion near the vertex be associated only with a change in the angles between the axes of the coordinate system x′0(а), x′1(а), x′2(а), x3′(а), while the lengths of these axes remain unchanged. In this case, it is sufficient to consider only the change in the angles between the basis vectors e′0(a), e′1(a), e′2(a), e′3(a) of the distorted reference system.
It is known from vector analysis that the basis vectors of the distorted 4-basis e′0(a), e′1(a), e′2(a), e′3(a) can be expressed in terms of the original basis vectors e0(a), e1(a), e2(a), e3(a) of an orthogonal 4-basis by means of the following system of the linear equations [3]:
where
are the guiding cosines.
e′0(a) = β00(a) e0(a) + β01(a) e1(a) + β02(a) e2(a) + β 03(a) e3(a);
e′1(a) = β10(a) e0(a) + β11(a) e1(a) + β12(a) e2(a) + β13(a) e3(a);
e′2(a) = β20(a) e0(a) + β21(a) e1(a) + β22(a) e2(a) + β23(a) e3(a);
e′3(a) = β30(a) e0(a) + β31(a) e1(a) + β32(a) e2(a) + β03(a) e3(a),
e′1(a) = β10(a) e0(a) + β11(a) e1(a) + β12(a) e2(a) + β13(a) e3(a);
e′2(a) = β20(a) e0(a) + β21(a) e1(a) + β22(a) e2(a) + β23(a) e3(a);
e′3(a) = β30(a) e0(a) + β31(a) e1(a) + β32(a) e2(a) + β03(a) e3(a),
β pm(a) = (e′p(a) ⋅em(a)) = cos (e′p(a) ^em(a))
The systems of Eqs. (1) and (3) can be represented in a compact form:
here and below, the "Einstein summation rule" is used.
x′i(a) = αij(a) x j(a),
e′p(a) = β pm(a) em(a).
For example, we write the vector (48) in [1]
in the distorted 4-basis, taking into account Exs. (5) and (6), vector (7) can be represented as
ds′(7)=e′i(7)dx′ i(7).
ds′(7)= β pm(7)em(7)αpj(7)dxj(7).
2.2. Curved metric 4-spaces
Consider two vectors (48) and (49) in [1], given in the 5th and 7th curved affine spaces
ds′ (5)= βln(5)en(5)αlj(5)dxj,
ds′ (7)= βpm(7)em(7)αpi(7)dxi.
Let's find the scalar product of these vectors
where
are the components of the metric tensor of the (7,5)-th metric 4-space.
ds′ (7,5)2 = ds′ (7)ds′ (5) = βpm(7)em(7)αpi(7)βln(5)en(5)αlj(5)dxidxj = сij(7,5)dxidx j,
сij(7,5)= βpm(7)em(7)αpi(7)βln(5)en(5)αlj(5)
Thus, we have obtained the metric of the (7,5)-th metric 4-space
with signature (53) in [1] (+ + + –) and metric tensor
ds′(7,5)2 = сij(7,5)dxidxj
Similarly, the scalar pairwise product of any two of the 16 vectors (9)
leads to the formation of an atlas consisting of 16 × 16 = 256 possible curved 4-sheets (that is, metric 4-spaces) with metrics
where a = 1,2,3,…,16; b = 1,2,3,…,16, with corresponding signatures (11) in [2] and metric tensors
where
are the components of the metric tensor of the (a,b)-th curved metric 4-space.
ds′ (a)= βpm(a)em(а)αpi(a)dxi,
ds′ (b) = βln(b)en(b)αlj(b)dxj
ds′ (a, b)2 = сij(a, b)dxidxj,
сij(a, b)= βpm(a)em(a)αpi(a)βln(b)en(b)αlj(b)
2.3. The first stage of compactification of curved measurements
Just as it was done in §2.3 in [2], at the first stage of the compactification of additional curved mathematical dimensions in the Algebra of Signatures, metric 4-spaces with the same signature are averaged.
For example, for metrics with signature (– + – +), we have the following averaged metric tensor
where p corresponds to the 14-th signature (– + – +), according to the following conditional numbering of signatures:
This matrix with numbered signatures corresponds to the matrix of signatures (32) in [2].
As a result of operation (21), the averaged metric is obtained
<ds(– + – +) 2> = сij(14)dxi dxj , with signature (– + – +).
Similarly, as a result of averaging type (21) – (23) out of 256 metrics (18) of curved metric 4-spaces, we can obtain 256 : 16 = 16 averaged metrics with 16 possible signatures
where ‹ › means averaging.
<ds(+– – –)2> <ds(+ + + +)2> <ds(– – – +)2> <ds(+ – – +)2 >
<ds(– – + –)2> <ds(+ + – –)2> <ds(– + – –)2> <ds(+ – + –)2>
<ds(– + + +)2> <ds(– – – – )2> <ds(+ + + –)2> <ds (– + + –)2>
<ds(+ + – +)2> <ds(– – + +)2> <ds(+ – + +)2> <ds(– + – +)2>,
<ds(– – + –)2> <ds(+ + – –)2> <ds(– + – –)2> <ds(+ – + –)2>
<ds(– + + +)2> <ds(– – – – )2> <ds(+ + + –)2> <ds (– + + –)2>
<ds(+ + – +)2> <ds(– – + +)2> <ds(+ – + +)2> <ds(– + – +)2>,
If the additive superposition (i.e., summation) of all these 16 averaged metrics (24) is equal to zero
then this expression can be used for an average flat λm,n-vacuum. At the same time, it is a condition for maintaining the λm,n-vacuum balance.
Recall that the “vacuum balance condition” was formulated in the introduction of the article [1], and this is the basic statement that, in particular, no matter what convex-concave curvatures (fluctuations) occur with the local area of the λm,n-vacuum, on average over the entire area they are equal to zero.
In this case, all 16×16=256 components of 16 averaged metric tensors сij(p) can be random functions of time. But, according to the condition of λm,n-vacuum balance, these metric-dynamic fluctuations should overflow into each other so that the total metric (25) on average remains equal to zero.
Based on the metric (25), λm,n-vacuum thermodynamics can be developed, which considers the most complex, near-zero “transfusions” of the local λm,n-vacuum curvatures. Concepts about λm,n-vacuum entropy and temperature (i.e., the essence of chaoticity and intensity of local λm,n-vacuum fluctuations) can be introduced. We can talk about the “cooling” of the λm,n-vacuum to “freezing”, its “heating“ to “evaporation” and many other effects similar to the processes occurring in atomistic continuous media.
Features of λm,n-vacuum thermodynamics are associated with processes when the gradients of λm,n-vacuum fluctuations approach the speed of light (dсij(p)/dxa ~ c) or zero (dсij(p)/dxa ~ 0). A detailed consideration of λm,n-vacuum thermodynamics and torsion fields is beyond the scope of this article. However, some aspects of this area of research are considered in [3,4,5].
2.4. The second stage of compactification of curved mathematical measurements
Just as it was done in §7.2 in [2], Ex. (25) can be reduced to two terms
where
is the quadratic form, which is the result of averaging seven metrics from the list (24) with signatures included in the numerator of the left rank (43) in [2] or (29);
is the quadratic form, which is the result of averaging seven averaged metrics from the list (24) with signatures included in the numerator of the right rank (43) in [2] or (29).
(+ + + +) + (– – – –) =0
(– – – +) + (+ + + –) =0
(+ – – +) + (– + + –) =0
(– – + –) + (+ + – +) =0
(+ + – –) + (– – + +) =0
(– + – –) + (+ – + +) =0
(+ – + –) + (– + – +) =0
(+ – – –)+ + (– + + +)+ =0
(– – – +) + (+ + + –) =0
(+ – – +) + (– + + –) =0
(– – + –) + (+ + – +) =0
(+ + – –) + (– – + +) =0
(– + – –) + (+ – + +) =0
(+ – + –) + (– + – +) =0
(+ – – –)+ + (– + + +)+ =0
Thus, from the complex λm,n-vacuum fluctuations, two averaged sides can be distinguished:
1) the averaged “outer” side of the 23-λm,n-vacuum (or subcont, see §2.7 in [2]) with the averaged metric
where
here
are components of the metric tensor of the subcont (i.e., the "outer" side of the 23-λm,n-vacuum).
2) the averaged “inner” side of the 23-λm,n-vacuum (or antisubcont, see §2.7 in [2]) with the averaged metric
where
here
are the components of the metric tensor of the antisubcont (i.e., the “inner” side of the 23-λm,n-vacuum).
Recall that the two-sided model of λm,n-vacuum, that is, the result of averaging complex metric-dynamic fluctuations of λm,n-vacuum to a two-sided level of consideration, is called in §2.7 in [2] “23-λm,n-vacuum”, because in this case, only 4 + 4 = 8 = 23 mathematical measurements remain within the framework of consideration.
To shorten the notation, the averaging signs < > of the components of the metric tensors (32) and (35) are omitted.
Once again, we note that two concepts were formally introduced in [1]:
- -
- subcont (i.e., the substantial continuum or the outer side of the 23-λm,n-vacuum with the averaged metric (30) and with the signature (+ – – –) of the Minkowski space);
- -
- antisubcont (i.e., antisubstantial continuum or inner side of 23-λm,n-vacuum with averaged metric (33) and signature (– + + +) of Minkowski antispace).
The fictitious concepts of subcont and antisubcont are introduced to simplify and facilitate our perception of the complex intra-vacuum processes.
Thus, from the complexly fluctuating λm,n-vacuum (see Figure 6), due to simplification and averaging, we singled out only one averaged 23-λm,n-vacuum with two mutually opposite 4-dimensional sides: subcont and antisubcont (see Figure 7).
Figure 6.
Fractal illustration of complex intra-vacuum processes.

On Figure 7 conditionally shows the average section of the two-sided 23-λm,n-vacuum, the outer side of which (subcont) is described by the averaged metric (30), and the inner side (antisubcont) is described by the averaged metric (33).
Figure 7.
The simplified illustration of a two-sided section of the 23-λm÷n-vacuum, the outer side of which (subcont) is described by the averaged metric (30) with the signature (+ – – –), and its inner side (antisubcont) is described by the metric (33) with the opposite signature (– + + +), as ε → 0.
Figure 7.
The simplified illustration of a two-sided section of the 23-λm÷n-vacuum, the outer side of which (subcont) is described by the averaged metric (30) with the signature (+ – – –), and its inner side (antisubcont) is described by the metric (33) with the opposite signature (– + + +), as ε → 0.

Let’s explain the importance of at least two-sided consideration with a simple example. Let's take a sheet of paper and draw lines (segments) of the same length on its two sides in the same place (see Figure 8 a,b)
Reference systemsХУ orХ′У′ on two opposite sides of a sheet of paper have different stignatures, respectively {+ +} and {+ –}. To understand this, take a sheet of paper and draw on it the XY reference system (as inFigure 8a). Then turn the sheet over, and on its reverse side depict theХ′У′ reference system in the same way in the same place. If you compare these reference systems, you will find that the X and X′ axes are directed in different directions, so their stignatures are different.
If the given sheet of paper is not curved, then the reference system with stignatures {+ +} and {+ –} are alike, i.e., any of them can set the coordinates of the drawn lines.
However, if this sheet is bent (see Figure 8c), then the line on the outer side of the sheet (see Figure 8a) will slightly expand, and the line on the inner side of the sheet (Figure 8b) will shrink by almost the same amount. In other words, the expansion of one line inevitably leads to the compression of another line drawn on the opposite side of the sheet.
Therefore, at least a two-sided consideration of the process of curvature of a sheet of paper is inevitable. Otherwise, one-sided consideration will lead not only to the loss of information about the process under study, but also in some cases to paradoxical and erroneous conclusions. In addition, the two-pronged approach immediately takes into account the vacuum balance condition, which states that any action is accompanied by a reaction. In the considered case, the expansion of one line is inevitably accompanied by compression of the other line, therefore, if we neglect the thickness of the sheet, then its average deformation of these lines tends to zero.
In fact, a sheet of paper has a thickness ε (see Figure 7). Therefore, in its thickness, one can always distinguish a cube with an edge length ε (see Figure 7 and Figure 9).
If the sheet is bent, all sixteen 3-bases associated with the trihedral angles of such a deformed cube will be distorted in different ways (see Figure 4). Therefore, the Algebra of Signatures develops a mathematical apparatus that initially takes into account the distortions of all sixteen 3-bases at once, shown in Figure 9.
Only in the case when the thickness of a sheet of paper is so small that it can be neglected, it is permissible to simplify the problem to a two-sided consideration.
Unilateral consideration, i.e. the study of the curvature of only one side of a sheet of paper is possible, but will inevitably lead to a fundamental incompleteness of the mathematical model of the process under study.
In relation to vacuum physics, we are forced to state that the void (i.e., vacuum), which plays the role of the space surrounding us, has at least two sides: 1) external, i.e. Minkowski space with signature (+ – – –) and 2) internal, i.e. Minkowski antispace with signature (– + + +) (see Figure 7). One-sided consideration will inevitably lead to dead-ends and unfinished areas of scientific research.
For example, a metric that is a solution to the equations of the general theory of relativity (GR) of A. Einstein with the signature (+ – – –) can only describe a one-sided cosmological model of the Universe, which, in principle, cannot be completed. We also need, at a minimum, a metric-solution of the same equations with the opposite signature (– + + +). A more complete cosmological model should take into account all 16 metric-solutions of GR equations with signatures (29). Only such a cosmological model can claim logical completeness. The project of the cosmological model taking into account metric-solutions with all 16 possible signatures will be presented in subsequent articles of this project.
2.5. Four-strain tensor of 23-λm,n-vacuum in the case of a simplified two-sided consideration
2.5.1. Four-strain tensors of two sides of 23-λm,n-vacuum
Let‘s assume that the simplified two-sided model of the 23-λm,n-vacuum presented in the previous paragraph (see Figure 7) satisfies the given consideration accuracy.
Let the initial uncurved state of the studied area of the outer side of the 23-λm,n-vacuum (i.e., subcont) be characterized by a zero averaged metric (30)
Here and below, to shorten the entries, the averaging sign < > is removed, while it is conditionally assumed that
In the Cartesian coordinate system, metric (36) takes the form
wherein
In the case of curvature of the same section of the subcont, its metric-dynamic state is determined by the averaged metric
The difference between the curved state of the studied section of the subcont and its non-curved state is determined by the Expression [3]
here
is the 4-deformation tensor of the local section of the subcont.
Whence it follows
Substituting (42) into (39), taking into account (40), we obtain [3]
or expanded
εij(+) = ½ [(1 + li(+))(1 + lj(+)) cosβij(+) – cosβij0(+)]gij0(+),
βij0(+) is the angle between the axes xi and xj of the reference system, "frozen" into the initial uncurved state of the subcont section under study;
βij(+) is the angle between the axes xi′and xj′ of the distorted reference system “frozen” into the distorted state of the same section of the subcont.
When βij0(+) = π/2, Ex. (44) takes the form
For the diagonal components of the 4-strain tensor εii(+), Ex. (45) is simplified
whence follows [3]
If deformations of the subcont section εij(+) are small, then, expanding Ex. (47) into a series, and, confining ourselves to the first member of this series, we obtain the relative elongation of the local subcont section [3]
Similarly, the deformation of the local section of the inner side of the 23-λm,n-vacuum (antisubcont) is determined by the Expression
where
is the 4-deformation tensor of the local section of the antisubcont;
is the metric of the uncurved state of the local section of the antisubcont. In the Cartesian coordinate system, metric (51) takes the form
where
in its turn
is the metric of the curved local section of the antisubcont.
The relative elongation (or contraction) of the local section of the antisubcont is determined by the Expression
2.5.2. The 4-strain tensor of 23-λm,n-vacuum in the case of two-sided consideration
We define the 4-strain tensor of a local section of a two-sided 23-λm,n-vacuum as the average value of the deformations of its two sides
or, taking into account expressions (40) and (50), we obtain
εij(±) = ½ (εij(+) + εij(–)) = ½ (εij(– + + +) + εij(+ – – –)),
because from the condition of 23-λm,n-vacuum balance (48) in [2] ds(+ – – –)2 + ds(– + + +)2 = 0 follows:
The relative elongation (or contraction) li(±) of the local section of the two-sided 23-λm,n-vacuum is determined by the Expression
where
because according to the condition of the λm,n-vacuum balance (56) ij0(–) = –ij0(+).
Substituting Exs. (58) and (59) into Ex. (57), we obtain
It can be seen from this Expression that the relative elongation (or contraction) of the local section of the two-sided 23-λm,n-vacuum, li(±) can be a complex number.
In this regard, we note the following important circumstance. If both sides of Ex. (55) are multiplied by dxidxj, then we obtain the averaged quadratic form
which resembles the Pythagorean theorem c2 = a2 + b2.
This means that the line segments ()1/2ds(–) and ()1/2ds(+), which lie on two mutually opposite sides of the two-sided 23-λm,n-vacuum, are always mutually perpendicular to each other, i.e. ds (–)⊥ ds (+) (Figure 10a).
In this case, two lines directed in the same direction can always be mutually perpendicular only if they form a double helix (see Figures 10b and 12).
Thus, the averaged metric (61) corresponds to a “braid” segment consisting of two mutually perpendicular spirals s(+) and s(–). In this case, just like the relative elongation of the local section of the two-sided 23-λm,n-vacuum li(±) (60), such a section of the “double helix” can be described by a complex number
the square of whose modulus is equal to Ex. (61).
Below, a k-braid is the result of averaging metrics with different signatures (where k is the number of averaged metrics, i.e., the number of “threads” in the “braid”). For example, the averaged metric (61) is called a 2-braid, since it is “twisted” from 2 lines (“threads”): ds(+) = ds (+ – – –) and ds(–) = ds(– + + +).
Here is another augment in favor of the mutual perpendicularity of the segments ds(+) and ds(–);
Figure 8 showed that the reference systems XY or X'Y' on two opposite sides of a sheet of paper have different stignatures {+ +} and {+ –}. In order to get a completely opposite stignature {– –}, it is necessary first to depict the reference system XY on the sheet, then turn this sheet 90 degrees clockwise, then turn it to the other side and similarly draw the reference system X'Y' on the same place where the reference system XY (see Figure 11). The result will be a reference system Y'X' with a completely opposite stignature {– –} in relation to the XY system.
If, on one side of the sheet, draw a line ds(+) along the X axis (see Figure 11), and on the other completely opposite side, draw the same line ds(–) along the X ′ axis, then it turns out that the lines ds(+) and ds(–) are mutually perpendicular. Such a crossing of the ds(+) and ds(–) lines takes place in each local area of the sheet, which resembles the crossing of fabric threads (see Figure 12a).
If a hypothetical sheet with two completely opposite sides (i.e., with opposite stignatures {+ +} and {– –}) is bent as shown in Figure 8c, then the line ds(+) will stretch, and the corresponding line ds(–) on the other side of such a sheet will remain the same (i.e., not deformed). In this case, the line will shrink on the perpendicular axis Y′. This ultimately leads to the twisting of the ds(+) and ds(–) lines into spiral bundles (see Figure 10 and Figure 12a).
As applied to the model of the two-sided section of the 23-λm,n-vacuum with completely opposite sides, i.e. with signatures (+ – – –) and (– + + +) (Figure 7), we conclude that the intertwined lines ds (+ – – –) and ds(– + + +) form a fabric of 3-dimensional extent. In other words, the 23-λm,n-vacuum is not just a two-sided 8-dimensional space, but is the result of the interlacing of its two sides, like the threads of a fabric (see Figure 12b).
Deformation of one side of such length inevitably leads to a perpendicular counter - response on its other side. This is the reason for the folding of local sections of such a two-sided space into double helixes (see Figure 10 a,b and Figure 12b).
It is difficult to imagine a 3-dimensional tissue structure of a two-sided 8-dimensional 23-λm÷n-vacuum, but the mathematics of the Algebra of Signatures takes into account the ubiquitous interweaving of the lines ds (+ – – –) and ds(– + + +) automatically, since not summed these lines themselves, but their quadratic forms ds (+ – – –)2 and ds(– + + +)2 (61), which define the metrics of these extensions.
2.5.3. The 4-strain tensor of a curved 26-λm,n-vacuum in the case of sixteen-sided consideration
At the next deeper 16-sided level of consideration, the number of mathematical dimensions is 16 × 4 = 64 = 26, so at this level the subject of consideration is the 26-λm,n-vacuum.
The metric-dynamic properties of the local region of the 26-λm,n-vacuum are characterized by a superposition (i.e., additive superposition or averaging) of sixteen metrics with all 16 possible signatures (29), i.e. 16-braid (according to the definition of k-braid after Ex. (61)):
dsΣ2 = 1/16 (ds(+ – – –)2 + ds(+ + + +)2 + ds(– – – +)2 + ds(+ – – +)2 +
+ ds(– – + –)2 + ds(+ + – –)2 + ds(– + – –)2 + ds(+ – + –)2 +
+ ds(– + + +)2 + ds(– – – – )2 + ds(+ + + –)2 + ds(– + + –)2 +
+ ds(+ + – +)2 + ds(– – + +)2 + ds(+ – + +)2 + ds(– + – +)2).
+ ds(– – + –)2 + ds(+ + – –)2 + ds(– + – –)2 + ds(+ – + –)2 +
+ ds(– + + +)2 + ds(– – – – )2 + ds(+ + + –)2 + ds(– + + –)2 +
+ ds(+ + – +)2 + ds(– – + +)2 + ds(+ – + +)2 + ds(– + – +)2).
In this case, we have 16 4-deformation tensors of all 16 types of curved 4-spaces with different signatures (or topologies, see §2.4 in [2])
where according to expression (25)
is the 4-deformation tensor of the p-th 4-space, p = 1,2,…,16, where:
εij(p) = ½ (сij(p) – сij0(p))
сij0(p) is the metric tensor of the non-curved region of the p-th 4-space with the corresponding signature;
сij(p) is the metric tensor of the same but curved region of the p-th 4-space with the same signature.
By analogy with Ex. (54), at the 16-sided level of consideration, the general tensor of 4-deformations of the local curved region of the 26-λm,n-vacuum is defined as the average value
In this case, the relative elongation of the local region of the 26-λm,n-vacuum in this case is given by the average hypercomplex number of the 16-th rank
where
liΣ(16) = 1/16 (η1 li (1) + η2 li (2) + η3 li (3) +…+ η16 li (16)),
ηm is an orthonormal basis of 16 unit objects (m = 1,2,3,…,16) satisfying the anticommutation relation of the Clifford algebra
where δnm is the identity 16×16-matrix.
ηmηn + ηnηm = 2δmn ,
In this case, the curved section of the 16-braid consists of sixteen intertwined "colored" lines (threads):
ds (16) = η1 ds(+– – –) + η2 ds(+ + + +) + η3 ds(– – – +) + η4 ds(+ – – +) +
+ η5 ds(– – + –) + η6 ds(+ + – –) + η7 ds(– + – –) + η8 ds(+ – + –) +
+ η9 ds(– + + +) + η10 ds(– – – –) + η11 ds(+ + + –) + η12 ds (– + + –) +
+ η13 ds(+ + – +) + η14 ds(– – + +) + η15 ds(+ – + +) + η16 ds(– + – +).
+ η5 ds(– – + –) + η6 ds(+ + – –) + η7 ds(– + – –) + η8 ds(+ – + –) +
+ η9 ds(– + + +) + η10 ds(– – – –) + η11 ds(+ + + –) + η12 ds (– + + –) +
+ η13 ds(+ + – +) + η14 ds(– – + +) + η15 ds(+ – + +) + η16 ds(– + – +).
The colors of these lines conditionally correspond to the colors of the signatures (i.e., the types of topologies of these 4-dimensional spaces), which are formally assigned to these spaces in the framework of vacuum chromodynamics:
Red (+ + + +) + (– – – –) Аnti-red
Yellow (– – – +) + (+ + + –) Аnti-yellow
Orange (+ – – +) + (– + + –) Аnti-orange
Green (– – + –) + (+ + – +) Аnti-green
Blue (+ + – –) + (– – + +) Аnti-blue
Dark blue (– + – –) + (+ – + +) Аnti-dark blue
Violet (+ – + –) + (– + – +) Аnti-violet
White (+ – – –)+ + (– + + +)+ BlackBlack
Yellow (– – – +) + (+ + + –) Аnti-yellow
Orange (+ – – +) + (– + + –) Аnti-orange
Green (– – + –) + (+ + – +) Аnti-green
Blue (+ + – –) + (– – + +) Аnti-blue
Dark blue (– + – –) + (+ – + +) Аnti-dark blue
Violet (+ – + –) + (– + – +) Аnti-violet
White (+ – – –)+ + (– + + +)+ BlackBlack
Formal coloring of 16 lines (or threads) ds(+– – –), ds(– + + +), ds(+ + + +), ds(+ + – +), … , ds(– + – +) makes it possible to represent the “fabric” of 26-λm,n-vacuum woven from them in color form (Figure 6 and Figure 13).
Each “colored” 4-space with the corresponding signature (i.e., topology) (70) can be formally represented as a continuous plastic-elastic medium of the corresponding color. Such 4-spaces, which have elastoplastic properties, can be interpreted as "colored" ethers. But unlike the ether theory, in the geometrized vacuum physics based on the Algebra of Signatures (Alsigna), there is not one ether, but depending on the level of consideration, there can be 2 such ethers (“white” and “black”) or 24 = 16 (with “colors” (70)) or 28 = 256 (with different shades of colors (70)) etc. to infinity. At the same time, all these formal “colored” ethers of Alsigna are intertwined into a single 3-dimensional “carpet” (see Figure 6 and Figure 14), i.e. form a single intertwined and seething 3-dimensional space. Such a 3-dimensional, mean-flat (i.e., "zero") space in each local area resembles a multi-dimensional Calabi-Yau manifold. In addition, the “colored” ethers of Alsigna are illusory in nature and are intended only to facilitate the perception and awareness of the most complex intra-vacuum processes.
If all curved linear forms ds(+ – – –), ds(– + + +), ds(+ + – +), … , ds(– + – +) can be represented in a diagonal form, then in accordance with (65) and (66) in [2], Ex. (69) can be represented in the spintensor form
Within the framework of the Algebra of Signatures, much deeper 2n-sided levels of consideration of the metric-dynamic properties of the curved region of the λm,n-vacuum are possible, while the number of components of the metric tensor characterizing its metric-dynamic properties can increase to infinity (see §2.9 in [2]).
6. Physical meaning of the components of the metric tensor
6.1. Nonzero components of the metric tensor
Let the averaged metric-dynamic states of two 4-dimensional sides of the local region of the 23-λm,n-vacuum be given by metrics (30) and (33) (see Figure 7). Consider the nonzero components of the metric tensors (32) and (35) of these metrics
here the Greek alphabet indices α, β correspond to the 3-dimensional consideration (i.e., α, β = 1,2,3).
The scalar curvature of the local two-sided region of the 23-λm,n-vacuum in the framework of the Algebra of Signatures is determined by the complex number
where the scalar curvature of each of the two sides of the 23-λm,n-vacuum is defined in the same way as in general relativity (GR)
where
is the Ricci tensor of the outer side of the local section of the 23-λm,n-vacuum (i.e., the subcont);
is the Ricci tensor of the inner side of the local section of the 23-λm,n-vacuum (i.e., the antisubcont);
are the Christoffel symbols of the local section of the subcont;
are the Christoffel symbols of the antisubcont.
A feature of the geometrized vacuum physics developed here is to ensure the vacuum balance condition
it means that “convexity” and “concavity” (or compression and tension) over the entire 3-dimensional deformed region of the 23-λm,n-vacuum, on average, completely compensate each other (see Figure 14).
Figure 14.
2D-illustration of a convex-concave two-sided surface with stignatures {+ +} and {– +}. This curved surface is such that it is, on average, flat. At the same time, the deformation that looks like a convexity from the outside, from the inside it looks like a concavity. At the same time, it should be taken into account that if the stignatures of the sides of such a two-sided surface are completely opposite {+ +} and {– –}, then the deformations on its two sides are not only mutually opposite, but also mutually perpendicular (see §5.2).
Figure 14.
2D-illustration of a convex-concave two-sided surface with stignatures {+ +} and {– +}. This curved surface is such that it is, on average, flat. At the same time, the deformation that looks like a convexity from the outside, from the inside it looks like a concavity. At the same time, it should be taken into account that if the stignatures of the sides of such a two-sided surface are completely opposite {+ +} and {– –}, then the deformations on its two sides are not only mutually opposite, but also mutually perpendicular (see §5.2).

So, in two-sided consideration, the non-zero components of the metric tensors (72) and ) are interconnected and describe the curvature of the 3-dimensional extension of the two-sided 23-λm,n-vacuum.
2.6.2. Zero components of the metric tensor
To clarify the physical meaning of the zero components of the metric tensors (32) and (35)
let’s use the kinematics of the layers of the two-sided 23-λm,n-vacuum.
Under the kinematics of vacuum layers is meant such a section of geometrized vacuum physics based on the Algebra of Signatures (Alsigna), in which the displacements (movements) of different sides of theλm,n-vacuum are considered independently of their deformations. With a more consistent approach, i.e. during the development of the dynamics of vacuum layers in subsequent articles of this cycle, it turns out that any displacement of the local region of one layer ofλm,n-vacuum is inevitably accompanied by its curvature. This, in turn, sets in motion the curvature of other adjacent layers of theλm,n-vacuum. At the same time, and vice versa, the curvature of the local region of one layer of theλm,n-vacuum is necessarily accompanied by its displacement (i.e., flow), which leads to a disturbance of all adjacent layers. In what follows, interconnected flows and curvatures of local sections of different layers of theλm,n-vacuum are considered as multidimensional 4-deformations using the mathematical apparatus of the general theory of relativity.
Despite the above shortcomings, kinematic models of the motion of various layers of the 23-λm,n-vacuum make it possible to elucidate the physical meaning of the zero components of metric tensors and theoretically predict a number of previously unknown vacuum effects that can be tested in practice.
Let the initial (stationary and non-curved) state of the two-sided 23-λm,n-vacuum be given by a set of pseudo-Euclidean metrics (36a) and (51a)
where the symbols are introduced
is affine aggregates, in particular, quaternions with a multiplication table of imaginary units, for example,
ds(+)′ = с dt′ + idx′+ jdy′+ kdz ′ is mask of the subcont;
ds(+)′′ = с dt′′+ idx′′+ jdy′′+ kdz ′′ is interior of the subcont;
ds(–)′ = – с dt′+ idx′+ jdy′+ kdz ′ is mask of the antisubcont;
ds(–)′′ = с dt′′– idx′′– jdy′′– kdz ′′ is interior of the antisubcont,
| i | j | k | (88) | |
| i | –1 | k | –j | |
| j | –k | 1 | i | |
| k | j | –i | –1 |
We consider three kinematic cases:
1). In the first case, let the mask and the interior of the outer and inner sides of the 23-λm,n-vacuum move relative to the initial stationary state along the x axis with the same velocity vx,, but in different directions. This is formally described by the transformation of coordinates [7]:
t′ = t, x′ = x + vx t, y′= y, z′= z – for the mask;
t′′= t, x′′ = x – vxt, y′′= y, z′′= z – for the interior.
The equality of the modules of the speeds of movement vx of the mask and the interior is due to the condition of 23-λm,n-vacuum balance, which requires that each movement in the 23-λm,n-vacuum corresponds to a similar anti-motion.
We differentiate expressions (89) and (90) taking into account that x′ is a function of two variables x′(x,t) and vx = const, and substitute the results of differentiation into metrics (83), as a result we obtain a set of metrics
which describe the kinematics of the joint motion of the outer side of the 23-λm,n-vacuum (i.e. subcont) and its inner side (antisubcont), subject to the vacuum balance condition
ds(–)2 + ds(+)2 = 0.
The zero components of the metric tensors (82) in this case are equal to
the mask and the interior of the outer and inner sides of the 23-λm,n-vacuum.
2). In the second case, let the mask and the interior of the outer and inner sides of the 23-λm,n-vacuum (i.e., of the subcont and antisubcont) move relative to their initial stationary state in the same direction - along the x axis with the same speed vx. This is formally described by the coordinate transformations:
t′= t, x′ = x – vx t, y′= y, z′= z – for the mask;
t′′= t, x′′ = x – vxt, y′′= y, z′′= z – for the interior.
We differentiate Exs. (94) and (95) taking into account that x′ is a function of two variables x′(x,t) and vx = const, and substitute the results of differentiation into metrics (83), as a result we obtain a set of metrics
In this case, 23-λm,n-vacuum balance is also observed, because ds(–)2 + ds(+)2 = 0, but additional cross terms vxdxdt are appear. In this case, the zero components of the metric tensors (82) are equal to
3) In the third case, let the mask and the interior of the outer and inner sides of the 23-λm,n-vacuum (i.e., of the subcont and antisubcont) rotate around the z-axis in the same direction with the angular velocity Ω. These processes are described by the coordinate transformations [7]:
t′ = t, x′ = x cosΩ t – y sinΩ t, z′ = z, y′= x sinΩ t + y cosΩ t,
t′′ = t, x′′ = x cosΩ t – y sinΩ t,
z′′ = z, y′′ = x sinΩ t + y cosΩ t.
t′′ = t, x′′ = x cosΩ t – y sinΩ t,
z′′ = z, y′′ = x sinΩ t + y cosΩ t.
We differentiate Exs. (89) and substitute the results of differentiation into metrics (83), as a result we obtain the metrics [7]
In this case, the 23-λm,n-vacuum balance ds(+)2 + ds(–)2 = 0 is observed, and the zero components of the metric tensors (82) are equal to
It can be seen from the considered kinematic examples that the zero components of the metric tensors (82) are associated not with deformations, but with the translational and/or rotational motion of various layers of the 23-λm,n-vacuum.
2.7. Predictions of the kinetics of vacuum layers
2.7.1. The limiting speed of movement of 23-λm,n-vacuum layers
Let’s consider one of the metrics (96)
ds(+)2 = (1– vx2/с2)c2dt2 + 2vxdxdt – dx2 – dy2– dz2.
In these notations, metric (102) takes the form [7]
corresponding to the propagation of a beam of light in the reference system of the observer, which moves together with the moving side of the 23-λm,n-vacuum.
In this case, the metric (102) describes the propagation of a light beam in a layer of vacuum, which moves with a constant speed vx relative to the reference system of a stationary observer. This is similar to how a stationary observer measures the speed of waves propagating along a moving surface of water (for example, a river). Such an observer will find that the speed of propagation of wave disturbances depends on the speed of the river flow, while relative to the water itself, the speed of wave propagation remains unchanged, and depends only on the properties of the water itself (its density, temperature, impurities, etc.).
From the first Ex. (103)
it can be seen that in the case of (94) – (96) the speed vx of the outer side of the 23-λm,n-vacuum (subcont) and its inner side (antisubcontact) cannot exceed the speed of light c (the velocity of propagation of wave disturbances along these sides), i.e. vx < c.
However, for the case (89) – (91) the situation is different. Let’s consider this variant of intravacuum processes using the example of subcont movement described by the metric (91)
ds(–)2 = (1+ vx2/с2)c2dt2– dx2 – dy2 – dz2.
In this case, the introduction of the notation
reduces the metric (106) to the form (104). At the same time, it can be seen from Еxs. (107) that there are no restrictions on the speeds vx of the mask and the interior of the subcont. This circumstance requires a separate detailed consideration, since it allows the possibility of organizing superluminal intra-vacuum communication channels by controlling the movement of vacuum layers. This is one of the possible theoretical predictions of the kinetics of the 23-λm,n-vacuum layers.
2.7.2. Inert properties of layers of 23-λm,n-vacuum
Let's return to the consideration of metrics (83)
ds(+ – – –)2 = ds(+)2 = c2dt2 – dx2 – dy2 – dz2 ,
ds(– + + +)2 = ds(–)2 = – c2dt2 + dx2 + dy2 + dz2 .
Let us take out the value с2dt2 on the right-hand sides of these metrics [7]
where v = (dx2 +dy2 +dz2)1/2/dt = dl/dt is the 3-dimensional velocity.
We extract the root of the two sides of Exs. (110) and (111). As a result, according to the symbols (84) – (87), we get
For example, consider the 4-dimensional velocity vector of the mask of the subcont
We substitute the linear form (112) into Ex. (116), as a result, we obtain the components of the 4-velocity of the mask of the subcont [7,8]
Let the mask of the subcont move only in the direction of the x-axis, then its 4-velocity has components
Let’s now define the 4-acceleration of the mask of the subcont [8]
and to simplify, consider only its x-component 4-velocity
where the value
has the dimension of the x-component of the 3-dimensional acceleration of the local section of the mask of the subcont.
On the left side of Ex. (121), we perform the differentiation operation [8]
and introduce the notation
dvx/dt = аx(+)'.
In this case, Ex. (122) takes the form
where
ax(+) is the actual acceleration section of the mask of the subcont, taking into account its inert properties;
аx(+)' is the ideal acceleration of the same section of the mask of the subcont, without taking into account its inert properties.
Let’s represent Ex. (124) in the following form
where
is the dimensionless kinematic coefficient of inertia of the local area of the mask of the subcont, which relates the actual and ideal accelerations of this 23-λm,n-vacuum layer. This coefficient shows, within the framework of the kinematic approach, how the inertness (i.e., resistance to a change in the state of movement) of this section of the mask of the subcont changes with a change in the speed of its movement.
It follows from Ex. (126) that at vх = 0 the kinematic inertia coefficient μх(+) = 1 and . This means that the section of the mask of the subcont does not exert any resistance to the beginning of its movement (or displacement). As vх approaches the speed of light c, the kinematic inertia coefficient μх(+) tends to infinity, while further acceleration of this 23-λm,n-vacuum layer becomes impossible.
Ex. (126) is a massless analog of Newton's second law
where Fx is the x-component of the force vector; m is body weight; ax′ is the x-component of its ideal acceleration.
Fx = max′,
Comparing Exs. (125) and (127), we find that the dimensionless coefficient of inertia μх(–) of the local area of the mask of the subcont is a dimensionless analogue of the density of the inertial mass of a continuous medium.
Sequentially performing actions (116) – (126) with metrics (113) – (115), we obtain the kinematic inertia coefficients μх(+)′′, μх(–)′, μх(–)′′ for three the remaining layers of the two-sided 23-λm,n-vacuum. The overall kinetic inertia coefficient of the local two-sided section of the 23-λm,n-vacuum is a function of all four inertia coefficients
μх(±) = f (μх(+)′ , μх(–)′′, μх(–)′, μх(–)′′ ).
The explicit form of this function is determined when describing the dynamics of 23-λm,n-vacuum, which will be presented in subsequent articles of this project.
2.7.3. Kinematics of the rupture of the local section of the 23-λm,n-vacuum
"For in much wisdom is much vexation, and he
who increases knowledge increases sorrow."
Kohelet (Ecclesiastes 1:18)
Let’s consider the kinematic aspects of the possibility of a "rupture" of the of the local section of the two-sided 23-λm,n-vacuum.
We integrate Ex. (121) [8]
Integrating expression (129) once more and setting x0 = 0 at t = 0, we obtain the following change in the length of the mask of the subcont of the 23-λm,n-vacuum along the x axis during its accelerated motion [8]:
Let the initial (i.e., immobile) state of the local section of the subcont be given by the metric (108)
The uniformly accelerated motion of this section along the x axis is formally specified by the coordinate transformation [8]:
Differentiating the coordinates (132) and substituting the results of differentiation into the metric (131), we obtain the following metric [8]
which describes the uniformly accelerated motion of the local section of the subcont (i.e., the outer side of the 23-λm,n-vacuum) in the direction of the x axis.
If, in the same area of the subcont, an additional flow with uniformly slow motion (i.e., with negative acceleration) is created,
then, doing mathematical calculations similar to (130) – (133), we get the metric
In this case, the average metric-dynamic state of the local section of the subcont will be characterized by the averaged metric
with signature (+ – – –).
It can be seen from the metric (106) that in the case
or |ах|t = c or |ах| = c /Δt, (136)
the first and second terms in the averaged metric (136) go to infinity. This singularity can be interpreted as a "rupture" of the subcont area under study (i.e., a "rupture" of the local region of the 23-λm,n-vacuum outer side).
Breaking a subcont is an incomplete action. For a complete "rupture" of the local section of the 23-λm,n-vacuum, it is necessary to “break” its inner side, described by the metric (109) with the signature (– + + +). To do this, it is necessary to create similar uniformly accelerated and uniformly retarded flows in the same region of space in the antisubcont (i.e., in the inner side of the 23-λm,n-vacuum), so that its average state is determined by the averaged metric
with a signature (– + + +), which "breaks" under the same conditions
or |ах|t = c, or |ах| = c /Δt. (138)
Averaging metrics (136) and (138) leads to the fulfillment of the vacuum condition
which in this situation is equivalent to Newton's third law: – "Action is equal to reaction":
Fx(+) – Fx(–) = max(+) – max(–) = ax(+) – ax(–).
That is, the process of "rupture" of the local region of the λm,n-vacuum is similar to the rupture of an ordinary (atomistic) solid body, to which sufficiently large equal forces (more precisely, accelerations) are applied from its two sides.
It is not excluded that the conditions of "rupture" described above λ -11, -13 -vacuum are formed in the collision of oncoming flows of elementary particles accelerated on the collider. It is possible that a strong collision of particles leads to the emergence of a web of vacuum "cracks", while the closed cracks scatter in the form of many new "particles" and "antiparticles".
To obtain "rupture" of the vacuum of large scales, it is necessary to initiate accelerated flows of different sides of the λ2,3 -vacuum (see §1 in the article [1]).
Apparently, "ruptures" of vacuum of various scales occur in the nature around us, for example, when new particles are born from the void during the collision of atmospheric molecules with cosmic radiation, or during lightning flashes in rain clouds. We are here only trying to describe these phenomena on the basis of a geometrized vacuum physics based on the Algebra of Signatures. However, the development of “zero” (vacuum) technologies is fraught with great dangers. Therefore, in parallel with the development of vacuum physics (in particular, the kinetics of vacuum layers), it is necessary to rethink the religious and philosophical aspects of modern science, to develop "Vacuum Ethics", "Vacuum Aesthetics" and "Vacuum Psychology". Otherwise, this knowledge will not benefit our civilization [5,9].
3. Conclusions
“Whoever fights monsters, you yourself
do not become a monster. And when you
gaze long into an Abyss, the Abyss also
gazes into you.”
Friedrich W. Nietzsche
“Jenseits Gut und böse” (Beyond Good and evil)
This article is the third part of a unified study under the general title "Geometrized vacuum physics based on the Algebra of Signatures".
In the first two parts of this study, presented in the author's articles [1,2], a method was proposed for studying an ideal (i.e., non-curved and stationary) vacuum region by probing it with mutually perpendicular light rays with different wavelengths λm,n. This method made it possible in the first two articles [1,2] to lay the foundations first of the Algebra of Stignatures, and then of the Algebra of Signatures.
This article considers the possibilities of describing the curved state of the same region of the vacuum on the basis of further development of the mathematical apparatus of the Algebra of Signatures.
We list the main results obtained in this article:
1) On the example of one of the λm,n-vacuums, it is shown that many levels of consideration of its curvatures are possible. The simplest of them is at least double-sided. This level of consideration implies that the empty space (vacuum) surrounding us has at least two 4-dimensional sides, which can be conditionally called: the outer side with the Minkowski space signature (+ – – –), and the inner side with the completely opposite signature of anti-Minkowski space (– + + +). In this case, to describe the curvature of the local region of the two-sided 23-λm,n-vacuum, not 16 components of the metric tensor are required, as in a one-sided theory, for example, in general relativity, but 16 + 16 = 32 = 25 components of the metric tensor. The next level of consideration is the λm,n-vacuum with 16 sides. At this level of consideration, to describe the curvature of the local region of the sixteen-sided 26-λm,n-vacuum, already 16 × 16 = 256 = 28 components of the metric tensor are required. In this case, the description of the curvature is much more accurate than with a two-sided description. The mathematical apparatus of the Algebra of Stignatures provides for the possibility of increasing the number of metric tensor components describing the curvature of the local region of the λm,n-vacuum to infinity (see §2.9 in [2]).
2) It is shown that a length element on one side of a two-sided space with completely opposite signatures corresponds to a perpendicular element on its other side (see §2.5.2 of this article). This, at first glance, simple observation entails very significant consequences. This circumstance is the reason why many intra-vacuum and macroscopic processes turn into spirals.
3) The physical meaning of the zero and non-zero components of the metric tensor is revealed in the framework of the proposed "Geometrized vacuum physics" (see §2.6 of this article).
4) The 4-strain tensor and the components of the relative elongation vector are introduced for two-sided and 16-sided consideration of the λm,n-vacuum curvatures (see §§2.5.2 and 2.5.3 of this article).
5) Some aspects of the kinematics of the layers of λm,n-vacuum are considered (see §2.7 of this article). This made it possible to analyze the inert properties of the λm,n-vacuum (see §2.7.1), and to predict the possibility of displacement of vacuum layers at a speed much higher than the speed of light (see §2.7.2). At the same time, it is shown that the longitudinal displacement of the outer and inner sides of the λm,n-vacuum relative to each other inevitably leads to their twisting into a spiral bundle.
6) A kinematic model of the possibility of a local “rupture” of λm,n-vacuum is considered (see §2.7.3).
The "Geometrized vacuum physics based on the Algebra of Signatures" proposed in articles [1,2] and in this article is far from being completed, but already now, within the framework of this research program, the possibility of developing “zero” (i.e., vacuum) technologies. At the same time, it is obvious that the development of these technologies requires a significant increase in the responsibility of mankind to the Universe [5,9].
Acknowledgments
I express my sincere gratitude to R. Gavriil Davydov, David Reid and R. Eliezer Rahman for their assistance. The discussion of the article was attended by Academician of the Russian Academy of Sciences Shipov G.I., Ph.D. Lukyanov V.A., Lebedev V.A., Prokhorov S.G. and Khramikhin V.P. Also, the author is grateful for the support of Salova M.N., Morozova T.S., Przhigodsky S.V., Maslov A.N., Bolotov A.Yu., Ph.D. Levi T.S., Musanov S.V., Batanova L.A., Ph.D. Myshelov E.P., Chivikov E.P.
References
- Batanov-Gaukhman, M. (2023) “Geometrized vacuum physics. Part I. Algebra of Stignatures“. [CrossRef]
- Batanov-Gaukhman, M. (2023) “Geometrized vacuum physics. Part II. Algebra of Signatures“. [CrossRef]
- Sedov, L.I. (1994) ”Continuum mechanics. T1”, – Moscow: Nauka, [in Russian]. (Available in English "A course in continuum mechanics", translation from the Russian, ed. by J. R. M. Radok).
- Shipov, G. (1998) ”A Theory of Physical Vacuum”, – Moscow ST-Center, Russia ISBN 5 7273-0011-8. (available in English).
- Gaukhman, M.Kh. (2007) "Algebra of Signatures "Void" (yellow Alsigna), – Moscow: URSS, p. 308, ISBN 978-5-382-00580-5, (available at www.alsigna.ru).
- Gaukhman, M.Kh. (2017) "Algebra of Signatures "Massless physics" (purple Alsigna). – Moscow: Filin, ISBN 978-5-9216-0104-8 (available in English http://metraphysics.ru/).
- Landau, L.D. & Lifshits, E.M. (1988) "Field theory". Vol. 2. – Moscow: Nauka, p. 509, ISBN 5-02-014420-7 [in Russian]. (available in English "The Classical theory of fields", Vol. 2. fourth English edition).
- Logunov, A.A. (1987) "Lectures on the theory of relativity and gravity", – Moscow: Nauka, p. 271 [in Russian].
- Gaukhman, M.Kh. (2007) "Algebra of Signatures "NAMES" (orange Alsigna), – Moscow: LKI, p.228, ISBN 978-5-382-00077-0 (available on site www.alsigna.ru) [in Russian].
Figure 1.
Discrete set of nested λm,n-vacuums of the same 3-dimensional volume of a vacuum, where λm,n > λm+1,n+1 >λ m+2,n+2 >λm+3,n+3.
Figure 1.
Discrete set of nested λm,n-vacuums of the same 3-dimensional volume of a vacuum, where λm,n > λm+1,n+1 >λ m+2,n+2 >λm+3,n+3.
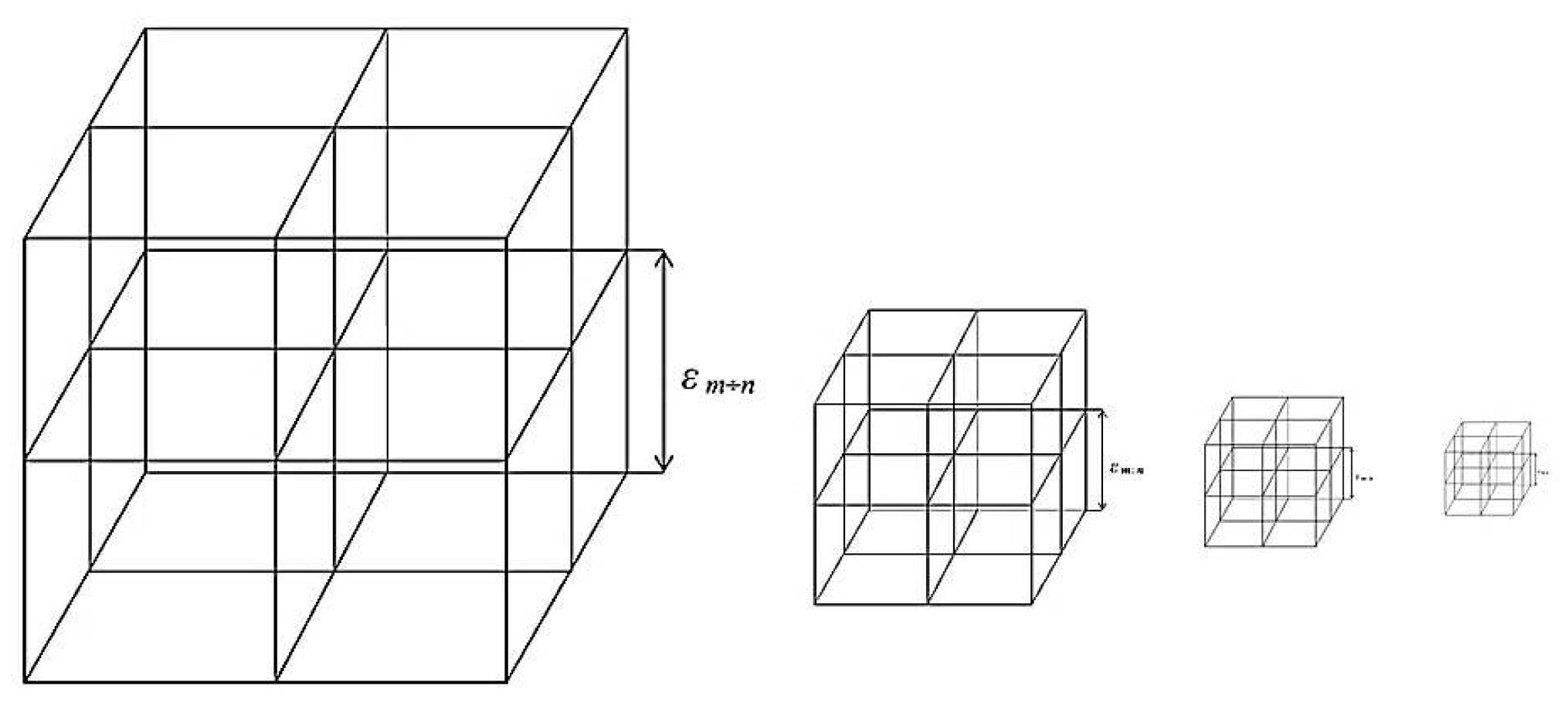
Figure 2.
Experimental data on the thickness of the laser beam depending on the length wave λ of the corresponding monochromatic electromagnetic radiation.
Figure 2.
Experimental data on the thickness of the laser beam depending on the length wave λ of the corresponding monochromatic electromagnetic radiation.

Figure 3.
Illustration of a curved λm,n-vacuum embedded in curved λf,d-vacuum (where λf,d > λm,n).

Figure 5.
Sixteen 4-bases associated with eight corners of the λm,n-vacuum cube (repeat of the Figure 7 in [1]).
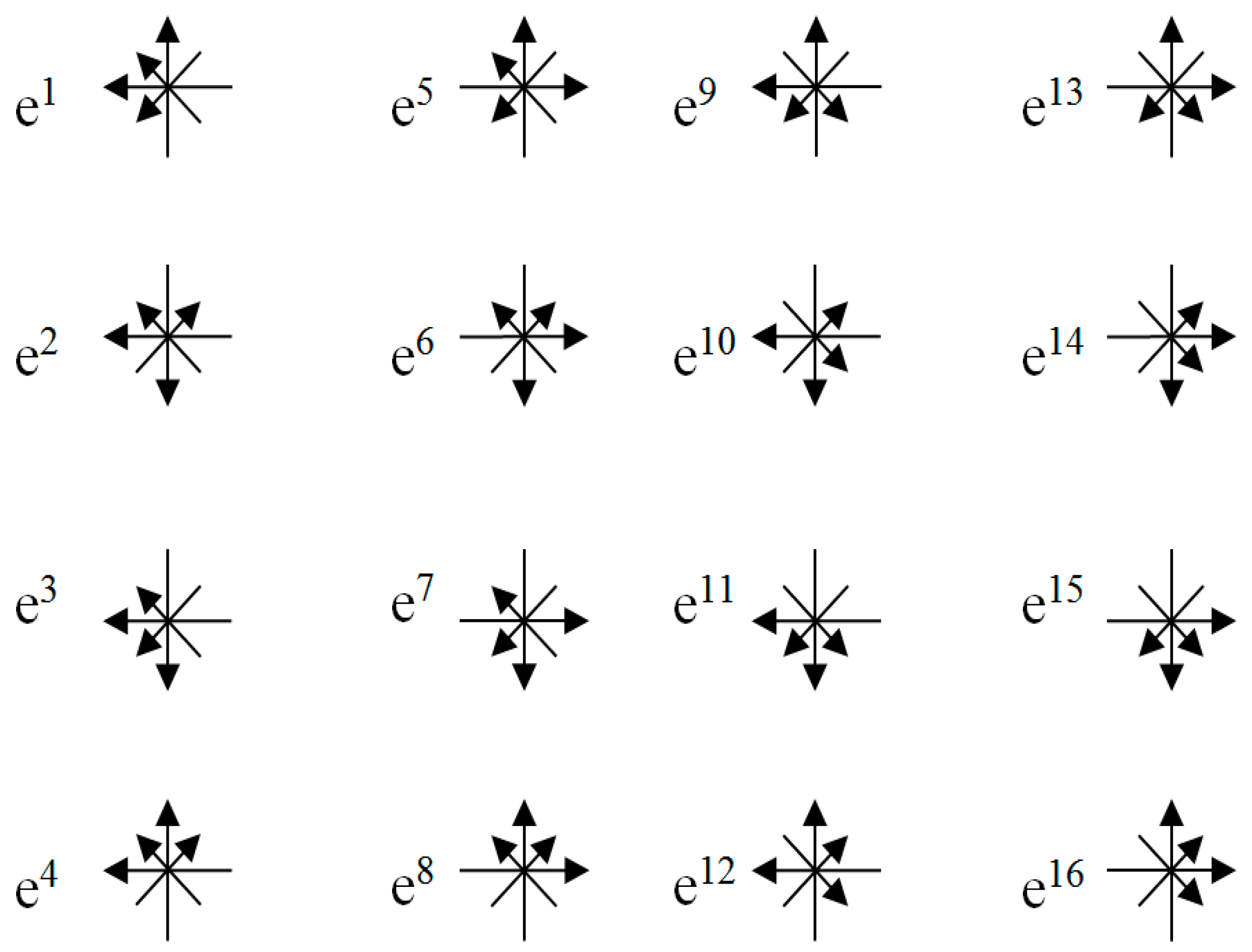
Figure 8.
Two sides of one sheet of paper.

Figure 9.
A cube isolated in the thickness of a sheet of paper (or in some other continuous medium, including vacuum).
Figure 9.
A cube isolated in the thickness of a sheet of paper (or in some other continuous medium, including vacuum).
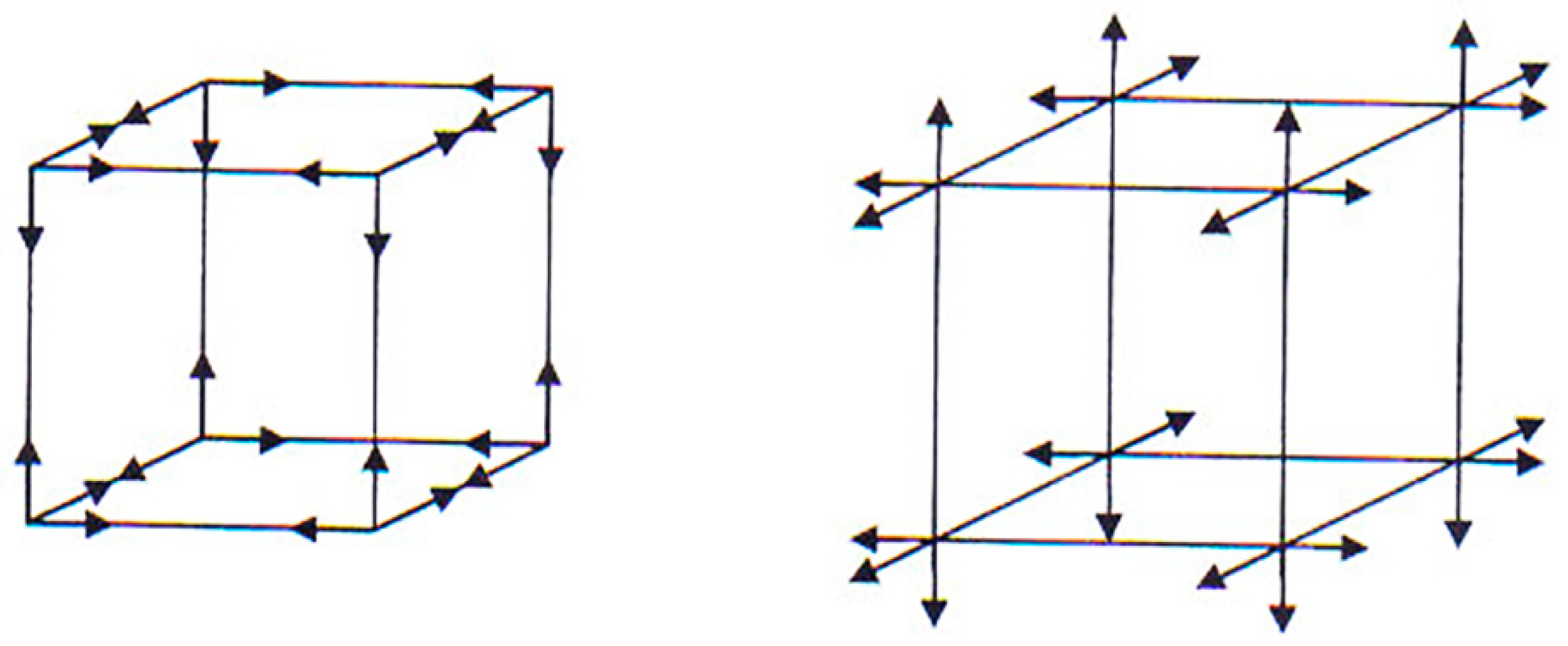
Figure 10.
a) Mutually perpendicular segments ()1/2ds(+) and ()1/2ds(–); b) If you project a double helix onto a plane, then at the intersection of its lines ds(–) and ds(+) are always mutually perpendicular.
Figure 10.
a) Mutually perpendicular segments ()1/2ds(+) and ()1/2ds(–); b) If you project a double helix onto a plane, then at the intersection of its lines ds(–) and ds(+) are always mutually perpendicular.

Figure 11.
Two sides of the space with completely opposite stignatures {+ +} and {– –}.

Figure 12.
Woven threads of the fabric.

Figure 13.
Fractal illustration of a warped 26-λm,n-vacuum fabric woven from 16 "color" lines (threads).
Figure 13.
Fractal illustration of a warped 26-λm,n-vacuum fabric woven from 16 "color" lines (threads).

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Copyright: This open access article is published under a Creative Commons CC BY 4.0 license, which permit the free download, distribution, and reuse, provided that the author and preprint are cited in any reuse.
Alerts
MDPI Initiatives
Important Links
© 2025 MDPI (Basel, Switzerland) unless otherwise stated





