Self-Interactions, Self-Energy and the Electromagnetic Contribution to the Anomalous g-Factor

Preprint

Article

Self-Interactions, Self-Energy and the Electromagnetic Contribution to the Anomalous g-Factor

Altmetrics

Downloads

260

Views

333

Comments

A peer-reviewed article of this preprint also exists.

Miroslav Georgiev^*

This version is not peer-reviewed

Submitted:

02 October 2023

Posted:

04 October 2023

Read the latest preprint version here

Alerts

Abstract

The present paper reports an exact approach quantifying the electromagnetic contribution to the anomalous magnetic moment occurring in isolated system comprised of non-composite particle carrying elementary electric charge. Essential averaging procedure and regularization of the electromagnetic field potentials necessary when quantifying the electromagnetic self-interactions and when deriving equations of motion without singularities and obeying the conservation laws are thoroughly discussed. The study shows that the dynamics of the considered system is associated to a unique classical transcendental equations of motion satisfied by the particle’s velocity and the electromagnetic contribution to the anomalous g-factor known from the quantum electrodynamics. The equations of motion predict a value of the anomalous g-factor that agrees with the experimentally measured one reported in the literature and that calculated with the aid of quantum electrodynamics. In the present study the computational accuracy is restricted to match one part in a billion, obtaining ae=0.001159652(23), thus reveling the potential of non-perturbative methods in predicting the electron’s anomalous g-factor.

Keywords:

Subject: Physical Sciences - Theoretical Physics

1. Introduction

The anomalous magnetic moment and the intrinsic dynamics of non-composite particles have been considered as unique features of the quantum field theory since the beginning of its elaboration [1,2,3,4,5]. The electron’s anomalous magnetic moment and its fundamental properties are the first to be studied and realized. With the aid of quantum electrodynamics the value of corresponding g-factor was predicted with a stunning accuracy [6,7,8,9,10,11], leaving no space for mistrusting its effectiveness. Following, were the properties of the two remaining leptons carrying elementary charge, the muon and tau. Determining with high accuracy the dynamics of both massive leptons is an active field of research. The muon’s anomalous magnetic moment is still puzzling the community aiming to reduce the gap between theory and experiment [12,13,14,15,16,17,18,19], recently known to be of about

0.58

%. On the other hand, having a very short lifetime and being the massive among all leptons, measuring and predicting tau anomalous magnetic moment is a challenging task requiring great efforts [20,21,22,23,24,25,26]. Although, there is a serious discrepancy between theory and experiment, such efforts may have the potential to shed more light on the contribution of high order hadronic terms thus aiding in resolving the inconsistency in the muon data.

The microscopic electrodynamics underlying the occurrence of anomalous magnetic moment is indispensably related to the nature of self-interactions [1,27,28]. The latter are believed to be uniquely addressable by the regularization and renormalization methods of quantum theory [29,30,31,32,33], with no classical analog. Yet, even within the standard methods of quantum mechanics, the evaluation of self-Coulomb energy in multi-electron systems still poses a challenge [34,35].

The quantum and classical theories are believed to have no interconnection pertaining to the occurrence of anomalous magnetic moment and self-energy divergence problem. Therefore, a classical method with the potential to quantify the anomalous magnetic moment and the self-interactions in the absence of singularities and electromagnetic radiation may contribute significantly in establishing a better interrelationship between both theories.

The present paper propose an exact approach quantifying the electromagnetic contribution to the anomalous magnetic moment and related dynamics of isolated non-composite particle carrying elementary electric charge. The approach build on the methods of classical electrodynamics in studying the microscopic dynamics of self-symmetric systems, like the considered one, by removing all singular points. It incorporates a particular spatial averaging procedure and regularization of the electromagnetic field potentials confining the field to the particle itself. Accordingly, the effect of self-repulsion is accounted for without singularity, or violation of the conservation laws, allowing a complete microscopic description of the system’s energy state. Two types of self-interactions are predicted, the self-Coulomb and self-Zeeman ones. Essentially, the considered approach results in a system of transcendental equations of motion satisfied by the particle’s velocity and the anomalous g-factor of electromagnetic origin. The derived system of equations ensure fast and accurate computational results and predicts the value of anomalous g-factor with the same success the perturbative approach of quantum electrodynamics does. In the present work, the computations are carried out with accuracy matching one prat in a billion.

The rest of the paper is organized as follows. The mathematical notation of all fundamental physical quantities characterizing the system under consideration along with essential interrelationships between the introduced observables are presented in Section 2. Furthermore, the section discusses the occurrence of self-interactions and their explicit representation. Lagrangian and Hamiltonian density representations are also given. The main results are outlined in Section 3, with computations carried out on Wolfram Mathematica. Section 4 summarizes the used approach and obtained results.

2. Theoretical Background

2.1. General Considerations

In the present study all representations are restricted to the mathematical framework of the classical relativistic mechanics and electrodynamics, overlooking all relevant quantum mechanical representations except the ones of immense significance. For the sake of clarity, all physical quantities and equations of motion are represented within the standard three-dimensional vector formalism. The four-vector convention is omitted, since the representation in Minkowski space with the relevant Lorentz group is straightforward [36,37,38].

Consider an isolated system composed of single non-composite particle of type q, with rest frame of reference

R

, rest mass

m_{q}

and electric charge

e_{q}^{\pm} = \pm e

\forall q

, where e denotes the elementary charge. Let

r_{c q} = α {\bar{λ}}_{c q}

be the particle’s electromagnetic radius at rest, where

α

and

{\bar{λ}}_{c q}

are the fine structure constant and reduced Compton wavelength, respectively. Let

u_{q}

, with

κ \cdot u_{q} = u_{q}

and

{\dot{u}}_{q} = 0

, be the velocity associated to the considered particle and

p_{q} = γ_{q} m_{q} u_{q}

the corresponding momentum in the observer’s rest frame

O

, where

γ_{q}

is the corresponding Lorentz factor. As we will see later in the discussion, for the considered system

u_{q} = 0 \Leftrightarrow ∄ e

. Furthermore, let

r_{q} = {\bar{λ}}_{q}

, with

λ_{q} = λ_{c q}

\sqrt{β_{q}^{- 2} - 1}

, be the particle’s electromagnetic radius in

O

, where

λ_{q}

is the particle’s intrinsic wavelength,

β_{q} = u_{q} c^{- 1}

and c denotes the light speed in vacuum. Since the particle alone represents the only center of symmetry in the system,

r_{q}

and

p_{q}

are conjugate intrinsic variables, satisfying

r_{q} p_{q} = {\bar{λ}}_{c q} m_{q} c

Let

ρ_{| e_{q}^{\pm} |}

and

ρ_{m_{q}}

, with

ρ_{s} = \frac{2}{V_{c q}} \int_{0}^{\infty} s δ (\bar{λ} - α {\bar{λ}}_{c q}) d \bar{λ}, s = {| e_{q}^{\pm} |, m_{q}},

be the charge and rest mass densities, respectively, such that

V_{c q} = 8 π \int_{0}^{\infty} \int_{0}^{\bar{λ}} δ (\bar{λ} - α {\bar{λ}}_{c q}) r^{2} d r d \bar{λ},

(1)

and

e_{q}^{\pm} = \pm \int_{Ω_{c q}} ρ_{| e_{q}^{\pm} |} d v, m_{q} = \int_{Ω_{c q}} ρ_{m_{q}} d v,

(2)

where the spatial domain

Ω_{c q} \in R^{3}

, with a boundary

\partial Ω_{c q}

, is such that

V_{c q} = \int_{Ω_{c q}} d v

Let for all q the system is characterized by an effective rest mass given by

M_{q} = \int_{Ω_{c q}} ρ_{M_{q}} d v,

(3)

where the corresponding density

ρ_{M_{q}} = ρ_{M_{q}} (r)

is a smooth function over

r \in (0, + \infty)

The system posses intrinsic angular momentum

f_{q}

and a corresponding magnetic one

μ_{q}

, with magnitudes

f_{q}

and

μ_{q}

, respectively. In accordance to (3), we have

f_{q} = \int_{Ω_{c q}} r_{q} \times j_{M_{q}} d v, μ_{q} = \frac{1}{2} \int_{Ω_{c q}} r_{q} \times G_{q} j_{e_{q}^{\pm}} d v,

(4)

where

j_{M_{q}} = γ_{q} ρ_{M_{q}} u_{q}

and

j_{e_{q}^{\pm}} = \pm γ_{q} ρ_{| e_{q}^{\pm} |} u_{q}

are the system’s mass and charge density currents, respectively. Here,

G_{q}

is the integrand g-factor, where the latter reads

g_{e} = \frac{1}{V_{c q}} \int_{Ω_{c q}} G_{q} d v, G_{q} = 2 \frac{| e_{q}^{\pm} | ρ_{M_{q}}}{m_{q} ρ_{| e_{q}^{\pm} |}} .

(5)

We further have

g_{e} = 2 (1 + a_{e})

, where

a_{e}

is the electromagnetic contribution to the anomalous g-factor.

Let us point out that according to (5), the fraction

M_{q} m_{q}^{- 1}

is a constant for all q. We would like to stress, furthermore, that

f_{q}

is not an orbital angular momentum, since the system does not posses a center of symmetry independent from the particle. In the vector diagram sketching the dynamics of the considered system, the position vector

r_{q}

associated to the density currents will remain conjugate to the particle’s momentum for an infinite time. In other words, the dynamics related to both physical quantities given in Equation (4) is not time and space independent event for an observer in

O

and hence it cannot be associated to a free spinning sphere of radius

r_{c q}

. The particle’s momentum and corresponding currents are intrinsic, not relative to an observer and not a consequence of independent external action.

All of the above considered relations and definitions hold for

u_{q} = v_{q} + {\tilde{v}}_{q}

, where

v_{q}

is the velocity component characterizing a node moving relative to the origin of

O

and

{\tilde{v}}_{q}

is the velocity component associated to an oscillation occurring with respect to that node. In this regard, the particle has a standing wave representation that is naturally related to some uncertainty. The corresponding oscillation dynamics can be further studied within the classical wave theory, or with the more rigorous methods of the quantum theory. Such studies lie beyond the goals of the present research and may be discussed elsewhere.

2.2. Field Observables

Since the system is isolated, or

u_{q} \neq u_{q} (t)

, and the particle posses elementary electric charge confined within the time independent spatial domain

Ω_{c q}

, the resulting electric current and its density are time and spatially independent. Accordingly, the electric

E_{q}

and magnetic

B_{q}

components of the corresponding electromagnetic field in

O

are time independent and spatially conjugated to the particle’s momentum. Thus, we have the relations

E_{q} = - \nabla φ_{q}

and

B_{q} = \nabla \times A_{q}

, where

φ_{q} = φ_{q} (r)

and

A_{q} = A_{q} (r)

are the scalar and vector potentials of the electromagnetic field in

O

. In particular, we have

φ_{q} = γ_{q} ϕ_{q}, A_{q} = γ_{q} \frac{u_{q}}{c^{2}} ϕ_{q}, B_{q} = \frac{1}{c^{2}} (u_{q} \times E_{q}),

(6)

where the function

ϕ_{q} = ϕ_{q} (r)

, given with respect to the observer’s rest frame, is regularized to the origin of

R

and reads

ϕ_{q} (r) = \frac{e_{q}^{\pm}}{4 π ε_{o} r} (1 - e^{- \frac{γ_{q} r}{(1 + a_{e}) λ_{c q}}}),

(7)

with

ε_{o}

denoting the vacuum permittivity. The function given in Equation (7) is solution to the superposition of Poisson and Helmholtz equations, with constant of integration equal to zero at the limit

r \to \infty

and minimal wavelength of the electromagnetic field equal to

(1 + a_{e}) λ_{c q}

\sqrt{1 - β_{q}^{2}}

r \to 0

. Graphical representation of the difference between the Coulomb potential and the one given in Equation (7) is shown in Figure 1.

We would like to note that in Cartesian coordinates, the components of the vector potential given in (6) read

A_{q}^{τ} = γ_{q} c^{- 2} u_{q}^{τ} ϕ_{q} (β, ν)

, where

τ \neq β \neq ν = {x, y, z}

and

u_{q}^{τ}

are the components of

u_{q}

. The potentials given in Equation (6) satisfy trivially both choices of gauge the Coulomb and Lorenz one.

Owing to Equation (7), we obtain the analytical expression of the electromagnetic field’s energy, with density

ε_{o} E_{q}^{2}

, for all q. Thus, integrating over

R^{3}

, we get

W_{q} (r) = α c^{2} γ_{q}^{2} m_{q} \frac{{\bar{λ}}_{c q}}{2 r} (2 - 4 e^{\frac{γ_{q} r}{(1 + a_{e}) λ_{c q}}} + 2 e^{2 \frac{γ_{q} r}{(1 + a_{e}) λ_{c q}}} + \frac{γ_{q} r}{(1 + a_{e}) λ_{c q}}) e^{- 2 \frac{γ_{q} r}{(1 + a_{e}) λ_{c q}}},

(8)

where the constant of integration is fixed at

r \to \infty

. Graphical representation is depicted in the inset in Figure 1.

From Equations (7) and (8), we obtain two important limits. We have,

lim_{r \to 0} φ_{q} (r) = \frac{γ_{q}^{2} e_{q}^{\pm}}{4 π ε_{o} (1 + a_{e}) λ_{c q}} and lim_{r \to 0} W_{q} (r) = \frac{α c^{2} γ_{q}^{3} m_{q}}{4 π (1 + a_{e})},

respectively. These results point out that the energy of generated electromagnetic field does not vanish at

r \to 0

and the potential energy is a constant ensuring that the self-interactions do not vanish at the given limit and do not associate to singularity points. Hence, on any gradient surface, including all points on the boundary

\partial Ω_{c q}

, the presence of electromagnetic field appears as a steady electromagnetic veil making the particle to appear as a very round object [39]. In addition, for the corresponding Umov-Poynting vector, we have

μ_{o}^{- 1} (E_{q} \times B_{q}) = ε_{o} E_{q}^{2} u_{q}

, where

μ_{o}

is the vacuum magnetic permeability.

Note that, the discussed electromagnetic field is consistently confined to the particle. It depends exclusively on the particle’s intrinsic characteristics and does not obey the Liénard-Wiechert representation [38,40,41,42]. Hence, the electromagnetic field does not propagate at speed c independently from the particle and does not classify as an on shell coupling between the electric and magnetic field components. We neither observe spontaneous emission nor absorption of photons. In this regard, the steady presence of electromagnetic field around the particle can be considered as a classical analog of the foam of virtual photons presented in quantum electrodynamics.

2.3. Electromagnetic Self-Energy and Self-Interactions

Since the system is closed, with the action of no additional fields, the particle exhibits no exchange of energy and hence momentum. Accordingly, neither external nor net self-forces [38,43,44] are acting on the particle and the energy of the system remains purely kinetic. However, the considered system definitely exhibits two types of self-interactions of electromagnetic nature, with average energy depending only on

u_{q}

The Hamiltonian describing the total energy of electromagnetically self-interacting particle read

H_{q} = γ_{q} m_{q} c^{2} + Σ_{q} + Z_{q},

(9)

where the energy terms

Σ_{q}

and

Z_{q}

are associated to the self-Coulomb and self-Zeeman interactions, respectively.

The self-Coulomb term is the spatial average over the domain

Ω_{c q}

of the interaction energy between the particle’s charge and its own electromagnetic field. It reads

Σ_{q} = γ_{q} c^{2} \int_{Ω_{c q}} ρ_{M_{q}} - ρ_{m_{q}} d v,

(10)

where

ρ_{M_{q}} = ρ_{m_{q}} (1 + η_{q} \frac{r_{c q}}{r} (1 - e^{- \frac{γ_{q} r}{(1 + a_{e}) λ_{c q}}})),

(11)

η_{q} = 1 + β_{q}^{2}

. Here, we have taken into account the relation

ρ_{m_{q}} c^{2} = | e_{q}^{\pm} | ρ_{| e_{q}^{\pm} |} {(4 π ε_{o} r_{c q})}^{- 1}

The self-Zeeman term represents the spatial average over the domain

Ω_{c q}

of the interaction energy between the system’s intrinsic magnetic moment presented in (4) and the induced magnetic field given in (6). Since the self-Zeeman interaction is time independent, the corresponding integrand satisfies

- μ_{q} \cdot B_{q} = - μ_{q} B_{q}

, where in accordance to (6), we have

B_{q} = c^{- 2} γ_{q} u_{q} | \nabla ϕ_{q} |

. Thus, taking into account relations (4), according to which

μ_{q} = e {\bar{λ}}_{c q} c \frac{M_{q}}{m_{q}} and f_{q} = {\bar{λ}}_{c q} M_{q} c,

we obtain

Z_{q} = - \frac{f_{q} p_{q}}{m_{q}^{2} η_{q}} \int_{Ω_{c q}} | \nabla ρ_{M_{q}} | d v .

(12)

The self-energy terms given in Equations (10) and (12) are intrinsic to the considered particle and hence invariant to an external influence. Therefore, represented in terms of quantum theory they will remain invariant with respect to the particle’s orbital state in many-body systems. That may be of benefit to the researchers studying multi-electron systems with the aid of computational methods that fail to account for the self-interactions without generating errors, see for example the case of Kohn-Sham density functional theory [35,45,46].

2.4. The Lagrangian and Hamiltonian Density

In Section 2.3 we discuss the two allowed by the conservation laws types of electromagnetic self-interactions that take place only within the domain

Ω_{c q}

and have particular energy densities.

In general, the Hamiltonian (9) has a corresponding density and Lagrangian. The latter reads

L_{q} = p_{q} \cdot {\tilde{u}}_{q} - γ_{q} c^{2} ρ_{M_{q}} + \frac{M_{q} p_{q}^{3}}{m_{q}^{3} η_{q}} κ \cdot \nabla_{p} ρ_{M_{q}},

(13)

where

{\tilde{u}}_{q} = κ \frac{2}{V_{c q}} \int_{0}^{\infty} u_{q} (\bar{λ}) δ (\bar{λ} - {\bar{λ}}_{q}) d \bar{λ}

(14)

is the generalized velocity and

ρ_{M_{q}} = ρ_{m_{q}} (1 + \frac{η_{q}}{m_{q}} \frac{α}{c} p_{q} (1 - e^{- \frac{γ_{q} m_{q} c}{2 π (1 + a_{e}) p_{q}}}))

(15)

is the momentum representation of the effective mass density. Here, we take into account that

κ \cdot \nabla_{p} ρ_{M_{q}} \in R_{+}

for all

u_{q} \in (0, c)

, such that

| \nabla ρ_{M_{q}} (r_{q}) | \to p_{q}^{2} {(λ_{c q} c m_{q})}^{- 1} κ \cdot \nabla_{p} ρ_{M_{q}} (p_{q})

For the corresponding Hamiltonian density, we have

H_{q} = γ_{q} c^{2} ρ_{M_{q}} - \frac{M_{q} p_{q}^{3}}{m_{q}^{3} η_{q}} κ \cdot \nabla_{p} ρ_{M_{q}},

(16)

where the Hamiltonian’s equations read

{\tilde{u}}_{q} = \nabla_{p} H_{q}, and {\dot{p}}_{q} = 0 .

(17)

We would like to point out that Equations (13) to (17) are derived by bearing in mind that the components of

u_{q}

are constrained over time. We have

u_{q} (t) \cdot u_{q} (t) = u_{q}^{2}

, with

u_{q} \neq u_{q} (t)

3. Results

3.1. Equations of Motion

Following the Hamiltonian’s equations (17), the generalized velocity representation (14) and (5), we obtain the system’s equations of motion.

Working with the magnitude of the particle’s velocity for convenience, we have

u_{q} = \int_{Ω_{c q}} κ \cdot \nabla_{p} H_{q} d v .

(18)

Integrating over the domain

Ω_{c q}

, we obtain one of the equations. It is a transcendental equation and reads

α c γ_{q} {\tilde{η}}_{q} (1 - (1 + \frac{c}{2 π (1 + a_{e}) u_{q}}) e^{- \frac{c}{2 π (1 + a_{e}) u_{q}}}) - \frac{α c γ_{q}^{2}}{4 π^{2} (1 + a_{e})} e^{- \frac{c}{2 π (1 + a_{e}) u_{q}}} - u_{q} = 0,

(19)

where

{\tilde{η}}_{q} = η_{q} + 3 (1 + a_{e}) γ_{q} β_{q}^{2}

On the other hand, taking into account the explicit representation of the effective mass density (11), from (5) we obtain a second equation. For all q, we have

3 η_{q} (\frac{1}{2} - (\frac{1 - e^{- \frac{γ_{q} α}{2 π (1 + a_{e})}} (1 + \frac{γ_{q} α}{2 π (1 + a_{e})})}{{(\frac{γ_{q} α}{2 π (1 + a_{e})})}^{2}})) - a_{e} = 0 .

(20)

Equations (19) and (20) represent system of transcendental equations of motion, with

u_{q} = u_{e}

for all q and

m_{q}

, where

u_{e} = c o n s t .

, for

t \in [0, + \infty)

. The solutions yield to the exact value of the particle’s energy represented by the Hamiltonian (9) and essentially to that of the electromagnetic contribution to the anomalous g-factor. It is worth noting that from Equation (19) follows that

β_{e} \approx α

Within the considered theoretical approach

u_{e}

is invariant for all observers, with relative quantities being the velocities

v_{q}

and

{\tilde{v}}_{q}

, see Section 2.1. Accordingly, although the system’s total energy associated to the Hamiltonian given in Equation (9) remains invariant, the particle’s behavior with respect to

O

remains relative. Thus, for different observers the particle will have different behavior. In the case

| {\tilde{v}}_{q} | ⋙ | v_{q} |

the particle will appear as more localized and associated to a high frequency oscillation in time. On the opposite, for

| v_{q} | ⋙ | {\tilde{v}}_{q} |

the particle will be associated to a traveling wave of lower frequency. In both cases, however, because of it’s electrical charge yielding

u_{e} \neq 0

for an infinite time the particle cannot be at rest in any of the observers’ rest frame of references. Moreover, since the magnitudes of

v_{q}

and

{\tilde{v}}_{q}

are time independent it follows that the oscillation dynamics will be underpinned by a plane of isotropy, with

v_{q} \cdot {\tilde{v}}_{q} = 0

3.2. Effective Mass-Energy Equivalence

Taking into account the contribution only of the electric part of the self-interactions in (9), we obtain the effective mass-energy relation. Accounting for the first two terms on the right hand side after the corresponding equality symbol, we get

E_{q} = γ_{e} M_{q} c^{2},

(21)

where for the self-Coulomb energy term we get

Σ_{q} = a_{e} γ_{e} m_{q} c^{2}

and for the effective rest mass we have

M_{q} = m_{q} (1 + a_{e})

. Here,

γ_{e}

is a constant for all q, obtained by solving Equations (19) and (20), see Section 3.1. We would like to point out once more that within the considered mathematical framework if

∄ e

, then

∄ a_{e}

and the particle’s speed equals zero. Thus, the energy of a free electrically charged non-composite particle of rest mass

m_{q}

is always higher than the energy of a free electrically neutral non-composite particle of the same rest mass. Furthermore, according to Equation (21) the former will always be characterized by an effective momentum given by

P_{q} = γ_{e} M_{q} u_{e}

and yielding to the effective energy-momentum representation

E_{q} = \sqrt{M_{q}^{2} c^{4} + P_{q}^{2} c^{2}} .

(22)

It is important to note that the effective rest mass, energy and momentum related by Equation (22) are physical characteristics of the spinor field

Ψ_{q} (x_{μ})

that effectively account for the self-Coulomb energy of the q-th particle and satisfies the Dirac equation

(i ℏ γ^{μ} \partial_{μ} - M_{q} c) Ψ_{q} (x_{μ}) = 0 .

(23)

With the aid of Equation (23) the free-particle representation of the corresponding field holds ensuing that the quantum system under consideration remains isolated and hence the conservation laws are not violated. Moreover, Equation (23) accounts for the electromagnetic contribution to the particle’s anomalous g-factor, predicting

g_{e} = 2 (1 + a_{e})

for all q.

3.3. The Anomalous g-Factor

The computations of the electromagnetic component of the particle’s anomalous g-factor and the corresponding speed are carried out for

α = {137.035999084}^{- 1}

[47]. Solving the system of transcendental Equations (19) and (20) for the particle’s speed we obtain

u_{e} = 2.18821606262 \times 10^{6}

^{- 1}

, with corresponding value of

a_{e}

given in the second row of Table 1. Compared to the experimentally obtained [11] and calculated with the aid of quantum electrodynamics [7,8] electron’s anomalous g-factor, the numerical value obtained by solving Equations (19) and (20), with the given value of the fine structure constant, is accurate to a one part in a billion. To the best of our knowledge, the result given in Table 1 is the most accurate classical evaluation of the electromagnetic contribution to the electron’s anomalous g-factor reported to the present days. We would like to point out that the obtained accuracy holds further for

α = {137.035999206}^{- 1}

[48], with

u_{e} = 2.18821616430 \times 10^{6}

^{- 1}

. The accuracy may be improved with additional refinement of the fine structure constant after the ninth digit behind the decimal point.

4. Summary

With the aid of essential regularization (Section 2.2) of the electromagnetic field potentials and spatial averaging procedure (Section 2.3), elaborated within the formalism of classical electrodynamics, the present paper reports an exact approach quantifying on a microscopic level the electrodynamics of isolated system comprised of non-composite particle of arbitrary rest mass and possessing elementary electric charge. The proposed approach overcomes all singularities arising in the conventional methods of classical electrodynamics thus uncovering in details the physical nature of electromagnetic self-interactions and the occurrence of anomalous magnetic moment in the system. It quantifies the particle’s dynamics exactly through a system of transcendental equations of motion obtained in the absence of any approximations and at the classical limit, see Section 3.1. Essentially, the solutions of these equations give the exact values of the particle’s intrinsic velocity and the electromagnetic contribution to the anomalous g-factor (see Section 3.3). The derived transcendental equations ensure fast computations and provide highly accurate results competitive to the perturbative method of quantum electrodynamics, see the comparison in Table 1.

In general, the proposed approach may be build on and integrated into the mathematical framework of the classical and quantum field theories. In that regard, more contributions to the particle’s magnetic moment may be calculated exactly. With the appropriate gauge fields and regularization one may introduce additional self-interactions and generalize the system of transcendental equations discussed in Section 3.1.

In conclusion, it appears that the present study supports a thesis pointing out that the anomalous magnetic moment may not be a unique feature to the quantum theory and its occurrence may have a solid classical description. Moreover, it may be the case that the perturbative method of quantum field theory is not unique to that feature and in addition there exist an exact method for its calculation. On that account, pushing the boundaries of classical or quantum field theories further to test the possibility for the exact calculation of even higher contributions in the anomalous magnetic moment of non-composite particles is worth a shot. The proposed regularization to the scalar potential and the related self-Coulomb and self-Zeeman interactions, furthermore, may significantly facilitate the optimization of some computational methods in solid state physics making them self-consistent. Especially these methods studying multi-electron systems that fail to address the self-interactions without the consideration of additional corrections.

Author Contributions

Not applicable.

Funding

This work was supported by National Program “Young scientists and postdoctoral researchers-2” of the Ministry of Education and Science, Bulgaria, approved by DCM 206/07.04.2022.

Institutional Review Board Statement

Not applicable.

Informed Consent Statement

Not applicable.

Data Availability Statement

The data generated within this research is included in the paper.

Conflicts of Interest

Not applicable.

References

Heisenberg, W. The self-energy of the electron. In Early Quantum Electrodynamics, 1st ed.; Miller, A.I., Ed.; Cambridge University Press, 1994; pp. 121–128. [Google Scholar] [CrossRef]
Roberts, B.L.; Marciano, W.J. Lepton Dipole Moments; Advanced Series on Directions in High Energy Physics; World Scientific: 2009; Volume 20. [CrossRef]
Keshavarzi, A.; Khaw, K.S.; Yoshioka, T. Muon g-2: A review. Nucl. Phys. B 2022, 975, 115675. [Google Scholar] [CrossRef]
Schwartz, M.D. Quantum Field Theory and the Standard Model. Cambridge University Press, 2013. [Google Scholar] [CrossRef]
Melnikov, K.; Vainshtein, A. Theory of the Muon Anomalous Magnetic Moment; Springer Tracts in Modern Physics; Springer: Berlin; New York, 2006.
Vogel, M. The anomalous magnetic moment of the electron. Contemp. Phys. 2009, 50, 437–452. [Google Scholar] [CrossRef]
Aoyama, T.; Hayakawa, M.; Kinoshita, T.; Nio, M. Tenth-Order QED Contribution to the Electron g-2 and an Improved Value of the Fine Structure Constant. Phys. Rev. Lett. 2012, 109, 111807. [Google Scholar] [CrossRef] [PubMed]
Aoyama, T.; Hayakawa, M.; Kinoshita, T.; Nio, M. Tenth-order electron anomalous magnetic moment: Contribution of diagrams without closed lepton loops. Phys. Rev. D 2015, 91, 033006. [Google Scholar] [CrossRef]
Aoyama, T.; Kinoshita, T.; Nio, M. Theory of the Anomalous Magnetic Moment of the Electron. Atoms 2019, 7, 28. [Google Scholar] [CrossRef]
Lin, F.; Huang, M. Magnetic correction to the anomalous magnetic moment of electrons. Commun. Theor. Phys. 2022, 74, 055202. [Google Scholar] [CrossRef]
Fan, X.; Myers, T.; Sukra, B.; Gabrielse, G. Measurement of the Electron Magnetic Moment. Phys. Rev. Lett. 2023, 130, 071801. [Google Scholar] [CrossRef]
Morte, M.D.; Francis, A.; Gülpers, V.; Herdoíza, G.; Von Hippel, G.; Horch, H.; Jäger, B.; Meyer, H.B.; Nyffeler, A.; Wittig, H. The hadronic vacuum polarization contribution to the muon g-2 from lattice QCD. J. High Energ. Phys. 2017, 2017, 20. [Google Scholar] [CrossRef]
Jegerlehner, F. The Anomalous Magnetic Moment of the Muon; Springer Tracts in Modern Physics; Springer International Publishing: Cham, 2017; Volume 274. [Google Scholar] [CrossRef]
Logashenko, I.B.; Eidelman, S.I. Anomalous magnetic moment of the muon. Phys.-Usp. 2018, 61, 480–510. [Google Scholar] [CrossRef]
Westin, A.; Kamleh, W.; Young, R.; Zanotti, J.; Horsley, R.; Nakamura, Y.; Perlt, H.; Rakow, P.; Schierholz, G.; Stüben, H. Anomalous magnetic moment of the muon with dynamical QCD + QED 2020, 245, 06035. [CrossRef]
Aoyama, T.; Asmussen, N.; Benayoun, M.; Bijnens, J.; Blum, T.; Bruno, M.; Caprini, I.; Carloni Calame, C.; Cé, M.; Colangelo, G.; et al. The anomalous magnetic moment of the muon in the Standard Model. Phys. Rep. 2020, 887, 1–166. [Google Scholar] [CrossRef]
Gérardin, A. The anomalous magnetic moment of the muon: status of lattice QCD calculations. Eur. Phys. J. A 2021, 57, 116. [Google Scholar] [CrossRef] [PubMed]
Leutgeb, J.; Mager, J.; Rebhan, A. Holographic QCD and the muon anomalous magnetic moment. Eur. Phys. J. C 2021, 81, 1008. [Google Scholar] [CrossRef]
Nedelko, S.; Nikolskii, A.; Voronin, V. Soft gluon fields and anomalous magnetic moment of muon. J. Phys. G: Nucl. Part. Phys. 2022, 49, 035003. [Google Scholar] [CrossRef]
Eidelman, S.; Passera, M. Theory of the τ lepton anomalous magnetic moment. Mod. Phys. Lett. A 2007, 22, 159–179. [Google Scholar] [CrossRef]
González-Sprinberg, G.A.; Vidal, J. Tau magnetic moment. J. Phys. Conf. Ser. 2017, 912, 012001. [Google Scholar] [CrossRef]
Özgüven, Y.; Billur, A.A.; İnan, S.C.; Bahar, M.K.; Köksal, M. Search for the anomalous electromagnetic moments of tau lepton through electron–photon scattering at CLIC. Nucl. Phys. B 2017, 923, 475–490. [Google Scholar] [CrossRef]
Chen, X.; Wu, Y. Search for the Electric Dipole Moment and anomalous magnetic moment of the tau lepton at tau factories. J. High Energ. Phys. 2019, 2019, 89. [Google Scholar] [CrossRef]
Arroyo-Ureña, M.A.; Hernández-Tomé, G.; Tavares-Velasco, G. Anomalous magnetic and weak magnetic dipole moments of the τ lepton in the simplest little Higgs model. Eur. Phys. J. C 2017, 77, 227. [Google Scholar] [CrossRef]
Dyndał, M.; Kłusek-Gawenda, M.; Szczurek, A.; Schott, M. Anomalous electromagnetic moments of τ lepton in γγ →τ+τ- reaction in Pb + Pb collisions at the LHC. Phys. Lett. B 2020, 809, 135682. [Google Scholar] [CrossRef]
Tran, H.M.; Kurihara, Y. Tau g-2 at e^-e⁺ colliders with momentum-dependent form factor. Eur. Phys. J. C 2021, 81, 108. [Google Scholar] [CrossRef]
Borges, L.H.C.; Barone, F.A.; Ferrari, A.F. The point-charge self-energy in a nonminimal Lorentz-violating Maxwell electrodynamics. EPL 2018, 122, 31002. [Google Scholar] [CrossRef]
Sebens, C.T. Eliminating Electron Self-repulsion. Found. Phys. 2023, 53, 65. [Google Scholar] [CrossRef]
Fetter, A.L.; Walecka, J.D. Quantum Theory of Many-Particle Systems; Dover Publications: Mineola, NY, 2003. [Google Scholar]
Weinberg, S. The Quantum Theory of Fields. Cambridge University Press, 1995; Volume 1. [Google Scholar] [CrossRef]
Weinberg, S. The Quantum Theory of Fields. Cambridge University Press, 1996; Volume 2. [Google Scholar] [CrossRef]
Desai, B.R. Quantum Mechanics with Basic Field Theory, 1st ed.; Cambridge University Press, 2009. [Google Scholar] [CrossRef]
Collins, J.C. Renormalization: An Introduction to Renormalization, the Renormalization Group and the Operator-Product Expansion, 1st ed.; Cambridge University Press, 2023. [Google Scholar] [CrossRef]
Perdew, J.P.; Zunger, A. Self-interaction correction to density-functional approximations for many-electron systems. Phys. Rev. B 1981, 23, 5048–5079. [Google Scholar] [CrossRef]
Bao, J.L.; Gagliardi, L.; Truhlar, D.G. Self-Interaction Error in Density Functional Theory: An Appraisal. J. Phys. Chem. Lett. 2018, 9, 2353–2358. [Google Scholar] [CrossRef] [PubMed]
Hehl, F.W.; Obukhov, Y.N. Foundations of Classical Electrodynamics: Charge, Flux, and Metric; Birkhäuser Boston: Boston, MA, 2003. [Google Scholar] [CrossRef]
Shanahan, D. A Case for Lorentzian Relativity. Found. Phys. 2014, 44, 349–367. [Google Scholar] [CrossRef]
Griffiths, D.J. Introduction to Electrodynamics, 4th ed.; Cambridge University Press, 2017. [Google Scholar] [CrossRef]
Roussy, T.S.; Caldwell, L.; Wright, T.; Cairncross, W.B.; Shagam, Y.; Ng, K.B.; Schlossberger, N.; Park, S.Y.; Wang, A.; Ye, J.; et al. An improved bound on the electron’s electric dipole moment. Science 2023, 381, 46–50. [Google Scholar] [CrossRef]
Greiner, W. Classical Electrodynamics; Theoretical Physics; Springer: New York, NY, 1998. [Google Scholar] [CrossRef]
Jackson, J.D. From Lorenz to Coulomb and other explicit gauge transformations. Am. J. Phys. 2002, 70, 917–928. [Google Scholar] [CrossRef]
Cohen-Tannoudji, C.; Dupont-Roc, J.; Grynberg, G. Photons and Atoms: Introduction to Quantum Electrodynamics; Physics Textbook; Wiley-VCH: Weinheim, 2004. [Google Scholar]
Rohrlich, F. The self-force and radiation reaction. Am. J. Phys. 2000, 68, 1109–1112. [Google Scholar] [CrossRef]
Gratus, J. Maxwell-Lorentz without self-interactions: conservation of energy and momentum. J. Phys. A Math. Theor. 2022, 55, 065202. [Google Scholar] [CrossRef]
Lonsdale, D.R.; Goerigk, L. The one-electron self-interaction error in 74 density functional approximations: a case study on hydrogenic mono- and dinuclear systems. Phys. Chem. Chem. Phys. 2020, 22, 15805–15830. [Google Scholar] [CrossRef]
Zope, R.R.; Yamamoto, Y.; Diaz, C.M.; Baruah, T.; Peralta, J.E.; Jackson, K.A.; Santra, B.; Perdew, J.P. A step in the direction of resolving the paradox of Perdew-Zunger self-interaction correction. J. Chem. Phys. 2019, 151, 214108. [Google Scholar] [CrossRef] [PubMed]
2018 CODATA Value: fine-structure constant. The NIST Reference on Constants, Units, and Uncertainty. NIST, 2019.
Morel, L.; Yao, Z.; Cladé, P.; Guellati-Khélifa, S. Determination of the fine-structure constant with an accuracy of 81 parts per trillion. Nature 2020, 588, 61–65. [Google Scholar] [CrossRef] [PubMed]

Figure 1. Graphical representations of the regularized electromagnetic scalar potential considered in the present study and the conventional (Coulomb) one depicted for comparison. For the sake of clarity both potentials are normalized by

C^{'}

. The energy of corresponding electromagnetic field as a function of the distance from the origin of particle’s rest frame of reference is depicted in the inset.

C^{'}

. The energy of corresponding electromagnetic field as a function of the distance from the origin of particle’s rest frame of reference is depicted in the inset.

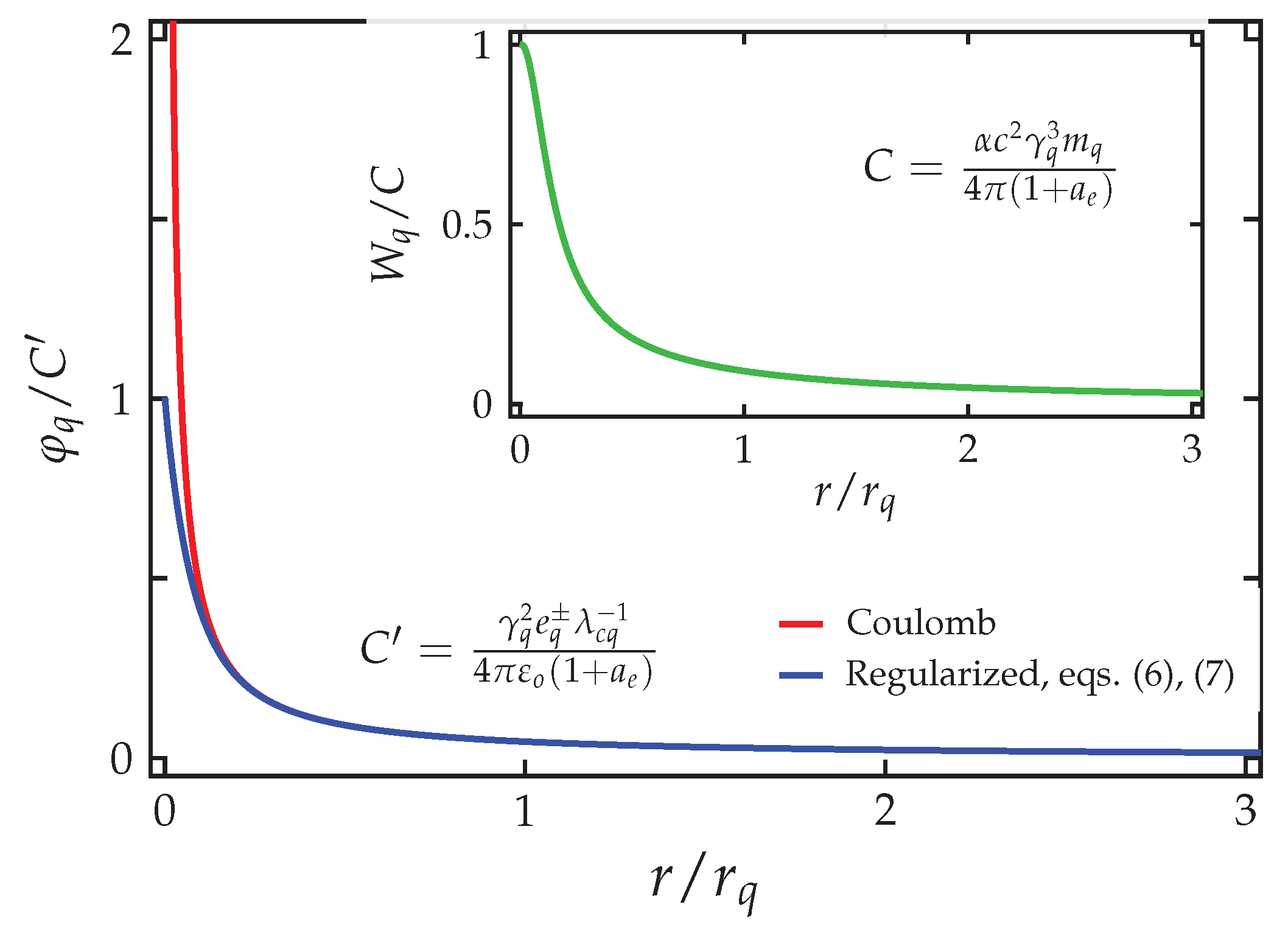
Table 1. Theoretical and experimental (EXP) values of the anomalous g-factor of electromagnetic origin. Second, third and fourth rows show the theoretical results, with prediction of classical electrodynamics (CED) discussed in the present study and some of the recent results based on renormalized quantum electrodynamics (QED). The last row shows the most recent experimental result, with measurements carried out on electrons. The asterisk symbol indicates that the given result is obtained for a value of the fine structure constant taken from NIST [47], see also Section 3.3.

Methods	$a_{e}$	Ref.
Exact CED	$0.001159652 {(23)}^{*}$	(19) & (20)
Perturbative QED	$0.00115965218178 (77)$	[7]
Perturbative QED	$0.001159652181643 (25)$	[8]
EXP	$0.00115965218059 (13)$	[11]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content.

© 2020 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).

Copyright: This open access article is published under a Creative Commons CC BY 4.0 license, which permit the free download, distribution, and reuse, provided that the author and preprint are cited in any reuse.

MDPI Initiatives

Important Links

Choose an area of interest and we will send you notifications of new preprints at your preferred frequency.

Disclaimer

Self-Interactions, Self-Energy and the Electromagnetic Contribution to the Anomalous g-Factor

Abstract

1. Introduction

2. Theoretical Background

2.1. General Considerations

2.2. Field Observables

2.3. Electromagnetic Self-Energy and Self-Interactions

2.4. The Lagrangian and Hamiltonian Density

3. Results

3.1. Equations of Motion

3.2. Effective Mass-Energy Equivalence

3.3. The Anomalous g-Factor

4. Summary

Author Contributions

Funding

Institutional Review Board Statement

Informed Consent Statement

Data Availability Statement

Conflicts of Interest

References

MDPI Initiatives

Important Links

Subscribe