Preprint
Article
Dark Energy and Dark Matter from Extra Dimensional Symmetry as Different Manifestations of Vacuum Energy
Altmetrics
Downloads
383
Views
349
Comments
0
This version is not peer-reviewed
Submitted:
21 September 2024
Posted:
23 September 2024
You are already at the latest version
Alerts
Abstract
Dark energy and dark matter are described as different manifestations of vacuum energy in the cyclic universe framework of Extra Dimensional Symmetry (EDS). Motivated by the cosmological constant problem, EDS doubles large spatial dimensions with microscopic partners of opposite chirality and dimension number (gravitational charge). In this framework, the bulk of vacuum energy, constrained by a Planck density constraint, exists in a gravitationally inert state where actual gravitational constant is zero G. This is in contrast with the gravitationally active state where actual gravitational constant is two Gs with each of these two states corresponding to opposite particle chirality. Dark energy is described as a small repulsive component of vacuum energy constrained to the active state by an asymptotically evolving asymmetry. Dark matter is described as the attractive component of vacuum energy, enabled by neutrino induced gravitational constant which also serves as neutrino mass generation mechanism. While ordinary neutrino interaction with dark energy yields light neutrino mass, chiraly inverted neutrino interaction with dense vacuum energy yields heavy neutrino mass. Transition to sterile neutrino phase can be activated by the gravitational effect of shear stress on the microscopic partners of the visible spatial dimensions. Observational and experimental tests are briefly discussed.
Keywords:
Subject: Physical Sciences - Astronomy and Astrophysics
1. Introduction
Dark energy is a mysterious energy component that has been observed to be driving the accelerated expansion of the universe, and have defied several explanations since its discovery in supernova observations [1]. Its existence has since been confirmed by several independent observations like the Planck measurements of the Cosmic Microwave Background (CMB), indicating that it accounts for about 68.3% of the gravitating energy content of the universe [2]. This is in addition to the older dark matter mystery [3] of a gravitationally attractive but invisible matter component constituting about 26.8% of the gravitating energy content of the universe. Only about 4.6% is in the form of visible baryonic matter.
The cosmological constant (Λ) earlier introduced into Einstein’s Field Equations, which appears to be the simplest solution, results in a serious conflict between measured density of dark energy and the 120 orders of magnitude larger value predicted from quantum field theory [4]. The Λ problem has defied logical solution from supersymmetric cancellation approaches, relaxation of vacuum energy [5] and anthropic approach [6]. It also defies an approach that simply makes the spacetime metric insensitive to Λ [7].
Slowly evolving scalar field models like quintessence [8,9] is one of the many alternative approaches to solve the dark energy mystery without the Λ route. We also have modified gravity models [10] and unification of dark energy and dark matter [11]. See Ref. [12] for detailed review and Refs.[13,14] for recent review.
The amount of theoretical and observational efforts that has been applied to understand dark energy and dark matter indicates that they require new physics beyond the existing standard model of cosmology and particle physics.
Attempts to resolve the dark matter mystery can be mainly classified as either a modification of gravity or introduction of new particles beyond the standard model of particle physics. However, both approaches of modified gravity and particle dark matter tends to fail in providing consistent explanations to the dark matter mystery as each approach tends to succeed in explaining some observations and fail at some others.
There is an approach that explains dark matter as gravitational polarization of vacuum energy by baryonic matter without invoking new particle or modifying gravity in the traditional sense [15]. It is based on the idea that matter and antimatter have opposite gravitational charges which requires a violation of the Weak Equivalence Principle. Preliminary findings from measurements of antiproton to proton charge to mass ratio imply that matter and antimatter gravitate the same way [16]. Furthermore, the recent result from the ALPHA collaboration has ruled out the possibility of gravitationally repulsive antimatter [17].
In this paper, the dark energy and dark matter puzzle are approached using the new framework of Extra Dimension Symmetry (EDS) that doubles the number of large extra dimensions with microscopic partners of opposite handedness. EDS provides a possible solution to the dark energy puzzle by placing vacuum energy (constrained by a Planck energy density constraint) in a gravitationally inert state where the actual gravitational constant is zero G. Due to a speed limit asymmetry between the two states and given the energy density constraint, a nonzero component of vacuum energy is constrained to the gravitationally active state, manifesting as dark energy. For dark matter, it relies on the background effect of neutrinos which induces nonzero gravitational constant in the inert state, enabling the gravitation of some dense component of vacuum energy which appears as dark matter and neutrino mass generation mechanism. See Ref [18] for a review on neutrino mass which is still an open question.
While neutrino interaction with dark energy produces light neutrino mass, chiraly inverted neutrino interaction with the bulk of vacuum energy produces heavy neutrino mass which manifests as cold dark matter. Transition to the heavy state can be enabled by the gravitational expansion of microscopic dimensions by shear stress or gravitational disturbance from sudden disappearance of negative pressure. This neutrino substrate approach for dark matter doesn’t require the polarization of the quantum vacuum or opposite gravitational charges for particles and antiparticles like in [15].
This paper is organized as follows. Section 2 discusses the key dimensional symmetry in which spatial dimensions are grouped as a set of dimension(s) with dimensional partners having opposite dimension numbers (gravitational charge) that determines either a positive or negative response to the stress energy tensor. It also discusses the selective response of the extra dimensions to the components of the stress energy tensor. It further discusses the speed constraint, the two gravitationally inert and active states, their speed limit asymmetry and density constraint, which is then applied in section 4 to provide a possible solution to the Λ problem of dark energy. Section 3 discusses General Relativity in two set of these extra dimensions which determines the dynamics of expansion and contraction as well as chiral transition of neutrinos. Section 4 discusses the emergence of an asymptotically evolving Λ dark energy due to the speed limit asymmetry and energy density constraint discussed in section 2. It also briefly discusses dynamics of the resulting dark energy and the cyclic universe scenario that results from the reversal of a dimensionality parameter. Section 5 discusses the neutrino induced gravitation of virtual particles which appears as dark matter, neutrino mass and chiral transition. Section 6 discusses observational and experimental probe of this form of dark matter. Discussion and conclusion follows in Section 7.
2. Extra Dimensional Symmetry Framework
In this framework, the spatial dimensions are grouped, with the number of large spatial dimensions described by the dimension number (analogous to baryon number) with a dimensionality parameter d that is an indication of handedness that can either be positive or negative (right handed or left handed) such that
where and with (right handed) in the present universe, a dimension with corresponds to which is a 1D time like spatial dimension that is invisible due to a speed constraint consistent with special relativity discussed later in Section 2.2. Dimensions with corresponds to which is the visible 3D set of spatial dimensions. Ref [19] discusses right handedness of space time although in the context of the geometry of the Euclidean version of twistor space in understanding the symmetries of the standard model.
Gravitational interaction in is not diluted by a large as they are compartmentalized partly by their opposite dimension number and the entropic nature of . While the positive dimension number (gravitational charge) of denotes positive response to the components of the stress energy tensor where mass energy is seen as positive and therefore attractive, the negative dimension number of denotes a negative response where mass energy appears negative and therefore repulsive.
The EDS framework further doubles these large set of right handed spatial dimensions ( and ) with left handed microscopic partners that have opposite dimension numbers where , such that the total dimension number of the universe can be zero as illustrated in Table 1. While the dimensional partner of , is a Planck size dimension, the dimensional partner of is denoted and the size of one of the component dimensions is constrained to 137 Planck units which determines the value of the fine structure constant. The and dimensions ( and as well as their left handed partners and respectively) appears to be stable and gravitationally active except for the inert dimension as discussed later in section 2.1.
The opposite dimension numbers of right handed spatial and left handed spatial dimensions implies the inversion of the sign of the stress energy tensor in which expansion becomes contraction while contraction becomes expansion. As a result of this, the expansion of the large spatial dimensions and in the early universe is equivalent to the gravitational collapse of their dimensional partners. This is partly enabled by the selective response to the stress energy tensor discussed later in section 2.1.
In this attempt to resolve the mysteries of dark energy and dark matter, the focus is on the large spatial dimensions and , their microscopic partners and illustrated in Figure 1 and how they interact with one another.
2.1. Selective Response of Extra Dimensions to Components of the Stress Energy Tensor
As part of the symmetries in the EDS framework, every gravitational interaction in the visible spatial dimensions is mirrored in either of the extra spatial dimensions with negative dimension numbers such as and . The dimension is presently inert since has the same dimension number with the visible dimensions.
The dimension is unable to respond to shear stress and negative pressure due to its one spatial dimensionality (shear stress requires at least two spatial dimensions) and its entropic nature. The size of associated with every cubic Planck volume of 3D space is a measure of its entropy such that, in Planck unit. It expands in response to energy density and positive pressure when , such as in the present universe, while the dimension only responds to shear stress and negative pressure. The responses of and to components of the stress energy tensor can serve as potential means of probing them alongside dark energy and dark matter which will be discussed later.
2.2. Speed Constraint and Invisibility of S0 Dimension
Despite its cosmic size, the dimension is invisible due to its time like behavior from the speed constraint in the EDS framework. Consistent with Special Relativity, the speed constraint requires that a particle’s velocity must always equal the maximum speed limit c in the – dimensions.
Massless particles like photons have zero velocity component along , while massive particles and antiparticles travel in opposite directions along as illustrated in Figure 2. This motion along can be quantitatively equivalent to the passage of time such that,
where µ is particle velocity along , and ν is particle velocity along visible spatial dimensions .
When for massive particles, and conversely, when ν , for massless particles, , to satisfy the speed constraint. This suggests that time can be an emergent temporal dimension driven by the velocity of particles along dimension. How fast time appears to pass for a massive particle can be equivalent to the ratio of its component of velocity to the speed limit c as,
where is the equivalent Lorentz factor for the dimension. Since ,
2.3. Speed Limit Asymmetry
The asymmetry in speed limits c and is described by the asymmetry parameter which is the ratio of the Planck size of to the size of dimension, illustrated in Figure 2. such that,
where () is the size of in Planck unit which expands with the gravitational contraction of as explained later in section 3, which implies a slightly larger value of in deep gravitational potential wells and hence smaller value of .
The asymmetry between the two speed limits and , as shown in Figure 2 can be expressed as,
2.4. Gravitational State and Chirality Oscillation
In the EDS framework, the opposite speed limit states discussed in the previous subsection as illustrated in Figure 2, are also opposite gravitational on and off states and further corresponds to opposite chirality. Thus, for a Higgs interacting massive particle undergoing chirality oscillation, it also oscillates between the two G and zero G states with slightly different speed limits c and respectively. They still however experience an average gravitational constant value of 1G.
2.5. Planck Energy Density Constraint
The Planck energy density constraint essentially constrains the total energy density in a given volume of spacetime to always equal the upper limit of the Planck density. This density constraint is analogous to the speed constraint in section 2.2 and can be expressed as,
where is the vacuum energy density, and is the total baryonic matter density.
However, Planck density is only obtainable in the gravitational active state where the speed limit is c while the bulk of the vacuum energy component exists in the inert state with lower speed limit and hence lower Planck density The key significance of this, is in the emergence of non zero Λ dark energy in section 4. However, such energy density constraint implies a Planck density limit to the density of black holes like that suggested in [20], where Planck stars replace black hole singularities.
3. General Relativity in
andDimensions
3.1. General Relativity in S0 Dimension
In 1+1 dimensionality the curvature term in Einstein Field Equations is zero [21]. In dimension where the dimension number is negative, it can be expressed as,
where is the equivalent cosmological constant in S0 dimension, is the metric tensor and is the stress energy tensor. The negative dimension number of the dimension which inverts the sign of the stress energy tensor, confers on it some unique features such as:
- i.
- 1
- i.
- ii. 2
Positive energy density in is equivalent to negative energy density everywhere in.
An energy density associated with a given Planck volume of 3d space appears as an equivalent negative energy density everywhere along the corresponding dimension as illustrated in Figure 3.
Positive pressure in is equivalent to negative pressure in Any form of positive pressure in appears as negative pressure in for the same reason of negative dimensionality. The result is a positive cosmological constant expansion of that is equivalent to the curvature of () as illustrated in Figure 4, such that,
3.2. General Relativity in
Dimensions
In 3D dimensions, the Einstein tensor is not zero unlike in 1D dimension. In addition, only responds to shear stress and negative pressure due to selective response to the stress energy tensor as discussed in section 2.1. The result is that the positive curvature effect () of shear stress on dimensions is equivalent to the negative curvature effect () of shear stress on the dimensions. Therefore,
Furthermore, the positive cosmological constant () expansion of due to negative pressure, is equivalent to the negative cosmological constant () contraction of .
The gravitational inversion of - dimensions as illustrated in Figure 5 results in the negative pressure driven collapse of until all its component dimensions have completely collapsed to their minimum length scale.
4. Emergence of Asymptotically Evolving Cosmological Constant
The asymmetry in speed limit described in Equation (6) between the two gravitational states also reflects in the values of their Planck densities such that (Since ),
Where is the vacuum energy density and equals the lower Planck density in state. is the Planck density in the state. is the asymmetry parameter that asymptotically approaches zero with the gravity driven growth of as discussed in the previous section.
In the presence of Baryons with energy density , Eq. (12) can be expressed as,
Since the gravitationally inert (lower speed) state is unable to contain the total vacuum energy components, the small component in Eq. (13) is therefore constrained to the gravitationally active state as a cosmological constant dark energy with an equation of state parameter as illustrated in Figure 6. That is,
4.1. Dynamics of
Dark Energy in a Cyclic Universe
Despite maintaining a constant equation of state, this form of dark energy varies spatially according to the suppression of by gravitational potential wells (Eq. (5) and Figure (3)). The deeper the gravitational well the more the suppression and this can show up in the precision measurement of the Integrated Sachs Wolf effect. In addition, the density of this form of dark energy also tracks the gravity driven growth of (as discussed in section 3.1) on cosmological time scale. A smaller in the early universe can result in with capable of driving an inflationary universe before asymptotically falling close to its current value. The recent result from the DESI collaboration indicates a possible evolution of dark energy density [22].
Due to the gravitational symmetry in this framework, the positive expansion of the visible spatial dimensions is equivalent to the negative contraction of until all its 3D components completely collapse to their characteristic minimum length scales.
The possible chiral oscillation of the dimensionality parameter in Eq. (1) on a cosmological time scale can result in a reverse cycle of the universe in which dimension number signs of the dimensions are reversed as well as their various responses to components of the stress energy tensor. In this scenario, in which the visible spatial dimensions illustrated in Table 1 as right handed, become left handed and their partners become right handed, the dark energy equation of state still remains constant. Rather, it is the response of the visible spatial dimensions to positive energy density that becomes negative while its response to negative pressure becomes negative as well.
The result is that baryonic matter and dark matter becomes repulsive with black holes becoming white holes until complete evaporation, while dark energy becomes attractive, driving the collapse of the visible universe while expanding the dimensions. Associated with an expanding , is the fall in the value of the fine structure constant since it is coupled to the size of one of its 3 dimensions in this framework. The corresponding contraction of increases the density of dark energy in reverse towards the Planck density scale, while inert dimension remains at the Planck scale, neither expanding nor contracting. This results in an accelerated collapse of the visible right handed spatial dimensions with the inflation of the left handed dimensions in a cyclic scenario which gets reversed with chiral oscillation of the dimensionality parameter on cosmological time scale.
Since the size of is a measure of entropy, its contraction when the dimensionality parameter is negative implies reduction in entropy of the universe. This further implies possible loss of entropy to the Bulk in a Multiverse scenario.
5. Dark Matter from Neutrino Enabled Gravitation of Virtual Particles
In the EDS framework, the existence of most of the vacuum energy component in the gravitationally inert state ensures that virtual particles in such state are gravitationally inert. However, neutrinos can induce small component of the gravitational constant in this inert state. It enables virtual particles within a spatial volume determined by the size of neutrinos, to gravitate with positive pressure and appear as dark matter.
In the absence of neutrino coupling to the Higgs field, the mass generation mechanism for neutrinos in EDS, is this gravitational coupling to vacuum energy components either as dark energy in the active state or the bulk of vacuum energy in the inert state through the induced gravitational constant component. This neutrino induced gravitational constant is restricted to the internal structure of neutrinos without a radiative term and can be expressed as,
where is the dimensionless phase parameter. It oscillates such that . is a dimensionless constant with a bound and may actually range from 0.1 to 0.9 for each of the neutrino flavours. is a dimensionless energy scale parameter. It is the ratio of neutrino energy to Planck energy such that .
where is neutrino energy and is Planck energy.
5.1. Neutrino Mass Generation Mechanism
When neutrinos are in the gravitationally active state as left handed neutrinos and right handed antineutrinos, they interact with dark energy through their induced gravitational constant component from Eq. 15 such that,
where is the resulting neutrino gravitational parameter, is dark energy density and is the spatial volume under the influence of the neutrino gravitational constant component.
The generated mass from ordinary neutrino interaction with dark energy can therefore be expressed as,
It is therefore expected that maximum light neutrino masses obtainable when , should coincide and track the mass scale of dark energy at about 10-3 eV. Indeed an attempt was made in [23] to justify a possible coincidence between the mass scale of dark energy to what we expect of the neutrino mass. However, since light neutrino mass in this framework is coupled to dark energy, the maximum mass should be slightly lower in deep gravitational potential wells which also suppress dark energy density as discussed in Section 4.1.
When light neutrinos chiraly transition to right handed neutrinos and left handed antineutrinos, they interact with the bulk of vacuum energy in the otherwise gravitationally inert state. The mass of the resulting sterile neutrinos becomes increasingly heavy with the oscillation of and begins to behave as cold and ultra cold dark matter since Eq. (18) becomes,
where is the mass of sterile neutrinos and is the bulk of vacuum energy density which has a mass scale of 30 orders of magnitude greater than that of dark energy. It is therefore expected that the maximum mass scale of sterile neutrinos should be up to 1030 times greater than that for ordinary light neutrinos.
The value of for the various neutrino flavours in Eq. (15) and by extension, Eq. (18) and Eq. (19) determines the neutrino mass hierarchy. For experimental determination of neutrino mass hierarchy, see Ref [24]
5.2. Neutrino Chiral transition
In this framework neutrino chiral oscillation depends on rotation with respect to the dimensions which have collapsed to microscopic scales below the required transition threshold under the influence of negative pressure such as that from dark energy as discussed in section 3. However the gravitation effect shear stress expands these dimensions as discussed in the same section 3. Once neutrinos pass through regions of spacetime with shear stress density (from baryons) above a certain threshold, dimensions can expand beyond the transition threshold, allowing light neutrino chiral transition to heavy neutrinos which manifests as cold dark matter. The required transition threshold can also be achieved with high frequency gravitational oscillation in that can be caused by sudden disappearance of high negative pressure.
6. Observational and Experimental Probe of Sterile Neutrino Dependent Dark Matter
6.1. Probe of Insensitivity to Baryonic Gravitational Potential Wells
Since sterile neutrinos and the associated cold dark matter exists in the zero G state in this framework, they should be insensitive to baryonic gravitational potential wells which exists in the two G state. However, baryonic matter and radiation can respond and there fore, fall into the gravitational potential well of cold dark matter and this should be observable in structure formation, gravitational lensing and even more discernible in the bullet cluster collision data [25].
6.2. Hierarchical Cold Dark Matter Halo Formation
When light neutrinos pass through shear stress generating baryonic structures such as planets and stars, their probability of chiral transition increases with the magnitude of shear stress and duration in such shallow gravitational potential. When chiral transition to sterile neutrinos eventualy occure, the phase parameter oscillates from zero to one over a transition period in the neutrino refrence frame. The transition distance therefore, will depend on relativistic time dilation and gravitational time dilation, resulting in a spectrum of transion distances that may range from a few kilometers for non relativistic cosmic neutrinos to several light years for ultra-relativistic neutrinos. While low energy neutrinos with short transition distances should have relatively short sterile life time, medium and high energy neutrinos, when they transition can have relativley long sterile life time on cosmological timescale.
The result is the formation of a herarchical halo profile around stress generating baryonic structures reflecting the spectrum of transition distances of the various neutrino energies and the stress profile of the baryons. It is also possible that diferent neutrino flavours have different transition period which can cause sterile neutrino flavour dispersion and contribute to the halo profile of this form of dark matter. See Ref [26] on Navaro-Frenk-White hierarchical halo profile.
6.3. Shear Activation and Indirect Detection of Sterile Cosmic Neutrinos
Since cosmic neutrinos have become non relativistic in the present universe their chiral transition probability should be relatively higher and should have shorter transition distance as justified in Section 5.4. For instance hundreds of meters of shear battery made of cells of tough alloys under shear stress of up to 2.4 Gpa (Maraging steel) can be installed vertically underground. Upward travelling cosmic neutrinos passing through such shear battery can chiraly transition to sterile neutrinos by the time they arrive the Earth surface. These sterile cosmic neutrinos can manifest as gravitational anomalies in the measurement of the gravitational constant.
6.4. Probe of Earth Quake Light from Possible Dent in the Fine Structure Constant
As earlier mentioned in section 2, the value of the fine structure constant is determined by the size (137 Planck units) of one of the component dimensions of. Beyond a certain threshold in strength of gravity from shear stress, it is possible that the dimensions can expand to the extent of causing a dent in the value of . It conjectured here that there is a possible coupling of sterile neutrinos to such dent in , making some sterile neutrinos observable in this regard.
There is a possibility that non relativistic cosmic neutrinos, on passing through regions of high tectonic stress, transition to sterile neutrinos that may show up above the surface not just as anomalies in measurements of the gravitational constant but as dent in . Spatial variations in have been reported in [27]. Such dent in can be observed as slight drop in atomic energy levels and possible glow resulting from energy level transitions in atoms within the dent. Spectral analysis of Earth Quake Lights [28,29] can be of potential interest in this regard.
There is also the possibility of relativistic MeV neutrinos such as solar neutrinos and Geoneutrinos, transitioning over hundreds of thousands of kilometers forming halos of dark matter around the planet which can be the target of future probes.
6.5. Measurement of the Gravitational Constant during Solar Eclipse
There is the possibility of sterile transition of solar neutrinos after passing through regions of shear stress in the lunar interior, mostly from tidal forces and possible moon quakes which are usually stronger during solar eclipse. The resulting sterile neutrinos from coincident lunar quake and solar eclipse event on reaching Earth can be observed as anomalies in measurements of the gravitational constant. Moreover there have been various reports of anomalies in measurements of the gravitational constant during some solar eclipse events [30,31]. This is in addition to other reports of anomalies in measurements of the gravitational constant such as in [32] that appears to be correlated with length of day variation. Neutrino detectors can also be used to look out for expected deficit in the detection rate of light neutrino flavours under the moon’s shadow during solar eclipse.
7. Discussion and Conclusions
EDS as a symmetry framework of spatial dimensions, doubles large spatial dimensions with microscopic partners of opposite handedness and dimension number which inverts the sign of gravitational interactions in these extra dimensions. It provides a framework for the possible resolution of the mysteries of dark energy, dark matter and neutrino mass. In doing so, it provides some potential insights into the dimensional structure of spacetime, gravity and the dynamics of a cyclic universe. It also implies that time could be an emergent property of a large extra spatial dimension with negative dimension number and size that is a measure of entropy.
Specifically, it places the bare vacuum energy component in a state where the gravitational field is switched off while real massive standard model particles chiraly oscillate between this gravitationally inert state and the active state.
Due to a Planck energy density constraint and a speed limit asymmetry described by the asymptotically evolving asymmetry parameter, a small component of vacuum energy is constrained to exist in the gravitationally active state and appear as dark energy. The density of this form of dark energy with a constant equation of state parameter, asymptotically evolves towards zero with the gravity driven growth of dimension as explained in section 3. However, the chiral oscillation of the dimensionality parameter from Eq. (1) on a cosmological timescale can still result in a cyclic universe scenario as discussed in section 4.1.
Furthermore, EDS describes dark matter as resulting from neutrino enabled gravitation of virtual particles, in which neutrinos induce small component of the gravitational constant in the gravitationally inert state. The induced gravitational constant serves as a mass generation mechanism for neutrinos through this gravitational coupling to vacuum energy. While light neutrino mass arises from its interaction with dark energy heavy sterile neutrino mass arises from its interaction with the bulk of vacuum energy. However, chiral transition to sterile neutrino phase is suppressed by negative pressure driven collapse of the dimension, but can be activated with the extra degree of freedom from shear stress driven expansion of .
Due to various transition distances which depends on time dilations, the resulting sterile neutrinos and cold dark matter readily form hierarchical halos, exhibiting hybrid particle and modified gravity behaviour like the gravitational polarization of vacuum energy approach [15] and superfluid dark matter [33]. Experimental and observational probes for this sterile neutrino dependent form of cold dark matter were briefly discussed in section 6.
Expected results from the trio of the JWST, Euclid and upcoming Nancy Grace Roman Telescope and Vera Rubin Observatory, are expected to provide precision measurements of dark energy density as well as the dynamics of dark matter. Such precision measurements should glean out the predicted suppression of dark energy density in the deep gravitational potential wells of baryonic matter.
EDS also provides a potential insight on baryon asymmetry which may arise if the universe has a net spin with respect to the dimension in a Multiverse scenario. Since spin in opposite directions of as illustrated in Figure 2 represents particle and antiparticle states, a net spin may result in baryon asymmetry particularly in the early universe. Such form of baryon asymmetry would have reduced in the present universe by a factor of with the expansion of the dimension.
In conclusion, the EDS framework offers new physics explanations for dark energy and dark matter as different manifestations of vacuum energy. While dark energy is the small repulsive component of vacuum energy in the active state, dark matter is simply virtual particles in the inert state that are enabled to gravitationally attract with neutrino induced gravitational constant and also serves as mass generation mechanism for neutrinos. The predicted dynamics of dark energy as well as the shear stress activation of sterile neutrinos provides observational and experiment probes with potential insights into a number of other unsolved problems.
Data availability
No data was used for the research described in the article.
Declaration of competing interest
The authors declare that they have no known competing financial interests or personal relationships that could have appeared to influence the work reported in this paper.
Author Contributions
Kabir Adinoyi Umar: Conceptualization, Investigation, Writing-original draft, Writing-review and editing, Resources.
Acknowledgments
The author gratefully acknowledges Benjamin G. Ayatunji for his criticisms, encouragement and mentorship. He is very grateful to S. X. K. Howusu for his inspiring advice and initial motivation for this work. The author is immensely grateful to Aminu M. Musa, and Buhari M. Abubakar.
References
- Riess, A.G.; Filippenko, A.V.; Challis, P.; Clocchiatti, A.; Diercks, A.; Garnavich, P.M.; Gilliland, R.L.; Hogan, C.J.; Jha, S.; Kirshner, R.P.; et al. Observational Evidence from Supernovae for an Accelerating Universe and a Cosmological Constant. Astron. J. 1998, 116, 1009–1038. [Google Scholar] [CrossRef]
- Ade P. A., R.; et al. Planck 2013 results. XVI. cosmological parameters, (2013). arXiv:1303.5076[astro-ph.CO].
- Bertone, G.; Hooper, D. History of dark matter. Rev. Mod. Phys. 2018, 90, 045002. [Google Scholar] [CrossRef]
- Carroll, S. M. , The cosmological constant, Living Rev. Rel. 4:1, (2001), arXiv:astro-ph/0004075.
- Kachru, S.; et al. , de Sitter vacua in string theory, Phys. Rev. D 68 (2003) 046005, arXiv:hep-th/0301240.
- Banks, T.; Dine, M.; Motl, L. On anthropic solutions of the cosmological constant problem. J. High Energy Phys. 2001, 2001, 031–031. [Google Scholar] [CrossRef]
- Arkani-Hamid, N. , A small cosmological constant from a large extra dimension, Phys. Lett. B480 (2000) 193-199, arXiv:hep-th/000197.
- Caroll, S. M. , Quintessence and the rest of the world: suppressing long-range interactions, Phys. Rev. Lett. 81 (1998) 3067. [CrossRef]
- Ratra, B. and Peebles, J., Cosmological consequences of a rolling homogenous scalar field, Phys. Rev. D 37 (1988) 321.
- Tsujikawa, S. , Modified gravity models of dark energy, Lec. Notes Phys. 800 (2010) 99-145.
- Bilic, N. , Tuper, G. B., Viollier, R. D., Unification of dark matter and dark energy: The inhomogenouschaplygin gas, Phys. Lett. B 535 (2002) 17-21.
- Copeland, E. J.; et al. , Dynamics of dark energy, Int. J. Mod. Phys. D15 (2006) 1753-1936, arXiv:hep-th/0603057.
- Oks, E. Brief review of recent advances in understanding dark matter and dark energy. New Astron. Rev. 2021, 93, 101632. [Google Scholar] [CrossRef]
- Arun, K.; et al. Dark matter, dark energy and alternate models: a review, Advances in Space Research, 60, (2017) 166-186. arXiv:1704.06155[physics.gen-ph].
- Hajdukovic D., S. , Quantum vacuum and dark matter, Astrophysics and Space Science 337 (2012) 9-14.
- Borchert M., J. , Devlin J. A., Ulmer S., et al., A 16 parts per trillion measurement of the antiproton to proton charge-mass ratio, Nature 601 (2022) 53-57.
- Anderson, E.K.; Baker, C.J.; Bertsche, W.; Bhatt, N.M.; Bonomi, G.; Capra, A.; Carli, I.; Cesar, C.L.; Charlton, M.; Christensen, A.; et al. Observation of the effect of gravity on the motion of antimatter. Nature 2023, 621, 716–722. [Google Scholar] [CrossRef] [PubMed]
- Dev, A. Neutrino oscillation and mass models. 1768; arXiv:2310.17685v1[hep-ph]. [Google Scholar]
- Woit, P. Spacetime is right-handed. 0060; arXiv:2311.00608v2[hep-th]. [Google Scholar]
- Rovelli, C. , Vidoto, F. Planck Stars, Int. J. Mod. Phys. D 23 (2014) 1442026. arXiv:1401.6562, 1442026.
- Boozer, A.D. General relativity in (1 + 1) dimensions. Eur. J. Phys. 2008, 29, 319–333. [Google Scholar] [CrossRef]
- Adame, A. G.; : Cosmological constraints from the measurements of Baryon Acoustic Oscillations, (2024); et al. arXiv:2404.03002 [astro-ph.CO].
- Bass, S.D.; Krzysiak, J. Vacuum energy with mass generation and Higgs bosons. Phys. Lett. B 2020, 803, 135351. [Google Scholar] [CrossRef]
- Ciuffoli, E. , Evslin, J. and Zhang, X., Confidence on neutrino mass hierarchy determinatination, JHEP 01 (2014) 095.
- Clowe, D.; Bradač, M.; Gonzalez, A.H.; Markevitch, M.; Randall, S.W.; Jones, C.; Zaritsky, D. A Direct Empirical Proof of the Existence of Dark Matter. Astrophys. J. 2006, 648, L109–L113. [Google Scholar] [CrossRef]
- Navarro, J.F.; Frenk, C.S.; White, S.D.M. A Universal Density Profile from Hierarchical Clustering. Astrophys. J. 1997, 490, 493–508. [Google Scholar] [CrossRef]
- Webb, J.K.; King, J.A.; Murphy, M.T.; Flambaum, V.V.; Carswell, R.F.; Bainbridge, M.B. Indications of a Spatial Variation of the Fine Structure Constant. Phys. Rev. Lett. 2011, 107, 191101. [Google Scholar] [CrossRef] [PubMed]
- Thériault, R.; St-Laurent, F.; Freund, F.T.; Derr, J.S. Prevalence of Earthquake Lights Associated with Rift Environments. Seism. Res. Lett. 2014, 85, 159–178. [Google Scholar] [CrossRef]
- Derr, J. , Earth quake lights: A review of observations and present theories. Bull Seismol. Soc. Am. 63 1973. [Google Scholar]
- Wang, Q. , Yang, X. and Tang, K., Gravity anomaly from Mohe total eclipse, Chin. Sci. Bull. 46 (2001) 1833-1836.
- Wang, Q.-S.; Yang, X.-S.; Wu, C.-Z.; Guo, H.-G.; Liu, H.-C.; Hua, C.-C. Precise measurement of gravity variations during a total solar eclipse. Phys. Rev. D 2000, 62. [Google Scholar] [CrossRef]
- Anderson, J.D.; Schubert, G.; Trimble, V.; Feldman, M.R. Measurements of Newton's gravitational constant and the length of day. EPL (Europhysics Lett. 2015, 110. [Google Scholar] [CrossRef]
- Hossenfelder, S.; Mistele, T. The Milky Way’s rotation curve with superfluid dark matter. Mon. Not. R. Astron. Soc. 2020, 498, 3484–3491. [Google Scholar] [CrossRef]
Figure 1.
Large spatial dimensions and and their microscopic dimensional partners and . The opposite dimension numbers which inverts the sign of the stress energy tensor implies that and collapsed toward their minimum microscopic sizes during the inflation of and in the early universe.
Figure 1.
Large spatial dimensions and and their microscopic dimensional partners and . The opposite dimension numbers which inverts the sign of the stress energy tensor implies that and collapsed toward their minimum microscopic sizes during the inflation of and in the early universe.

Figure 2.
Ring structure of - dimensions with two slightly unequal speed limit states C and C0 (which are of opposite chirality) at the two ends of dimension that can be seen as two brane surfaces. Traveling in opposite directions of represents particle and antiparticle states.
Figure 2.
Ring structure of - dimensions with two slightly unequal speed limit states C and C0 (which are of opposite chirality) at the two ends of dimension that can be seen as two brane surfaces. Traveling in opposite directions of represents particle and antiparticle states.

Figure 3.
A gravitational well in is inverted into a gravitational hill with the expansion of by the gravitational interaction of a test particle. The test particle is replicated about 1060 times everywhere along dimension.
Figure 3.
A gravitational well in is inverted into a gravitational hill with the expansion of by the gravitational interaction of a test particle. The test particle is replicated about 1060 times everywhere along dimension.
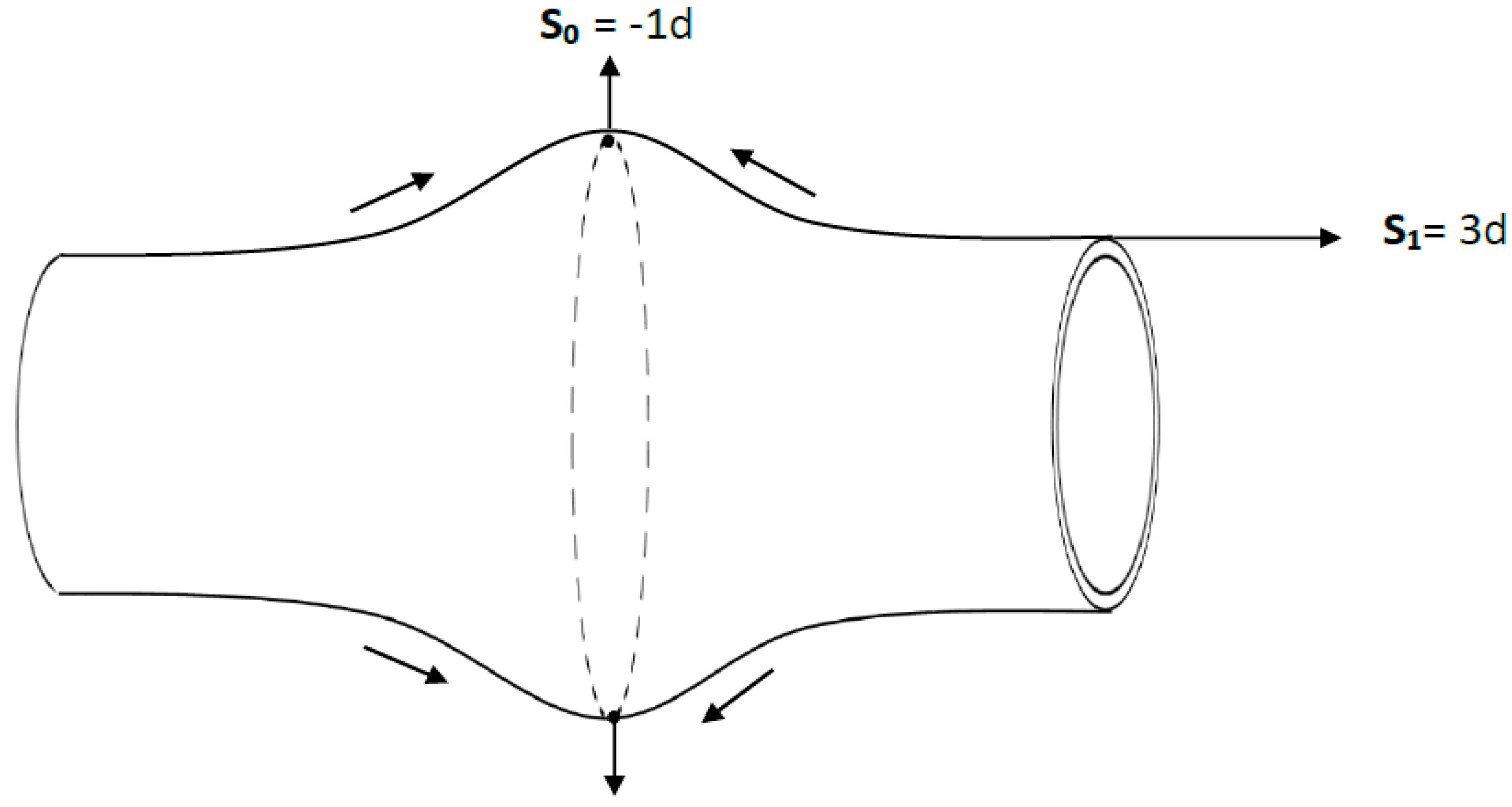
Figure 4.
Gravitational inversion in S1-S0 dimensions. A positive cosmological constant expansion of S0 is equivalent to the curvature of S1 in the present universe with a positive dimensionality parameter. This is reversed in a cyclic universe when dimensionality parameter becomes negative.
Figure 4.
Gravitational inversion in S1-S0 dimensions. A positive cosmological constant expansion of S0 is equivalent to the curvature of S1 in the present universe with a positive dimensionality parameter. This is reversed in a cyclic universe when dimensionality parameter becomes negative.

Figure 5.
Gravitational inversion in - dimensions. Positive curvature of resulting from shear stress, is equivalent to the negative curvature of due to the same shear stress component. Also, a positive cosmological constant expansion of is equivalent to a negative cosmological constant contraction of .
Figure 5.
Gravitational inversion in - dimensions. Positive curvature of resulting from shear stress, is equivalent to the negative curvature of due to the same shear stress component. Also, a positive cosmological constant expansion of is equivalent to a negative cosmological constant contraction of .

Figure 6.
While the bulk of the vacuum energy components occupy the gravitationally inert state to its limit, there has to be a component in the gravitationally active state to satisfy the energy density constraint. The lower Planck density is associated with the lower speed limit state .
Figure 6.
While the bulk of the vacuum energy components occupy the gravitationally inert state to its limit, there has to be a component in the gravitationally active state to satisfy the energy density constraint. The lower Planck density is associated with the lower speed limit state .

Table 1.
Periodic table of spatial dimensions indicating dimension number symmetry between the sets of right handed spatial and left handed spatial dimensions within the EDS framework. With positive dimensionality parameter d, the right handed spatial dimensions expanded in the early universe while their left handed partners of opposite dimensionality parameter, collapsed to their minimum length scales.
Table 1.
Periodic table of spatial dimensions indicating dimension number symmetry between the sets of right handed spatial and left handed spatial dimensions within the EDS framework. With positive dimensionality parameter d, the right handed spatial dimensions expanded in the early universe while their left handed partners of opposite dimensionality parameter, collapsed to their minimum length scales.
|
Microscopic Spatial dimensions (Left Handed) |
Large Spatial dimensions (Right Handed) |
|
| n | ||
| 1 | ||
| 0 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Copyright: This open access article is published under a Creative Commons CC BY 4.0 license, which permit the free download, distribution, and reuse, provided that the author and preprint are cited in any reuse.
MDPI Initiatives
Important Links
© 2024 MDPI (Basel, Switzerland) unless otherwise stated






