New Types Of M-fractional Wave Solitons To The Mathematical Physics Model By Three Distinct Techniques

1. Introduction

Soliton theory based on water waves, plasmas, optical fibers etc., was developed in 1960-1970. This is significant branch of applied mathematics as well as mathematical physics. It has significant uses in non-linear optics, fluid mechanics, plasmas etc. This theory is applied in various natural sciences, including telecommunication, biology, chemistry, mathematics and many fields of physics including fluid dynamics, condensed matter, plasma physics etc. Distinct kinds of naturally occurring phenomenon are shown as a non-linear fractional partial differential equations (NLFPDEs). Distinct schemes are made to attain exact results of non-linear partial differential equations i.e modified direct algebraic technique [1], modified Khater scheme [2], Kudryashov technique [3], novel $(G^{\prime }/G)$-expansion method [4], extended mapping scheme [5].

In our study we use three simple, useful and significant methods named as modified simplest equation method, Sardar sub-equation method and generalized Kudryashov method. There are various uses of these methods. Instantly; some solitary wave solutions of BBM and Chan-Hilliard equations by utilizing modified simplest equation method [6], exact solitons of Boussinesq and coupled Boussinesq equations have been attained by using this technique in [7]. Some solitons solutions of perturbed Fokas-lenells model have been obtained with the help of Sardar sub-equation technique [8], new kind of solitons of (2+1)-dimensional Sawada-Kotera (SK) model have been gained by this method [9], some exact wave solitons of new Hamiltonian Ampltude equation have been attained [10], the dark, bright and singular optical solitons of higher order non-linear Schrödinger have been gained by utilizing Sardar sub-equation scheme [11], the singular, bright, dark, periodic singular, combined solitons and other solutions of strain wave model have been achieved with the use of this technique [12]. Similarly, the bell, anti-bell, dark, kink, flat kink and other wave solutions of Fokas-Lenelles by applying generalized Kudryashov technique [13], exact solitons of KdV-Burger model are obtained in [14] etc.

Our mathematical physical important model is the (1+1)-dimensional non-linear simplified Modified Camassa-Holm model (SMCHM). Different types of exact solitons of our concerned model have been achieved with the use of different methods. Likely, some distinct kind of travelling wave solitons of SMCHM have been attained with the help of generalized $(G^{\prime }/G)$-expansion scheme in [15], solitary wave solitons are gained through Exp-function technique in [16], different types of wave solutions have been gained by utilizing the Riccati-Bernoulli sub-ODE scheme in [17] etc.

The purpose of our research is to discover the new types of M-fractional wave solitons to the (1+1)-dimensional non-linear simplified Modified Camassa-Holm model by utilizing modified simplest equation, Sardar sub-equation and generalized Kudryashov techniques.

The paper have distinct sections; In section 2: we explain the our concerned model and it’s mathematical analysis. In section 3: we describe the modified simplest equation technique and it’s application to obtain the soliton solutions. In section 4: we explain the Sardar sub-equation technique and apply it to gain the new soliton solutions of our concerned model. In section 5: we describe the generalized Kudryashov technique and it’s application to obtain the soliton solutions. In section 6: we explain the some obtained solutions through 2-dimensional, 3-dimensional and contour plots. In section 7: we give a conclusion of our research work.

2. The concerning model and It’s mathematical analysis

Let’s assume the non-linear M-fractional simplified Modified Camassa-Holm model from the family of important equations known as the modified $\beta$-equations explained by Wazwaz [18].

$$D_{M,t}^{\alpha ,\gamma }g-D_{M,t}^{\alpha ,\gamma }\left(D_{M,2x}^{2\alpha ,\gamma }g\right)+(\beta +1)g^2{D}_{M,2x}^{2\alpha ,\gamma }g-\beta D_{M,x}^{\alpha ,\gamma }g{D}_{M,2x}^{2\alpha ,\gamma }g-D_{M,3x}^{3\alpha ,\gamma }g=0.\beta >0.$$

(1)

here g=g(x,t) represents the wave profile while $\Omega$ and $\theta$ are nonzero parameters. Putting $\beta =2$ in the Eq.(1), we get

$$D_{M,t}^{\alpha ,\gamma }g-D_{M,t}^{\alpha ,\gamma }\left(D_{M,2x}^{2\alpha ,\gamma }g\right)+3g^2{D}_{M,2x}^{2\alpha ,\gamma }g-2D_{M,x}^{\alpha ,\gamma }g{D}_{M,2x}^{2\alpha ,\gamma }g-D_{M,3x}^{3\alpha ,\gamma }g=0.$$

(2)

This form is called modified Camassa-Holm model. Furthermore simplified form is of Eq.(2) given in [19].

$$D_{M,t}^{\alpha ,\gamma }g+2\Omega D_{M,x}^{\alpha ,\gamma }g-D_{M,t}^{\alpha ,\gamma }\left(D_{M,2x}^{2\alpha ,\gamma }g\right)+\theta g^2{D}_{M,x}^{\alpha ,\gamma }g=0.\Omega \in \Re \beta >0$$

(3)

here

$$D_{M,t}^{\alpha ,{\rm Y}}g\left(t\right)=\underset{\tau \to 0}{lim}\frac{g\left(t{E}_{{\rm Y}}\left(\tau t^{1-\alpha }\right)\right)-g\left(t\right)}{\tau },\alpha \in (0,1],{\rm Y}>0,$$

(4)

where $E_{{\rm Y}}(.)$ is the truncated Mittag-Leffler (ML) function of one parameter shown in [20,21].

Assume the wave transformation given as follows:

$$g=G\left(\xi \right),\xi =\frac{\Gamma (1+{\rm Y})}{\alpha }(\mu x^{\alpha }+\delta t^{\alpha })$$

(5)

where $\mu$ and $\delta$ are the nonzero constants. By substituting the Eq.(5) into Eq.(3) we attain

$$(\delta +2\Omega \mu )G-\delta {\mu }^2{G}^{\prime \prime }+\frac{\theta \mu }{3}{G}^3=0.$$

(6)

3. Modified simplest equation technique

The fundamental points of this scheme are given as:

Step 1:

Consider a NLPDE:

$$V(h,h^2,h^2{h}_x,h_y,h_{yy},h_{xx},h_{xy},h_{xt},...)=0,$$

(7)

here $h=h(x,y,t)$ represents wave profile.

Consider the wave transformation:

$$h(x,y,t)=H\left(\xi \right),\xi =x-\mu y+\lambda t.$$

(8)

Inserting Eq. (8) into Eq. (7), we gain a NLODE:

$$V(H\left(\xi \right),H^2\left(\xi \right)H^{{}^{\prime }}\left(\xi \right),H^{{}^{\prime \prime }}\left(\xi \right),...)=0.$$

(9)

Step 2:Consider Eq.(9) has below shape solution:

$$G\left(\xi \right)=\sum _{j=1}^m{b}_j{\psi }^j\left(\xi \right)$$

(10)

where $b_j(j=1,2,3,...,m)$ are undetermined and $b_m\ne 0$.

A new profile $\psi \left(\xi \right)$ fulfil below ODE:

$${\psi }^{\prime }\left(\xi \right)={\psi }^2\left(\xi \right)+\omega$$

(11)

where $\omega$ is a parameter.

Eq.(11) have solutions for different cases of $\omega$:

if $\omega <0$,

$$\psi \left(\xi \right)=-\sqrt{-\omega }tanh\left(\sqrt{-\omega }\xi \right)$$

(12)

$$\psi \left(\xi \right)=-\sqrt{-\omega }coth\left(\sqrt{-\omega }\xi \right)$$

(13)

$$\psi \left(\xi \right)=\sqrt{-\omega }\left(-tanh\left(2\sqrt{-\omega }\xi \right)\pm isech\left(2\sqrt{-\omega }\xi \right)\right),$$

(14)

$$\psi \left(\xi \right)=\sqrt{-\omega }\left(-coth\left(2\sqrt{-\omega }\xi \right)\pm csch\left(2\sqrt{-\omega }\xi \right)\right),$$

(15)

$$\psi \left(\xi \right)=-\frac{\sqrt{-\omega }}{2}\left(tanh\left(\frac{\sqrt{-\omega }}{2}\xi \right)+coth\left(\frac{\sqrt{-\omega }}{2}\xi \right)\right).$$

(16)

if $\omega >0$,

$$\psi \left(\xi \right)=\sqrt{\omega }tan\left(\sqrt{\omega }\xi \right)$$

(17)

$$\psi \left(\xi \right)=-\sqrt{\omega }cot\left(\sqrt{\omega }\xi \right)$$

(18)

$$\psi \left(\xi \right)=\sqrt{\omega }\left(tan\left(2\sqrt{\omega }\xi \right)\pm sec\left(2\sqrt{\omega }\xi \right)\right),$$

(19)

$$\psi \left(\xi \right)=\sqrt{\omega }\left(-cot\left(2\sqrt{\omega }\xi \right)\pm csc\left(2\sqrt{\omega }\xi \right)\right),$$

(20)

$$\psi \left(\xi \right)=\frac{\sqrt{\omega }}{2}\left(tan\left(\frac{\sqrt{\omega }}{2}\xi \right)-cot\left(\frac{\sqrt{\omega }}{2}\xi \right)\right).$$

(21)

If $\omega =0$,

$$\psi \left(\xi \right)=-\frac{1}{\xi }$$

(22)

Step 3:

Putting Eq.(10) into Eq.(9) with Eq.(11) and collecting the coefficients of every order of ${\psi }^j$. Substituting co-efficients of equal to 0, we obtain a set of equations involving $b_i$, $\lambda$, $\mu$. Manipulating the gained set of equations, we gain results for parameters.

Step 4:

Inserting Eq.(9) of which $b_j$, $\lambda$, $\mu$ has been obtained into Eq.(10), we attain solutions of Eq.(7).

3.1. New soliton solutions of Eq.(6) by MSET

Eq.(10) changes into given form for m=1:

$$G\left(\xi \right)=b_0+b_1\psi \left(\xi \right)$$

(23)

Using Eq.(23) into Eq.(6) with Eq.(11). By collecting coefficients of every order of $\psi \left(\xi \right)$ and taking equal to 0, we obtain a system of equations. Manipulating the achieved set by Mathematica software, we attain sets.

Set 1:

$$\left\{b_0=0,b_1=-\frac{2\sqrt{3}\mu \sqrt{\Omega }}{\sqrt{2\theta {\mu }^2\omega -\theta }},\delta =\frac{2\mu \Omega }{2{\mu }^2\omega -1}\right\}$$

(24)

Case 1: if $\omega <0$.

$$g(x,t)=-\frac{2\sqrt{3}\mu \sqrt{\Omega }}{\sqrt{2\theta {\mu }^2\omega -\theta }}(-\sqrt{-\omega }tanh\left(\sqrt{-\omega }\frac{\Gamma (1+{\rm Y})}{\alpha }(\mu x^{\alpha }+\left(\frac{2\mu \Omega }{2{\mu }^2\omega -1}\right)t^{\alpha })\right))$$

(25)

$$g(x,t)=-\frac{2\sqrt{3}\mu \sqrt{\Omega }}{\sqrt{2\theta {\mu }^2\omega -\theta }}(-\sqrt{-\omega }coth\left(\sqrt{-\omega }\frac{\Gamma (1+{\rm Y})}{\alpha }(\mu x^{\alpha }+\left(\frac{2\mu \Omega }{2{\mu }^2\omega -1}\right)t^{\alpha })\right))$$

(26)

$$\begin{array}{c}g(x,t)=-\frac{2\sqrt{3}\mu \sqrt{\Omega }}{\sqrt{2\theta {\mu }^2\omega -\theta }}\left(\sqrt{-\omega }\right(-tanh\left(2\sqrt{-\omega }\frac{\Gamma (1+{\rm Y})}{\alpha }(\mu x^{\alpha }+\left(\frac{2\mu \Omega }{2{\mu }^2\omega -1}\right)t^{\alpha })\right)\hfill \\ \hfill \pm isech\left(2\sqrt{-\omega }\frac{\Gamma (1+{\rm Y})}{\alpha }(\mu x^{\alpha }+\left(\frac{2\mu \Omega }{2{\mu }^2\omega -1}\right)t^{\alpha })\right)\left)\right)\end{array}$$

(27)

$$\begin{array}{c}g(x,t)=-\frac{2\sqrt{3}\mu \sqrt{\Omega }}{\sqrt{2\theta {\mu }^2\omega -\theta }}\left(\sqrt{-\omega }\right(-coth\left(2\sqrt{-\omega }\frac{\Gamma (1+{\rm Y})}{\alpha }(\mu x^{\alpha }+\left(\frac{2\mu \Omega }{2{\mu }^2\omega -1}\right)t^{\alpha })\right)\hfill \\ \hfill \pm csch\left(2\sqrt{-\omega }\frac{\Gamma (1+{\rm Y})}{\alpha }(\mu x^{\alpha }+\left(\frac{2\mu \Omega }{2{\mu }^2\omega -1}\right)t^{\alpha })\right)\left)\right)\end{array}$$

(28)

$$\begin{array}{c}g(x,t)=-\frac{2\sqrt{3}\mu \sqrt{\Omega }}{\sqrt{2\theta {\mu }^2\omega -\theta }}(-\frac{\sqrt{-\omega }}{2}(tanh\left(\frac{\sqrt{-\omega }}{2}\frac{\Gamma (1+{\rm Y})}{\alpha }(\mu x^{\alpha }+\left(\frac{2\mu \Omega }{2{\mu }^2\omega -1}\right)t^{\alpha })\right)\hfill \\ \hfill +coth\left(\frac{\sqrt{-\omega }}{2}\frac{\Gamma (1+{\rm Y})}{\alpha }(\mu x^{\alpha }+\left(\frac{2\mu \Omega }{2{\mu }^2\omega -1}\right)t^{\alpha })\right)\left)\right)\end{array}$$

(29)

Case 2: if $\omega >0$.

$$g(x,t)=-\frac{2\sqrt{3}\mu \sqrt{\Omega }}{\sqrt{2\theta {\mu }^2\omega -\theta }}(\sqrt{\omega }tan\left(\sqrt{\omega }\frac{\Gamma (1+{\rm Y})}{\alpha }(\mu x^{\alpha }+\left(\frac{2\mu \Omega }{2{\mu }^2\omega -1}\right)t^{\alpha })\right))$$

(30)

$$g(x,t)=-\frac{2\sqrt{3}\mu \sqrt{\Omega }}{\sqrt{2\theta {\mu }^2\omega -\theta }}(-\sqrt{\omega }cot\left(\sqrt{\omega }\frac{\Gamma (1+{\rm Y})}{\alpha }(\mu x^{\alpha }+\left(\frac{2\mu \Omega }{2{\mu }^2\omega -1}\right)t^{\alpha })\right))$$

(31)

$$\begin{array}{c}g(x,t)=-\frac{2\sqrt{3}\mu \sqrt{\Omega }}{\sqrt{2\theta {\mu }^2\omega -\theta }}\left(\sqrt{\omega }\right(tan\left(2\sqrt{\omega }\frac{\Gamma (1+{\rm Y})}{\alpha }(\mu x^{\alpha }+\left(\frac{2\mu \Omega }{2{\mu }^2\omega -1}\right)t^{\alpha })\right)\hfill \\ \hfill \pm sec\left(2\sqrt{\omega }\frac{\Gamma (1+{\rm Y})}{\alpha }(\mu x^{\alpha }+\left(\frac{2\mu \Omega }{2{\mu }^2\omega -1}\right)t^{\alpha })\right)\left)\right)\end{array}$$

(32)

$$\begin{array}{c}g(x,t)=-\frac{2\sqrt{3}\mu \sqrt{\Omega }}{\sqrt{2\theta {\mu }^2\omega -\theta }}\left(\sqrt{\omega }\right(-cot\left(2\sqrt{\omega }\frac{\Gamma (1+{\rm Y})}{\alpha }(\mu x^{\alpha }+\left(\frac{2\mu \Omega }{2{\mu }^2\omega -1}\right)t^{\alpha })\right)\hfill \\ \hfill \pm csc\left(2\sqrt{\omega }\frac{\Gamma (1+{\rm Y})}{\alpha }(\mu x^{\alpha }+\left(\frac{2\mu \Omega }{2{\mu }^2\omega -1}\right)t^{\alpha })\right)\left)\right)\end{array}$$

(33)

$$\begin{array}{c}g(x,t)=-\frac{2\sqrt{3}\mu \sqrt{\Omega }}{\sqrt{2\theta {\mu }^2\omega -\theta }}\left(\frac{\sqrt{\omega }}{2}\right(tan\left(\frac{\sqrt{\omega }}{2}\frac{\Gamma (1+{\rm Y})}{\alpha }(\mu x^{\alpha }+\left(\frac{2\mu \Omega }{2{\mu }^2\omega -1}\right)t^{\alpha })\right)\hfill \\ \hfill -cot\left(\frac{\sqrt{\omega }}{2}\frac{\Gamma (1+{\rm Y})}{\alpha }(\mu x^{\alpha }+\left(\frac{2\mu \Omega }{2{\mu }^2\omega -1}\right)t^{\alpha })\right)\left)\right)\end{array}$$

(34)

Case 3: if $\omega =0$.

$$g(x,t)=\frac{2\sqrt{3}\mu \sqrt{\Omega }}{\sqrt{-\theta }\frac{\Gamma (1+{\rm Y})}{\alpha }(\mu x^{\alpha }-2\mu \Omega t^{\alpha })}$$

(35)

Set 2:

$$\left\{b_0=0,b_1=\frac{2\sqrt{3}\mu \sqrt{\Omega }}{\sqrt{2\theta {\mu }^2\omega -\theta }},\delta =\frac{2\mu \Omega }{2{\mu }^2\omega -1}\right\}$$

(36)

Case 1:if $\omega <0$.

$$g(x,t)=\frac{2\sqrt{3}\mu \sqrt{\Omega }}{\sqrt{2\theta {\mu }^2\omega -\theta }}(-\sqrt{-\omega }tanh\left(\sqrt{-\omega }\frac{\Gamma (1+{\rm Y})}{\alpha }(\mu x^{\alpha }+\left(\frac{2\mu \Omega }{2{\mu }^2\omega -1}\right)t^{\alpha })\right))$$

(37)

$$g(x,t)=\frac{2\sqrt{3}\mu \sqrt{\Omega }}{\sqrt{2\theta {\mu }^2\omega -\theta }}(-\sqrt{-\omega }coth\left(\sqrt{-\omega }\frac{\Gamma (1+{\rm Y})}{\alpha }(\mu x^{\alpha }+\left(\frac{2\mu \Omega }{2{\mu }^2\omega -1}\right)t^{\alpha })\right))$$

(38)

$$\begin{array}{c}g(x,t)=\frac{2\sqrt{3}\mu \sqrt{\Omega }}{\sqrt{2\theta {\mu }^2\omega -\theta }}\left(\sqrt{-\omega }\right(-tanh\left(2\sqrt{-\omega }\frac{\Gamma (1+{\rm Y})}{\alpha }(\mu x^{\alpha }+\left(\frac{2\mu \Omega }{2{\mu }^2\omega -1}\right)t^{\alpha })\right)\hfill \\ \hfill \pm isech\left(2\sqrt{-\omega }\frac{\Gamma (1+{\rm Y})}{\alpha }(\mu x^{\alpha }+\left(\frac{2\mu \Omega }{2{\mu }^2\omega -1}\right)t^{\alpha })\right)\left)\right)\end{array}$$

(39)

$$\begin{array}{c}g(x,t)=\frac{2\sqrt{3}\mu \sqrt{\Omega }}{\sqrt{2\theta {\mu }^2\omega -\theta }}\left(\sqrt{-\omega }\right(-coth\left(2\sqrt{-\omega }\frac{\Gamma (1+{\rm Y})}{\alpha }(\mu x^{\alpha }+\left(\frac{2\mu \Omega }{2{\mu }^2\omega -1}\right)t^{\alpha })\right)\hfill \\ \hfill \pm csch\left(2\sqrt{-\omega }\frac{\Gamma (1+{\rm Y})}{\alpha }(\mu x^{\alpha }+\left(\frac{2\mu \Omega }{2{\mu }^2\omega -1}\right)t^{\alpha })\right)\left)\right)\end{array}$$

(40)

$$\begin{array}{c}g(x,t)=\frac{2\sqrt{3}\mu \sqrt{\Omega }}{\sqrt{2\theta {\mu }^2\omega -\theta }}(-\frac{\sqrt{-\omega }}{2}(tanh\left(\frac{\sqrt{-\omega }}{2}\frac{\Gamma (1+{\rm Y})}{\alpha }(\mu x^{\alpha }+\left(\frac{2\mu \Omega }{2{\mu }^2\omega -1}\right)t^{\alpha })\right)\hfill \\ \hfill +coth\left(\frac{\sqrt{-\omega }}{2}\frac{\Gamma (1+{\rm Y})}{\alpha }(\mu x^{\alpha }+\left(\frac{2\mu \Omega }{2{\mu }^2\omega -1}\right)t^{\alpha })\right)\left)\right)\end{array}$$

(41)

Case 2: if $\omega >0$.

$$g(x,t)=\frac{2\sqrt{3}\mu \sqrt{\Omega }}{\sqrt{2\theta {\mu }^2\omega -\theta }}(\sqrt{\omega }tan\left(\sqrt{\omega }\frac{\Gamma (1+{\rm Y})}{\alpha }(\mu x^{\alpha }+\left(\frac{2\mu \Omega }{2{\mu }^2\omega -1}\right)t^{\alpha })\right))$$

(42)

$$g(x,t)=\frac{2\sqrt{3}\mu \sqrt{\Omega }}{\sqrt{2\theta {\mu }^2\omega -\theta }}(-\sqrt{\omega }cot\left(\sqrt{\omega }\frac{\Gamma (1+{\rm Y})}{\alpha }(\mu x^{\alpha }+\left(\frac{2\mu \Omega }{2{\mu }^2\omega -1}\right)t^{\alpha })\right))$$

(43)

$$\begin{array}{c}g(x,t)=\frac{2\sqrt{3}\mu \sqrt{\Omega }}{\sqrt{2\theta {\mu }^2\omega -\theta }}\left(\sqrt{\omega }\right(tan\left(2\sqrt{\omega }\frac{\Gamma (1+{\rm Y})}{\alpha }(\mu x^{\alpha }+\left(\frac{2\mu \Omega }{2{\mu }^2\omega -1}\right)t^{\alpha })\right)\hfill \\ \hfill \pm sec\left(2\sqrt{\omega }\frac{\Gamma (1+{\rm Y})}{\alpha }(\mu x^{\alpha }+\left(\frac{2\mu \Omega }{2{\mu }^2\omega -1}\right)t^{\alpha })\right)\left)\right)\end{array}$$

(44)

$$\begin{array}{c}g(x,t)=\frac{2\sqrt{3}\mu \sqrt{\Omega }}{\sqrt{2\theta {\mu }^2\omega -\theta }}\left(\sqrt{\omega }\right(-cot\left(2\sqrt{\omega }\frac{\Gamma (1+{\rm Y})}{\alpha }(\mu x^{\alpha }+\left(\frac{2\mu \Omega }{2{\mu }^2\omega -1}\right)t^{\alpha })\right)\hfill \\ \hfill \pm csc\left(2\sqrt{\omega }\frac{\Gamma (1+{\rm Y})}{\alpha }(\mu x^{\alpha }+\left(\frac{2\mu \Omega }{2{\mu }^2\omega -1}\right)t^{\alpha })\right)\left)\right)\end{array}$$

(45)

$$\begin{array}{c}g(x,t)=\frac{2\sqrt{3}\mu \sqrt{\Omega }}{\sqrt{2\theta {\mu }^2\omega -\theta }}\left(\frac{\sqrt{\omega }}{2}\right(tan\left(\frac{\sqrt{\omega }}{2}\frac{\Gamma (1+{\rm Y})}{\alpha }(\mu x^{\alpha }+\left(\frac{2\mu \Omega }{2{\mu }^2\omega -1}\right)t^{\alpha })\right)\hfill \\ \hfill -cot\left(\frac{\sqrt{\omega }}{2}\frac{\Gamma (1+{\rm Y})}{\alpha }(\mu x^{\alpha }+\left(\frac{2\mu \Omega }{2{\mu }^2\omega -1}\right)t^{\alpha })\right)\left)\right)\end{array}$$

(46)

Case 3: if $\omega =0$.

$$g(x,t)=-\frac{2\sqrt{3}\mu \sqrt{\Omega }}{\sqrt{-\theta }\frac{\Gamma (1+{\rm Y})}{\alpha }(\mu x^{\alpha }-2\mu \Omega t^{\alpha })}$$

(47)

4. Description of Sardar sub-equation technique

Here, we explain the fundamental points of Sardar sub-equation method [22]. Assume the nonlinear fractional PDE:

$$J(g,g_z,g_{zz},g_{zt},g{g}_{tt},g_{zzt},...)=0.$$

(48)

here $g=g(z,t)$ is a wave profile.

Substituting a wave transformation shown as:

$$g(z,t)=G\left(\zeta \right),\zeta =\lambda z+\mu t$$

(49)

We obtain a non-linear ODE shown as:

$$Y(G,G^{\prime \prime },G{G}^{\prime \prime },G^{\prime }{G}^2,...)=0.$$

(50)

Consider Eq.(50) posses the results in the given shape:

$$G\left(\zeta \right)=\sum _{i=0}^m{b}_i{\psi }^i\left(\zeta \right).$$

(51)

here $\psi \left(\zeta \right)$ fulfill the ODE shown as:

$${\psi }^{{}^{\prime }}\left(\zeta \right)=\sqrt{\sigma +\kappa {\psi }^2\left(\zeta \right)+{\psi }^4\left(\zeta \right)}.$$

(52)

here $\sigma$ and $\kappa$ are constants.

Using Eq.(51) into Eq.(50) along Eq.(52) and collecting the coefficients of every power of ${\psi }^i$. Inserting co-efficient of every power equal to 0, we achieve a set of equations having $b_i$, $\lambda$, $\mu$. Solving the achieved set of equation, we gain the results for unknowns.

Case 1: if $\kappa >0$ and $\sigma =0$, we have

$${\psi }_1^{\pm }=\pm \sqrt{-\kappa ab}sec{h}_{ab}\left(\sqrt{\kappa }\zeta \right),$$

(53)

$${\psi }_2^{\pm }=\pm \sqrt{\kappa ab}csc{h}_{ab}\left(\sqrt{\kappa }\zeta \right),$$

(54)

where, $sec{h}_{ab}\left(\zeta \right)=\frac{2}{a{e}^{\zeta }+b{e}^{-\zeta }}$, $csc{h}_{ab}\left(\zeta \right)=\frac{2}{a{e}^{\zeta }-b{e}^{-\zeta }}$

Case 2: if $\kappa <0$ and $\sigma =0$, we have

$${\psi }_3^{\pm }=\pm \sqrt{-\kappa ab}{sec}_{ab}\left(\sqrt{-\kappa }\zeta \right),$$

(55)

$${\psi }_4^{\pm }=\pm \sqrt{-\kappa ab}{csc}_{ab}\left(\sqrt{-\kappa }\zeta \right),$$

(56)

where, ${sec}_{ab}\left(\zeta \right)=\frac{2}{a{e}^{\iota \zeta }+b{e}^{-\iota \zeta }}$, ${csc}_{ab}\left(\zeta \right)=\frac{2\iota }{a{e}^{\iota \zeta }-b{e}^{-\iota \zeta }}$

Case 3: if $\kappa <0$ and $\sigma =\frac{{\kappa }^2}{4}$, we have

$${\psi }_5^{\pm }=\pm \sqrt{-\frac{\kappa }{2}}{tanh}_{ab}\left(\sqrt{-\frac{\kappa }{2}}\zeta \right),$$

(57)

$${\psi }_6^{\pm }=\pm \sqrt{-\frac{\kappa }{2}}{coth}_{ab}\left(\sqrt{-\frac{\kappa }{2}}\zeta \right),$$

(58)

$${\psi }_7^{\pm }=\pm \sqrt{-\frac{\kappa }{2}}({tanh}_{ab}\left(\sqrt{-2\kappa }\zeta \right)\pm \iota \sqrt{ab}sec{h}_{ab}\left(\sqrt{-2\kappa }\zeta \right)),$$

(59)

$${\psi }_8^{\pm }=\pm \sqrt{-\frac{\kappa }{2}}({coth}_{ab}\left(\sqrt{-2\kappa }\zeta \right)\pm \sqrt{ab}csc{h}_{ab}\left(\sqrt{-2\kappa }\zeta \right)),$$

(60)

$${\psi }_9^{\pm }=\pm \sqrt{-\frac{\kappa }{8}}({tanh}_{ab}\left(\sqrt{-\frac{\kappa }{8}}\zeta \right)+{coth}_{ab}\left(\sqrt{-\frac{\kappa }{8}}\zeta \right)),$$

(61)

where, ${tanh}_{ab}\left(\zeta \right)=\frac{a{e}^{\zeta }-b{e}^{-\zeta }}{a{e}^{\zeta }+b{e}^{-\zeta }}$, ${coth}_{ab}\left(\zeta \right)=\frac{a{e}^{\zeta }+b{e}^{-\zeta }}{a{e}^{\zeta }-b{e}^{-\zeta }}$

Case 4: if $\kappa >0$ and $\sigma =\frac{{\kappa }^2}{4}$, we have

$${\psi }_{10}^{\pm }=\pm \sqrt{\frac{\kappa }{2}}{tan}_{ab}\left(\sqrt{\frac{\kappa }{2}}\zeta \right),$$

(62)

$${\psi }_{11}^{\pm }=\pm \sqrt{\frac{\kappa }{2}}{cot}_{ab}\left(\sqrt{\frac{\kappa }{2}}\zeta \right),$$

(63)

$${\psi }_{12}^{\pm }=\pm \sqrt{\frac{\kappa }{2}}({tan}_{ab}\left(\sqrt{2\kappa }\zeta \right)\pm \sqrt{ab}{sec}_{ab}\left(\sqrt{2\kappa }\zeta \right)),$$

(64)

$${\psi }_{13}^{\pm }=\pm \sqrt{\frac{\kappa }{2}}({cot}_{ab}\left(\sqrt{2\kappa }\zeta \right)\pm \sqrt{ab}{csc}_{ab}\left(\sqrt{2\kappa }\zeta \right)),$$

(65)

$${\psi }_{14}^{\pm }=\pm \sqrt{\frac{\kappa }{8}}({tan}_{ab}\left(\sqrt{\frac{\kappa }{8}}\zeta \right)+{cot}_{ab}\left(\sqrt{\frac{\kappa }{8}}\zeta \right)),$$

(66)

where, ${tan}_{ab}\left(\zeta \right)=-\iota \frac{a{e}^{\iota \zeta }-b{e}^{-\iota \zeta }}{a{e}^{\iota \zeta }+b{e}^{-\iota \zeta }}$, ${cot}_{ab}\left(\zeta \right)=\iota \frac{a{e}^{\iota \zeta }+b{e}^{-\iota \zeta }}{a{e}^{\iota \zeta }-b{e}^{-\iota \zeta }}$

4.1. Solutions through Sardar sub-equation method

Eq.(51) changes into given form for m=1.

$$G\left(\zeta \right)=b_0+b_1\psi \left(\zeta \right)$$

(67)

Putting Eq.(67) into Eq.(6) by using Eq.(52). Collecting co-efficients of every power of $\psi \left(\zeta \right)$ and taking equal to 0, we attain a set of equations. Solving the attained set of equations by Mathematica tool, we gain a set.

Set :

$$\left\{b_0=0,b_1=\pm \frac{2\sqrt{3}\mu \sqrt{\Omega }}{\sqrt{\theta \kappa {\mu }^2-\theta }},\delta =\frac{2\mu \Omega }{\kappa {\mu }^2-1}\right\}$$

(68)

Case 1:

$$g(x,t)=\pm \frac{2\sqrt{3}\mu \sqrt{\Omega }}{\sqrt{\theta \kappa {\mu }^2-\theta }}\left(\sqrt{-\kappa ab}sec{h}_{ab}\left(\sqrt{\kappa }\frac{\Gamma (1+{\rm Y})}{\alpha }(\mu x^{\alpha }+\left(\frac{2\mu \Omega }{\kappa {\mu }^2-1}\right)t^{\alpha })\right)\right)$$

(69)

$$g(x,t)=\pm \frac{2\sqrt{3}\mu \sqrt{\Omega }}{\sqrt{\theta \kappa {\mu }^2-\theta }}\left(\sqrt{\kappa ab}csc{h}_{ab}\left(\sqrt{\kappa }\frac{\Gamma (1+{\rm Y})}{\alpha }(\mu x^{\alpha }+\left(\frac{2\mu \Omega }{\kappa {\mu }^2-1}\right)t^{\alpha })\right)\right)$$

(70)

Case 2:

$$g(x,t)=\pm \frac{2\sqrt{3}\mu \sqrt{\Omega }}{\sqrt{\theta \kappa {\mu }^2-\theta }}\left(\sqrt{-\kappa ab}{sec}_{ab}\left(\sqrt{-\kappa }\frac{\Gamma (1+{\rm Y})}{\alpha }(\mu x^{\alpha }+\left(\frac{2\mu \Omega }{\kappa {\mu }^2-1}\right)t^{\alpha })\right)\right)$$

(71)

$$g(x,t)=\pm \frac{2\sqrt{3}\mu \sqrt{\Omega }}{\sqrt{\theta \kappa {\mu }^2-\theta }}\left(\sqrt{-\kappa ab}{csc}_{ab}\left(\sqrt{-\kappa }\frac{\Gamma (1+{\rm Y})}{\alpha }(\mu x^{\alpha }+\left(\frac{2\mu \Omega }{\kappa {\mu }^2-1}\right)t^{\alpha })\right)\right)$$

(72)

Case 3:

$$g(x,t)=\pm \frac{2\sqrt{3}\mu \sqrt{\Omega }}{\sqrt{\theta \kappa {\mu }^2-\theta }}\left(\sqrt{-\frac{\kappa }{2}}{tanh}_{ab}\left(\sqrt{-\frac{\kappa }{2}}\frac{\Gamma (1+{\rm Y})}{\alpha }(\mu x^{\alpha }+\left(\frac{2\mu \Omega }{\kappa {\mu }^2-1}\right)t^{\alpha })\right)\right)$$

(73)

$$g(x,t)=\pm \frac{2\sqrt{3}\mu \sqrt{\Omega }}{\sqrt{\theta \kappa {\mu }^2-\theta }}\left(\sqrt{-\frac{\kappa }{2}}{coth}_{ab}\left(\sqrt{-\frac{\kappa }{2}}\frac{\Gamma (1+{\rm Y})}{\alpha }(\mu x^{\alpha }+\left(\frac{2\mu \Omega }{\kappa {\mu }^2-1}\right)t^{\alpha })\right)\right)$$

(74)

$$\begin{array}{c}g(x,t)=\pm \frac{2\sqrt{3}\mu \sqrt{\Omega }}{\sqrt{\theta \kappa {\mu }^2-\theta }}\left(\sqrt{-\frac{\kappa }{2}}\right({tanh}_{ab}\left(\sqrt{-2\kappa }\frac{\Gamma (1+{\rm Y})}{\alpha }(\mu x^{\alpha }+\left(\frac{2\mu \Omega }{\kappa {\mu }^2-1}\right)t^{\alpha })\right)\hfill \\ \hfill \pm \iota \sqrt{ab}sec{h}_{ab}\left(\sqrt{-2\kappa }\frac{\Gamma (1+{\rm Y})}{\alpha }(\mu x^{\alpha }+\left(\frac{2\mu \Omega }{\kappa {\mu }^2-1}\right)t^{\alpha })\right)\left)\right)\end{array}$$

(75)

$$\begin{array}{c}g(x,t)=\pm \frac{2\sqrt{3}\mu \sqrt{\Omega }}{\sqrt{\theta \kappa {\mu }^2-\theta }}\left(\sqrt{-\frac{\kappa }{2}}\right({coth}_{ab}\left(\sqrt{-2\kappa }\frac{\Gamma (1+{\rm Y})}{\alpha }(\mu x^{\alpha }+\left(\frac{2\mu \Omega }{\kappa {\mu }^2-1}\right)t^{\alpha })\right)\hfill \\ \hfill \pm \sqrt{ab}csc{h}_{ab}\left(\sqrt{-2\kappa }\frac{\Gamma (1+{\rm Y})}{\alpha }(\mu x^{\alpha }+\left(\frac{2\mu \Omega }{\kappa {\mu }^2-1}\right)t^{\alpha })\right)\left)\right)\end{array}$$

(76)

$$g(x,t)=\pm \frac{2\sqrt{3}\mu \sqrt{\Omega }}{\sqrt{\theta \kappa {\mu }^2-\theta }}\left(\sqrt{\frac{\kappa }{2}}{tan}_{ab}\left(\sqrt{\frac{\kappa }{2}}\frac{\Gamma (1+{\rm Y})}{\alpha }(\mu x^{\alpha }+\left(\frac{2\mu \Omega }{\kappa {\mu }^2-1}\right)t^{\alpha })\right)\right)$$

(77)

Case 4:

$$g(x,t)=\pm \frac{2\sqrt{3}\mu \sqrt{\Omega }}{\sqrt{\theta \kappa {\mu }^2-\theta }}\left(\sqrt{\frac{\kappa }{2}}{cot}_{ab}\left(\sqrt{\frac{\kappa }{2}}\frac{\Gamma (1+{\rm Y})}{\alpha }(\mu x^{\alpha }+\left(\frac{2\mu \Omega }{\kappa {\mu }^2-1}\right)t^{\alpha })\right)\right)$$

(78)

$$\begin{array}{c}g(x,t)=\pm \frac{2\sqrt{3}\mu \sqrt{\Omega }}{\sqrt{\theta \kappa {\mu }^2-\theta }}\left(\sqrt{\frac{\kappa }{2}}\right({tan}_{ab}\left(\sqrt{2\kappa }\frac{\Gamma (1+{\rm Y})}{\alpha }(\mu x^{\alpha }+\left(\frac{2\mu \Omega }{\kappa {\mu }^2-1}\right)t^{\alpha })\right)\hfill \\ \hfill \pm \sqrt{ab}{sec}_{ab}\left(\sqrt{2\kappa }\frac{\Gamma (1+{\rm Y})}{\alpha }(\mu x^{\alpha }+\left(\frac{2\mu \Omega }{\kappa {\mu }^2-1}\right)t^{\alpha })\right)\left)\right)\end{array}$$

(79)

$$\begin{array}{c}g(x,t)=\pm \frac{2\sqrt{3}\mu \sqrt{\Omega }}{\sqrt{\theta \kappa {\mu }^2-\theta }}\left(\sqrt{-\frac{\kappa }{8}}\right({tanh}_{ab}\left(\sqrt{-\frac{\kappa }{8}}\frac{\Gamma (1+{\rm Y})}{\alpha }(\mu x^{\alpha }+\left(\frac{2\mu \Omega }{\kappa {\mu }^2-1}\right)t^{\alpha })\right)\hfill \\ \hfill +{coth}_{ab}\left(\sqrt{-\frac{\kappa }{8}}\frac{\Gamma (1+{\rm Y})}{\alpha }(\mu x^{\alpha }+\left(\frac{2\mu \Omega }{\kappa {\mu }^2-1}\right)t^{\alpha })\right)\left)\right)\end{array}$$

(80)

$$\begin{array}{c}g(x,t)=\pm \frac{2\sqrt{3}\mu \sqrt{\Omega }}{\sqrt{\theta \kappa {\mu }^2-\theta }}\left(\sqrt{\frac{\kappa }{2}}\right({cot}_{ab}\left(\sqrt{2\kappa }\frac{\Gamma (1+{\rm Y})}{\alpha }(\mu x^{\alpha }+\left(\frac{2\mu \Omega }{\kappa {\mu }^2-1}\right)t^{\alpha })\right)\hfill \\ \hfill \pm \sqrt{ab}{csc}_{ab}\left(\sqrt{2\kappa }\frac{\Gamma (1+{\rm Y})}{\alpha }(\mu x^{\alpha }+\left(\frac{2\mu \Omega }{\kappa {\mu }^2-1}\right)t^{\alpha })\right)\left)\right)\end{array}$$

(81)

$$\begin{array}{c}g(x,t)=\pm \frac{2\sqrt{3}\mu \sqrt{\Omega }}{\sqrt{\theta \kappa {\mu }^2-\theta }}\left(\sqrt{\frac{\kappa }{8}}\right({tan}_{ab}\left(\sqrt{\frac{\kappa }{8}}\frac{\Gamma (1+{\rm Y})}{\alpha }(\mu x^{\alpha }+\left(\frac{2\mu \Omega }{\kappa {\mu }^2-1}\right)t^{\alpha })\right)\hfill \\ \hfill +{cot}_{ab}\left(\sqrt{\frac{\kappa }{8}}\frac{\Gamma (1+{\rm Y})}{\alpha }(\mu x^{\alpha }+\left(\frac{2\mu \Omega }{\kappa {\mu }^2-1}\right)t^{\alpha })\right)\left)\right)\end{array}$$

(82)

5. The generalized Kudryashov technique

The basic steps of this technique are given as [23,24]:

Step 1:

Take a nonlinear PDE:

$$Y(q,q^2{q}_{\gamma },q_{\theta },q_{\theta \theta },q_{\gamma \gamma },q_{\gamma \theta },...)=0$$

(83)

here q is a wave profile.

Assume the transformation given as:

$$q(\gamma ,\theta )=Q\left(\xi \right),\xi =\gamma -\nu \theta$$

(84)

Inserting Eq. (84) into Eq. (83), we gain the nonlinear ODE:

$$F(Q,Q^{{}^{\prime }},Q^2{Q}^{{}^{\prime }},Q^{{}^{\prime \prime }},Q^2{Q}^{{}^{\prime }},...)=0.$$

(85)

Step 2:

Consider the roots of Eq. (85) is of form:

$$Q\left(\xi \right)={\alpha }_0+\sum _{j=1}^m\frac{{\alpha }_p}{{(1+\psi \left(\xi \right))}^p}$$

(86)

here ${\alpha }_0$ and ${\alpha }_j$, $(j=1,2,3,...,m)$ are undetermined parameters and $\psi$ is a new wave profile of $\xi$ that is a root of the general Riccati equation shown as:

$${\psi }^{\prime }\left(\xi \right)=a+b\psi \left(\xi \right)+c{\psi }^2\left(\xi \right)$$

(87)

where a, b and c are the constants. The roots of Eq.(87) are given in the below cases [25]:

Case 1: If all a, b and c are nonzero, we have $\psi \left(\xi \right)$ is shown by

$$\psi \left(\xi \right)=\frac{1}{2c}\left(\sqrt{4ac-b^2}tan\left(\frac{1}{2}\sqrt{4ac-b^2}\left(d_0+\xi \right)\right)-b\right),4ac>b^2$$

(88)

$$\psi \left(\xi \right)=\frac{-1}{2c}\left(\sqrt{4ac-b^2}cot\left(\frac{1}{2}\sqrt{4ac-b^2}\left(d_0+\xi \right)\right)+b\right),4ac>b^2$$

(89)

$$\psi \left(\xi \right)=\frac{-1}{2c}\left(\sqrt{4ac-b^2}tanh\left(\frac{1}{2}\sqrt{4ac-b^2}\left(d_0+\xi \right)\right)+b\right),4ac<b^2$$

(90)

$$\psi \left(\xi \right)=\frac{-1}{2c}\left(\sqrt{4ac-b^2}coth\left(\frac{1}{2}\sqrt{4ac-b^2}\left(d_0+\xi \right)\right)+b\right),4ac<b^2$$

(91)

$$\psi \left(\xi \right)=\frac{-1}{c}\left(\frac{1}{d_0+\xi }+\frac{b}{2}\right),4ac=b^2$$

(92)

Case 2:If a=0 and $c\ne 0$, we have

$$\psi \left(\xi \right)=\frac{-1}{2c}\left(btanh\left(\frac{b}{2}\left(d_0+\xi \right)\right)+b\right),b^2>0$$

(93)

$$\psi \left(\xi \right)=\frac{-1}{2c}\left(bcoth\left(\frac{b}{2}\left(d_0+\xi \right)\right)+b\right),b^2>0$$

(94)

$$\psi \left(\xi \right)=\frac{1}{2c}\left(\sqrt{-b^2}tan\left(\frac{\sqrt{-b^2}}{2}\left(d_0+\xi \right)\right)-b\right),b^2<0$$

(95)

$$\psi \left(\xi \right)=\frac{-1}{2c}\left(\sqrt{-b^2}cot\left(\frac{\sqrt{-b^2}}{2}\left(d_0+\xi \right)\right)+b\right),b^2<0$$

(96)

$$\psi \left(\xi \right)=\frac{b}{bexp(-b(d_0+\xi ))-c},b\ne 0$$

(97)

$$\psi \left(\xi \right)=\frac{-1}{c\xi },b=0$$

(98)

Case 3:If b=0 and $c\ne 0$, we have

$$\psi \left(\xi \right)=\frac{\sqrt{ac}}{c}tan\left(\sqrt{ac}\left(d_0+\xi \right)\right),ac>0.$$

(99)

$$\psi \left(\xi \right)=-\frac{\sqrt{ac}}{c}cot\left(\sqrt{ac}\left(d_0+\xi \right)\right),ac>0.$$

(100)

$$\psi \left(\xi \right)=-\frac{\sqrt{ac}}{c}tanh\left(\sqrt{-ac}\left(d_0+\xi \right)\right),ac<0.$$

(101)

$$\psi \left(\xi \right)=-\frac{\sqrt{ac}}{c}coth\left(\sqrt{-ac}\left(d_0+\xi \right)\right),ac<0.$$

(102)

$$\psi \left(\xi \right)=\frac{-1}{c(d_0+\xi )},a=0$$

(103)

Case 4:If c=0 and $b\ne 0$, then

$$\psi \left(\xi \right)=\frac{1}{b}\left(exp\left(b(d_0+\xi )\right)-a\right)$$

(104)

Step 3:

By substituting Eq.(86) into Eq.(85) and collecting co-efficients of every order of $\psi \left(\xi \right)$. Leting the co-efficient of every order equal to 0, we attain a set of equations containing ${\alpha }_0$ and ${\alpha }_j$, $(j=1,2,3,...,m)$ and other parameters. By manipulating the attain set by Mathematica tool, we achieve the results for undetermined.

Set 4:

By substituting results of ${\alpha }_0$ and ${\alpha }_j$, $(j=1,2,3,...,m)$ into Eq.(86) and using Eq.(88)-Eq.(104), we gain results for Eq.(6).

5.1. New wave solutions through generalized Kudryashov technique

For $m=1$, Eq. (86) changes into:

$$G\left(\xi \right)={\alpha }_0+\frac{{\alpha }_1}{1+\psi \left(\xi \right)}$$

(105)

here ${\alpha }_0$ and ${\alpha }_1$, are unknowns. Inserting Eq. (105) into Eq. (6) along Eq. (87) and collecting the co-efficients every power of $\psi \left(\xi \right)$, we obtain the set of equations having ${\alpha }_0$,${\alpha }_1$ and other parameters. With the Mathematica tool, we achieve the set given as:

Set:

$$\left\{{\alpha }_0=\pm \frac{i\mu \sqrt{6\Omega }(b-2c)}{\sqrt{\theta (-4ac{\mu }^2+b^2{\mu }^2+2)}},{\alpha }_1=\pm \frac{2i\mu \sqrt{6\Omega }(a-b+c)}{\sqrt{\theta (2-4ac{\mu }^2+b^2{\mu }^2)}},\delta =-\frac{4\mu \Omega }{2-4ac{\mu }^2+b^2{\mu }^2},\Delta =\sqrt{4ac-b^2}\right\}$$

(106)

Case 1:

$$g(x,t)=\pm \frac{i\mu \sqrt{6\Omega }}{\sqrt{\theta (2-4ac{\mu }^2+b^2{\mu }^2)}}((b-2c)+\frac{2(a-b+c)}{1+\left(\frac{1}{2c}(\Delta tan(\frac{1}{2}\Delta (d_0+\frac{\Gamma (1+{\rm Y})}{\alpha }(\mu x^{\alpha }-\left(\frac{4\mu \Omega }{2-4ac{\mu }^2+b^2{\mu }^2}\right)t^{\alpha })))-b)\right)})$$

(107)

$$g(x,t)=\pm \frac{i\mu \sqrt{6\Omega }}{\sqrt{\theta (2-4ac{\mu }^2+b^2{\mu }^2)}}((b-2c)+\frac{2(a-b+c)}{1+\left(\frac{-1}{2c}(\Delta cot(\frac{1}{2}\Delta (d_0+\frac{\Gamma (1+{\rm Y})}{\alpha }(\mu x^{\alpha }-\left(\frac{4\mu \Omega }{2-4ac{\mu }^2+b^2{\mu }^2}\right)t^{\alpha })))+b)\right)})$$

(108)

$$g(x,t)=\pm \frac{i\mu \sqrt{6\Omega }}{\sqrt{\theta (2-4ac{\mu }^2+b^2{\mu }^2)}}((b-2c)+\frac{2(a-b+c)}{1+\left(\frac{-1}{2c}(\Delta tanh(\frac{1}{2}\Delta (d_0+\frac{\Gamma (1+{\rm Y})}{\alpha }(\mu x^{\alpha }-\left(\frac{4\mu \Omega }{2-4ac{\mu }^2+b^2{\mu }^2}\right)t^{\alpha })))+b)\right)})$$

(109)

$$g(x,t)=\pm \frac{i\mu \sqrt{6\Omega }}{\sqrt{\theta (2-4ac{\mu }^2+b^2{\mu }^2)}}((b-2c)+\frac{2(a-b+c)}{1+\left(\frac{-1}{2c}(\Delta coth(\frac{1}{2}\Delta (d_0+\frac{\Gamma (1+{\rm Y})}{\alpha }(\mu x^{\alpha }-\left(\frac{4\mu \Omega }{2-4ac{\mu }^2+b^2{\mu }^2}\right)t^{\alpha })))+b)\right)})$$

(110)

$$g(x,t)=\pm \frac{i\mu \sqrt{6\Omega }}{\sqrt{\theta (2-4ac{\mu }^2+b^2{\mu }^2)}}((b-2c)+\frac{2(a-b+c)}{1+\left(\frac{-1}{c}(\frac{1}{d_0+\frac{\Gamma (1+{\rm Y})}{\alpha }(\mu x^{\alpha }-\left(\frac{4\mu \Omega }{2-4ac{\mu }^2+b^2{\mu }^2}\right)t^{\alpha })}+\frac{b}{2})\right)})$$

(111)

Case 2:

$$g(x,t)=\pm \frac{i\mu \sqrt{6\Omega }}{\sqrt{\theta (b^2{\mu }^2+2)}}((b-2c)+\frac{2(c-b)}{1+\left(\frac{-1}{2c}(btanh\left(\frac{b}{2}(d_0+\frac{\Gamma (1+{\rm Y})}{\alpha }(\mu x^{\alpha }-\left(\frac{4\mu \Omega }{2-4ac{\mu }^2+b^2{\mu }^2}\right)t^{\alpha }))\right)+b)\right)})$$

(112)

$$g(x,t)=\pm \frac{i\mu \sqrt{6\Omega }}{\sqrt{\theta (b^2{\mu }^2+2)}}((b-2c)+\frac{2(c-b)}{1+\left(\frac{-1}{2c}(bcoth\left(\frac{b}{2}(d_0+\frac{\Gamma (1+{\rm Y})}{\alpha }(\mu x^{\alpha }-\left(\frac{4\mu \Omega }{2-4ac{\mu }^2+b^2{\mu }^2}\right)t^{\alpha }))\right)+b)\right)})$$

(113)

$$g(x,t)=\pm \frac{i\sqrt{6}\mu \sqrt{\Omega }}{\sqrt{\theta (b^2{\mu }^2+2)}}((b-2c)+\frac{2(-b+c)}{1+\left(\frac{1}{2c}(\sqrt{-b^2}tan\left(\frac{\sqrt{-b^2}}{2}(d_0+\frac{\Gamma (1+{\rm Y})}{\alpha }(\mu x^{\alpha }-\left(\frac{4\mu \Omega }{-4ac{\mu }^2+b^2{\mu }^2+2}\right)t^{\alpha }))\right)-b)\right)})$$

(114)

$$g(x,t)=\pm \frac{i\sqrt{6}\mu \sqrt{\Omega }}{\sqrt{\theta (b^2{\mu }^2+2)}}((b-2c)+\frac{2(-b+c)}{1+\left(\frac{-1}{2c}(\sqrt{-b^2}cot\left(\frac{\sqrt{-b^2}}{2}(d_0+\frac{\Gamma (1+{\rm Y})}{\alpha }(\mu x^{\alpha }-\left(\frac{4\mu \Omega }{-4ac{\mu }^2+b^2{\mu }^2+2}\right)t^{\alpha }))\right)+b)\right)})$$

(115)

$$g(x,t)=\pm \frac{i\sqrt{6}\mu \sqrt{\Omega }}{\sqrt{\theta (b^2{\mu }^2+2)}}((b-2c)+\frac{2(-b+c)}{1+\left(\frac{b}{bexp(-b(d_0+\frac{\Gamma (1+{\rm Y})}{\alpha }(\mu x^{\alpha }-\left(\frac{4\mu \Omega }{-4ac{\mu }^2+b^2{\mu }^2+2}\right)t^{\alpha })))-c}\right)})$$

(116)

$$g(x,t)=\pm \frac{i\sqrt{6}\mu \sqrt{\Omega }}{\sqrt{2\theta }}(-2c+\frac{2c}{1+\left(\frac{-1}{c\frac{\Gamma (1+{\rm Y})}{\alpha }(\mu x^{\alpha }-\left(\frac{4\mu \Omega }{-4ac{\mu }^2+b^2{\mu }^2+2}\right)t^{\alpha })}\right)})$$

(117)

Case 3:

$$g(x,t)=\pm \frac{i\sqrt{6}\mu \sqrt{\Omega }}{\sqrt{\theta (-4ac{\mu }^2+2)}}(-2c+\frac{2(a+c)}{1+(\frac{\sqrt{ac}}{c}tan\left(\sqrt{ac}(d_0+\frac{\Gamma (1+{\rm Y})}{\alpha }(\mu x^{\alpha }-\left(\frac{4\mu \Omega }{-4ac{\mu }^2+b^2{\mu }^2+2}\right)t^{\alpha }))\right))})$$

(118)

$$g(x,t)=\pm \frac{i\sqrt{6}\mu \sqrt{\Omega }}{\sqrt{\theta (-4ac{\mu }^2+2)}}(-2c+\frac{2(a+c)}{1+(-\frac{\sqrt{ac}}{c}cot\left(\sqrt{ac}(d_0+\frac{\Gamma (1+{\rm Y})}{\alpha }(\mu x^{\alpha }-\left(\frac{4\mu \Omega }{-4ac{\mu }^2+b^2{\mu }^2+2}\right)t^{\alpha }))\right))})$$

(119)

$$g(x,t)=\pm \frac{i\sqrt{6}\mu \sqrt{\Omega }}{\sqrt{\theta (-4ac{\mu }^2+2)}}(-2c+\frac{2(a+c)}{1+(-\frac{\sqrt{ac}}{c}tanh\left(\sqrt{-ac}(d_0+\frac{\Gamma (1+{\rm Y})}{\alpha }(\mu x^{\alpha }-\left(\frac{4\mu \Omega }{-4ac{\mu }^2+b^2{\mu }^2+2}\right)t^{\alpha }))\right))})$$

(120)

$$g(x,t)=\pm \frac{i\sqrt{6}\mu \sqrt{\Omega }}{\sqrt{\theta (-4ac{\mu }^2+2)}}(-2c+\frac{2(a+c)}{1+(-\frac{\sqrt{ac}}{c}coth\left(\sqrt{-ac}(d_0+\frac{\Gamma (1+{\rm Y})}{\alpha }(\mu x^{\alpha }-\left(\frac{4\mu \Omega }{-4ac{\mu }^2+b^2{\mu }^2+2}\right)t^{\alpha }))\right))})$$

(121)

$$g(x,t)=\pm \frac{i\sqrt{6}\mu \sqrt{\Omega }}{\sqrt{2\theta }}(-2c+\frac{2c}{1+\left(\frac{-1}{c(d_0+\frac{\Gamma (1+{\rm Y})}{\alpha }(\mu x^{\alpha }-\left(\frac{4\mu \Omega }{-4ac{\mu }^2+b^2{\mu }^2+2}\right)t^{\alpha }))}\right)})$$

(122)

Case 4:

$$g(x,t)=\pm \frac{i\sqrt{6}\mu \sqrt{\Omega }}{\sqrt{\theta (b^2{\mu }^2+2)}}(b+\frac{2(a-b)}{1+\left(\frac{1}{b}(exp\left(b(d_0+\frac{\Gamma (1+{\rm Y})}{\alpha }(\mu x^{\alpha }-\left(\frac{4\mu \Omega }{-4ac{\mu }^2+b^2{\mu }^2+2}\right)t^{\alpha }))\right)-a)\right)})$$

(123)

6. Physical explanation

Here, we explain some of our obtained solutions through different kind of graphs. The effect of fractional order is also shown through the graphs.

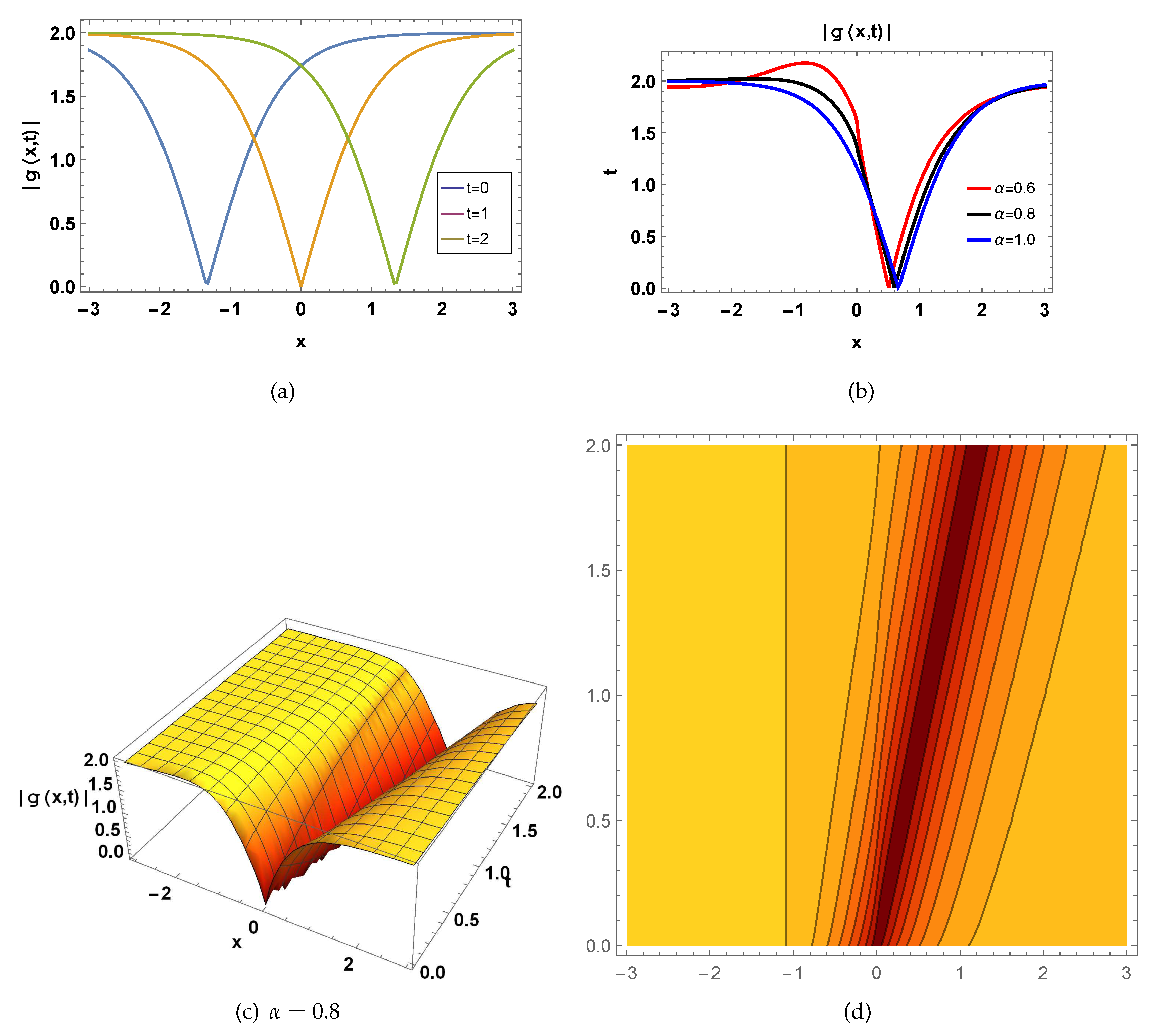
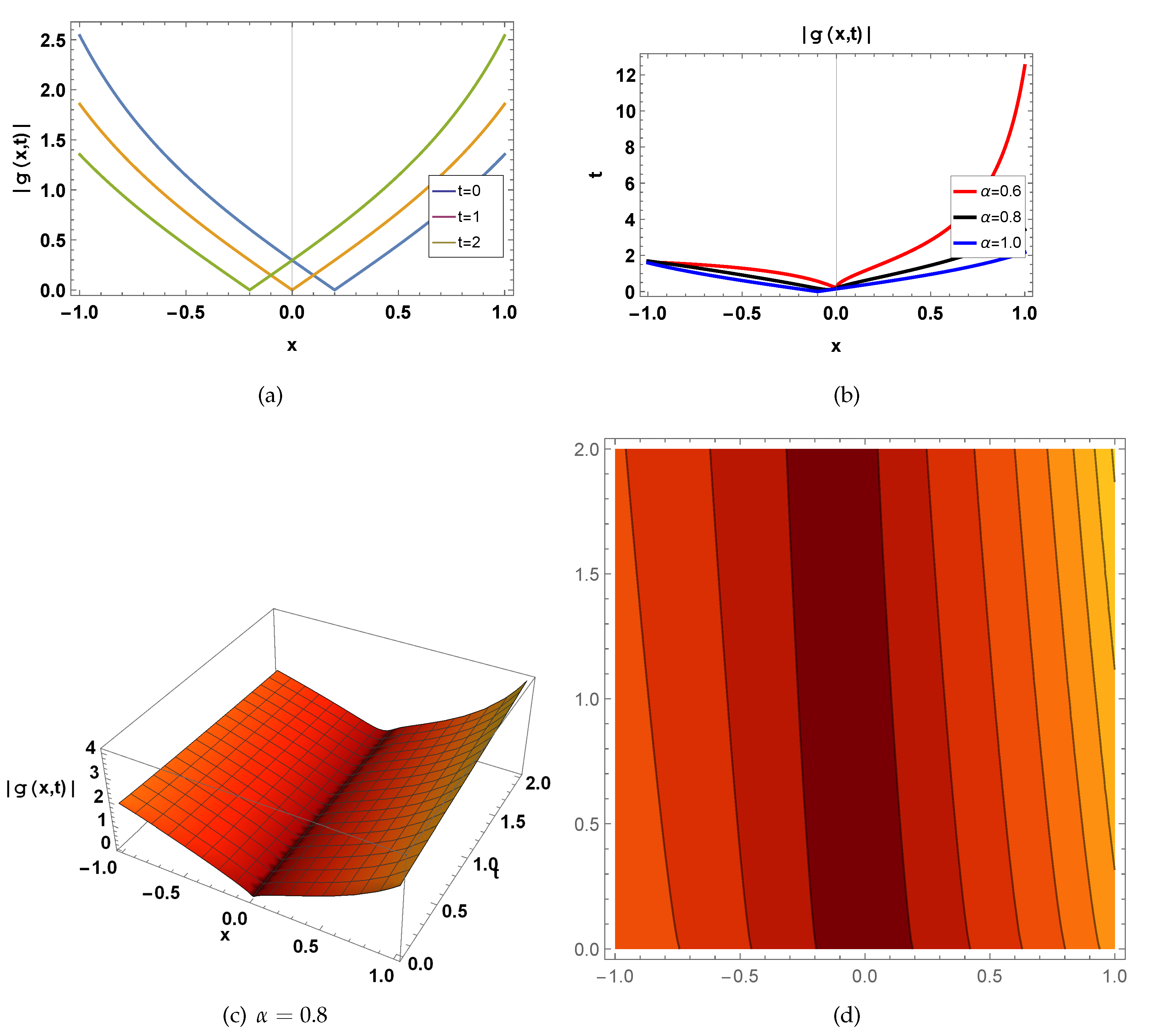
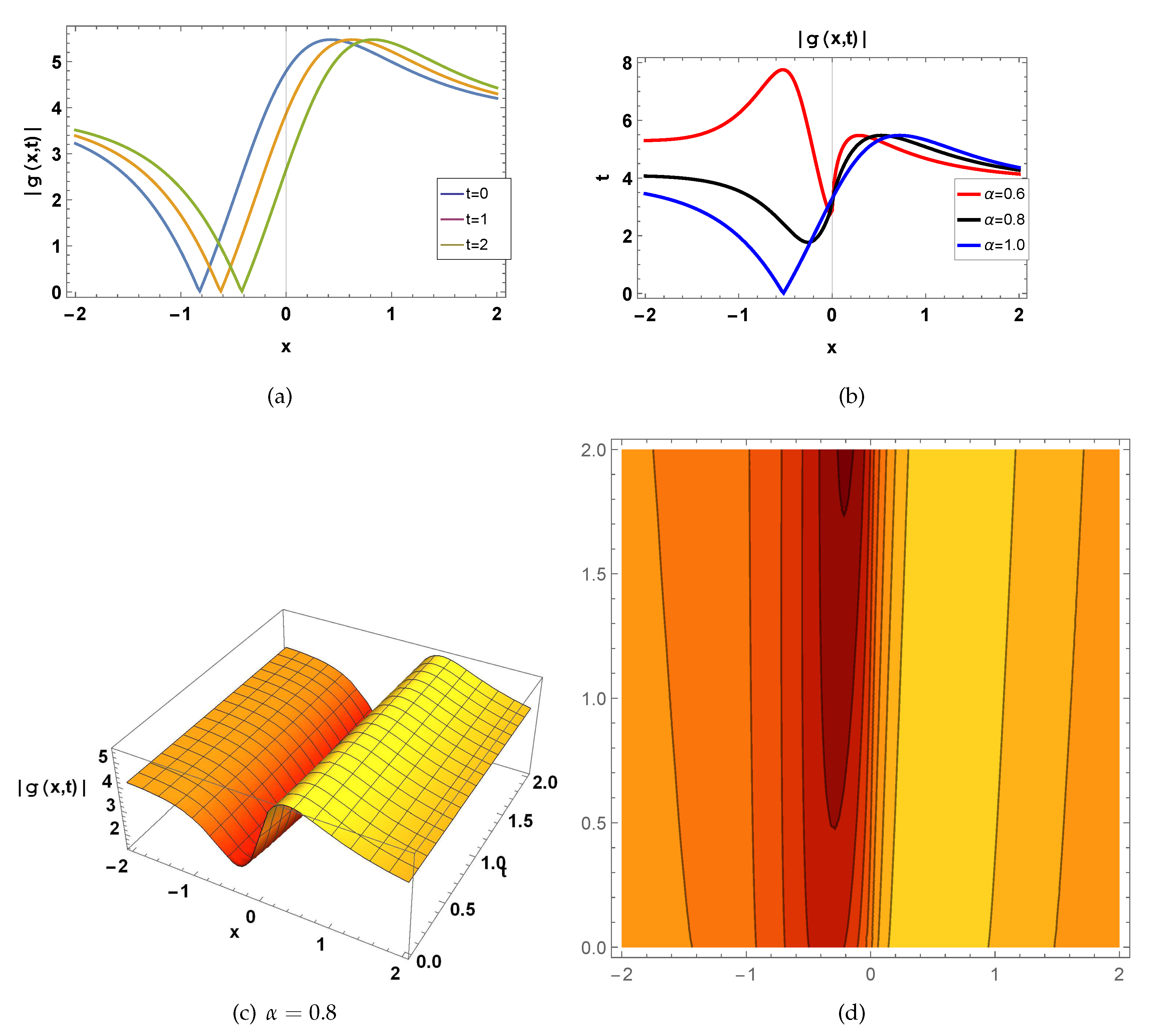
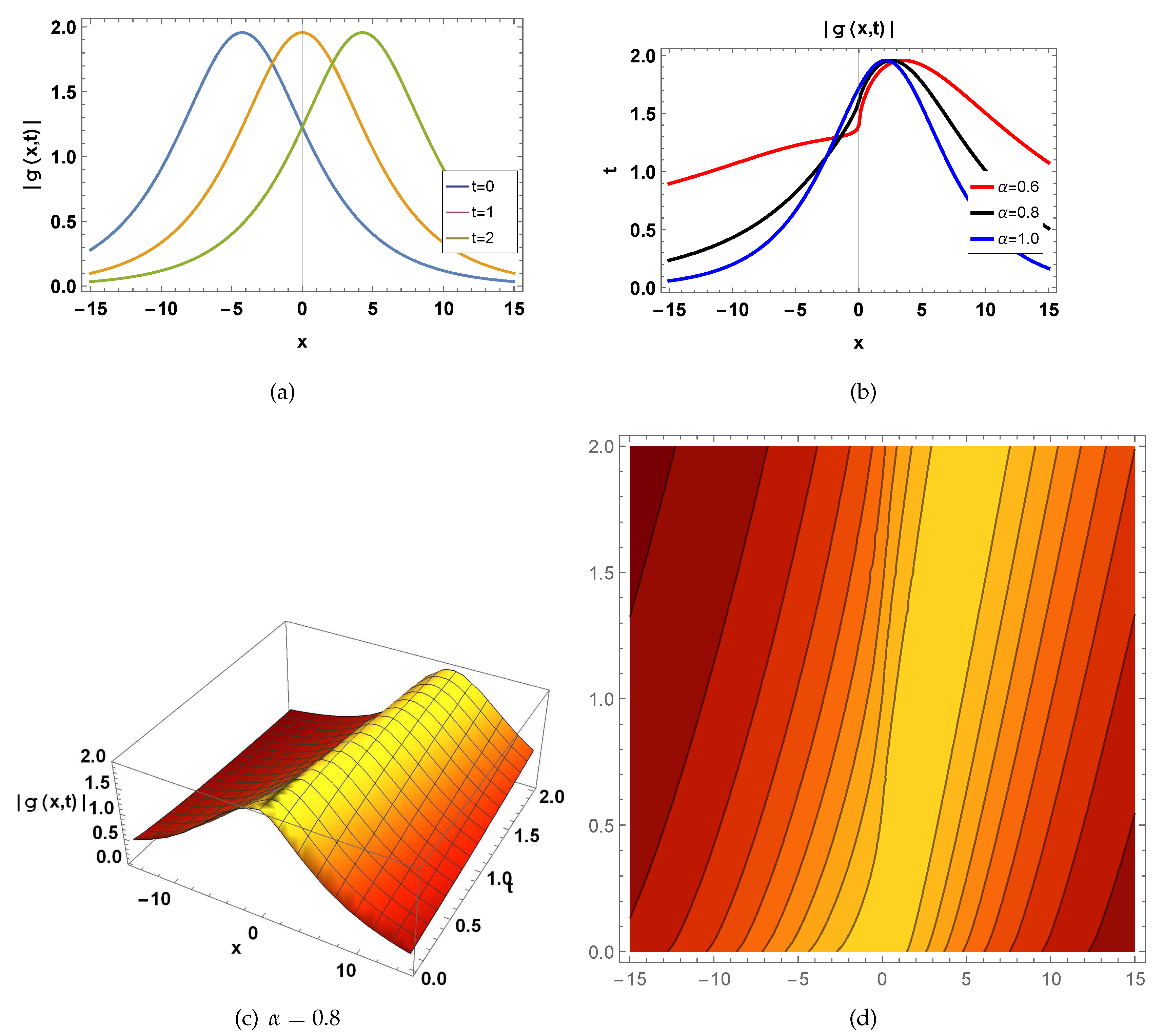
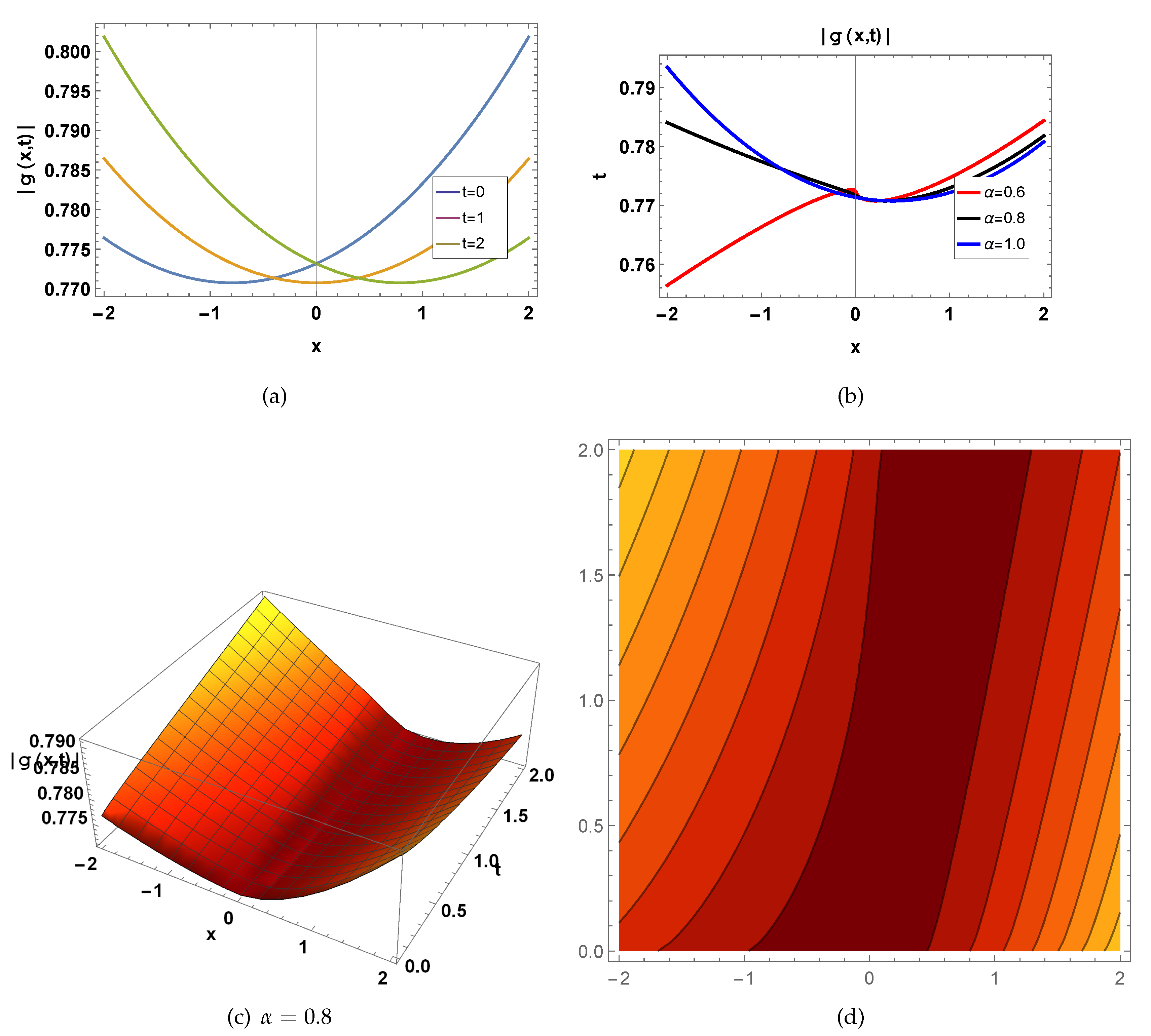
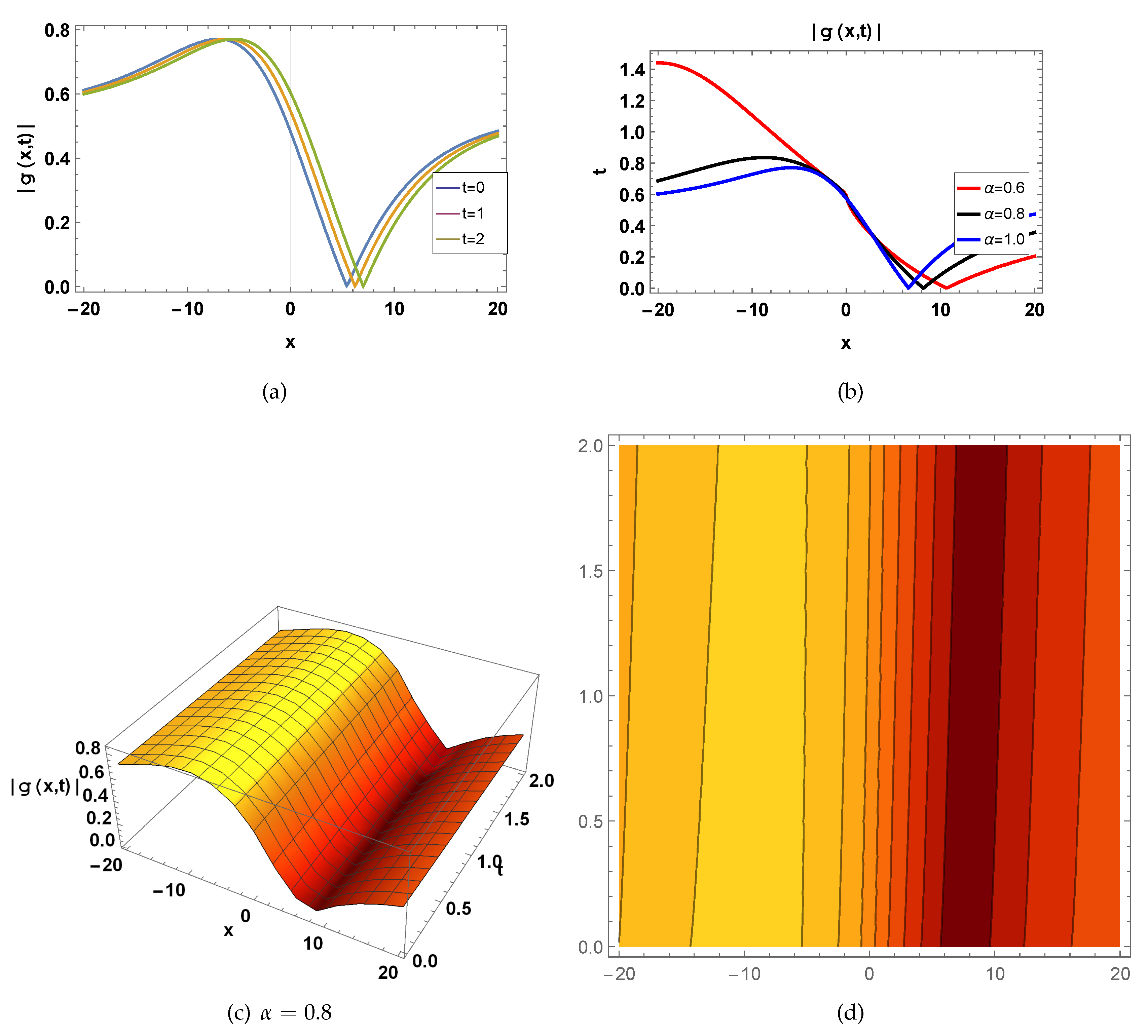
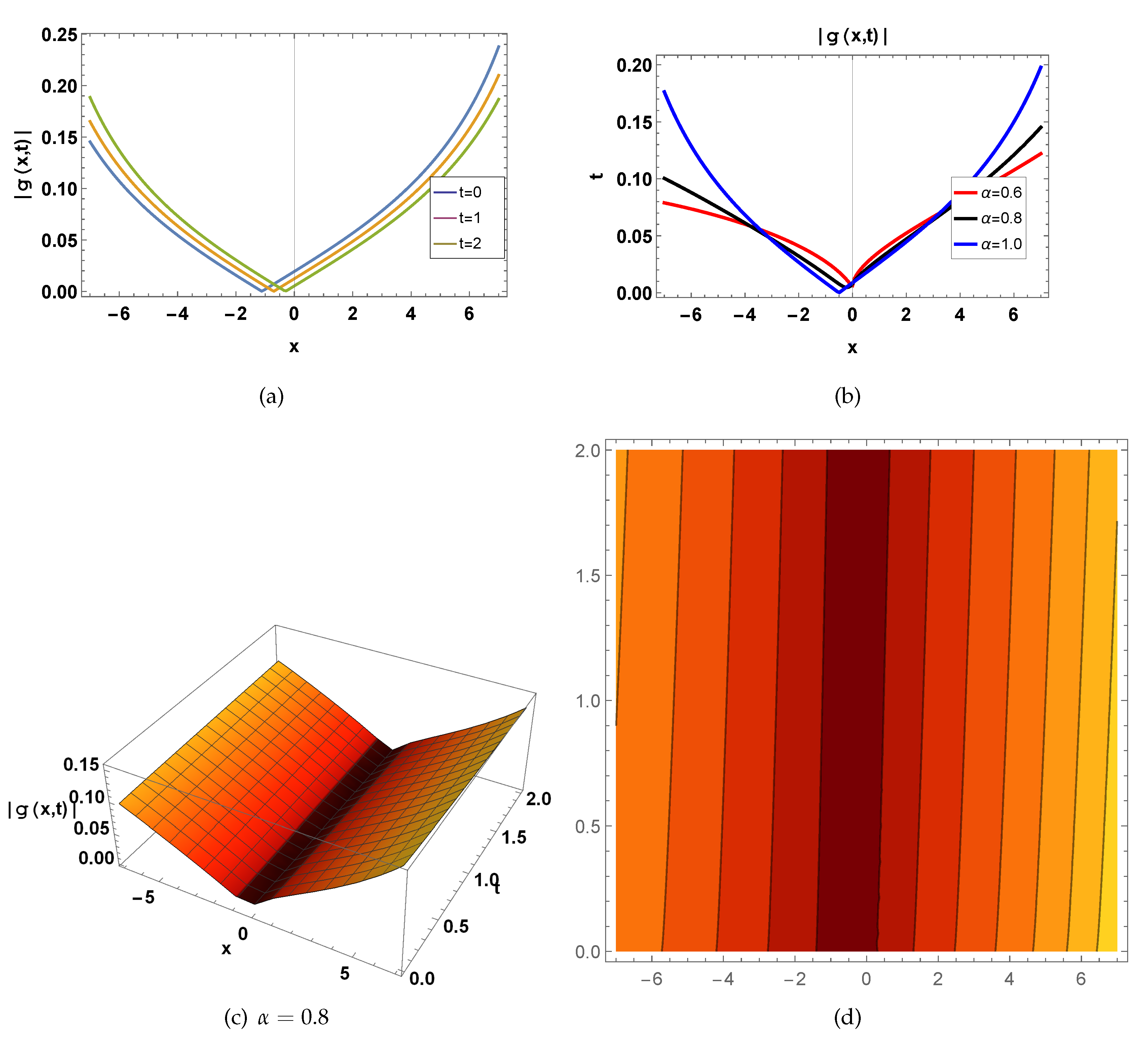
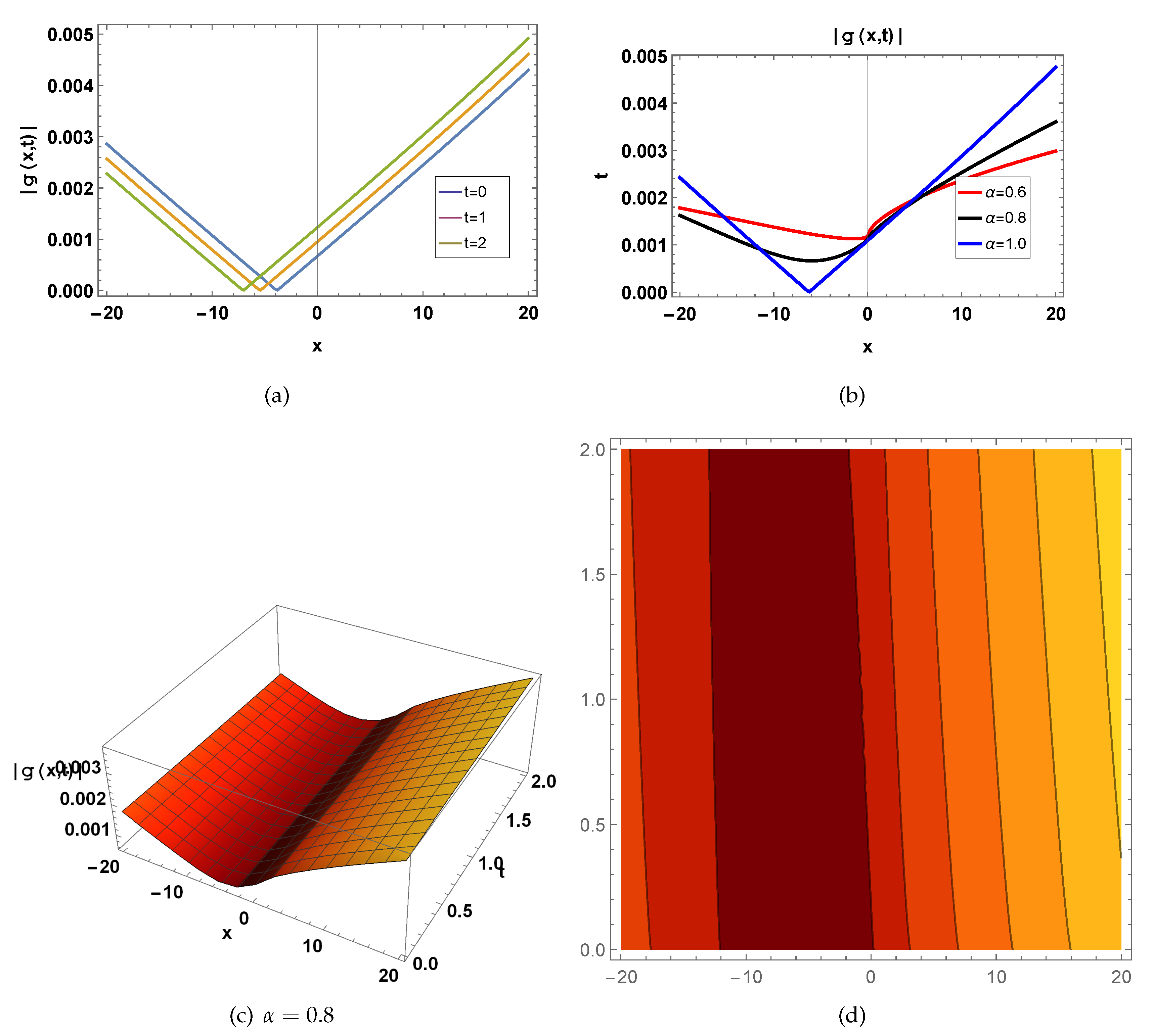
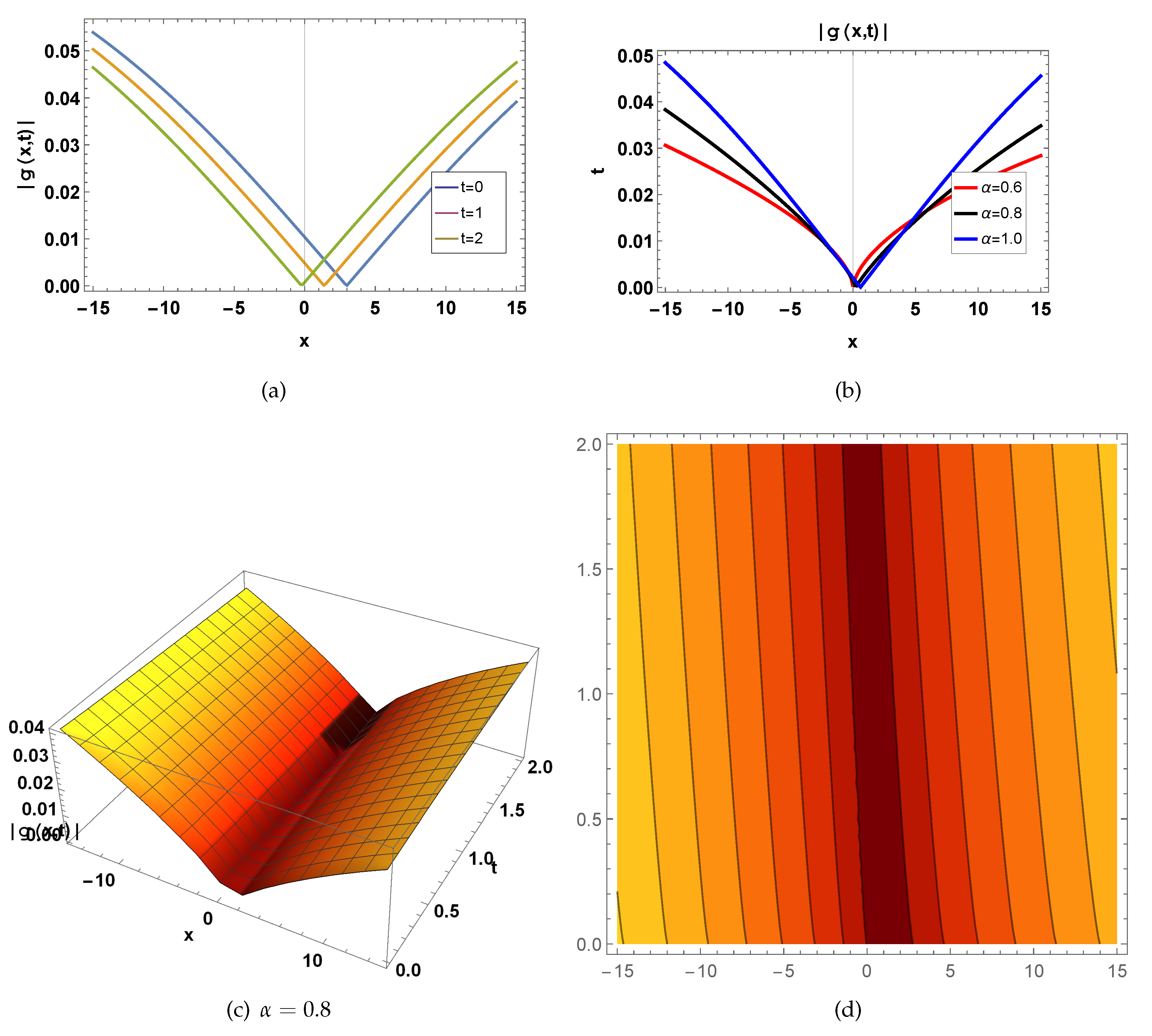
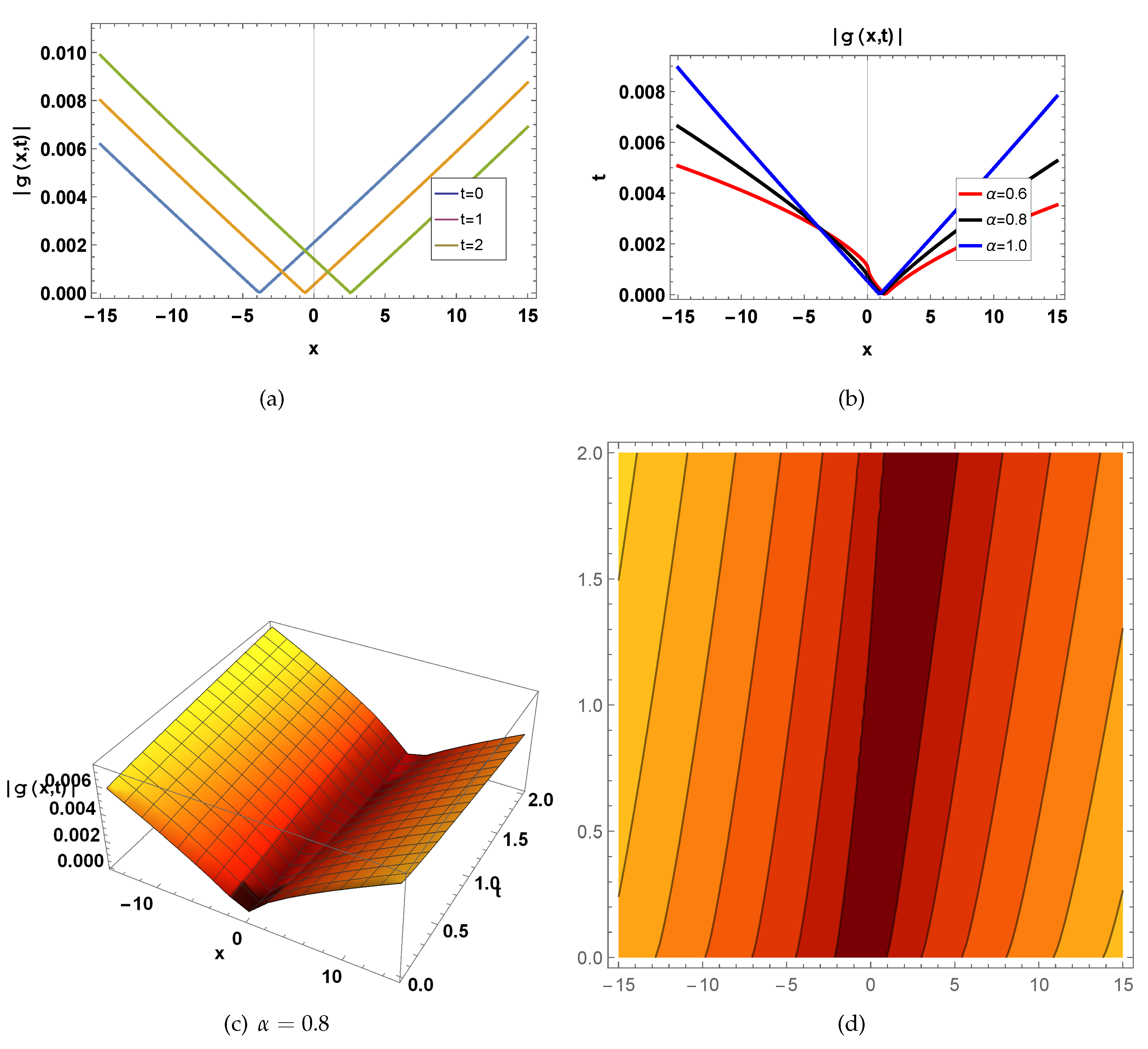
Figure 1. represents a wave function of $\left|g\right(x,t\left)\right|$ shown in Eq.(25) for $\mu =1;\Omega =1;\theta =-1;\omega =-1;{\rm Y}=1;$, fig(a) $-3<x<3$ in 2D for $\alpha =1$, blue curve draw for $t=0$, orange curve draw for $t=1$, green curve draw for $t=2$, fig(b) $-3<x<3$ in 2D for $0<t<2$, red curve draw for $\alpha =0.6$, black curve draw for $\alpha =0.8$, blue curve draw for $\alpha =1$, fig(c) in 3D for $\alpha =0.8$ and $0<t<2$, and fig(d) in contour for $\alpha =0.8$ and $0<t<2$.

Figure 1. represents a wave function of $\left|g\right(x,t\left)\right|$ shown in Eq.(25) for $\mu =1;\Omega =1;\theta =-1;\omega =-1;{\rm Y}=1;$, fig(a) $-3<x<3$ in 2D for $\alpha =1$, blue curve draw for $t=0$, orange curve draw for $t=1$, green curve draw for $t=2$, fig(b) $-3<x<3$ in 2D for $0<t<2$, red curve draw for $\alpha =0.6$, black curve draw for $\alpha =0.8$, blue curve draw for $\alpha =1$, fig(c) in 3D for $\alpha =0.8$ and $0<t<2$, and fig(d) in contour for $\alpha =0.8$ and $0<t<2$.

Figure 2. represents a wave function of $\left|g\right(x,t\left)\right|$ shown in Eq.(30) for $\mu =1;\Omega =0.01;\theta =0.1;\omega =0.6;{\rm Y}=1;$, fig(a) $-1<x<1$ in 2-D for $\alpha =1$, blue curve draw for $t=0$, orange curve draw for $t=1$, green curve draw for $t=2$, fig(b) $-1<x<1$ in 2-D for $0<t<2$, red curve draw for $\alpha =0.6$, black curve draw for $\alpha =0.8$, blue curve draw for $\alpha =1$, fig(c) in 3-D for $\alpha =0.8$ and $0<t<2$, and fig(d) in contour for $\alpha =0.8$ and $0<t<2$.

Figure 2. represents a wave function of $\left|g\right(x,t\left)\right|$ shown in Eq.(30) for $\mu =1;\Omega =0.01;\theta =0.1;\omega =0.6;{\rm Y}=1;$, fig(a) $-1<x<1$ in 2-D for $\alpha =1$, blue curve draw for $t=0$, orange curve draw for $t=1$, green curve draw for $t=2$, fig(b) $-1<x<1$ in 2-D for $0<t<2$, red curve draw for $\alpha =0.6$, black curve draw for $\alpha =0.8$, blue curve draw for $\alpha =1$, fig(c) in 3-D for $\alpha =0.8$ and $0<t<2$, and fig(d) in contour for $\alpha =0.8$ and $0<t<2$.

Figure 3. represents a wave function of $\left|g\right(x,t\left)\right|$ shown in Eq.(37) for $\mu =1;\Omega =0.1;\theta =0.02;\omega =-0.5;{\rm Y}=1;$, fig(a) $-2<x<2$ in 2-D for $\alpha =1$, blue curve draw for $t=0$, orange curve draw for $t=1$, green curve draw for $t=2$, fig(b) $-2<x<2$ in 2-D for $0<t<2$, red curve draw for $\alpha =0.6$, black curve plotted at $\alpha =0.8$, blue curve draw for $\alpha =1$, fig(c) in 3-D for $\alpha =0.8$ and $0<t<2$, and fig(d) in contour for $\alpha =0.8$ and $0<t<2$.

Figure 3. represents a wave function of $\left|g\right(x,t\left)\right|$ shown in Eq.(37) for $\mu =1;\Omega =0.1;\theta =0.02;\omega =-0.5;{\rm Y}=1;$, fig(a) $-2<x<2$ in 2-D for $\alpha =1$, blue curve draw for $t=0$, orange curve draw for $t=1$, green curve draw for $t=2$, fig(b) $-2<x<2$ in 2-D for $0<t<2$, red curve draw for $\alpha =0.6$, black curve plotted at $\alpha =0.8$, blue curve draw for $\alpha =1$, fig(c) in 3-D for $\alpha =0.8$ and $0<t<2$, and fig(d) in contour for $\alpha =0.8$ and $0<t<2$.

Figure 4. represents a wave function of $\left|g\right(x,t\left)\right|$ shown in Eq.(69) for $\mu =1;\Omega =1;\theta =0.2;\kappa =0.06;{\rm Y}=1;$, fig(a) $-15<x<15$ in 2-D for $\alpha =1$, blue curve draw for $t=0$, orange curve plotted at $t=1$, green curve draw for $t=2$, fig(b) $-15<x<15$ in 2-D for $0<t<2$, red curve draw for $\alpha =0.6$, black curve draw for $\alpha =0.8$, blue curve draw for $\alpha =1$, fig(c) in 3-D for $\alpha =0.8$ and $0<t<2$, and fig(d) in contour for $\alpha =0.8$ and $0<t<2$.

Figure 4. represents a wave function of $\left|g\right(x,t\left)\right|$ shown in Eq.(69) for $\mu =1;\Omega =1;\theta =0.2;\kappa =0.06;{\rm Y}=1;$, fig(a) $-15<x<15$ in 2-D for $\alpha =1$, blue curve draw for $t=0$, orange curve plotted at $t=1$, green curve draw for $t=2$, fig(b) $-15<x<15$ in 2-D for $0<t<2$, red curve draw for $\alpha =0.6$, black curve draw for $\alpha =0.8$, blue curve draw for $\alpha =1$, fig(c) in 3-D for $\alpha =0.8$ and $0<t<2$, and fig(d) in contour for $\alpha =0.8$ and $0<t<2$.

Figure 5. represents a wave function of $\left|g\right(x,t\left)\right|$ shown in Eq.(71) for $\mu =1;\Omega =0.2;\theta =0.04;\kappa =-0.01;{\rm Y}=1;$, fig(a) $-2<x<2$ in 2-D for $\alpha =1$, blue curve draw for $t=0$, orange curve draw for $t=1$, green curve draw for $t=2$, fig(b) $-2<x<2$ in 2-D for $0<t<2$, red curve draw for $\alpha =0.6$, black curve draw for $\alpha =0.8$, blue curve draw for $\alpha =1$, fig(c) in 3-D for $\alpha =0.8$ and $0<t<2$, and fig(d) in contour for $\alpha =0.8$ and $0<t<2$.

Figure 5. represents a wave function of $\left|g\right(x,t\left)\right|$ shown in Eq.(71) for $\mu =1;\Omega =0.2;\theta =0.04;\kappa =-0.01;{\rm Y}=1;$, fig(a) $-2<x<2$ in 2-D for $\alpha =1$, blue curve draw for $t=0$, orange curve draw for $t=1$, green curve draw for $t=2$, fig(b) $-2<x<2$ in 2-D for $0<t<2$, red curve draw for $\alpha =0.6$, black curve draw for $\alpha =0.8$, blue curve draw for $\alpha =1$, fig(c) in 3-D for $\alpha =0.8$ and $0<t<2$, and fig(d) in contour for $\alpha =0.8$ and $0<t<2$.

Figure 6. represents a wave function of $\left|g\right(x,t\left)\right|$ shown in Eq.(75) for $\mu =1;\Omega =0.2;\theta =0.04;\kappa =-0.01;{\rm Y}=1;$, fig(a) $-20<x<20$ in 2-D for $\alpha =1$, blue curve draw for $t=0$, orange curve draw for $t=1$, green curve draw for $t=2$, fig(b) $-20<x<20$ in 2-D for $0<t<2$, red curve draw for $\alpha =0.6$, black curve draw for $\alpha =0.8$, blue curve draw for $\alpha =1$, fig(c) in 3-D with $\alpha =0.8$ and $0<t<2$, and fig(d) in contour for $\alpha =0.8$ and $0<t<2$.

Figure 6. represents a wave function of $\left|g\right(x,t\left)\right|$ shown in Eq.(75) for $\mu =1;\Omega =0.2;\theta =0.04;\kappa =-0.01;{\rm Y}=1;$, fig(a) $-20<x<20$ in 2-D for $\alpha =1$, blue curve draw for $t=0$, orange curve draw for $t=1$, green curve draw for $t=2$, fig(b) $-20<x<20$ in 2-D for $0<t<2$, red curve draw for $\alpha =0.6$, black curve draw for $\alpha =0.8$, blue curve draw for $\alpha =1$, fig(c) in 3-D with $\alpha =0.8$ and $0<t<2$, and fig(d) in contour for $\alpha =0.8$ and $0<t<2$.

Figure 7. represents a wave function of $\left|g\right(x,t\left)\right|$ shown in Eq.(79) for $\mu =1;\Omega =0.1;\theta =0.4;\kappa =0.01;{\rm Y}=1;$, fig(a) $-7<x<7$ in 2-D for $\alpha =1$, blue curve draw for $t=0$, orange curve draw for $t=1$, green curve draw for $t=2$, fig(b) $-7<x<7$ in 2-D for $0<t<2$, red curve draw for $\alpha =0.6$, black curve draw for $\alpha =0.8$, blue curve draw for $\alpha =1$, fig(c) in 3-D for $\alpha =0.8$ and $0<t<2$, and fig(d) in contour for $\alpha =0.8$ and $0<t<2$

Figure 7. represents a wave function of $\left|g\right(x,t\left)\right|$ shown in Eq.(79) for $\mu =1;\Omega =0.1;\theta =0.4;\kappa =0.01;{\rm Y}=1;$, fig(a) $-7<x<7$ in 2-D for $\alpha =1$, blue curve draw for $t=0$, orange curve draw for $t=1$, green curve draw for $t=2$, fig(b) $-7<x<7$ in 2-D for $0<t<2$, red curve draw for $\alpha =0.6$, black curve draw for $\alpha =0.8$, blue curve draw for $\alpha =1$, fig(c) in 3-D for $\alpha =0.8$ and $0<t<2$, and fig(d) in contour for $\alpha =0.8$ and $0<t<2$

Figure 8. represents a wave function of $\left|g\right(x,t\left)\right|$ shown in Eq.(108) for $\mu =0.01;\Omega =-0.4;\theta =1;{\rm Y}=1;a=0.8;b=0.08;c=0.5;d_0=0.8$, fig(a) $-20<x<20$ in 2-D for $\alpha =1$, blue curve draw for $t=0$, orange curve draw for $t=1$, green curve draw for $t=2$, fig(b) $-20<x<20$ in 2-D with $0<t<2$, red curve draw for $\alpha =0.6$, black curve draw for $\alpha =0.8$, blue curve draw for $\alpha =1$, fig(c) in 3-D for $\alpha =0.8$ and $0<t<2$, and fig(d) in contour for $\alpha =0.8$ and $0<t<2$.

Figure 8. represents a wave function of $\left|g\right(x,t\left)\right|$ shown in Eq.(108) for $\mu =0.01;\Omega =-0.4;\theta =1;{\rm Y}=1;a=0.8;b=0.08;c=0.5;d_0=0.8$, fig(a) $-20<x<20$ in 2-D for $\alpha =1$, blue curve draw for $t=0$, orange curve draw for $t=1$, green curve draw for $t=2$, fig(b) $-20<x<20$ in 2-D with $0<t<2$, red curve draw for $\alpha =0.6$, black curve draw for $\alpha =0.8$, blue curve draw for $\alpha =1$, fig(c) in 3-D for $\alpha =0.8$ and $0<t<2$, and fig(d) in contour for $\alpha =0.8$ and $0<t<2$.

Figure 9. represents a wave function of $\left|g\right(x,t\left)\right|$ shown in Eq.(113) for $\mu =1;\Omega =-0.4;\theta =1;{\rm Y}=1;b=0.08;c=0.5;d_0=0.8$, fig(a) $-15<x<15$ in 2-D for $\alpha =1$, blue curve draw for $t=0$, orange curve draw for $t=1$, green curve draw for $t=2$, fig(b) $-15<x<15$ in 2-D for $0<t<2$, red curve draw for $\alpha =0.6$, black curve draw for $\alpha =0.8$, blue curve draw for $\alpha =1$, fig(c) in 3-D for $\alpha =0.8$ and $0<t<2$, and fig(d) in contour with $\alpha =0.8$ and $0<t<2$.

Figure 9. represents a wave function of $\left|g\right(x,t\left)\right|$ shown in Eq.(113) for $\mu =1;\Omega =-0.4;\theta =1;{\rm Y}=1;b=0.08;c=0.5;d_0=0.8$, fig(a) $-15<x<15$ in 2-D for $\alpha =1$, blue curve draw for $t=0$, orange curve draw for $t=1$, green curve draw for $t=2$, fig(b) $-15<x<15$ in 2-D for $0<t<2$, red curve draw for $\alpha =0.6$, black curve draw for $\alpha =0.8$, blue curve draw for $\alpha =1$, fig(c) in 3-D for $\alpha =0.8$ and $0<t<2$, and fig(d) in contour with $\alpha =0.8$ and $0<t<2$.

Figure 10. represents a wave function of $\left|g\right(x,t\left)\right|$ shown in Eq.(119) for $\mu =0.10;\Omega =0.8;\theta =2;{\rm Y}=1;a=0.05;c=0.5;d_0=2$, fig(a) $-15<x<15$ in 2-D for $\alpha =1$, blue curve draw for $t=0$, orange curve draw for $t=1$, green curve draw for $t=2$, fig(b) $-15<x<15$ in 2-D with $0<t<2$, red curve draw for $\alpha =0.6$, black curve draw for $\alpha =0.8$, blue curve draw for $\alpha =1$, fig(c) in 3-D for $\alpha =0.8$ and $0<t<2$, and fig(d) in contour for $\alpha =0.8$ and $0<t<2$.

Figure 10. represents a wave function of $\left|g\right(x,t\left)\right|$ shown in Eq.(119) for $\mu =0.10;\Omega =0.8;\theta =2;{\rm Y}=1;a=0.05;c=0.5;d_0=2$, fig(a) $-15<x<15$ in 2-D for $\alpha =1$, blue curve draw for $t=0$, orange curve draw for $t=1$, green curve draw for $t=2$, fig(b) $-15<x<15$ in 2-D with $0<t<2$, red curve draw for $\alpha =0.6$, black curve draw for $\alpha =0.8$, blue curve draw for $\alpha =1$, fig(c) in 3-D for $\alpha =0.8$ and $0<t<2$, and fig(d) in contour for $\alpha =0.8$ and $0<t<2$.

7. Conclusion

We succeed to contributes to our understanding of the truncated M-fractional (1+1)-dimensional non-linear simplified Modified Camassa-Holm model and provides a useful methods for handling nonlinear fractional partial differential equations. This paper describes the successful application of modified simplest equation, Sardar sub-equation and generalized Kudryashov techniques to explore new type of M-fractional soliton solutions for the truncated M-fractional (1+1)-dimensional non-linear simplified Modified Camassa-Holm model. The obtained solutions are fruitful for further studies of the concerned model. The modified simplest equation, Sardar sub-equation and generalized Kudryashov techniques are shown to be a simple, fruitful, and reliable techniques for handling nonlinear fractional partial differential equations. The solutions are verified and also described graphically through 2-dimensional, 3-dimensional and contour graphs using Mathematica software.