Prerpints.org logo
Preprint
Article

Inverse magnetocaloric effect in Heusler Ni44.4Mn36.2Sn14.9Cu4.5 alloy at low temperatures

Altmetrics

Downloads

170

Views

60

Comments

0

A peer-reviewed article of this preprint also exists.

Submitted:

10 October 2023

Posted:

13 October 2023

You are already at the latest version

Alerts
Abstract
The direct measurements of the magnetocaloric effect were performed in Heusler Ni44.4Mn36.2Sn14.9Cu4.5 alloy at cryogenic temperatures in magnetic fields up to 10 T. The maximum value of the inverse magnetocaloric effect in a field of 10 T was observed ∆Tad = –2.7 K in the vicinity of the 1st order magnetostructural phase transition at T0 = 117 K. The ab initio and Monte Carlo calculations were performed to discuss the effect Cu doping into Ni-Mn-Sn compound on the ground state structural and magnetic properties. It is shown that with increasing Cu content the martensitic transition temperature decreases and the Curie temperature of austenite slightly increases. In general, calculated transition temperatures, magnetization values are correlated well with the experimental ones.
Keywords: 
Subject: Physical Sciences  -   Condensed Matter Physics

1. Introduction

The promising idea of solid-state magnetic cooling (SMC) by means of adiabatic demagnetization of paramagnetic salts was used in the early 1930s to develop new methods to obtain the low temperatures below 1 K [1]. The SMC is based on the magnetocaloric effect (MCE), which is a reversible change in an entropy ΔSiso under isothermal conditions or in a temperature ΔTad under adiabatic conditions of a magnetic material due to the external magnetic field changes [2,3]. The MCE is an efficient tool in the study of magnetic phase transitions (PTs), which is among the most urgent problems in modern solid-state physics. The maximum of the MCE is reached in the vicinity of magnetic PTs [3,4]. The magnetic materials with magnetic PTs in the required range of working temperatures are chosen for SMC. The promising applications of the SMC at low temperatures in particular for the liquefaction of gases such as N2, He, H2 or natural gases have been mentioned in [5,6]. The advantages of such materials for SMC at low temperatures are related to the fact that the magnetic heat capacity increases in the region of the magnetic PT and becomes comparable (and sometimes exceeds) to the heat capacity of the crystal lattice of a solid. This circumstance makes SMC more promising at low temperatures, where the lattice heat capacity of metals is much higher than that at room temperature. It is commonly accepted at present that low (cryogenic) temperatures are temperatures below 120 K [7].
The MCE can be either direct or inverse under a magnetization of a magnetic material [2,3,4]. The direct MCE is a decrease in entropy at isothermal conditions or an increase in temperature at adiabatic conditions. Against, the inverse MCE is a positive isothermal change in entropy or a negative adiabatic change in temperature under magnetization. Both direct and inverse MCE can be used for SMC at low temperatures. The inverse MCE can be useful when it is necessary to rapidly cool an area in the presence of magnetic field., It is sufficed to exert magnetic field on the refrigerant for this purpose. Thus, the refrigerant will cool down, and it will also cool the area of magnetic field. The effect can be used to cool a superconducting magnet and the surrounding liquefied gas [8], which will make it possible to reduce the consumption of liquefied gas.
The rare-earth metals [4] and their intermetallic compounds [9] are the most promising materials for SMC at low temperatures. The magnetostructural PTs in such alloys are normally observed at temperatures above 120 K [2]. Most often they show a direct MCE in the vicinity of the PTs. The inverse MCE is observed in their intermetallic compounds with antiferromagnetic (AFM) ordering: RCu2, R2Fe17, RFe3 (R – heavy rare-earth metal) [9,10], Gd2In [11]. The inverse MCE can also be observed as a result of magnetization rotation upon magnetization of a highly anisotropic single-crystal sample along the hard magnetization axis in RCo5 and Tb–Gd [12,13]. However, the rare earth metals are expensive, and their compounds are breakable and rapidly oxidize on the air, so they are difficult to use in the proposed scheme of the SMC.
Inverse MCE at low temperatures can also be exhibited by Mn-based compounds, for example in Mn5Si3 [14]. Significant inverse MCE can be observed in several ferromagnetic (FM) Heusler alloys based on Ni–Mn–Z (Z = In, Sb, Sn), but usually they show it closer to the room temperatures [15,16,17,18]. Some compositions of the Heusler alloys show the inverse MCE at low temperatures, for example the Ni(-Co)-Mn-Ti Heusler alloys [19]. We investigated the Ni44.4Mn36.2Sn14.9Cu4.5 Heusler alloy with the inverse MCE at low temperature perspective for the SMC and tried to explain its properties theoretically in this paper.

2. Materials and Methods

2.1. Samples Characterization

Initially, the composition of the Heusler alloy was chosen according to [20]. Polycrystalline ingot of the Ni44.4Mn36.2Sn14.9Cu4.5 Heusler alloy was synthesized by the argon arc-melting method from high purity elements. The ingot was remelted seven times for the elimination the chemical segregation of the composition. The obtained ingot had the mass of about 80 g. The samples in the form of the 1 mm thick plates were cut and sealed into evacuated quartz ampoules. As is shown earlier the melting point this alloy is 1313 K [21] due to the temperature of the homogenization annealing was selected as 1133 K. The sealed samples were subjected to homogenization annealing for 24 hours, after that they were quenched in water.
The X-ray diffraction and the X-ray phase analysis was performed on a Rigaku Ultima V X-ray diffractometer using Cu-Kα radiation. The crystal structure of the studied sample at room temperature is cubic (space group Fm-3m) with lattice parameter a0 = 5.991 A.The analysis of the microstructure and elemental composition were carried out on a Vega 3-SBH Tescan scanning electron microscope (SEM) equipped with sensors for the backscattered electrons and the energy-dispersive X-ray (EDX) analysis (Oxford Instruments). The specimens were prepared by electropolishing in an electrolyte with 90 % n-butyl alcohol (C4H9OH) and 10 % HCl. The microstructure of the alloy by SEM is represented by a single-phase state and is shown in Figure 1a. The dark points on the thin section are etching pits. The grains have size in order of 100 μm according to the orientational contrast. The microstructure can be characterized as an equiaxed one in general. The not blurred contrast means that the grain boundaries are high-angle ones. The chemical elements maps of the section area are obtained by EDX analysis and presented in Figure 1b. The distribution of the Ni, Mn, Sn and Cu is uniform, and the areas with localization of the any elements are absent. It can be concluded, that annealing at 1133 K for 24 hours followed by quenching makes possibly to obtain a single-phase state of the sample so within the possibility of EDX analysis.
The magnetic properties of Ni44.4Mn36.2Sn14.9Cu4.5 Heusler alloy samples were studied by standard magnetometry methods (ZFC-FC-FH protocols) using a SQUID magnetometer in low magnetic field of 10 mT (inset in Fig.1a) and in fields of 1 T and 3 T in wide temperature range of 2–400 K (Figure 1a). The alloy demonstrates two magnetic PTs of the 1st and the 2nd orders. The magnetostructural PT of the 1st order from a ferrimagnetic (FiM) martensitic phase to a FM austenitic phase is observed at the cryogenic temperatures. The characteristic start and finish temperatures of the martensite and the austenite states obtained by the tangential method and are denoted as MS = 101 K, MF = 54 K, AS = 70 K, and AF = 118 K, respectively. The temperature hysteresis about 20 K is observed during the heating/cooling process (inset in Fig.1a). The application of high magnetic fields shifts the region of the martensitic phase to lower temperatures with the rate of –2.2 K/T (Figure 1a), which makes it possible to realize the inverse MCE in this alloy in relatively low magnetic fields at cryogenic temperatures. The magnetic PT of the 2nd order is the Curie temperature at TC ≈ 350 K.
Magnetic field dependence of the magnetization of the Ni45.3Mn35.9Sn14.3Cu4.5 Heusler alloy for different temperatures in the vicinity of the magnetostructural PT from 50 to 130 K with increasing and decreasing of the magnetic fields are shown in Figure 2b. The results were obtained in Bitter magnet’s magnetic fields up to 10 T by using an original technique detail described in [22].

2.2. Computational Methods

In order to study the role of Cu addition on the structural and magnetic properties of Ni-Mn-Sn alloy, we also carried out theoretical studies in the framework of ab initio calculations and Monte Carlo (MC) simulations.
First-principles calculations of the ground state properties of the austenitic and martensitic phases of Cu-doped Ni-Mn-Sn alloys were performed using the projector augmented wave (PAW) method implemented in the VASP package [23,24]. The generalized gradient approximation in the formulation of Perdue, Burke, and Ernzerhof [25] was chosen to calculate the exchange-correlation energy. Electron-ion interactions are described by PAW potentials with the electronic configurations as follows: 3p63d84s2 for Ni, 3p63d64s1 for Mn, 3d104s24p2 for Sn, and 3p63d104s1 for Cu. The cutoff energy of plane waves was 450 eV. The Brillouin zone was sampled by a 5×5×4 mesh of k points centered at Gamma point. The convergence criteria for the total energy is 10-6 eV/at. A 2×2×2 supercell of 64 atoms with 8 atom tetragonal unit cell (L10, Im3 ̅m, #139) was considered.
The geometric optimization procedure was carried out for the compounds Ni32-xCuxMn23Sn9 (x = 1, 2, and 3), which correspond to Ni50-xCuxMn35.94Sn14.06 (x = 1.56, 3.12 и 4.69 at.%), where the latter is close to the experimental Ni44.4Cu4.5Mn36.2Sn14.9. Two magnetic configurations were considered: FM, where the Mn1, Mn2 and Ni magnetic moments are parallelly aligned, and FiM, where magnetic moments of Mn2 atoms are reversed with respect to the Mn1 and Ni magnetic moments. Here Mn1 and Mn2 are Mn atoms located in the regular Mn sublattice and Sn sublattice, respectively.
The spin polarized relativistic Korringa-Kohn-Rostoker code implemented in the SPR-KKR package [26] was applied for calculating of the magnetic exchange coupling constants in austenitic and martensitic phase of Ni-Cu-Mn-Sn. The formation of non-stoichiometric compositions was performed in the coherent potential approximation. Brillouin zone integration was performed using a special point method on a 57×57×57 k-grid (4495 k-points) to calculate self-consistent potentials and exchange interaction integrals, respectively. The energy convergence threshold was set to 0.01 mRy.
The temperature dependencies of the magnetization were modeled using the MC method, the Heisenberg Hamiltonian and Metropolis algorithm [27]. The model lattice with periodic boundary conditions is consisted of 5488 atoms (for Ni2MnSn: 2744 Ni atoms, 1372 Mn and Ga atoms) and is obtained by multiplying 7×7×7 times the 16-atom unit cell. To form Ni50-xCuxMn35.94Sn14.06 (x = 1.56, 3.12 и 4.69 at.%), the Mn2 and Cu atoms were randomly distributed at Sn and Ni sites. The number of MC steps per temperature value was 1×105. To achieve thermal equilibrium in the system, the first 104 steps of the MC were discarded. Magnetization averaging was performed for 225 configurations for every 400 MC steps.

3. Results

3.1. Indirect MCE Estimation

The isothermal change of magnetic entropy ΔSiso was obtained from Maxwell's thermodynamic relations (1) by using the magnetization curves with increasing of field from 0 to 10 T and presented in Figure 2b.
Δ S i s o = ∫ 0 H ∂ M Т ,   Н ∂ T H d H .
The maximum value of the magnetic entropy change was ΔSiso = 9.5 J/(kg K) at T = 113 K in μ0H = 10 T (Figure 3a). The ΔSiso values obtained in the vicinity of hysteresis of the magnetostructural PT are irreversible, because reversible MCE values in this region can be obtained by demagnetizing of the sample or by turning on the magnetic field again.
The isothermal heat ∆Q is also an important parameter of the working body of a magnetic refrigerator, it allows to estimate the amount of heat that can be taken from the cooled bath as a result of one ideal cycle of the isothermal magnetization/demagnetization processes. Figure 3b shows the ΔQ values calculated from the ΔSiso values by using Equation (2)
∆Q = –T∆Siso,
The maximum value was ΔQ = 1.06 kJ/kg at T = 113 K in magnetic field of 10 T, which is an order of magnitude lower than the maximum known value for the MnAs compound at room temperature in the same magnetic field [28].
The presence of field hysteresis (Figure 2a) in the vicinity of the magnetostructural PT in the sample’s magnetization/demagnetization cycle leads to irreversible heat release δQ, which was calculated by using the Equation (3)
∂ Q = μ 0 ∮ H d M .
Figure 3b shows the δQ values calculated for different temperatures with the maximal value δQ = 0.09 kJ/kg at T = 110 K. This value is only 8.5% of the ΔQ maximal value, which is made by the Heusler Ni44.4Mn36.2Sn14.9Cu4.5 alloy is interesting for using as a magnetocaloric working body in the temperature range of natural gas liquefaction [8], despite the presence of field hysteresis of the magnetostructural PT.

3.2. Direct MCE Measurements

The extraction method in Bitter magnet’s field up to 10 T was used for direct measurements of the MCE in the Heusler Ni44.4Mn36.2Sn14.9Cu4.5 alloy under adiabatic conditions ∆Tad. This method in details was described in [29]. The studies were carried out in two different regimes: (1) sequential heating of the sample, (2) thermal cycling of the sample. In the first case, the sample temperature was successively raised and ∆Tad was measured at selected temperatures. In the second case, the sample was pre-cooled down to 4.2 K, and then its temperature was raised to the required temperature for measurement. Figure 4 shows the results of measurements of the ∆Тad value in magnetic fields of 1.8 T and 10 T: closed circles – sequential heating, open circles – thermal cycling.
The inverse MCE is observed in the region of low temperatures in a field of 1.8 T in both regimes of measuring (Figure 4). The maximum value of the inverse MCE in a field of 1.8 T was observed in the vicinity of the 1st order PT is ∆Tad = –0.5 K at an initial temperature T0 = 117 K (Figure 4). The inverse MCE increases with increasing of the magnetic field up to 10 T, reaching the value ∆Tad = –2.7 K at T0 = 117 K (Figure 4). It is interesting that direct MCE in a magnetic field of 10 T is observed in the FiM martensite phase and reaching the value ∆Tad = 0.7 K (Figure 4) in the sequential heating regime in the temperature range of 20–60 K.

3.3. Computational Results

We proceed to discuss the results of geometric optimization of the crystal structures of the austenitic and martensitic phases as well as the calculation of magnetic properties for Heusler Ni50-xCuxMn35.94Sn14.06 alloys.
Figure 5 shows the energy landscape E(c/a) of Ni50-xCuxMn35.94Sn14.06 with response to volume-conserving elongations and compressions of the cubic L21 structure along c axis. The results are shown for both FM and FiM solutions (Figure 5a). One can see that the FM solution exhibits only one global cubic minimum at c/a = 1 being 11 meV/atom higher in energy than the FiM one and indicating an instability of FM tetragonal phase. For all compounds, the FiM ordering is energetically preferable compared to the FM one for both cubic austenitic and tetragonal martensitic phases. The global minimum for the FiM tetragonal phase takes place around c/a = 1.25.
A close look at the FiM E(c/a) curves, shown in Figure 5b, reveals that the c/a ratio of tetragonal phase varies slightly from 1.25 to 1.27 with increasing Cu content due to a reduce (an increase) of tetragonal lattice constant at (c), respectively (see Table 1). In addition, it is found that the optimized cubic lattice constant increases slightly with the addition of Cu. This is explained by the slightly larger atomic radius of Cu (r = 1.28 Å) compared to Ni (r = 1.24 Å). It should be noted that partial substitution of Ni by Cu reveals an almost linear decrease in the energy difference ( ∆ E L 2 1 − L 1 0 ) between the FIM cubic and tetragonal structure, as evident from Figure 5b. For the parent compound with x = 0, ∆ E L 2 1 − L 1 0 is calculated to be 7.145 meV/atom, whereas in the case of x = 4.69 at.%, it reduces to 3.89 meV/atom. The decrease in the energy barrier with the Cu content indicates indirectly the reduce of the martensitic transition temperature Tm. The Tm temperature can be estimated from a rough approximation: ∆ E L 2 1 − L 1 0 ≈ k B T m where kB is the Boltzmann constant. According to this expression, Tm ≈ 83 K for Ni50Mn35.94Sn14.06 and 45 K for Ni45.31Cu4.69Mn35.94Sn14.06.
A more correct way to estimate the martensitic transition temperature is to calculate the free energies of the austenitic and martensitic phases. For simplicity of calculations, we considered the lattice contribution to the free energy only, which at low temperatures plays a predominant role compared to the electronic and magnetic contributions:
F T , V = E V + F l a t T , V ,
where E(V) is the ground state energy calculated at T = 0 K, Flat(T,V) is the lattice contribution calculated within the Debye model [30].
In Figure 6, we illustrate the free energies of L21 cubic and L10 tetragonal phases as well as the free energy difference ∆ F = F L 2 1 − F L 1 0 for Ni45.31Cu4.69Mn35.94Sn14.06 alloy as an example. For ∆ F   > 0, the L10 phase (matensite) is more preferable and vica verse for ∆ F   < 0, the L21 phase (austenite) is stable. As evident from the figure, both free energy curves reveal a non-linear behavior with a temperature and intersect with each other at low temperature. The martensitic transition temperature extracted from ∆ F = 0 is about 88 K, which agrees well with the experimental one (85.75 K) for Ni44.4Mn36.2Sn14.9Cu4.5, where Tm is computed via Tm = (Ms + Mf + Af + As)/4. The low Tm temperature suggests that the zero-point vibrational energy ( 9 8 k B Θ D ) plays a predominant role as compared to a vibrational entropy in the Flat term. Thus, Tm is mainly contributed by ∆ E L 2 1 − L 1 0 at T = 0 K and ∆ Θ D L 2 1 − L 1 0 at T > 0 K. For Ni45.31Cu4.69Mn35.94Sn14.06, ∆ Θ D L 2 1 − L 1 0 is 13 K in absolute value. We would like to note that Tm is sensitive to ∆ Θ D L 2 1 − L 1 0 , and an increase in ∆ Θ D L 2 1 − L 1 0 ( Θ D L 2 1 < Θ D L 1 0 ) leads to a reduce in Tm, the austenitic phase becomes stable in the whole temperature range at ∆ Θ D L 2 1 − L 1 0 is about 30 K in absolute value. On the hand, an increase in ∆ Θ D L 2 1 − L 1 0 ( Θ D L 2 1 > Θ D L 1 0 ) shifts Tm to higher temperatures.
Figure 7 shows the pairwise exchange coupling constants Jij as function of distance between i and j atoms for FiM-L21 and FiM-L10 Ni45.31Cu4.69Mn35.94Sn14.06 alloy as an example. We would like to note that magnetic interactions reveal a similar behavior for all compounds under study since Cu atoms are nonmagnetic. For both phases, intra-sublattice Jij constants between Mn atoms (Mn1-Mn1 and Mn2-Mn2) show similar damped oscillatory behavior as a function of the distance between atoms up to d/a ≈ 2, except that Jij (Mn2-Mn2) within the 1st and the 2nd coordination shell of the cubic structure exhibits the AFM character.
The cubic inter-sublattice interactions between the nearest Mn1-Mn2 atoms located at a smaller distance (d/a = 0.5) compared to the nearest pairs Mn1(2)-Mn1(2) (d/a = 2 / 2 ) are characterized by a strong AFM interaction, which gradually decreases with increasing d/a. In contrast to cubic structure, the strongest AFM exchange (≈ - 20 meV) between the four nearest atoms Mn1-Mn2 located in the (110) plane is found for tetragonal structure due to the smallest distance (d/a = 0.5), whereas two next nearest atoms Mn1-Mn2 (d/a ≈ 0.627) strongly interact ferromagnetically with Jij ≈ 11.5 meV. As for the Mn1(2)-Ni interactions, they show similar behavior for both phases, demonstrating the FM exchange only between the nearest neighbors. Generally, the behavior of Jij coupling constants is a similar to those of Ni-Mn-(Ga, In, Sb) alloys reported previously [31,32,33,34].
Let us make a general remark concerning the behavior of the exchange interaction parameters for the remaining compounds in the cubic and tetragonal phases. Since the substitution of Ni by Cu influences a small change in the parameters of crystal structures for both phases, it will also affect weakly the exchange constants between the nearest pairs of Ni Mn1, Mn2 (See Table 2). For cubic structure, a slight enhancement of the FM interactions JMn1(2)-Mn1(2) and JMn1(2)-Ni, as well as weakening of the AFM interaction JMn1-Mn2 with increasing Cu content is observed, which also affects the Curie temperature of austenite (TCA). In the case of tetragonal structure, a weakening of FM and AFM interactions between Ni, Mn1, Mn2 atoms is observed. However, the Curie temperature of martensite TCM increases in a similar way as TCA. The increase in TCM is caused by the weakening of the strong AFM interaction between the nearest Mn1-Mn2, the change of which has a larger contribution to the magnetic energy compared to the change of JMn1(2)-Mn1(2) and JMn1(2)-Ni interactions as a function of Cu content.
Figure 8a shows the T-x phase diagram for Ni50-xCuxMn35.94Sn14.06, which includes the predicted Tm and TC temperatures and experimentally measured values of Tm and TCA. With increasing the Cu doping level, Tm reduces nonlinearly from 133 K (x = 0 at.%) to 88 K (x = 4.69 at.%). This is mainly due to a decrease in the energy barrier ∆ E L 2 1 − L 1 0 and ∆ Θ D L 2 1 − L 1 0 , which have a maximum at x = 0 (see Figure 5 and Table 1). The predicted value of Tm for Ni45.31Cu4.69Mn35.94Sn14.06 is close to the experimental one for Ni44.4Cu4.5Mn36.2Sn14.9. As for Curie temperature, MC simulations and mean-field approximation (MFA) show a similar trend of TCA with the increase in Cu content. Nevertheless, the experimental value of TCA for Ni44.4Cu4.5Mn36.2Sn14.9 is less than the theoretical one by about 100 K.

4. Discussions

Let us next discuss the calculated total magnetic moment μ t o t for Ni45.31Cu4.69Mn35.94Sn14.06 and its relation to the experimental one for Ni44.4Cu4.5Mn36.2Sn14.9. It follows from Figure 6 that the magnetic reference state for both austenite and martensite is FiM one, where all magnetic moments of M n 1 ↑ and M n 2 ↓ are oppositely aligned. As a consequence, the μ t o t is calculated to be almost equal 2.16 and 2.12 μ B or 45.6 and 44.8 emu/g, respectively. The latter value is close to the experimental magnetization of martensite (≈ 53 emu/g). However, there is some discrepancy between the calculated and measured values of μ t o t for austenite. The experiment yields twice larger μ t o t . On the other hand, the calculated value of μ t o t for FM austenite, which is energetically unfavorable, is about 5.94 μ B or 125.6 emu/g is larger than experimental one. The dashed line in Figure 2a are calculated curve (approximation) of the temperature dependence of the magnetization in μ0H = 3T for the sample in austenite phase without a magnetostructural PT. This curve was calculated by using the approach proposed in [35]. The obtained magnetization value is 110 emu/g by using this approach (Figure 2a).
To explain the observed discrepancy, we suggest that not all Mn2 atoms located at the Sn sublattice have antiparallel magnetic moment with respect to Mn1 atoms located at the regular Mn sublattice. To prove this, we additionally preformed CPA calculations of μ t o t for L21-Ni45.31Cu4.69Mn35.94Sn14.06 (or N i 45.31 C u 4.69 M n 25 ↑ M n 10.94 − x ↓ M n x ↑ S n 14.06 ) using SPR-KKR code as function of Mn2 atoms at Sn sites with a parallel magnetic moment to that of Mn1 atoms, as shown in Figure 8b. As can be seen from the figure, μ t o t increases linearly with a fraction of M n 2 ↑ atoms and reaches 5.94 μ B or 125.6 emu/g for 100 % amount of M n 2 ↑ spins aligned parallel to M n 1 ↑ ones (FM order). We find that the experimental value of μ t o t can be reached theoretically if only ≈40 % of Mn2 atoms interact antiferromagnetically with Mn1 atoms. This finding allows to conclude that the experimental sample has some kind of FiM order with the predominant amount of FM ordered Mn atoms.
The inverse MCE at low temperatures in the Heusler Ni33.7Co14.8Mn35.4Ti16.1 alloy show the similar values at low temperatures [19]: maximal ∆Tad = –2.7 K at T0 = 90 K in magnetic field of 20 T. Perhaps this is a physical limit on the value of the inverse MCE for the Heusler alloys with PTs at low temperatures, which is significantly lower than in known alloys with inverse MCE at room temperatures [36].

5. Conclusions

The inverse MCE in the Heusler Ni33.7Co14.8Mn35.4Ti16.1 alloy in the region of low temperatures is observed in magnetic field of 1.8 T in both regimes of measuring: at sequential heating and at thermal cycling. The maximum value of the inverse MCE in the vicinity of the 1st order PT in magnetic field of 1.8 T was observed ∆Tad = –0.5 K at an initial temperature T0 = 117 K. The inverse MCE is reaching up to ∆Tad = –2.7 K at T0 = 117 K with increasing of the magnetic field up to 10 T. The direct MCE in the FiM martensite phase is observed in magnetic field of 10 T and reaching the mximal value ∆Tad = 0.7 K at the sequential heating regime in the temperature range of 20–60 K.
First principles and MC approaches applied to determine the phase diagram for Ni50-xCuxMn35.94Sn14.06 (x = 0, 1.56, 3.12, and 4.69) compounds FM ordered, which are similar in composition with experimental sample. It is shown that the Cu doping leads to decrease in Tm temperature and an increase in TCA temperature. This is mainly due to the slight change in the optimized lattice constants, magnetic exchange interactions and Debye temperatures for austenite and martensite. From the analysis of our computational results, we suggest that the experimental sample exhibits some type of FiM order in the austenite, with a predominance of ferromagnetically ordered Mn atoms. The theory is well confirmed by experiment and will make it possible to predict the transition temperatures, the magnetizations and the MCE properties of Heusler Ni-Mn-Sn family when the part of Sn atoms replaced by Cu.

Author Contributions

Conceptualization, A.P.K., V.G.S.; Methodology, Y.S.K. and V.D.B.; Software, V.V.S.; Formal analysis, A.P.K., Y.S.K. and V.V.S.; Investigation, Y.S.K., A.V.K., A.V.M., R.Y.G, I.I.M., J.C. and V.V.S.; Writing—original draft, A.P.K., Y.S.K., and V.V.S.; Supervision, V.D.B. and V.G.S.; Project administration, V.G.S. All authors of the manuscript contributed equally to data acquisition and analysis, writing, editing and formatting. All authors have read and agreed to the published version of the manuscript.

Funding

The magnetization and MCE measurements were carried out within the framework of RSF project № 20-19-00745-П, https://rscf.ru/project/23-19-45040/. The alloy preparing and the structure analysis were carried out by R.Y.G and I.I.M. within the framework of the state task of IMSP RAS. V.V.S. acknowledges the financial support from the Priority-2030 Program of NUST “MISiS” (grant No. K2-2022-022) (the total energy calculations). V.D.B. acknowledge the Ministry of Science and Higher Education of the Russian Federation within the Russian State Assignment, under Contract 075-01493-23-00 (the phonon calculations).

Data Availability Statement

The data presented in this study are available on request from the corresponding author.

Acknowledgments

In this section, you can acknowledge any support given which is not covered by the author contribution or funding sections. This may include administrative and technical support, or donations in kind (e.g., materials used for experiments).

Conflicts of Interest

The authors declare no conflict of interest.

References

  1. Giauque, W.F.; MacDougall, D.P. Attainment of Temperatures Below 1 Absolute by Demagnetization of Gd2(SO4)3·8H2O. Phys. Rev. 1933, 43, 768. [Google Scholar] [CrossRef]
  2. Koshkid’ko, Yu. S.; Dilmieva, E.T.; Kamantsev, A.P.; Mashirov, A.V.; Cwik, J.; Kol’chugina, N.B.; Koledov, V.V.; Shavrov, V.G. Magnetocaloric Materials for Low-Temperature Magnetic Cooling. J. Commun. Technol. Electr. 2023, 68, 379–388. [Google Scholar] [CrossRef]
  3. Tishin, A.M.; Spichkin, Y.I. The Magnetocaloric Effect and its Applications. Publisher: IOP Publ., Bristol, 2003; 476 p.
  4. Belov, K.P. The Magnetothermal Phenomena in Rare-Earth Magnetics. Publisher: Nauka, Moscow, 1990.
  5. Khovaylo, V.V.; Taskaev, S.V. Magnetic Refrigeration: From Theory to Applications. Encycl. Smart Mater. 2022, 5, 407–417. [Google Scholar]
  6. Zhang, H.; Sun, Y.J.; Niu, E.; Yang, L.H.; Shen, J.; Hu, F.X.; Sun, J.R.; Shen, B.G. Large magnetocaloric effects of RFeSi (R = Tb and Dy) compounds for magnetic refrigeration in nitrogen and natural gas liquefaction. Appl. Phys. Lett. 2013, 103, 202412. [Google Scholar] [CrossRef]
  7. Timmerhaus, K.D.; Reed, R.P. Cryogenic Engineering: Fifty Years of Progress. Publisher: Springer Science & Business Media, New York, 2007.
  8. Suslov, D.A.; Shavrov, V.G.; Koledov, V.V.; Mashirov, A.V.; Terentyev, Yu. A.; Petrov, A.O.; Kamantsev, A.P.; Samvelov, A.V.; Yasev, S.G.; Taskaev, S.V.; Kolesov, K.A. Comparison of thermodynamic efficiency of cryogenic gas and solid-state magnetocaloric cycles. Chel. Phys.-Math. J. 2020, 5, 612–617. [Google Scholar] [CrossRef]
  9. Andreenko, A.S.; Belov, K.P.; Nikitin, S.A.; Tishin, A.M. Magnetocaloric effects in rare-earth magnetic materials. Sov. Phys. Usp. 1989, 32, 649–664. [Google Scholar] [CrossRef]
  10. Zheng, X.Q.; Xu, Z.Y.; Zhang, B.; Hu, F.X.; Shen, B.G. The normal and inverse magnetocaloric effect in RCu2 (R= Tb, Dy, Ho, Er) compounds. J. Magn. Magn. Mater. 2017, 421, 448–452. [Google Scholar] [CrossRef]
  11. Kamantsev, A.P.; Koshkidko, Y.S.; Taskaev, S.V.; Khovaylo, V.V.; Koshelev, A.V.; Cwik, J.; Shavrov, V.G. Inverse Magnetocaloric Effect and Kinetic Arrest Behavior in As-Cast Gd2In at Cryogenic Temperatures. J. Supercond. Nov. Magn. 2022, 35, 2181–2186. [Google Scholar] [CrossRef]
  12. Nikitin, S.A.; Skokov, K.P.; Koshkid’ko, Yu. S.; Pastushenkov, Yu. G.; Ivanova, T.I. Giant Rotating Magnetocaloric Effect in the Region of Spin-Reorientation Transition in the NdCo5 Single Crystal. Phys. Rev. Lett. 2010, 105, 137205. [Google Scholar] [CrossRef]
  13. Nikitin, S.A.; Ivanova, T.I.; Zvonov, A.I.; Koshkid'ko, Yu.S.; Ćwik, J.; Rogacki, K. Magnetization, magnetic anisotropy and magnetocaloric effect of the Tb0.2Gd0.8 single crystal in high magnetic fields up to 14 T in region of a phase transition. Acta Mater. 2018, 161, 331. [Google Scholar] [CrossRef]
  14. Kuznetsov, A.S.; Mashirov, A.V.; Musabirov, I.I.; Mitsiuk, V.I.; Anikin, M.S.; Kamantsev, A.P.; Koledov, V.V.; Shavrov, V.G. Inverse Magnetocaloric Effect in Mn5Si3 Compound. J. Commun. Technol. Electr. 2023, 68, 413–419. [Google Scholar] [CrossRef]
  15. Koshkid'ko, Y.; Cwik, J.; Dilmieva, E.; Pandey, S.; Quetz, A.; Aryal, A.; Dubenko, I.; Ali, N.; Granovsky, A.; Lähderanta, E.; Zhukov, A.; Stadler, S. Inverse magnetocaloric effects in metamagnetic Ni-Mn-In-based alloys in high magnetic fields. J. Alloy. Compd. 2017, 695, 3348–3352. [Google Scholar] [CrossRef]
  16. Dilmieva, E.T.; Koshkid’ko, Yu. S.; Kamantsev, A.P.; Koledov, V.V.; Mashirov, A.V.; Shavrov, V.G.; Khovaylo, V.V.; Lyange, M.V.; Cwik, J.; Gonzalez-Legarreta, L.; Grande, H.B. Research of magnetocaloric effect of Ni-Mn-In-Co Heusler alloys by the direct method in magnetic fields up to 14 T. IEEE Trans. Magn. 2017, 53, 2503705. [Google Scholar] [CrossRef]
  17. Konoplyuk, S.M.; Kokorin, V.V.; Mashirov, A.V.; Kamantsev, A.P.; Koledov, V.V.; Shavrov, V.G.; Koshelev, A.V. Direct measurements of adiabatic temperature change in Ni49.9Mn37.03Sb12.3Fe0.77 alloy due to magnetocaloric effect in the temperature range of martensitic transformation. IEEE Trans. Magn. 2018, 54, 2500204. [Google Scholar] [CrossRef]
  18. Sokolovskii, V.V.; Nachinova, D.V.; Buchel’nikov, V.D.; Dilmieva, E.T.; Koshkidko, Yu. S.; Emelyanova S., M.; Marchenkova, E.B.; Marchenkov, V.V. Magnetic and magnetocaloric properies of Heusler alloys Ni-Mn-Sn with an excess of Mn within the theoreticaland experimental approaches. Chel. Phys.-Math. J. 2020, 5, 493–503. [Google Scholar] [CrossRef]
  19. Beckmann, B.; Koch, D.; Pfeuffer, L.; Gottschall, T.; Taubel, A.; Adabifiroozjaei, E.; Miroshkina, O.N.; Riegg, S.; Niehoff, T.; Kani, N.A.; Gruner, M.E.; Molina-Luna, L.; Skokov, K.P.; Gutfleisch, O. Dissipation losses limiting first-order phase transition materials in cryogenic caloric cooling: A case study on all-d-metal Ni (-Co)-Mn-Ti Heusler alloys. Acta Mater. 2023, 246, 118695. [Google Scholar] [CrossRef]
  20. Elerman, Y.; Dinçer, I.; Yüzüak, E.; Emre, B.; Yüce, S.; Ener, S.; Akarca, B. Ferromanyetik Ni-ve Co-tabanlı Heusler alaşımlarının yapısal, manyetokalorik, ısısal, elektriksel ve spintronik özelliklerinin incelenmesi. Publisher: Ankara Üniversitesi Bilimsel Araştırma Projeleri, 2011, 48 p. http://hdl.handle.net/20.500. 1257. [Google Scholar]
  21. Gaifullin, R.Yu.; Kirilyuk, K.K.; Safarov, I.M.; Musabirov, I.I. Structure of Ni44.4Mn36.2Sn14.9Cu4.5 alloy applicable for thermomechanical treatment. Lett. Mater., 2023, 13(2), 164-170.
  22. Anand, A.; Manjuladevi, M.; Veena, R.K.; Veena, V.S.; Koshkid'ko, Y.S.; Sagar, S. A study on spin memory, nature of magnetic transition, and magnetocaloric effect in Nd0.5Ca0.5MnO3. J. Magn. Magn. Mater. 2021, 528, 167810. [Google Scholar] [CrossRef]
  23. Kresse, G.; Furthmüller, J. Efficient iterative schemes for ab initio total-energy calculations using a plane- wave basis set. Phys. Rev. B. 1996, 54, 11169–11186. [Google Scholar] [CrossRef] [PubMed]
  24. Kresse, G.; Joubert, D. From ultrasoft pseudopo-tentials to the projector augmented-wave method. Phys. Rev. B 1999, 59, 1758–1775. [Google Scholar] [CrossRef]
  25. Perdew, J.P.; Burke, K.; Ernzerhof, M. Generalized Gradient Approximation Made Simple. Phys. Rev. Lett. 1996, 77, 3865–3868. [Google Scholar] [CrossRef]
  26. Ebert, H.; Ködderitzsch, D.; Minár, J. Calculating condensed matter properties using the KKR-Green’s function method-recent developments and applications. Rep. Prog. Phys. 2011, 74, 096501–48. [Google Scholar] [CrossRef]
  27. Landau, D.; Binder, K. A guide to Monte Carlo simulations in statistical physics; Publisher: Cambridge university press, 2021; p. 578. [Google Scholar]
  28. Koshkid'ko, Y.S.; Dilmieva, E.T.; Cwik, J.; Rogacki, K.; Kowalska, D.; Kamantsev, A.P.; Koledov, V.V.; Mashirov, A.V.; Shavrov, V.G.; Valkov, V.I.; Golovchan, A.V.; Sivachenko, A.P.; Shevyrtalov, S.N.; Rodionova, V.V.; Shchetinin, I.V.; Sampath, V. Giant reversible adiabatic temperature change and isothermal heat transfer of MnAs single crystals studied by direct method in high magnetic fields. J. Alloyю Comp. 2019, 798, 810–819. [Google Scholar] [CrossRef]
  29. Koshkid'ko, Y.S.; Ćwik, J.; Ivanova, T.I.; Nikitin, S.A.; Miller, M.; Rogacki, K. Magnetocaloric properties of Gd in fields up to 14 T. J. Magn. Magn. Mater. 2017, 433, 234–238. [Google Scholar] [CrossRef]
  30. Moruzzi, V.L.; Janak, J.F.; Schwarz, K. Calculated thermal properties of metals. Phys. Rev. B 1988, 37, 790–799. [Google Scholar] [CrossRef]
  31. Comtesse, D.; Gruner, M.E.; Ogura, M.; Sokolovskiy, V.V.; Buchelnikov, V.D.; Grünebohm, A.; Arróyave, R.; Singh, N.; Gottschall, T.; Gutfleisch, O.; et al. First-principles calculation of the instability leading to giant inverse magnetocaloric effects. Phys. Rev. B 2014, 89, 184403–6. [Google Scholar] [CrossRef]
  32. Sokolovskiy, V.V.; Buchelnikov, V.D.; Zagrebin, M.A.; Entel, P.; Sahool, S.; Ogura, M. First-principles investigation of chemical and structural disorder in magnetic Ni2Mn1+xSn1−x Heusler alloys. Phys. Rev. B. 2012, 86, 134418–11. [Google Scholar] [CrossRef]
  33. Sokolovskiy, V.; Grünebohm, A.; Buchelnikov, V.; Entel, P. Ab initio and Monte Carlo approaches for the magnetocaloric effect in Co- and In-doped Ni-Mn-Ga Heusler alloys. Entropy. 2014, 16, 4992–5019. [Google Scholar] [CrossRef]
  34. Buchelnikov, V.D.; Sokolovskiy, V.V.; Zagrebin, M.A.; Klyuchnikova, M.A.; Entel, P. First-principles study of the structural and magnetic properties of the Ni45Co5Mn39Sn11 Heusler alloy. J. Magn. Magn. Mater. 2015, 383, 180–185. [Google Scholar] [CrossRef]
  35. Kuz’min, M.D. Landau-type parametrization of the equation of state of a ferromagnet. Phys. Rev. B, 2008, 77, 184431. [Google Scholar] [CrossRef]
  36. Kamantsev, A.P.; Amirov, A.A.; Zaporozhets, V.D.; Gribanov, I.F.; Golovchan, A.V.; Valkov, V.I.; Pavlukhina, O.O.; Sokolovskiy, V.V.; Buchelnikov, V.D.; Aliev, A.M.; Koledov, V.V. Effect of Magnetic Field and Hydrostatic Pressure on Metamagnetic Isostructural Phase Transition and Multicaloric Response of Fe49Rh51 Alloy. Metals. 2023, 13, 956. [Google Scholar] [CrossRef]
Preprints 87426 g001
Figure 1. (а) Microstructure of the Ni44.4Mn36.2Sn14.9Cu4.5 Heusler alloy is obtained by SEM. (b) Maps of the chemical element’s distribution of the section area of the Ni44.4Mn36.2Sn14.9Cu4.5 Heusler alloy are obtained by EDX analysis.
Figure 1. (а) Microstructure of the Ni44.4Mn36.2Sn14.9Cu4.5 Heusler alloy is obtained by SEM. (b) Maps of the chemical element’s distribution of the section area of the Ni44.4Mn36.2Sn14.9Cu4.5 Heusler alloy are obtained by EDX analysis.
Preprints 87426 g001
Preprints 87426 g002
Figure 2. (a) Temperature dependences of the magnetization of the Ni45.3Mn35.9Sn14.3Cu4.5 Heusler alloy in high magnetic fields of 1 and 3 T, inset: in low field of 10 mT. The dashed line are calculated curve (approximation) of the temperature dependence of the magnetization in μ0H = 3 T for the sample in austenite phase (without a magnetostructural PT). (b) Field dependences of the magnetization of the Ni45.3Mn35.9Sn14.3Cu4.5 Heusler alloy at different temperatures from 50 to 130 K are obtained with increasing and decreasing of the field.
Figure 2. (a) Temperature dependences of the magnetization of the Ni45.3Mn35.9Sn14.3Cu4.5 Heusler alloy in high magnetic fields of 1 and 3 T, inset: in low field of 10 mT. The dashed line are calculated curve (approximation) of the temperature dependence of the magnetization in μ0H = 3 T for the sample in austenite phase (without a magnetostructural PT). (b) Field dependences of the magnetization of the Ni45.3Mn35.9Sn14.3Cu4.5 Heusler alloy at different temperatures from 50 to 130 K are obtained with increasing and decreasing of the field.
Preprints 87426 g002
Preprints 87426 g003
Figure 3. (а) Temperature dependence of the isothermal entropy changes ΔSiso in the magnetic field μ0H = 10 T for the Heusler Ni44.4Mn36.2Sn14.9Cu4.5 alloy. (b) Temperature dependences of the isothermal heat ΔQ and the irreversible magnetization losses δQ in μ0H = 10 T for the Heusler Ni44.4Mn36.2Sn14.9Cu4.5 alloy.
Figure 3. (а) Temperature dependence of the isothermal entropy changes ΔSiso in the magnetic field μ0H = 10 T for the Heusler Ni44.4Mn36.2Sn14.9Cu4.5 alloy. (b) Temperature dependences of the isothermal heat ΔQ and the irreversible magnetization losses δQ in μ0H = 10 T for the Heusler Ni44.4Mn36.2Sn14.9Cu4.5 alloy.
Preprints 87426 g003
Preprints 87426 g004
Figure 4. Temperature dependences of the adiabatic temperature change ∆Tad for the Ni44.4Mn36.2Sn14.9Cu4.5 Heusler alloy, measured in magnetic fields of 1.8 T (green curves) and 10 T (violet curves) in two different regimes: the solid circles – during sequential heating of the sample, the open circles – after pre-cooling down to 4.2 K and subsequent heating to the measurement temperature.
Figure 4. Temperature dependences of the adiabatic temperature change ∆Tad for the Ni44.4Mn36.2Sn14.9Cu4.5 Heusler alloy, measured in magnetic fields of 1.8 T (green curves) and 10 T (violet curves) in two different regimes: the solid circles – during sequential heating of the sample, the open circles – after pre-cooling down to 4.2 K and subsequent heating to the measurement temperature.
Preprints 87426 g004
Preprints 87426 g005
Figure 5. (a) Energy difference as function of the tetragonal c/a ratio for Ni50-xCuxMn35.94Sn14.06 (x = 0, 1.56, 3.12, and 4.69). For all compounds, the energy zero corresponds to the FiM cubic structure. Filled and open symbols denote the FiM and FM solution, respectively. (b) Enlarged scale for the FiM solution. The Inset shows the energy difference between FiM cubic and tetragonal phases as a function of Cu content.
Figure 5. (a) Energy difference as function of the tetragonal c/a ratio for Ni50-xCuxMn35.94Sn14.06 (x = 0, 1.56, 3.12, and 4.69). For all compounds, the energy zero corresponds to the FiM cubic structure. Filled and open symbols denote the FiM and FM solution, respectively. (b) Enlarged scale for the FiM solution. The Inset shows the energy difference between FiM cubic and tetragonal phases as a function of Cu content.
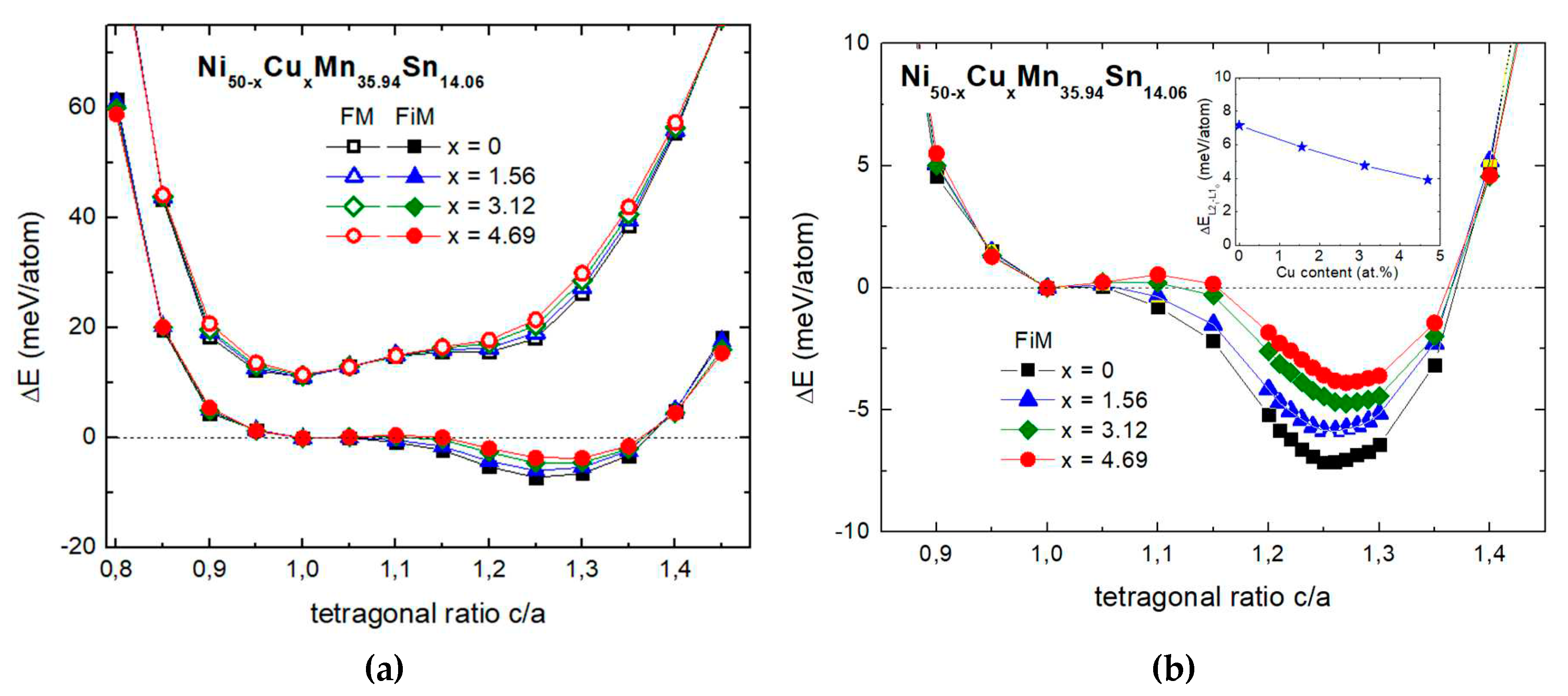
Preprints 87426 g005
Preprints 87426 g006
Figure 6. Temperature dependencies of the free energy of cubic and tetragonal phases of Ni45.31Cu4.69Mn35.94Sn14.06. The inset shows the free energy difference between L21 and L10 structures.
Figure 6. Temperature dependencies of the free energy of cubic and tetragonal phases of Ni45.31Cu4.69Mn35.94Sn14.06. The inset shows the free energy difference between L21 and L10 structures.
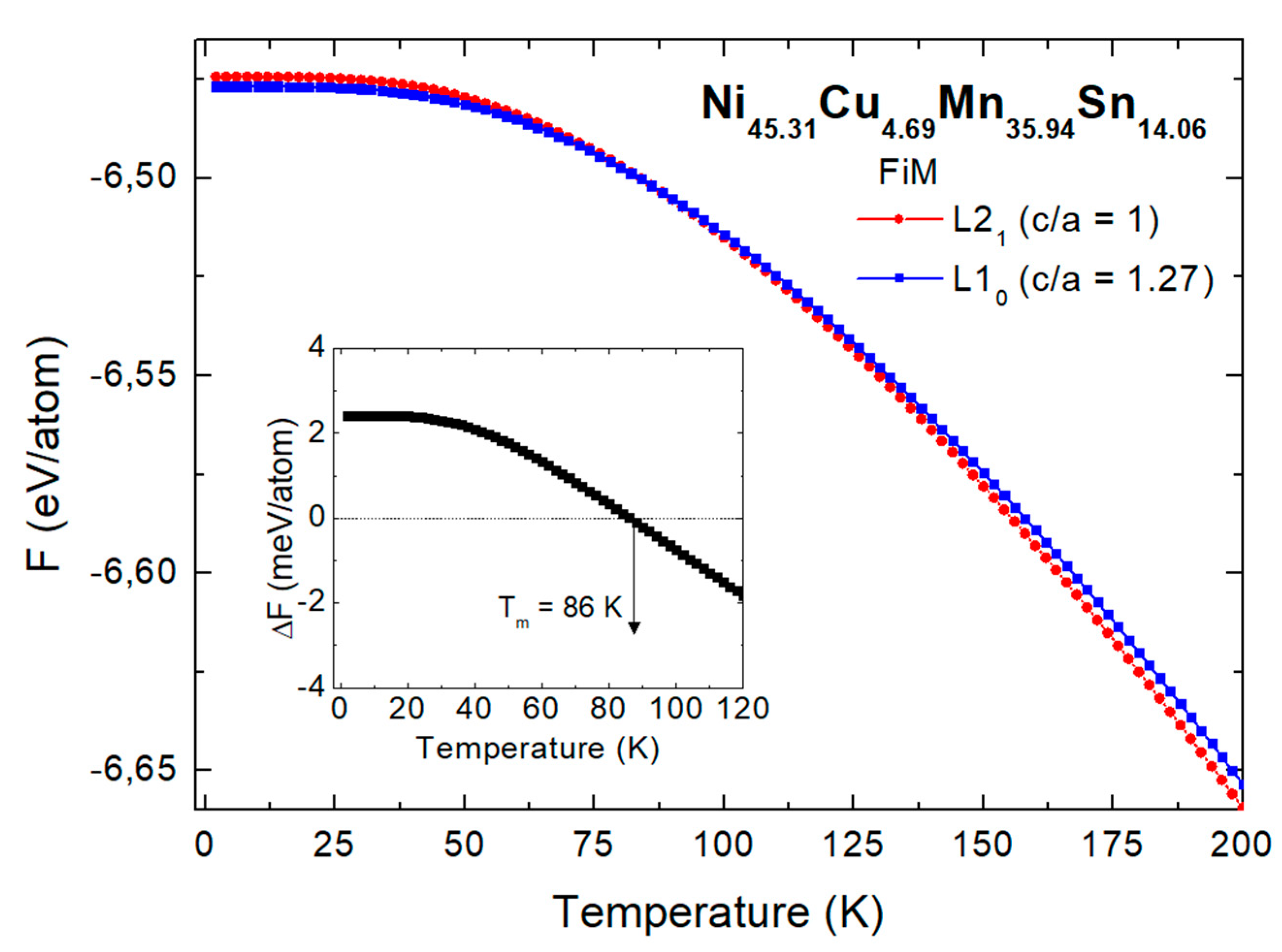
Preprints 87426 g006
Preprints 87426 g007
Figure 7. Calculated magnetic exchange parameters of (a) FiM L21- and (b) L10-Ni45.31Cu4.69Mn35.94Sn14.06 as functions of the distance between the atoms in units of the lattice constant a. Positive Jij denote FM interactions, negative ones denote AFM interactions.
Figure 7. Calculated magnetic exchange parameters of (a) FiM L21- and (b) L10-Ni45.31Cu4.69Mn35.94Sn14.06 as functions of the distance between the atoms in units of the lattice constant a. Positive Jij denote FM interactions, negative ones denote AFM interactions.
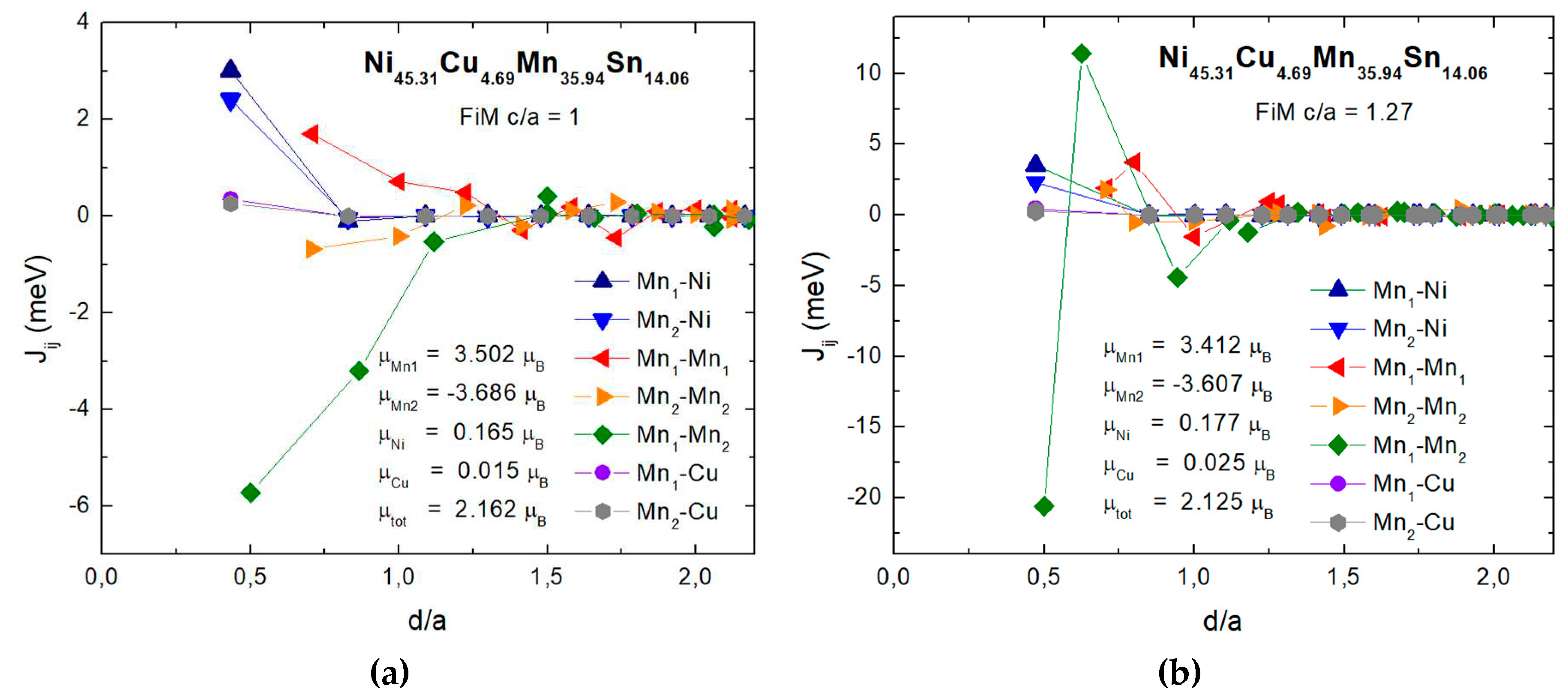
Preprints 87426 g007
Preprints 87426 g008
Figure 8. (a) Calculated phase diagram for Ni50-xCuxMn35.94Sn14.06 (x = 0, 1.56, 3.12, and 4.69). Here, Tm ( ∆ E ) and Tm ( ∆ F ) are the martensitic transformation temperatures calculated from energy difference at T = 0 K, which has been converted to a temperature scale, and from free energy difference considering only lattice contribution, respectively; TCA and TCM are the Curie temperatures of austenite and martensite calculated by MC method and MFA. The experimental values of Tm and TCA refer to Ni44.4Mn36.2Sn14.9Cu4.5, Tm is computed via Tm = (Ms + Mf + Af + As)/4. (b) The calculated total magnetic moment (filled symbol) of Ni45.31Cu4.69Mn35.94Sn14.06 with the cubic structure as a function of Mn2 atoms at Sn sites with a parallel magnetic moment to that of Mn1 atoms. The experimental magnetization for austenitic phase of Ni44.4Mn36.2Sn14.9Cu4.5 is highlighted by the dash line.
Figure 8. (a) Calculated phase diagram for Ni50-xCuxMn35.94Sn14.06 (x = 0, 1.56, 3.12, and 4.69). Here, Tm ( ∆ E ) and Tm ( ∆ F ) are the martensitic transformation temperatures calculated from energy difference at T = 0 K, which has been converted to a temperature scale, and from free energy difference considering only lattice contribution, respectively; TCA and TCM are the Curie temperatures of austenite and martensite calculated by MC method and MFA. The experimental values of Tm and TCA refer to Ni44.4Mn36.2Sn14.9Cu4.5, Tm is computed via Tm = (Ms + Mf + Af + As)/4. (b) The calculated total magnetic moment (filled symbol) of Ni45.31Cu4.69Mn35.94Sn14.06 with the cubic structure as a function of Mn2 atoms at Sn sites with a parallel magnetic moment to that of Mn1 atoms. The experimental magnetization for austenitic phase of Ni44.4Mn36.2Sn14.9Cu4.5 is highlighted by the dash line.
Preprints 87426 g008
Table 1. Optimized lattice parameters and Debye temperatures of cubic and tetragonal structures of Ni50-xCuxMn35.94Sn14.06. The Debye temperatures were calculated from elastic moduli.
Table 1. Optimized lattice parameters and Debye temperatures of cubic and tetragonal structures of Ni50-xCuxMn35.94Sn14.06. The Debye temperatures were calculated from elastic moduli.
Phase Parameters x=0 x=1.56 x=3.12 x=4.69
FiM cubic (c/a = 1) a0 (Å) 5.953 5.955 5.959 5.963
Θ D (K) 316 308 293 282


FiM tetragonal (c/a = 1.27)		 at (Å) 5.526 5.513 5.502 5.500
c (Å) 6.908 6.947 6.988 6.994
c/a 1.25 1.26 1.27 1.27
Θ D (K) 333 325 308 295
Table 2. The nearest exchange coupling constants and Curie temperatures calculated within the MC method and MFA for cubic and tetragonal structures of Heusler Ni50-xCuxMn35.94Sn14.06 alloys.
Table 2. The nearest exchange coupling constants and Curie temperatures calculated within the MC method and MFA for cubic and tetragonal structures of Heusler Ni50-xCuxMn35.94Sn14.06 alloys.
x Mn1-Ni Mn2-Ni Mn1-Mn1 Mn2-Mn2 Mn1-Mn2 TC (MC) TC (MFA)
FiM cubic structure
0 2.961 2.341 1.082 -0.936 -6.065 425 423.4
1.56 2.977 2.364 1.201 -0.837 -5.925 430 433.5
3.12 2.988 2.384 1.492 -0.772 -5.859 452 444.8
4.69 2.999 2.402 1.702 -0.678 -5.722 464 453.1
FiM tetragonal structure
0 3.514 2.308 2.035 1.859 -21.902 484 544.9
1.56 3.502 2.299 1.985 1.826 -21.513 506 547.5
3.12 3.495 2.294 1.942 1.798 -21.071 514 549.6
4.69 3.489 2.287 1.906 1.779 -20.598 523 551.4
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content.
Copyright: This open access article is published under a Creative Commons CC BY 4.0 license, which permit the free download, distribution, and reuse, provided that the author and preprint are cited in any reuse.
Prerpints.org logo

Preprints.org is a free preprint server supported by MDPI in Basel, Switzerland.

facebook logotwitter logolinkedin logo
MDPI Initiatives
Important Links
Subscribe

Disclaimer

Terms of Use

Privacy Policy

© 2024 MDPI (Basel, Switzerland) unless otherwise stated