Preprint
Article
Shannon Entropy of Chemical Elements
Altmetrics
Downloads
269
Views
138
Comments
1
A peer-reviewed article of this preprint also exists.
This version is not peer-reviewed
Submitted:
20 October 2023
Posted:
23 October 2023
Read the latest preprint version here
Alerts
Abstract
It was recently discovered that electron populations within an orbital not only maximize spin multiplicity but also minimize Shannon entropy, which seems to be the physical basis for the Hund rule. This study extends these findings to the Aufbau rule. We observed that some elements that violate the Aufbau rule have the same entropies in actual and Aufbau configurations. On the other hand, a lower entropy of the actual element's configuration is associated with a higher or equal spin multiplicity of this configuration compared to the Aufbau configuration. The only exception to this rule is palladium. It follows from Hund rule that the entries s$^1$, p$^1$-p$^3$, d$^1$-d$^5$ and f$^1$-p$^7$ of the configuration of an element do not contribute to its entropy, but only to the spin multiplicity; entries s$^2$, p$^6$, d$^{10}$, and f$^{14}$ contribute with $\log_b(2)$ to entropy, but do not contribute to spin multiplicity; and the remaining entries contribute both to the entropy and to the spin multiplicity.
Keywords:
Subject: Physical Sciences - Atomic and Molecular Physics
1. Introduction
Hund’s rule of maximum multiplicity is a powerful empirical tool for determining the electron population of electronic shells. It was recently reported [1] that the real driving force behind Hund’s rule appears to be the second law of infodynamics [2]
where in this time derivative is the information entropy proportional to Shannon entropy
of a discrete random variable that attains maximum if the events are equiprobable (i.e., if the probabilities ) and vanishes for certain and impossible events1 In other words, electron populations within an orbital minimize Shannon entropy (2).
2. Hund’s rule and Shannon entropy
A simple but inventive procedure for calculating Shannon entropies of electron populations was disclosed in [1]. Any set of N electrons satisfies , where and denote, respectively, the number of up- and down-spin electrons. Thus, the probabilities of finding ↑ and ↓ electrons within the set of N electrons are and .
Definition 1
(Electron set entropy). An electron set entropy is
This definition was disclosed in [1] but is given here for clarity. Electrons are fermions, so electron sets populating chemical elements’ orbitals must satisfy the Pauli exclusion principle. For the s orbital, for example, which can accommodate a maximum of electrons, the corresponding probabilities are if the orbital accommodates one electron and if the orbital accommodates two electrons. Thus, possible electron set entropies (4) are .
We do not assume any particular logarithm base b, noting that nature uses natural logarithm in Landauer’s principle [18] and Boltzmann/Gibbs physical entropy . Furthermore, the author of [1] assumes that the electron set entropies of states s and s are the same, but this assumption is unjustified, as we shall discuss later (cf. Figure 8).
The situation becomes more diverse for orbitals with a larger angular momentum quantum number ℓ as multiple sets with different electron set entropies and spin multiplicities are possible, as shown in Figure 1, Figure 2, Figure 3 and Figure 4 for orbitals p-g.
However:
- for , ;
- for , only one electron set is possible; and
- for , ;
wherein nature selects this electron set among the ones allowed by the Pauli exclusion principle that maximizes spin multiplicity. However, as shown in [1], for , nature also selects this electron population among the allowed ones, which minimizes the orbital Shannon entropy. This rule of populating the sublevels can be stated in the simple theorem illustrated in Figure 5.
Theorem 1
(Orbital entropy). For any orbital capable of storing , electrons and storing N electrons and populated to maximize spin multiplicity (i.e. according to Hund’s rule), the orbital Shannon entropy vanishes iff and amounts
otherwise.
Proof.
According to Hund’s rule, for the spin multiplicity is equal to , while for is equal to . For electrons can freely populate available sublevels to maximize the spin multiplicity and therefore (the same for ↓). For the electrons will begin to repopulate the available sublevels following the Pauli exclusion principle up to , where , which completes the proof. □
Some researchers postulate that certain elements are exceptions to Hund’s rule. Chromium, for example, having the atomic number is between vanadium (, electron configuration [Ar]3d4s) and manganese (, [Ar]3d4s) within the periodic table of elements. Thus, it should have electron configuration [Ar]3d4s, following Hund’s rule, but instead, it has [Ar]3d4s, as one electron from 4s moves to 3d to make it more stable. But it is not Hund’s rule that is violated. It is the Aufbau rule. Hund’s rule governs the electron population of a solitary orbital only.
3. Aufbau rule and Shannon entropy
The Aufbau or Madelung energy ordering rule is another powerful empirical tool for predicting the electron configurations of chemical elements corresponding to the ground state. It correctly predicts the electron configurations of most of the elements. However, about twenty chemical elements violate the Aufbau rule, leading to intriguing exceptions and anomalies. Chromium and copper violations are attributed to a delicate balance of electron-electron repulsion and the energy gap between the 3d and 4s orbitals. Only palladium exhibits double electron promotion with all ten electrons filling the 4d orbital and therefore is often called a "double anomaly". Palladium also possesses the smallest differential entropy [16]. This exceptional behavior is attributed to the compactness of 3d orbitals and complex electron interactions. In addition, there are no chemical elements that have orbital f in their electron configurations, although the Aufbau rule predicts f8 for gadolinium () as [Xe]+6s+4f and for curium () as [Rn]+7s+5f. Furthermore, there are only two nondoubleton sets of consecutive elements violating the Aufbau rule. We note that for , actual ground states are predicted. Thus, perhaps other elements, such as darmstadtium () and roentgenium () also violate the Aufbau rule.
The elements that violate the Aufbau rule are listed in Table 1, which shows their actual electron configurations and the configurations predicted by the Aufbau rule.
==layoutwidth=297mm,layoutheight=210 mm, left=2.7cm,right=2.7cm,top=1.8cm,bottom=1.5cm, includehead,includefoot [LO,RE]0cm [RO,LE]0cm
==
As the entropies of independent systems are additive quantities, we can calculate the Shannon entropies of chemical elements by summing the orbital entropies of electron configurations using the relation (5).
Definition 2.
An element’s (ground-state) Shannon entropy is the sum of the orbital entropies in the electron configuration of this element, neglecting the principal quantum number.
For example, the electron configuration of oxygen is [He]2s2p→ ssp, where and is given by the relation (5). Thus, the Shannon entropy for oxygen is equal to
Similarly, for chromium .
Shannon entropies and for (Hassium is the heaviest element with known properties) are shown in Figure 6 based on actual ground state configurations and configurations obtained using the Aufbau rule.
Table 1 lists the spin multiplicities of the elements that violate the Aufbau rule, i.e., . Figure 7 shows the spin multiplicities of elements . if Z is even and otherwise.
The Aufbau rule reflects the periodicity of the elements within the windows defined by the noble gases’ atomic numbers, as shown in Figure 7 and Table 2. A simple conclusion of Theorem 1 is that the entries s, p-p, d-d, f-f, etc. of the configuration of an element do not contribute to the entropy of the element, but only to the spin multiplicity. On the other hand, entries s, p, d, f, etc. contribute with to entropy, but do not contribute to spin multiplicity. The remaining entries contribute both to the entropy and to the spin multiplicity.
Definition 3.
The Aufbau core entropy of an element is , where k increases by one from for with every set of elements (cf. Table 2) to for , and so on.
For example, the oxygen core entropy is , while Cr, as an exception to the Aufbau rule, has core entropy .
We can, therefore, calculate Shannon entropy for different numbers of rows of Table 2. Figure 8, for example, shows the entropy for five rows, yielding . This value is well beyond any considerable physical limit, such as Feynmanium (), unoctquadium () or , at which point the Compton wavelength of such an atom becomes smaller than the Planck length [8,13], which is physically implausible because the Planck area is the smallest area required to encode one bit of information [8,19,20,21]. However, Figure 8 illustrates a trend of increasing the entropy of the elements. We conjecture that this trend continues until the element’s half-life is equal to Planck time and for is bounded by (cf. blue curve in the inset of Figure 8).
Figure 8.
Shannon entropy of elements as given by the Hund and Aufbau rules (red), and its approximations (green) and (blue); , . Inset for .
Figure 8.
Shannon entropy of elements as given by the Hund and Aufbau rules (red), and its approximations (green) and (blue); , . Inset for .

4. Conclusions
We observed that the following holds for the 21 chemical elements violating the Aufbau rule:
- for 13 of them, the element’s entropy is lower for the actual and Aufbau configurations; the remaining ones have the same entropies in actual and Aufbau configurations;
- 6 of them have a spin multiplicity different from that predicted by the Aufbau rule, including palladium, the only element with a lower multiplicity;
- the first nondoubleton set contains four elements having lower entropies and the same spin multiplicities.
- the second nondoubleton set contains five elements having the same entropies and spin multiplicities.
Overall, these results significantly strengthen the meaning of the Aufbau rule.
We finally note that the Shannon entropy of hydrogen is zero, not . In emergent dimensionality [7,8,9,10,11,12,13,14], neither one nor two electrons exist. Therefore, s is populated before s not because one electron is less than two electrons, but because the Shannon entropy for one electron is lower than the Shannon entropy for two electrons.
Data Availability Statement
The public repository for the code written in Matlab computational environment is given under the link https://github.com/szluk/Second-Law-of-Infodynamics (accessed on October 17, 2023).
Acknowledgments
I truly thank my wife Magdalena Bartocha for her unwavering support and motivation. I thank my partner and friend, Renata Sobajda, for her prayers. I truly thank my godson, Wawrzyniec Bieniawski, for the state-of-the-art input.
References
- M. M. Vopson, “The second law of infodynamics and its implications for the simulated universe hypothesis,” AIP Advances, vol. 13, p. 105308, Oct. 2023. [CrossRef]
- M. M. Vopson and S. Lepadatu, “Second law of information dynamics,” AIP Advances, vol. 12, p. 075310, July 2022. [CrossRef]
- P. T. de Chardin, The Phenomenon of Man. Harper, New York, 1959.
- I. Prigogine and I. Stengers, Order out of Chaos: Man’s New Dialogue with Nature. Bantam Books, 1984.
- R. Melamede, “Dissipative structures and the origins of life,” in Unifying Themes in Complex Systems IV (A. A. Minai and Y. Bar-Yam, eds.), (Berlin, Heidelberg), pp. 80–87, Springer Berlin Heidelberg, 2008.
- V. Vedral, Decoding Reality: The Universe as Quantum Information. Oxford University Press, 2010.
- S. Łukaszyk, “Four Cubes,” 2020.
- S. Łukaszyk, Black Hole Horizons as Patternless Binary Messages and Markers of Dimensionality, ch. 15, pp. 317–374. Nova Science Publishers, 2023.
- S. Łukaszyk, “Life as the Explanation of the Measurement Problem,” tech. rep., Cornell University, 2018. [CrossRef]
- S. Łukaszyk, “A new concept of probability metric and its applications in approximation of scattered data sets,” Computational Mechanics, vol. 33, pp. 299–304, 2004. [CrossRef]
- S. Łukaszyk, “Novel Recurrence Relations for Volumes and Surfaces of n-Balls, Regular n-Simplices, and n-Orthoplices in Real Dimensions,” Mathematics, vol. 10, no. 13, 2022.
- S. Łukaszyk and A. Tomski, “Omnidimensional Convex Polytopes,” Symmetry, vol. 15, Mar. 2023. [CrossRef]
- S. Łukaszyk, “The Imaginary Universe,” preprint, PHYSICAL SCIENCES, Mar. 2023.
- S. Lukaszyk, “A No-go Theorem for Superposed Actions (Making Schrödinger’s Cat Quantum Nonlocal),” in New Frontiers in Physical Science Research Vol. 3 (D. J. Purenovic, ed.), pp. 137–151, Book Publisher International (a part of SCIENCEDOMAIN International), Nov. 2022. arXiv:1801.08537 [quant-ph].
- D. Bonchev, V. Kamenska, and T. C., “Equations for the Elements in the Periodic Table, Based on Information Theory,” MATCH Communications in Mathematical and in Computer Chemistry, vol. 2, pp. 117–122, 1976.
- D. Bonchev and V. Kamenska, “Information Theory in Describing the Electronic Structures of Atoms,” CROATICA CHEMICA ACTA, vol. 51, pp. 19–27, 1978.
- N. Flores-Gallegos, “Shannon Informational Entropies and Chemical Reactivity,” in Advances in Quantum Mechanics (P. Bracken, ed.), InTech, Apr. 2013.
- R. Landauer, “Irreversibility and Heat Generation in the Computing Process,” IBM Journal of Research and Development, vol. 5, no. 3, pp. 183–191, 1961. [CrossRef]
- J. D. Bekenstein, “Black Holes and Entropy,” Phys. Rev. D, vol. 7, pp. 2333–2346, Apr 1973.
- G. t. Hooft, “Dimensional Reduction in Quantum Gravity,” 1993.
- E. Verlinde, “On the origin of gravity and the laws of Newton,” J. High Energ. Phys., vol. 2011, p. 29, April 2011. [CrossRef]
| 1 | In the latter case is not defined. It is, by convention, taken as 0. |
Figure 1.
Orbital p. , for , p, p, p.
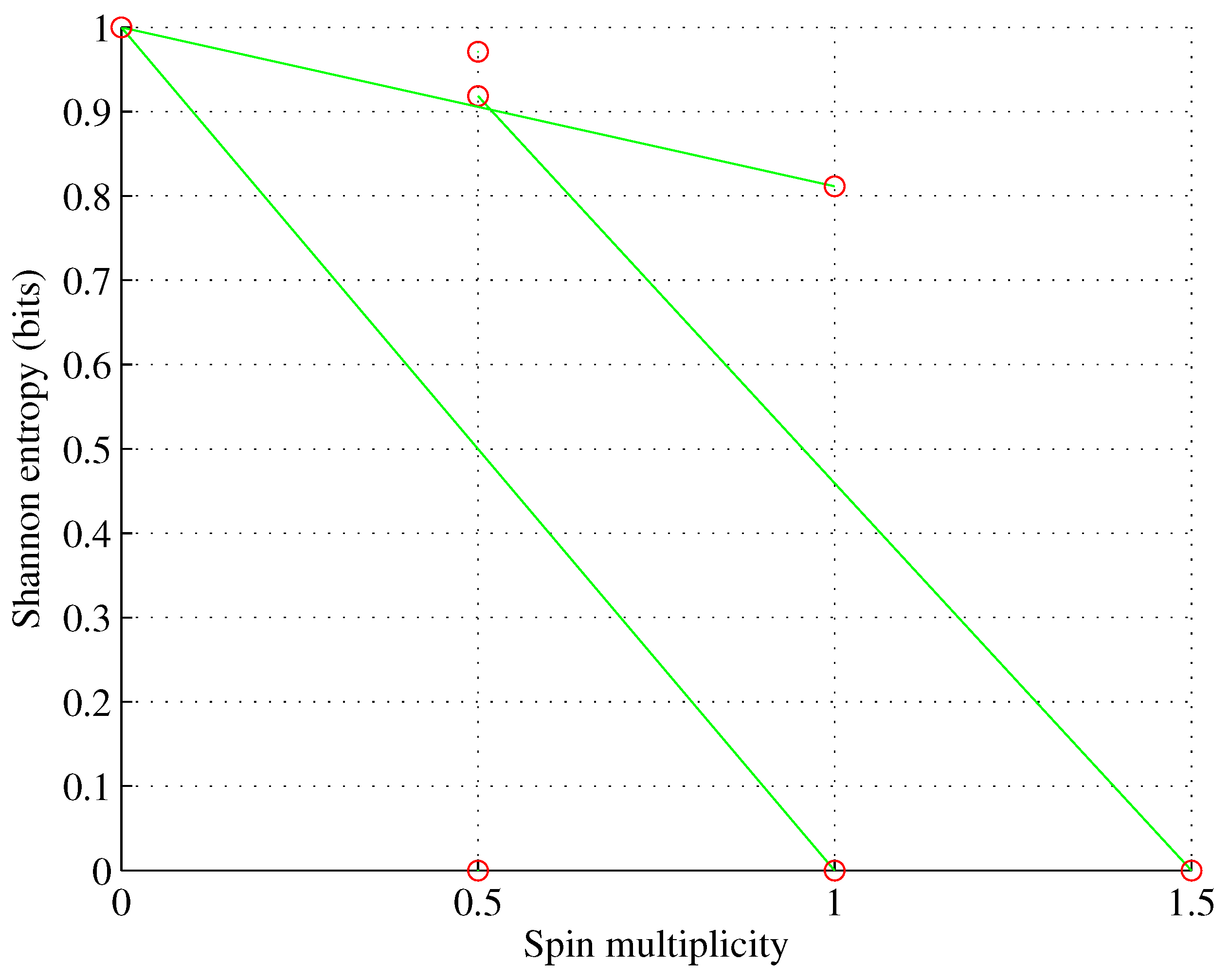
Figure 2.
Orbital d. , for , d.

Figure 3.
Orbital f. , for , f.

Figure 4.
Orbital g. , for , g.

Figure 5.
Orbital g. Spin multiplicity (red, rescaled) and associated orbital entropy (green).

Figure 6.
Shannon entropy of chemical elements (green) showing Aufbau rule violations (red) and elements of the same entropy (blue). Thirteen elements have entropy different than predicted by the Aufbau rule.
Figure 6.
Shannon entropy of chemical elements (green) showing Aufbau rule violations (red) and elements of the same entropy (blue). Thirteen elements have entropy different than predicted by the Aufbau rule.

Figure 7.
Spin multiplicity of chemical elements (green) showing Aufbau rule violations (red) and elements of the same multiplicity (blue). Six elements have a spin multiplicity different from that predicted by the Aufbau rule, including palladium, the only one with a lower multiplicity.
Figure 7.
Spin multiplicity of chemical elements (green) showing Aufbau rule violations (red) and elements of the same multiplicity (blue). Six elements have a spin multiplicity different from that predicted by the Aufbau rule, including palladium, the only one with a lower multiplicity.

Table 1.
Chemical elements violating Aufbau rule.
| Ground state electron configuration | Spin multiplicity | Shannon entropy | |||||
|---|---|---|---|---|---|---|---|
| Z | actual | Aufbau | |||||
| Cr | 24 | [Ar]3d4s | [Ar]3d4s | 3 | 2 | ||
| Ni | 28 | [Ar]3d4s (or [Ar]3d4s) | [Ar]3d4s | 1 | 1 | ||
| Cu | 29 | [Ar]3d4s | [Ar]3d4s | 1/2 | 1/2 | ||
| Nb | 41 | [Kr]4d5s | [Kr]4d5s | 5/2 | 3/2 | ||
| Mo | 42 | [Kr]4d5s | [Kr]4d5s | 3 | 2 | ||
| Ru | 44 | [Kr]4d5s | [Kr]4d5s | 2 | 2 | ||
| Rh | 45 | [Kr]4d5s | [Kr]4d5s | 3/2 | 3/2 | ||
| Pd | 46 | [Kr]4d | [Kr]4d5s | 0 | 1 | ||
| Ag | 47 | [Kr]4d5s | [Kr]4d5s | 1/2 | 1/2 | ||
| La | 57 | [Xe]5d6s | [Xe]4f6s | 1/2 | 1/2 | ||
| Ce | 58 | [Xe]4f5d6s | [Xe]4f6s | 1 | 1 | ||
| Gd | 64 | [Xe]4f5d6s | [Xe]4f6s | 4 | 3 | ||
| Pt | 78 | [Xe]4f5d6s | [Xe]4f5d6s | 1 | 1 | ||
| Au | 79 | [Xe]4f5d6s | [Xe]4f5d6s | 1/2 | 1/2 | ||
| Ac | 89 | [Rn]6d7s | [Rn]5f7s | 1/2 | 1/2 | ||
| Th | 90 | [Rn]6d7s | [Rn]5f7s | 1 | 1 | ||
| Pa | 91 | [Rn]5f6d7s | [Rn]5f7s | 3/2 | 3/2 | ||
| U | 92 | [Rn]5f6d7s | [Rn]5f7s | 2 | 2 | ||
| Np | 93 | [Rn]5f6d7s | [Rn]5f7s | 5/2 | 5/2 | ||
| Cm | 96 | [Rn]5f6d7s | [Rn]5f7s | 4 | 3 | ||
| Lf | 103 | [Rn]5f7s7p | [Rn]5f6d7s | 1/2 | 1/2 | ||
Exceptional configurations have the same entropy and spin multiplicity for italic Z and lower entropy and higher multiplicity for underlined Z. Palladium is the only exception to this relation between entropy and spin multiplicity.
Table 2.
Pattern in the Aufbau rule and element entropies.
| Z | g | f | d | p | g | f | d | p | ||
|---|---|---|---|---|---|---|---|---|---|---|
| 2-17 | 2 | 6 | 2 | 6 | ||||||
| 18-53 | 2 | 6 | 2 | 6 | ||||||
| 54-117 | 2 | 6 | 2 | 6 | ||||||
| 118-217 | 2 | 6 | 2 | 6 |
The subscripts indicate the number of exceptions to the Aufbau rule. The last line shows the continuation of this pattern with orbital g.
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Copyright: This open access article is published under a Creative Commons CC BY 4.0 license, which permit the free download, distribution, and reuse, provided that the author and preprint are cited in any reuse.
MDPI Initiatives
Important Links
© 2024 MDPI (Basel, Switzerland) unless otherwise stated






