Preprint
Article
Shannon Entropy of Chemical Elements
Altmetrics
Downloads
269
Views
138
Comments
1
A peer-reviewed article of this preprint also exists.
This version is not peer-reviewed
Submitted:
16 November 2023
Posted:
17 November 2023
You are already at the latest version
Alerts
Abstract
Hund's rule of maximum multiplicity is a powerful empirical tool for determining the electron population of electronic shells. It was recently discovered that it is also the rule of minimum Shannon entropy. This study extends these findings to the Aufbau rule and discusses them from the perspective of emergent dimensionality, providing a monotonically increasing entropy function. We observed that some elements that violate the Aufbau rule have the same entropies in the actual and Aufbau electron configurations. On the other hand, lower entropies of all actual elements' configurations are associated with higher or equal numbers of unpaired electrons in these configurations compared to the Aufbau configurations. The only exception to this rule is palladium.
Keywords:
Subject: Physical Sciences - Atomic and Molecular Physics
1. Introduction
It was recently reported [1] that the real driving force behind Hund’s rule of maximum spin multiplicity appears to be the second law of infodynamics [2]
where in this time derivative is the information entropy proportional to Shannon entropy
of a discrete random variable that attains maximum if the events are equiprobable (i.e., if the probabilities ) and vanishes for certain and impossible events1 In other words, electron populations within an orbital minimize Shannon entropy (2).
It is now generally accepted [1,2,3,4,5,6,7,8,9,10,11,12,13,14,15,16] that information in the universe evolves, decreasing the information entropy . Assuming that the total entropy of the universe S is constant and is the sum of the information entropy and the physical entropy , we obtain [1]
This study extends the findings of [1] to the Aufbau rule and discusses them from the perspective of emergent dimensionality [5,9,10,11,12,13,14,17]. Other applications of Shannon entropy in chemistry [18,19,20,21,22] and in particular in the context of hydrogen [23,24,25] are known from the state of the art.
2. Hund’s rule and Shannon entropy
A simple but inventive procedure for determining Shannon entropy of an electron population was disclosed in [1]. Any set of N electrons satisfies , where and denote, respectively, the number of up- and down-spin electrons. Thus, the probabilities of finding ↑ and ↓ electrons within the set of N electrons are and .
Definition 1
(Electron set entropy). An electron set entropy is
This definition was disclosed in [1] but is given here for clarity Electrons are fermions, so electron sets populating chemical elements’ orbitals must satisfy the Pauli exclusion principle. For the s orbital, for example, which can accommodate a maximum of electrons, the corresponding probabilities are if the orbital accommodates one electron and if the orbital accommodates two electrons. Thus, possible electron set entropies (4) are . The author of [1] assumes that the electron set entropies of states s1 and s2 are the same, but this assumption is unjustified, as we shall discuss later.
In general, any orbital can store up to
electrons, where is the angular momentum quantum number and n is the principal quantum number. Thus, the situation becomes more diverse for orbitals with larger ℓ as multiple sets with different electron set entropies and numbers of unpaired electrons are possible, as shown in Figure 1, Figure 2, Figure 3 and Figure 4. In general, the number of distinct electron sets allowed by the Pauli exclusion principle and available for an orbital is given by triangular numbers as
and therefore for , only one distinct electron set is possible. If there is more than one electron set available, nature selects this electron set that maximizes the number of unpaired electrons, so the electron set entropy is
However, as shown in [1], for , nature also selects this electron population among the allowed ones, which minimizes the orbital Shannon entropy. This rule of populating the sublevels can be stated in the simple theorem illustrated in Figure 5.
Theorem 1
(Orbital entropy). For any orbital capable of storing (5) electrons, storing N electrons, and populated to maximize the number of unpaired electrons (i.e. according to Hund’s rule), the orbital Shannon entropy amounts
where for we call the entropy intermediate and for pure.
Proof.
According to Hund’s rule, for the number of unpaired electrons is equal to , while for is equal to . For electrons can freely populate available sublevels and therefore (the same for ↓). For the electrons will begin to repopulate the available sublevels following the Pauli exclusion principle up to , where . This completes the proof. □
Some researchers postulate that certain elements are exceptions to Hund’s rule. Chromium, for example, having the atomic number is between vanadium (, electron configuration [Ar]3d34s2) and manganese (, [Ar]3d54s2) within the periodic table of elements. Thus, it should have electron configuration [Ar]3d44s2, following Hund’s rule, but instead, it has [Ar]3d54s1, as one electron from 4s moves to 3d to make it more stable. But it is not Hund’s rule that is violated. It is the Aufbau rule. Hund’s rule governs the electron population of a solitary orbital only.
3. Aufbau rule and Shannon entropy
The Aufbau or Madelung energy ordering rule is another powerful empirical tool for predicting the electron configurations of chemical elements corresponding to the ground state. It correctly predicts the electron configurations of most of the elements. However, about twenty chemical elements (with only two nondoubleton sets of consecutive ones) violate the Aufbau rule, leading to intriguing exceptions and anomalies. Chromium and copper violations are attributed to a delicate balance of electron-electron repulsion and the energy gap between the 3d and 4s orbitals. Only palladium exhibits double electron promotion with all ten electrons filling the 4d orbital and therefore is often called a "double anomaly". Palladium also has the smallest differential entropy [19]. This exceptional behavior is attributed to the compactness of 3d orbitals and complex electron interactions. Furthermore, there are no chemical elements that have orbital f8 in their electron configurations, although the Aufbau rule predicts f8 for gadolinium and curium (). The elements that violate the Aufbau rule are listed in Table 1, which shows their actual electron configurations and the configurations predicted by the Aufbau rule.
We note that for , actual ground states are predicted. Thus, other elements, such as darmstadtium () and roentgenium () may also violate the Aufbau rule. Furthermore, the electron configuration of nickel () is disputed, but we included it in the list of exceptions.
Similarly to orbitals, we can calculate the number of unpaired electrons of a chemical element, as shown in Figure 6 for . We can also calculate the Shannon entropy of a chemical element. In two ways.
First, we can consider an element as an electron set and calculate its entropy using Definition 1, as shown in Figure 7. For example, the electron configuration of oxygen is [He]2s22p4→ 2·s2+p4 and thus , , , and .
However, the entropy according to this definition does not increase monotonically and, in addition, palladium has a lower entropy in the Aufbau configuration. Therefore, noting that the entropies of independent systems are additive quantities, we provide another, monotonically increasing entropy.
Definition 2.
An element’s Shannon entropy is the sum of the orbital entropies (8) in the electron configuration of this element, neglecting the principal quantum number n.
The principal quantum number represents the average distance of electrons from the nucleus, and therefore its meaning in the context of the emergent dimensionality is restricted. Only the Pythagorean theorem introduces negative distances (as non-principal square roots), and distance non-negativity is not an axiom of diffuse metrics [26], such as the Łukaszyk–Karmowski metric [9].
For example, for oxygen is [He]2s22p4→ s2s2p4. Since , and is given by the relation (8), the entropy for oxygen is equal to
and the number of unpaired electrons . Similarly, for chromium () , so its entropy is and the number of unpaired electrons .
Elements’ entropies and for (Hassium is the heaviest element with known properties) are shown in Figure 8 based on actual electron configurations and configurations obtained using the Aufbau rule. Furthermore, Table 1 lists the entropies and numbers of unpaired electrons of the Aufbau rule violators.
The Aufbau rule reflects the periodicity of the elements within the windows defined by the noble gases’ atomic numbers, as shown in Figure 6, Figure 7 and Figure 8. Subsequent atomic orbitals form the Janet’s sequence (OEIS A167268) that can be generated by the relation (5) with given by
where and are floor and ceiling functions2.
The atomic numbers of noble gases form the sequence OEIS A018227 that can be generated using formulas
where is the sum of the elements of a given period of the periodic table (OEIS A093907) that can be generated using formulas
Figure 9.
The periodic table of chemical elements arranged according to their entropy. The Aufbau rule violators (red symbols). Spin-only-contributors (yellow frames). Entropy-only-contributors (green frames). Spin-and-entropy-contributors (blue frames). are exceptional spin-only-contributors. are exceptional spin-and-entropy-contributors having pure entropy. is an exceptional entropy-only-contributor, which is not a spin-contributor.
Figure 9.
The periodic table of chemical elements arranged according to their entropy. The Aufbau rule violators (red symbols). Spin-only-contributors (yellow frames). Entropy-only-contributors (green frames). Spin-and-entropy-contributors (blue frames). are exceptional spin-only-contributors. are exceptional spin-and-entropy-contributors having pure entropy. is an exceptional entropy-only-contributor, which is not a spin-contributor.

However, the sequence (10) is also valid for , as shown in Table 2. In particular for
and thus, 2’s generated by positive l’s are denser, as these l’s include pronic numbers (OEIS A002378) and for . A mirror situation occurs for imaginary , , in which case for
and 2’s generated by negative l’s are denser, as shown in Figure 10.
Figure 10.
Janet’s sequence (red) and its reflection (green).

Furthermore, although the sequences (13) are equivalent to each other their elements no longer represent the sums of the elements of a given period of the periodic table if . Also, the sequences (11) and (12) are different for , as shown in Figure 11.
Figure 11.
The atomic numbers of noble gases generated by the OEIS formula (12) (red) and recurrence formula (11) (blue).

A simple conclusion of Theorem 1 (cf. Figure 5) is that the entries s1, p1-p3, d1-d5, f1-f7, etc. of the configuration of an element do not contribute to the entropy of the element, but only to the number of unpaired electrons; entries s2, p6, d10, f14, etc., contribute with to the entropy but do not contribute to the number of unpaired electrons; and the remaining entries contribute both to entropy and to the number of unpaired electrons. Therefore, we can introduce the following definitions.
Definition 3.
An entropy-only-contributor is the element that has entropy , where and the number of unpaired electrons .
These elements are defined by
where is given by (10) (cf. Figure 6) and l increases from for to for , etc.
Definition 4.
A spin-only-contributor is the element that has the number of unpaired electrons greater than the preceding element and entropy , where .
These elements range between (16) and
Definition 5.
A spin-and-entropy-contributor is the element that has the number of unpaired electrons lower than the preceding element and entropy , where .
These elements range between (17) and (16) and are responsible for soft transitions between the entropy steps shown in Figure 8 and Figure 13.
Thus, for the Aufbau electron configurations each element is either a spin-only-contributor, an entropy-only-contributor, or a spin-and-entropy-contributor. There are only four exceptions to this rule for actual electron configurations, which occur for orbital d, namely which are spin-and-entropy-contributors having entropy equal to . For example, d10 in silver’s configuration [Kr]4d105s1 makes it entropy equal to (krypton has entropy ) and s1 makes it a spin-contributor. Furthermore, palladium is an entropy-only-contributor instead of being a spin-and-entropy-contributor and (orbitals f) are spin-only-contributors instead of being spin-and-entropy-contributors. Furthermore, all Aufbau rule non-singleton violators, have entropies higher or equal than those of the preceding exceptional elements. These results strengthen the meaning of the Aufbau rule.
The fact that hydrogen is a spin-only-contributor, while helium is an entropy-only-contributor suggests a different arrangement of the periodic table of elements shown in Figure 9, where period terminating entropy-only-contributors define the separations of the elements. The outline of the extended periodic table is shown in Figure 12 for . From the 3rd period, the left-hand rows of this periodic table increase by four every two periods , which represents the linear part of the curve.
Figure 12.
The outline of the periodic table of elements arranged to isolate pure-entropy-contributors for (cf. Figure 9).
Figure 12.
The outline of the periodic table of elements arranged to isolate pure-entropy-contributors for (cf. Figure 9).
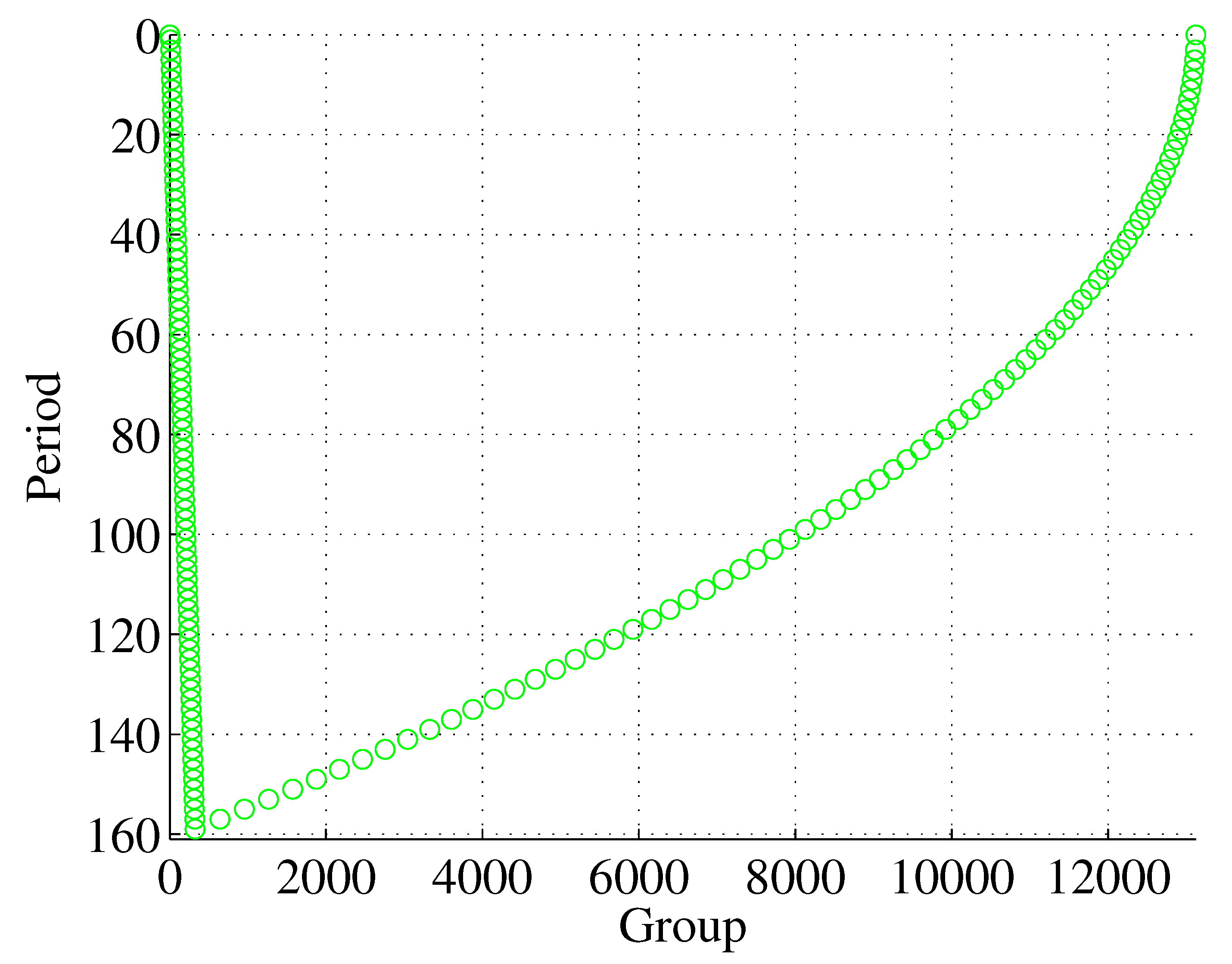
Figure 13.
Shannon entropy of elements as given by Hund’s and Aufbau rules (red), and its approximations (green) and (blue). Inset for .
Figure 13.
Shannon entropy of elements as given by Hund’s and Aufbau rules (red), and its approximations (green) and (blue). Inset for .

Figure 13 shows the element entropies for , yielding . This value is well beyond any considerable physical limit, although elements as heavy as (or even ) are predicted to exist in nature [27] due to doubly-magic nuclei [27,28]. However, the Compton wavelength of an atom becomes smaller than the Planck length for [10,14], which is physically implausible because the Planck area is the smallest area required to encode one bit of information [10,29,30,31]. Figure 13 illustrates a trend of increasing the entropy of the elements. We conjecture that this trend continues until the element’s half-life is equal to Planck time and for is bounded by (cf. the blue curve in the inset of Figure 13).
4. Conclusions
We observed that the following holds for the 21 chemical elements violating the Aufbau rule:
- for 13 of them, the entropy is lower for the actual and Aufbau configurations; the remaining ones have the same entropies in actual and Aufbau configurations;
- 6 of them have the number of unpaired electrons different from that predicted by the Aufbau rule, including palladium, the only element with a lower number of unpaired electrons;
- the first nondoubleton set contains four elements having lower entropies and the same numbers of unpaired electrons with the exception of having lower number of unpaired electrons;
- the second nondoubleton set contains five elements having the same entropies and numbers of unpaired electrons.
Furthermore, for Aufbau electron configurations, each element contributes either to the number of unpaired electrons but not to entropy, to entropy with but not to the number of unpaired electrons, or both to the number of unpaired electrons and to the intermediate entropy, wherein for actual configurations only violate this rule. Overall, these results strengthen the meaning of the Aufbau rule and invite further research. Perhaps the Aufbau rule violators can be explained within the framework of assembly theory [8,15,16].
We finally note that the Shannon entropy H of hydrogen is zero, not . In emergent dimensionality [5,9,10,11,12,13,14,17], neither one nor two electrons exist. Therefore, s1 is populated before s2 not because one electron is less than two electrons, but because the Shannon entropy for one electron is lower than the Shannon entropy for two electrons.
Acknowledgments
I truly thank my godson, Wawrzyniec Bieniawski, for the state-of-the-art input. I thank Andrzej Tomski for finding the analytical formula for the number of distinct electron sets available for an orbital (6). I thank my partner and friend, Renata Sobajda, for her prayers. I truly thank my wife Magdalena Bartocha for her unwavering support and motivation.
Data Availability Statement
The public repository for the code written in MATLAB computational environment is given under the link https://github.com/szluk/Evolution_of_Information (accessed on November 03, 2023).
References
- Vopson, M.M. The second law of infodynamics and its implications for the simulated universe hypothesis. AIP Advances 2023, 13, 105308. [CrossRef]
- Vopson, M.M.; Lepadatu, S. Second law of information dynamics. AIP Advances 2022, 12, 075310. [CrossRef]
- de Chardin, P.T. The Phenomenon of Man; Harper, New York, 1959.
- Prigogine, I.; Stengers, I. Order out of Chaos: Man’s New Dialogue with Nature; Bantam Books, 1984.
- Łukaszyk, S. A new concept of probability metric and its applications in approximation of scattered data sets. Computational Mechanics 2004, 33, 299–304. [CrossRef]
- Melamede, R. Dissipative Structures and the Origins of Life. Unifying Themes in Complex Systems IV; Minai, A.A.; Bar-Yam, Y., Eds.; Springer Berlin Heidelberg: Berlin, Heidelberg, 2008; pp. 80–87.
- Vedral, V. Decoding Reality: The Universe as Quantum Information; Oxford University Press, 2010. [CrossRef]
- Murray, A.; Marshall, S.; Cronin, L. Defining Pathway Assembly and Exploring its Applications 2018. [CrossRef]
- Łukaszyk, S. Life as the Explanation of the Measurement Problem. Technical report, Cornell University, 2018. [CrossRef]
- Łukaszyk, S., Black Hole Horizons as Patternless Binary Messages and Markers of Dimensionality. In Future Relativity, Gravitation, Cosmology; Nova Science Publishers, 2023; chapter 15, pp. 317–374. [CrossRef]
- Łukaszyk, S. Four Cubes, 2020. [CrossRef]
- Łukaszyk, S. Novel Recurrence Relations for Volumes and Surfaces of n-Balls, Regular n-Simplices, and n-Orthoplices in Real Dimensions. Mathematics 2022, 10. [CrossRef]
- Łukaszyk, S.; Tomski, A. Omnidimensional Convex Polytopes. Symmetry 2023, 15. [CrossRef]
- Łukaszyk, S. The Imaginary Universe. preprint, PHYSICAL SCIENCES, 2023.
- Marshall, S.M.; Moore, D.G.; Murray, A.R.G.; Walker, S.I.; Cronin, L. Formalising the Pathways to Life Using Assembly Spaces. Entropy 2022, 24, 884. [CrossRef]
- Sharma, A.; Czégel, D.; Lachmann, M.; Kempes, C.P.; Walker, S.I.; Cronin, L. Assembly theory explains and quantifies selection and evolution. Nature 2023, 622, 321–328. [CrossRef]
- Lukaszyk, S. A No-go Theorem for Superposed Actions (Making Schrödinger’s Cat Quantum Nonlocal). In New Frontiers in Physical Science Research Vol. 3; Purenovic, D.J., Ed.; Book Publisher International (a part of SCIENCEDOMAIN International), 2022; pp. 137–151. arXiv:1801.08537 [quant-ph], . [CrossRef]
- Bonchev, D.; Kamenska, V.; C., T. Equations for the Elements in the Periodic Table, Based on Information Theory. MATCH Communications in Mathematical and in Computer Chemistry 1976, 2, 117–122.
- Bonchev, D.; Kamenska, V. Information Theory in Describing the Electronic Structures of Atoms. CROATICA CHEMICA ACTA 1978, 51, 19–27.
- Angulo, J.C.; Dehesa, J.S. Tight rigorous bounds to atomic information entropies. The Journal of Chemical Physics 1992, 97, 6485–6495. [CrossRef]
- Flores-Gallegos, N. Shannon Informational Entropies and Chemical Reactivity. In Advances in Quantum Mechanics; Bracken, P., Ed.; InTech, 2013. [CrossRef]
- Stahura, F.L.; Godden, J.W.; Bajorath, J. Differential Shannon Entropy Analysis Identifies Molecular Property Descriptors that Predict Aqueous Solubility of Synthetic Compounds with High Accuracy in Binary QSAR Calculations. Journal of Chemical Information and Computer Sciences 2002, 42, 550–558. [CrossRef]
- Jiao, L.G.; Zan, L.R.; Zhang, Y.Z.; Ho, Y.K. Benchmark values of Shannon entropy for spherically confined hydrogen atom. International Journal of Quantum Chemistry 2017, 117, e25375. [CrossRef]
- Martínez-Sánchez, M.A.; Vargas, R.; Garza, J. Shannon Entropy for the Hydrogen Atom Confined by Four Different Potentials. Quantum Reports 2019, 1, 208–218. [CrossRef]
- Toranzo, I.V.; Puertas-Centeno, D.; Sobrino, N.; Dehesa, J.S. Analytical Shannon information entropies for all discrete multidimensional hydrogenic states. International Journal of Quantum Chemistry 2020, 120, e26077. [CrossRef]
- Castro, P.S.; Kastner, T.; Panangaden, P.; Rowland, M. MICo: Improved representations via sampling-based state similarity for Markov decision processes. Advances in Neural Information Processing Systems 2021, 34, 30113–30126. [CrossRef]
- Maly, J.; Walz, D.R. Search for Superheavy Elements Among Fossil Fission Tracks in Zircon 1980.
- Koura, H.; Chiba, S. Single-Particle Levels of Spherical Nuclei in the Superheavy and Extremely Superheavy Mass Region. Journal of the Physical Society of Japan 2013, 82, 014201. [CrossRef]
- Bekenstein, J.D. Black Holes and Entropy. Phys. Rev. D 1973, 7, 2333–2346. [CrossRef]
- Hooft, G.t. Dimensional Reduction in Quantum Gravity, 1993. [CrossRef]
- Verlinde, E. On the origin of gravity and the laws of Newton. J. High Energ. Phys. 2011, 2011, 29. [CrossRef]
| 1 | In the latter case is not defined. It is, by convention, taken as 0. |
| 2 | Or alternatively by OEIS A167268. |
Figure 1.
Orbital p. , for , , , .
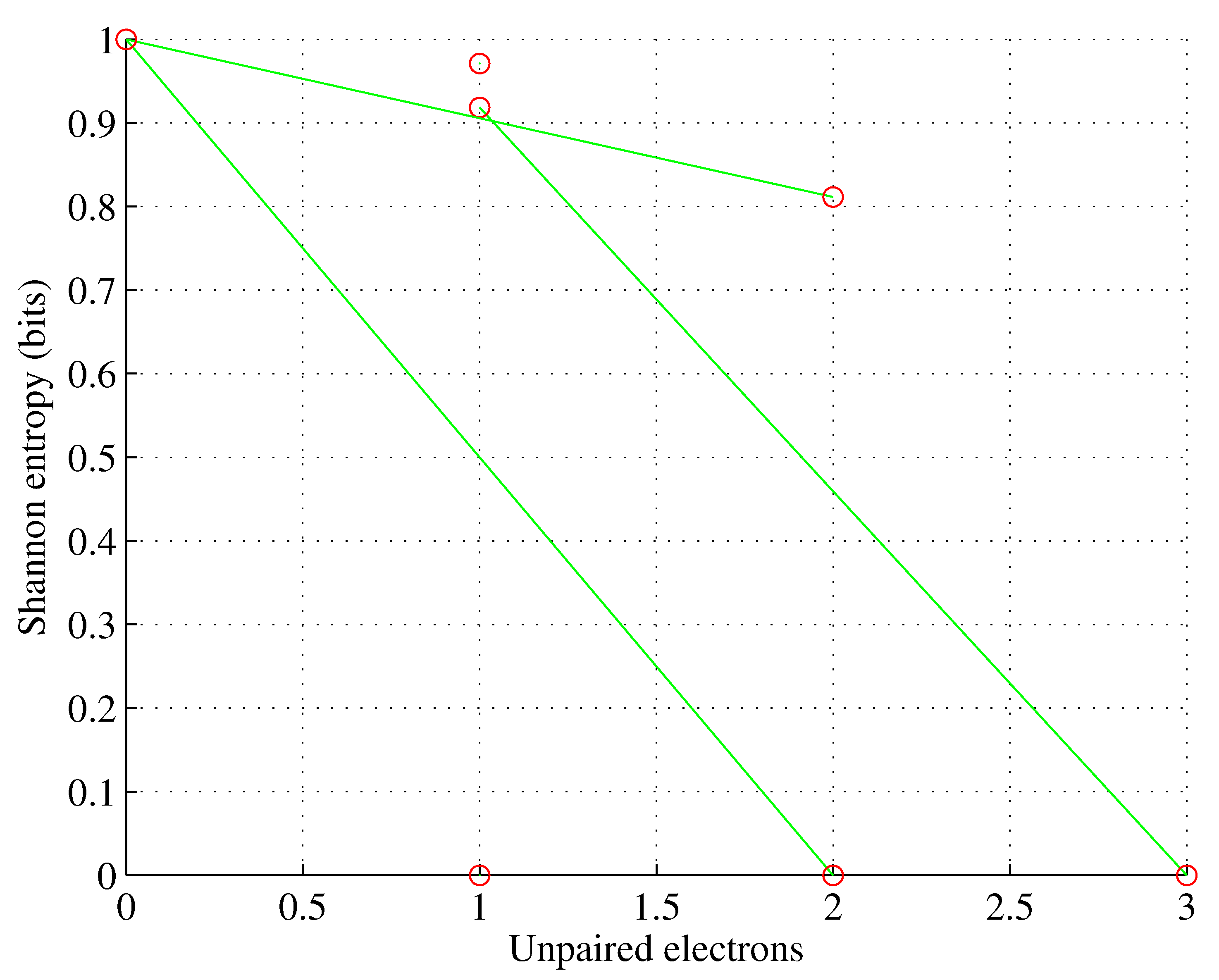
Figure 2.
Orbital d. , for , .

Figure 3.
Orbital f. , for , .

Figure 4.
Orbital g. , for , .

Figure 5.
Orbital g. The number of unpaired electrons L (red, rescaled) and associated orbital entropy H (green) as functions of the number of the orbital electrons N.
Figure 5.
Orbital g. The number of unpaired electrons L (red, rescaled) and associated orbital entropy H (green) as functions of the number of the orbital electrons N.

Figure 6.
Number of unpaired electrons of chemical elements (green) showing the Aufbau rule violators (red) and elements of the same L (blue). Five elements () have L higher than that predicted by the Aufbau rule. Only palladium () has a lower L.
Figure 6.
Number of unpaired electrons of chemical elements (green) showing the Aufbau rule violators (red) and elements of the same L (blue). Five elements () have L higher than that predicted by the Aufbau rule. Only palladium () has a lower L.
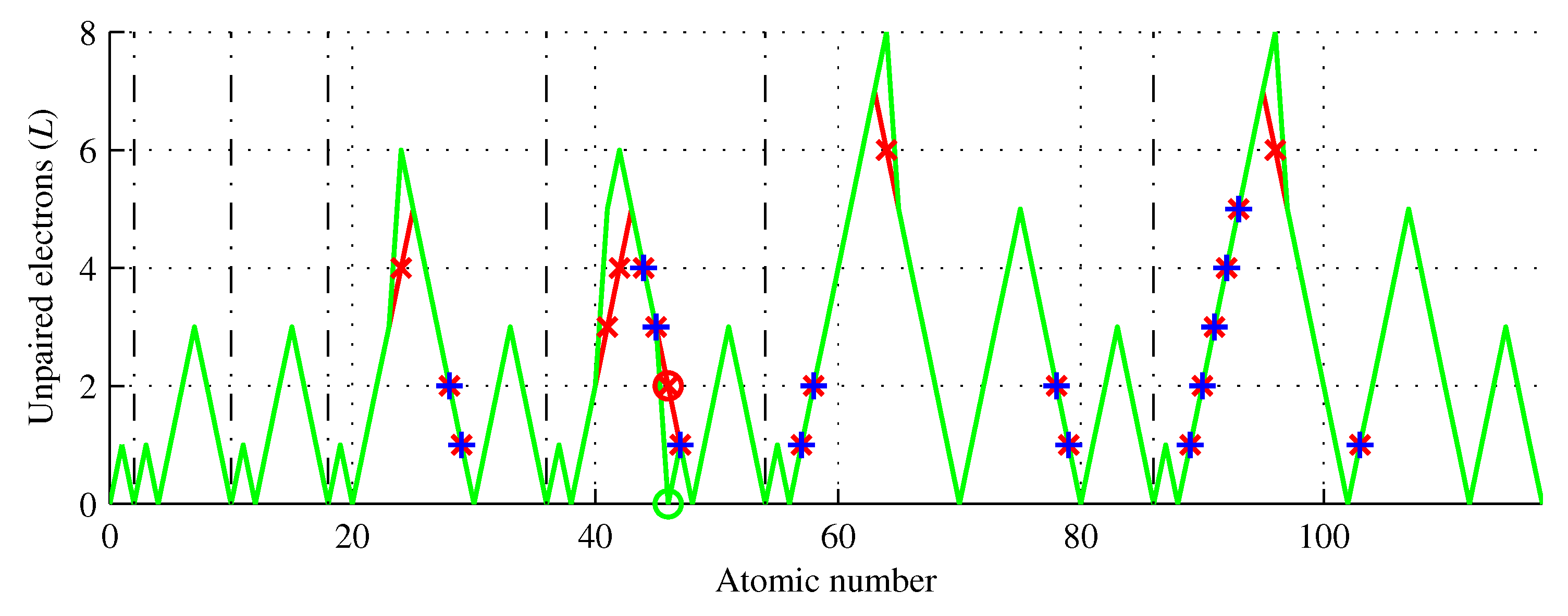
Figure 7.
Electron set entropy of chemical elements (green curve) showing the Aufbau rule violators (red x) and elements of the same entropy (blue +). Five elements () have electron entropy lower than that predicted by the Aufbau rule. This function does not increase monotonically and .
Figure 7.
Electron set entropy of chemical elements (green curve) showing the Aufbau rule violators (red x) and elements of the same entropy (blue +). Five elements () have electron entropy lower than that predicted by the Aufbau rule. This function does not increase monotonically and .

Figure 8.
Shannon entropy of chemical elements (green) showing Aufbau rule violators (red) and elements of the same entropy (blue). Thirteen elements ( including palladium) have entropy lower than that predicted by the Aufbau rule. Furthermore, .
Figure 8.
Shannon entropy of chemical elements (green) showing Aufbau rule violators (red) and elements of the same entropy (blue). Thirteen elements ( including palladium) have entropy lower than that predicted by the Aufbau rule. Furthermore, .

Table 1.
Chemical elements violating Aufbau rule.
| Electron configuration | Unpaired electrons | Shannon entropy | Electron set entropy | ||||||
| Z | actual | Aufbau | |||||||
| Cr | 24 | [Ar]3d54s1 | [Ar]3d44s2 | 6 | 4 | 0.9544 | 0.9799 | ||
| Ni | 28 | [Ar]3d84s2 (or [Ar]3d94s1) | [Ar]3d84s2 | 2 | 0.9963 | ||||
| Cu | 29 | [Ar]3d104s1 | [Ar]3d94s2 | 1 | 0.9991 | ||||
| Nb | 41 | [Kr]4d45s1 | [Kr]4d35s2 | 5 | 3 | 0.9892 | 0.9961 | ||
| Mo | 42 | [Kr]4d55s1 | [Kr]4d45s2 | 6 | 4 | 0.9852 | 0.9934 | ||
| Ru | 44 | [Kr]4d75s1 | [Kr]4d65s2 | 4 | 0.9940 | ||||
| Rh | 45 | [Kr]4d85s1 | [Kr]4d75s2 | 3 | 0.9968 | ||||
| Pd | 46 | [Kr]4d10 | [Kr]4d85s2 | 0 | 2 | 1 | 0.9986 | ||
| Ag | 47 | [Kr]4d105s1 | [Kr]4d95s2 | 1 | 0.9997 | ||||
| La | 57 | [Xe]5d16s2 | [Xe]4f16s2 | 1 | 0.9998 | ||||
| Ce | 58 | [Xe]4f15d16s2 | [Xe]4f26s2 | 2 | 0.9991 | ||||
| Gd | 64 | [Xe]4f75d16s2 | [Xe]4f86s2 | 8 | 6 | 0.9887 | 0.9937 | ||
| Pt | 78 | [Xe]4f145d96s1 | [Xe]4f145d86s2 | 2 | 0.9995 | ||||
| Au | 79 | [Xe]4f145d106s1 | [Xe]4f145d96s2 | 1 | 0.9999 | ||||
| Ac | 89 | [Rn]6d17s2 | [Rn]5f17s2 | 1 | 0.9999 | ||||
| Th | 90 | [Rn]6d27s2 | [Rn]5f27s2 | 2 | 0.9996 | ||||
| Pa | 91 | [Rn]5f26d17s2 | [Rn]5f37s2 | 3 | 0.9992 | ||||
| U | 92 | [Rn]5f36d17s2 | [Rn]5f47s2 | 4 | 0.9986 | ||||
| Np | 93 | [Rn]5f46d17s2 | [Rn]5f57s2 | 5 | 0.9979 | ||||
| Cm | 96 | [Rn]5f76d17s2 | [Rn]5f87s2 | 8 | 6 | 0.9950 | 0.9972 | ||
| Lf | 103 | [Rn]5f147s27p1 | [Rn]5f146d17s2 | 1 | 0.9999 | ||||
Z - the same H and L. Z - lower H, higher L. Z - palladium: higher , lower H, lower L. Electron set entropy calculated for .
Table 2.
Extended Aufbau rule. Periodic table for .
| k | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| 2 | 10 | |||||||||
| 2 | 8 | |||||||||
| 2 | 6 | |||||||||
| 2 | 0 | 4 | ||||||||
| 2 | 2 | 2 | ||||||||
| 0 | 0 | 2 | 2 | 0 | ||||||
| 1 | 1 | 2 | 2 | 2 | ||||||
| 2 | 2 | 3 | 2 | 6 | 8 | 10 | ||||
| 3 | 4 | 5 | 2 | 6 | 8 | 18 | ||||
| 4 | 6 | 8 | 2 | 6 | 18 | 36 | ||||
| 5 | 9 | 11 | 2 | 6 | 18 | 54 | ||||
| 6 | 12 | 15 | 2 | 6 | 32 | 86 | ||||
| 7 | 16 | 19 | 2 | 6 | 32 | 118 | ||||
| 8 | 20 | 24 | 2 | 18 | 14 | 10 | 6 | 50 | 168 | |
| 9 | 25 | 29 | 2 | 18 | 14 | 10 | 6 | 50 | 218 | |
| 10 | 30 | 35 | 2 | 22 | 18 | 14 | 10 | 6 | 72 | 290 |
| 11 | 36 | 41 | 2 | 22 | 18 | 14 | 10 | 6 | 72 | 362 |
The subscripts indicate the number of exceptions to the Aufbau rule. Setting 18 instead of 22 yields , the conjectured limit on the heaviest physically significant element.
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Copyright: This open access article is published under a Creative Commons CC BY 4.0 license, which permit the free download, distribution, and reuse, provided that the author and preprint are cited in any reuse.
MDPI Initiatives
Important Links
© 2024 MDPI (Basel, Switzerland) unless otherwise stated






