Preprint
Review
Thermal Conductivity and Temperature Dependency of MR Fluids and Application Systems—A Chronologically Review
Altmetrics
Downloads
147
Views
54
Comments
0
A peer-reviewed article of this preprint also exists.
This version is not peer-reviewed
Submitted:
18 October 2023
Posted:
19 October 2023
You are already at the latest version
Alerts
Abstract
Many studies on magnetorheological fluid (MRF) have been carried out over last three decades several salient advantages such as fast phase change, easy control of the yield stress and so forth. Especially, several review articles of MRF technology have been reported for last two decades summarizing the development of MRFs and their applications. As specific examples, the review articles including the optimization of the particles and carrier liquid to achieve minimum off-state viscosity and maximum yield stress at on-state, the formulation of many constitutive models in-cluding Casson model and Herschel-Bulkley (H-B) model, the sedimentation enhancement using additives and nanosized particles, many types of dampers for automotive suspension and civil structures, medical and rehabilitation devices, MRF polishing technology, the methods of mag-netic circuit design, and the synthesis of various controllers have been published. More recently, the effect of the temperature and thermal conductivity on the properties of MRFs and application systems are actively being investigated by several works. However, there is no review article on this issue so far despite of that the thermal problem is one of crucial factors to be seriously con-sidered for the development of advanced MRFs and commercial products of application systems. In this work, studies on the thermal conductivity and temperature in MRFs themselves and tem-perature-dependent application systems are reviewed, respectively, and principal results are summarized emphasizing on the followings: how to reduce the temperature effect on the field-dependent properties of MRFs and how to design application system to minimize the thermal effect. It is noted here that the review summary is organized in a chronological format using tables.
Keywords:
Subject: Engineering - Mechanical Engineering
1. Introduction
Since Rabinow discovered a new type of magnetic fluid at the National Bureau of Standards in 1948 [1], numerous works on the development of advanced magnetorheological fluids (MRFs) and semi-active control systems utilizing them such as vehicle suspension damper have been actively carried out since early of 1990s. In the Rabinow’s work, many research issues were arisen to be seriously considered for high control performance of the proposed clutch. These issues include the saturation of the solid phase under the magnetic field, an optimal mixture between particles and carrier liquid to avoid the de-energized problem, sealing issue to protect the liquid leakage, design of an appropriate magnetic circuit, power consumption, wear problem and lubrication issue which is different from dry friction clutch. Therefore, many scholars who are actively working in the rheology-related societies of rheological group, chemical group, chemistry group and mechanical engineering associated with control logics have tried to solve the above issues. As a consequence of the great effort and long-term research, MRFs are commercialized by Lord Company [2,3]. They sell several different MRFs naming MRF-122EG, MRF-132DG, MRF-140CG and also several application systems such as MR damper. In the homepage of Lord Company, there are many questions and answers on the commercial MRF products: (i) How does the iron affect the properties of MRF? (ii) How is MRF viscosity measured? (iii) What determines the viscosity of MRF? (iv) What is the best model for how MRFs behave? (v) What is the density of MRF? (vi) What is the molecular weight of MRF? (vii) What kind of iron particles are used in making Lord MRF? (viii) Does the MRF change volume during activation? (ix) How does fluid settling affect the performance of MRF devices? (x) Iron particles in oil sound abrasive? (xi) What is the time response of MRF? (xii) How does temperature and thermal conductivity affect MRF? So far, numerous works on the above issues have been done to resolve and hence make more advanced MRFs and their applications. Since there are many works on the same or similar research topics, several review reports on the above-mentioned studies have been published for last 20 years. As for the development of advanced MRFs, the review of the sedimentation stability, the review of constitutive models of MRFs, the review of the role of particle shape and morphology in MRFs, the review of challenges and solutions in the preparation of MRFs, the review of abrasive finishing using MR fluids, the review of physical mechanism and microchemical models. On the other hand, as for the development of MRF application systems, many review articles have been published in last two decades including the type of MR damper, medical and rehabilitation applications, the field-dependent characteristics of clutches and brakes, self-powered MR damper, vibration control for automotive suspension system and civil structures, sensor applications and types of mount systems for impact mitigation.
It is obvious from the above literature survey on the review articles, the review article focusing on the last question (xii) made by Lord Company has not been reported as far as the author’s knowledge even though the number of research on this topic is gradually increasing in these days. In the answer to this question, the catalog has stated that the temperature effect at the off-state largely dependent and at the on-state magnetic coil and working conditions. However, there is no accurate information on the properties of MRFs at lower temperatures ranging from 0~ -40°C. Moreover, there is no detail how to mitigate the temperature effect and how to design the application system to minimize the thermal effect and hence prevent the performance degradation. Consequently, a state-of-art on the temperature effect on MRF itself and its applications needs to be summarized based on recent works. In this work, major review articles on the development of enhanced MRFs and some review articles on the practical development of MRF application systems are firstly revisited. Subsequently, the temperature and thermal conductivity effect on the properties of MRFs, and thermal effect on the performances of the application systems are reviewed in detail and summarized in terms of chronological order using the tables. Since the tables provide the main issue on the temperature problems, the potential readers can easily understand the related important research history and can get several creative insights regarding to some challenging works to be resolved in future.
2. Review Articles for Magnetorheological Fluids
Carlson [4] have firstly written a review article introducing commercial magnetorheological fluids (MRFs) made by Lord Company stating a series of figures-of-merit. It has been introduced commercial MRFs and the first application device which is a small controllable brake applicable to aerobic exercise equipment. In 1998, a small MR seat damper was commercialized for heavy-duty truck, followed by several types of MR dampers for suspension systems of race and sedan vehicles. In this review article, the ingredients of MRFs developed by Lord Corporation in 2004 and the field-dependent rheological properties are also presented. For example, MRF consisting of hydrocarbon carrier liquid and iron particle volume fraction of 22% can produce a maximum yield stress 23 kPa at 200kA/m and response time of less than 1ms. Several advantages of semi-active controllable actuators introduced in this review article have significantly triggered numerous research works in diverse areas with a creative design philosophy which can replace traditional systems or devices by high performance systems showing design simplicity, low power consumption, excellent adaptability to various control strategies. Muhammad et al [5] summarized several requirements to make high performance of MRFs by stating principal properties including lowest coercivity, highest saturation magnetization, fastest response time, non-abrasive particles, spherical shapes particles and high purity particles (carbonyl iron powder: CIP). In addition, the particle volume fraction and particle size dependence of viscosity has been described mentioning the higher volume fraction, the higher viscosity, but the faster sedimentation due to the increased density. Some constitutive models of MRFs relating to the shear stress versus shear rate is also given by classifying geometry into concentric cylinder, parallel plate, cone and plate, and double concentric. Ierardi and Bombard [6] introduced the optimized mixture method of CIPs and carrier liquid (hydrocarbon) to achieve minimal off-state viscosity as well as maximal the field-dependent yield stress. In the investigation, three different BSAF CIPs have been used to obtain the proposed target because the CIPs have several merits over other magnetic particles such as excellent absorption of radar and microwaves, high purity, outstanding quality and consistency, reliable delivery, compatible with most polymer matrices and easily compoundable. Therefore, the CIPs are currently used to develop innovative solutions for a whole spectrum of different applications including automotives since BASF discovered the CIP processing recipe 85 years ago. Three samples are classified into Sample A(coarse), Sample B(medium) and Sample C(fine) and the dispersing additive of the thixotropic additives are used in all mixture formulations. It has been found from this investigation that the Sample A produces the highest field-dependent magnetization effect indicating the larger particle size contributes the high yield stress. de Vicente [7] comprehensively revisited the most salient properties of the field-dependent rheological fluids of MRFs, specially focusing on the understanding of the flow motion, yield stress and viscoelastic behavior under shear mode operation. Firstly, reviewing the manufacturing recipes of MRFs via traditional approaches, followed by new methods to reduce the particle sedimentation by reducing the particle size to the nanometer range. Then, they discussed about particle magnetization model (constitutive model of MR fluids) from both microscopic and macroscopic manners by adopting Brownian motion, Mason number, Reynolds number, Peclet number and van der Waals and body forces, Bingham model, Herschel Bulkley model and Casson method. Subsequently, the relationships between the shear stress and shear rate, between the viscosity and Mason number have reviewed at various magnetic field intensity to understand the constitutive models of MRFs. Ashtiani et al [8] reviewed overall content of MRFs, especially emphasizing on different methods of preparation, stabilization, the field-dependent rheological models and application. MR effect is characterized by a reversible increase in the viscosity and showing the yield stress due to the introduction of a magnetic field which can be explained by the particles chain formation. MR effect can be controlled by the magnetic field intensity and rheological characteristics of MR fluid constituents. This review article pointed out the difficulty of practical use due to the particle sedimentation caused from the density mismatch between the magnetic particles and carrier liquids. As solution to mitigate this problem, several approaches have been suggested: particle coating, use of various additives, use of various carrier liquid, use of porose particle, use of nano-sized particles, use of different shape of the particles and so forth. A detailed schematic images of MR effect with and without adding nanoparticles in which the nanoparticles are added to MRF dispersed in the voids between the microparticles resulting in the yield stress by strengthening the particle chains have been shown. The significance of the carrier liquids has been also discussed by adopting one potential carrier liquid: polyethylene oxide (PEO) which is a widely used polymer since it is a linear polymer soluble in organic media. Other potential carrier liquids include hydro-carbonic oil, ferrofluid, silicone oil, mineral oil, ionic liquid, cedar wood oil and so on.
Choi [9] comprehensively reviewed and analyzed the recipes to improve sedimentation stability from the aspects of the three ingredients: particle modification, carrier liquid modification and adjustment of additives. In addition, a few conceptual methodologies to prevent the sedimentation occurred in the bottle’s storage and application systems are also discussed as possible challenging works for developing successful MR applications. In the particle modifications, several types of magnetic particles including CIP, iron oxide, iron carbide, low carbon steel, silicone steel, nickel and cobalt have been used and their coated particles are employed, and as for the carrier liquid, many types of carrier liquids to reduce the density mismatch such as mineral oil, modified silicone oil, hydrocarbon oil, polyalphaolefin (PAO), 1-ethyl-3-methylimidazolium diethyl phosphate, and 1-hexyl-3-methylimidazolium chloride are investigated. The additives also crucial role for sedimentation stability of MRF and hence the sedimentation effect of MRFs using additives such as thixotropic agent, carboxylate soap, antioxidant, lubricant, viscosity modifier, metal-oxide powders, sulfur-containing, thioesters, xanthan gum, stearate-carboxylic are discussed. The review article [10] also analyzed the sedimentation stability of MRFs emphasizing on the use of different additives: iron oxide additives, ferrofluids, organic additives, carbon allotropes and inorganic additives. In addition, the relationship between the carrier liquid and sedimentation was investigated by adopting several carrier liquids: linear polydimethylsiloxane, hyperbranched polycarbosilane, CI dispersed in silicone oil (Si), synthetic oil (Sy), sunflower oil and polytetrafluoroethylene (PTFE). Pei and Peng [11] summarized diverse constitutive models of MRFs which are useful tools for prediction and analysis of the field-dependent characteristics such as yield shear stress and complex modulus. After describing constituents and rheological properties of MRFs, constitutive modeling methods are discussed in two different ways: macroscopic models and microscopic models. The macroscopic models, which are generally data-based models, have been popularly used due to their simplicity and accuracy. Among many macroscopic parametric models, Bingham model is the simplest, but provides useful properties including the shear stress versus shear rate at different magnetic field intensity. The macroscopic parameter models include Biplastic model, Casson model, Biviscous model, Herschel-Bulkley (H-B) model, Eyring model, Robertson model, Pa-Casson model, Mizrahi-Berk (M-B) model and so forth. The prediction of accuracy of these models are functions of many factors such as particle type and size, temperature, types of carrier fluids and properties of additives. In general, the parametric models lose the prediction accuracy at both very high and low shear rates. Thus, several types of macroscopic nonparametric models which can be applied for the prediction of the field-dependent shear stress of MRFs subjected to uncertainties of varying magnetic fields and varying currents. Unlike the parametric models, nonparametric models are hard to express specific equations containing experimental coefficients. One of the best ways to deal the nonparametric problem is to use artificial neural network (ANN) and support vector regression (SVR) technique. Using the methods, the time varying data are trained and tested by defining the temperature and shear rate as the inputs and the shear stress as the output. Another approach to resolve the uncertainties is to use extreme learning machine which can demonstrate both the ANN and SVR with a smaller training time. On the hand, the nonlinearity and saturation of the particle magnetization and the calculation of the resistance force of the chains at the field-on microscopic models have been studied by several scholars. Some representative microscopic models include the finite element model, micro-macro model, dipole model-based micro-macro model, initial tilt chain model and micro model based on hexagonal closed packed structure.
Kumar et al [12] summarized on a few challenging methods for the development of advanced MRFs considering types of the magnetic particles obtained by chemical vapor deposition of iron pentacarbonyl, and particle shapes affecting wear on the walls of the container or device inside in which MRFs operate. To increase sedimentation stability of MRFs, several recipes are discussed: the use of the coated particles and the use of additives such as carboxymethyl cellulose, polyethylene oxide, polyvinyl butyral, fibrous carbon, oleic acid, cholesteryl chloroformate and magnetic nanoparticles. When the additives are used to reduce the sedimentation is to be carefully adjusted the percentage of the volume or weight. In addition, fast time response of MRFs by the input current and the friction between the particles in fluid flow are to be carefully investigated. Matharu and Sehgal [13] summarized the field dependent rheological MRFs by considering the particle type, particle shape and particle size because these factors significantly influence the yield shear stress, sedimentation stability, wear and durability, and the magnetic intensity. Firstly, MRFs are classified into monodispersed, bi-dispersed, ploy-dispersed, ferrofluid based and dimorphic fluid stating inherent characteristics such as sedimentation and off-state viscosity. Particulate materials include carbonyl iron, Fe3O4, cobalt, nickel, carbon nano tube, graphene oxide, glass, hard magnetic metal, soft magnetic metal, magnetic stainless steel, and alloy iron. On the other, particulate size is ranged from nanometers to 30-50 micrometers. Durability of MRFs causes several disadvantages such as the degradation due to oxidation of the particles, the degradation due to wear of the particles, the degradation due to breakage or spalling of the particles, and the decrease in loss modulus over time due to shear thinning. On the other hand, Khairi et al [14] reviewed numerous studies focused on the ability of these materials to alter their rheological properties in response to applied magnetic fields. Especially, in this review, the influences of additives on the rheological properties of MR solids including MR elastomers and MR greases were presented. It has been shown that plasticizers soften the rubber matrix increasing MR effect by lowering the zero-field moduli, and carbon-based additives provide superior bonding with the rubber matrix and improve the dispersion of CIPs at the same time. Chromium-based additives enhance the stability of CIPs in the dispersion media by acting as coating agents resulting in preventing agglomeration. It has been also demonstrated that a combination of plasticizer, multi-walled carbon nanotube (MWCNT), and carbon black during the fabrication of anisotropic MR elastomers enhance the MR effect. Notably, MWCNTs provide an enhancement of MR elastomers, MR gels, and MR plastomers. It is noted that to get better additives to improve MR effect in various aspect, mathematical relationships must be established to predict the best composition of additives by systematic investigations on the interparticle forces between particles and additives, as well as additives and matrices.
Table 1 presents several review articles related to temperature and thermal conductivity of MRFs. As seen from the table, MRF products are on the market in 2000-2009 showing high yield stress at the on-state, and low viscosity at the off-state, and several approaches to enhance temperature robustness of MRFs are proposed from 2010-2019, and many works to reduce the particle sedimentation, constitutive models and effect of several additives to the field-dependent properties of MRFs have been undertaken after 2020.
Table 1.
Summary of review articles for MRFs.
| Years | Main Reviews | Related References |
|---|---|---|
| ~1999 | N/A | |
| 2000~2009 |
|
[4,5] |
| 2010~2019 |
|
[6,7,8] |
| 2020~Present |
|
[9,10,11,12,13,14] |
3. Review Articles for MRF Applications
As mentioned in Introduction, various types of systems and devices have been proposed and developed utilizing MRFs. The most significant MRF technology has been highlighted by MR damper for semi-active suspension system in automotive engineering field. Therefore, the review articles considering vehicle shock absorbers (dampers) have been reported by many scholars. Imaduddin et al [15] reviewed rotary MR dampers instead of traditional linear MR dampers focusing on several merits of the rotary type including design compactness, weight reduction and cost down due to the requirement of small MRF because there are no pressure chambers filled with MRF. However, since the field-dependent yield shear stress should be produced by rotational motion, the structural configurations of the rotary MR damper is relatively complex. The rotational applications such as MR clutch, MR brake and hybrid types of MR brakes and clutches are relatively easy to make by just installing the magnetic coils in an appropriate place. The authors also investigated the modeling method of rotary MR dampers: Bingham based torque model, Herschel-Bulkley based torque model, Bouc-Wen model and Dahl model. It has been remarked at the end of the discussion that an accurate modeling of the hysteresis model of rotary MR damper, an innovation application adaptable to the rotary damper, an accurate formulation of the constitutive model and design an appropriate controller with high stability and robustness under severe uncertain operating conditions are challenging future works to be resolved for the successful development of the rotary MR dampers. On the other hand, Kaluvan et al [16] summarized sensor applications of MRFs instead of actuator applications done by numerous scholars. Especially, the design and principle of following novel sensors are briefly introduced: resonant sensor, current sensor, magnetic flux sensor and tactile sensors. This article introduces a novel electrical current measurement technique using MRF in shear mode of operation, and the tactile display are discussed for the robotic system applicable to minimally invasive surgery (MIS) to provide a surgeon tactile information of touching remote biological tissues or organs change. Do and Choi [17] comprehensively reviewed design configurations of high loaded MR mount which are applicable to construction vehicles and ship engine mount for reduction of unwanted vibrations or impact loads. It has been firstly summarized from the design review that, in the design process of high loaded mounts, many principal factors such as damping gaps, cross sections, and equations of damping forces should be critically considered. In addition, identification models have to be carried out simultaneously for the achievement of superior vibration control performance. It has been found that the high loaded MR mount is designed based on two main modes of MR fluid: flow mode and shear mode. The distribution of parts inside the mount determines the establishing pressures, which are directly related to the damping force of the mount. It has been also identified that the high loaded MR mount based on the squeeze mode is still difficult in design and manufacturing due to the low damping force and leakage of the fluid itself. Hence, a breakthrough design configuration using the squeeze mode with large deformation values needs to be created in future. Ahamed et al [18] reviewed both traditional MR damper and self-powered MR damper considering structural configuration and governing dynamic model, energy generation. Various MR applications including automotive damper, industry brakes and clutch, glass polishing, hydraulic valve, composite core structure, active servo valve and rotary seal have been investigated and their operation performances are discussed in the sense of design simplicity, power consumption, high performance and leakage issue. Subsequently, several mechanical models of MR damper associated with the governing equations are discussed by adopting Bingham model, Bouc-Wen hysteretic operator-based model, simple Bouc-Wen model, modified Bouc-Wen model, hyperbolic tangent function based model and nonlinear bi-viscous model. In addition, energy harvesting (self-powered) MR dampers have been introduced describing the structural configuration integrated with the electromagnetic induction (EMI) and permanent magnet in which the EMI system can produce the generated voltage linearly proportional to the relative velocity across the MR damper. Finally, other methods to self-powered MR damper such as the use of the induction coil, rack and pinion mechanism, ball and screw mechanism, and generator and motor were also briefly summarized. Choi et al [19] investigated several control strategies which have been applied to various devices or systems utilizing MRFs and MREs. The mandatory requirements for successful applications of MRF and MRE include several factors: advanced material properties, optimal mechanisms, suitable physical modeling, and appropriate control schemes. Among these requirements, the use of an appropriate control scheme is a crucial factor since it is the final action stage of the application systems to achieve desired output responses. There are numerous different control strategies which have been applied to many different application systems of MRF and MRE: skyhook controller, PID controller, LQR/LQG (linear quadratic regulator/linear quadratic Gaussian) controller, sliding mode controller, adaptive fuzzy controller, neural network controller, and hybrid controller combining more than two different control schemes. Advantages and disadvantages of each control scheme are discussed so that potential researchers can develop more effective control strategies to achieve higher control performances of many application systems utilizing MR materials.
Kumari and Chak [20] comprehensively surveyed and described on the surface finishing (or polishing) utilizing MRFs. The magnetically assisted abrasive finishing (MAAF) processes are the precision material removal processes that have been applied to a large variety of materials from brittle to ductile and from magnetic to non-magnetic. As well known, the MAAF process relies on a unique “smart fluid”, known as magnetorheological (MR) fluid or electrorheological (ER) fluid. Nowadays, MR fluids are more popular for surface finishing which contains the micron sized magnetizable particles such as carbonyl iron, dispersed in a non-magnetic carrier medium like silicone oil, mineral oil or water. The mechanism involved in MRF of material removal and surface finish is basically comprises three different modes: (i) the abrasive particle held by chains of iron particles, (ii) the bunch of iron and abrasive particles move in the forward direction and shears/removes a very small amount of material in the form of micro-chip and (iii) when this bunch of iron and abrasive particles moves further, it separates the micro-/nano-chip from the work piece. There are several finishing or polishing approaches based on the above three modes: ball and MR finishing, permanent magnetic finishing, chemical-based MRF processing and ultrasonic based MRF processing. As for effective applications, machining of electronics and industrial components, machining of optical components, and machining of biomedical component are fully discussed, followed by challenging works for successful commercialization. For example, how to reduce the finishing time when ball and MR fluid (BMRF) process is applied to hard material? And how to solve when MRAFF process is applied to the specimens which have sharp edges? This review article is very helpful to know the surface processing utilizing MR fluids and create new methodologies over existing disadvantages. Daniel et al [21] summarized MR damper focusing on civil structure application to suppress unwanted vibrations through passive and semi-active control means. The main difference between MR damper for automotive suspension and civil structure is that much higher yield stress (actuating force) is required for the civil structures. Many researchers proposed MR damper which can generate more than 20 kN at around 3A and applied to the precision table structure, high story building, bridge cables and seismic structure to reduce the vibration as soon as possible. As controllers for the semi-active system, skyhook controller, linear quadratic Gaussian (LQG) and fuzzy controllers have been applied to vibration control of high story building and seismic occurrence. A couple of works were also introduced where the optimization method using the genetic algorithm was used and bang-bang and clipped optimal controller were implemented to reduce the vertical acceleration. The finally remarked that the important factors to develop successful technology in civil structures are fast reaction time, design with less moving parts and choice of appropriate control strategies. Sohn et al [22] briefly reviewed applications of MRF and ERF to robotic systems. Since ERF has lower stiffness effect, flexible robot arm, soft robot, flexible gantry arm, tactile robot display and long and thin robot arms such as space robot have studied by several scholars. However, many studies on the robotic area using MRF have been actively undertaken. Among many different types, haptic robot, collaborative robot, climbing robot, spherical robot, planar robot, deformable gripper and many different rehabilitation systems such as knee prosthetics. Advantages of robots fabricated from MRFs include accurate interaction information between human and robot by controlling the current and easy fabrication using 3D printing method. This review paper can motivate the creativity of researchers seeking to harness MRF for robotic applications. Ahamed et al [23] reviewed the field-dependent properties of MR materials and comprehensively investigated MR materials-based applications. They summarized the properties of MR materials including MRF, MRE, MR foam, MR grease and MR plastomer studied in the last two decades. Them, in this review article, applications are focused on several engineering and technology fields such as automotive industry, civil environment, military sector and medical devices. More specifically, they summarized the development procedures of MRF brake, MRF damper, MRF polishing, MRF damper for civil structures, Diverse MRF seat damper, MRF damper for washing machine, MRF damper for above-knee amputees and human body palpation sensor using MRF. Oh and Choi [24] reviewed various types of MR dampers applicable to different purpose have been surveyed and their design configurations are discussed. After briefly explain the salient characteristics of MR fluids, MR damper for automotive, MR damper for the bridge cable, MR seat damper for commercial vehicles such as truck and bus, MR damper for washing machine to suppress both vibration and noise, lateral type of MR damper for high-speed railway vehicles to improve operating stability at curve lines, special MR damper or military vehicles subjected to severe environmental conditions of temperature and road condition are discussed in terms of current status and future works to be implemented. In addition, performances of many different types of MR dampers applicable to human rehabilitation devices such as prosthesis and above-knee amputees are described. In this review article are also surveyed on the development, application, and classification of MR dampers focusing on the structure components, operating principle, control performance and developing trends for various systems and devices to be operated by semi-active control strategies.
Kolekar et all [25] reviewed the research trend on sandwich structures, whose stiffness and damping properties could be controllable utilizing MRF as cores or layers. It has firstly shown that both damping property and stiffness property of the sandwich structures can be effectively controlled by several ways: the change of the field intensity, the location of cores zones, the partial and full treatment and boundary conditions of the structures. In addition, it has found that the modal parameters such as natural frequency of the sandwich structures are controllable in real time by supplying external input current to the specific points and hence the resonance cased from the external excitations can be easily avoided by changing the natural frequencies of the sandwich structures. In addition, the controllability of the mode shapes of the sandwich plates can provide several advantages in flexible structures systems such as aircraft wing, automobile hood, and appendage of space robot. Despite many works and several advantages of these smart sandwich structures, there is no specific practical application in real environment so far. This directly indicates that further research works should be explored in the future focusing on the optimal coil structure for MRF, the maximization of controllable range of the stiffness and damping properties, the buckling problem as a function of controllable parameters, the field-dependent acoustic characteristics with controllable cores. Yuan et al [26] investigated types of MR dampers along with the position of the magnetic circuit core and flow modes. It has been found that controlling viscosity of MR damper in a limited axial channel can extend the variation of both the damping ranges and force due to different positions and turns of coils. In addition, adopting flow mode MR damper with the radial and circumferential direction, a longer length of damping channels can be obtained. Especially, combining radial and circumferential flow modes in a miniature external valve can provide more excellent performances. As future works, creative MR damper based on longer damping channel, high magnetic field utilization, larger damping range, weak magnetic field excitation, smaller volume, less energy consumption, better generality, and lower costs haven been pointed out. Lu et al [27] reviewed on the MRF polishing technology which had done from 2016 to 2020 focusing on major advantages including recycled MRF, updated abrasive in real time, few tool wear, stable removal function, and controlled hardness of MRF micro-grinding head. In addition, the application methods, operation modes, and structural configurations of machining equipment with MRF are discussed considering different polishing approaches. Two significant factors to achieve accurate polishing or machining, both the type of the platforms such as MR damper and polishing principles such as slurry polishing technique are also discussed. Merits and demerits of various polishing technics have been analyzed by adopting several different approaches: dual rotation MR polishing, magnetic compound slurry, MR solid core rotating polishing, belt MR finishing, multiple polishing heads, MR gear profile finishing, reciprocal MR polishing and wheel-based MR finishing. Eshgarf et al [28] analyzed appropriate applications of MRFs corresponding to the field-dependent yield shear stress in terms of the field magnitude, particle volume fraction by adopting several types of vehicle MR dampers which are operated with the flow mode, shear mode and squeeze mode. Especially, the power to operate MRF application systems has been discussed by adopting three types of batteries including chemical batteries, physical batteries and biological batteries. It has been demonstrated that the fuel battery included in the chemical batteries shows several advantages: (i) the process of direct conversion of chemical potential energy into electrical energy prevents thermal blockage, (ii) it is easy to deal and very safe due to the lack of moving components, (iii) environmentally friendly manner due to the production of hydrogen. On the other hand, Liu et al [29] surveyed and systematically reviewed the progress of medical applications of MRFs mainly emphasizing on six categories: lower limb prosthesis, exoskeleton, orthosis, rehabilitation device, haptic master, and tactile display. With MRFs, one can devise stable limb motions in lower limb prostheses, exoskeletons, orthoses, flexible muscle trainings in rehabilitation devices, and high transparency and resolution haptic feedback. In exoskeleton applications, exoskeleton for force/strength enhancement and exoskeleton for motion assistance are included, while for the orthosis applications lists the knee assistance device and ankle foot orthoses. Among applications of medical field of MRF, the research work on the haptic master has been mostly done for robot surgery in which a surgeon operating the haptic master can feel same force or torque occurred in the surgical areas by controlling the magnetic field. In addition, in the robot surgery a tactile display device using MRF is used to mimic human tissues or organs by controlling the input current. Aziz et al [30] presented a state-of-art on the large-sized MR dampers, which can produce high enough damping force with a relatively small power, applied to several industries. The application of fields is classified into civil infrastructure, automotives, gun recoil system, vibration isolation systems, railway vehicles, powertrain mount, polishing industry and military vehicles. For example, 400kN large MR damper was developed for vibration control of civil structures, 600kN MR damper was made based on the hyperbolic tangent function model and applied it vibration control of building structures with proper control logics. The largest MR damper which has a capacity of 6000kN was made in China and applied it for vibration control of Binzhou Yellow River Bridge. Most of large-sized MR dampers have been designed using Bingham model-based monotube large MR damper (single-ended), hyperbolic tangent function-based mono tube single-ended large MR damper, modified Bouc-wen model-based monotube large MR damper (double-ended), phenomenological Bouc–Wen model-based monotube large MR damper (double-ended), phenomenological Dhel friction model-based mono-tube large MR damper (double-ended), Bingham model-based monotube large MR damper (double-ended), and Herschel-Bulky model-based monotube large MR damper (double-ended).
Do and Choi [31] reviewed six different smart materials actuators applied to diverse control applications from the aspect of control performances. Among actuators, MRF actuator was investigated on the various application systems including vibration control automotive suspension system, vibration isolator, tiny vibration control of precision table. To achieve enhanced performances of these application systems, a proper control strategy corresponding to the motion characteristics of each application should be designed. In the review article, several control strategies which are popularly used for MRF application systems have been discussed by choosing sliding mode controller, H-infinity controller, fuzzy controller, and neural networks model-based controller, model free fuzzy controller and phase-based fuzzy logic control. Some advantages and disadvantages of each control strategy have been discussed in terms of control performance, practical implementation, cost-effectiveness, signal processing of the feedback states and wide applicability to diverse MRF applications. Fardan et al [32] summarized the research trend of auxetic metamaterials research which have the potential to be developed primarily for use in prosthetic devices, protective devices, robotic applications, and aerospace engineering by treating MRF as one of metamaterials since its inherent characteristics are controllable by the input current. Among many applications, the prosthetic design was fully discussed showing some features such as the negative Poisson’s ratio effect and other relevant properties. Masa’id et al [33] surveyed various control strategies applied to smart MR materials in a semi-active manner. It is well known that MR damper can dissipate the energy only, and hence the semi-active controller needs to be implemented to increase damping performance due to the magnetic field. One representative semi-active controller is the skyhook controller proposed firstly by Karnopp et al [34]. Later on, a sky-ground hook controller was developed for vibration control of both body mode and wheel mode of vehicles suspension systems. In this review paper, each controller has been arranged to appropriate application systems. For example, LQR or LQG provides high control performances of automotive, railway train, aero-foil shape and building structures, while the sliding mode controller brings good performances of several uncertain systems, and the fuzzy logic controller is well adaptable to military vehicle. Each control system has been discussed with advantages and disadvantages, followed by future works such as how to make a simple and cheap microprocessor which can be easily implemented in real environment with relatively small power to operate MR applications.
Table 2 summarizes major review articles published in the last two decades. It is clearly seen that various application devices and systems utilizing MRFs have been proposed and studied including linear and rotary MR damper, MR mount, medical and rehabilitation devices, polishing technology, and civil structures such as bridge cables. In addition, diverse semi-active control strategies such as skyhook controller, sliding mode controller, optimal controller and adaptive fuzzy controller are applied to the MRF systems to achieve desirable performances.
Table 2.
Summary of review articles of MRF applications.
| Years | Main Reviews | Related References |
|---|---|---|
| ~1999 | N/A | |
| 2000~2009 | N/A | |
| 2010~2019 |
|
[15,16,17,18,19,20,21,22,23,24,25,26] |
| 2020~present |
|
[27,28,29,30,31,32,33] |
4. Temperature Effect and Thermal Conductivity
As mentioned earlier, one of most significant issues for successful development in MRF technology in real environment is to reduce or eliminate the temperature effect on the MRFs and thermal effect of the application systems to maintain advanced properties and performances regardless of the temperature variation. Most of MRFs consist of magnetic particles, carrier liquids and additives, and hence the main characteristics of MRF itself is very sensitive to the temperature induced from the magnetic coil (or input current). Thus, the heat needs to be effectively dissipated to achieve maximum performance of the application system which brings the work of the heat transfer analysis of the design structures associated with the magnetic circuit position. Furthermore, the temperature issue is closely related to energy consumption during dynamic motions, maximum input magnetic field due to the saturation and operational durability. In subsequent two sections, major research studies on the temperature and thermal conductivity of MRFs and thermal effect in application systems are surveyed and summarized.
Zschunke et al [35] presented an approach to the solution of the variation reduction due to the magnetic field strength, geometry and temperature. They used an Arrhenius relationship in which the fluid viscosity is a function of the shear rate, magnetic field and the temperature. It has been shown that the viscosity of the MR fluid follows the Arrhenius expression stating that the temperature effect depends only in the change of viscosity of the matrix fluid. Heine et al [36] analyzed thermal energy transport in sheared MRFs by ignoring viscous dissipation. The particle-level simulation was carried out to determine the suspension structure in terms of Mason number and volume fractions. It has been demonstrated that a small Mason number results in a larger effective thermal conductivity than at large Mason number. It has been also shown that the effective thermal conductivity of the sheared MRFs can be roughly approximated double as the Mason number is decreased from the large to the small Mason number limits. Ocalan [37] conducted the literature survey in the thesis focusing on the temperature effects on the rheological properties of MRFs and theoretical predictions to changes in field-dependent properties of MRFs with temperatures. The dependence of magnetic force on temperature arises from the effects of thermal fluctuations on ferromagnetic ordering within the particles, while the ferromagnetic ordering is completely lost at the Curie temperature in the absence of the magnetic field. In addition, the saturation magnetization of the ferromagnetic material is a monotonically decreasing function of temperature at below Curie temperature. On the other hand, Brownian force resulting from a temperature rise from 25°C to 70°C only corresponds to half an order magnitude change. It has been also shown that the effect of temperature on magnetorheological response is evaluated for three levels of magnetic flux density. The measurements on five samples were taken at three temperatures each: 20, 75 and 130°C. From this test, it has been demonstrated that at higher flux densities, the current requirement is higher at elevated temperatures, and the high temperature results show a systematic and statistically significant decline in magnetorheological stress. Yildirim and Genc [38] experimentally studied the thermal conductivity of MRFs synthesized with iron powder and silicone oil with several particle volume fractions (5, 20, and 40 vol%) of two different grades of iron (Fe) and magnetic field strengths. The thermal conductivity behavior of the MR fluids in different temperature ranges was analyzed and found that the heat transfer is more effective at higher temperature, while the thermal conductivity increases in the temperature range from 0°C to 50°C, and from 50°C to 100°C, but decrease from 20°C to 100°C. This decrease could be due to the decrease of the thermal conductivity of the silicone oil. As a result, silicone oil-based MR fluids may not be suitable for low temperature heat transfer applications. It has been identified that the heat transfer coefficient is high for higher particle concentration and the percentage increase is more pronounced for lower particle concentrations. This may be due to the close packing of particles in the higher volume fractions of the magnetic phase. For lower concentrations, the particles which are farther away from each other in the off-state lined up in a chain-like formation and the interaction is more increased under the magnetic field. Chang et al [39] investigated thermal conductivity of a set of MRFs made of CIP and three different oils. Especially, the concentration of MRFs is chosen as a key parameter to formulate the relationship considering the thermal conductivity. It has been shown that there is a linear correlation between thermal conductivity and concentration of MRFs. Applying this trend, the concentration variation of MRFs can be acquired on the basis of the thermal conductivity variation.
Bilyk et al [40] formulated a mathematical model of MRF flow in an annular channel of the MR shock-absorber taking into account forces of dry friction and gas friction in a pneumatic camera to investigate the dependence rheological properties of the MRF on shear rate, temperature and magnetic flux density. It has been observed that the resistance force with growth of magnetic flux density increases 40 times, but the resistance force with growth of temperature from 20°C to 80°C decreases 7 times in the magnetic field of 500 mT, and 2 times without the field. This temperature dependence can be used by development of a control algorithm of shock-absorber performance characteristics which needs to be integrated with the electronic control unit practical implementation. Wang et al [41] investigated the effects of temperature on the material properties of MRF components. Both theoretical analysis and experimental investigation were performed on the temperature-dependent material properties of MRF components. It has been shown from experimental that both the mass magnetization and coercivity of MR particles decreased as the temperature increased and the phenomenon is particularly obvious at high temperatures. It is also identified that the shear viscosity and a relatively large thermal expansion of the carrier fluid severely are decreased by increasing temperature further, and the magnetization performance of MR particles declines sharply after a long-term exposure to high-temperature environment due to the formation of surface oxidation layer. In addition, the shear viscosity of the carrier fluid decreases as the temperature increases, and a carrier fluid with a higher viscosity is more sensitive to the temperature variation. Mistik et al [42] investigated the compression effect due to the temperature of MRF contained in a fabric subjected to the magnetic field. The compression test was carried out on the MRF-filled spacer fabric with and without the magnetic field in the temperature range from 15°C to 70°C. Thermal conductivity of the MRF-filled spacer fabric was also tested to see the effect of the change in the magnetization and rigidity. The compression tests exhibit an increase with increase in the displacement value showing the intensity of the temperature dependence. On the other hand, it has been seen that the encapsulation of the MRF has a considerable effect on the thermal conductivity of the spacer fabric resulting in nearly fivefold increase in the thermal conductivity of the spacer fabric even in the absence of an applied magnetic field. Chen et al [43] presented the temperature effect of the shearing transmission performance of MR transmission device under the external magnetic field and investigated the influence of temperature on the shearing stress and transmission performance. It has been demonstrated that the torque transferred by MRF gradually declines as temperature rises under the same magnetic field strength and rotating speed, but the variation is continuous and uniform. Once the temperature is higher than 100∘C, the torque transferred by MRF gradually increases and varies irregularly, which cause instability of the transmission performance due to the thickening behavior of MRF after the temperature is higher than 100∘C.
Rahim and Ismail [44] investigated the thermal behavior of MRF and nanofluid focusing on the understanding of their thermal conductivity property with several parameters including particle volume fraction, shape and size of particles, materials of particles, base fluid, and magnetic field. It has been shown that the increase with particle volume fraction and magnetic field strength may increase the thermal conductivity of MRF. From the theory and experimental given, the relationship between these parameters and thermal conductivity can be found. Although there are several studies on the enhancement of thermal conductivity of MRFs from 0.42 W/m K to 0.54 W/m K, they are still room for improvement to enable optimization of the fluids to be used in practical applications. The potential applications of magnetorheological fluids with better thermal properties would be like thermomagnetic convection and heat pipe. Sherman et al [45,49] studied non-Brownian suspension theory applied to explain and find the temperature dependent behavior of the carrier fluid. To carry out this, two fluids were sheared with the same carrier fluid, then their fluid properties were scaled in temperature similarly. This method is first validated by measuring the viscosity across temperature for custom model fluids (commercial MRFs) to conform to the theory showing temperature scaling within 5% for both the MRFs and their carrier fluid. It is shown that the MRFs exhibit common scaling to within 4% and the effect of magnetic hysteresis is a negligible in yield stress increment. The proposed measurement method of the viscosity across temperature for a pair of custom MRFs and corresponding carrier liquid is very useful to identify the temperature dependence of MRFs in fewer experiments. Yang et al [46] carried out experimental works on the investigation of the hyperthermia effect of magnetic functional fluids using three samples: Fe73.5Nb3Cu1Si13.5B9 amorphous particles, CoFe2O4 nanoparticles and Fe3O4 nanoparticles dispersed in water. It has been observed from the hyperthermia experiment that when alternating electrical current is 150 A, the temperature of the functional fluids based on amorphous particles could rise to 20°C to 80°C 33◦C in 1500 s. When the current is 300 A, the final stable temperature could reach to 20°C to 80°C 60◦C. This study demonstrates that the Fe73.5Nb3Cu1Si13.5B9 magnetic functional fluids may have potential on biomedical applications. The hyperthermia experimental results indicate that the Fe73.5Nb3Cu1Si13.5B9 MRF exhibits more significant heating effect than that of CoFe2O4FF and Fe3O4FF in an alternating magnetic field. As a result, it has been shown from the hyperthermia experiment that the Fe73.5Nb3Cu1Si13.5B9 MRF has the best potential on hyperthermia therapy of tumor in biomedical applications. Rabbani et al [47] studied the stability and rheological properties of MRF consisting of carbonyl iron microparticles and silicone oil within a temperature range of 10°C to 85°C. In the preparation of MRF, 3 wt% of stearic acid has been added to enhance fluid stability resulted in 92% stability enhancement for a period of a month, which is eight times more than common MRF. It has been also shown that as the temperature increased, the viscosity and maximum yield stress decreased. The investigation of the type and weight fraction of MRF additives and also particle polydispersity on the MR effect and MRF stability at high temperatures over 80°C can be the significant subject of the future research areas. Shah et al [48] presented the field-dependent rheological properties of a nano-sized magnetic particle-based ferrofluid (NMPFF) investigated in dynamic mode at different temperatures. The NMPFF has several inherent rheological properties such as improved shear thinning, elevated dynamic moduli, and thermo-rheological complexity. These properties came from the presence of a long chain- like structure in the NMPFF under the influence of an applied magnetic field. The proposed NMPFF is a magnetic colloidal suspension of a mixture of two different nano-sized magnetic particles, which are dispersed in a carrier liquid in an appropriate weight fraction. Both the structural and morphological properties of the particles are firstly investigated using small-angle neutron scattering and a transmission electron microscope is observed. Subsequently, the temperature-dependent rheological properties of the NMPFF are measured in an oscillatory mode using the magneto-rheometer. It has been shown that the crossover strain value increases with augmenting temperature at low field, and it decreases with increasing the magnetic field strength. It has been also found from the frequency sweep test that a slight change in the magnitude of the storage modulus with the increased temperature and magnetic field strength is occurred.
Rahim et al [50,52] investigated the thermal issue which needs to be seriously resolved in the heat dissipation technology such as MRF filled inside a PMMA (polymethylmethacrylate) by carrying out the measurement of thermal conductivity in both parallel and perpendicular orientations with the magnetic field. The magnetized distribution is undertaken using finite element method (FEM) and three parameters are being looked into namely, PMMA diameter, PMMA height and gap thickness. By utilizing the FEM software, simulation results were produced and magnetic flux density for all designs have been achieved. It has been clearly found that gap thickness played a significant factor in determining the optimal design. For both vertical and horizontal magnet arrangements, the highest magnetic flux densities were produced with the gap thickness at 5 mm. Meanwhile, the geometries of PMMA container indicates a minimal flux density change at all parameter levels. For vertical magnet arrangement, the low level of PMMA diameter resulted in higher flux density regardless of the PMMA height and this is a complete opposite to horizontal magnet arrangement in which the PMMA height at low level (30 mm) is more dominant, irrespective of PMMA diameter. Therefore, it has been concluded that the optimal design for the thermal conductivity measurement instrument is possible by having PMMA geometries at medium level with 5 mm of gap thickness. Forero-Sandoval et al [51] studied the thermal conductivity and viscosity of MRF composed of CIP immersed in silicone oil. Thermal wave resonant cavity was employed to measure the thermal diffusivity of the MRF as a function of an externally applied magnetic field. The dynamic viscosity was also measured and its relationship with the concentration of the particles and the magnetic field strength was investigated. It has been shown that higher concentrations of CIPs as well as higher magnetic field intensities lead to a significant increase in thermal conductivity. Thus, the relationship between the thermal conductivity and the dynamic viscosity can be explored using highly viscous materials. It has been also found that the addition of CIP combined with the magnetic field induce the formation of chains that increase significantly the thermal conductivity and viscosity. McKee et al [53] studied the temperature effect on performance of compressible MRF. MRF is a temperature-dependent material where its compressibility and rheological properties change with the temperature. Firstly, it has been found that the shear yield stress of the MRF remains unchanged within the testing range while both the plastic viscosity, using the Bingham plastic model, and the bulk modulus of the MRF decrease as the temperature of the fluid increases (25°C ~ 70°C). A theoretical model integrated with the temperature-dependent properties of MRF has been also proposed to predict behavior of a compressible MRF. It has been demonstrated from experimental results that the accuracy of the proposed model is good to predict the stiffness and energy dissipation of the compressible MRF showing that both the stiffness and the energy dissipation decrease with an increase in the temperature of MRF. Maroofi and Hashemabadi [54] adopted Discrete Phase Model (DPM) to simulate the effects of influencing parameters on thermal conductivity of MRFs and computer fluid dynamics (CFD) simulation results are validated with experimental data from Transient Hot Wire (THW). It has been shown that the magnetic field intensity variations are a proper factor for changing the thermal conductivity of MRF and if the external magnetic field is parallel to the temperature gradient, chains of magnetic particles act as a facilitator of heat conduction increasing the thermal conductivity of MRF. It has been identified that the conditions of larger dispersed particles volume fraction and intensified applied magnetic field and also smaller dispersed particles increase the thermal conductivity anisotropy in the MRFs. Therefore, the thermal conductivity of MRFs is a controllable property can be increased or decreased based on the direction of the magnetic field, magnetic field intensity, size and volume fraction of dispersed particles, and this salient characteristic can control the thermal effect and heat transfer in MRF application systems.
Maroofi et al [55] studied a variation of thermal conductivity of the MRF via the visualization of the cluster formation inside the fluid which can be achieved CFD simulations. It has been shown that the more and longer chains of particles are formed, the variation of thermal conductivity of MRF is more considerable. In addition, it has been identified from experimental test that the thermal conductivity of the MRF with 15% dispersed particle volume fraction is increased up to 117% by applying 11.16 kA∕m magnetic field. Thus it has been found that whenever the magnetic field strength and the dispersed volume fraction are greater and the size of particles is smaller, the thermal conductivity of MRF is more increased. Pisuwala [56] presented the thermal properties of MRFs made of transformer oil and 2 wt. % of Fe3O4 particles. Firstly, it has been shown that the thermal conductivity is enhanced by 219%, 304%, and 356% by changing the iron concentration from 15 vol. %, 20 vol. %, and 25 vol. %, respectively. This increment is higher than the normal spherical-shaped iron particles-based MRFs. The increase in the thermal conductivity of transformer oil-based MRF is due to the shape effect as well as the reduction in thermal resistance due to a higher particle-particle interaction compared to spherical particles. In addition, on adding nanoparticles, the friction between the particles reduces and nanoparticles facilitate flake shaped particles to form a stronger chain. This results in an enhancement of the thermal conductivity. Therefore, the observed reduction in the viscosity of MRFs with nanoparticles supports the concept of the increased orientation of flakes in the direction of the field. It has been concluded that the observed enhancement with the field is due to the shape of particles, the increase in ordering of particle chains and the reduction in friction and viscosity of MRF with the nanoparticles. Hemmatian et al [57] investigated the influence of temperature on the linear and nonlinear viscoelastic behavior of MRFs. In this work, the shear flow and oscillatory shear strain experiments were conducted over a wide temperature range (-5 to 50°C) for different levels of magnetic flux density, and strain amplitude and rate. Firstly, it has been shown that the MRF is highly dependent on the temperature and shear stress increases by reducing the temperature especially below 10℃. Moreover, it has been observed that applying the magnetic field decreases the effect of the carrier fluid and consequently the dependency of the MRF to the temperature decreases. In addition, it has been shown that in the linear region, the temperature mostly affects the storage and loss moduli in the absence of the applied flux density. On the other hand, the influence of the driving frequency on the viscoelastic properties of the MRFs was studied resulting in the increment both the storage and loss moduli as the frequency increase in the absence of magnetic field. Li et al [58] studied the influence of temperature rise on the properties of MRFs using different constitutive models considering the temperature rising from 20°C to 70°C. In the viscosity test, the zero-field viscosity of the MRFs decreases with increasing temperature as expected, and this phenomenon is more obvious at higher shear rates. The shear stress of MRFs also decreases significantly with an increasing temperature in the presence of the magnetic field, and further decrease appears at higher shear rates. It has been shown that the Herschel-Bulkley model with high accuracy at room temperature is no longer able to accurately describe the shear stress of MRFs at high temperatures causing the error up to 21.4% at 70 °C. The decrease in shear stress caused by the temperature rises is mainly due to the change in hydrodynamic force between particles and base carrier fluid. The hydrodynamic force follows the Navier-Stokes law where the temperature-dependent viscosity of base carrier fluid follows the Andrade’s formula. Therefore, a new constitutive model with temperature prediction was formulated and tested showing the small error at different temperatures and magnetic field strengths. Xiong et al [59] investigated the temperature effect by making the yield stress testing device of MRFs in which the temperature field model is considered including enameled wire and assembly gap. And simulation works were carried out to compare with the measured results of the thermal conductivity of the coil winding and assembly gap. Then, the following results have been drawn: (i) When the temperature field reaches approximately 90 min, the steady-state balance is reached. (ii) The greater the thermal conductivity of the winding, the stronger the thermal conductivity to the surrounding material and the lower the maximum temperature of the testing device. As the gap increases, the heat dissipation of the testing device decreases, the steady-state temperature rises, and the average gap increases by 0.01mm. (iii) The thermal conductivity of the enameled wire is determined to be 3.06W/m2K and the assembly gap is 0.03mm. Kariganaur et al [60] presented the temperature effect of MRF on performance while the damper device subjected to different load parameters was working using an embedded thermocouple. The results reveal that the fluid temperature rises significantly from atmospheric to 125.39 ◦C with decrease in damping force by 66.32% at higher loading parameters. More specifically, the following results were obtained: (i) From the sedimentation measurements, the sedimentation rate was found to be reducing exponentially with the rise in temperature of the MRF. (ii) The viscosity of MRF decreases exponentially as the temperature increases and increases significantly with external applied magnetic field strength. (iii) The reduction in damping force as temperature increases is an exponential drop with R2 = 0.9989. The saturation point of magnetization before the synthesis of MRFs reveals an 8.28% decrease at higher temperatures than atmosphere temperature. (iv) The heat treatment study of CIP showed that destabilization of the damper is possible if the system operates at higher temperatures for a prolonged time.
Ji et al [61] studied the sedimentation stability, expansion rate, volatilization rate, and temperature–viscosity property of MRFs in a high-temperature environment. MRFs were composed of soft magnetic particles, surfactants and the base carrier liquid since both the soft magnetic particles and base liquid influence the properties of MRF at high temperatures. It has been shown that the proposed MRF has excellent sedimentation stability, of which the sedimentation rate is only 4.42% after heat treatment at 150°C for one-week placement. It has been also observed that at 30°C, the shear yield stress of the novel fluid is 9.47 kPa under the magnetic field of 817 mT showing higher than the general MRFs with the same mass fraction. The yield shear stress is less affected by the high-temperature environment, reducing by only 4.03% from 10°C to 70°C. Zakinyan et al [62] investigated the effect of thermal percolation in a magnetic fluid composite by changing the content of conducting microparticles in a low-conducting medium. It has been shown that the effect of the magnetic field on the thermal conductivity of the composite material is significant at the concentration of conducting microparticles above the percolation threshold and much less pronounced otherwise. The relative increase in thermal conductivity can exceed 600% under the influence of a magnetic field parallel to the heat flux and is achieved in fields of intensity. In addition, it has been found that the magnetic field parallel to the heat flux in the sample significantly affects the thermal conductivity of magnetic fluid composites. In the home page of Lord Company [63], several types of commercial products of MR fluids such as MRF-122EG, MRF-132DG and MRF-140CG are provided. In this home page, two questions on the temperature problem of MRFs are appeared: (i) How does temperature affect MRFs? (ii) Is the heat dissipation an issue if MRF applications for a long-term operation? For the first question, the answer has been written as follows. In the off-state (no magnetic field applied), temperature effects are largely dependent on the liquid carrier fluid. Oil and silicone oil-based fluids can typically operate from -40 to 150°C. Water based fluids are rated from 0 to 70°C. Glycol can extend water-based fluids to operate below normal freezing temperatures. In the on-state (magnetic field applied), the magnetic viscosity effect is typically an order of magnitude greater than temperature viscosity effect, and so device performance is uniform and controllable across a wide range of temperatures. However, there is no information on the specific properties of MRFs at lower temperatures ranging from 0°C~ -40°C. As for the second question, the following answer was given. The heat dissipation depends on whether the damper (device) is also being stroked. If the damper coil is energized to produce the magnetic field but the piston is not moving, no energy is dissipated and there is no substantial generation of heat. When the damper is on and the piston is moving, the energy is dissipated as heat. When stroking the damper on a test machine under constant current for extended periods of time, active cooling may be required to keep the damper from overheating. For example, the RD-1005-3 MR linear damper reaches 120-140°C (external body temperature) if it is continuously stroked at 2 Hz and +/- 0.5 inches at 0.5 amp current in a room temperature environment with no active cooling. The recommended upper temperature for continuous use is 160°F (70°C). The heat dissipation heavily depends on the mechanism configuration of applications and number of magnetic coils (required power). In addition, an exact analysis of the temperature effect on the dynamic or control performances of MRF applications depends on the location and number of the temperature sensors such as thermocouple.
Table 3 summarizes principal results of major studies on the temperature effect on MRFs describing the problems and recipes to reduce the drawbacks due to the temperature effect. In the middle of 2000s, both Arrhenius relationship and Mason number are used to identify the thermal characteristics of MRFs and the relationship between the thermal conductivity and Mason number, saturation magnetization and Brownian suspension theory After that, several methods to release the temperature problem have been proposed by several scholars: addition of nanoparticles, addition of nano-sized additives, the use of non-spherical particles, the use of new constitutive models incorporating the temperature effect and proper choice of carrier liquids which are typically operated from -40 to 150°C. It is noted here that despite many recipes to reduce the temperature effect, there exist the trade-off properties such as the reduction of the viscosity and degradation of MRF application systems to be discussed in a subsequent section.
Table 3.
Studies on temperature and thermal conductivity in MRFs.
| Years | Summary of Main Results | Related References |
|---|---|---|
| ~1999 | N/A | |
| 2000~2009 |
|
[35,36] |
| 2010~2019 |
|
[37,38,39,40,41,42,43,44,45,46,47,48,49,50,51,52,53,54,55,56] |
| 2020~present |
|
[57,58,59,60,61,62,63] |
5. Thermal Effect in Application Systems
Among numerous applications as summarized in section 3, MR dampers are mostly studied and commercially available for automotive suspension systems. So far, various different types of MR dampers in terms of the structural configuration and magnetic circuit core have been applied to vibration control of the vehicle suspension system, vibration control of civil structures such as bridge cable and many types of the prosthesis including leg. However, one of significant drawbacks of MRF application systems including MR damper is the weakness to the operation in a wide range of the temperature, which results in performance degradation of the applications. Breese and Gordaninejad [64,65] firstly studied on the heat generation and dissipation of field controllable, MR damper via both experimental and theoretical studies. It is well known that MR dampers are energy-dissipating devices, and hence the issues of heat generation and dissipation are important. As a first step, applying various types of sinusoidal input motions to the damper, both heat dissipation and heat generation within the MR damper was tested and compared with the proposed theoretical model developed to predict the temperature increase of the MR dampers. It has been demonstrated that the theoretical model well predicts the measured results showing good accuracy, and a non-dimensional form of the governing equations has been developed to assess the effect of physical parameters on the heat dissipation and heat generation. Batterbee and Sims [66] presented the effects of temperature variation on MR damper in the temperature range from 15C to75°C by identifying the meaningful parameters of MR damper model considering the temperature. It has been firstly shown that the analysis from the model provide 34% drop in viscosity, 22% reduction in yield stress, and 300% increase in damper stiffness with rising temperature under certain conditions. Secondly, it has been shown that the temperature effect on the broadband excited single-degree-of-freedom mass isolator is evaluated by adopting proportional-integral-derivative (PID) controller and gain scheduling and on/off controller. From this test, it has been identified that RMS acceleration is enhanced, but RMS working space is degraded by increasing the temperature for each controller. Wilsom and Wereley [67] investigated the variation of the pressures of the fluid and the pneumatic accumulator due to the operating temperature. In this work, a theoretical model which can capture this change in stiffness due to self-heating while preserving the connection to the physical realization of the MR damper was proposed and validated considering the temperature from 0°C to 100°C. It was observed that the controllable yield force was found to decrease by up to 20%, the post-yield damping was found to decrease by over 60%, and the stiffness at high piston velocity was found to increase significantly. In addition, the proposed model considering the temperature effect was well validated to capture better stiffness behavior over a large operating temperature range. Zhang et al [68] presented the multi-physics of a new MR damper with a multistage piston and independent input currents. In order to analyze the multi-physics problem, a software COMSOL was used to analyze considering electromagnetic, thermal dynamic, and fluid mechanic. To evaluate the performance, the measurement index involving total damping force, dynamic range, and induction time needed for magnetic coil was put forward. For thermal analysis, it is considered that the heat is transferred in both the solid and the fluid domains and generated from self-inductance of the electromagnetic coil with fast-changing current and friction of the relative motion between the piston and the cylinder. It has been found that the majority of the temperature rise is caused by the friction inside the MR damper rather than self-induction from electromagnetic coils, and the maximum temperature occurs in the piston head around the coils. In addition, it has been identified that the temperature appears to be an exponential rising in the working gap at the beginning followed by slows down when the maximum temperature reaches about 45°C due to low thermal conductivity of MRF.
Saravanakumar and Ravikumar [69] studied the dynamic characteristics of MR squeeze film dampers used for the rotating balance (or vibration) of the aircraft jet engine rotor. As a first step, both the viscosity and dynamic characteristics due to the temperature increment were conducted showing that both characteristics are found to be decreasing with increase in temperature. Then, the temperature effects and the length/diameter (L/D) ratio effect on MR squeeze film dampers were tested considering the zone of high temperature gradients and high heat generation due to the current in the magnetic coils. It has been found that the dynamic characteristics is decreased with increase in shear strain rate but gets improved when the magnetic field intensity is increased. This behavior tends to deteriorate when the temperature is increased as the viscosity decreases. Thus, it has been suggested that both the damping and stiffness coefficients of the squeeze film damper can be enhanced by increasing the magnetic field intensity. Ferdaus et al [70] studied the temperature effect on the performances of MR dampers since conventional MR dampers normally face wide variations in temperature due to the magnetic coils attached in the piston head resulting in the creation of the heat. It has been shown that fluid viscosity has a direct relation with temperature, and hence dynamic performance of MR dampers. One of possible candidates to resolve the temperature effect is to change the design configuration by positioning the electromagnet coils to minimize the heat generation under the magnetic field. As for the MRF itself, the use of carrier liquid which is not sensitive to the temperature is one of possible solutions. Yu et al [71] formulated a theoretical model of temperature change of a self-decoupling magnetorheological (SDMR) and the prototype was manufactured to test the heat dissipation requirements. As a first step, the temperature performance test has been undertaken showing that as the temperature rises, the damping force decline significantly since a high heat generation is occurred due to the operate the large-sized SDMR damper applicable to wind vibration control in civil engineering. In addition, it has been suggested that the finding of the optimal location of the magnetic circuits and exact analysis of the heat transfer of the MR damper depending on the geometrical configuration with proper dimensions. Huang et al [72] proposed a transmission method of MRF in disc type driven by shape memory alloy (SMA) spring with the thermal effect and tis torque responses due to the variation of the temperature. The flow and transmission of MRF between two discs were analyzed, and the equations of flow velocity and transmission torque of MRF between two discs were obtained. It has been found that MRFs flow between two discs can be approximated to laminar flow, and the transmission torque increased with the applied magnetic field. On the other hand, MRF was driven to work chamber by SMA spring and hence the electric current was also adjusted by temperature. Therefore, the transmission torque of MRF is consecutively controlled and the value of it increased with the temperature. Sarkar and Hirani [73] investigated the heat transfer when MRF is operated like a brake friction motion by assuming that the heat generation during the braking action is inevitably occurred. In this work, MRF with nano-silver and nano-copper particles was synthesized and investigated on the characteristics of the heat transfer rate and the demagnetizing effect of those particles on the shear stress of MRF. Five different MRFs, containing a different percentage of silver and copper nano particles, were synthesized. Shear stresses of all five MRFs were measured using magneto-rheometer and the flywheel-based MR brake experimental setup was used to analyze the performance of synthesized MRFs. “T” type thermocouples were used to measure the temperature distribution of the fabricated MR brake. The results of the temperature distribution of brakes containing five synthesized MRFs have been measured and compared. In addition, the maximum temperature on the MR brake housing surface of five different MR fluids were measured and found that the temperature difference (maximum temperature reading-minimum temperature reading) for MRF85 (without silver particles), MRF85_0.25Ag (o.25% silver particles), MRF85_0.5Ag (o.5% silver particles), MRF85_0.25Cu (0.25% copper), MRF85_0.50Cu (0.5% copper) were identified by 18°C, 12°C, 14°C, 16°C and 9°C respectively. Therefore, the increase in silver nano-particle percentage helps in uniform temperature distribution on the MR brake. The difference in the temperature around the periphery of the disk of MR brake may be due to misalignment between the disk and the MR brake housing. It was also observed that the difference in the MR gap and non-uniform distribution of MR particles caused the non-uniform distribution of temperature.
Paul et al [74] studied the vibration reduction when MRF was used for metal cutting. In the metal cutting, MRF is used to reduce the tool vibration, but a major problem in this case, both durability and temperature effect on the MRF properties. This work proposed one method to reduce temperature and to improve viscosity of MR fluids by infusing nanoparticles along with conventional MRFs. Both aluminum oxide and titanium oxide nanoparticles of 0.1% and 0.2% concentration by weight were considered and experimental tests to investigate the influence of nanoparticles on the performance of MRFs. In the cutting process, major parameters such as cutting temperature, cutting force, surface roughness and tool vibration during hard turning of AISI 4340 steel were considered. Two different nanoparticles Al2O3 and TiO2 having 0.1% and 0.2% weight, respectively, were used separately and series of experiments were carried out resulting in the following results: (i) The impregnation of the nanoparticles can provide better stability, higher viscosity and better cutting performance during hard process. (ii) For achieving better damping capability and cutting performance, the damper should be made with MRFs impregnated with titanium oxide ferro and magnetized with direct current of higher magnitude. (iii) Nanoparticles used along with MRFs reduce cutting temperature in MRF, especially outside the core. Mitrouchev et al [75] proposed a methodology for calculation some principal characteristics of the damper such as electromagnet’s magnetic field value, emitted heat and damping force. The methodology was based on analytic calculations of the characteristics and finite element method analysis. It has been found that two main geometrical characteristics of the damper, namely, the piston thickness and electromagnet width are optimally chosen, thus allowing to reach maximum damping force. In addition, it has been identified that the heating of solenoid is the biggest uncertainty at the highest voltage. Specifically, the maximum power of the electromagnet was identified 15 W when the heating up did not exceed 100 °C within 30 min of continuous operation where the maximum damping force was reached at the piston thickness of 10mm and electromagnet width of 26 mm.
Parlak et al [76] experimentally investigated the performances of MR damper considering specified parameters including the gap width, the active length, the gap length and the radius of the piston core. The tests of manufactured nine dampers were carried out under constant damping velocity at constant 300°C with checking by the thermocouples placed in the piston head where the varying temperatures affect to the performance of the damper is most significant resulting in degradation of the damper responses. It has been shown that the effect of the gap width, which is the most dominant, increases with the increasing velocity by just 5.56 %, while the effect of the active length decreases by 5.13% to achieve the maximum damping force. In addition, it has been found that the effects of all parameters are almost unchanged with velocity for maximum dynamic range directly indicating that both the velocity to achieve maximum force and dynamic range could not be realized in real environment. Wang et al [77] investigated the effect of temperature on the transmission characteristics of high-torque MR brakes by theoretically analyzing a disc brake, the pressure characteristics of the MRF in axial direction, and the heat dissipation. A high-torque squeezing MR brake equipped with a water cooling system for heat dissipation was designed and manufactured. It has been found that even though MR brake is manufactured to be operated in the desired workable pressure characteristics for the high-torque transmission range, the braking torque exhibited a variation trend that has been initially increasing then decreasing when the temperature increased from 25 °C to 150 °C. The magnitude of decrease was approximately 210 N m, which decreased from 1800 to 1590 N m. It has been also observed that the temperature of the MRF presents an almost linear increase at different slip powers and the rate of temperature rise increases with an increase in slip power. The results presented in this work directly indicate that an effective cooling method can be vital to the stable operation of the heat dissipation in high-torque and high-power MR devices including high-torque MR brake. Gołdasz and Sapinski [78] carried out a numerical study to analyze both heat generation and heat dissipation mechanisms within MR damper operating in squeeze-mode. In this numerical experiment, an exemplary squeeze-mode device was evaluated for temperature changes (0°C~40°C) resulting from mechanical and electrical inputs, respectively. The proposed model based on the Stefan model was coupled considering the lumped parameters of the electromagnetic circuits. It has been shown that the obtained data reveal the effects of temperature rise and force changes upon stroking, and hence control studies involving operating and environmental conditions such as long operation times and low-temperature (cold) warm-up cycles should be carried out in future. Song et al [79] reviewed thermal and tribological properties of a cylindrical MR brake operated by the shear mode. As a first step. A theoretical analysis of the heating and heat dissipation of the MR brake was performed, and the transient temperature behaviors of the MR brake were simulated using the finite element method. It has been identified that the increment of the temperature of MRF domain is heavily dependent on the working gap. The smaller working gap, the higher increment of the temperature is occurred. This directly indicates that when the working gap is very small, an appropriate cooling measure needs to be adopted to avoid the degradation of the braking performances. In addition, several heating and wear tests were conducted on the MR brake and worn surfaces of the friction plates were observed using SEM to understand tribological characteristics of the MR brake. It has been shown from the results that the working gap significantly affects the wear property and tribological characteristics, and these behaviors cause the performance degradation of MR Brake.
Versaci et al [80] presented a steady-state magnetic induction fields with associated steady-state thermal stresses and a consequent constant yield stress of MRF using the finite element method. Especially, since steady-state electric current circulates in the coil of the device, a magneto-static analysis of the device and particularly of the strip of MRF contained therein is analyzed since the device is subject to heating during operation, and a thermo-static analysis is also carried out considering different external temperature. Since MRFs change their rheological behaviors as the absolute temperature T changes, MR damper subject to temperature changes due to climates of intense cold or particularly overheating conditions, a thermostatic analysis to evaluate the temperature distribution needs to be carried out. It has been observed that the influence of the temperature on both the magnetization of the particles and the MRF is significant in the MRF strip. On the other hand, the coercivity decreased as the temperature increased mainly due to the fact that the temperature rise accelerates the atomic motion and therefore disorders the magnetic moment orientation of the particles, which results in a reduction in the magnetic moment. In addition, it has been identified that the influence of temperature affects its global viscosity in the sense that, indeed, by increasing the temperature. Liu and Wu [81] analyzed mechanical properties of the two special dampers installed in the naval gun test shell using FEA and CFD models. It has been found that both models well analyze the dynamic characteristics of MR damper and colloidal damper. Especially, the results of quasi-static experiment and simulation have a high matching degree, which proves that the model is accurate. The energy absorption rate of colloidal dampers under impact is 97.8%, while the MR damper has a higher energy absorption rate, which reaches to almost 100%. In addition, it has been shown that multiple vortices with different sizes appeared in MR damper during the dynamic impact process, while the streamline of colloidal damper is stable without vortex generation. On the other hand, the heat of damper was mainly generated in the annular gap. Due to the improved fluidity, the final temperature distribution of MR damper was more uniform, and the temperature of colloidal damper was concentrated near the annular gap. This means that MR damper has higher resistance peaks, longer recovery time, and lower recovery velocity compared with colloidal fluid damper. Du et al [82] proposed a new MR damper by adding an aluminum foil bubble insulation material with a low thermal conductivity in the cavity between the electromagnetic coil and the MRF to avoid a rapid rise in the temperature of the MRF in the working process of the damper. First, the temperature-dependent rheological properties of the MRF were tested by a rheometer, squeezing and stretching tests at different temperatures to identify the relationship between the dynamic viscosity and the shear stress, squeezing stress, stretching stress and temperature. It has been found that the dynamic viscosity decreases when the temperature and shear rate increase. The fitting formula presented in this paper can reflect the change in the dynamic viscosity of MRF with the temperature and shear rate. In addition, the squeezing stress and stretching stress decrease with increasing temperature, and the amplitude of decrease will increase with increasing strain. When the temperature of the electromagnetic coil rises from 30°C to 70°C, the numerical simulation shows that compared with the rate of increase in the MRF of the damper without the heat insulation device, that of the damper with the heat insulation device will decrease by 57.4% in the working area. In addition, with the increasing temperature of the electromagnetic coil, the temperature decreases by 22.9%∼46.0% with insulation, and this difference will continue to increase with the temperature of the electromagnetic coil. It has been also identified that the rate of increase in MRF temperature in the working area of the damper with the insulation material could be reduced by 57.4% compared to that of the damper without the insulation material. Parlak et al [83] identified the relationship between damping performance and temperature in control algorithms using a new methodology which considers dynamic behaviors of MR damper depending on temperature based on the Bouc-Wen model. After evaluating seven parameters in the Bouc-Wen model related with the temperature, damping force has been defined depending on temperature with a single equation to simplify the control process. Thus, damping force has been defined depending on temperature with a single equation that significantly simplifies the control process. The temperature considered in this work is ranging from 20°C to 70°C. It has been demonstrated that the damping force error evaluated from experiment and model is small varying from %0.89 to %8.4. The reduction of damping force of MR damper was caused from both the reduced viscosity of the carrier liquid and the reduced yield stress of MRF as the temperature increased. Chen et al [84] investigated on the heat dissipation of high-power MR transmission devices owing to wall slip, particle friction, and exciting current by utilizing a new water-cooling structure in which the negative effect of centrifugal force on heat dissipation is operated. The magnetic circuit of the MR transmission device was designed on the basis of electromagnetic theory, and the magnetic field was investigated by finite element calculation using the exciting coil with diameter of 1 mm and turns of 2400. It has been shown that the magnetic field strength increases with exciting current and decreases with the increase in the width of working gap, but it does not change significantly when the magnetic conduction arc becomes thicker. The proposed MR transmission device is set as the designed maximum transmission power (7.5 kW) to verify its heat dissipation effects under extreme condition. After cooling water flowing into the cooling channel, the temperature of MRF in the device drops sharply from 70 °C to 28 °C, decreasing by 60 %. It has been found that the power transmission device has excellent heat dissipation effect indicating better performance considering new structures with the radius and of the magnetic conduction arc and the number of transmission discs.
Xiao et al [85] investigated the transient temperature distribution pattern inside the magnetorheological MR grease torsional vibration damper. As a first step, a theoretical heat transfer model of MR torsional vibration damper with dual heat source structural feature was established based on the Bingham constitutive model and the temperature-dependent viscosity characteristics. Form the theoretical model, it has been shown that the temperature increment of MR greases in the working domain is the fastest, but a gradual slowing of the temperature rise rate. The magnitude and rate of temperature rise are maximum when the 1A current is applied to the torsional vibration damper. The current–temperature curve was obtained by fitting the simulation results. The results of the analysis reveal the internal temperature distribution and temperature rise characteristics of the torsional vibration damper. In addition, it has been demonstrated that the temperature of both end surfaces gradually increases from the center of the circle along the radial direction. The maximum temperature of each working condition was collected and fitted to obtain the relationship between current and temperature. By analyzing the five working conditions, when the applied current is 0~1A, the increase in MR greases viscosity leads to an increase in the rate and magnitude of temperature rise inside the device. Jacob et al [87] presented a hybrid damper concept using a combination of MRF and shape memory alloy (SMA)-based energy dissipation. Especially, in this work, it has been demonstrated that the operation of MR and SMA dampers complement each other to compensate for each other’s weaknesses. In particular, the slow response from the MR damper is compensated by passive SMA damping using the pseudoplastic effect of martensite reorientation, which can dissipate the significant amount of shock energy at the beginning of the shock occurrence. The MR damper compensates for the incapability of the SMA to dampers. The inability of the SMA to continuously dampen the subsequent vibration was taken care of by the MR damper, which could dissipate the energy as long as a magnetic field was applied. Therefore, the SMA damper can be used in wider application, while the energy consumption of the MR damper can be reduced. The energy needed for the MR damper decreases with increasing shock loading amplitudes, showing improved settling behavior owing to enhanced energy dissipation by the SMA damper. Specifically, a reduction of up to 96% in energy consumption was achieved for the MR damper, as the current excitation requirement was reduced from 5 A to 1 A. This mutual integration concept in a hybrid form can open the possibility of reducing the MR damper’s construction volume and the temperature effect. Szelag et al [86] proposed a field model of coupled phenomena occurring in an axisymmetric MR brake focusing on transient fluid dynamics, electromagnetic property and thermal fields. The influence of temperature on the electromagnetic and rheological properties of the used materials has been also taken into account using the finite element method and a step-by-step algorithm to figure out coupled phenomena considered MR brake. The nonlinearity of the magnetic circuit and rheological properties of MRF as well as the influence of temperature on the properties of materials have been tested and the Newton–Raphson method and the coupled block over-relaxation method have been implemented. The elaborated algorithm has been successfully used in the analysis of the phenomena in the considered MR brake. It has been verified that the accuracy of the developed model and its usefulness have been demonstrated by a comparative analysis of the results of simulation and laboratory tests of MR bake. It was also remarked that the successful development of this technology required on the durability of MRF in a wide temperature range and the robustness of the applied systems.
Table 4 presents the summary of the temperature effect on the working performances of various MRF application systems. It is clearly seen that MRFs are applied to special systems such as a high-power transmission system to investigate the heat generation and temperature distribution. Many studies have been focused on the several recipes to reduce the thermal effect during the working operation. As one of potential methodologies to minimize the thermal effect of MRF application systems is to optimize the location of the magnetic circuits considering both the temperature distribution and distribution of the heat generation. In addition, it has been known that there are several trade-off behaviors between the temperature effect and application performance. For example, the addition of nanoparticles and proper additives can mitigate the temperature effect, but the field-dependent damper force of MR damper is decreased.
Table 4.
Studies on thermal behaviors in MRF applications.
| Years | Summary of Main Results | Related References |
|---|---|---|
| ~ 1999 | The heat generation and dissipation of MR damper was studied and a theoretical model was developed which could predict the temperature variation of MR damper. It has been shown that the result achieved from the developed non-dimensional model is well agreed with experimental result by assessing the physical parameters. | [63] |
| 2000~2009 | The performance of MR damper such as damper stiffness has been investigated in the temperature range from 15°C to 75°C. It has been shown that 34% drop in viscosity, 22% reduction in yield stress, and 300% increase in damper stiffness with rising temperature under certain conditions. | [64,65] |
| 2010~2019 |
|
[66,67,68,69,70,71,72,73,74,75,76,77,78,79] |
| 2020~present |
|
[80,81,82,83,84,85,86,87,88] |
6. Concluding Remarks
In this paper, review articles about MRFs and their application systems were revisited and summarized in a chronologically manner to comprehensively figure out the state-of-the-art on the MRF technology. Then, as a major technical contribution, many studies on the temperature and thermal conductivity of MRFs and heat generation of MRFs applications were investigated and principal results were summarized which have been mostly achieved for last two decades. It has been identified from the research survey that even though many different recipes to reduce the temperature effect of MRFs have been carried out, there are still challenging works to be resolved. For example, the optimal ratio of the additives over the particle volume, the proper ratio of the nano-sized particles over the micro-sized particles, the optimal flow gap based on the magnetic circuits and so forth. As for the application system works, even though many devices and systems have been proposed and tested in a laboratory level, there are impediments to solve for successful realization in real environment. For example, the number of magnetic circuit cores, the analysis of the heat transfer, the optimal geometry considering the diameter and length, the hybrid design with different materials and so on are to be resolved. In the review investigation on the temperature effect, the formulation of the accurate model of temperature rise due to the magnetic coils driven by the input current, the analysis of the relationship between the semi-active system (energy dissipation only) and temperature variation, electrical analysis using power circuit consisting of the resister and capacitor, and the analysis of the temperature distribution of application system have been discussed in detail.
The main review results on the temperature effect are shown in Figure 1 from several aspects. It is clearly seen that the inherent properties of MRFs are weakened due to the temperature increment, and hence several studies to reduce the temperature effect have been done. For example, the addition of the nano-sized particles and additives and use of carrier liquids which are less sensitive to the temperature can reduce the property degradation caused from the temperature increment. The temperature problem directly affects dynamic or control performances of many MRF application systems. Thus, recently several works are being actively studied on the formulation of the constitutive models of MRFs considering the temperature effect. These models are used from the beginning of the design stage to compensate the temperature effect. Despite many works on the temperature and thermal effect in MRF technology, there are many things to be resolved for successful realization in practical fields. As one of challenging works is to find the optimal location of the flow gap, in which MRF flows in a narrow gap, and magnetic circuit cores causing the temperature increment from the heat generation. Therefore, thermal effect of application systems can be significantly reduced through the analysis of the heat transfer of the flow path associated with the magnetic distribution.
It is finally remarked that several review articles on MRFs and their application systems have not been cited in this paper since major review contents of the articles are similar to those presented in the references cited in this work. Moreover, a few studies on the temperature effect and thermal conductivity of MRFs and their application systems have been investigated, but not cited in this article to avoid the redundancy of the research contents.
Figure 1.
Temperature effects in MRFs and application systems.
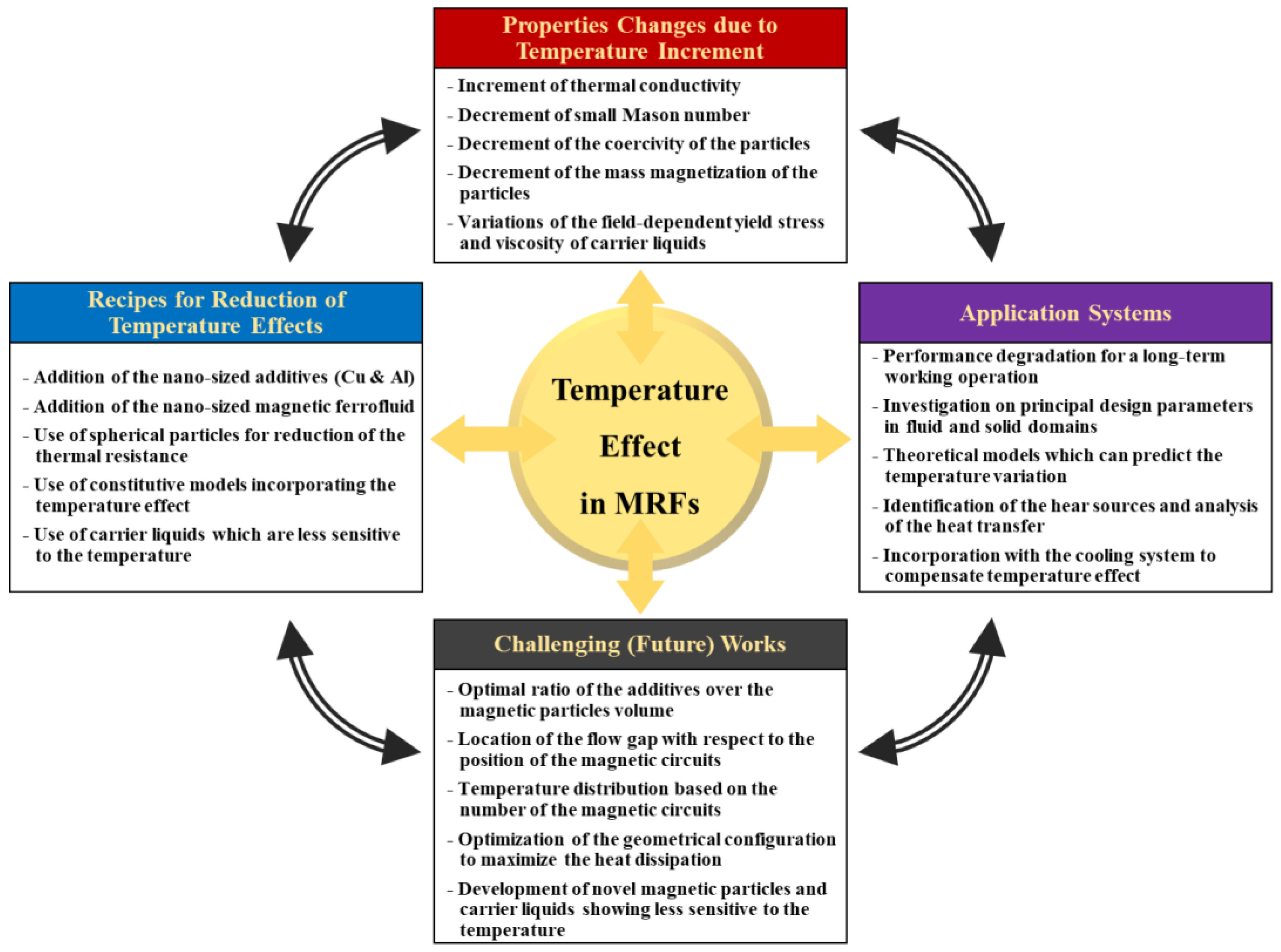
Author Contributions
Conceptualization, Methodology, Data Collection and Analysis, Writing and Review: S. B. C.
Funding
None.
Conflicts of Interest
The author declares that there is no conflict of interest.
References
- The Magnetic Fluid Clutch by J. Rabinow. AIEE Transactions 1948, 67, 1308–1315.
- Lord Company homepage: https://en.wikipedia.org/wiki/Lord_Corporation. 2023.
- Lord Magneto-Rheological Fluids: https://www.shoplordmr. 2023.
- Carlson, J.D. MR fluids and devices in the real world. International Journal of Modern Physics B 2005, 19, 1463–1470. [Google Scholar] [CrossRef]
- Muhammad, A.; Yao, X.L.; Deng, Z.C. Review of magnetorheological (MR) fluids and its applications in vibration control. Journal of Marine Science and Application 2006, 15, 17–29. [Google Scholar] [CrossRef]
- Ierardi, R.F.; Bombard, A.J.F. Off-state viscosity and yield stress optimization of magnetorheological fluids: a mixture design of experimental approach. Journal of Physics: Conference Series 2009, 149, 1–5. [Google Scholar] [CrossRef]
- de Vicente, J.; Klingenberg, D.J.; Hidalgo-Alvarez, R. Magnetorheological fluids: a review. Soft Matter 2011, 7, 3701–3710. [Google Scholar] [CrossRef]
- Ashtiani, M.; Hashemabadi, S.H.; Ghaffari, A. A review on the magnetorheological fluid preparation and stabilization. Journal of Magnetism and Magnetic Materials 2015, 374, 716–730. [Google Scholar] [CrossRef]
- Choi, S.B. Sedimentation Stability of Magnetorheological Fluids: The State of the Art and Challenging Issues. Micromachines 2022, 3. [Google Scholar] [CrossRef] [PubMed]
- Thiagarajan, S.; Koh, A.S. Performance and Stability of Magnetorheological Fluids—A Detailed Review of the State of the Art. Advanced Engineering Materials 2021, 2001458. [Google Scholar] [CrossRef]
- Pei, P.; Peng, Y. Constitutive modeling of magnetorheological fluids: A review. Journal of Magnetism and Magnetic Materials 2022, 550. [Google Scholar] [CrossRef]
- Kumar, J.S.; Paul, P.S.; Raghunathan, G.; Alex, D.G. A review of challenges and solutions in the preparation and use of magnetorheological fluids. International Journal of Mechanical and Materials Engineering 2019, 14. [Google Scholar] [CrossRef]
- Matharu, M.S.; Sehgal, S.S. Role of Particle Shape, Material and Morphology in Magnetorheological Fluids- A Systematic Review. International Journal of Scientific &Technology Research 2020, 9. [Google Scholar]
- Khairi, M.H.A.; Mazlan, S.A.; Ubaidillah, U.; Choi, S.B.; Aziz, S.S.A.; Mohamad, N.; Hapipi, N.; Nordin, N. Role of Additives in Enhancing the Rheological Properties of Magnetorheological Solids: A Review. Advanced Engineering Materials 2019, 21, 1800696. [Google Scholar] [CrossRef]
- Imaduddin, F.; Mazlan, S.A.; Zamzuri, H. A Design and Modeling Review of Rotary Magnetorheological Damper. Materials & Design 2013, 51, 575–591. [Google Scholar] [CrossRef]
- Kaluvan, S.; Kim, H.C.; Oh, J.S.; Choi, S.B. A State-of Art of Creative Sensor Devices Utilizing Magneto- Rheological Fluids. International Journal of Mechanical Systems Engineering 2015, 1, 1–7. [Google Scholar] [CrossRef]
- Do, X.P.; Choi, S.B. High Loaded Mounts for Vibration Control Using Magnetorheological Fluids: Review of Design Configuration. Shock and Vibration 2015, 915859. [Google Scholar] [CrossRef]
- Ahamed, R.; Ferdaus, M.M.; Li, Y. Advancement in energy harvesting magneto-rheological fluid damper: A review. Korea-Australia Rheology Journal 2016, 28, 355–379. [Google Scholar] [CrossRef]
- Choi, S.B.; Li, W.; Yu, M.; Du, H.; Fu, J.; Do, P.X. State of the art of control schemes for smart systems featuring magneto-rheological materials. Smart Materials and Structures 2016, 25, 043001. [Google Scholar] [CrossRef]
- Kumari, C.; Chak, S.K. A review on magnetically assisted abrasive finishing and their critical process parameters. Manufacturing Review 2018, 5. [Google Scholar] [CrossRef]
- Daniel, C.; Hemalatha, G.; Jebadurai, S.V.S.; Sarala, L.; Tensing, D.; Christy, S.S.J.E. A Review on the Magnetorheological Fluid, Damper and Its Applications for Seismic Mitigation. Civil Engineering Journal 2018, 4. [Google Scholar] [CrossRef]
- Sohn, J.W.; Kim, G.W.; Choi, S.B. A State-of-the-Art Review on Robots and Medical Devices Using Smart Fluids and Shape Memory Alloys. Applied Sciences 2018, 8, 1928. [Google Scholar] [CrossRef]
- Ahamed, R.; Choi, S.B.; Ferdaus, M.M. A state of art on magneto-rheological materials and their potential applications. Journal of Intelligent Material Systems and Structures 2018, 29, 2051–2095. [Google Scholar] [CrossRef]
- Oh, J.S.; Choi, S.B. A Review on the Development of MR Dampers Utilizing Smart Magnetorheological Fluids. Current Smart Materials 2019, 4, 15–21. [Google Scholar] [CrossRef]
- Kolekar, S.; Venkatesh, K.; Oh, J.S.; Choi, S.B. Vibration Controllability of Sandwich Structures of Electrorheological Fluids and Magnetorheological Materials: A Review. Journal of Vibration Engineering & Technologies 2019, 7, 359–377. [Google Scholar] [CrossRef]
- Yuan, X.; Tian, T.; Ling, H.; Qiu, T.; He, H. A Review on Structural Development of Magnetorheological Fluid Damper. Shock and Vibration 2019, 1498962. [Google Scholar] [CrossRef]
- Lu, H.; Hua, D.; Wang, B.; Yang, C.; Hnydiuk-Stefan, A.; Królczyk, G.; Liu, X.; Li, X. The Roles of Magnetorheological Fluid in Modern Precision Machining Field: A Review. Frontiers in Materials 2021, 8. [Google Scholar] [CrossRef]
- Eshgarf, H.; Nadooshan, A.A.; Raisi, A. An overview on properties and applications of magnetorheological fluids: Dampers, batteries, valves and brakes. Journal of Energy Storage 2022, 50. [Google Scholar] [CrossRef]
- Liu, G.; Gao, F.; Wang, D.; Liao, W.H. Medical applications of magnetorheological fluid: a systematic review. Smart Materials and Structures 2022, 31. [Google Scholar] [CrossRef]
- Aziz, M.A.; Mohtasim, S.M.; Ahammed, R. State-of-the-art recent developments of large magnetorheological (MR) dampers: a review. Korea-Australia Rheology Journal 2022, 34, 105–136. [Google Scholar] [CrossRef]
- Do, X.P.; Choi, S.B. A state-of-the-art on smart materials actuators over the last decade: control aspects for diverse applications. Smart Materials and Structures 2022, 31, 053001. [Google Scholar] [CrossRef]
- Fardan, M.F.; Lenggana, B.W.; Ubaidillah, U.; Choi, S.B.; Susilo, D.D.; Khan, S.Z. Revolutionizing Prosthetic Design with Auxetic Metamaterials and Structures: A Review of Mechanical Properties and Limitations. Micromachines 2023, 14, 1165. [Google Scholar] [CrossRef] [PubMed]
- Masa’id, A.; Lenggana, B.W.; Ubaidillah, U.; Susilo, D.D.; Choi, S.B. A Review on Vibration Control Strategies Using Magnetorheological Materials Actuators: Application Perspective. Actuators 2023, 12. [Google Scholar] [CrossRef]
- Karnopp, D. Active and Semi-Active Vibration Control, ASME Special 50th Anniversary Design Issue 1995, 117. https://mechanicaldesign.asmedigitalcollection.asme.org/ on 01/29/2016.
- Zschunke, F.; Rivas, R.; Brunn, P.O. Temperature Behavior of Magnetorheological Fluids. Applied Rheology 2005, 15, 116–121. [Google Scholar] [CrossRef]
- Heine, M.C.; de Vincente, J.; Klingenberg, D.D. Thermal Transport in Sheared Electro-and Magnetorheological Fluids. Physics of Fluids 2006, 18. [Google Scholar] [CrossRef]
- Ocalan, M. Magnetorheological Fluids for Extreme Environments: Stronger, Lighter, Hotter, Ph.D. Degree, Mechanical Engineering of MIT, 2011.
- Yildirim, G.; Genc, S. Experimental Study on Heat Transfer of the Magnetorheological Fluids. Smart Materials and Structures 2013, 22. [Google Scholar] [CrossRef]
- Chang, H.; Hong; Liu, C. Study on preparation and thermal conductivity of high stability magnetorheological fluids. Journal of the Chinese Advanced Materials Society 2014, 2, 130–137. [CrossRef]
- Bilyk, V.; Korobko, E.; Bubulis, A. Estimation of performance characteristics of a controlled shock-absorber affected by the magnetic field and temperature on rheological properties of the magnetorheological fluid, Vibro-engineering PROCEDIA, 2014 3. 331-336, ISSN 2345-0533.
- Wang, D.; Zi, B.; Zeng, Y.; Hou. Y.; Meng, Q. Temperature-dependent material properties of the components of magnetorheological fluids. Journal of Material Science 2014, 49, 8459–8470. [CrossRef]
- Mistik, S.I.; Shah, T.; Hadimani, R.L.; Siores, E. Compression and Thermal conductivity Characteristics of Magnetorheological Fluid-spacer Fabric Smart Structures. Journal of Intelligent Material Systems and Structures 2015, 23. [Google Scholar] [CrossRef]
- Chen. S.; Huang, J.; Jian, K.; Ding, J. Analysis of Influence of Temperature on Magnetorheological Fluid and Transmission Performance. Advances in Materials Science and Engineering 2015, 583076. [CrossRef]
- Rahim, M.S.; Ismail, I. Review of magnetorheological fluids and nanofluids thermal behaviour, IOP Conf. Series: Materials Science and Engineering 2015, 100, 012040. [Google Scholar] [CrossRef]
- Sherman, S.G.; Powell, L.A.; Becnel, A.C.; Wereley, N.M. Scaling temperature dependent rheology of magnetorheological fluids. Journal of Applied Physics 2015, 117, 17C751. [Google Scholar] [CrossRef]
- Yang, C.; Bian, X.; Qin, J.; Guo.; Zhao, S. Fabrication and hyperthermia effect of magnetic functional fluids based on amorphous particles. Applied Surface Science 2015, 330, 216–220. [CrossRef]
- Rabbani, Y.; Ashtiani, M.; Hashemabadi, S.H. An experimental study on the effects of temperature and magnetic field strength on the magnetorheological fluid stability and MR effect. Soft Matter 2015, 22. [Google Scholar] [CrossRef]
- Shah, K.; Choi, S.B.; Choi, H.J. Thermorheological properties of nano-magnetorheological fluid in dynamic mode: experimental investigation. Smart Materials and Structures 2015, 24, 057001. [Google Scholar] [CrossRef]
- Sherman, S.G.; Powell, L.A.; Becnel, A.C.; Wereley, N.M. Scaling temperature dependent rheology of magnetorheological fluids. Journal of Applied Physics 2015, 117, 17C751. [Google Scholar] [CrossRef]
- Rahim, M.S.A.; Ismail, I.; Wahid, S.A.; Aqida, S.N. Magnetic field simulation of a thermal conductivity measurement instrument for magnetorheological fluid. MATEC Web of Conferences 2016, 90, 01061. [Google Scholar] [CrossRef]
- Forero-Sandoval, I.Y.; Vega-Flick, A.; Alvarado-Gil, J.J.; Medina-Esquivel, R.A. Study of thermal conductivity of magnetorheological fluids using the thermal wave resonant cavity and its relationship with the viscosity. Smart Materials and Structures 2017, 26. [Google Scholar] [CrossRef]
- Rahim, M.S.A.; Ismail, I.; Choi, S.B.; Azmi, W.H.; Aqida, S.N. Thermal conductivity enhancement and sedimentation reduction of magnetorheological fluids with nano-sized Cu and Al additives. Smart Materials and Structures 2017, 26. [Google Scholar] [CrossRef]
- McKee, M.; Gordaninejad, F.; Wang, X. Effects of temperature on performance of compressible magnetorheological fluid suspension systems. Journal of Intelligent Material Systems and Structures 2018, 29, 41–51. [Google Scholar] [CrossRef]
- Maroofi, J.; Hashemabadi, S.H. Experimental and numerical investigation of parameters influencing anisotropic thermal conductivity of magnetorheological fluids. Heat and Mass Transfer 2019, 55, 2751–2767. [Google Scholar] [CrossRef]
- Maroofi, J.; Hashemabadi, S.H.; Rabbani, Y. Investigation of the Chain Formation Effect on Thermal Conductivity of Magnetorheological Fluids. Journal of Thermophysics and Heat Transfer 2019. [Google Scholar] [CrossRef]
- Pisuwala, M.S.; Upadhyay, R.V.; Parekh, K. Contribution of magnetic nanoparticle in thermal conductivity of flake-shaped iron particles based magnetorheological (MR) fluid. Journal of Applied Physics 2019, 126, 055104. [Google Scholar] [CrossRef]
- Hemmatian, M.; Sedaghati, R.; Rakheja, S. Temperature dependency of magnetorheological fluids’ properties under varying strain amplitude and rate. Journal of Magnetism and Magnetic Materials 2020, 498, 166109. [Google Scholar] [CrossRef]
- Li, H.; Jönkkäri, I.; Sarlin, E.; Chen, F. Temperature effects and temperature-dependent constitutive model of magnetorheological fluids. Rheological Acta 2021, 60, 719–728. [Google Scholar] [CrossRef]
- Xiong, H.; Luo, Y.; Ji, D.; Ren, H.; Wei, D.; Liu, W. Analysis and evaluation of temperature field and experiment for magnetorheological fluid testing devices. Advances in Mechanical Engineering 2021, 13, 1–12. [Google Scholar] [CrossRef]
- Kariganaur, A.K.; Kumar, H.; M Arun, A. Influence of temperature on magnetorheological fluid properties and damping performance. Smart Materials and Structures 2022, 31, 055018. [Google Scholar] [CrossRef]
- Ji, J.; Wu, X.; Tian, Z.; Xie, F.; Chen, F.; Haopeng Li, H. Materials 2023, 16, 4207. [CrossRef]
- Zakinyan, A.R.; Zakinyan, A.A.; Mesyatseva, L.S. Thermal percolation in a magnetic field responsive composite. Chemical Physics Letters 2023, 813. [Google Scholar] [CrossRef]
- Lord Catalog. https://www.shoplordmr.com/. 2023.
- Darrell, G.; Breese, D.G.; Gordaninejad, F. Heating of Magnetorheological Fluid Dampers. Journal of Intelligent Materials Systems and Structures 1999, 10. [Google Scholar] [CrossRef]
- Douoz, M.B.; Gordaninejadh, F.; Eñc, L.; Stipanovichb, W.A.J. Heat transfer from magneto-rheological fluid dampers, In Smart Structures and Materials 2000: Smart Systems for Bridges, Structures, and Highways, 84 S. C. Liu. Editor, 2000, Proceedings of SPIE Vol. 3988, 0277-786X/OO/$15.00.
- Batterbee, D.; Sims, N.D. Temperature sensitive controller performance of MR dampers. Journal of Intelligent Material Systems and Structures 2009, 20, 297–309. [Google Scholar] [CrossRef]
- Wilsom, N.L.; and Wereley, N. Analysis of a Magnetorheological Fluid Damper Incorporating Temperature Dependence, 51st AIAA/ASME/ASCE/AHS/ASC Structures, Structural Dynamics, and Materials Conference, 2010, 12 April 2010 - 15 April 2010, Orlando, Florida, 2012. 12 April. [CrossRef]
- Zheng, J.; Li, Z.; Koo, J.H.; Wang, J. Magnetic Circuit Design and Multiphysics Analysis of a Novel MR Damper for Applications under High Velocity. Advances in Mechanical Engineering 2014, 402501. [Google Scholar] [CrossRef]
- Saravanakumar, G.; Ravikumar, L. 2, Effect of L/D Ratio and the Temperature on MR Fluid Squeeze Film Damper Performance. Asian Journal of Engineering and Applied Technology 2014, 3, 37–41. [Google Scholar] [CrossRef]
- Ferdaus, M.M.; Rashid, M.M.; Hasan, M.H.; Yosuf, H.B.M.; Bhuiyan, M.M.I.; Abdulaziz Alraddadi, A. Temperature Effect Analysis on Magneto-Rheological Damper’s Performance. Journal of Automation and Control Engineering 2014, 2. [Google Scholar] [CrossRef]
- Yu, G.; Du, C.; Tiger Sun, T. Thermodynamic Behaviors of a Kind of Self-Decoupling Magnetorheological Damper. Shock and Vibration 2015, 502747. [Google Scholar] [CrossRef]
- Huang, J.; Qiao, Z.; Fan, B. Properties of MR Transmission under Thermal Affect. International Conference on Advanced Manufacturing and Industrial Application 2015, 5890827. [Google Scholar] [CrossRef]
- Sarkar, C.; Hirani, H. Synthesis and Characterization of Nano-Particles Based Magnetorheological Fluids for Brake. Japanese Society of Tribologists 2015, 10. [Google Scholar] [CrossRef]
- Paul, P.S.; Iasanth, J.A.; Vasanth, X.A.; Varadarajan, A.S. Effect of nanoparticles on the performance of magnetorheological fluid damper during hard turning process. Friction 2015, 3, 333–343. [Google Scholar] [CrossRef]
- Mitrouchev, P.; Klevinskis, A.; Bucinskas, V.; Dragasius, E.; Udris, D.; Morkvenaite-Vilkonciene, I. Analytical research of damping efficiency and heat generation of magnetorheological damper. Smart Materials and Structures 2017, 26, 065026. [Google Scholar] [CrossRef]
- Parlak, Z.; Soyemez, M.E.; Kemerli, M.; Sahin, I. Multiphysics Numerical Analysis of MR Damper with Experimental Validation. International Journal of Multidisciplinary Studies and Innovative Technologies 2018, 2, 43–50. [Google Scholar]
- Wang, N.; Liu, X.; Krolczyk, G.; Li, Z.; Li, W. Effect of temperature on the transmission characteristics of high-torque magnetorheological brakes. Smart Materials and Structures 2019, 28. [Google Scholar] [CrossRef]
- Gołdasz, J.; Sapinski, B. Influence of Temperature on the MR Squeeze-Mode Damper, 2019 20th International Carpathian Control Conference (ICCC), Krakow-Wieliczka, Poland, 2019, pp. 1–6. [CrossRef]
- Song, W.L.; Wang, S.Y.; Choi, S.B.; Wang, N.; Xiu, S.C. Thermal and Tribological Characteristics of a Disc-Type Magnetorheological Brake Operated by the Shear Mode. Journal of Intelligent Material Systems and Structures 2019, 30, 722–733. [Google Scholar] [CrossRef]
- Versaci, M.; Cutrupi, A.; Palumbo, A. Magneto-Thermo-Static Study of a Magneto-Rheological Fluid Damper: A Finite Element Analysis. IEEE Xplore 2021, 57. [Google Scholar] [CrossRef]
- Liu, X.; Wu, A.D. Performance Evaluation of Dampers Under Dynamic Impact Based on Magnetic Field FE and CFD. IEEE Access 2020, 8, 174643–174653. [Google Scholar] [CrossRef]
- Du, C.; Zeng, F.; Liu, B.; Fu, Y. A novel magnetorheological fluid damper with a heat insulation function. Smart Materials and Structures 2021, 30, 075001. [Google Scholar] [CrossRef]
- Parlak, Z.; Soylemez, M.E.; Sahin, I. A New Methodology to Describe Non-Linear Characterization Depending on Temperature of a Semi-Active Absorber Based on Bouc-Wen Model. Gazi University Journal of Science 2022, 35, 624–1638. [Google Scholar] [CrossRef]
- Chen, F.; Wang, X.; Li, H.; Li, A.; Deng, Y.; Tian, Z.; Wu, X. A novel water-cooling magnetorheological transmission device. Journal of Mechanical Science and Technology 2022, 36. [Google Scholar] [CrossRef]
- Xiao, Z.; Hu, H.; Ouyang, Q.; Shan, L.; Su, H. 3, Multi-Condition Temperature Field Simulation Analysis of Magnetorheological Grease Torsional Vibration Damper. Frontiers in Materials 2022, 9, 930825. [Google Scholar] [CrossRef]
- Jacob, K.; Tan, A.S.; Sattel, T.; Kohl, M. Enhancement of Shock Absorption Using Hybrid SMA-MRF Damper by Complementary Operation. Actuators 2022, 11, 280. [Google Scholar] [CrossRef]
- Szelag, W.; Jedryczka, C.; Myszkowski, A.; Wojciechowski, R.M. Coupled Field Analysis of Phenomena in Hybrid Excited Magnetorheological Fluid Brake. Sensors 2023, 23, 358. [Google Scholar] [CrossRef] [PubMed]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Copyright: This open access article is published under a Creative Commons CC BY 4.0 license, which permit the free download, distribution, and reuse, provided that the author and preprint are cited in any reuse.
MDPI Initiatives
Important Links
© 2024 MDPI (Basel, Switzerland) unless otherwise stated





