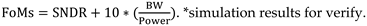Prerpints.org logo
Preprint
Article

Low-power Multi-bit Delta-Sigma Modulator based on Passive and Attenuationless Summation Scheme

Altmetrics

Downloads

214

Views

79

Comments

1

Submitted:

04 December 2023

Posted:

05 December 2023

You are already at the latest version

Alerts
Abstract
In the field of delta-sigma modulators, reducing system power consumption without sacrificing accuracy has become a challenge. The summing circuit, as the main part of the cascade-of-integrator-in-feedforward delta-sigma modulator, consumes a significant amount of power in active summing. On the other hand, passive summing circuits have low power consumption but can cause attenuation in the summing results. This article proposes a low-power multi-bit delta-sigma modulator based on passive and attenuationless summation scheme. The summation circuit achieves multiplication of the voltage signal carried on the summation capacitor through bidirectional sampling technique, compensating for the inherent attenuation caused by passive summation, thus eliminating the need for active OTA to achieve perfect summation. A 2-order delta-sigma modulator based on 4-bit 1-order passive noise-shaping (NS) SAR quantizer is designed to verify the scheme. By combining a passive NS SAR as the 4-bit quantizer and the third-stage integrator of the system, and incorporating an energy-efficient cascode floating inverter amplifier (FIA) structure as the core active OTA, these elements have significantly enhanced the energy-efficiency of the modulator. Simulation results show that at a supply voltage of 1.2 V and a bandwidth of 20 kHz, the SNDR reaches 102.62 dB, power consumption is only 148.32 μW, and Schreier figure-of-merit (FoM) of SNDR is 183.92 dB.
Keywords: 
Subject: Engineering  -   Electrical and Electronic Engineering

1. Introduction

With the rapid development of IoT, sensors powered by batteries are needed, so in addition to high accuracy, low power consumption is also an important requirement for delta-sigma modulators (DSM) [1,2,3,4,5,6,7]. Therefore, how to balance between high accuracy and low power consumption has become the focus of exploration in the field of delta-sigma modulators.
With the popularity of the full feedforward delta-sigma topology [8,9,10,11], the summation circuit has become an important component of multi-bit DSM. Conventional summation circuits use active operational transconductance amplifiers (OTAs) as the core to sum up the signals at each node of the circuit, consuming a large amount of active power [12,13,14,15]. In order to reduce this part of power consumption, passive summation schemes have been widely adopted. However, passive summation circuits directly parallel capacitors carrying the voltage signals of each branch [1,16,17,18,19,20], which reduces power consumption but also causes attenuation of the summation result. This makes the design requirements for multi-bit quantizers more stringent, which is not desirable.
This paper proposes a low-power multi-bit DSM based on passive and attenuationless summation. This circuit aims to achieve coefficient multiplication of the summation through bidirectional sampling technology compared to conventional passive summation schemes, thereby compensating the attenuation caused by summation and achieving attenuationless summation in a passive manner. This saves active power and greatly relaxes the design requirements for multi-bit quantizers.
Due to the recent popularity of the noise-shaping (NS) SAR ADC structure, which improves on the SAR structure by sampling and integrating the residual voltage generated by SAR, and achieving noise-shaping characteristics similar to the DSM structure in a passive manner [21,22,23,24,25] , the NS SAR structure can not only be used as a quantizer for coarse quantization of DSM, but also replace the active integrator in DSM in a passive manner, with high energy-efficiency. Therefore, in order to improve energy-efficiency, the DSM proposed in this paper integrates a 1-order NS SAR based on 4-bit SAR quantizater, allowing the NS SAR to act as a third-stage integrator and quantizer in a passive manner.
The first and second stage integrators in the DSM must use active OTA as the core module. In order to further improve energy-efficiency, the floating inverter amplifier (FIA) architecture with self-extinguishing characteristics is used by DSM [17,26,27,28,29,30]. Since the essence of FIA is to work in the form of inverters, which have great energy-efficiency advantages, FIA optimizes the power supply method of inverters on this basis, transforming the constant power supply mode into a floating capacitor power supply mode. Due to the limited power of capacitors, FIA has a natural self-extinguishing characteristic, which greatly saves the power consumption of the circuit. This characteristic also makes FIA particularly suitable for the half-cycle DSM structure.
This paper is organized as follows: Section 2 introduces the basic principles of conventional passive summation. The proposed passive and attenuationless summation scheme is analyzed in detail in Section 3. Section 4 presents a passive summation case study based on a second-order 4-bit DSM. The simulation results are shown in Section 5 and the conclusion is drawn in Section 6.

2. Conventional passive Summation

Conventional discrete-time DSM passive summation circuits are generally based on the principle of charge redistribution. To simplify, take the example of using passive summation to add two voltages Vip1 and Vip2.The working principle is shown in Figure 1.
During Φ2, Vip1 and Vip2 respectively charge capacitors Cf1 and Cf2. At this time, the voltage signals carried by capacitors Cf1 and Cf2 are Vip1 and Vip2, respectively. During Φ1, these two voltage signals are transmitted to VDACp for summation. Due to the parallel connection of Cf1 and Cf2, the voltage at the final summation node VDACp will be attenuated to:
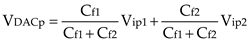
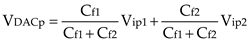
Preprints 92179 i004
Based on the above analysis, passive summation will cause the final summation voltage to attenuate to a coefficient less than 1, which is undesirable. The attenuation of the summation coefficient will increase the design requirements of the multi-bit quantizer.

3. Proposed passive and attenuationless summation scheme

In conventional passive summation, for example, if Cf1 = Cf2, only 0.5 Vip1 and 0.5 Vip2 are transmitted to the signal summation node VDACp, causing attenuation. To compensate for this attenuation, a simple and effective method is to increase the multiplication factor of the voltage signal carried by the capacitor. As we know, high-precision delta-sigma ADCs are often implemented in differential form, which allows the use of Vin1 (Vin2) signals that are opposite in phase to Vip1 (Vip2) for bidirectional sampling, thus achieving doubling for the summation coefficient.
Figure 2 shows the improved bidirectional sampling passive summation scheme proposed in this paper. The timing is the same as Figure 1(b), and the only difference from Figure 1 is that the bottom plates of Cf1 and Cf2 are connected to Vin1 and Vin2, respectively. Through bidirectional sampling of Vip1 and Vin1, during Φ2, the voltage carried by Cf1 is Vip1-Vin1. Since Vip1 and Vin1 are a pair of differential voltages, there is a relationship Vip1-Vin1 = 2Vip1, which is equivalent to the voltage signal carried by Cf1 being 2Vip1. The same applies to Cf2. Therefore, the voltage signals carried by Cf1 and Cf2 are doubled through bidirectional sampling technology. During Φ1, these two voltage signals are transmitted to VDACp for summation, and the voltage at the final summation node VDACp is:
VDACp = 2 Cf 1 Cf 1 + Cf 2 Vip 1 + 2 Cf 2 Cf 1 + Cf 2 Vip 2
If Cf1=Cf2, then exactly 1 times the voltage signals of Vip1 and Vip2 are transmitted at the summation node. Thus, the voltage signals carried by Cf1 and Cf2 were successfully multiplied by a factor of 2 through bidirectional sampling, compensating for the attenuation caused by direct parallel connection of the summation capacitors. If a larger multiplication factor is required, a more aggressive expansion scheme can be used, such as increasing the multiplication factor by serially connecting capacitors.
Figure 3 shows the bidirectional sampling and capacitive series passive summation scheme proposed in this paper, which can achieve higher coefficient multiplication. The timing is the same as Figure 1(b). Similar to Figure 2, during Φ2, the sampling is completed, and the voltage signals carried by Cf1 and Cf2 are equivalent to 2Vip1 and 2Vip2, respectively. During Φ1, since two Cf1 capacitors are connected in series, the carried voltages are superimposed, and the series circuit composed of two Cf1 capacitors carries an equivalent voltage signal of 4Vip1. The same applies to Cf2, so that the voltage signals carried by the summation capacitor are quadrupled through bidirectional sampling and capacitive series connection. These quadrupled voltage signals are transmitted to the summation node VDACp for summation, and the voltage at the final summation node VDACp is:
VDACp = 4 Cf 1 Cf 1 + Cf 2 Vip 1 + 4 Cf 2 Cf 1 + Cf 2 Vip 2
Similarly, if Cf1=Cf2, then at the signal summation node VDACp, twice the voltage signals of Vip1 and Vip2 are transmitted, providing the possibility to compensate for higher attenuation and increasing the applicability and flexibility of the passive and attenuationless summation scheme.
It is worth noting that this method can only series connect a maximum of two capacitors, otherwise the parasitic capacitance of the switch will greatly affect the final summation result, and the maximum coefficient multiplication is limited to 4 and can only be an integer multiple. In conventional passive summation, the attenuation increases as the number of summation paths increases, making this scheme less attractive. However, fortunately, in DSMs, the number of summation paths does not exceed 4, and the sum of the summation coefficients (attenuation) is also smaller than this value. Using dynamic-range scaling technology to design summation coefficients as digital numbers such as 0.25、0.5、1 and 2 can help the summation capacitor arrays achieve better matching. In addition, we can parallel additional capacitors to ground at the summation node to control the attenuation value, making it easy to round off the attenuation to an integer.
For DSMs with a summation coefficient sum ≤ 2, it can use bidirectional summation alone to compensate for the attenuation. With a coefficient sum ≤ 4, it need to use the bidirectional sampling and capacitive series connection scheme with greater coefficient multiplication. With a coefficient sum > 4, we can first try to reduce the coefficient sum by loop coefficient scaling or reducing the summation path. If we cannot reduce the summation coefficients, although we cannot compensate for attenuation perfectly, we can try to suppress this attenuation as much as possible.

4. Design example based on passive and attenuationless summation scheme

4.1. System architecture topology

To verify the reliability of the passive and attenuationless summation scheme, we designed a 2-order DSM based on energy-efficient 4-bit 1-order passive NS-SAR quantizer. It can implement a 3-order noise-shaping loop filter. This case is significant because the third-stage is implemented in a passive manner, reducing the number of summation paths and making the proposed scheme even more attractive.
Figure 4 shows the system block diagram of a low-power multi-bit DSM based on passive and attenuationless summation. In this DSM, an NS SAR ADC is integrated to serve as the quantizer of the modulator. The NS SAR achieves shaping effects by sampling and integrating the residual voltage of the SAR, thereby replacing the active OTA and reducing the quantization noise, leading to improved energy-efficiency of the modulator. The NS SAR is designed as a 1-order loop based on 4-bit 1-order passive NS SAR quantizer. The signal and noise transfer functions can be described as follows:
STFNS = 1 + ( 1 - d ) Z - 1 1 - ( 1 - d ) Z - 1 1 + ( 1 - d ) Z - 1 1 - ( 1 - d ) Z - 1 = 1
NTFNS = 1 1 + ( 1 - d ) Z - 1 1 - ( 1 - d ) Z - 1 = 1 - ( 1 - d ) Z - 1
As can be seen, the signal transfer function of the NS SAR system is strictly equal to 1, and it achieves 1-order shaping effects on the quantization noise introduced by the SAR. The system cascades the NS SAR with a 2-order CIFF modulator structure to implement the third integrator passively. The signal transfer diagram of the entire DSM after cascading can be equivalently represented as Figure 5.
As a result, the overall signal and noise transfer function of the system becomes:
STF = STFNS ( Z - 1 + c 1 a 2 Z - 1 1 - Z - 1 + c 1 c 2 a 1 Z - 1 ( 1 - Z - 1 ) 2 ) 1 + STFNS ( c 1 a 2 Z - 1 1 - Z - 1 + c 1 c 2 a 1 Z - 1 ( 1 - Z - 1 ) 2 ) = Z - 1 + c 1 a 2 Z - 1 1 - Z - 1 + c 1 c 2 a 1 Z - 1 ( 1 - Z - 1 ) 2 1 + c 1 a 2 Z - 1 1 - Z - 1 + c 1 c 2 a 1 Z - 1 ( 1 - Z - 1 ) 2
NTF = NTFNS 1 + STFNS ( c 1 a 2 Z - 1 1 - Z - 1 + c 1 c 2 a 1 Z - 1 ( 1 - Z - 1 ) 2 ) = 1 - ( 1 − d ) Z - 1   ( 1 - Z - 1 ) 2 1 + ( c 1 a 2 + c 1 c 2 a 1 − 2 ) Z - 1 + ( 1 − c 1 a 2 ) Z - 2
Due to the low input signal frequency of the DSM, Z-1 is approximately equal to 1 for the input signal. Therefore, the signal transfer function of the system is 1. From equation (7), in this system, by cascading the NS SAR with a 2-order CIFF modulator structure, we obtain a 3-order shaping modulator system. The coefficients of the system are set as follows: d = 0.15, c1 = 2, c2 = 2, a1 = 0.25, a2 = 0.25.
Additionally, since there are always three summing paths in this system and the sum of the summing coefficients is 1.5 < 2, we can use bidirectional summation technique to compensate for the attenuation.

4.2. Circuit implementation

4.2.1. Cascode FIA in the integrator

As is well known, the OTA in the integrator is a core structure of the DSM and also the main source of power consumption in DSM. As shown in Figure 6, the FIA is used in the two-stage integrator of the DSM designed in this project, utilizing its natural self-extinguishing effect to further improve the energy efficiency of the DSM. During Φ1, in the sampling cycle, the integrator does not work, and the power supply charges the floating power supply FIA. During Φ2, in the integration cycle, the FIA works. Unlike conventional fixed power supply, the FIA uses a fully floating capacitor Cres to provide the power voltage and current required by this inverter. The voltage and current provided by Cres gradually decrease over time. When the voltage difference of Cres is less than twice the threshold voltage of the transistor, the FIA stops working. This self-extinguishing effect is naturally suitable for half-cycle DSM structures and can further improve the energy efficiency of the entire DSM. The equivalent gm provided by FIA to the system during the integration cycle is estimated as [17]:
gm _ avg = 8 Cres T s Δ VD VT
Where TS is the period of Φ1 and Φ2, ∆VD is the voltage drop during the charging and discharging of the floating capacitor Cres, and VT is the thermal voltage. It is worth noting that ∆VD itself is also proportional to VT, so this FIA structure has strong PVT tolerance. Furthermore, since the power consumption of FIA depends only on ∆VD and the clock frequency, this means that using FIA as the core operational amplifier of DSM allows for the flexible adjustment of the clock frequency to control performance and power consumption.
Since the capacitor is fully floating, the FIA is also insensitive to common-mode inputs and automatically modulates the power supply voltage based on real-time common-mode inputs. The FIA does not require dedicated common-mode feedback and only needs to use a small capacitor Cx to sample the voltage of Vcm during Φ1 for simple common-mode feedback.
In order to overcome the disadvantage of low gain in simple FIA, we also applied a cascode FIA structure based on the cascade transistor, which increases the gain of the OTA to the square of the transistor’s intrinsic gain. The drawback is that this will result in a decrease in output swing. However, for DSMs that can adjust the output swing using scaling techniques, this is not a disadvantage.

4.2.2. Noise-Shaping SAR ADC used as a quantizer

As shown in Figure 7, the 1-order passive NS SAR quantizer enhances the noise-shaping capability of the loop filter while improving the system’s energy efficiency. The specific principle is that during Φ2, Vip and Vin charge the capacitor Csum, and the charge of CDAC is cleared. Here, the capacitance value of Csum is set to be equal to the total summing capacitance in the DSM and is set to be equal to CDAC. During Φs1, Cns is cleared. During Φ_SAR, NS SAR works, sharing the charge on Csum with CDAC and inputting it into the SAR quantization comparator with the integration voltage Vint = Vintp-Vintn from the previous period in a ratio of 1:1/d. The Φc signal is generated asynchronously, automatically generating the next Φc when NS SAR starts working or when the previous comparison bit is compared. After CDAC is also switched by SAR Logic, the value of Vp-Vn is the residual voltage Vres generated by the comparison. Vres represents the error generated by SAR quantization. NS SAR relies on sampling and integrating Vres to complete noise-shaping. It is worth noting that although the signal is attenuated by half due to the shared charge of Csum and CDAC, the final comparison result is not affected because CDAC is half of the sum of CDAC + Csum. During Φns, the residual charge on CDAC and Csum continues to be shared with Cns. Since Cns is set to be dCDAC/(1-d), the voltage on Cns is (1-d)Vres. During Φ2, the charge on Cns is shared with the charge on Cint in the previous period, and the integrated voltage Vint on Cint is a(1-d)Vres+(1-d)Vint·Z-1. Its equivalent model is shown in Figure 8.

4.2.3. Overall circuit of the DSM

Figure 9 shows the circuit and timing diagram of the low-power multi-bit DSM based on passive and attenuationless summation. The circuit consists of two integrators, passive summation circuits, a 1-order loop shaping based on 4-bit NS SAR quantizer, DWA module, and feedback DAC arrays. In order to meet the performance requirements of DSM to achieve 100dB at a power supply voltage of 1.2V and OSR=62.5, according to the thermal noise limit=4kT/(OSR·CS), CS1=14p, CS2=2p are set. It should be noted that the summation circuit completes the passive and attenuationless summation through bidirectional sampling. The signals to be summed by the summation circuit are Vip-Vin, Vo1p-Vo1n, and Vo2p-Vo2n, and the required summation coefficients are 1 : 0.25 : 0.25. During Φ2d, the input signal and OTA1 charge the positive terminals Cf1 and Cf2 through bidirectional sampling technology, causing the voltage carried by Cf1 and Cf2 to be equivalent to 2 times Vip and Vo1p, achieving a coefficient doubling. Additionally, the voltage of Cf3 is cleared. During Φ1d, the voltage of the positive terminals Cf1 and Cf2 is pushed to the summing node VDACp for summation. At the same time, OTA2 charges Cf3. The same applies to the negative terminal VDACn. The final summing node VDACp-VDACn is:
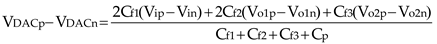
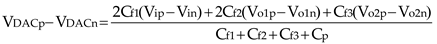
Preprints 92179 i002
Where Cp is the estimated parasitic capacitance. If Cf1 : Cf2 : Cf3 : Cp = 4 : 1 : 2 : 1, then the final VDACp-VDACn is:
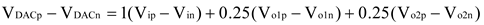
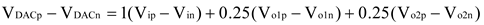
Preprints 92179 i003
Therefore, by using bidirectional sampling technology to achieve coefficient doubling for the voltage signals carried by Cf1 and Cf2, a passive and attenuationless summation method is achieved.
It should be noted that during summation, the left plate of the Cf2 capacitor is connected to the output of the second-stage op-amp. The CDAC capacitor switching inside NS SAR will increase the driving pressure on the op-amp, which is catastrophic for FIA. Therefore, we can use a differential positive and negative feedback switching of the CDAC to replicate a capacitor array with the same environment for VFp and VFn nodes, but with opposite CDAC switching feedback, to compensate for the two nodes, thereby eliminating the driving pressure on OTA2. This feedback mechanism only generates very small power during comparison and can be ignored relative to the entire system.

5. The simulation results

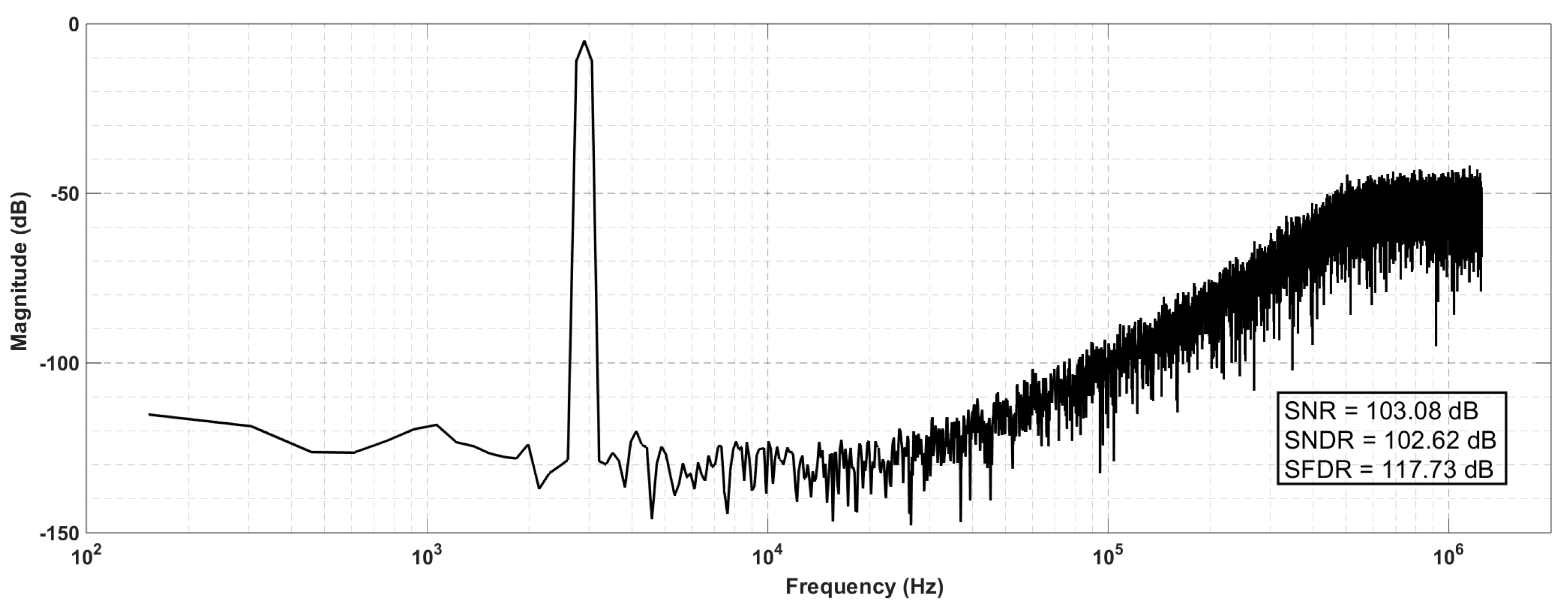
This DSM based on passive and attenuationless summation was designed using the 180nm CMOS process. The DSM runs at 2.5 MHz with a 1.2 V supply. Under an input signal of 2.9 kHz, the 4-bit code stream output by the modulator was analyzed as shown in Figure 10. In a bandwidth of 20 kHz, the simulated SNR, SNDR and SFDR are 103.08 dB, 102.62 dB and 117.73 dB. The total power consumption is 148.32 μW, with analog power consumption at 78 μW and digital power consumption at 70.32 μW.
Table 1. Performance comparison.
Table 1. Performance comparison.
Parameter This work JSSC[31] JSSC[32] TCAS-II[33]
Technology 180nm 65nm 65nm 65nm
Year 2020 2019 2021
Supply(V) 1.2 0.8 1 1
BW (kHz) 20 24 25 19.5
SNDR (dB) 102.62 89.6 94.6 88.5
Power (μW) 148.32 60 175 43.5
FoMs (dB) 183.92 175.6 176.2 175
Preprints 92179 i001

6. The simulation results

This paper proposes a low-power multi-bit DSM based on passive and attenuationless summation. By using bidirectional sampling and capacitor series connection, the voltage signals carried by the summation circuit are multiplied, successfully compensating for the attenuation caused by the parallel connection of the summation capacitors and greatly relaxing the design requirements of the subsequent multi-bit quantizer. Moreover, this scheme does not introduce additional active overhead. By combining the use of FIA and NS SAR, the validation case of a 3rd-order DSM with a 4-bit quantizer in the 180nm CMOS process demonstrates excellent performance in simulation results. The design case used provides a new approach to reduce passive summation paths, making the proposed passive and attenuationless summation scheme highly advantageous in multi-bit DSMs design.

Author Contributions

Rongshan Wei: Conceptualization, Methodology, Software. Lijie Huang: Data curation, Writing- Original draft preparation. Gongxing Huang: Conceptualization, Methodology. Renping Wang: Visualization, Investigation. Cong Wei: Methodology, Reviewing.

Funding

This work was supported by the Natural Science Foundation of Fujian Province, China (Grant No. 2023J01398).

Conflicts of Interest

The authors declare no conflict of interest.

References

  1. Y. Chae.: G. Han. Low Voltage, Low Power, Inverter-Based Switched-Capacitor Delta-Sigma Modulator. IEEE Journal of Solid-State Circuits. 2009, 44, 458–472. [CrossRef]
  2. Je-Kwang Cho. Low-power sigma-delta modulator with half-sample delayed-input feedforward. IEICE Electronics Express. 2014, 11, 1349–2543. [CrossRef]
  3. D. Laouej.; H. Daoud.; M. Loulou. A Very Low Power Delta Sigma Modulator Using Optimized Bulk Driven Telescopic OTA for Biomedical Devices. In 2020 IEEE International Conference on Design & Test of Integrated Micro & Nano-Systems (DTS), Hammamet, Tunisia, 2020, pp. 1-6. [CrossRef]
  4. J. Kim.; H. Shin.; S. Na. A 860.8-nW Low-Power Continuous-Time Delta-Sigma Modulator With Switched Resistors for Sensor Applications. In 2023 IEEE International Symposium on Circuits and Systems (ISCAS), Monterey, CA, USA, 2023, pp. 1-5. [CrossRef]
  5. B. Zhao.; Y. Lian.; A. M. Niknejad. A Low-Power Compact IEEE 802.15.6 Compatible Human Body Communication Transceiver With Digital Sigma-Delta IIR Mask Shaping. IEEE Journal of Solid-State Circuits. 2019, 54, 346–357. [CrossRef]
  6. T. -C. Wang.; Y. -H. Lin. A 0.022 mm2 98.5 dB SNDR Hybrid Audio ΔΣ Modulator With Digital ELD Compensation in 28 nm CMOS. IEEE Journal of Solid-State Circuits. 2015, 50, 2655–2664. [CrossRef]
  7. M. Jang.; C. Lee. Analysis and Design of Low-Power Continuous-Time Delta-Sigma Modulator Using Negative-R Assisted Integrator. IEEE Journal of Solid-State Circuits. 2019, 54, 277–287. [CrossRef]
  8. J. Silva.; U. Moon. Wideband low-distortion delta-sigma ADC topology. Electron. Lett.. 2001, 37, 737–738. [CrossRef]
  9. M. A. Mokhtar.; P. Vogelmann.; J. Wagner. Incremental Sturdy-MASH Sigma-Delta Modulator with Reduced Sensitivity to DAC Mismatch. In 2019 IEEE International Symposium on Circuits and Systems (ISCAS), Sapporo, Japan, 2019, pp. 1-5. [CrossRef]
  10. P. P. Bora.; D. Borggreve.; F. Vanselow. A 0.8 V Low-Power 3rd order Sigma-Delta Modulator in 22 nm FDSOI CMOS Process for Sensor Interfaces. In 2019 17th IEEE International New Circuits and Systems Conference (NEWCAS), Munich, Germany, 2019, pp. 1-4. [CrossRef]
  11. H. Park.; K. Nam. A 0.7-V 870-μ W Digital-Audio CMOS Sigma-Delta Modulator. IEEE Journal of Solid-State Circuits. 2009, 44, 1078–1088. [CrossRef]
  12. Y.Tang.; S.Gupta.; J. Paramesh. A digital-summingfeedforward Σ−∆ modulator and its application to a cascade ADC. In TheProceedings of the IEEEInternational Symposium of Circuits and Systems(ISCAS ), New Orleans, USA, 07, pp. 485-488. 20 May. [CrossRef]
  13. Y. Ye.; L. Liu.; J. Li. A 120dB SNDR audio sigma-delta modulator with an asynchronous SAR quantizer. In 2012 IEEE International Symposium on Circuits and Systems (ISCAS), Seoul, Korea (South), 2012, pp. 2357-2360. [CrossRef]
  14. S. Mehrotra.; E.Eland.; S. Karmakar. A 590 µW, 106.6 dB SNDR, 24 kHz BW Continuous-Time Zoom ADC with a Noise-Shaping 4-bit SAR ADC. In ESSCIRC 2022- IEEE 48th European Solid State Circuits Conference (ESSCIRC), Milan, Italy, 2022, pp. 253-256. [CrossRef]
  15. E. Eland.; S. Karmakar. A 440-μW, 109.8-dB DR, 106.5-dB SNDR Discrete-Time Zoom ADC With a 20-kHz BW. IEEE Journal of Solid-State Circuits. 2021, 56, 1207–1215. [CrossRef]
  16. Y. Tang.; X. Chen.; H Zhu. A 108-dB SNDR 2–1 MASH ΔΣ Modulator with First-Stage Multibit for Audio Application. In 2018 IEEE 3rd International Conference on Integrated Circuits and Microsystems (ICICM), Shanghai, China, 2018, pp. 336-340. [CrossRef]
  17. R. S. A. Kumar.; N. Krishnapura. Analysis and Design of a Discrete-Time Delta-Sigma Modulator Using a Cascoded Floating-Inverter-Based Dynamic Amplifier. IEEE Journal of Solid-State Circuits, 2022, 57, 3384–3395. [CrossRef]
  18. M. Zhao.; Y. Zhao. A 4-μW Bandwidth/Power Scalable Delta–Sigma Modulator Based on Swing-Enhanced Floating Inverter Amplifiers. IEEE Journal of Solid-State Circuits. 2022, 57, 709–718. [CrossRef]
  19. J. -S. Huang.; S. -C. Kuo. A Multistep Multistage Fifth-Order Incremental Delta Sigma Analog-to-Digital Converter for Sensor Interfaces. IEEE Journal of Solid-State Circuits. 2023, 58, 2733–2744. [CrossRef]
  20. Jinho Noh. ; Jisoo Lee. An analog sigma-delta modulator with shared operational amplifier for low-power class-D audio amplifier. IEICE Electronics Express. 2015, 12, 1349–2543. [CrossRef]
  21. H. Zhuang.; W. Guo. A Second-Order Noise-Shaping SAR ADC With Passive Integrator and Tri-Level Voting. IEEE Journal of Solid-State Circuits. 2019, 54, 1636–1647. [CrossRef]
  22. S. Li.; B. Qiao. A 13-ENOB Second-Order Noise-Shaping SAR ADC Realizing Optimized NTF Zeros Using the Error-Feedback Structure. IEEE Journal of Solid-State Circuits. 2018, 53, 3484–3496. [CrossRef]
  23. H. Hu.; V. Vesely.; U. -K. Moon. Ultra-Low OSR Calibration Free MASH Noise Shaping SAR ADC. In 2022 IEEE International Symposium on Circuits and Systems (ISCAS), Austin, TX, USA, 2022, pp. 1244-1248. [CrossRef]
  24. Aprile.; M. Folz.; D. Gardino. A 0.06-mm2 Current-Mode Noise-Shaping SAR based Temperature-to-Digital Converter with a 4.9-nJ Energy/Conversion. In 2023 IEEE Custom Integrated Circuits Conference (CICC), San Antonio, TX, USA, 2023, pp. 1-2. [CrossRef]
  25. K. Li.; S. -W. Sin.; L. Qi. A Robust Hybrid CT/DT 0-2 MASH DSM with Passive Noise-Shaping SAR ADC. In 2022 IEEE International Symposium on Circuits and Systems (ISCAS), Austin, TX, USA, 2022, pp. 551-555. [CrossRef]
  26. X. Tang.; L. Shen. An Energy-Efficient Comparator With Dynamic Floating Inverter Amplifier. IEEE Journal of Solid-State Circuit. 2020, 55, 1011–1022. [CrossRef]
  27. Y. Hu.; Y. Zhao.; W. Qu. A 2.87μW 1kHz-BW 94.0dB-SNDR 2-0 MASH ADC Using FIA with Dynamic-Body-Biasing Assisted CLS Technique. In 2022 IEEE International Solid- State Circuits Conference (ISSCC), San Francisco, CA, USA, 2022, pp. 410-412. [CrossRef]
  28. X. Hao.; J. Chen.; L. Meng. A 94.6dB-SNDR 50kHz-BW 1-1-1 MASH ADC Using OTA-FIA Based Integrators. In 2023 IEEE International Symposium on Circuits and Systems (ISCAS), Monterey, CA, USA, 2023, pp. 1-4. [CrossRef]
  29. X. Wu.; Y. Liu.; H. Lu. A 77.2-dB SNDR SAR ADC With a Segmented Comparator Logic Based on Compensatory SA Latch for FIA. In 2021 IEEE MTT-S International Wireless Symposium (IWS), Nanjing, China, 2021, pp. 1-3. [CrossRef]
  30. H. Li.; Z. Tan. Energy-Efficient CMOS Humidity Sensors Using Adaptive Range-Shift Zoom CDC and Power-Aware Floating Inverter Amplifier Array. IEEE Journal of Solid-State Circuits. 2021, 56, 3560–3572. [CrossRef]
  31. S. Ma.; L. Liu.; T. Fang. A Discrete-Time Audio ΔΣ Modulator Using Dynamic Amplifier With Speed Enhancement and Flicker Noise Reduction Techniques. IEEE Journal of Solid-State Circuits. 2020, 55, 333–343. [CrossRef]
  32. S. -H. Liao.; J. -T. Wu. A 1-V 175-μW 94.6-dB SNDR 25-kHz Bandwidth Delta-Sigma Modulator Using Segmented Integration Techniques. IEEE Journal of Solid-State Circuits. 2019, 54, 2523–2531. [CrossRef]
  33. Matsuoka. ; T. Nezuka.; T. Iizuka. Fully Dynamic Discrete-Time ΔΣ ADC Using Closed-Loop Two-Stage Cascoded Floating Inverter Amplifiers. IEEE Transactions on Circuits and Systems II: Express Briefs. 2022, 69, 944–948. [CrossRef]
Preprints 92179 g001
Figure 1. Conventional passive summation circuit diagram and timing: (a) circuit; (b) timing.
Figure 1. Conventional passive summation circuit diagram and timing: (a) circuit; (b) timing.
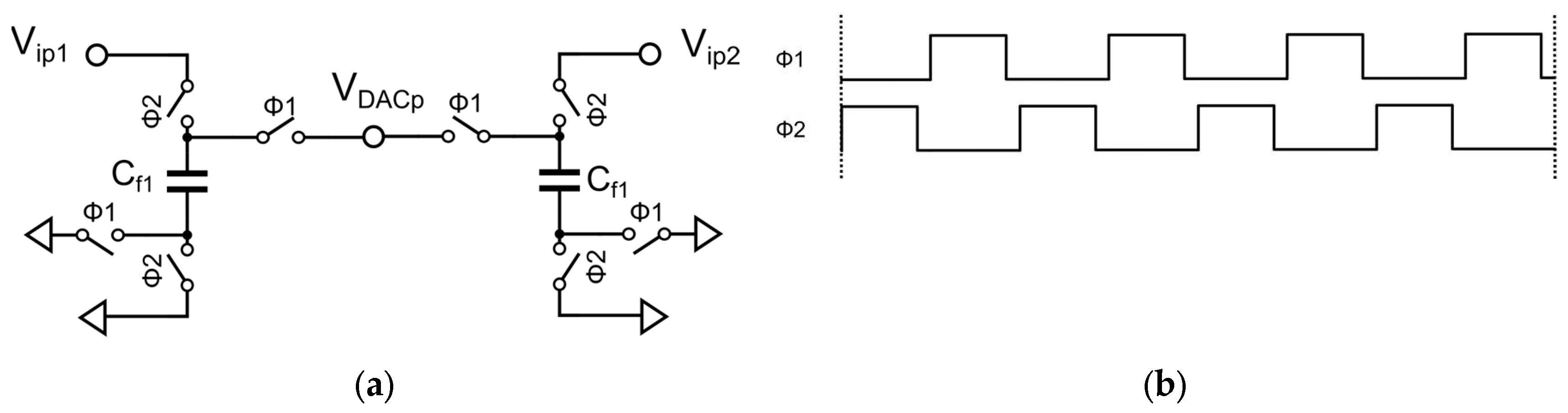
Preprints 92179 g001
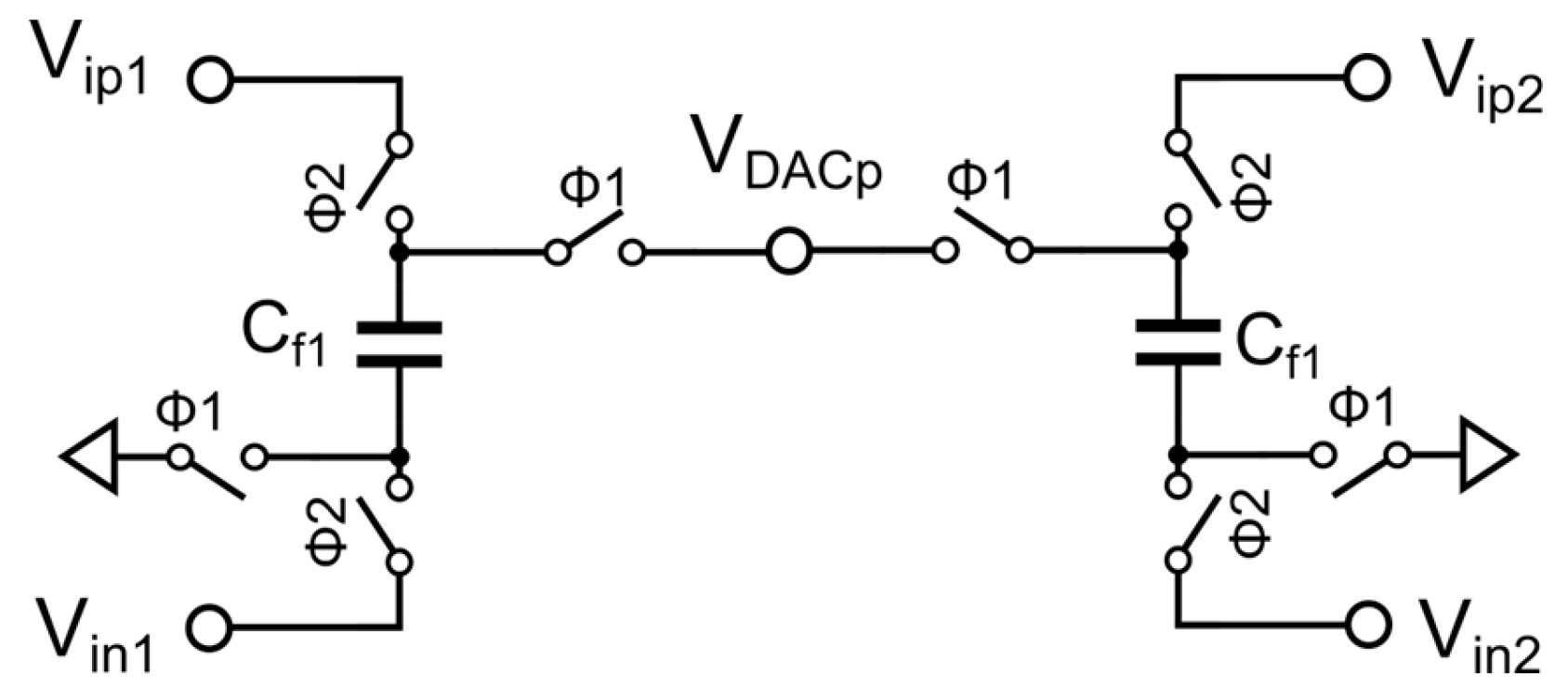
Preprints 92179 g002
Figure 2. Bidirectional sampling passive summation circuit diagram.
Figure 2. Bidirectional sampling passive summation circuit diagram.
Preprints 92179 g002
Preprints 92179 g003
Figure 3. Bidirectional sampling and capacitor series connection passive summation circuit diagram.
Figure 3. Bidirectional sampling and capacitor series connection passive summation circuit diagram.
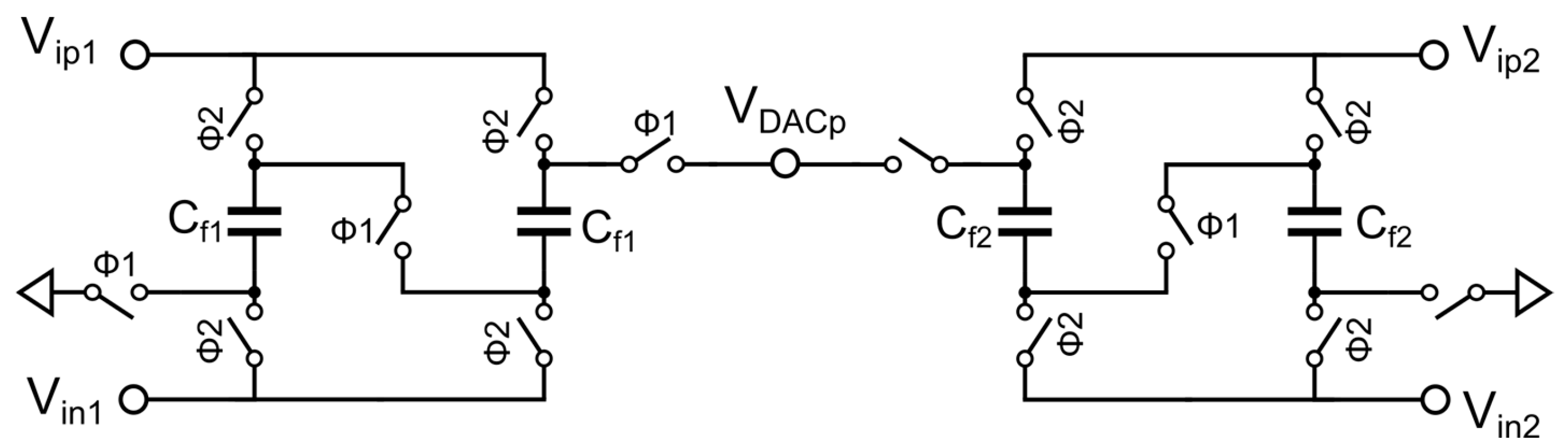
Preprints 92179 g003
Preprints 92179 g004
Figure 4. System block diagram.
Figure 4. System block diagram.
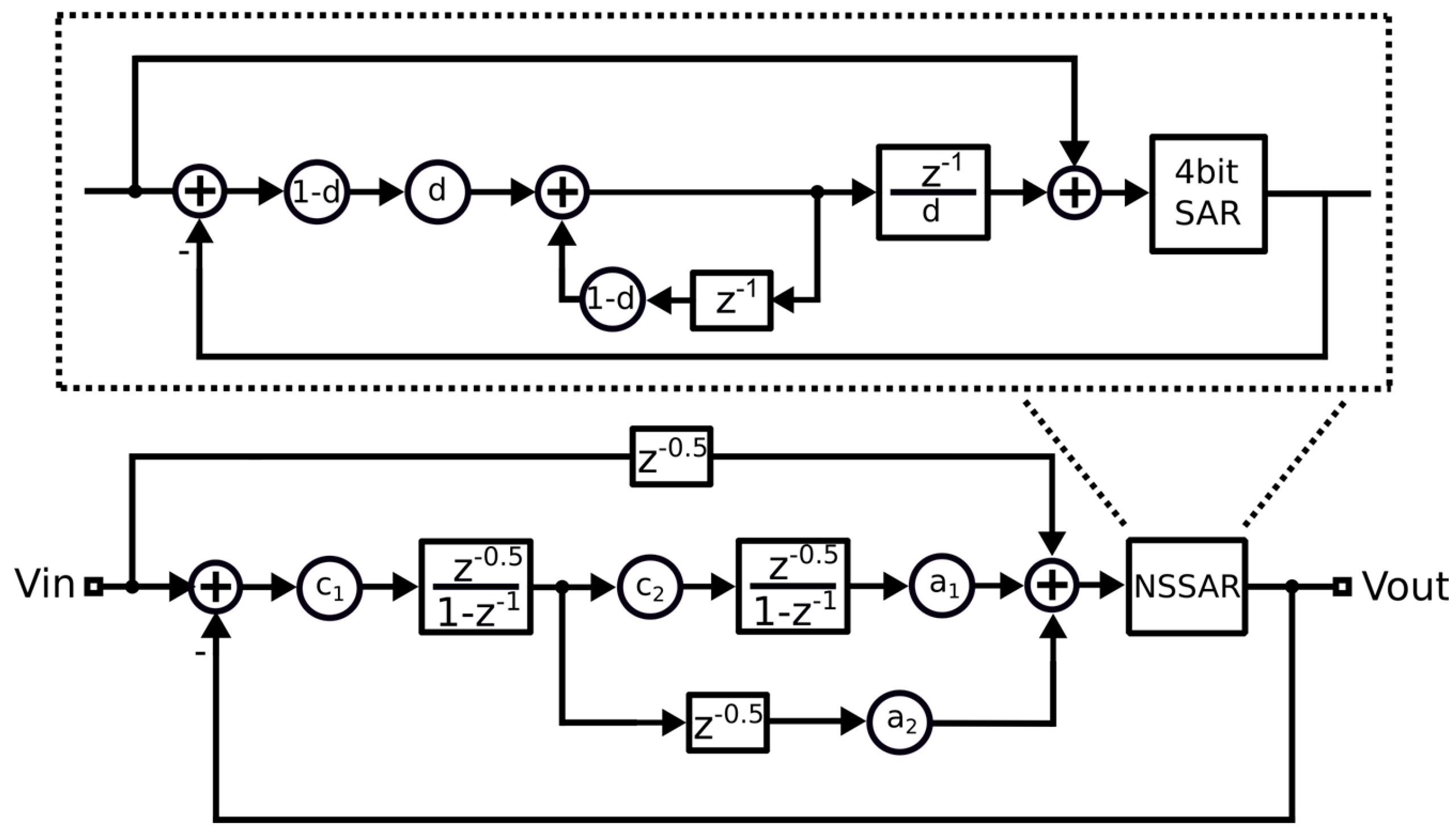
Preprints 92179 g004
Preprints 92179 g005
Figure 5. Equivalent system diagram.
Figure 5. Equivalent system diagram.
Preprints 92179 g005
Preprints 92179 g006
Figure 6. Cascode FIA.
Figure 6. Cascode FIA.
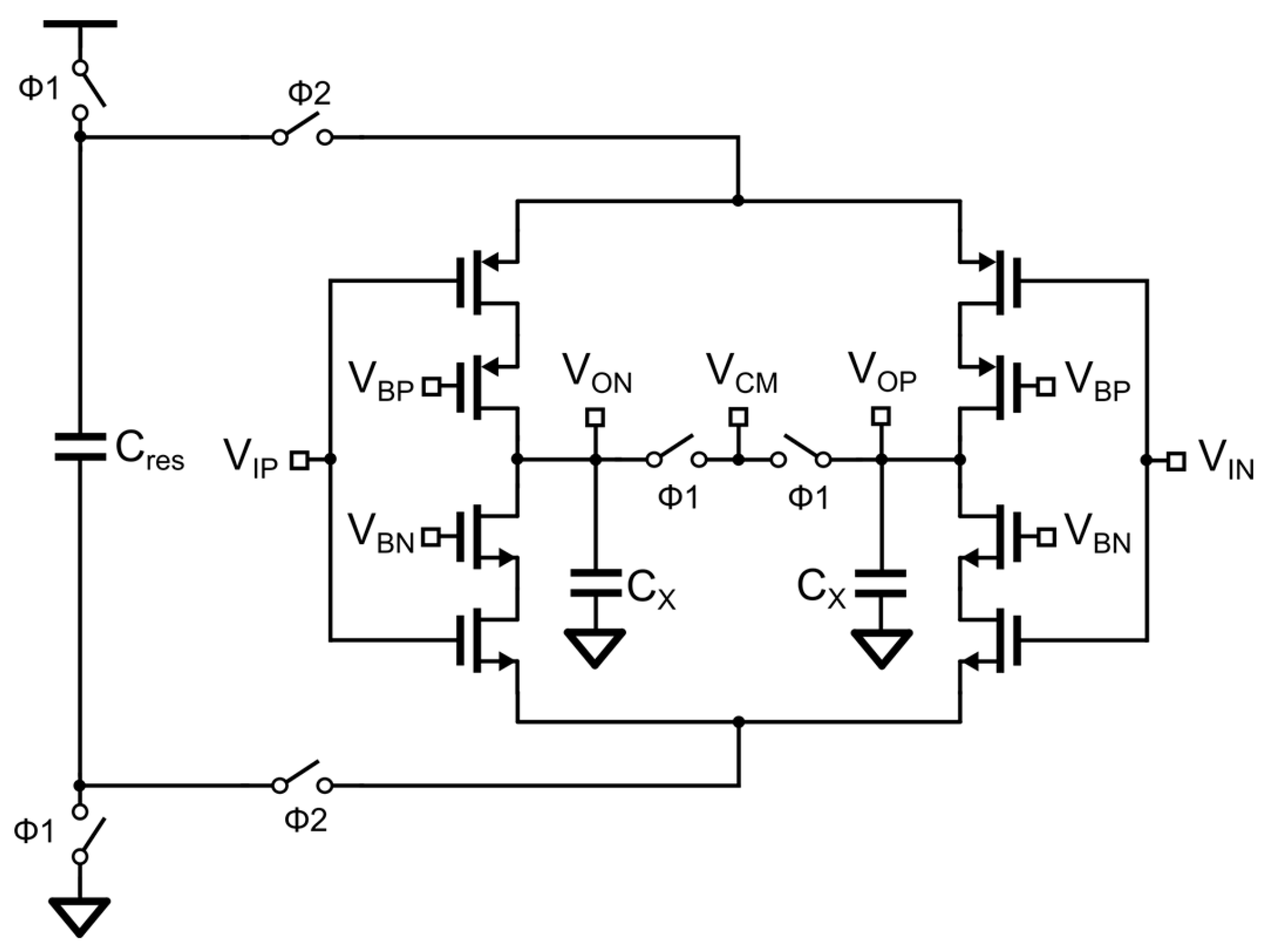
Preprints 92179 g006
Preprints 92179 g007
Figure 7. Circuit structure and timing of Noise-Shaping SAR ADC: (a) circuit; (b) timing.
Figure 7. Circuit structure and timing of Noise-Shaping SAR ADC: (a) circuit; (b) timing.
Preprints 92179 g007
Preprints 92179 g008
Figure 8. Equivalent model of NSSAR.
Figure 8. Equivalent model of NSSAR.
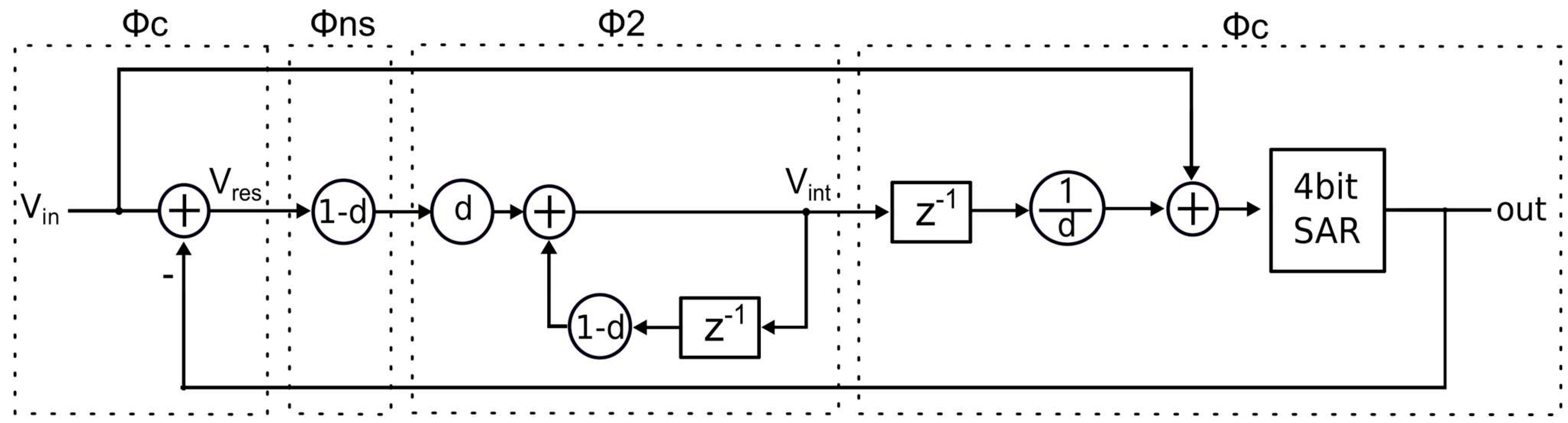
Preprints 92179 g008
Preprints 92179 g009
Figure 9. DSM circuit and timing:(a) circuit; (b) timing.
Figure 9. DSM circuit and timing:(a) circuit; (b) timing.
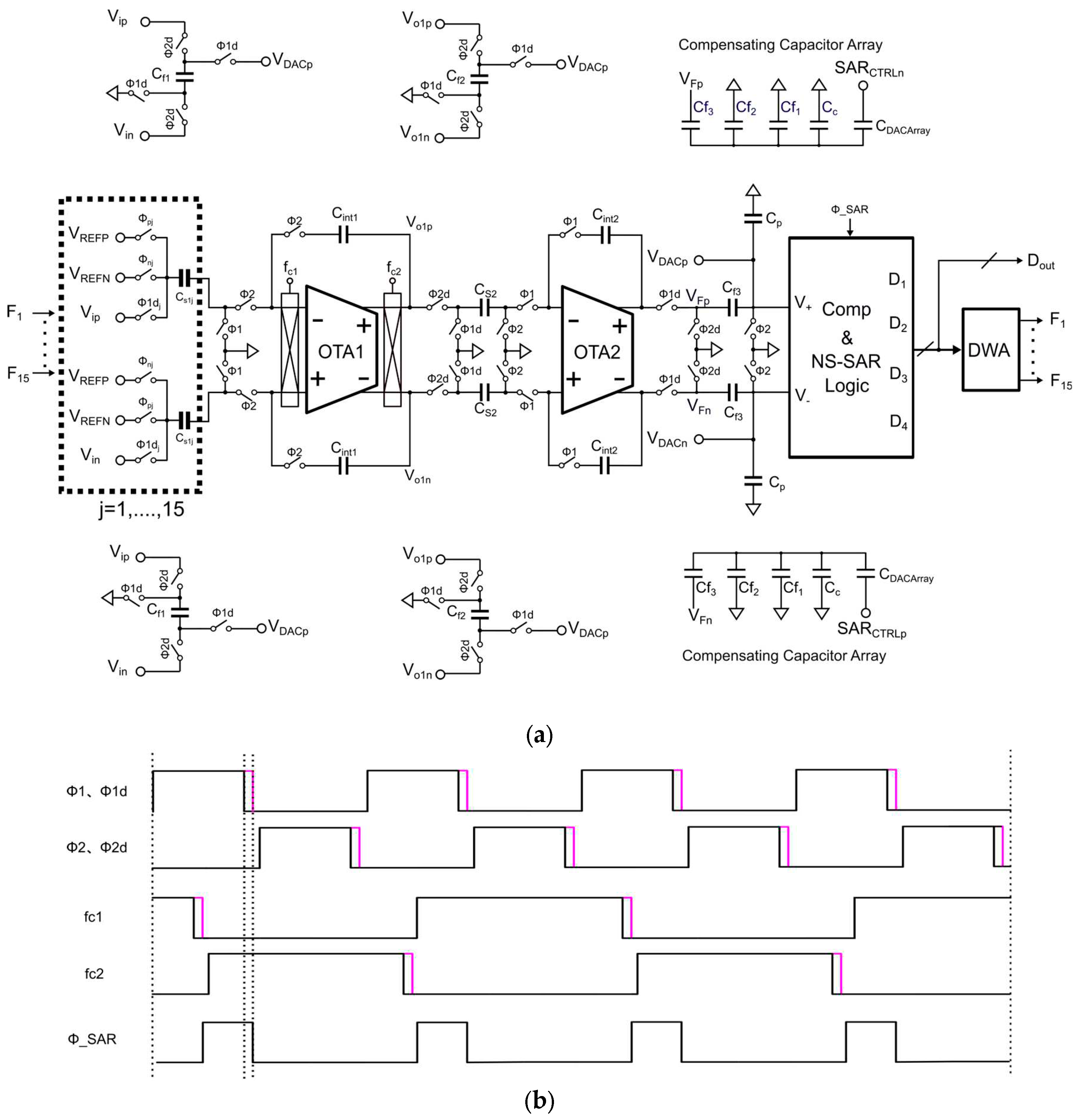
Preprints 92179 g009
Preprints 92179 g010
Figure 10. Simulation Output spectrum.
Figure 10. Simulation Output spectrum.
Preprints 92179 g010
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content.
Copyright: This open access article is published under a Creative Commons CC BY 4.0 license, which permit the free download, distribution, and reuse, provided that the author and preprint are cited in any reuse.
Prerpints.org logo

Preprints.org is a free preprint server supported by MDPI in Basel, Switzerland.

facebook logotwitter logolinkedin logo
MDPI Initiatives
Important Links
Subscribe

Disclaimer

Terms of Use

Privacy Policy

© 2024 MDPI (Basel, Switzerland) unless otherwise stated