Prerpints.org logo
Preprint
Article

Identification of Aggregation Processes in Hexamethylenetetramine Aqueous Solutions: A Comprehensive Raman and Acoustic Spectroscopic Study Combined with Molecular Orbital Calculations

Altmetrics

Downloads

72

Views

17

Comments

0

A peer-reviewed article of this preprint also exists.

Submitted:

01 November 2023

Posted:

02 November 2023

You are already at the latest version

Alerts
Abstract
Raman scattering has been employed to study in detail the concentration dependence of the vibrational modes for the hexamethylenetetramine (HMTA) aqueous solutions. The formation of protonated and/or aggregated species has been clarified by comparing the experimental with the theoretically predicted vibrational spectra by means of quantum mechanical calculations. The analysis has shown that the vibrational modes of the solutions arise from a contribution of the vibrational modes of the HMTA self-aggregates and hetero-aggregates of HMTA with water molecules that are formed in the low- and intermediate-concentration region, respectively. The protonation of HMTA is ruled out due to the large differences between the experimental and the theoretically calculated spectra of the protonated molecules of HTMA in the fingerprint region. In the low-concentration solutions, the hetero-aggregation reaction of HMTA with water is the dominant mechanism, while at higher concentrations a self-aggregation mechanism occurs. Ultrasonic absorption and velocity measurements were carried out for hexamethylenetetramine aqueous solutions. The acoustic spectra reveal the presence of only one single Debye-type relaxation process that is assigned to the aggregation mechanism of HMTA. The sound absorption data follow two different dependencies on HMTA mole fraction. The crossover 0.018 mole fraction signifies two separate regions with distinct structural characteristics. The relaxation mechanism observed in dilute solutions is attributed to hetero-association of HMTA with water molecules, while at higher concentrations the observed relaxation process is assigned to the self-association reaction of HMTA molecules. This structural transformation is also reflected in several physicochemical properties of the system, including the kinematic viscosity, the mass density, the sound speed, and the adiabatic compressibility of the HMTA aqueous solutions. The combination of vibrational and acoustic spectroscopies with molecular orbital calculations allowed us to disentangle the underlying processes and to elucidate the observed relaxation mechanism in the HMTA aqueous solutions.
Keywords: 
Subject: Chemistry and Materials Science  -   Physical Chemistry

1. Introduction

Hexamethylenetetramine (HMTA) is a tertiary polyamine, whose structure resembles that of a cage. HMTA is also known as methenamine, hexamine or urotropine. Hexamethylenetetramine is used in medicine for the treatment of urinary tract infection (UTI) and it is effective as a daily low-dose antibiotics at preventing UTIs [1]. It is also used for heating camping food or military rations, since it burns without smoke and it has a high energy density, it does not liquify while burning, and leaves no ashes, although its fumes are toxic [2]. The wide range of applications also includes its use as a food additive/preservative corresponding to the International Numbering System for Food Additives (INS number) 239 [3]. HMTA is used as the base component to produce a variety of high explosive organic compound including RDX (abbreviation of Research Department eXplosive), C-4, octogen, HMTD (Hexamethylene Triperoxide Diamine) and others [4]. Finally, HMTA is a versatile reagent in organic synthesis [5], while its dominant use is in the production of powdery or liquid preparations of phenolic resins, where it is added as a hardening component [4].
Hexamethylenetetramine is a heterocyclic organic compound with a tetrahedral cage-like structure similar to adamantane. Four vertices are occupied by nitrogen atoms that are connected by methylene groups. Despite the molecular cage-like shape of HTMA, at the interior of the molecule no void space is available to incorporate other atoms or smaller molecules. The HMTA molecule behaves like an amine base, undergoing protonation and molecular self- and hetero-aggregation reactions with solvent molecules. Its rigid molecular structure does not permit any conformational change.
Deep understanding of the HMTA structural and dynamical properties is essential for predicting several macroscopic properties of this amine that may have important implications in the already wide range of applications. The combination of vibrational (Raman) spectroscopy with acoustic relaxational spectroscopy has been proved a powerful tool in investigating the association-dissociation [6,7], the proton-transfer [8,9] mechanisms and generally in reaction engineering [10]. Ultrasonic relaxation spectroscopy may provide dynamical and structural information about the studied system in the frequency domain covering a wide range from 10 kHz to ∼10 GHz that corresponds to relaxation times between 10−5 to 10−11 s, without any restriction on the type of process studied [11,12,13,14]. Additionally, Raman spectroscopy provides vibrational relaxation and structural information at molecular level in a time scale of 10−12 to 10−14 s. By combining Raman with ultrasonic relaxation spectroscopy, one may provide a complete and frank account for the physicochemical behavior of the system. On the other hand, molecular orbital calculations may be used to help us disentangle the underlying processes and to elucidate the observed relaxation mechanism in the acoustic spectra.
Our aim in this study is to provide insights on the structure and dynamics of aqueous solutions of HMTA by performing vibrational and acoustic concentration-dependent measurements. The possibility of formation of protonated and/or aggregated species has been clarified by comparing the experimental spectroscopic data with quantum mechanical calculations on isolated species in the gas phase free of any interactions.

2. Results and Discussion

2.1. Structural processes in HMTA aqueous solutions

The HMTA molecule acts as an amine base, and thus it may undergo protonation reactions with solvent molecules. The proton-transfer mechanism can be described as:
H M T A + z H 2 O   ⇄ H M T A + z + z O H −
The possible proton transfer reactions that may occur in HMTA aqueous solutions are shown in Figure 1. Each reaction corresponds to a subsequent protonation step.
The molecular structure of HMTA permits, not only a molecular hetero-aggregation, but also a self-aggregation reaction. In addition, other processes such as a proton-transfer reaction of HMTA with the solvent (water) may also occur. Processes related with conformational changes are unlikely due to the rigid molecular structure of HMTA.
The hetero-aggregation reaction of HMTA with water is likely to occur at low concentrations of amine, where the solute–solvent interactions are expected to be stronger, and can be described as through the following scheme:
H 2 O m + H M T A ⇄ H M T A ∙ m H 2 O
The (H2O)m and HMTA ∙ mH2O complexes denote the corresponding water clathrate and the mixed aggregate between water and HMTA molecules, respectively and both are constituted through hydrogen bonds [15,16,17,18]. Parameter m is the number of water molecules that are associated in the formation of the water clathrate and mixed aggregate. The possible hetero-aggregation reactions that may occur in HMTA aqueous solutions are presented schematically in Figure 2. Each reaction corresponds to a subsequent aggregation step.
At intermediate concentrations of HMTA aqueous solutions, a self-aggregation reaction of HMTA may occur that can be described as:
n H M T A   ⇄   H M T A n
with n designating the aggregation number and (HMTA)n the HMTA aggregate. The self-aggregation mechanism is usually a stepwise reaction [19]. Nevertheless, for low values of the aggregation number, this reaction can be approximated as a mean mechanism that is described by equation (3). The possible self-aggregation reactions that may occur in HMTA aqueous solutions are illustrated in Figure 3.
In an effort to verify which mechanism occurs upon dilution of HMTA in water, we recorded the Raman spectra of solutions in the dense concentration region at 20 °C. The polarized (VV) spectra in the 0.00018 to 0.083 mole fraction region are presented in Figure 4. The depolarized (VH) spectrum is shown only for the solution with the 0.083 mole fraction of HMTA. No significant spectral changes are observed with varying the HMTA mole fraction in solutions. At mole fractions below 0.0018, the spectra are dominated by the presence of the solvent bands, especially in the frequency range above 3000 cm−1. The bands observed in the fingerprint region, that is below 1500 cm−1, are due to CNC deformation and stretching, CN stretching and CH2 deformation, twisting and wagging vibrations [20,21]. In the 2800–3000 cm−1 region, the high-intensity bands are assigned to the symmetric and asymmetric C-H stretching modes, while above 3000 cm−1 the spectrum is dominated by the presence of broad and strongly overlapping bands that are attributed to the O-H stretching modes of the hydroxyl functional groups that belong to water molecules. It is also detected a relatively sharp band near ~3636 cm−1, which is assigned to the O-H stretching modes of the hydroxyl of water molecules that are hydrogen bonded with HMTA molecule. As solutions become more concentrated in the amine, the intensity of the ~3636 cm−1 peak reduces, nevertheless it continues to exist. This means that even in the concentrated amine solutions, HMTA complexation with water takes place. From the depolarized spectrum, it seems that the 785 cm−1 band, assigned to the stretching mode of the CNC with A1 symmetry, is strongly polarized. The rest of the bands are only partially polarized. The experimental and theoretically estimated Raman frequencies of HMTA in solution and in vacuum, respectively, as well as a tentative assignment are presented in Table 1.
In Figure 5, the calculated spectra of HMTA protonated species with one, two, three and four protons are illustrated. The experimental polarized (VV) Raman spectrum of the HMTA solution corresponding to 0.051 mole fraction is also presented for direct comparison. The results indicate that we can rule out the protonation of HMTA due to the large differences between the experimental and the theoretically calculated spectra of the protonated molecules of HTMA. The spectra of the protonated molecules of HMTA yield way more peaks compared to the experimental spectrum, especially in the fingerprint region.
In Figure 6, we present the calculated spectra of HMTA aggregated species with one, two, three and four water molecules. The experimental polarized (VV) Raman spectrum of an HMTA solution corresponding to 0.051 mole fraction is again shown for comparison reasons. Almost all bands coincide in frequency, while they slightly differ only in their relative intensities in the low-frequency region. The results reveal that the theoretical findings are in close agreement with the experimental Raman spectrum corresponding to 0.051 mole fraction even though the calculation is carried out in the vapor state. In addition to the very good identification of the experimental spectrum with the theoretically calculated ones in the range from 250–1500 cm−1, we also have an identification of the peak at ~3636 cm−1 of the experimental spectrum, which is attributed to the O-H stretching mode of the water molecule that is hydrogen bonded to the HMTA amine molecule. The reason why this peak appears at higher wavenumbers in the theoretical compared to the experimental spectrum is because all the theoretical spectra were calculated in the gas phase. This is also the reason why the C-H vibrations are also shifted to higher wavenumbers in the theoretical spectra. The CH2 groups are in the outer space of the molecule, and as such, are the most strongly affected by the presence of other neighboring molecules. The peak observed slightly above 3500 cm−1 in the theoretical spectra is attributed to the N-H stretching vibration of the hydrogen bond with water. In the experimental spectra, this peak is not detected probably because it is overwhelmed by the spectrum of water in that frequency region.
In Figure 7, the calculated spectrum of the HMTA· 20H2O aggregate species with twenty water molecules is presented. This aggregate was chosen to be studied theoretically because it closely mimics the experimental solution with 0.051 mole fraction (molality equal 3), in which the HMTA:H2O molar ratio is 1:18.5. The experimental polarized Raman spectra of three HMTA solutions corresponding to 0.051, 0.067 and 0.083 mole fractions are also presented for comparison.
Also in this case, a very good resemblance is observed between the experimental and theoretical spectrum in the fingerprint region. Furthermore, the intense peak observed in the highest frequency of the HMTA· 20H2O aggregate’s theoretical spectrum is attributed to the O-H stretching mode of the water molecule that is hydrogen bonded to the HMTA amine molecule and is related with the peak located at ~3636 cm−1 in the experimental spectrum. This peak appears at higher wavenumbers because the calculation was performed in the gas phase without considering any intermolecular interactions. The same also holds for the theoretically predicted frequencies of the C-H vibrations that are also calculated at higher wavenumbers relative to the experimental frequencies. Based on this more complex theoretical calculation, we can safely attribute this peak to the hydroxyl groups of the water molecules that are in the outer part of the aggregate. One hydrogen atom of these water molecules is bound to another water molecule of the aggregate, while the second hydrogen atom is free and not bounded to other water molecules of the aggregate.
Let us now examine if a self-aggregation of HMTA is likely to occur at higher mole fractions. We present in Figure 8, the calculated spectrum of HMTA self-aggregated species with aggregation number n equal to one, two, three and four. The experimental polarized (VV) Raman spectra of two HMTA solutions with 0.00018 and 0.083 mole fractions corresponding to two limiting concentrations are also presented for comparison. We observe an adequate matching between the experimental spectrum of the concentrated amine solution with the theoretically calculated spectra for the self-aggregation reaction between HMTA molecules (see Table 1). These results indicate that a self-aggregation mechanism may occur upon dilution of HMTA in aqueous environment.
From the above discussion, we can conclude that in the dilute solutions the dominating mechanism is the hetero-aggregation reaction of HMTA with water, while at higher mole fractions a self-aggregation mechanism occurs. Experimental evidence for protonation of HMTA was not found.

2.2. Sensing of the aggregation processes via ultrasonic absorption measurements

The sound absorption coefficient a for a non-electrolytic system is given by [22,23]:
a f 2 t o t a l = a f 2 r e l a x i n g   p a r t + a f 2 n o n − r e l a x i n g
The first term of the sum is related to the relaxing part that is frequency dependent and is associated with the relaxation processes that may be present in the system studied. The second term is related with the non-relaxing or classical part, which is frequency independent at least in the frequency range where the measurements are performed. The so-called non-relaxing term is also labelled with B and includes contributions from several co-existing effects such as, the vibrational relaxation, the viscous absorption, the thermal absorption and the radiation, as:
B = a f 2 v i b r a t i o n a l + a f 2 v i s c o u s + a f 2 t h e r m a l + a f 2 r a d i a t i o n
Usually, the contribution of the latter is ignored because it is insignificant relative to the rest three contributions. The sound absorption coefficient due to vibrational relaxation is a linear function of temperature and appears constant when the system is at a given temperature under thermodynamic equilibrium. Viscous contribution to the sound absorption or Stokes term is given by:
a f 2 v i s c o u s = 2 π 2 3 ρ u 3 3 η V + 4 η S
with ηV and ηS denoting the shear and volume viscosity, respectively. Other symbols have their usual meanings, that is density ρ and sound velocity u. The third contribution is the thermal sound absorption or Kirchhoff term and is a function of several thermodynamic parameters including thermal conductivity Q of the solution and the specific heats under constant pressure Cp and volume Cp/Cv, respectively. The equation for the estimation of the thermal losses is:
a f 2 t h e r m a l = 2 π 2 ρ u 3 γ − 1 Q C P
where γ is the ratio Cp/Cv. For most systems, thermal losses may be considered as negligible relative to the viscous losses and in general B is frequency independent [22,23].
For simple liquids, the sound absorption coefficient can be fitted in the frequency-domain by a single Debye-type equation through the equation [22,23,24,25]:
a f 2 = A 1 + f f r 2 + B
with fr denoting the characteristic relaxation frequency of the process. The corresponding relaxation time of the process is given by:
τ r = 1 2 π f r
In the a/f2 vs f semi-log plot, the classical absorption B is observed as a straight line, while the relaxation process appears as an excess sigmoidal form over this straight line. The single Debye-type process appears as a simple exponential relaxation in the time-domain.
The absorption spectra in the frequency reduced form (a/f2) as a function of frequency for all concentrations of HMTA aqueous solutions at 20 °C are shown in Figure 9. Symbols represent experimental points, while the solid sigmoidal lines denote the single Debye-type relaxation profiles for each concentration. The solvent ultrasound absorption coefficient was found constant in the MHz frequency range studied in this study and equal to ~20×10−17 s2/cm. The results reveal that the relaxation amplitude increases in the mole fraction range 0.035–0.083 and decreases in the range 1.8×10−7–1.8×10−2. The trend of the relaxation amplitude is shown by arrows in the graph. The fitting using Debye-type profiles was adequate to fit all the experimental spectra as indicated by the goodness of fit observed in Figure 9. The single relaxation mechanism observed in the acoustic spectra of the HMTA aqueous solutions is attributed to the aggregation reaction, which is clearly a structural process. These processes are related to shear (structural) viscosity and dominate in strongly associated solutions and neat liquids consisting of polar molecules, such as alcohols, amines, water, etc. that are bonded with hydrogen bonds.
The characteristic relaxation frequency fr, the amplitude of the relaxation A and the classical ultrasound absorption B were the only free fitting parameters in the non-linear least-mean square fitting procedure and their values as a function of HMTA mole fraction are presented in Figure 10 (a) and (b), respectively.
As already discussed, the association mechanism is two-fold. Amine molecules form structures with several water molecules in the region of low concentrations and amine molecules form structures of different sizes ranging from monomers to clusters of monomers in the range of intermediate concentrations. As is evident from the experimental acoustic spectra, all steps of the association reaction are detected as one “mean” Debye-type relaxation. It is interesting to note that the relaxation frequency experiences a drastic change below and above 0.018 mole fraction of HMTA. Initially, the characteristic frequency slightly increases, while above this mole fraction suddenly decreases. Analogous behavior is demonstrated by the relaxation amplitude in the same mole fraction. The only difference between the two acoustic parameters is that they exhibit exactly the opposite trend. Furthermore, for each acoustic parameter the rate below and above the 0.018 mole fraction is quite different.
The crossover 0.018 mole fraction signifies two separate regions with distinct structural characteristics. The relaxation observed in dilute solutions is attributed to hetero-association of HMTA with water molecules, while at higher concentrations the observed relaxation is assigned to the self-association reaction of HMTA molecules. The behavior demonstrated in Figure 10 is assigned as a “spectral signature” of the system’s structural transition from hetero- to self-aggregation of HMTA. Indeed, the amplitude of the relaxation initially decreases with concentration and then suddenly increases implying the enhancement of the self-aggregation of HMTA at the expense of the hetero-aggregation mechanism of HMTA with water molecules which is stronger in the low-concentration limit. The classical contribution B to ultrasound absorption coefficient per squared frequency a/f2 appears almost constant for all solutions as expected.
The structural model proposed for the HMTA aqueous solutions considering intermolecular interactions is expected to be reflected in several physicochemical properties of the system. In Figure 11 (a) to (d), we present the concentration dependence of the kinematic viscosity, the mass density, the sound speed, and the adiabatic compressibility of all HMTA aqueous solutions studied. All measurements were performed at 20 °C. Interestingly, all four properties exhibit the same behavior with relaxation frequency and amplitude illustrated in Figure 10 revealing two separate regions with distinct characteristics attributed to the structural variation taking place. These physicochemical properties were not chosen accidentally. The viscosity of a fluid is a measure of its resistance to deformation at a given rate and thus, it is a physicochemical parameter that is very sensitive to intermolecular relaxation due to molecular association in these solutions through hydrogen-bonding interactions. Sound velocity is an important thermo-physical property mirroring the dynamic response of the condensed phase. The velocity of sound in a liquid is sensitive to molecules’ changes and is dependent on the degree of order of the molecules. On the contrary, the sound absorption coefficient is sensitive to changes in particle size and molecular interactions. Mass density is very important in the investigation of concentration-induced structural changes in the liquid state. The transition from a loose to a more rigid structure, and vice versa is related to changes in the coordination and the cross-linking of interstitial spaces in the overall structure, which is reflected in the concentration dependency of the mass density. Finally, the adiabatic compressibility provides important information concerning specific interactions between like and unlike molecules in the binary liquid mixtures. The expression for the adiabatic compressibility is:
κ S = − 1 V ∂ V ∂ p S = 1 ρ u 2
and it is a measure of the instantaneous relative volume change of the fluid as a response to a pressure change. The results presented in Figure 11 reveal a transition from a more loose-packed structure to a more rigid one in agreement with the proposed structural model proposed based on the vibrational and acoustic spectroscopic data. The mass density and especially the kinematic viscosity data of the HMTA aqueous solutions reveal that amine molecules would exert an influence on the water structural organization.
Our results are in line with the reported X-ray data that have revealed a novel type of clathrate structure, where each amine molecule is found to occupy cavities within water clathrates constituted by hydrogen-bonded water molecules [26]. Furthermore, HMTA aqueous solutions exhibited a strong deviation from the ideal behavior suggesting the dominant role of the solute-solvent interactions [27].

3. Materials and Methods

3.1. Materials

Solid Hexamethylene tetramine (HMTA) with the molecular formula (CH2)6N4 and 99% purity was obtained from Alfa Aesar and was used without further treatment or purification. At room temperature HMTA is a white solid with ammonia like odor. HMTA does not melt, although it sublimes at 260–295 °C. Aqueous solutions of HTMA were prepared gravimetrically in a wide concentration range using triply distilled water. All solutions were clear and colorless. Its solubility in water at 20 °C was estimated ~0.67 g/mL. Molal concentration and the corresponding mole fractions are presented in Table 2. For all measurements fresh samples were used, while special attention has been paid due to HMTA’s ability to absorb carbon dioxide.

3.2. Raman Spectroscopy

The procedures for acquiring polarized and depolarized Raman spectra have already reported in the past [28,29,30,31]. All samples were excited by a 532 nm continuous-wave and linearly polarized laser in a backscattering geometry. A fully integrated confocal Raman microscope instrument (Labram SoleilTM, Horiba Scientific, France) equipped with 1800 gratings was used to analyze the scattered light. The analyzed light after the gratings was detected by a thermoelectrically cooled CCD camera down to −70 °C. The spectral resolution for all Raman measurements was fixed at 1.5 cm−1. Rayleigh scattering was reduced by means of a notch filter appropriate for the working laser wavelength. The incident power on the liquid samples was less than 3 mW to avoid thermal heating. Short accumulation times near 200 s and cumulation number equal to 5 were sufficient to achieve Raman spectra with high signal-to-noise ratio of all samples. Both vertical-vertical (VV) and horizontal-vertical (VH) polarization configurations were employed. A reference CCl4 sample was used to calibrate possible monochromator’s drift and polarization. All Raman spectroscopic measurements were carried out at 20 °C.

3.3. Ultrasonic relaxation spectroscopy

Ultrasonic absorption measurements were obtained in the low-MHz frequency region under isobaric conditions by employing the transmission method [32,33,34]. A cylindrical acoustic cell was used to accommodate approximately 2 mL of the liquid sample at the desired temperature. The cell was made of fused silica glass to endure chemical durability. The temperature was controlled by an external circulation system with an accuracy of ±0.01 °C. Two identical broadband transducers (Olympus V111, central frequency 10 MHz) were attached to the parallel faces of the cylindrical acoustic cell. A common medical couplant was placed between the piezoelectric element and the cell surface to ensure perfect transmission of the ultrasound. The first piezoelectric element, which acts as transmitter, is triggered by a pulse generator (TTi, TGP3151) at the desired frequency. The time duration of the pulse was less than 3.5 microseconds, and the pulse repetition rate was fixed at 5 milliseconds. The so-obtained ultrasonic wave propagates back and forth through the sample many times after multiple reflections in the parallel faces of the acoustic cell. The transmitted pulse, as well as the consequential echoes, were detected by the receiving transducer and sent to a digital oscilloscope (Tektronix, TBS 1202B) for further signal analysis. For each frequency, the attenuation coefficient of the solution sample can be directly estimated from the exponential decrease of the signal amplitude. The accuracy of the sound absorption measurements is better than ± 5%. The ultrasound speed is calculated by the time required for the ultrasonic wave to travel the fixed path length of the acoustic cell with an accuracy of ± 0.01% [32,33,34]. All ultrasonic relaxation spectroscopic measurements were carried out at 20 °C.

3.4. DFT calculations

Quantum–mechanical calculations were carried out for the HMTA and the relevant aggregated and protonated species in gas phase with the Gaussian 09 W Revision D.01 package of programs [35]. Density functional theory (DFT) using B3LYP functional and 6-311++G(d,p) basis set was employed to optimize the molecular structure of HMTA-based species, and the corresponding vibrational frequencies [36]. More details concerning the methodology of the theoretical calculations of the reaction volume change can be found in reference [34]. The molecular structure of HMTA was received as SDF digital file from the PubChem electronic database and was used for structure optimization and further calculations.
To elucidate potential protonation reaction of HMTA, we performed theoretical calculations on the protonated species of HMTA with one, two, three and four protons, respectively. We chose these structures for the evaluation of the protonation reaction. To interpret possible self-aggregation of HMTA, we performed calculations on four structures of the solute associated species (HMTA)n with aggregation number n=2, 3 and 4. To clarify the hetero-aggregation mechanism of HMTA with water molecules, we constructed five aggregates HMTA·xH2O, x=1-4, 20 that consist of one HMTA molecule and 1, 2, 3, 4 and 20 molecules of H2O, respectively. In all cases, water molecules were aggregated with the solute through hydrogen bonding between a hydrogen atom from water and a nitrogen atom from HMTA molecule. The theoretical study of the species with the 20 water molecules surrounding the HMTA solute will provide a more realistic simulation of the dilute region. All theoretically predicted molecular structures of HMTA molecule and all HMTA protonated and aggregated species can be found in Supplementary Material.

4. Conclusions

An experimental concentration dependent Raman and ultrasonic relaxation spectroscopic study of HMTA aqueous solutions has been undertaken to study structural changes in short- and medium-range order.
By comparing the experimental Raman spectroscopic data with quantum mechanical calculations on isolated species, the protonation of HMTA was excluded due to the extended spectral differences in the fingerprint region. In dilute solutions, the dominating mechanism is the hetero-aggregation reaction of HMTA with water, while in the intermediate concentration region a self-aggregation mechanism is also present. Processes related with conformational changes are unlikely due to the rigid molecular structure of HMTA.
The acoustic spectra of the HMTA aqueous solutions revealed a single relaxation mechanism, which is attributed to the aggregation reaction. The concentration dependence of the relaxation frequency and amplitude exhibits a drastic change below and above 0.018 mole fraction of HMTA, although with the opposite trend. This characteristic mole fraction signifies two separate regions with distinct structural characteristics. The relaxation observed in dilute solutions is attributed to hetero-association of HMTA with water molecules, while at higher concentrations the observed relaxation is assigned to the self-association reaction of HMTA molecules. In the low-concentration region and up to the crossover mole fraction, the relaxation amplitude decreases, while above this characteristic concentration suddenly increases indicating the strengthening of the self-aggregation of HMTA process at the expense of the hetero-aggregation mechanism of HMTA with water molecules, which is expected to be robust in the low-concentration limit.
The concentration dependence of the kinematic viscosity and mass density indicate the passage from a loose packed to a more rigid structure composed of HMTA self-aggregates. Analogous behavior is exhibited by the ultrasound velocity and the adiabatic compressibility. All four physicochemical properties experience drastic changes when approaching the crossover mole fraction supporting our proposed structural model proposed based on the vibrational and acoustic spectroscopic data.

Supplementary Materials

The following supporting information can be downloaded at the website of this paper posted on Preprints.org.

Author Contributions

Conceptualization, A.G.K. and T.A.K.; methodology, A.G.K.; validation, A.G.K.; S.T. and T.A.K.; formal analysis, A.G.K. and S.T.; investigation, S.T.; M.G.P.; T.A.K. and A.G.K.; resources, T.A.K. and A.G.K.; data curation, S.T.; T.A.K. and A.G.K.; writing–original draft preparation, S.T. and A.G.K.; writing–review and editing, S.T.; M.G.P., T.A.K. and A.G.K.; supervision, A.G.K.; project administration, A.G.K.; funding acquisition, T.A.K. and A.G.K. All authors have read and agreed to the published version of the manuscript.

Funding

This research received no external funding.

Data Availability Statement

Data are available upon request from the corresponding author.

Acknowledgments

This work was carried out in fulfilment of the requirements for the Ph.D thesis of S. Tsigoias according to the curriculum of the Department of Chemistry, University of Ioannina, under the supervision of A. G. Kalampounias.

Conflicts of Interest

The authors declare no conflict of interest.

References

  1. Bakhit, M.; Krzyzaniak, N.; Hilder, J.; Clark, J.; Scott, A.M.; Mar, C.D. Use of methenamine hippurate to prevent urinary tract infections in community adult women: a systematic review and meta-analysis. Br. J. Gen. Pract. 2021, 71, e528–e537. [Google Scholar] [CrossRef] [PubMed]
  2. Schoen A.H. Re: Equivalence of methenamine Tablets Standard for Flammability of Carpets and Rugs. Report of U.S. Consumer Product Safety Commission, July 29, 2004.
  3. Retrieved from UK Food Standards Agency: “Current EU approved additives and their E Numbers”. https://www.food.gov.uk/business-guidance/approved-additives-and-e-numbers.
  4. Eller K, Henkes E, Rossbacher R, Höke H (2000). “Amines, Aliphatic”. Ullmann’s Encyclopedia of Industrial Chemistry. Wiley-VCH Verlag GmbH.
  5. Blažzević, N.; Kolbah, D.; Belin, B.; Šunjić, V.; Kajfež, F. Hexamethylenetetramine, A Versatile Reagent in Organic Synthesis. Synthesis 1979, 3, 161–176. [Google Scholar] [CrossRef]
  6. Tryfon, A.; Siafarika, P.; Kouderis, C.; Kaziannis, S.; Boghosian, S.; Kalampounias, A.G. Evidence of Self-Association and Conformational Change in Nisin Antimicrobial Polypeptide Solutions: A Combined Raman and Ultrasonic Relaxation Spectroscopic and Theoretical Study. Antibiotics 2023, 12, 221. [Google Scholar] [CrossRef] [PubMed]
  7. Kalampounias, A.G.; Kirillov, S.A.; Steffen, W.; Yannopoulos, S.N. Raman spectra and microscopic dynamics of bulk and confined salol. J. Molec. Struct. 2003, 651–653, 475–4831.
  8. Tsigoias, S.; Kouderis, C.; Mylona-Kosmas, A.; Boghosian, S.; Kalampounias, A.G. Proton-transfer in 1,1,3,3 tetramethyl guanidine by means of ultrasonic relaxation and Raman spectroscopies and molecular orbital calculations. Spectrochim. Acta A 2020, 229, 117958. [Google Scholar] [CrossRef]
  9. Tsigoias, S.; Papanikolaou, M.G.; Kabanos, T.A.; Kalampounias, A.G. Structure and dynamics of aqueous norspermidine solutions: an in situ ultrasonic relaxation spectroscopic study. J. Phys.: Condens. Matter, 2021, 33, 495104.
  10. Bernasconi, C.F. Relaxation kinetics. Academic Press, New York, 1976.
  11. Herzfeld, K.F.; Litovitz, T.A. Absorption and Dispersion of Ultrasonic Waves (Academic Press, New York, 1959).
  12. Mpourazanis, P.; Stogiannidis, G.; Tsigoias, S.; Kalampounias, A.G. Transverse phonons and intermediate-range order in Sr-Mg fluorophosphate glasses Spectrochim. Acta A. 2019, 212, 363–370. [Google Scholar] [CrossRef]
  13. Mpourazanis, P.; Stogiannidis, G.; Tsigoias, S.; Papatheodorou, G.N.; Kalampounias, A.G. Ionic to covalent glass network transition: Effects on elastic and vibrational properties according to ultrasonic echography and Raman spectroscopy. J. Phys. Chem. Solids. 2019, 125, 43–50. [Google Scholar] [CrossRef]
  14. Stogiannidis, G.; Tsigoias, S.; Mpourazanis, P.; Boghosian, S.; Kaziannis, S.; Kalampounias, A.G. Dynamics and vibrational coupling of methyl acetate dissolved in ethanol. Chem. Phys. 2019, 522, 1–9. [Google Scholar] [CrossRef]
  15. Ivanov, E.V.; Batov, D.V. Unusual behavior of temperature-dependent solvent H/D isotope effects in the enthalpy and heat capacity of hexamethylenetetramine (urotropine) hydration. J. Molec. Liq. 2019, 285, 508–514. [Google Scholar] [CrossRef]
  16. Ivanov, E.V. Temperature-dependent standard volumetric properties of hexamethylenetetramine in ordinary and deuterated water: A study resolving debatable issues being commented in the [Journal of Molecular Liquids, 248 (2017) 48–52]. J. Molec. Liq. 2018, 272, 218–225. [Google Scholar] [CrossRef]
  17. Thomas, C.W. Hexamethylenetetramine Hexahydrate: A New Type of Clathrate Hydrate. J. Chem. Phys. 1965, 43, 2799–2805. [Google Scholar]
  18. Davidson, D.W. Dielectric relaxation and orientational ordering of water molecules in hexamethylenetetramine hexahydrate. Division of Applied Chemistry, National Research Council of Cariada, Ottawa, Canada 1967.
  19. Chettiyankandy, P.; Chand, A.; Ghosh, R.; Sarkar, S.K.; Das, P.; Chowdhuri, S. Effects of hexamethylenetetramine (HMTA) on the aqueous solution structure, dynamics and ion solvation scenario: A concentration and temperature dependent study with potential HMTA models. J. Molec. Liq. 2019, 296, 111820. [Google Scholar] [CrossRef]
  20. Santos, P.S. ; Raman Spectra of Some Adducts of Hexamethylenetetramine, J. Raman Spectr. 1989, 20, 405–408. [Google Scholar] [CrossRef]
  21. Bertie, J.E.; Solinas, M. Infrared and Raman spectra and the vibrational assignment of hexamethylenetetramine-h12 and -d12. J. Chem. Phys. 1974, 61, 1666–1677. [Google Scholar] [CrossRef]
  22. Litovitz, T.A.; Davis, C.M. Physical Acoustics, ed. W. P. Mason, Vol. IIA, (1965), Academic Press, London and New York.
  23. Herzfeld, K.F.; Litovitz, T.A. Absorption and Dispersion of UltrasonicWaves, Academic Press, New York, 1959.
  24. Ensminger, D.; Bond, L.J. Ultrasonics: Fundamentals, Technologies, and Applications, Third edition CRC Press, New York, 2011.
  25. Blandamer, M.J. Introduction to Chemical Ultrasonics, Academic Press, New-York, 1973.
  26. Davidson, D.W. Dielectric relaxation and orientational ordering of water molecules in hexamethylenetetramine hexahydrate. Can. J. Chem. 1968, 46, 1024–1027. [Google Scholar] [CrossRef]
  27. Crescenzi, V.; Quadrifoglio, F.; Vitagliano, V. Hexamethylenetetramine Aqueous Solutions. Isopiestic Data at 25° and Density and Viscosity Data in the Range 3–34o, J. Phys. Chem. 1967, 71, 2313–2318.
  28. Kalampounias, A.G.; Tsilomelekis, G.; Boghosian, S. Glass-forming ability of TeO2 and temperature induced changes on the structure of the glassy, supercooled, and molten states. J. Chem. Phys. 2015, 142, 154503. [Google Scholar] [CrossRef] [PubMed]
  29. Kalampounias, A.G.; Yannopoulos, S.N.; Papatheodorou, G.N. Temperature- induced structural changes in glassy, supercooled, and molten silica from 77 to 2150 K. J. Chem. Phys. 2006, 124, 014504. [Google Scholar] [CrossRef] [PubMed]
  30. Latsis, G.K.; Banti, C.N.; Kourkoumelis, N.; Papatriantafyllopoulou, C.; Panagiotou, N.; Tasiopoulos, A.; Douvalis, A.; Kalampounias, A.G.; Bakas, T.; Hadjikakou, S.K. Poly Organotin Acetates against DNA with Possible Implementation on Human Breast Cancer. Int. J. Mol. Sci. 2018, 19, 2055. [Google Scholar] [CrossRef] [PubMed]
  31. Kalampounias, A.G.; Kirillov, S.A.; Steffen, W.; Yannopoulos, S.N. Raman spectra and microscopic dynamics of bulk and confined salol. J. Molec. Struct. 2003, 651–653, 475–483.
  32. Kouderis, C.; Siafarika, P.; Kalampounias, A.G. Disentangling proton-transfer and segmental motion relaxations in poly-vinyl-alcohol aqueous solutions by means of ultrasonic relaxation spectroscopy. Polymer 2021, 217, 123479. [Google Scholar] [CrossRef]
  33. Kalampounias, A.G. Establishing the role of shear viscosity on the rate constants of conformational fluctuations in unsaturated aldehydes. Chem. Phys. 2022, 561, 111618. [Google Scholar] [CrossRef]
  34. Kouderis, C.; Siafarika, P.; Kalampounias, A.G. Molecular relaxation dynamics and self-association of dexamethasone sodium phosphate solutions. Chem. Pap. 2021, 75, 6115–6125. [Google Scholar] [CrossRef]
  35. Frisch, M.J. et al., Gaussian 09, Revision A.02, Gaussian, Inc, Wallingford CT, 2009.
  36. Becke, A. Density-Functional Thermochemistry. III. The Role of Exact Exchange. J. Chem. Phys. 1993, 98, 5648–5652. [Google Scholar]
Preprints 89399 g001
Figure 1. Possible proton transfer reactions that may occur in HMTA aqueous solutions. Each reaction corresponds to a subsequent protonation step.
Figure 1. Possible proton transfer reactions that may occur in HMTA aqueous solutions. Each reaction corresponds to a subsequent protonation step.
Preprints 89399 g001
Preprints 89399 g002
Figure 2. Possible hetero-aggregation reactions that may occur in HMTA aqueous solutions. Each reaction corresponds to a subsequent aggregation step.
Figure 2. Possible hetero-aggregation reactions that may occur in HMTA aqueous solutions. Each reaction corresponds to a subsequent aggregation step.
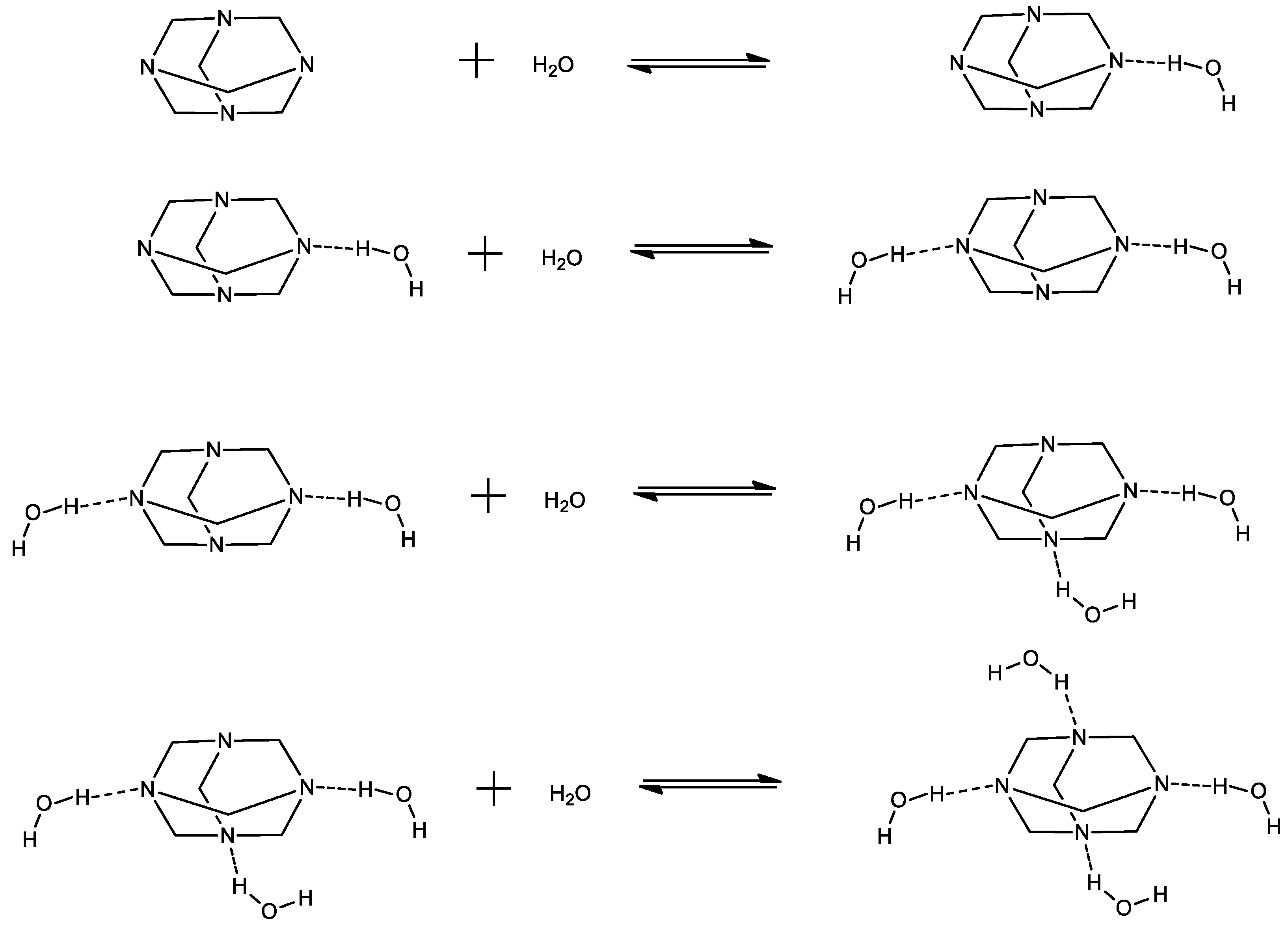
Preprints 89399 g002
Preprints 89399 g003
Figure 3. Possible self-aggregation reactions that may occur in HMTA aqueous solutions. The second reaction corresponds to a mean reaction of the usually stepwise aggregation mechanism. This hypothesis is valid for relatively low values of the aggregation number n.
Figure 3. Possible self-aggregation reactions that may occur in HMTA aqueous solutions. The second reaction corresponds to a mean reaction of the usually stepwise aggregation mechanism. This hypothesis is valid for relatively low values of the aggregation number n.
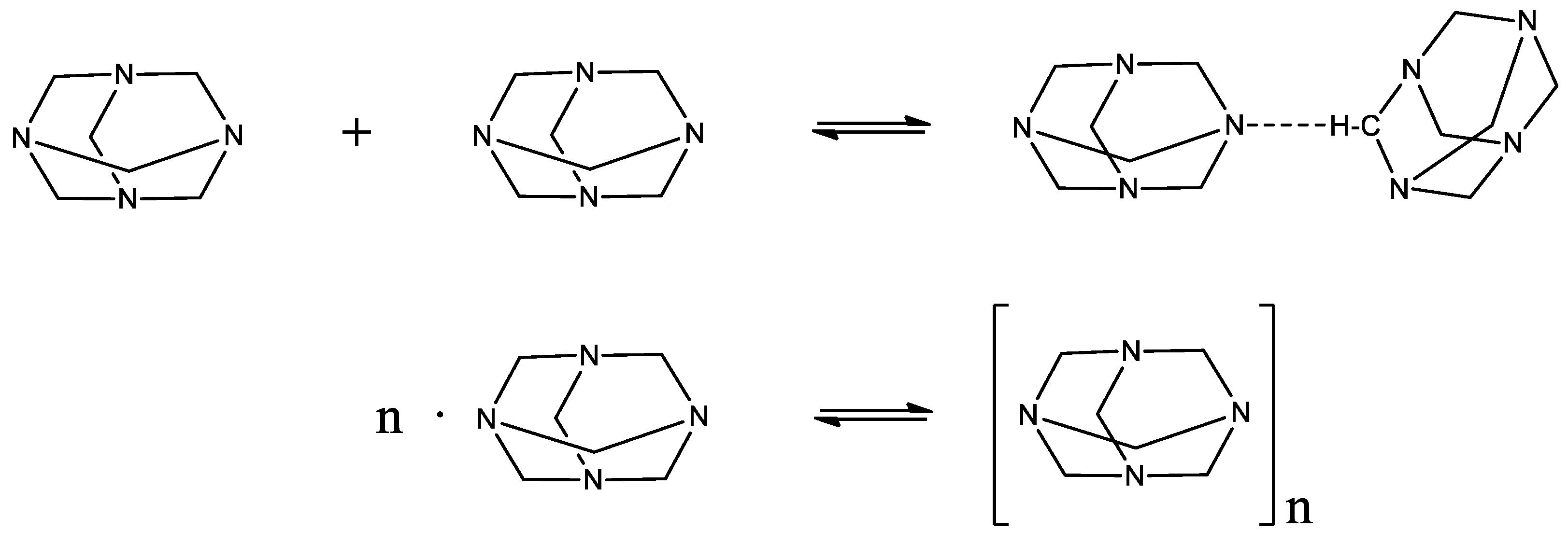
Preprints 89399 g003
Preprints 89399 g004
Figure 4. Concentration dependence of the experimental polarized (VV) Raman spectra of HMTA aqueous solutions in the dense concentration region at 20 °C. The depolarized (VH) spectrum is presented only for one solution corresponding to 0.083 mole fraction of HMTA. The intensity of the depolarized spectrum is multiplied by a factor of 2 to facilitate the observation of the bands in the fingerprint region (<1500 cm−1).
Figure 4. Concentration dependence of the experimental polarized (VV) Raman spectra of HMTA aqueous solutions in the dense concentration region at 20 °C. The depolarized (VH) spectrum is presented only for one solution corresponding to 0.083 mole fraction of HMTA. The intensity of the depolarized spectrum is multiplied by a factor of 2 to facilitate the observation of the bands in the fingerprint region (<1500 cm−1).
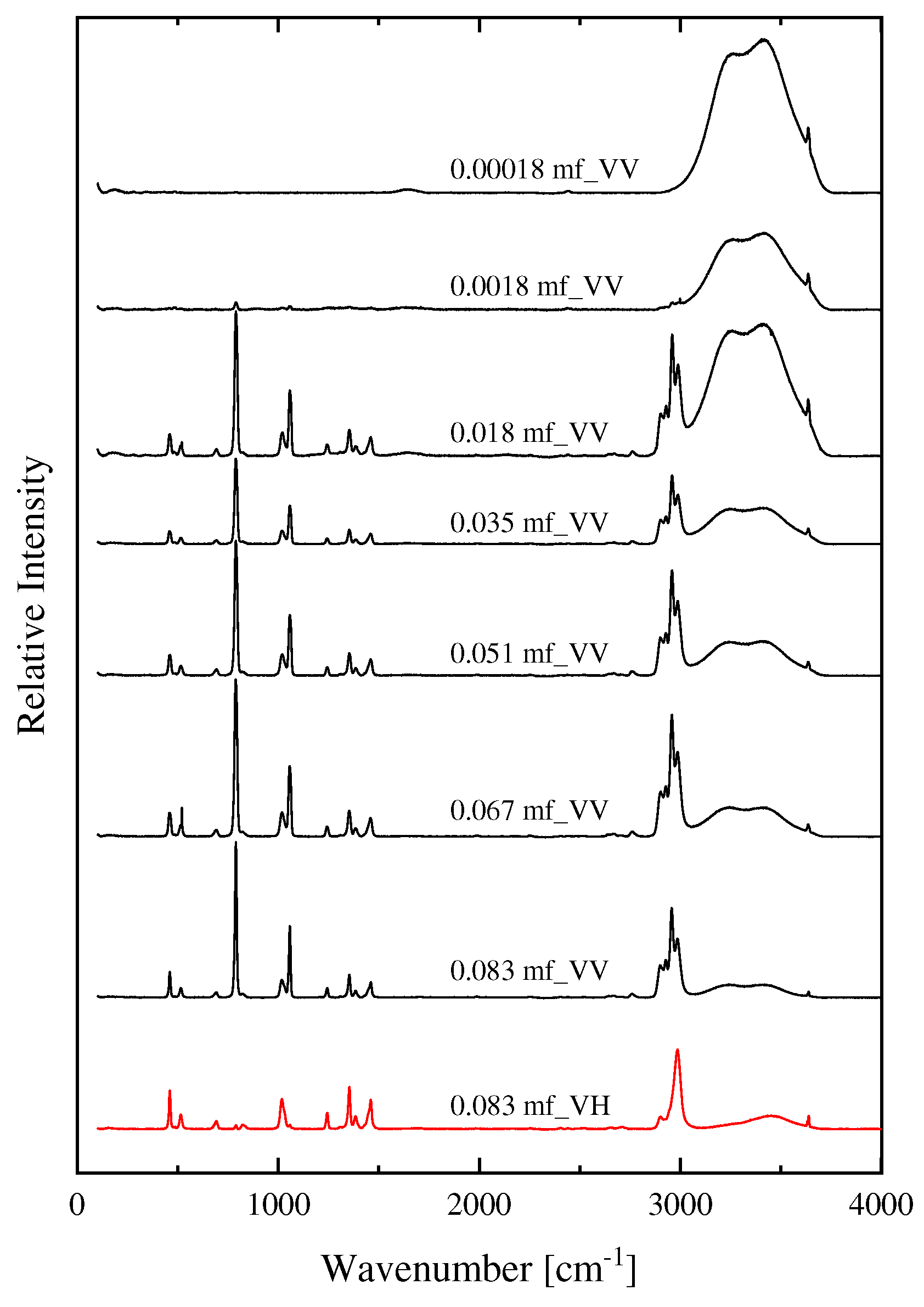
Preprints 89399 g004
Preprints 89399 g005
Figure 5. The calculated spectra of HMTA protonated species with one, two, three and four protons. See text for details concerning the calculation procedure. The experimental polarized Raman spectrum of the HMTA solution corresponding to 0.051 mole fraction is also presented for comparison.
Figure 5. The calculated spectra of HMTA protonated species with one, two, three and four protons. See text for details concerning the calculation procedure. The experimental polarized Raman spectrum of the HMTA solution corresponding to 0.051 mole fraction is also presented for comparison.
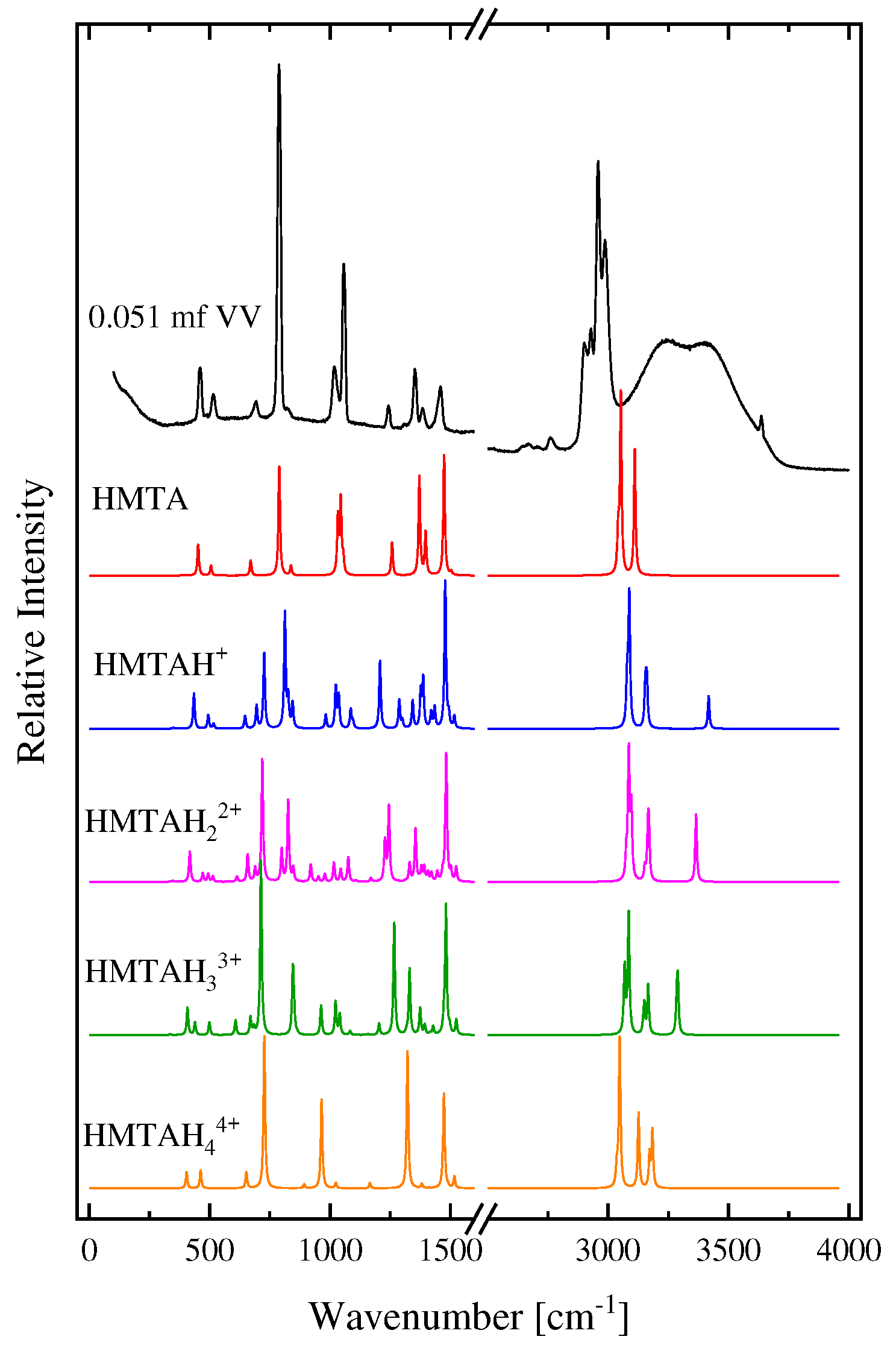
Preprints 89399 g005
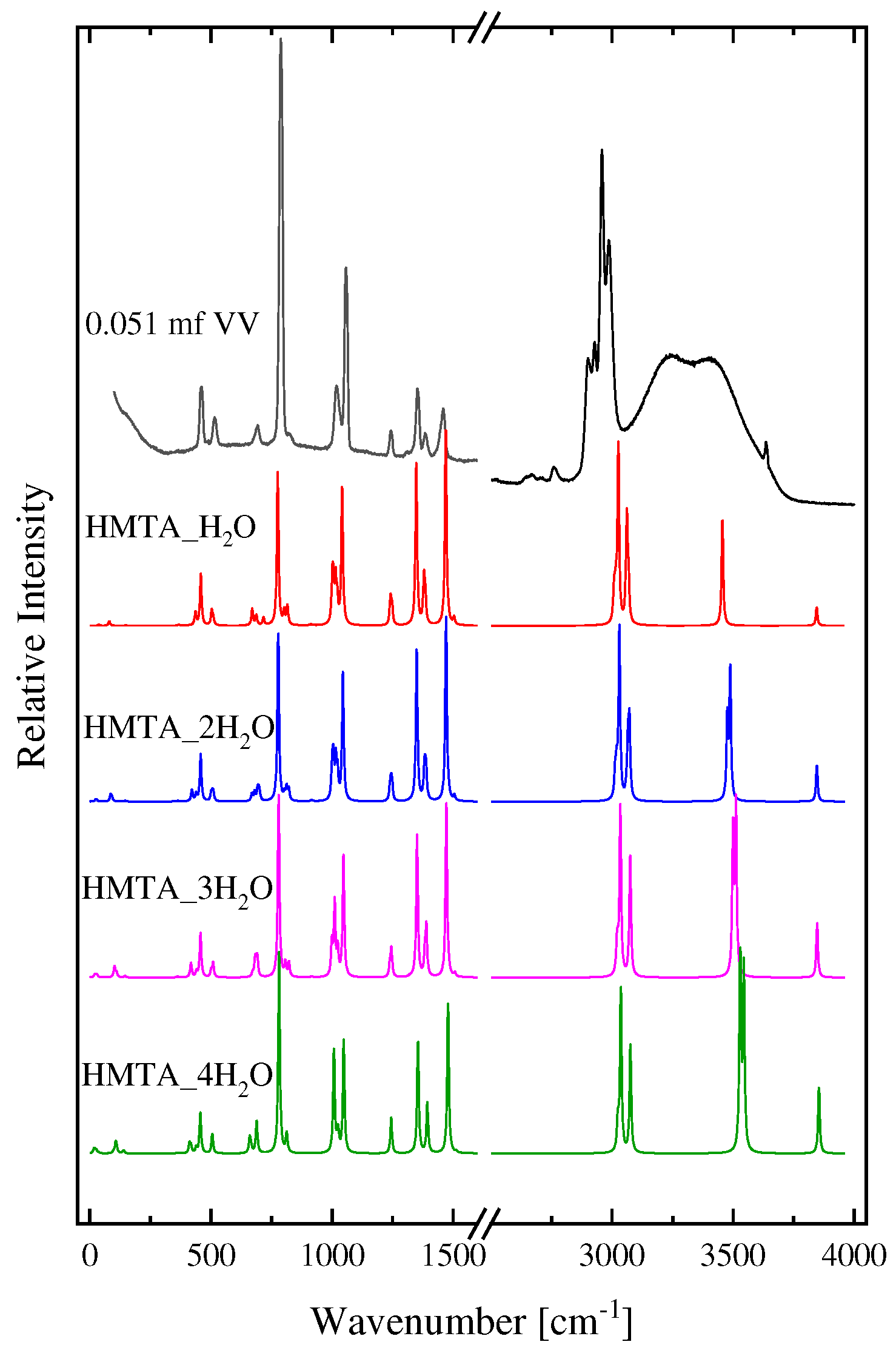
Preprints 89399 g006
Figure 6. The calculated spectra of HMTA aggregated species with one, two, three and four water molecules. See text for details concerning the calculatino procedure. The experimental polarized Raman spectrum of an HMTA solution corresponding to 0.051 mole fraction is also presented for comparison.
Figure 6. The calculated spectra of HMTA aggregated species with one, two, three and four water molecules. See text for details concerning the calculatino procedure. The experimental polarized Raman spectrum of an HMTA solution corresponding to 0.051 mole fraction is also presented for comparison.
Preprints 89399 g006
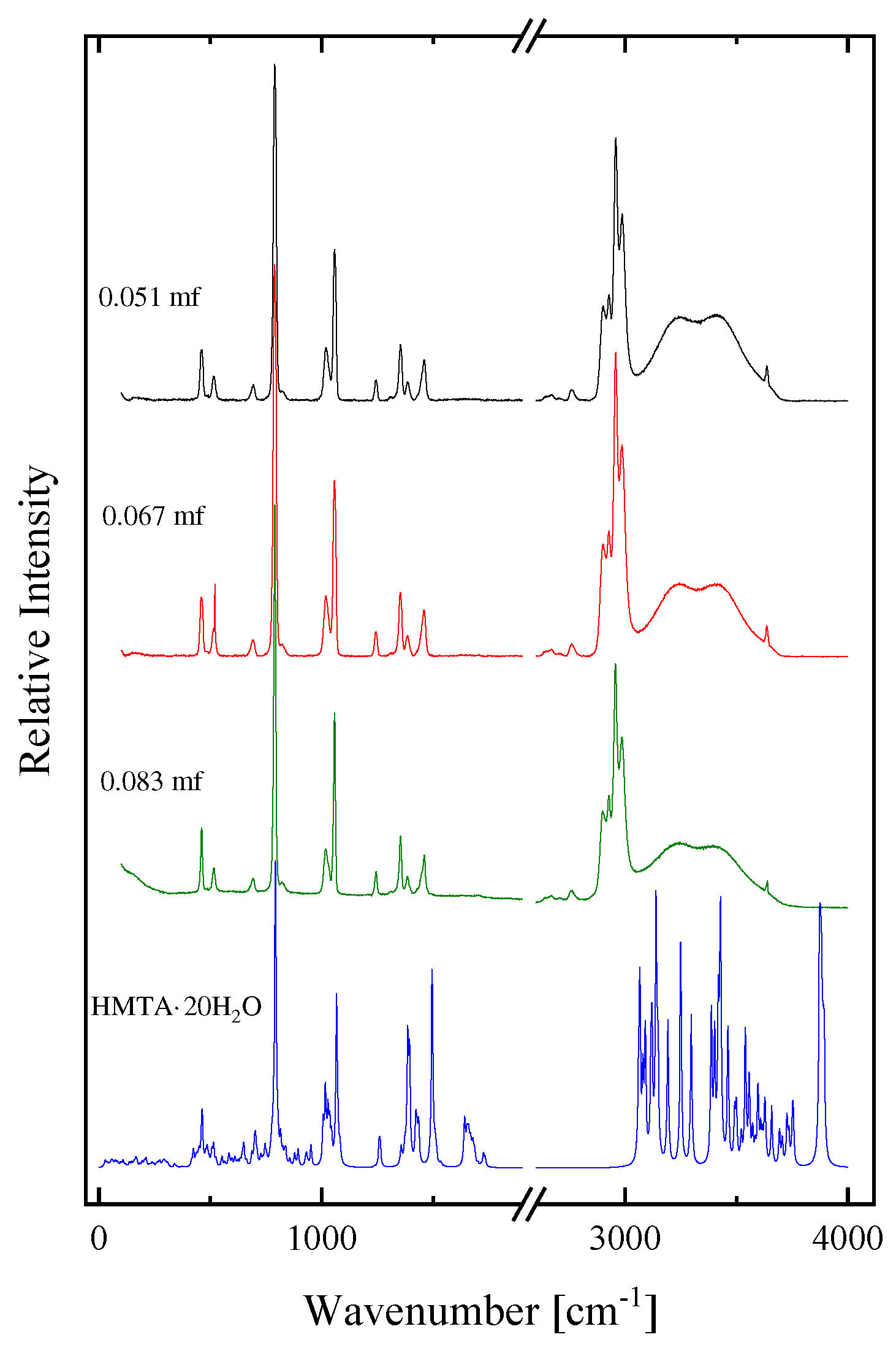
Preprints 89399 g007
Figure 7. The calculated spectrum of HMTA aggregated species with twenty water molecules. See text for details concerning the calculation procedure. The HMTA· 20H2O aggregate closely mimics the experimental solution with 0.051 mole fraction (molality equal to 3), in which the HMTA:H2O molar ratio is 1:18.5. The experimental polarized Raman spectra of three different HMTA solutions corresponding to 0.051, 0.067 and 0.083 mole fractions are also peresented for comparison.
Figure 7. The calculated spectrum of HMTA aggregated species with twenty water molecules. See text for details concerning the calculation procedure. The HMTA· 20H2O aggregate closely mimics the experimental solution with 0.051 mole fraction (molality equal to 3), in which the HMTA:H2O molar ratio is 1:18.5. The experimental polarized Raman spectra of three different HMTA solutions corresponding to 0.051, 0.067 and 0.083 mole fractions are also peresented for comparison.
Preprints 89399 g007
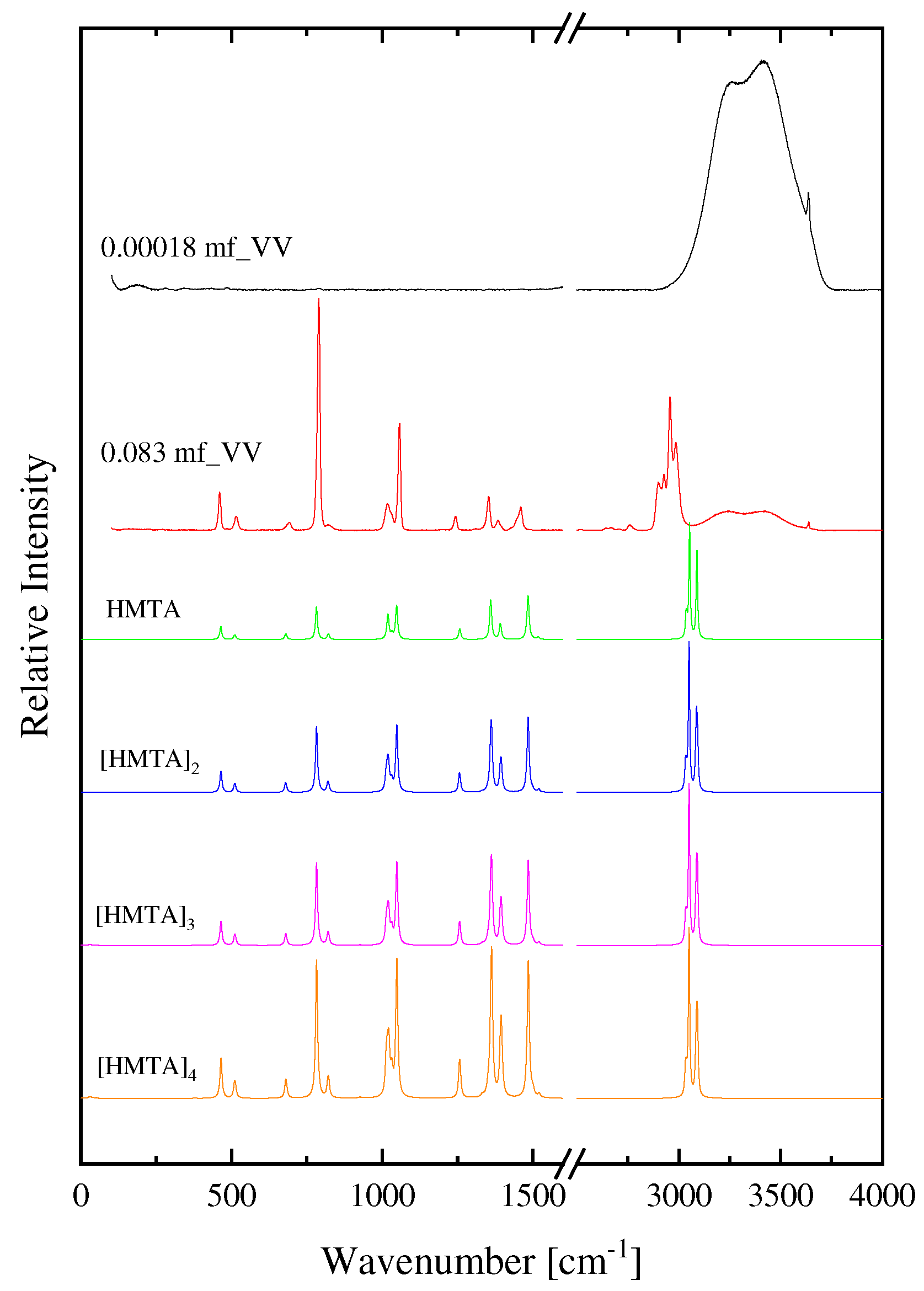
Preprints 89399 g008
Figure 8. The calculated spectrum of HMTA self-aggregated species with aggregation number n equal to one, two, three and four. See text for details concerning the calculation procedure. The experimental polarized Raman spectra of two HMTA solution with 0.00018 an 0.083 mole fractions corresponding to low and intermediate concentration are also presented for comparison.
Figure 8. The calculated spectrum of HMTA self-aggregated species with aggregation number n equal to one, two, three and four. See text for details concerning the calculation procedure. The experimental polarized Raman spectra of two HMTA solution with 0.00018 an 0.083 mole fractions corresponding to low and intermediate concentration are also presented for comparison.
Preprints 89399 g008
Preprints 89399 g009
Figure 9. Ultrasound absorption spectra in the frequency reduced form (a/f2) for all concentrations of HMTA aqueous solutions at 20 °C. Squared symbols represent experimental points and solid lines denote the single Debye-type relaxation profiles for each concentration. See text for more details concerning the fitting of the experimental spectra. The ultrasound absorption coefficient of the solvent (water) was constant in the MHz frequency range studied in this study. Arrows denote that the relaxation amplitude increases in the range 0.035–0.083 and decreases in the range 1.8×10−7–1.8×10−2.
Figure 9. Ultrasound absorption spectra in the frequency reduced form (a/f2) for all concentrations of HMTA aqueous solutions at 20 °C. Squared symbols represent experimental points and solid lines denote the single Debye-type relaxation profiles for each concentration. See text for more details concerning the fitting of the experimental spectra. The ultrasound absorption coefficient of the solvent (water) was constant in the MHz frequency range studied in this study. Arrows denote that the relaxation amplitude increases in the range 0.035–0.083 and decreases in the range 1.8×10−7–1.8×10−2.
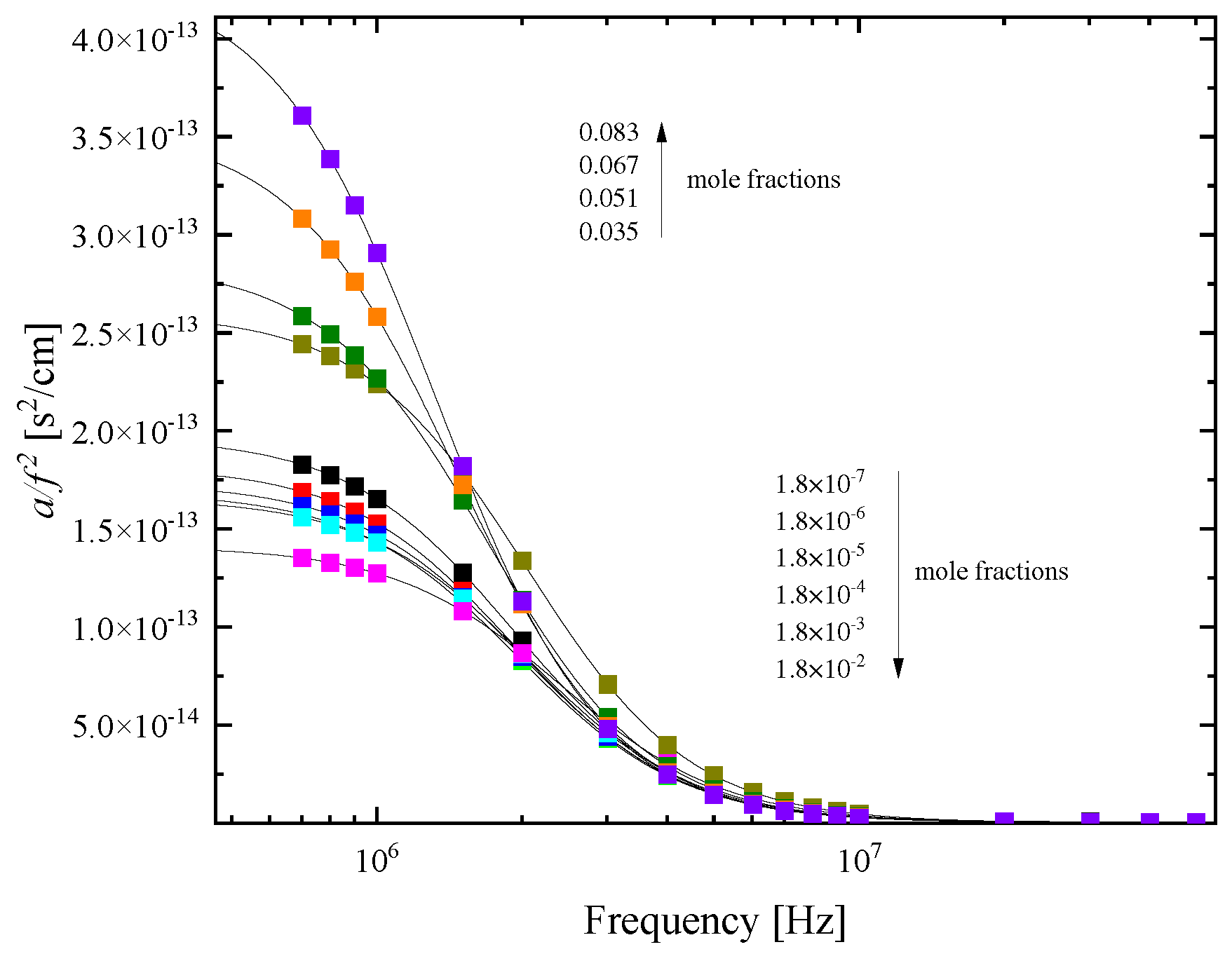
Preprints 89399 g009
Preprints 89399 g010
Figure 10. (a) Characteristic ultrasonic relaxation frequency fr as a function of mole fraction for HMTA aqueous solutions at 20 °C. (b) Concentration dependence of the relaxation amplitude A and classical contribution to a/f2 denoted as B.
Figure 10. (a) Characteristic ultrasonic relaxation frequency fr as a function of mole fraction for HMTA aqueous solutions at 20 °C. (b) Concentration dependence of the relaxation amplitude A and classical contribution to a/f2 denoted as B.
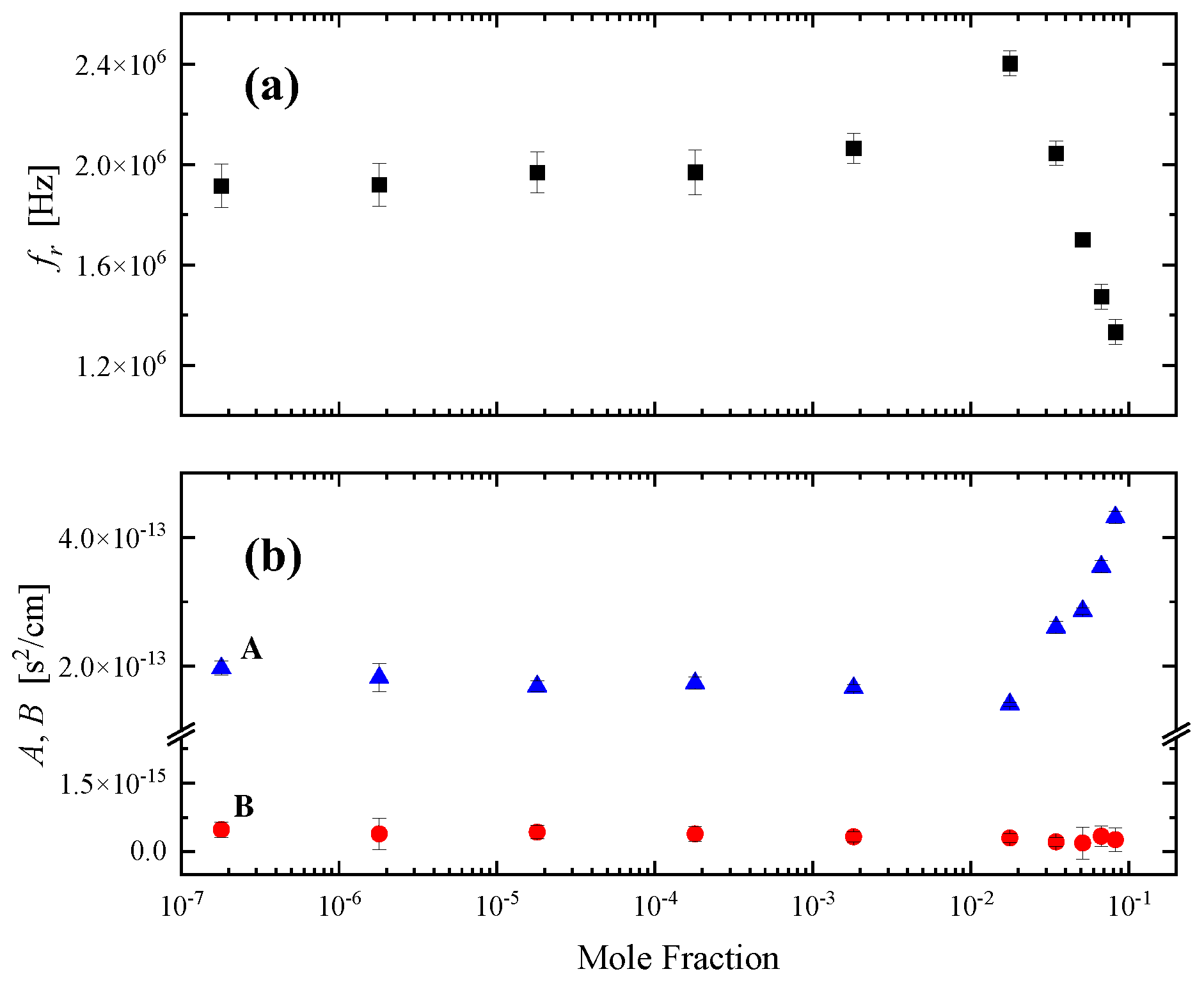
Preprints 89399 g010
Preprints 89399 g011
Figure 11. Concentration dependence of the kinematic viscosity (a), mass density (b), ultrasound velocity (c) and adiabatic compressibility (d) for all HMTA aqueous solutions studied. All physical properties and acoustic parameters were measured at 20 °C. Lines denote two distinct regions of interest.
Figure 11. Concentration dependence of the kinematic viscosity (a), mass density (b), ultrasound velocity (c) and adiabatic compressibility (d) for all HMTA aqueous solutions studied. All physical properties and acoustic parameters were measured at 20 °C. Lines denote two distinct regions of interest.
Preprints 89399 g011
Table 1. Experimental and theoretical Raman Frequencies of HMTA in solution and vacuum, respectively and band assignments.
Table 1. Experimental and theoretical Raman Frequencies of HMTA in solution and vacuum, respectively and band assignments.
Experimental Raman frequencies (cm−1) in solution Theoretical Raman frequencies (cm−1) in vacuum Assignment Symmetry
59 463 CNC deformation E Fingerprint region (<1500 cm−1)
515 510 CNC deformation F2
692 680 CNC deformation F2
785 789 CNC stretching A1
823 821 CH2 rocking F2
1020 1019 CN stretching F2
1056 1048 CNC deformation A1
1242 1257 CN stretching F2
1353 1359 CH2 twisting E
1384 1392 CH2 wagging F2
1455 1483 CH2 deformation E
2902 3037 Symmetric and asymmetric C-H stretching C-H region
2931 3039
2959 3052
2988 3088
3253 - O-H stretching of water molecules O-H region
3410 - O-H stretching of water molecules
3636 - O-H stretching of water molecules that are hydrogen bonded with HMTA molecule
3648 - O-H stretching of water molecules
Table 2. Molal concentration and the corresponding mole fractions.
Table 2. Molal concentration and the corresponding mole fractions.
Molal Concentration
(mol/Kgsolvent)		 Mole fraction
1 × 10−5 1.80 × 10−7
1 × 10−4 1.80 × 10−6
1 × 10−3 1.80 × 10−5
0.01 1.80 × 10−4
0.1 1.82 ×10−3
1.0 0.018
2.0 0.035
3.0 0.051
4.0 0.067
5.0 0.083
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content.
Copyright: This open access article is published under a Creative Commons CC BY 4.0 license, which permit the free download, distribution, and reuse, provided that the author and preprint are cited in any reuse.
Prerpints.org logo

Preprints.org is a free preprint server supported by MDPI in Basel, Switzerland.

facebook logotwitter logolinkedin logo
MDPI Initiatives
Important Links
Subscribe

Disclaimer

Terms of Use

Privacy Policy

© 2024 MDPI (Basel, Switzerland) unless otherwise stated