Process Parameters of High Frequency Welding

Preprint

Article

Process Parameters of High Frequency Welding

Altmetrics

Downloads

157

Views

Comments

A peer-reviewed article of this preprint also exists.

This version is not peer-reviewed

Submitted:

27 November 2023

Posted:

27 November 2023

You are already at the latest version

Alerts

Abstract

So far, scientific studies on high frequency welding (HF) have observed the three main parameters: anode current, welding time and pressure of upper electrode. Some authors have also observed and studied the welding frequency, the power, and the values of the dielectric constants of the materials or the angle of dielectric losses. Due to the complex effects that the parameters have on the quality of the welded joint, it is necessary to extend the research and study the mutual relationships between a large numbers of process parameters on the welded joint in order to achieve satisfactory strength of the welded joint. In this research, the number of considered parameters is increased to 17, each of which is relevant to the processes of HF welding, and their mathematical equations are presented. The research is carried out on a HF welding machine improved with a linear pneumatic actuator for lowering the upper electrode and regulating the compressive force, and a measuring machine with the appropriate measuring method for determining the breaking forces with a tension gage and performing the „T“ test is made. Numerous researches were carried out on the functional changes of the process parameters of the percentage share of the values of the coupling capacitor, the anode current and the welding time on the values of the breaking forces of the welded joints. Based on the conducted research, it was concluded that the optimization of the process parameters of HF machine must be performed before the welding process, which is determined by a certain amount of energy that must be introduced into the material. These parameters are the capacity of the output capacitor, i.e., the value of the electric current flowing through the material and the time for the development of the operating power to produce welded joints with a certain strength and quality of welded joints for a specific material used in the process.

Keywords:

Subject: Chemistry and Materials Science - Paper, Wood and Textiles

1. Introduction

The use of high frequency (HF) electromagnetic field for welding parts of clothing is related to high technological techniques of welding, such as ultrasonic welding, welding using heat in the form of conduction and convection, and welding with infrared lasers. The aforementioned techniques are displacing the traditional techniques for joining clothing parts, namely sewing and gluing, due to their favourable characteristics such as lower energy consumption, high productivity and environmental awareness. They are characterised by exceptional strength of the produced welded joints, air and water impermeability and are used in almost all joining techniques in apparel technology.

The oldest available research paper on high frequency heating dates from 1944 and is titled “High frequency Heating.” In this paper, not only the physical aspects of the heat phenomenon in treated materials, the technique for generating a HF electric field that causes rapid and uniform heating through the depth of the material, as well as the drying of wooden laminates for the aircraft industry, the drying of agricultural raw materials and food, diathermy (heat treatment of the human body at low wavelengths) are described, but also the possibility of joining flat textile products [1]. Later, in 1946, a new paper entitled “Radio Frequency Heating” was published, covering the same subject as the previous one. In this paper, it is described how HF vibration, induction and electrical heating can be achieved [2]. This paper mentions for the first time the phase change between current and voltage in a dielectric and uses the equation for the power dissipation in the material, which depends on the frequency, the tangent of the phase change angle, the square of the input voltage and the dielectric properties of the treated material, but it does not specify which these properties are. In 1974, the standard DIN 16960 entitled “Welding of Thermoplastics Principles” defined practically all joining techniques of thermoplastic materials and specified the methods and basic parameters of HF welding [3]. It states that the welding techniques are suitable for thermoplastic materials with dissipation factor (tg δ) that should be equal to or higher than 0.01, measured at a frequency of 1 MHz and a temperature of 20°C. The welded joint is heated between electrode plates that form a capacitor while the material is under a certain compressive force. The heat develops inside the material, the electrodes remain cold, and the maximum temperature develops in the middle of the welded joint of the materials, when the layers of the materials to be joined have the same thickness. The mentioned technique is mainly used for welding foils and flat textile materials. The welding frequency of 27.120 MHz ± 0,6% is stated in the standard, the elements of welding techniques and welded joints are shown, but other process parameters are not given. Apart from the two parameters mentioned, the standard gives a list of basic welding parameters: temperature, velocity, and pressure force, but the values of the parameters are not given.

Later books and guides appeared mentioning the subject of HF techniques of welding. Dixon and Grewell mention some of the most important parameters for HF welding, namely welding time (2-5 s), material thickness (0.03-1.27 mm) and chemical composition of thermoplastic materials [4]. They mention five main activities of welding (positioning of the sample, loading pressure of the electrode, influence of the high frequency field, cooling phase, disposal procedure) and for each activity a time interval is given, of which the time of HF field influence (1-5 s) is the most important. They also give the mathematical derivation for the power which is dissipated in the dielectric and the power needed to weld a centimetre square of the welded joint. It also gives the main properties of the materials that can be welded using the HF technique, such as high dielectric constant, dielectric losses, and resistance to electrical arcing, a list of materials with their weldability grades, and the most common defects that can occur in HF welding. Wu [5] described the technique of microwave welding, which is rarely available due to its high frequencies. Park and Grewell prepared a detailed guide for the selection of HF welding techniques [6]. Pokharel and Karki, in their book Electric engineering materials [7], describe in great detail the dielectric properties of materials that are particularly important for HF welding. In the book Handbook of Plastics Joining, author Troughton [8] describes a group of the most important welding parameters (output power of the machine, time of heating and cooling, thickness of the material, pressure and temperature of the upper electrode) and some basic properties but does not mention the values of the parameters. In his book Joining textiles – Principles and applications, author Jones [9] lists good and bad properties of HF welding and gives a table of suitable materials for HF welding divided into five categories. He describes and gives numerical values for welding time, specific pressure of the electrode during welding, voltage of the electrode, frequency of the generator and temperature of the electrode. Thus, he gives the area of the welded joint that can be achieved with a power of 1 kW. In the same book, the author Pourmohammadi [10] has dealt with problems of welding of non-woven flat products with special reference to the HF welding technology, but he mentions only the frequency (27.12 MHz) and the thickness of the materials (< 1.5 mm).

In a publication, Shah [11] investigated the possibilities of using the HF technique for welding samples used in medicine at welding frequency of 27.12 MHz and gives a table of materials indicating the values of dielectric losses and the degrees of suitability of the welding materials, divided into four classes. Bajsić et al. [12] have presented the changes of the loss angles depending on the temperature change, which is very significant for the technique of HF welding when the temperatures increase from room temperature to a dozen °C. Pierlot [13] gives the basic characteristics of the HF welding and of the processing parameters mentions only that with 1 kW HF power 10 to 30 square centimetres of material area can be joined. Later, Wu et al. [14] extended the research on the behaviour of the dependence of the materials on the electrical permittivity and the loss angle with respect to the frequency. Ingle and Deshmukh [15] published table of thermoplastic materials, giving the abbreviations of the names, the welding temperatures, and the specific density of the materials. Hollande et al. [16] performed tests on the behaviour of dielectric properties when welding thermoplastic polyurethane at a frequency of 27.12 MHz. They mention formulas for calculating the complex permittivity and the cooling time, as well as their setup for determining the breaking forces of welded joints using the so-called “T” test of tearing. As of other process parameters they state that the voltage developed on electrode was 8 kV and work pressure of 5 bar. In the guide 3M [17], there is a mention of HF welding of reflective tapes on clothes at frequency of 27.12 MHz, thickness of material 0.3 mm and area of welding from 10 to 20 square centimetres at power of 1 kW, welding time from 1 to 6 s and method of visual evaluation of welded joints and ways of testing the strength with depiction of the facts that can affect delamination of the welded joints, damage on the welded joints when too much heat energy is injected into the welded joint or if electrical arcing happens. Yousefpour et al. [18] describe the basic methods of so-called dielectric welding and mention the importance of dielectric loss factor and the HF generator power, material thickness, welding area, material properties, welding time and pressure during welding. Grewell et al. [19] only give values for material thickness from 0.03 to 1.27 mm, a frequency of 27.12 MHz, and the required relative dielectric constant of the material, which must be higher than 2. Other process parameters they specify are voltage, welding time, material properties, pressure force, and vertical displacement of the electrode. Mitelea et al. [20] have worked on optimizing parameters of welding in a way where they changed in three groups anode current from 2.5 to 5 mA, welding time from 1 to 3 s, welding pressure and cooling from 5 bar and cooling time from 2 s. Authors evaluated the achieved optimal process parameters through rise in strength of the welded joints. Podržaj et al. [21] studied changes in anode current and electrode displacement during welding as a function of welding time. They also gave a wide range of process parameters and variables needed to calculate the required power, as well as mathematical derivations to calculate the required welding power and voltage. Patil et al. [22] performed an interesting work on the effect of frequency changes on the dielectric constant in the range of 1 to 500 MHz used in radio frequency and microwave heating of nanotubes in thermoplastic polymer materials. Amanat et al. [23] studied the air and water permeability of the welded joints fabricated using the HF electrical field in area of making medical equipment hermetically enclosed and they stated properties of a large number of high-technology welding techniques. The paper focuses on the type of materials to be welded and the required strength of the welded joints for hermetic enclosure. Sano et al. [24] mention the use of the HF welding technology in the aircraft and automotive industries and their uniqueness is using frequencies of 40 MHz to create welds, instead of using usual frequencies. They did test of materials dielectric constant in range of 300 MHz which are generally constants but the dielectric loss angle decreases with the applied frequency. Yang et al. [25] used the HF welding at a frequency of 27.12 MHz and studied the welding temperature, welding time, welding pressure, and anode current and voltage, and tested the welding strength using the “T” method. This is the only paper in which the authors point out the problematic of the effects of the electromagnetic field on conventional and electronic measurement equipment during the welding process. Čebular et al. [26] determine the quality of the welded joint when welding PVC films. They use the material thickness, five groups of the used HF power and the anode current, and then use the “T” test method to test the strength of the welded joints. They concluded that the possible classification of the quality of the HF welding technology is by measuring the thickness of the welded joint. Kaappa et al. [27] showed a new application of the HF welding technique in the integration of electronic components into textile flat products. They use the type of material, the specific pressure of the welding electrodes, the welding time and cooling, and the percentage of the HF generator power. In the results of the paper, the effect of the HF field on the quality of integrated electronic components in textiles is tested. On the project HRZZ IP-2018-01-6363 characteristics of all HF welding techniques are studied and process parameters used are anode current and voltage, pressure, HF generator power, area of electrode, type and thickness of material [28].

1.1. Process parameters of the HF welding and their interdependence

High frequency welding of polymer materials is performed using a high frequency alternating electromagnetic field in which the material to be welded is located and in which heat is generated under the effect of the field. The material to be welded is positioned between the upper movable electrode and the lower plate, which represents the immovable electrode. The electrodes are made of metal (aluminium or brass) and generally represent a capacitor having an upper plate (upper movable electrode), a lower plate (immovable electrode, work table) and a dielectric between the surfaces (material to be welded). Figure 1 (a) shows the circuit of the capacitor with the upper plate of area A (upper movable electrode), the material to be welded with relative dielectric constant ε_r, and the lower plate (lower electrode or work table WT). On the plates of such formed capacitor a high frequency generator of alternating current G is connected, Figure 1 (a), with a frequency f of usually 27.12 MHz. The voltage u_g of the generator is relatively high, usually about 800 to 2,000 V, at which a current i_g flows through the capacitor, reaching values between 0.2 and 0.6 A in practise. The power of the HF generator, which is used in clothing technology, reaches values up to 800 W when used for shorter welded joints on thinner materials (e.g., raincoats), and can be increased to values of 4 kW for longer and durable welded joints on thicker materials (tarpaulins, sunshades).

Any real capacitor can be represented as an equivalent or substitute scheme circuit consisting of a parallel connection of the resistor R through which the current i_R flows and an ideal capacitor through which the current ic flows, Figure 1 (b).

From the technological point of view, it is interesting and significant that the electric power converted into heat is manifested as power dissipation at a resistor R, Figure 1 (b), during the time of influence of the high frequency alternating field, and its value is the same:

P = u_{G} \cdot i_{R}

(1)

where:

P – electric power [W]

u_G – generator voltage [V]

i_R – current which flows through the resistor [A]

Using the phase diagram, the current flowing through the resistor doing the work can be calculated using the equation:

i_{R} = i_{G} \cdot \cos θ

(2)

where:

i_R – current which flows through the resistor [Ω]

i_G – current which flows through the generator [A]

θ – displacement phase angle [rad]

so, the power of alternating current is calculated from the equation:

P = {u_{G} \cdot i}_{G} \cdot \cos θ

(3)

From the Figure 1 (c) it can also be seen that:

i_{C} = i_{G} \cdot \sin θ \to i_{G} = \frac{i_{C}}{\sin θ}

(4)

Inserting the expression (4) into expression (3) is gives:

P = u_{G} \cdot \frac{i_{C}}{\sin θ} \cdot \cos θ \to P = {u_{G} \cdot i}_{C} \cdot cotg θ

(5)

From the Figure 1 (c) it can also be seen that:

θ = 90 ° - δ

(6)

Inserting expression (6) into expression (5) gives:

P = {u_{G} \cdot i}_{C} \cdot cotg (90 ° - δ)

(7)

By knowing that:

cotg (90 ° - δ) = tg δ

expression (7) can be written as:

P = {u_{G} \cdot i}_{C} \cdot tg δ

(8)

Spurious resistance of the capacitor X_c is equal to:

X_{c} = \frac{1}{ω \cdot C} = \frac{1}{2 \cdot π \cdot f \cdot C}

(9)

where:

X_C - spurious resistance of the capacitor [Ω]

ω – angular frequency [Hz]

C- capacity [F]

f – frequency [Hz]

Determining the current flowing through the capacitor, can be written as:

i_{C} = \frac{u_{G}}{X_{c}} = \frac{u_{G}}{\frac{1}{2 \cdot π \cdot f \cdot C}} = u_{G} \cdot 2 \cdot π \cdot f \cdot C

(10)

Inserting the expression (10) into expression (8) gives:

P = u_{G} \cdot 2 \cdot π \cdot f \cdot C \cdot u_{G} \cdot tg δ = u_{G}^{2} \cdot 2 \cdot π \cdot f \cdot C \cdot tg δ

(11)

During the high frequency welding the upper electrode of area A which welds the polymer material of thickness d and relative dielectric constant ε_r together with the lower electrode form the capacitor with capacitance:

C = ε_{0} \cdot ε_{r} \cdot \frac{A}{d}

(12)

so, by inserting expression (12) into the expression (11), it is obtained the expression for the calculation of the generator power P from its voltage, i.e., the voltage of the upper electrode:

P = u_{G}^{2} \cdot 2 \cdot π \cdot f \cdot ε_{0} \cdot ε_{r} \cdot \frac{A}{d} \cdot tg δ

(13)

In practise, it is better to use an expression based on generator current rather than generator voltage, since this can be measured and adjusted. Thus, if the generator voltage is expressed as:

u_{G} = i_{G} \cdot X_{c} = \frac{i_{G}}{2 \cdot π \cdot f \cdot C}

(14)

inserting the expression (14) into expression (13) gives:

P = {(\frac{i_{G}}{2 \cdot π \cdot f \cdot C})}^{2} \cdot 2 \cdot π \cdot f \cdot ε_{0} \cdot ε_{r} \cdot \frac{A}{d} \cdot tg δ

P = \frac{i_{G}^{2} \cdot tg δ}{2 \cdot π \cdot f \cdot C}

(15)

Furthermore, inserting expression (12) into expression (15), gives the expression for calculating the generator power P via the anode current of the generator, i.e., the current of the upper electrode:

P = \frac{i_{G}^{2} \cdot d \cdot tg δ}{2 \cdot π \cdot f \cdot ε_{0} \cdot ε_{r} \cdot A}

(16)

To achieve heating of the weld from room temperature T₁ to the melting temperature of the polymer material T₂, it is necessary to supply specific heat:

Q_{H} = c \cdot m \cdot (T_{2} - T_{1})

(17)

where:

Q_H - specific heat to warm up the material from room temperature to the melting temperature [J],

c - specific warm up heat of the polymer [J/kg°C]

m - mass of the material to be welded under the upper electrode [kg]

T₂ – melting temperature of the polymer material [°C]

T₁ – room temperature [°C]

The mass of the material to be welded can be expressed as follows:

m = ρ \cdot V

(18)

where:

m – mass of the material to be welded [kg]

ρ – specific density of the material [kg/m³]

V – volume of the material [m³]

As the volume of the welded joint is equal to:

V = A \cdot d

(19)

where:

V – volume of the welded joint [m³]

A – area of the material [m²]

d – thickness of the material [m]

by inserting the expression (19) into expression (18) the mass can be determined with expression:

m = ρ \cdot A \cdot d

(20)

Furthermore, inserting expression (20) into expression (17) gives:

Q_{H} = c \cdot ρ \cdot A \cdot d \cdot (T_{2} - T_{1})

(21)

In the same way, the expression for latent heat or heat of melting must be defined for the polymer material to be welded:

Q_{L} = m \cdot L

(22)

where:

Q_L – melting heat of the material [J]

m – mass of the material to be welded [kg]

L – specific latent heat to melt the material [J/kg]

Inserting expression (20) into expression (22) gives:

Q_{L} = ρ \cdot A \cdot d \cdot L

(23)

The total heat Q_T required to weld the polymer material is expressed as:

Q_{T} = Q_{H} + Q_{L}

(24)

Inserting expression (21) and expression (23) into expression (24) gives:

Q_{T} = c \cdot ρ \cdot A \cdot d \cdot (T_{2} - T_{1}) + ρ \cdot A \cdot d \cdot L

(25)

that is:

Q_{T} = ρ \cdot A \cdot d \cdot [c \cdot (T_{2} - T_{1}) + L]

(26)

As Q_T = P∙t, where t is time of the HF energy influence on the welded joint and P is the power of that field, it follows:

t = \frac{Q_{T}}{P}

(27)

Inserting expression (26) and (13) into the expression (27), we get an expression for the necessary time of the HF energy influence on the welded joint, expressed as the work of the HF generator.

Total heat Q_T, required for welding polymeric materials, can be calculated with expression (25), and the power of the influence of the high frequency energy on the welded joint with expression (14). Thus, inserting the expression (13) and (26) into (27), it is obtained the expression for the required time of the high- frequency energy influence on the welded joint, expressed by the voltage of the high- frequency generator:

t = \frac{ρ \cdot A \cdot d \cdot [c \cdot (T_{2} - T_{1}) + L]}{u_{G}^{2} \cdot 2 \cdot π \cdot f \cdot ε_{0} \cdot ε_{r} \cdot \frac{A}{d} \cdot tg δ}

t = \frac{ρ \cdot d^{2} \cdot [c \cdot (T_{2} - T_{1}) + L]}{u_{G}^{2} \cdot 2 \cdot π \cdot f \cdot ε_{0} \cdot ε_{r} \cdot tg δ}

(28)

In the same manner inserting expression (26) and (16) into (27), it is obtained the expression for the required time of the influence of the high frequency power on the polymer joint expressed by the anode current:

t = \frac{ρ \cdot d \cdot [c \cdot (T_{2} - T_{1}) + L]}{\frac{i_{G}^{2} \cdot tg δ \cdot d}{2 \cdot π \cdot f \cdot ε_{0} \cdot ε_{r} \cdot A}}

t = \frac{ρ \cdot d \cdot [c \cdot (T_{2} - T_{1}) + L] \cdot 2 \cdot π \cdot f \cdot ε_{0} \cdot ε_{r} \cdot A}{i_{G}^{2} \cdot tg δ \cdot d}

t = \frac{ρ \cdot [c \cdot (T_{2} - T_{1}) + L] \cdot 2 \cdot π \cdot f \cdot ε_{0} \cdot ε_{r} \cdot A}{i_{G}^{2} \cdot tg δ}

(29)

Expression (29) can be used to calculate the required time of exposure of the high frequency generator, if the welding current is specified, which is measured with an amperemeter in the cathode circuit, since it is considered technically easier to achieve and relatively harmful (in contrast to the anode voltages, which can reach voltages up to several kV). In the same way, the most important technological parameter, the time t, is determined, which is required for the influence of the high frequency energy on the welded joint, when all known relevant parameters for welding polymer materials are known:

ρ - specific density of the polymer material to be welded [kg/m³],

d – thickness of the polymer material to be welded, [m],

Q_H - specific heat to warm the material from room temperature to the melting temperature [J],

c - heat of the polymer material to be welded [J/kgm],

T₂ – softening temperature of the polymer material to be welded [°C],

T₁ – initial temperature of the polymer material to be welded [°C],

Q_L – heat to melt the polymer material [J],

L – specific latent heat of the polymer material to be welded [J/kg],

Q_T - total necessary heat to weld the polymer materials [J],

f – frequency of the HF generator [Hz],

u_G – generator voltage [V],

i_G – generator anode current [A],

P – generator power [W],

ε_r – relative dielectric constant of the polymer material to be welded,

A – area of the upper electrode [m²],

tgδ – dielectric loss angle of the polymer material to be welded and

t – welding time of the HF joint [s]

All the above parameters are known or can be measured before the technological process of welding the polymer materials, and the required welding time can be specified in advance using expression (29). After that, by fine-tuning only two parameters, the welding time t, and the anode current i_g, the welded joint with the desired properties can be set (visual appearance and strength of the weld).

Considering the previously listed key parameters of high frequency welding used in the mathematical derivation, it can be concluded that these are also most of the parameters on which the HF technique of welding depends. In addition to the above parameters, we can also mention the area and shape of the electrode, the pressure force of the upper electrode, the maximum power of the HF generator and the time required for cooling the sample after welding.

2. Materials and Methods

2.1. Investigation of process parameters of the HF welding

The investigation of the process parameters of the HF welding in the Laboratory for process parameters was carried out on a machine equipped with a linear pneumatic actuator for lowering the upper electrode and regulating the pressure force, Figure 2. The figure shows the added instruments: oscilloscope for measuring the voltage waveforms at the upper electrode, electronic voltmeter for measuring effective values, and a digital frequency counter. All three of the above instruments are fed attenuated voltages through a high voltage probe.

On the right side below the oscilloscope is a small screen for data display with PLC control of the machine. The original machine with integrated PLC circuit was upgraded according to the idea of the research team with an industrial PC for measuring and storing data and the application package for displaying the changes in process parameters [29].

Figure 3 shows the screen of the upgraded machine. On the left side of the screen, the icons of the main control circuits are displayed in a vertical column, and next to them are the currently stored values and elements for data entry, which can be changed by pressing the control buttons on the monitor or by using a PC mouse and keyboard.

The first in the column is the pressure force of the upper electrode, which can be used to adjust the pressure of the compressed air from 0 to 750 N. Below it is the value of the capacitor in the range from 0 to 100%. This capacitor was designed as a coupling capacitor between the titrated circuit of the oscillator in the HF generator made of anode triode, and upper electrode. It has two functions: it protects the user of the machine from electric shock when the electrode is touched during positioning of the sample, and it transfers the energy of the HF generator to the sample when its values are changed. The third symbol represents the time setting of three parameters: the initial pause before activating the HF generator, the welding time when the HF generator is active, and the time of cooling after welding, when the heat is transferred from the welded joint to the cooler electrode, which is a way to cool the sample so that the chains in the macromolecular structure of the polymer are reformed. The last symbol in the column is a window for displaying the set power of the generator (0 – off, 1 – power of 1kW, 2 – power of 2kW and 3 – power of 4kW).

In the upper part of the screen there is a segment for selecting the type of machine control (manual, current, automatic and time) and to the right of it there is a copy of the PLC screen with the parameters for the position of the upper electrode, the controls for archiving the diagrams of the interdependence of the parameters and the currently loaded control segments from the memory for the welding recipes.

The largest part of the screen is used to display the diagrams of changes in the main process parameters of welding in real time during the process. The pressure force of the upper electrode, the value of the capacitor and the value of the anode current are graphically recorded. Besides drawing the diagrams, the measured values are stored in a matrix that can be read in numerical format and transferred to a program for statistical processing.

A measuring device and a method of measurement have been developed to determine the breaking force using a tensile gauge and the application of the “T”test.

3. Results and Discussion

Numerous studies of the functional variations of the process parameters were carried out with the shown machine, such as the influence of the percentages of the coupling capacitor, the anode current and the welding time on the values of the breaking forces of welded joints made with the HF welding technique.

Figure 4 shows the diagram of the dependence of the welded joint strength on the capacity of the couplingt capacitor (F-C) of the machine for the HF welding for welding times of 3.5 and 7 s. It can be seen that the values of the breaking forces for low values of the coupling capacitor at a value of 55% are relatively low (10 to 17 N). This can be attributed to an insufficient amount of the HF that could not be transferred to the upper electrode, i.e., to the sample.

With the increase of the capacitor value, the breaking force of the welded joints increases, i.e., the strength, so the curve of interdependence of breaking forces and values of the coupling capacitor shows a continuous increase, as more and more HF energy is transferred into the welded joint. For values of the capacity of the coupling capacitor higher than 80%, the energy injected into the welded joint is too big so the sample overheats and melts, its thickness decreases due to the greater amount of extruded edges, and an electric arc occurs. The reduced thickness of the welded material cannot withstand the strong electric field, so an electric arc occurs. The material damage shows up as a carbonised hole at the location of the arc and the machine’s safety system shuts down the active working mechanisms, after which the initial procedure of activation must be performed.

In conducting the experiments, it was found that the samples behaved ambivalently during the testing of breaking forces. When testing samples where less energy was injected into the welded joint, it was observed that the layers in the welded joint are separated by gradual peeling. These welded joints are usually welded joints with reduced energy input, where the extruded edges of the welded joint are less visible and the welded joint has excellent visual properties. On the other hand, if more energy is supplied by increasing the value of the coupling capacitor, or if it remains the same and the welding time is increased, a more pronounced extruded edge will appear. Extrusion of the material at the edges significantly deteriorates the aesthetic component of the welded joint, the welded joint becomes uneven and harder, and its elastic and bending properties deteriorate [28,30]. Welded joints with pronounced extruded edges are not separated by peeling, but by tearing the material directly on one side of the extruded edge.

In Figure 5, you can see a symbolic representation of the test phases and two characteristic types of the HF welded joints tearing, from the initial visual appearance of the welded joint, after insertion into the tensile gauge grips, before elongation, during elongation and when the welded joint breaks both separate cases, peeling and tearing at the extruded edge.

In the first phase of the test, the sample is not clamped with the tensile gage, so the test force is not applied, and the sample is not changed. In the second phase, the sample is fixed in the grips, the force is still not applied, and the sample is not changed. In the third phase, the test force is applied. During a short period of time, the force gradually increases, the sample elongates, and after a certain time, the layers of the sample separate. While the force increases gradually, a long elongation of the material is observed in some samples, before and during the separation of the layers of the welded joint by peeling. In the fourth phase, a breakage of the sample in the welded segment is observed. There are two ways in which the welded joint separates from the sample. One is peeling and the other is tearing of the material. Peeling means that each layer of the welded joint separates, and tearing means that the material breaks in the area where the extruded edge is located.

This unusual phenomenon of material separation of the HF welded joints (gradual peeling of the entire welded joint and tearing off of the material in the area of the extruded edge) can be directly attributed to the amount of the HF energy injected into the welded joint. As the original mathematical derivation by the authors of this article states, the total energy Q_T injected into the welded joint is expression (24), which is composed of the specific heat to warm the material from room temperature to the melting temperature Q_H and the latent heat of fusion of the material Q_L. In the melting process of the welded joint, HF energy is first used to generate the specific heat and the temperature of the material, which increases rapidly until the melting temperature is reached, after which the energy is converted into latent heat as the temperature of the material does not increase, but the material melts in its volume under the upper electrode.

The described phenomenon can be explained as well as the inflexion point in the diagram of the breaking force of the welded joint against the capacity of the coupling capacitor for values of the abscissa starting from 65% on Figure 4. The phenomenon of the inflexion point for this abscissa value is very specific because the slope of the dependence curve of the welding force on the coupling capacity changes unexpectedly. In the range of the coupling capacitor values from 55 to 65%, the dependence curve rises rapidly at first and then slowly, and one might expect it to continue to rise slowly after the abscissa value of 65%, when it reaches a kind of saturation. However, when visually observing the HF welded joints breaking under different forces, the mentioned characteristic separation of the welded joint was observed by gradual peeling and tearing in the location of the extruded edge. It was confirmed that this phenomenon of gradual separation of the HF welded joints occurs by characteristic peeling in the first part of the curve until the point of inflexion. After this point, extruded edges are formed, an increase in the breaking force is observed and the quality of the welded joints decreases rapidly.

From the diagram of the dependence of the breaking forces of the HF welded joints on the welding time (F-t) for different values of the capacity of the coupling capacitor in Figure 6, the amounts of injected HF energy in the welded joint and the effects of the breaking forces of the welded joint, i.e., the welded joint strength, can be observed.

A particularly interesting diagram is shown in Figure 6 for the lowest value of coupling capacitor capacity of 55% and welding times of 3 to 5 s. This is also the lowest amount of the HF energy injected into the welded joint. It can be observed that during the welding time of the machine, in the time range of 3 and 4 s and when the capacity of the coupling capacitor is 55%, a small amount of energy is injected into the welded joint. In these short times and with a small amount of the HF energy of the generator, the energy was mainly used for heating the welded joint (by specific heat), but the material was not heated to the melting point, there was no melting of the material (phenomenon of latent heat), so the material layers could not mix at the intermolecular level and the welded joint was not realised. Thus, the breaking force is zero. After a welding time of the HF field of 5 s, the amount of energy injected into the weld was sufficient so that the melting temperature was reached, the material layers began to melt and join together, but only to a small extent, resulting in modest values of the breaking forces.

Increasing the values of the coupling capacitor may result in injecting enough energy into the HF welded joint to form joints with higher breaking forces. At values of 60% of the coupling capacitor, breaking forces of 25 N are achieved, at 65% around 30 N and with a particularly fine shape of the formed welded joint. With a continuous increase of the capacitor value from 70 to 80%, a higher amount of the HF energy is injected into the welded joint, which is unnecessarily used for undesirable melting of the material, resulting in poor properties and a poor technological process.

In accordance with the obtained data, it is considered that the amount of HF energy can be achieved by adjusting the value of the coupling capacitor, which means that a higher amount of energy is transferred to the upper electrode when the value of the coupling capacitor increases. Another option is to keep the value of the capacitor constant and increase the welding time. From the technical point of view, it is better to keep the welding time as short as possible, because with shorter times the load on the HF generator and the consumption of electrical energy are lower. From the technological point of view, this method is appropriate because it shortens the time of technological operation and increases production.

With these investigations it is possible to study the interdependence of the HF welded joint breaking forces with the values of the anode current, Figure 7. In the case shown in the diagram, for the time of HF field influence on the sample in the duration of 5 s, the anode currents are in the range from 0.30 to 0.46 A.

The diagram shown also shows that the breaking forces of the welded joints increase with increasing anode current of the generator, in the case shown up to a value of 0.42 A. After that, the strength decreases because too much energy is injected into the welded joint, which can lead to dielectric arcing in the welded joint.

The diagram of the dependence of the breaking forces on the anode current (F-i_g) is more suitable for the scientific study of the process parameters of HF welding, but from the practical point of view it is easier to use the diagram of the dependence of the breaking forces on the capacity of the coupling capacitor (F-C). This is since the value of the coupling capacitor can be changed during the industrial operation of the process parameters by manual adjustment of the spindle and with computer-controlled machines using servomotors.

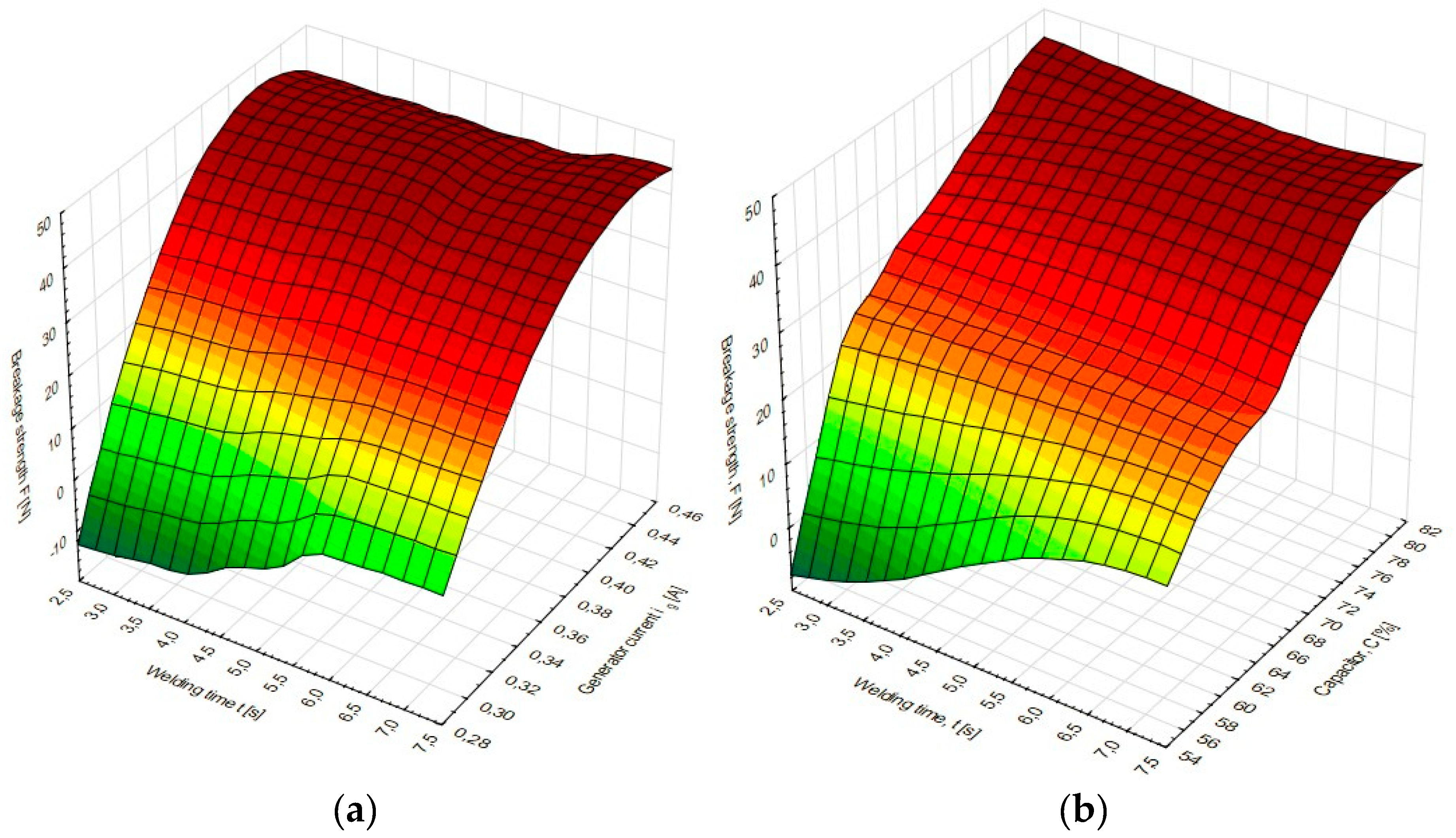
Based on the investigation carried out, in addition to a 2D diagram, it is also possible to draw 3D diagrams on the dependence of the breaking forces of HF welded joints on the welding time and the anode current (F-t-i_g), Figure 8(a), or of the welding time and the capacitor value (F-t-C), Figure 8(b).

In both cases, the optimal path must be found so that the time of the HF field is shorter, and the values of the coupling capacitor or anode current ensure an optimal breaking force and visual shape of the welded joint.

From the extensive literature on the HF welding of polymer materials, it appears that many authors consider three main parameters, namely, anode current, welding time, and upper electrode pressure. However, some authors have also worked with the welding frequency, power, and values of dielectric constants of the material or dielectric loss angle. In our research, the scope of parameters in the mathematical derivation is extended to 17 parameters that have been shown to be crucial for the HF welding processes. Their mathematical relationships are shown, and formulas for calculating the parameters are given, as well as forms of diagrams showing the change in many parameters that have been determined experimentally. From all this we can conclude that the field of study of process parameters of high frequency welding, as one of the high-technology welding methods, is very interesting and exciting, and that many more interesting studies can be expected for this field, which will advance the development of clothing technology.

4. Conclusions

In this paper, the dependence of the breaking forces in the HF welding, i.e., the strength of the welded joints, on the values of the coupling capacitor, the anode current, and the welding time is studied.

Considering the interdependence of the values of coupling capacitor, it was found that due to insufficient energy transfer to the sample, when applying lower percentage values of the capacity of the coupling capacitor at a value of 55% and at welding times of 3.5 and 7 s, the values of the breaking forces of the welded joints are low (10 – 17 N). At higher capacity values of the capacitor, with which a higher amount of HF energy is injected into the welded joint, the breaking force of the welded joint increases, i.e., the strength of the welded joint. However, too high capacity value of the coupling capacitor, which in this paper was of value more than 80%, leads to a reduction in the welded joint thickness and the appearance of extremely extruded edges, since too much energy is injected into the welded joint, and causes electric arcing as well as damage to the material, so that a satisfactory welded joint is not produced.

Observing the dependence of the HF welded joint breaking forces on the values of the anode current for welding times of 5 s and values of the anode current from 0.36 to 0.46 A, it was found that when the anode current is increased to 0.42 A, the breaking force increases, and when the anode current is further increased, an electric arc occurs on the welded joint due to excessive energy injected into the welded joint. It is important to emphasize that from a practical point of view the use of the dependence of the welded joint strength on the capacity of the coupling capacitor is easier to handle, since in production practice the capacity of the coupling capacitor spindle is more quickly set manually or with computer-controlled machines.

HF welded joint strength also depends on the welding time and it is observed in this paper using different values of the capacity of the coupling capacitor, i.e., the amount of HF energy injected into the welded joint. At welding times of 3 and 4 s, with values of the coupling capacitor of 55%, not enough energy is injected into the welded joint, i.e., the material does not manage to heat up to the melting point, so the welded joint does not materialise and its breaking force is zero. The injected amount of energy sufficient for reaching the melting temperature is reached at the time of HF exposure of 5 s, during which the welded joint is formed, but in small amounts and with low values of the breaking forces, which means that no satisfactory welded joint strength is achieved. As the value of the coupling capacitor increases, higher breaking forces are obtained with satisfactory shapes of the welded joint formed. But when the value of the capacitor increases from 70 to 80%, the material melts and the welded joint deteriorates.

When testing the welded joint strength with the “T” test, two types of HF welded joint breakage occur, namely, gradual peeling of the entire welded joint and tearing of the material at the extruded edge, which depends on the capacity of the coupling capacitor. It was found that the separation of the welded joint by peeling results in the small amount of extruded edges and the separation of the welded joint by tearing at the extruded edge results in the increased amount of extruded edges, which are characterised by higher strength, and for this reason process optimization of the welded joint formation is required.

Based on the results shown in this paper it was found that in order to achieve optimum welded joint strength, the amount of HF energy can be regulated by adjusting the values of the coupling capacitor, i.e., by increasing the energy transferred to the sample or by fixing the capacitor value and increasing the welding time. It is necessary to determine the optimum so that the time of action of the HF field is as short as possible and the value of the coupling capacitor or the anode current ensures optimum breaking force and visual shape of the welded joint. From the technological point of view, it is better to shorten the welding time, as this reduces the duration of the technological process and increases productivity.

With further research in the field of high frequency welding, further development of the clothing technology is possible.

Author Contributions

For research articles with several authors, a short paragraph specifying their individual contributions must be provided. The following statements should be used “Conceptualization, D.R., S.F.R., S.F.; methodology, D.R., S.F.R., D.C.V.; software, D.R.; validation, S.F.R., Ž.K.; S.F. formal analysis, D.R., S.F.R., Ž.K.; investigation, D.R., S.F.R.; D.C.V., N.J.; resources, D.R., Ž.K.; data curation, D.R., D.C.V., Ž.K., S.F.R.; N.J.; writing—original draft preparation, D.R., S.F.R.; writing—review and editing, D.R., S.F., S.F.R.; visualization, S.F.R.; N.J.; supervision, D.R.; project administration, S.F.R.; funding acquisition, D.R. All authors have read and agreed to the published version of the manuscript.” Please turn to the CRediT taxonomy for the term explanation. Authorship must be limited to those who have contributed substantially to the work reported.

Funding

This research was funded by the Croatian Science Foundation through the project “Development and thermal properties of intelligent clothing (ThermIC), grant number IP-2018-01-6363.

Conflicts of Interest

The authors declare no conflict of interest.

References

Hartshorn, L. High frequency Heating. Journal of the Royal Society of Arts. 1944, 93, 38–49. [Google Scholar]
Hartshorn, L. Radio-frequency heating. Nature. 1946, 157, 607–610. [Google Scholar] [CrossRef]
DIN 16960 „Welding of Thermoplastics – Principles“, 1974, German National Standard.
Dixon, J. P., Grewell, D. Radio Frequency Welding. In Plastics and Composites Welding Handbook, Grewell, D. A., Benatar, A., Park, J. B., Eds.; Hanser Gardener, 2003, 249-270.
Wu, C. Y. Microwave Welding. In Plastics and Composites Welding Handbook, Grewell, D. A., Benatar, A., Park, J. B., Eds.; Hanser Gardener, 2003, 313-334.
Park, J., Grewell, D. Guidelines for Process Selection. In Plastics and Composites Welding Handbook, Grewell, D. A., Benatar, A., Park, J. B., Eds.; Hanser Gardener, 2003, 335-360.
Pokharel, B. P.; Nava, R. K. Electrical engineering materials, 1st ed; Narosa Publishing House, 2007, 73-94.
Troughton, M. J. Handbook of plastics joining: a practical guide, 2nd ed; William Andrew Inc., United States of America, 2008, 57-63.
Jones, I. Ultrasonic and dielectric welding of textiles. In: Joining Textiles: Principles and Applications, Jones, I., Stylios, G.K., Eds.; Woodhead Publishing, 2013, 374-397.
Pourmohammadi, A. (2013) Nonwoven materials and joining techniques. Joining Textiles: Principles and Applications. 374-397.
Shah, T. Polyurethane thin-film welding for medical device applications. Medical Device and Diagnostic Industry. 2002, 24, 62-69.
Bajsić, E. G.; Šmit, I.; Leskovac, M. Blends of thermoplastic polyurethane and polypropylene. I. Mechanical and phase behavior. Journal of applied polymer science 2007, 104, 3980–3985. [Google Scholar] [CrossRef]
Pierlot, T. (CSIRO Materials Science and Engineering). Welding technology for joining textile media. 2011. (accessed on February 2023).
Wu, C.; Xingyi, H.; Genlin, W. and Xinfeng, W., Ke, Y.; Shengtao, L.; Pingkai, J. Hyperbranched-polymer functionalization of graphene sheets for enhanced mechanical and dielectric properties of polyurethane composites. Journal of Materials Chemistry. 2012, 22, 7010–7019. [Google Scholar] [CrossRef]
Ingle, S.; Deshmukh, M. J. Parametric Study and Development of Different Plastics and It’s Welding Techniques. International Research Journal of Engineering and Technology. 2019, 6, 1792–1795. [Google Scholar]
Hollande, S.; Laurent, J.-L.; Lebey, T. High frequency welding of an industrial thermoplastic polyurethane elastomer-coated fabric. ” Polymer. 1998, 39, 5343–5349. [Google Scholar] [CrossRef]
3M Science. Applied to Life. Available online: https://multimedia.3m.com/mws/media/157027O/high-gloss-film.pdf (accessed on February 2023).
Yousefpour, A.; Hojjati, M.; Immarigeon, J.-P. Fusion bonding/welding of thermoplastic composites. Journal of Thermoplastic composite materials. 2004, 17, 303–341. [Google Scholar] [CrossRef]
Grewell, D.; Benatar, A. Welding of plastics: fundamentals and new developments. International polymer processing. 2007, 22, 43–60. [Google Scholar] [CrossRef]
Mitelea, I.; Varzaru, N.; Bordeasu, I.; Popescu, M. The high frequency welding aptitude of thermoplastic polymers. Materiale Plastice. 2009, 46, 101–107. [Google Scholar]
Podržaj, P.; Čebular, A. The application of LVQ neural network for weld strength evaluation of RF-welded plastic materials. IEEE/ASME Transactions on Mechatronics. 2015, 21, 1063–1071. [Google Scholar] [CrossRef]
Patil, N.; Camacho, A. C.; Mishra, N. K.; Singhla, P.; Sweeney, C. B.; Saed, M. A.; Radovic, M.; Green, M. J. Radio frequency and microwave heating of preceramic polymer nanocomposites with applications in mold-free processing. Advanced Engineering Materials. 2019, 21, 1900276 (1–8). [Google Scholar] [CrossRef]
Amanat, N.; James, N. L.; McKenzie, D. R. Welding methods for joining thermoplastic polymers for the hermetic enclosure of medical devices. Medical engineering & physics. 2010, 32, 690–699. [Google Scholar]
Sano, M.; Oguma, H.; Masahiro, S.; Sato, C. High frequency welding of polypropylene using dielectric ceramic compounds in composite adhesive layers. International Journal of Adhesion and Adhesives, 2013, 47, 57–62. [Google Scholar] [CrossRef]
Yang, Y.; Zeng, P.; Lei, L. High frequency dielectric heating and the strength of flexible polymer seams. Journal of Materials Processing Technology. 2015, 215, 105–113. [Google Scholar] [CrossRef]
Čebular, A.; Stepanova, A.; Šorli, I.; Podržaj, P. Weld quality evaluation in radio-frequency PVC welding process. Informacije MIDEM. 2011, 41, 290–296. [Google Scholar]
Kaappa, E.; Myry, M.; gren, S.; Halme, A.; Vanhala, J. High Frequency Welding: Applications and Opportunities to Integrate Electronics into Textiles. In Proceedings of the 1st World Textile Conference SMARTEX, Kafrelsheikh University, Egypt, 22-24 November, 2011.
Bobovčan Marcelić, M.; Geršak, J.; Rogale, D.; Firšt Rogale, S. Study of the compression properties of welded seams formed using hot wedge, hot air, ultrasonic, and high frequency welding techniques. Textile Research Journal. 2022, 92, 4736–4752. [Google Scholar] [CrossRef]
Rogale, D.; Firšt Rogale, S. Project Results HRZZ IP-2018-01-6363, Development and thermal properties of intelligent clothing (ThermIC). University of Zagreb Faculty of Textile Technology, Croatia, 2023, 1-124.
Bobovčan Marcelić, M. Process parameters of high-tech welding methods and properties of welds on protective and intelligent clothing. Ph.D. Thesis, Faculty of Textile Technology University oh Zagreb, Zagreb, Croatia, 2023. [Google Scholar]

Figure 1. High frequency heating of polymer material: (a) principle of high frequency heating; (b) the equivalent current circuit; (c) phase diagram of the currents of the equivalent circuit.

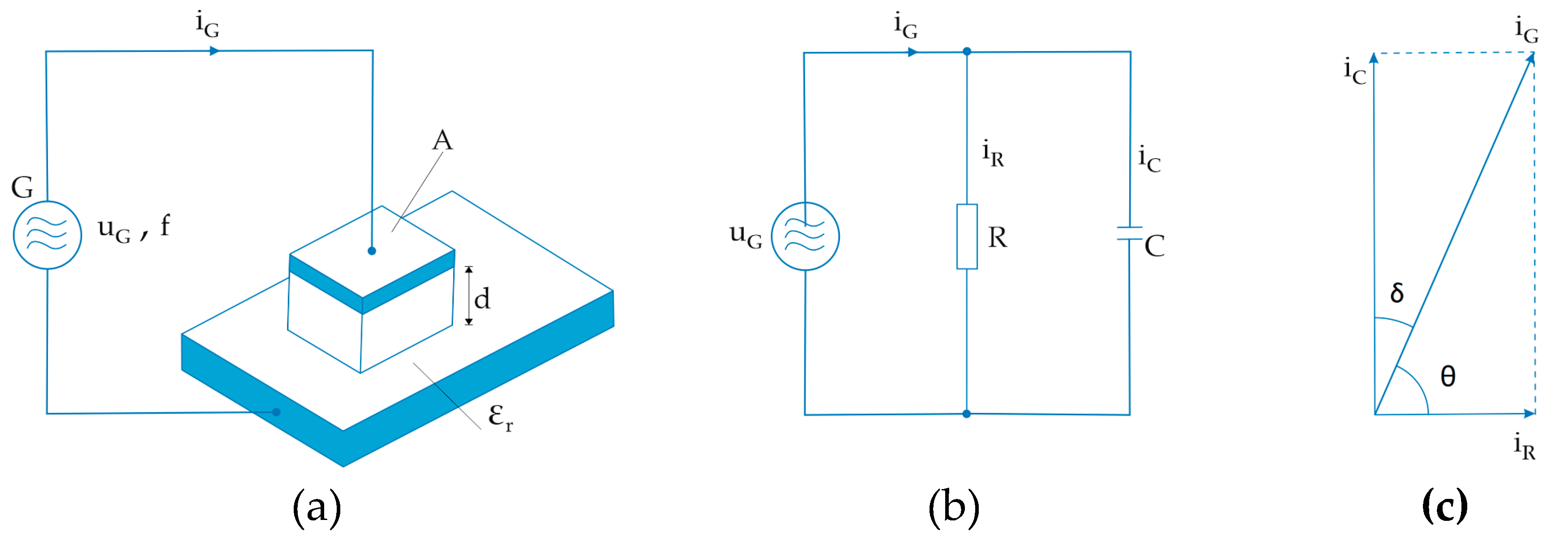
Figure 2. Machine for the HF welding with added measuring instruments.

Figure 3. Screen layout of the monitor attached to the HF machine.

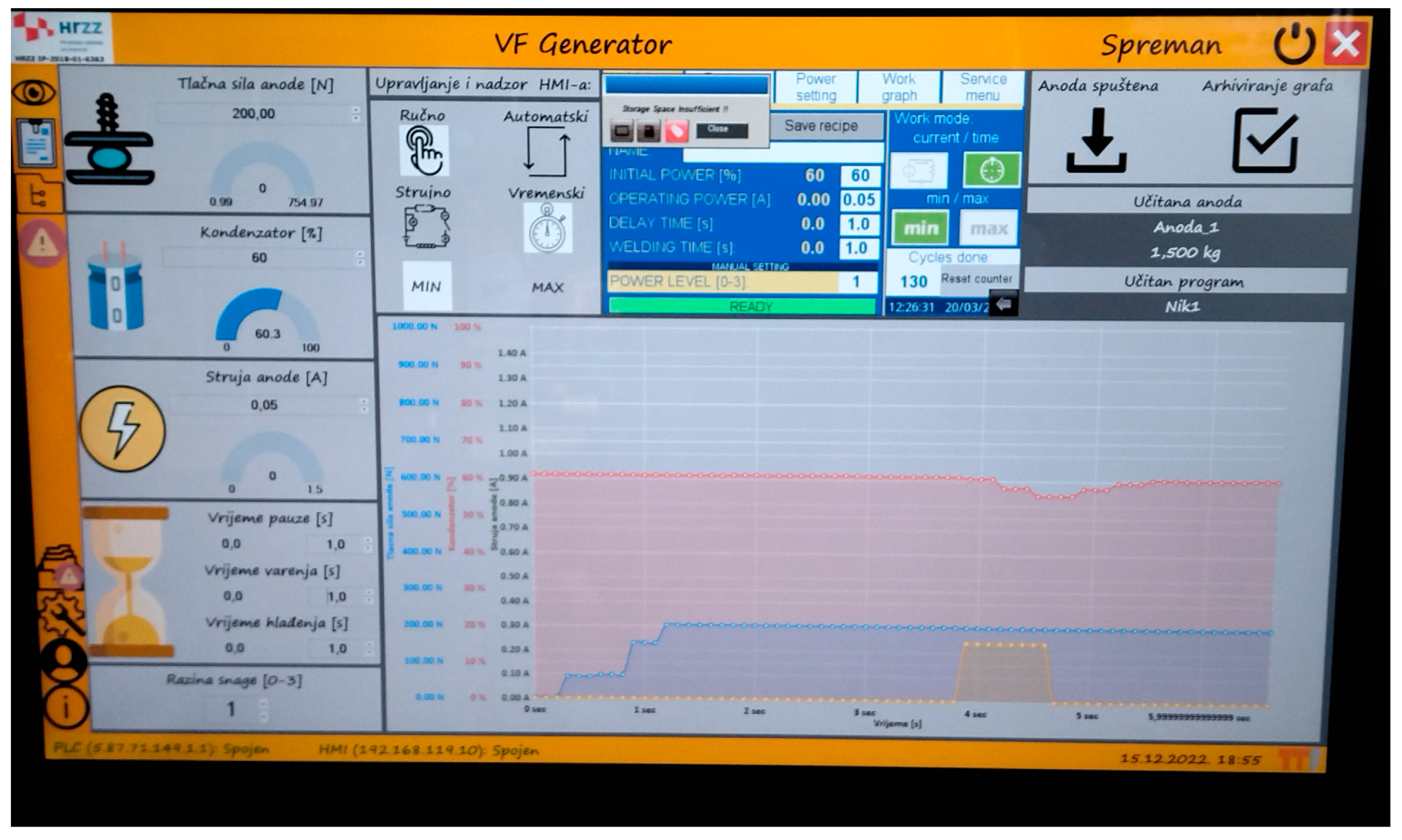
Figure 4. Interdependence of the welded joint strength in relation to capacity of the coupling capacitor.

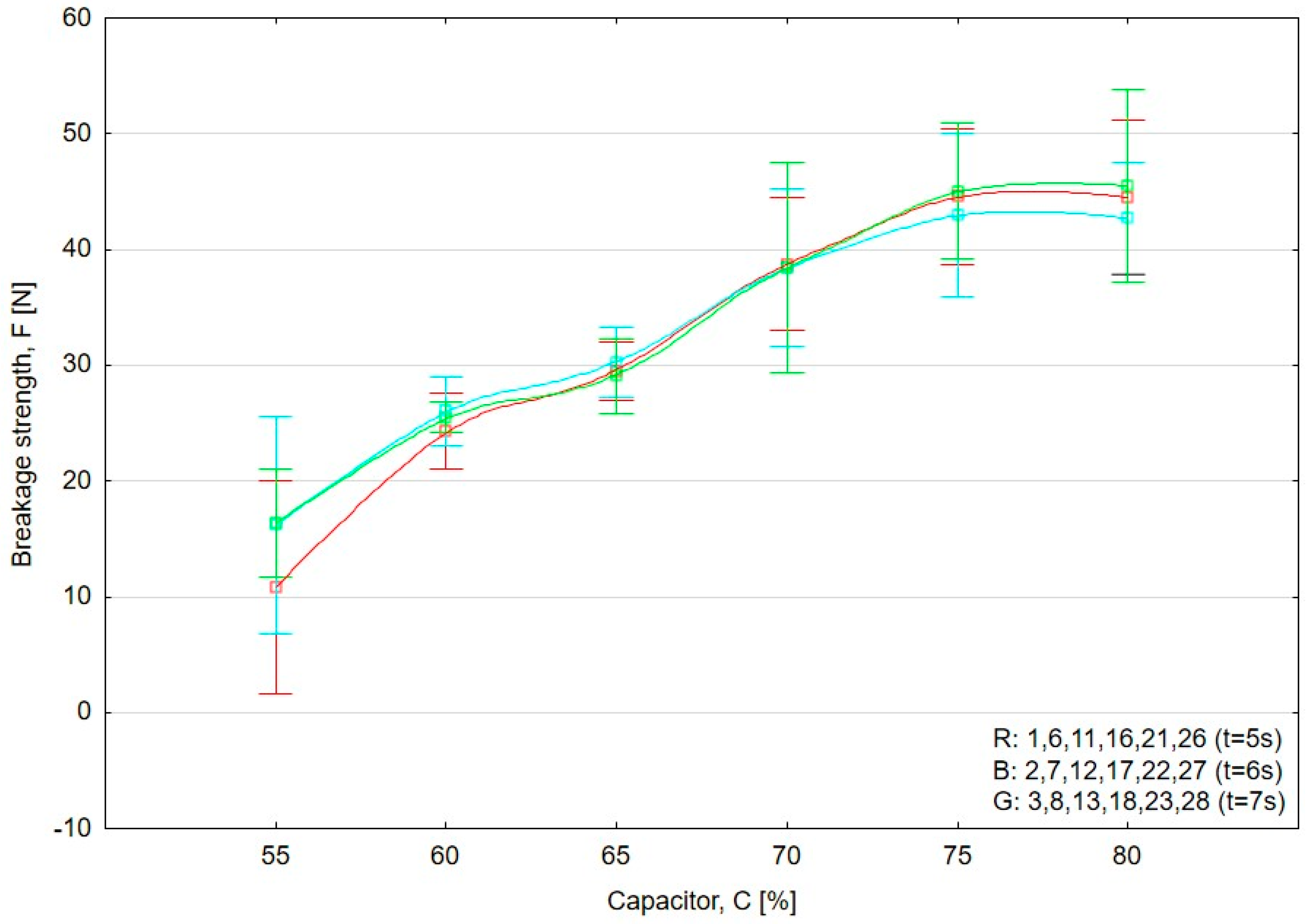
Figure 5. Phases of testing breaking forces of the HF welded joints.

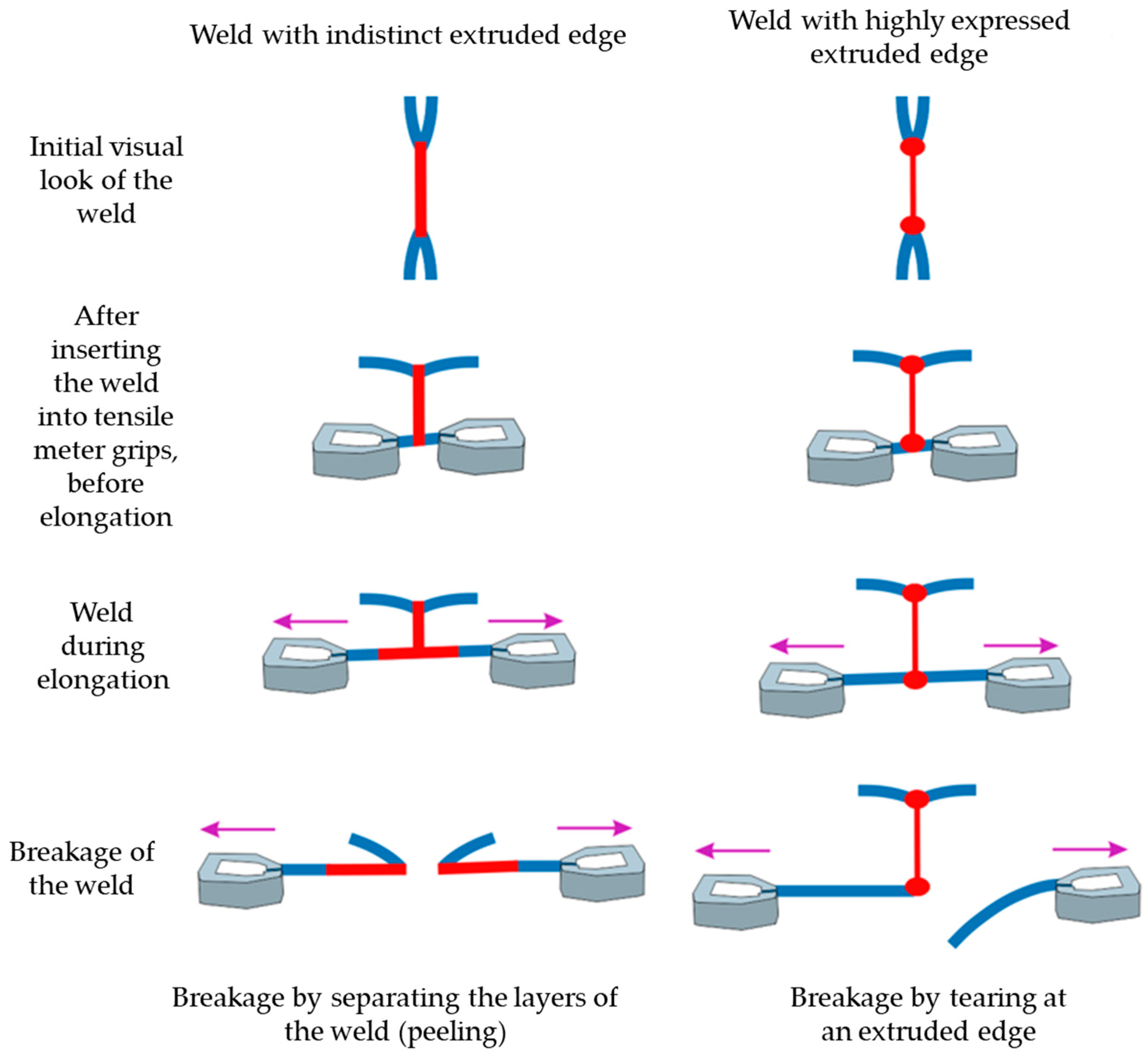
Figure 6. Dependencies of breaking forces of the HF welded joints on the connection time for different values of the coupling capacitor capacity.

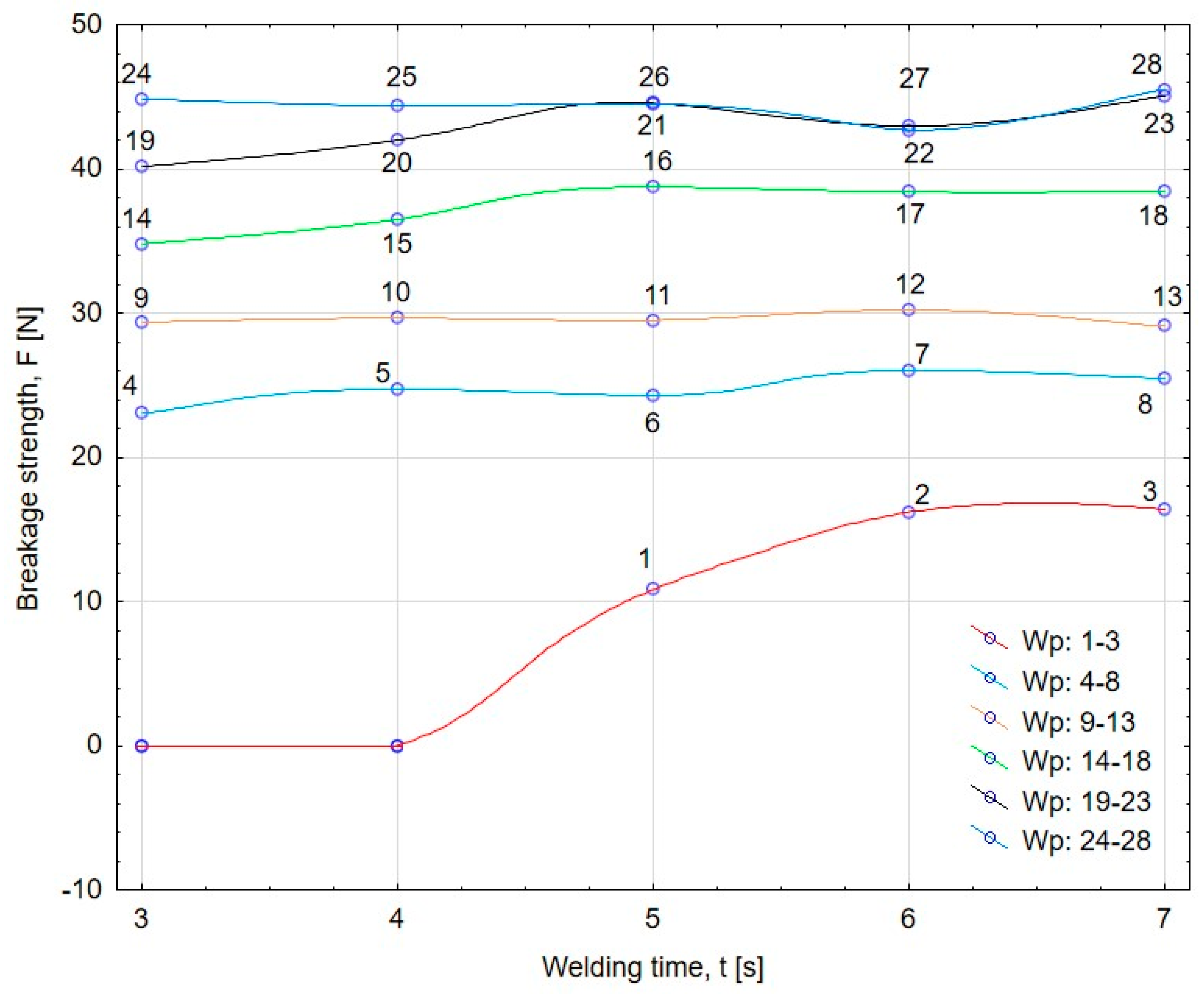
Figure 7. Dependence of breaking forces of the HF welded joints depending on the value of the anode current.

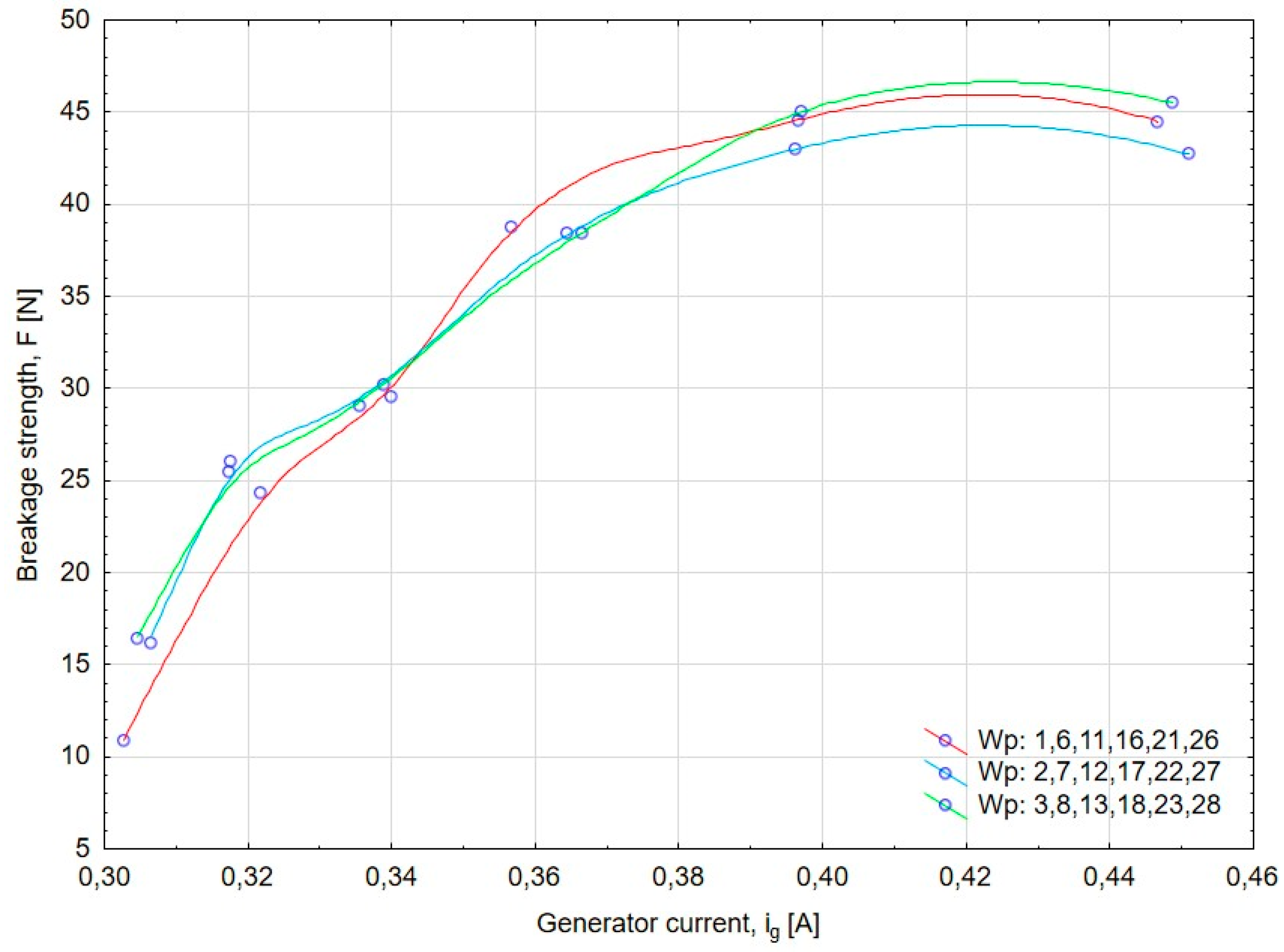
Figure 8. Diagram of: (a) dependence of breaking forces of HF welded joints on welding time and anode current; (b) dependence of breaking forces of HF welded joints on welding time and capacitor value.

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content.

© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).

Copyright: This open access article is published under a Creative Commons CC BY 4.0 license, which permit the free download, distribution, and reuse, provided that the author and preprint are cited in any reuse.

MDPI Initiatives

Important Links

Choose an area of interest and we will send you notifications of new preprints at your preferred frequency.

Disclaimer

Process Parameters of High Frequency Welding

Abstract

1. Introduction

1.1. Process parameters of the HF welding and their interdependence

2. Materials and Methods

2.1. Investigation of process parameters of the HF welding

3. Results and Discussion

4. Conclusions

Author Contributions

Funding

Conflicts of Interest

References

MDPI Initiatives

Important Links

Subscribe