Preprint
Article
Novel Fuzzy Contractions and Applications to Engineering Science
Altmetrics
Downloads
94
Views
24
Comments
0
A peer-reviewed article of this preprint also exists.
This version is not peer-reviewed
Submitted:
30 November 2023
Posted:
30 November 2023
You are already at the latest version
Alerts
Abstract
The article outlines a creative approach by fusing ideas from fuzzy contractions, graph mappings, and Kannan mappings to create a brand-new notion known as "Kannan-graph-fuzzy contraction." This combination of concepts makes it possible to create a unique framework for resolving real-world issues controlled by nonlinear models. It is anticipated that using Kannan-graph-fuzzy contractions will offer a fresh approach to discussing solution existence in situations where these ideas overlap. This strategy might have a significant impact on a lot of real-world applications, proving how practical multidisciplinary techniques are for tackling issues in the real world originating from technology and engineering. The article includes several illustrated examples based on computer simulations, making the results more explicit.
Keywords:
Subject: Computer Science and Mathematics - Mathematics
1. Introduction
Fixed point () theory is a powerful mathematical tool with numerous applications in various domains. It has played a crucial role in creating vital concepts and approaches and is an active field of study and development. It is commonly used in pure and applied mathematics as a bridge between topology and analysis. theory is a burgeoning scientific field with significant applications in various sectors. It is concerned with research demonstrating that, under certain conditions, a self-map on a non-empty set, M, admits one or more s. theorems prove the existence and uniqueness of s. Because of its application in both pure and applied mathematics, the metric theorem has a considerable effect on academia. Banach made an unexpected discovery in 1922 that became known as the Banach contraction principle. One of the most adaptive conclusions in theory and approximation theory is the Banach contraction principle. It changed and generalized in a variety of ways over time. The Banach contraction concept is frequently used in the literature on theory. Numerous authors have generalized this conclusion by employing other contraction mappings in other metric spaces. The classical principle of contraction mapping states if is a complete metric space and such that for all , where, , then ℶ admits a unique . Banach’s [19] hypothesis helps compute mathematics for examining the existence of solutions to nonlinear integral equations, systems of linear equations, and nonlinear differential equations, as well as manifesting algorithms related to convergence. Many variations on Banach’s theorem have been proposed; see [12].
In 1971, Cirić [20], Reich [21], and Rus [16] established a theorem for mappings , which has been around for decades, meets the following requirement:
where , and . If , condition 1.1 reduces to Banach contraction.
where , whereas for condition 1.1 reduces to Kannan’s contraction.
where, . Kannan mappings are essential in mathematics and its applications because they provide a flexible framework for establishing the existence and uniqueness of s in metric spaces. They are an important instrument in the study of nonlinear analysis, numerical techniques, and various scientific and technical domains. Their significance stems from their capacity to determine the convergence of iterative algorithms and solve a wide range of mathematical and practical problems. In fact, Kannan’s fixed-point theorem is a noteworthy finding in mathematics, especially regarding complete metric spaces. The theorem gives circumstances in which it is possible to derive a unique in such spaces without continuous mapping. This is important because continuity of the mapping is a necessary assumption in many traditional fixed-point theorems.
Kannan’s theorem has several applications.
1. Eliminating the Continuity Requirement: Kannan’s theorem is applicable in a wider range of scenarios, encompassing non-continuous maps, in contrast to several previous fixed-point theorems that need continuous mapping. It may, therefore, be used to solve an enormous variety of mathematical and real-world issues.
2. Metric Completeness Characterization: Kannan’s theorem is remarkable since it describes a space’s metric completeness in terms of the presence of a . That is to say, a metric space is complete if and only if there is only one for a certain kind of mapping. This offers a profound relationship between the existence of s and the notion of metric completeness.
3. Valuable and Interesting Mappings: Since it illuminates the characteristics of full metric spaces and their topological structure, studying mappings that meet Kannan’s theorem requirements is important. There are several practical applications for comprehending these mappings and the corresponding s in mathematics and its applications, such as functional analysis and engineering mathematics, see, eg., [??]. In conclusion, Kannan’s fixed-point theorem is a key finding in mathematics that sheds light on the relationship between metric completeness and the presence of s and provides a fresh viewpoint on fixed-point theory in complete metric spaces. It is extensively researched and used in many different mathematical fields, and it has had a long-lasting influence on mathematical study. Therefore, the results established in [20,21], under slightly different forms, are true generalizations of Banach’s contraction principle [19] and Kannan’s [22] theorem.
On the other side, a significant expansion of classical metric spaces, the notion of fuzzy metric spaces (developed by Kramosil and Michalek [5]) for a more adaptable and comprehensive treatment of distances and continuity. There are uses for fuzzy metric spaces in many domains, such as fixed-point theory and topology. The concepts of fuzzy metric spaces and Hausdorff topology on fuzzy metric spaces were proposed by George and Veeramani [8]. It was demonstrated that this topology is metrizable when applied to specific fuzzy metric spaces, offering a more reliable framework for researching topological characteristics. It is observed that related fuzzy metric spaces may be generated from any classical metric space. This connection demonstrates how flexible fuzzy metric spaces represent various notions of distance. theorems in various mathematical spaces are now better understood because of the introduction of graphical structures and graph theory to theory. These advancements have applications in engineering sciences, functional analysis, and optimization. Fixed-point solutions for set-valued graphical contraction mappings were recently provided by Chen et al.[14,15]. This work extends the study of convexity to the context of graphical structures by introducing the idea of a graphical convex metric space. Younis et al. (2022) [33] discussed the graphical structure of extended b-metric spaces. Studying the graphic structure of such generalized graphical metric spaces might provide insights into fixed-point theory and allow for greater flexibility in the definition of distances. Applications for fuzzy graph s are found in many technical disciplines where imprecision and uncertainty are typical. They make it possible to make the best decisions in situations like network design with erratic connections, environmental management with fluctuating parameters like weather and pollution levels, and transportation route optimization in the face of changing traffic circumstances. They also impact production scheduling in unpredictable events, power grid control in the face of variable renewable energy sources, and robotics motion planning in intricate, dynamic situations. They support the development and management of cities in the face of changing demographic and economic forces in urban planning and smart cities. Engineers may use fuzzy graph s as a flexible tool to address real-world issues with ambiguous data and make defensible choices in changing environments.
This article’s primary goal is to highlight and study many engineering applications of the current theory. To do this, the study offers a set of innovative fuzzy contraction mappings and uses them to construct fixed-point outcomes. Notably, in the setting of fuzzy metric spaces, these discoveries are the first of their kind. This study advances the practical use of advanced fixed-point theory in engineering applications and emphasizes its potential to handle complicated real-world challenges in various scientific domains.
This paper has two parts. Using Kannan-type contractions, we first derive and common theorems for single-valued mappings in the domain of fuzzy metric spaces. Conversely, we offer the £-Kannan-contractions as an extension of fuzzy £-contraction to show s. Our results include a generalization of well-known results of Gregori and Sapna [24] and an extension of Jachymski’s notable results [13] in fuzzy metric spaces. Our primary objective is to generate several applications of the defined fuzzy contractions to different nonlinear models originating from engineering science and technology.
2. Preliminary Concepts
To facilitate the reader, we will now review key concepts and properties that are relevant to this study. Throughout the paper, we will write fuzzy metric spaces as spaces, and the sets of natural numbers be denoted by . The set of integers i.e., , and . The symbols denotes the set of non-negative real numbers. Throughout the paper, all sets are assumed to be nonempty.
Definition 2.1.
([26]) The binary function is a continuous ⋄-norm if is topological monoid with unit and satisfies the condition whenever, , , and Examples of ⋄-norm.
- (i)
- Minimum ⋄-norm
- (ii)
- Product ⋄-norm
- (iii)
- Lukasiewicz ⋄-norm .
Definition 2.2.
([8]) The 3-tuple is termed space if ∁ is an arbitrary set, * is a continuous ⋄-norm, and is fuzzy set on satisfies the following conditions: for all , and
- ⇔
- is the left continuous.
For all and
Definition 2.3.
([8]) Let is a space. The sequence is called convergent in ∁⇔, whenever .
For further synthesis and basic concepts like the Cauchy sequence, the convergence of the Cauchy sequence, and completeness in fuzzy setup, we refer to the readers’ [8].
Definition 2.4.
([8]) Let be a metric space. The triplet is called standard fuzzy metric generated by metric d, where,
It’s worth noting that the topologies generated by the standard fuzzy metric and its corresponding measure are similar to one another.
Lemma 2.1.
([8]) Let is a space. Then is continuous function on
Lemma 2.2.
([29]) Let is a space. Then is nondecreasing for all .
Definition 2.5.
([24]) A self-mapping within the context of a space is called ℶ-uniformly continuous if ∀ , ∃ , such that
for all .
Definition 2.6.
([24]) A self-mapping defined on is called fuzzy contractive mapping, if there exists such that
for every .
Proposition 2.1.
([23]) Let is a space. If is fuzzy contractive mapping then ℶ is uniformly continuous.
Let £ be a directed graph with vertices and . In this context, a path in £ is a sequence of vertices , where there are vertices, and it satisfies the conditions: the first vertex is , the last vertex is , and for every j from 1 to , there is a directed edge from to in the graph.
A graph £ is considered linked or connected when a path exists connecting any pair of its vertices. This definition of connectivity applies to directed graphs. When the graph £ is undirected, we refer to it as weakly connected, meaning there is a path connecting every pair of vertices, and the direction of the edges is not considered in determining connectivity. Shukla et al. [28] are to be credited with the following annotations [28]:
- a:
- = In the graph £, there is a directed path with length l that connects and .
- b:
- If there is a path leading from to in £, and , then is on the path.
Unless otherwise stated, we consider all the graphs to be direct.
In a graph £, a path of length from vertex directing to another vertex is represented by the sequence of vertices such that , and , .
Definition 2.7.
([27]) A mapping is called a £-fuzzy contraction in the context of space if the following assertions are contented:
- GF1
- ℶ is edge-preserving i.e., ;
- GF2
Example 2.1.
([28]) Any constant function , that is , where, fixed is a £-fuzzy contraction with arbitrary value of .
Definition 2.8.
([28]) Let is a space, and be a self mapping. We denote the iterate of ℶ on by , and for all with The mapping ℶ is called Picard operator, if ℶ has a unique u, and for all The maping ℶ is called a weak Picard operator, if for all ∃ a (which may depend on ϖ) of ℶ such that for all .
It is worth noting that each Picard operator is also a weak Picard operator. The of a weak Picard operator cannot be truely unique.
Definition 2.9.
([28]) A subset A is called ℶ-invariant if
The next lemma will help with what comes next.
Lemma 2.3.
([27]) Let is a £-fuzzy contraction, then given , and , we have for all .
The component of £ that contains is made up of edges, vertices, and a path that starts at . In this instance, the relation R is defined on by the following rule:
if there is a path in £ from to . In this scenario, , where, the equivalence class of this relation is . Thus, the relationship between is self-evident.
We will now talk about different sorts of mapping continuity. The first is well-known in metric theory and is frequently employed.
Definition 2.10.
A mapping is called orbitally continuous if for all , the sequence of positive integers, implies as
Definition 2.11.
A mapping is called orbitally £-continuous if given , and the sequence ,
Recent findings provide the necessary conditions for mapping to a Picard Operator (PO) when the space has a graph structure. This discovery, first revealed by Jachymski [13], expands on its relationship with the Kelisky-Rivlin theorem about iterates of the Bernstein operators in the space .
Definition 2.12.
([13]) A self map is called a Banach £-contraction (£-contraction), if f preserves edges, i.e.,
and edge weights decrease as follows in £:
The notion of £-Kannan operators is proposed in this study to evaluate the s for Kannan operators in spaces given a graph £. We assume that is a metric space and that £ is a directed graph with the criteria that and that £ has no parallel edges. An instance of is a collection of all s for a mapping ℶ.
The ensuing notion forms the basis for the recommended results throughout the course of the work.
Definition 2.13.
([3]) A mapping in the framework of metric space is called £-Kannan operator if the following assumptions hold true:
- (1)
- ;
- (2)
-
for each , we have:where .
Lemma 2.4.
Let £ is a graph endowing metric space , and is a £-Kannan operator. If the assertion is satisfied by , then we have
for all .
Proof.
Let with . An easy induction shows for all ,
which implies
where, .
A repetition of the above will result in the following
□
3. Common for Fuzzy-Kannan Contractions
In the context of spaces, we generate fixed-point and common fixed solutions for single-valued fuzzy-Kannan-type contractions.
Definition 3.1.
Let is complete space. A self-mapping is called a fuzzy-Kannan-contraction of type-I, if
with , for all .
The primary outcome about common for two single-valued mappings within the context of spaces is as follows.
Theorem 3.1.
Suppose is a complete space. Let ∁ be self-mappings such that;
for for all . Then , and ℶ have at most one common in ∁.
Proof.
Suppose is an arbitrary point, and define the sequence by
Using (5.2), and (3.2) we can write
This implies
where, since .
Similarly
Continuing in this manner, we obtain
Repeating the above procedure, we infer
Hence,
Taking 3.3 into account, we deduce
since , for any there exists some such that;
which yields that is a Cauchy sequence in Since is complete, there exists such that;
For proving v is a of S, Assume Then
We arrive at
Which implies
Noting that , , i.e., In the same way, we suppose , we have
This can be put as follows
Then
Which implies
Noticing that , i.e.,
Let is any of ℶ and in ∁ i.e., . Then
This proves that , and . That is ℶ yields a unique .
The following provides the convergence result for fuzzy-Kannan-contraction of type-I for a single-valued mapping ℶ.
Corollary 3.1.
Suppose be a complete space. Let is a self-mapping such that;
for all and . Then, there is only one in ∁ for the map ℶ.
The fuzzy-Kannan-contraction of type-II within the fuzzy-metric setup for two mappings forms the basis of the common result that follows.
Corollary 3.2.
Suppose is complete space. Let be self-mappings. Suppose that for such that;
for all . Then and ℶ have at most one common in ∁.
Proof.
Assuming that is an arbitrary point, define the sequence as follows:
Using 5.2, and 3.2 we can write
This implies
where, Similarly
The procedure is the same as in Theorem 3.1 to derive the common of the maps and ℶ. □
The convergence result of a single mapping ℶ for fuzzy-Kannan-contraction of type-II is as follows.
Theorem 3.2.
Suppose is a complete space. Let be self-mapping. Suppose that for such that;
for all . Then mapping ℶ has at most one common in ∁.
4. Convergence results for Fuzzy-Graph-Kannan Contractions
Definition 4.1.
Let is a space endowed with graph £, and the operator is called fuzzy-graph-£-Kannan operator, if:
- (1)
- ;
- (2)
- the values are positive (), and for every pair , we have:
Remark 4.1.
Any Kannan contraction is a Kannan -contraction, with is defined by .
Theorem 4.1.
Let is a complete metric space endowed with a directed graph £, and is a £-Kannan contraction. Under the assumption that:
- (1)
- For any sequence in ∁, if and for all , then there is a subsequence of , such that for all .
If £ is ℶ-connected, then ℶ is a .
Specifically, we consider as a -space with a graph indicated by £. In this case, £ represents a directed graph, with its vertex set being ∁ and . We ensure that there are no parallel edges in £. Each edge corresponds to a different element from . The following findings are essential in demonstrating the upcoming theorems.
Definition 4.2.
Let is a space endowed with a graph £, and is a mapping. We say that the graph £ is ℶ-connected if for all vertices of £ with , there exists a path in from ϖ to ς such that and for all . A graph £ is weak ℶ-connected, if is ℶ-connected.
Lemma 4.1.
Let is a space endowed with a graph £, and is a fuzzy-graph-£-Kannan operator such that the graph £ is ℶ-connected. For all the sequence is a Cauchy sequence.
Proof.
For a fixed value , two cases arise:
- () If then by Lemma 2.4, we havefor all , where, . Because we getdemonstrating that the Cauchy sequences have the form .
-
() If . There is a path from to in , where, with all , and for all . As a result of employing the inequality of the fuzzy metric, we obtainDefineandWe infer,We can put the above inequality asBy elementary calculations, we havefor all . Since , we find thatthereby showing that is a Cauchy sequence.
The primary outcome of this section is expressed in the subsequent theorem. □
Theorem 4.2.
Let be a complete space endowed with a graph £, and be a fuzzy-graph-£-Kannan operator. We assume that:
- (i)
- £ is ℶ-connected;
- (ii)
- for any in ∁, if , and for then a subsequence with for . Then ℶ is a PO.
Proof.
By Lemma 4.1, is a Cauchy sequence for all , and from hypothesis, we have is convergent. Let then , and , as .
- (a)
- If , we have for all , thenfor all . Letting we obtain because we attain .
- (b)
- If , there is path in from to such that with for all , and for all . Then for all , and , and by the triangle inequality we get
By Lemma 4.1 and by the given hypothesis, the series is convergent, as does the continuity, which states that the sequence is convergent. Let for all . Let obtain for all that is , hence . Therefore, for all there exists a unique such that;
Now we will prove that . Because the graph £ is ℶ-connected, there is at least such that so for all . But , then there is a subsequence with for all , we have the following
Now, letting , we obtain that is, . Furthermore, if we have, for some , then from above, we must have , so that , and therefore, ℶ is a PO. □
Example 4.1.
Let us consider a set ∁, which is defined as the union of two subsets of the real numbers, : Q and . is a fuzzy metric that is defined as follows: . It can be expressed as follows: , where d denotes the standard metric on ∁. Now, we define a transformation by the following
Let , for all , and if , then and Also,
when . Then and . In this case, we have
If and or , and , then and or and . For , we acquire
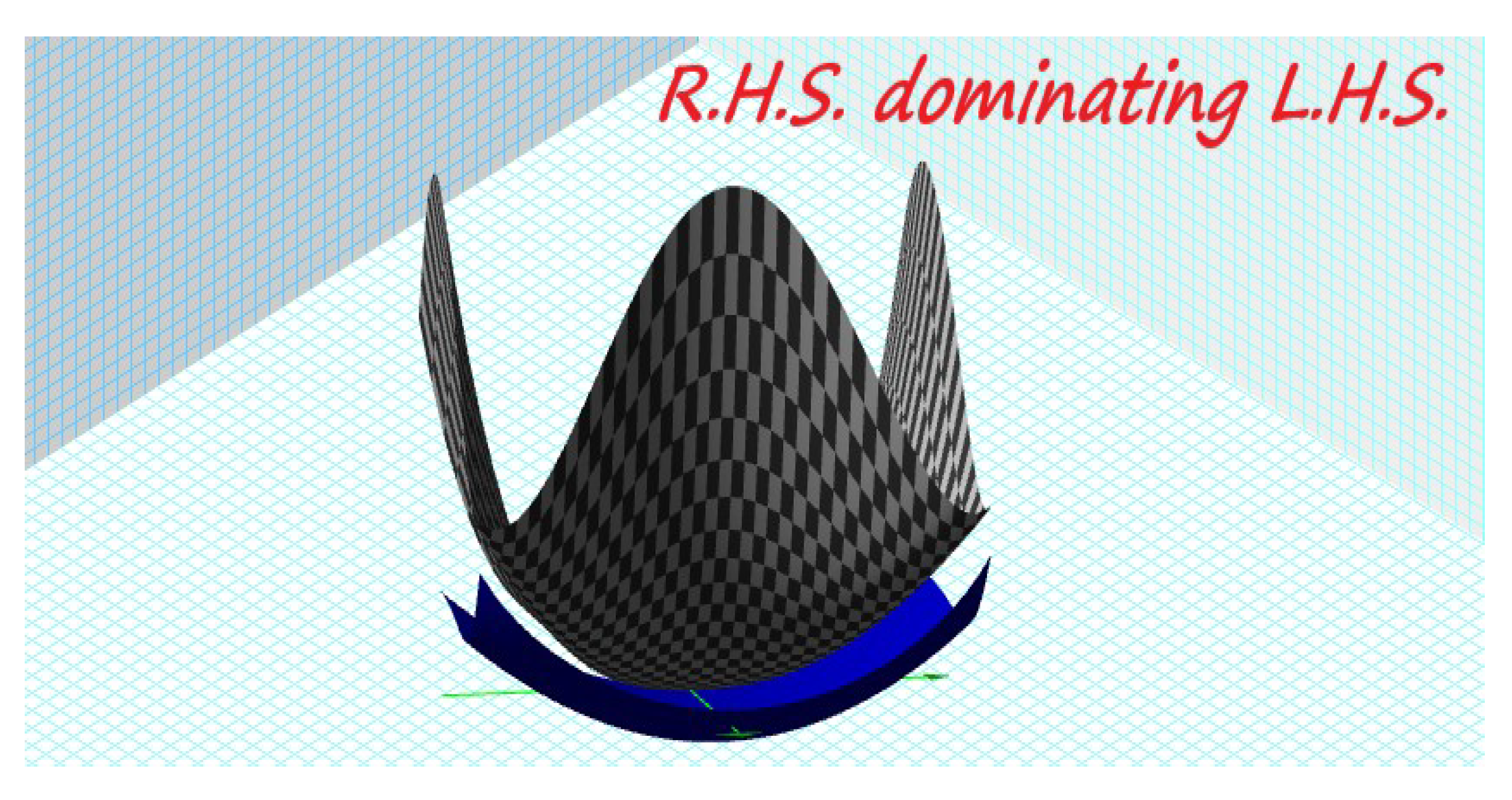
Figure 1.
Validation of inequality fuzzy-graph-Kannan contraction

Note that . As a result, Theorem 4.2 meets all of the specified requirements.
Theorem 4.3.
Let is a complete space, and is a mapping satisfying the following
where,
and
for all , and . Then ℶ has a unique .
Proof.
By taking , and applying Theorem 4.2, we obtain the result. □
5. Fractional-Differential Equations and Fixed-Point Solution
Fractional differential equations are practical tools for modeling and analyzing physical systems with continuous distributions or interactions. In engineering research, they are frequently used to derive correlations between numbers or to describe phenomena in greater detail than differential equations alone can. They offer a framework for comprehending complicated behaviors and interactions in various engineering systems. Differential equations of the implicit kind, particularly s, have a wide range of applications in engineering research. In this section, we prove the existence of a unique solution of the . These kinds of differential equations are frequently employed in engineering. Digital circuit evaluation, mechanical design strain and fluid circulation simulation, structural evaluation, control mechanisms, data processing operations, material scientific research, heat exchange, and magnetic field assessment for radars all depend on them. They also help with inverse problems associated with ophthalmology and acoustically for wave propagation and diffraction studies, as well as in geophysics, non-destructive testing, and medical imaging. These formulas offer an adaptable framework for comprehending and evaluating continuous distributions and interactions across a range of engineering specialties.
s are essential in modeling real-world scientific, engineering, and decision-making events. They provide a nuanced viewpoint by including linearity, nonlinearity, determinism, and stochasticity in mathematical models. Khan et al. [23] investigated the presence, stability, and computational characteristics of s in aquatic illness models. They used fractal-fractional derivatives and a modified Atangana-Baleanu derivative to prove the solution’s existence, demonstrating convergence approaches. Notably, their work demonstrated the power of fractal fractional operators, providing new insights into system dynamics. More synthesis on how s have been the center of research for the fixed point theorists also, we recommend [1].
Let us start by going over the basic terminology of fractional calculus.
The Riemann-Liouville-fractional derivative of order for a function is written as follows:
The expression
If the right side is point-wise defined on the interval , then this is true. In this case, the Euler-gamma function is denoted by , while the integer portion of p is represented as .
Let us now consider the following
where f is a continuous function on , and is the Caputo-fractional-derivative having order , defined by
Denote by , the space of all continuous functions taken on the interval [0,1]. Define a metric d on by
Then is a complete metric-space. The binary operation * is defined by product ⋄-norm
A standard fuzzy metric is given as
Then, easily one can verify that is triangular and is a complete fuzzy metric space.
Theorem 5.1.
Consider the nonlinear (5.1). If the underlying conditions are met:
- (i) For and , the following is true
-
(ii) there exists withThen the (5.1) has necessarily at most one solution in .
Proof.
The equivalent for (5.1) is the following
for all and , where
If for the map defined by
is a , then is a solution of the problem (5.1).
Taking into account the given conditions, for , we infer
This shows that
Put , we can write
The above expression can be written as
for all . This leads to the conclusion that ℶ satisfies the Fuzzy-contraction of the Theorem 3.2. As a result, ℶ concedes a unique fixed-point in , implying that the (5.1) has a unique solution. □
6. An Application to the Equation of Motion
The equations of motion provide the mathematical foundation for comprehending the complex dynamics of particles and systems impacted by forces. These equations are crucial in kinematics, correctly portraying position, velocity, acceleration, and displacement connections. Furthermore, they lead to the development of differential equations, allowing for modeling and predicting system behaviors across time. This mathematical basis lies at the heart of numerical analytic techniques, enabling the creation of algorithms that imitate real-world dynamics.
These equations are common and vital in engineering across many fields. They are essential in mechanical engineering for assessing and optimizing the performance of machinery, engines, and vehicles. Civil engineers use these equations to examine the structural integrity and behavior of buildings, bridges, and infrastructure under various loads and environmental circumstances. They are critical in aeronautical engineering for forecasting trajectories, assuring stability, and building airplanes and spacecraft.
The applications also include control systems, where equations of motion are used to help develop strategies that govern how robots, automation, and manufacturing equipment behave. Electrical engineering also uses these equations to explain how electrical systems and parts, including motors and generators, behave. These formulas are fundamental to comprehending, assessing, and forecasting the behavior of physical systems and are used in a wide range of scientific and technical contexts.
Problem Statement: A particle of mass m is at rest at , . A force starts activity on it in a particular direction such that its velocity immediately jumps from 0 to 1 after . Find the position of the particle at time ⋏. The corresponding motion of the particle is governed by the following second-order differential equation.
for all and , is a continuous function. We are interested in finding the solution of the equation (6.1), which tells us the nature of the particle’s motion, i.e., how the particle moves.
The Green’s function of the problem defined in (6.1) is equivalent to
We now prove the existence of a solution to the second-order differential equation (6.1).
Theorem 6.1.
Suppose for the mappings the following holds:
Then, the second order differential equation (6.1) governing the equation of motion of a particle has a solution in .
Proof.
We note that the solution of (6.1) is equivalent to finding the solution of the following integral equation
Integral equation (6.2) is equivalent to the second order IVP (6.1), which is constructed with the help of Green’s function .
If is a of the map defined by
then is a solution of the problem (6.1).
Taking the same metric defined in Section 5 with , the space of all continuous functions taken on the interval [0,1], and following the same procedure as processed in Theorem 5.1, we can easily see that Hence, concedes a fixed point, endorsing that the second order differential equation (6.1) governing the equation of motion has a solution.
□
7. Conclusion and Future Scope
In the situation of spaces, we developed novel and suitable extensions of Kannan-contraction and discovered their applicability to the solution of integral equations. This is the first attempt to solve £-graph-Kannan contraction in spaces that we are aware of. A few non-trivial examples and an application confirm the solution’s uniqueness. Finally, we have used the manuscript’s significant contents to offer a novel application in which we can derive the existence of a solution to the class of integral equations under very generic conditions.
On the one hand, additional research is needed into how to reformulate the contractivity condition so that theory can be developed in more general abstract metric spaces.
Author Contributions
All authors had equal contributions to this article regarding results, conception, and drafting of the manuscript. The authors read and approved the final manuscript.
Acknowledgments
We are grateful to the reviewers for their helpful suggestions.
Conflicts of Interest
The authors declare that they have no competing interests.
References
- Abdou, A.A.N. Solving a Nonlinear Fractional Differential Equation Using Fixed Point Results in Orthogonal Metric Spaces. Fractal Fract. 2023, 7, 817. [Google Scholar] [CrossRef]
- Bojor, F. Fixed pont theorems for Reich type contractions on metric spaces with a graph. Nonlinear Anal. 2012, 75, 3895–3901. [Google Scholar] [CrossRef]
- Bojor, F. Fixed points of Kannan mappings in metric spaces endowed with a graph. An. S¸t. Univ. Ovidius Constanta 2012, 20, 31–40. [Google Scholar] [CrossRef]
- Zadeh, L.A. Fuzzy sets. Information, and Control. 1965; 338–353. [Google Scholar]
- Kramosil, I.; Michálek, J. Fuzzy metrics and statistical metric spaces. Kybernetika (Prague) 1975, 11, 336–344. [Google Scholar]
- Deng, Z.K. Fuzzy pseudometric spaces. J. Math. Anal. Appl. 1982, 86, 74–95. [Google Scholar] [CrossRef]
- Kaleva, O.; Seikkala, S. On FM-spaces. Fuzzy Sets and Systems 1984, 12, 215–229. [Google Scholar] [CrossRef]
- George, A.; Veeramani, P. On some results in FM-spaces. Fuzzy Sets and Systems 1994, 64, 395–399. [Google Scholar] [CrossRef]
- Erceg, M.A. Metric space in fuzzy set theory. J. Math. Anal. Appl. 1979, 69, 205–230. [Google Scholar] [CrossRef]
- Chugh, R.; Kumar, S. Common fixed point theorem in FM-spaces. Bull. Calcutta Math. Soc. 2002, 94, 17–22. [Google Scholar]
- Grabiec, M. Fixed points in FM-spaces. Fuzzy Sets and Systems 1988, 27, 385–389. [Google Scholar] [CrossRef]
- Tiammee, J. Suantai, Coincidence point theorems for graph-preserving multi-valued mappings. Fixed Point Theory Appl. 2014. [Google Scholar] [CrossRef]
- Jachymski, J. The contraction principle for mappings on a metric space with a graph. Proc. Amer. Math. Soc. 2008, 136, 1359–1373. [Google Scholar] [CrossRef]
- Chen, L.; Yang, N.; Zhao, Y. Fixed point theorems for set-valued G-contractions in a graphical convex metric space with applications. J. Fixed Point Theory Appl. 2020, 22, 88. [Google Scholar] [CrossRef]
- Chen, L.; Yang, N.; Zhao, Y. Fixed point theorems for the Mann’s iteration scheme in convex graphical rectangular b-metric spaces. Optimization 2022, 70, 1359–1373. [Google Scholar] [CrossRef]
- Petrusel, A.; Rus, I.A. Fixed point theorems in ordered L-spaces. Proc. Amer. Math. Soc. 2006, 134, 411–418. [Google Scholar] [CrossRef]
- Schweizer, B.; Sklar, A. Statistical metric spaces. Pacic J. Math. 1960, 10, 133–34. [Google Scholar] [CrossRef]
- Klement, E.P.; Mesiar, R. Triangular Norms, Trends in Logics, Kluwer Academic Publishers, Dordrecht, Boston, London, 2000.
- Banach, S. Sur les operations dans les ensembles abstraits et leur application aux equations integrales. Fund. Math. 1922, 3, 133–181. [Google Scholar] [CrossRef]
- Ćiric, L.B. A generalization of Banach’s contraction principle. Proc. Amer. Math. Soc. 1974, 45, 267–273. [Google Scholar]
- Reich, S. Fixed points of contractive functions. Boll. Unione Mat. Ital. 1972, 26–42. [Google Scholar]
- Kannan, R. Some remarks on fixed points. Bull. Calcutta Math. Soc. 1960, 60, 71–76. [Google Scholar]
- Khan, H.; Alzabut, J.; Tunç, O.; Kaabar, M.K. A fractal–fractional COVID-19 model with a negative impact of quarantine on the diabetic patients. Results Control Optim. 2023, 10, 100199. [Google Scholar] [CrossRef]
- Gregori, V.; Sapena, A. On fixed-point theorems in FM-spaces. Fuzzy Sets Syst. 2002, 125, 245–252. [Google Scholar] [CrossRef]
- Grabiec, M. Fixed points in FM-spaces. Fuzzy Sets and Systems 1988, 27, 385–389. [Google Scholar] [CrossRef]
- Schweizer, B.; Sklar, A. Statistical metric spaces. Pacific J. Math. 1960, 10, 313–334. [Google Scholar] [CrossRef]
- Jabeen, S.; et al. Some Fixed Point Results of Weak-Fuzzy Graphical Contraction Mappings with Application to Integral Equation. Mathematics 2021, 9, 541. [Google Scholar] [CrossRef]
- Shukla, S. Fixed point theorems of £-fuzzy contractions in FM-spaces endowed with a graph. Communications in Mathematics 2014, 22, 1–12. [Google Scholar]
- Choudhur, B.S.; Das, K.; Das, P. Extensions of Banach’s and Kannan’s Results in FM-spaces. Commun. Korean Math. Soc. 2012, 27, 265–277. [Google Scholar] [CrossRef]
- Petric, M.A. Some remarks concerning Ćiric-reich-rus operators. Creat. Math. Inform. 2009, 18, 188–193. [Google Scholar]
- Younis, M.; Singh, D.; Abdou, A.N. A fixed point approach for tuning circuit problem in b-dislocated metric spaces. Mathematical Methods in the Applied Sciences 2022, 45, 2234–2253. [Google Scholar] [CrossRef]
- Younis, M.; Bahuguna, D. A unique approach to graph-based metric spaces with an application to rocket ascension. Computational and Applied Mathematics 2023, 42, 44. [Google Scholar] [CrossRef]
- Younis, M.; Ahmad, H.; Chen, L.; Han, M. Computation and convergence of fixed points in graphical spaces with an application to elastic beam deformations. Journal of Geometry and Physics. 2023, 192, 104955. [Google Scholar] [CrossRef]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Copyright: This open access article is published under a Creative Commons CC BY 4.0 license, which permit the free download, distribution, and reuse, provided that the author and preprint are cited in any reuse.
MDPI Initiatives
Important Links
© 2024 MDPI (Basel, Switzerland) unless otherwise stated





