Preprint
Article
Effect of Thermal Aging on Viscoelastic Behavior of Thermosetting Polymers under Mechanical and Cyclic Temperature Impact
This version is not peer-reviewed.
Submitted:
12 December 2023
Posted:
13 December 2023
You are already at the latest version
Abstract
Development of load-bearing polymer composite constructions in civil engineering, such as industrial chimneys and gas ducts, requires filling some research gaps. These gaps are related to long-term behavior under mechanical and temperature loads, cyclic ones as well. Such conditions mean that viscoelasticity should be considered. First of all, viscoelastic parameters should be calculated, based on the used inner structure model. Then, due to the high working temperatures, thermal aging should be considered as well. This research is devoted to effects of thermal aging on viscoelastic behavior of polymers. Two sets of experiments were conducted: creep tensile tests and cyclic heating in constrained state. The Kelvin-Voigt viscoelasticity model was used to determine the rheological parameters of binder from experimental creep curves. Thermally-aged binder turned out to lose its viscous properties and became elastic. Cyclic heating was used to compare the behavior of normal and thermally-aged binder and to evaluate the possibility of temperature stress accumulation. Similar to creep tests, cyclic heating showed that aged binder lost viscosity and worked almost perfectly elastic. Non-aged binder was prone to stress accumulation much more. As a result, the rheological behavior of aged and non-aged binder was compared and showed a significant difference. In addition, the influence of temperature stress accumulation on stress—strain state was evaluated and turned out to be significant as well. The obtained results can be further used to predict the mechanical behavior of structures made of polymer composites operating for a long time at elevated temperatures, including those exceeding the glass transition temperature.
Keywords:
thermal aging
; polymer composites
; glass fiber-reinforced plastics
; thermo-relaxation
; ; viscoelasticity
; creep
; elasticity
; thermal load
Advanced Polymeric Composites: Processing, Characterization and Mechanical Behavior
1. Introduction
Polymer composites are prospective modern materials for civil engineering due to their low weight, high corrosion resistance and high strength [1-3]. They are effective for constructions which are exposed to aggressive environment, e.g. industrial chimneys and gas ducts. Such constructions operate in specific conditions: they are under constant mechanical load for a long period of time (up to decades) and high temperature as well. Thus, long-term behavior of material should be determined and considered. In addition to that, there can be a lot of cycles of heating and cooling, which could lead to accumulation of temperature stresses. If such stresses do occur and turn out to be significant, they can affect the stress-strain state of the structure. Such stresses, not considered in the design, can complement the stresses from the load and, for example, in total exceed the critical stresses of loss of stability. Therefore, temperature cycles and time must be considered while designing load-bearing polymer constructions.
These days, FRP gas exhaust systems are usually not designed as bearing constructions and the loads are transferred to supporting constructions (e.g., steel frameworks)[4]. Using full material’s capacity could make polymer composite gas exhaust systems cheaper and more reliable. First of all, if the composite’s bearing capacity was considered, supporting constructions could be made weaker or not used at all. Moreover, the supporting constructions tend to corrode quickly and need repairs which cost a lot and sometimes are impossible (due to the fact that it is very expensive and problematic to pause the process of an industrial facility). These days it is especially relevant as industrial facilities are moving from coal fuel to gas, which results in lower exhaust temperatures and more active condensation and corrosion.
It is known that polymers have a viscous behavior [5,6,7,8,9,10,11,12,13,14,15] and change their properties under heating [6,16,17]. However, the long-term behavior of polymer composites is not fully researched and there are no standardized solutions which are needed for civil engineering. In spite of the fact that the viscous behavior was studied in a number of papers [5], temperature influence on it is yet to be determined. In some papers, the influence of working temperature on stiffness is studied [6,16], but for immediate response, without considering viscosity of material. Although there are papers about creep of polymers [5,18,19], they use different models and materials and it’s difficult to turn the results in practical solution. Thermal behavior of polymers and FRPs have been also studied before [17,20,21] and is researched sufficiently. The research gap is how the material works when all these factors are combined: when the construction operates for a long period of time under constant load and changing temperature conditions. To develop the usage of load-bearing FRP constructions in civil engineering, we need to study the long-term behavior of polymers considering rheological behavior and temperature dependencies at the same time.
Previous research of thermal aging effects showed that the polymer structure right after curing includes a lot of weak bonds with different bond strengths [22]. These bonds are responsible for viscous behavior of material. As it was stated, thermal aging results in destroying these weak bonds, leading to increase in elasticity. Therefore, in this paper we propose the hypothesis that thermal aging shifts the material behavior from viscoelastic to elastic to some extent. If it turns out to be true, thermal aging would become one more factor to be considered while designing polymer constructions as it will directly influence the stress-strain state.
The viscoelastic behavior can be assessed through such effects as, for example, creep and relaxation. In order to describe the long-term behavior of polymers and obtain the rheological parameters, mathematical modeling can be used. There is a number of such models, having the same idea of modeling the inner structure of polymers as a combination of primitive elastic and viscous elements, connected in series or parallel[23]. The creep (or relaxation) curves are based on exponential law. One of the promising models is the Kelvin-Voigt model, which is well-known[23,24,25,26,27] and applicable for polymers as well. In this paper, we are going to use the Kelvin-Voigt model (which consists of one elastic and one viscous element, connected in parallel) connected to one more elastic element in series. Such three-element model was described before in classical creep theory [23]. The rheological parameters are acquired through numerical approximation of theoretical and experimental creep curves.
Thus, the aim of this research is to compare the viscoelastic behavior of material in normal and thermally-aged state and experimentally evaluate the difference of their work in cyclic heating environment. It includes:
- -
- Testing both materials for creep and acquiring creep curves.
- -
- Applying the mathematical model of viscoelasticity to get rheological parameters and comparing them.
- -
- Testing the specimens for cycling heating in constrained conditions and comparing the accumulation of stresses.
- -
- Assessing the influence of thermal aging on behavior of material for such conditions.
2. Materials and Methods
2.1. Materials
In this research we studied the epoxy binder. The same materials as in our previous researches were used [7,12,18,19,20,21,22] (presented in Table 1).
The specimens were cured for 2 hours under 80°C and then kept for 24 hours under 150°C. This post-curing was conducted in order to change the specimen from extremely plastic under-cured state closer to the real construction material.
Table 1.
Types of binders investigated.
| № | Composition | Name |
|---|---|---|
| 1 | Epoxy (Ker 828 52.5% + MTHPA 44.5% + alkofen 3%) (without thermal aging) |
EP |
| 2 | Epoxy (Ker 828 52.5% + MTHPA 44.5% + alkofen 3%) (thermally-aged, see 2.2.1) |
EP-TR |
2.2. Methods
2.2.1. Long Heat Treatment (Thermal Aging)
The same aging conditions were used as in previous studies [28].
2.2.2. Tensile testing chamber
The tests were carried out in a specially manufactured chamber that allowed the ends of the sample to be pinched from longitudinal displacements and to test it for tension by moving one of the chamber parts. Heating elements and a fan were installed in the chamber to create hot air movement and uniformity of heating inside the chamber.
The chamber was installed on a Tinius Olsen h100ku machine (characteristics were presented in previous studies [17,28,31]). The upper part of the camera (see Figure 1) was clamped in the grip of the machine and is able to slip freely relative to the lower part. Clamps had flexibility for turns, so bending moments from eccentricities did not occur in the sample, and it worked only for longitudinal force.
The strain of the sample was controlled by clock-type sensors to eliminate the effect of slippage in the grips of the machine and the test chamber. 4 sensors with an accuracy of 0.01 mm were used, mounted in pairs crosswise (to account for possible slopes relative to both axes of symmetry of the sample section).
To increase the accuracy of determining the calculated length, the contact of the corner shelf with the sample along its length was minimized by inserting the spacer and making sure that the contact of corner and the specimen is concentrated in one spot. The test scheme and the photo of real installation are presented at Figure 2.
2.2.3. Testing for creep
The Tinius testing machine allowed us to make a program for constant loading. The machine automatically increases the strain if the tensile force falls. Consequently, it was used for creep test as it represents the conditions for creep: constant stress and unconstrained displacement.
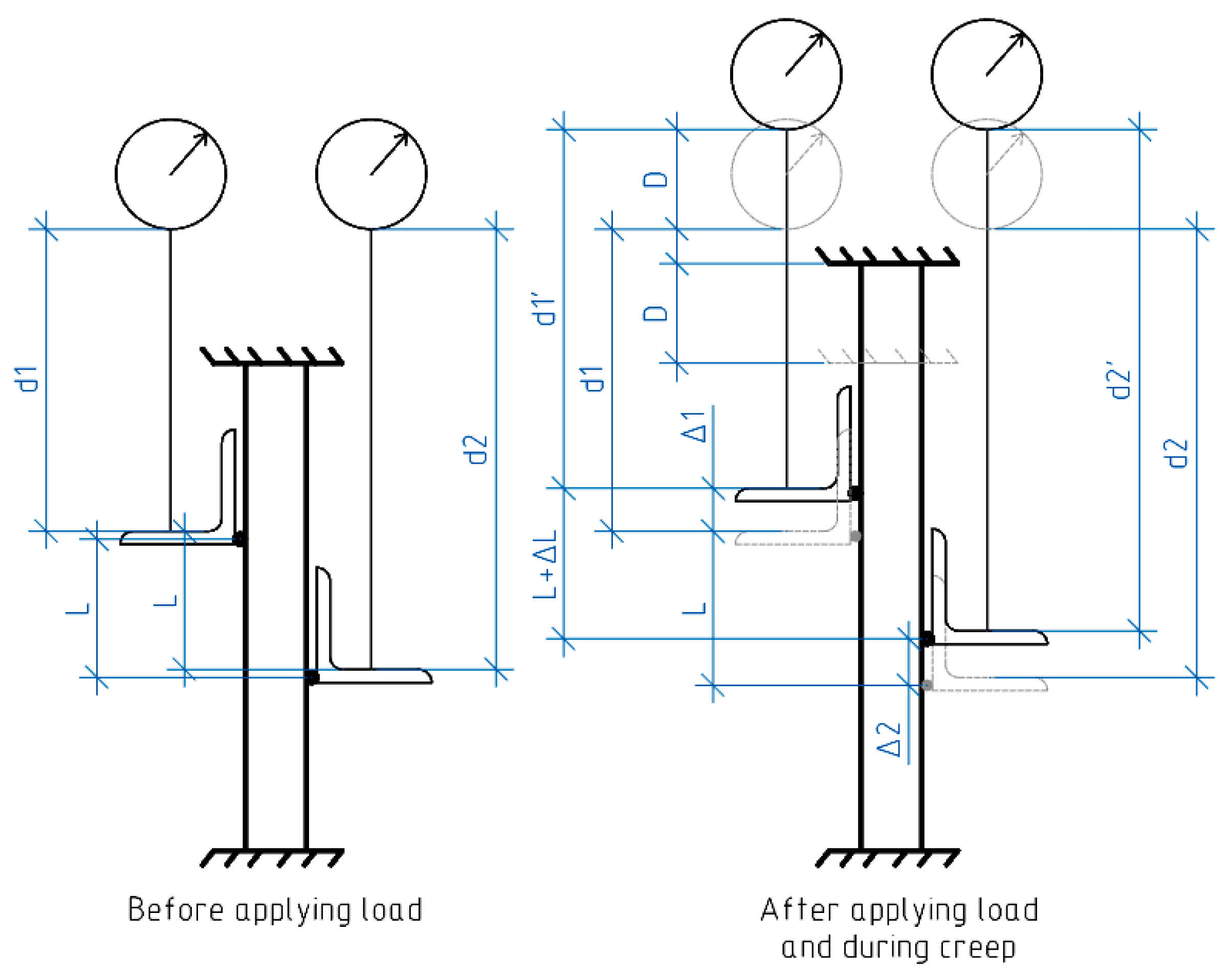
The sensor readings were recorded at zero force, immediately after applying load and then with the steps of 1, 5, 10, 15, 30, 60 minutes. They were transformed into span change using formulas (1-7) (see Figure 3). Then, the strain was calculated by dividing the displacement by the initial span. As the result, creep curves were obtained ( – time).
Where readings and are average for 2 crosswise sensors for near and far sections, respectively.
Figure 3.
The scheme for formulas (1-7).
2.2.4. Acquiring rheological parameters
In this research we used the three-element model which is based on Kelvin-Voigt model. This model is described in classical creep theory [23]. The appearance of model is shown at Figure 4.
and are Young’s modulus for elastic elements. is a parameter of viscosity of viscous element. are normal stresses in elastic elements, viscous element and the material overall, respectively. These parameters are connected by following relations [23] (formulas 8-9):
is a long-term elastic modulus, is a relaxation time.
The creep law is described by (10):
Experimental creep curves were imported into Mathcad program, which created a system of equations (see (10)), where strain, time and elastic modulus are known parameters and are unknown. The elastic modulus was obtained in previous research [17,31] and checked again in this one at initial loading. By equalizing the theoretical and experimental strain values at given times, the program numerically approximated them and came up with which provided convergence of theoretical and experimental curves.
As [23], there are 2 unknown parameters left: and . By solving the system of equations (8, 9), they were defined.
As a result, all parameters of the model were determined.
2.2.5. Cyclic heating test
The specimen was clamped on both sides and pre-stressed for a load of 1000 N. It was done in order to get rid of possible shifts in installation. After the initial load, the specimen was left to rest for 5 minutes to let the initial fast relaxation slow down and to not disturb the results.
The temperature was automatically controlled by a thermostat, and also checked according to a reference sample of the same thickness as the test sample, with a thermocouple glued inside.
The testing process was: turning on the heating until the desired temperature was reached, turning heating off until the specimen cools down to room temperature, then repeating the cycle a number of times.
The measurements were recorded each 5 minutes during the whole testing period. The temperature was recorded from the reference sample and the variation from the thermostat was no more than 2°C.
From previous research [17], the dependences of the modulus of elasticity () and coefficient of thermal expansion (CTE) from temperature are known. Previously, the relationship between and CTE was established and it was found that up to a temperature of about 80 °C, they change weakly and almost linearly. After 80 °C, there is a sharp change in the behavior of the material and a non-linear growth of and CTE. At this stage of research, it was assumed that the viscoelastic behavior of the material will change in a similar way, since all these processes are related to the internal structure of the material. Since the determination of rheological parameters was carried out at normal temperature (see further), heating tests were carried out at 70°C in order to exclude the possible influence of non-linearities that have not yet been studied.
3. Results and Discussion
3.1. Creep tests
To assess the correctness of measured strains, the Young’s modulus was calculated, using the Hooke’s law. The modulus was , what corresponds to our previous research [17,31] and other scientist’s data [6]. Thus, the experimental data was considered to be correct.
The testing equipment did not allow us to test both EP and EP-TR samples under the same stresses. However, the rheological parameters should not depend on stress, which was confirmed in this research (see 3.2).
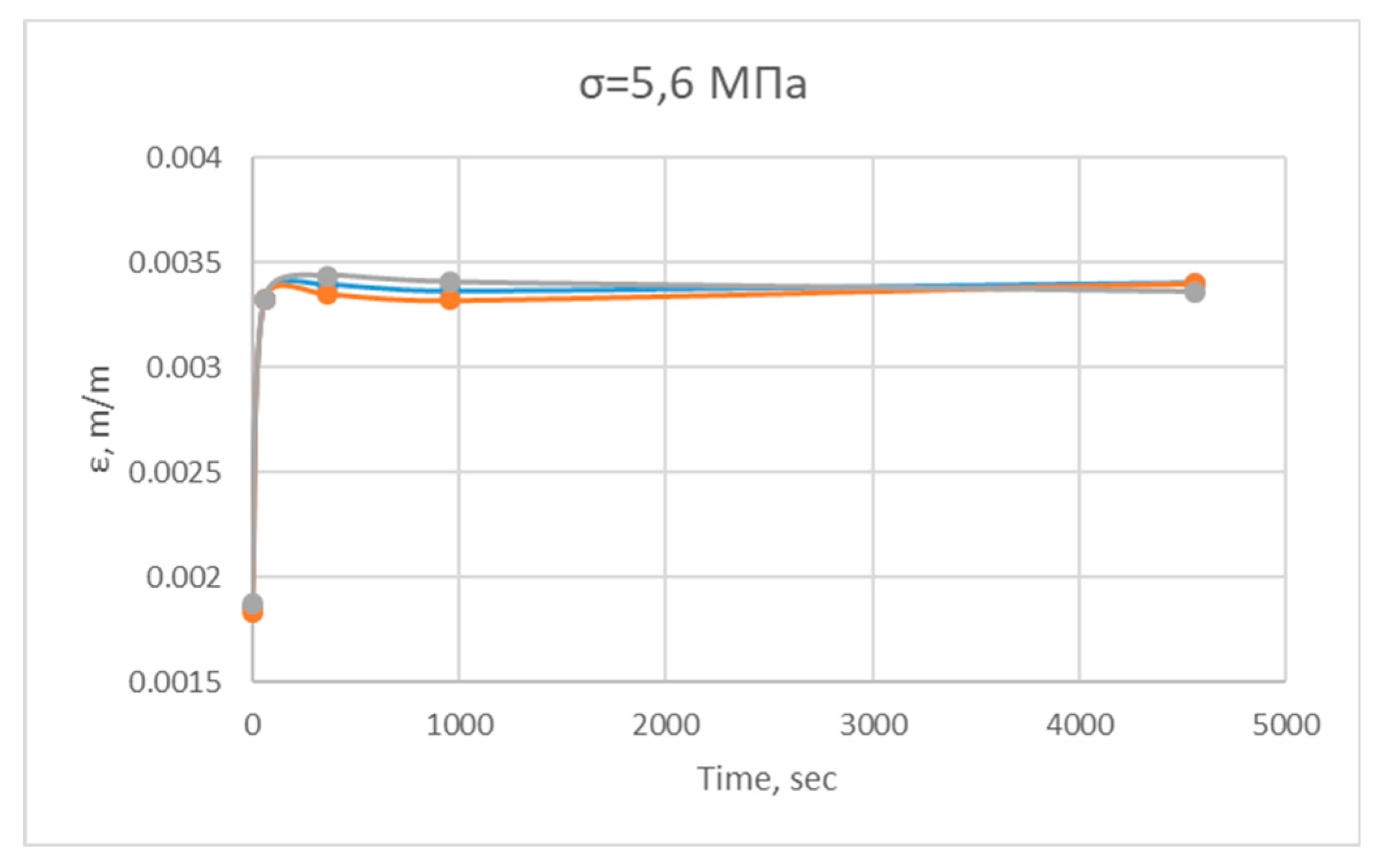
As we can see at Figure 4 and Figure 5, the behavior of normal and thermally-relaxed binder under constant load is different. EP samples gave us classical creep curves, with strain increasing over time, gradually slowing down. On the other hand, EP-TR show almost no viscosity. There is a rapid increase in strain in the beginning (which was followed by audible crackling) and then the strain change is negligible.
Figure 5.
Creep curves for EP.
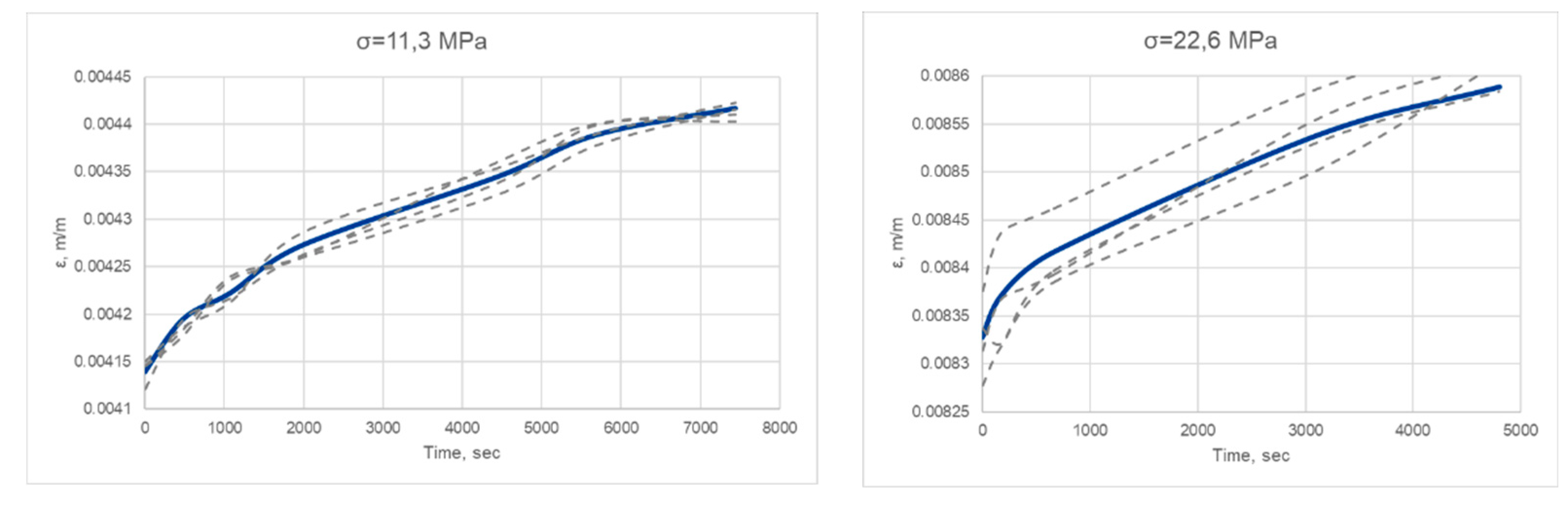
Figure 6.
Creep curves for EP-TR.
This difference can be explained by the changes in internal structure of material. As weak volatile bonds are destroyed during thermal aging, the material loses its ability for plastic deformation, becoming brittle[22]. Thus, the hypothesis, mentioned in Introduction (that thermal aging decreases viscosity), seems to be proved by the results of creep tests.
3.2. Obtaining rheological parameters
First of all, we used the model to calculate the parameters of EP for both stress levels. The average results are presented in Table 2.
As we can see, the results for both stress levels were quite close. It was expected, as the parameters in such models as the one we used should not depend on stress level. These parameters represent the inner structure of material as well as the Young’s modulus. The results seem to be adequate.
The rheological parameters of model’s elements were calculated for average values from Table 2 and are shown in Table 3.
Using the parameters, theoretical creep curves were created and compared to experimental. The result is shown at Figure 7.
Indeed, the calculated rheological parameters represent the real material behavior.
The biggest error is at the end of the curve for 22,64 MPa. The experimental value here is 0.00859 and theoretical is 0.00867. Thus, the error is 0.93%, which is satisfying.
The situation is different for EP-TR. As it was mentioned before, aged binder became non-viscous and brittle. It’s creep curve (Figure 6) does not correspond to the law (formula 10). The initial strain change is, presumably, caused by appearance of inner cracks. Therefore, the is no rheology in EP-TR’s behavior. It has both advantages and disadvantages. On the one hand, elastic behavior is good for calculations as it can be easily determined and it does not have such effects as stress accumulation. On the other hand, brittleness is a negative factor for constructions. Cracks have to be considered in calculating stiffness (in analogy with concrete) and the destruction is immediate and dangerous.
3.3. Cyclic heating
The time-temperature-force dependencies are presented at Figure 8 and Figure 9 for EP and EP-TR, respectively.
As the figures show, the temperature stress accumulation was much lower in EP-TR. By the end of 3th cycle, EP had lost 11.6% of tensile force, while EP-TR had lost 6.5%.
By the end of shown cycles (5 for EP and 3 for TR), there was almost no further difference.
The minimum force for EP got 25.5% lower at the end, and 9.7% lower for EP-TR.
As a result, the data from creep tests, which showed the decrease in viscosity, correlates with cyclic heating results. The change in longitudinal force during cycles is the sum of accumulated temperature compression stress and the relaxation, accelerated by high temperature. Both of them are connected to viscosity. Therefore, smaller change means less viscosity.
Again, thermal aging has been proven to shift the material from viscoelastic to elastic. The hypothesis, stated in Introduction, was proved by 2 separate experiments.
In both cases, slower or faster, we see that over some cycles the changes become negligible. The viscoelastic model we used earlier have a special feature: as can be seen from the formula (10), the creep slows down over time, stopping at some point:
It also proves the applicability of the selected model for the studied material. This feature can be used in future to make construction’s calculations easier as the maximum creep strain could be defined from (11).
4. Conclusions
In this study, following goals were achieved:
- -
- EP and EP-TR binders were tested for creep and curves were obtained.
- -
- The mathematical model of viscoelasticity was used to get rheological parameters of EP. The EP-TR turned out to lack viscous behavior and was brittle.
- -
- The long-term behavior of EP and EP-TR was compared.
- -
- Both binder variants were tested for cyclic heating in restrained conditions and their behavior was also compared.
- -
- The influence of thermal aging on viscoelastic behavior was assessed.
Non-aged epoxy binder, as expected, shows viscoelastic behavior which can be described, using the three-element Kelvin-Voigt model. It showed similar material constants for 2 different stress levels; the behavior of theoretical strain-time creep curves corresponded to the experimental curve. Future research will be based on this model.
Thermal aging, as it was previously stated in our previous research [22,28] and by other authors [20,21,32], influences the properties of material. Based on this knowledge, the new hypothesis was carried out: aging also influences the viscoelasticity. This hypothesis was proved by 2 independent experiments: creep testing and cyclic heating. Indeed, EP-TR appeared to behave non-viscously in creep test and almost elastic in cyclic heating.
Thermal aging should be investigated more, as it not only has positive effects, but increases brittleness and crack formation.
Stress accumulation from cyclic heating-cooling directly influences the stress-strain state of construction and should be considered in future when designing such constructions. Depending on the temperature regime, the relaxation or accumulation can be up to 25% of stress (based on current research data). It cannot be ignored while designing load-bearing polymer composite constructions and requires more research to get safety coefficients to account for these phenomena. Therefore, further research of viscoelasticity and thermal behavior is needed.
Author Contributions
Conceptualization, A.K. and M.M.; methodology, A.K., M.M. and A.Z.; validation, A.K. and M.M.; formal analysis, A.K., M.M. and A.Z.; investigation, A.Z. and M.M.; resources, A.K.; data curation, A.Z. and M.M.; writing—original draft preparation, A.Z.; writing—review and editing, A.Z.; visualization, A.Z.; supervision, M.M. and A.K.; project administration, A.K. and M.M.; funding acquisition, M.M. All authors have read and agreed to the published version of the manuscript.
Funding
The research was funded by Russian Science Foundation, grant number 23-29-00425
Conflicts of Interest
The authors declare no conflict of interest.
References
- El Damatty, A.A.; Awad, A.S.; Vickery, B.J. Thermal analysis of FRP chimneys using consistent laminated shell element. Thin-Walled Structures. 2000, 37, 57–76. [Google Scholar] [CrossRef]
- Astashkin, V.M.; Mishnev, M.V. On the Development of the Manufacturing Technology of Fiberglass Cylindrical Shells of Gas Exhaust Trunks by Buildup Winding. Procedia Engineering. 2016, 150, 1636–1642. [Google Scholar] [CrossRef]
- Bochicchio, V.A. FRP chimney liners for power plant flue gas desulfurization - A chimney supplier’s perspective. NACE - International Corrosion Conference Series. 2011. [Google Scholar]
- Astashkin, V.M.; Zholudov, V.S. Chimneys and Elements of Gas-Escape Paths Made of Polymer Composites. Chelyabinsk, Russia: Abris-Print, 2011.
- Drozdov, A.D.; Høj Jermiin, R.; de Claville Christiansen, J. Lifetime Predictions for High-Density Polyethylene under Creep: Experiments and Modeling. Polymers (Basel). 2023, 15, 334. [Google Scholar] [CrossRef] [PubMed]
- Tefera, G.; Adali, S.; Bright, G. Flexural and Viscoelastic Properties of FRP Composite Laminates under Higher Temperatures: Experiments and Model Assessment. Polymers (Basel). 2022, 14, 2296. [Google Scholar] [CrossRef] [PubMed]
- Ribeiro, J.G.T.; Castro, J.T.P.; De Meggiolaro, M.A. Modeling concrete and polymer creep using fractional calculus. Journal of Materials Research and Technology. 2021, 12, 1184–1193. [Google Scholar] [CrossRef]
- Shokuhfar, A.; et al. Predictive modeling of creep in polymer/layered silicate nanocomposites. Polym Test. 2012, 31, 345–354. [Google Scholar] [CrossRef]
- Hassanzadeh-Aghdam, M.K.; et al. Effect of nanoparticle aggregation on the creep behavior of polymer nanocomposites. Compos Sci Technol. 2018, 162, 93–100. [Google Scholar] [CrossRef]
- Amjadi, M.; Fatemi, A. Creep behavior and modeling of high-density polyethylene (HDPE). Polym Test. 2021, 94, 107031. [Google Scholar] [CrossRef]
- Göbel, L.; et al. Viscoelastic behavior of polymer-modified cement pastes: Insight from downscaling short-term macroscopic creep tests by means of multiscale modeling. Applied Sciences (Switzerland). 2018, 8, 4. [Google Scholar] [CrossRef]
- Chang, Z.; et al. Creep behavior of polymer nanocomposites: Insights from molecular dynamics simulation. Polymer (Guildf). 2021, 228, 123895. [Google Scholar] [CrossRef]
- Bochnia, J.; Blasiak, S. Stress relaxation and creep of a polymer-aluminum composite produced through selective laser sintering. Polymers (Basel). 2020, 12, 4. [Google Scholar] [CrossRef] [PubMed]
- Guedes, R.M. Creep and fatigue in polymer matrix composites. Creep and Fatigue in Polymer Matrix Composites. 2019.
- Raghavan, J.; Meshii, M. Creep of Polymer Composites. Compos Sci Technol. 1998, 57, 1673–1688. [Google Scholar] [CrossRef]
- Manalo, A.; et al. Flexural behavior of an FRP sandwich system with glass-fiber skins and a phenolic core at elevated in-service temperature. Compos Struct. 2016, 152, 96–105. [Google Scholar] [CrossRef]
- Korolev, A.; et al. Relaxation Model of the Relations between the Elastic Modulus and Thermal Expansivity of Thermosetting Polymers and FRPs. Polymers (Basel). 2023, 15, 699. [Google Scholar] [CrossRef] [PubMed]
- Lainé, E.; et al. Methodology of Accelerated Characterization for long-term creep prediction of polymer structures to ensure their service life. Polym Test. 2019, 79. [Google Scholar] [CrossRef]
- Cheng, K.; et al. Experimental Investigation and Prediction for Bending Creep of Glass Fiber-Reinforced Polymer Pultruded Tube. Buildings. 2023, 13, 2714. [Google Scholar] [CrossRef]
- Hannou, A.; et al. Thermal Aging Effect on the Compression Behavior of Thermoplastic Polymers—Proposed Phenomenological Model. Journal of Failure Analysis and Prevention. 2023, 23, 781–794. [Google Scholar] [CrossRef]
- Bahrololoumi, A.; et al. Thermal aging coupled with cyclic fatigue in cross-linked polymers: Constitutive modeling & FE implementation. Int J Solids Struct. 2022, 252. [Google Scholar] [CrossRef]
- Korolev, A.; et al. Prolonged thermal relaxation of the thermosetting polymers. Polymers (Basel). 2021, 13, 4104. [Google Scholar] [CrossRef] [PubMed]
- Rzanitsyn, A.R. Theory of Creep. Moscow: Stroyizdat, 1968.
- Farno, E.; Baudez, J.C.; Eshtiaghi, N. Comparison between classical Kelvin-Voigt and fractional derivative Kelvin-Voigt models in prediction of linear viscoelastic behaviour of waste activated sludge. Science of the Total Environment 2018, 613-614, 1031–1036. [Google Scholar] [CrossRef] [PubMed]
- Cao, T.; et al. Viscoelastic analytical model and design of polymer-based bimodal piezoelectric motor. Mech Syst Signal Process. 2020, 145, 106960. [Google Scholar] [CrossRef]
- Serra-Aguila, A.; et al. Viscoelastic models revisited: characteristics and interconversion formulas for generalized Kelvin–Voigt and Maxwell models. Acta Mechanica Sinica/Lixue Xuebao. 2019, 35, 1191–1209. [Google Scholar] [CrossRef]
- Elele, E.; et al. Mechanical properties of polymeric microfiltration membranes. J Memb Sci. 2019, 591. [Google Scholar] [CrossRef]
- Mishnev, M.; et al. Effect of Long-Term Thermal Relaxation of Epoxy Binder on Thermoelasticity of Fiberglass Plastics: Multiscale Modeling and Experiments. Polymers (Basel). 2022, 14, 1712. [Google Scholar] [CrossRef] [PubMed]
- Korolev, A.; Mishnev, M.; Ulrikh, D.V. Non-Linearity of Thermosetting Polymers’ and GRPs’ Thermal Expanding: Experimental Study and Modeling. Polymers (Basel). 2022, 14, 4281. [Google Scholar] [CrossRef] [PubMed]
- Mishnev, M.; et al. Solid Particle Erosion of Filled and Unfilled Epoxy Resin at Room and Elevated Temperatures. Polymers (Basel). 2023, 15, 1. [Google Scholar] [CrossRef] [PubMed]
- Mishnev, M.V.; et al. Based on the hybrid hot-curing epoxy binder fiberglass and evaluation of its effectiveness in load-bearing chimneys. Construction of Unique Buildings and Structures. 2020, 93, 9302. [Google Scholar]
- Yang, Y.; et al. Thermal aging of an anhydride-cured epoxy resin. Polym Degrad Stab. 2015, 118, 111–119. [Google Scholar] [CrossRef]
Figure 1.
Testing chamber.
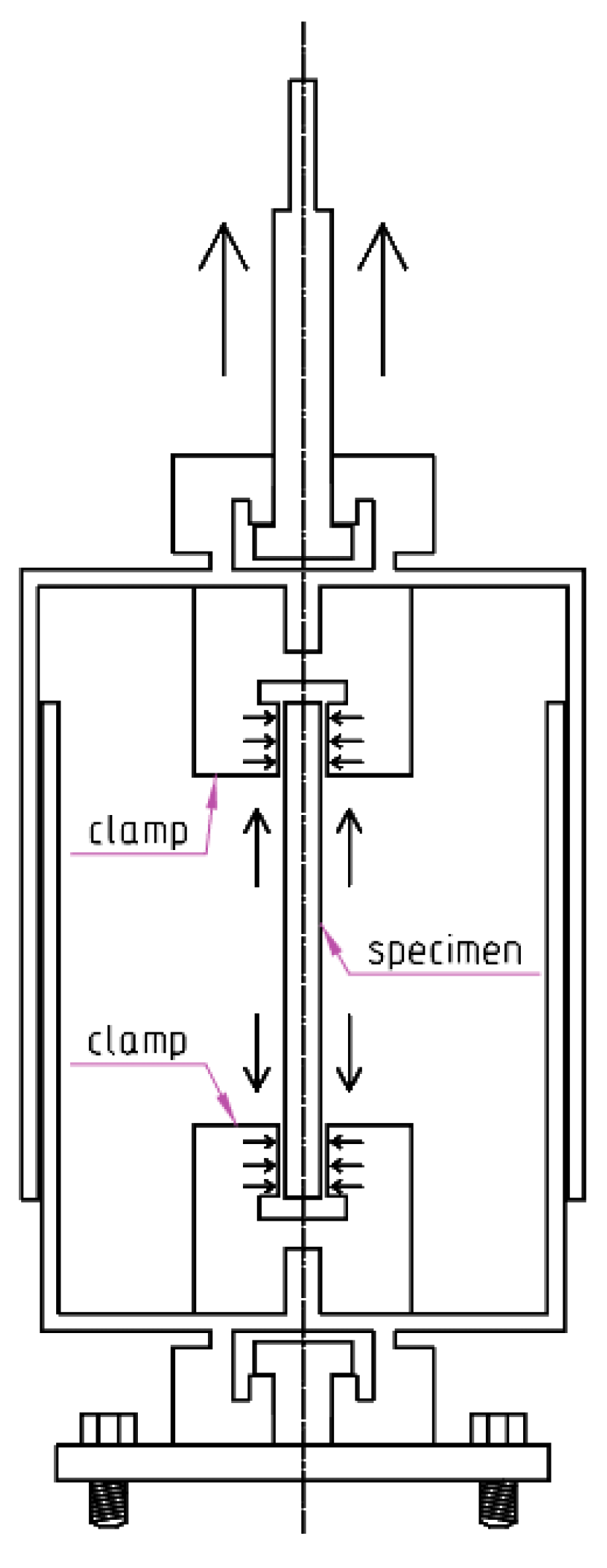
Figure 2.
The test scheme (left) and the real photo (right).
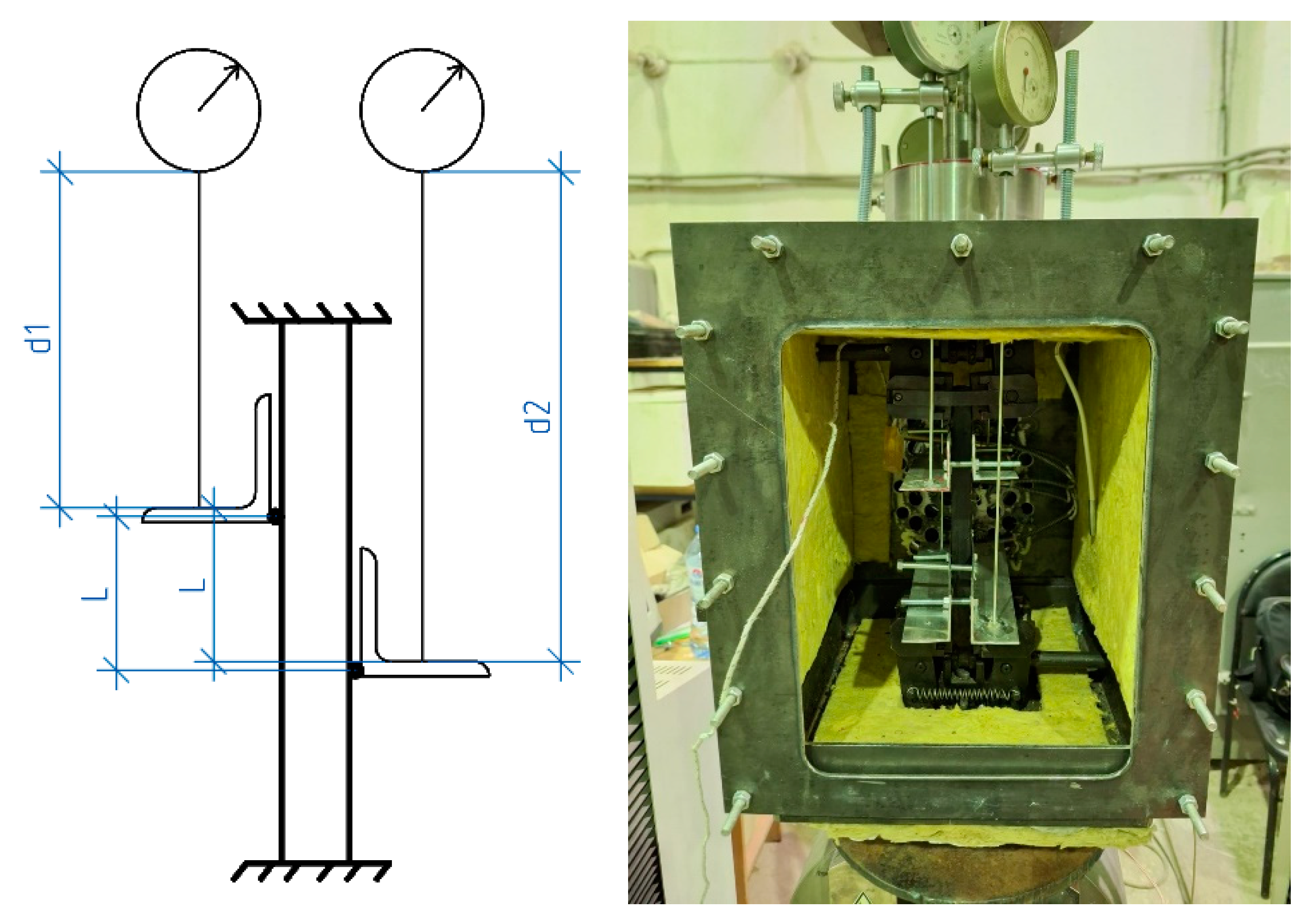
Figure 4.
Three-element viscoelastic model.
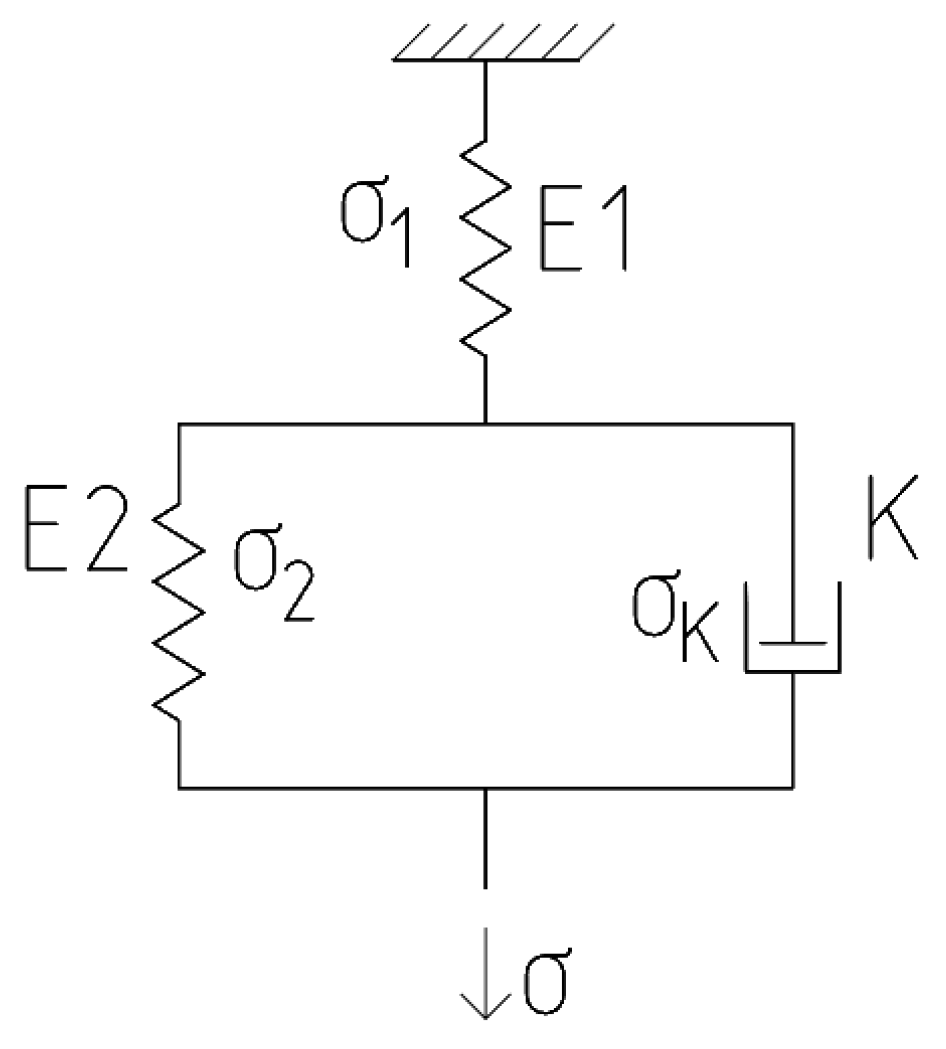
Figure 7.
Comparing theoretical and experimental creep curves for EP.
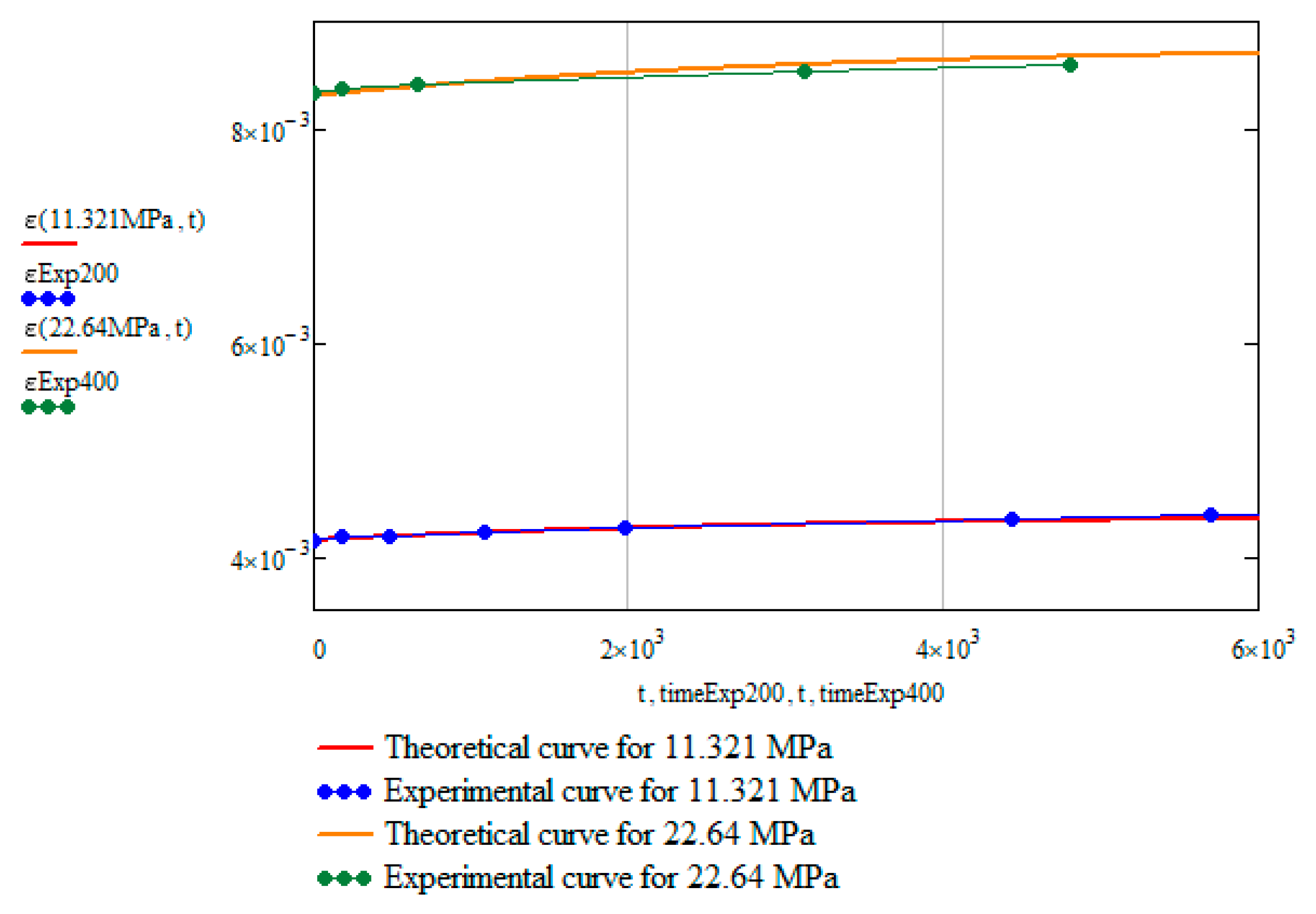
Figure 8.
Cyclic heating of EP.
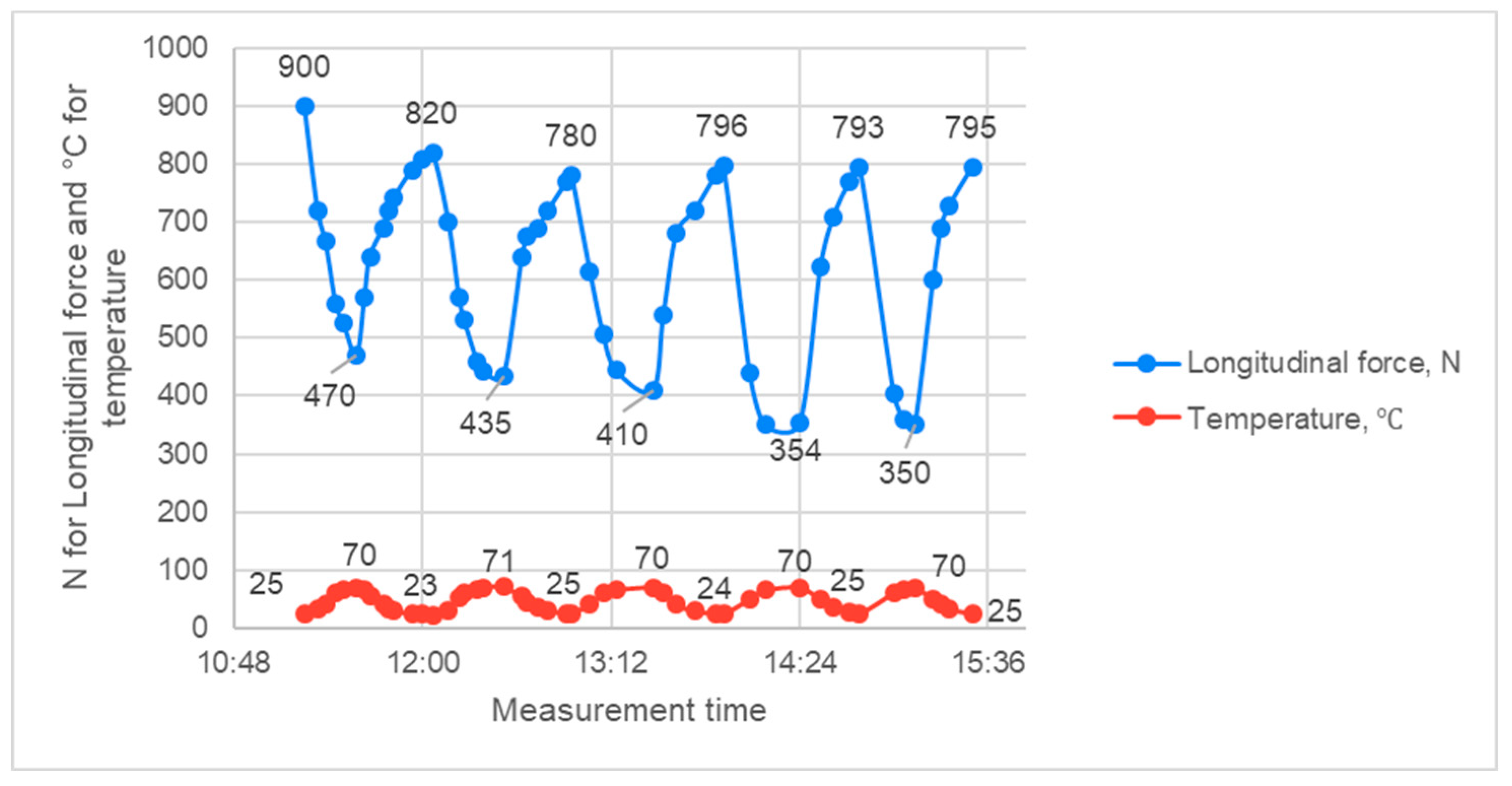
Figure 9.
Cyclic heating of EP-TR.
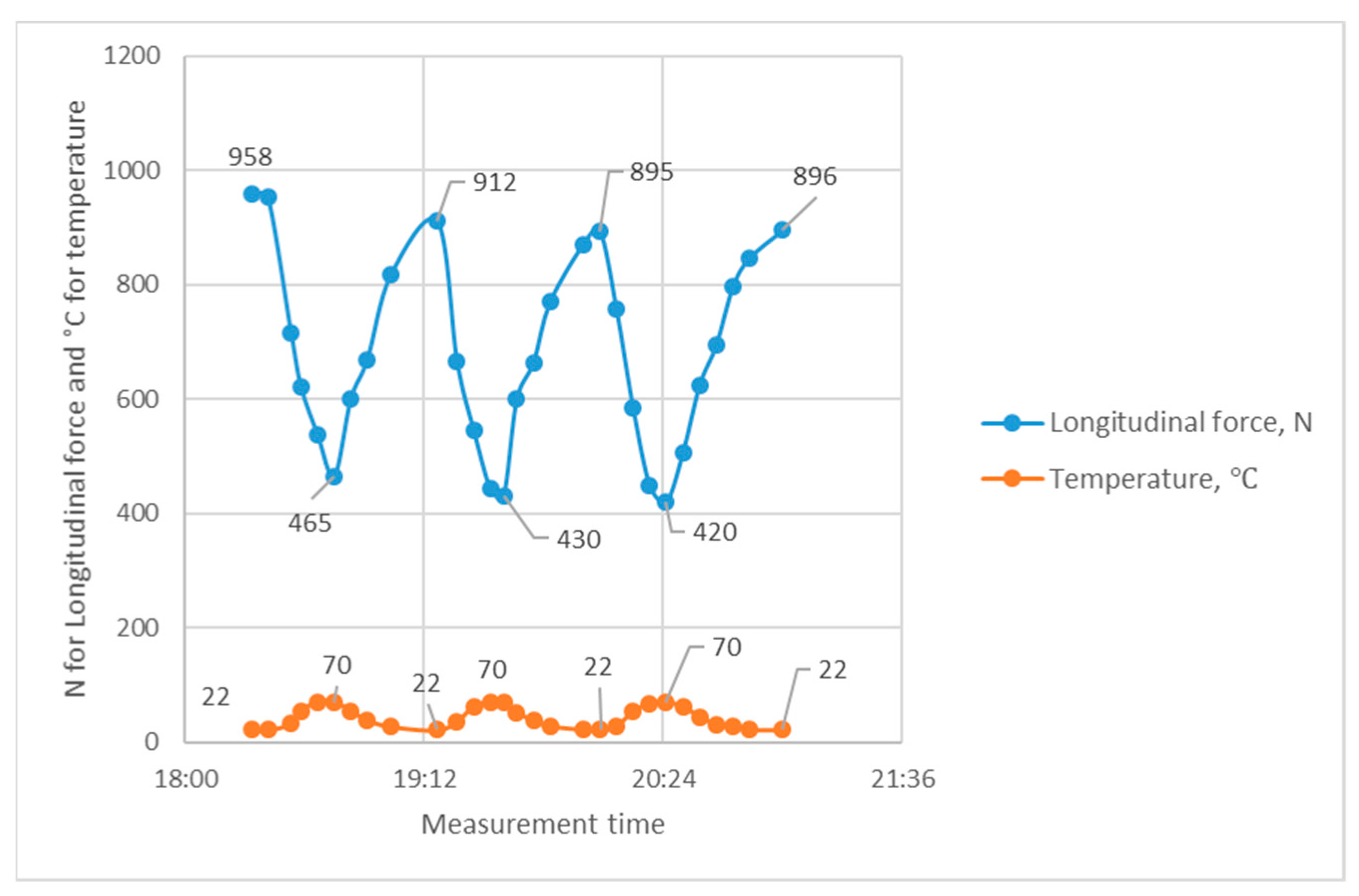
Table 2.
Parameters for EP.
| Composition | σ, MPa | E, MPa | H, MPa | n, min |
|---|---|---|---|---|
| EP | 11.32 | 2735 | 2550 | 51.4 |
| 22.64 | 2719 | 2620 | 48.7 |
Table 3.
Element’s parameters for EP.
| Composition | E1, MPa | E2, MPa | s |
|---|---|---|---|
| EP | 2735 | 2550 | 51.4 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Copyright: This open access article is published under a Creative Commons CC BY 4.0 license, which permit the free download, distribution, and reuse, provided that the author and preprint are cited in any reuse.
Downloads
113
Views
35
Comments
0
Subscription
Notify me about updates to this article or when a peer-reviewed version is published.
MDPI Initiatives
Important Links
© 2025 MDPI (Basel, Switzerland) unless otherwise stated







