Preprint
Article
Impact of Biodiversity Loss on the Structure and Stability of a Marine Antarctic Food Web
Altmetrics
Downloads
189
Views
93
Comments
0
A peer-reviewed article of this preprint also exists.
This version is not peer-reviewed
Submitted:
14 December 2023
Posted:
15 December 2023
You are already at the latest version
Alerts
Abstract
The consequences of climate change and anthropogenic stressors, such as habitat loss and overexploitation, are threatening the subsistence of species and communities across the planet. Therefore, it is crucial to analyze the impact of environmental perturbations on the diversity, structure and function of ecosystems. In this work, in silico simulations of biodiversity loss were carried out on the marine food web of Caleta Potter (25 de Mayo Island, Antarctica) where global warming has caused critical changes in the abundance and distribution of benthic and pelagic communities during the last 30 years. We performed species removal considering their degree and trophic level and including four different thresholds on the occurrence of secondary extinctions. We examined the impact of extinctions on connectance, modularity and stability of the food web. We found different responses of these properties depending on the extinction criteria used, e.g., large increase in modularity and rapid decrease in stability when most-connected and relatively high-trophic level species were removed. Additionally, we studied the complexity-stability relationship of the food web founding two regimes: 1) high sensitivity to small perturbations suggesting that Potter Cove would be locally unstable, and 2) high persistence to long-range perturbations suggesting global stability of this ecosystem.
Keywords:
Subject: Biology and Life Sciences - Ecology, Evolution, Behavior and Systematics
1. Introduction
Climate change, together with impacts of human activities such as habitat fragmentation, pollution, and overexploitation of natural resources, is driving an unprecedented ecological crisis that threatens ecosystems, both terrestrial [1,2] and marine [3,4]. These stressors are changing the patterns of species abundance, distribution, and interactions, placing numerous species towards the brink of extinction, causing alterations at high levels of organization (e.g., food webs) and threatening the persistence of ecological communities [5].
There are many biotic and abiotic factors ─ ultimately associated with climate change ─ that can cause species extinction (e.g., changes in temperature, temporal mismatch between interacting species, freshwater scarcity, etc.), however, changes in biotic interactions that lead to a variation in food availability are one of the most evident factors [6,7,8]. Furthermore, since every species is functionally unique, the risk of a species becoming extinct will also depend on different biological and ecological species traits, such as body size, habitat type or diet breadth [9]. For this reason, it is of great importance to establish and quantify the effects of species extinction on food webs.
Generally, extinction simulations in food webs have been developed considering that a species goes extinct when it loses all its prey due to primary extinctions [10,11,12,13,14,15,16,17,18]. This assumption does not address the possibility that a consumer may become extinct when a certain percentage of its prey is lost; only few works have included different extinction thresholds in this sense. For example, Bellingeri & Bodini [19] have introduced thresholds as an energetic criterion to define species extinction and analyzed the robustness of ten food webs against random species loss. Cordone et al. [20] have simulated ordered and random extinctions in an Antarctic food web including extinction thresholds to study changes in connectance and number of secondary extinctions. However, the effects of biodiversity loss on food webs properties considering thresholds and different species-extinction criteria have been poorly studied.
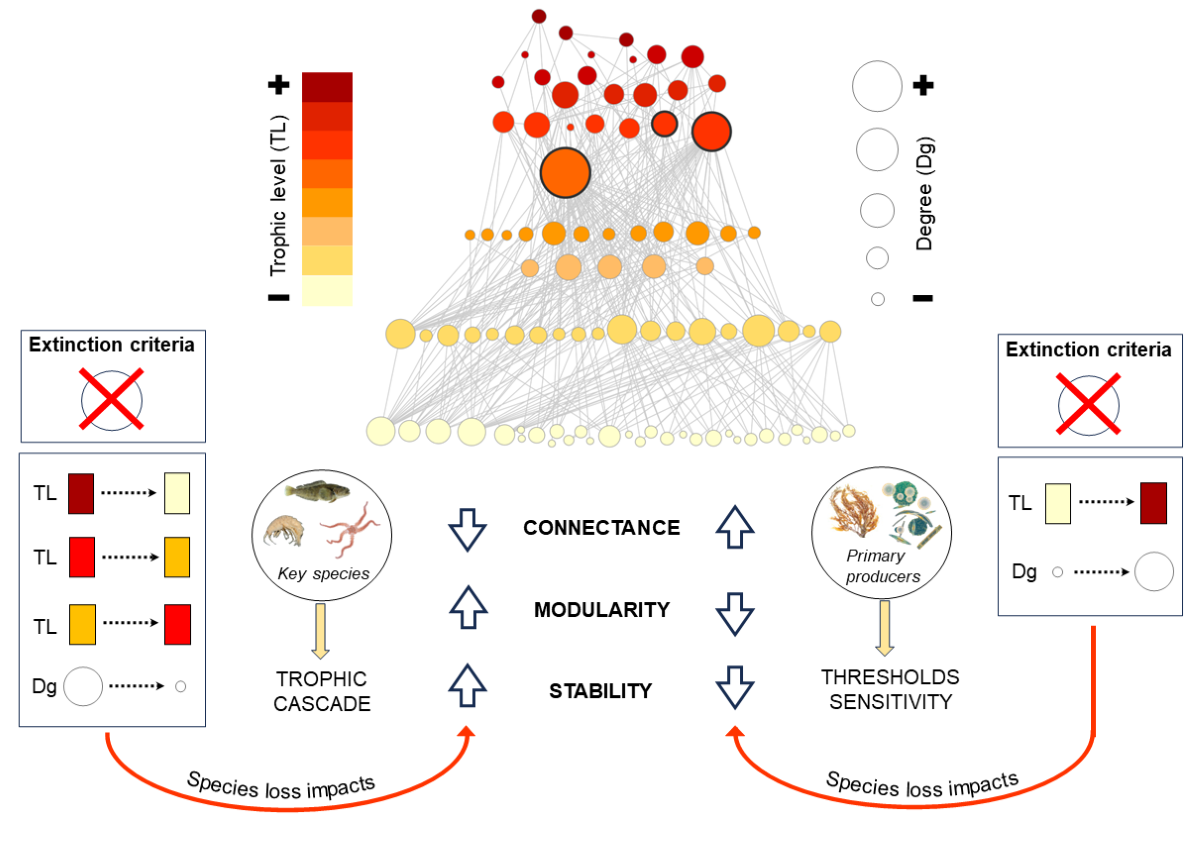
Food webs comprised the trophic interactions between species in an ecosystem resuming the flows of matter and energy among organisms; therefore, food webs provide information about key factors that can modify ecosystem’s structure, function, and stability [12,21,22,23,24,25]. Hence, it is crucial to understand how food web properties change due environmental perturbations that cause biodiversity loss [17,26,27], especially in threatened ecosystems, such as polar regions, where climate change is causing warming at a faster rate than other places in the world [28,29,30]. During the last 30 years, many works have studied the effects of biodiversity loss in food web stability [11,13,14,31,32,33,34] embracing this complex concept that contains multiple facets such as resilience, resistance, persistence or robustness [35]. One of the most studied concepts when simulating species loss in food webs is its robustness, defined as the fraction of primary species loss that induces at least 50% total species loss [12,13,15,16,20,36,37,38,39]. Around this concept, many studies have highlighted the high number of secondary extinctions after removing most-connected species, addressing it cascading effect on food webs [11,12,13,26,40,41]. Recent works have assessed food webs robustness by including different network properties [42,43], however, little is known about how predator sensitivity to prey loss and how extinction criteria (e.g., removing species by trophic level) affect the complexity and stability of food webs.
In this work, we performed in silico extinctions on the highly-resolve food web of the Potter Cove marine ecosystem (25 de Mayo/King George Is., Antarctica). This ecosystem is considered a biodiversity hotspot where global warming has caused the decline of coastal glaciers and the increase in suspended particles due to ice melting [44,45,46], leading profound implications on the benthic and pelagic communities [47,48,49,50]. We performed species removal by degree and by trophic level considering four different proposed thresholds for secondary extinctions, i.e., the proportion of prey that a consumer loss to become extinct. We examined the impact of extinction simulations on the following network properties: connectance (C), modularity (M) and stability (Quasi-Sign Stability). Finally, we analyzed cumulative secondary extinctions vs primary extinctions and evaluated the dependency of M and QSS on food web connectance.
2. Materials and Methods
2.1. Study Site
Potter Cove (62°14´S, 58°40´W) is a tributary inlet of 25 de Mayo/King George Island, Antarctica, and one of the largest fjords of the central South Shetland Islands measuring 4 km long and 2.5 km wide (Figure 1).
2.2. Food web data set
Potter Cove food web, firstly described by Marina et al. [51], was assembled based on trophic information about benthic and pelagic species inhabiting the fjord. This high-resolved food web includes 91 trophic species (nodes), defined as a group of taxa collapsed into a single node in the food web, and 307 feeding interactions (links). Although most of the trophic species were identified at species level (e.g., Euphausia superba), some were defined at a lower taxonomic level due to the lack of detailed trophic information (e.g., ascidians) or when species share the same set of predators and prey (e.g., copepods) [52,53]. From now on, “trophic species” will be called “species”. More details on the Potter Cove food web assembly process can be found in Marina et al. [51].
2.3. Extinction simulations
Species were removed from the network based on two removal criteria: 1) degree (total number of trophic interactions) where species were removed from most-connected to least-connected species (Descending) and vice versa (Ascending), and 2) trophic level (position of species in the food web with respect to the source of matter and energy), where species were removed from high-trophic level to low-trophic level (High) and vice versa (Low). In addition, we established an extinction sequence considering mid-trophic level species (2.5 ≤TL≤3.5) and performed it in both ascending (MidAsc: from 2.5 to 3.5) and descending (MidDes: from 3.5 to 2.5) orders. The last criterion was conducted to understand how the removal of basal, intermediate, and top trophic-level species affect the structure and processes of the ecosystem. Species elimination was carried out until the network was reduced by 90% of its original size (91 species) except for the mid-trophic level extinction sequence, where a fixed number of species was eliminated. Species degree and TL can be found in the Appendix A (Table A1).
2.4. Thresholds on secondary extinctions
For each extinction step, we calculated the number of species lost considering both primary (eliminated species) and secondary extinctions. It has been proposed that a secondary extinction occurs when one consumer species loses all its prey species [11]. This definition is based on a topological approach which solely requires the network structure as input, simplifying its application to complex networks. However, this approach presents two limitations: 1) that a secondary extinction occurs “only” if the consumer loses all its prey due to primary extinctions and 2) that all species have the same baseline probability of extinctions, although in natural systems some species are more vulnerable than others [20,54]. To deal with such limitations, we considered a wide range (0.2-0.8) of secondary extinction thresholds. Following Bellingeri & Bodini [19], we applied different thresholds based on the proportion of prey that a consumer loses. For example, a threshold of 0.2 indicates that a consumer goes extinct when it loses 80% of its prey, representing the 20% of the original incoming items. In this regard, we included four thresholds: 0.2, 0.4 , 0.6 and 0.8.
2.5. Effect on food web properties
In order to measure the impact of extinction simulations on the food web, we considered the following properties: connectance (C), modularity (M) and stability (Quasi-Sign Stability). Connectance (C) is a standard food web metric representing the proportion of possible links that are actually realized. It is considered an estimator of community sensitivity to perturbations that strongly covaries with many network properties [12,22,55,56,57]. Modularity (M) measures how strongly sub-groups (modules) of species interact between them compared with the strength of interaction with other sub-groups. It is related to network persistence since the impacts of a perturbation are retained within modules minimizing impacts on the network [58,59,60]; values closer to 1 indicate more persistance. The stability of the food web was measured using the Quasi-Sign Stability index (QSS), that is the proportion of community matrices that are locally stable preserving the sign structure [61]. We calculated QSS considering the mean of the maximum eigenvalue of the random community matrices for easier analysis and visualization; values closer to zero indicate a more stable food web. This index is directly related to network local stability representing the ‘return time’ of the community needed to return to the original equilibrium after a sufficiently small disturbance [62,63,64]. We calculated C, M, and QSS along the extinction’s sequences, analyzed cumulative secondary extinctions vs primary extinctions and studied properties dependency on connectance.
To enhance the robustness and reliability of our results, we used two different Integrated Development Environments (IDE): RStudio and MatLab. Most network metrics were calculated in R version 4.2.2 (R Core Team 2022), using 'igraph' [65] and ‘multiweb’ [66] packages. Matlab (R2020a) code was used to set the network for each extinction criteria (i.e., ordering the matrix) and identify the species in each extinction step. Source codes and data are available in Supplementary Material.
3. Results
3.1. Effects on connectance (C)
We observed two expectable trends of C when extinctions were performed by degree: 1) in ascending order, C increased along the extinction sequence reaching values six times higher than the original value (0.037): the higher the threshold, the lower the increase; 2) in descending order, C values decreased abruptly, approaching zero: the higher the threshold, the faster the decrease (Figure 2).
When high-trophic level species were removed sequentially (High criterion), C values barely varied from its initial value (0.037) during the first 20 steps, approximately, where it plummeted to the half rapidly. The same trend was observed in subsequent steps (>20). Such a trend did not change among thresholds. When low-trophic level species were removed (Low criterion), C displayed an increasing trend that accelerated at high thresholds (≥0.6). In the case of MidAsc criterion, two opposite trends were observed: a monotonous slight increase in the first 15 steps approximately and a decreasing trend afterwards, where the high thresholds drove at faster decrease. In the case of MidDes criterion, C showed a trend similar to that observed when high-trophic level species were removed (High criterion). Here, the collapse was between steps 15-17 approximately (Figure 2).
3.2. Effects on modularity (M)
We observed that modularity presented opposite trends regarding species degree deletion: when most-connected species were removed (Descending), M increased during the first 15-20 steps and then it rapidly plummeted; when least-connected species were removed (Ascending), M decreased displaying oscillations and faster decreasing at high thresholds (≥0.6) (Figure 3).
For trophic level extinction criteria, we observed that M increased at the beginning and then slightly decreases except for the Low criterion that remains unaltered until a high threshold (≥0.6) when it decreased. There is a slight difference between MidDes and MidAsc criteria, the former one presents a sudden increase in modularity, similar to the High criterion, near the 20th step. No variation in the trend of M for High criterion and no-significant variation for MidDes criterion were observed among the thresholds. Basal and MidAsc criteria displayed variation at high thresholds (≥0.6) (Figure 3).
3.3. Effects on stability (QSS)
The QSS decreased rapidly when deleting from most to least-connected species (Descending). When deleting in Ascending order, it decreased showing a slight variation depending on the threshold: the higher the thresholds, the faster the decrease. When trophic level was considered, it decreased for all criteria and thresholds. Except for the Low criterion, QSS started to rapidly decrease around the 20th step. There were no changes among thresholds for High and MidDes criteria (Figure 4).
3.4. Cumulative secondary extinctions
When most-connected species (Descending) were removed, we observed that the number of secondary extinctions rapidly increased in the first steps (<23) regardless the threshold. On contrary, when least-connected species (Ascending) were removed, the number of secondary extinctions variated among thresholds: low thresholds (≤0.4) displayed a relatively low number of secondary extinctions, while high thresholds (≥0.6) displayed a relatively high number of secondary extinctions (Figure 5).
Removing species by trophic level displayed that the number of secondary extinctions varied for each criterion and thresholds except for the High criterion where no secondary extinctions were registered before 0.8 threshold. The higher number of secondary extinctions was observed for the Low criterion followed by MidAsc criterion where the higher the threshold the higher the number of secondary extinctions. There were no changes for MidDes criterion among thresholds (Figure 5).
3.5. Properties dependency on food web connectance
3.5.1. Modularity
We observed that M decreases when C increases for all thresholds and criteria except when most-connected (Descending) and high-trophic level (High) species were removed, in which M displayed an increasing trend for low values of C (<0.037) (Figure 6).
3.5.2. Quasi-Sign Stability
We observed different trends of the QSS for the different extinction criteria. On one hand, when most-connected species (Descending) were removed, it rapidly increased for low values of C (<0.037); when least-connected species (Ascending) were removed, it softly decreased for values of C higher than 0.037. On the other hand, when species were removed by trophic level, the QSS displayed a fluctuating and increasing trend for low values of C (<0.037) for all criteria excepting Low criterion, in which QSS displayed a fluctuating and decreasing trend for C >0.037 (Figure 7).
4. Discussion
4.1. Topological role of species
It is widely known that not all species have the same role in a community (i.e., they make different contributions to the structure and processes), so removing some of them could lead to additional species losses causing dramatic changes (e.g., trophic cascade) on the whole ecosystem [67,68,69]. These species, also called “keystone species” [70,71], play critical roles in ecological communities and ecosystem function by interacting directly or indirectly with other species [72], hence, the need to get deep on network analysis to quantify their importance in an ecosystem [73]. In this regard, keystone species have been related with the position they occupy within its community [74], ultimately related to properties such as degree (feeding links in which species participates) and trophic level (species vertical position in the food web) since they are good descriptors of the species’ potential to affect the rest of its community [75].
In Potter Cove, three biological species were identified as potential keystone species: the demersal fish Notothenia coriiceps, the brittle star Ophionotus victoriae and the amphipod Bovallia gigantea. Independently of the extinction criteria used (by degree or trophic level), the removal of these species displayed cascade effects on connectance, modularity and quasi-sign stability index. On one hand, these effects can be explained by a combination of species properties where degree and trophic level have a major prominence. Regarding the number of interactions, the three species are relatively high-connected species: N. coriiceps (49), O. victoriae (33) and B. gigantea (18). The demersal fish and the brittle star are the two most-connected species in the food web and the most important predators due to their omnivorous diet. The fish N. coriiceps is a top predator, i.e., it has no predators due to the limits of the food web assembly that did not consider marine mammals or seabirds [51]. The brittle star O. victoriae has only two predators in the food web. The amphipod B. gigantea has a degree that places it as the fourth most-connected species with only five predators in the food web. The case of the third most-connected species (Gondogeneia antarctica (20)) will be discussed later in this section. Regarding trophic level, the three species present a similar and relatively high trophic level with respect to the mean trophic level (2.1): N. coriiceps (2.80), O. victoriae (2.97) and B. gigantea (3.00). Although trophic level alone is not a strong predictor of cascading effect, it is widely known that major predators and primary producers are expected to have particularly large effects on the rest of their communities through top-down and bottom-up control, respectively [75,76,77]. In the food web of Potter Cove, N. coriiceps and O. victoriae are high-connected species and major predators, which can explain the fact that their removal causes cascading effects on the network properties suggesting top-down control. On contrary, although B. gigantea has a similar trophic position than N. coriiceps and O. victoriae, its degree is relatively low compare with them, in fact, there is another amphipod with higher degree than B. gigantea: the above-mentioned G. antarctica. The case of these two species and their roles in the food web deserves to be addressed. Even though G. antarctica is more connected in the food web than B. gigantea, the effect of its removal on network properties was lower. This result might be explained considering that: 1) their trophic levels are 2 and 3 respectively, ultimately linked to their diet (i.e., herbivorous vs. carnivorous), highlighting the importance of the species’ trophic position in the food web over its number of interactions (when this number is not higher with respect to the mean species’ degree), and 2) because there are coexisting species that are trophically-equivalent to G. antarctica and replace the energy paths when it is removed, which does not occur in the case of top predators from a trophic guild, such as B. gigantea. On the other hand, another way to explain the results of removing these three species from the food web could be through the extent to which species interact with different modules (hyperconnected groups) within the network. A recent study that compared the stability of sub-Antarctic and Antarctic food webs, discussed the topological role of the species of Potter Cove food web [78]. They found that N. coriiceps is the only species with high connectivity between and within modules, playing the role of “network connector”, while B. gigantea and O. victoriae were identified as “module connectors” or species whose interactions are mostly between modules. Thus, they suggested that N. coriiceps represent the most important species responsible for linking modules and connecting the entire food web.
4.2. Effects of thresholds on food web properties
Biodiversity loss simulations have been extensively conducted by many studies in the last 30 years in different ecosystems [10,11,12,13,14,15,16,17,18], but only few have considered thresholds on secondary extinctions to assess biodiversity loss [19,20]. Furthermore, the study on how these thresholds impact on food web properties beyond considering species degree as the only extinction scenario has not been addressed so far.
In Potter Cove marine food web, the inclusion of thresholds to assess secondary extinctions in the different scenarios (e.g., remove species by trophic level) displayed different patterns. We observed that the effects of biodiversity loss on food web properties (i.e., connectance, modularity and Quasi-Sign Stability index) displayed similar trends among thresholds in most of the scenarios considered, except for those in where basal or low-trophic-level species were the first species removed from the network. These results might be related to the high number of basal and low-trophic level species in the food web Marina et al. [51] that constitute the food items of most consumers in the network (90% of consumers are connected to at least one basal species). Then, increasing consumers’ sensitivity to the loss of their primary resources (i.e., the consumer becoming extinct after a certain fraction of its prey were removed), implicates the highest impact on food web properties among thresholds compared with other extinction scenarios, increasing food web sensitivity that could suggest a “bottom-up” control. In the last decades, many studies have documented the importance of primary producers for consumers in polar ecosystems [79,80,81], especially in Antarctic communities where micro- and macroalgae have been regarded as primary food sources providing an important proportion of carbon to Antarctic benthic consumers [82,83,84,85]. In Potter Cove, the role of macroalgae in the regulation of the food web has been recently discussed by performing in silico experiments and considering different bottom types [20,86] showing that no cascading effects were observed under macroalgae extinction events until a high threshold is reached. This fact highlights the food web sensitivity to the elimination of other basal species (e.g., phytoplankton) that can cause a trophic cascade. Furthermore, Cordone et al. [20,86] have shown that Potter Cove ecosystem displayed a robust response against environmental perturbations which can be linked to food web redundancy. In Potter Cove, biodiversity loss experiments showed that the removal of most of the species does not have a cascading effect on the ecosystem, which can be related to species having similar functional roles in a community since many species of Potter Cove are similar in their trophic [20] and non-trophic [87] interactions and their loss is not critical for the ecosystem. Thus, species with similar roles may be able to compensate if one species becomes extinct, increasing food web resistance by means of availability of alternative prey [67,88]. Finally, due the complexity of this ecosystem where the species establish different types of interactions (i.e., trophic, commensalism, mutualism) in an intricate manner, deciding whether regulation of number or biomass is primarily controlled by bottom-up, top-down or wasp-waist effects remain a challenge [25].
4.3. Multidimensional stability criteria
Disentangling the complexity-stability relationship of food webs is a challenge that sparks interest and debates among environmentalists even today [89]. We understand stability as a complex concept with numerous ways to be analyzed, which is one of the reasons that drove this challenge. In this work, stability was discussed under the assumption that Potter Cove is in a steady state, considering complexity and structural properties: connectance, modularity and Quasi-Sign Stability index. Connectance ─ directly linked to food web complexity and probably the most-studied property in the last three decades [90] ─ displayed a high impact when most-connected species were removed (i.e., cascading effect), which is consistent with many previous works that suggested that food web sensitivity to perturbations is ruled by the number species and their degree [11,12,13,26,40,41]. As we discussed above, species’ degree and their interactions partners play a fundamental role when analyzing food web persistence against biodiversity loss. Depending on the interactions’ distribution, some species provide more interactions between modules than within modules, so, it would be expected that their elimination increases module robustness reducing the propagation of perturbations and therefore increasing network stability [91]. In Potter Cove food web, the highest values of modularity were reached when the aforementioned keystone species (N. coriiceps, O. victoriae and B. gigantea) were removed which is consistent with their topological role, i.e., network and module connectors. Furthermore, the loss of these species displayed similar effects on the network stability. We observed a rapid change in stability along the extinction sequences that suggest a transition from less to more stable state. This result might be explained by the relationship between complexity and stability: the lowest complexity, the higher stability.
Potter Cove complexity and structure have been initially studied by Marina et al. [51] that suggested potential fragility of the food web due its low values of connectance; however, subsequent studies found the network relatively robust against disturbances [20,78,86]. This apparent contradiction highlighted the need to address a deep understanding of ecosystem complexity by considering the types of species interactions and the relationships between species functional roles with ecosystem structure [25,87]. Taking these suggestions into account, we extended previous findings by analyzing the complexity-stability relationship of Potter Cove food web through the correlation between modularity and stability with connectance. Whilst connectance provides information about food web complexity, modularity and Quasi-Sign Stability index bring out an idea about global and local stability of the ecosystem, respectively. This method revealed two types of stability regimes around food web complexity. On one hand, we found relatively high values of modularity around the original connectance where the lower the connectance the higher the modularity, which suggested high persistence against perturbations. On the other hand, we observed an abrupt change on Quasi-Sign Stability index in a close vicinity of the original connectance: a small variation in the values of connectance caused a large decrease of this index bringing it closer to zero and suggesting a high sensitivity to small disturbances on complexity. In general, this behavior was observed in both modularity and quasi-sign stability regardless of the extinction thresholds and criteria used. Finally, we may explain the apparent contradiction previously mentioned suggesting that Potter Cove would be locally unstable in its initial state due to the sensitivity to small perturbations but globally stable against long-range perturbations.
Supplementary Materials
The following supporting information can be downloaded at the website of this paper posted on Preprints.org. Source codes and data about extinction simulations are available in the public GitHub repository (https://github.com/vasalinas/Extinction-simulations).
Author Contributions
Conceptualization, VS, GC and TIM; methodology, VS and TIM; software, VS, GC and TIM; validation, VS and GC; formal analysis, VS, GC and TIM.; investigation, VS; writing—original draft preparation, VS; writing—review and editing, GC, TIM and FRM; visualization, TIM; supervision, FRM; project administration, VS; funding acquisition, VS. All authors have read and agreed to the published version of the manuscript.
Funding
This research was funded by the European Union’s Horizon 2020 research and innovation program under the Marie Skłodowska-Curie grant agreement N° 872690, Alfred Wegener Institute (AWI) - Universidad Nacional de General Sarmiento (UNGS Res. N°209/98).
Institutional Review Board Statement
Not applicable.
Data Availability Statement
Source codes and data about extinction simulations are available in the public GitHub repository (https://github.com/vasalinas/Extinction-simulations).
Acknowledgments
This research was support by Ghent University (UGhent, Belgium) and Universidad Nacional de General Sarmiento (UNGS, Argentina). The work was conducted in the frame of VS participation in the EU CoastCarb project (N° 872690), Horizon2020 Program, funded by Marie Skłodowska-Curie Actions RISE (Research and Innovation Staff Exchange).
Conflicts of Interest
The authors declare no conflict of interest. The funders had no role in the design of the study; in the collection, analyses, or interpretation of data; in the writing of the manuscript; or in the decision to publish the results.
Appendix A
| Species | TL | Degree |
|---|---|---|
| Urticinopsis antartica | 4.27 | 4 |
| Octopus | 4.13 | 4 |
| Chaenocephalus aceratus | 4.02 | 4 |
| Protomyctophum | 3.70 | 1 |
| Diplasterias brucei | 3.67 | 1 |
| Trematomus newnesi | 3.65 | 10 |
| Trematomus bernacchi | 3.59 | 7 |
| Parachaenichthys charcoti | 3.50 | 1 |
| Perknaster fuscus antarticus | 3.46 | 4 |
| Parborlasia corrugatus | 3.41 | 9 |
| Odontaster meridionalis | 3.35 | 7 |
| Hyperiids | 3.33 | 6 |
| Harpagifer antarcticus | 3.32 | 11 |
| Notothenia rossii | 3.25 | 8 |
| Margarella antarctica | 3.25 | 10 |
| Perknaster aurorae | 3.25 | 2 |
| Sterechinus neumayeri | 3.21 | 17 |
| Glyptonotus antarcticus | 3.13 | 8 |
| Lepidonotothen nudifrons | 3.07 | 7 |
| Austrodoris kerguelensis | 3.07 | 10 |
| Odontaster validus | 3.06 | 10 |
| Bovallia gigantea | 3.00 | 18 |
| Ophionotus victoriae | 2.97 | 33 |
| Notothenia coriiceps | 2.80 | 49 |
| Salps | 2.70 | 8 |
| Neobuccinum eatoni | 2.67 | 11 |
| Dacrydyum sp. | 2.50 | 3 |
| Euphausia superba | 2.50 | 11 |
| Copepods | 2.50 | 5 |
| Ascidians | 2.50 | 5 |
| Oligochaetes | 2.50 | 3 |
| Hydrozoans | 2.50 | 4 |
| Bryozoans | 2.50 | 5 |
| Priapulids | 2.50 | 2 |
| Mysids | 2.50 | 3 |
| Malacobelmnon daytoni | 2.50 | 2 |
| Laternulla elliptica | 2.33 | 6 |
| Haliclonidae sp. | 2.25 | 11 |
| Stylo-Myca | 2.25 | 13 |
| Rosella sp. | 2.25 | 11 |
| Dendrilla antarctica | 2.25 | 6 |
| Nereidae | 2.00 | 17 |
| Eatoniella sp. | 2.00 | 7 |
| Nacella concinna | 2.00 | 9 |
| Laevilacunaria antarctica | 2.00 | 9 |
| Paradexamine sp. | 2.00 | 7 |
| Eurymera monticulosa | 2.00 | 9 |
| Pontogeneiella sp. | 2.00 | 8 |
| Gondogeneia antarctica | 2.00 | 20 |
| Pariphimedia integricauda | 2.00 | 3 |
| Cheirimedon femoratus | 2.00 | 4 |
| Gitanopsis antarctica | 2.00 | 5 |
| Prostebbingia gracilis | 2.00 | 14 |
| Waldeckia obesa | 2.00 | 6 |
| Hippo-Orcho | 2.00 | 3 |
| Oradarea bidentata | 2.00 | 3 |
| Serolis sp. | 2.00 | 3 |
| Plakarthrium puncattissimum | 2.00 | 5 |
| Hemiarthrum setulosum | 2.00 | 3 |
| Zooplankton | 2.00 | 17 |
| Callophyllis atrosanguinea | 1.00 | 1 |
| Curdiea racovitzae | 1.00 | 3 |
| Georgiella confluens | 1.00 | 3 |
| Gigartina skottsbergii | 1.00 | 5 |
| Iridaea cordata | 1.00 | 5 |
| Myriogramme manginii | 1.00 | 2 |
| Neuroglossum delesseriae | 1.00 | 1 |
| Palmaria decipiens | 1.00 | 9 |
| Pantoneura plocamioides | 1.00 | 1 |
| Picconiella plumosa | 1.00 | 1 |
| Plocamium cartilagineum | 1.00 | 4 |
| Pyropia plocamiestris | 1.00 | 1 |
| Trematocarpus antarcticus | 1.00 | 1 |
| Adenocystis utricularis | 1.00 | 3 |
| Ascoseira mirabilis | 1.00 | 3 |
| Desmarestia anceps | 1.00 | 2 |
| Desmarestia antarctica | 1.00 | 3 |
| Desmarestia menziesii | 1.00 | 5 |
| Geminocarpus geminatus | 1.00 | 2 |
| Phaeurus antarcticus | 1.00 | 3 |
| Lambia antarctica | 1.00 | 1 |
| Monostroma hariotii | 1.00 | 3 |
| Urospora penicilliformis | 1.00 | 1 |
| Ulothrix sp. | 1.00 | 1 |
| Epiphytes diatoms | 1.00 | 8 |
| Benthic diatoms | 1.00 | 15 |
| Phytoplankton | 1.00 | 16 |
| Aged detritus | 1.00 | 5 |
| Squids | 1.00 | 3 |
| Fresh detritus | 1.00 | 12 |
| Necromass | 1.00 | 9 |
References
- Seddon, A. W.; Macias-Fauria, M.; Long, P. R.; Benz, D.; Willis, K. J. Sensitivity of global terrestrial ecosystems to climate variability. Nature 2016, 531, 229–232. [Google Scholar] [CrossRef] [PubMed]
- Nolan, C.; Overpeck, J. T.; Allen, J. R.; Anderson, P. M.; Betancourt, J. L.; Binney, H. A. ; ... Jackson, S. T. Past and future global transformation of terrestrial ecosystems under climate change. Science 2018, 361, 920–923. [Google Scholar] [CrossRef] [PubMed]
- Hoegh-Guldberg, O.; Bruno, J. F. The impact of climate change on the world’s marine ecosystems. Science 2010, 328, 1523–1528. [Google Scholar] [CrossRef] [PubMed]
- Doney, S. C.; Ruckelshaus, M.; Emmett Duffy, J.; Barry, J. P.; Chan, F.; English, C. A. ; ... Talley, L. D. Climate change impacts on marine ecosystems. Annu. Rev. Mar. Science 2012, 4, 11–37. [Google Scholar] [CrossRef] [PubMed]
- Heleno, R. H.; Ripple, W. J.; Traveset, A. Scientists' warning on endangered food webs. Web Ecol. 2020, 20, 1–10. [Google Scholar] [CrossRef]
- Cahill, A. E.; Aiello-Lammens, M. E.; Fisher-Reid, M. C.; Hua, X. , Karanewsky, C. J.; Yeong Ryu, H.; ... Wiens, J. J. How does climate change cause extinction? Proc. R. Soc. Ser. B Biol. Sci. 2013, 280, 20121890. [Google Scholar] [CrossRef] [PubMed]
- Mastrantonis, S.; Craig, M. D.; Renton, M.; Kirkby, T.; Hobbs, R. J. Climate change indirectly reduces breeding frequency of a mobile species through changes in food availability. Ecosphere 2019, 10, e02656. [Google Scholar] [CrossRef]
- Hirt, M. R.; Barnes, A. D.; Gentile, A. , Pollock, L. J., Rosenbaum, B.; Thuiller, W.; ... Brose, U. Environmental and anthropogenic constraints on animal space use drive extinction risk worldwide. Ecol. Lett. 2021, 24, 2576–2585. [Google Scholar] [CrossRef] [PubMed]
- Chichorro, F.; Juslén, A.; Cardoso, P. A review of the relation between species traits and extinction risk. Biol. Conserv. 2019, 237, 220–229. [Google Scholar] [CrossRef]
- Albert, R.; Jeong, H.; Barabasi, A. L. Error and attack tolerance of complex networks. Nature 2000, 409, 542–542. [Google Scholar] [CrossRef]
- Sole, R. V.; Montoya, M. Complexity and fragility in ecological networks. Proc. R. Soc. Ser. B Biol. Sci. 2001, 268, 2039–2045. [Google Scholar] [CrossRef] [PubMed]
- Dunne, J. A.; Williams, R. J.; Martinez, N. D. Network Topology and Species Loss in Food Webs: Robustness Increases with Connectance. In SFI Working Papers; Santa Fe Institute: Santa Fe, NM 87501, USA, 2002. [Google Scholar]
- Dunne, J. A.; Williams, R. J.; Martinez, N. D. Network structure and robustness of marine food webs. Mar. Ecol. Prog. Ser. 2004, 273, 291–302. [Google Scholar] [CrossRef]
- Srinivasan, U. T.; Dunne, J. A.; Harte, J.; Martinez, N. D. Response of complex food webs to realistic extinction sequences. Ecology 2007, 88, 671–682. [Google Scholar] [CrossRef]
- Riede, J. O.; Binzer, A.; Brose, U.; de Castro, F.; Curtsdotter, A.; Rall, B. C.; Eklöf, A. Size-based food web characteristics govern the response to species extinctions. Basic Appl. Ecol. 2011, 12, 581–589. [Google Scholar] [CrossRef]
- Carscallen, W. M. A.; Romanuk, T. N. Structure and robustness to species loss in Arctic and Antarctic ice-shelf meta-ecosystem webs. Ecol. Modell. 2012, 245, 208–218. [Google Scholar] [CrossRef]
- Donohue, I.; Petchey, O. L.; Kéfi, S.; Génin, A.; Jackson, A. L.; Yang, Q.; O'Connor, N. E. Loss of predator species, not intermediate consumers, triggers rapid and dramatic extinction cascades. Global Change Biol. 2012, 23, 2962–2972. [Google Scholar] [CrossRef] [PubMed]
- Keyes, A. A.; McLaughlin, J. P.; Barner, A. K.; Dee, L. E. An ecological network approach to predict ecosystem service vulnerability to species losses. Nat. Commun. 2021, 12, 1586. [Google Scholar] [CrossRef]
- Bellingeri, M.; Bodini, A. Threshold extinction in food webs. Theor. Ecol. 2013, 6, 143–152. [Google Scholar] [CrossRef]
- Cordone, G.; Marina, T. I.; Salinas, V.; Doyle, S. R.; Saravia, L. A.; Momo, F. R. Effects of macroalgae loss in an Antarctic marine food web: applying extinction thresholds to food web studies. PeerJ 2018, 6, e5531. [Google Scholar] [CrossRef]
- Dunne, J. A.; Williams, R. J. Cascading extinctions and community collapse in model food webs. Philos. Trans. R. Soc. London, Ser. B 2009, 364, 1711–1723. [Google Scholar] [CrossRef]
- Montoya, J. M.; Pimm, S. L.; Solé, R. V. Ecological networks and their fragility. Nature 2006, 442, 259–264. [Google Scholar] [CrossRef] [PubMed]
- Hastings, A.; McCann, K. S.; de Ruiter, P. C. Introduction to the special issue: theory of food webs. Theor. Ecol. 2016, 9, 1–2. [Google Scholar] [CrossRef]
- Barnes, A. D.; Jochum, M.; Lefcheck, J. S.; Eisenhauer, N.; Scherber, C.; O’Connor, M. I. ; ... Brose, U. Energy flux: the link between multitrophic biodiversity and ecosystem functioning. Trends Ecol. Evol. 2018, 33, 186–197. [Google Scholar] [CrossRef] [PubMed]
- Momo, F. R.; Cordone, G.; Marina, T. I.; Salinas, V.; Campana, G. L.; Valli, M. A. ; ... Saravia, L. A. Seaweeds in the Antarctic Marine Coastal Food Web. In Antarctic Seaweeds: Diversity, Adaptation and Ecosystem Services, 1st ed.; Gómez, I., Huovinen, P., Eds.; Springer Cham: Springer Nature, Switzerland AG, 2020; Volume 1, pp. 293–307. ISBN 978-3-030-39447-9. [Google Scholar]
- Allesina, S.; Bodini, A. Who dominates whom in the ecosystem? Energy flow bottlenecks and cascading extinctions. J. Theor. Biol. 2004, 230, 351–358. [Google Scholar] [CrossRef] [PubMed]
- Bascompte, J. Disentangling the web of life. Science 2009, 325, 416–419. [Google Scholar] [CrossRef] [PubMed]
- Eayrs, C.; Li, X.; Raphael, M. N.; Holland, D. M. Rapid decline in Antarctic Sea ice in recent years hints at future change. Nat. Geosci. 2012, 14, 460–464. [Google Scholar] [CrossRef]
- Rantanen, M.; Karpechko, A. Y.; Lipponen, A.; Nordling, K.; Hyvärinen, O.; Ruosteenoja, K. ; ... Laaksonen, A. The Arctic has warmed nearly four times faster than the globe since 1979. Commun. Earth Environ. 2022, 3, 168. [Google Scholar] [CrossRef]
- Constable, A. J.; Harper, S.; Dawson, J.; Holsman, K.; Mustonen, T.; Piepenburg, D.; ... Van Dam, B. Cross-chapter paper 6: polar regions. In Climate Change 2022: Impacts, adaptation and vulnerability; Contribution of the WGII to the 6th assessment report of the intergovernmental panel on climate change, IPCC AR WGII; Cambridge University Press, 2022. [Google Scholar]
- Ives, A. R.; Cardinale, B. J. Food-web interactions govern the resistance of communities after non-random extinctions. Nature 2004, 429, 174–177. [Google Scholar] [CrossRef] [PubMed]
- Thébault, E.; Huber, V.; Loreau, M. Cascading extinctions and ecosystem functioning: contrasting effects of diversity depending on food web structure. Oikos 2007, 116, 163–173. [Google Scholar] [CrossRef]
- Sanders, D.; Thébault, E.; Kehoe, R.; Frank van Veen, F. J. Trophic redundancy reduces vulnerability to extinction cascades. PNAS 2018, 115, 2419–2424. [Google Scholar] [CrossRef]
- Kehoe, R.; Frago, E.; Sanders, D. Cascading extinctions as a hidden driver of insect decline. Ecol. Entomol. 2021, 46, 743–756. [Google Scholar] [CrossRef]
- Pimm, S. L. The complexity and stability of ecosystems. Nature 1984, 307, 321–326. [Google Scholar] [CrossRef]
- Allesina, S.; Bodini, A.; Bondavalli, C. Secondary extinctions in ecological networks: bottlenecks unveiled. Ecol. Modell. 2006, 194, 150–161. [Google Scholar] [CrossRef]
- Bellingeri, M.; Vincenzi, S. Robustness of empirical food webs with varying consumer's sensitivities to loss of resources. J. Theor. Biol. 2013, 333, 18–26. [Google Scholar] [CrossRef] [PubMed]
- Berg, S.; Pimenov, A.; Palmer, C.; Emmerson, M.; Jonsson, T. Ecological communities are vulnerable to realistic extinction sequences. Oikos 2015, 124, 486–496. [Google Scholar] [CrossRef]
- Ávila-Thieme, M. I.; Corcoran, D.; Pérez-Matus, A.; Wieters, E. A.; Navarrete, S. A.; Marquet, P. A.; Valdovinos, F. S. Alteration of coastal productivity and artisanal fisheries interact to affect a marine food web. Sci. Rep. 2021, 11, 1765. [Google Scholar] [CrossRef] [PubMed]
- Calizza, E.; Costantini, M. L.; Rossi, L. Effect of multiple disturbances on food web vulnerability to biodiversity loss in detritus-based systems. Ecosphere 2015, 6, 1–20. [Google Scholar] [CrossRef]
- Mendonça, V.; Madeira, C.; Dias, M.; Flores, A. A.; Vinagre, C. Robustness of temperate versus tropical food webs: comparing species trait-based sequential deletions. Mar. Ecol. Prog. Ser. 2022, 691, 19–28. [Google Scholar] [CrossRef]
- Mestre, F.; Rozenfeld, A.; Araújo, M. B. Human disturbances affect the topology of food webs. Ecol. Lett. 2022, 25, 2476–2488. [Google Scholar] [CrossRef]
- Mérillet, L.; Robert, M.; Hernvann, P. Y.; Pecuchet, L.; Pavoine, S.; Mouchet, M. ; ... Kopp, D. Effects of life-history traits and network topological characteristics on the robustness of marine food webs. Global Ecol. Conserv. 2022, 34, e02048. [Google Scholar] [CrossRef]
- Bers, A. V.; Momo, F.; Schloss, I. R.; Abele, D. Analysis of trends and sudden changes in long-term environmental data from King George Island (Antarctica): relationships between global climatic oscillations and local system response. Clim. Change 2013, 116, 789–803. [Google Scholar] [CrossRef]
- Lagger, C.; Nime, M.; Torre, L.; Servetto, N.; Tatián, M.; Sahade, R. Climate change, glacier retreat and a new ice-free island offer new insights on Antarctic benthic responses. Ecography 2018, 41, 579–591. [Google Scholar] [CrossRef]
- Barlett, E. R.; Sierra, M. E.; Costa, A. J.; Tosonotto, G. V. Interannual variability of hydrographic properties in Potter Cove during summers between 2010 and 2017. Antarct. Sci. 2021, 33, 281–300. [Google Scholar] [CrossRef]
- Pasotti, F.; Manini, E.; Giovannelli, D.; Wölfl, A. C.; Monien, D.; Verleyen, E. ; ... Vanreusel, A. Antarctic shallow water benthos in an area of recent rapid glacier retreat. Mar. Ecol. 2015, 36, 716–733. [Google Scholar] [CrossRef]
- Sahade, R.; Lagger, C.; Torre, L.; Momo, F.; Monien, P.; Schloss, I. ; ... Abele, D. Climate change and glacier retreat drive shifts in an Antarctic benthic ecosystem. Sci. Adv. 2015, 1, e1500050. [Google Scholar] [CrossRef] [PubMed]
- Garcia, M. D.; Severini, M. D. F.; Spetter, C.; Abbate, M. C. L.; Tartara, M. N.; Nahuelhual, E. G. ; ... Hoffmeyer, M. S. Effects of glacier melting on the planktonic communities of two Antarctic coastal areas (Potter Cove and Hope Bay) in summer. Reg. Stud. Mar. Sci. 2019, 30, 100731. [Google Scholar] [CrossRef]
- Hoffmann, R.; Al-Handal, A. Y.; Wulff, A.; Deregibus, D.; Zacher, K.; Quartino, M. L. ; ... Braeckman, U.Implications of glacial melt-related processes on the potential primary production of a microphytobenthic community in Potter Cove (Antarctica). Front. Mar. Sci. 2019, 6, 655. [Google Scholar] [CrossRef]
- Marina, T. I.; Salinas, V.; Cordone, G.; Campana, G.; Moreira, E.; Deregibus, D. ; ... Momo, F. R. The food web of Potter Cove (Antarctica): complexity, structure and function. Estuarine Coastal Shelf Sci. 2018, 200, 141–151. [Google Scholar] [CrossRef]
- Briand, F.; Cohen, J. E. Community food webs have scale-invariant structure. Nature 1984, 307, 264–267. [Google Scholar] [CrossRef]
- Martinez, N. D. Artifacts or attributes? Effects of resolution on the Little Rock Lake food web. Ecol. Monogr. 1991, 61, 367–392. [Google Scholar] [CrossRef]
- Eklöf, A.; Tang, S.; Allesina, S. Secondary extinctions in food webs: a Bayesian network approach. Methods Ecol. Evol. 2013, 4, 760–770. [Google Scholar] [CrossRef]
- Martinez, N. D. Constant connectance in community food webs. Am. Nat. 1992, 139, 1208–1218. [Google Scholar] [CrossRef]
- Vermaat, J. E.; Dunne, J. A.; Gilbert, A. J. Major dimensions in food-web structure properties. Ecology 2009, 90, 278–282. [Google Scholar] [CrossRef] [PubMed]
- Poisot, T.; Gravel, D. When is a network complex? Connectance drives degree distribution and emerging network properties. PeerJ Preprints, 2013, 1, e50v1. [Google Scholar] [CrossRef]
- Newman, M. E.; Girvan, M. Finding and evaluating community structure in networks. Phys. Rev. E 2004, 69, 026113. [Google Scholar] [CrossRef]
- Fortuna, M. A.; Stouffer, D. B.; Olesen, J. M.; Jordano, P.; Mouillot, D.; Krasnov, B. R. ; ... Bascompte, J. Nestedness versus modularity in ecological networks: two sides of the same coin? J. Anim. Ecol. 2010, 811–817. [Google Scholar] [CrossRef] [PubMed]
- Grilli, J.; Rogers, T.; Allesina, S. Modularity and stability in ecological communities. Nat. Commun. 2016, 7, 12031. [Google Scholar] [CrossRef]
- Allesina, S.; Pascual, M. Network structure, predator–prey modules, and stability in large food webs. Theor. Ecol. 2008, 1, 55–64. [Google Scholar] [CrossRef]
- Pimm, S. L.; Lawton, J. H. Number of trophic levels in ecological communities. Nature 1977, 268, 329–331. [Google Scholar] [CrossRef]
- Pimm, S. L. Food webs. In Food Webs. Population and Community Biology; Springer: Dordrecht, The Netherlands, 1982; pp. 1–11. ISBN 978-94-009-5927-9. [Google Scholar]
- Moore, J. C.; McCann, K.; de Ruiter, P. C. Modeling trophic pathways, nutrient cycling, and dynamic stability in soils. Pedobiologia 2005, 49, 499–510. [Google Scholar] [CrossRef]
- Csardi, G.; Nepusz, T. The igraph software package for complex network research. InterJournal, complex systems 2006, 1695, 1–9. [Google Scholar]
- Saravia, L. A.; Marina, T. I.; Kristensen, N. P.; De Troch, M.; Momo, F. R. Ecological network assembly: how the regional metaweb influences local food webs. J. Anim. Ecol. 2022, 91, 630–642. [Google Scholar] [CrossRef]
- Borrvall, C.; Ebenman, B.; Tomas Jonsson, T. J. Biodiversity lessens the risk of cascading extinction in model food webs. Ecol. Lett. 2000, 3, 131–136. [Google Scholar] [CrossRef]
- Myers, R. A.; Baum, J. K.; Shepherd, T. D.; Powers, S. P.; Peterson, C. H. Cascading effects of the loss of apex predatory sharks from a coastal ocean. Science 2007, 315, 1846–1850. [Google Scholar] [CrossRef]
- Murphy, G. E.; Romanuk, T. N.; Worm, B. Cascading effects of climate change on plankton community structure. Ecol. Evol. 2020, 10, 2170–2181. [Google Scholar] [CrossRef] [PubMed]
- Paine, R. T. Food web complexity and species diversity. Am. Nat. 1966, 100, 65–75. [Google Scholar] [CrossRef]
- Paine, R. T. A note on trophic complexity and community stability. Am. Nat. 1969, 103, 91–93. [Google Scholar] [CrossRef]
- Wu, J.; Liu, Y.; Sui, H.; Xu, B.; Zhang, C.; Ren, Y.; Xue, Y. Using network analysis to identify keystone species in the food web of Haizhou Bay, China. Mar. Freshwater Res. 2019, 71, 469–481. [Google Scholar] [CrossRef]
- Gouveia, C.; Móréh, Á.; Jordán, F. Combining centrality indices: maximizing the predictability of keystone species in food webs. Ecol. Indic. 2021, 126, 107617. [Google Scholar] [CrossRef]
- Delmas, E.; Besson, M.; Brice, M. H.; Burkle, L. A.; Dalla Riva, G. V.; Fortin, M. J. ; ... Poisot, T. Analysing ecological networks of species interactions. Biol. Rev. 2019, 94, 16–36. [Google Scholar] [CrossRef]
- Cirtwill, A. R.; Dalla Riva, G. V.; Gaiarsa, M. P.; Bimler, M. D.; Cagua, E. F.; Coux, C.; Dehling, D. M. A review of species role concepts in food webs. Food Webs 2018, 16, e00093. [Google Scholar] [CrossRef]
- Baum, J. K.; Worm, B. Cascading top-down effects of changing oceanic predator abundances. J. Anim. Ecol. 2009, 78, 699–714. [Google Scholar] [CrossRef]
- Lynam, C. P.; Llope, M.; Möllmann, C.; Helaouët, P.; Bayliss-Brown, G. A.; Stenseth, N. C. Interaction between top-down and bottom-up control in marine food webs. PNAS 2017, 114, 1952–1957. [Google Scholar] [CrossRef]
- Rodriguez, I. D.; Marina, T. I.; Schloss, I. R.; Saravia, L. A. Marine food webs are more complex but less stable in sub-Antarctic (Beagle channel, Argentina) than in Antarctic (Potter cove, Antarctic peninsula) regions. Mar. Environ. Res. 2022, 174, 105561. [Google Scholar] [CrossRef]
- Harrison, W. G.; Cota, G. F. Primary production in polar waters: relation to nutrient availability. Polar Res. 1991, 10, 87–104. [Google Scholar] [CrossRef]
- Tremblay, JÉ. ; Robert, D.; Varela, D.E. et al. Current state and trends in Canadian Arctic marine ecosystems: I. Primary production. Clim. Change 2012, 115, 161–178. [Google Scholar] [CrossRef]
- Pinkerton, M. H.; Boyd, P. W.; Deppeler, S.; Hayward, A.; Höfer, J.; Moreau, S. Evidence for the impact of climate change on primary producers in the Southern Ocean. Front. Ecol. Evol. 2021, 9, 592027. [Google Scholar] [CrossRef]
- Amsler, C. D.; Rowley, R. J.; Laur, D. R.; Quetin, L. B.; Ross, R. M. Vertical distribution of Antarctic peninsular macroalgae: cover, biomass and species composition. Phycologia 1995, 34, 424–430. [Google Scholar] [CrossRef]
- Wiencke, C.; Clayton, M. N.; Gómez, I.; Iken, K.; Lüder, U. H.; Amsler, C. D. ; ... Dunton, K. Life strategy, ecophysiology and ecology of seaweeds in polar waters. Rev. Environ. Sci. Bio/Technol. 2007, 6, 95–126. [Google Scholar] [CrossRef]
- Wiencke, C.; Amsler, C. D. Seaweeds and their communities in polar regions. In Seaweed biology. Ecological Studies; Wiencke, C., Bischof, K., Eds.; Springer Berlin Heidelberg: Berlin, Germany, 2012; Volume 219, pp. 265–291. ISBN 978-3-642-28451-9. [Google Scholar]
- Quartino, M. L.; Saravia, L. A.; Campana, G. L.; Deregibus, D.; Matula, C. V.; Boraso, A. L.; Momo, F. R. Production and biomass of seaweeds in newly ice-free areas: implications for coastal processes in a changing Antarctic environment. In Antarctic Seaweeds: Diversity, Adaptation and Ecosystem Services, 1st ed.; Gómez, I., Huovinen, P., Eds.; Springer Cham: Springer Nature, Switzerland AG, 2020; Volume 1, pp. 155–171. ISBN 978-3-030-39447-9. [Google Scholar]
- Cordone, G.; Salinas, V.; Marina, T. I.; Doyle, S. R.; Pasotti, F.; Saravia, L. A.; Momo, F. R. Green vs brown food web: Effects of habitat type on multidimensional stability proxies for a highly-resolved Antarctic food web. Food Webs 2020, 25, e00166. [Google Scholar] [CrossRef]
- Salinas, V.; Marina, T. I.; Cordone, G.; Momo, F. R. Ecological networks of an Antarctic ecosystem: a full description of non-trophic interactions. Mar. Biol. 2023, 170, 9. [Google Scholar] [CrossRef]
- Petchey, O. L.; Beckerman, A. P.; Riede, J. O.; Warren, P. H. Size, foraging, and food web structure. PNAS 2008, 105, 4191–4196. [Google Scholar] [CrossRef] [PubMed]
- Yonatan, Y.; Amit, G.; Friedman, J.; Bashan, A. Complexity–stability trade-off in empirical microbial ecosystems. Nat. Ecol. Evol. 2022, 6, 693–700. [Google Scholar] [CrossRef] [PubMed]
- van Altena, C.; Hemerik, L.; de Ruiter, P. C. Food web stability and weighted connectance: the complexity-stability debate revisited. Theor. Ecol. 2016, 9, 49–58. [Google Scholar] [CrossRef]
- Eskuche-Keith, P.; Hill, S. L.; Hollyman, P.; Taylor, M. L.; O’Gorman, E. J. Trophic structuring of modularity alters energy flow through marine food webs. Front. Mar. Sci. 2023, 9, 1046150. [Google Scholar] [CrossRef]
Figure 1.
Map of Potter Cove, 25 de Mayo/King George Is., where Argentinian scientific Carlini Base is highlighted.
Figure 1.
Map of Potter Cove, 25 de Mayo/King George Is., where Argentinian scientific Carlini Base is highlighted.
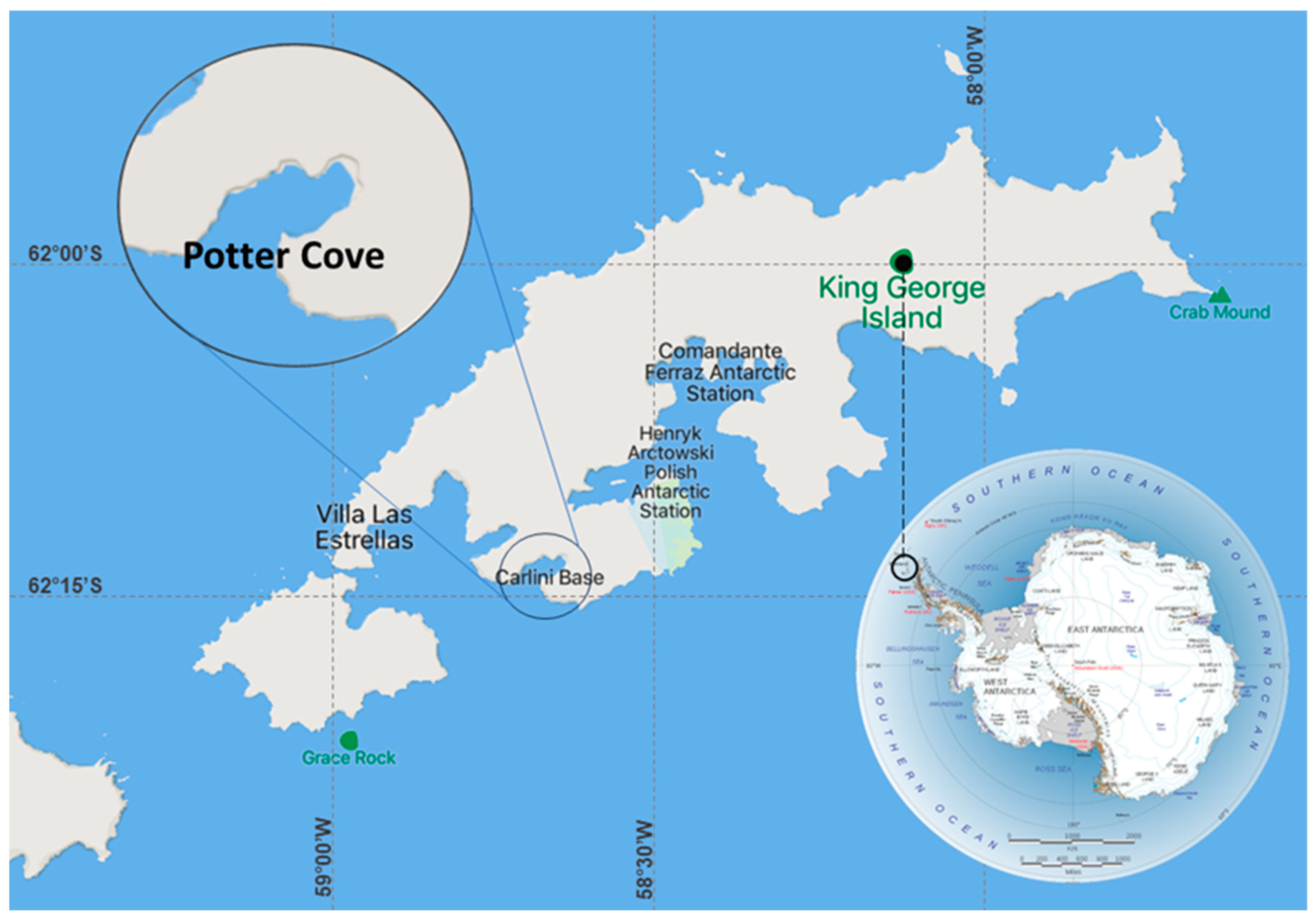
Figure 2.
Connectance changes when removing species by degree (top panels) and trophic level (bottom panels) considering four thresholds on secondary extinctions (0.2, 0.4, 0.6 and 0.8). Black triangle indicates the original connectance (0.037).
Figure 2.
Connectance changes when removing species by degree (top panels) and trophic level (bottom panels) considering four thresholds on secondary extinctions (0.2, 0.4, 0.6 and 0.8). Black triangle indicates the original connectance (0.037).
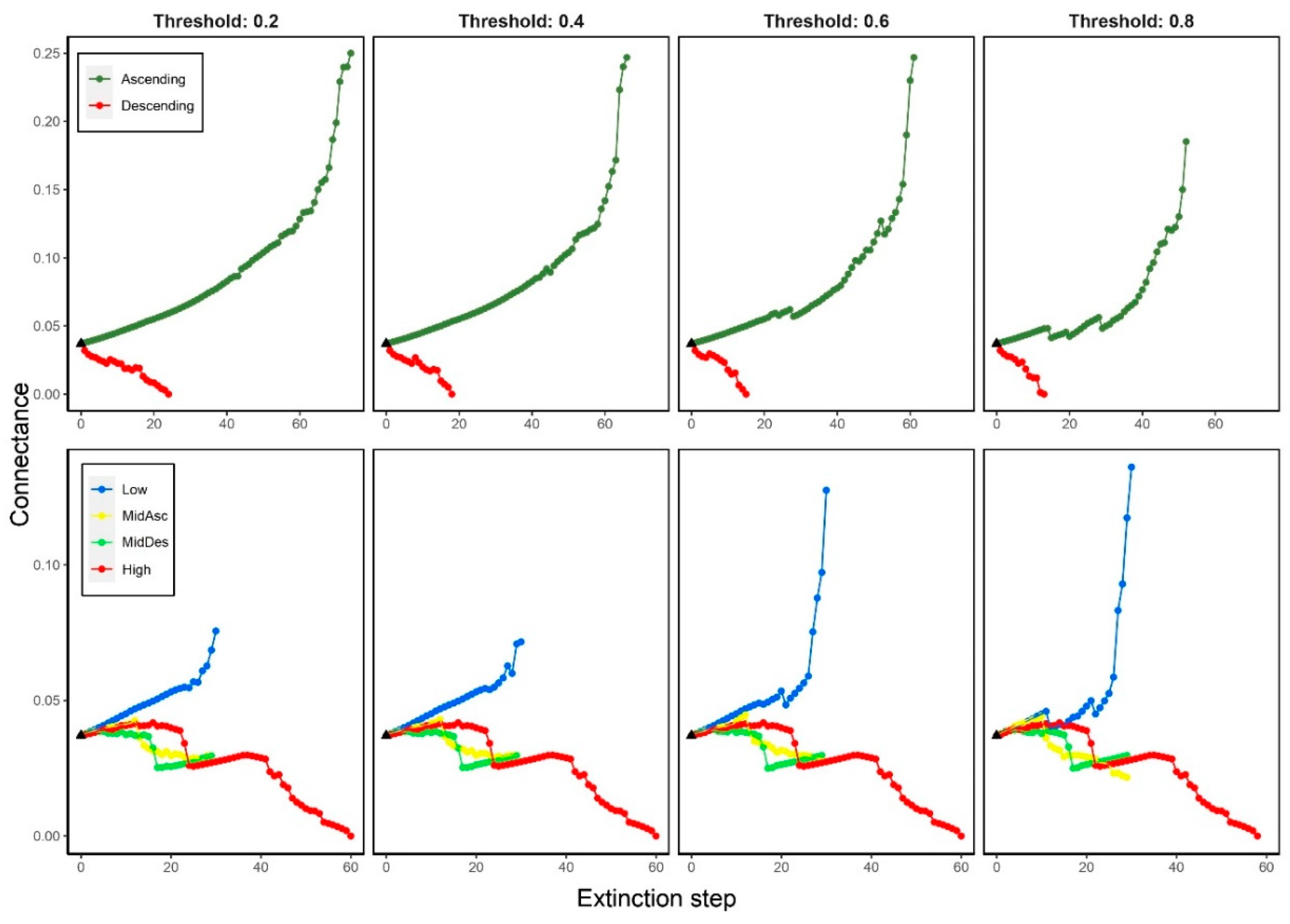
Figure 3.
Modularity changes when removing species by degree (top panels) and trophic level (bottom panels) considering four thresholds on secondary extinctions (0.2, 0.4, 0.6 and 0.8). Black triangle indicates the original modularity (0.37).
Figure 3.
Modularity changes when removing species by degree (top panels) and trophic level (bottom panels) considering four thresholds on secondary extinctions (0.2, 0.4, 0.6 and 0.8). Black triangle indicates the original modularity (0.37).

Figure 4.
Quasi-Sign Stability changes when removing species by degree (top panels) and trophic level (bottom panels) considering four thresholds on secondary extinctions (0.2, 0.4, 0.6 and 0.8). Black triangle indicates the original QSS (2.76).
Figure 4.
Quasi-Sign Stability changes when removing species by degree (top panels) and trophic level (bottom panels) considering four thresholds on secondary extinctions (0.2, 0.4, 0.6 and 0.8). Black triangle indicates the original QSS (2.76).
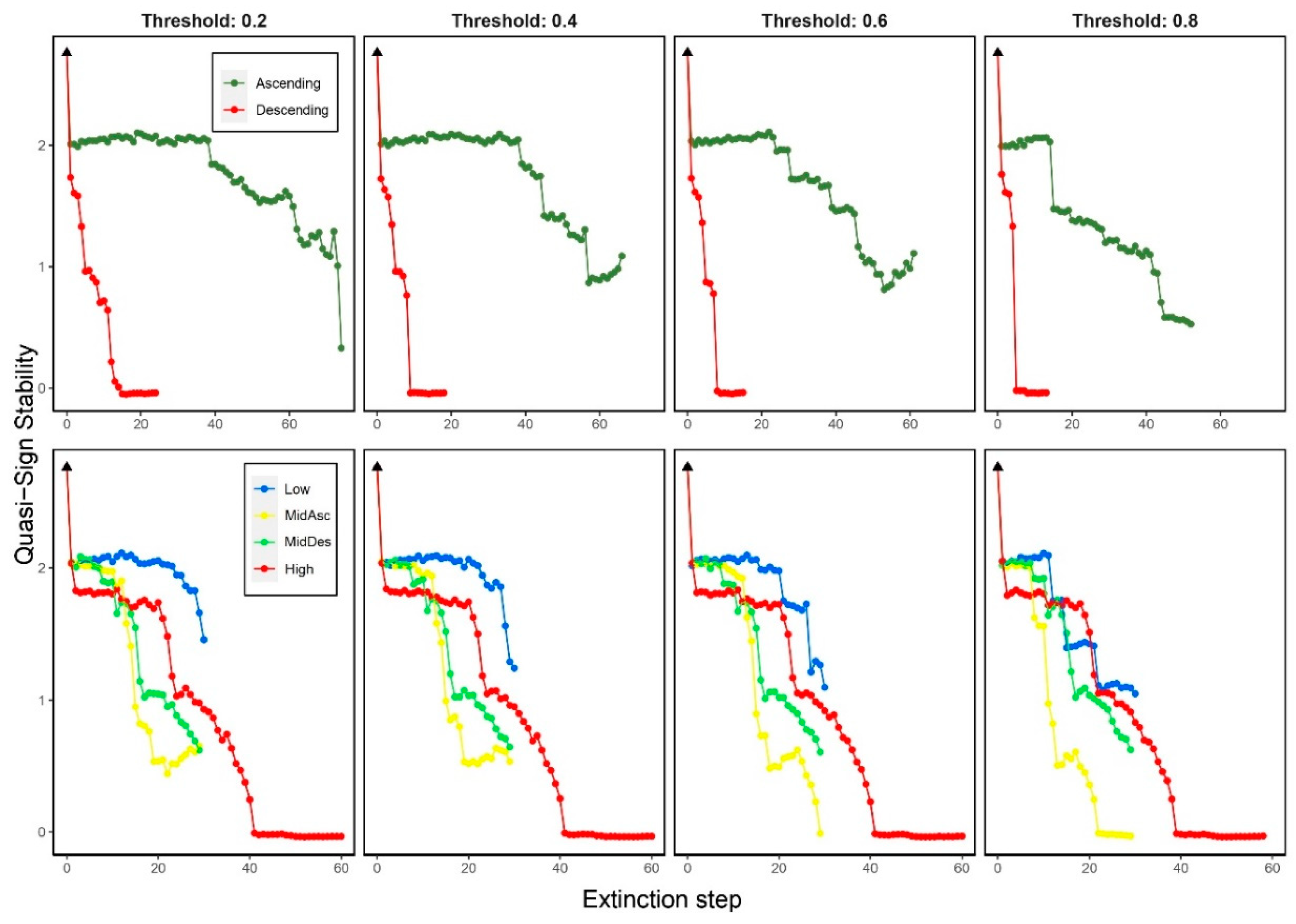
Figure 5.
Cumulative secondary extinctions versus primary extinctions when removing species by degree (top panels) and trophic level (bottom panels) considering four thresholds on secondary extinctions (0.2, 0.4, 0.6 and 0.8).
Figure 5.
Cumulative secondary extinctions versus primary extinctions when removing species by degree (top panels) and trophic level (bottom panels) considering four thresholds on secondary extinctions (0.2, 0.4, 0.6 and 0.8).
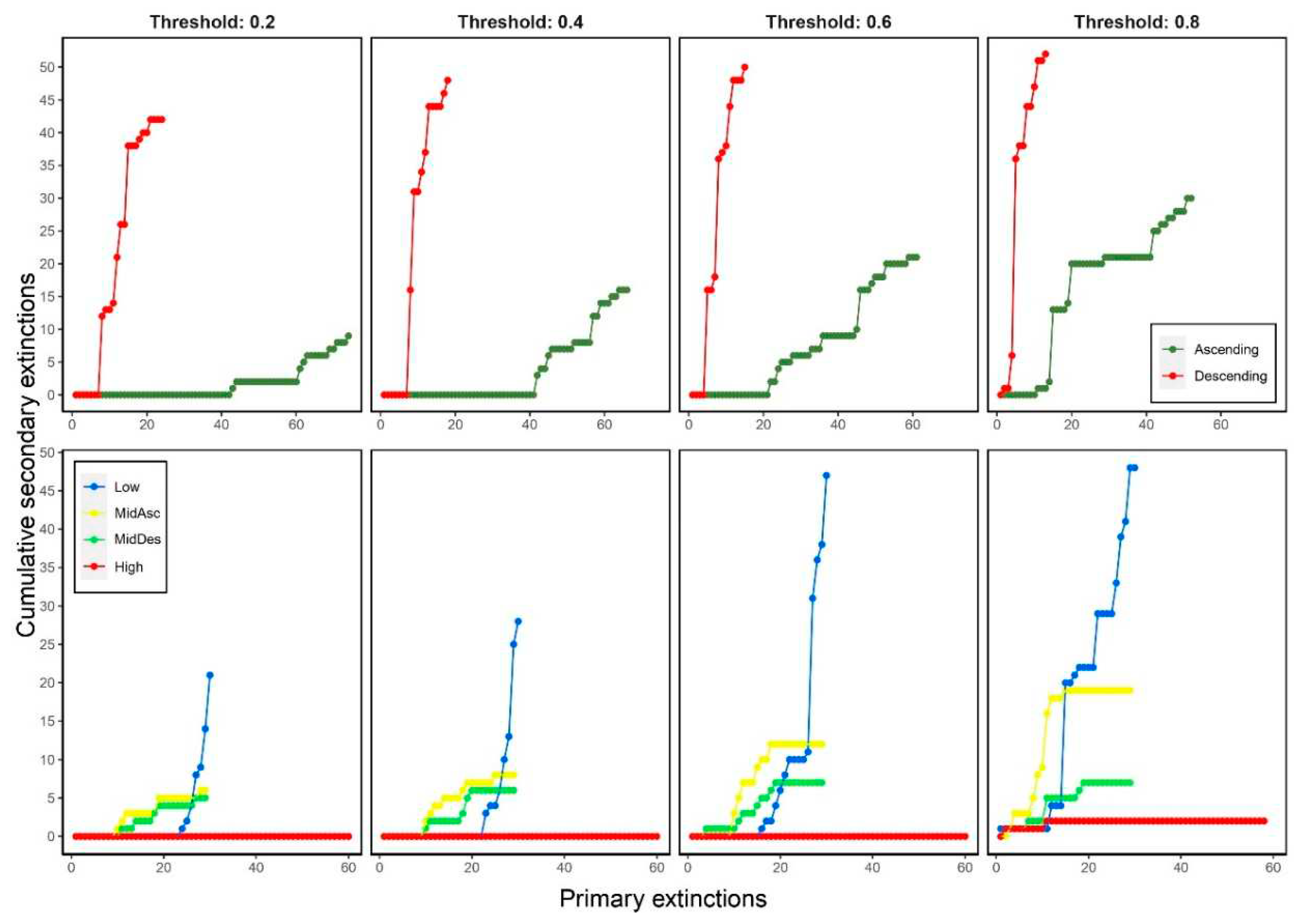
Figure 6.
Modularity versus connectance when removing species by degree (top panels) and trophic level (bottom panels), considering four thresholds on secondary extinctions (0.2, 0.4, 0.6 and 0.8). Black triangle indicates the original connectance (0.04).
Figure 6.
Modularity versus connectance when removing species by degree (top panels) and trophic level (bottom panels), considering four thresholds on secondary extinctions (0.2, 0.4, 0.6 and 0.8). Black triangle indicates the original connectance (0.04).

Figure 7.
Quasi-Sign Stability versus connectance when removing species by degree (top panels) and trophic level (bottom panels), considering four thresholds on secondary extinctions (0.2, 0.4, 0.6 and 0.8). Black triangle indicates the original connectance (0.037).
Figure 7.
Quasi-Sign Stability versus connectance when removing species by degree (top panels) and trophic level (bottom panels), considering four thresholds on secondary extinctions (0.2, 0.4, 0.6 and 0.8). Black triangle indicates the original connectance (0.037).

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Copyright: This open access article is published under a Creative Commons CC BY 4.0 license, which permit the free download, distribution, and reuse, provided that the author and preprint are cited in any reuse.
MDPI Initiatives
Important Links
© 2024 MDPI (Basel, Switzerland) unless otherwise stated








