Quaternion-Spin and Some Consequences

Preprint

Article

Quaternion-Spin and Some Consequences

Altmetrics

Downloads

179

Views

Comments

Bryan Sanctuary^*

This version is not peer-reviewed

Submitted:

19 August 2024

Posted:

21 August 2024

You are already at the latest version

Alerts

Abstract

Changing the symmetry of spin from SU(2) to the quaternion group, $Q_8$, has a number of ramifications. In this paper we first summarize the properties of Q-spin and then discuss some of those resulting changes. Many are counter to well-established ideas which change our view of the microscopic.

Keywords:

Subject: Physical Sciences - Quantum Science and Technology

1. Introduction

In 1925, one hundred years ago,

Schr ö dinger

[1] and Heisenberg, [2] formulated quantum mechanics. In 1927, Hendrik Lorentz organized the Solvay conference with the theme of electrons and photons, [3]. He, and many others, wanted to understand the meaning of wave mechanics. The Einstein-Bohr debates began and became focused in 1935 when EPR [4] published their famous paper. The debate continues today and Lorentz’s theme is just as relevant.

The EPR paradox motivated the development of quaternion, or Q-spin, [5]. Rather than accept Bell’s theorem, which asserts non-locality is necessary to account for the violation of Bell’s Inequalities, (BI), we have shown it is due to the coherent property of spin, its helicity, [5,6,7]. A simulation, [7], confirms this and Bell’s Theorem is disproven by counterexample, [6]. Despite defying rational explanation, however, acceptance of Bell’s Theorem has led to the vast field of quantum information theory which rests upon his classical treatment of correlation. Violation of BI, [8,9,10]is not evidence for non-locality, the reason for 2022 Nobel Prize, but rather evidence for local realism. Entanglement cannot extend over spacetime, and quantum teleportation is unfeasible, [11].

There are, however, several other changes that Q-spin reveals and the purpose of this article is to discuss these relative to our appreciation of the microscopic. Critical to this is a clear description of Q-spin, and in the first sections we attempt a pedagogical explanation. Some of the consequences that follow are as controversial as disproving Bell’s Theorem, because they question well established results. For example, there is no antimatter production from the Dirac equation. This motivates re-examination of his reasoning that was established and accepted almost 100 years ago. We explore these, and other ramification of complexifying the Dirac field.

2. Quaternion Spin

Spin is defined as a point particle of intrinsic angular momentum of magnitude

\frac{1}{2}

, with two polarized states of

|\pm, \hat{n}〉

where

\hat{n}

is a unit vector on the Bloch sphere. It is even to parity, has SU(2) symmetry and is one of the two solutions to the Dirac equation. Call this Dirac spin, [12].

A polarizing field is required to lift the degeneracy in order to form these states,

|\pm, \hat{n}〉

, which are used, for example, to form qubits. In contrast, in the absence of a polarizer, the two states cannot form and they are degenerate. Qubits then don’t exist. We call a spin in the absence of a polarizing field, a free-flight spin. As shown elsewhere, [5,6,7], its properties are crucial to resolving the EPR paradox, and several other problems within the foundations of physics. Generally, we change the symmetry from SU(2) to the quaternion group

Q_{8}

, and the spin that emerges carries the property of coherence in addition to polarization. We call this quaternion, or Q-spin.

The well known expression for the geometric product of two Pauli spin components is, [13],

σ_{i} σ_{j} = δ_{i j} + ε_{i j k} i σ_{k}

(1)

The first term is symmetric and leads to the polarized states,

|\pm, \hat{n}〉

, and the second is anti-symmetric, in terms of the Levi-Civita tensor and a bivector,

i σ

, which leads to the helicity. The two terms in Eq.(1) are complementary, by which we mean they are mutually exclusive.

There is no bivector in the Dirac Equation, and introducing one is the only change made from the usual approach. To do this, one gamma matrix is multiplied by the imaginary number, i,

γ^{2} \to i γ_{s}^{2} \equiv {\tilde{γ}}_{s}^{2}

. This renders the Dirac equation non-Hermitian, and complexifies spin spacetime (subscript s), [5].

we us The gamma matrices represent the Dirac field in Minkowski space, which is a four dimensional real manifold where we live and observe. It is our Lab Fixed Frame, LFF, with dimensions of time and three spacial components,

(β = c t, X, Y, Z)

. The signature, or Clifford algebra is

C ℓ_{1, 3}

. Each Q-spin has its own Body Fixed Frame, BFF, called spin spacetime denoted by,

(β_{s}, e_{1}, e_{2}, e_{3})

. It is a boost and rotation away from Minkowski space.

Spin spacetime is governed by the non-Hermitian Dirac equation, which, due to the bivector, has algebra of

C ℓ_{2, 2}

. The gamma matrices,

(γ^{0}, γ^{1}, γ^{2}, γ^{3})

are changed

(γ_{s}^{0}, γ_{s}^{1}, \pm {\tilde{γ}}_{s}^{2}, γ_{s}^{3})

in spin spacetime. Notice there are two gamma fields

(\pm)

, due to the non-Hermiticity. These are related by complex conjugation.

The bivector complexifies the Dirac field and extends spin beyond our real LFF space. The underlying motivation behind Twistor Theory, [14,15], is Nature is fundamentally complex and we live in the real part. The results obtained here support this idea. Indeed the bivector leads directly to the definition of helicity as the complementary attribute of spin. The definition of spin is changed from a Hermitian Pauli spin vector, to a non-Hermitian complex spin operator which is an element of reality:

σ \to Σ = σ + \underset{̲}{\underset{̲}{h}}

(2)

The geometric helicity is defined by,

\underset{̲}{\underset{̲}{h}} = \underset{̲}{\underset{̲}{\underset{̲}{ε}}} \cdot i σ

(3)

Consider an EPR pair, two spins initially in a singlet state and separate into a product state devoid of entanglement. The correlation, [6], from an entangled state is

E (a, b) = - cos θ_{a b}

with

θ_{a b} = (θ_{a} - θ_{b})

. Dropping the entanglement to give a product state, loses correlation giving the contribution from polarization, (pol),

E {(a, b)}_{p o l} = a \cdot 〈σ^{1}〉 〈σ^{2}〉 \cdot b = - cos θ_{a} cos θ_{b}

. The conclusion follows from Bell’s Theorem that the missing correlation is a result of entanglement that must persists over spacetime. That is non-locality is a property of Nature. However, a similar calculation, [6], gives the contribution from coherence, (coh),

E {(a, b)}_{c o h} = a \cdot 〈{\underset{̲}{\underset{̲}{h}}}^{1}〉 \cdot 〈{\underset{̲}{\underset{̲}{h}}}^{2}〉 \cdot b = - sin θ_{a} sin θ_{b}

. This shows that helicity accounts for the correlation that leads to the violation of BI,

E (a, b) = E {(a, b)}_{p o l} + E {(a, b)}_{c o h}

(4)

which is conserved, [6], upon the separation of an EPR pair into a product state. Non-locality is not needed.

EPR coincidence experiments, [8,9,10], measure correlation from both polarization and coherence. The former is between the two fermion spins of Alice and Bob. The latter is between two boson spins of Alice and Bob. These statements are now explained.

Upon complexifying the Dirac field, the Dirac equation is non-Hermitian, and the two solutions, complex conjugates of each other, are mirror states, [16] and have no parity,

P ψ^{\pm} = ψ^{\mp}

. They are reflective twins, and Dirac interpreted them as a matter-antimatter pair, [17], two spins with two states each.

A further observation is that under parity, the non-Hermitian Dirac equation separates once again into two complementary spaces governed by two distinct equations, see Eqs.(10), (11) of reference [5]. Equation (10) is an even parity 2D Dirac equation which describes a plane formed from the (31) axes. The odd parity Equation (11) is that for a massless Weyl spinor and solves to give a unit quaternion that spins the axis, Y, Figure 1. These two states of opposite parity are formed from the mirror states,

P Ψ^{\pm} = \pm Ψ^{\pm}

, where

Ψ^{\pm} = 1 / \sqrt{2} (ψ^{+} \pm ψ^{-})

Q-spin is one particle in the complex Dirac field with four states: two polarized states of up and down,

|\pm, \hat{n}〉

, and two helicity states of L and R. The two mirror states,

ψ^{\pm}

, describe the two orthogonal spin axes on the same particle, and both these are also orthogonal to the axis of linear momentum. Polarization spacetime now has algebra

C ℓ_{1, 2}

The states that are complementary to the polarization are in coherent space (not spacetime) which is beyond our LFF. It cannot be directly observed. It is the

S^{3}

hypersphere of quaternions. Under parity, the equation for a massless Weyl spinor solves to give a unit quaternion which spins the axis of linear momentum,

Y = e_{2}

The two point-particle Dirac spins becomes one particle with two orthogonal axes,

Σ_{1}

and

Σ_{3}

, shown in Figure 1. Each is a fermion of spin-

\frac{1}{2}

, and each carries a magnetic momentum of

μ

. Therefore, Q-spin is defined only in the disc in the BFF,

Σ_{31} = e_{3} Σ_{3} + e_{1} Σ_{1}

(5)

and the two components couple to form a composite boson which bisects the

e_{3} e_{1}

quadrant. In free-flight, an electron is a boson spin-1,

e_{B}^{-}

, odd to parity, and spinning with two helicity states of L or R. The magnetic moment of the boson is twice that of each fermion axis, being

2 μ

In the presence of a polarizing field,

a

, spin is a unit quaternion,

a \cdot Σ_{13}

, and the two spin axes are no longer indistinguishable. Two general situations arise: if the field lies close to the boson axis, it precesses as a vector with Larmor frequency of

2 μ

. If the field lies close to one of the two fermion spin axes each with Larmor frequency of

μ

, then the boson decouples and one of the spin axes aligns with the external field. These ideas are visualized in Figure 2, L and R panels. If one aligned axis carries state,

|+, \hat{n}〉

, then the other carries the state,

|-, \hat{n}〉

Whether the boson spin remains intact or decouples, depends upon the orientation of the spin in the field, and upon the field strength, relative to the spin-spin coupling within the boson. The EPR paradox is resolved by including the correlation from both polarization and helicity, and the transition between the two. To determine which axis aligns, we use the Least Action Principle that states the axis with the greatest attraction to the field will align.

Q-spin embodies wave-particle duality showing the properties of spin depend upon its environment. The polarization (particle),

σ

, and the coherence (wave),

i σ

are manifest as complementary attributes such that the polarization exists only in an anisotropic environment as a fermion, whereas the coherence exists only in an isotropic environment as a boson,

e_{F}^{-} (anisotropy) \leftrightarrow e_{B}^{-} (isotropy)

(6)

Q-spin gives a boson electron of odd parity in free-flight and a fermion electron of even parity when measured. This is the wave-particle duality.

When we complexified the Dirac field, we did not expect a 2D spinning disc, a World sheet, [18], nor spin being an anyon, [19]. We did not expect that antimatter production by Dirac’s interpretation would be unnecessary.

2.1. Identifying EPR Coherence

In reference [7] it is shown the violation of BI is due to the transition from a boson in free-flight to a fermion when measured. A simulation confirms this and generates the EPR correlation in Figure 3 which plots the correlation between EPR pairs versus their filter angle difference,

θ_{a b}

. The correlation from the usual singlet state,

- cos θ_{a b}

is plotted in Figure 3 which has a CHSH, [8], of

2 \sqrt{2} = 2.828

. If the entanglement is dropped, then so is the coherence. This leaves only a product state displayed as the triangle, with CHSH = 2 which does not violate BI. The difference between the two is the part that violates BI, called the mustache function, with CHSH = 0.828.

The simulation results are also shown on the same figure along with their CHSH values. The simulation algorithm, [7] for the vector polarization has one spinning axis,

σ_{i}, i = 1

or 3, and the bivector has two counter spinning axes,

i σ_{2} = σ_{3} σ_{1}

, but only one aligns. Concisely, when both Alice and Bob are fermions, the correlation contributes to the triangle. When both are bosons, the correlation contributes to the mustache. When one of Alice or Bob is a boson and the other a fermion, then only polarized correlation is possible, [5].

Consider a correlation experiment with Alice and Bob’s filters set to differ by

\frac{π}{4}

. Both spins are anti-correlated as they approach their filters, the two space-like separated. Again, Least Action deterministically predicts the outcomes for both. Since the magnetic moment of the boson is twice that of the fermion, the boson attraction is double that of the fermions. We conclude that the boson persists uncoupled over a larger range than do the fermions, and precesses intact until the field is close to either the

e_{3}

e_{1}

axes. In the process, the boson begins to nutate until finally it decouples.

Figure 4 illustrates the four experiments used in the CHSH experiment, with the filter settings for Alice of

a_{3}

and

a_{1}

, and Bob’s at

\pm c

and

d

. The four

s =

CHSH experiments give correlation of

E (a_{1}, d)

E (a_{1}, c)

E (a_{3}, d)

, and

E (a_{3}, - c)

. Any orientations of these field vectors can be used, but those shown in Figure 4 have the maximum correlation and violates BI. Using the quantum result,

E (a, b) = - a \cdot b

gives,

\begin{matrix} s & = | E (a_{1}, d) + E (a_{1}, c) + E (a_{3}, d) + E (a_{3}, - c) | \\ = a_{1} \cdot (d + c) + a_{3} \cdot (d - c) \\ = \frac{1}{\sqrt{2}} (d + c) \cdot (d + c) + \frac{1}{\sqrt{2}} (d - c) \cdot (d - c) \\ = 2 \sqrt{2} \end{matrix}

(7)

Whereas each of the filter settings of Alice and Bob are fixed in an experiment, the orientations of Q-spin are not. Rather Q-spin impinges on a filter at random orientations,

θ

, determined at the source. Both spins-1 bosons of Alice and Bob are anti-correlated up until they encounter their space-like separated filters. Then, independently, Alice is pulled to her filter direction, and Bob to his. Each decouples either directly to a fermion, or indirectly as a boson, depending on how far each is from its filter.

However, within a 45∘ wedge, both Alice and Bob can simultaneously exist as bosons and therefore they decouple using the boson algorithm. Outside the wedge, a boson starts to directly decouple into fermions, and the product state takes over.

We conclude that in EPR coincidence experiments, Figure 3, as

θ_{a b}

approaches

\frac{π}{4}

both Alice and Bob’s spins precess at

2 μ

as bosons, giving the mustache function. On either side of

\frac{π}{4}

, the spins start to decouple into fermions which precess at

μ

and give the triangle correlation. The bosons precession is shown in Figure 5. A spin makes an angle of

cos χ = \frac{m}{\sqrt{S (S + 1)}}

with the field direction. For a spin-

\frac{1}{2}

, the angle is

54 . 74^{\circ}

while a spin-1 has an angle of

45^{\circ}

, thereby supporting the assertion that the boson is precessing in the field before decoupling and is responsible for the violation.

The classical simulation gives more correlation than from QM. CHSH = 2 (2.027) for polarization and 1 (0.968) for the coherence, giving a total of 3 (2.995). Although neither polarization nor coherence violate BI their sum does because EPR coincidence experiments cannot yet distinguish between coincidences that are from polarization and coherence.

The simulated correlation also violates the Tsirel’son bound, [20]. We assert this is because the quantum calculation uses a singlet state. Note that the classical triangle is reproduced by QM, but the main disagreement is between the simulated and quantum contributions to the coherence. The reason is that the singlet state is an approximation and misses coherent terms. This is shown later in this paper.

The simulation shows that persistent, non-local connectivity between separated EPR pairs is not needed to account for the violation. Rather the missing correlation, given by the mustache function, is due to spin coherence. There is no quantum weirdness.

2.2. EPR Correlation without Non-Locality

Q-spin is defined in Eq.(2), and has two components in the 3 and 1 directions, Eq.(5), in the BBF. To compare with experiment, we must calculate the expectation values,

〈Σ〉 = Tr [Σ ρ]

, in the LFF. For this we choose the pure state operator,

ρ

, to be, [7],

ρ = \frac{1}{2} (I + \frac{1}{\sqrt{2}} (e_{3} + e_{1}) \cdot σ)

(8)

Note this is defined in the BFF and there are no contributions from the complementary quaternions since we are not observing in the

S^{3}

hyperspace. Observation of complementary spaces cannot be simultaneous.

For the two components we find the expectation values are complex,

\begin{matrix} 〈Σ_{1}〉 = \frac{1}{\sqrt{2}} (e_{1} + i e_{3} Y) \\ 〈Σ_{3}〉 = \frac{1}{\sqrt{2}} (e_{3} - i e_{1} Y) \end{matrix}

(9)

where

P_{13} 〈Σ_{1}〉 = {〈Σ_{3}〉}^{*}

, and

P_{13}

a permutation operator, [5]. The BFF vectors are then transformed to the LFF. An advantage of Q-spin is the expectation values are all unit quaternions which are easy to use since a product of quaternions is also a quaternion.

Consider the correlation between an EPR pair approaching their filters, [5],

\begin{matrix} E (a, b) = & a \cdot (〈Σ_{31}^{A}〉 {〈Σ_{31}^{B}〉}^{*} + {〈Σ_{31}^{A}〉}^{*} 〈Σ_{31}^{B}〉) \cdot b \\ = - cos (θ_{a} - θ_{b}) \end{matrix}

(10)

The complex conjugation changes helicity from L to R, and Alice and Bob’s spins have opposite helicities. Also the correlation must be real, so we take into account the two opposite helicity states by the second term. This is similar to forming polarized light from photons. We obtain the full quantum EPR correlation,

E (a, b) = - cos θ_{a b}

, the same as the entangled result, but without any non-local entanglement. The full correlation is obtained from a product state. For this, it is essential that Alice and Bob’s spins both be complex, [14,15], and carry helicity.

2.3. Dirac: No Antimatter

Linearizing the Klein-Gordon equation, as Dirac did, led to his four dimensional gamma matrices defined in Minkowski space,

(γ^{0}, γ^{1}, γ^{2}, γ^{3})

. The necessary requirement is they anticommute. From this field the matter-antimatter pair emerged, and spin formalized. In contrast, we use the spin field represented by

(γ_{s}^{0}, γ_{s}^{1}, {\tilde{γ}}_{s}^{2}, γ_{s}^{3})

. Since they too anticommute, they present an alternate and viable linearization of the Klein-Gordon equation.

A perplexing point for Dirac was the twin spins have opposite, or negative, energies from its partner and these went to minus infinity, [17], which is non-physical. Dirac resolved this by proposing a fermionic continuum of negative energy, and added electrons according to the Pauli principle until the continuum was filled. Holes of antimatter positrons are formed as electrons jump to positive energy. Although this structure might exist in solid state physics, with the bound and conduction bands distinguished, for other systems and single particles, hole theory is a rationalization, not satisfying, and is contrived to resolve a problem.

Since Q-spin has two spins that precess oppositely and in phase, Figure 2, the two energies must be equal and opposite. This interpretation resolves the negative energy problem Dirac encountered. No sea of electrons is needed.

We do not dispute the existence of antimatter, [21,22], but question the Dirac interpretation of its production. Experimentally, only trace amounts are found in the universe from the decay of unstable nuclei. At higher energies, pair particle production also occurs.

If Q-spin was formed at the Big Bang, rather than fermion matter-antimatter pairs, the baryon asymmetry problem is resolved [23].

3. Bell’s Theorem Is Irrelevant

Bell’s Inequalities, [24], show that the correlation between real classical properties is bounded. Noting that QM can violate these inequalities, Bell concluded this can only happen if certain hidden variables account for the violation, however these must be non-local. We accept BI, but reject Bell’s Theorem, [25], which states in his own words,

“If [a hidden-variable theory] is local it will not agree with quantum mechanics, and if it agrees with quantum mechanics it will not be local."

A more formal statement is the impossibility that local hidden variables can reproduce the results of quantum mechanics, with the conclusion that non-locality is a property of Nature. Our work shows that a local theory can violate BI, thereby disproving Bell’s theorem by counterexample, [6].

We point out that in formulating Q-spin, there are no hidden variables, local or not, and consequently there can be no loopholes, nor hidden mechanism to create non-locality. The only variable in this work is the angle

θ

which orients a spin on the Bloch sphere and is a local variable.

Bell only examines classical correlation with BI giving the upper bound. Classical variables are all real; and they form a single commuting convex set. Pure states are extreme points and mixed states are interior points. The proof of Bell’s Theorem is also classical. He used the example for Bertlmann’s different and fading coloured classical socks to show non-local connectivity is needed. Bell had already proven classical correlation is bounded. He then examined under what conditions those classical variables could violate that bound even though he knew no classical correlation could. He arrive, therefore, at the conclusion that the classical hidden variables must be non-local. Since non-locality makes no sense, Bell’s proof should be considered as

r e d u c t i o

a d

a b s u r d u m

,26]. Attention would logically then shift to finding what is missing that gives the quantum violation of BI. Bell, however, did not take that course. Rather he assumed that quantum variables and classical variables have the same properties.

Quantum systems, in stark contrast to the hidden variables of Bell, do not commute; are complex; are complementary; and form two convex sets. All particles are produced in pure states which remain pure until encountering a polarizing field. On the other hand, Q-spin does not violate BI when viewed as two complementary sources of correlation. That is, polarization and coherence are complementary elements of reality. If experiments could separate the two, then the correlation for these would be CHSH = 2 for polarization and CHSH = 1 for coherence. Neither violates BI and each can be considered a separate experiment.

Bell’s theorem is inapplicable to quantum systems. Q-spin shows that few of Bell’s conclusions about QM are correct, [25]. BI are an exception. They quantitatively define the “Infamous Boundary” [27] between the classical and the quantum. For the CHSH form of Bell’s inequalities, the Infamous boundary is 2, [8].

Bell’s work inspired experiments, [8,9,10], which confirmed QM violates BI. This is regarded as proof that local hidden variable theories must be rejected, and established non-locality as a property of Nature, as evidenced by the 2022 Nobel Prize in physics. This conclusion cannot be supported with Q-spin. Although Bell’s work stimulated research into the foundations and philosophy of quantum mechanics, the view of this work is that non-locality has led physics into unfathomable directions which have hindered progress.

There are a number of no-go theorems in QM. In addition to Bell’s Theorem, [25], there is the no-cloning theorem, [28], with its many in-offs, all relying entirely on quantum teleportation, which, as shown below, is unfeasible. The Bell-Kochen-Specker theory, [25,29], discusses quantum contextuality. Although this theorem is useful, it is not applicable to Q-spin since their conclusions are valid for three dimensions or more, and Q-spin is two dimensional.

Q-spin is contextual. The outcomes are not known until the particle has reached its filter and is pulled to

θ_{a}

. Additionally, we deal with pure states only, so there are no compatible observables to influence the outcomes.

Parenthetically, we note in Figure 3, there is a small simulated correlation from the polarizations that violates Bell’s Theorem with a CHSH = 2.027. It is known that classical simulations can violate BI without non-locality, [30,31,32,33].

Despite the wide acceptance of Bell’s theorem, there exist a number of critics who also raise cogent doubts about its applicability and use, see [30,31,32,33,34,35,36,37,38,39,40,41,42,43,44,45].

3.1. Non-Locality vs. Local Realism

An immediate consequence of the inapplicability of Bell’s Theorem is that non-local connectivity is not viable. Out of the myriad descriptions we feel that Wikipedia sums up non-locality the best, [46]:

“The paradox is that a measurement made on either of the particles apparently collapses the state of the entire entangled system—and does so instantaneously, before any information about the measurement result could have been communicated to the other particle ... and hence assured the “proper" outcome of the measurement of the other part of the entangled pair."

Scholarpedia states [47], “Bell’s theorem asserts that if certain predictions of QM are correct then our world is non-local. “Non-local" here means that there exist interactions between events that are too far apart in space and too close together in time for the events to be connected even by signals moving at the speed of light."

Without Bell’s Theorem, these definitions cannot be supported.

The violation of BI now takes on an entirely different meaning. Spin polarization and helicity both simultaneously exist as elements of reality; coincidence experiments are sensitive to both; the experimental violation of Bell’s inequalities confirms local realism. Quantum weirdness, [48], is explained by complex helicity.

The diametrically opposite conclusion is reached in Bell-type experiments of which an enormous literature exists, see

e . g .

“The Big Bell Test" [49]. The paper’s conclusion is incorrect claiming local realism is challenged. Rather their data shows just the opposite: evidence for local realism by the existence of elements of reality that account for the violation.

3.2. Quantum Teleportation Is Unfeasible

Quantum teleportation, [11], depends solely upon long range, non-local, correlation between three entangled particles. The opening paragraph in that paper confirms, “The existence of long range correlations between Einstein-Podolsky-Rosen (EPR) pairs of particles raises the question of their use for information transfer." This statement rests on the now defunct Bell’s Theorem.

Teleportation claims that non-locality is mediated by “Einstein, Podolsky, Rosen Channels", but nowhere are these “channels" formulated and there is no evidence they exist other than Bell’s Theorem. Our work shows they are not needed, and do not exist. In particular the steps up to their Eq.(5), which are critical to quantum teleportation, cannot be justified. This requires swapping entanglement over spacetime separations. When Alice’s spin, 1, is far from Bob’s entangled pair, 23, then the following process is applied which is physically unfeasible:

|ϕ_{1}〉 |Ψ_{23}^{(-)}〉 \overset{unfeasible}{⟷} |Ψ_{12}^{(-)}〉 |φ_{3}〉

(11)

Therefore their Eq.(5) is impossible and the notion of quantum teleportation collapses. Recall Longuet-Higgins [50] made the distinction between mathematical operations and feasible operations, like ammonia, NH₃, and methyl fluoride, FCH₃, both have the possibility of umbrella symmetry, but only for ammonia is it feasible.

The concept of quantum teleportation is unfeasible, along with emerging technologies [51,52,53] [28], that rely it.

The question arises about the results from the above experiments which appear to support non-locality. Quantum cryptography relies on a third party interloper entangling with the communication between Alice and Bob. Using Q-spin, in contrast, any interloper will introduce a local phase change in the helicity, disrupting the transmission, and giving similar outcomes had entanglement persisted. The same approach effects all Bell-type coincidence experiments which rely on non-locality. Examples are entanglement swapping, [54], delayed choice quantum eraser, [55], and loop-hole free Bell tests, [56,57].

3.3. Quantum Computing

The prospects of having large working quantum computers (QC) has not been realized, [58]. Major obstacles have inhibited the engineering development which is motivated by extensive theoretical research showing considerable advantages over classical computation.

However, QC uses quantum teleportation and non-local entanglement. As shown here, this approach is unfeasible and must be abandoned, in favour of controlling

θ

. Another issue is the stability of qubits which decohere. Therefore, much effort is spent on finding suitable two-state quantum systems that can be controlled and are stable.

Presently the use of electrons in circuitry is based upon EM theory. If spin can be controlled, then the two spin components, with opposite magnetic moments, might serve as stable qubits. Consider the qubit form of Q-spin in the BFF,

a \cdot 〈Σ_{31}〉 = a \cdot \frac{1}{\sqrt{2}} (e_{3} exp (+ i \frac{π}{4} Y) + e_{1} exp (- i \frac{π}{4} Y))

(12)

The two axes,

e_{3}

and

e_{1}

have opposite magnetic moments, and these project along the field axis,

\begin{matrix} a \cdot e_{3} = cos (θ_{a} - θ) \\ a \cdot e_{1} = sin (θ_{a} - θ) \end{matrix}

(13)

By controlling

θ_{a}

and

θ

, the experimenter can determine which axis will align with the field, thereby giving a deterministic two bit outcome of

\pm 1

. Helicity maintains the orientational information encoded at the source. Spin is a stable property of particles. We note techniques that use NMR are candidates for QC, [59]. NMR spectroscopy routinely transports polarization and coherence between states, [60], performing spin "gymnastics", [61], and producing echos, [62].

An advantage of Q-spin qubits is the algorithms are products of quaternions.

4. The Singlet State Is Approximate

Entanglement, as Schrödinger famously said [63] is

\underset{̲}{the}

difference from classical systems. Without Bell’s theorem, persistence of entanglement between separated pairs is not possible. Therefore we accept entanglement as a vital property of QM but reject that it persists after separation, [6], to non-local situations.

A singlet state is always expressed by opposing polarized states which are anti-symmetrized,

↑ ↓ - ↓ ↑

, where

|\hat{n}, +〉 = ↑

e t c .

| Ψ_{12}^{-} 〉 = \frac{1}{\sqrt{2}} [{| ↑ 〉}_{1} {| ↓ 〉}_{2} - {| ↓ 〉}_{1} {| ↑ 〉}_{2}]

(14)

We suggest that the

|\hat{n}, \pm〉

states be replaced by Q-spins. Using

|\hat{n}, \pm〉

is an approximation and the reason the Tsirel’son bound, [20], is less than the simulated result of CHSH =3.

We suggest a singlet state of an electron is more realistically expressed as shown in Figure 6 where the two magnetic axes are attracted which are balanced by the repulsion of the charges. The helicity now spins the axis as shown.

To show the missing parts of the quantum form of the singlet state, Eq.(14), first take its outer product, and then separate the state operator,

ρ_{Ψ_{12}^{-}}

, into a sum of eight non-Hermitian product states, [64],

\begin{matrix} ρ_{Ψ_{12}^{-}} = |Ψ_{12}^{-}〉 〈Ψ_{12}^{-}| = \frac{1}{2} \sum_{\begin{matrix} n_{1}, n_{2}, \\ n_{3} = \pm 1 \end{matrix}} [ρ^{1} (n_{1} n_{2} n_{3}) \otimes ρ^{2} {(- n_{1} - n_{2} - n_{3})}^{†}] \\ = \frac{1}{8} \sum_{\begin{matrix} n_{1}, n_{2}, \\ n_{3} = \pm 1 \end{matrix}} {(I^{1} + n_{3} σ_{3}^{1} + n_{1} σ_{1}^{1} + n_{2} i σ_{2}^{1}) \otimes (I^{2} - n_{3} σ_{3}^{2} - n_{1} σ_{1}^{2} - n_{2} i σ_{2}^{2})}^{†} \\ = \frac{1}{4} (I^{1} I^{2} - σ^{1} \cdot σ^{2}) = \frac{1}{2} (\begin{matrix} 0 & 0 & 0 & 0 \\ 0 & 1 & - 1 & 0 \\ 0 & - 1 & 1 & 0 \\ 0 & 0 & 0 & 0 \end{matrix}) \end{matrix}

(15)

The final matrix displays the diagonal polarized states, and the off-diagonal coherent states. This expression leads to the

E (a, b) = - cos θ_{a b}

correlation between the two spins. The off-diagonal coherent terms are responsible for entanglement, [6]. Dropping them gives a product state,

E (a, b) = - cos θ_{a} cos θ_{b}

with no entanglement, [7].

The eight product states in Eq.(15 are the possible ways an EPR pair can separate. Each separates randomly into only one of the eight terms in the above sum which represent states of definite polarization and helicity. One term describe left and the other right helicity for each quadrant. We suggest that after initial separation, each spin settles into a trajectory with the disc of polarization spinning along the axis of linear momentum, see Figure 1, governed by the Intermediate Axis theorem, [65].

Inserting the Pauli spin matrices into Eq.(15), and summing the eight terms reproduces the entangled singlet matrix shown in the last line. In the process, coherent terms cancel, and those, we suggest, are responsible for the difference between QM and the classical simulation.

To demonstrate this consider the first quadrant in Eq.(15) with

(n_{1} = n_{2} = n_{3} = 1)

, giving the tensor product of two

2 \times 2

matrices, one for Alice and one for Bob. They have opposite polarization and helicity. Using these non-Hermitian matrices requires tacit acceptance that polarization and coherence simultaneously exist.

Upon separation, either the left or right handed persists. That is, for each quadrant, at separation, the EPR pair is one of the eight products, for example for the first quadrant,

(n_{1} = n_{3} = 1)

ρ^{1} ρ^{2 †} = (\begin{matrix} 1 & 1 \\ 0 & 0 \end{matrix}) \otimes (\begin{matrix} 0 & 0 \\ - 1 & 1 \end{matrix}) = (\begin{matrix} 0 & 0 & 0 & 0 \\ 0 & 0 & 0 & 0 \\ - 1 & - 1 & 1 & 1 \\ 0 & 0 & 0 & 0 \end{matrix})

(16)

The state with opposite helicity is also possible in the first quadrant, and adding the left

ρ^{1} {ρ^{2}}^{†}

and right

{ρ^{1}}^{†} ρ^{2}

(n_{2} = \pm 1)

matrices, Eq.(15), leads to a Hermitian matrix for each quadrant,

\begin{matrix} ρ (1^{st} quadrant, L and R) = ρ_{1} ρ_{2}^{†} + ρ_{1}^{†} ρ_{2} \\ = (\begin{matrix} 0 & 0 & 0 & 0 \\ 0 & 0 & 0 & 0 \\ - 1 & - 1 & 1 & 1 \\ 0 & 0 & 0 & 0 \end{matrix}) + (\begin{matrix} 0 & 0 & - 1 & 0 \\ 0 & 0 & - 1 & 0 \\ 0 & 0 & 1 & 0 \\ 0 & 0 & 1 & 0 \end{matrix}) = (\begin{matrix} 0 & 0 & - 1 & 0 \\ 0 & 0 & - 1 & 0 \\ - 1 & - 1 & 2 & 1 \\ 0 & 0 & 1 & 0 \end{matrix}) \end{matrix}

(17)

Permutations of

n_{i} s

gives similar matrices for the other three quadrants. Finally summing over the quadrants gives the entangled singlet, Eq.(15). Comparing the final singlet matrix in Eq.(17), shows the outer coherent terms, indicated as bold font, cancel in the final sum. We suggest these terms are responsible for violating the Tsirel’son bound, [20], which leads to the difference between quantum, CHSH

= 2 \sqrt{2}

and the simulation, CHSH

= 3

We conclude that use of the singlet state in QM, Eq.(14) is an approximation which misses these canceled terms. That is entanglement is a property of QM, but not of Nature. Entanglement simplifies calculation but missing correlation is the price.

5. Black Holes and Wormholes

Suggestions by Maldacena and Susskind [18] are expressed by the relationship ER=EPR where ER stands for Einstein-Rosen Bridges, (wormholes), [66], while the EPR [4], refers to QM and entanglement as a way to quantize spacetime [67].

Wormholes are solutions to the Einstein Field Equations [68] and are purely geometric distortions of spacetime, mathematically connecting different spacetime spaces. Maldacena and Susskind suggest that the wormholes are equivalent to, or created by, a pair of entangled black holes, with Alice at one and Bob at the other performing gedanken experiments with credible outcomes.

Although non-local entanglement is not possible, [7], Q-spin presents an alternative that replaces non-locality. We point to [6,69],

{(\underset{̲}{\underset{̲}{U}})}_{i j} = δ_{i j}

σ σ = \underset{̲}{\underset{̲}{U}} + \underset{̲}{\underset{̲}{\underset{̲}{″}}} \cdot i oe

(18)

as perhaps the smallest quantum entity which could be the building blocks of spacetime, with the LHS indicating the smallest component of quantum spin in free-flight, and the RHS indicating the connection to geometry,

σ σ = EPR = ER = \underset{̲}{\underset{̲}{U}} + \underset{̲}{\underset{̲}{\underset{̲}{ε}}} \cdot i σ

(19)

The following construction is speculative: consider Minkowski space as composed of a diffuse gas, a lattice, of particles in free-flight with spin

{oe}^{(2)} \equiv oe oe

. Each particle has a mass of at least a rest mass, and energy of at least a zero point. These particles attract each other according to an inverse power law like Newton’s Law of gravity, but because space is so diffuse and the masses so small, there is little attraction between the particles. We are assuming that throughout the vastness of space, these particles form the microscopic fabric of spacetime.

In regions where the density and mass increase, these particles pull closer and eventually they merge and coalesce. For N coalesced points the structure is now

σ^{(2) N}

, with Black Holes in the limit that

N \to \infty

. Examination of n-tuples of spin operators, [70], suggests the first step is to find the irreducible components under the Lorentz Group, and consider the quantization of the resulting structures, not unlike depicted in Figure 9.

We have found Q-spin with a microscopic

4^{t h}

dimensional hyperspace. Quantum gravity is a macroscopic force with a far reach. It is suggested to be mediated by a boson with spin of magnitude 2. Whereas we have found that reality must extend to the forth dimensional quaternion projective space, the

S^{3}

hypersphere, one can only suggest that gravity is more complicated and likely extends to the next credible projective space of octonions in the

S^{7}

hypersphere, or beyond. We do not know the nature of matter and energy in the dark regions of these vast hyperspaces beyond our visualization. All we can know are their stereographic projections into our dimension, and from these observations we must try to visualize what lies beyond our senses.

6. Standard Model

The success of the Standard Model, SU(3)⊗SU(2)⊗U(1), is marred by problems with the Weak Force, [71], with Lepton number [72] and parity violations, [73]. Our work suggests replacing SU(2) with the quaternion group,

Q_{8}

. In this section we explore some consequences of this change on beta decay and parity violation.

6.1. Neutrinos Do Not Exist

Beta decay, [74], can be expressed as a neutron in a nucleus decaying into a proton by emitting a beta particle,

i . e .

a fermion electron, and an anti-neutrino,

\begin{matrix} {}_{0}^{1}n \to_{1}^{1} p + e_{F}^{-} +_{0}^{0} \bar{ν} \\ \frac{1}{2} \to \frac{1}{2} + \frac{1}{2} + \frac{1}{2} \end{matrix}

(20)

The second line gives the total spin magnitudes, each with two magnetic quantum numbers of

\pm \frac{1}{2}

. These components will always conserve spin angular momentum,

e . g . \pm \frac{1}{2} = \pm \frac{1}{2} \pm \frac{1}{2} \mp \frac{1}{2}

. Without the neutrino, a fermion electron alone cannot,

e . g . \pm \frac{1}{2} \neq \pm \frac{1}{2} \pm \frac{1}{2}

These issues of conservation of spin angular momentum and energy led Pauli, [75,76] to hypothesize neutrinos to be fermions, with neither mass nor charge, and with the fermion axis colinear to the axis of linear momentum. This immediately reveals that neutrinos have no mirror image and violate parity. Neutrinos are only left handed while antineutrinos are only right handed. They carry no magnetic moment. We consider these properties peculiar.

Moreover, neutrinos have an exceedingly small collision cross section, so they are not significantly removed by reactions. That is, once produced, neutrinos are basically of no use, yet they are postulated to keep on being produced at enormous rates. All particles have a purpose. For neutrinos, once they have balanced energy and spin in nuclear reactions, they have no further use. They are already the most abundant particles in the Universe by far, [77]. Neutrinos have a source but no known sinks. In that case, consider the back-of-an-envelope calculation:

There are about

10^{22}

stars in the universe with an average neutrino flux of

10^{50}

neutrinos/star/second. Assume that production is constant for the last 14 billion years

= 4.42 \times 10^{17}

sec. With no neutrino sinks,

4.42 \times 10^{89}

neutrinos presently exist in the universe. The value of the average mass of a neutrino is not certain, with most stating one millionth that of an electron, [78]. We used the smallest value we could find,

2.14 \times 10^{- 37}

kg, [79] giving the present mass of neutrinos in the Universe of about about

10^{50}

tons. This is about ten times the estimated visible mass of the Universe. Either neutrinos have sinks, or they do not exist.

Boson beta electrons,

e_{B}^{-}

, in contrast, have a spin of 1 and internal structure. The latter should account for the energy distribution, and therefore not violate the conservation of energy. The spin-1 boson balances the spin without a neutrino,

{}_{0}^{1}n \to_{1}^{1} p + e_{B}^{-}

(21)

\frac{1}{2} \to \frac{1}{2} + 1

(22)

With almost no detection; strange parity properties; their persistence after production; and now the boson electron obviating the

r a i s o n d^{'} \hat{e} t r e

for a fermion electron neutrino, we assert again they do not exist.

This contradicts experimental evidence, [80,81,82,83,84] and we suggest that it be reconsidered in the light of boson beta electrons. No direct detection has been observed. Detected is based upon collision product using the Standard Model, with the Weak Force of SU(2) symmetry. Nonetheless, using this method, over enormous fluxes of neutrinos, very few events are recorded.

We assert this puts into doubt the experimental existence of neutrinos.

6.2. Parity Is Not Violated

In the 1950’s, Yang and Lee, [85], suggested that the weak force may not obey parity which motivated experiments. The 1956 experiment, conducted by Chien-Shiung Wu and her colleagues, [86], confirmed parity violation which was a crucial contribution to the field, [74]. Parity conservation was a widely accepted principle that stated that the mirror image of a physical process should be indistinguishable from the original process in the real world. Parity violation is as weird as quantum weirdness.

Wu’s experiment focused on the beta decay of cobalt-60 nuclei, emitting an electron (

e_{F}^{-}

), and an electron antineutrino, Eq.(20). According to the law of parity conservation, this process should be isotropic in all directions. However, Wu’s team observed a clear asymmetry in the emitted electrons’ distribution. Wu stated, [86],

“If an asymmetry in the distribution between

θ

and

(180 - θ)

... is observed, it provides unequivocal proof that parity is not conserved in beta decay.”

Q-spin challenges this statement.

Under quaternion symmetry, a beta particle is a boson of odd parity,

e_{B}^{-}

. As discussed in the last section, this immediately shows that for beta decay, the antineutrino is not needed to balance spin, and the internal structure of Q-spin conserves the energy. Therefore, without neutrinos, we assert the beta decay process for cobalt is given by,

{}_{60}^{27}{Co} \to_{28}^{60} Ni + e_{B}^{-} + 2 γ

(23)

In the experiment, [86], the cobalt sample was placed in a solenoid that produced a polarizing field along the polar axis and which can be reversed. Wu measured the gamma rays, the distribution of which is the same as that for the beta particles. Equatorial counts were used to normalize the polarization. By reversing the current in the solenoids, the experiment detected an asymmetry in the number of counts between the north and south. The mirror symmetry is not faithful and parity is violated.

This is shown in Figure 7. The first column is the real world. The second column depicts the parity transformations, and the last column performs a

π

rotation, needed so the first and third columns are mirror images.

If parity is conserved the expected distribution must be symmetrical, which is shown in the first row,

(a) Since the magnetic field; the cobalt spin; and the fermion electron; are all axial vectors, they are symmetrical under parity. As stated above, Wu expected this result if parity is conserved.

The real world image in the second row,

(b) This is what Wu actually observed, an asymmetrical distribution of beta events. Performing the same transformations as in (a), it is a clear that the mirror reflection is not faithful, showing parity is violated. For this reason it is concluded that parity is violated in Nature.

In contrast, if the beta particle is a boson electron with odd parity, then row

(c) of Figure 7 shows the real world data is faithfully reproduced for any distribution observed. Parity is not violated under the quaternion group.

6.3. Twistor Theory

The motivation behind Twistor theory, [14,15], is the concept that Nature is complex. At the microscopic level, particles are indistinguishable and described by wave mechanics, including polarization and coherence. Macroscopically, the Random Phase Approximation, (RPM) averages away the complex phases, leaving us in the real world.

A goal of Twistor Theory is to unify quantum mechanics and general relativity by transforming Minkowski space into a complex geometric Twistor space, T. As a complex space, it has two projections into helicity states of + and −, denoted by

P T^{\pm}

. The boundary between the two,

P N

, is a real space of null vectors, which are light rays from the light cone.

From this, using the Penrose transform and other techniques, a number of mathematical developments followed, along with incorporating twistors into string theory [87], and QFT, [88,89].

Here, we complexify the Dirac field. In analogy to Twistor space, T, denote complex spin spacetime by the Dirac field,

D_{s}

. This leads to two projective helicity states of

P D_{s}^{\pm}

. Upon measurement, the real part is observed. No additional parameters are introduced, and a non-Hermitian Dirac equation determines the fields. Under parity, this splits further into complementary spaces.

The purpose of our complexification is to introduce a bivector into spin, and, again, consistent with Twistor Theory, helicity is formulated. At the microscopic level, neglecting coherence gives incorrect results, leading us to accept Nature is complex.

7. Quantum Coherence

Quantum mechanics is also called wave mechanics because states are expressed as waves. As such they can superpose, interfere, have phase relations and decohere. Waves are expressed using complex functions and superposition creates different states. Above we mentioned the feasible umbrella motion of NH₃, and this can be treated as a double well potential separated by a surmountable barrier. The umbrella motion is an example of quantum coherence.

Generally, coherence is distinguished from polarized states by the latter being diagonal elements of the density matrix, and the former being off-diagonal elements, see,

e . g .

Eq.(15). We have shown, [6], that dropping the off-diagonal terms results in a product state devoid of coherence. Without coherence, QM would not violate BI.

Parity arguments, [5], separate spin spacetime into complementary spaces of even and odd parity. The

Q_{8}

symmetry renders one of the BFF axes imaginary,

i e_{2}

. This axis connects spin to the

S^{3}

hyperspace of quaternions. Since beyond our spacetime, we assert quaternions are unobservable elements of reality that generate L or R coherent helicity states in spin spacetime. In free-flight it spins the disc and averages away the polarization. Therefore a boson spin displays no polarization, only helicity, to give the wave part of the wave-particle duality.

We deduce the spinning axis of linear momentum carries a quantum of energy. Upon encountering a polarizing field, that energy is transferred to one of the two fermion axes, 3 or 1. When a system absorbs an electron,

e . g .

the Fermi Golden Rule, that quantum of energy is accepted.

Now consider the two axes, 3 and 1. The upper middle panel of Figure 2, depicts these two axes as the mirror states,

ψ^{\pm}

. That is, both precess in tandem in order to maintain their mirror property. They carry the same frequency, but in opposite directions. The small arrow on each precession indicates they are in phase. As such, they constructively interfere to produce the boson spin, which has no physical axis. It is a pure resonance state, like a tuning folk, and is the manifestation of the bivector,

i σ_{2} = σ_{3} σ_{1}

A spin-1 boson has no

m = 0

magnetic quantum number. Suppose the precessions as shown in Figure 2 are responsible for the

m = 1

component. Reversing both precessions then gives

m = - 1

. The

m = 0

component cannot be formed because it would break the mirror symmetry. Neither a photon nor a boson electron has an

m = 0

component.

The correlation between bosons is responsible for the mustache function. Note the coherent correlation,

E_{q}

, resembles two opposing sine waves reflected at

π

. The following gives a good fit,

E_{q} = \{\begin{matrix} - \frac{1}{4} sin (2 θ_{a b}) 0 \leq θ_{a b} \leq π \\ + \frac{1}{4} sin (2 θ_{a b}) π < θ_{a b} \leq 2 π \end{matrix}

(24)

This is plotted in Figure 8 along with the simulated data points which match Eq.(24). Also plotted for comparison is the correlation from quantum theory. The simulation gives more correlation, CHSH = 1, than obtained from the singlet state and QM, CHSH = 0.828. We have proposed the difference is due to the singlet state being an approximation.

The doubling of the filter angles,

2 θ_{a b}

is due to the boson magnetic moment of

2 μ

which gives two periods over

θ_{a b}

. The first period in Figure 3 starts off at

θ_{a b} = 0

where Alice and Bob’s spins remain anti-correlated. As

θ_{a b}

moves to

\frac{π}{4}

, the anti-correlation means that the bosons’ magnetic quantum numbers, m, must be opposite, making the mustache negative up to

\frac{π}{2}

. At that point, the two bosons begin to correlate, with equal magnetic quantum numbers, rendering the boson correlation positive.

The second period starts at

π

, but the bosons remain correlated up to

\frac{3 π}{2}

, after which they are again anti-correlated. Therefore, the boson correlation in the second period starts off positive. The sign of the boson correlation is in tandem with the sign of the correlation from polarization. Note also the sign of the small residual correlation, Figure 3, is also in tandem. Therefore all three contributions add to the correlation and none subtract, as they physically should.

We view the correlations from polarization (pol) and coherencies (coh) as distinct. By dropping the coherent terms, only fermion electrons remain and the correlation is the triangle in Figure 3. Putting back the boson spins gives the mustache function, Eq.(24). However, recall that to get correlation from coherence, both Alice and Bob must be bosons, and both in coherent states, coh-coh. There are four types of coincidences between them: pol-pol, pol-coh, coh-pol, and coh-coh. Therefore, only

\frac{1}{4}

of the coincidences lead to coherence, which is the origin of that factor in Eq.(24).

8. Electrons Are Anyons

Although we cannot measure a free-flight electron, we can envision one. In our spacetime, it is a charged spinning disc, with no net magnetic polarization. Under quaternion symmetry, we have shown, [5], the complementary properties are respectively the disc, governed by a Hermitian 2D Dirac equation with algebra,

C ℓ_{1, 2}

; and the anti-Hermitian quaternion in

S^{3}

, governed by the massless Weyl equation, which spins the disc.

We have proposed the two spatial components are not two particles, but two axes on the same particle. We permute the indistinguishable axes rather than indistinguishable particles. An electron is an anyon, [19],without being a quasiparticle. One might call the anyon state between the boson and fermion a quasistate since it emerges in the presence of an external field, and is a transition state between the fermion and boson forms.

e_{F}^{-} (anisotropy) \underset{transition}{\overset{anyon}{⟷}} e_{B}^{-} (isotropy)

(25)

In a polarizing field, the indistinguishability is lost, the two axes respond differently until one becomes the fermion we measure, and the other randomizes, Figure 2, left and right panels.

We suggest the anyon transition state is the boson decoupling by nutating. One axis rotates about the other, thereby forming braids, [90,91]. The braiding depends upon

(θ_{a} - θ)

, with

θ

common to both Alice and Bob. The coherent correlation, and violation of BI, can possibly be quantitatively described by this common braiding.

9. Interpretations of Quantum Mechanics

With Bell’s theorem gone, then so are objections to the locality assumption EPR made. Quantum mechanics is incomplete, [4]. In our treatment here, we deviate from standard practice, yet stay within a philosophy of the microscopic.

Elements of reality can be non-Hermitian, $e . g .$ Eq.(2).
Calculate the expectation values using the usual state operator which is Hermitian, positive semi-definite of trace 1, $e . g .$ . Eq.(8)
Expectation values of non-Hermitian operators can be complex with two complex conjugate states, $e . g .$ Eq.(9).
Take the real part to obtain the measured value , $e . g .$ Eq.(10).
When dealing in complex spaces, non-Hermitian operators lead to the separation of entangled states, $e . g .$ Eq.(15)

QM must be extended to accommodate spaces beyond our LFF. However, state operators remain in our LFF from which we observe.

In linearizing the Klein-Gordon equation, we did not consider complexifying more than one

γ_{s}^{μ}

matrix since a spin has only one axis to spin. The ± indicates two helicity spaces, [14,15]. We have complexified the gamma field in spin spacetime which is governed by a non-Hermitian Dirac equation.

The quaternion field is more fundamental than Dirac’s. The latter leads to the two-spin matter-antimatter pair,

i . e .

two point particles with two states each, while the former leads to one structured particle, Q-spin, with four states: two polarized up or down and two coherent, with helicity L or R.

This section argues that Q-spin gives a deeper and more realistic view of spin than Dirac. First, however, Q-spin becomes a particle in a polarizing field, where most interest lies. In that case, the two possible fermions (left and right panels of Figure 2) cannot be distinguished from Dirac spin of up and down. We expect quantum calculations based on Q-spin in a field to be identical to those of Dirac spin, albeit with no antimatter twin.

We know of no experiments other than coincidence EPR types, [8,9,10], which are sensitive to the anyon transition. However, one expects that transition to be ubiquitous in Nature.

Figure 9. Parentage Scheme. The number of linearly independent irreducible representations of weight ℓ in a spinor of order p,96].

Q-spin is also the first building block of a parentage scheme to higher spins, similar to the Clebsch-Gordan series, [92]. The dyadic, Eq.(1), stops at the bivector, but higher n-tuples contain trivectors and higher structures which can be decomposed into irreducible components under some group, [93] to [96]. The usual parentage scheme is based upon the addition of spinors, see Figure 9. Extension to complex Q-spin includes a phase for each spin, and any parentage scheme will likely contain insightful relationships as Q-spin couples to produce spins of higher weight. The way these couple may be crucial steps to understanding some process, like polarization transfer, which depends upon coherence. This implies we must maintain the phase relations in calculations, and only project into our real space when observation is needed.

9.1. Symmetry Change

The results of this paper logically follow by changing the symmetry from one continuous group, SU(2), to another,

Q_{8}

, consistent with Noether’s Theorem [97]. Several of the changes are profound, overturning well-established ideas. These changes remove certain features of QM which, succinctly, do not make sense. Non-locality is called quantum weirdness, [48], because it defies rational interpretation, yet a vast literature exists based upon non-local entanglement. Additionally, parity violation defies logic. How can a mirror not reflect an actual image of the real world? The existences of an infinitely deep fermionic continua of Dirac seas; non-locality; and persistent entanglement; are indeed “Spooky,” [98], and, according to Issac Newton, “an absurdity,” [26]. Q-spin resolves these issues.

Simply finding that a boson electron is odd to parity and a fermion electron is even to parity changes our view of the weak force, the beta particle is a boson, neutrinos are not needed, and parity is no longer violated. Our view of antimatter changes, which subsequently resolves the baryon asymmetry problem. This allows the definition of helicity; defines the complementary spin space of coherence; makes intrinsic angular momentum extrinsic; and provides a clear formulation and mechanism of the wave-particle duality of spin.

9.2. Measurement

If we accept quaternions as elements of reality, we arrive at a description of Nature that extends beyond our spacetime. Despite being a theory of measurements, QM must first treat what we cannot measure,

e . g .

Eq.(2) and then project that onto our dimensions,

e . g .

Eq.(10). Therefore, QM must include these higher dimensions and treat them as objectively real.

A state density operator is Hermitian,

e . g .

Eq.(8). Despite non-Hermitian operators, like

Σ

, a state density operator is unchanged in representing what we can observe. However, expectation values can be complex, Eq.(9), otherwise we miss coherence in our treatments.

Non-Hermitian state operators can separate entangled states into products, [64]. Whereas the state density operator is defined in Minkowski space, we propose non-Hermitian state operators be used when extending QM to include higher dimensions. All 16 components of Eq.(15) are non-Hermitian state operators, like each

2 \times 2

matrix in Eq.(16). That is, both polarization, (diagonal element) and coherence (off-diagonal element) are needed to separate the singlet into product states. Once separated, each term can be evaluated and then projected onto our dimensions in the LFF.

Each spin has eight orientations in spin spacetime to form a singlet: four BFF quadrants, and each quadrant has two helicity states. The simulation treats all orientations, [7] whereas the singlet state alone, as we have shown, cancels coherent terms, Eq.(17).

All four Bell states can be written as a product of non-Hermitian states, [64], and these, in turn, can be combined to give Hermitian operators,

\begin{matrix} ρ_{Ψ_{12}^{-}} = |Ψ_{12}^{-}〉 〈Ψ_{12}^{-}| = \frac{1}{4} (I^{1} I^{2} - σ^{1} \cdot σ^{2}) \\ ρ_{Ψ_{12}^{+}} = |Ψ_{12}^{+}〉 〈Ψ_{12}^{+}| = \frac{1}{4} (I^{1} I^{2} + σ^{1} \cdot σ^{2} - 2 σ_{Z}^{1} σ_{Z}^{2}) \\ ρ_{Φ_{12}^{-}} = |Φ_{12}^{-}〉 〈Φ_{12}^{-}| = \frac{1}{4} (I^{1} I^{2} + σ^{1} \cdot σ^{2} - 2 σ_{X}^{1} σ_{X}^{2}) \\ ρ_{Φ_{12}^{+}} = |Φ_{12}^{+}〉 〈Φ_{12}^{+}| = \frac{1}{4} (I^{1} I^{2} + σ^{1} \cdot σ^{2} - 2 σ_{Y}^{1} σ_{Y}^{2}) \end{matrix}

(26)

For a fuller description of the microscopic, non-Hermitian operators can resolve entangled states into products, and remove approximations. We suggest that any quantum operator can be expressed as a product state when using non-Hermitian states.

9.3. No Wave Function Collapse

Wave function collapse, [99,100] describes the change in a quantum system when it undergoes a non-deterministic transition from a superposition of states to a single state due to measurement. This concept primarily applies to individual quantum systems, such as particles like electrons or photons, rather than macroscopic objects like liquids or solids. For such macroscopic systems, the quantum effects are usually averaged out due to the large number of particles involved, [101,102,103]. The collective behavior of atoms and molecules in liquids and solids generally conforms to classical physics rather than quantum mechanics. Therefore, wave function collapse applies at the quantum level, while its direct application to the bulk properties of liquids and solids is limited.

We restrict the discussion, therefore, to single particle processes, like EPR coincidence experiments, molecular beams,

e t c

, for simplicity. We ignore particle-particle interactions. Q-spin perhaps provides the simplest system that undergoes wave function collapse that we can follow.

We have shown a boson-fermion transition occurs when the boson encounters its filter. From its source, a boson electron carries the two possible outcomes as a spin-1, and not as two fermions states of

\pm \frac{1}{2}

. Bosons do not describe matter; do not obey Pauli exclusion, and certainly do not describe a particle in two different states (or places) at the same time, as many express to astonish the layman. When measured, the boson wave "collapses" into a fermion particle, being one of the states

|\pm, \hat{n}〉

, Figure 2, left and right panels. As we have shown, the outcome depends upon the initial orientation of the boson axis,

θ

, and that of the filter angle,

θ_{a}

. The above "collapse" is deterministic, not random, nor instantaneous. The information is carried by the angle

θ

from the source. This example shows there is no wave function collapse.

Define a particle as the solution to the single particle Schrödinger equation. If we know the initial state, then when measured, that pure state is the only one detected. The outcome is pre-determined at the source. Those initial states are always pure, and remain pure until encountering a field that induces transitions. Inducing a transition is what measurement does.

Quantum mechanics, however, allows us to anticipate the outcome before "collapse" using the Born rule. We simply find the normal modes of the system,

i . e .

the eigenvalue problem, and this allows us to know the possible outcomes as a superposition, along with a probability for each. That does not reflect the wave before measurement or in free-flight, but rather the possible outcomes if measured over an ensemble of similarly prepared states, [104].

However, the pure states of particles often carry internal motions, coherences and resonances. Ammonia undergoes its umbrella motion in a few femtoseconds. All structured particles display internal motions. Consider a child blows a bubble. The ground state is a sphere, but that bubble undulates reflecting its creation when the child blew. Molecules are similar, albeit quantum. Valence Bond theory gives resonance structures: Molecular Orbital theory delocalizes electrons over molecules for stability: bonds vibrate; and spins couple. However, in free flight a molecule is like the bubble, it is a wave which expresses its pure state, so when measured, it is the only state observable. When encountering the field, that undulating wave changes in response as the pure state is measured.

9.3.1. Hydrogen Deuteride

To visualize the stages a system might undergo in wave function collapse, we follow hydrogen deuteride, HD, from zero magnetic field to a large value, [105]. This shows state preparation into a pure state leads to "collapse" into the same pure state at any field value. HD is complicated enough to bring out features, yet simple enough that we can visualize the processes.

HD has three angular momentum: a proton spin,

I_{p} = \frac{1}{2}

; a deuterium spin,

I_{d} = 1

, and rotational angular momentum,

J = 1

. At room temperature, only the

J = 1

state is significantly populated. In a magnetic field, each displays Zeeman splitting. We note the three gryromagnetic ratios are ordered as

γ_{p} ≫ γ_{d} > γ_{J}

. There is also a coupling Hamiltonian,

H_{c}

, that gives the fine structure and involves spin-spin, spin-rotation and quadruple interactions, etc. From early molecular beam experiments all the coupling constants are known, [105]. Therefore, the eigenvalue problem is solved and the energy diagram shown in Figure 10 from zero to high fields.

The proton spin has two states, the deuterium and rotation have three each for a total of 18. From Figure 10 three regions are identified as these 18 states experience varying field strengths. At zero field, all three spins couple to give the sum, F. The spectrum of the coupling Hamiltonian gives states of

F = \frac{5}{2}

, and two states each of

\frac{3}{2}

and

\frac{1}{2}

. As the field increases, those states undergo changes, displaying a variety of rich features: avoiding crossings, line crossings, repulsive and attractions between states and other features are encountered as the 18 zero field states finally settle down into linear Zeeman terms at high fields,

\begin{matrix} F \to I_{p} + κ \to I_{p} + I_{d} + J \\ (\begin{matrix} \frac{5}{2} \\ a \frac{3}{2}, b \frac{3}{2} \\ a \frac{1}{2}, b \frac{1}{2} \end{matrix}) \to \frac{1}{2} \otimes (\begin{matrix} 2 \\ 1 \\ 0 \end{matrix}) \to \frac{1}{2} \otimes 1 \otimes 1 \\ \underset{zero field}{\underset{︸}{|α, F, m_{F}〉}} \to \underset{intermediate field}{\underset{︸}{|m_{p}〉 |κ, m_{κ}〉}} \to \underset{high field}{\underset{︸}{|m_{p}〉 |m_{d}〉 |m_{J}〉}} \end{matrix}

(27)

where

α = a, b

. Figure 11 shows these three cases for low, intermediate and high fields. At zero field the vector F precesses with two internal precessions. As the field increases, the proton decouples leaving the other two coupled to give

κ = I_{p} + J

, middle panel, showing one internal precession and two Zeeman terms. Finally at high field, all three are decoupled and precess independently about the field direction, third panel. The analogy with the boson electron "collapsing" to the L and R panels of Figure 2 is obvious.

In an experiment, each particle is prepared at zero field, and then travels in free flight until encountering a field. It responds continuously and deterministically, as seen in Figure 10. The states labels remain with its state from low to high fields,

\begin{matrix} |α, F, m_{F}〉 \to_{field}^{intermediate} |m_{p}〉 |κ, m_{κ}〉 \to_{field}^{high} |m_{p}〉 |m_{d}〉 |m_{J}〉 \\ e . g . \\ state D = |b, \frac{3}{2}, \frac{3}{2}〉 \to_{field}^{intermediate} |- \frac{1}{2}〉 |2, 2〉 \to_{field}^{high} |- \frac{1}{2}〉 |+ 1〉 |+ 1〉 = state D \\ state M = |b, \frac{1}{2}, \frac{1}{2}〉 \to_{field}^{intermediate} |+ \frac{1}{2}〉 |0, 0〉 \to_{field}^{high} |+ \frac{1}{2}〉 |- 1〉 |+ 1〉 = state M \\ e t c . \end{matrix}

(28)

This illustrates our point: at the source a single particle is pure in one state. That prepared state is the same final state that is measured, albeit the measuring process can change that state, like the boson changes to a fermion; and the D and M states for HD. Even if the particle displays undulating resonance motions, like the bubble, it is still in a pure state. We might take that state and decompose it into objects we prefer, like spherical harmonics. No matter what we do, a single particle in free flight is in a pure state, because it cannot be in a mixed one. Therefore, when it is measured, there is no collapse.

Spectroscopy is usually done at high fields, but not always, [106,107]. NMR spectroscopy is done on the proton,

|\pm \frac{1}{2}〉 |m_{d}〉 |m_{J}〉

, on the deuterium,

|m_{p}〉 |0, \pm 1〉 |m_{J}〉

and rotational spectroscopy,

|m_{p}〉 |m_{d}〉 |0, \pm 1〉

. These reveal fine structure due to the coupling Hamiltonian,

H_{c}

Usually we do not know as much about a particle as we know for HD. Nonetheless, by definition, single particles are pure. Even though we might not know which state is prepared, Nature does not care. So we use QM to tell us the possible outcomes. Then, when measured, we get the initial pure state we did not know before. We add it to a bin, and wait for the next. After statistically adding, we compare with our predictions. Deviations from experimental data motivate changes to the Hamiltonian.

Every system has its unique properties like HD, but the above details are typical, with different techniques to prepare states for measurement. However, whether known or not, prepare any quantum state like

|α, F, m_{F}〉

and release it in free flight, Figure 10, then that is the only state which can interact with the filter when measured. Although we say a wave function can be a superposition of all the states, when measured we observe only the initially prepared pure state. Rather than collapse from a contrived superposed wave function, the observed outcome is predetermined, and the "old One" does not play dice, [108].

9.4. The Complex Interpretation

The approach used here is fundamentally different from all other interpretations, and an apt names might be the Twistor, Quaternion or Complex Interpretation. Alternatively, since we always treat pure states, it might be called the Pure State Interpretation. We are compelled to deal with a single particle in a pure state since more than one would include possible decoherence.

The existence of helicity impacts the Einstein-Bohr debates [3,108,109]. Bohr claimed that contrary to Einstein, only the measured property exists in his philosophy of complementarity [109]. Einstein believed that both elements of reality exist simultaneously. Indeed, both properties of Q-spin exist simultaneously, see

e . g .

Eqs.(2),(16), supporting Einstein. Only one is manifest at any instant, supporting Bohr.

All interpretations, including the Copenhagen, are concerned with the measurement problem and the meaning of reality. Most rely on non-deterministic wave function collapse which we reject. Many also rely the role of the observer, which lacks clarity, is ambiguous and subjective. Ballentine, [104], rejects interpretations that give the observer a central role in determining reality, which he calls a move to a solipsistic viewpoint. We agree, but from our perspective, QM is burdened by phenomena which defies rationality. The measurement problem, wave function collapse, Bell’s Theorem’s spooky action-at-a-distance, nondeterminism, Dirac’s hole theory, point particle spin, parity violation,

e t c .

, we assert, are the reasons for confusion, and lack of clarity in the interpretation of QM. Philosophers are put in an untenable position of trying to explain the impossible. This has resulted in exhausting semantic debates, too many to reference, most speculative, confusing, unpublished, and mostly irrelevant, which surround all current interpretations: the resolution of which is doubtful under existing concepts. This has resulted in the mantra of "Shut up and calculate", [110], and during his famous lectures on physics, Richard Feynman said, "I think I can safely say that nobody understands quantum mechanics". Such pithy phrases are intimidating for many, and undermine the tenet of our intelligent. It is unacceptable that we accept phenomena which defy reason, like non-locality. Our confusion is not due to our inability to follow the logic of Nature, but rather our incomplete and incorrect description of the microscopic. One guiding principle should be, "if it doesn’t make sense, it is probably wrong". We should trust our intuition.

Only two interpretations are microscopic: the ensemble, [104] and the de Broglie-Bohm, [111] interpretations. The latter is a hidden variable approach of Bohmian mechanics, but in order to be successful, its “quantum potential” must be non-local.

The Ensemble approach, [104] is closest to the details of an experiment. It assumes the wave function is an ensemble of similarly prepared systems consistent with the statistical mechanical view. Measurement is ensemble averaging over distinct convex sets, [112]. We have used von Neumann’s, [99], treatment of projective measure on a state operator by self-adjoint operators, which are the observables, [6]. We use the same approach when using non-Hermitian operators which are elements of reality and not directly observable.

Although we believe the Statistical Interpretation provides a realistic and pragmatic approach by focusing on distributions of ensembles to give the statistical nature of quantum predictions, it does not apply to individual particles in pure states and complex fields, which is our approach.

Realizing that Nature exists beyond our ability to measure, we must accept that information is lost upon measurement, [113], like determining which slit a particle passes. That knowledge disrupts the helicity of spin. The epistemological question is to find methods to account for real properties of Nature we cannot observe. This is not easy, as spin taught us, taking almost 100 years to find helicity as the complementary attribute of spin, the cryptic evidence being the violation of BI, [24].

Quantum mechanics gives an incomplete description of Nature. Instead, it is a theory of measurement restricted to our spacetime.

10. Conclusions

The ideas presented here are quantum yet provide an alternate view of the microscopic. Important and satisfying features of the macro classical view, carry over to the micro. Both are locally real, albeit reality of the microscopic is complex. Equally satisfying is determinism prevails in all dimensions: you measure only what you prepare. Our classical simulation relies on Least Action between vectors, and gives more correlation than from QM. Generally, however, the initial conditions are unknown, and the successes of quantum methods are the predictions we rely on.

We do not believe that the identical geometric structure of Q-spin and a photon is a coincidence, but rather shows a continuity of properties between microscopic particles. Presently, all particles in the standard model are bosons or fermions, and we suspect those particles should be treated under the quaternion group.

Quaternions, [114], one of the many legacies of Hamilton, [115] are more than mathematical constructs defined in the hypersphere,

S^{3}

with four spatial dimensions. Quaternions are elements of reality possessed by spin, complementary to its vector polarizations. Reality extends beyond our three spatial dimensions. The origin of the unit quaternions coincides with the origin of the Bloch sphere and extends it by adding helicity to each Bloch vector, and these spin either L or R with a constant frequency. Spin is not purely a vector quantity but includes the

S^{3}

hypersphere of unit quaternions, and only the 2×2 vector part of a spin,

S

, is projected into our spacetime.

Hamilton’s Eureka moment, discovering that rotations in 3D are generated by quaternions in 4D, set the stage for understanding spin. Spin is a quaternion.

References

Schrödinger, E. (1926). Quantisierung als Eigenwertproblem; von Erwin Schrödinger. Annalen der Physik, 384(4), 361-377. [CrossRef]
Heisenberg, W. (1925). Quantum-theoretical re-interpretation of kinematic and mechanical relations. Z. Phys, 33, 879-893.
Jammer, M. (1974). Philosophy of Quantum Mechanics. the interpretations of quantum mechanics in historical perspective.
Einstein, A, Podolsky, rn and Rosen, N, “Can quantum mechanical description of physical reality be considered complete?” Phys Rev 47, 777-780, (1935).
Sanctuary, B. Quaternion Spin. Mathematics 2024, 12, 1962. [CrossRef]
Sanctuary, B. Spin with Hyper-helicity. Preprints 2023, 2023010571. [CrossRef]
Sanctuary, B. C., Non-local EPR Correlations using Quaternion Spin. Quantum Rep. 2024, 6(3), 409-425. [CrossRef]
Clauser, J. F., Horne, M. A., Shimony, A., & Holt, R. A. (1969). Proposed experiment to test local hidden-variable theories. Physical review letters, 23(15), 880. [CrossRef]
Aspect, Alain, Jean Dalibard, and Gérard Roger. “Experimental test of Bell’s inequalities using time-varying analyzers.” Physical review letters 49.25 (1982): 1804.
Weihs, G., Jennewein, T., Simon, C., Weinfurter, H., Zeilinger, A. (1998). Violation of Bell’s inequality under strict Einstein locality conditions. Physical Review Letters, 81(23), 5039. [CrossRef]
Bennett, C. H., Brassard, G., Crépeau, C., Jozsa, R., Peres, A., Wootters, W. K. (1993). Teleporting an unknown quantum state via dual classical and Einstein-Podolsky-Rosen channels. Physical review letters, 70(13), 1895. [CrossRef]
Dirac, P. A. M. (1928). The quantum theory of the electron. Proceedings of the Royal Society of London. Series A, Containing Papers of a Mathematical and Physical Character, 117(778), 610-624. [CrossRef]
Wikipedia contributors. (2024, July 12). Pauli matrices. In Wikipedia, The Free Encyclopedia. Retrieved 12:06, August 15, 2024, https://en.wikipedia.org/w/index.php?title=Pauli_matrices&oldid=1234105559.
Penrose, Roger. "Twistor algebra." Journal of Mathematical Physics 8.2 (1967): 345-366. [CrossRef]
Penrose, Roger. "Solutions of the Zero-Rest-Mass Equations." Journal of Mathematical Physics 10.1 (1969): 38-39. [CrossRef]
Okun, Lev B. “Mirror particles and mirror matter: 50 years of speculation and searching." Physics-Uspekhi 50.4 (2007): 380. [CrossRef]
Dirac, P. A. M. (1930). "A Theory of Electrons and Protons". Proc. R. Soc. Lond. A. 126 (801): 360–365. [CrossRef]
Susskind, Leonard (1970). “Dual-symmetric theory of hadrons, I.". Nuovo Cimento A. 69 (1): 457–496 781-811. [CrossRef]
Wilczek, F. (1982). Quantum mechanics of fractional-spin particles. Physical review letters, 49(14), 957. [CrossRef]
Tsirel’son, B. S. (1987). Quantum analogues of the Bell inequalities. The case of two spatially separated domains. Journal of Soviet Mathematics, 36, 557-570. [CrossRef]
Ahmadi, M.; Alves, B.X.R.; Baker, C.J.; Bertsche, W.; Butler, E.; Capra, A.; Carruth, C.; Cesar, C.L.; Charlton, M.; Cohen, S.; et al. Observation of the 1S–2S transition in trapped antihydrogen. Nature 2017, 541, 506–510. [CrossRef]
Anderson, E. K., Baker, C. J., Bertsche, W., Bhatt, N. M., Bonomi, G., Capra, A., ... & Wurtele, J. S. (2023). Observation of the effect of gravity on the motion of antimatter. Nature, 621(7980), 716-722. [CrossRef]
Baryon Asymmetry. In Wikipedia. Available online: https://en.wikipedia.org/wiki/Baryon_asymmetry (accessed on 6 April 2024).
Bell, John S. "On the Einstein Podolsky Rosen paradox." Physics Physique Fizika 1.3 (1964): 195. [CrossRef]
Bell, J. S. "Speakable and Unspeakable in Quantum Mechanics" (Cambridge et al., 1987), 2004. See "Locality in quantum mechanics: reply to critics. Epistemological Letters", Nov. 1975, pp 2–6.".
Original letter from Isaac Newton to Richard Bentley Author: Isaac Newton, Source: 189.R.4.47, ff. 7-8, Trinity College Library, Cambridge, UK: extract: “That gravity should be innate inherent and essential to matter so that one body may act upon another at a distance through a vacuum without the mediation of any thing else by and through which their action or force may be conveyed from one to another is to me so great an absurdity that I believe no man who has in philosophical matters any competent faculty of thinking can ever fall into it.".
Wick, David. The Infamous Boundary: Seven decades of heresy in quantum physics. Springer Science and Business Media, 2012.
Scarani, V., Iblipedir, S., Gisin, N., & Acín, A. (2005). Quantum cloning. Reviews of Modern Physics, 77(4), 1225-1256. [CrossRef]
Kochen, S., and Specker, E. P. (1990). The problem of hidden variables in quantum mechanics. Ernst Specker Selecta, 235-263. [CrossRef]
Annila, A. (2021). Quantum Entanglement: Bell’s Inequality Trivially Violated Also Classically. Available at SSRN 4388586.
Arto Annila, Marten Wikstrom, 2021, viXra:2112.0118 Quantum Entanglement: Bell’s Inequality Trivially Violated Also Classically. Category: Quantum Physics.
Geurdes, H. (2023). Requiring Negative Probabilities From’The Thing’Researched, Else That Thing Doesn’t Exist, is Insufficient Ground for Any Conclusion. Else That Thing Doesn’t Exist, is Insufficient Ground for Any Conclusion (August 21, 2023).
Jakumeit, J., & Hess, K. (2024). Breaking a Combinatorial Symmetry Resolves the Paradox of Einstein-Podolsky-Rosen and Bell. Symmetry, 16(3), 255. [CrossRef]
Nordén, Bengt. “Quantum entanglement: facts and fiction–how wrong was Einstein after all?." Quarterly reviews of biophysics 49 (2016). [CrossRef]
Cetto, A. M., Casado, A., Hess, K., & Valdés-Hernández, A. (2021). “Towards a Local Realist View of the Quantum Phenomenon." in Towards a Local Realist View of the Quantum Phenomenon, 4.
Hess, Karl, “A critical review of works pertinent to the Einstein-Bohr debate and Bell’s Theorem" pre-print, November 2021.
Khrennikov, A. (2019). Get rid of nonlocality from quantum physics. Entropy, 21(8), 806. [CrossRef]
Oaknin, D. H. (2020). The Bell theorem revisited: geometric phases in gauge theories. Frontiers in Physics, 8, 142. [CrossRef]
De Raedt, H., Jattana, M. S., Willsch, D., Willsch, M., Jin, F., & Michielsen, K. (2020). Discrete-event simulation of an extended Einstein-Podolsky-Rosen-Bohm experiment. Frontiers in physics, 8, 160. [CrossRef]
Dieks, D. (2002). Inequalities that test locality in quantum mechanics. Physical Review A, 66(6), 062104. [CrossRef]
Kupczynski, M. (2020). “Is the Moon there if nobody looks: Bell Inequalities and Physical Reality." Frontiers in Physics, 8, 273. [CrossRef]
Christian, J. (2014). Disproof of Bell’s Theorem: Illuminating the Illusion of Entanglement. Universal-Publishers.
Hooft, Gerard T. “Ontology in quantum mechanics." arXiv preprint (2021). arXiv:2107.14191.
Hooft, Gerard T. Deterministic quantum mechanics: the mathematical equations. Frontiers in Physics, 8, 253, (2020). [CrossRef]
Santos, E. (2020). “Local realistic interpretation of entangled photon pairs in the Weyl-Wigner formalism. Frontiers in Physics, 8, 191. [CrossRef]
See “Quantum entanglement" Wikipedia.
Scholarpedia, see “Bell’s theorem".
Mullin, W. J. (2017). Quantum weirdness, Oxford University Press.
Big Bell Test Collaboration. “Challenging local realism with human choices." Nature 557.7704 (2018): 212-216. [CrossRef]
Longuet-Higgins, Hugh C. “The symmetry groups of non-rigid molecules." Molecular Physics 6.5 (1963): 445-460. [CrossRef]
Bashar, M. A., Chowdhury,M. A., Islam, R., Rahman, M. S., and Das,S. K. , “A Review and Prospects of Quantum Teleportation," 2009 International Conference on Computer and Automation Engineering, 2009, pp. 213-217. [CrossRef]
National Academies of Sciences, Engineering, and Medicine. (2019). Quantum computing: progress and prospects.
Gisin, N., Ribordy, G., Tittel, W., Zbinden, H., Quantum cryptography. Reviews of modern physics, 74(1), 145, 2002. [CrossRef]
Pan, J. W., Bouwmeester, D., Weinfurter, H., & Zeilinger, A. (1998). Experimental entanglement swapping: Entangling photons that never interacted. Physical Review Letters, 80(18), 3891-3894. [CrossRef]
Kim, Y. H., Yu, R., Kulik, S. P., Shih, Y., & Scully, M. O. (2000). Delayed "choice" quantum eraser. Physical Review Letters, 84(1), 1-5. [CrossRef]
Hensen, B., Bernien, H., Dréau, A. E., Reiserer, A., Kalb, N., Blok, M. S., ... & Hanson, R. (2015). Loophole-free Bell inequality violation using electron spins separated by 1.3 kilometers. Nature, 526(7575), 682-686. [CrossRef]
Giustina, M., Versteegh, M. A., Wengerowsky, S., Handsteiner, J., Hochrainer, A., Phelan, K., ... & Zeilinger, A. (2015). Significant-loophole-free test of Bell’s theorem with entangled photons. Physical Review Letters, 115(25), 250401. [CrossRef]
Brooks, M. (2023). Quantum computers: What are they good for. [CrossRef]
Zhang, Z., Chen, G., Diao, Z., Hemmer, P.R. (2009). NMR Quantum Computing. In: Gao, D., Sherali, H. (eds) Advances in Applied Mathematics and Global Optimization. Advances in Mechanics and Mathematics, vol 17. Springer, Boston, MA. [CrossRef]
Sanctuary, B. C., and Halstead, T. K. (1990). Multipole NMR. In Advances in magnetic and optical resonance (Vol. 15, pp. 79-161). Academic Press.
Freeman, R., and Kupce, E. (1994). Spin gymnastics with selective radiofrequency pulses.
Sanctuary, B. C., & Halstead, T. K. (1990). Multipole NMR. In Advances in magnetic and optical resonance (Vol. 15, pp. 79-161). Academic Press.
Schrödinger E., “Discussion of probability relations between separated systems". Mathematical Proceedings of the Cambridge Philosophical Society. 31 (4): 555–563 (1935). [CrossRef]
Sanctuary, B. C. “Separation of Bell states." arXiv preprint (2007). arXiv:0705.3657 (2007).
Poinsot, Louis. New theory of the rotation of bodies extracted from a memoir read at the Academy of Sciences of the Institute, May 19, 1834 by M. Poinsot . Bachelor, 1834.
Einstein, Albert, and Nathan Rosen. “The particle problem in the general theory of relativity." Physical Review 48.1 (1935): 73. [CrossRef]
Van Raamsdonk, M. (2010). Building up spacetime with quantum entanglement. General Relativity and Gravitation, 42(10), 2323-2329. [CrossRef]
Carroll, S. M. (2019). Spacetime and geometry. Cambridge University Press.
Doran, C., Lasenby, J., (2003). Geometric algebra for physicists. Cambridge University Press.
Sanctuary, B. C. (1976). Multipole operators for an arbitrary number of spins. The Journal of Chemical Physics, 64(11), 4352-4361. [CrossRef]
Bose, T., Boveia, A., Doglioni, C., Griso, S. P., Hirschauer, J., Lipeles, E., ... & Wang, L. T. (2021). Physics beyond the standard model.
Fonseca, R. M. (2020, August). Violation of lepton number in 3 units. In Journal of Physics: Conference Series (Vol. 1586, No. 1, p. 012003). IOP Publishing.
Schwartz, M. D. (2014). Quantum field theory and the standard model. Cambridge University Press.
Griffiths, David (2009). Introduction to Elementary Particles. pp. 59–60. ISBN 978-3-527-40601-2.
Pauli, W. (1930). Pauli letter collection: letter to Lise Meitner (No. CERN-ARCH-PLC, pp. Letter-2412).
Fermi, E. (1934). Versuch einer Theorie der β-Strahlen. I. Zeitschrift für Physik, 88(3-4), 161-177. [CrossRef]
Close, F. Particle physicsavery short introduction,Oxford: Oxford University Press(2004).
Weiler, T. Neutrinos, Einstein, time and paradox: Tom Weiler at TEDxNashville [Presentation],Nashville, TN(2012).
Immerman, Neil. Sacramento Peak: The Universe. University of Massachusetts Amherst. 21 May 2001.
Fukuda, S., Fukuda, Y., Hayakawa, T., Ichihara, E., Ishitsuka, M., Itow, Y., ... & Ichikawa, Y. (2003). The super-kamiokande detector. Nuclear Instruments and Methods in Physics Research Section A: Accelerators, Spectrometers, Detectors and Associated Equipment, 501(2-3), 418-462. [CrossRef]
Abbasi, R., Ackermann, M., Adams, J., Ahlers, M., Ahrens, J., Andeen, K., ... & Madsen, J. (2009). The IceCube data acquisition system: Signal capture, digitization, and timestamping. Nuclear Instruments and Methods in Physics Research Section A: Accelerators, Spectrometers, Detectors and Associated Equipment, 601(3), 294-316. [CrossRef]
Bellerive, A., Klein, J. R., McDonald, A. B., Noble, A. J., & Poon, A. W. (2016). The Sudbury Neutrino Observatory. ArXiv. [CrossRef]
Waxman, E. (2007). Neutrino astrophysics: A new tool for exploring the universe. Science, 315(5808), 63-65. [CrossRef]
Mertens, S. (2016, May). Direct neutrino mass experiments. In Journal of Physics: Conference Series (Vol. 718, No. 2, p. 022013). IOP Publishing.
Lee, T. D., & Yang, C. N. (1956). Question of parity conservation in weak interactions. Physical Review, 104(1), 254. [CrossRef]
Wu, C. S., Ambler, E., Hayward, R. W., Hoppes, D. D., & Hudson, R. P. (1957). Experimental test of parity conservation in beta decay. Physical review, 105(4), 1413. [CrossRef]
Witten, E. (2004). "Perturbative Gauge Theory As A String Theory In Twistor Space." Communications in Mathematical Physics, 252, 189-258. [CrossRef]
Hodges, A. (1990). Twistor diagrams and Feynman diagrams (pp. 339-366). Cambridge Univ. Press, Cambridge.
Mason, L., & Skinner, D. (2010). Scattering amplitudes and BCFW recursion in twistor space. Journal of High Energy Physics, 2010(1), 1-66. [CrossRef]
Freedman, M., Kitaev, A., Larsen, M., & Wang, Z. (2003). Topological quantum computation. Bulletin of the American Mathematical Society, 40(1), 31-38.
Kauffman, L. H., & Lomonaco, S. J. (2004). Braiding operators are universal quantum gates. New Journal of Physics, 6(1), 134. [CrossRef]
Edmonds, A. R. (1957). Angular Momentum in Quantum Mechanics. Princeton, New Jersey: Princeton University Press. ISBN 978-0-691-07912-7.
Coope, J. A. R., Snider, R. F., & McCourt, F. R. (1965). Irreducible cartesian tensors. The Journal of Chemical Physics, 43(7), 2269-2275. [CrossRef]
Coope, J. A. R., & Snider, R. F. (1970). Irreducible cartesian tensors. II. General formulation. Journal of Mathematical Physics, 11(3), 1003-1017. [CrossRef]
Coope, J. A. R. (1970). Irreducible Cartesian Tensors. III. Clebsch-Gordan Reduction. Journal of Mathematical Physics, 11(5), 1591-1612. [CrossRef]
Snider, R. F. (2017). Irreducible Cartesian Tensors (Vol. 43). Walter de Gruyter GmbH & Co KG.
Emmy Noether. Invariante Variationsprobleme. Gott. Nachr., pages 235–257, 1918.
Einstein, Albert. Born-Einstein Letters 1916-1955: Friendship, Politics and Physics in Uncertain Times. Palgrave Macmillan, 2014.
Von Neumann, John. Mathematical foundations of quantum mechanics. Princeton university press, 1955.
Wave function collapse." Wikipedia, The Free Encyclopedia, 6 Jul. 2024. Web. 10 Aug. 2024.
Zurek, W. H. (2003). "Decoherence, einselection, and the quantum origins of the classical." Reviews of Modern Physics, 75(3), 715. [CrossRef]
Joos, E., Zeh, H. D., Kiefer, C., Giulini, D. J., Kupsch, J., & Stamatescu, I. O. (2003). Decoherence and the Appearance of a Classical World in Quantum Theory. Springer.
Leggett, A. J. (2002). "Testing the limits of quantum mechanics: motivation, state of play, prospects." Journal of Physics: Condensed Matter, 14(15), R415.
Ballentine, Leslie E. “The statistical interpretation of quantum mechanics." Reviews of Modern Physics 42.4 (1970): 358. [CrossRef]
Ramsey, N. F., & Lewis, H. R. (1957). Theory of hydrogen deuteride in magnetic fields. Physical Review, 108(5), 1246. [CrossRef]
Smith, G. W., & Scott, G. G. (1969). Dynamic behavior of the thermomagnetic gas torque. Physical Review, 188(1), 433. [CrossRef]
Coope, J. A. R., Sanctuary, B. C., & Beenakker, J. J. M. (1975). The influence of nuclear spins on the transport properties of molecular gases and application to HD. Physica A: Statistical Mechanics and its Applications, 79(2), 129-170. [CrossRef]
Born, M., Born, H., Born, I., & Einstein, A. (1971). The Born-Einstein letters: correspondence between Albert Einstein and Max and Hedwig Born from 1916 to 1955. Walker.
Bohr, Niels. “Can quantum-mechanical description of physical reality be considered complete?." Physical review 48.8 (1935): 696. [CrossRef]
Mermin, N. D. (2004). Could Feynman have said this?. Physics Today, 57(5), 10-11. [CrossRef]
Bohm, David (1952). “A Suggested Interpretation of the Quantum Theory in Terms of ’Hidden Variables’ I". Physical Review. 85 (2): 166–179. [CrossRef]
Leonard, I. E., & Lewis, J. E. (2015). Geometry of convex sets. John Wiley & Sons.
Braunstein, Samuel L., and Arun K. Pati. “Quantum information cannot be completely hidden in correlations: implications for the black-hole information paradox." Physical review letters 98.8 (2007): 080502. [CrossRef]
Snygg, J. (1997). Clifford Algebra: A Computational Tool for Physicists. Oxford University Press on Demand.
Gsponer, A., Hurni, J. P. (2002). The physical heritage of Sir WR Hamilton. arXiv preprint math-ph/0201058.

Figure 1. Two properties of Q-spin in free-flight: its polarization axes,

Σ_{3}

and

Σ_{1}

, are perpendicular to its helicity. These two fermionic axes couple to give a boson of spin 1,

Σ_{31}

. The helicity is in the direction of propagation and averages out the polarizations. Q-spin is geometrically identical to a photon in free-flight.

Figure 1. Two properties of Q-spin in free-flight: its polarization axes,

Σ_{3}

and

Σ_{1}

, are perpendicular to its helicity. These two fermionic axes couple to give a boson of spin 1,

Σ_{31}

. The helicity is in the direction of propagation and averages out the polarizations. Q-spin is geometrically identical to a photon in free-flight.

Figure 2. The longer arrow denotes the direction of the polarizing field. Middle top: The two mirror states in free flight,

e_{1}

and

e_{3}

, couple to give a boson spin-1. Left and right: The fermionic axis closer to the field axis aligns, and the boson decouples. These two panels do not indicate two particles but one Q-spin depicted with one or the other axis aligned with the field. Middle bottom: When the boson spin is close to the field, it initially precesses as a spin-1 without decoupling.

Figure 2. The longer arrow denotes the direction of the polarizing field. Middle top: The two mirror states in free flight,

e_{1}

and

e_{3}

Figure 3. Plotting EPR correlation versus the angle difference

(θ_{a} - θ_{b})

. The blue points give the results of the simulation. The CHSH values are listed and the full correlation is the sum of polarization, the triangle, and coherence, the mustache. Note the, hardly discernible, residual quaternion correlation along the horizontal axis.

Figure 3. Plotting EPR correlation versus the angle difference

(θ_{a} - θ_{b})

Figure 4. Showing both Alice and Bob’s spins by looking along the axis of linear momentum, orthogonal to the screen. Within the two

45^{\circ}

wedges both Alice and Bob’s spins are bosons. The heavy lines are possible filter settings, such that if one axis is Alice’s filter setting, the adjacent setting is Bob’s and so on alternating from Alice and Bob.

Figure 4. Showing both Alice and Bob’s spins by looking along the axis of linear momentum, orthogonal to the screen. Within the two

45^{\circ}

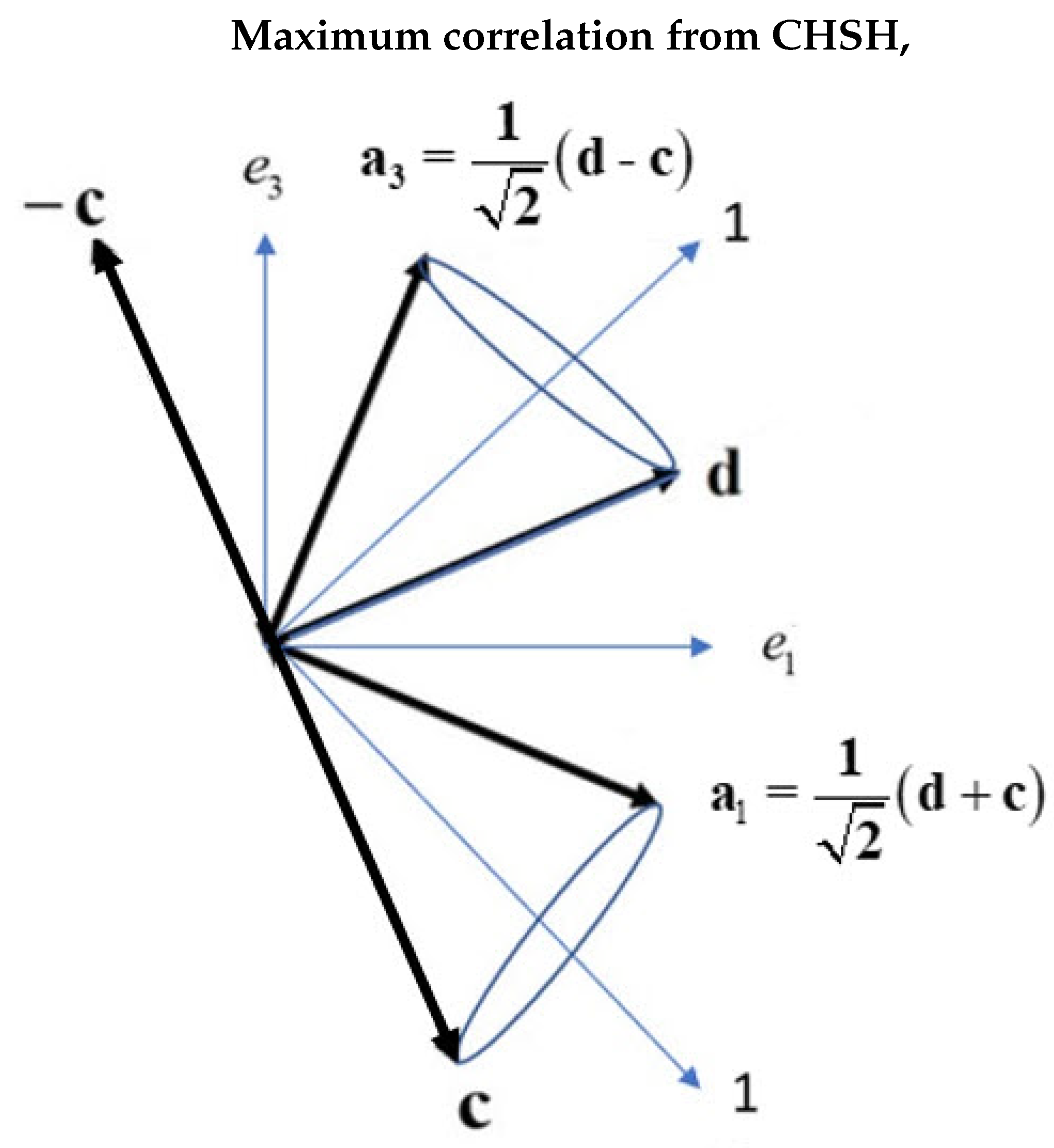
Figure 5. Displaying the maximum correlation from coherence. Alice sets her filter to

a_{3}

and the boson spin-1 aligns. Bob has two settings that will give the maximum violation by applying his filter either along

d

or along

- c

45^{\circ}

from

a_{3}

. Alternately, Alice can set her filter angle to

a_{1}

and Bob has two filter settings,

d

and

c

that lead to the maximum correlation. Note that a spin-1 makes an angle of 45∘ with filter directions.

Figure 5. Displaying the maximum correlation from coherence. Alice sets her filter to

a_{3}

and the boson spin-1 aligns. Bob has two settings that will give the maximum violation by applying his filter either along

d

or along

- c

45^{\circ}

from

a_{3}

. Alternately, Alice can set her filter angle to

a_{1}

and Bob has two filter settings,

d

and

c

that lead to the maximum correlation. Note that a spin-1 makes an angle of 45∘ with filter directions.

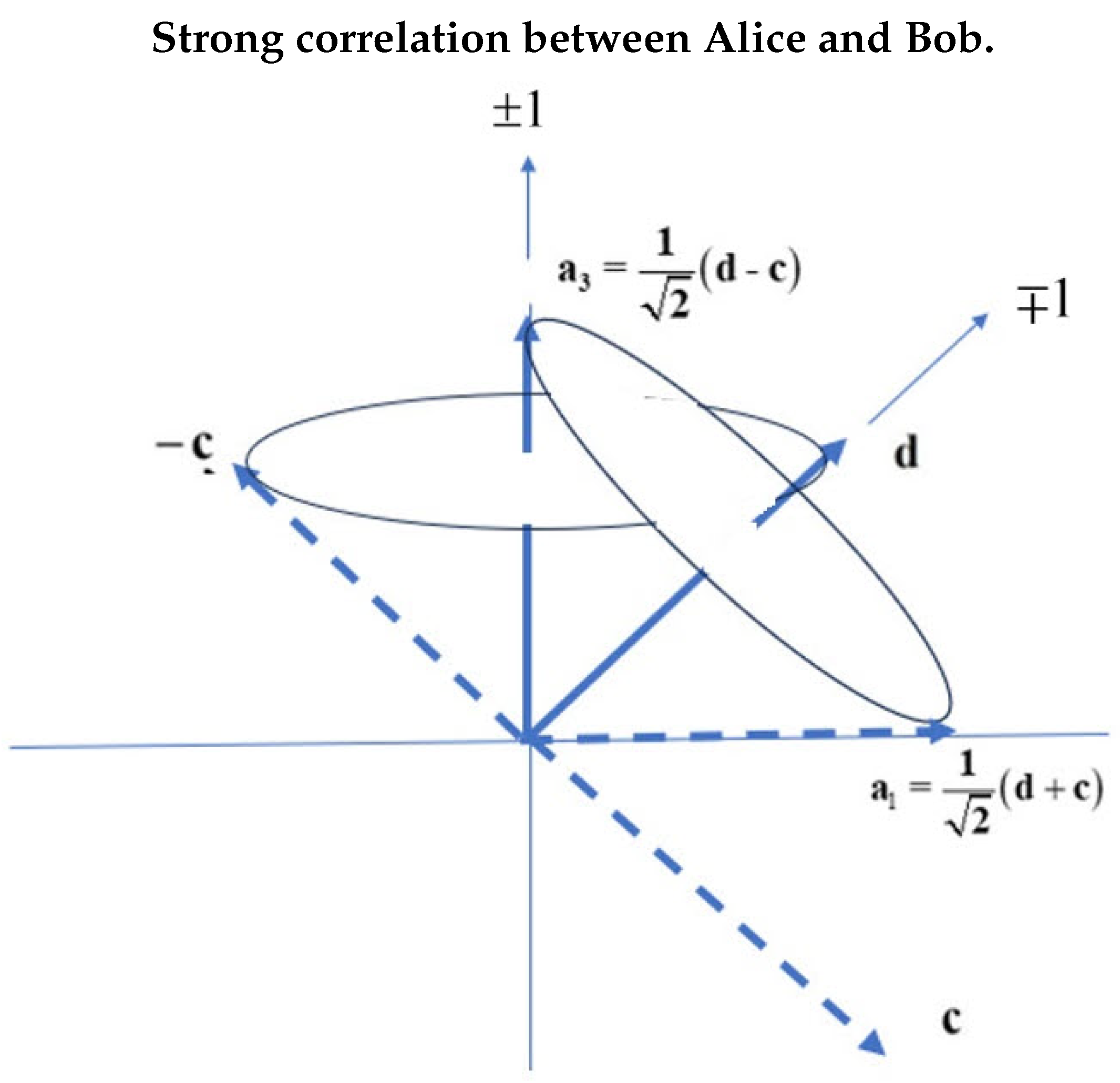
Figure 6. A possible structure of the singlet with two electrons. While the electric charges repel, the magnetic moments attract. If one spin is in a LH frame, the other is in a RH frame. The helicities are opposite.

Figure 7. The cobalt nuclei are the large spheres. The thick arrows are the magnetic fields. The small arrows are beta particles. The first column is the real world and the last is the mirror world. a) For a symmetrical distribution of beta fermion electrons parity is conserved. b) For a asymmetrical distribution of beta fermion electrons parity is not conserved. c) Using boson electrons, parity is conserved for any distribution.

Figure 8. The

E_{q}

function given in Eq.(24) compared to the simulation. Also shown is the correlation from QM showing less than the simulation.

Figure 8. The

E_{q}

function given in Eq.(24) compared to the simulation. Also shown is the correlation from QM showing less than the simulation.

Figure 10. Decoupling of HD: the three angular momenta,

I_{p} = \frac{1}{2}, I_{d} = 1, J = 1

produce 18 states and each is uniquely connected as the field varies from low to high. The unique structures are related to the details of the energy diagram. That diagram is expanded on the LHS to show the low field splitting of the degenerate states.

Figure 10. Decoupling of HD: the three angular momenta,

I_{p} = \frac{1}{2}, I_{d} = 1, J = 1

Figure 11. The three angular momenta,

I_{p} = \frac{1}{2}, I_{d} = 1, J = 1

couple together at low fields to produce the states

|α, F, m_{f}〉

. At intermediate fields, the proton decouples while the deuterium and rotation remained coupled to

|κ, m_{κ}〉

. Finally at high fields, all are decoupled,

|m_{p}〉 |m_{d}〉 |m_{J}〉

. All states are uniquely connected as the field varies. Note that each case has three precessions.

Figure 11. The three angular momenta,

I_{p} = \frac{1}{2}, I_{d} = 1, J = 1

couple together at low fields to produce the states

|α, F, m_{f}〉

. At intermediate fields, the proton decouples while the deuterium and rotation remained coupled to

|κ, m_{κ}〉

. Finally at high fields, all are decoupled,

|m_{p}〉 |m_{d}〉 |m_{J}〉

. All states are uniquely connected as the field varies. Note that each case has three precessions.

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content.

© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).

Copyright: This open access article is published under a Creative Commons CC BY 4.0 license, which permit the free download, distribution, and reuse, provided that the author and preprint are cited in any reuse.

MDPI Initiatives

Important Links

Choose an area of interest and we will send you notifications of new preprints at your preferred frequency.

Disclaimer

Quaternion-Spin and Some Consequences

Abstract

1. Introduction

2. Quaternion Spin

2.1. Identifying EPR Coherence

2.2. EPR Correlation without Non-Locality

2.3. Dirac: No Antimatter

3. Bell’s Theorem Is Irrelevant

3.1. Non-Locality vs. Local Realism

3.2. Quantum Teleportation Is Unfeasible

3.3. Quantum Computing

4. The Singlet State Is Approximate

5. Black Holes and Wormholes

6. Standard Model

6.1. Neutrinos Do Not Exist

6.2. Parity Is Not Violated

6.3. Twistor Theory

7. Quantum Coherence

8. Electrons Are Anyons

9. Interpretations of Quantum Mechanics

9.1. Symmetry Change

9.2. Measurement

9.3. No Wave Function Collapse

9.3.1. Hydrogen Deuteride

9.4. The Complex Interpretation

10. Conclusions

References

MDPI Initiatives

Important Links

Subscribe