Photon Acceleration by Superluminal Ionization Fronts

1. Introduction

Interaction of light with ionization fronts is an old theoretical problem in plasma physics [1,2], and led to the foundations of the concept of photon acceleration [3,4,5]. This concept is intimately related with the effect of self-phase modulation that is studied in nonlinear optical media [6], as shown in [7]. One of the most spectacular manifestations of self-phase modulation is the supercontinuum laser source (see [8]). Another extension of the problem of propagation in ionization fronts is associated with the possible use of superluminal fronts. This leads to the exploration of interesting space-time symmetries, as discussed here.

The theory of superluminal fronts was first considered in terms of geometric optics, showing that very large values of frequency shifts could be attained [9]. It should be noticed that the velocity of an ionization front is not directly associated with any material motion, because it is defined as an optical boundary between two regions of a gas, the neutral region and the ionized gas region, with two different refractive indices. As such, it can in principle be achieved with particles at rest (neutral atoms, electrons and ions), in the same way as a front fire can move in a forest with the trees staying at rest.

In the past, several experiments explored the use of relativistic ionization fronts moving with velocities close to, but lower than, the speed of light c [10,11,14,17]. Until recently, there was no clear indication that superluminal fronts, moving with velocities above c, could be generated experimentally. However, in recent years, the practical implementation of superluminal boundaries became possible due to the proposed schemes of flying-focus [18,19,20,21] (see also [22]). They are mainly based on chromatic optics, but achromatic schemes can also in principle be conceived. We could therefore use the flying-focus, or some other equivalent concept, to propose a new type of radiation experiments based on superluminal fronts. It is the purpose of the present work to describe the basic radiation processes induced by such fronts, and show the underlying symmetry breaking processes that can take place in space and time boundaries.

The content of this paper is the following. In Section II, we describe superluminal fronts in the laboratory frame S, and in the time frame $S^{\prime }$. We show that this new frame $S^{\prime }$ moves with respect to the laboratory frame with a subluminal velocity $V<c$, and can therefore be characterized by a proper Lorentz transformation. The interest of this new frame is that the superluminal front is reduced to a purely temporal process, to which the model of time-refraction can be directly applied [12,13]. We define two front shapes, a simple front with constant electron density, and a modulated front, with electron density oscillations. Simple fronts can easily be created by short laser pulses, because the recombination times are much longer than the ionization times, and the electron density stays nearly constant behind the pulse front [14]. The case of a modulated front is more speculative, although some recent simulations show that laser wakefields sometimes seem to behave as superluminal density waves, similar to the model examined here [15]. But this is only valid under quite special conditions, and the use of this approach to created superluminal density oscillations is questionable.

In Section III, we consider a simple front shape and derive the frequency shifts of a probe laser beam interacting with the front. We show that they strongly depend on the direction of propagation of the probe beam with respect to the ionization front. In Section IV, we use the field transformation formulas to derive expressions for the reflection and transmission coefficients in both reference frames. They can be seen as temporal Fresnel’s formulas. In Section V, we consider the case of a modulated front, and show that resonant scattering conditions characterized by a temporal Bragg formula can also be defined. If possible, the use of such modulated fronts with oscillating density perturbations behind the front could lead to the formation of a time-crystal. Finally, in Section VI, we state some conclusions.

The present work is valid in the frame of classical radiation theory, but the quantum optical description could equally be possible [16,23,24]. We adapt our previous results of time-refraction to the case of a time frame. Notice that time-refraction is a general process, symmetric to the well-know optical refraction, that exists in the classical as well as in the quantum regime. Time-refraction was recently extended to the case of a Dirac field [25,26], where photons are replaced by electron-positron pairs.

2. Time Frame

We consider the plasma density perturbations associated with an ionization front, moving with superluminal velocity $u>c$, along some arbitrary $Ox$-direction. We first describe the front in the laboratory frame S, and then introduce the time frame $S^{\prime }$, where the front will appear as a purely temporal process, enabling a simpler discussion of the wave propagation through the front.

The front can be generically described by the electron plasma frequency profile, ${\omega }_p(\mathbf{r},t)$. We use the square of this frequency, because it is proportional to the electron plasma density, and it is a relativistic invariant. In the lab frame, a front moving with velocity u along a given axis Ox, can be described by the following expression

$${\omega }_p^2(x,t)={\omega }_{p0}^2\left[1+ϵg(x,t)\right]f(x,t),$$

(1)

where $f(x,t)$ describes the shape of the front itself, as it is created by a laser pulse and moves through the medium, and $g(x,t)$ describes the eventual density structure with amplitude $ϵ$, left behind the front. This structure can be a plasma density oscillation moving with the front, as considered later. But, for the moment we consider a flat front, with no structure, and assume the amplitude $ϵ=0$. For simplicity, we also ignore the transverse dimension, ${\mathbf{r}}_{\perp }$, which can easily be included if necessary. In our description, it is appropriate to use

$$f(x,t)=\frac{1}{2}\left\{1+tanh\left[k_{f}q(x,t)\right]\right\},q(x,t)=x-ut.$$

(2)

where the quantity $k_f$ defines the front width, and we assume a superluminal front velocity, $u>c$. It is particularly useful to consider a Lorentz transformation from the lab frame S to a moving frame $S^{\prime }$, where the new front velocity becomes infinite, $u^{\prime }\to \infty$. In this case, we are reduced to a purely temporal process, where the plasma density suddenly changes instantly everywhere. To define this frame we consider the new space and time variables $(x^{\prime },t^{\prime })$, defined by the Lorentz transformations

$$x=\gamma \left(x^{\prime }+V{t}^{\prime }\right),t=\gamma \left(t^{\prime }+\frac{\beta }{c}{x}^{\prime }\right),$$

(3)

where V is the velocity of the new frame $S^{\prime }$ with respect to the lab, $\beta =V/c$ and $\gamma ={(1-{\beta }^2)}^{-1/2}$. Using the formula for addition of velocities, we have a new front velocity $u^{\prime }$ determined by

$$u^{\prime }=\frac{u-V}{1-u\beta /c},$$

(4)

which shows that $u^{\prime }$ diverges for $u=c/\beta \equiv c^2/V$. We conclude that this is a proper Lorentz transformation defined by a subluminal velocity $V=c^2/u<c$. Replacing this in the expression of $q(x,t)$, we get

$$q(x,t)=x-vt=\gamma \left(x^{\prime }-\frac{c^2}{u}{t}^{\prime }\right)-u\gamma \left(t^{\prime }-\frac{x^{\prime }}{u}\right)=\gamma \left(V-u\right)t^{\prime }.$$

(5)

We conclude that the plasma frequency, given by eqs. (1)-(2) with $ϵ=0$, only changes with time in the moving frame $S^{\prime }$. We are therefore reduced to a purely temporal problem. For that reason, we will call $S^{\prime }$ the time frame. Noting that the plasma frequency is a relativistic invariant, we can conclude from this discussion that, in the new frame, the ionization front it is given by

$${\omega }_p^2\left(t^{\prime }\right)=\frac{{\omega }_{p0}^2}{2}\left[1+tanh\left({\nu }_f^{\prime }{t}^{\prime }\right)\right],$$

(6)

with ${\nu }_f^{\prime }=k_f\gamma (V-u)$.

Figure 1. Superluminal ionization front, (a) in the laboratory frame S, and (b) in the time frame $S^{\prime }$. The incident wave is represented in both frames.

Figure 1. Superluminal ionization front, (a) in the laboratory frame S, and (b) in the time frame $S^{\prime }$. The incident wave is represented in both frames.

3. Frequency shifts

Let us now assume a photon beam (for instance a laser probe) interacting with the ionization front. Before interacting with the front, it will have an initial frequency ${\omega }_i$, defined in the lab frame. For propagation along the same axis Ox, we can define the corresponding wavevector as ${\mathbf{k}}_i=k_i{\mathbf{e}}_x$, with $k_i=s{\omega }_i/c$, where $s=+1$ corresponds to co-propagation, and $s=-1$ to counter-propagation with respect to the front.

In the time frame $S^{\prime }$, this initial beam will be characterized by new values of frequency ${\omega }_i^{\prime }$ and wavevector ${{\mathbf{k}}^{\prime }}_i=k_i^{\prime }{\mathbf{e}}_x$, defined by

$$k_i^{\prime }=\gamma \left(k_i-\frac{\beta }{c}{\omega }_i\right),{\omega }_i^{\prime }=\gamma \left({\omega }_i-V{k}_i\right).$$

(7)

This can be rewritten as

$$k_i^{\prime }=\gamma \left(s-\beta \right)\frac{{\omega }_i}{c},{\omega }_i^{\prime }=\gamma {\omega }_i\left(1-s\beta \right).$$

(8)

From here, we immediately conclude that

$$k_i^{\prime }=s\frac{{\omega }_i^{\prime }}{c},{\omega }_i^{\prime 2}={\omega }_i^2\frac{1-s\beta }{1+s\beta }.$$

(9)

It means that, for co-propagation $(s=1)$, we have $k_i^{\prime }>0$, and ${\omega }_i^{\prime }\ll {\omega }_i$ is expected. In contrast, for counter-propagation $(s=-1)$, we have $k_i^{\prime }<0$, and ${\omega }_i^{\prime }\gg {\omega }_i$.

Let us consider the interaction of this incident beam with the front. In the time frame $S^{\prime }$, the front suffers a transformation process when it crosses the temporal boundary at $t^{\prime }=0$. This is the process of time-refraction, which is already well-understood [5,23]. We known that this process conserves momentum (in the time frame), and induces a frequency shift. To simplify the discussion, we assume that the front width is negligible, $1/{\nu }_f^{\prime }\to 0$, but for a finite width, the final result would be the same. Assuming that the local dispersion relation is satisfied, for $t^{\prime }>0$, reflected and transmitted signals, the wavevectors and frequencies of the transmitted and reflected signal are given by

$${{\mathbf{k}}^{\prime }}_r=-{{\mathbf{k}}^{\prime }}_i,{{\mathbf{k}}^{\prime }}_t={{\mathbf{k}}^{\prime }}_i,{\omega }_r^{\prime }={\omega }_t^{\prime }=\sqrt{{\omega }_{po}^2+k_t^{\prime 2}{c}^2}\ne {\omega }_i^{\prime }.$$

(10)

Similarly, we can write

$${\omega }_t^{\prime }=\frac{|k_t^{\prime }|c}{n^{\prime }},n^{\prime }=\sqrt{1-\frac{{\omega }_{p0}^2}{{\omega }_t^{\prime 2}}},$$

(11)

where $n^{\prime }$ is the refractive index of the medium in the moving frame. Using eq. (9) we can write the new frequencies in terms of the initial frequency ${\omega }_i$, defined in the lab frame, as

$${\omega }_t^{\prime 2}={\omega }_i^2\frac{1-s\beta }{1+s\beta }+{\omega }_{p0}^2.$$

(12)

As a final step, we need to establish the value of the transmitted frequency in the lab frame, ${\omega }_t$. We use the Lorentz formula

$${\omega }_t=\gamma ({\omega }_t^{\prime }-V{k}_t^{\prime })=\gamma {\omega }_t^{\prime }(1+s\beta n^{\prime }).$$

(13)

From here, we get

$${\omega }_t^2={\omega }_i^2\frac{{(1+s\beta n^{\prime })}^2}{{(1+s\beta )}^2}+{\omega }_{p0}^2.$$

(14)

A similar description can be made for the reflected beam, the only difference being the direction of propagation. The result is

$${\omega }_r^2={\omega }_i^2\frac{{(1-s\beta n^{\prime })}^2}{{(1-s\beta )}^2}+{\omega }_{p0}^2.$$

(15)

It becomes clear that the reflected and transmitted beams have very distinct frequencies in the lab frame. Similarly, the corresponding wavevectors ${\mathbf{k}}_r$ and ${\mathbf{k}}_t$ will be quite different, in contrast with what happens in the time frame. This leads to the obvious conclusion that the superluminal front introduces a space and time symmetry breaking simultaneously, thereby changing the frequencies and momenta of the reflected and transmitted photons. This is illustrated in Figure 2, for an initial frequency slight above cutoff, ${\omega }_i=\sqrt{2}{\omega }_{p0}$. It should be noticed that large values of $\beta$ can easily be accessed experimentally, because they correspond to weakly superluminal front velocities. This means that very large frequency shifts should be expected.

To complete the description in the lab frame S, we only need to relate $n^{\prime }$ with the refractive index valid in this frame, n. This can be done using again the Lorentz relations. They allow us to write formulas similar to eqs. (7), but with the refractive index included, as

$$k_t^{\prime }=\gamma \left(sn-\beta \right)\frac{{\omega }_t}{c},{\omega }_t^{\prime }=\gamma {\omega }_t\left(1-s\beta n\right).$$

(16)

Now, using the relation $k_t^{\prime }c=s{\omega }_t^{\prime }{n}^{\prime }$, we easily get an expression for the refractive index in the moving frame, as

$$n^{\prime }=\frac{(n-s\beta )}{(1-s\beta n)},n=\frac{(n^{\prime }+s\beta )}{(1+s\beta n^{\prime })},$$

(17)

Obviously, the refractive index for the reflected and transmitted signals ise different, because they have different frequencies and the medium is dispersiove, $n\left({\omega }_r\right)\ne n\left({\omega }_t\right)$. This contrasts with what happens in the time frame $S^{\prime }$, where we have $n^{\prime }\left({\omega }_r^{\prime }\right)=n^{\prime }\left({\omega }_t^{\prime }\right)$, because the frequencies are equal, although distinct from the initial frequency ${\omega }_i^{\prime }$.

4. Field Transformations

We now focus on the field transformations, and establish the transmission and reflection coefficients in the two reference frames S and $S^{\prime }$. We start with the electric field ${\mathbf{E}}_i$, associated with the incident photon beam in the laboratory frame S. Assuming plane wave propagation along the axis Ox, and neglecting the radial beam structure, we have

$${\mathbf{E}}_i(x,t)={\mathbf{E}}_{i}exp(i{k}_{i}x-i{\omega }_{i}t),$$

(18)

with $k_i=s{\omega }_i/c$. Assuming that this field is linearly polarized in the Oy direction, ${\mathbf{E}}_i=E_i{\mathbf{e}}_y$, the wave magnetic field will be ${\mathbf{B}}_i=s(E_i/c){\mathbf{e}}_z$. In the moving frame $S^{\prime }$, the field is described by a similar expression

$${{\mathbf{E}}^{\prime }}_i(x,t)={{\mathbf{E}}^{\prime }}_{i}exp(i{k}_i^{\prime }{x}^{\prime }-i{\omega }_i^{\prime }{t}^{\prime }),$$

(19)

where $k_i^{\prime }$ and ${\omega }_i^{\prime }$, are determined by eqs. (9). The field amplitudes will be determined by the Lorentz formulas, as

$${{\mathbf{E}}^{\prime }}_i=\gamma \left({\mathbf{E}}_i+\mathbf{V}\times {\mathbf{B}}_i\right)=\gamma (1-s\beta ){\mathbf{E}}_i,$$

(20)

and

$${{\mathbf{B}}^{\prime }}_i=\gamma \left[{\mathbf{B}}_i-(\mathbf{V}\times {\mathbf{E}}_i)/c\right]=\gamma (1-sV){\mathbf{B}}_i,$$

(21)

In order to derive the fields resulting from the interaction with the ionization front, we use the reflection and transmission coefficients valid for a time-refraction event, as $E_r^{\prime }=R^{\prime }{E}_i^{\prime }$ and $E_t^{\prime }=T^{\prime }{E}_i^{\prime }$, such that [24]

$$T^{\prime }=\frac{{\alpha }^{\prime }}{2}({\alpha }^{\prime }+1),R^{\prime }=\frac{{\alpha }^{\prime }}{2}({\alpha }^{\prime }-1),$$

(22)

where the parameter ${\alpha }^{\prime }$ describes the change in refractive index associated with the temporal transition, from vacuum with refractive index $n_0^{\prime }=1$ to a plasma with refractive index $n^{\prime }$ as defined above. This is given by

$${\alpha }^{\prime }=\frac{n_0^{\prime }}{n^{\prime }}=\frac{(1-s\beta n)}{(n-s\beta )}.$$

(23)

Noting that ${\omega }_t^{\prime 2}={\omega }_i^{\prime 2}+{\omega }_{p0}^2$, we can also write

$${\alpha }^{\prime }=\frac{1}{n^{\prime }}=\frac{{\omega }_t^{\prime }}{\sqrt{{\omega }_t^{\prime 2}-{\omega }_{p0}^2}}=\frac{{\omega }_t^{\prime }}{{\omega }_i^{\prime }}.$$

(24)

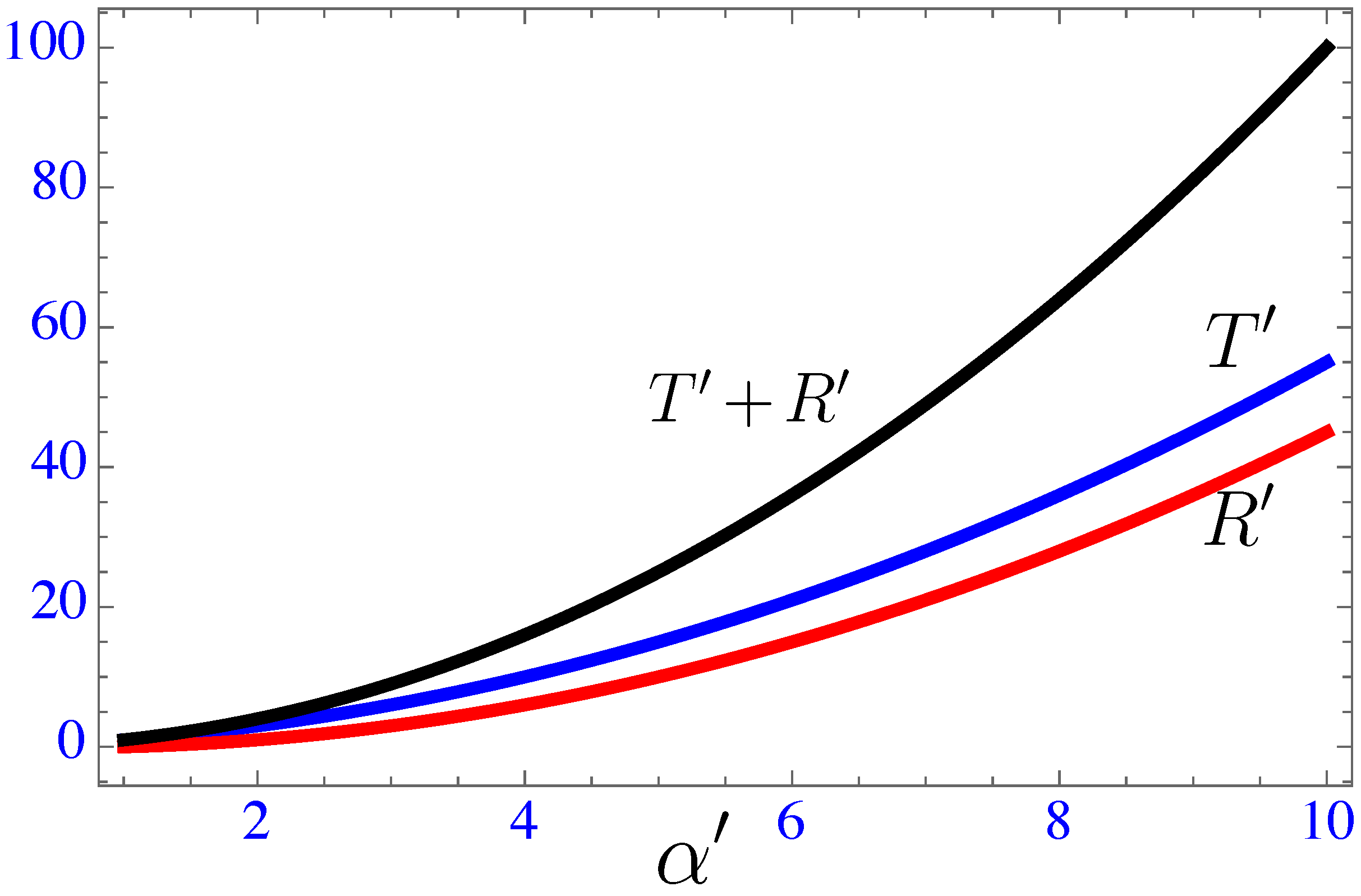
In previous papers on time-refraction, these expressions for $T^{\prime }$ and $R^{\prime }$ were sometimes called temporal Fresnel’s formulae. We can see that $T^{\prime }+R^{\prime }={\alpha }^{\prime 2}$, which is an indication that the energy is not conserved. See Figure 3, for an illustration. This could be explained at the elementary quantum level, as the result of emission of photon pairs from vacuum [23,24]. Let us now use the expression for the transmitted field in the lab frame, but now with the reversed sign of V in the transformation (21) and the inclusion of the refractive index. This gives

$$E_t=\gamma (1+s\beta n^{\prime })E_t^{\prime }=T{E}_i,E_r=\gamma (1-s\beta n^{\prime })E_r^{\prime }=R{E}_i.$$

(25)

We then get for the reflection and transmission coefficients in the lab frame, as

$$T={\gamma }^2(1+s\beta n^{\prime })(1-s\beta )T^{\prime },R={\gamma }^2(1-s\beta n^{\prime })(1-s\beta )R^{\prime },$$

(26)

and, finally

$$T=\frac{(1+s\beta n^{\prime })}{(1+s\beta )}\frac{1+n^{\prime }}{2n^{\prime 2}},R=\frac{(1-s\beta n^{\prime })}{(1+s\beta )}\frac{1-n^{\prime }}{2n^{\prime 2}},$$

(27)

where $n^{\prime }$ can be describe in terms of the refractive index in the laboratory frame n, using eq. (17). In the absence of ionization, we would have $n=n^{\prime }=1$, and these expressions would reduce to $T=1$, $R=0$, as expected. But this result is not very illuminating, because T and R are implicit functions of the transmitted and reflected frequencies. A more direct way to estimate the energy gain associated with this temporal process is to note that no dissipation is involved (apart from eventual scattering losses). Therefore, for each photon that crosses the time boundary we have an energy gain that this exactly given by the frequency ratio ${\omega }_t/{\omega }_i$ and ${\omega }_r/{\omega }_i$, where these quantities are determined by eqs. (14) and (15).

5. Modulated fronts

Let us now consider the case of modulated fronts, where now the amplitude of the oscillations in eq. (1) is non-zero, $ϵ\ne 0$, and the oscillating structure moves with the same superluminal speed as the front itself, $u>c$. For that purpose, we use a periodic function of the form

$$g(x,t)=\sum _{\ell }{g}_{\ell }cos(\ell k_{w}q),$$

(28)

where $q=x-ut$ as before, $g_{\ell }\le 1$ are constant coefficients, and $k_w\ll k_f$ is the wavenumber of the periodic structure, defining a scale that is much larger than the front width. In the time frame $S^{\prime }$, the plasma frequency structure is now transformed into

$${\omega }_p^2\left(t^{\prime }\right)=\frac{{\omega }_{p0}^2}{2}\left[1+ϵ\sum _{\ell }{g}_{\ell }cos(\ell {\omega }_w^{\prime }t)\right]\left[1+tanh\left({\nu }_f^{\prime }{t}^{\prime }\right)\right],$$

(29)

with ${\nu }_f^{\prime }=k_f\gamma (V-u)$, as before, and ${\omega }_w^{\prime }=(k_w/k_f){\nu }_f^{\prime }$. Let us assume an incident wave with frequency ${\omega }_i$, characterized by ${\omega }_i^{\prime }$ and ${{\mathbf{k}}^{\prime }}_i$ in the moving frame $S^{\prime }$, as defined by eqs. (9). Assuming that the local dispersion relation is satisfied, after the transition time $t^{\prime }=0$, we have two wave modes with wavevectors ${{\mathbf{k}}^{\prime }}_r=-{{\mathbf{k}}^{\prime }}_i$ and ${{\mathbf{k}}^{\prime }}_t={{\mathbf{k}}^{\prime }}_i$, and frequencies

$${\omega }_r^{\prime }={\omega }_t^{\prime }=\sqrt{{\omega }_p^2\left(t^{\prime }\right)+k_t^{\prime 2}{c}^2}\ne {\omega }_i^{\prime }.$$

(30)

Here, the plasma frequency ${\omega }_p\left(t^{\prime }\right)$ is determined by eq. (29) and defines a time-varying dispersion relation. Notice that the wavenumbers remain fixed in this frame, but the frequencies are time-dependent. This means that we have to write the total field as

$${{\mathbf{E}}^{\prime }}_i(x^{\prime },t^{\prime })={{\mathbf{E}}^{\prime }}_t\left(t^{\prime }\right)exp[(i{k}_t^{\prime }{x}^{\prime }-i\phi \left(t^{\prime }\right)]+{{\mathbf{E}}^{\prime }}_r\left(t^{\prime }\right)exp[(i{k}_r^{\prime }{x}^{\prime }+i\phi \left(t^{\prime }\right)]+c.c.,$$

(31)

with $\phi \left(t^{\prime }\right)={\int }^{t^{\prime }}{\omega }_{r,t}^{\prime }(t")dt"$, valid for ${\omega }_r^{\prime }={\omega }_t^{\prime }\ne {\omega }_i^{\prime }$. From the field continuity relations, we can derive the following evolution equations for the field amplitudes [27]

$$\frac{d{E}_t^{\prime }}{d{t}^{\prime }}=-\frac{1}{2}\frac{dln{n}^{\prime }}{d{t}^{\prime }}\left\{3E_t^{\prime }+E_r^{\prime }exp\left[2i\phi \left(t^{\prime }\right)\right]\right\},$$

(32)

and

$$\frac{d{E}_r^{\prime }}{d{t}^{\prime }}=-\frac{1}{2}\frac{dln{n}^{\prime }}{d{t}^{\prime }}\left\{3E_r^{\prime }+E_t^{\prime }exp[-2i\phi \left(t^{\prime }\right)]\right\},$$

(33)

where $n^{\prime }\equiv n^{\prime }\left(t^{\prime }\right)=\sqrt{1-{\omega }_p^2\left(t^{\prime }\right)/{\omega }^{\prime 2}}$ is the local refractive index. These expressions can be derived using a succession of infinitesimal time-refraction processes. They can be used to describe propagation in arbitrary time-varying media, and are formally identical to the field equations for propagation ins static but arbitrarily inhomogeneous media [28,29]. Now, introducing the notation

$$E_{r,t}^{\prime }=A_{r,t}^{\prime }{e}^{-3\Gamma \left(t^{\prime }\right)},\Gamma \left(t^{\prime }\right)=\frac{1}{2}{\int }^{t^{\prime }}\frac{dln{n}^{\prime }}{dt"}dt",$$

(34)

we can reduce the above coupled equations for the amplitudes to the simple coupled form

$$\frac{d{A}_t^{\prime }}{d{t}^{\prime }}=\eta \left(t^{\prime }\right)A_r^{\prime },\frac{d{A}_r^{\prime }}{d{t}^{\prime }}={\eta }^{*}\left(t^{\prime }\right)A_r^{\prime },$$

(35)

with the new coefficient

$$\eta \left(t^{\prime }\right)=-\frac{1}{2}\left(\frac{dln{n}^{\prime }}{d{t}^{\prime }}\right)exp\left[2i\phi \left(t^{\prime }\right)\right].$$

(36)

The solution of these equations is very easy to find, and takes the form

$$A_t^{\prime }\left(t^{\prime }\right)=\alpha \left(t^{\prime }\right)A_t^{\prime }\left(0\right)-\beta \left(t^{\prime }\right)A_r^{\prime }\left(0\right),A_r^{\prime }\left(t^{\prime }\right)=\alpha \left(t^{\prime }\right)A_r^{\prime }\left(0\right)-\beta \left(t^{\prime }\right)A_t^{\prime }\left(0\right),$$

(37)

where

$$\alpha \left(t^{\prime }\right)=cosh\left[r\left(t^{\prime }\right)\right],\beta \left(t^{\prime }\right)=sinh\left[r\left(t^{\prime }\right)\right],r\left(t^{\prime }\right)={\int }^{t^{\prime }}\eta (t")dt".$$

(38)

The temporal evolution described by the coefficients $\alpha \left(t^{\prime }\right)$ and $\beta \left(t^{\prime }\right)$ can be seen as a squeezing transformation, where the quantity $r\left(t^{\prime }\right)$ is the squeezing parameter. Let us consider the initial conditions corresponding to the absence of a reflected field at the transition time $t^{\prime }=0$. Using $A_t^{\prime }\left(0\right)\ne 0$ and $A_t^{\prime }\left(0\right)=0$ in eqs. (33), we can then derive the time reflection and time transmission coefficients in the time frame $S^{\prime }$, as

$$T\left(t^{\prime }\right)=\frac{E_t^{\prime }\left(t^{\prime }\right)}{E_t^{\prime }\left(0\right)}=cosh\left[r\left(t^{\prime }\right)\right]e^{-3\Gamma \left(t^{\prime }\right)},R\left(t^{\prime }\right)=\frac{E_r^{\prime }\left(t^{\prime }\right)}{E_t^{\prime }\left(0\right)}=sinh\left[r\left(t^{\prime }\right)\right]e^{-3\Gamma \left(t^{\prime }\right)},$$

(39)

These coefficients strongly depend on the amplitude of the moving structure, $ϵ$. Noting that $d{n}^{\prime }/d{t}^{\prime }\propto ϵ$, we conclude that for very small modulation amplitudes, the following approximate solutions are valid

$$T\left(t^{\prime }\right)\simeq 1,R\left(t^{\prime }\right)\simeq r\left(t^{\prime }\right)=-\frac{1}{2}{\int }^{t^{\prime }}\left(\frac{dln{n}^{\prime }}{dt"}\right)exp\left[2i\phi (t")\right]dt",$$

(40)

On the other hand, we maximize the value of $\left|R\right(t^{\prime }\left)\right|$ when the quantity in the integrand of eq. (40) is nearly constant. For a cosine perturbation of the refractive index, such that in eq. (29) the constant coeficients are defined by the Kroeneker symbol, as $g_{\ell }={\delta }_{\ell 1}$, this maximum reflection condition is approximately given by

$$\nu {\omega }_w^{\prime }{t}^{\prime }-2\phi \left(t^{\prime }\right)=0,$$

(41)

where $\nu$ is an integer. Noting that $\phi \left(t^{\prime }\right)\simeq {({\omega }_i^{\prime 2}+{\omega }_{p0}^2)}^{1/2}{t}^{\prime }$, and using the explicit expression for ${\omega }_w^{\prime }$, we can rewrite this condition as

$$\nu \gamma k_w(V-u)=2{({\omega }_i^{\prime 2}+{\omega }_{p0}^2)}^{1/2}.$$

(42)

This approximate condition defines what could be called a temporal Bragg law. It characterizes the occurrence of resonant backscattering from a temporal periodic perturbation, or in other words, of resonant backscattering from a time-crystal. In order to relate this to the value of the incident frequency, as seen in the lab frame, we can use the Lorentz transformations (8), and write

$$\nu \gamma k_w(V-u)=2\sqrt{{\gamma }^2{\omega }_i^2{(1-s\beta )}^2+{\omega }_{p0}^2}.$$

(43)

Finally, we obtain

$${\omega }_i^2=\frac{1}{4{\beta }^2}\frac{{(1-\beta )}^2}{{(1-s\beta )}^2}\left[{\nu }^2{k}_w^2{c}^2+{\omega }_{p0}^2{\beta }^2(1+\beta )\right].$$

(44)

For incident frequencies ${\omega }_i$ satisfying this relation, we should be able to observed the formation of a Bragg maximum of the backscattered signal from a plasma time-crystal. On the other hand, for a very long time structure, we see from eq. (39) that the energy of the time-reflected signal can grow exponentially, due to the sine hyperbolic function, $sinh\left[r\left(t^{\prime }\right)\right]$. Furthermore, due to the need for momentum conservation, the transmitted signal will necessarily grow as well, thus showing the formation of a temporal driven instability. This is nothing but a classical analogue of the dynamical Casimir effect. We should however notice that the above temporal Bragg scattering, and the associated instability, can only be observed with a modulated ionization front with shape defined by eq. (29), which is not easy to produce experimentally.

6. Conclusions

We have considered wave propagation in the presence of superluminal ionization fronts. These fronts can be use to produce a considerable frequency shift and to amplify radiation. They can be created by chromatic optical arrangements and are not in contradiction with the causality principle. We have discussed two kinds of ionization fronts, simple fronts and modulated fronts, and we used a simple theoretical description based on the time frame, which is a reference frame where the fronts reduce to a purely temporal process.

The results of this paper could eventually inspire the development of new radiation sources, where phase coherence is conserved and frequency tunning could be achieved for different values of the front velocity. This process is, in principle, hundred percent efficient, because all the photons interacting with the front are equally phase shifted, and energy amplification can be considerably larger than one. This means that part of the energy of the superluminal front can be converted into radiation, a process that is not explicitly described by the present model, because the energy balance between the front and the radiation field was not established.

The present work can be extended in several different directions including, apart from this energy balance, a systematic numerical study of the spectral energy density, and an estimate of the losses due to scattering in the transverse beam direction. It could also be applied to the optics of metamaterials, where the study of temporal processes is particularly relevant [30,31]. Some of these extensions will be examined in a future publication.