Preprint
Communication
Metallic Ratios and Angles of a Real Argument
Altmetrics
Downloads
223
Views
96
Comments
0
A peer-reviewed article of this preprint also exists.
This version is not peer-reviewed
Submitted:
01 February 2024
Posted:
02 February 2024
You are already at the latest version
Alerts
Abstract
We extend the concept of metallic ratios to the real argument n considered as a dimension by analytic continuation showing that they are defined by an argument of a normalized complex number, and for rational n ≠ {0, ±2}, they are defined by Pythagorean triples. We further extend the concept of metallic ratios to metallic angles.
Keywords:
Subject: Computer Science and Mathematics - Mathematics
1. Introduction
Each rectangle contains at least one square with an edge h equal to the shorter edge of the rectangle. If a rectangle contains n such squares and its edges and h satisfy
they satisfy a metallic ratio; the golden ratio for , the silver ratio for , shown in Figure 1, the bronze ratio for , etc.
Metallic ratios (3) have interesting properties, such as
Furthermore, as n goes to ifinity, the factor in the square root becomes negligible, and for large n.
It was shown [1] that for positive metallic ratios (3) can be expressed by primitive Pythagorean triples, as
and for
where is the angle between a longer cathetus b and hypotenuse c of a right triangle defined by a Pythagorean triple, as shown in Figure 3, whereas for it is the angle between a hypotenuse and a shorter cathetus a ( and are defined by the same Pythagorean triples, respectively, and ), and
For example the Pythagorean triple defines , the Pythagorean triple defines , the Pythagorean triple defines , and so on.
Since the edge lengths of a metallic rectangle are assumed to be nonnegative, generally only the positive principal square root of (2) is considered. However, the nonnegativity of distances (corresponding to the ontological principle of identity of indiscernibles) does not hold for the LK-metric [2], for example; such an axiomatization is misleading [3]. Furthermore, fractal dimensions have been verified to be consistent with experimental observations [4,5] which justifies the analytic continuation of metallic ratios to the real argument n considered as a dimension [6,7]. This is discussed in Section 2. Section 3 extends the concept of metallic ratios to metallic angles of a real argument n. Section 4 concludes the findings of this study.
2. Metallic Ratios of a Real Number
Theorem 1.
The metallic ratio of is defined by an acute angle of a right triangle .
Proof.
We express the RHS of the Equation (5) using half-angle formulas and substituting
since (we exclude degenerated triangles), so .
We can extend the domain of Theorem 1 by analytic continuation to as in this range. However, extending it further to we note that in this range . Thus, the quadratic Equation (9) becomes
and its roots are
Theorem 2.
The metallic ratio of is defined by an angle .
Proof.
Equating relations (3) and (10) and solving for n gives
for . This identity can also be obtained directly from the second property (4) applied to the ratio (10). Solving the relation (13) for yields
Similarly, applying the second property (4) to the ratio (12) gives
for . Solving the relation (15) for yields
as a conjugate of the relation (14). The relations (14) and (16) remove the singularity of in the relations (13), (15), and . □
Equations (14) and (16) relate which defines a metallic ratio (3) to the normalized complex number . The angles and are shown in Figure 4. There are two axes of symmetry.
In summary, metallic ratios as functions of are
where the first ± defines the range of and the second ± corresponds to positive or negative form of the ratio. Therefore, the first and second properties (4) hold for and but the third property (4) holds as .
Proof.
Plugging rational into the relation (14) gives
and is a possible solution. It is easy to see that , which is valid . implies and ; implies and . □
Table 1 shows the generalized Pythagorean triples that define the metallic ratios for .
Theorem 4.
For , the positive metallic ratio .
Proof.
Direct calculation of the defining relation (3) for . Furthermore, . □
3. Metallic Angles of a Real Number
We can extend the concept of metallic ratios (1) to angles as
where for well known golden angle , shown in Figure 7, is obtained.
In this case, both their products and sums
are dependent on n, where and .
4. Conclusions
The positive golden ratio (3) and the negative golden angle (22) are observed in nature. In flower petals, sunflowers and pinecones, tree branches, shells’ shapes, spiral galaxies, hurricanes, reproductive dynamics, etc. But why has nature chosen corresponding to the complex number (14) remains to be researched. We note that forms the smallest Pythagorean triple, which hints at the relation of such a nature’s choice to the second law of thermodynamics.
Acknowledgments
I thank my wife Magdalena Bartocha for her unwavering motivation and my friend, Renata Sobajda, for her prayers.
References
- Rajput, C. Metallic Ratios in Primitive Pythagorean Triples: Metallic Means embedded in Pythagorean Triangles and other Right Triangles. JOURNAL OF ADVANCES IN MATHEMATICS 2021, 20, 312–344. [Google Scholar] [CrossRef]
- Łukaszyk, S. A new concept of probability metric and its applications in approximation of scattered data sets. Computational Mechanics 2004, 33, 299–304. [Google Scholar] [CrossRef]
- Łukaszyk, S.; Tomski, A. Omnidimensional Convex Polytopes. Symmetry 2023, 15, 755. [Google Scholar] [CrossRef]
- Yu, B. Fractal Dimensions for Multiphase Fractal Media. Fractals 2006, 14, 111–118. [Google Scholar] [CrossRef]
- Yu, B.; Zou, M.; Feng, Y. Permeability of fractal porous media by Monte Carlo simulations. International Journal of Heat and Mass Transfer 2005, 48, 2787–2794. [Google Scholar] [CrossRef]
- Łukaszyk, S. Novel Recurrence Relations for Volumes and Surfaces of n-Balls, Regular n-Simplices, and n-Orthoplices in Real Dimensions. Mathematics 2022, 10, 2212. [Google Scholar] [CrossRef]
- Łukaszyk, S. Life as the Explanation of the Measurement Problem. Journal of Physics: Conference Series 2024. [CrossRef]
Figure 1.
Silver rectangle an ratio .

Figure 2.
Metallic ratios: positive (red), negative (green) as continuous functions of .

Figure 3.
Right triangle showing a longer (b), shorter (a) hypotenuse, catheti (c) and angles and .

Figure 4.
Phases of the complex number (red, ) and its conjugate (green, ) for . , .

Figure 5.
Metallic ratios: positive (red), negative (green) as a function of (dotted), and (solid), and (positive: solid blue; negative: solid cyan) for .
Figure 5.
Metallic ratios: positive (red), negative (green) as a function of (dotted), and (solid), and (positive: solid blue; negative: solid cyan) for .

Figure 6.
The triples corresponding to the angle (14), (16) as functions of . The positive metallic ratio for .

Figure 7.
Golden angle .
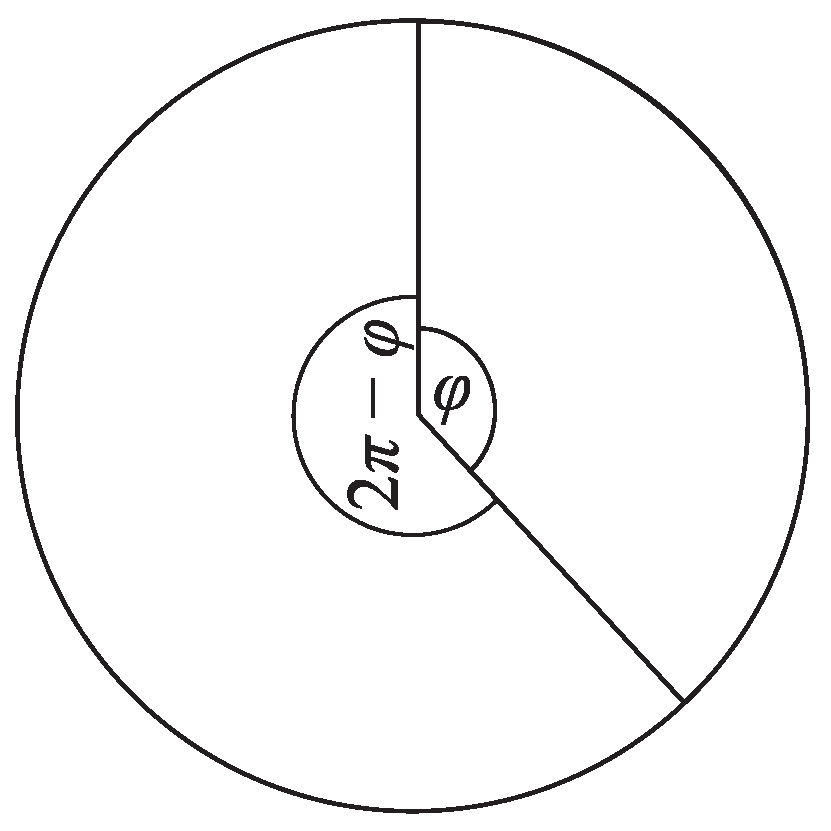
Figure 8.
Metallic angles (solid) and ratios (dotted), positive (red), negative (green), as continuous functions of .
Figure 8.
Metallic angles (solid) and ratios (dotted), positive (red), negative (green), as continuous functions of .

Table 1.
Pythagorean triples associated with metallic ratios for rational .
| n | a | b | c | n | a | b | c |
|---|---|---|---|---|---|---|---|
| 0.1 | -399 | 40 | 401 | 3.6 | 28 | 45 | 53 |
| 0.2 | -99 | 20 | 101 | 3.7 | 969 | 1480 | 1769 |
| 0.3 | -391 | 120 | 409 | 3.8 | 261 | 380 | 461 |
| 0.4 | -12 | 5 | 13 | 3.9 | 1121 | 1560 | 1921 |
| 0.5 | -15 | 8 | 17 | 4 | 3 | 4 | 5 |
| 0.6 | -91 | 60 | 109 | 4.1 | 1281 | 1640 | 2081 |
| 0.7 | -351 | 280 | 449 | 4.2 | 341 | 420 | 541 |
| 0.8 | -21 | 20 | 29 | 4.3 | 1449 | 1720 | 2249 |
| 0.9 | -319 | 360 | 481 | 4.4 | 48 | 55 | 73 |
| 1 | -3 | 4 | 5 | 4.5 | 65 | 72 | 97 |
| 1.1 | -279 | 440 | 521 | 4.6 | 429 | 460 | 629 |
| 1.2 | -8 | 15 | 17 | 4.7 | 1809 | 1880 | 2609 |
| 1.3 | -231 | 520 | 569 | 4.8 | 119 | 120 | 169 |
| 1.4 | -51 | 140 | 149 | 4.9 | 2001 | 1960 | 2801 |
| 1.5 | -7 | 24 | 25 | 5 | 21 | 20 | 29 |
| 1.6 | -9 | 40 | 41 | 5.1 | 2201 | 2040 | 3001 |
| 1.7 | -111 | 680 | 689 | 5.2 | 72 | 65 | 97 |
| 1.8 | -19 | 180 | 181 | 5.3 | 2409 | 2120 | 3209 |
| 1.9 | -39 | 760 | 761 | 5.4 | 629 | 540 | 829 |
| 2 | 5.5 | 105 | 88 | 137 | |||
| 2.1 | 41 | 840 | 841 | 5.6 | 171 | 140 | 221 |
| 2.2 | 21 | 220 | 221 | 5.7 | 2849 | 2280 | 3649 |
| 2.3 | 129 | 920 | 929 | 5.8 | 741 | 580 | 941 |
| 2.4 | 11 | 60 | 61 | 5.9 | 3081 | 2360 | 3881 |
| 2.5 | 9 | 40 | 41 | 6 | 4 | 3 | 5 |
| 2.6 | 69 | 260 | 269 | 6.1 | 3321 | 2440 | 4121 |
| 2.7 | 329 | 1080 | 1129 | 6.2 | 861 | 620 | 1061 |
| 2.8 | 12 | 35 | 37 | 6.3 | 3569 | 2520 | 4369 |
| 2.9 | 441 | 1160 | 1241 | 6.4 | 231 | 160 | 281 |
| 3 | 5 | 12 | 13 | 6.5 | 153 | 104 | 185 |
| 3.1 | 561 | 1240 | 1361 | 6.6 | 989 | 660 | 1189 |
| 3.2 | 39 | 80 | 89 | 6.7 | 4089 | 2680 | 4889 |
| 3.3 | 689 | 1320 | 1489 | 6.8 | 132 | 85 | 157 |
| 3.4 | 189 | 340 | 389 | 6.9 | 4361 | 2760 | 5161 |
| 3.5 | 33 | 56 | 65 | 7 | 45 | 28 | 53 |
For set . E.g. for n = −7, {45,28,53}↔{45, −28,53}.
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Copyright: This open access article is published under a Creative Commons CC BY 4.0 license, which permit the free download, distribution, and reuse, provided that the author and preprint are cited in any reuse.
MDPI Initiatives
Important Links
© 2024 MDPI (Basel, Switzerland) unless otherwise stated






