Preprint
Article
On the Dual Ontological Structure and Mixed Dynamics of the Universe
Altmetrics
Downloads
184
Views
138
Comments
0
This version is not peer-reviewed
Submitted:
07 January 2024
Posted:
09 January 2024
You are already at the latest version
Alerts
Abstract
Ontological premises have profound theoretical implications. This analysis posits a single, universal structure composed of two ontic entities: discrete, quantized units of space ("Planck Spheres") and a State of Absolute Nothingness (the "SOAN"). Planck Spheres and the SOAN form a physical, ultra-high (3 x N) dimensional space ("Planck Space") and a discrete 3D space (or "4D spacetime"). Together, Planck Space and 4D spacetime form a tightly integrated ((3 x N) + 3) hyperspace (the "Dual Ontology"). Critically, a one-to-one mapping and identity exists between the Planck Spheres comprising 4D spacetime and Planck Space. The Dual Ontology conjecture supports radically simplified physical explanations for three distinct areas of theoretical physics: First, the conjecture reconciles fundamental issues in Special Relativity and quantum mechanics by explaining why single and N-body quantum states (a) dynamically evolve in 4D spacetime subject to the speed of light and (b) instantaneously collapse in a physical Planck Space that is not subject to Special Relativity. Second, the temporal and physical asymmetry between the dynamic evolution of quantum states in 4D spacetime subject to Special Relativity and the instantaneous collapse of quantum states in a Planck Space where Special Relativity is inapplicable explains quantum path irreversibility and the arrow of time. Third, at or near the heat death of 4D spacetime, the instantaneous collapse of the energy content of Planck Space and the simultaneous transition of 4D spacetime's extremely widely dispersed energy content to a non-singular, generally localized volume at t = 0 explains 4D spacetime's isotropy, homogeneity, extremely high energy, pressure, and temperature, flatness, and low gravitational entropy. It also explains the horizon and fine-tuning problems.
Keywords:
Subject: Physical Sciences - Quantum Science and Technology
1. The Dual Ontology Conjecture and Synopsis
1.1. Introduction
The Dual Ontology conjecture posits a single, universal structure composed of two ontic entities: discrete, quantized units of space ("Planck Spheres") and a State of Absolute Nothingness (the "SOAN") without positive physical properties or structure.1Planck Spheres and the SOAN form a physical, ultra-high (3 x N) dimensional "Planck Space" and a 3D space (or "4D spacetime")." Together, Planck Space and 4D spacetime form a tightly integrated ((3 x N) + 3) hyperspace (the "Dual Ontology"). A one-to-one mapping and identity between the Planck Spheres comprising 4D spacetime and Planck Space ensures that the dynamic movement and collapse of all quantum states are reflected simultaneously in 4D spacetime and Planck Space.2 3
Unlike a mathematical 3N quantum configuration space, Planck Space is a physical ultra-high (3 x N) dimensional space in the form of an ordered N-tuple of ordered triples where each Planck Sphere represents a unique set of x, y, and z coordinates.4 Each N in Planck Space forms a single three-dimensional space. Planck Space does not have a time dimension nor the physical properties of space or volume. Many physical laws of 4D spacetime, including Special and General Relativity and the second law of thermodynamics, do not apply to Planck Space.5
The Dual Ontology conjecture:
- Reconciles fundamental issues in Special Relativity and quantum mechanics by explaining why all quantum states (a) dynamically evolve in 4D spacetime subject to the speed of light and (b) instantaneously collapse in a physical Planck Space that is not subject to Special Relativity.
- Holds that the temporal and physical asymmetry between the dynamic evolution of quantum states in a 4D spacetime governed by the speed of light and the collapse of all quantum states in a Planck Space where Special Relativity is inapplicable is the physical source of quantum path irreversibility and the arrow of time.
- Explains why, at or near 4D spacetime’s heat death, the instantaneous collapse of the energy content of Planck Space and the simultaneous transition of 4D spacetime's widely dispersed energy content to a non-singular, generally localized volume at t = 0 is the physical cause for 4D spacetime's isotropy, homogeneity, extremely high energy, pressure and temperature, flatness, and low gravitational entropy. The collapse process also explains the horizon and fine-tuning problems.
Several preliminary points are noted before a synopsis of the Dual Ontology conjecture. First, the analysis does not attempt to determine the origin of the SOAN, Planck Spheres, 4D spacetime, Planck Space, or the energy content of the Dual Ontology. Second, the ontological existence of the SOAN and Planck Spheres is coeval, as is the ontological existence of 4D spacetime and Planck Space. Third, the analysis does not consider the implications of quantum gravity. Fourth, the Dual Ontology, rather than 4D spacetime, represents a closed physical system. Fifth, subatomic entities are "quantum states," not "particles." All quantum states are ontic, but the mathematical wavefunctions that describe the dynamic evolution of all quantum states in 4D spacetime and their collapse in Planck Space are not.6
1.2. The Dual Ontology's Structure
The analysis begins with the Dual Ontology's fundamental ontological units: the SOAN and Planck Spheres. The SOAN is a concept considered extensively by philosophers but largely ignored by physicists.7 Other than "onticness," the absence of other positive attributes characterizes the SOAN.8 The SOAN has no space, time, size, structure, matter, energy, gravity, or boundary. Consequently, 4D spacetime's physical laws do not apply to the SOAN.
In contrast, Planck Spheres are discrete quanta of volume.9 All Planck Spheres, or their equivalent, are the same size, with a volume of approximately 2.2 ×10−108 cubic meters.10 As the smallest independent structural unit in the universe, no smaller space units exist within the interstices of Planck Spheres. Instead, the SOAN rather than "space" is ontic within the interstices of Planck Spheres.
Together, the SOAN and Planck Spheres form 4D spacetime and Planck Space. 4D spacetime and Planck Space form the Dual Ontology's tightly integrated ((3 x N) + 3) hyperspace.11 Critically, on a one-to-one mapping and identity basis, an individual Planck Sphere in 4D spacetime corresponds to the same Planck Sphere in Planck Space (the "Planck Identity"). At the microscopic level, the x, y, and z coordinates of each of the Planck Spheres that form 4D spacetime not only map directly to the same Planck Spheres in Planck Space but also the physical attributes of the individual Planck Spheres are identical.
Cosmologically, the Planck Identity also ensures that the energy content of the Planck Spheres that comprise 4D spacetime and Planck Space is identical. Accordingly, the energy content of the Planck Spheres that comprise 4D spacetime (the “4D Energy Field”) equals the energy content of Planck Space (the “Planck Energy Hyper-Point”).
1.3. The Dual Ontology and Quantum State Dynamics
The existence of a physical, ultra-high dimensional Planck Space not only explains why single and N-body quantum states dynamically evolve in 4D spacetime subject to the laws of Special Relativity but also why all quantum states instantaneously collapse in Planck Space, where Special Relativity is inapplicable. In the case of a single quantum state, for example, as the quantum state dynamically evolves in 4D spacetime, it physically occupies Planck Spheres. Each Planck Sphere occupied by a quantum state forms a "Bell Quantum Sphere" in 4D spacetime. A one-to-one mapping and identity exists between each Bell Quantum Sphere in 4D spacetime and the same Bell Quantum Sphere in Planck Space (the "Bell Identity"). Cumulatively, all the Bell Quantum Spheres occupied by a quantum state simultaneously form the quantum state's "Bell Quantum Field" in 4D spacetime and its ultra-high dimensional "Bell Quantum Hyper-Point" in Planck Space.
In the case of an electron in the ground state of a hydrogen atom, the 1.92 x 1074 Bell Quantum Spheres that comprise a quantum state's Bell Quantum Field in 4D spacetime simultaneously comprise the electron's ultra-high dimensional (3 x 1.92 x 1074) Bell Quantum Hyper-Point in Planck Space.12 Consequently, the physical attributes of a quantum state, including its energy content, are simultaneously represented by the Bell Quantum Spheres that comprise the quantum state's Bell Quantum Field and its Bell Quantum Hyper-Point.
The dynamic evolution of an N-body quantum state is similar to that of a single quantum state. Following the generation of a bipartite quantum state, the system forms two separate Bell Quantum Fields in 4D spacetime. Simultaneously, the bi-partite quantum state forms a single ultra-high dimensional Bell Quantum Hyper-Point in Planck Space. Assume that one of the two electrons that comprise the bipartite quantum state is sent to Princeton and the other to Copenhagen. As the system spreads, the number of Bell Quantum Spheres that comprise the system's two Bell Quantum Fields and its single Bell Quantum Hyper-Point increases. The Bell Identity ensures that there is always a one-to-one mapping and identity between the Bell Quantum Spheres that comprise the bi-partite quantum state's two Bell Quantum Fields in 4D spacetime and its single Bell Quantum Hyper-Point in Planck Space.
The collapse of a quantum state's Bell Quantum Hyper-Point is an instantaneous event in Planck Space and 4D spacetime.13 14 In the case of a single quantum state, the instantaneous collapse of the quantum state's Bell Quantum Hyper-Point causes a decrease in the number of Bell Quantum Spheres that comprise a quantum state's Bell Quantum Hyper-Point in Planck Space. Simultaneously, the Bell Identity ensures that the decrease in the number of Bell Quantum Spheres that comprise the newly collapsed Bell Quantum Hyper-Point is reflected on a one-to-one basis with a reduction in the number of Bell Quantum Spheres that comprise the quantum state's new Bell Quantum Field in 4D spacetime.
Following the instantaneous reduction in the number of Bell Quantum Spheres that comprise the quantum state's Bell Quantum Field, the quantum state is now generally localized in 4D spacetime.15 Nevertheless, Special Relativity has not been violated. The generalized localization of the quantum state is an event extraneous to 4D spacetime caused by 1) the collapse of the quantum state's Bell Quantum Hyper-Point, 2) the instantaneous reduction in the number of Bell Quantum Spheres that comprise the newly collapsed Bell Quantum Hyper-Point, and 3) the simultaneous decrease in the number of Bell Quantum Spheres that comprise the quantum's state's generally localized Bell Quantum Field in 4D spacetime.
In the slightly more complicated case of a bipartite quantum state, concurrently with the instantaneous collapse of its Bell Quantum Hyper-Point, the quantum state transitions from an entangled state to a product state. Using a notation based on a physical (3 x N) Planck Space rather than a mathematical 3N space, the transition would be ψ1,2((x1,y1,z1)1,(x2,y2,z2)2) → ψ1(x1,y1,z1)1 ⊗ ψ2(x2,y2,z2)2.16 As a result, quantum state x1 forms Bell Quantum Hyper-Point x1 in Planck Space, and quantum state x2 forms Bell Quantum Hyper-Point x2. The collapse of the bipartite quantum state reduces the number of Bell Quantum Spheres that comprise Bell Quantum Hyper-Point x1 and Bell Quantum Hyper-Point x2, respectively. Simultaneously, the Bell Identity ensures that the reduction in the number of Bell Quantum Spheres that comprise Bell Quantum Hyper-Point x1, and Bell Quantum Hyper-Point x2 is instantaneously reflected in the number of Bell Quantum Spheres comprising Bell Quantum Field x1 and Bell Quantum Field x2 in 4D spacetime. Bell Quantum Field x1 and Bell Quantum Field x2 are now generally localized in 4D spacetime, but Special Relatively has not been violated.17
The Bell Identity serves a secondary function: the strict one-to-one mapping and identity between the Bell Quantum Spheres of a quantum state in 4D spacetime and Planck Space ensures that a quantum state's Bell Quantum Field cannot suddenly appear anywhere in 4D spacetime. A quantum state's generally localized position in 4D spacetime after a collapse must be to a subset of the Bell Quantum Spheres that formerly comprised the quantum state's Bell Quantum Hyper-Point and its Bell Quantum Field immediately before the quantum state's collapse.18
1.4. Quantum Path Irreversibility
The Dual Ontology conjecture posits that "quantum path irreversibility" following the instantaneous collapse of a quantum state in Planck Space is an absolute physical impossibility rather than extremely unlikely or nearly impossible. Stated most succinctly, the space and time-asymmetry between the dynamic evolution of all quantum states in 4D spacetime subject to Special Relativity’s maximum speed of light and the instantaneous collapse of all quantum systems in Planck Space, where Special Relativity is inapplicable, precludes quantum path reversibility regardless of whether a quantum state is or is not space-like separated.
Briefly, assume quantum states z1 and z2 are in the singlet state in the z-direction. In 4D spacetime, the entangled state forms Bell Quantum Field z1 near Mars and Bell Quantum Field z2 near Earth. In Planck Space, quantum states z1 and z2 form Bell Quantum Hyper-Point z1z2. Following the instantaneous collapse of Bell Quantum Hyper-Point z1z2, the z1 and the z2 quantum states form a product state in Planck Space ψ1,2((x1,y1,z1)1,(x2,y2,z2)2) → ψ1(x1,y1,z1)1 ⊗ ψ2(x2,y2,z2)2,. The formerly entangled singlet state now forms Bell Quantum Hyper-Point z1 and Bell Quantum Hyper-Point z2 in Planck Space and Bell Quantum Field z1 and Bell Quantum Field z2 in 4D spacetime, respectively. The collapse instantaneously reduces the number of Bell Quantum Spheres that comprise Bell Quantum Hyper-Point z1 and z2 in Planck Space and the number of Bell Quantum Spheres comprising Bell Quantum Fields z1 and z2 in 4D spacetime. Bell Quantum Field z1 is now generally localized near Mars, approximately 225,000,000 miles from Bell Quantum Field z2, which is now generally localized near Earth.
Given the physical structure of the Dual Ontology and the difference between the dynamic laws that govern the spreading of the z1 and z2 quantum states in 4D spacetime and the instantaneous collapse of the singlet state in Planck Space, path reversibility is now physically impossible. Path reversibility requires the instantaneous path reversal along the identical collapse path taken by the entangled quantum state's Bell Quantum Hyper-Point in Planck Space. Under the Dual Ontology conjecture, however, no such physical structure exists. The z1 and z2 quantum states can only spread in 4D spacetime and only do so subject to the laws of Special Relativity. They must travel at least 112,500,000 miles to become entangled once more.
Accordingly, following the collapse of any quantum state (or the quantum states that comprise a macrostate, including the proverbial egg), the asymmetrical spatial and temporal differences between the dynamic evolution of the quantum state in 4D spacetime and its collapse in Planck Space preclude quantum path reversibility. The increased entropy of a cracked egg in 4D spacetime is directly related to the physical process associated with the collapse of the cracked egg's quantum states in Planck Space. As a result, in 4D spacetime, the arrow of time moves in a single temporal direction.
1.5. The Dual Ontology at t = 0 and Heat Death
The Dual Ontology's structure and dynamics form the basis for re-evaluating the cosmogony and cosmology of 4D spacetime. The conjecture posits that the instantaneous collapse of the Planck Energy Hyper-Point at or near 4D spacetime's heat death simultaneously causes a reduction in the number of Planck Spheres that comprise 4D spacetime's 4D Energy Field.19 The reduction not only causes 4D spacetime's instantaneous transition from an extremely widely separated physical state at heat death to a generally localized status at t = 0 but also explains 4D spacetime's isotropy, homogeneity, flatness, and low gravitational entropy at t = 0 and the horizon and flatness problems.
1.5.1. 4D Spacetime at t = 0 and Heat Death
The analysis begins by briefly comparing 4D spacetime's ontological status at two temporal bookends, t = 0 and heat death. As we have seen, the Dual Ontology conjecture posits a discrete 4D spacetime composed of Planck Spheres and the SOAN instead of General Relativity's dimensionless initial singularity. Consequently, at t = 0, the Dual Ontology replaces General Relativity's divide by zero mathematics and infinite curvature, density, pressure, and temperature with a generally localized 4D spacetime characterized by extreme, but not infinite, energy densities, pressures, and temperatures.
Using the CMB as a baseline, approximately 380,000 years after t = 0, the temperature anisotropies of the CMB indicate that temperature variations across the sky varied by approximately 1 part in 105.20 Based on these variations, the CMB indirectly supports 4D spacetime's nearly isotropic and homogenous energy density, pressure, and temperature at t =0 and its near thermodynamic equilibrium. It also suggests the existence of anisotropies and inhomogeneities. When the angular power spectrum around the first peak of the anisotropies of the CMB is extrapolated backward toward t = 0, the temperature variations indirectly support a 4D spacetime curvature that appears nearly flat (k = 0), and that 4D spacetime's total energy density equaled the critical density ρ4D = ρcrit.21 Although 4D spacetime's energy density and pressure entropy were nearly maximal, the presence of anisotropies and inhomogeneities, the future expansion of 4D spacetime, and the capacity of 4D spacetime to do work all support a near-zero gravitational entropy.22
At or near heat death, 4D spacetime is in a state of near or maximum entropy. Its energy density, pressure, and temperature are nearly homogeneous and isotropic and asymptotically approach zero. 4D spacetime is in a state of near thermodynamic equilibrium. 4D spacetime has no large-scale structures, is extremely widely dispersed, and its spatial curvature is flat or nearly flat (k =0). Since little or no heat can flow in a near-zero thermal equilibrium, physical changes cannot occur at the macrostate level in 4D spacetime. Without work, little or no additional gravitational clumping can occur. Accordingly, although 4D spacetime's spatial geometry, energy density, and pressure are homogeneous and isotropic, 4D spacetime is in a state of near-maximal gravitational entropy.
Beginning with the similarities between the two temporal periods, 4D spacetime's energy density, pressure, and temperature are nearly homogeneous and isotropic, and its energy density and pressure are near maximum entropy. 4D spacetime is also near thermodynamic equilibrium. 4D spacetime's spatial curvature is nearly flat, and, as a result, 4D spacetime's total energy density is nearly equal to the critical density. 4D spacetime does not have any large-scale structures at either time.23
Significant dissimilarities exist between 4D spacetime at t = 0 and near heat death. At heat death, 4D spacetime's spatial structure is widely dispersed, its energy density, pressure, and temperature are extremely low, and its gravitational entropy is near maximal. In contrast, 4D spacetime is generally localized at t = 0, its energy density, pressure, and temperature are extremely high, and its gravitational entropy state is very low.
1.5.2. Planck Space at t = 0 and Heat Death
Although the structural status of Planck Space at t = 0 and heat death remains the same, the Planck Identity ensures that dynamic changes to the energy content of the individual Planck Spheres that comprise 4D spacetime's 4D Energy Field and Planck Space's Planck Energy Hyper-Point occur simultaneously. Accordingly, at t = 0 and heat death, the energy density, pressure, and temperature of the individual Planck Spheres that comprise the 4D Energy Field and the Planck Energy Hyper-Point are identical. At t = 0, the energy density, pressure, and temperature of the Planck Spheres that comprise the Planck Energy Hyper-Point were not extremely high but also highly homogeneous. In contrast, at heat death, the Planck Energy Hyper-Point's Planck Spheres remain homogeneous, but their energy density, pressure, and temperature asymptotically approach zero.
1.5.3. The Collapse of the Planck Energy Hyper-Point
Although the preceding analysis clarifies the ontological status and relationship of 4D spacetime and Planck Space at t = 0 and heat death, it is the instantaneous collapse of the Planck Energy Hyper-Point that explains 4D spacetime's transition from heat death to t = 0. The collapse of the Planck Energy Hyper-Point is similar to the collapse of an ultra-high dimensional N-partite Bell Quantum Hyper-Point. The Planck Identity ensures that the collapse of the Planck Energy Hyper-Point simultaneously reduces the number of Planck Spheres that contain the energy content of the Planck Energy Hyper-Point and the 4D Energy Field.
Regardless of the extremely wide spatial size of the 4D Energy Field at heat death, the instantaneous reduction in the number of Planck Spheres that comprise 4D spacetime's new 4D Energy Field results in the discrete, generalized localization of 4D spacetime at t = 0. In turn, the generalized localization of the 4D Energy Field instantly transforms 4D spacetime's energy density, pressure, and temperature from near-zero at heat death to extremely high energy density, pressure, and temperature at t = 0.24 Instantaneity has a secondary effect: it ensures that the localization process does not introduce temporal deviations that alter the homogeneity or isotropy of 4D spacetime's energy density, pressure, or temperature at t = 0.
The collapse of the Planck Energy Hyper-Point also explains 4D spacetime's instantaneous transition from a state of near maximal gravitational entropy at heat death to near-zero gravitational entropy at t = 0. Following the generalized localization of the 4D Energy Field at t = 0, the combination of 4D spacetime's extreme energy density, pressure, and temperature, the presence of anisotropies and inhomogeneities, and 4D spacetime's future expansion all contribute to the transition from a state of near-maximal gravitational entropy at heat death to near-zero gravitational entropy at t = 0.
1.5.4. The Horizon Problem and Causality
The inability to explain the exceptionally high homogeneity and isotropy of 4D spacetime at or near t = 0 is the source of the horizon problem. The Dual Ontology's resolution of the causality problem is premised upon three critical factors. First, the Dual Ontology replaces the concept of an initial singularity at t = 0 with a discrete 4D spacetime comprised of Planck Spheres and the SOAN. Second, the near maximal homogeneity and isotropy of the 4D Energy Field at heat death result from the expansion and cooling of 4D spacetime over extremely long-time scales. Expansion and cooling are internal physical processes without independent or external parameters and apply whether or not disparate regions of 4D spacetime were ever in causal contact.
Third, the instantaneous collapse of the Planck Energy Hyper-Point and the generalized localization of the 4D Energy Field at t = 0 does not alter the homogeneity or isotropy of 4D spacetime's energy density, pressure, or temperature, nor does it alter the existence of anisotropies and inhomogeneities at or near the heat death of 4D spacetime.
1.5.5. The Flatness Problem and Fine-Tuning
The precision of 4D spacetime's flatness at t = 0 and the sensitivity of 4D spacetime's spatial curvature to small deviations from flatness is known as the flatness problem. The Dual Ontology conjecture does not provide a theoretical basis or physical data that explains why the total energy or the total energy density of the 4D Energy Field has the particular values that it does. Nevertheless, the fine-tuning of independent or external parameters is not required to maintain a flat 4D spacetime (k = 0) from t = 0 to heat death or from heat death to t =0. In other words, ρ4DEF = ρcrit from t = 0 to heat death and from heat death back to t =0. Flatness is an internal condition of the Dual Ontology, based upon its dual ontological structure and mixed dynamics, the physical properties and laws governing 4D spacetime, and the physical properties and laws that govern (and do not govern) Planck Space.
1.6. Analytical Structure
The specific sections of the analysis are structured as follows. Section 1 contains an introduction and a synopsis of the conjecture. Section 2 introduces the SOAN, Planck Spheres, the Planck Identity, and Planck Space. Section 3 examines the dynamic evolution of all quantum states in 4D spacetime, the instantaneous collapse of all quantum states in Planck Space, and the role of both the Bell Identity and the Bell Quantum Hyper-Point in the collapse process. Section 3 also reviews the EPR thought experiment, the double-slit experiment, and a which-way experiment from the perspective of the Dual Ontology conjecture. Section 4 reviews the physical implications of the Dual Ontology conjecture, including quantum indeterminacy, quantum state emergence and annihilation, the physical triggers of quantum state collapse, and the generalized localization of a quantum state following the collapse of a Bell Quantum Hyper-Point. Section 5 reviews the historical tension between Special Relativity and quantum mechanics. Section 6 describes the physical basis for quantum path irreversibility and the arrow of time. Section 7 provides a physical explanation for the instantaneous collapse of the Planck Energy Hyper-Point and 4D Spacetime's simultaneous transition at or near heat death to a generally localized, non-singular volume at t = 0. Section 8 contains a short conclusion.
2. The Physical Structure of the Universe
2.1. The State of Absolute Nothingness
4D spacetime's physical laws do not apply to a State of Absolute Nothingness. Nevertheless, a central premise of this analysis is that the SOAN possesses a single physical characteristic that is fundamental to the structure of the Dual Ontology: it is ontic. A "state of absolute nothingness" or simply "nothingness" in cosmology and quantum mechanics has varied interpretations. For this analysis, in a State of Absolute Nothingness:25
There is no spacetime, dimension, boundary, size, structure, volume, gravity, energy, pressure, temperature, force, fields, ground states, vacuum states, virtual particles, quantum fluctuations, dynamical properties, frame of reference, matter, strings, information, mathematical entities, potentials, concepts, abstractions, consciousness, physical laws, possibilities, or entropy.26 27
The SOAN's highly restrictive definition means it plays no active role in the universe. Nevertheless, the absence of positive attributes other than onticness is a feature, not a bug. The very absence of physical characteristics and laws creates a physical environment in which the SOAN fundamentally alters the physical structure and dynamics of the universe.
It is impossible to determine directly whether the SOAN is ontic. Nevertheless, over 100 years of quantum experiments, the physical basis for quantum irreversibility and the arrow time and 4D spacetime's instantaneous transition from heat death to t = 0 all indirectly suggest that the SOAN is not only ontic but also plays a fundamental physical role in the universe's Dual Ontology.
2.2. Planck Spheres, 4D Spacetime, and Planck Space
Whether space is continuous or discrete is an unsettled and controversial subject.28 HHeiHeHHeisHeidafd eH29 In general, quantum gravity theories view the discretization of space as a critical concept. In addition to preventing singularities at the center of black holes or upon the initial emergence of the 4D spacetime at t = 0, the discretization of space prevents the infinities that appear in the quantization of General Relativity.30 31
The analysis makes several basic assumptions regarding Planck Spheres. First, an individual Planck Sphere and the SOAN represent a discrete, ontic unit in 4D spacetime and Planck Space.32 Second, an individual Planck Sphere is the smallest structural unit in the universe. Third, a one-to-one mapping and identity exists between a Planck Sphere in 4D spacetime and the same Planck Sphere in Planck Space. Consequently, the x, y, and z coordinates that identify a Planck Sphere in 4D spacetime also identify the same Planck Sphere in Planck Space (the "Planck Identity").
In Planck Space, every Planck Sphere represents a (3 x N) dimensional space where N equals 1. Structurally, all of the Planck Spheres in the observable and unobservable universe form an ultra-high dimensional space composed of N-tuples of ordered triples; Qn = ((x1,y1,z1),(x2,y2,z2),...,(xn, yn, zn)).33 Moreover, any combination of (3 x N) dimensions where N is 2 or greater also forms an ultra-high dimensional space. Accordingly, unlike a mathematical 3N quantum configuration space composed of mutually orthogonal vectors,34 the 5.58 x 10186 Planck Spheres that currently comprise the observable portion of 4D spacetime also form an ultra-high dimensional Planck Energy Hyper-Point in Planck Space composed of (3 x 5.58 x 10186) dimensions. Although the number of Planck Spheres in Planck Space can be counted, individual Planck Spheres do not represent a specific numbered dimension.
Finally, within the (3 x N) structure of Planck Space, there is no concept of time, nor is there any space, spatial curvature, volume, or gravity.35 Accordingly, the laws of Special and General Relativity, the 2nd law of thermodynamics, and gravitational entropy, among other 4D spacetime laws, are inapplicable in Planck Space.
3. Quantum States and the Dual Ontology
A central premise of the Dual Ontology conjecture is that the traditional dichotomy between the dynamic evolution of a single, physical quantum state in 4D spacetime and the dynamic evolution of a non-physical N-body quantum state in a 3N configuration space is unwarranted.36 Instead, the Dual Ontology conjecture not only posits that all quantum states are "real" and dynamically evolve in 4D spacetime but also that the collapse of all quantum states is a physical process that occurs in a physical ultra-high dimensional Planck Space. More generally, there is no theoretical or experimental basis to support different laws for single and N-body quantum states.
3.1. Single Quantum States and 4D Spacetime
The analysis begins with the dynamic evolution of a single quantum state in 4D spacetime. As the quantum state dynamically evolves in 4D spacetime, it occupies Planck Spheres. Each Planck Sphere occupied by a quantum state constitutes a Bell Quantum Sphere, and a one-to-one mapping and identity exists between a Bell Quantum Sphere in 4D spacetime and the same Bell Quantum Sphere in Planck Space (the "Bell Identity"). Consequently, the Bell Identity holds that the x, y, and z coordinates of a Bell Quantum Sphere not only map to the same Bell Quantum Sphere in 4D spacetime and Planck Space but also the physical attributes of the Bell Quantum Sphere are identical in both spaces.37
Cumulatively, all the Bell Quantum Spheres occupied by a quantum state simultaneously form the quantum state's "Bell Quantum Field" in 4D spacetime and its ultra-high dimensional "Bell Quantum Hyper-Point" in Planck Space. For example, in the case of an electron in the ground state of a hydrogen atom, the 1.92 x 1074 Bell Quantum Spheres that comprise the electron's Bell Quantum Field in 4D spacetime simultaneously form an ultra-high dimensional (3 x 1.92 x 1074) Bell Quantum Hyper-Point in Planck Space.
As a quantum state spreads in 4D spacetime, the number of Bell Quantum Spheres occupied by the quantum state increases. The Bell Identity ensures that the increase in the number of Bell Quantum Spheres that comprise the quantum state's Bell Quantum Field in 4D spacetime is simultaneously reflected in an increase in the number of Bell Quantum Spheres that comprise the quantum state's Bell Quantum Hyper-Point in Planck Space. Accordingly, on a one-to-one basis, as the number of Bell Quantum Spheres occupied by the quantum state in 4D spacetime increases, the number of Bell Quantum Spheres comprising the quantum state's Bell Quantum Hyper-Point also increases.
3.1.1. The Collapse of a Single Quantum State
The Bell Identity not only links the dynamic evolution of a quantum state's Bell Quantum Field in 4D spacetime with its Bell Quantum Hyper-Point in Planck Space, but it also links the collapse of a quantum state's Bell Quantum Hyper-Point in Planck Space with its Bell Quantum Field in 4D spacetime. More specifically, the Bell Identity links the instantaneous decrease in the number of Bell Quantum Spheres that comprise a quantum state's Bell Quantum Hyper-Point in Planck Space with a simultaneous reduction in the number of Bell Quantum Spheres that comprise the quantum state's Bell Quantum Field in 4D spacetime.38
For example, assume Box A is a three-dimensional box with impenetrable walls. Box A is 2 inches wide, 1 inch deep, and 1 inch tall. There is zero potential inside Box A. The instant a single quantum state ("quantum state A") is generated, it simultaneously forms Bell Quantum Field A in 4D spacetime and Bell Quantum Hyper-Point A in Planck Space. Quantum state A is inserted into Box A. The quantum state can spread throughout Box A but never has zero energy.

As quantum state A spreads, the Bell Identity ensures that the increase in the number of Bell Quantum Spheres that comprise Bell Quantum Field A is simultaneously reflected in a one-to-one increase in the number of Bell Quantum Spheres that comprise Bell Quantum Hyper-Point A. The opening of Box A triggers the instantaneous collapse of Bell Quantum Hyper-Point A.39 The collapse instantaneously reduces the number of Bell Quantum Spheres that formerly composed Bell Quantum Hyper-Point A, and the Bell Identity ensures that the collapse is simultaneously linked to the instantaneous reduction in the number of Bell Quantum Spheres that now comprise Bell Quantum Field A. Quantum state A is now generally localized in Box A and begins to spread again.40.
3.1.2. The Einstein/de Broglie Box Thought Experiment
The Einstein/de Broglie Box thought experiment is a more complex example of the dynamics of a single quantum state.41 Assume Box B is initially identical to Box A. A single quantum state ("quantum state B") is generated, simultaneously forming Bell Quantum Field B in 4D spacetime and Bell Quantum Hyper-Point B in Planck Space. Quantum state B is inserted into Box B and begins to spread. As quantum state B spreads, the number of Bell Quantum Spheres that comprise Bell Quantum Field B within Box B increases, and so does the number of Bell Quantum Spheres that form Bell Quantum Hyper-Point B in Planck Space.
Without becoming entangled with quantum state B, an impenetrable divider is inserted into the middle of Box B, separating it into two identical boxes, C and D. Ignoring the physical dimensions of the divider, Box C and Box D are now 1 inch wide, 1 inch deep, and 1inch tall. Inside Boxes C and D, the potential energy remains zero.

The insertion of the impenetrable divider has separated the quantum state into two equal Bell Quantum Fields in 4D spacetime: Bell Quantum Field C and Bell Quantum Field D. Nevertheless, the quantum state continues to form a single Bell Quantum Hyper-Point in Planck Space, now designated as "Bell Quantum Hyper-Point CD." The mathematical wave function indicates that the quantum state is in a superposition of location with a 50% chance that following the quantum state's collapse, the quantum state is found in Box B and a 50% chance that the quantum state is found in Box C.
Box C remains in Princeton, and Box D is shipped to Copenhagen, 6,252 kilometers away. Although the movement of Box D's physical location has altered the x, y, and z coordinates of the Bell Quantum Spheres that comprise Bell Quantum Field D in 4D spacetime, the Bell Quantum Spheres that comprise Bell Quantum Field C and Bell Quantum Field D continue to comprise Bell Quantum Hyper-Point CD. In other words, so long as Bell Quantum Hyper-Point CD does not collapse, the quantum state remains in a superposition of location with a 50% chance of being found in Box C and a 50% chance of being found in Box D.
Regardless of whether Box B or Box C is opened first, the opening of either box is the physical trigger that causes the collapse of Bell Quantum Hyper-Point CD. In turn, the collapse instantaneously reduces the number of Planck Spheres that formerly comprised Bell Quantum Hyper-Point CD. The reduction in the number of Planck Spheres that formerly comprised Bell Quantum Hyper-Point CD has several significant ramifications.
If Box C is opened first and the entire quantum state ψc (x1,y1,z1)c is found in Box C, 1) the number of Planck Spheres that comprise Bell Quantum Field C and Bell Quantum Hyper-Point C have been instantaneously reduced, 2) Bell Quantum Field C is generally localized in Box C, and 3) there is a one to one mapping and identity between the Bell Quantum Spheres that comprise Bell Quantum Field C in 4D spacetime and Bell Quantum Hyper-Point C in Planck Space. In addition, the mathematical wave function formerly representing Bell Quantum Field D and Bell Quantum Hyper-Point D no longer has meaning. A similar analysis applies if Box C is opened and the quantum state is not found in Box C or if Box D is opened first and the quantum state is or is not found in Box D.
Finally, the collapse of Bell Quantum Hyper-Point CD is not a "jump in 4D spacetime."42 Recall that a Bell Quantum Hyper-Point is an ultra-high dimensional point in a Planck Space where time, space, and volume do not exist. When Bell Quantum Hyper-Point CD collapses, there is a reduction in the number of dimensions that comprise the quantum state's new Bell Quantum Hyper-Point, and the Bell Identity ensures that there is a simultaneous reduction in the number of Bell Quantum Spheres that comprise the quantum state's new Bell Quantum Field. What appears to be a "jump" in 4D spacetime is the collapse of Bell Quantum Hyper-Point CD in Planck Space and the reduction in the number of Bell Quantum Spheres that comprise the quantum state's Bell Quantum Field.43
3.2. N-Body Quantum States
The introduction of the Dual Ontology, the dynamic evolution of all quantum states in 4D spacetime, and their collapse in Planck Space sets the stage for re-examining the ontic status of N-body quantum states. Instead of relying on a 3N configuration space to describe the dynamic motion of N-body quantum states, the Dual Ontology conjecture posits that all N-body quantum states are physical entities that dynamically evolve in a 4D spacetime and collapse in Planck Space.44
3.2.1. Evolution and Collapse of N-Body Quantum States
The issues raised by the dynamic evolution of an N-body quantum state in 4D spacetime and its collapse in Planck Space are highlighted by a re-examination of the Bohm version of the EPR experiment.45 A pair of electrons is prepared in the singlet state. The wave function of the entangled quantum state is in a superposition, and its combined angular momentum is 0. The instant the entangled quantum state is generated, the quantum state simultaneously forms two Bell Quantum Fields in 4D spacetime (Bell Quantum Fields E and F, respectively) and an ultra-high dimensional Bell Quantum Hyper-Point ("Bell Quantum Hyper-Point EF") in Planck Space. Quantum state E is sent toward Princeton, and quantum state F is sent toward Copenhagen. Testing equipment is configured to conduct a Stern-Gerlach experiment in the z direction on either Bell Quantum Field E or Bell Quantum Field F.

Regardless of the space-like separation of Bell Quantum Fields E and F in 4D spacetime, the Bell Identity ensures that the Bell Quantum Spheres that comprise Bell Quantum Fields E and F also comprise Bell Quantum Hyper-Point EF. As Bell Quantum Fields E and F dynamically spread in 4D spacetime, the number of Bell Quantum Spheres that comprise their respective Bell Quantum Fields increases, as does the number of Bell Quantum Spheres that comprise Bell Quantum Hyper-Point EF.
Bell Quantum Hyper-Point EF collapses following a Stern-Gerlach experiment in the z direction in either Princeton or Copenhagen.46 The collapse instantaneously reduces the number of Bell Quantum Spheres that formerly composed Bell Quantum Hyper-Point EF. The Bell Identity ensures that the collapse is simultaneously linked to the reduced number of Bell Quantum Spheres that comprise Bell Quantum Field E and Bell Quantum Field F, respectively. Unlike the case with a single Bell Quantum Hyper-Point, Bell Quantum Hyper-Point EF now forms two independent Bell Quantum Hyper-Points designated as Bell Quantum Hyper-Point E and Bell Quantum Hyper-Point F. Bell Quantum Hyper-Point E shares a one-to-one mapping and identity with the Bell Quantum Spheres that now form Bell Quantum Field E, and Bell Quantum Hyper-Point F shares a one-to-one mapping and identity with the Bell Quantum Spheres that now form Bell Quantum Field F. Following the collapse of Bell Quantum Hyper-Point EF, Bell Quantum Hyper-Point E and Bell Quantum Hyper-Point F form a product state rather than an entangled state ψ1,2((x1,y1,z1)1,(x2,y2,z2)2) → ψ1(x1,y1,z1)1 ⊗ ψ2(x2,y2,z2)2,t). Finally, the reduction in the number of Bell Quantum Spheres that comprise Bell Quantum Field E and Bell Quantum Field F instantaneously causes the generalized localization of both Bell Quantum Field E and Bell Quantum Field F regardless of how spread out each of the individual Bell Quantum Fields may have been before the collapse of Bell Quantum Hyper-Point EF.
If the Stern-Gerlach experiment in the z direction is conducted in Princeton on quantum state E, and the quantum state is found in the z spin-up direction, quantum state F is instantly in the z spin-down direction in Copenhagen. Conversely, if the Stern-Gerlach experiment in the z direction is conducted in Princeton on quantum state E and the quantum state is found in the z spin-down direction, quantum state F is instantly in the z spin-up direction in Copenhagen. The same analysis applies if the Stern-Gerlach experiment in the z direction is conducted on quantum state F in Copenhagen instead of quantum state E in Princeton.
3.2.2. The Double-slit Experiment
The double-slit experiment and its multiple incarnations have been reviewed extensively by physicists and philosophers. Notwithstanding the numerous controversies surrounding the experiment, the experimental results of the various double-slit experiments are subject to the same ontological structure and physical laws that govern the collapse of all quantum states under the Dual Ontology conjecture.
Individual electrons ("quantum states") are sent one at a time towards "Wall (W)" with two narrow Gaussian slits (A) and (B). In comparison with a quantum state's wavelength, slits (A) and (B) are sufficiently narrow so that a quantum state diffracts if it passes through slits (A) and (B). Some of the individual quantum states that move towards Wall (W) hit the wall, while others pass through slits (A) and (B). Once a quantum state passes through slit (A), it spreads uniformly as a spherical wave toward a detector "Detector (D)." Similarly, as the quantum state passes through slit (B), it too spreads uniformly as a spherical wave toward Detector (D). The two spherical waves interfere as they spread toward Detector (D). A single flash occurs whenever a quantum state hits Detector (D). Only one flash is detected per quantum state. After many flashes, Detector (D) indicates an interference pattern.
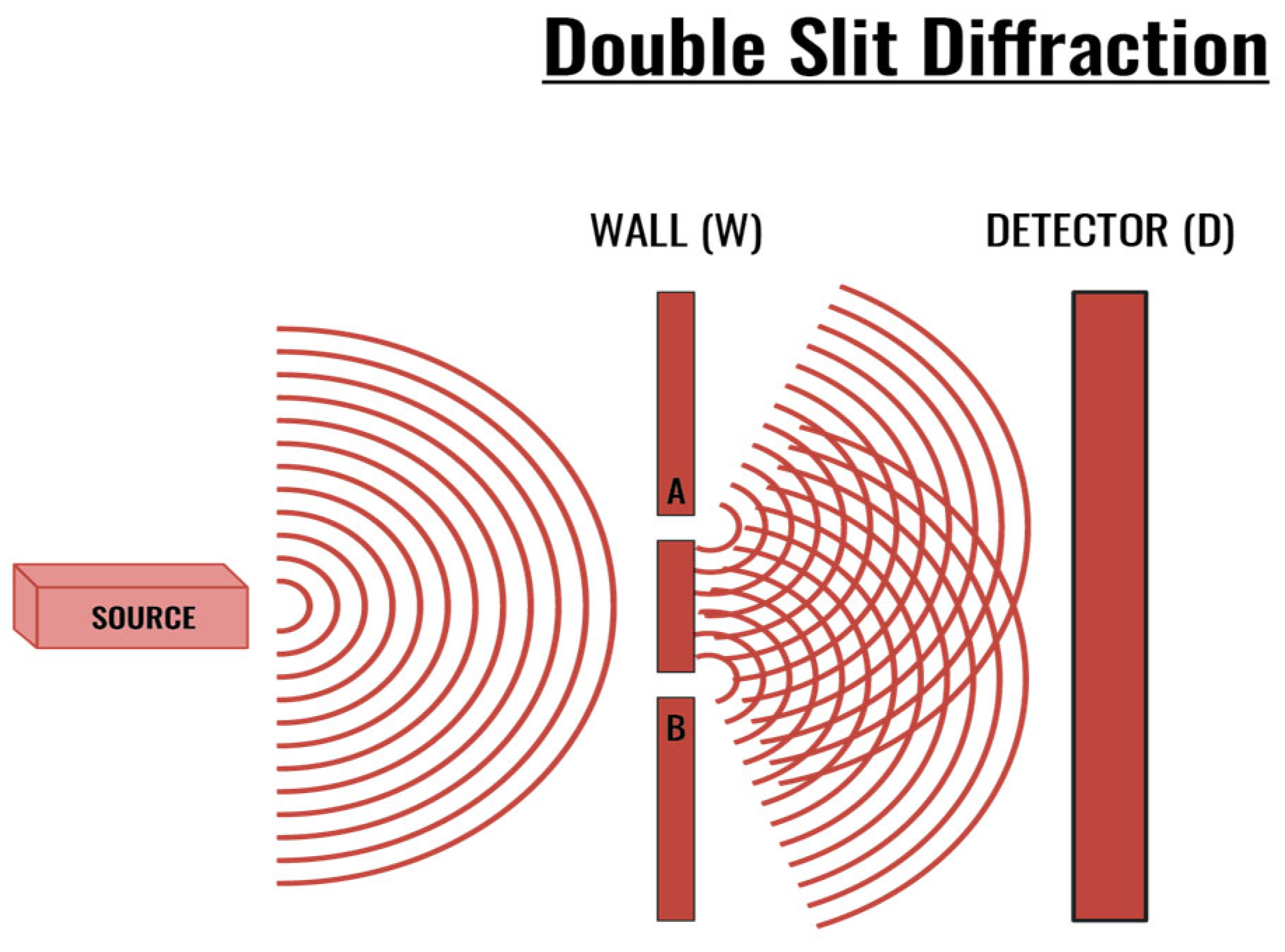
When each quantum state is generated, it forms a Bell Quantum Field in 4D spacetime and an ultra-high dimensional Bell Quantum Hyper-Point in Planck Space. Accordingly, when a quantum state is fired toward Wall W, the quantum state is simultaneously comprised of a Bell Quantum Field in 4D spacetime and a Bell Quantum Hyper-Point in Planck Space. As the quantum state travels toward Wall W, it spreads spherically in 4D spacetime but continuously forms a single Bell Quantum Hyper-Point in Planck Space. A one-to-one mapping and identity exists between the Bell Quantum Spheres that comprise the quantum state’s Bell Quantum Field in 4D spacetime and the quantum state's Bell Quantum Hyper-Point in Planck Space.
As the quantum state passes through slits (A) and (B), both slits independently diffract the quantum state, and the quantum state begins to spread from both slits towards Detector D in a spherical pattern. The Bell Quantum Spheres of the quantum state that passes through slit A forms Bell Quantum Field A in 4D spacetime, and the Bell Quantum Spheres of the quantum state that passes through slit B form Bell Quantum Field B in 4D spacetime. The quantum state forms a single Bell Quantum Hyper-Point AB in Planck Space. Mathematically, the quantum state's wavefunction is typically described as .47
Before reaching Detector D, the two wave functions combine linearly, and the linear combination causes interference. The modulus squared of the wavefunction is greatest where the linear combination is constructive and approaches zero where the interference is destructive. Whether or not the mathematical wavefunction of the quantum state forms a peak or a trough, the quantum state always continues to form a single Bell Quantum Hyper-Point AB in Planck Space.
In 4D spacetime, a flash anywhere on Detector (D) indicates that a quantum state's Bell Quantum Hyper-Point has physically collapsed. The collapse of a quantum state is an instantaneous physical process in Planck Space. Following its collapse, the quantum state forms a single Bell Quantum Hyper-Point. However, the number of dimensions that comprise the quantum state's new Bell Quantum Hyper-Point is reduced to a subset of the Bell Quantum Spheres that formerly comprised its Bell Quantum Hyper-Point. Simultaneously, the Bell Identity ensures that the collapse of the quantum state's Bell Quantum Hyper-Point is, on a one-to-one basis, linked to an instantaneous reduction in the number of Bell Quantum Spheres that now comprise the quantum state's single Bell Quantum Field in 4D spacetime.
Regardless of how spread out the quantum state's Bell Quantum Field may have been in 4D spacetime before its collapse, and regardless of the interference caused by the interaction of the diffracted waves spreading from slits (A) and (B), the collapse of a quantum state's Bell Quantum Hyper-Point ensures that the entire quantum state is generally localized in 4D spacetime. The collapse of the quantum state to a generally localized position anywhere on Detector (D) is instantaneous, and the mathematical wave function of Bell Quantum Spheres that are no longer occupied by the quantum state following its collapse has no meaning.
Following many flashes caused by the collapse of individual electrons, an interference pattern emerges on the Detector (D). The interference pattern is the combined result of the collapse of individual Bell Quantum Hyper-Points in Planck Space and the simultaneous reduction in the number of Bell Quantum Spheres that comprise each quantum state's Bell Quantum Field.
3.2.3. A Simple Which-Way Experiment
Historically, "which way" experiments have compounded the theoretical issues of double-slit experiments. Once again, however, from the perspective of the Dual Ontology conjecture, the theoretical analysis of a which-way experiment is the same as it is for all other collapse-based quantum events.
The baseline experiment repeats the double-slit example in section 3.2.2 but is modified by inserting a proton into an empty rectangular box at the center of Wall (W).48 The proton is positively charged, and each electron ("quantum state") fired towards Wall (W) is negatively charged. Assuming the experiment is 100% reliable, slit (A) flashes if the proton is attracted towards slit (A), and slit (B) flashes if the proton is attracted towards slit (B). Experimentally, 50% of the flashes are recorded at slit (A) and 50% at slit (B) 50%.

As an electron spreads in 4D spacetime, it forms a Bell Quantum Field in 4D spacetime and simultaneously forms a single Bell Quantum Hyper-Point in Planck Space. If slit (A) flashes, the electron's Bell Quantum Hyper-Point collapses, and the number of Bell Quantum Spheres that comprise the electron's Bell Quantum Hyper-Point is reduced. Simultaneously, on a one-to-one basis, the Bell Identity ensures that the number of Bell Quantum Spheres comprising the electron's Bell Quantum Field is reduced. The electron is now generally localized at slit A. The analysis is the same if slit B flashes rather than slit A.
Once the electron is generally localized at either slit (A) or slit (B), it will once again continue to spread towards Detector (D). Critically, however, since the electron passed through either slit (A) or slit (B), but not both, an interference pattern is not formed at detector D. Conversely, if neither slit (A) nor slit (B) flashes, as the electron passes through slits (A) and (B) the analysis of the double-slit experiment described in Section 3.2.2 above is once again applicable.
4. Physical Considerations
4.1. Indeterminacy and the Bell Quantum Hyper-Point
Indeterminacy, like many other quantum terms, has disparate meanings. This analysis adopts a simple definition: Indeterminacy occurs when a quantum system "has a determinable property, but no determinate value for the determinable."49 For a singlet state in the z-direction, spin is a determinable property, and spin in the z spin-up and z spin-down directions are determinant values. Assuming quantum states z1 and z2 form a singlet state in the z-direction, the mathematical representation would be ψ = (↑z1↓z2 −↓z1↑z2 ).
Regardless of whether the singlet state is space-like separated in 4D spacetime, the Bell Identity ensures that the singlet state forms a single Bell Quantum Hyper-Point in Planck Space and two Bell Quantum Fields in 4D spacetime. Although the singlet state’s spin is physically indeterminate, both in Planck Space and in 4D spacetime, the singlet state’s ultra-high dimensional Bell Quantum Hyper-Point is not space-like separated. After the collapse of the singlet state, the system transitions to a product state, and the spins of the z1 and z2 quantum states become determinate rather than indeterminate ψ = (↑z1↓z2 −↓z1↑z2 )→↑z1↓z2 in both Planck Space and 4D spacetime. The z1 and z2 quantum states now form independent Bell Quantum Hyper-Points in Planck Space and two generally localized Bell Quantum Fields in 4D spacetime.50
4.2. Quantum State Emergence and Annihilation
Quantum state emergence and annihilation have been well-documented experimentally. Nevertheless, the physical acts of quantum state emergence and annihilation have been theoretically problematic when viewed from the perspective of the non-relativistic Schrödinger equation because of the fixed nature of mathematical quantum configuration spaces.51 Since the size of the configuration space is based on the number of quantum states represented in that space, the number of quantum states is typically conserved. As a result, it is difficult to account for either the decrease or increase in the number of quantum states in a given system. Relativistic Quantum Field Theory ("QFT"), unlike non-relativistic quantum theory, targets both quantum state emergence and annihilation, and within QFT, the number of quantum states may vary.
The Dual Ontology provides a physical, ultra-high dimensional ontological solution to the quantum state annihilation and emergence issue even though the number of dimensions in Planck Space is potentially finite.52 As we have seen, the Bell Identity links a quantum state's Bell Quantum Spheres in 4D spacetime with the same Bell Quantum Spheres in Planck Space. Given that there are approximately 1090 quantum states in the observable universe, the aggregate number of Bell Quantum Spheres occupied by all quantum states in the observable universe at any one moment depends upon the spread of their respective Bell Quantum Fields. To put this number into perspective, if a single quantum's state's field is limited to the ground state of a hydrogen atom, that field would be composed of approximately 1.92E+74 Bell Quantum Spheres, an incredibly large number under ordinary circumstances, but an incredibly small number compared to the 5.58E+186 Planck Spheres in the observable universe. Ignoring the Pauli exclusionary principle, the number of Planck Spheres in the observable universe is a tiny fraction of the 25.58186-1 unique combinations formed by the 1090 quantum states in the observable universe. Since the number of unique quantum combinations of Planck Spheres in the observable universe is orders of magnitude greater than the total number of quantum states, there is no apparent physical or mathematical reason that the number of Planck Spheres occupied by quantum states must remain constant.
During the process of quantum annihilation, a quantum state's Bell Quantum Hyper-Point instantaneously collapses. The quantum state's Bell Quantum Hyper-Point and Bell Quantum Field no longer exist, and the mathematical wave function describing the quantum state no longer has any meaning. Conversely, when a quantum state is generated, the quantum state forms a Bell Quantum Field in 4D spacetime and a Bell Quantum Hyper-Point in Planck Space.
4.3. Physical Triggers
This analysis does not identify the physical cause or causes in 4D spacetime that precipitate quantum state collapse under the Dual Ontology conjecture. Nevertheless, the analysis provides a physical framework to investigate the role of physical triggers in quantum collapse.53 The starting point is the dynamic, physical evolution of all quantum states in a 4D spacetime governed by the three known classical forces: electromagnetism, the strong nuclear force, the weak nuclear force as well as the warping of spacetime by gravity (the "Classical Forces")54 Consequently, not only do the Classical Forces underlie all quantum state motion in 4D spacetime, but they are also the cause of all known physical interactions ("Physical Interactions") between quantum states including all physical triggers that precede the collapse of a quantum system.55
There are no fine-tuning parameters in the Dual Ontology conjecture. All triggers in 4D spacetime are independent time and location events.56 Physical triggers occur more frequently or less frequently, depending on time and location. Heat and cold will, for example, affect the rate of Physical Interactions.57 Human beings can not only trigger Physical Interactions or be the proximate cause of a physical trigger, but they can also determine the time and location of such triggers.58 Individual Physical Interactions are not based upon human consciousness or observation, do not give any special significance to human beings or human action, are not based upon a single universal cause, a single white or non-white noise source,59 are not spontaneous,60 universally continuous, or discontinuous, and are not predicated upon rules governing the frequency or probability of occurrence.
4.4. The Generalized Localization of a Quantum State
Although the Bell Identity links the collapse of a quantum state to the simultaneous reduction in the number of Bell Quantum Spheres that comprise a quantum state's Bell Quantum Hyper-Point in Planck Space and its Bell Quantum Field in 4D spacetime, there is nothing in the Dual Ontology conjecture itself that provides a physical basis to determine the precise localization of a quantum state in 4D spacetime. Nevertheless, the Dual Ontology provides a possible structural framework for further analysis that differs considerably from current theoretical approaches to quantum localization.61 62
As we have seen, the Bell Identity ensures a one-to-one mapping and identity between Bell Quantum Spheres in 4D spacetime and Planck Space. The collapse of a Bell Quantum Hyper-Point must be to a subset of the Bell Quantum Spheres that had previously represented the quantum state's original Bell Quantum Hyper-Point in Planck Space and its Bell Quantum Field in 4D spacetime. In this sense, the Bell Identity is always restrictive. It places a strict boundary on the collapse outcome. A quantum state's Bell Quantum Hyper-Point cannot collapse anywhere in the universe; instead, it is a linked collapse restricted to the Bell Quantum Spheres that comprise the quantum state before its collapse.
Although the Bell Identity places strict boundaries on the generalized location of a quantum state immediately following its collapse in Planck Space, it does not set a specific limit on the size or location of a quantum state's Bell Quantum Field in 4D spacetime following a collapse. The generalized location of a quantum state may be related to the physical trigger that initiated collapse in the first instance. The size of a quantum state's Bell Quantum Field may also vary based on the physical composition of the quantum state in question. Photons, neutrinos, electrons, muons, and other sub-atomic quantum states may localize to Bell Quantum Fields of varying sizes. In addition, high or low-energy collapses may have different localization characteristics, and a quantum state's momentum in 4D spacetime may also affect its localization.
4.5. The Dual Ontology, Time, and Instantaneous Collapse
The instantaneous nature of quantum collapse is related to the Dual Ontology's integrated structure and dynamics and the concept of time. Neither Planck Space nor 4D spacetime alone supports the concept of instantaneous collapse. Planck Space does not have a dimension of time, and except for the collapse process, Planck Space does not have an independent concept of dynamic movement. 4D spacetime, on the other hand, has dynamic movement and a time dimension, but Special Relativity constrains the time dimension. Since the instantaneous collapse process in 4D spacetime is a single point in time, the event occurs in the three spatial dimensions of 4D spacetime. However, there is no movement in 4D spacetime's time dimension.
The explanation for the "instantaneous" nature of quantum collapse lies in the Dual Ontology's integrated structure and mixed dynamics. Since the Bell Identity posits a one-to-one mapping and identity between each Bell Quantum Sphere in 4D spacetime and Planck Space, dynamic motion in 4D spacetime is always reflected in the Bell Quantum Spheres that comprise a Bell Quantum Hyper-Point in Planck Space. When a Physical Interaction in a 4D spacetime subject to the laws of Special Relativity triggers the collapse of a quantum state, the trigger directly affects the quantum state's Bell Quantum Hyper-Point in Planck Space, where Special Relativity is inapplicable. Since neither time, space, nor volume separates the Bell Quantum Spheres that comprise the Bell Quantum Hyper-Point, the collapse of the Bell Quantum Hyper-Point is instantaneous. Once again, the Bell Identity ensures that the collapse of the Bell Quantum Spheres in Planck Space is instantly reflected in the reduction in the number of Bell Quantum Spheres that comprise the quantum state’s Bell Energy Field in 4D spacetime.
5. Special Relativity and Quantum Mechanics
The theoretical conflict between Special Relativity and quantum mechanics has had a long and unresolved history. John Bell, for example, recognized that the conflict was at the forefront of theoretical physics:
"…I think, any sharp formulation of quantum mechanics, has a very surprising feature: the consequences of events at one place propagate to other places faster than light…For me then this is the real problem with quantum theory: the apparently essential conflict between any sharp formulation and fundamental relativity. That is to say, we have an apparent incompatibility, at the deepest level, between the two fundamental pillars of contemporary theory."63
The apparent incompatibility is typically described by a set of terms and concepts derived from 4D spacetime rather than Planck Space or the Dual Ontology. Despite their usefulness in the context of a closed 4D spacetime, the use of common terms such as space-like separated, non-separability, entangled, instantaneous, superluminal, faster than light, local and non-local, discrimination, and unattenuation, as well as more complex concepts such as the Relativity of Simultaneity and Relativistic Energy Increase have had the unintended effect of magnifying a theoretical and experimental conflict that does not exist.64
5.1. Space-Like Separated
The term "space-like separated" is based upon a 4D spacetime structure composed of three dimensions of space and one dimension of time. The term is directly related to the concepts of space and time, the theory of Special Relativity, and the spatial distance between two or more events outside of one another's light cones. Nevertheless, the term has no meaning in conjunction with an ultra-high dimensional Bell Quantum Hyper-Point in Planck Space. Planck Space does not have a time dimension, space, or volume, and the concepts of Special and General Relativity have no meaning.
5.2. Non-separability
Einstein may have been the first physicist who raised concerns regarding the theoretical implications of separability. His primary concern was based upon the conjunction of two assumptions related to his incompleteness argument: that spatially separate systems are real states and that the physical effects of space-like separated quantum systems could not communicate faster than the speed of light.65
In 4D spacetime, a singlet state in the z-direction ψ = (↑z1↓z2 −↓z1↑z2 ) is non-separable, even though it is often regarded as an abstract mathematical concept. Key attributes of a non-separable singlet state are 1) the spatial separation of the z1 and z2 states, 2) the temporal separation of z1 and z2 states, and 3) the existence of a single system.
Without time, space, or volume, an ultra-high dimensional Bell Quantum Hyper-Point is not spatially or temporally separated in Planck Space.66 Instead, the Bell Quantum Hyper-Point is a single, non-separable entity linked by the Bell Identity to the Bell Quantum Spheres that comprise the quantum state's Bell Quantum Field(s) in 4D spacetime. Although the issue of non-separability raises significant theoretical issues in 4D spacetime, the non-separability of a Bell Quantum Hyper-Point in Planck Space does not challenge Special Relativity. Instead, it reinforces the integrated status of the Dual Ontology's structure and the importance of the Bell Identity as an explanatory tool that simultaneously links a quantum state's Bell Quantum Field in 4D spacetime and its Bell Quantum Hyper-Point in Planck Space.67
5.3. Instantaneous, Superluminal, and Faster than Light
The temporal terms instantaneous, superluminal, and faster than light are often used to describe the collapse of a quantum state in 4D spacetime and the apparent violation of the laws of Special Relativity. The terms are closely associated with space and time and are directly related to the maximum speed of light in a vacuum. Following the collapse of a quantum state, the terms instantaneous, superluminal, and faster are typically used to describe the quantum state's speed of 1) communication, 2) signaling or the absence of signaling, 3) information transmission, and 4) matter and energy transfer.
In contrast, the instantaneous collapse of a Bell Quantum Hyper-Point is an event that has physical ramifications in both Planck Space and 4D spacetime. The Dual Ontology's ontological structure and the Bell Identity ensure that the reduction in the number of Bell Quantum Spheres that comprise a quantum state's Bell Quantum Hyper-Point in Planck Space is simultaneously reflected in the reduction in the number of Bell Quantum Spheres that comprise the quantum state's new Bell Quantum Field in 4D spacetime. Although that reduction is, in fact, instantaneous, it does not violate Special Relativity.
5.4. The Quantum Connection
In 4D spacetime, quantum discrimination describes a quantum state's ability to maintain an exclusive "connection" to the exclusion of all other quantum states in 4D spacetime, and unattenuated denotes the strength (or non-attenuation) of a quantum state's connection in 4D spacetime.68 The terms are typically used to denote the connection between space-like separated entangled states in 4D spacetime. In this context, discrimination and non-attenuation imply an instantaneous and continuous connection that violates the maximum speed of light.
In the context of the Dual Ontology, quantum discrimination and non-attenuation are directly related to the Bell Identity and the one-to-one mapping and identity between each of the Bell Quantum Spheres that comprise a quantum state's Bell Quantum Field(s) in 4D spacetime and the Bell Quantum Spheres that comprise its Bell Quantum Hyper-Point in Planck Space. There is nothing in either the structure of the Dual Ontology or in the dynamics of a quantum state's spreading or collapse that suggests that the ability to discriminate or the lack of attenuation implies a violation of Einstein's theory of Special Relativity. On the contrary, the Dual Ontology conjecture explains why a quantum state's ability to discriminate and its non-attenuation are physical phenomena and why quantum discrimination and attenuation do not violate Special Relativity.69
5.5. Locality, Bell's Theorem, and Bell Quantum Hyper-Points
No development in quantum theory in the latter half of the 20th century has been more important than John Bell's inequality theorem.70 The theorem posits that relativistic local causation theories cannot physically account for the statistical predictions of quantum theory (for spin experiments of entangled quantum states in the singlet state).71 More generally, Bell's theorem posits that theories that conform with the statistical results of quantum experiments cannot be local. Although there have been numerous attempts to describe the precise meaning and import of "locality" and "non-locality," those discussions are well beyond the scope of this analysis.72 For this analysis, the terms local and non-local are causal concepts connected with the 4D spacetime concepts of time and space and the maximum speed of light.73
Despite the importance of the local vs. non-local debate in 4D spacetime, the concepts of time, space, and volume do not apply to Planck Space. In Planck Space, a quantum state's Bell Quantum Hyper-Point is neither space-like separated nor separable.74 Accordingly, the Dual Ontology alters the 4D spacetime local causality vs. non-local causality discussion, but it does not alter the import of Bell's inequality theorem. Recall that Bell's inequality theory posits that relativistic local causation theories cannot physically account for the statistical predictions of quantum theory (for spin experiments in the singlet state), and more generally, theories that conform with the statistical results of quantum experiments cannot be local. Neither the existence nor the collapse of a quantum state's Bell Quantum Hyper-Point alters the correctness of either of these statements.75
Nevertheless, the Dual Ontology conjecture alters the assumption that quantum state collapse is evidence of non-local causality in 4D spacetime. Quantum state collapse is evidence of a physical event external to 4D spacetime.76 Accordingly, the Dual Ontology conjecture strengthens rather than weakens the core of the Bell Inequality theorem.77 Instead of a theoretically troublesome non-local event in 4D spacetime, the collapse of a Bell Quantum Hyper-Point in Planck Space and the generalized localization of a quantum state in 4D spacetime by-passes the non-locality issue. Perhaps even more satisfying to John Bell, the Dual Ontology conjecture eliminates "…the real problem with quantum theory: the apparently essential conflict between any sharp formulation and fundamental relativity."78
5.6. The Relativity of Simultaneity
The historical tension between Special Relativity and quantum mechanics extends to Einstein's Relativity of Simultaneity theory. The theory of Special Relativity holds that 1) there are no privileged frames of reference – all inertial reference frames (frames that move relative to one another at a constant speed) are equally valid, and 2) for all observers in all inertial frames, the speed of light in a vacuum is invariant. Consequently, Einstein's Relativity of Simultaneity theory posits that 1) whether two events that are spatially separated occur at the same time depends on an inertial observer's frame of reference, and 2) two observers with different inertial reference frames will conclude that the same event occurred at two separate times.
In the case of space-like separated entangled electrons in the singlet state in the z-direction (↑z1↓z2 −↓z1↑z2), an experiment that causes the instantaneous collapse of the z1 electron simultaneously causes the instantaneous collapse of the z2 electron. Since the Relativity of Simultaneity theory posits that whether a cause (the collapse of the z1 electron) precedes an effect (the collapse of the z2 electron) is dependent upon an inertial observer's frame of reference, the simultaneous nature of the experiment appears to violate Einstein's Relativity of Simultaneity theory. It implies both a violation of Lorentz Invariance and a preferred frame of reference.79
Based on the Dual Ontology conjecture, the instantaneous collapse of a quantum state is a physical event but does not violate the 4D spacetime concept of Relativity of Simultaneity. In the case of an entangled pair of electrons in the singlet state in the z-direction, it does not matter whether a quantum experiment in 4D spacetime is first conducted on the z1 electron or the z2 electron, nor does it matter whether the z1 and z2 electrons are space-like separated in 4D spacetime. The Bell Identity automatically ensures that an experiment on either the z1 or z2 electron in 4D spacetime is conducted simultaneously on the entangled quantum state's single Bell Quantum Hyper-Point. Moreover, the Bell Identity simultaneously links the instantaneous collapse of the Bell Quantum Spheres that comprise the quantum state's single Bell Quantum Hyper-Point in Planck Space with a reduction in the number of Bell Quantum Spheres that comprise the now generally localized Bell Quantum Fields of both the z1 and z2 electrons in 4D spacetime. The formerly entangled electrons now form a product state; the collapse was instantaneous and simultaneous, but the 4D spacetime laws of Special Relativity have not been violated.
5.7. Relativistic Energy Increase
The instantaneous nature of quantum state collapse also appears to violate the related concept of Relativistic Energy Increase. Einstein's theory posits that the relativistic energy of a body in motion relative to an observer or another body increases as its velocity accelerates. As the velocity of an object accelerates as it approaches the speed of light, its relativistic kinetic energy theoretically approaches infinity, but its speed is limited by Special Relativity.80 In quantum mechanics, momentum is a more commonly used term than velocity. Accordingly, as the momentum of a quantum state increases, its associated energy increases. For a quantum state to reach or exceed the speed of light, as with instantaneous collapse, the energy required would be infinite.81
Although the collapse of a quantum state's Bell Quantum Hyper-Point in Planck Space is instantaneous, the collapse is a physical event extraneous to 4D spacetime. The Dual Ontology and the Bell Identity ensure that the instantaneous collapse of a Bell Quantum Hyper-Point decreases the number of Bell Quantum Spheres that comprise a quantum state's Bell Quantum Hyper-Point and its Bell Quantum Field. As a result, the reduction in the number of Bell Quantum Spheres that comprise a Bell Quantum Field is instantaneous. However, there is no Relativistic Energy Increase of the quantum state in 4D spacetime or Planck Space.
6. Quantum Path Irreversibility and The Arrow of Time
The ontological and dynamic implications of the Dual Ontology include the subjects of quantum path irreversibility, the arrow of time, the second law of thermodynamics, and entropy, among others.82 This section focuses on a narrow subset of these subjects: the physical basis that precludes path reversibility following the non-linear collapse of a quantum state in the first instance and its physical implications for the arrow of time.
The Dual Ontology conjecture posits that "quantum path irreversibility" is an absolute impossibility rather than extremely unlikely or nearly impossible. Path irreversibility means it is physically impossible for any quantum state to instantaneously reverse its path in space or time following its collapse.83 84 85 Two primary factors within the Dual Ontology conjecture preclude path reversibility: the dynamic motion of all quantum states in 4D spacetime subject to the physical laws of 4D spacetime and the instantaneous collapse of all quantum states in Planck Space, where the laws of Special and General Relativity are not applicable.
A re-examination of the Bohm version of the EPR experiment demonstrates why the collapse of all quantum states is physically irreversible. Assume that two quantum states, z1 and z2, are entangled in the singlet state in the z-direction ψ = (↑z1↓z2 −↓z1↑z2 ). The Bell Quantum Field of the z1 quantum state is spread out in orbit around Mars (Bell Quantum Field z1), and the Bell Quantum Field of the z2 quantum state is spread out in orbit around Earth (Bell Quantum Field z2). The z1 and z2 quantum states are separated by approximately 225 million kilometers. In Planck Space, the singlet state forms Bell Quantum Hyper-Point z1z2. The singlet state is non-separable, and its spin is indeterminate.
Following the instantaneous collapse of Bell Quantum Hyper-Point z1z2 in Planck Space, the z1 and the z2 quantum states form a product state ψ1,2((x1,y1,z1)1,(x2,y2,z2)2) → ψ1(x1,y1,z1)1 ⊗ ψ2(x2,y2,z2)2 rather than an entangled singlet state. The Bell Identity ensures that the reduction in the number of Bell Quantum Spheres that comprised Bell Quantum Hyper-Point z1z2 before its collapse is simultaneously linked to the reduction in the number of Bell Quantum Spheres that comprise Bell Quantum Fields z1 and z2 in 4D spacetime. Bell Quantum Field z1 is generally localized in orbit around Mars, and Bell Quantum Field z2 is generally localized in orbit around Earth. The z1 and the z2 quantum states are separable rather than non-separable and form Bell Quantum Hyper-Point z1 and Bell Quantum Hyper-Point z2 in Planck Space, respectively. If the z1 quantum state is in the spin-up direction, the z2 quantum state is in the spin-down direction, and if the z1 quantum state is in the spin-down direction, the z2 quantum state is in the spin-up direction. In either case, the spin of the respective quantum state's electrons is now determinate rather than indeterminate.
We can now analyze why path irreversibility is an impossibility based upon the difference between the physical mechanism by which quantum states spread in 4D spacetime and instantaneously collapse in Planck Space. As we have seen, the spreading of a singlet state is subject to all of the physical laws of 4D spacetime, including the laws of Special Relativity. In contrast, Planck Space has no time, space, or volume, and Bell Quantum Hyper-Point z1z2's instantaneous collapse is not subject to Special Relativity’s laws.
Before the collapse of the singlet state, 1) the z1 and z2 quantum states were separated by 225 million kilometers in 4D spacetime, 2) the z1 quantum state was spread out in orbit around Mars, and the z2 quantum state was spread out in orbit around Earth, 3) the singlet state formed Bell Quantum Hyper-Point z1z2 in Planck Space, 4) the singlet state was non-separable, and 5) its spin was indeterminate. Following the instantaneous collapse of Bell Quantum Hyper-Point z1z2, 1) the z1 and z2 quantum states are still separated by approximately 225,000,000 kilometers, 2) Bell Quantum Field z1 is generally localized in orbit around Mars, and Bell Quantum Field z2 is generally localized in orbit around Earth, 3) the singlet state has separated into a product state composed of Bell Quantum Hyper-Point z1 and Bell Quantum Hyper-Point z2, in Planck Space and Bell Quantum Field z1 and Bell Quantum Field z2, respectively, 4) the formerly entangled singlet state is now separable, and 5) the spins of quantum states z1 and z2 are determinate even if they are unknown.
Given the physical structure of the Dual Ontology and the dynamic laws that govern the spreading of the z1 and z2 quantum states in 4D spacetime and the collapse of the singlet state in Planck Space, path reversibility is physically impossible. Path reversibility requires the instantaneous path reversal along the identical collapse path taken by the entangled Bell Quantum Hyper-Point in Planck Space in the first instance. Under the Dual Ontology conjecture, however, no such physical structure exists. The z1 and z2 quantum states can only spread in 4D spacetime and only do so subject to the laws of Special Relativity.
More specifically, following the collapse of Bell Quantum Hyper-Point z1z2 in Planck Space, the physical connection in Planck Space between Bell Quantum Field z1 on Mars and Bell Quantum Sphere z2 on Earth no longer exists. Since Bell Quantum Field z1 and Bell Quantum Field z2 are now generally located in orbit around Mars and Earth, each quantum state must travel at least 112,500,000 miles before they can become entangled again.86Even if there is an infinitely small chance that the z1 and z2 quantum states will in the distant future spread and once again form Bell Quantum Hyper-Point z1z2, at the moment the singlet state collapses, path reversibility becomes an impossibility.
The Dual Ontology's ontological structure and the asymmetric rules that govern the dynamic motion of quantum states in 4D spacetime and the collapse of quantum states in Planck are the physical basis for 4D spacetime's arrow of time. Without an instantaneous reversible path, the arrow of time for all quantum states, including quantum states z1 and z2 and those of the proverbial egg, can only move in a single temporal and spatial direction.
7. Quantum Cosmology, Cosmogony, and the Dual Ontology
The Dual Ontology's analysis of the structural and dynamic relationship of 4D spacetime and Planck Space is the basis for a fundamental re-evaluation of the universe's quantum cosmology and cosmogony. The Dual Ontology conjecture not only explains why the transition from a widely dispersed 4D spacetime at or near heat death to a generally localized, non-singular volume at t = 0 is caused by the instantaneous physical collapse of the Planck Energy Hyper-Point but also why the collapse is the physical mechanism that explains 1) 4D spacetime's isotropy, homogeneity, extremely high energy, pressure, and temperature, flatness, and low gravitational entropy at t = 0, and 2) the horizon and fine-tuning problems.87
7.1. 4D spacetime at t = 0 and Heat Death
The ΛCDM model, the CMB, and related data88 indirectly indicate that approximately 13.8 billion years ago, 4D spacetime was a hot, dense state characterized by extreme energy densities, pressures, and temperatures.89 Since the Dual Ontology conjecture posits a 4D spacetime composed of Planck Spheres and the SOAN, the Dual Ontology conjecture replaces the dimensionless initial singularity of General Relativity with a discrete spacetime at t = 0. Consequently, at t = 0, 4D spacetime is characterized by a discrete, generally localized 4D spacetime with extreme, rather than infinite, energy densities, pressures, and temperatures.90
Approximately 380,000 years after t = 0, the temperature anisotropies of the CMB across the sky varied by approximately 1 part in 105. The temperature variations indirectly suggest that 4D spacetime's energy density and pressure at t = 0 are 1) nearly isotropic and homogeneous and 2) very small anisotropies and inhomogeneities also exist. When the angular power spectrum around the first peak of the anisotropies is extrapolated backward to t = 0, the CMB and related data indirectly support a 4D curvature that is nearly flat (k = 0).91 A nearly flat 4D curvature strongly suggests that 4D spacetime’s total energy density at t = 0 nearly equaled the critical density ρ4D = ρcrit. Since the total energy content of 4D spacetime and the 4D Energy Field (4DEF) are equal, the critical density of the 4D Energy Field also nearly equals the critical density ρ4DEF = ρcrit. Although 4D spacetime's energy density and pressure entropy are nearly maximal, the presence of anisotropies and inhomogeneities, the capacity of 4D spacetime to do work, and the future expansion of 4D spacetime not only support a very low gravitational entropy, but they also support the capacity to do work.
At or near heat death, 4D spacetime is in a state of near or maximum entropy. 4D spacetime's spatial geometry, energy density, pressure, and temperature are very nearly homogeneous and isotropic, and, as a result, 4D spacetime is very close to thermodynamic equilibrium.92 At or near heat death, 4D spacetime's energy density, pressure, and temperature asymptotically approach zero. In addition, 4D spacetime has no large-scale structures, is extremely widely dispersed, and its spatial curvature is flat or nearly flat (k =0). Once again, the total energy density of 4D spacetime's 4D Energy Field nearly equals the critical density ρ4DEF = ρcrit. Since little or no heat can flow near a thermal equilibrium approaching zero, little or no work or gravitational clumping can occur. At the macrostate level in 4D spacetime, no additional physical changes can occur without work.93 Although 4D spacetime's spatial geometry, energy density, and pressure are homogeneous and isotropic, 4D spacetime is in a state of near-maximal gravitational entropy.
Significant similarities and dissimilarities exist between 4D spacetime at t = 0 and heat death. Beginning with the similarities, at t = 0 and heat death, 4D spacetime's energy density, pressure, and temperature are nearly homogeneous and isotropic, and 4D spacetime's energy density and pressure are near maximum entropy. 4D spacetime's spatial curvature is nearly flat, and, as a result, 4D spacetime's total energy density equals the critical density ρ4D = ρcrit. There are no large-scale structures at t = 0 or heat death.
Significant dissimilarities exist between 4D spacetime at t = 0 and heat death. In contrast to 4D spacetime at t = 0, at heat death, 4D spacetime's spatial structure is widely dispersed rather than generally localized; its energy density, pressure, and temperature asymptotically approach zero, and no further work can occur since 4D spacetime is near thermal equilibrium at the macroscopic level. Without future gravitational clumping, no other macrostates are physically possible. Accordingly, at heat death, 4D spacetime is in a state of near-maximal gravitational entropy rather than near-zero entropy. The extreme energy density, pressure, and temperature of 4D spacetime at t = 0 prevent the formation of quantum states, but quantum states continue to exist at heat death.
7.2. Planck Space at t = 0 and Heat Death
The ontological structure of a (3 x N) Planck Space is identical at t = 0 and heat death. Although the number of Planck Spheres that comprise Planck Space remains constant and may be infinite, Planck Space does not have a time dimension or the physical characteristics of space or volume. Nevertheless, the Planck Identity ensures that dynamic changes to the energy content of the Planck Spheres comprising the 4D Energy Field are simultaneously reflected in the same Planck Spheres of the Planck Energy Hyper-Point. Accordingly, at both temporal periods, the Planck Identity ensures that the energy density, pressure, and temperature of the individual Planck Spheres that comprise the 4D Energy Field and the Planck Energy Hyper-Point are identical. As a result, at t = 0, the Planck Spheres comprising the Planck Energy Hyper-Point have extremely high energy densities, pressures, and temperatures. At heat death, the same Planck Spheres have energy densities, pressures, and temperatures that asymptotically approach zero.94 Since the Planck Spheres comprising the Planck 4D Energy Field were homogeneous at both periods, so were the Planck Spheres comprising the Planck Energy Hyper-Point.
7.3. Planck Energy Hyper-Point Collapse and t = 0
Although the physical event that triggers the collapse of the Planck Energy Hyper-Point is unknown, the trigger is a Physical Interaction in 4D spacetime.95 The physical trigger causes an instantaneous reduction in the number of Planck Spheres that comprise the Planck Energy Hyper-Point. Simultaneously, and without violating the physical laws that govern 4D spacetime, including the laws of Special and General Relativity and the second law of thermodynamics, the Planck Identity ensures that the reduction in the number of Planck Spheres that comprise Planck Space's Planck Energy Hyper-Point corresponds to a one-to-one reduction of the number of Planck Spheres that comprise 4D spacetime's 4D Energy Field at t = 0.96
The enormity of the instantaneous collapse of the number of Planck Spheres that comprise the Planck Energy Hyper-Point at t = 0 and the simultaneous reduction of the number of Planck Spheres in 4D spacetime that comprise the 4D Energy Field cannot be overstated. Regardless of the physical size or space-like dispersion of the 4D Energy Field at heat death, the instantaneous reduction in the number of Planck Spheres that comprise the 4D spacetime's new 4D Energy Field following the collapse process results in the discrete, generalized localization of the field at t = 0.
Although the precise size of the 4D Energy Field at t =0 remains unknown, the instantaneous transition from heat death to t = 0 radically alters 4D spacetime's energy density, pressure, and temperature. At the moment of heat death, 4D spacetime's energy density, pressure, and temperature asymptotically approach zero. At t = 0, however, the general localization of the 4D Energy Field causes an instantaneous and exponential increase in energy density, pressure, and temperature. Notwithstanding the extreme conditions of 4D spacetime at t = 0, the ΛCDM model, the CMB, and other experimental data indirectly confirm that the energy density, pressure, and temperature of the new 4D Energy Field at t = 0 continue to reflect the homogeneous and isotropic conditions of 4D spacetime at heat death.97
The collapse of the Planck Energy Hyper-Point at or near heat death also explains 4D spacetime's instantaneous transition from a state of near maximal gravitational entropy at heat death to near-zero gravitational entropy at t = 0. At heat death, 4D spacetime's energy density, pressure, and temperature approach zero. As a result, despite the presence of anisotropies and inhomogeneities and the continuing expansion of 4D spacetime, no additional work takes place at the macroscopic level, and in the absence of future gravitational clumping, 4D spacetime's gravitational entropy is near maximal. In contrast, following the collapse of the Planck Energy Hyper-Point and the general localization of the 4D Energy Field at t = 0, 4D spacetime's anisotropies and inhomogeneities, 2) the future expansion of 4D spacetime98, and 3) the extreme energy density, pressure, and temperature all contribute to 4D spacetime's near-zero gravitational entropy. 99 Instantaneity has a secondary effect: the localization process does not cause temporal deviations that introduce additional anisotropies and inhomogeneities at t = 0.
7.4. The Horizon Problem and Causality
The inability to explain the exceptionally high homogeneity and isotropy of 4D spacetime at or near t = 0 is the source of the horizon problem. Given an initial singularity premised on an infinite density, pressure, curvature, and temperature and the constraints of the speed of light c, space-like separated regions of 4D spacetime following t = 0 could not have been in causal contact. The problem is exacerbated by experimental data, including data from the CMB that indicates 4D spacetime temperature variations were approximately 1 part in 100,000 380,000 years after t = 0. More specifically, the amplitude of the fluctuations of the angular power spectrum of the CMB indirectly supports the conclusion that 4D spacetime was nearly homogeneous and isotropic at or near t =0. More technically, data from the Planck satellite and other sources indicates that the curvature parameter Ωk is -0.0005 ± 0.0005 at the last scattering of photons, corresponding to a 4D spacetime within 0.1% of being flat.
The Dual Ontology's resolution of the causality problem is premised upon three critical factors. First, the Dual Ontology replaces the concept of an initial singularity at t = 0 with a discrete 4D spacetime with quanta of volume approximately the size of a Planck Sphere. Second, the near maximal homogeneity and isotropy of the 4D Energy Field at heat death is an internal physical process caused by the expansion and cooling of 4D spacetime over extremely long-time scales. Expansion and cooling are physical processes without independent or external parameters. Moreover, given sufficient time, the expansion and cooling of 4D spacetime cause homogeneity and isotropy whether or not 4D spacetime's energy and matter were ever in causal contact. Stated somewhat differently, the near maximal homogeneity and isotropy of 4D spacetime at heat death result from the physical properties and laws that govern 4D spacetime and apply to disparate regions of space that need not have been in causal contact.
Third, the instantaneous collapse of the Planck Energy Hyper-Point causes the generalized localization of the 4D Energy Field at t = 0. The collapse does not alter the homogeneity or isotropy of 4D spacetime's energy density, pressure, or temperature, nor does it alter the existence of anisotropies and inhomogeneities at or near the heat death of 4D spacetime. However, it dramatically reduces the number of Planck Spheres comprising the new 4D Energy Field and the new Planck Energy Hyper-Point at t = 0. The reduction in Planck Spheres comprising the new 4D Energy Field is the basis for the generalized localization of the 4D Energy Field and the exponential increase in 4D spacetime's energy density, pressure, and temperature. Similarly, the collapse of the Planck Energy Hyper-Point is an internal process without independent or external parameters.100
7.5. The Flatness Problem and Fine-Tuning
The flatness of 4D spacetime's spatial curvature and its sensitivity to minor deviations from flatness (from k = 0 to either k =1 or k = -1) is known as the flatness problem. Since the value of k is calculated by Ω, and Ω is equal to the ratio of the 4D Energy Field ρ4DEF to the critical density ρcrit, the total energy density of the 4D Energy Field must equal the critical density for k to equal 1.
The Dual Ontology does not provide a theoretical basis or physical data that explains why the total energy density of the 4D Energy Field has the particular values that it does. Nevertheless, as we have already seen, independent and external parameters are not required to maintain a flat 4D spacetime from t = 0 to heat death or from heat death to t =0. In other words, from t = 0 to heat death and to t =0 ρ4DEF = ρcrit = ρ4DEF. Flatness, then, is an internal condition of the Dual Ontology, not a fine-tuning of independent and external parameters.101 102
8. Conclusion
The Dual Ontology conjecture supports a single, closed hyperspace ontology and mixed dynamics that differs fundamentally from current theories. Ontologically, the universe is comprised of two fundamental units, the SOAN, and discrete, quantized Planck Spheres. The SOAN and Planck Spheres form a single, tightly integrated ((3 x N) +3) Dual Ontology composed of 4D spacetime and Planck Space. Dynamically, the Dual Ontology is governed by a mixed set of integrated physical laws. The physical laws of 4D spacetime govern the dynamic movement of all quantum states in 4D spacetime, but many of those laws, including the laws of Special and General Relativity, are inapplicable to the collapse of quantum states in Planck Space.
The Dual Ontology's integrated structure and mixed dynamics provide a theoretical model that simultaneously unifies aspects of relativistic quantum mechanics, quantum path irreversibility and the arrow of time, and cosmology and cosmogony. It reconciles key aspects of the tension between Special Relativity and quantum mechanics and provides a physical explanation for quantum path irreversibility and the arrow of time. The analysis also explains why 4D spacetime's instantaneous transition at or near heat death to a non-singular, generally localized volume at t = 0 is not only the source of 4D spacetime's isotropy and homogeneity and extremely high energy, pressure, and temperature but also resolves the horizon and fine-tuning problems.
The largely unsung lynchpin of the Dual Ontology conjecture is the State of Absolute Nothingness. The SOAN's lack of positive physical attributes is the basis for many key physical concepts, including the Dual Ontology, Planck Space, the Planck Identity, the Bell Identity, the Bell Quantum Hyper-Point, the 4D Energy Field, the Planck Energy Hyper-Point. These concepts, in turn, ensure that the laws governing Planck Space are integrated with and complement 4D spacetime's physical laws.
To a greater or lesser extent, the Dual Ontology conjecture raises questions regarding the SOAN, Planck Spheres, time, space, energy, 4D spacetime, Planck Space, cosmogony, cosmology (including the formation of the large-scale structure of 4D spacetime), black holes, the second law of thermodynamics, statistical mechanics, the laws of General Relativity, quantum gravity, the cyclic nature of the universe, determinism, the physical nature of a quantum state, quantum tunneling, and the mathematical formalism that properly describes a quantum state's evolution in 4D spacetime and its collapse in Planck Space, among others.
These questions require considerable theoretical thought and experimental inquiry. Perhaps the most direct path for theoretical investigation and experimental testing lies with the Cosmogony and Cosmology of 4D spacetime. Future indirect experimental data regarding t = 0 and the period that immediately follows may provide support for the instantaneous collapse of 4D spacetime at or near its heat death. Similarly, future work may demonstrate that quantum state collapse, as described in the Dual Ontology conjecture, is a primary cause of the large-scale structure of 4D spacetime.
Acknowledgments
The Dual Ontology conjecture benefited immeasurably from lengthy and vigorous debates as well as penetrating criticism from Dr. Stanley Kahan and Mr. Joseph Atsmon. Over many years, Dr. Kahan’s intuitive insights and Mr. Atsmon’s analytical probing not only helped shape the analysis but also explored all of its fundamental topics, from the nature of the SOAN and Planck Space and their relationship to 4D spacetime and quantum mechanics to the collapse of 4D spacetime. I cannot thank them enough. I would also like to express a very special thanks to Ms. Naomi Kahan for her patience and technical expertise in preparing the manuscript.
References
- Adams, F. C. (2019). The degree of fine-tuning in our universe — and others. Physics Reports, 807, 1–111. [CrossRef]
- Aghanim, N., et al. (2019). Planck 2018 results. VI. Cosmological parameters. ArXiv. https://arxiv.org/abs/1807.06209.
- Aerts, D. (1984). The missing elements of reality in the description of quantum mechanics of the EPR paradox situation. Helvetica Physica Acta, 57(4), 421–428.
- Albert, D. Z. (1992). Quantum mechanics and experience. Harvard University Press. https://www.jstor.org/stable/j.ctv1bzfptv.
- Albert, D. Z. (1996). Elementary quantum metaphysics. In J. T. Cushing, A. Fine, & S. Goldstein (Eds.), Bohmian Mechanics and Quantum Theory: an Appraisal 184, 277–284. Kluwer Academic Publishers. [CrossRef]
- Albert, D. Z. (2003). Time and chance. Harvard University Press. https://www.jstor.org/stable/j.ctvjsf57g.
- Albert, D. Z., & Loewer, B. (1996). Tails of Schrodinger’s cat. In R. Clifton (Ed.), Perspectives on Quantum Reality: Non-relativistic, Relativistic, and Field-Theoretic (pp. 81–92). Kluwer Academic Publishers. https://link.springer.com/chapter/10.1007/978-94-015-8656-6_7.
- Albert, D. Z., & Ney, A. (2013). The wave function: Essays on the metaphysics of quantum mechanics. Oxford University Press. https://philpapers.org/rec/ALBTWF.
- Albert, D., & Loewer, B. (1990). Wanted dead or alive: Two attempts to solve Schrödinger’s paradox. In PSA: Proceedings of the Biennial Meeting of the Philosophy of Science Association (pp. 277–285). University of Chicago Press. https://www.jstor.org/stable/192710.
- Alberto, P., Das, S., & Vagenas, E. C. (2011). Relativistic particle in a three-dimensional box. Physics Letters A, 375(12), 1436–1440. [CrossRef]
- Allori, V. (2022). What is it like to be a relativistic GRW theory? Or: Quantum mechanics and relativity, still in conflict after all these years. Foundations of Physics, 52(4), 1–28. [CrossRef]
- Allori, V. (2013a). On the metaphysics of quantum mechanics. In S. Lebihan (Ed.), Precis de la Philosophie de la Physique (pp. 116–151). Vuibert. https://philpapers.org/rec/ALLOTM-2.
- Allori, V. (2013b). Primitive ontology and the structure of fundamental physical theories. In A. Ney & D. Alberts (Eds.), The Wave Function: Essays on the Metaphysics of Quantum Mechanic (pp. 58–75). Oxford University Press. https://philarchive.org/rec/ALLPOA.
- Allori, V. (2015). Primitive ontology in a nutshell. International Journal of Quantum Foundations, 1(3), 107–122. https://philsci-archive.pitt.edu/11651/.
- Allori, V. (2016). How to make sense of quantum mechanics: Fundamental physical theories and primitive ontology. PhilPapers. https://philpapers.org/rec/ALLQTM.
- Allori, V. (2020). Scientific realism without the wave-function: An example of naturalized quantum metaphysics. In S. French & J. Saatsi (Eds.), Scientific Realism and the Quantum (pp. 212–228). Oxford University Press. https://philarchive.org/rec/ALLSRW.
- Allori, V. (2022). Spontaneous localization theories. In G. Bacciagaluppi & O. Freire Jr. (Eds.), Oxford Handbook of the History of Interpretations and Foundations of Quantum Mechanics (pp. 1103–1134). Oxford University Press. https://philpapers.org/rec/ALLSLT-3.
- Allori, V., Bassi, A., Durr, D., & Zanghi, N. (2021). Do wave functions jump?: perspectives of the work of GianCarlo Ghirardi. Springer. https://link.springer.com/book/10.1007/978-3-030-46777-7.
- Allori, V., Goldstein, S., Tumulka, R., & Zanghi, N. (2008). On the common structure of Bohmian mechanics and the Ghirardi-Rimini-Weber theory. The British Journal for the Philosophy of Science, 59(3), 353–389. [CrossRef]
- Allori, V., Goldstein, S., Tumulka, R., & Zanghi, N. (2010). Many worlds and Schrodinger’s first quantum theory. The British Journal for the Philosophy of Science, 62(1), 1–27. [CrossRef]
- Ashtekar, A., & Barrau, A. (2015). Loop quantum cosmology: From pre-inflationary dynamics to observations. Classical and Quantum Gravity, 32(23), 234001. [CrossRef]
- Ashtekar, A., & Sloan, D. A. (2011). Probability of inflation in loop quantum cosmology. General Relativity and Quantum Cosmology, 43(12), 3619–3655. [CrossRef]
- Azhar, F., & Loeb, A. (2021). Finely tuned models sacrifice explanatory depth. Foundations of Physics, 51(5), 1–36. [CrossRef]
- Bacciagaluppi, G., & Valentini, A. (2009). Quantum theory at the crossroads: Reconsidering the 1927 Solvay conference. ArXiv (Cornell University. https://arxiv.org/abs/quant-ph/0609184.
- Bahrami, M., Bassi, A., Donadi, S., Ferialdi, L., & León, G. (2015). Irreversibility and collapse models. In A. von Müller & T. Filk (Eds.), Re-Thinking Time at the Interface of Physics and Philosophy, (Vol. 4, pp. 125–146). Springer International Publishing. [CrossRef]
- Baker, D. J. (2009). Against field interpretations of quantum field theory. The British Journal for the Philosophy of Science, 60(3), 585–609. [CrossRef]
- Banerjee, S., Bera, S., & Singh, T. P. (2016). Quantum nonlocality and the end of classical spacetime. International Journal of Modern Physics D, 25(12), 1644005–1644005. [CrossRef]
- Barrett, J. A. (2005). The preferred-basis problem and the quantum mechanics of everything. The British Journal for the Philosophy of Science, 56(2), 199–220. [CrossRef]
- Barrow, J. D. (2001). The book of nothing. Vintage. https://philpapers.org/rec/BARTBO-11.
- Bassi, A. (2009). Collapse models: Analysis of the free particle dynamics. ArXiv. [CrossRef]
- Bassi, A. (2021). Philosophy of quantum mechanics: Dynamical collapse theories. Oxford Research Encyclopedia of Physics. [CrossRef]
- Bassi, A., & Ghirardi, G. (2003). Dynamical reduction models. Physics Reports, 379(5-6), 257–426. [CrossRef]
- Bassi, A., Lochan, K., Satin, S., Singh, T. P., & Ulbricht, H. (2013). Models of wave-function collapse, underlying theories, and experimental tests. Reviews of Modern Physics, 85(2), 471–527. [CrossRef]
- Bassi, A., & Ulbricht, H. (2014). Collapse models: From theoretical foundations to experimental verifications. Journal of Physics: Conference Series, 504. [CrossRef]
- Becker, A. (2018). What is real? Basic Books.
- Bedingham, D. (2021). Collapse models, relativity, and discrete spacetime. In V. Allori, A. Bassi, D. Durr, & N. Zanghi (Eds.), Do Wave Functions Jump? Perspectives of the Work of GianCarlo Ghirardi (pp. 191–203). Springer. [CrossRef]
- Bedingham, D. (2016). Collapse models and spacetime symmetries. ArXiv. [CrossRef]
- Bell, J. S. (2004). Speakable and unspeakable in quantum mechanics: collected papers on quantum philosophy. Cambridge University Press.
- Bell, M., & Gao, S. (2016). Quantum nonlocality and reality: 50 years of Bell’s theorem. Cambridge University Press. about:blank.
- Belot, G. (2011). Quantum states for primitive ontologists. European Journal for Philosophy of Science, 2(1), 67–83. [CrossRef]
- Blandford, R. D., Dunkley, J., Frenk, C. S., Lahav, O., & Shapley, A. E . (2020). Coming of age of the standard model. Nature Astronomy, 4(2), 122–123. [CrossRef]
- Bohm, D. (1952a). Quantum theory. Prentice-Hall; Constable.
- Bohm, D. (1952b). A suggested interpretation of the quantum theory in terms of “hidden” variables. I. Physical Review, 85(2), 166–179. [CrossRef]
- Bohm, D., & Broglie, L. de. (2016). Causality and chance in modern physics. Routledge. [CrossRef]
- Bohr, N., & Einstein, A. (1949). Discussion with Einstein on epistemological problems in atomic physics. Open Court Publishing Company. https://www.marxists.org/reference/subject/philosophy/works/dk/bohr.htm.
- Bojowald, M. (2008). Loop quantum cosmology. Living Reviews in Relativity, 11(1). [CrossRef]
- Bojowald, M. (2015). Quantum cosmology: A review. Reports on Progress in Physics, 78(2). [CrossRef]
- Boyer, R. W. (2014). Did the universe emerge from nothing? Reductive vs. holistic cosmology. NeuroQuantology, 12(4), 424–454. [CrossRef]
- Boyle, L., Finn, K., & Turok, N. (2018). CPT-symmetric universe. Physical Review Letters, 121(25). [CrossRef]
- Boyle, L., & Turok, N. (2022). Thermodynamic solution of the homogeneity, isotropy, and flatness puzzles (and a clue to the cosmological constant). ArXiv (Cornell University). [CrossRef]
- Brandenberger, R. H., & Martin, J. (2013). Trans-Planckian issues for inflationary cosmology. Classical and Quantum Gravity, 30(11), 113001. [CrossRef]
- Brandenberger, R., & Peter, P. (2017). Bouncing cosmologies: Progress and problems. Foundations of Physics, 47(6), 797–850. [CrossRef]
- Brenner, A. (2016). What do we mean when we ask “why is there something rather than nothing?” Erkenntnis, 81(6), 1305–1322. https://philarchive.org/rec/BREWDW.
- Bricmont, J. (2016). Making sense of quantum mechanics. Springer. https://philpapers.org/rec/BRIMSO.
- Brunner, N., Cavalcanti, D., Pironio, S., Scarani, V., & Wehner, S. (2014). Bell nonlocality. Reviews of Modern Physics, 86(2), 419–478. [CrossRef]
- Broglie, L. de. (1964). The current interpretation of wave mechanics. Elsevier Publishing Company.
- Callender, C. (2014). One world, one beable. Synthese, 192(10), 3153–3177. [CrossRef]
- Callender, C. (2020). Can we quarantine the quantum blight? In Scientific Realism and the Quantum (pp. 57–77). Oxford University Press. http://philsci-archive.pitt.edu/15450/.
- Callender, C. (2021). Quantum mechanics: Keeping it real? The British Journal for the Philosophy of Science. [CrossRef]
- Calmet, X. (2008). On the precision of a length measurement. European Physical Journal C, 54(3), 501–505. [CrossRef]
- Calmet, X., Graesser, M., & Hsu, S. (2005). Minimum length from first principles. International Journal of Modern Physics D, 14(12), 2195–2199. [CrossRef]
- Carrol, S. M. (2019). Something deeply hidden: Quantum worlds and the emergence of spacetime. Dutton.
- Carroll, S. (2018, October 31). What is nothing? Vice Magazine Online. https://www.vice.com/en/article/vbk5va/what-is-nothing.
- Carroll, S. M. (2014). In what sense is the early universe fine-tuned? ArXiv. [CrossRef]
- Carroll, S. M. (2018). Why is there something, rather than nothing? ArXiv. [CrossRef]
- Carroll, S. M., & Chen, J. (2004). Spontaneous inflation and the origin of the arrow of time. ArXiv. https://arxiv.org/abs/hep-th/0410270.
- Carroll, S. M., & Singh, A. (2018). Mad-dog Everettianism: Quantum mechanics at its most minimal. ArXiv. [CrossRef]
- Castro, P., Gatta, M., Croca, J. R., & Moreira, R. N. (2018). Spacetime as an emergent phenomenon: A possible way to explain entanglement and the tunnel effect. Journal of Applied Mathematics and Physics, 6, 2107–2118. [CrossRef]
- Chen, E. K. (2017). Our fundamental physical space: An essay on the metaphysics of the wave function. The Journal of Philosophy, 114(7), 333–365. https://arxiv.org/abs/2110.14531.
- Chen, E. K. (2018). The intrinsic structure of quantum mechanics. ArXiv. [CrossRef]
- Chen, E. K. (2019). Realism about the wave function. Philosophy Compass, 14(7). [CrossRef]
- Chen, E. K. (2020a). The past hypothesis and the nature of physical laws. ArXiv. [CrossRef]
- Chen, E. K. (2020b). Bell’s theorem, quantum probabilities, and superdeterminism. ArXiv. [CrossRef]
- Clifton, R., & Monton, B. (1999). Losing your marbles in wavefunction collapse theories. The British Journal for the Philosophy of Science, 50(4), 697–717. [CrossRef]
- Coleman, S., & De Luccia, F. (1980). Gravitational effects on and of vacuum decay. Physical Review D, 21(12), 3305–3315. [CrossRef]
- Cordero, A. (2001). Realism and underdetermination: Some clues from the practices-up. Philosophy of Science, 68(S3), S301–S312. [CrossRef]
- Coutinho, F. A. B., & Wreszinski, W. F. (2016). Instantaneous spreading versus space localization for nonrelativistic quantum systems. Brazilian Journal of Physics, 46(4), 462–470. [CrossRef]
- Crouse, D. T. (2016). On the nature of discrete space-time: The atomic theory of space-time and its effects on Pythagoras’s theorem, time versus duration, inertial anomalies of astronomical bodies, and special relativity at the Planck scale. ArXiv. [CrossRef]
- Crouse, D., & Skufca, J. (2018). On the nature of discrete space-time: Part 1: The distance formula, relativistic time dilation, and length contraction in discrete space-time. [CrossRef]
- Davies, P. (1996, April 27). The day time began. New Scientist. https://www.newscientist.com/article/mg15020274-200-the-day-time-began/.
- Davies, P. (1997). The last three minutes: Conjectures about the ultimate fate of the universe. Phoenix.
- Davies, P. (2011, November 16). Nothingness: The turbulent life of empty space. New Scientist. https://www.newscientist.com/article/mg21228390-800-nothingness-the-turbulent-life-of-empty-space/.
- Davis, T. M., & Lineweaver, C. H. (2004). Expanding confusion: Common misconceptions of cosmological horizons and the superluminal expansion of the universe. Publications of the Astronomical Society of Australia, 21(1), 97–109. [CrossRef]
- DeWitt, B. S. (1967). Quantum theory of gravity. I. the canonical theory. Physical Review, 160(5), 1113–1148. [CrossRef]
- Diez-Tejedor, A., & Sudarsky, D. (2012). Towards a formal description of the collapse approach to the inflationary origin of the seeds of cosmic structure. Journal of Cosmology and Astroparticle Physics, 2012(07), 045–045. [CrossRef]
- Doyle, R. (2014). The origin of irreversibility. Department of Astronomy, Harvard University. https://www.informationphilosopher.com/problems/reversibility/Irreversibility.pdf.
- Durham, I. T. (2018). Bell’s theory of beables and the concept of `universe’. ArXiv (Cornell University). [CrossRef]
- Dürr, D., Goldstein, S., Taylor, J. O., Tumulka, R., & Zanghi, N. (2006). Topological factors derived from Bohmian mechanics. Annales Henri Poincaré, 7(4), 791–807. [CrossRef]
- Dyson, F. J. (1979). Time without end: Physics and biology in an open universe. Reviews of Modern Physics, 51(3), 447–460. [CrossRef]
- Eddington, A. S. (1929). The nature of the physical world. Cambridge University Press. https://henry.pha.jhu.edu/Eddington.2008.pdf.
- Egg, M. (2021). Quantum ontology without speculation. European Journal for Philosophy of Science, 11(1). [CrossRef]
- Egg, M., & Esfeld, M. (2014). Primitive ontology and quantum state in the GRW matter density theory. Synthese, 192(10), 3229–3245. [CrossRef]
- Egg, M., & Saatsi, J. (2021). Scientific realism and underdetermination in quantum theory. Philosophy Compass, 16(11). [CrossRef]
- Einstein, A. (2011). Letters on wave mechanics: Correspondence with H.A. Lorentz, Max Planck, and Erwin Schrodinger (K. Przibram, Ed.). Open Road Integrated Media.
- Einstein, A., Podolsky, B., & Rosen, N. (1935). Can quantum-mechanical description of physical reality be considered complete? Physical Review, 47(10), 777–780. [CrossRef]
- Esfeld, M. (2012). Ontic structural realism and the interpretation of quantum mechanics. European Journal for Philosophy of Science, 3(1), 19–32. [CrossRef]
- Esfeld, M. (2014). Science and metaphysics: The case of quantum physics. In A. Reboul (Ed.), Mind, Values, and Metaphysics Vol.1 (pp. 267–285). Springer International Publishing. http://philsci-archive.pitt.edu/3401/1/Esfeld-MetScience.pdf.
- Esfeld, M. (2016). Collapse or no collapse? What is the best ontology of quantum mechanics in the primitive ontology framework? In S. Gao (Ed.), Collapse of the Wave Function: Models, Ontology, Origin, and Implications. Cambridge University Press. http://philsci-archive.pitt.edu/12666/.
- Esfeld, M. (2019). From the measurement problem to the primitive ontology programme. ArXiv. https://arxiv.org/abs/1907.11058.
- Esfeld, M., & Gisin, N. (2014). The GRW flash theory: A relativistic quantum ontology of matter in space-time? Philosophy of Science, 81(2), 248–264. [CrossRef]
- Feynman, R. P. (2017). The character of physical law. The MIT Press. https://www.ling.upenn.edu/~kroch/courses/lx550/readings/feynman1-4.pdf (Original work published 1967).
- Fine, A. (1996). The shaky game. University of Chicago Press. https://www.jstor.org/stable/2215622.
- Fleming, G. (1996). Just how radical is hyperplane-dependence? In R. Clifton (Ed.), Perspectives on Quantum Reality: Non-Relativistic, Relativistic and Field Theoretic (pp. 11–28). Kluwer Academic Publishers. https://link.springer.com/chapter/10.1007/978-94-015-8656-6_2.
- Forrest, P. (1988). Quantum metaphysics. Blackwell. https://philpapers.org/rec/FORQM.
- Fortin, S., Alberto, J., & Arriaga, J. (2018). About the nature of the wave function and its dimensionality: The case of quantum chemistry. PhilSci Archive. http://philsci-archive.pitt.edu/14868/1/3N-Dimensions.pdf.
- Frigg, R. (2018). Properties and the Born rule in GRW theory. In S. Gao (Ed.), Collapse of the Wave Function: Models, Ontology, Origin, and Implications. (pp. 124–133). Cambridge University. http://philsci-archive.pitt.edu/20933/.
- Gao, S. (2013). A discrete model of energy-conserved wavefunction collapse. Royal Society Publishing. https://arxiv.org/abs/1304.0439.
- Gao, S. (2013b). Three possible implications of spacetime discreteness. PhilArchive. https://philarchive.org/archive/GAOTIOv1.
- Gao, S. (2016). The meaning of the wave function: In search of the ontology of quantum mechanics. Cambridge University Press. ArXiv. https://arxiv.org/abs/1611.02738.
- Gao, S. (2017). Is an electron a charge cloud? A reexamination of Schrödinger’s charge density hypothesis. Foundations of Science, 23(1), 145–157. [CrossRef]
- Gao, S. (2018a). Against the field ontology of quantum mechanics. PhilSci Archive. http://philsci-archive.pitt.edu/id/eprint/15476.
- Gao, S. (2018b). Collapse of the wave function: Models, ontology, origin, and implications. Cambridge University Press.
- Gao, S. (2019). Quantum theory is incompatible with relativity: A new proof beyond Bell’s theorem and a test of unitary quantum theories. PhilSci Archive. http://philsci-archive.pitt.edu/16155/.
- Gao, S. (2020). A puzzle for the field ontologists. Foundations of Physics, 50(11), 1541–1553. [CrossRef]
- Gao, S. (2022). Reality of mass and charge and its implications for the meaning of the wave function. PhilSci Archive. http://philsci-archive.pitt.edu/21317/.
- Garfinkle, D., Ijjas, A., & Steinhardt, P. J. (2023). Initial conditions problem in cosmological inflation revisited. Physics Letters B, 843, 138028. [CrossRef]
- Geert, D. (2006). The ontology of spacetime (D. Dieks, Ed.). Elsevier. https://philpapers.org/rec/DIETOO.
- Genovese, M. (2016). Experimental tests of Bell inequalities. In S. Gao & M. Bell (Eds.), Quantum Nonlocality and Reality: 50 Years of Bells Theorem. Cambridge University Press. https://journals.aps.org/prl/abstract/10.1103/PhysRevLett.49.1804.
- Genovese, M. (2023). Can quantum non-locality be connected to extra-dimensions? International Journal of Quantum Information, 21(07), 2340003. [CrossRef]
- Genovese, M., & Gramegna, M. (2019). Quantum correlations and quantum non-locality: A review and a few new ideas. Applied Sciences, 9(24), 5406. [CrossRef]
- Ghirardi, G. (2009a). The interpretation of quantum mechanics: Where do we stand? Journal of Physics: Conference Series, 174, 012013. [CrossRef]
- Ghirardi, G. (2009b). Does quantum nonlocality irremediably conflict with special relativity? Foundations of Physics, 40(9-10), 1379–1395. [CrossRef]
- Ghirardi, G. (2021). Sneaking a look at god’s cards. Princeton University Press.
- Ghirardi, G. C., Pearle, P., & Rimini, A. (1990). Markov processes in Hilbert space and continuous spontaneous localization of systems of identical particles. Physical Review A, 42(1), 78–89. [CrossRef]
- Ghirardi, G. C., Rimini, A., & Weber, T. (1986). Unified dynamics for microscopic and macroscopic systems. Physical Review D, 34(2), 470–491. [CrossRef]
- Giddings, S. B. (2019). Black holes in the quantum universe. Philosophical Transactions of the Royal Society A: Mathematical, Physical and Engineering Sciences, 377(2161), 20190029. [CrossRef]
- Goldstein, S. (1998a). Quantum theory without observers—part one. Physics Today, 51(3), 42–46. [CrossRef]
- Goldstein, S. (1998b). Quantum theory without observers—part two. Physics Today, 51(4), 38–42. [CrossRef]
- Goldstein, S., Norsen, T., Tausk, D., & Zanghi, N. (2011). Bell’s theorem. Scholarpedia, 6(10), 8378. [CrossRef]
- Graham, P. W., Kaplan, D. E., & Rajendran, S. (2018). Born again universe. Physical Review D, 97(4). [CrossRef]
- Greenberger, D. M., Horne, M. A., & Zeilinger, A. (2007). Going beyond Bell’s theorem. ArXiv. https://arxiv.org/abs/0712.0921.
- Griffiths, D. J., & Schroeter, D. F. (2018). Introduction to quantum mechanics (2nd ed.). Cambridge University Press.
- Gröblacher, S., Paterek, T., Kaltenbaek, R., Brukner, Č., Żukowski, M., Aspelmeyer, M., & Zeilinger, A. (2007). An experimental test of non-local realism. Nature, 446(7138), 871–875. [CrossRef]
- Grünbaum, A. (2009). Why is there a world AT ALL, rather than just nothing? Ontology Studies, 9, 7–19.
- Guerreiro, T., Sanguinetti, B., Zbinden, H., Gisin, N., & Suárez, A. (2012). Single-photon space-like antibunching. Physics Letters, 376(32), 2174–2177. [CrossRef]
- Guth, A. H. (2007). Eternal inflation and its implications. Journal of Physics A: Mathematical and Theoretical, 40(25), 6811–6826. [CrossRef]
- Hagar, A. (2015). Discrete or continuous?: The quest for fundamental length in modern physics. Choice Reviews Online, 52(06), 52–3157. [CrossRef]
- Harrigan, N., & Spekkens, R. W. (2010). Einstein, incompleteness, and the epistemic view of Quantum states. Foundations of Physics, 40(2), 125–157. [CrossRef]
- Hartle, J. B. (1997). Quantum cosmology: Problems for the 21st century. Physics in the 21st Century, 179–199. [CrossRef]
- Hartle, J. B., & Hawking, S. W. (1983). Wave function of the universe. Physical Review D, 28(12), 2960–2975. [CrossRef]
- awking, S. W., & Penrose, R. (1970). The singularities of gravitational collapse and cosmology. Proceedings of the Royal Society of London, 314(1519), 529–548. [CrossRef]
- Hayes, B. (2012). The science of sticky spheres. American Scientist, 100(6), 442–442. [CrossRef]
- Hegerfeldt, G. C. (1998). Instantaneous spreading and Einstein causality in quantum theory. Annalen Der Physik, 7(7-8), 716–725. about:blank.
- Hitchcock, C. (2004). Contemporary debates in philosophy of science. Blackwell Pub.
- Hobson, A. (2013). There are no particles, there are only fields. American Journal of Physics, 81(3), 211–223. [CrossRef]
- Hobson, A. (2019). Realist analysis of six controversial quantum issues. In M. R. Matthews (Ed.), Mario Bunge: A Centenary Fetschrift (pp. 329–348). Springer Nature Switzerland. https://browse.arxiv.org/pdf/1901.02088.pdf.
- Holman, M. (2018). How problematic is the near-Euclidean spatial geometry of the large-scale universe? Foundations of Physics, 48(11), 1617–1647. [CrossRef]
- Holt, J. (2012). Why does the world exist?: An existential detective story. W. W. Norton & Company.
- Hooft, G. t. (2018). Time, the arrow of time, and quantum mechanics. Frontiers in Physics, 6. [CrossRef]
- Hossenfelder, S. (2013). Minimal length scale scenarios for quantum gravity. Living Reviews in Relativity, 16(1). [CrossRef]
- Hossenfelder, S. (2014). Theory and phenomenology of space-time defects. Advances in High Energy Physics, 2014, 1–6. [CrossRef]
- Hossenfelder, S. (2018). Lost in math. Basic Books.
- Howard, D. (1985). Einstein on locality and separability. Studies in History and Philosophy of Science, 16(3), 171–201. [CrossRef]
- Howard, D. (1989). Holism, separability, and the metaphysical implications of the bell experiments. In E. McMullin (Ed.), Philosophical consequences of quantum theory: Reflections on Bell’s theorem (pp. 224–253). University of Notre Dame Press. https://philpapers.org/rec/HOWHSA-2.
- Howard, D. (1990). “Nicht sein kann was nicht sein darf,” or the prehistory of EPR, 1909–1935: Einstein’s early worries about the quantum mechanics of composite systems. In A. I. Miller (Ed.), Sixty-Two Years of Uncertainty (pp. 61–111). Cambridge University. [CrossRef]
- Howard, D. (2004). Who invented the “Copenhagen Interpretation”? A study in mythology. Philosophy of Science, 71(5), 669–682. [CrossRef]
- Hubert, M., & Romano, D. (2018). The wave-function as a multi-field. European Journal for Philosophy of Science, 8(3), 521–537. [CrossRef]
- Huggett, N., & Wuthrich, C. (2021). Out of nowhere: Introduction: The emergence of spacetime. PhilArchive. [CrossRef]
- Huggett, N., & Wüthrich, C. (2013). Emergent spacetime and empirical (in)coherence. Studies in History and Philosophy of Science Part B: Studies in History and Philosophy of Modern Physics, 44(3), 276–285. [CrossRef]
- Ijjas, A., & Steinhardt, P. J. (2022). Entropy, black holes, and the new cyclic universe. Physics Letters B, 824, 136823. [CrossRef]
- Ijjas, A., Steinhardt, P. J., & Loeb, A. (2013). Inflationary paradigm in trouble after Planck2013. ArXiv. [CrossRef]
- Isam, A. (2018). Spacetime discreteness: Shedding light on two of the simplest observations in physics. Journal of Modern Physics, 09(07), 1415–1431. [CrossRef]
- Jammer, M. (1974). The philosophy of quantum mechanics. Wiley-Interscience.
- Jones, R. H. (2018). Mystery 101: Introduction to the big questions and the limits of human knowledge. State University of New York. https://philpapers.org/rec/JONMA-2.
- Karam, R. (2020). Why are complex numbers needed in quantum mechanics? Some answers for the introductory level. American Journal of Physics, 88(1), 39–45. [CrossRef]
- Kastner, R. (2017). On quantum collapse as a basis for the second law of thermodynamics. Entropy, 19(3), 106. [CrossRef]
- Klevgard, P. A. (2020). The Mach-Zehnder interferometer and photon dualism. In J. A. Shaw, K. Creath, & V. Lakshminarayanan (Eds.), In Light in Nature VIII (pp. 61–81). SPIE. [CrossRef]
- Kochen, S., & Specker, E. (1967). The problem of hidden variables in quantum mechanics. Indiana University Mathematics Journal, 17(1), 59–87. [CrossRef]
- Kragh, H. (2013). What’s in a name: History and meanings of the term “big bang.” ArXiv. [CrossRef]
- Krauss, L. M. (2012). A universe from nothing: Why is there something rather than nothing? Free Press.
- Kumar, M. (2011). Quantum : Einstein, Bohr, and the great debate about the nature of reality. W.W. Norton & Co.
- Lam, V., & Wüthrich, C. (2018). Spacetime is as spacetime does. Studies in History and Philosophy of Modern Physics, 64, 39–51. [CrossRef]
- Landsman, K., & Reuvers, R. (2013). A flea on Schrodinger’s cat. ArXiv. [CrossRef]
- Lechuga, R. L., & Sudarsky, D. (2023). Eternal inflation and collapse theories. ArXiv. [CrossRef]
- Leggett, A. J. (2003). Nonlocal hidden-variable theories and quantum mechanics: An incompatibility theorem. Foundations of Physics, 33(10), 1469–1493. [CrossRef]
- Leifer, M. S. (2014). Is the quantum state real? An extended review of ψ-ontology theorems. Quanta, 3(1), 67. [CrossRef]
- León, G., Landau, S. J., & Piccirilli, M. P. (2014). Quantum collapse as a source of the seeds of cosmic structure during the radiation era. Physical Review, 90(8). [CrossRef]
- Leslie, J., & Kuhn, R. L. (2013). The mystery of existence. John Wiley & Sons.
- Lewis, P. (1995). GRW and the tails problem. Topoi, 14(1), 23–33. [CrossRef]
- Lewis, P. (2004). Life in configuration space. The British Journal for the Philosophy of Science, 55(4), 713–729. [CrossRef]
- Lewis, P. J. (2013). Dimension and illusion. In A. Ney & D. Albert (Eds.), The Wave Function: Essays on the Metaphysics of Quantum Mechanics (pp. 110–125). Oxford University Press. http://philsci-archive.pitt.edu/8841/.
- Lewis, P. J. (2016). Quantum ontology: A guide to the metaphysics of quantum mechanics. Oxford University Press.
- Lewis, P. J. (2017). On the status of primitive ontology. In S. Gao (Ed.), Collapse of the Wave Function: Models, Ontology, Origin, and Implications (pp. 154–166). Cambridge University. http://philsci-archive.pitt.edu/14421/.
- Lineweaver, C. H., & Davis, T. M. (2005, March). Misconceptions about the Big Bang. Scientific American, 292(3), 36–45. [CrossRef]
- Lucia, U., & Grisolia, G. (2022). Thermodynamic definition of time: Considerations on the EPR paradox. Mathematics, 10(15), 2711. [CrossRef]
- Mack, K. (2020). The end of everything: (Astrophysically speaking). Scribner.
- Mariani, C. (2022a). Does the primitive ontology of GRW rest on shaky ground? In V. Allori (Ed.), Quantum Mechanics and Fundamentality (pp. 127–139). Springer, Cham. [CrossRef]
- Mariani, C. (2022b). Non-accessible mass and the ontology of GRW. Studies in History and Philosophy of Science, 91, 270–279. [CrossRef]
- Martin, J., & Brandenberger, R. H. (2001). Trans-Planckian problem of inflationary cosmology. 63(12). ArXiv. https://arxiv.org/abs/hep-th/0005209.
- Maudlin, T. (1995). Three measurement problems. Topoi, 14(1), 7–15. [CrossRef]
- Maudlin, T. (2007). Completeness, supervenience, and ontology. Journal of Physics A, 40(12), 3151–3171. [CrossRef]
- Maudlin, T. (2010). Can the world be only wavefunction? In S. Saunders, J. Barrett, A. Kent, & D. Wallace (Eds.), Everett, Quantum Theory, & Reality (pp. 121–143). [CrossRef]
- Maudlin, T. (2011). Quantum non-locality & relativity : Metaphysical intimations of modern physics. (3rd ed.). John Wiley.
- Maudlin, T. (2013a). Philosophy of physics: Space and time. Princeton University Press, 50(05), 50–2595. [CrossRef]
- Maudlin, T. (2013b). The nature of the quantum state. In D. Albert & A. Ney (Eds.), The Wave Function: Essays on the Metaphysics of Quantum Mechanics (pp. 126–153). [CrossRef]
- Maudlin, T. (2014). What Bell did. Journal of Physics A: Mathematical and Theoretical, 47(42), 424010. [CrossRef]
- Maudlin, T. (2015). The universal and the local in quantum theory. Topoi, 34(2), 349–358. [CrossRef]
- Maudlin, T. (2016). Local beables and the foundations of physics. In M. Bell & S. Gao (Eds.), Quantum Nonlocality and Reality: 50 Years of Bell’s Theorem (pp. 317–330). Cambridge University Press. [CrossRef]
- Maudlin, T. (2018). Ontological clarity via canonical presentation: Electromagnetism and the Aharonov–Bohm effect. Entropy, 20(6), 465. [CrossRef]
- Maudlin, T. (2019). Philosophy of physics: Quantum theory (Vol. 9). Princeton University Press.
- Maudlin, T. (2020). Appreciating what he did. In V. Allori, A. Bassi, D. Durr, & N. Zanghi (Eds.), Do Wave Functions Jump? Perspectives of the Work of GianCarlo Ghirardi (pp. 55–61). Springer. [CrossRef]
- Maudlin, T., Okon, E., & Sudarsky, D. (2020). On the status of conservation laws in physics: Implications for semiclassical gravity. Studies in History and Philosophy of Science Part B: Studies in History and Philosophy of Modern Physics, 69, 67–81. [CrossRef]
- McCoy, C. D. (2015). Does inflation solve the hot Big Bang model’s fine-tuning problems? Studies in History and Philosophy of Science Part B: Studies in History and Philosophy of Modern Physics, 51, 23–36. [CrossRef]
- McQueen, K. J. (2015). Four tails problems for dynamical collapse theories. Studies in History and Philosophy of Science Part B: Studies in History and Philosophy of Modern Physics, 49, 10–18. [CrossRef]
- Melia, F. (2020). The cosmic spacetime. CRC Press.
- Melia, F. (2021a). Thermodynamics of the rh = ct universe: A simplification of cosmic entropy. The European Physical Journal C, 81(234). [CrossRef]
- Melia, F. (2021b). Classicization of quantum fluctuations at the Planck scale in the rh = ct universe. Physics Letters B, 818, 136362. [CrossRef]
- Melia, F. (2022a). A candid assessment of standard cosmology. Publications of the Astronomical Society of the Pacific, 134(1042), 121001. [CrossRef]
- Melia, F. (2022b). Initial energy of a spatially flat universe -- a hint of its possible origin. ArXiv. [CrossRef]
- Melia, F. (2023). The Friedmann–Lemaître–Robertson–Walker metric and the principle of equivalence. Zeitschrift Für Naturforschung, 78(6), 525–533. [CrossRef]
- Mermin, N. D. (1981). Quantum mysteries for anyone. The Journal of Philosophy, 78(7), 397–408. [CrossRef]
- Monton, B. (2002). Wave function ontology. Synthese, 130(2), 265–277.
- Monton, B. (2004). The problem of ontology for spontaneous collapse theories. Studies in History and Philosophy of Science Part B: Studies in History and Philosophy of Modern Physics, 35(3), 407–421. [CrossRef]
- Monton, B. (2006). Quantum mechanics and 3n-dimensional space. Philosophy of Science, 73(5), 778–789. [CrossRef]
- Monton, B. (2013). Against 3n-dimensional space. In A. Ney & D. Albert (Eds.), The Wave Function: Essay in the Metaphysics of Quantum Mechanics (pp. 154–167). Oxford University Press. [CrossRef]
- Musser, G. (2015). Spooky action at a distance: The phenomenon that reimagines space and time and what it means for black holes, the big bang, and theories of everything. Scientific American/Farrar, Straus and Giroux.
- Myrvold, W. C. (2002). On peaceful coexistence: Is the collapse postulate incompatible with relativity? Studies in History and Philosophy of Modern Physics, 33(3), 435–466. [CrossRef]
- Myrvold, W. C. (2015). What is a wavefunction? Synthese, 192(10), 3247–3274. [CrossRef]
- Myrvold, W. C. (2017). Ontology for collapse theories. In S. Gao (Ed.), Collapse of the Wave Function: Models, Ontology, Origin, and Implications (pp. 97–123). Cambridge University Press. http://philsci-archive.pitt.edu/13318/.
- Myrvold, W. C. (2019a). Ontology for relativistic collapse theories. In O. Lombardi, S. Fortin, & C. Lopez (Eds.), Quantum Worlds: Perspectives on the Ontology of Quantum Mechanics (pp. 9–31). Cambridge University Press. [CrossRef]
- Myrvold, W. C. (2019b). How could relativity be anything other than physical? Studies in History and Philosophy of Science Part B: Studies in History and Philosophy of Modern Physics, 67, 137–143. [CrossRef]
- Myrvold, W. C. (2019c). On the status of quantum state realism. ArXiv. [CrossRef]
- Narasimhan, A., Chopra, D., & Kafatos, M. C. (2019). The nature of the Heisenberg-von Neumann cut: Enhanced orthodox interpretation of quantum mechanics. Activitas Nervosa Superior, 61(12-17), 12–17. [CrossRef]
- Neumaier, A. (2019). Foundations of quantum physics I. A critique of the tradition. ArXiv. [CrossRef]
- Ney, A. (2016). Separability, locality, and higher dimensions in quantum mechanics. Philarchive.org. https://philarchive.org/rec/NEYSLA.
- Ney, A. (2021). The world in the wave function : A metaphysics for quantum physics. Oxford University Press.
- Ney, A. (2023). Three arguments for wave function realism. European Journal for Philosophy of Science, 13(4). [CrossRef]
- Norsen, T. (2005). Einstein’s boxes. American Journal of Physics, 73(2), 164–176. [CrossRef]
- Norsen, T. (2010). The theory of (exclusively) local beables. Foundations of Physics, 40(12), 1858–1884. [CrossRef]
- Norsen, T. (2011). John S. Bell’s concept of local causality. American Journal of Physics, 79(12), 1261–1275. [CrossRef]
- Norsen, T. (2016). Quantum solipsism and nonlocality. In M. Bell & S. Gao (Eds.), Quantum Nonlocality and Reality: 50 Years of Bell’s Theorem (pp. 204–237). Cambridge University Press.
- Norsen, T. (2017). Foundations of quantum mechanics: An exploration of the physical meaning of quantum theory. Springer.
- Norsen, T. (2018). On the explanation of Born-rule statistics in the de Broglie-Bohm Pilot-Wave theory. Entropy, 20(6), 422. [CrossRef]
- Norsen, T., Marian, D., & X. Oriols. (2014). Can the wave function in configuration space be replaced by single-particle wave functions in physical space? Synthese, 192(10), 3125–3151. [CrossRef]
- North, J. (2013). The structure of a quantum world. In A. Ney & D. Albert (Eds.), The Wave Function Essays on the Metaphysics of Quantum Mechanics (pp. 183–202). Oxford University Press. https://philpapers.org/rec/NORTSO-5.
- Ojo, A. (2005). On a thermodynamic basis for inflationary cosmology. ArXiv. [CrossRef]
- Okon, E., & Sudarsky, D. (2014). Benefits of objective collapse models for cosmology and quantum gravity. Foundations of Physics, 44(2), 114–143. [CrossRef]
- Oldofredi, A. (2018). Particle creation and annihilation: Two Bohmian approaches. Lato Sensu: Revue de La Société de Philosophie Des Sciences, 5(1), 77–85. [CrossRef]
- OpenAI. (2023). ChatGPT (Mar 14 version) [Large language model]. https://chat.openai.com/chat.
- Patel, V., & Lineweaver, C. (2017). Solutions to the cosmic initial entropy problem without equilibrium initial conditions. Entropy, 19(8), 411. [CrossRef]
- Pearle, P. (1989). Combining stochastic dynamical state-vector reduction with spontaneous localization. Physical Review E, 39(5), 2277–2289. [CrossRef]
- Pearle, P. (2000). Wavefunction collapse and conservation laws. ArXiv. https://arxiv.org/abs/quant-ph/0004067.
- Pearle, P. (2009). How stands collapse II. In W. Myrvold & J. Christian (Eds.), Quantum Reality, Relativistic Causality and Closing the Epistemic Circle: Essays in Honor of Abner Shimony (pp. 257–292). Springer. [CrossRef]
- Penrose, R. (1989a). The emperor’s new mind: Concerning computers, minds, and the laws of physics. Oxford University Press.
- Penrose, R. (1989b). Difficulties with inflationary cosmology. Annals of the New York Academy of Sciences, 571(1), 249–264. [CrossRef]
- Penrose, R. (1996). On gravity’s role in quantum state reduction. General Relativity and Gravitation, 28(5), 581–600. [CrossRef]
- Penrose, R. (1997). Physics and the mind. In M. Longair (Ed.), The Large, the Small and the Human Mind (pp. 93–143). Cambridge University Press.
- Penrose, R. (2006). Before the big bang: An outrageous new perspective and its implications for particle physics. Proceedings of EPAC 2006, 060626, 2759–2767.
- Penrose, R. (2009). Black holes, quantum theory and cosmology. Journal of Physics: Conference Series, 174, 012001. [CrossRef]
- Penrose, R. (2018). The big bang and its dark-matter content: Whence, whither, and wherefore. Foundations of Physics, 48(10), 1177–1190. [CrossRef]
- Pérez, A., Sahlmann, H., & Sudarsky, D. (2006). On the quantum origin of the seeds of cosmic structure. Classical and Quantum Gravity, 23(7), 2317–2354. [CrossRef]
- Price, H. (1995). Cosmology, time’s arrow, and that old double standard. In S. Savitt (Ed.), Time’s Arrows Today: Recent Physical and Philosophical Work on the Direction of Time (pp. 66–94). Cambridge University Press. [CrossRef]
- Price, H. (1996). Time’s arrow & Archimedes’ point : New directions for the physics of time. Oxford University Press. https://philpapers.org/rec/PRITAA-2.
- Price, H. (2004). On the origins of the arrow of time: Why there is still a puzzle about the low entropy past. In C. Hitchcock (Ed.), Contemporary Debates in the Philosophy of Science (pp. 219–239). Blackwell Publishing Ltd. https://philpapers.org/rec/PRIOTO-2.
- Pusey, M. F., Barrett, J., & Rudolph, T. (2012). On the reality of the quantum state. Nature Physics, 8(6), 475–478. [CrossRef]
- Redd, N. T. (2013). What is dark energy? Space.com. https://www.space.com/20929-dark-energy.html.
- Renou, M.-O., Trillo, D., Weilenmann, M., Thinh, L. P., Tavakoli, A., Gisin, N., Acín, A., & Navascués, M. (2021). Quantum physics needs complex numbers. Research Gate. https://www.researchgate.net/publication/348802613_Quantum_physics_needs_complex_numbers.
- Romano, D. (2020). Multi-field and Bohm’s theory. Synthese, 198(11), 10587–10609. [CrossRef]
- Rovelli, C. (2006). The disappearance of space and time. In D. Dieks (Ed.), The Ontology of Spacetime (Vol. 1, pp. 25–36). Elsevier. https://www.sciencedirect.com/science/article/abs/pii/S1871177406010023.
- Rovelli, C. (2011). “Forget time.” Foundations of Physics, 41(9), 1475–1490. [CrossRef]
- Rovelli, C. (2019). Where was past low-entropy? Entropy, 21(5), 466. [CrossRef]
- Rovelli, C., Carnell, S., & Segre, E. (2018). Reality is not what it seems: The journey to quantum gravity. Riverhead Books.
- Rovelli, C., & Smolin, L. (1995). Discreteness of area and volume in quantum gravity. Nuclear Physics B, 442(3), 593–619. [CrossRef]
- Rovelli, C., & Speziale, S. (2003). Reconcile Planck-scale discreteness and the Lorentz-Fitzgerald contraction. Physical Review D, 67(6). [CrossRef]
- Rovelli, C., & Vidotto, F. (2022). Philosophical foundations of loop quantum gravity. ArXiv. [CrossRef]
- Schlosshauer, M. (2005). Decoherence, the measurement problem, and interpretations of quantum mechanics. Reviews of Modern Physics, 76(4), 1267–1305. [CrossRef]
- Schlosshauer, M. (2019). The quantum-to-classical transition and decoherence. ArXiv. [CrossRef]
- Sebens, C. T. (2021). Electron charge density: A clue from quantum chemistry for quantum foundations. Foundations of Physics, 51(4). [CrossRef]
- Sebens, C. T. (2023). Eliminating electron self-repulsion. Foundations of Physics, 53(4). [CrossRef]
- Sedley, D. (1982). Two conceptions of vacuum. Phronesis, 27(1-2), 175–193. [CrossRef]
- Shankar, R. (1994). Principles of quantum mechanics (2nd ed.). Springer US. [CrossRef]
- Singh, T. P. (2017). Wave function collapse, non-locality, and space-time structure. In S. Gao (Ed.), Collapse of the Wave Function: Models, Ontology Origin, and Implications (pp. 295–311). [CrossRef]
- Singh, T. P. (2018). Space-time from collapse of the wave-function. Zeitschrift Für Naturforschung A, 74(2), 147–152. [CrossRef]
- Slowik, E. (2016). A historical defense of non-spacetime hypotheses: Non-Local beables and Leibnizian Ubeity. Philosophia Scientiae, 20(3), 149–166. [CrossRef]
- Smolin, L. (2004). Atoms of space and time. Scientific American, 290(1), 66–75. https://www.jstor.org/stable/26172656.
- Smolin, L. (2008). Generic predictions of quantum theories of gravity. In D. Oriti (Ed.), Approaches to Quantum Gravity: Toward a New Understanding of Space, Time, and Matter (pp. 548–570). Cornell University. https://arxiv.org/abs/hep-th/0605052.
- Smolin, L. (2017). Three roads to quantum gravity. Basic Books.
- Snyder, D. M. (2000). Irreversibility and measurement in quantum mechanics. ArXiv. [CrossRef]
- Solé, A. (2013). Bohmian mechanics without wave function ontology. Studies in History and Philosophy of Science Part B: Studies in History and Philosophy of Modern Physics, 44(4), 365–378. [CrossRef]
- Solé, A., & Hoefer, C. (2015). Introduction: Space–time and the wave function. Synthese, 192(10), 3055–3070. [CrossRef]
- Sorensen, R. (2020). Nothingness. In E. N. Zalta & U. Nodelman (Eds.), Stanford Encyclopedia of Philosophy (Spring 2020). Metaphysics Research Lab, Stanford University. https://plato.stanford.edu/archives/spr2020/entries/nothingness/.
- Srinivas, M. (1980). Collapse postulate for observables with continuous spectra. Communications in Mathematical Physics, 71(2), 131–158. [CrossRef]
- Stapp, H. P. (2017). Quantum theory and free will. In Springer eBooks. Springer International Publishing. [CrossRef]
- Steinhardt, P. J. (2011, April 1). The inflation debate: Is the theory at the heart of modern cosmology deeply flawed? Scientific American, 18–25. https://www.scientificamerican.com/article/the-inflation-summer/.
- Steinhardt, P. J., & Turok, N. (2007). Endless universe : Beyond the big bang. Broadway Books.
- Stoica, O. C. (2019). Representation of the wave function on the three-dimensional space. Physical Review A, 100(4). [CrossRef]
- Sudarsky, D. (2010). The quantum origin of the seeds of cosmic structure: The case for a missing link. AIP Conference Proceedings, 1256, 107–121. [CrossRef]
- Sudarsky, D. (2011). Shortcomings in the understanding of why cosmological perturbations look classical. International Journal of Moder Physics D, 20(04), 509–552. [CrossRef]
- Tatum, E. T. (2018). Why flat space cosmology is superior to standard inflationary cosmology. Journal of Modern Physics, 09(10), 1867–1882. [CrossRef]
- Tatum, E. T., & Seshavatharam, U. (2021). Flat space cosmology. Universal-Publishers.
- Torre, A. (2016). Do we finally understand quantum mechanics? ArXiv. [CrossRef]
- Tumulka, R. (2017). Paradoxes and primitive ontology in collapse theories of quantum mechanics. In S. Gao (Ed.), Collapse of the Wave Function: Models, Ontology, Origin and Implications (pp. 134–153). Cambridge University Press. https://arxiv.org/pdf/1102.5767.pdf?origin=publication_detail.
- Turok, N., & Boyle, L. (2022). Gravitational entropy and the flatness, homogeneity and isotropy puzzles. ArXiv. [CrossRef]
- Turok, N., & Boyle, L. (2023). A minimal explanation of the primordial cosmological perturbations. ArXiv. [CrossRef]
- Unger, R. M., & Smolin, L. (2015). The singular universe and the reality of time : A proposal in natural philosophy. Cambridge University Press.
- Vagnozzi, S., & Loeb, A. (2022). The challenge of ruling out inflation via the primordial graviton background. The Astrophysical Journal Letters, 939(2), L22. [CrossRef]
- Vaidman, L. (2020). Derivations of the born rule. In M. Hemmo & O. Shenko (Eds.), Jerusalem Studies in Philosophy and History of Science (pp. 567–584). Springer. [CrossRef]
- Vaidman, L. (2021a). Many-Worlds interpretation of quantum mechanics. The Stanford Encyclopedia of Philosophy. https://plato.stanford.edu/archives/fall2021/entries/qm-manyworlds.
- Vaidman, L. (2021b). Wave function realism and three dimensions. In V. Allori (Ed.), Quantum Mechanics and Fundamentality; Naturalizing Quantum Theory between Scientific Realism and Ontological Indeterminacy . Springer. http://philsci-archive.pitt.edu/19979/.
- Verlinde, E. (2000). On the holographic principle in a radiation dominated universe. ArXiv. [CrossRef]
- Verlinde, E. (2011). On the origin of gravity and the laws of Newton. Journal of High Energy Physics, 4, 1–27. [CrossRef]
- Vilenkin, A. (1982). Creation of universes from nothing. Physics Letters B, 117(1-2), 25–28. [CrossRef]
- von Neumann, J. (1955). Mathematical foundations of quantum mechanics (R. Beyer, Trans.). Princeton University Press.
- Wald, R. M. (2006). The arrow of time and the initial conditions of the universe. Studies in History and Philosophy of Science Part B: Studies in History and Philosophy of Modern Physics, 37(3), 394–398. [CrossRef]
- Wallace, D. (2010). Gravity, entropy, and cosmology: In search of clarity. The British Journal for the Philosophy of Science, 61(3), 513–540. [CrossRef]
- Wallace, D. (2017). Against wavefunction realism. In S. Dasgupta, R. Dotan, & B. Weslake (Eds.), Current Controversies in Philosophy of Science (pp. 63–74). Routledge. https://philpapers.org/rec/WALAWR-3.
- Wallace, D. (2018). On the plurality of quantum theories: Quantum theory as a framework, and its implications for the quantum measurement problem. In S. French & J. Saatsi (Eds.), Scientific Realism and the Quantum Measurement Problem (pp. 78–102). Oxford University Press. http://philsci-archive.pitt.edu/15292/.
- Wallace, D. (2019). What is orthodox quantum mechanics? In A. Cordero (Ed.), Philosophers Look at Quantum Mechanics (pp. 285–312). Springer International Publishing. [CrossRef]
- Wallace, D. (2018). Why black hole information loss is paradoxical. ArXiv.org. [CrossRef]
- Wallace, D., & Timpson, C. G. (2010). Quantum mechanics on spacetime I: Spacetime state realism. The British Journal for the Philosophy of Science, 61(4), 697–727. [CrossRef]
- Weatherall, J. O. (2016). Void. Yale University Press.
- Webb, J. (2014). Nothing : Surprising insights everywhere from zero to oblivion. Profile Books Ltd.
- Wechsler, S. (2021). About the nature of the quantum system—an examination of the random discontinuous motion interpretation of the quantum mechanics. Journal of Quantum Information Science, 11(03), 99–111. [CrossRef]
- Wechsler, S. D. (2020). In praise and in criticism of the model of continuous spontaneous localization of the wave-function. Journal of Quantum Information Science, 10(04), 73–103. [CrossRef]
- Wechsler, S. D. (2021). The quantum mechanics needs the principle of wave-function collapse—but this principle shouldn’t be misunderstood. Journal of Quantum Information Science, 11(01), 42–63. [CrossRef]
- Wiseman, H. M. (2006). From Einstein’s theorem to Bell’s theorem: a history of quantum non-locality. Contemporary Physics, 47(2), 79–88. [CrossRef]
- Witherall, A. (2001). The fundamental question. Journal of Philosophical Research, 26, 53–87. https://philpapers.org/rec/WITTFQ.
- Wittgenstein, L., & Russell, B. (2021). Tractatus logico-philosophicus (C. K. Ogden, Trans.). Routledge. https://www.gutenberg.org/files/5740/5740-pdf.pdf (Original work published 1921).
- Wolf, W. J., & Thébault, K. P. Y. (2023). Explanatory depth in primordial cosmology: A comparative study of inflationary and bouncing paradigms. The British Journal for the Philosophy of Science. [CrossRef]
| 1 | Despite the Dual Ontology’s unique structure, “It may be that a real synthesis of quantum and relativity theories requires not just technical developments but radical conceptual renewal.” (Bell, 2004, p. 171). |
| 2 | See (Monton, 2002, 2006) regarding mixed ontologies and the problems associated with ontologies that are not based upon a one-to-one identity and mapping. See also (Maudlin, 2013b). |
| 3 | In the abstract, a ((3 x N) + 3) hyperspace formed by a (3 x N) Planck Space and the three spatial dimensions of 4D spacetime appears to be an imposing physical structure. From a non-technical perspective, however, the closed universe formed by the Dual Ontology is a combination of the 4D spacetime of everyday experience and a many-dimensional Planck Space. The Planck Spheres that comprise 4D spacetime on a one-to-one basis also comprise Planck Space. As a simple example, assume that there are 5 Planck Spheres in 4D spacetime, one each somewhere on Venus, Mars, the Milky Way Galaxy, the Andromeda Galaxy, and the Orion Constellation. The 5 Planck Spheres in 4D spacetime form a (3 x N) space in Planck Space where N equals five, and the number of dimensions equals 15. Unlike 4D spacetime, the Planck Spheres in Planck Space are not separated by time, space, or volume. All of the Planck Spheres in 4D spacetime and the corresponding Planck Spheres in Planck Space form a closed ((3 x N) +3) hyperspace where (3 x N) represents Planck Space and “3” represents the three spatial dimensions of 4D spacetime. |
| 4 | For a discussion regarding a mathematical (3 x N) dimensional space composed of an ordered N-tuple of ordered triples and the difference between a (3 x N) space and a 3N space, see (Lewis, 2013, pp. 116-118). |
| 5 | “My own view is that…belief in the completeness of Relativity as an account of space-time structure has been irrationally fetishized just as belief in the completeness of the quantum-mechanical description had been by Bohr and company.” (Maudlin, 2014, p. 24). |
| 6 | See generally (Maudlin, 2013b, 2019, pp. 36-37 and 79-93); (Monton, 2006); (Pusey et al., 2012). |
| 7 | (Barrow, 2002); (Grunbaum, 2009); (Holt, 2012). The existence of the SOAN turns one of the greatest philosophical questions of all time on its head. The question is not, “Why is there something rather than nothing.” Rather, the question is, “Why is there something AND nothing.” |
| 8 | See section 2.1 for a complete definition. |
| 9 | See generally (Bedingham, 2021); (Gao, 2018, pp. 248-253); (Smolin, 2004). |
| 10 | The calculation assumes the diameter of a Planck Sphere equals the Planck length. The designation of a Planck Sphere as the smallest unit of space is illustrative only. The actual shape and size of the smallest unit of space may differ. |
| 11 | See (Howard, 1989, pp. 247-253) for an early consideration of higher dimensional spaces. |
| 12 | See also (Albert, 1992, 2013); (Chen, 2019); (Ney, 2021) regarding the ontic nature of a wave function in higher dimensional 3N spaces. |
| 13 | See also (Genovese, 2023); (Genovese and Gramenga, 2022). |
| 14 | See Section 4.3 for a discussion on the physical triggers for quantum state collapse and Section 4.5 for a more comprehensive discussion regarding instantaneity. |
| 15 | The location of a quantum state following its collapse is unknown. Accordingly, the term “generally localized” denotes the general location of a quantum state in 4D spacetime. See Section 4.4 for a more complete discussion. |
| 16 | For a mathematical 3N notation the transition would be ∣ψ 1,2⟩→∣ψ 1⟩⊗∣ψ 2⟩. The (3 x N) notation describes the transition of an entangled state to a product state in a physical (3 x N) Planck Space in which there is a one-to-one mapping and identity between a Planck Sphere in 4D spacetime and Planck Space. |
| 17 | See also (Ney, 2021, pp. 10-11). |
| 18 | This conclusion is at odds with the Born rule and its probability density interpretation of wave-function collapse for continuous variables. Not only does the physical structure of the Dual Ontology differ from the ontology used to derive the Born Rule, but the collapse of a quantum state to a discrete subset of the Bell Quantum Spheres occupied by the quantum state prior to collapse is a probability, not a probability density. Rather than integrating a density function of the quantum state over a continuous space, the likelihood of generally locating the electron in a “discrete, constrained space” is a probability based on the square modulus of the quantum state’s wave function. OpenAI. (2023). Background information on the Born Rule. See also (Norsen, 2017, pp. 238-239) regarding the use of two real functions to replace a complex wave function in 4D spacetime. |
| 19 | Note that the “Planck Energy Hyper-Point” and “4D Energy Field” include dark energy and any other form of energy not yet identified. |
| 20 | For extensive information regarding the Planck data, see (Aghanim et al., 2018). |
| 21 | More technically, data from the Planck satellite and other sources indicates that the curvature parameter Ωk is -0.0005 ± 0.0005, corresponding to a 4D spacetime within 0.1% of being flat. Accordingly, ρ4D =
|
| 22 | See generally (Carroll & Chen, 2004); (Penrose, 1991, 2006). |
| 23 | The CMB and related data indirectly indicate that the spatial geometry of 4D spacetime was nearly homogeneous and isotropic near t = 0. The FLRW metric supports a spatial geometry that is homogeneous and isotropic at each 3D time slice. |
| 24 | The generalized localization of 4D Spacetime at t = 0 does not involve the destruction or localization of the Planck Spheres that comprise Planck Space, nor does it affect the SOAN. Although the energy content of the 4D Energy Field and the Planck Energy Hyper-Point are localized at t = 0, Planck Spheres and the SOAN continue to form an ultra-high dimensional (3 x N) space. See Section 7. |
| 25 | See (Holt, 2012) for an extensive discussion on the issues associated with a physical state of nothingness. See also (Barrow, 2002); (Kuhn, 2013). |
| 26 | The SOAN is a difficult concept to grasp fully. For example, see (Davies, 2013, p. 126). “The concept of a true void…strikes many people as preposterous or even meaningless. If two bodies are separated by nothing, should they not be in contact? How can ‘emptiness’ keep things apart or have properties such as size or boundaries?” |
| 27 | See also, Interview with Sean Carroll, Vice Magazine On Line. What is Nothing? with Nick Rose, October 31, 2018. |
| 28 | (Bedingham, 2016); (Hagar, 2014); Hossenfelder, 2013); (Rovelli, 2017); OpenAI (2023). |
| 29 | Arguments in favor of the discretization of space have been proposed based upon mathematics, electrodynamics, quantum electrodynamics, loop quantum gravity, loop quantum cosmology, string theory, discrete lattice, asymptotic safe gravity, causal sets, spin foams, deformed special relativity, causal dynamical triangulation, quantum graphity, and black hole theory among others. (Crouse, 2016); (Crouse & Skufca, 2018); (Hagar, 2014); (Hossenfelder, 2014). |
| 30 | (Hossenfelder, 2013). |
| 31 | The non-relativistic Schrödinger equation is based on a continuous 3D space and treats time as a separate parameter. |
| 32 | Black holes are also composed of Planck Spheres. |
| 33 | See also footnote 4. |
| 34 | (Lewis, 2013, p. 116); (Ney, 2013). Note also that a (3N + 3) hyperspace raises serious theoretical and mathematical issues. A wavefunction that describes a quantum state in a 3N space cannot simultaneously describe the same quantum state in a discrete 3D space. |
| 35 | “We first note that most authors agree that habitable universes should have only one time dimension [56, 84, 506, 507]. If space-time had more than one temporal dimension, then closed time-like loops could be constructed. Such loops, in turn, allow for observers to revisit the “past” and thereby affect causality. In addition to violations of causality, multiple time dimensions can lead to violations of unitarity, tachyons, and ghosts [212].” (Adams, 2019, p. 158). |
| 36 | Unlike quantum mechanics, quantum chemistry often approaches N-body quantum states differently. See generally (Fortin et al., 2018); (Sebens, 2021). |
| 37 | For an alternative approach, see (Norsen et al., 2015). |
| 38 | The preferred basis for all observations of a quantum state is the position basis. “The second moral is that in physics the only observations we must consider are position observations, if only the positions of instrument pointers.” (Bell, 204, p. 161). See also (Maudlin, 2019, pp. 48-50). |
| 39 | See section 4.3 below for additional information on “physical triggers.” |
| 40 | See section 4.4 for additional information on generalized localization. |
| 41 | (Allori, 2022); (Bricmont, 2016); (Broglie, 1964); (Norsen, 2005). |
| 42 | See generally (Allori et al., 2021). |
| 43 | Despite its metaphorical usefulness, “quantum tunneling” is neither a jump nor a tunneling process. The instantaneous collapse of a quantum state at some point after the quantum state’s leading edge has passed through a physical barrier that is classically impenetrable reflects the collapse of the quantum state’s Bell Quantum Hyper-Point, not the tunneling of the entire quantum state through an otherwise impassible barrier. Following the quantum state’s collapse, its Bell Quantum Field is generally localized on the other side of the physical barrier. See generally (Castro et al., 2018). |
| 44 | The dynamic evolution and collapse of quantum states also apply to black hole information loss. See generally (Giddings, 2019); (Wallace D., 2018). |
| 45 | (Bohm, 1951, pp. 611-619). |
| 46 | Although the Stern-Gerlach experiment in the z direction is conducted in 4D spacetime on either Bell Quantum Field E in Princeton or Bell Quantum Field F in Copenhagen, the Bell Identity ensures that the experiment is simultaneously reflected on Bell Quantum Hyper-Point EF in Planck Space. More generally, any quantum experiment in 4D spacetime is always reflected simultaneously on the quantum state’s Bell Quantum Hyper-Point in Planck Space. |
| 47 | Double-slit experiments describe a quantum state by its wave function rather than the considerably more amorphous terms “charge density” or “energy content.” Nevertheless, since all quantum states are real if an electron passes through slits A and B, the charge density, and the energy content of the electron, however ill-defined after it has passed through slits A and B, must also do so. See (Sebens C. T., 2021). |
| 48 | The “which way” monitoring experiment is based upon the example discussed in (Maudlin, 2019). |
| 49 | (Lewis, 2016, pp. 72-107). |
| 50 | In Planck Space, a single Bell Quantum Hyper-Point is neither space-like separated nor a separable system. See Sections 5.1 and 5.2 below. See also (Ney, 2021, pp. 112-128); (Howard, 1985, p. 197). |
| 51 | For a detailed discussion, see (Ney, 2021). |
| 52 | The unobservable Universe is considerably larger than the observable universe and may be infinite. |
| 53 | Although decoherence extensively considers environmental triggers and the loss of coherence rather than a specific quantum collapse mechanism, the Dual Ontology conjecture supports a very specific collapse mechanism initiated exclusively by 4D spacetime Physical Interactions. |
| 54 | No assumptions are made regarding dark matter or dark energy. |
| 55 | See also (Licata & Chiatti, 2019). |
| 56 | Although physical triggers such as nuclear interactions on the sun may be statistically determinable, individual quantum state collapses are still time and location-dependent. |
| 57 | The collapse rate of individual quantum states on the sun is directly related to temperature and location. |
| 58 | Humans can, at will, use a scanning tunneling microscope (STM) to control and precisely vary the collapse rate of electrons. |
| 59 | (Bassi, et al., 2012). |
| 60 | (Ghirardi, 2004, p. 406). |
| 61 | Note that the Dual Ontology conjecture conflicts with mathematical models that describe quantum state collapse to a single dimensionless point, a Dirac delta function, or an eigenstate of position with a single discrete value. |
| 62 | From the perspective of the Dual Ontology conjecture, "The problem is that mathematics has become too dominant in physics. We have become so focused on finding mathematical descriptions of nature that we have forgotten to ask if these descriptions are actually true. We have become so entranced by the beauty of mathematics that we have lost sight of the goal of physics, which is to understand the real world." (Hossenfelder, 2018, p. 8). “Physics is not mathematics, and mathematics is not physics.” (Feynman, 1985, p. 55). |
| 63 | (Bell, 2004, p. 171); See also (Norsen, 2011, p. 1216). |
| 64 | The terminology that describes 4D spacetime may confirm Ludwig Wittgenstein’s concern that “The limits of my language means the limits of my world.” (Wittgenstein, 1922, p. 74). |
| 65 | Although the term “non-separable” has multiple definitions, a stronger version of that term holds that “…the non-separability of states is the claim that spatio-temporal separation is not a sufficient condition for the individuating systems themselves, that under certain circumstances the contents of two spatio-temporally separated regions of space-time constitute just a single system”. (Howard, 1989). For a slightly different perspective, see (Ney, 2016). Einstein’s primary concern was not with non-separability per se but with the possibility that non-separability implied a violation of his theory of Special Relativity. (Howard, 1985, pp. 172-173); (Howard, 1989, p. 232). |
| 66 | See also (Ney, 2016, 2021). |
| 67 | The non-separability of a Bell Quantum Hyper-Point addresses a concern raised by Einstein. Einstein questioned whether spatially separated quantum states in 4D spacetime had an independent reality. (Wiseman, 2006). The existence of a single ultra-high dimensional Bell Quantum Hyper-Point would prove two points. First, space-like quantum states separated in 4D spacetime are “real,” and second, they are not physically independent. |
| 68 | See (Maudlin, 2011, pp. 21-22). |
| 69 | The ability of a Bell Quantum Hyper-Point to maintain a “quantum connection” also answers the self-interference puzzle outlined in (Gao, 2020). An electron’s Bell Quantum Hyper-Point contains all of the information regarding an electron’s charge distribution regardless of whether the electron is or is not space-like separated in 4D spacetime. The electron’s Bell Quantum Hyper-Point not only contains the charge distribution of the electron, but it also quantum discriminates and is non-attenuated. In this sense, all electrons are not the same. The electron’s Bell Quantum Hyper-point does not interfere with itself. See also (Sebens, 2021, 2022); (Wechsler S. D., 2021). |
| 70 | (Brunner et al., 2014). |
| 71 | (Goldstein et al., 2011); (Maudlin, 2014, p. 21); See also (Bell & Gao, 2016). |
| 72 | See (Maudlin, 2011, pp. 53, Note 1). |
| 73 | (Ney, 2021, p. 96). See (Allori, 2022) regarding different uses of the term “non-local” in Einstein/de Broglie Box experiments. |
| 74 | See Banerjee et al. 2016: “We conclude that the problem of time in quantum theory is intimately connected with the vexing issue of quantum non-locality and acausality in entangled states. Addressing the former compels us to revise our notions of space-time structure, which in turn provides a resolution for the latter.” |
| 75 | See also (Genovese, 2023). |
| 76 | Stated somewhat differently, Planck Space is “extra” local. |
| 77 | See also (Ney, 2021 Sections 3.7 - 3.8). |
| 78 | See also (Penrose, 1997, p. 137): “My own view is that, to understand quantum non-locality, we shall require a radical new theory. This theory will not just be a slight modification of quantum mechanics but something as different from standard quantum mechanics as General Relativity is different from Newtonian gravity. It would have to be something which has a completely different conceptual framework. In this picture, quantum non-locality would be built into the theory”. |
| 79 | (Maudlin. 2011, p. 185). |
| 80 | The mass of a body is always reference frame-dependent. See generally (Maudlin T., 2011, pp. 58-64). |
| 81 | OpenAI (2023). Background information on Special Relativity. |
| 82 | See (Bahrami, et al., 2015); (Doyle, 2014); (Lucia & Grisolia, 2022). |
| 83 | (Albert D. Z., 2000, pp. 150-162); (Doyle, 2014). |
| 84 | See generally (Price, 2004). |
| 85 | See also (Snyder, 2000) Footnote 1: “Generally, this irreversibility means that it is highly unlikely that the physical interaction that is the measurement could occur in the opposite direction of time to the one in which it is occurring or has occurred.” |
| 86 | Moreover, the Bell Identity does not support path reversibility. Instead, it simply posits that the Bell Quantum Spheres comprising the Bell Quantum Hyper-Point z1 in Planck Space are identical to those comprising Bell Quantum Field z1 on Mars and the Bell Quantum Spheres comprising Bell Quantum Hyper-Point z2 in Planck Space are identical to those comprising Bell Quantum Field z2 on Earth. |
| 87 | For a general introduction to quantum cosmology, see (Bojowald, 2015). |
| 88 | Including Type Ia Supernovae Observations, Hubble's Law and Redshift Observations, Baryon Acoustic Oscillations, Galaxy Redshift Surveys, Stellar Evolution Models, and Globular Cluster Age Estimates. |
| 89 | See generally (Davies, 1994); (Aghanim, 2018); Background source. OpenAI (2023). |
| 90 | The FLRW metric also assumes a homogeneous and isometric spatial structure. |
| 91 | “The Planck satellite data reported in 2013 … shows with high precision that we live in a remarkably simple universe. The measured spatial curvature is small; the spectrum of fluctuations is nearly scale-invariant; there is a small spectral tilt, consistent with there having been a simple dynamical mechanism that caused the smoothing and flattening; and the fluctuations are nearly Gaussian, eliminating exotic and complicated dynamical possibilities, such as inflationary models with noncanonical kinetic energy and multiple fields.” (Ijjas et al., 2013). |
| 92 | OpenAI (2023). Background on 4D spacetime’s heat death. |
| 93 | Changes may still occur at the microstate level. |
| 94 | Note that the terms “spatial curvature” and “spatial isometry” do not apply to an ultra-high dimensional Planck Space without space, volume, or distance. |
| 95 | See section 4.3 above. |
| 96 | The collapse of the Planck Energy Hyper-Point and the reduction in the number of Planck Spheres that comprise the new 4D Energy Field does not involve the destruction or localization of the Planck Spheres that comprise Planck Space, nor does it affect the SOAN. Although the energy content of the 4D Energy Field and the Planck Energy Hyper-Point are localized at t = 0, Planck Spheres and the SOAN continue forming an ultra-high dimensional (3 x N) space. |
| 97 | The collapse of the Planck Energy Hyper-Point and the generalized localization of 4D spacetime at t = 0 differs in two important respects from the collapse of a single or N-body quantum state. First, the collapse includes all of the energy content of the Planck Energy Hyper-Point, including, without limitation, dark energy. Second, the collapse generally localizes all of 4D spacetime without regard to a specific subset of Planck Spheres. |
| 98 | See (Melia, 2023) regarding the cosmological principle and the constant expansion rate of 4D spacetime. |
| 99 | The Dual Ontology conjecture also posits that the ubiquity of quantum state collapse plays a central role in the existence of the anisotropies and inhomogeneities at the last scattering of the CMB and the formation of the large-scale structure of 4D spacetime. See generally (Le´on et al., 2014); (Perez et al., 2006); (Sudarsky, 2010). |
| 100 | The theory of General Relativity regards the expansion of spacetime as an intrinsic process rather than an extrinsic expansion. Although the vast majority of the Planck Spheres that comprise all of Planck Space at t =0 are extrinsic to 4D spacetime, the existence of an ultra-high dimensional Planck Space composed of Planck Spheres does not appear to violate General Relativity. |
| 101 | See (Azhar & Loeb, 2021) regarding explanatory depth. (Adams, 2019); (Wolf & Thebault, 2022). |
| 102 | (Wald, 2005): “[T]he presently observed universe should not merely be a (highly unlikely) possibility that is allowed in the model but rather should be a prediction of the model.” |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Copyright: This open access article is published under a Creative Commons CC BY 4.0 license, which permit the free download, distribution, and reuse, provided that the author and preprint are cited in any reuse.
MDPI Initiatives
Important Links
© 2024 MDPI (Basel, Switzerland) unless otherwise stated




