Preprint
Article
The Fundamental Flaw in the Theory of Time Dilation and the Vector Nature of Light
Altmetrics
Downloads
143
Views
304
Comments
0
This version is not peer-reviewed
Submitted:
10 January 2024
Posted:
11 January 2024
You are already at the latest version
Alerts
Abstract
In Einstein’s Special Relativity(SR), time is treated as one of the four dimensions within spacetime and is relative in nature. Typically, a photon clock serves as the illustrative device in the time dilation thought experiment, featuring a single photon bouncing between two mirrors. However, the present paper identifies a fundamental flaw in this thought experiment, hence challenging its validity. Contrary to SR, this paper asserts that time is a consequence of motion. Space and time should be treated differently as proposed by classical mechanics. Importantly, this stance does not challenge the concept of curved space in General Relativity (GR), but rather questions the idea of relative time, as employed in both SR and GR. The absence of time dilation also dispels the twin paradox, prompting a necessary reassessment of the second postulate of relativity to ensure a more precise depiction of relativistic effects.
Keywords:
Subject: Physical Sciences - Theoretical Physics
1. Introduction
SR proposes that the observed rate at which time passes for an object depends on the object’s velocity relative to the observer[1]. Time dilation stems from the consistent speed of light observed in all reference frames, a principle governed by the second postulate of SR, asserting that the speed of light remains constant, irrespective of the motion of the source[1]. This further implies that the speeds of material objects and light are non-additive. This letter challenges the conventional argument that it is impossible to alter the perceived speed of light by moving towards or away from the light source. It argues for treating light as a vector, not a scalar, emphasizing the significance of considering its directional motion. While the speed of light remains constant, its velocity varies based on direction. Consequently, the observed speed of light for an object in an inertial frame of reference becomes relative, governed by the principles of vector addition and subtraction.
2. The fundamental flaw
The photon clock comprises of a single photon reflecting back and forth between two mirrors. To facilitate comprehension, let’s consider a photon clock of a reasonable height, for instance, c/2. In this scenario, the stationary observer would register two clicks of the clock, signifying the passage of 1 second. Figure 1 depicts the standard illustration used to depict the zig-zag path followed by the photon when the photon clock moves with a certain velocity with respect to a stationary observer.
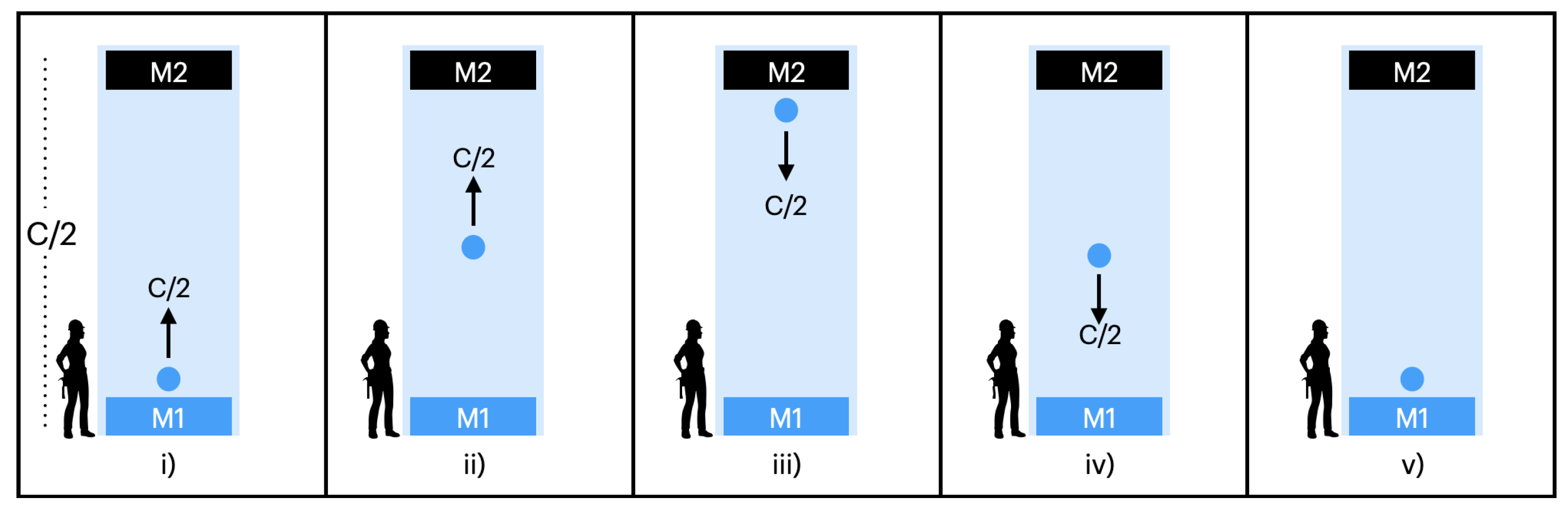
Initially, the photon keeps travelling vertically between mirrors M1 and M2. Now, envision the photon clock initiating horizontal motion, Figure 2, just before the photon reflects off mirror M1, attaining a certain speed. According to the second postulate, the horizontal motion of the photon clock doesn’t impart any additional horizontal velocity to the photon. Consequently, the photon continues to travel vertically as it was doing previously.
As the photon clock moves horizontally, covering some distance, the photon effectively departs the confines of the moving clock. Remarkably, there is no deviation from its initial vertical trajectory; it maintains a straight path without undergoing any horizontal motion. Hence, there will be no zig-zag motion at all in any scenario. This fundamental flaw of actual photon path leads to inconsistencies in the theories of time dilation and twin paradox. Figure 1 is the wrong depiction and illustration of the path taken by the photon leading to inconsistencies and paradoxes.
2.1. Redesigning the photon clock for inertial frame
Let us redesign the photon clock by replacing the mirror M1 with a one-way mirror as shown in Figure 3. In this photon clock, there is a photon emitter which is angled at 30 degrees with respect to horizontal.
2.2. Photon path observed by stationary observer
Let us first analyse the path of photon, as observed by a stationary observer, when the photon clock is moving horizontally at a velocity /2c as shown in Figure 4. At this speed, the photon will be contained inside the photon clock. As clearly observed in Figure 4, the stationary observer would observe two ticks amounting to a total time elapse of 2 seconds.
It is important to note here that we will be able to contain the photon in the moving photon clock only when the mentioned initial conditions are exactly met. If the photon clock moves at a speed other than /2c, the photon will leave its confinement within the photon clock.
2.3. Photon path observed in photon clock’s frame of reference
Since the photon has been emitted at an angle of 30 degrees and the photon clock is moving horizontally at a speed of /2c, the photon will be contained inside the moving photon clock. For the observer in this photon clock’s frame of reference, the observed speed of the photon will be c/2. Since the height of the clock is c/2, the total time elapsed for two ticks will be 2 seconds.
Figure 5.
’Contained photon’ positions at various instances of time
3. The Vector nature of light
3.1. Velocity of light for stationary observers
Figure 6 shows 3 different scenarios for two stationary observers S1 & S2 where S1 sends a photon in various directions. These 3 cases are discussed below :
- A: S1 sends photon towards the observer S2 in the horizontal direction. The speed of photon observed by S2 will be ’c’ and the photon will be detected by S2.
- B: S1 sends photon horizontally away from the observer S2 in the opposite direction. The speed of photon observed by S2 will be ’-c’, hence the photon will never be detected by S2.
- C: S1 sends photon in all directions except towards S2. The speed of photon observed by S2 will be of various values in vector form and the photon will never be detected by S2.
Though, even when both the observers are stationary, the speed of light is still ’c’ but based upon the direction the observed speed of light can change for stationary observer.
3.2. Velocity of light for observer in inertial frame
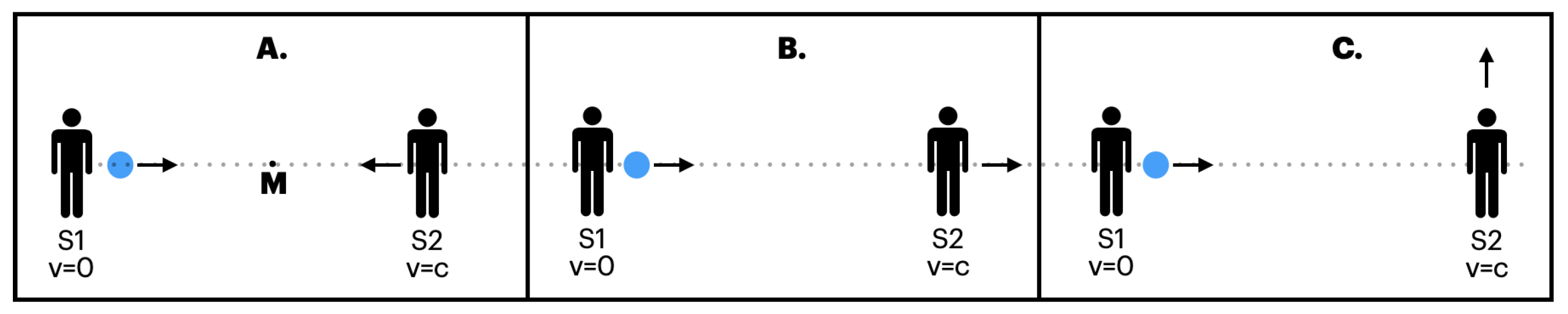
Figure 7 shows 3 different scenarios for a stationary observer S1 & a moving observer S2. S1 sends a photon in the horizontal direction towards S2 while S2 moves at the speed of ’c’ albeit in different directions as shown. These 3 cases are discussed below :
- A: S1 sends photon horizontally towards the observer S2. Since S2 is moving towards S1 at the speed of ’c’, S2 will exactly meet the photon at the midpoint M and S2 will be able to detect the photon. The speed of light in the frame of reference of S2 would be ’2c’.
- B: S1 sends photon horizontally towards the observer S2. Since S2 is moving away from the S1 at the speed of ’c’, the photon will never reach S2. The observed speed of photon in the frame of reference of S2 will be 0.
- C: S1 sends photon horizontally towards the observer S2. Since S2 is moving vertically at the speed of ’c’, the photon will never reach S2. The observed speed of photon in the frame of reference of S2 will be 0 since the velocity of photon has no vertical component.
It can be observed that the speed of light is not always constant in inertial frames of reference and is dependent on the direction of photon travel.
4. Conclusions
- The speed of light c is a constant, independent of the relative motion of the source and the speed of material objects and light are not additive. But the observed speed of light can certainly change for an observer who is either stationary or in inertial frame.
- Light should be treated as a vector
- There is no time dilation and hence no twin paradox
- Space and time are not intertwined. They are fundamentally separate and time is a consequence of motion.
References
- Einstein, Albert. "On the Electrodynamics of Moving Bodies." Annalen der Physik, vol. 17, no. 10, 1905, pp. 891-921.
Figure 1.
Zig-zag path based on standard model
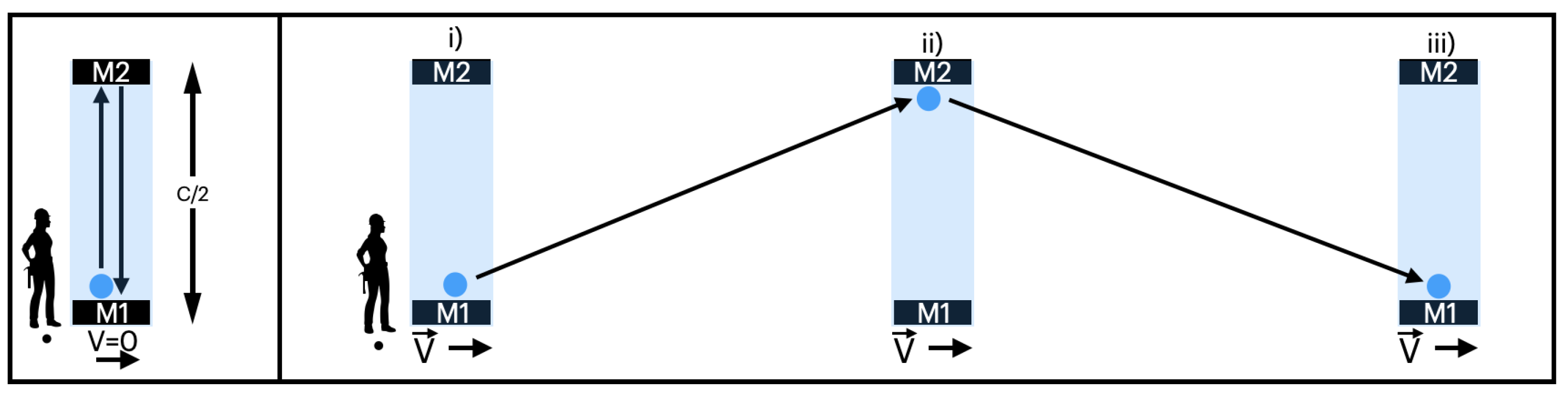
Figure 2.
Actual path of photon at various instances of time

Figure 3.
Modified Photon Clock
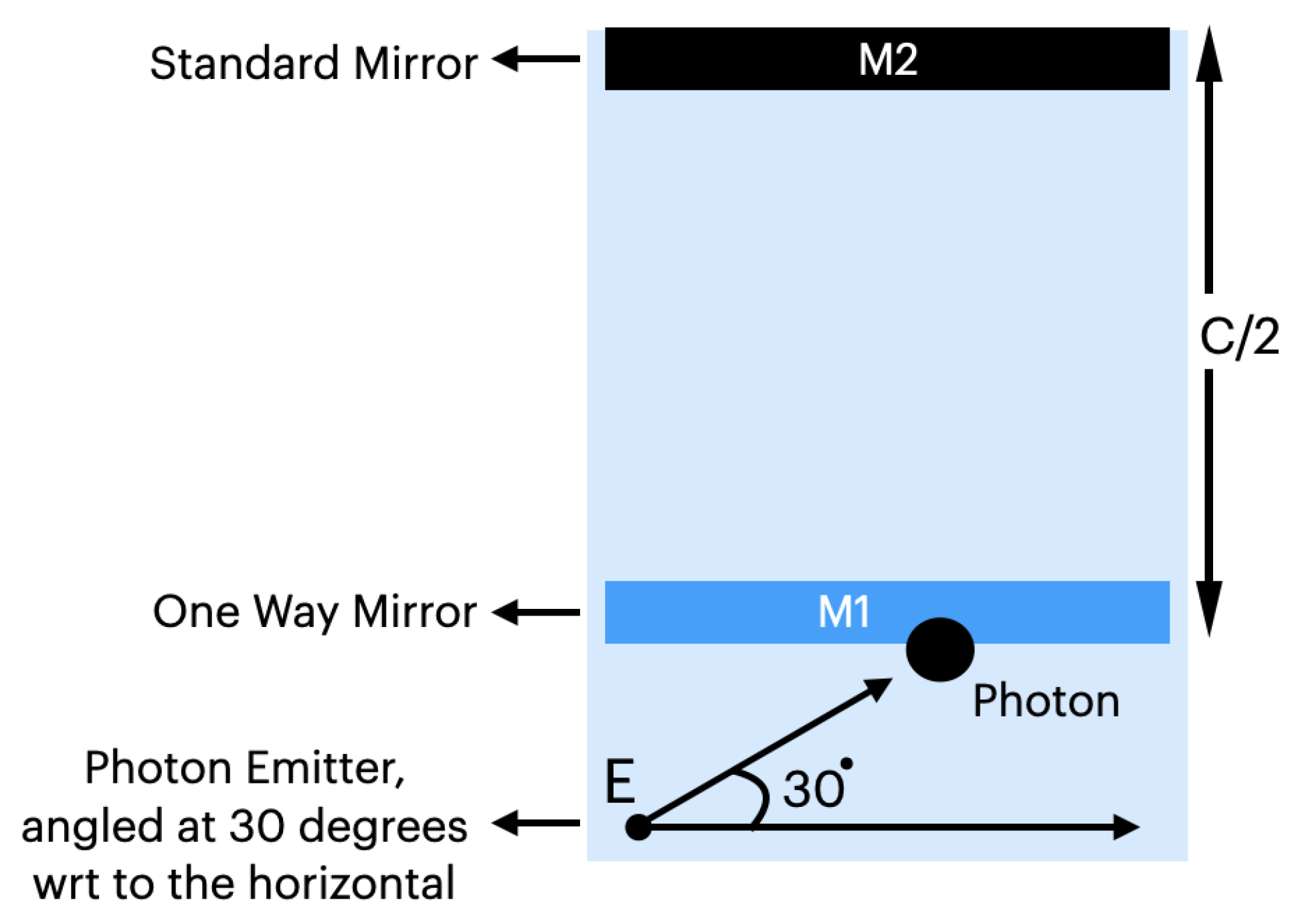
Figure 4.
Zig-zag path observed by the stationary observer
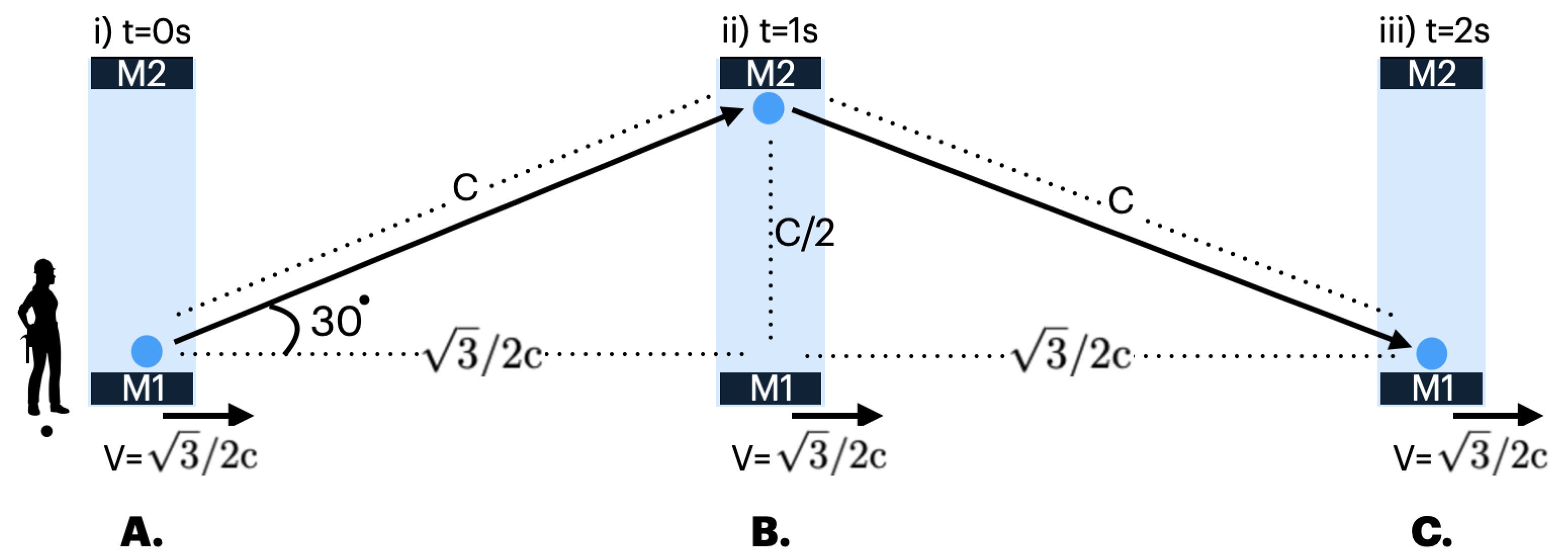
Figure 6.
3 Instances

Figure 7.
3 Instances
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Copyright: This open access article is published under a Creative Commons CC BY 4.0 license, which permit the free download, distribution, and reuse, provided that the author and preprint are cited in any reuse.
MDPI Initiatives
Important Links
© 2024 MDPI (Basel, Switzerland) unless otherwise stated




