Preprint
Article
Critical Pseudo-Double Impulse Analysis Evaluating Seismic Energy Input to Reinforced Concrete Buildings with Steel Damper Columns
This version is not peer-reviewed.
Submitted:
12 January 2024
Posted:
16 January 2024
You are already at the latest version
A peer-reviewed article of this preprint also exists.
Abstract
Steel damper columns (SDCs) are energy-dissipating members that are suitable for reinforced concrete (RC) buildings and are often used for multistory housing. The evaluation of the peak deformation and hysteretic dissipated energy of such building structures is essential for the rational seismic design of RC buildings with SDCs. In a previous study, the authors proposed an energy-based prediction procedure for the peak and cumulative response of an RC frame building with SDCs. In this procedure, the accuracy of the equivalent velocity of the maximum momentary input energy (VΔE1*)–peak equivalent displacement (D1*max) relationship is essential for high quality predictions. In this article, the VΔE1*–D1*max relationships of RC moment-resisting frames with and without SDCs are invested using a critical pseudo-double impulse (PDI) analysis based on a study by Takewaki and coauthors. The results show that the VΔE1*–D1*max relationship obtained from the critical PDI analysis agrees well with that calculated from the equations proposed in the previous study.
Keywords:
reinforced concrete building
; steel damper column (SDC)
; pseudo double impulse (PDI)
; energy input
; pushover analysis
1. Introduction
1.1. Background and Motivation
A dual system with sacrificial members that absorb the seismic energy prior to the beams and columns, e.g., a damage-tolerant structure (Wada et al., 2000), is one solution for creating structures with superior seismic performance. Unlike traditional earthquake-resistant structures, beams and columns in such dual systems are damage free (or have limited damage) after large earthquakes because most of the seismic energy input is absorbed by the sacrifice members. Therefore, buildings with such dual systems are more resilient than those without sacrificial members.
Steel damper columns (SDCs; Katayama et al., 2000) are energy-dissipating sacrificial members that are suitable for reinforced concrete (RC) buildings and are often used for multistory housing. The purpose of SDCs is to mitigate damage to beams and columns during strong seismic events. The author’s research group has been studying the seismic rehabilitation of existing RC buildings using SDCs (Fujii and Miyagawa, 2018; Fujii et al., 2019) and the seismic design of new RC moment-resisting frames (MRFs) with SDCs (Mukouyama et al., 2021).
The peak deformation and cumulative strain energy are essential parameters in assessing the seismic performance of structural members. Specifically, the peak deformation is an essential parameter for RC members dominated by flexural behavior, as long as the story drift does not exceed 2.0 % (Elwood et al., 2021). Meanwhile, both the peak deformation and the cumulative strain energy are important for the steel damper panels within SDCs. In a previous paper, an energy-based prediction procedure for the peak and cumulative responses of an RC MRF building with SDCs was proposed (Fujii and Shioda, 2023). In this procedure, the building model is converted to an equivalent single-degree-of-freedom (SDOF) model that represents the first modal response. Then, two energy-related seismic intensity parameters are considered, namely, the maximum momentary input energy (Hori and Inoue, 2002) and the total input energy (Akiyama, 1985). The peak displacement is predicted by considering the energy balance during a half cycle of the structural response using the maximum momentary input energy. Meanwhile, the energy dissipation demand of the dampers is predicted considering the energy balance during an entire response cycle using the total input energy.
This procedure has been verified by comparing nonlinear time-history analysis (NTHA) results using non-pulse-like artificial ground motions (Fujii and Shioda, 2023) and 30 recorded pulse-like ground motions (Fujii, 2023). However, the following issues remain.
- In the presented procedure (Fujii and Shioda, 2023), the accuracy of the equivalent velocity of the maximum momentary input energy of the first modal response ()–equivalent displacement of the first modal response () relationship is essential for high quality predictions of the peak displacement. Accordingly, a monotonic pushover analysis was performed to evaluate the – relationship. However, the strain hardening effect observed in low-yield steel shear panels subjected to cyclic loading (Nakashima, 1995) cannot be considered in a monotonic pushover analysis.
- For the prediction of the peak equivalent displacement () and cumulative input energy of the first modal response, the equivalent velocities of the maximum momentary input energy () and the total input energy () are predicted from the linear elastic spectrum (the and spectra, respectively). In the presented procedure (Fujii and Shioda, 2023), the effective period of the first modal response () calculated from the predicted – relationship is used for the predictions of and . Although the accuracies of the predicted and values have been examined by comparing the predicted results with the NTHA results, the accuracy of has not yet been examined. The response period of the first modal response (), which is defined as twice ( where is the interval of a half cycle of the structural response), is a good index for evaluating in NTHA results. However, the value of obtained from the NTHA results is unstable because of the complexity of the characteristics of ground motions and the influence of the higher modal responses of a structure.
The relationship between the energy and the peak deformation has been studied by several researchers. There are two main approaches: the first approach is to define a parameter that relates the cumulative input energy (or cumulative strain energy) and the peak deformation and the second approach is to define an energy-based seismic intensity parameter that is directly related to the peak deformation. Akiyama (1988) stated that the cumulative inelastic deformation ratio should be assumed to be 4 times the inelastic deformation ratio for the seismic design of structures with elastic–perfectly plastic behavior, such as ductile steel MRFs. Then, the equivalent number of cycles can be formulated as the ratio of the cumulative inelastic deformation to the peak inelastic deformation in the simplified energy-based seismic design method (Akiyama, 1999). Manfredi et al. (2003) investigated the relationship between the equivalent number of plastic cycles and the seismological parameters in the near field based on 128 near-fault and 122 far-fault records. They concluded that “the relative importance of the cyclic damage for structures grows at the higher distance from the fault, whereas in the near-source conditions structural response is governed by the peak demand, confirming the damage observations after destructive earthquakes.” Mota-Páez et al. (2021) noted that, for the seismic retrofit design of an RC soft-story building with a hysteresis damper under near-fault earthquakes, the equivalent number of cycles should be reduced. This is because, in the case of a near-fault earthquake, a large amount of seismic energy input occurs within a few cycles. Within the first approach, Fajfar (1992) proposed another dimensionless parameter normalizing the cumulative hysteresis (strain) energy by the peak deformation. This parameter has been applied to the pushover-based damage analysis method of RC MRFs (Gaspersic et al, 1992; Fajfar and Gaspersic, 1996) and the seismic design procedure of new RC MRFs (Teran-Gilmore, 1998). Decanini et al. (2000) studied the relationship between the cumulative input energy and the peak displacement of RC MRFs subjected to near-source earthquakes; they concluded that a reliable relationship between the cumulative input energy and the peak displacement can be constructed, using either the cumulative hysteretic energy or the cumulative input energy. Mollaioli et al. (2011) analyzed the correlations between the energy and the peak displacement for linear and nonlinear SDOF and multi-degree-of-freedom (MDOF) models; they concluded that the degree of correlation between the energy and the displacement quantities is noticeably more stable when the cumulative input energy is considered, rather than the cumulative hysteresis energy. Following these studies, Angelucci et al. (2023b) studied the relationship between the cumulative input energy and the peak displacement of RC MRFs with infills. Meanwhile, Benavent-Climent (Benavent-Climent et al., 2004; Benavent-Climent, 2011) proposed an energy-based assessment method for existing buildings; they focused on the strain energy under the monotonic loading of stories until the ultimate state, instead of the ultimate story drift.
Inoue and his research group (Hori et al., 2000, Inoue et al., 2000, Hori and Inoue 2002) proposed the maximum momentary input energy as an energy-based seismic intensity parameter that is directly related to the peak displacement of RC structures. Note that a similar energy-based seismic intensity parameter was proposed by Kalkan and Kunnath (2007). The present authors formulated the time-varying function of the momentary energy input of an elastic SDOF mode using Fourier series (Fujii et al, 2019). Then, the concept of the momentary input energy was extended to bidirectional horizontal excitation (Fujii and Murakami, 2021; Fujii 2021). In addition, Fajfar’s parameter was re-formulated using the maximum momentary input energy and the total input energy for RC structures (Fujii, 2021). Similarly, for base-isolated structures with hysteresis dampers, Akiyama’s equivalent number of cycles was reformulated using the maximum momentary input energy and the total input energy (Fujii, 2023). Angelucci et al. (2023a) studied the relationship between the energy-related seismic intensity parameters proposed by Kalkan and Kunnath (2007) and the peak displacement of bare RC MRFs.
The above-discussed studies are based on NTHA results using recorded ground motions. Conversely, Takewaki and his research group (Kojima et al., 2015; Kojima and Takewaki, 2015a, 2015b, and 2015c; Akehashi and Takewaki, 2021; Akehashi and Takewaki, 2022) studied simplifying the seismic input as a series of impulsive forces. First, Kojima et al. (2015) introduced the concept of the “critical double impulse input,” which represents the upper bound of the earthquake energy input for a given pulse velocity (). Next, Kojima and Takewaki introduced the double impulse input as a substitute for the fling-step near-fault ground motion (2015a). Following this study, they introduced the triple impulse input as a substitute for the forward-directivity near-fault ground motion (2015b) and the multiple impulse input as a substitute for long-duration earthquake ground motion (2015c). Then, Akehashi and Takewaki introduced pseudo-double impulse (PDI) (2021) and pseudo-multi impulse (PMI) (2022) analyses. In PDI and PMI analyses, the MDOF model oscillates predominantly in the single mode, considering the impulsive lateral force corresponding to a certain mode vector; when the impulsive lateral force corresponding to the first mode vector is considered, the MDOF model oscillates predominantly in the first mode.
The author believes that PDI is suitable to discuss the above two issues for the following reasons: (i) the momentary input energy can easily be calculated as the energy input because of the acting pseudo lateral force and (ii) the interval of a half cycle of the structural response () can easily be evaluated and is expected to be stable because the MDOF model oscillates predominantly in a single mode.
1.2. Objectives
Given the above-outlined background, this study addresses the following questions.
- (i)
- What is the – relationship when considering the response of an RC MRF with SDCs subjected to critical PDI input? Does it agree with the predicted – relationship from the simplified equation proposed in the author’s previous study (Fujii and Shioda, 2023)?
- (ii)
- What is the relationship between the response period () and the effective period () calculated from and in the case of an RC MRF with SDCs subjected to critical PDI input?
In this study, critical PDI analyses of RC MRF models are performed. These critical PDI analyses are based on studies by Akehashi and Takewaki (2021) with one modification: in this study, the change in the first mode shape in the nonlinear range is considered to maintain consistency with the assumptions applied in the procedure (Fujii and Shioda, 2023). Six 8- and 16-story RC MRFs with and without SDCs are analyzed considering various intensities of the pulse velocity . Then, the predicted – and – relationships calculated according to the procedure (Fujii and Shioda, 2023) are compared with those obtained from the critical PDI analysis results.
The rest of this paper is organized as follows. Section 2 outlines the critical PDI analysis based on Akehashi and Takewaki (2021). Section 3 presents the six RC MRFs with and without SDCs and the analysis methods. Section 4 describes the responses of the six RC MRFs obtained from the critical PDI analysis results. Section 5 discusses the comparisons with the predicted results based on the author’s previous study (Fujii and Shioda, 2023) and the critical PDI analysis results, focusing particularly on (i) the – relationship and (ii) the – relationship. The conclusions drawn from this study and the directions of future research are discussed in Section 6.
2. Critical PDI Analysis
2.1. Outline of the Critical PDI Analysis
Figure 1 outlines the critical PDI analysis. This analysis is based on the studies by Akehashi and Takewaki (2021, 2022), and one modification is made to maintain consistency with the assumptions applied in the procedure (Fujii and Shioda, 2023): in this study, the change in the first mode vector () in the nonlinear range is considered for the calculation of the first modal response at time and the second pseudo impulsive lateral force.
Consider a planer frame building model (number of stories, ) subjected to a pseudo impulsive lateral force proportional to the first mode vector (pulse velocity: ). Here, is the mass matrix of the building model; , , and are the relative displacement, velocity, and acceleration vectors, respectively; and and are the restoring force and damping force vectors, respectively. The equivalent displacement (), equivalent velocity (), and equivalent relative acceleration () of the first modal response are defined as
where is the effective first modal mass. Note that and depend on the local maximum equivalent displacement within the range . In this study, the first mode vector at time is updated assuming that is proportional to the displacemt vector at the time when the maximum equivalent displacement occurs (). The first mode vector at time is updated such that
The equivalent acceleration is defined as
2.1.1. First pseudo impulsive lateral force
At time , the first pseudo impulsive lateral force acts on the building model, as shown in Figure 1. Note that, before the first pseudo impulsive force acts on the building model (), the building model is in the stationary state (, , ). The equivalent velocity of the first modal response just after the first pseudo impulsive lateral force acts () is calculated such that
and the corresponding velocity vector () is
where is the first mode vector at the initial stage. The increment of the input energy of the first modal response () is calculated such that
where is the first modal mass at the initial stage. The cumulative input energy of the first modal response () is
To calculate the response following the action of the first pseudo impulsive lateral force, the equivalent velocity () and the velocity vector () are updated such that
2.1.2. Free vibration after the first pseudo impulsive lateral force
Following the action of the first pseudo impulsive lateral force, the building model oscillates without external forces (free vibration) until the arrival of the second pseudo impulsive lateral force. The kinetic energy, damping dissipated energy, cumulative strain energy, and cumulative input energy of the first modal response (, , , and , respectively) are expressed as
Because the first pseudo impulsive lateral force is proportional to the first mode vector, the building model oscillates predominantly in the first mode. Therefore, the kinetic energy, damping dissipated energy, cumulative strain energy, and cumulative input energy (, , , and , respectively) are approximated as
Note that the first mode vector () updates any step according to Equation (5) until reaches its local peak ( shown in Figure 1) and that the effective first modal mass () is re-calculated according to Equation (4). The time is defined as the time when reaches .
The timing of the second second pseudo impulsive lateral force () is determined from the following condition:
This condition (Equation (17)) is equivalent to the condition of critical timing given by Akehashi and Takewaki (2021, 2022).
2.1.3. Second pseudo impulsive lateral force
At time , the second pseudo impulsive lateral force acts on the building model, as shown in Figure 1. The equivalent velocity of the first modal response just after the arrival of the second pseudo impulsive lateral force () is calculated such that
Here, is the equivalent velocity of the first modal response just before the action of the second pseudo impulsive lateral force. Assuming that the velocity vector just prior to the action of the second pseudo impulsive lateral force () can be approximated by the first modal response, the corresponding velocity vector () can be expressed as
The increment of the input energy of the first modal response () is calculated such that
Note that Equation (17) is obtained by differentiating Equation (20) with respect to and equating it to zero. Therefore, the timing of the arrival of the second pseudo impulsive lateral force is the timing that maximizes .
The cumulative input energy of the first modal response immediately following the action of the second pseudo impulsive lateral force () is calculated such that
To calculate the response following the action of the second pseudo impulsive lateral force, the equivalent velocity () and the velocity vector () are updated such that
2.1.4. Free vibration after the second pseudo impulsive lateral force
Following the action of the second pseudo impulsive lateral force, the building model oscillates without external forces (free vibration) until .
The time is defined as the time when reaches its local peak (). The peak equivalent displacement of the first modal response over the course of the entire seismic event () is
2.2. Momentary Input Energy in the Critical PDI Analysis
Consider the energy response of the equivalent SDOF model representing the first modal response subjected to the ground acceleration (). The cumulative input energy of the first modal response per unit mass over the course of the entire seismic event () is calculated from the time derivative of the equivalent displacement () and the ground acceleration () such that
According to Hori and Inoue (2002), the momentary input energy of the first modal response per unit mass () is calculated such that
In Equation (25), and are the beginning and ending times of a half cycle of the structural response, respectively. The maximum momentary input energy per unit mass () is defined as the maximum value of over the course of the entire seismic event.
Following the study by Kojima and Takewaki (2015a), the ground acceleration () in the case of a critical DI analysis can be written as
In Equation (26), is the Dirac delta function, which satisfies
Next, the momentary input energy of the first modal response per unit mass at the first and
second half cycles and respectively) are calculated from Equations
(25) and (26). Assuming that the intervals of the first and second half cycles of the structural response
are and , respectively, as shown in Figure 1,
and can be calculated such that
Note that, in Equation (28), the interval of integration is changed from to to calculate the integrals that contain the Dirac delta fuction. To calculate Equations (28) and (29), the equivalent velocities () at times and are rewritten as
Therefore, and can be calculated such that
The calculated and shown in Equations (32) and (33) are consistent with the above-shown increments of the energy input (Equations (9) and (20), respectively). This implies that, in the case of a critical PDI analysis, the momentary input energy is calculated as the increment of the energy input as a result of the pseudo impulsive lateral force.
The maximum momentary input energy of the first modal response per unit mass () is obtained such that
The cumulative input energy of the first modal response per unit mass () is calculated such that
The equivalent velocity of the maximum momentary input energy of the first modal response () is calculated such that
Similarly, the equivalent velocity of the cumulative input energy of the first modal response () is calculated such that
In this study, the response period of the first modal response () is defined as twice the interval between the two local peaks ( and ) such that
2.3. Analysis Flow
Figure 2 shows the flow of the critical PDI analysis. In this flow, the damping force increment resulting from the velocity vector changing at analysis step () is treated as the unbalanced force to be corrected in the next step. In addition, the timing of the second pseudo impulsive lateral force is determined by checking the sign of the equivalent relative acceleration (). The timing of the second pseudo impulsive lateral force is determined according the following condition:
When Equation (39) is satisfied, the second pseudo impulsive lateral force acts.
The analysis procedure was implemented in the computer code used in the previous analysis (Fujii and Miyagawa, 2018).
3. Analysis Data and Methods
3.1. Building Data
The six planar building models analyzed in this study are 8- and 16-story RC MRFs with and without SDCs. Figure 3 shows the simplified plans and elevations of the RC MRF building models. The two models labeled Type B are the same as those used in the previous study (Fujii and Shioda, 2023). Meanwhile, the two models made from the Type B models by removing all SDCs are referred to as Type O. The models referred to as Type A were made from the Type B models by reducing the number of SDCs. All RC MRFs analyzed herein were designed according to the strong-column/weak-beam concept, except for the foundation level beam and in the case of SDCs installed in an RC frame. In the latter case, at the joints between an RC beam and a steel damper column, the RC beam was designed to be sufficiently stronger than the yield strength of the steel damper column considering strain hardening. Sufficient shear reinforcement of all RC members was provided to prevent premature shear failure. The failure of the beam–column joints was not considered because it was assumed that sufficient reinforcement was provided. The natural periods of the first modal response in the elastic range () of the 8-story models are 0.740 s, 0.627 s, and 0.561 s for the Type O, A, and B models, respectively. Similarly, the values of the 16-story models are 1.41 s, 1.21 s, and 1.12 s for the Type O, A, and B models, respectively.
The nonlinear behavior of the RC members and SDCs was modeled as in previous studies (Mukoyama et al., 2021; Fujii, 2022; Fujii and Shioda, 2023), except for the hysteresis rule used for the SDCs. Figure 4 shows the hysteresis rule. The same hysteresis model (stiffness degradation model) was used for the flexural springs in the RC members. Meanwhile, for the damper panel in the SDCs, two hysteresis models were considered to investigate the influence of strain hardening on the energy response. The first model was the normal bilinear model (LB); its yield strength is set to the initial yielding strength of the damper panel (), and the strain hardening effect is neglected. The second model was the trilinear model used in previous studies (Mukoyama et al., 2021; Fujii, 2022; Fujii and Shioda, 2023) with the strain-hardening effect (SH); its upper bound strength () was set such that the ratio equaled 300/205 = 1.46. Other details concerning the six structural models can be found in previous studies (Fujii, 2022; Fujii and Shioda, 2023). In this study, the viscous damping ratio of the first modal response of the RC MRFs in the elastic range () was set to 0.03.
Figure 5 shows the equivalent acceleration–equivalent displacement relationships of the six models. In this study, a displacement-based mode-adaptive pushover (DB-MAP) analysis (Fujii, 2014) was applied to obtain the relationship between the equivalent acceleration (whole building: , RC MRF: , and SDCs: ) and the equivalent displacement (). The pushover analyses were performed until the equivalent displacement () reached 1/75 of the assumed equivalent height (): the assumed values were 18.9 m and 35.9 m for the 8- and 16-story models, respectively. The target equivalent displacement for the pushover analysis was 0.252 m for the 8-story model, while that for the 16-story model was 0.479 m. Note that, in the pushover analyses of the Type A and B models, the influence of the strain hardening of the SDCs was neglected. The idealized – curves for the Type O models and the idealized – and – curves for the Type A and B models are shown in Figure 5. The values of and at the “yield point” ( for RCF and for SDC) are also shown. The bilinear idealization of each curve was performed following the methods shown in Fujii (2022).
The following observations can be drawn from Figure 5.
- The “yield displacement” of RCF () in Type A and B models is smaller than that in Type O models. The equivalent drift ratio at the “yielding of RCF” () of the Type O 8-story model is 1/170, while those of the Type A and B models are 1/196 and 1/205, respectively. Similarly, the ratio of the Type O 16-story model is 1/187, while those of the Type A and B models are 1/215 and 1/218, respectively. This is because of the shortening of the beam span resulting from the presence of the SDCs.
- The “yield acceleration” of RCF () in the Type A and B models is nearly the same as that in the Type O models. In case of the 8-story models, the values of the Type O, A, and B models are 2.561 m/s2, 2.567 m/s2, and 2.596 m/s2, respectively. Similarly, in the case of the 16-story models, the values of the Type O, A, and B models are 1.288 m/s2, 1.304 m/s2, and 1.326 m/s2, respectively.
- The “yield acceleration” of SDC () in the Type B models is approximately twice of that in the Type A models. The ratio of the “yield acceleration” of SDC to that of RCF () of the Type A 8-story model is 0.234, while that of the Type B model is 0.458. Similarly, the ratio of the Type A 16-story model is 0.242, while that of the Type B model is 0.471.
- The ratio of the “yield displacement” of SDC to that of RCF () of the 8-story models is smaller than that of the 16-story models. For the 8-story models, the ratios of the Type A and B models are 0.563 and 0.598, respectively. Meanwhile for the 16-story models, the ratios of the Type A and B models are 0.731 and 0.780, respectively.
Note that the ratio influences the effectiveness of the SDCs with respect to seismic energy absorption. This is discussed in the analysis results.
3.2. Analysis Method
In this study, the pulse velocity () was set from 0.10 m/s, with an interval of 0.05 m/s, until was close to 1/75 of the assumed equivalent height (). In each analysis, the ending time of the analysis () was determined as the ending of the 32nd half cycle of free vibration following the action of the second pseudo impulsive lateral force.
4. Analysis Results
In this section, the responses of the building models subjected to a pseudo impulsive lateral force proportional to the first mode vector are compared and discussed. For the Type A and B models, only the results considering strain hardening are shown here.
4.1. Response of the Overall Building Model
Figure 6 compares the relationships between the seismic intensity parameters (, , and ) and the peak equivalent displacement ().
The following conclusions can be drawn from Figure 6.
- The seismic intensity parameters (, , and ) increase as increases. The – and – curves are very similar to the – curve of the same model: the ratio ranges from 1.8 to 2.0, while the ratio ranges from 1.5 to 1.7. The differences in the and ratios between models are very small.
- For the same value of , the values of the Type B models are the smallest while those of the Type O models are the largest. Comparing the values of the 8-story models considering the case where = 0.55 m/s, the value for the Type O models is 0.252 m, while those for the Type A and B models are 0.190 m (−24.6 %) and 0.162 m (−35.7 %), respectively. Similarly, comparing the values of the 16-story models considering the case where = 0.55 m/s, the value for the Type O models is 0.487 m, while those for the Type A and B models are 0.377 m (−22.6 %) and 0.325 m (−33.3 %), respectively.
Figure 7 compares the ratios of the cumulative energy at the end of the simulation (), i.e., the ratios of the kinetic energy (), damping dissipated energy (), cumulative strain energy of the RC MRF (), and cumulative strain energy of the SDCs (). In this figure, the vertical dotted lines indicate the “yield displacement” of the SDCs () and the RC MRF ().
The following conclusions can be drawn from Figure 7.
- In all cases, the ratio of the kinetic energy () is close to zero.
- For the Type O 8-story model, the ratio is close to 0.8 when is smaller than 0.111 m (= ). Meanwhile, the ratio decreases and the ratio increases as increases when is larger than 0.111 m. When is 0.251 m, is 0.148 while is 0.852. Because no SDCs are installed in the Type O models, the ratio is zero.
- For the Type A 8-story model, the ratio increases as increases when is larger than 0.054 m (= ). The ratio increases as increases when is larger than 0.096 m (= ). Meanwhile, the ratio decreases as increases. When is 0.254 m, is 0.097 while is 0.553 and is 0.350.
- For the Type B 8-story model, similar observations can be made as for the Type A 8-story model. When is 0.250 m, is 0.084 while is 0.447 and is 0.467.
- For the Type O 16-story model, similar observations can be made as for the Type O 8-story model. When is 0.487 m, is 0.129 while is 0.871. Because no SDCs are installed in the Type O models, the ratio is zero.
- For the Type A 16-story model, the ratio increases as increases when is larger than 0.122 m (= ). The ratio increases as increases when is between 0.167 m (= ) and 0.332 m. However, the ratio is nearly constant when is larger than 0.332 m. When is 0.492 m, is 0.091 while is 0.600 and is 0.310.
- For the Type B 16-story model, similar observations can be made as for the Type A 16-story model. When is 0.480 m, is 0.084 while is 0.499 and is 0.417.
The differences in the ratios for the 8- and 16-story models can be explained by the diffrences in the ratios shown in Figure 5. As shown in Figure 5, the ratios in the 8-story models are 0.563 (Type A) and 0.598 (Type B), while those in the 16-story models are 0.731 (Type A) and 0.780 (Type B). Therefore, the effectiveness of the SDCs in 8-story models is better than that in 16-story models because the ratio is smaller for the 8-story models than for the 16-story models.
Figure 8 shows the hysteresis loops of the first modal response (the – relationship) for each model. In this figure, the points at which the first and second pseudo impulsive lateral forces act ( and , respectively), and the points of the local peak responses ( and , respectively) are shown. The – curves obtained from the pushover analysis results (as in Figure 5) are also shown Figure 8.
The following conclusions can be drawn from Figure 8.
- In all models, larger equivalent displacements occur in the positive direction: is larger than . This means that the peak equivalent displacement of the first modal response over the course of the entire seismic event () occurs at the end of the second half cycle of the response, where the momentary energy input occurs.
- For the Type O models (both 8- and 16-story), the – curves obtained from the pushover analysis results (black dotted curve) agree very well with the hysteresis loops obtained via the critical PDI analyses: the points at the local peak response ( and ) are on the – curves obtained from the pushover analysis results.
- For the Type A and B models (both 8- and 16-story), the – curves obtained from the pushover analyses are slightly different from the hysteresis loops obtained via the critical PDI analyses: the points at the second local peak response () are above the – curve obtained from the pushover analysis results. For the 8-story models, the ratios of the values obtained from the critical PDI analysis and the pushover analysis at the point are 1.086 for the Type A model and 1.141 for the Type B models. Similary, for the 16-story models, the ratios of the values from the critical PDI analysis and the pushover analysis are 1.083 for the Type A model and 1.149 for the Type B model.
Figure 9 shows the ratio of the energy input () to the local peak equivalent displacement (). Here, the ratio is defined as
The ratio is 1/3 if the structure exhibits undamped linear elastic behavior. Meanhile, the ratio is 1 if the structure exhibits rigid–perfectly plastic behavior, that is, no strain energy is released after the first local peak () occurs.
The ratio is defined as
The ratio is 0.5 if the structure exhibitis undamped linear elastic behavior. Meanwhile, the ratio is 1 if the structural response is symmetric in the positive and negative directions.
The following conclusions can be drawn from Figure 9.
- In all models, the ratio is nearly constant: most of the plots are distributed within a narrow range between 1/3 and 0.4.
- In all models, the ratio is nearly constant: most of the plots are distributed within a narrow range between 0.4 and 0.5.
Figure 10 shows the response periods of the first modal response () and the ratios of the effective period of the first modal response () and the response period (). Here, is defined as in Fujii and Shioda (2023) as
In Equation (42), is the complex damping ratio of the equivalent linear system. Here, is set to 0.10.
The following conclusions can be drawn from Figure 10.
- In all models, increases as increases.
- When comparing in the case of similar , of the Type O models is the largest while that of the Type B models is the smallest. This means that becomes smaller as the number of SDCs increases.
- In all models, the ratio is nearly constant: all of the plots are distributed within a narrow range between 1.0 and 1.2.
4.2. Local Response
Figure 11 compares the peak responses of all model types for = 0.55 m/s. The following local response quantities are compared: (i) the peak relative displacement; (ii) the peak story drift; (iii) the peak plastic rotation at the beam end (); and (iv) the peak shear strain of the damper panel (). Note that, because the span length is different due to the presence of SDCs, for the beam end at the right of column X2 is shown for the Type O and B models; meanwhile, for the beam end at the left of column X2 is shown for the Type A models. The plot of for the Type O models is not shown because no SDCs were installed in this model type.
The following conclusions can be drawn from Figure 11.
- For both the 8- and 16-story models, the responses of the Type O models are the largest, while those of the Type B models are the smallest.
- For the 8-story models, the largest peak story drift is observed at the third floor level. The largest is observed at the third or forth floor levels. The largest is observed at the forth floor level.
- For the 16-story models, the largest peak story drift is observed at the sixth or seventh floor levels. The largest is observed at the seventh floor level. The largest is observed at the seventh floor level.
Figure 12 compares the normalized cumulative strain energies of all the model types for = 0.55 m/s. The following local response quantities are compared: (i) the normalized cumulative strain energy at the beam end () and (ii) the normalized cumulative strain energy of the damper panel (). Here, is defined as
In Equation (43), and are the yield moment and the chord rotation, respectively, at the yielding of the th beam end and is the cumulative strain energy of the th beam end.
is defined as
In Equation (44), and are the initial yield shear force and the initial yielding shear strain, respectively, of the th damper panel; is the height of the th damper panel; and is the cumulative strain energy of the th damper panel.
The following conclusions can be drawn from Figure 12.
- For both 8- and 16-story models, the values of the Type O models are the largest, while those of the Type B models are the smallest. The largest values are observed at the forth floor level in the 8-story models and at the seventh floor level in the 16-story models.
- For both the 8- and 16-story models, the values of the Type A models are larger than those of the Type B models. In the 8-story models, the largest is observed at the forth floor level in the Type A models, while it is observed at the first floor level in the Type B models. In the 16-story models, the largest is observed at the seventh floor level in both model types.
4.3. Summary of the Analysis Results
This section summarizes the responses of the RC frame building models with and without SDCs subjected to a pseudo impulsive lateral force proportional to the first mode vector. The analysis results can be summarized as follows.
- A)
- In the critical PDI analysis results shown herein, the peak equivalent displacement of the first modal response over the course of the entire seismic event () occurs at the end of the second half cycle of the response, when the second pseudo impulsive lateral force acts. The momentary input energy corresponding to the second pseudo impulsive lateral force () is larger than that corresponding to the first pseudo impulsive lateral force ().
- B)
- The equivalent acceleration ()–equivalent displacement () curve obtained from the pushover analysis results agrees very well with the hysteresis loop (the – relationship) obtained by the critical PDI analysis in the case of the Type O models. Meanwhile, in the case of the models with SDCs, the – curve obtained from the pushover analysis is slightly different from the hysteresis loop obtained by the critical PDI analysis. This is due to the strain hardening effect of the damper panel.
- C)
- The ratio of the the effective period of the first modal response (), calculated from Equation (42), to the response period of the first modal response (), , is nearly constant: the ratio is within a narrow range between 1.0 and 1.2.
Point (A) is important for discussing the relationship between the maximum momentary input energy () and the peak displacement (). In the prediction procedure for the peak displacement of Hori and Inoue (2002), as well as that of Fujii and Shioda (2023), the peak displacement is calculated considering the energy balance during the half cycle of the structural response: it is assumed that the peak displacement () occurs at the end of the response, when the maximum momentary energy input occurs. Therefore, point (A) is consistent with the assumption of the prediction procedure.
Point (B) indicates that the – curve obtained from the pushover analysis results agrees well with the critical PDI analysis results, as far as the model without the strain hardening effect is concerned. This implies that, for the bare RC MRF studied herein, the – curve constructed from the critical PDI analysis results of various by plotting the peak response point will be the same as the the – curve obtained from the pushover analysis.
Point (C) indicates that calculated from and the equivalent velocity of the maximum momentary input energy of the first modal response () via Equation (42) is clearly related to the response period (). This may indicate that Equation (42) is valid for calculating when evaluating and from the and spectra as discussed in previous studies (Fujii and Shioda, 2023; Fujii 2023).
5. Comparisons with the Predicted Results
This section focuses on comparisons with the predicted results based on the study of Fujii and Shioda (2023) and the critical PDI analysis results, particularly (i) the – relationship and (ii) the – relationship. Details concerning calculating and from the pushover analysis results can be found in Fujii and Shioda (2023).
5.1. Bare RC Frame Models
Figure 13 shows comparisons between the predicted results and the critical PDI analysis results for the Type O models. The upper two panels show comparisons of the predicted – curve and the – plots obtained from the critical PDI results, while the lower two panels show comparisons of the predicted – curve and the – plots obtained from the critical PDI results.
The following conclusions can be drawn from Figure 13.
- The predicted – curves agree well with the – plots obtained from the critical PDI results. More specifically, the predicted – curve of the 8-story model agrees very well with the critical PDI results when is larger than 0.111 m (= ). Similarly, the predicted – curve of the 16-story model agrees very well with the critical PDI results when is larger than 0.192 m.
- The predicted – curves are above the – plots obtained from the critical PDI results, although its trend is similar. The predicted – curves show a gradual increase in as increases, which is consistent with the – plots. However, the difference between and becomes significant when is small.
5.2. RC Frame Models with SDCs
Figure 14 shows comparisons between the predicted results and the critical PDI analysis results for the Type A and B models. Similar to Figure 13, the upper two panels show comparisons of the predicted – curves with the – plots obtained from the critical PDI results, while the lower two panels show comparisons of the predicted – curves with the – plots obtained from the critical PDI results. In this figure, the critical PDI results obtained by the models without the strain hardening effect (LB) and with the strain hardening effect (SH) are shown for comparison.
The following conclusions can be drawn from Figure 14.
- As far as the – and – plots are concerned, the differences between the LB (without strain hardening) and SH (with strain hardening) plots are very small in the critical PDI analysis results shown herein.
- The predicted – curves agree well with the – plots obtained from the critical PDI results for all models shown herein.
- The predicted – curves are above the – plots obtained from the critical PDI results, and the difference between the predicted – curves and the – plots decreases as increases.
5.3. Discussion
This section compares the predicted results based on the study of Fujii and Shioda (2023) with the critical PDI analysis results, focusing on (i) comparisons between the predicted – curves and the – plots obtained from the critical PDI results and (ii) comparisons between the predicted – curves and the – plots. Based on these comparisons, the following conclusions can be drawn.
- A)
- The predicted – curves agree well with the – plots obtained from the critical PDI results for all models shown herein.
- B)
- The predicted – curves are slightly above the – plots obtained from the critical PDI results. Although their trends are similar, the predicted – curves show a gradual increase in as increases, which is consistent with the – plots.
- C)
- The influence of the strain hardening of the damper panels on the – and – plots of the RC MRF models with SDCs is negligibly small.
Conclusion (A) indicates that the accuracy of the predicted – curves is satisfactory, as far as the bare RC MRF models and RC MRF models with SDCs are concerned. However, the difference between the predicted and is not small when is small, as evident in conclusion (B). The predicted can be closer to if the idealization of the – curve is made via a tri-linear curve. Conclusion (C) indicates that the strain hardening effect of the damper panel can be neglected in calculations of the – curve.
6. Conclusions
In this study, critical PDI analyses of six RC MRF models with and without SDCs were performed. Then, the predicted – and – relationships calculated according to Fujii and Shioda (2023) were compared with those obtained from the critical PDI analysis results. The main results and conclusions can be summarized as follows.
- The equivalent acceleration ()–equivalent displacement () curves obtained from the pushover analysis results agree very well with the hysteresis loops (– relationship) obtained by the critical PDI analysis in the case of models without SDCs. In the case of models with SDCs, the – curve obtained from the pushover analysis differs slightly from the hysteresis loop obtained by the critical PDI analysis.
- The effective period of the first modal response (), calculated from the equation of the peak equivalent displacement () and the equivalent velocity of the maximum momentary input energy (), is clearly related to the response period (); the ratio is within a narrow range between 1.0 and 1.2.
- The predicted – curves agree well with the – plots obtained from the critical PDI results for all models shown herein. Meanwhile, the predicted – curves are slightly above the – plots obtained from the critical PDI results. Although their trends are similar, the predicted – curves show a gradual increase in as increases, which is consistent with the – plots.
- The influence of the strain hardening of the damper panels on the – and – plots of the RC MRF models with SDCs is negligibly small.
The above conclusions support the accuracy of the prediction procedure (Fujii and Shioda, 2023): the predicted – curves are sufficiently accurate for RC MRFs with and without SDCs. In addition, considering the unavoidable scatter in evaluating and from the linear spectrum, the accuracy of the predicted – curves may be acceptable.
Another finding of interest is that the – curve can be directly evaluated from the critical PDI (or PMI) analysis in the case of buildings with a significant cyclic loading effect. In the DB-MAP analysis, there are several limitations imposed to avoid instability problems in the numerical analysis, e.g, the envelope of the force–deformation relationship of members must be symmetric, and a severe strength degredation (severe negative slope) in the force–deformation relationship of brittle members may cause numerical stability. In addition, because the DB-MAP analysis is a monotonic loading analysis, the influence of the cyclic loading effect cannot be directly included. Meanwhile, the critical PDI analysis shown herein has no such limitations; a critical PDI analysis of a structural model can be performed as long as the structural model is stable for NTHA. The flow of the critical PDI analysis shown herein can easily be extended to a critical PMI analysis. Therefore, the influence of the number of cyclic loadings on the – curve can easily be evaluated by increasing the number of impulsive inputs in the critical PMI analysis. Therefore, critical PDI analyses have great potential for seismic performance evaluations of structures.
Note that the results shown in this study are, so far, valid only for RC MRF models with and without SDCs. Therefore, apart from further verifications using additional building models, the following questions remain unanswered. This list of questions is not comprehensive.
- What is the dependence of the – curve on the number of impulsive inputs? It is expected that the ratio of the amplitude of in the positive and negative directions changes as the number of impulsive inputs increases. Therefore, the relationship between the increment of the energy input in each half cycle and the local peak equivalent displacement should vary depending on the number of impulsive inputs.
- Can the distribution of the cumulative strain energy of the SDCs in the critical PDI analysis at each floor level be properly evaluated from the pushover analysis results? Because the pushover analysis cannot consider the strain hardening effect, the distribution of the deformation of the SDCs may be different from the critical PDI analysis results. It is expected that the influence of the strain hardening effect on the distribution of the cumulative strain energy of the SDCs may be significant when the number of impulsive inputs increases.
Author Contributions
The author confirms that they are the sole contributor to this work and approve its publication.
Funding
This study received financial support from JSPS KAKENHI Grant Number JP23K41046.
Data Availability Statement
The raw data presented in this study are available from the corresponding author upon request.
Acknowledgments
The original frame model data used in this study were provided by Momoka Shioda, who is a former graduate student of the Chiba Institute of Technology. This work was supported by JSPS KAKENHI Grant Number JP23K41046. We thank Martha Evonuk, PhD, from Edanz (https://jp.edanz.com/ac), for editing a draft of this manuscript.
Conflict of Interest
The author has no conflicts of interest to declare.
Abbreviations
DB-MAP = displacement-based mode-adaptive pushover
MDOF = multi-degree-of-freedom
MRF = moment-resisting frame
NTHA = nonlinear time-history analysis
PDI = pseudo double impulse
RC = reinforced concrete
SDC = steel damper column
SDOF = single-degree-of-freedom
References
- Angelucci, G., Quaranta, G., Mollaioli, F., Kunnath, SK. (2023a). “Correlation between seismic energy demand and damage potential under pulse-like ground motions”, In: Varum, H., Benavent-Climent, A., Mollaioli, F. (eds) Energy-Based Seismic Engineering. IWEBSE 2023. Lecture Notes in Civil Engineering, vol 236. Springer, Cham.
- Angelucci, G., Mollaioli, F., Quaranta, G. (2023b). Correlation between energy and displacement demands for infilled reinforced concrete frames. Frontiers in Built Environment. 9, 1198478. [CrossRef]
- Akehashi, H., Takewaki, I. (2021). Pseudo-double impulse for simulating critical response of elastic-plastic MDOF model under near-fault earthquake ground motion. Soil Dynamics and Earthquake Engineering. 150, 106887. [CrossRef]
- Akehashi, H., Takewaki, I. (2022). Pseudo-multi impulse for simulating critical response of elastic-plastic high-rise buildings under long-duration, long-period ground motion. The Structural Design of Tall and Special Buildings. 31(14), e1969. [CrossRef]
- Akiyama, H. (1985). Earthquake Resistant Limit-State Design for Buildings. Tokyo: University of Tokyo Press.
- Akiyama, H. (August 1988). “Earthquake resistant design based on the energy concept”, in Proceedings of the 9th World Conference on Earthquake Engineering, Tokyo-Kyoto, Japan.
- Akiyama, H. (1999). Earthquake-Resistant Design Method for Buildings Based on Energy Balance. Tokyo: Gihodo Shuppan.
- Benavent-Climent, A., Akiyama, H., López-Almansa, F., Pujades, LG. (2004). Prediction of ultimate earthquake resistance of gravity-load designed RC buildings. Engineering Structures. 26. 1103-1103. [CrossRef]
- Benavent-Climent, A. (2011). A seismic index method for vulnerability assessment of existing frames: application to RC structures with wide beams in Spain. Bulletin of Earthquake Engineering, 9. 491-517. [CrossRef]
- Decanini, L., Mollaioli, F., Saragoni, R. (January 2000). “Energy and displacement demands imposed by near-source ground motions,” in Proceedings of the 12th World Conference on Earthquake Engineering, Auckland, New Zealand.
- Elwood, K.J.; Sarrafzadeh, M.; Pujol, S.; Liel, A.; Murray, P.; Shah, P.; Brooke, N.J. (April 2021). “Impact of prior shaking on earthquake response and repair requirements for structures—Studies from ATC-145,” in Proceedings of the NZSEE 2021 Annual Conference, Christchurch, New Zealand.
- Fajfar, P. (1992). Equivalent ductility factors, taking into account low-cycle fatigue. Earthquake Engineering and Structural Dynamics. 21, 837–848. [CrossRef]
- Fajfar, P., Gaspersic, P. (1996). The N2 method for the seismic damage analysis of RC buildings. Earthquake Engineering and Structural Dynamics.25, 31-46. [CrossRef]
- Fujii, K. (2014). Prediction of the largest peak nonlinear seismic response of asymmetric buildings under bi-directional excitation using pushover analyses. Bulletin of Earthquake Engineering. 12, 909–938. [CrossRef]
- Fujii, K., Miyagawa, K. (June 2018). “Nonlinear seismic response of a seven-story steel reinforced concrete condominium retrofitted with low-yield-strength-steel damper columns” in Proceedings of the 16th European Conference on Earthquake Engineering (Thessaloniki).
- Fujii, K., Sugiyama, H., Miyagawa, K. (2019). Predicting the peak seismic response of a retrofitted nine-storey steel reinforced concrete building with steel damper columns. Earthquake Resistant Engineering Structures XII, WIT Transactions on The Built Environment. 185, PII75–85.
- Fujii, K., Kanno, H., and Nishida, T. (2019). Formulation of the time-varying function of momentary energy input to a SDOF system by Fourier series. Journal of Japan Association for Earthquake Engineering. 19, 247–266. [CrossRef]
- Fujii, K., Murakami, Y. (September 2021). “Bidirectional momentary energy input to a one-mass two-DOF system. In Proceedings of the 17th World Conference on Earthquake Engineering,” Sendai, Japan.
- Fujii, K. (2021). Bidirectional seismic energy input to an isotropic nonlinear one-mass two-degree-of-freedom system. Buildings. 11, 143. [CrossRef]
- Fujii, K. (2022). Peak and cumulative response of reinforced concrete frames with steel damper columns under seismic sequences. Buildings. 12, 275. [CrossRef]
- Fujii, K., and Shioda, M. (2023). Energy-based prediction of the peak and cumulative response of a reinforced concrete building with steel damper columns. Buildings. 13, 401. [CrossRef]
- Fujii, K. (2023), Energy-based response prediction of reinforced concrete buildings with steel damper columns under pulse-like ground motions. Frontiers in Built Environment. 9, 1219740. [CrossRef]
- Fujii, K. (July 2023). “Equivalent Number of Cycles Formulation for a Base-Isolated Building,” In: Varum, H., Benavent-Climent, A., Mollaioli, F. (eds) Energy-Based Seismic Engineering. IWEBSE 2023. Lecture Notes in Civil Engineering, vol 236. Springer, Cham.
- Gaspersic, P.; Fajfar, P.; Fischinger, M. (July 1992). “An approximate method for seismic damage analysis of buildings,” in Proceedings of the 10th World Conference on Earthquake Engineering, Madrid, Spain.
- Hori, N., Iwasaki, T., Inoue, N. (January 2000). “Damaging properties of ground motions and response behavior of structures based on momentary energy response,” in Proceedings of the 12th World Conference on Earthquake Engineering, Auckland, New Zealand.
- Hori, N., Inoue, N. (2002). Damaging properties of ground motion and prediction of maximum response of structures based on momentary energy input. Earthquake Engineering and Structural Dynamics. 31, 1657–1679. [CrossRef]
- Inoue, N., Wenliuhan, H., Kanno, H., Hori, N., Ogawa, J. (2000). “Shaking table tests of reinforced concrete columns subjected to simulated input motions with different time durations, “ in Proceedings of the 12th World Conference on Earthquake Engineering, Auckland, New Zealand.
- Kalkan, E., Kunnath, SK. (2007). Effective Cyclic Energy as a Measure of Seismic Demand, Journal of Earthquake Engineering, 11(5), 725-751. [CrossRef]
- Katayama, T., Ito, S., Kamura, H., Ueki, T., Okamoto, H. (January 2000). “Experimental study on hysteretic damper with low yield strength steel under dynamic loading,” in Proceedings of the 12th World Conference on Earthquake Engineering, Auckland, New Zealand.
- Kojima, K., Fujita, K., Takewaki, I. (2015). Critical double impulse input and bound of earthquake input energy to building structure. Frontiers in Built Environment. 1, 5. [CrossRef]
- Kojima, K., Takewaki, I. (2015a). Critical earthquake response of elastic–plastic structures under near-fault ground motions (Part 1: Fling-step input). Frontiers in Built Environment. 1, 12. [CrossRef]
- Kojima, K., Takewaki, I. (2015b). Critical earthquake response of elastic–plastic structures under near-fault ground motions (Part 2: Forward-directivity input). Frontiers in Built Environment. 1, 13. [CrossRef]
- Kojima, K., Takewaki, I. (2015c). Critical input and response of elastic–plastic structures under long-duration earthquake ground motions. Frontiers in Built Environment. 1, 15. [CrossRef]
- Manfredi, G., M., Cosenza, E. (2003). Cumulative demand of the earthquake ground motions in the near source. Earthquake Engineering and Structural Dynamics. 32, 1853–1865. [CrossRef]
- Nakashima, M. (1995). Strain-hardening behaviour of shear panels made of low-yield steel 1: test. Journal of Structural Engineering, ASCE. 121(12), 1742-1749. [CrossRef]
- Mollaioli, F., Bruno, S., Decanini, L., Saragoni, R. (2011). Correlations between energy and displacement demands for performance-based seismic engineering. Pure and Applied Geophysics. 168, 237-259. [CrossRef]
- Mota-Páez, S., Escolano-Margarit, D., Benavent-Climent, A. (2021). Seismic response of RC frames with a soft first story retrofitted with hysteretic dampers under near-fault earthquakes, Applied Sciences. 2021, 11, 1290. [CrossRef]
- Mukoyama, R., K. Fujii, K., Irie, C., Tobari, R., Yoshinaga, M., K. Miyagawa, K. (October 2021). “Displacement-controlled Seismic Design Method of Reinforced Concrete Frame with Steel Damper Column,” in Proceedings of the 17th World Conference on Earthquake Engineering, Sendai, Japan.
- Teran-Gilmore, A. (1998). A parametric approach to performance-based numerical seismic design. Earthquake Spectra. 14(3), 501-520. [CrossRef]
- Wada, A., Huang, YH., Iwata, M. (2000). Passive damping technology for buildings in Japan. Progress in Structural Engineering and Materials. 2(3), 335-350. [CrossRef]
Figure 1.
Outline of the critical pseudo-double impulse (PDI) analysis.

Figure 2.
Flow of the critical PDI analysis.

Figure 3.
Simplified structural plans and elevations of the reinforced concrete (RC) moment-resisting frame (MRF) building models.
Figure 3.
Simplified structural plans and elevations of the reinforced concrete (RC) moment-resisting frame (MRF) building models.
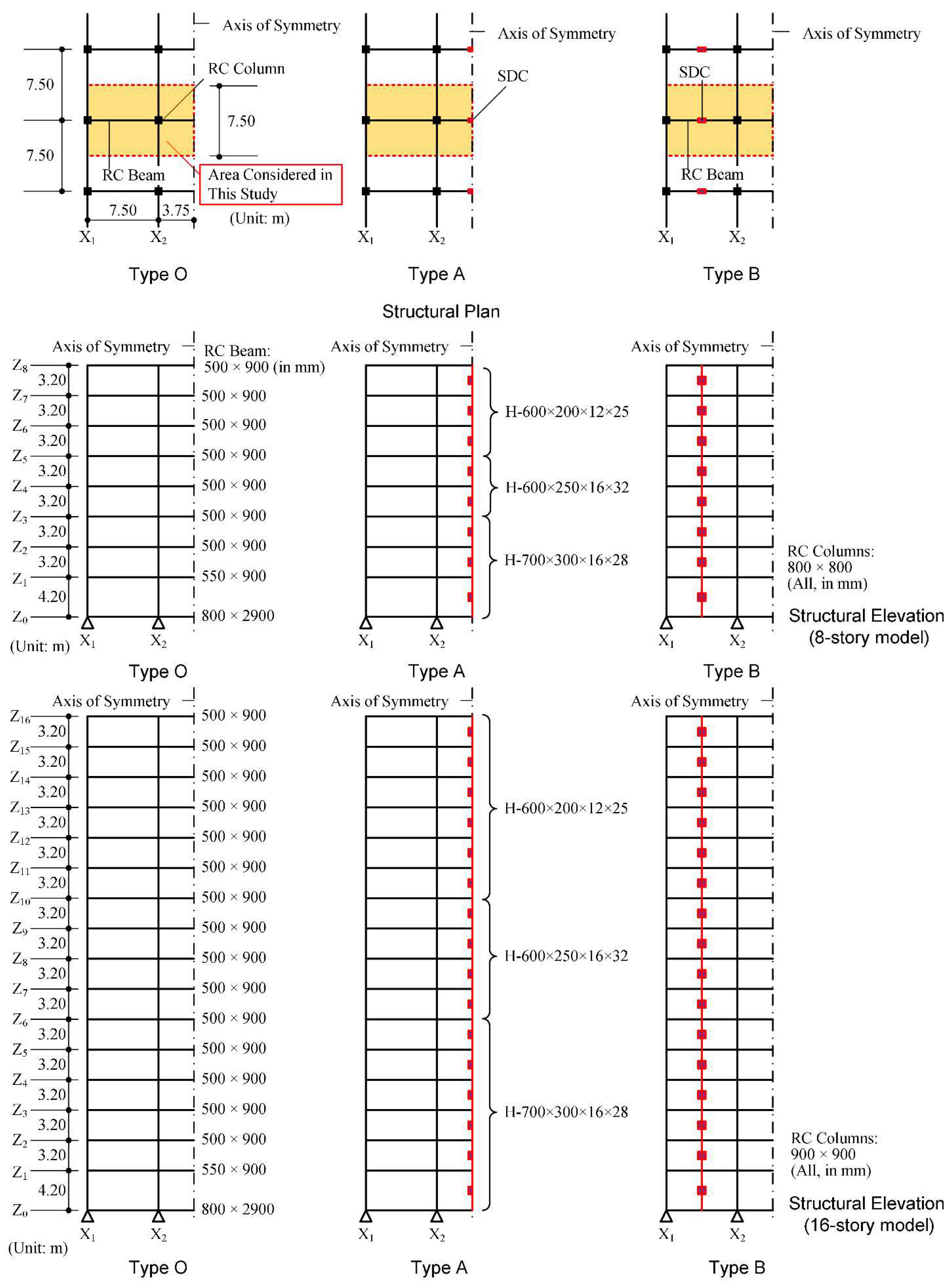
Figure 4.
Hysteresis model for RC members and steel damper columns (SDCs).

Figure 5.
Equivalent acceleration–equivalent displacement relationships of the building models.

Figure 6.
Relationship between the seismic intensity parameters and the peak equivalent displacement.
Figure 6.
Relationship between the seismic intensity parameters and the peak equivalent displacement.

Figure 7.
Ratios of the cumulative energy at the end of the simulation.

Figure 8.
Hysteresis loop of the first modal response.
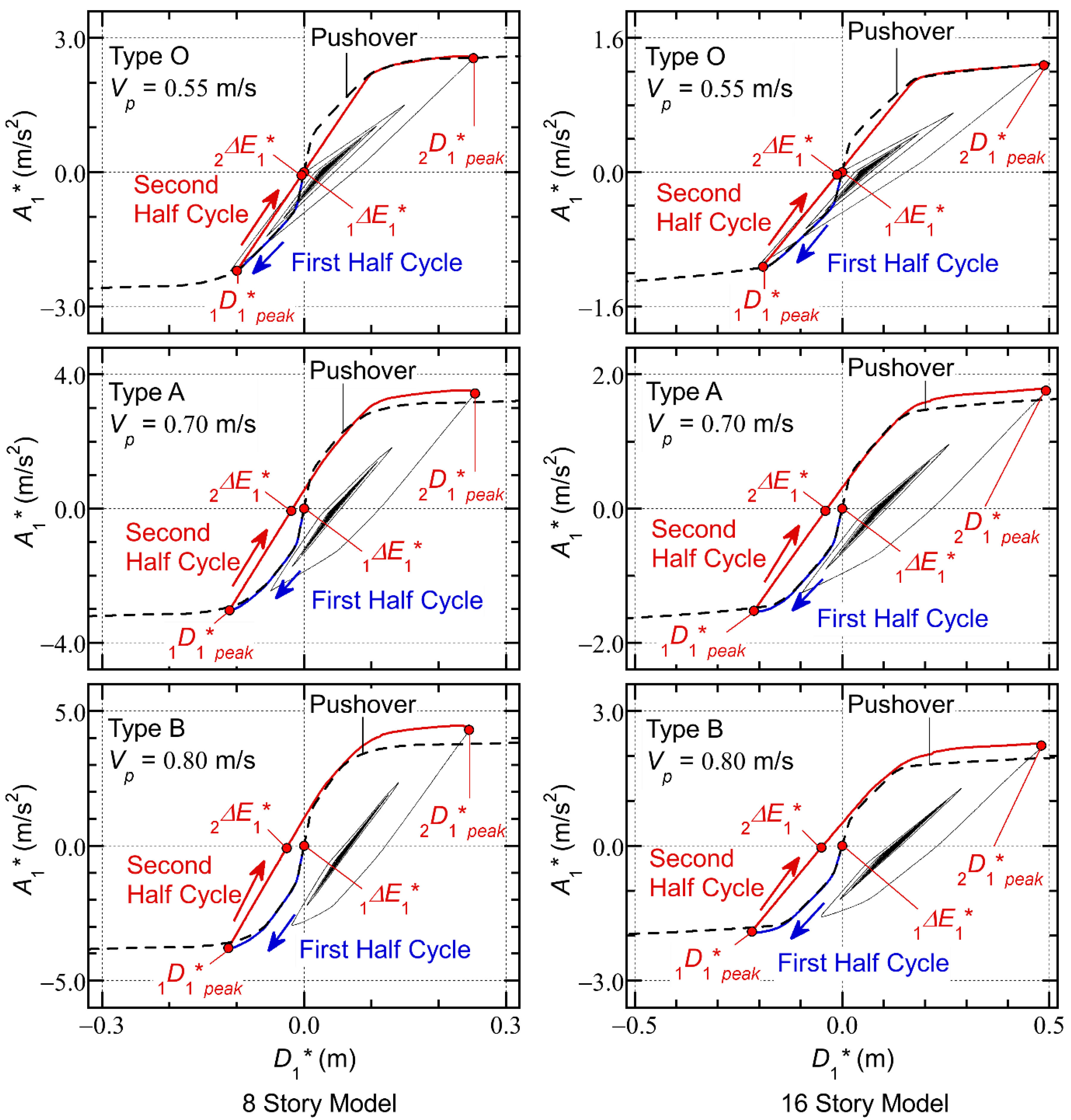
Figure 9.
Input energy ratio (ηE) and local peak equivalent displacement ratio (ηD).
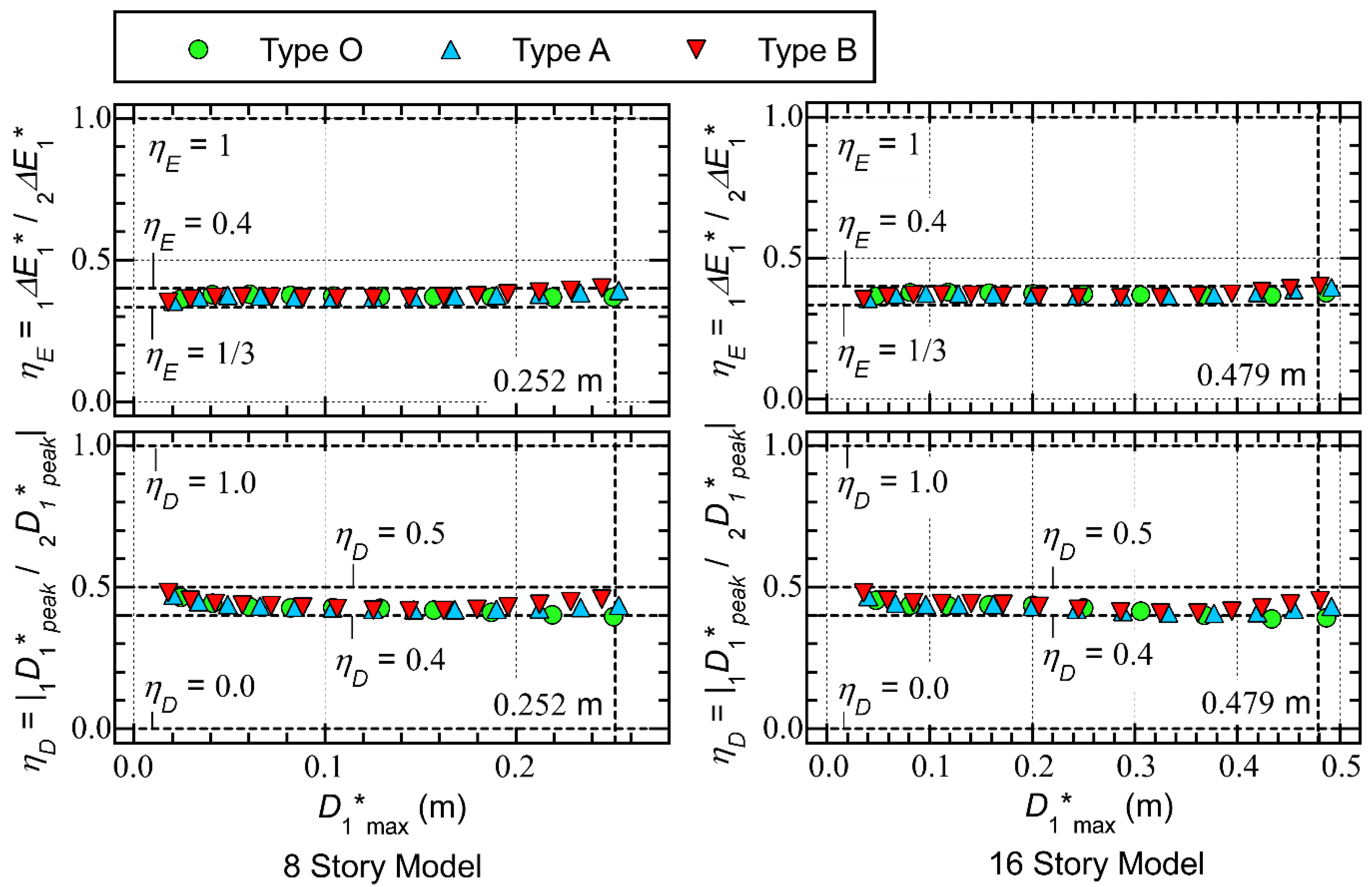
Figure 10.
Response period (T1res).

Figure 11.
Comparisons of the peak response (Vp = 0.55 m/s).
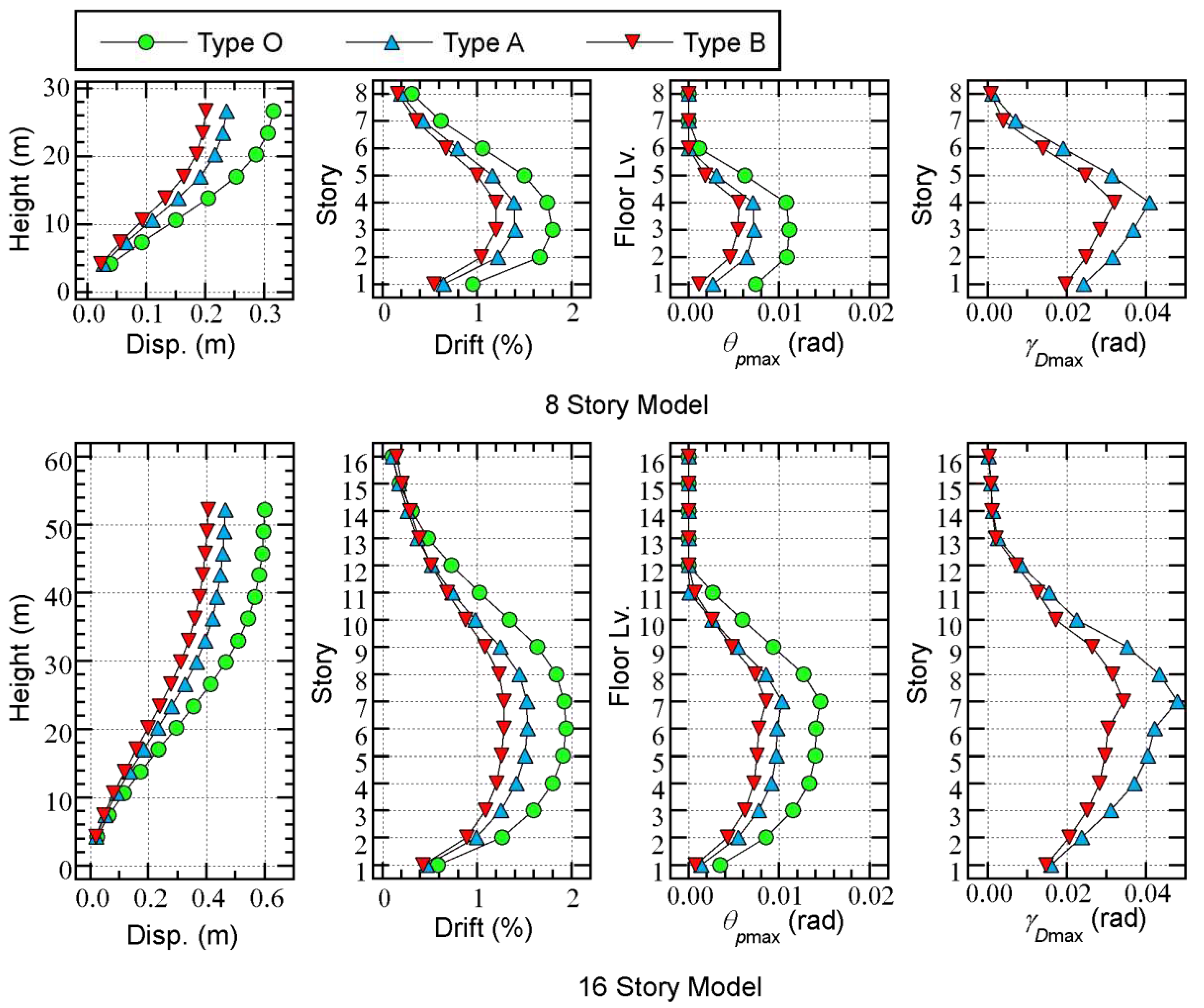
Figure 12.
Comparisons of the normalized cumulative strain energy (Vp = 0.55 m/s).
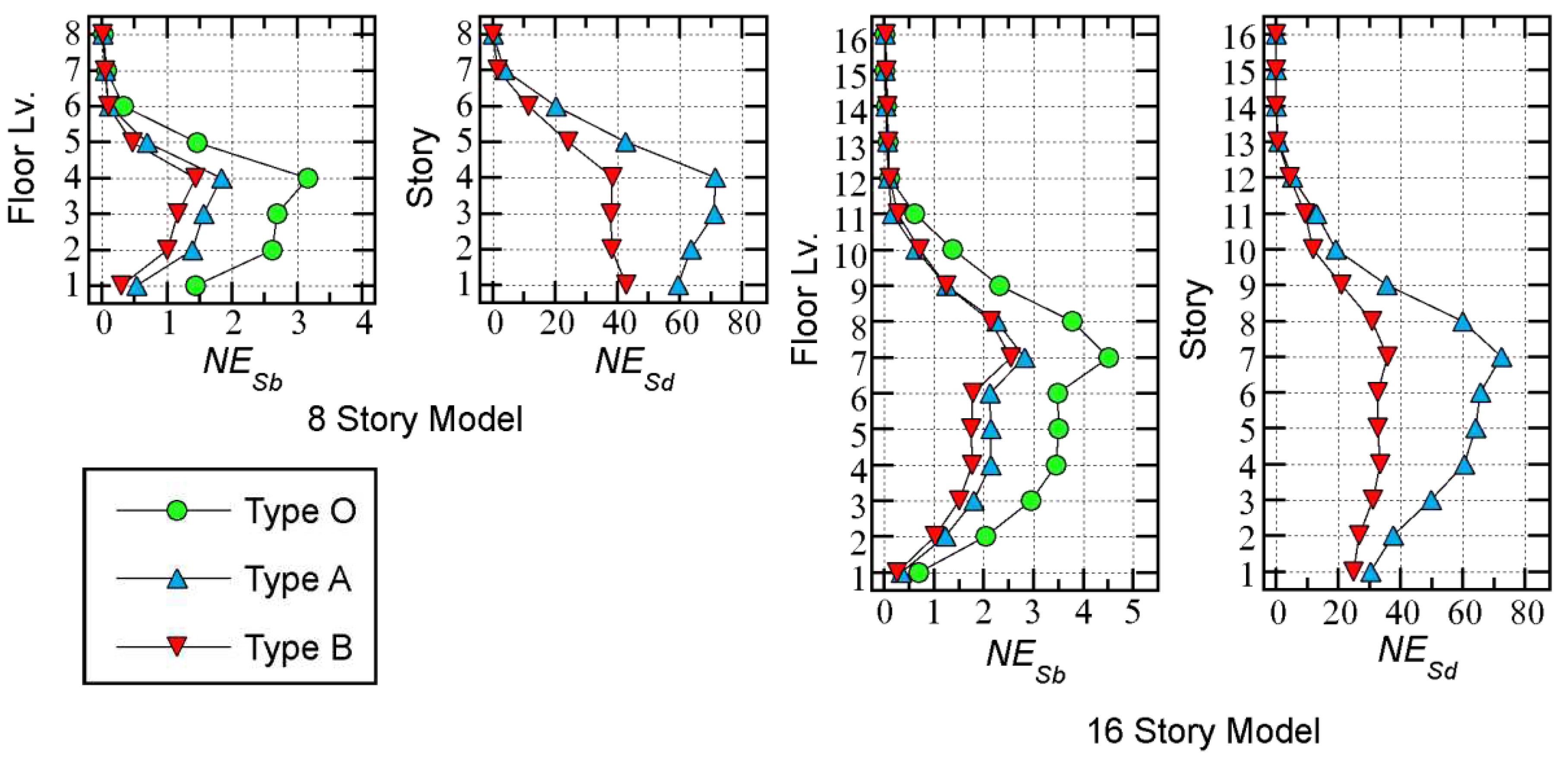
Figure 13.
Comparisons of the predicted results with the critical PDI analysis results (Type O).

Figure 14.
Comparisons of the predicted results with the critical PDI analysis results (Types A and B).
Figure 14.
Comparisons of the predicted results with the critical PDI analysis results (Types A and B).

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Copyright: This open access article is published under a Creative Commons CC BY 4.0 license, which permit the free download, distribution, and reuse, provided that the author and preprint are cited in any reuse.
Downloads
98
Views
41
Comments
0
Subscription
Notify me about updates to this article or when a peer-reviewed version is published.
MDPI Initiatives
Important Links
© 2025 MDPI (Basel, Switzerland) unless otherwise stated





