An Efficient Analytical Approaches to Investigate Nonlinear Two-Dimensional Time-Fractional Rosenau-Hyman Equations within the Yang Transform

Preprint

Article

An Efficient Analytical Approaches to Investigate Nonlinear Two-Dimensional Time-Fractional Rosenau-Hyman Equations within the Yang Transform

Altmetrics

Downloads

156

Views

Comments

A peer-reviewed article of this preprint also exists.

This version is not peer-reviewed

Submitted:

02 February 2024

Posted:

02 February 2024

You are already at the latest version

Alerts

Abstract

The goal of the current study is to analyse several nonlinear two-dimensional time-fractional Rosenau Hymanequations. The two-dimensional fractional Rosenau-Hyman equation has extensive use in engineering and applied sciences. The fractional view analysis of two-dimensional time-fractional Rosenau-Hyman equations is discussed using the homotopy perturbation approach, adomian decomposition method, and Yang transformation. Some examples involving two-dimensional time-fractional Rosenau-Hyman equations are provided in order to better understand the suggested approaches. The solutions appear as infinite series. We offer a comparison between the accurate solutions and those that are generated employing the proposed approaches in order to demonstrate the effectiveness and applicability of the proposed techniques. The results are graphically illustrated using 2D and 3D graphs. It has been noted that the obtained results and the targeted problems real solutions are quite similar. Calculated solutions at various fractional levels describe some of the problems useful dynamics. Other fractional problems that arise in other fields of science and engineering can be solved using a modified version of the current techniques.

Keywords:

Subject: Computer Science and Mathematics - Computational Mathematics

1. Introduction

Fractional calculus (FC) is considered to be one of best area of defining problems in the field of science and engineering. The basic concept of FC arise from the notation of derivative used by Leibniz in his publications. L’Hopital was the one who arise a question that what the result would be if the derivative order is non-integer [1]. In recent years, derivatives of any order have been extensively used as a potent mathematical tool for modelling challenging issues. In reality their non-local feature and higher degree of freedom than ordinary derivatives have been two key factors in capturing scholars’ interest in them [1,2,3,4]. One significant class of classical fractional derivatives that has attracted a lot of attention is the caputo derivative. In fact, this form of derivative is a possible modification of the usual derivative. The initial conditions needed to solve fractional problems involving this type of derivative are the same as the initial conditions needed to solve problems with conventional derivatives, which is one of the good advantages of this derivative (in addition to useful properties expressed for conventional fractional derivatives) [1]. Recent uses of this kind of fractional derivative include designing an optimization model for the mosaic disease [5], more accurate modelling of the transmission of the coronavirus disease (COVID-19) [6], worm transmission design in wireless sensor networks [7], modelling of climate change [8] and many others.

Interesting extensions of conventional fractional differentiations are distributed-order (DO) fractional derivatives. It is widely used to determine such derivatives by integrating simple fractional differentiations with respect to their orders [9,10]. The behaviour of viscoelastic substances with spatially varying holdings is a well-known example that illustrates the capability of this family of derivatives [11]. They make a beneficial relationship between the common and traditional fractional differentiations, as shown in [12]. It is possible to think of fractional differential equations (FDEs) involving this form of derivative as generalised fractional equations [10,13]. During the last decades, FDEs have been extensively used in numerous fields of engineering and applied science [14,15,16,17,18,19,20]. Nonlinear differential equations defined most of phenomena in nature. So researchers pay more attention in different branches of science and engineering try to solve them. But due to involvement of nonlinear part in these types of equations, finding an exact solution is hard. The solutions of nonlinear FDEs have great concern both in mathematics and in useful applications. Consequently it is of great interest in the field of research of fractional models that how to build a reliable technique for to get the approximate solution or exact solution of FDEs.

Due to its extensive and considerable applications across many fields of sciences and engineering, fractional partial differential equations (FPDEs) have caught the attention of scientists. Numerous analytical, semi-analytical, and computational approaches have been developed and put into use to solve these nonlinear mathematical models that have been found in the literature. We frequently noticed that non-linear (FPDEs) were difficult to numerically solve. It has been established that analytical methods are essential for resolving these issues. Numerous analytical techniques have been used for finding the solution of these problems. Such as Laplace Variational Iteration Method [21], Iterative Laplace transform method [22], Laplace-Adomian decomposition method [23], approximate-analytical method [24], Reduced Differential Transform Method [25], Optimal Homotopy Asymptotic Method [26], Natural Transform Decomposition Method [27], Homotopy Analysis Method [28], Adomian Decomposition Method [29] and many more.

In this study, we have explored an effective classical method for solving two-dimensional Rosenau-Hyman equations based on the Homotopy perturbation method, the Adomian decomposition method, and the Yang transform. The Rosenau-Hyman equation was introduced by Rosenau and Hyman [30], and it appears in the formation of patterns in liquid drops with compaction solutions. In applied sciences and mathematical physics, the Rosenau-Hyman equation compacton investigations are helpful [31,32,33]. The general form of two-dimensional Rosenau-Hyman equation is:

\frac{\partial^{β} T}{\partial Y^{β}} + a (\frac{\partial}{\partial ς} (T^{n}) + \frac{\partial}{\partial φ} (T^{n})) + (\frac{\partial^{3}}{\partial ς^{3}} (T^{n}) + \frac{\partial^{3}}{\partial φ^{3}} (T^{n})) = 0,

(1)

in some continuous domain with initial conditions

T (ς, φ, 0) = f (ς, φ) .

Here,

β

is any real number.

In the current study, we suggested two novel computational techniques called the Yang transform decomposition method (YTDM) and Yang homotopy perturbation method (YHPM). The suggested methods combine the Yang transform with the homotopy perturbation approach and the adomian decomposition method to produce analytical solutions for the non-integer two-dimensional Rosenau-Hyman equations. The homotopy perturbation technique was introduced by He [34,35] in 1998. In this technique, the solution is assumed to be the sum of an infinite sequence that converges rapidly to the exact results. This approach was used to solve both linear and nonlinear equations. To create YTDM, the decomposition method and the Yang transform are merged. By eliminating the requirement to calculate the fractional integrals or fractional derivative in the recursive mechanism, the suggested method enables estimating the series terms easier than the conventional Adomian method [36,37].

The structure of this research study is as follows. Section 2 has covered an introduction to Yang transforms and their characteristics. In Section 3, the Yang transformation-based Homotopy perturbation method (YHPM) was covered. In Section 4, the Yang transformation-based adomian decomposition method (YTDM) was covered. To demonstrate the clarity and precision of the suggested methodologies, a few numerical problems have been done in Section 5 for examining the solution to the two-dimensional fractional Rosenau-Hyman equation. In Section 6, the conclusion is covered.

2. Preliminaries

In this section, along with Yang transform (YT) theory and properties, we have given some basic definitions of the fractional calculus.

Definition 1.

Fractional derivative in Caputo’s manner is given as [38]

\begin{matrix} D_{Y}^{β} T (ς, Y) = \frac{1}{Γ (k - β)} \int_{0}^{Y} {(Y - γ)}^{k - β - 1} T^{(k)} (ς, γ) d γ, k - 1 < β \leq k, k \in N . \end{matrix}

(2)

Definition 2.

The YT of the function is [35]

Y {T (Y)} = M (u) = \int_{0}^{\infty} e^{\frac{- Y}{u}} T (Y) d Y, Y > 0,

(3)

with u representing the transform variable.

Few properties of YT is stated as.

\begin{matrix} Y [1] = & u, \\ Y [Y] = & u^{2}, \\ Y [Y^{q}] = & Γ (q + 1) u^{q + 1}, \end{matrix}

(4)

illustrating the inverse YT as

Y^{- 1} {M (u)} = T (Y) .

(5)

Definition 3.

The YT nth order derivative function is as [35]

Y {T^{n} (Y)} = \frac{M (u)}{u^{n}} - \sum_{k = 0}^{n - 1} \frac{T^{k} (0)}{u^{n - k - 1}}, \forall n = 1, 2, 3, \dots

(6)

Definition 4.

The YT of non-integer order derivative function is as [35]

Y {T^{β} (Y)} = \frac{M (u)}{u^{β}} - \sum_{k = 0}^{n - 1} \frac{T^{k} (0)}{u^{β - (k + 1)}}, n - 1 < β \leq n .

(7)

3. Configuration for HPTM

To describe the fundamental idea of this approach we take the given equation:

D_{Y}^{β} T (ς, Y) = R [ς] T (ς, Y) + S [ς] T (ς, Y), 0 < β \leq 1,

(8)

with initial guess

T (ς, 0) = ξ (ς) .

where

D_{Y}^{β} = \frac{\partial^{β}}{\partial Y^{β}}

is Caputo’s derivative,

R [ς]

S [ς]

are linear and nonlinear operators.

By taking YT, we can write (8) as

Y [D_{Y}^{β} T (ς, Y)] = Y [R [ς] T (ς, Y) + S [ς] T (ς, Y)],

(9)

\frac{1}{u^{β}} {M (u) - u T (0)} = Y [R [ς] T (ς, Y) + S [ς] T (ς, Y)],

(10)

which simplifies to

M (u) = u T (0) + u^{β} Y [R [ς] T (ς, Y) + S [ς] T (ς, Y)] .

(11)

Now by taking inverse YT, we have

T (ς, Y) = T (0) + Y^{- 1} [u^{β} Y [R [ς] T (ς, Y) + S [ς] T (ς, Y)]] .

(12)

Now in terms of homotopy perturbation method (HPM), Eq. (12) become

T (ς, Y) = T (0) + ϵ [Y^{- 1} [u^{β} Y [R [ς] T (ς, Y) + S [ς] T (ς, Y)]]] .

(13)

Now the HPM having parameter

ϵ

is given as

T (ς, Y) = \sum_{k = 0}^{\infty} ϵ^{k} T_{k} (ς, Y),

(14)

with

ϵ \in [0, 1]

The nonlinear term can be decomposed as

S [ς] T (ς, Y) = \sum_{k = 0}^{\infty} ϵ^{k} H_{n} (T) .

(15)

where

H_{k}

are He’s polynomials and can be determined as

H_{k} (T_{0}, T_{1}, . . ., T_{n}) = \frac{1}{Γ (n + 1)} D_{ϵ}^{k} {[S (\sum_{k = 0}^{\infty} ϵ^{i} T_{i})]}_{ϵ = 0},

(16)

where

D_{ϵ}^{k} = \frac{\partial^{k}}{\partial ϵ^{k}} .

Using relation (14) and (15) in (13), we have

\sum_{k = 0}^{\infty} ϵ^{k} T_{k} (ς, Y) = T (0) + ϵ \times (Y^{- 1} [u^{β} Y {R \sum_{k = 0}^{\infty} ϵ^{k} T_{k} (ς, Y) + \sum_{k = 0}^{\infty} ϵ^{k} H_{k} (T)}]) .

(17)

On equating

ϵ

coefficients both sides, we get

\begin{matrix} ϵ^{0} : T_{0} (ς, Y) = T (0), \\ ϵ^{1} : T_{1} (ς, Y) = Y^{- 1} [u^{β} Y (R [ς] T_{0} (ς, Y) + H_{0} (T))], \\ ϵ^{2} : T_{2} (ς, Y) = Y^{- 1} [u^{β} Y (R [ς] T_{1} (ς, Y) + H_{1} (T))], \\ . \\ . \\ . \\ ϵ^{k} : T_{k} (ς, Y) = Y^{- 1} [u^{β} Y (R [ς] T_{k - 1} (ς, Y) + H_{k - 1} (T))], \\ k > 0, k \in N . \end{matrix}

(18)

The component

T_{k} (ς, Y)

is determined easily, which leads us to the convergent series.

T (ς, Y) = lim_{M \to \infty} \sum_{k = 1}^{M} T_{k} (ς, Y) .

(19)

4. Configuration for YTDM

To describe the fundamental idea of this approach we take the given equation:

D_{Y}^{β} T (ς, Y) = R (ς, Y) + S (ς, Y), 0 < β \leq 1,

(20)

with initial guess

T (ς, 0) = ξ (ς) .

where

D_{Y}^{β} = \frac{\partial^{β}}{\partial Y^{β}}

is Caputo’s derivative,

R

S

are linear and nonlinear operators.

By taking YT, we can write (20) as

\begin{matrix} Y [D_{Y}^{β} T (ς, Y)] = Y [R (ς, Y) + S (ς, Y)], \\ \frac{1}{u^{β}} {M (u) - u T (0)} = Y [R (ς, Y) + S (ς, Y)], \end{matrix}

(21)

which simplifies to

M (T) = u T (0) + u^{β} Y [R (ς, Y) + S (ς, Y)] .

(22)

Now by taking inverse YT, we have

T (ς, Y) = T (0) + Y^{- 1} [u^{β} Y [R (ς, Y) + S (ς, Y)] .

(23)

Also the solution in series form is determined as

T (ς, Y) = \sum_{m = 0}^{\infty} T_{m} (ς, Y) .

(24)

The nonlinear term can be decomposed as

S (ς, Y) = \sum_{m = 0}^{\infty} A_{m},

(25)

with

A_{m} = \frac{1}{m!} {[\frac{\partial^{m}}{\partial ℓ^{m}} \{S (\sum_{k = 0}^{\infty} ℓ^{k} ς_{k}, \sum_{k = 0}^{\infty} ℓ^{k} Y_{k})\}]}_{ℓ = 0} .

(26)

Using relation (24) and (25) into (23), we have

\sum_{m = 0}^{\infty} T_{m} (ς, Y) = T (0) + Y^{- 1} [u^{β} Y \{R (\sum_{m = 0}^{\infty} ς_{m}, \sum_{m = 0}^{\infty} Y_{m}) + \sum_{m = 0}^{\infty} A_{m}\}] .

(27)

So, we get the below approximation

T_{0} (ς, Y) = T (0),

(28)

T_{1} (ς, Y) = Y^{- 1} [u^{β} Y {R (ς_{0}, Y_{0}) + A_{0}}] .

Finally for

m \geq 1

, we have

T_{m + 1} (ς, Y) = Y^{- 1} [u^{β} Y {R (ς_{m}, Y_{m}) + A_{m}}] .

5. Applications

Example 1.

Assume the nonlinear two-dimensional fractional Rosenau-Hyman equation:

D_{Y}^{β} T (ς, φ, Y) + T_{ς}^{2} (ς, φ, Y) + T_{φ}^{2} (ς, φ, Y) + T_{ς ς ς}^{2} (ς, φ, Y) + T_{φ φ φ}^{2} (ς, φ, Y) = 0, 0 < β \leq 1,

(29)

having initial guess

T (ς, φ, 0) = ς + φ .

By taking YT, we may write (29) as

Y (\frac{\partial^{β} T}{\partial Y^{β}}) = Y (- T_{ς}^{2} (ς, φ, Y) - T_{φ}^{2} (ς, φ, Y) - T_{ς ς ς}^{2} (ς, φ, Y) - T_{φ φ φ}^{2} (ς, φ, Y)),

(30)

which simplifies to

\frac{1}{u^{β}} {M (u) - u T (0)} = Y (- T_{ς}^{2} (ς, φ, Y) - T_{φ}^{2} (ς, φ, Y) - T_{ς ς ς}^{2} (ς, φ, Y) - T_{φ φ φ}^{2} (ς, φ, Y)),

(31)

M (u) = u T (0) + u^{β} (- T_{ς}^{2} (ς, φ, Y) - T_{φ}^{2} (ς, φ, Y) - T_{ς ς ς}^{2} (ς, φ, Y) - T_{φ φ φ}^{2} (ς, φ, Y)) .

(32)

Now by taking inverse YT, we obtain

\begin{matrix} T (ς, φ, Y) = T (0) - Y^{- 1} [u^{β} \{Y (T_{ς}^{2} (ς, φ, Y) + T_{φ}^{2} (ς, φ, Y) + T_{ς ς ς}^{2} (ς, φ, Y) + T_{φ φ φ}^{2} (ς, φ, Y))\}], \\ T (ς, φ, Y) = (ς + φ) - Y^{- 1} [u^{β} \{Y (T_{ς}^{2} (ς, φ, Y) + T_{φ}^{2} (ς, φ, Y) + T_{ς ς ς}^{2} (ς, φ, Y) + T_{φ φ φ}^{2} (ς, φ, Y))\}] . \end{matrix}

(33)

By applying HPM to (33), we have

\begin{matrix} \sum_{k = 0}^{\infty} ϵ^{k} T_{k} (ς, φ, Y) = (ς + φ) - ϵ (Y^{- 1} [u^{β} Y [{(\sum_{k = 0}^{\infty} ϵ^{k} H_{k} (T))}_{ς} + {(\sum_{k = 0}^{\infty} ϵ^{k} H_{k} (T))}_{φ} + \\ {(\sum_{k = 0}^{\infty} ϵ^{k} H_{k} (T))}_{ς ς ς} + {(\sum_{k = 0}^{\infty} ϵ^{k} H_{k} (T))}_{φ φ φ}]]) . \end{matrix}

(34)

The first three nonlinear terms are calculated as

\begin{matrix} H_{0} (T) = T_{0}^{2}, \\ H_{1} (T) = 2 T_{0} T_{1}, \\ H_{2} (T) = 2 T_{0} T_{2} + {(T_{1})}^{2} . \end{matrix}

On equating ϵ coefficients both sides, we get

\begin{matrix} ϵ^{0} : T_{0} (ς, φ, Y) = ς + φ, \\ ϵ^{1} : T_{1} (ς, φ, Y) = Y^{- 1} (u^{β} Y [- {(H_{0} (T))}_{ς} - {(H_{0} (T))}_{φ} - {(H_{0} (T))}_{ς ς ς} - {(H_{0} (T))}_{φ φ φ}]) = \\ [- 4 (ς + φ)] \frac{Y^{β}}{Γ (β + 1)}, \\ ϵ^{2} : T_{2} (ς, φ, Y) = Y^{- 1} (u^{β} Y [- {(H_{1} (T))}_{ς} - {(H_{1} (T))}_{φ} - {(H_{1} (T))}_{ς ς ς} - {(H_{1} (T))}_{φ φ φ}]) = \\ [32 (ς + φ)] \frac{Y^{β + 1}}{Γ (β + 2)}, \\ ϵ^{3} : T_{3} (ς, φ, Y) = Y^{- 1} (u^{β} Y [- {(H_{2} (T))}_{ς} - {(H_{2} (T))}_{φ} - {(H_{2} (T))}_{ς ς ς} - {(H_{2} (T))}_{φ φ φ}]) = \\ [- 384 (ς + φ)] \frac{Y^{β + 2}}{Γ (β + 3)}, \\ ⋮ \end{matrix}

Finally, the analytical solution leads us to the convergent series rapidly as

\begin{matrix} T (ς, φ, Y) = T_{0} (ς, φ, Y) + T_{1} (ς, φ, Y) + T_{2} (ς, φ, Y) + T_{3} (ς, φ, Y) + \dots \\ T (ς, φ, Y) = (ς + φ) + [- 4 (ς + φ)] \frac{Y^{β}}{Γ (β + 1)} + [32 (ς + φ)] \frac{Y^{β + 1}}{Γ (β + 2)} + [- 384 (ς + φ)] \frac{Y^{β + 2}}{Γ (β + 3)} + \dots \end{matrix}

Implementation of YTDM

By taking YT, we may write (29) as

Y \{\frac{\partial^{β} T}{\partial Y^{β}}\} = Y (- T_{ς}^{2} (ς, φ, Y) - T_{φ}^{2} (ς, φ, Y) - T_{ς ς ς}^{2} (ς, φ, Y) - T_{φ φ φ}^{2} (ς, φ, Y)),

(35)

which simplifies to

\frac{1}{u^{β}} {M (u) - u T (0)} = Y (- T_{ς}^{2} (ς, φ, Y) - T_{φ}^{2} (ς, φ, Y) - T_{ς ς ς}^{2} (ς, φ, Y) - T_{φ φ φ}^{2} (ς, φ, Y)),

(36)

M (u) = u T (0) + u^{β} Y (- T_{ς}^{2} (ς, φ, Y) - T_{φ}^{2} (ς, φ, Y) - T_{ς ς ς}^{2} (ς, φ, Y) - T_{φ φ φ}^{2} (ς, φ, Y)) .

(37)

Now by taking inverse YT, we obtain

\begin{matrix} T (ς, φ, Y) = T (0) - Y^{- 1} [u^{β} \{Y (T_{ς}^{2} (ς, φ, Y) + T_{φ}^{2} (ς, φ, Y) + T_{ς ς ς}^{2} (ς, φ, Y) + T_{φ φ φ}^{2} (ς, φ, Y))\}], \\ T (ς, φ, Y) = (ς + φ) - Y^{- 1} [u^{β} \{Y (T_{ς}^{2} (ς, φ, Y) + T_{φ}^{2} (ς, φ, Y) + T_{ς ς ς}^{2} (ς, φ, Y) + T_{φ φ φ}^{2} (ς, φ, Y))\}] . \end{matrix}

(38)

Also the series form solution is determined as

T (ς, φ, Y) = \sum_{m = 0}^{\infty} T_{m} (ς, φ, Y) .

(39)

The nonlinear term can be decomposed as. So, we get

\begin{matrix} \sum_{m = 0}^{\infty} T_{m} (ς, φ, Y) = T (ς, φ, 0) - Y^{- 1} [u^{β} Y [{(\sum_{m = 0}^{\infty} A_{m})}_{ς} + {(\sum_{m = 0}^{\infty} A_{m})}_{φ} + {(\sum_{m = 0}^{\infty} A_{m})}_{ς ς ς} + \\ {(\sum_{m = 0}^{\infty} A_{m})}_{φ φ φ}]], \\ \sum_{m = 0}^{\infty} T_{m} (ς, φ, Y) = (ς + φ) - Y^{- 1} [u^{β} Y [{(\sum_{m = 0}^{\infty} A_{m})}_{ς} + {(\sum_{m = 0}^{\infty} A_{m})}_{φ} + {(\sum_{m = 0}^{\infty} A_{m})}_{ς ς ς} \\ {(\sum_{m = 0}^{\infty} A_{m})}_{φ φ φ}]] . \end{matrix}

(40)

The first three nonlinear terms are calculated as

\begin{matrix} A_{0} = T_{0}^{2}, \\ A_{1} = 2 T_{0} T_{1}, \\ A_{2} = 2 T_{0} T_{2} + T_{1}^{2} . \end{matrix}

So, we get the below approximation

T_{0} (ς, φ, Y) = ς + φ .

m = 0

T_{1} (ς, φ, Y) = [- 4 (ς + φ)] \frac{Y^{β}}{Γ (β + 1)} .

m = 1

T_{2} (ς, φ, Y) = [32 (ς + φ)] \frac{Y^{β + 1}}{Γ (β + 2)} .

m = 2

T_{3} (ς, φ, Y) = [- 384 (ς + φ)] \frac{Y^{β + 2}}{Γ (β + 3)} .

Finally, the analytical solution leads us to the convergent series rapidly as

T (ς, φ, Y) = \sum_{m = 0}^{\infty} T_{m} (ς, φ, Y) = T_{0} (ς, φ, Y) + T_{1} (ς, φ, Y) + T_{2} (ς, φ, Y) + T_{3} (ς, φ, Y) + \dots

\begin{matrix} T (ς, φ, Y) = (ς + φ) + [- 4 (ς + φ)] \frac{Y^{β}}{Γ (β + 1)} + [32 (ς + φ)] \frac{Y^{β + 1}}{Γ (β + 2)} + [- 384 (ς + φ)] \frac{Y^{β + 2}}{Γ (β + 3)} + \dots \end{matrix}

The solution at

β = 1

leads to the exact solution as

T (ς, φ, Y) = \frac{ς + φ}{1 + 4 Y} .

(41)

Figure 1. Surface (a) demonstrates the exact solution behavior (b) demonstrates our methods solution behavior (c) demonstrates our methods solution behavior at

β = 1

(d) demonstrates our methods solution behavior at

β = 1

Figure 1. Surface (a) demonstrates the exact solution behavior (b) demonstrates our methods solution behavior (c) demonstrates our methods solution behavior at

β = 1

(d) demonstrates our methods solution behavior at

β = 1

Figure 2. Surface (a) demonstrates the 3-D behavior of our methods solution at different fractional-orders (b) demonstrates the 2-D behavior of our methods solution at different fractional-orders (c) demonstrates absolute error of our methods solution at

β = 1

β = 1

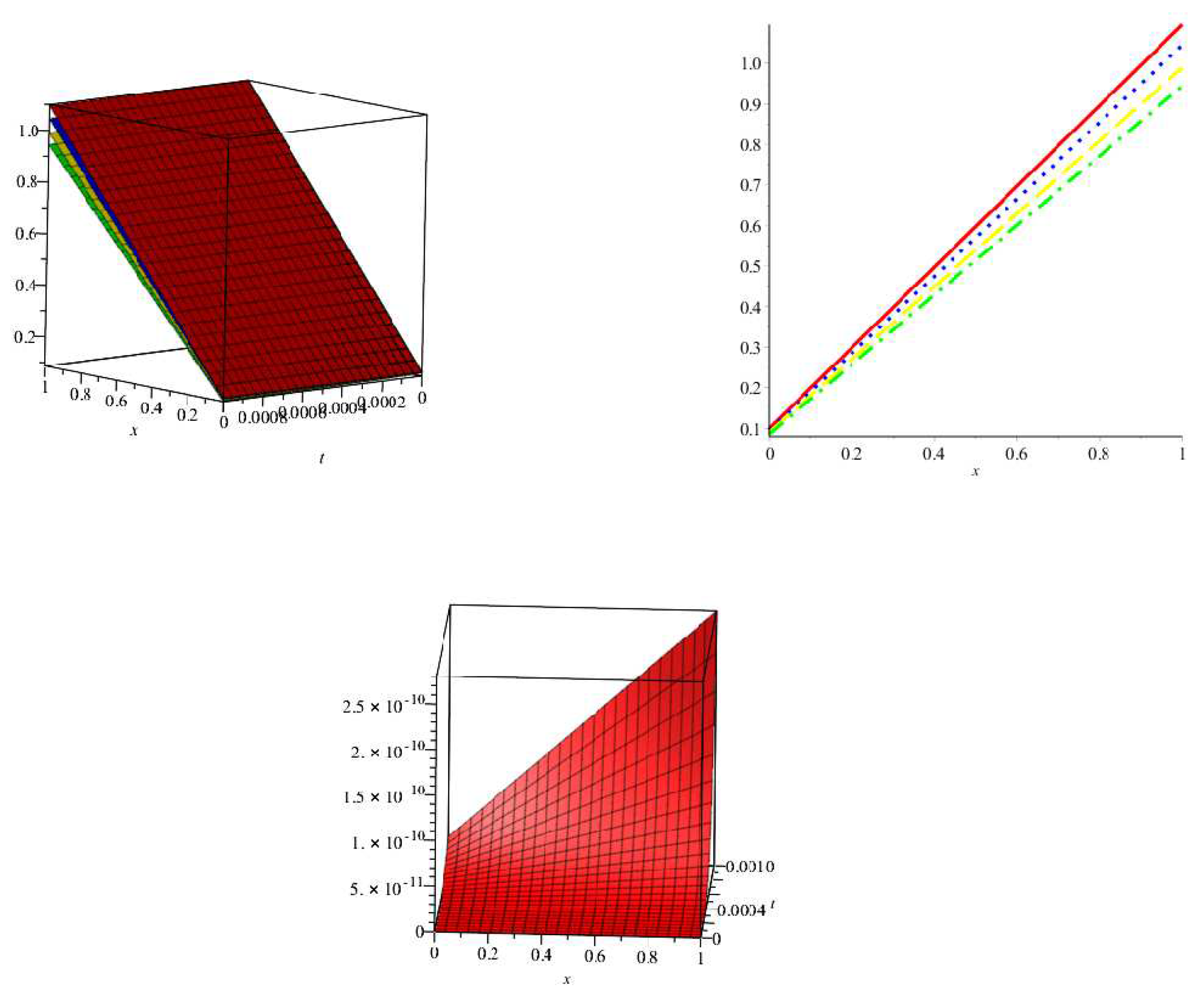
Table 1. Behavior of the exact as well as our methods solution for

F (ω, ζ)

at different orders of

β

of example 1.

Table 1. Behavior of the exact as well as our methods solution for

F (ω, ζ)

at different orders of

β

of example 1.

$(ω, ζ)$	Our solution at $β =$ 0.85	Our solution at $β =$ 0.90	Our solution at $β =$ 0.95	Our solution at $β =$ 1	Exact solution
(0.2,0.001)	0.69169056	0.69421552	0.69598017	0.69721115	0.69721115
(0.4,0.001)	0.88931644	0.89256282	0.89483165	0.89641434	0.89641434
(0.6,0.001)	1.08694232	1.09091011	1.09368313	1.09561753	1.09561753
(0.8,0.001)	1.28456820	1.28925740	1.29253461	1.29482071	1.29482071
(0.2,0.003)	0.67903959	0.68458136	0.68867761	0.69169959	0.69169960
(0.4,0.003)	0.87305090	0.88017603	0.88544265	0.88932804	0.88932806
(0.6,0.003)	1.06706222	1.07577071	1.08220768	1.08695649	1.08695652
(0.8,0.003)	1.26107353	1.27136538	1.27897272	1.28458495	1.28458498
(0.2,0.005)	0.66791671	0.67578334	0.68175305	0.68627440	0.68627450
(0.4,0.005)	0.85875006	0.86886429	0.87653964	0.88235280	0.88235294
(0.6,0.005)	1.04958341	1.06194525	1.07132623	1.07843120	1.07843137
(0.8,0.005)	1.24041676	1.25502620	1.26611282	1.27450960	1.27450980

Example 2.

Assume the nonlinear two-dimensional fractional Rosenau-Hyman equation:

\begin{matrix} D_{Y}^{β} T (ς, φ, Y) = T (ς, φ, Y) T_{ς} (ς, φ, Y) + T (ς, φ, Y) T_{φ} (ς, φ, Y) + T (ς, φ, Y) T_{ς ς ς} (ς, φ, Y) + T (ς, φ, Y) \\ T_{φ φ φ} (ς, φ, Y) + 3 T_{ς} (ς, φ, Y) T_{ς ς} (ς, φ, Y) + 3 T_{φ} (ς, φ, Y) T_{φ φ} (ς, φ, Y), 0 < β \leq 1, \end{matrix}

(42)

having initial guess

T (ς, φ, 0) = - \frac{8}{3} {cos}^{2} (\frac{ς + φ}{4}) .

By taking YT, we may write (42) as

\begin{matrix} Y (\frac{\partial^{β} T}{\partial Y^{β}}) = Y (T (ς, φ, Y) T_{ς} (ς, φ, Y) + T (ς, φ, Y) T_{φ} (ς, φ, Y) + T (ς, φ, Y) T_{ς ς ς} (ς, φ, Y) + T (ς, φ, Y) \\ T_{φ φ φ} (ς, φ, Y) + 3 T_{ς} (ς, φ, Y) T_{ς ς} (ς, φ, Y) + 3 T_{φ} (ς, φ, Y) T_{φ φ} (ς, φ, Y)), \end{matrix}

(43)

which simplifies to

\begin{matrix} \frac{1}{u^{β}} {M (u) - u T (0)} = Y (T (ς, φ, Y) T_{ς} (ς, φ, Y) + T (ς, φ, Y) T_{φ} (ς, φ, Y) + T (ς, φ, Y) T_{ς ς ς} (ς, φ, Y) + \\ T (ς, φ, Y) T_{φ φ φ} (ς, φ, Y) + 3 T_{ς} (ς, φ, Y) T_{ς ς} (ς, φ, Y) + 3 T_{φ} (ς, φ, Y) T_{φ φ} (ς, φ, Y)), \end{matrix}

(44)

\begin{matrix} M (u) = u T (0) + u^{β} (T (ς, φ, Y) T_{ς} (ς, φ, Y) + T (ς, φ, Y) T_{φ} (ς, φ, Y) + T (ς, φ, Y) T_{ς ς ς} (ς, φ, Y) + T (ς, φ, Y) \\ T_{φ φ φ} (ς, φ, Y) + 3 T_{ς} (ς, φ, Y) T_{ς ς} (ς, φ, Y) + 3 T_{φ} (ς, φ, Y) T_{φ φ} (ς, φ, Y)) . \end{matrix}

(45)

Now by taking inverse YT, we obtain

\begin{matrix} T (ς, φ, Y) = T (0) + Y^{- 1} [u^{β} {Y (T (ς, φ, Y) T_{ς} (ς, φ, Y) + T (ς, φ, Y) T_{φ} (ς, φ, Y) + T (ς, φ, Y) T_{ς ς ς} (ς, φ, Y) \\ + T (ς, φ, Y) T_{φ φ φ} (ς, φ, Y) + 3 T_{ς} (ς, φ, Y) T_{ς ς} (ς, φ, Y) + 3 T_{φ} (ς, φ, Y) T_{φ φ} (ς, φ, Y))}], \\ T (ς, φ, Y) = - \frac{8}{3} {cos}^{2} (\frac{ς + φ}{4}) + Y^{- 1} [u^{β} {Y (T (ς, φ, Y) T_{ς} (ς, φ, Y) + T (ς, φ, Y) T_{φ} (ς, φ, Y) + \\ T (ς, φ, Y) T_{ς ς ς} (ς, φ, Y) + T (ς, φ, Y) T_{φ φ φ} (ς, φ, Y) + 3 T_{ς} (ς, φ, Y) T_{ς ς} (ς, φ, Y) + 3 T_{φ} (ς, φ, Y) T_{φ φ} (ς, φ, Y))}] . \end{matrix}

(46)

By applying HPM to (46), we have

\begin{matrix} \sum_{k = 0}^{\infty} ϵ^{k} T_{k} (ς, φ, Y) = - \frac{8}{3} {cos}^{2} (\frac{ς + φ}{4}) + ϵ (Y^{- 1} [u^{β} Y [(\sum_{k = 0}^{\infty} ϵ^{k} H_{k}^{1} (T)) + (\sum_{k = 0}^{\infty} ϵ^{k} H_{k}^{2} (T)) + \\ (\sum_{k = 0}^{\infty} ϵ^{k} H_{k}^{3} (T))]]) . \end{matrix}

(47)

The first three nonlinear terms are calculated as:

\begin{matrix} H_{0}^{1} (T) = T_{0} {(T_{0})}_{ς} + T_{0} {(T_{0})}_{φ}, \\ H_{1}^{1} (T) = T_{1} {(T_{0})}_{ς} + T_{0} {(T_{1})}_{ς} + T_{1} {(T_{0})}_{φ} + T_{0} {(T_{1})}_{φ}, \\ H_{2}^{1} (T) = T_{2} {(T_{0})}_{ς} + T_{1} {(T_{1})}_{ς} + T_{0} {(T_{2})}_{ς} + T_{2} {(T_{0})}_{φ} + T_{1} {(T_{1})}_{φ} + T_{0} {(T_{2})}_{φ}, \\ ⋮ \\ H_{0}^{2} (T) = T_{0} {(T_{0})}_{ς ς ς} + T_{0} {(T_{0})}_{φ φ φ}, \\ H_{1}^{2} (T) = T_{1} {(T_{0})}_{ς ς ς} + T_{0} {(T_{1})}_{ς ς ς} + T_{1} {(T_{0})}_{φ φ φ} + T_{0} {(T_{1})}_{φ φ φ}, \\ H_{2}^{2} (T) = T_{2} {(T_{0})}_{ς ς ς} + T_{1} {(T_{1})}_{ς ς ς} + T_{0} {(T_{2})}_{ς ς ς} + T_{2} {(T_{0})}_{φ φ φ} + T_{1} {(T_{1})}_{φ φ φ} + T_{0} {(T_{2})}_{φ φ φ}, \\ ⋮ \\ H_{0}^{3} (T) = {(T_{0})}_{ς} {(T_{0})}_{ς ς} + {(T_{0})}_{φ} {(T_{0})}_{φ φ}, \\ H_{1}^{3} (T) = {(T_{1})}_{ς} {(T_{0})}_{ς ς} + {(T_{1})}_{ς} {(T_{0})}_{ς ς} + {(T_{0})}_{φ} {(T_{1})}_{φ φ} + {(T_{0})}_{φ} {(T_{1})}_{φ φ}, \\ H_{2}^{3} (T) = {(T_{2})}_{ς} {(T_{0})}_{ς ς} + {(T_{1})}_{ς} {(T_{1})}_{ς ς} + {(T_{0})}_{ς} {(T_{2})}_{ς ς} + {(T_{2})}_{φ} {(T_{0})}_{φ φ} + {(T_{1})}_{φ} {(T_{1})}_{φ φ} + {(T_{0})}_{φ} {(T_{2})}_{φ φ}, \end{matrix}

On equating ϵ coefficients both sides, we get

\begin{matrix} ϵ^{0} : T_{0} (ς, φ, Y) = - \frac{8}{3} {cos}^{2} (\frac{ς + φ}{4}), \\ ϵ^{1} : T_{1} (ς, φ, Y) = Y^{- 1} (u^{β} Y [H_{0}^{1} (T) + H_{0}^{2} (T) + H_{0}^{3} (T)]) = - \frac{4}{3} sin (\frac{ς + φ}{2}) \frac{Y^{β}}{Γ (β + 1)}, \\ ϵ^{2} : T_{2} (ς, φ, Y) = Y^{- 1} (u^{β} Y [H_{1}^{1} (T) + H_{1}^{2} (T) + H_{1}^{3} (T)]) = \frac{4}{3} cos (\frac{ς + φ}{2}) \frac{Y^{β + 1}}{Γ (β + 2)}, \\ ϵ^{3} : T_{3} (ς, φ, Y) = Y^{- 1} (u^{β} Y [H_{2}^{1} (T) + H_{2}^{2} (T) + H_{2}^{3} (T)]) = \frac{4}{3} sin (\frac{ς + φ}{2}) \frac{Y^{β + 2}}{Γ (β + 3)}, \\ ⋮ \end{matrix}

Finally, the analytical solution leads us to the convergent series rapidly as

\begin{matrix} T (ς, φ, Y) = T_{0} (ς, φ, Y) + T_{1} (ς, φ, Y) + T_{2} (ς, φ, Y) + T_{3} (ς, φ, Y) + \dots \\ T (ς, φ, Y) = - \frac{8}{3} {cos}^{2} (\frac{ς + φ}{4}) - \frac{4}{3} sin (\frac{ς + φ}{2}) \frac{Y^{β}}{Γ (β + 1)} + \frac{4}{3} cos (\frac{ς + φ}{2}) \frac{Y^{β + 1}}{Γ (β + 2)} + \frac{4}{3} \\ sin (\frac{ς + φ}{2}) \frac{Y^{β + 2}}{Γ (β + 3)} + \dots \end{matrix}

Implementation of YTDM

By taking YT, we may write (42) as

\begin{matrix} Y \{\frac{\partial^{β} T}{\partial Y^{β}}\} = Y (T (ς, φ, Y) T_{ς} (ς, φ, Y) + T (ς, φ, Y) T_{φ} (ς, φ, Y) + T (ς, φ, Y) T_{ς ς ς} (ς, φ, Y) + T (ς, φ, Y) \\ T_{φ φ φ} (ς, φ, Y) + 3 T_{ς} (ς, φ, Y) T_{ς ς} (ς, φ, Y) + 3 T_{φ} (ς, φ, Y) T_{φ φ} (ς, φ, Y)), \end{matrix}

(48)

which simplifies to

\begin{matrix} \frac{1}{u^{β}} {M (u) - u T (0)} = Y (T (ς, φ, Y) T_{ς} (ς, φ, Y) + T (ς, φ, Y) T_{φ} (ς, φ, Y) + T (ς, φ, Y) T_{ς ς ς} (ς, φ, Y) + \\ T (ς, φ, Y) T_{φ φ φ} (ς, φ, Y) + 3 T_{ς} (ς, φ, Y) T_{ς ς} (ς, φ, Y) + 3 T_{φ} (ς, φ, Y) T_{φ φ} (ς, φ, Y)), \end{matrix}

(49)

\begin{matrix} M (u) = u T (0) + u^{β} Y (T (ς, φ, Y) T_{ς} (ς, φ, Y) + T (ς, φ, Y) T_{φ} (ς, φ, Y) + T (ς, φ, Y) T_{ς ς ς} (ς, φ, Y) + T (ς, φ, Y) \\ T_{φ φ φ} (ς, φ, Y) + 3 T_{ς} (ς, φ, Y) T_{ς ς} (ς, φ, Y) + 3 T_{φ} (ς, φ, Y) T_{φ φ} (ς, φ, Y)) . \end{matrix}

(50)

Now by taking inverse YT, we obtain

\begin{matrix} T (ς, φ, Y) = T (0) + Y^{- 1} [u^{β} {Y (T (ς, φ, Y) T_{ς} (ς, φ, Y) + T (ς, φ, Y) T_{φ} (ς, φ, Y) + T (ς, φ, Y) T_{ς ς ς} (ς, φ, Y) \\ + T (ς, φ, Y) T_{φ φ φ} (ς, φ, Y) + 3 T_{ς} (ς, φ, Y) T_{ς ς} (ς, φ, Y) + 3 T_{φ} (ς, φ, Y) T_{φ φ} (ς, φ, Y))}], \\ T (ς, φ, Y) = - \frac{8}{3} {cos}^{2} (\frac{ς + φ}{4}) + Y^{- 1} [u^{β} {Y (T (ς, φ, Y) T_{ς} (ς, φ, Y) + T (ς, φ, Y) T_{φ} (ς, φ, Y) + \\ T (ς, φ, Y) T_{ς ς ς} (ς, φ, Y) + T (ς, φ, Y) T_{φ φ φ} (ς, φ, Y) + 3 T_{ς} (ς, φ, Y) T_{ς ς} (ς, φ, Y) + 3 T_{φ} (ς, φ, Y) T_{φ φ} (ς, φ, Y))}] . \end{matrix}

(51)

Also the series form solution is determined as

T (ς, φ, Y) = \sum_{m = 0}^{\infty} T_{m} (ς, φ, Y) .

(52)

The nonlinear term can be decomposed as. So, we get

\begin{matrix} \sum_{m = 0}^{\infty} T_{m} (ς, φ, Y) = T (ς, φ, 0) + Y^{- 1} [u^{β} Y [(\sum_{m = 0}^{\infty} A_{m}) + (\sum_{m = 0}^{\infty} B_{m}) + (\sum_{m = 0}^{\infty} C_{m})]], \\ \sum_{m = 0}^{\infty} T_{m} (ς, φ, Y) = - \frac{8}{3} {cos}^{2} (\frac{ς + φ}{4}) + Y^{- 1} [u^{β} Y [(\sum_{m = 0}^{\infty} A_{m}) + (\sum_{m = 0}^{\infty} B_{m}) + (\sum_{m = 0}^{\infty} C_{m})]] . \end{matrix}

(53)

The first three nonlinear terms are calculated as

\begin{matrix} A_{0} = T_{0} {(T_{0})}_{ς} + T_{0} {(T_{0})}_{φ}, \\ A_{1} = T_{1} {(T_{0})}_{ς} + T_{0} {(T_{1})}_{ς} + T_{1} {(T_{0})}_{φ} + T_{0} {(T_{1})}_{φ}, \\ A_{2} = T_{2} {(T_{0})}_{ς} + T_{1} {(T_{1})}_{ς} + T_{0} {(T_{2})}_{ς} + T_{2} {(T_{0})}_{φ} + T_{1} {(T_{1})}_{φ} + T_{0} {(T_{2})}_{φ}, \\ ⋮ \\ B_{0} = T_{0} {(T_{0})}_{ς ς ς} + T_{0} {(T_{0})}_{φ φ φ}, \\ B_{1} = T_{1} {(T_{0})}_{ς ς ς} + T_{0} {(T_{1})}_{ς ς ς} + T_{1} {(T_{0})}_{φ φ φ} + T_{0} {(T_{1})}_{φ φ φ}, \\ B_{2} = T_{2} {(T_{0})}_{ς ς ς} + T_{1} {(T_{1})}_{ς ς ς} + T_{0} {(T_{2})}_{ς ς ς} + T_{2} {(T_{0})}_{φ φ φ} + T_{1} {(T_{1})}_{φ φ φ} + T_{0} {(T_{2})}_{φ φ φ}, \\ ⋮ \\ C_{0} = {(T_{0})}_{ς} {(T_{0})}_{ς ς} + {(T_{0})}_{φ} {(T_{0})}_{φ φ}, \\ C_{1} = {(T_{1})}_{ς} {(T_{0})}_{ς ς} + {(T_{1})}_{ς} {(T_{0})}_{ς ς} + {(T_{0})}_{φ} {(T_{1})}_{φ φ} + {(T_{0})}_{φ} {(T_{1})}_{φ φ}, \\ C_{2} = {(T_{2})}_{ς} {(T_{0})}_{ς ς} + {(T_{1})}_{ς} {(T_{1})}_{ς ς} + {(T_{0})}_{ς} {(T_{2})}_{ς ς} + {(T_{2})}_{φ} {(T_{0})}_{φ φ} + {(T_{1})}_{φ} {(T_{1})}_{φ φ} + {(T_{0})}_{φ} {(T_{2})}_{φ φ}, \end{matrix}

So, we get the below approximation

T_{0} (ς, φ, Y) = - \frac{8}{3} {cos}^{2} (\frac{ς + φ}{4}) .

m = 0

T_{1} (ς, φ, Y) = - \frac{4}{3} sin (\frac{ς + φ}{2}) \frac{Y^{β}}{Γ (β + 1)} .

m = 1

\begin{matrix} T_{2} (ς, φ, Y) = \frac{4}{3} cos (\frac{ς + φ}{2}) \frac{Y^{β + 1}}{Γ (β + 2)} . \end{matrix}

m = 2

\begin{matrix} T_{3} (ς, φ, Y) = \frac{4}{3} sin (\frac{ς + φ}{2}) \frac{Y^{β + 2}}{Γ (β + 3)} . \end{matrix}

Finally, the analytical solution leads us to the convergent series rapidly as

T (ς, φ, Y) = \sum_{m = 0}^{\infty} T_{m} (ς, φ, Y) = T_{0} (ς, φ, Y) + T_{1} (ς, φ, Y) + T_{2} (ς, φ, Y) + T_{3} (ς, φ, Y) + \dots

\begin{matrix} T (ς, φ, Y) = - \frac{8}{3} {cos}^{2} (\frac{ς + φ}{4}) - \frac{4}{3} sin (\frac{ς + φ}{2}) \frac{Y^{β}}{Γ (β + 1)} + \frac{4}{3} cos (\frac{ς + φ}{2}) \frac{Y^{β + 1}}{Γ (β + 2)} + \frac{4}{3} \\ sin (\frac{ς + φ}{2}) \frac{Y^{β + 2}}{Γ (β + 3)} + \dots \end{matrix}

The solution at

β = 1

leads to the exact solution as

T (ς, φ, Y) = - \frac{8}{3} {cos}^{2} (\frac{ς + φ - 2 Y}{4}) .

(54)

Figure 3. Surface (a) demonstrates the exact solution behavior (b) demonstrates our methods solution behavior (c) demonstrates our methods solution behavior at

β = 1

(d) demonstrates our methods solution behavior at

β = 1

Figure 3. Surface (a) demonstrates the exact solution behavior (b) demonstrates our methods solution behavior (c) demonstrates our methods solution behavior at

β = 1

(d) demonstrates our methods solution behavior at

β = 1

Figure 4. Surface (a) demonstrates the 3-D behavior of our methods solution at different fractional-orders (b) demonstrates the 2-D behavior of our methods solution at different fractional-orders (c) demonstrates absolute error of our methods solution at

β = 1

β = 1

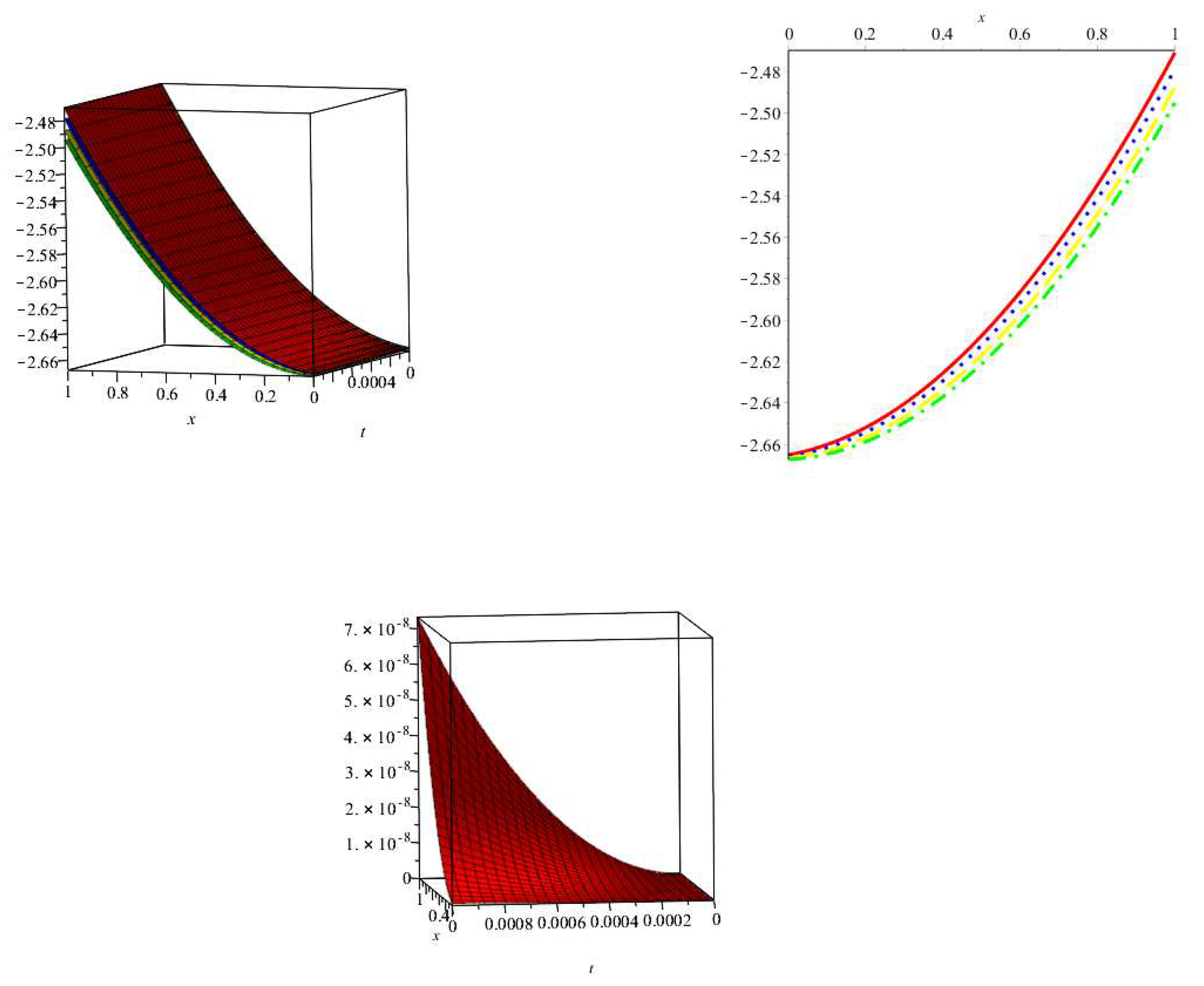
Table 2. Behavior of the exact as well as our methods solution for

F (ω, ζ)

at different orders of

β

of example 2.

Table 2. Behavior of the exact as well as our methods solution for

F (ω, ζ)

at different orders of

β

of example 2.

$(ω, ζ)$	Our solution at $β =$ 0.85	Our solution at $β =$ 0.90	Our solution at $β =$ 0.95	Our solution at $β =$ 1	Exact solution
(0.2,0.001)	-2.58719083	-2.58677734	-2.58648838	-2.58628682	-2.58628685
(0.4,0.001)	-2.53565592	-2.53513121	-2.53476453	-2.53450877	-2.53450882
(0.6,0.001)	-2.47210777	-2.47147710	-2.47103638	-2.47072897	-2.47072904
(0.8,0.001)	-2.39718136	-2.39645103	-2.39594066	-2.39558468	-2.39558478
(0.2,0.003)	-2.58928105	-2.58836815	-2.58769353	-2.58719596	-2.58719623
(0.4,0.003)	-2.53831126	-2.53715197	-2.53629530	-2.53566348	-2.53566392
(0.6,0.003)	-2.47530161	-2.47390754	-2.47287741	-2.47211766	-2.47211832
(0.8,0.003)	-2.40088168	-2.39926680	-2.39807352	-2.39719345	-2.39719436
(0.2,0.005)	-2.59114070	-2.58983720	-2.58884842	-2.58809984	-2.58810060
(0.4,0.005)	-2.54067731	-2.53902074	-2.53776422	-2.53681298	-2.53681422
(0.6,0.005)	-2.47815022	-2.47615722	-2.47464557	-2.47350122	-2.47350305
(0.8,0.005)	-2.40418420	-2.40187476	-2.40012314	-2.39879716	-2.39879968

6. Conclusions

The fractional view analysis of the two-dimensional time-fractional Rosenau-Hyman equations has been computed in the current study. In order to lead the solution, the Yang transformation is combined with the adomian decomposition method and the Homotopy perturbation method. To highlight its physical characteristics at the chosen values for the assuming considering parameters, some of the obtained findings have been represented in two and three dimensions. The fractional problems solution are convergent toward the integer-order solutions. By investigating two problems, the adequacy of the methods were shown. Additionally, the suggested methods have good accuracy and required less calculations. The offered approaches can be applied to additional fractional-order problems due to their straightforward implementation and ease of use.

Author Contributions

A.H.G., S.M., A.K. developed the idea. R.M.A. wrote the manuscript, N.A., W.G., A.S.B. reviewed and edited the manuscript. All authors have read and agreed to the published version of the manuscript.

Funding

The authors extend their appreciation to the Deanship of Scientific Research at King Khalid University for funding this work through large group Research Project under grant number RGP2/402/44.

Data Availability Statement

The numerical data used to support the findings of this study are included within the article.

Acknowledgments

The authors extend their appreciation to the Deanship of Scientific Research at King Khalid University for funding this work through large group Research Project under grant number RGP2/402/44.

Conflicts of Interest

The authors declare that they have no competing interests.

References

Podlubny, I. 1998. Fractional differential equations: an introduction to fractional derivatives, fractional differential equations, to methods of their solution and some of their applications. Elsevier.
Li, M. 2022. Multi-fractal traffic and anomaly detection in computer communications. CRC Press.
Li, M. , 2022. Fractal Teletraffic Modeling and Delay Bounds in Computer Communications. CRC Press.
Das, N.; Saha Ray, S. Dispersive optical soliton wave solutions for the time-fractional perturbed nonlinear Schrödinger equation with truncated M-fractional conformable derivative in the nonlinear optical fibers. Optical and Quantum Electronics 2022, 54, 544. [Google Scholar] [CrossRef]
Vellappandi, M., Kumar, P., Govindaraj, V. and Albalawi, W. An optimal control problem for mosaic disease via Caputo fractional derivative. Alexandria Engineering Journal 2022, 61, 8027–8037. [CrossRef]
Bavi, O., Hosseininia, M., Heydari, M.H. and Bavi, N., 2022. SARS-CoV-2 rate of spread in and across tissue, groundwater and soil: A meshless algorithm for the fractional diffusion equation. Engineering analysis with boundary elements, 138, pp.108-117. [CrossRef]
Achar, S.J., Baishya, C. and Kaabar, M.K., 2022. Dynamics of the worm transmission in wireless sensor network in the framework of fractional derivatives. Mathematical Methods in the Applied Sciences, 45(8), pp.4278-4294. [CrossRef]
Din, A. , Khan, F.M., Khan, Z.U., Yusuf, A. and Munir, T., 2022. The mathematical study of climate change model under nonlocal fractional derivative. Partial Differential Equations in Applied Mathematics, 5, p.100204. [CrossRef]
Yuttanan, B. and Razzaghi, M., 2019. Legendre wavelets approach for numerical solutions of distributed order fractional differential equations. Applied Mathematical Modelling, 70, pp.350-364. [CrossRef]
Zaky, M.A. and Machado, J.T., 2017. On the formulation and numerical simulation of distributed-order fractional optimal control problems. Communications in Nonlinear Science and Numerical Simulation, 52, pp.177-189. [CrossRef]
Lorenzo, C.F. and Hartley, T.T., 2002. Variable order and distributed order fractional operators. Nonlinear dynamics, 29, pp.57-98. [CrossRef]
Li, Y. , Sheng, H. and Chen, Y.Q., 2011. On distributed order integrator/differentiator. Signal Processing, 91(5), pp.1079-1084. [CrossRef]
Yuttanan, B. , Razzaghi, M. and Vo, T.N., 2021. A numerical method based on fractional-order generalized Taylor wavelets for solving distributed-order fractional partial differential equations. Applied Numerical Mathematics, 160, pp.349-367. [CrossRef]
Mishra, N.K. , AlBaidani, M.M., Khan, A. and Ganie, A.H., 2023. Two Novel Computational Techniques for Solving Nonlinear Time-Fractional Lax’s Korteweg-de Vries Equation. Axioms, 12(4), p.400. [CrossRef]
Mishra, N.K. , AlBaidani, M.M., Khan, A. and Ganie, A.H., 2023. Numerical Investigation of Time-Fractional Phi-Four Equation via Novel Transform. Symmetry, 15(3), p.687. [CrossRef]
Ray, S.S. and Bera, R.K., 2006. Analytical solution of a fractional diffusion equation by Adomian decomposition method. Applied Mathematics and Computation, 174(1), pp.329-336. [CrossRef]
Ganie, A.H. , AlBaidani, M.M. and Khan, A., 2023. A Comparative Study of the Fractional Partial Differential Equations via Novel Transform. Symmetry, 15(5), p.1101. [CrossRef]
Ray, S.S. , 2008. A new approach for the application of Adomian decomposition method for the solution of fractional space diffusion equation with insulated ends. Applied Mathematics and Computation, 202(2), pp.544-549. [CrossRef]
Sunthrayuth, P. , Alyousef, H.A., El-Tantawy, S.A., Khan, A. and Wyal, N., 2022. Solving Fractional-Order Diffusion Equations in a Plasma and Fluids via a Novel Transform. Journal of Function Spaces, 2022. [CrossRef]
Alderremy, A.A. , Aly, S., Fayyaz, R., Khan, A., Shah, R. and Wyal, N., 2022. The analysis of fractional-order nonlinear systems of third order KdV and Burgers equations via a novel transform. Complexity, 2022.
Jafari, H. and Jassim, H.K., 2014. Local fractional Laplace variational iteration method for solving nonlinear partial differential equations on Cantor sets within local fractional operators. Journal of Zankoy Sulaimani-Part A, 16(4), pp.49-57. [CrossRef]
Yan, L. , 2013. Numerical solutions of fractional Fokker-Planck equations using iterative Laplace transform method. In Abstract and applied analysis (Vol. 2013). Hindawi. [CrossRef]
Hendi, F.A. , 2011. Laplace Adomian decomposition method for solving the nonlinear Volterra integral equation with weakly kernels. Studies in Nonlinear Sciences, 2(4), pp.129-134.
El-Ajou, A. , Arqub, O.A. and Momani, S., 2015. Approximate analytical solution of the nonlinear fractional KdV-Burgers equation: a new iterative algorithm. Journal of Computational Physics, 293, pp.81-95. [CrossRef]
Rawashdeh, M. , 2013. Using the reduced differential transform method to solve nonlinear PDEs arises in biology and physics. World Applied Sciences Journal, 23(8), pp.1037-1043. [CrossRef]
Hashmi, M.S. , Khan, N. and Iqbal, S., 2012. Optimal homotopy asymptotic method for solving nonlinear Fredholm integral equations of second kind. Applied Mathematics and Computation, 218(22), pp.10982-10989. [CrossRef]
Shah, R. , Khan, H., Kumam, P., Arif, M. and Baleanu, D., 2019. Natural transform decomposition method for solving fractional-order partial differential equations with proportional delay. Mathematics, 7(6), p.532. [CrossRef]
Dehghan, M. , Manafian, J. and Saadatmandi, A., 2010. Solving nonlinear fractional partial differential equations using the homotopy analysis method. Numerical Methods for Partial Differential Equations: An International Journal, 26(2), pp.448-479. [CrossRef]
El-Wakil, S.A. , Elhanbaly, A. and Abdou, M.A., 2006. Adomian decomposition method for solving fractional nonlinear differential equations. Applied Mathematics and Computation, 182(1), pp.313-324. [CrossRef]
Rosenau P, Hyman JM (1993) Compactons: solitons with finite wavelength. Phys Rev Lett 70:564-567. [CrossRef]
Mihaila B, Cardenas A, Cooper F, Saxena A (2010) Stability and dynamical properties of Rosenau Hyman compactons using Padé approximants. Phys Rev E. [CrossRef]
Bazeia D, Das A, Losano L, Santos MJ (2010) Traveling wave solutions of nonlinear partial differential equations. Appl Math Lett 23:681-686. [CrossRef]
Rus F, Villatoro FR (2007) Self-similar radiation from numerical Rosenau-Hyman compactons. J Comput Phys 227:440-454. [CrossRef]
He, J.H. , 1999. Homotopy perturbation technique. Computer methods in applied mechanics and engineering, 178(3-4), pp.257-262. [CrossRef]
He, J.H. , 2000. A coupling method of a homotopy technique and a perturbation technique for non-linear134 problems. International journal of non-linear mechanics, 35(1), pp.37-43. [CrossRef]
G. Adomian, R. Rach, Inversion of nonlinear stochastic operators, J. Math. Anal. Appl. 91 (1983)39-46. [CrossRef]
R. E. Bellman, G. Adomian, Partial Differential Equations: New Methods for their Treatment and Solution, D. Reidel, Dordrecht, 1985.
Podlubny, I. and Kacenak, M. (2001) Isoclinal matrices and numerical solution of fractional differential equations, 2001 European Control Conference (ECC). [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content.

© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).

Copyright: This open access article is published under a Creative Commons CC BY 4.0 license, which permit the free download, distribution, and reuse, provided that the author and preprint are cited in any reuse.

MDPI Initiatives

Important Links

Choose an area of interest and we will send you notifications of new preprints at your preferred frequency.

Disclaimer

An Efficient Analytical Approaches to Investigate Nonlinear Two-Dimensional Time-Fractional Rosenau-Hyman Equations within the Yang Transform

Abstract

1. Introduction

2. Preliminaries

3. Configuration for HPTM

4. Configuration for YTDM

5. Applications

6. Conclusions

Author Contributions

Funding

Data Availability Statement

Acknowledgments

Conflicts of Interest

References

MDPI Initiatives

Important Links

Subscribe