You are currently viewing a beta version of our website. If you spot anything unusual, kindly let us know.

Preprint

Communication

Antenna Array on a Multilayer Dielectric Structure as a Nanostructure

Altmetrics

Downloads

Views

Comments

Marian Wnuk^*

This version is not peer-reviewed

Abstract

The work contains a detailed analysis of the electromagnetic field originating from a point source placed above the layered structure.The surface impedance of the multilayer dielectric structure was determined. Based on the boundary conditions, a method for determining the surface impedance of a layered structure with a perfectly conductive screen and or a magnetic screen is presented. The resonance conditions and the conditions for the excitation of surface waves are derived. The integral expression defining the generalized impedance was analyzed with a view to adapting it to numerical implementation. This allowed the development of a computer program based on which calculations were made of the effect of excitation of waves on multilayer dielectric substrates.

Keywords:

Subject: Engineering - Electrical and Electronic Engineering

1. Introduction

Printed antennas have been one of the most innovative fields of antenna technology for over a dozen years. In the early 1950s, radiation from strip lines and microstrip lines was observed. However, from the point of view of the use of transmission lines, it was an undesirable phenomenon, so apart from a few articles suggesting its use in the construction of antennas, it did not arouse much interest. Only Munson’s article [1] showed that microstrip antennas can have practical applications. In 1979, the first international conference was held in La Cruses, devoted to a comprehensive approach to antennas on dielectric substrates, i.e. materials, construction plans, configuration systems and theoretical foundations.

Microstrip antennas have many interesting features, such as:

• accurate representation on the surface,

• low manufacturing cost,

• high repeatability of workmanship,

• insignificant volume,

• masking the operating frequency,

• simplicity of production provided that relatively advanced technologies are used,

• flat shape and low mass allow the use of dielectric antennas on fast flying objects without fear of deterioration of their aerodynamic properties.

Microstrip antennas are characterized by many interesting features. However, the single- or multi-layer dielectric substrate used favors the excitation of surface waves, outgoing waves, and guided waves, which propagate along the dielectric plane and disrupt the normal operation of the antenna.

Other disadvantages of microstrip antennas are:

• narrow operating band,

• limited power load.

These antennas allow for miniaturization of the antenna system, thus increasing its density. This causes mutual couplings that change the field distributions on aperture antennas and current distributions in linear antennas. This state of affairs, in turn, causes a change in the spatial characteristics of the antenna radiation and their input impedance.

The basic design of a microstrip antenna consists of a metallic strip printed on a thin, grounded (shield) dielectric substrate [2,3]. A single element can be powered by: a coaxial line run perpendicularly through the substrate (coaxial probe fed antenna) [4,5] or a microstrip line run on a dielectric substrate in the plane of the antenna [6,7]. This is particularly important when implementing planar antenna arrays (periodic antennas) due to the possibility of achieving high repeatability of execution and reducing production costs.

The first studies and publications concerned single radiating elements, which have large transverse dimensions of the antenna radiation characteristics and, therefore, small antenna gain. These defects can be removed by using periodic antenna arrays, taking into account their mutual coupling (through their mutual impedance).

There are several methods for determining mutual couplings in the periodic antenna wall. Among them, the mutual impedance method is a commonly used method. Within this approach, it is assumed that the antenna supply currents clearly determine the current distribution on the antenna dipoles and hence its radiation field (or scattered field) Es. We use the method of moments to determine these currents. Using the method developed by Richmond [8] for obtaining the elements of the impedance matrix based on the concept of reaction between sections of the base and testing functions. The power supply currents and voltages at the input of one antenna element (cell) are related to their counterparts at the input of the other antenna element through the mutual impedance matrix of the antenna system composed of many interacting elements. Hence, the supply currents of all system elements are related to the supply voltages of all system elements through the mutual impedance matrix.

Therefore, to determine the mutual impedance, it is necessary to know the electromagnetic field generated by the Hertz dipole, which leads to the need to know the Green’s function for the multilayer dielectric structure. We determine it by solving the non-homogeneous Helmholtz equation.

The spectral response of a periodic antenna structure placed in a dielectric homogeneous medium depends on the antenna geometry, medium parameters, incidence angle, polarization and excitation field geometry [17]. However, in many applications the above number of parameters determining the operation of the antenna is not sufficient to model its desired directional characteristics. Increasing the number of parameters of the antenna structure can be achieved by introducing a multilayer dielectric medium with a specific number of metalized periodic surfaces placed on flat boundaries between dielectric layers.

2. Surface impedance of the layered structure. reflectivity. transverse resonance conditions

2.1. Surface impedance of a layered structure

We assume the geometry of the system and the excitation independent of the y coordinate (Figure 1), thus reducing the issue to a two-dimensional problem

Surface impedance binds the appropriate components of the electromagnetic field tangential to the surface z=0 according to the formula [9]

- \underline{n} \times \underline{n} \times \underline{E} (k_{x}) = z_{0} η (k_{x}) \cdot \underline{n} \times \underline{H} (k_{x})

(1)

where:

z_{0} = \sqrt{\frac{μ_{0}}{ε_{0}}}

specific vacuum impedance z>0,

\underline{n}

vector normal to the impedance surface (Figure 1),

η (k_{x}) = [\begin{matrix} 0 & η^{e} \\ - 1 / η^{h} & 0 \end{matrix}]

relative (relative to the medium) surface impedance z=0.

For a TM type field (E-polarization) with components

\underline{H} = [0, H_{y}, 0] \underline{E} = [E_{x}, 0, E_{z}]

from the definition of surface impedance (1) we obtain

{\tilde{E}}_{x} = - z_{0} \cdot η^{e} \cdot {\tilde{H}}_{y},

(2)

from Maxwell’s equations

{\tilde{E}}_{x} = - i \cdot \frac{z_{o}}{k} \cdot \frac{\partial {\tilde{H}}_{y}}{\partial z},

(3)

by comparing expression (2) and (3) we obtain the differential impedance condition for the TM problem.

\frac{\partial {\tilde{H}}_{y}}{\partial z} + i \cdot k \cdot η^{e} \cdot {\tilde{H}}_{y} = 0 .

(4)

We assume the following form of the field at the boundary of the centers

{\tilde{H}}_{y} = e^{i \cdot (k_{x} \cdot x - k_{z} \cdot z)} + R^{e} \cdot e^{i \cdot (k_{x} \cdot x + k_{z} \cdot z)} .

(5)

From the condition of continuity (boundary) of the tangential components of the field in the z = 0 plane, we obtain the expression for the reflection coefficient

R^{e} (k_{x}) = \frac{k_{z} - k \cdot η^{e} (k_{x})}{k_{z} + k \cdot η^{e} (k_{x})} .

(6)

For a TE type field (H-polarization) with components . By definition we get

{\tilde{H}}_{x} = Y_{o} \cdot η^{h} \cdot {\tilde{E}}_{y},

(7)

and from Maxwell’s equations

{\tilde{H}}_{x} = i \cdot \frac{Y_{o}}{k} \cdot \frac{\partial {\tilde{E}}_{y}}{\partial z}

(8)

from (7) and (8) we obtain the boundary admittance condition for the TE problem

\frac{\partial {\tilde{E}}_{z}}{\partial z} + i \cdot k \cdot η^{h} \cdot {\tilde{E}}_{y} = 0 .

(9)

Assuming the field form analogous (as for the TM problem), we obtain the reflection coefficient from the condition of continuity in the z = 0 plane

R^{h} (k_{x}) = \frac{k_{z} - k \cdot η^{h} (k_{x})}{k_{z} + k \cdot η^{h} (k_{x})} .

(10)

2.2 Reflectance and surface impedance of a dielectric layer lying on a perfectly conductive plane

Figure 2. Dielectric layer with an electric shield.

We consider the TM field (E-polarization) - an even field. To determine the reflection coefficient appearing in equations (6) and (10), it should be noted that it does not depend on the angle ψ in the (x, y) plane. Thus, we can analyze the issue in the selected plane from here

y = 0

from where

ρ = x; k_{ρ} = k_{x} k_{y} = 0

For the considered TM field with components

\underline{E} = [E_{x}, 0, E_{z}] \underline{H} = [0, H_{y}, 0]

we assume the following form of the field

{\tilde{H}}_{y} (k_{x}) = \frac{I d l}{8 \cdot π^{2}} \cdot e^{i k x} \{\begin{matrix} \pm e^{\pm i \cdot k_{z} z} + R^{e} (k_{x}) e^{i k_{z} z} d l a z > - z_{o} \\ A \cdot \frac{\cos χ (z + z_{o} + b)}{\cos χ b} - z - b < z < - z_{o} . \end{matrix}

(11)

From Maxwell’s equations we get:

{\tilde{E}}_{x} = - \frac{i}{ω \cdot ε \cdot ε_{o}} \cdot \frac{\partial {\tilde{H}}_{y}}{\partial z}

(12)

ε = \{\begin{matrix} 1 f o r z > z_{o} \\ ε (r e l a t i v e) - z_{o} - b < z < - z_{o} . \end{matrix}

(13)

Meaning that

{\tilde{E}}_{x} (k_{x}) = - \frac{i}{ω \cdot ε_{o}} \cdot \frac{I d l}{8 \cdot π^{2}} \cdot e^{i \cdot k_{x} x} \{\begin{matrix} i k_{z} e^{\pm i \cdot k_{z} z} + R^{e} (k_{x}) \cdot e^{i \cdot k_{z} z} d l a z > - z_{o} \\ - A \cdot \frac{χ}{ε} \cdot \frac{\sin χ (z + z_{o} + b)}{\cos χ b} - z_{o} - b < z < - z_{o} \end{matrix}

(14)

where:

χ (k_{x}) = χ (k_{ρ}) = \sqrt{k^{2} \cdot n^{2} - k_{ρ}^{2}} .

(15)

Equations (11) and (12) take into account the condition of zeroing of the electric field component on the screen

z = - z_{o} - b

. We will call the obtained solution an even TM field, i.e., symmetrical with respect to the plane of the screen (perfectly conductive).

From the conditions of continuity of components in the plane we obtain:

i \cdot k_{z} (e^{i \cdot k_{z} \cdot z} + R_{p}^{e} (k_{x}) \cdot e^{- i \cdot k_{z} \cdot z_{o}}) = - A \cdot \frac{χ}{ε} \cdot tg (χ \cdot b) .

(16)

Hence the reflection coefficient for the even field TM

R_{p}^{e} (k_{ρ}) = - e^{2 \cdot i \cdot k_{z} \cdot z} \cdot \frac{k_{z} + i \frac{χ}{ε} tg (χ \cdot b)}{k_{z} - i \frac{χ}{ε} tg (χ \cdot b)} = - e^{2 \cdot i \cdot k_{z} \cdot z} \cdot \frac{k_{z} - k \cdot η_{p}^{e} (k_{ρ})}{k_{z} + k \cdot η_{p}^{e} (k_{ρ})}

(17)

and surface impedance

η_{p}^{e} (k_{ρ}) = - i \cdot \frac{χ}{k \cdot ε} tg (χ \cdot b) .

(18)

2.3 Odd TE reflection coefficient, surface impedance

We consider the TE (H-polarization) odd field. We are analyzing the problem

in the plane from here

x = 0

ρ = y, k_{ρ} = k_{y}

. For the considered field TE with components

\underline{E} = [E_{x}, 0,0] \underline{H} = [0, H_{y}, H_{z}]

we adopt its following representation.

{\tilde{E}}_{x} (k_{y}) = - \cdot \frac{I d l}{8 \cdot π^{2}} \cdot ω \cdot μ_{0} \cdot \frac{1}{k_{z}} e^{i \cdot k_{y} y} \{\begin{matrix} e^{\pm i \cdot k_{z} z} + {R_{n}}^{(h)} (k_{y}) \cdot e^{i \cdot k_{z} z} d l a z > - z_{o} \\ A \cdot \frac{\sin χ (z + z_{o} + b)}{\sin (χ b)} - z_{o} - b < z < - z_{o} \end{matrix}

(19)

from Maxwell’s equations for the medium

{\tilde{H}}_{x} = - \frac{i}{ω \cdot μ \cdot μ_{o}} \cdot \frac{\partial {\tilde{E}}_{x}}{\partial z}

(20)

μ = \{\begin{matrix} 1 d l a z > z_{o} \\ μ - z_{o} - b < z < - z_{o} \end{matrix}

(21)

{\tilde{H}}_{x} (k_{y}) = - i \cdot \frac{I d l}{8 \cdot π^{2}} \cdot \frac{1}{k_{z}} e^{i \cdot k_{y} y} \{\begin{matrix} (\pm e^{\pm i \cdot k_{z} \cdot z} + {R_{n}}^{(h)} (k_{y}) \cdot e^{i \cdot k_{z} \cdot z}) \cdot i \cdot k_{z} \\ A \cdot \frac{χ}{μ} \cdot \frac{\cos χ (z + z_{o} + b)}{\sin (χ \cdot b)} . \end{matrix}

(22)

Considering the condition of zeroing of the electric field component E_x on the screen

z = - z_{0} b

and the continuity of the electromagnetic field components Ex, H_y in the plane

z = - z_{0}

, we obtain

(e^{i \cdot k_{z} \cdot z} + R_{n}^{h} (k_{y})) e^{- i \cdot k_{z} \cdot z_{o}} = A

(23)

i \cdot k_{z} (- e^{+ i \cdot k_{z} \cdot z} + R_{n}^{h} (k_{y}) e^{- i \cdot k_{z} \cdot z_{o}}) = A \cdot \frac{χ}{μ} \cdot ctg (χ \cdot b)

(24)

Hence, the reflection coefficient for the odd field and TE polarization is described by the relationship.

R_{n}^{h} (k_{ρ}) = e^{2 \cdot i \cdot k_{z} \cdot z} \frac{k_{z} - k \cdot η_{n}^{h} \cdot (k_{ρ})}{k_{z} + k \cdot η_{n}^{h} (k)}

(25)

and surface admittance

η_{n}^{h} (k_{ρ}) = \frac{i}{k} \cdot \frac{χ}{μ} \cdot ctg (χ \cdot b) .

(26)

2.4. Reflection coefficient for TE field (H-polarization) even field, surface impedance

We analyze the issue in the plane, hence

x = 0, k_{x} = 0

, stąd

ρ = y, k_{ρ} = k_{y}

Figure 3. Dielectric layer with a magnetic screen.

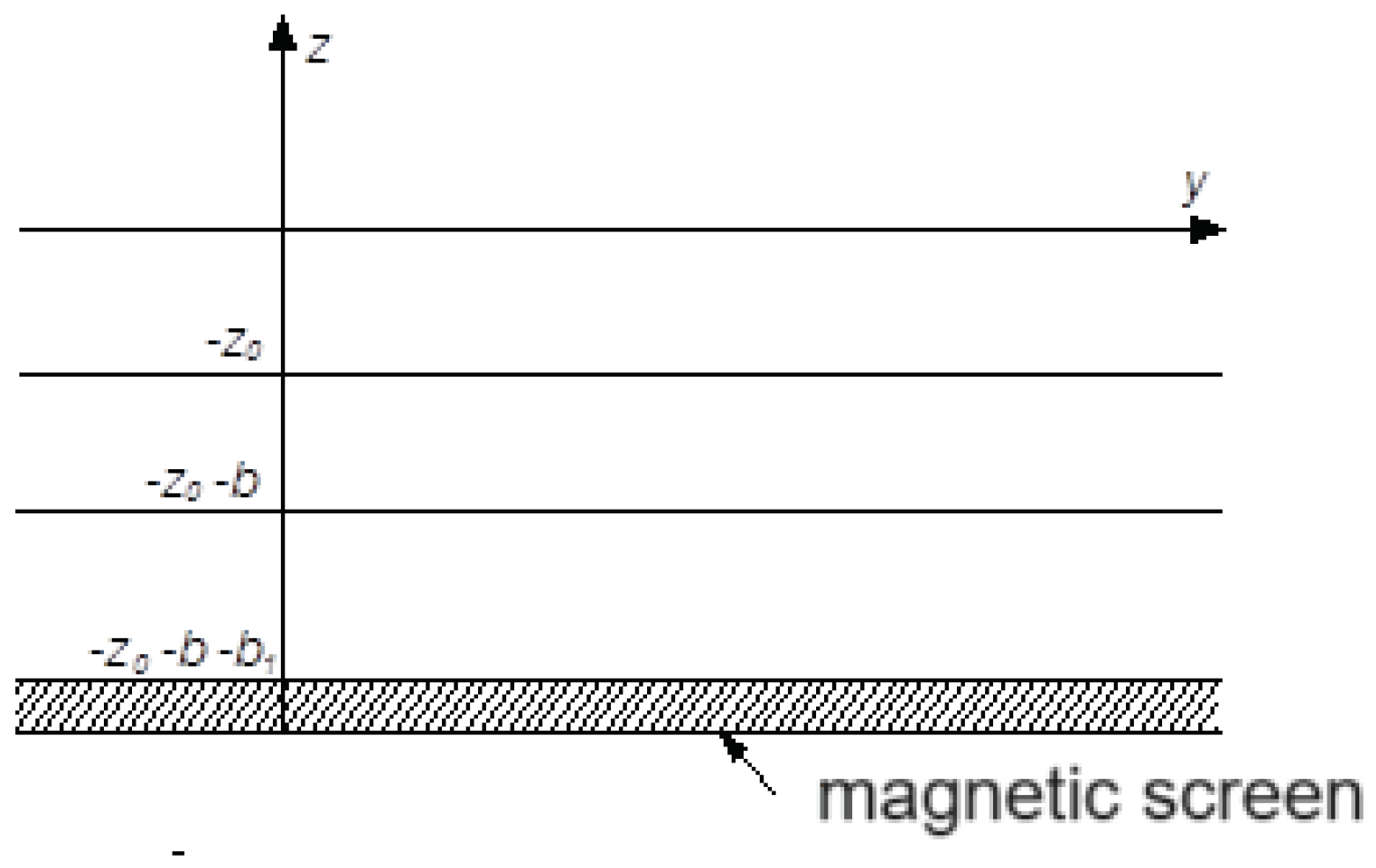
For the considered TE field with components:

\underline{E} = [E_{x}, 0,0] i \underline{H} = [0, H_{y}, H_{z}]

We adopt the following representation.

{\tilde{E}}_{x} (k_{y}) = + \frac{I d l}{8 \cdot π^{2}} \cdot ω \cdot μ_{0} \cdot \frac{e^{i \cdot k_{y} \cdot y}}{k_{z}} \{\begin{matrix} e^{\pm i \cdot k_{z} z} + R_{p}^{h} (k_{y}) \cdot e^{i \cdot k_{z} \cdot z} d l a z > - z_{o} \\ A \cdot \frac{\cos χ (z + z_{o} + b)}{\cos (χ \cdot b)} - z_{o} - b < z < - z_{o} \end{matrix}

(27)

from Maxwell’s equation for the medium

{\tilde{H}}_{y} = - \frac{i}{ω \cdot μ \cdot μ_{0}} \cdot \frac{\partial {\tilde{E}}_{x}}{\partial z}

(28)

μ = \{\begin{matrix} 1 z > - z_{0} \\ μ_{r} z_{0} - b < z < z_{0} . \end{matrix}

(29)

In numerical calculations, we assume μ=1 for a perfect dielectric

{\tilde{H}}_{y} (k_{y}) = - i \cdot \frac{I d l}{8 \cdot π^{2}} \cdot \frac{e^{i \cdot k_{y} \cdot y}}{k_{z}} \{\begin{matrix} \pm e^{\pm i \cdot k_{z} z} + R_{p}^{h} (k_{y}) \cdot e^{i \cdot k_{z} \cdot z} d l a z > - z_{o} \\ - A \cdot \frac{χ}{μ} \cdot \frac{\sin χ (z + z_{o} + b)}{\cos (χ \cdot b)} - z_{o} - b < z < - z_{o} . \end{matrix}

(30)

Considering the condition of zeroing of the magnetic field component on the screen

z = - z_{0} - b

and the continuity of the electromagnetic field components E_x, H_y in the plane

z = - z_{0}

, we obtain

e^{i \cdot k_{z} \cdot z} + R_{p}^{h} (k_{y}) = A

(31)

i \cdot k_{z} (- e^{i \cdot k_{z} \cdot z} + R_{p}^{h} (k_{y}) \cdot e^{- i \cdot k_{z} \cdot z} = - A \cdot \frac{χ}{μ} \cdot tg (χ \cdot b)

(31)

Hence, the reflection coefficient for the even TE field is described by the expression

R_{p}^{h} = e^{2 \cdot i \cdot k_{z} \cdot z} \frac{k_{z} + i \cdot \frac{χ}{μ} \cdot tg (χ \cdot b)}{k_{z} - i \cdot \frac{χ}{μ} \cdot tg (χ \cdot b)} = e^{2 \cdot i \cdot k_{z} \cdot z} \frac{k_{z} - k \cdot η_{p}^{h} \cdot (k_{ρ})}{k_{z} + k \cdot η_{p}^{h} \cdot (k_{ρ})}

(32)

and surface admittance

η_{p}^{h} (k_{ρ}) = - \frac{i}{k} \cdot \frac{χ}{μ} \cdot tg (χ b) .

(33)

2.5. Reflection coefficient for the TM field (E - polarization), odd field, surface impedance.

Carrying out analogous considerations as in the previous points, we obtain:

R_{n}^{e} = - e^{2 \cdot i \cdot k_{z} \cdot z} \frac{k_{z} - k \cdot η_{n}^{e} \cdot (k_{ρ})}{k_{z} + k \cdot η_{n}^{e} \cdot (k_{ρ})}

(35)

η_{n}^{e} (k_{ρ}) = \frac{i}{k} \cdot \frac{χ}{ε} \cdot ctg (χ \cdot b) .

(36)

2.6. Transverse resonance conditions for the dielectric layer on the screen - surface wave poles for the TM and |TE fields

Zeroing of the denominator of the reflection coefficient R(k_ρ) for a specific polarization and field symmetry

k_{z} + k \cdot η^{e (h)} (k_{ρ}) = 0

(37)

gives us the condition for transverse resonance of the dielectric layer, now treated as a waveguide structure. The solution of this condition determines first order pole type singularities in the integral expressions in the field representation. Singularities of this type located on the physical Rieman lobe Im(k_z)>0 correspond to surface waves carried by an open waveguide structure. For H polarization we obtain the following resonance conditions for the even part of the field

k_{z} - i \cdot \frac{χ}{μ} \cdot tg (χ \cdot b) =

(38)

for the odd part of the field

k_{z} + i \cdot \frac{χ}{μ} \cdot ctg (χ \cdot b) = 0 .

(39)

For E- polarization for the even part of the field

k_{z} - i \cdot \frac{χ}{ε} \cdot tg (χ \cdot b) = 0

(40)

for the odd part of the field

k_{z} + i \cdot \frac{χ}{ε} \cdot ctg (χ \cdot b) = 0 .

(41)

The occurrence of this phenomenon should be taken into account when analyzing linear antennas on a dielectric substrate.

2.7. Reflection coefficient, surface impedance for the dielectric layer at a distance b from the perfectly conducting plane (screen)

We analyze the system presented in Figure 4. We consider the TM field (E polarization), an even field with a component

\underline{E} = [E_{x} 0, E_{z}] \underline{H} = [0, H_{y}, 0]

We analyze the problem in the y=0 plane, hence ρ=x , k_ρ=k_y. We assume the following field representation

{\tilde{H}}_{y}

{\tilde{H}}_{y} (k_{x}) = \frac{I \cdot d l}{8 \cdot π^{2}} e^{i \cdot k_{x} \cdot x} \{\begin{matrix} (1 + R^{e} (k_{x})) \cdot e^{i \cdot k_{z} \cdot z} z > - z_{0} \\ - e^{- i \cdot k_{z} \cdot z} + R^{e} (k_{x})) \cdot e^{i \cdot k_{z} \cdot z} - z_{0} < z < 0 \\ \frac{A (k_{x})}{\sin (χ \cdot b)} {\sin χ (z + z_{0} + b) - f (k_{x}) \sin χ (z + z_{0})} - z_{o} - b < z < - z_{0} \\ A (k_{x}) f (k_{x}) \frac{\cos k_{z} (z + z_{0} + b + b_{1})}{\cos (k_{z} \cdot b)} - z_{0} - b - b_{1} < z < - z - b . \end{matrix}

(42)

Using Maxwell’s equations for medium III

{\tilde{E}}_{x} (k_{x}) = - \frac{i}{ω \cdot ε \cdot ε_{o}} \cdot \frac{\partial {\tilde{H}}_{y}}{\partial z}

(43)

we designate:

{\tilde{E}}_{x} (k_{x}) = \frac{- i \cdot I \cdot d l \cdot e^{i \cdot k_{x} \cdot x}}{8 \cdot π^{2} \cdot ω \cdot ε_{0}} \{\begin{matrix} i \cdot k_{z} (1 + R^{e} (k_{x})) \cdot e^{i \cdot k_{z} \cdot z} z > - z_{0} \\ i k_{z} [e^{- i \cdot k_{z} \cdot z} + R^{e} (k_{x})] \cdot e^{i \cdot k_{z} \cdot z} - z_{0} < z < 0 \\ \frac{A (k_{x}) [χ \cos χ (z + z_{0} + b) - f (k_{x}) χ \cos χ (z + z_{0})]}{\sin (χ \cdot b)} - z_{o} - b < z < - z_{0} \\ A (k_{x}) \cdot f (k_{x}) \cdot \frac{k_{z} \sin k_{z} (z + z_{0} + b + b_{1})}{\cos (k_{z} \cdot b_{1})} - z_{0} - b - b_{1} < z < - z - b \end{matrix}

(44)

where:

f (x) = \frac{χ}{ε \cdot \sin (χ \cdot b)} \cdot {\{\frac{χ}{ε} \cdot ctg (χ \cdot b) - k_{z} \cdot tg (k_{z} \cdot b_{1})\}}^{- 1} .

(45)

Taking into account the continuity of the field

{\tilde{H}}_{y} i {\tilde{E}}_{x}

in the i plane

z = - z_{0} - b

and

z = z_{0}

, we obtain an expression for the reflection coefficient

R^{e} (k_{ρ}) = - e^{- 2 \cdot i \cdot k_{z} \cdot z_{0}} \cdot \frac{k_{z} - k \cdot η^{e} (k_{ρ})}{k_{z} + k η^{e} (k_{ρ})}

(46)

and surface impedance

η_{e} (k_{ρ}) = η_{n}^{e} \cdot \frac{η_{p}^{e} + η_{o p}}{η_{n}^{e} + η_{o p}}

(47)

where : are

η_{p}^{e} a n d η_{n}^{e}

described by dependencies (18) and (36), respectively

η_{o p} (k_{ρ}) = - i \cdot \frac{k_{z}}{k} \cdot tg (k_{z} \cdot b_{1}) .

(48)

The condition of transverse resonance is expressed by the following relationship:

k_{z} + k \cdot η^{e} = \frac{η_{o p} (k_{ρ}) (k_{z} + k \cdot η_{n}^{e}) + η_{m}^{e} (k_{z} + k \cdot η_{n}^{e})}{η_{n}^{e} + η_{o p}}

(49)

2.8. Reflectance and surface impedance of the TE field (H-polarization) even field

We analyze the system shown in Figure 5

We consider the TE field - an even field with components

\underline{E} = [E_{x}, 0,0] i \underline{H} = [0, H_{y}, H_{z}]

We assume the following field representation ρ=y , k_ρ=k_y. We assume the following field representation E_x(k_y)

{\tilde{E}}_{x} (k_{y}) = \frac{I \cdot d l}{8 \cdot π^{2}} ω μ_{0} {\frac{e}{k_{z}}}^{i \cdot k_{y} \cdot y} \{\begin{matrix} (1 + R^{h} (k_{y})) \cdot e^{i \cdot k_{z} \cdot z} z > - z_{0} \\ - e^{- i \cdot k_{z} \cdot z} + R^{h} (k_{y})) \cdot e^{i \cdot k_{z} \cdot z} - z_{0} < z < 0 \\ A (k_{y}) \frac{\{\sin χ (z + z_{0} + b) - g (k_{y}) \sin χ (z + z_{0})\}}{\sin (χ \cdot b)} - z_{o} - b < z < - z_{0} \\ A (k_{y}) \cdot g (k_{y}) \cdot \frac{\cos k_{z} (z + z_{0} + b + b_{1})}{\cos (k_{z} \cdot b_{1})} - z_{0} - b - b_{1} < z < - z - b . \end{matrix}

(50)

They use Maxwell’s equations (28) to calculate the area

{\tilde{H}}_{y} (k_{y})

{\tilde{H}}_{y} (k_{y x}) = \frac{- i I \cdot d l}{8 \cdot π^{2}} \frac{e^{i \cdot k_{x} \cdot x}}{k_{z}} \{\begin{matrix} i \cdot k_{z} (1 + R^{h} (k_{y x})) \cdot e^{i \cdot k_{z} \cdot z} z > - z_{0} \\ i k_{z} [e^{- i \cdot k_{z} \cdot z} + R^{h e} (k_{y x})] \cdot e^{i \cdot k_{z} \cdot z} - z_{0} < z < 0 \\ \frac{A (k_{y}) χ \cos χ (z + z_{0} + b) - g (k_{y x}) χ \cos χ (z + z_{0})}{\sin (χ \cdot b)} - z_{o} - b < z < - z_{0} \\ A (k_{y}) \cdot g (k_{y}) \cdot \frac{k_{z} \sin k_{z} (z + z_{0} + b + b_{1})}{\cos (k_{z} \cdot b_{1})} - z_{0} - b - b_{1} < z < - z - b . \end{matrix}

(41)

where:

g (k_{y}) = \frac{χ}{μ \cdot \sin (χ \cdot b)} \cdot \frac{χ}{μ} \cdot {\{ctg (χ \cdot b) - k_{z} \cdot tg (k_{z} \cdot b_{1})\}}^{- 1}

(52)

Taking into account field continuity

{\tilde{H}}_{y}, {\tilde{E}}_{x}

in the plane

z = - z_{0} - b

z = z_{0}

we obtain an expression for the reflectance coefficient

R^{h} (k_{ρ}) = e^{+ 2 \cdot i \cdot k_{z} \cdot z_{0}} \cdot \frac{k_{z} - k \cdot η^{h} (k_{ρ})}{k_{z} + k η^{h} (k_{ρ})}

(53)

and surface admittance of the surface structure

η^{h} (k_{ρ}) = η_{n}^{h} \cdot \frac{η_{p}^{h} + η_{o p}}{η_{n}^{h} + η_{o p}}

(54)

The resonance condition is expressed by the following relationship:

k_{z} + k \cdot η^{h} = \frac{η_{o p} (k_{z} + k \cdot η_{n}^{h}) + η_{n}^{h} (k_{z} + k \cdot η_{p}^{h})}{η_{n}^{h} + η_{o p}} = 0 .

(55)

2.8. Reflectance coefficient and surface impedance for TE fields (H - polarization) odd field and TM fields (E - polarization) odd field

Carrying out the considerations as in the previous section, we obtain an odd field for the TE field (H-polarization).

Reflectance coefficient

R^{h} (k_{ρ}) = e^{2 \cdot i \cdot k_{z} \cdot z} \frac{k_{z} - k \cdot η^{h} (k_{ρ})}{k_{z} + k \cdot η^{h} (k_{ρ})}

(56)

surface admittance

η^{h} = η_{n}^{h} \cdot \frac{η_{p}^{h} + η_{o n}}{η_{n}^{h} + η_{o n}}

(57)

where

η_{o n} = i \frac{k_{z}}{k} ctg (k_{z} \cdot b_{1}),

(58)

η_{n}^{h} = i \frac{χ}{k} ctg (χ \cdot b),

(59)

η_{p}^{h} = - i \frac{χ}{k} tg (χ \cdot b) .

(60)

Resonance equation

k_{z} + k \cdot η^{h} = \frac{η_{o n} (k_{z} + k \cdot η_{n}^{h}) + η_{n}^{h} (k_{z} + k \cdot η_{p}^{h})}{η_{n}^{p} + η_{o p}}

(61)

and for E polarization

R^{e} (k_{ρ}) = e^{2 i \cdot k_{z} \cdot z_{0}} \frac{k_{z} - k \cdot η^{e} (k_{ρ})}{k_{z} + k \cdot η^{e} (k_{ρ})}

(62)

surface impedance

η^{e} = η_{n}^{e} \frac{η_{p}^{e} + η_{o n}}{η_{n}^{e} + η_{o n}}

(63)

where:

η_{o n}, η_{p}^{e}, η_{n}^{e}

are described by dependencies (58), (18) and (26), respectively. And the resonance equation has the form:

k_{z} + k \cdot η^{e} = \frac{η_{o n} (k_{z} + k \cdot η_{p}^{e}) + η_{n}^{e} (k_{z} + k \cdot η_{p}^{e})}{η_{n}^{e} + η_{o n}} = 0 .

(64)

3. Distribution coefficients Generalized impedance z_mn

There are two complementary approaches to the analysis of an antenna structure placed in a dielectric homogeneous medium. In the first, the composite antenna system is analyzed by constructing supermodes of the entire structure, in the second, the system is considered as a cascade assembly of flat discrete elements, i.e. boundaries between two dielectrics, periodic metalized planes and dielectric layers [10,11]. The second approach leads to the definition of the scattering matrix, transmission or impedance of the entire structure by cascading appropriate matrices assigned to individual discrete elements of the antenna structure. It is particularly useful in modeling dielectric multilayer antenna walls, where the stored data regarding one planar antenna element can be repeatedly used in the analysis of various antenna systems with modified parameters of other discrete elements of the structure [18,19]. We consider the composite periodic antenna structure shown in Figure 6. The system consists of many layered elements limited at the top and bottom by half-spaces (upper and lower) of homogeneous dielectric media. The third axis (y) of the orthogonal coordinate system (x,y,z) is perpendicular to the drawing plane. The basic components of the structure are: a metalized, infinitely periodic in the (x, y) plane, an antenna surface, a boundary between two dielectrics and a homogeneous dielectric layer.

After determining the Green’s function for the adopted form of the base current, further considerations come down to presenting the generalized impedance in the form of a double integral, where the integrating function will be presented in an explicit form [12]. We obtain the following expression describing the generalized impedance:

z_{m n} = - {(2 π)}^{- 4} \int_{- \infty}^{\infty} \int_{- \infty}^{\infty} \int_{- \infty}^{\infty} \int_{- \infty}^{\infty} \int_{- \infty}^{\infty} \int_{- \infty}^{\infty} \{F [G (k_{x} - k_{x}^{’}; k_{y} - k_{y}^{’})] F [J_{o} (k_{x} - k_{x}^{’})] \cdot

F [J_{o} (k_{x} - k_{x}^{’})] \cdot F [J_{o} (k_{x})] e^{- i (k_{x} - k_{x}^{’}) x_{m} - k_{x}^{’} x_{n} - i (k_{y} - k_{y}^{’}) y_{m} - i k_{y}^{’} y_{n} + i k_{x} x + i k_{y} y} d k_{y}^{’} d k_{x}^{’} d k_{y} d k_{x} d y d x

(65)

The integral described by expression (66) converges slowly, moreover, it contains poles related to the occurrence of a surface wave and requires great caution when calculating in the vicinity of the source [20]. There are two ways to calculate the integral. When calculating in the spatial domain, the spectral variables k_x and k_y are converted into variables in the polar coordinate systemϕ and K. Using the properties of the spectral Green’s functions, integration over the variable Preprints 99982 i001

is performed analytically. The integral (66) therefore reduces to the form:

\int_{x} \int_{y} \int_{x_{0}} \int_{y_{0}} \int_{0}^{\infty} J_{m} (x) G (x, y, x_{0}, y_{0}, K) J_{n} (x_{0}, y_{0}) d K d y_{0} d x_{0} d y d x

(66)

By integrating the function G over the variable K, we obtain the field at point (x, y) produced by the source placed at point (x_o, y_o), hence the method is similar to the standard method of moments used to solve linear antennas [13] and can be used in calculations previously learned and developed techniques (e.g. use of Toeplitz matrix symmetry). The main inconvenience of this method, which makes the calculation of antennas with very thin substrates very difficult or even impossible, is the singularity that occurs when the point (x,y) approaches the source in the integration process. Despite this, it is used by some authors [14].

The second approach to calculating the integral requires moving to the spectral domain. The integration over the spatial variables in (66) can then be performed analytically. Occurrence. exponential factors means the Fourier transform of the functions J_m and J_n. Then the integral (66) reduces to

\int_{- \infty}^{\infty} \int_{- \infty}^{\infty} F_{m} (k_{x}, k_{y}) \underline{G} (k_{x}, k_{y}) F_{n} (k_{x}, k_{y}) d k_{y} d k_{x}

(57)

where F_m and F_n are the Fourier transforms of the basis and test functions, respectively.

This integral is calculated numerically, but it is the most time-consuming process due to the slow convergence of the integral [15,16]. Slow convergence is caused by the fact that the singularities of the integral in the spatial domain have been removed by converting the domain to a spectral one and have become "scattered" in this domain. Calculating the integral (66) in the spectral domain allows it to be easily extended to the case of an infinite array of antennas [21]. Further analysis is carried out in the spectral domain.We wzorach tych are the reflectance coefficients described by the relations:

for single-layer system (18, 26, 33, 35)
for a multi-layer system, dependencies (18, 26, 33, 36, 48, 58)

In the above expressions (based on the solution of equations (38÷41), the quantity k_z may assume purely imaginary values. This is due to the fact that the dielectric substrate is non-lossy

tg δ \leq 1 0^{- 4}

But the size

ε_{0}, μ_{0}, ε, μ, x_{m} - x_{n} . y_{m} - y_{n}, k_{e f}, k_{n} k, L_{N}, b, i ω

are real, non-negative parameters that change discreetly within certain ranges.

We will now examine the behavior of the integrand functions in the above formulas. Studies of the integrand functions appearing in the formulas indicate the existence of singular points of the type - branches for:

\sqrt{k^{2} - k_{x}^{2} - k_{y}^{2}} = 0

\sqrt{k^{2} n^{2} - k_{x}^{2} - k_{y}^{2}} = 0

(68)

-: apparent pole for:

k_{x}^{2} - k_{e f}^{2} = 0

(this is a removable singularity)

-: isolated poles (which may occur)

for 1 + \frac{ε \sqrt{k^{2} - k_{x}^{2} - k_{y}^{2}} - \sqrt{k^{2} n^{2} - k_{x}^{2} - k_{y}^{2}}}{ε \sqrt{k^{2} - k_{x}^{2} - k_{y}^{2}} + \sqrt{k^{2} n^{2} - k_{x}^{2} - k_{y}^{2}}} e^{2 i b_{1} \sqrt{k^{2} n - k_{x}^{2} - k_{y}^{2}}} = 0

(69)

for 1 - \frac{μ \sqrt{k^{2} - k_{x}^{2} - k_{y}^{2}} - \sqrt{k^{2} n^{2} - k_{x}^{2} - k_{y}^{2}}}{μ \sqrt{k^{2} - k_{x}^{2} - k_{y}^{2}} + \sqrt{k^{2} n^{2} - k_{x}^{2} - k_{y}^{2}}} e^{2 i b_{1} \sqrt{k^{2} n - k_{x}^{2} - k_{y}^{2}}} = 0

(6)

hence, after transformation, we get that for

k^{2} - k_{x}^{2} - k_{y}^{2} > 0 or k^{2} n^{2} - k_{x}^{2} - k_{y}^{2} < 0

(7)

poles do not exist.

It is possible for a pole to occur only for

(k n)^{2} - k_{x}^{2} - k_{y}^{2} > 0 i k^{2} - k_{x}^{2} - k_{y}^{2} < 0 (k < |\sqrt{k_{x}^{2} + k_{y}^{2}}| < k n)

(8)

In order to determine the value of isolated poles for

R_{p}^{e}

and

R_{n}^{h}

, the relationship should be examined:

R_{p}^{e} : 1 + \frac{ε \sqrt{k^{2} - k_{x}^{2} - k_{y}^{2}} - \sqrt{k^{2} n^{2} - k_{x}^{2} - k_{y}^{2}}}{ε \sqrt{k^{2} - k_{x}^{2} - k_{y}^{2}} + \sqrt{k^{2} n^{2} - k_{x}^{2} - k_{y}^{2}}} e^{2 i b_{1} \sqrt{k^{2} n - k_{x}^{2} - k_{y}^{2}}}

(9)

R_{n}^{h} : 1 - \frac{μ \sqrt{k^{2} - k_{x}^{2} - k_{y}^{2}} - \sqrt{k^{2} n^{2} - k_{x}^{2} - k_{y}^{2}}}{μ \sqrt{k^{2} - k_{x}^{2} - k_{y}^{2}} + \sqrt{k^{2} n^{2} - k_{x}^{2} - k_{y}^{2}}} e^{2 i b_{1} \sqrt{k^{2} n - k_{x}^{2} - k_{y}^{2}}} = 0

(10)

From the above it follows that if

\begin{matrix} \begin{matrix} a) k^{2} - k_{x}^{2} - k_{y}^{2} > 0 \\ b) k^{2} n^{2} - k_{x}^{2} - k_{y}^{2} < 0 \end{matrix}\} \\ c) k^{2} - k_{x}^{2} - k_{y}^{2} < 0 i k^{2} n^{2} - k_{x}^{2} - k_{y}^{2} > 0 \end{matrix}

(11)

If conditions a) and b) are met, the poles do not occur, but if condition c) is met, then the necessary condition for the presence of poles is met.

Let us therefore examine the sufficient condition from the condition mentioned above (74) point. c it follows that:

k < |\sqrt{k_{x}^{2} + k_{y}^{2}}| < k n

(12)

For the situation under consideration, the equations will be written in the form:

R_{p}^{e} : 1 + \frac{i ε \sqrt{k_{x}^{2} + k_{y}^{2} - k^{2}} - \sqrt{k^{2} n^{2} - k_{x}^{2} - k_{y}^{2}}}{i ε \sqrt{k_{x}^{2} + k_{y}^{2} - k^{2}} + \sqrt{k^{2} n^{2} - k_{x}^{2} - k_{y}^{2}}} e^{2 i b_{1} \sqrt{k^{2} n^{2} - k_{x}^{2} - k_{y}^{2}}} = 0

(13)

R_{n}^{h} : 1 - \frac{i μ \sqrt{k_{x}^{2} + k_{y}^{2} - k^{2}} - \sqrt{k^{2} n^{2} - k_{x}^{2} - k_{y}^{2}}}{i μ \sqrt{k_{x}^{2} + k_{y}^{2} - k^{2}} + \sqrt{k^{2} n^{2} - k_{x}^{2} - k_{y}^{2}}} e^{2 i b_{1} \sqrt{k^{2} n^{2} - k_{x}^{2} - k_{y}^{2}}} = 0

(14)

Hence, after transformations we have:

1 + e^{- 2 i a r c t g} (\frac{ε \sqrt{k_{x}^{2} + k_{y}^{2} - k^{2}}}{\sqrt{k^{2} n^{2} - k_{x}^{2} - k_{y}^{2}}}) e^{2 i b_{1} \sqrt{k^{2} n^{2} - k_{x}^{2} - k_{y}^{2}}} = 0

(15)

1 - e^{- 2 i a r c t g} \frac{μ \sqrt{k_{x}^{2} + k_{y}^{2} - k^{2}}}{\sqrt{k^{2} n^{2} - k_{x}^{2} - k_{y}^{2}}} e^{2 i b_{1} \sqrt{k^{2} n^{2} - k_{x}^{2} - k_{y}^{2}}} = 0

For a multilayer system, the equation determining the singularities (poles) of the function takes the form:-

dla R^{e} \to \infty tg χ b_{1} = \frac{2 χ k_{z} ε}{(χ^{2} + ε {|k_{z}|}^{2}) (1 + \frac{χ^{2} - ε^{2} {|k_{z}|}^{2}}{χ^{2} + ε^{2} {|k_{z}|}^{2}}) e^{- 2 b_{2} |k_{z}|}} \Rightarrow k_{ρ}^{2} = x^{e} is a \sin gular point : k^{2} < x^{e} < k^{2} \cdot n^{2}

(16)

- for

R^{h} \to \infty

tg χ b_{1} = \frac{2 χ |k_{z}| μ}{(χ^{2} + μ^{2} {|k_{z}|}^{2}) (1 - \frac{χ^{2} - μ_{r}^{2} {|k_{z}|}^{2}}{χ^{2} + μ_{r}^{2} {|k_{z}|}^{2}}) e^{- 2 b_{2} |k_{z}|}} \Rightarrow k_{ρ}^{2} = x^{h} is a \sin gular point : k^{2} < x^{h} < k^{2} \cdot n^{2} |k_{z}| = |\sqrt{k_{ρ}^{2} - k^{2}}|

(17)

The remaining patterns remain as for a single-layer system.

The appropriate branches of the elements are specified in individual ranges

in the following way:1⁰

dla k_{ρ}^{2} > k^{2} n^{2} > k^{2} k_{z} = \pm i \sqrt{k_{ρ}^{2} - k^{2}} χ = \pm i \sqrt{k_{ρ}^{2} - k^{2} n^{2}} R^{e, h} = - e^{- 2 z \sqrt{k_{p}^{2} - k^{2}}} = \frac{e^{- 2 b \sqrt{k_{p}^{2} - k^{2} n^{2}}} + η_{e, h}}{1 + η_{e, h} e^{- 2 b \sqrt{k_{p}^{2} - k^{2} n^{2}}}}

(18)

η_{e} = \frac{ε \sqrt{k_{p}^{2} - k^{2}} - \sqrt{k_{p}^{2} - k^{2} n^{2}}}{ε \sqrt{k_{p}^{2} - k^{2}} + \sqrt{k_{p}^{2} - k^{2} n^{2}}} η_{h} = \frac{μ \sqrt{k_{p}^{2} - k^{2}} - \sqrt{k_{p}^{2} - k^{2} n^{2}}}{μ \sqrt{k_{p}^{2} - k^{2}} + \sqrt{k_{p}^{2} - k^{2} n^{2}}}

(19)

2⁰

2^{0} for k^{2} n^{2} > k_{ρ}^{2} > k^{2} k_{ρ} \in (- k n, - k) \cup (k, k n) k_{z} = \pm i \sqrt{k_{ρ}^{2} - k^{2}} κ = \pm i \sqrt{k^{2} n^{2} - k_{ρ}^{2}} R^{e, h} = - e^{- 2 z \sqrt{k_{p}^{2} - k^{2}}} = \frac{e^{2 i b \sqrt{k_{p}^{2} - k^{2} n^{2}}} + η_{e, h}}{1 + η_{e, h} e^{2 b \sqrt{k_{p}^{2} - k^{2} n^{2}}}}

(20)

η_{e} = \frac{i ε \sqrt{k_{p}^{2} - k^{2}} - \sqrt{k^{2} n^{2} - k_{p}^{2}}}{i ε \sqrt{k_{p}^{2} - k^{2}} + \sqrt{k^{2} n^{2} - k_{p}^{2}}} η_{h} = \frac{i μ \sqrt{k_{p}^{2} - k^{2}} - \sqrt{k^{2} n^{2} - k_{p}^{2}}}{i μ \sqrt{k_{p}^{2} - k^{2}} + \sqrt{k^{2} n^{2} - k_{p}^{2}}}

(21)

3^{0} dla k^{2} n^{2} > k^{2} > k_{ρ}^{2} k_{ρ} \in (- k, + k) k_{z} = \pm i \sqrt{k_{ρ}^{2} - k^{2}} κ = \pm i \sqrt{k^{2} n^{2} - k_{ρ}^{2}} R^{e, h} = - e^{- 2 z \sqrt{k_{p}^{2} - k^{2}}} = \frac{e^{2 i b \sqrt{k_{p}^{2} - k^{2} n^{2}}} + η_{e, h}}{1 + η_{e, h} e^{2 b \sqrt{k_{p}^{2} - k^{2} n^{2}}}}

(22)

η_{e} = \frac{ε \sqrt{k^{2} - k_{p}^{2}} - \sqrt{k^{2} n^{2} - k_{p}^{2}}}{ε \sqrt{k^{2} - k_{p}^{2}} + \sqrt{k^{2} n^{2} - k_{p}^{2}}} η_{h} = \frac{μ \sqrt{k^{2} - k_{p}^{2}} - \sqrt{k^{2} n^{2} - k_{p}^{2}}}{μ \sqrt{k^{2} - k_{p}^{2}} + \sqrt{k^{2} n^{2} - k_{p}^{2}}}

(23)

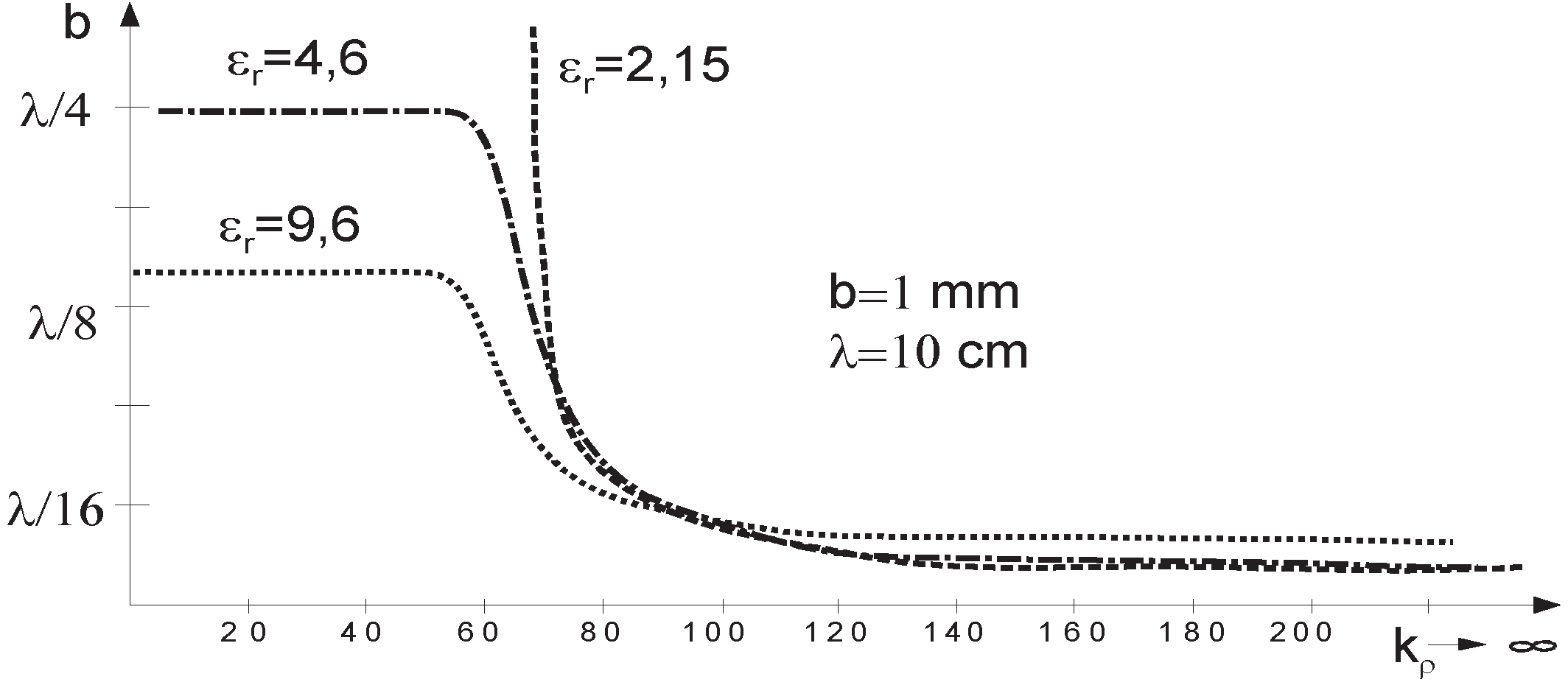
Numerical analysis of submitted above expression was made. Results of calculation for typical dielectrics are shown in Figure 7.

Conclusions

Microstrip antennas combine field and peripheral issues and require the use of analytical methods with a high degree of complexity. Therefore, at present, there are no standard methods that can be used in engineering practice. The work is a step towards filling these gaps. It covers all issues related to the analysis of coupling of arbitrarily placed linear antennas on a multilayer dielectric and generalizes the obtained results to antenna arrays.

The problem was reduced to an exact solution of the Hertzian dipole radiation for a layered system. The obtained solution was used to synthesize a solution for the case of finite dimensions of the antenna, using the standard formalism of Green’s functions, representing the field coming from a point source. These functions satisfy the inhomogeneous Helmholtz equation, the Sommerfeld radiation condition and the appropriate boundary conditions. Using Fourier transforms and their properties, an analytical expression for the generalized impedance was determined in the form of a double integral. Due to the fact that there are singular points in the integrand function, the behavior of this function was examined. The conditions for the generation of surface waves were determined. Surface waves in microstrip antennas can be eliminated by selection of base type. It can be done by minimization of

b / ε_{r}

proportion. Even three-layer dielectric system causes minimization of this proportion but it requires high-guide work. Condition of surface waves minimization is at variance with the antenna bandwidth requirements.

References

Munson R.: „Conformal microstrip antennas and microstrip phased arrays”, IEEE Transactions on Antennas and Propagation Vol. 22 No.11 November 1974. [CrossRef]
Minatti, G.; Faenzi, M.; Martini, E.; Caminita, F.; de Vita, P.; Gonz’alez-Ovejero, D.; Sabbadini, M.; Maci, S. “Modulated metasurface antennas for space: Synthesis, analysis and realizations”. IEEE Transactions on Antennas and Propagation 2014, 63, 1288–1300.
Sharawi S. M. “Printed Multi-Band MIMO Antenna Systems and Their Performance Metrics” IEEE Antennas and Propagation Magazine 2013, 218 - 232.
Oltman G.H. "Electromagnetically coupled microstrip dipoles", IEEE Transactions on Antennas and Propagation AP-29, No 1, 1981.M. N. Sadiku, “Elements of electromagnetics”. Oxford University Press, 2014.
Malaisamy K., Haridoss, Pandian A., Rima S. “Design of Crossed Dipole Yagi–Uda MIMO Antenna for Radar Applications Internationa” Journal of Antennas and Propagation 2022 Volume12.
Haobo Y, Hongguang Z , Hongwei L, Xiaojie D, and Xi C “Computing Mutual Impedance of Antennas by Spherical Harmonic Transform” IEEE Transactions on antennas and propagation, VOL. 67, NO. 7, JULY 2019 4377.
Aourik S. , Erkik A. , Oukaira A. , Dhaou S. Jamal, Z. Lakhssassi A. „An Advanced Array Configuration Antenna Based on Mutual Coupling Reduction” Electronics 2023, 12(7), 1707;
Richmond J.H., Otto D.V.: „Digital computer solutions of the rigorous equations of electromagnetics”, IEEE Transactions on Antennas and Propagation Vol.17, 1969.
Liu S., Sun S., and Chew W. C., “A potential-based integral equation method for low-frequency electromagnetic problems,” IEEE Transactions on Antennas and Propagation, vol. 66, no. 3, pp. 1413–1426, 2018.
Gao, X.; Han, X.; Cao, W.-P.; Li, H.O.; Ma, H.F.; Cui, T.J. “Ultrawideband and high-efficiency linear polarization converter based on double v-shaped metasurface”. IEEE Transactions on Antennas and Propagation. 2015, 63, 3522–3530. [Google Scholar] [CrossRef] . [CrossRef]
Cao Y. F. ; Zhang X. Y. ; Xue Q. “Compact Shared-Aperture Dual-Band Dual-Polarized Array Using Filtering Slot Antenna and Dual-Function Metasurface” IEEE Transactions on Antennas and Propagation, Publication Year: 2022, Page(s):1120 – 1131 04.
Wnuk M. “Multilayer dielectric periodic antenna structure in a cascade view” Appl. Sci. 2022, Volume 12, Issue 9, pp.1-14.
Liu, Q.S.; Sun, S.; Chew, W.C. “A potential-based integral equation method for low-frequency electromagnetic problems”. IEEE Transactions on Antennas and Propagation. 2018, 66, 1413–1426. [Google Scholar] [CrossRef. [CrossRef]
Yaghjian, A.D. “Reflections on Maxwell’s treatise.” Prog. Electromagnetic. Res. 2014, 149, 217–249.
Khaleghi S. S. M., Moradi G. , Jafargholi R. S. S. A. “Microstrip Line Impedance Matching Using ENZ Metamaterials”, Design, and Application Transactions on antennas and propagation, Vol. 67, No. 4, 2019 2243.
Radavaram S., Pour M. , “Wideband Radiation Reconfigurable Microstrip Patch Antenna Loaded With Two Inverted” IEEE Transactions on Antennas and Propagation, Vol. 67, No. 3, 2019 1501-1505.
Balanis, C.A. “Antenna Theory: Analysis and Design”; John Wiley & Sons: Hoboken, NJ, USA, 2016.
Kong, J.A. “Electromagnetic Wave Theory”; EMW Publishing: Cambridge, MA, USA, 2008.
Sadiku, M.N. “Elements of Electromagnetics”; Oxford University Press: Oxford, UK, 2014.
Bender, C.M.; Orszag, S.A. “Advanced Methods for Scientists and Engineers I: Asymptotic Methods and Perturbation Theory”; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2013.
Oppenheim, A.V.; Schafer, R.W. “Electromagnetic Field Theory Discrete-Time Signal Processing”; Pearson Education: London, UK, 2014.

Figure 1. Surface impedance.

Figure 4. Multilayer dielectric structure with an electric shield.

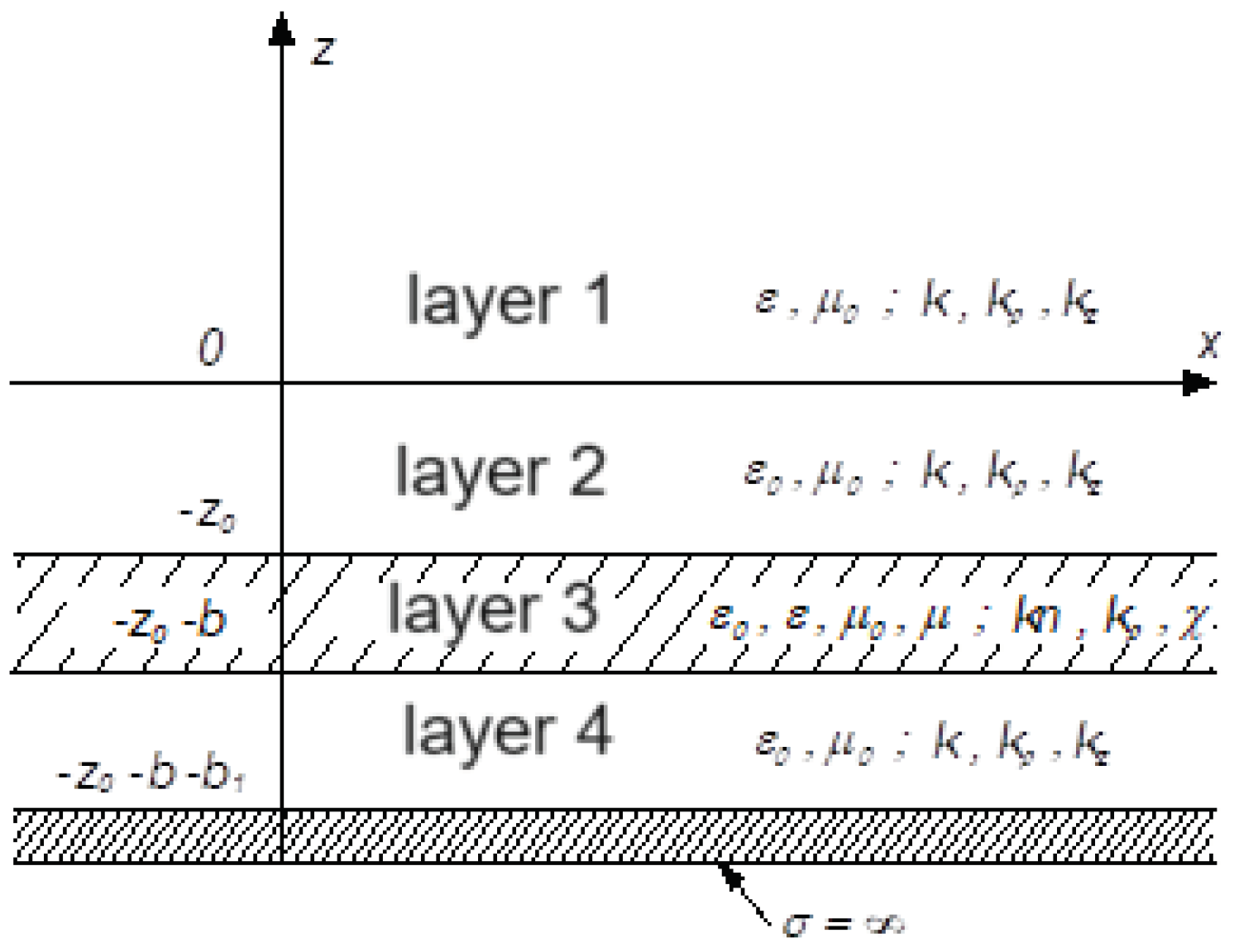
Figure 5. Multilayer dielectric structure with a magnetic screen.

Figure 6. Plane periodic antenna structure infinite in the (x,y) plane, multi-layer structure.

Figure 7. Distribution of singular points with thickness of dielectric layer.

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content.

© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).

Copyright: This open access article is published under a Creative Commons CC BY 4.0 license, which permit the free download, distribution, and reuse, provided that the author and preprint are cited in any reuse.

Submitted:

27 February 2024

Posted:

28 February 2024

You are already at the latest version

Alerts

Abstract

Keywords:

Subject: Engineering - Electrical and Electronic Engineering

1. Introduction

Microstrip antennas have many interesting features, such as:

• accurate representation on the surface,

• low manufacturing cost,

• high repeatability of workmanship,

• insignificant volume,

• masking the operating frequency,

• simplicity of production provided that relatively advanced technologies are used,

• flat shape and low mass allow the use of dielectric antennas on fast flying objects without fear of deterioration of their aerodynamic properties.

Other disadvantages of microstrip antennas are:

• narrow operating band,

• limited power load.

2. Surface impedance of the layered structure. reflectivity. transverse resonance conditions

2.1. Surface impedance of a layered structure

We assume the geometry of the system and the excitation independent of the y coordinate (Figure 1), thus reducing the issue to a two-dimensional problem

Surface impedance binds the appropriate components of the electromagnetic field tangential to the surface z=0 according to the formula [9]

- \underline{n} \times \underline{n} \times \underline{E} (k_{x}) = z_{0} η (k_{x}) \cdot \underline{n} \times \underline{H} (k_{x})

(1)

where:

z_{0} = \sqrt{\frac{μ_{0}}{ε_{0}}}

specific vacuum impedance z>0,

\underline{n}

vector normal to the impedance surface (Figure 1),

η (k_{x}) = [\begin{matrix} 0 & η^{e} \\ - 1 / η^{h} & 0 \end{matrix}]

relative (relative to the medium) surface impedance z=0.

For a TM type field (E-polarization) with components

\underline{H} = [0, H_{y}, 0] \underline{E} = [E_{x}, 0, E_{z}]

from the definition of surface impedance (1) we obtain

{\tilde{E}}_{x} = - z_{0} \cdot η^{e} \cdot {\tilde{H}}_{y},

(2)

from Maxwell’s equations

{\tilde{E}}_{x} = - i \cdot \frac{z_{o}}{k} \cdot \frac{\partial {\tilde{H}}_{y}}{\partial z},

(3)

by comparing expression (2) and (3) we obtain the differential impedance condition for the TM problem.

\frac{\partial {\tilde{H}}_{y}}{\partial z} + i \cdot k \cdot η^{e} \cdot {\tilde{H}}_{y} = 0 .

(4)

We assume the following form of the field at the boundary of the centers

{\tilde{H}}_{y} = e^{i \cdot (k_{x} \cdot x - k_{z} \cdot z)} + R^{e} \cdot e^{i \cdot (k_{x} \cdot x + k_{z} \cdot z)} .

(5)

From the condition of continuity (boundary) of the tangential components of the field in the z = 0 plane, we obtain the expression for the reflection coefficient

R^{e} (k_{x}) = \frac{k_{z} - k \cdot η^{e} (k_{x})}{k_{z} + k \cdot η^{e} (k_{x})} .

(6)

For a TE type field (H-polarization) with components . By definition we get

{\tilde{H}}_{x} = Y_{o} \cdot η^{h} \cdot {\tilde{E}}_{y},

(7)

and from Maxwell’s equations

{\tilde{H}}_{x} = i \cdot \frac{Y_{o}}{k} \cdot \frac{\partial {\tilde{E}}_{y}}{\partial z}

(8)

from (7) and (8) we obtain the boundary admittance condition for the TE problem

\frac{\partial {\tilde{E}}_{z}}{\partial z} + i \cdot k \cdot η^{h} \cdot {\tilde{E}}_{y} = 0 .

(9)

Assuming the field form analogous (as for the TM problem), we obtain the reflection coefficient from the condition of continuity in the z = 0 plane

R^{h} (k_{x}) = \frac{k_{z} - k \cdot η^{h} (k_{x})}{k_{z} + k \cdot η^{h} (k_{x})} .

(10)

2.2 Reflectance and surface impedance of a dielectric layer lying on a perfectly conductive plane

Figure 2. Dielectric layer with an electric shield.

y = 0

from where

ρ = x; k_{ρ} = k_{x} k_{y} = 0

For the considered TM field with components

\underline{E} = [E_{x}, 0, E_{z}] \underline{H} = [0, H_{y}, 0]

we assume the following form of the field

{\tilde{H}}_{y} (k_{x}) = \frac{I d l}{8 \cdot π^{2}} \cdot e^{i k x} \{\begin{matrix} \pm e^{\pm i \cdot k_{z} z} + R^{e} (k_{x}) e^{i k_{z} z} d l a z > - z_{o} \\ A \cdot \frac{\cos χ (z + z_{o} + b)}{\cos χ b} - z - b < z < - z_{o} . \end{matrix}

(11)

From Maxwell’s equations we get:

{\tilde{E}}_{x} = - \frac{i}{ω \cdot ε \cdot ε_{o}} \cdot \frac{\partial {\tilde{H}}_{y}}{\partial z}

(12)

ε = \{\begin{matrix} 1 f o r z > z_{o} \\ ε (r e l a t i v e) - z_{o} - b < z < - z_{o} . \end{matrix}

(13)

Meaning that

{\tilde{E}}_{x} (k_{x}) = - \frac{i}{ω \cdot ε_{o}} \cdot \frac{I d l}{8 \cdot π^{2}} \cdot e^{i \cdot k_{x} x} \{\begin{matrix} i k_{z} e^{\pm i \cdot k_{z} z} + R^{e} (k_{x}) \cdot e^{i \cdot k_{z} z} d l a z > - z_{o} \\ - A \cdot \frac{χ}{ε} \cdot \frac{\sin χ (z + z_{o} + b)}{\cos χ b} - z_{o} - b < z < - z_{o} \end{matrix}

(14)

where:

χ (k_{x}) = χ (k_{ρ}) = \sqrt{k^{2} \cdot n^{2} - k_{ρ}^{2}} .

(15)

Equations (11) and (12) take into account the condition of zeroing of the electric field component on the screen

z = - z_{o} - b

. We will call the obtained solution an even TM field, i.e., symmetrical with respect to the plane of the screen (perfectly conductive).

From the conditions of continuity of components in the plane we obtain:

i \cdot k_{z} (e^{i \cdot k_{z} \cdot z} + R_{p}^{e} (k_{x}) \cdot e^{- i \cdot k_{z} \cdot z_{o}}) = - A \cdot \frac{χ}{ε} \cdot tg (χ \cdot b) .

(16)

Hence the reflection coefficient for the even field TM

R_{p}^{e} (k_{ρ}) = - e^{2 \cdot i \cdot k_{z} \cdot z} \cdot \frac{k_{z} + i \frac{χ}{ε} tg (χ \cdot b)}{k_{z} - i \frac{χ}{ε} tg (χ \cdot b)} = - e^{2 \cdot i \cdot k_{z} \cdot z} \cdot \frac{k_{z} - k \cdot η_{p}^{e} (k_{ρ})}{k_{z} + k \cdot η_{p}^{e} (k_{ρ})}

(17)

and surface impedance

η_{p}^{e} (k_{ρ}) = - i \cdot \frac{χ}{k \cdot ε} tg (χ \cdot b) .

(18)

2.3 Odd TE reflection coefficient, surface impedance

We consider the TE (H-polarization) odd field. We are analyzing the problem

in the plane from here

x = 0

ρ = y, k_{ρ} = k_{y}

. For the considered field TE with components

\underline{E} = [E_{x}, 0,0] \underline{H} = [0, H_{y}, H_{z}]

we adopt its following representation.

{\tilde{E}}_{x} (k_{y}) = - \cdot \frac{I d l}{8 \cdot π^{2}} \cdot ω \cdot μ_{0} \cdot \frac{1}{k_{z}} e^{i \cdot k_{y} y} \{\begin{matrix} e^{\pm i \cdot k_{z} z} + {R_{n}}^{(h)} (k_{y}) \cdot e^{i \cdot k_{z} z} d l a z > - z_{o} \\ A \cdot \frac{\sin χ (z + z_{o} + b)}{\sin (χ b)} - z_{o} - b < z < - z_{o} \end{matrix}

(19)

from Maxwell’s equations for the medium

{\tilde{H}}_{x} = - \frac{i}{ω \cdot μ \cdot μ_{o}} \cdot \frac{\partial {\tilde{E}}_{x}}{\partial z}

(20)

μ = \{\begin{matrix} 1 d l a z > z_{o} \\ μ - z_{o} - b < z < - z_{o} \end{matrix}

(21)

{\tilde{H}}_{x} (k_{y}) = - i \cdot \frac{I d l}{8 \cdot π^{2}} \cdot \frac{1}{k_{z}} e^{i \cdot k_{y} y} \{\begin{matrix} (\pm e^{\pm i \cdot k_{z} \cdot z} + {R_{n}}^{(h)} (k_{y}) \cdot e^{i \cdot k_{z} \cdot z}) \cdot i \cdot k_{z} \\ A \cdot \frac{χ}{μ} \cdot \frac{\cos χ (z + z_{o} + b)}{\sin (χ \cdot b)} . \end{matrix}

(22)

Considering the condition of zeroing of the electric field component E_x on the screen

z = - z_{0} b

and the continuity of the electromagnetic field components Ex, H_y in the plane

z = - z_{0}

, we obtain

(e^{i \cdot k_{z} \cdot z} + R_{n}^{h} (k_{y})) e^{- i \cdot k_{z} \cdot z_{o}} = A

(23)

i \cdot k_{z} (- e^{+ i \cdot k_{z} \cdot z} + R_{n}^{h} (k_{y}) e^{- i \cdot k_{z} \cdot z_{o}}) = A \cdot \frac{χ}{μ} \cdot ctg (χ \cdot b)

(24)

Hence, the reflection coefficient for the odd field and TE polarization is described by the relationship.

R_{n}^{h} (k_{ρ}) = e^{2 \cdot i \cdot k_{z} \cdot z} \frac{k_{z} - k \cdot η_{n}^{h} \cdot (k_{ρ})}{k_{z} + k \cdot η_{n}^{h} (k)}

(25)

and surface admittance

η_{n}^{h} (k_{ρ}) = \frac{i}{k} \cdot \frac{χ}{μ} \cdot ctg (χ \cdot b) .

(26)

2.4. Reflection coefficient for TE field (H-polarization) even field, surface impedance

We analyze the issue in the plane, hence

x = 0, k_{x} = 0

, stąd

ρ = y, k_{ρ} = k_{y}

Figure 3. Dielectric layer with a magnetic screen.

For the considered TE field with components:

\underline{E} = [E_{x}, 0,0] i \underline{H} = [0, H_{y}, H_{z}]

We adopt the following representation.

{\tilde{E}}_{x} (k_{y}) = + \frac{I d l}{8 \cdot π^{2}} \cdot ω \cdot μ_{0} \cdot \frac{e^{i \cdot k_{y} \cdot y}}{k_{z}} \{\begin{matrix} e^{\pm i \cdot k_{z} z} + R_{p}^{h} (k_{y}) \cdot e^{i \cdot k_{z} \cdot z} d l a z > - z_{o} \\ A \cdot \frac{\cos χ (z + z_{o} + b)}{\cos (χ \cdot b)} - z_{o} - b < z < - z_{o} \end{matrix}

(27)

from Maxwell’s equation for the medium

{\tilde{H}}_{y} = - \frac{i}{ω \cdot μ \cdot μ_{0}} \cdot \frac{\partial {\tilde{E}}_{x}}{\partial z}

(28)

μ = \{\begin{matrix} 1 z > - z_{0} \\ μ_{r} z_{0} - b < z < z_{0} . \end{matrix}

(29)

In numerical calculations, we assume μ=1 for a perfect dielectric

{\tilde{H}}_{y} (k_{y}) = - i \cdot \frac{I d l}{8 \cdot π^{2}} \cdot \frac{e^{i \cdot k_{y} \cdot y}}{k_{z}} \{\begin{matrix} \pm e^{\pm i \cdot k_{z} z} + R_{p}^{h} (k_{y}) \cdot e^{i \cdot k_{z} \cdot z} d l a z > - z_{o} \\ - A \cdot \frac{χ}{μ} \cdot \frac{\sin χ (z + z_{o} + b)}{\cos (χ \cdot b)} - z_{o} - b < z < - z_{o} . \end{matrix}

(30)

Considering the condition of zeroing of the magnetic field component on the screen

z = - z_{0} - b

and the continuity of the electromagnetic field components E_x, H_y in the plane

z = - z_{0}

, we obtain

e^{i \cdot k_{z} \cdot z} + R_{p}^{h} (k_{y}) = A

(31)

i \cdot k_{z} (- e^{i \cdot k_{z} \cdot z} + R_{p}^{h} (k_{y}) \cdot e^{- i \cdot k_{z} \cdot z} = - A \cdot \frac{χ}{μ} \cdot tg (χ \cdot b)

(31)

Hence, the reflection coefficient for the even TE field is described by the expression

R_{p}^{h} = e^{2 \cdot i \cdot k_{z} \cdot z} \frac{k_{z} + i \cdot \frac{χ}{μ} \cdot tg (χ \cdot b)}{k_{z} - i \cdot \frac{χ}{μ} \cdot tg (χ \cdot b)} = e^{2 \cdot i \cdot k_{z} \cdot z} \frac{k_{z} - k \cdot η_{p}^{h} \cdot (k_{ρ})}{k_{z} + k \cdot η_{p}^{h} \cdot (k_{ρ})}

(32)

and surface admittance

η_{p}^{h} (k_{ρ}) = - \frac{i}{k} \cdot \frac{χ}{μ} \cdot tg (χ b) .

(33)

2.5. Reflection coefficient for the TM field (E - polarization), odd field, surface impedance.

Carrying out analogous considerations as in the previous points, we obtain:

R_{n}^{e} = - e^{2 \cdot i \cdot k_{z} \cdot z} \frac{k_{z} - k \cdot η_{n}^{e} \cdot (k_{ρ})}{k_{z} + k \cdot η_{n}^{e} \cdot (k_{ρ})}

(35)

η_{n}^{e} (k_{ρ}) = \frac{i}{k} \cdot \frac{χ}{ε} \cdot ctg (χ \cdot b) .

(36)

2.6. Transverse resonance conditions for the dielectric layer on the screen - surface wave poles for the TM and |TE fields

Zeroing of the denominator of the reflection coefficient R(k_ρ) for a specific polarization and field symmetry

k_{z} + k \cdot η^{e (h)} (k_{ρ}) = 0

(37)

k_{z} - i \cdot \frac{χ}{μ} \cdot tg (χ \cdot b) =

(38)

for the odd part of the field

k_{z} + i \cdot \frac{χ}{μ} \cdot ctg (χ \cdot b) = 0 .

(39)

For E- polarization for the even part of the field

k_{z} - i \cdot \frac{χ}{ε} \cdot tg (χ \cdot b) = 0

(40)

for the odd part of the field

k_{z} + i \cdot \frac{χ}{ε} \cdot ctg (χ \cdot b) = 0 .

(41)

The occurrence of this phenomenon should be taken into account when analyzing linear antennas on a dielectric substrate.

2.7. Reflection coefficient, surface impedance for the dielectric layer at a distance b from the perfectly conducting plane (screen)

We analyze the system presented in Figure 4. We consider the TM field (E polarization), an even field with a component

\underline{E} = [E_{x} 0, E_{z}] \underline{H} = [0, H_{y}, 0]

We analyze the problem in the y=0 plane, hence ρ=x , k_ρ=k_y. We assume the following field representation

{\tilde{H}}_{y}

{\tilde{H}}_{y} (k_{x}) = \frac{I \cdot d l}{8 \cdot π^{2}} e^{i \cdot k_{x} \cdot x} \{\begin{matrix} (1 + R^{e} (k_{x})) \cdot e^{i \cdot k_{z} \cdot z} z > - z_{0} \\ - e^{- i \cdot k_{z} \cdot z} + R^{e} (k_{x})) \cdot e^{i \cdot k_{z} \cdot z} - z_{0} < z < 0 \\ \frac{A (k_{x})}{\sin (χ \cdot b)} {\sin χ (z + z_{0} + b) - f (k_{x}) \sin χ (z + z_{0})} - z_{o} - b < z < - z_{0} \\ A (k_{x}) f (k_{x}) \frac{\cos k_{z} (z + z_{0} + b + b_{1})}{\cos (k_{z} \cdot b)} - z_{0} - b - b_{1} < z < - z - b . \end{matrix}

(42)

Using Maxwell’s equations for medium III

{\tilde{E}}_{x} (k_{x}) = - \frac{i}{ω \cdot ε \cdot ε_{o}} \cdot \frac{\partial {\tilde{H}}_{y}}{\partial z}

(43)

we designate:

{\tilde{E}}_{x} (k_{x}) = \frac{- i \cdot I \cdot d l \cdot e^{i \cdot k_{x} \cdot x}}{8 \cdot π^{2} \cdot ω \cdot ε_{0}} \{\begin{matrix} i \cdot k_{z} (1 + R^{e} (k_{x})) \cdot e^{i \cdot k_{z} \cdot z} z > - z_{0} \\ i k_{z} [e^{- i \cdot k_{z} \cdot z} + R^{e} (k_{x})] \cdot e^{i \cdot k_{z} \cdot z} - z_{0} < z < 0 \\ \frac{A (k_{x}) [χ \cos χ (z + z_{0} + b) - f (k_{x}) χ \cos χ (z + z_{0})]}{\sin (χ \cdot b)} - z_{o} - b < z < - z_{0} \\ A (k_{x}) \cdot f (k_{x}) \cdot \frac{k_{z} \sin k_{z} (z + z_{0} + b + b_{1})}{\cos (k_{z} \cdot b_{1})} - z_{0} - b - b_{1} < z < - z - b \end{matrix}

(44)

where:

f (x) = \frac{χ}{ε \cdot \sin (χ \cdot b)} \cdot {\{\frac{χ}{ε} \cdot ctg (χ \cdot b) - k_{z} \cdot tg (k_{z} \cdot b_{1})\}}^{- 1} .

(45)

Taking into account the continuity of the field

{\tilde{H}}_{y} i {\tilde{E}}_{x}

in the i plane

z = - z_{0} - b

and

z = z_{0}

, we obtain an expression for the reflection coefficient

R^{e} (k_{ρ}) = - e^{- 2 \cdot i \cdot k_{z} \cdot z_{0}} \cdot \frac{k_{z} - k \cdot η^{e} (k_{ρ})}{k_{z} + k η^{e} (k_{ρ})}

(46)

and surface impedance

η_{e} (k_{ρ}) = η_{n}^{e} \cdot \frac{η_{p}^{e} + η_{o p}}{η_{n}^{e} + η_{o p}}

(47)

where : are

η_{p}^{e} a n d η_{n}^{e}

described by dependencies (18) and (36), respectively

η_{o p} (k_{ρ}) = - i \cdot \frac{k_{z}}{k} \cdot tg (k_{z} \cdot b_{1}) .

(48)

The condition of transverse resonance is expressed by the following relationship:

k_{z} + k \cdot η^{e} = \frac{η_{o p} (k_{ρ}) (k_{z} + k \cdot η_{n}^{e}) + η_{m}^{e} (k_{z} + k \cdot η_{n}^{e})}{η_{n}^{e} + η_{o p}}

(49)

2.8. Reflectance and surface impedance of the TE field (H-polarization) even field

We analyze the system shown in Figure 5

We consider the TE field - an even field with components

\underline{E} = [E_{x}, 0,0] i \underline{H} = [0, H_{y}, H_{z}]

We assume the following field representation ρ=y , k_ρ=k_y. We assume the following field representation E_x(k_y)

{\tilde{E}}_{x} (k_{y}) = \frac{I \cdot d l}{8 \cdot π^{2}} ω μ_{0} {\frac{e}{k_{z}}}^{i \cdot k_{y} \cdot y} \{\begin{matrix} (1 + R^{h} (k_{y})) \cdot e^{i \cdot k_{z} \cdot z} z > - z_{0} \\ - e^{- i \cdot k_{z} \cdot z} + R^{h} (k_{y})) \cdot e^{i \cdot k_{z} \cdot z} - z_{0} < z < 0 \\ A (k_{y}) \frac{\{\sin χ (z + z_{0} + b) - g (k_{y}) \sin χ (z + z_{0})\}}{\sin (χ \cdot b)} - z_{o} - b < z < - z_{0} \\ A (k_{y}) \cdot g (k_{y}) \cdot \frac{\cos k_{z} (z + z_{0} + b + b_{1})}{\cos (k_{z} \cdot b_{1})} - z_{0} - b - b_{1} < z < - z - b . \end{matrix}

(50)

They use Maxwell’s equations (28) to calculate the area

{\tilde{H}}_{y} (k_{y})

{\tilde{H}}_{y} (k_{y x}) = \frac{- i I \cdot d l}{8 \cdot π^{2}} \frac{e^{i \cdot k_{x} \cdot x}}{k_{z}} \{\begin{matrix} i \cdot k_{z} (1 + R^{h} (k_{y x})) \cdot e^{i \cdot k_{z} \cdot z} z > - z_{0} \\ i k_{z} [e^{- i \cdot k_{z} \cdot z} + R^{h e} (k_{y x})] \cdot e^{i \cdot k_{z} \cdot z} - z_{0} < z < 0 \\ \frac{A (k_{y}) χ \cos χ (z + z_{0} + b) - g (k_{y x}) χ \cos χ (z + z_{0})}{\sin (χ \cdot b)} - z_{o} - b < z < - z_{0} \\ A (k_{y}) \cdot g (k_{y}) \cdot \frac{k_{z} \sin k_{z} (z + z_{0} + b + b_{1})}{\cos (k_{z} \cdot b_{1})} - z_{0} - b - b_{1} < z < - z - b . \end{matrix}

(41)

where:

g (k_{y}) = \frac{χ}{μ \cdot \sin (χ \cdot b)} \cdot \frac{χ}{μ} \cdot {\{ctg (χ \cdot b) - k_{z} \cdot tg (k_{z} \cdot b_{1})\}}^{- 1}

(52)

Taking into account field continuity

{\tilde{H}}_{y}, {\tilde{E}}_{x}

in the plane

z = - z_{0} - b

z = z_{0}

we obtain an expression for the reflectance coefficient

R^{h} (k_{ρ}) = e^{+ 2 \cdot i \cdot k_{z} \cdot z_{0}} \cdot \frac{k_{z} - k \cdot η^{h} (k_{ρ})}{k_{z} + k η^{h} (k_{ρ})}

(53)

and surface admittance of the surface structure

η^{h} (k_{ρ}) = η_{n}^{h} \cdot \frac{η_{p}^{h} + η_{o p}}{η_{n}^{h} + η_{o p}}

(54)

The resonance condition is expressed by the following relationship:

k_{z} + k \cdot η^{h} = \frac{η_{o p} (k_{z} + k \cdot η_{n}^{h}) + η_{n}^{h} (k_{z} + k \cdot η_{p}^{h})}{η_{n}^{h} + η_{o p}} = 0 .

(55)

2.8. Reflectance coefficient and surface impedance for TE fields (H - polarization) odd field and TM fields (E - polarization) odd field

Carrying out the considerations as in the previous section, we obtain an odd field for the TE field (H-polarization).

Reflectance coefficient

R^{h} (k_{ρ}) = e^{2 \cdot i \cdot k_{z} \cdot z} \frac{k_{z} - k \cdot η^{h} (k_{ρ})}{k_{z} + k \cdot η^{h} (k_{ρ})}

(56)

surface admittance

η^{h} = η_{n}^{h} \cdot \frac{η_{p}^{h} + η_{o n}}{η_{n}^{h} + η_{o n}}

(57)

where

η_{o n} = i \frac{k_{z}}{k} ctg (k_{z} \cdot b_{1}),

(58)

η_{n}^{h} = i \frac{χ}{k} ctg (χ \cdot b),

(59)

η_{p}^{h} = - i \frac{χ}{k} tg (χ \cdot b) .

(60)

Resonance equation

k_{z} + k \cdot η^{h} = \frac{η_{o n} (k_{z} + k \cdot η_{n}^{h}) + η_{n}^{h} (k_{z} + k \cdot η_{p}^{h})}{η_{n}^{p} + η_{o p}}

(61)

and for E polarization

R^{e} (k_{ρ}) = e^{2 i \cdot k_{z} \cdot z_{0}} \frac{k_{z} - k \cdot η^{e} (k_{ρ})}{k_{z} + k \cdot η^{e} (k_{ρ})}

(62)

surface impedance

η^{e} = η_{n}^{e} \frac{η_{p}^{e} + η_{o n}}{η_{n}^{e} + η_{o n}}

(63)

where:

η_{o n}, η_{p}^{e}, η_{n}^{e}

are described by dependencies (58), (18) and (26), respectively. And the resonance equation has the form:

k_{z} + k \cdot η^{e} = \frac{η_{o n} (k_{z} + k \cdot η_{p}^{e}) + η_{n}^{e} (k_{z} + k \cdot η_{p}^{e})}{η_{n}^{e} + η_{o n}} = 0 .

(64)

3. Distribution coefficients Generalized impedance z_mn

z_{m n} = - {(2 π)}^{- 4} \int_{- \infty}^{\infty} \int_{- \infty}^{\infty} \int_{- \infty}^{\infty} \int_{- \infty}^{\infty} \int_{- \infty}^{\infty} \int_{- \infty}^{\infty} \{F [G (k_{x} - k_{x}^{’}; k_{y} - k_{y}^{’})] F [J_{o} (k_{x} - k_{x}^{’})] \cdot

F [J_{o} (k_{x} - k_{x}^{’})] \cdot F [J_{o} (k_{x})] e^{- i (k_{x} - k_{x}^{’}) x_{m} - k_{x}^{’} x_{n} - i (k_{y} - k_{y}^{’}) y_{m} - i k_{y}^{’} y_{n} + i k_{x} x + i k_{y} y} d k_{y}^{’} d k_{x}^{’} d k_{y} d k_{x} d y d x

(65)

is performed analytically. The integral (66) therefore reduces to the form:

\int_{x} \int_{y} \int_{x_{0}} \int_{y_{0}} \int_{0}^{\infty} J_{m} (x) G (x, y, x_{0}, y_{0}, K) J_{n} (x_{0}, y_{0}) d K d y_{0} d x_{0} d y d x

(66)

\int_{- \infty}^{\infty} \int_{- \infty}^{\infty} F_{m} (k_{x}, k_{y}) \underline{G} (k_{x}, k_{y}) F_{n} (k_{x}, k_{y}) d k_{y} d k_{x}

(57)

where F_m and F_n are the Fourier transforms of the basis and test functions, respectively.

for single-layer system (18, 26, 33, 35)
for a multi-layer system, dependencies (18, 26, 33, 36, 48, 58)

In the above expressions (based on the solution of equations (38÷41), the quantity k_z may assume purely imaginary values. This is due to the fact that the dielectric substrate is non-lossy

tg δ \leq 1 0^{- 4}

But the size

ε_{0}, μ_{0}, ε, μ, x_{m} - x_{n} . y_{m} - y_{n}, k_{e f}, k_{n} k, L_{N}, b, i ω

are real, non-negative parameters that change discreetly within certain ranges.

\sqrt{k^{2} - k_{x}^{2} - k_{y}^{2}} = 0

\sqrt{k^{2} n^{2} - k_{x}^{2} - k_{y}^{2}} = 0

(68)

-: apparent pole for:

k_{x}^{2} - k_{e f}^{2} = 0

(this is a removable singularity)

-: isolated poles (which may occur)

for 1 + \frac{ε \sqrt{k^{2} - k_{x}^{2} - k_{y}^{2}} - \sqrt{k^{2} n^{2} - k_{x}^{2} - k_{y}^{2}}}{ε \sqrt{k^{2} - k_{x}^{2} - k_{y}^{2}} + \sqrt{k^{2} n^{2} - k_{x}^{2} - k_{y}^{2}}} e^{2 i b_{1} \sqrt{k^{2} n - k_{x}^{2} - k_{y}^{2}}} = 0

(69)

for 1 - \frac{μ \sqrt{k^{2} - k_{x}^{2} - k_{y}^{2}} - \sqrt{k^{2} n^{2} - k_{x}^{2} - k_{y}^{2}}}{μ \sqrt{k^{2} - k_{x}^{2} - k_{y}^{2}} + \sqrt{k^{2} n^{2} - k_{x}^{2} - k_{y}^{2}}} e^{2 i b_{1} \sqrt{k^{2} n - k_{x}^{2} - k_{y}^{2}}} = 0

(6)

hence, after transformation, we get that for

k^{2} - k_{x}^{2} - k_{y}^{2} > 0 or k^{2} n^{2} - k_{x}^{2} - k_{y}^{2} < 0

(7)

poles do not exist.

It is possible for a pole to occur only for

(k n)^{2} - k_{x}^{2} - k_{y}^{2} > 0 i k^{2} - k_{x}^{2} - k_{y}^{2} < 0 (k < |\sqrt{k_{x}^{2} + k_{y}^{2}}| < k n)

(8)

In order to determine the value of isolated poles for

R_{p}^{e}

and

R_{n}^{h}

, the relationship should be examined:

R_{p}^{e} : 1 + \frac{ε \sqrt{k^{2} - k_{x}^{2} - k_{y}^{2}} - \sqrt{k^{2} n^{2} - k_{x}^{2} - k_{y}^{2}}}{ε \sqrt{k^{2} - k_{x}^{2} - k_{y}^{2}} + \sqrt{k^{2} n^{2} - k_{x}^{2} - k_{y}^{2}}} e^{2 i b_{1} \sqrt{k^{2} n - k_{x}^{2} - k_{y}^{2}}}

(9)

R_{n}^{h} : 1 - \frac{μ \sqrt{k^{2} - k_{x}^{2} - k_{y}^{2}} - \sqrt{k^{2} n^{2} - k_{x}^{2} - k_{y}^{2}}}{μ \sqrt{k^{2} - k_{x}^{2} - k_{y}^{2}} + \sqrt{k^{2} n^{2} - k_{x}^{2} - k_{y}^{2}}} e^{2 i b_{1} \sqrt{k^{2} n - k_{x}^{2} - k_{y}^{2}}} = 0

(10)

From the above it follows that if

\begin{matrix} \begin{matrix} a) k^{2} - k_{x}^{2} - k_{y}^{2} > 0 \\ b) k^{2} n^{2} - k_{x}^{2} - k_{y}^{2} < 0 \end{matrix}\} \\ c) k^{2} - k_{x}^{2} - k_{y}^{2} < 0 i k^{2} n^{2} - k_{x}^{2} - k_{y}^{2} > 0 \end{matrix}

(11)

If conditions a) and b) are met, the poles do not occur, but if condition c) is met, then the necessary condition for the presence of poles is met.

Let us therefore examine the sufficient condition from the condition mentioned above (74) point. c it follows that:

k < |\sqrt{k_{x}^{2} + k_{y}^{2}}| < k n

(12)

For the situation under consideration, the equations will be written in the form:

R_{p}^{e} : 1 + \frac{i ε \sqrt{k_{x}^{2} + k_{y}^{2} - k^{2}} - \sqrt{k^{2} n^{2} - k_{x}^{2} - k_{y}^{2}}}{i ε \sqrt{k_{x}^{2} + k_{y}^{2} - k^{2}} + \sqrt{k^{2} n^{2} - k_{x}^{2} - k_{y}^{2}}} e^{2 i b_{1} \sqrt{k^{2} n^{2} - k_{x}^{2} - k_{y}^{2}}} = 0

(13)

R_{n}^{h} : 1 - \frac{i μ \sqrt{k_{x}^{2} + k_{y}^{2} - k^{2}} - \sqrt{k^{2} n^{2} - k_{x}^{2} - k_{y}^{2}}}{i μ \sqrt{k_{x}^{2} + k_{y}^{2} - k^{2}} + \sqrt{k^{2} n^{2} - k_{x}^{2} - k_{y}^{2}}} e^{2 i b_{1} \sqrt{k^{2} n^{2} - k_{x}^{2} - k_{y}^{2}}} = 0

(14)

Hence, after transformations we have:

1 + e^{- 2 i a r c t g} (\frac{ε \sqrt{k_{x}^{2} + k_{y}^{2} - k^{2}}}{\sqrt{k^{2} n^{2} - k_{x}^{2} - k_{y}^{2}}}) e^{2 i b_{1} \sqrt{k^{2} n^{2} - k_{x}^{2} - k_{y}^{2}}} = 0

(15)

1 - e^{- 2 i a r c t g} \frac{μ \sqrt{k_{x}^{2} + k_{y}^{2} - k^{2}}}{\sqrt{k^{2} n^{2} - k_{x}^{2} - k_{y}^{2}}} e^{2 i b_{1} \sqrt{k^{2} n^{2} - k_{x}^{2} - k_{y}^{2}}} = 0

For a multilayer system, the equation determining the singularities (poles) of the function takes the form:-

dla R^{e} \to \infty tg χ b_{1} = \frac{2 χ k_{z} ε}{(χ^{2} + ε {|k_{z}|}^{2}) (1 + \frac{χ^{2} - ε^{2} {|k_{z}|}^{2}}{χ^{2} + ε^{2} {|k_{z}|}^{2}}) e^{- 2 b_{2} |k_{z}|}} \Rightarrow k_{ρ}^{2} = x^{e} is a \sin gular point : k^{2} < x^{e} < k^{2} \cdot n^{2}

(16)

- for

R^{h} \to \infty

tg χ b_{1} = \frac{2 χ |k_{z}| μ}{(χ^{2} + μ^{2} {|k_{z}|}^{2}) (1 - \frac{χ^{2} - μ_{r}^{2} {|k_{z}|}^{2}}{χ^{2} + μ_{r}^{2} {|k_{z}|}^{2}}) e^{- 2 b_{2} |k_{z}|}} \Rightarrow k_{ρ}^{2} = x^{h} is a \sin gular point : k^{2} < x^{h} < k^{2} \cdot n^{2} |k_{z}| = |\sqrt{k_{ρ}^{2} - k^{2}}|

(17)

The remaining patterns remain as for a single-layer system.

The appropriate branches of the elements are specified in individual ranges

in the following way:1⁰

dla k_{ρ}^{2} > k^{2} n^{2} > k^{2} k_{z} = \pm i \sqrt{k_{ρ}^{2} - k^{2}} χ = \pm i \sqrt{k_{ρ}^{2} - k^{2} n^{2}} R^{e, h} = - e^{- 2 z \sqrt{k_{p}^{2} - k^{2}}} = \frac{e^{- 2 b \sqrt{k_{p}^{2} - k^{2} n^{2}}} + η_{e, h}}{1 + η_{e, h} e^{- 2 b \sqrt{k_{p}^{2} - k^{2} n^{2}}}}

(18)

η_{e} = \frac{ε \sqrt{k_{p}^{2} - k^{2}} - \sqrt{k_{p}^{2} - k^{2} n^{2}}}{ε \sqrt{k_{p}^{2} - k^{2}} + \sqrt{k_{p}^{2} - k^{2} n^{2}}} η_{h} = \frac{μ \sqrt{k_{p}^{2} - k^{2}} - \sqrt{k_{p}^{2} - k^{2} n^{2}}}{μ \sqrt{k_{p}^{2} - k^{2}} + \sqrt{k_{p}^{2} - k^{2} n^{2}}}

(19)

2⁰

2^{0} for k^{2} n^{2} > k_{ρ}^{2} > k^{2} k_{ρ} \in (- k n, - k) \cup (k, k n) k_{z} = \pm i \sqrt{k_{ρ}^{2} - k^{2}} κ = \pm i \sqrt{k^{2} n^{2} - k_{ρ}^{2}} R^{e, h} = - e^{- 2 z \sqrt{k_{p}^{2} - k^{2}}} = \frac{e^{2 i b \sqrt{k_{p}^{2} - k^{2} n^{2}}} + η_{e, h}}{1 + η_{e, h} e^{2 b \sqrt{k_{p}^{2} - k^{2} n^{2}}}}

(20)

η_{e} = \frac{i ε \sqrt{k_{p}^{2} - k^{2}} - \sqrt{k^{2} n^{2} - k_{p}^{2}}}{i ε \sqrt{k_{p}^{2} - k^{2}} + \sqrt{k^{2} n^{2} - k_{p}^{2}}} η_{h} = \frac{i μ \sqrt{k_{p}^{2} - k^{2}} - \sqrt{k^{2} n^{2} - k_{p}^{2}}}{i μ \sqrt{k_{p}^{2} - k^{2}} + \sqrt{k^{2} n^{2} - k_{p}^{2}}}

(21)

3^{0} dla k^{2} n^{2} > k^{2} > k_{ρ}^{2} k_{ρ} \in (- k, + k) k_{z} = \pm i \sqrt{k_{ρ}^{2} - k^{2}} κ = \pm i \sqrt{k^{2} n^{2} - k_{ρ}^{2}} R^{e, h} = - e^{- 2 z \sqrt{k_{p}^{2} - k^{2}}} = \frac{e^{2 i b \sqrt{k_{p}^{2} - k^{2} n^{2}}} + η_{e, h}}{1 + η_{e, h} e^{2 b \sqrt{k_{p}^{2} - k^{2} n^{2}}}}

(22)

η_{e} = \frac{ε \sqrt{k^{2} - k_{p}^{2}} - \sqrt{k^{2} n^{2} - k_{p}^{2}}}{ε \sqrt{k^{2} - k_{p}^{2}} + \sqrt{k^{2} n^{2} - k_{p}^{2}}} η_{h} = \frac{μ \sqrt{k^{2} - k_{p}^{2}} - \sqrt{k^{2} n^{2} - k_{p}^{2}}}{μ \sqrt{k^{2} - k_{p}^{2}} + \sqrt{k^{2} n^{2} - k_{p}^{2}}}

(23)

Numerical analysis of submitted above expression was made. Results of calculation for typical dielectrics are shown in Figure 7.

Conclusions

b / ε_{r}

References

Munson R.: „Conformal microstrip antennas and microstrip phased arrays”, IEEE Transactions on Antennas and Propagation Vol. 22 No.11 November 1974. [CrossRef]
Minatti, G.; Faenzi, M.; Martini, E.; Caminita, F.; de Vita, P.; Gonz’alez-Ovejero, D.; Sabbadini, M.; Maci, S. “Modulated metasurface antennas for space: Synthesis, analysis and realizations”. IEEE Transactions on Antennas and Propagation 2014, 63, 1288–1300.
Sharawi S. M. “Printed Multi-Band MIMO Antenna Systems and Their Performance Metrics” IEEE Antennas and Propagation Magazine 2013, 218 - 232.
Oltman G.H. "Electromagnetically coupled microstrip dipoles", IEEE Transactions on Antennas and Propagation AP-29, No 1, 1981.M. N. Sadiku, “Elements of electromagnetics”. Oxford University Press, 2014.
Malaisamy K., Haridoss, Pandian A., Rima S. “Design of Crossed Dipole Yagi–Uda MIMO Antenna for Radar Applications Internationa” Journal of Antennas and Propagation 2022 Volume12.
Haobo Y, Hongguang Z , Hongwei L, Xiaojie D, and Xi C “Computing Mutual Impedance of Antennas by Spherical Harmonic Transform” IEEE Transactions on antennas and propagation, VOL. 67, NO. 7, JULY 2019 4377.
Aourik S. , Erkik A. , Oukaira A. , Dhaou S. Jamal, Z. Lakhssassi A. „An Advanced Array Configuration Antenna Based on Mutual Coupling Reduction” Electronics 2023, 12(7), 1707;
Richmond J.H., Otto D.V.: „Digital computer solutions of the rigorous equations of electromagnetics”, IEEE Transactions on Antennas and Propagation Vol.17, 1969.
Liu S., Sun S., and Chew W. C., “A potential-based integral equation method for low-frequency electromagnetic problems,” IEEE Transactions on Antennas and Propagation, vol. 66, no. 3, pp. 1413–1426, 2018.
Gao, X.; Han, X.; Cao, W.-P.; Li, H.O.; Ma, H.F.; Cui, T.J. “Ultrawideband and high-efficiency linear polarization converter based on double v-shaped metasurface”. IEEE Transactions on Antennas and Propagation. 2015, 63, 3522–3530. [Google Scholar] [CrossRef] . [CrossRef]
Cao Y. F. ; Zhang X. Y. ; Xue Q. “Compact Shared-Aperture Dual-Band Dual-Polarized Array Using Filtering Slot Antenna and Dual-Function Metasurface” IEEE Transactions on Antennas and Propagation, Publication Year: 2022, Page(s):1120 – 1131 04.
Wnuk M. “Multilayer dielectric periodic antenna structure in a cascade view” Appl. Sci. 2022, Volume 12, Issue 9, pp.1-14.
Liu, Q.S.; Sun, S.; Chew, W.C. “A potential-based integral equation method for low-frequency electromagnetic problems”. IEEE Transactions on Antennas and Propagation. 2018, 66, 1413–1426. [Google Scholar] [CrossRef. [CrossRef]
Yaghjian, A.D. “Reflections on Maxwell’s treatise.” Prog. Electromagnetic. Res. 2014, 149, 217–249.
Khaleghi S. S. M., Moradi G. , Jafargholi R. S. S. A. “Microstrip Line Impedance Matching Using ENZ Metamaterials”, Design, and Application Transactions on antennas and propagation, Vol. 67, No. 4, 2019 2243.
Radavaram S., Pour M. , “Wideband Radiation Reconfigurable Microstrip Patch Antenna Loaded With Two Inverted” IEEE Transactions on Antennas and Propagation, Vol. 67, No. 3, 2019 1501-1505.
Balanis, C.A. “Antenna Theory: Analysis and Design”; John Wiley & Sons: Hoboken, NJ, USA, 2016.
Kong, J.A. “Electromagnetic Wave Theory”; EMW Publishing: Cambridge, MA, USA, 2008.
Sadiku, M.N. “Elements of Electromagnetics”; Oxford University Press: Oxford, UK, 2014.
Bender, C.M.; Orszag, S.A. “Advanced Methods for Scientists and Engineers I: Asymptotic Methods and Perturbation Theory”; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2013.
Oppenheim, A.V.; Schafer, R.W. “Electromagnetic Field Theory Discrete-Time Signal Processing”; Pearson Education: London, UK, 2014.

Figure 1. Surface impedance.

Figure 4. Multilayer dielectric structure with an electric shield.

Figure 5. Multilayer dielectric structure with a magnetic screen.

Figure 6. Plane periodic antenna structure infinite in the (x,y) plane, multi-layer structure.

Figure 7. Distribution of singular points with thickness of dielectric layer.

MDPI Initiatives

Important Links

Choose an area of interest and we will send you notifications of new preprints at your preferred frequency.

Disclaimer

Antenna Array on a Multilayer Dielectric Structure as a Nanostructure

Abstract

1. Introduction

2. Surface impedance of the layered structure. reflectivity. transverse resonance conditions

2.1. Surface impedance of a layered structure

2.2 Reflectance and surface impedance of a dielectric layer lying on a perfectly conductive plane

2.3 Odd TE reflection coefficient, surface impedance

2.4. Reflection coefficient for TE field (H-polarization) even field, surface impedance

2.5. Reflection coefficient for the TM field (E - polarization), odd field, surface impedance.

2.6. Transverse resonance conditions for the dielectric layer on the screen - surface wave poles for the TM and |TE fields

2.7. Reflection coefficient, surface impedance for the dielectric layer at a distance b from the perfectly conducting plane (screen)

2.8. Reflectance and surface impedance of the TE field (H-polarization) even field

2.8. Reflectance coefficient and surface impedance for TE fields (H - polarization) odd field and TM fields (E - polarization) odd field

3. Distribution coefficients Generalized impedance zmn

Conclusions

References

Abstract

1. Introduction

2. Surface impedance of the layered structure. reflectivity. transverse resonance conditions

2.1. Surface impedance of a layered structure

2.2 Reflectance and surface impedance of a dielectric layer lying on a perfectly conductive plane

2.3 Odd TE reflection coefficient, surface impedance

2.4. Reflection coefficient for TE field (H-polarization) even field, surface impedance

2.5. Reflection coefficient for the TM field (E - polarization), odd field, surface impedance.

2.6. Transverse resonance conditions for the dielectric layer on the screen - surface wave poles for the TM and |TE fields

2.7. Reflection coefficient, surface impedance for the dielectric layer at a distance b from the perfectly conducting plane (screen)

2.8. Reflectance and surface impedance of the TE field (H-polarization) even field

2.8. Reflectance coefficient and surface impedance for TE fields (H - polarization) odd field and TM fields (E - polarization) odd field

3. Distribution coefficients Generalized impedance zmn

Conclusions

References

MDPI Initiatives

Important Links

Subscribe

3. Distribution coefficients Generalized impedance z_mn

3. Distribution coefficients Generalized impedance z_mn