Prerpints.org logo
Preprint
Article

An Improved Zero-Flowrate Switching Control Method to Reduce Switching Losses in a Hydraulic Actuator

Altmetrics

Downloads

88

Views

16

Comments

0

This version is not peer-reviewed

Submitted:

06 March 2024

Posted:

08 March 2024

You are already at the latest version

Alerts
Abstract
Abstract—Hydraulic switching actuators are high efficiency, fast response, and low cost solutions for hydraulic control systems. One of the challenging problems is throttling losses during valve transitions. Previously the authors proposed a zero-flowrate switching method to reduce throttling energy loss of the switching valve, where a hydraulic resonator is applied to make the flowrates through the lines approaching zero before the valves are switched off. The major challenge of this approach is fast switching valves whose transition times are less than 2 ms. In this paper, an improved zero-flowrate switching method is presented. It utilizes the capacity with independent inlet/outlet ports to regulate flowrates through the lines. Models of capacity applied in a simple line with different pressure signals are developed to explore characteristics of the capacity, based on which a complete actuation system is developed. In the complete model, resistance and inductance are optimized to achieve desired flowrate response. The improved zero-flowrate switching method reduces throttling energy losses by 99.945% compared to a hard switching system. The simulation results demonstrated that the improved zero-flowrate switching method performs as expected in the design condition. A capacity with proper volume is able to regulate flowrates through all the lines to zero, with the help of appropriate resistance and inductance. Compared to previous zero-flowrate switching method, the novel strategy allows slower switching valves applied in hydraulic actuation systems and achieves better efficiency performance. This research paves a new avenue for reducing throttling energy losses and improves system efficiency in hydraulic switching actuators, as well as most of the hydraulic switch-mode circuits.
Keywords: 
Subject: Engineering  -   Mechanical Engineering

I. INTRODUCTION

In last decades, switching hydraulics has been more and more popular for high-efficient and fast response performance, as well as low cost [1,2,3,4,5,6]. However, the technology has not penetrated the marketplace, partially due to the energy loss during transition events of the valves. More frequent the valve switches, more energy loss it produces. Although tons of research have been on switching energy loss, eliminating flowrate or pressure drop through/across the switching valve during transition events is still a challenge. It has been identified that such energy loss origins from transition times, pressure drops across and throttled flow through the switching valves, fluid compressibility, hysteresis in the accumulator bladder, fluid friction, and leakage [7]. Among them, the transition time is dominated (about 60%) [8,9]; other significant losses in the system are generated by compressibility (20%), full open throttling (19%), and leakage (1%). According to comparison of the numbers, transition time and throttling result in 79% energy loss in total. James D. Van de Ven presented volumetric efficiencies that ranged from 51% to 95% in three different circuits which shows that the dominant power loss is due to throttling through the ports of the switching valve during valve transition events [10]. Thus, the focus to improve efficiencies of switching hydraulic actuators is to explore solutions to attenuate energy consumption during transitions of valves.
Energy loss is the production of flowrates and pressure drops; therefore, it is possible to reduce power loss by decreasing either flowrate through or pressure drop across the valve, which is inspired by “Soft Switching Technology” in electronic technology. In last decades, some researchers have proposed different soft switching methods to increase the efficiency of a hydraulic switching actuator. One of the methodologies is to switch off a control valve when pressure drop across the valve is zero. In 2009, Michael B. Rannow and Perry Y. Li [11] proposed a soft switch where a piston chamber with a spring at the back of piston is applied parallel to the switching valve. When the switch valve is to switch off, a tank valve is switched on to accelerate the flowrate; then the tank valve is switched off; as a result, the fluid is forced to go to the piston chamber and the momentum is converted to pressure of the piston chamber. Once the pressure is up to the cracking pressure of the check valve parallel to the switching valve, the check valve is opened and the pressure drop across the switching valve becomes zero. Therefore, the switching valve is able to switch off with very small energy loss. By applying such a soft switching configuration, the transition and compressibility losses is reduced by 81% and total system losses by 64%. In 2017, Alexander C. Yudell and James D. Van de Ven applied a similar principle to an inertance hydraulic circuit aiming at reducing switching power loss caused by high-speed valves whose power loss is up to 44% of overall losses. Their simulation results demonstrates that the soft switching controller increases system efficiency by 42% at peak load power and extends the power delivery capabilities by 77%.
These research work indicates that soft switching technology is a potential solution for transition loss in a switching hydraulic system. However, there are some limitations for the above work. Firstly, very fast valves are needed for such a soft switch. To minimize the switching period in hydraulic switch mode circuits, the transition time of the valve is on the order of 5% of the switching period. The high-speed valve used in the research transitions in 0.8 ms and is capable of 100 Hz switching. Since a high-speed valve whose opening/closing times are as small as 0.8 ms are very expensive and difficultly obtained commercially, it limits the application of such soft switching technologies in conditions where very fast switching valves are used.
Since 2016 S. Peng has proposed a zero-flowrate switching control technology where the flowrate through a switching valve is reduced to zero and then the valve is switched off [12,13]. In the work, a hydraulic resonator is applied to regulate flowrates to zero and small pressure waves from a normal piston pump is used as the excitation. Since the base frequency of the pressure waves is about 113 Hz, the frequency of excited flowrate waves is also about 113 Hz, which means the transition time of the switching valve should be smaller than 8.8 ms (one period for 113 Hz). In above work, the system efficiency is improved by 89% with fast-switching valves whose transition time is 2 ms. The transition time of 2 ms still makes a valve very expensive and be a great limitation of applications; besides, to make the resonator work, the capacitance and inductance should be designed carefully to match the natural frequency of the resonator, thus the dimensions of the resonator can be too large to limit the application of for this technology.
In this paper, an improved zero-flowrate switching method is proposed for efficiency improvement of hydraulic switching actuators. The control scheme consists of two control branches: one is the main control line, and the other is the auxiliary control line. The flowrate-regulation function is achieved by the capacity in the auxiliary line with the help of resistance and inductance in two branches. As a result, the proposed method does not require the natural frequency of the capacity to match a specified frequency. The suggested solution improves the energy efficiency of a hydraulic switching actuator by reducing throttling loss during transitions of the valves. A thorough analysis is carried out numerically against a hard-switching actuator in order to confirm the effectiveness and efficiency performance of the suggested method. In conclusion, the paper’s contribution consists of the following.
1)
Proposing an improved zero-flowrate switching method for a hydraulic switching actuator. The suggested approach is created as an alternative to a hard-switching controller, to achieve higher efficiency in applications that allow relatively slower switching valves.
2)
The improved control method utilizes a capacity to regulate flowrates through the lines, thus the dimension of capacity can be more flexible because resistance and inductance in the lines help to further adjust flowrates as required. While in previous method, a hydraulic resonator that consists of a capacitance and inductance is applied; the frequencies of the capacitance and inductance should match the natural frequency of the resonator, which can make the volume of capacity too large, or inductor too long, or inappropriate for the application.
3)
The characteristic of the capacity with different input pressure signals is analyzed and the effectiveness of applying a capacity to regulate flowrates in a hydraulic switching actuator in desired conditions is validated numerically. Efficiency improvement is confirmed numerically with lumped models of resistance, inductance and capacitance.
The focus of this paper is on presenting a high-efficient hydraulic actuator by applying a novel mechanism to enable zero-flowrate switching in hydraulic switching actuators. In Section II, the general principles of zero-flowrate switching technology are presented. Next, the mechanism of an improved zero-flowrate switching controller is explained in Section III. To validate the mechanism, the simulation models are developed in Section IV to explore the feasibility of this novel method. Section V presents a complete model of an improved zero-flowrate switching actuator, and the Simulation results are presented in Sec. VI and discussed in Section VII. Section VIII provides the conclusion remarks.

II. Concept of Zero-Flowrate Switching Technology

Zero-flowrate switching technology is a switching methodology where flowrate across the control valve is reduced to approximately zero when the valve is switched off. This concept is inspired by zero-current switching method in electronic engineering [14]. The methodology aims to decrease transition losses by reducing flowrates through the switching valve dramatically during a transition event.
As shown in Figure 1, a switching valve is used to switch on/off the control line. Since it takes some time for the valve spool to move from totally-open position to totally-closed position, there must be some flowrates and pressure drops during a valve transition event. As shown in Figure 2, flowrates and pressure drops lead to certain energy loss. The more frequently the valve switches, the larger the energy losses are. Thus, it is important to reduce transition loss for such a switching valve control line.
As explained in Figure 2, power loss during valve transition is the production of pressure drop and flowrate, thus, one potential solution to reduce switching power loss is to actively reduce the flowrate to approximately zero before the switching-off process starts. As shown in Figure 3, the flowrate through the valve is already small enough when the valve starts to switch off; thus, the transition power loss is greatly reduced compared to that in Figure 2.
In an existing zero-flowrate switching controller (as shown in Figure 4) developed by S. Peng [6,7], two parallel control lines are designed to regulate flowrates through the lines: a main control line is in charge of switching on/off the control line between power source and node N; an auxiliary control line is an assist to regulate the flowrates through the two lines and realize a zero-flowrate switching controller. When there is a need to switch off the control line, instead of switch off the control line directly, the auxiliary control line is switched on to connect a hydraulic resonator parallelly to the main control line. Therefore, flowrates through the main control line will be reduced when the resonant flowrates of the auxiliary line increases; the main control valve can be switched off when the flowrate is reduced to minimum. After the main control valve is switched off, the flowrate before the capacity keeps resonating until it drops to zero; then it is time to switch off the valve in the auxiliary control line. In the end, all the valves in this hydraulic system are switched off at minimum flowrates. Transition power losses have been greatly reduced.
In above research work, a hydraulic resonator in the auxiliary control line, consisting of a hydraulic capacity and a hydraulic inductance, produces harmonic flowrate waves at resonance frequency
ω C = 1 ω L ,
where C is capacitance; L is the inductance; and ω is angular frequency of the resonator. The flowrate waves make flowrate minimum (approaching zero) at some moments when they are best to switch off the switching valve.
This design offers an feasibility to reduce transition power loss while it is only suitable for the switching valves whose transition times are no more than 2 ms. Considering about the high-cost and difficulties of obtaining such valves, it is practical to apply those hydraulic controllers with normal switching valves. In following section, the authors are going to propose an improved zero-flowrate switching controller where the transition time of the switching valve is as large as 20 ms.

III. An Improved Zero-Flowrate Switching Controller

To better regulate flowrates through the lines and effecitively reduce switching power loss with valves whose transition times are larger than those in previous research work, a novel capacity-based solution is proposed. The improved zero-flowrate switching controller, shown in Figure 5, utilizes capacity to absorb large flowrate and regulate flowrate through the lines. Similar to previous zero-flowrate switching controller in [6,7], there are two parallel control lines: the main control line and the auxiliary control line. In the schematic presented in Figure 5, conduit 1 is a lumped model which represents total resistance through the main control line; conduit 2 is a lumped model that represents total inductance/resistance through the auxiliary control line; capacity C is a lumped model representing all the capacity through the auxiliary control line. Capacitance and inductance through the main control line are minor for system performance and ignored in the models of this paper. The load is simplified as an orifice in this system.
The capacity in auxiliary control line has two independent ports: inlet connected with power source and outlet connected to load direction, which leads to independent control of flowrates at inlet/outlet ports. In an ideal capacity model, the pressure differential at inlet makes flowrate changes as the following equation explains.
d p S d t = B Q o u t v o l 0
where Ps is the pressure of power source, Qout is the flowrate into the load, B is bulk modulus, vol0 is the volume of the capacity, t is the time. By inputting proper pressure difference at inlet of capacity, there will be a flowrate increase through the auxiliary control line, and accordingly a flowrate drop through the main control line, as Figure 4 explains; in the same time, the flowrates at outlet of capacity is stabled zero by the inductance of conduit 2 so that enough transition time for valve 3 is guaranteed. The resistance ratio of conduit 1 and conduit 2 is related to flowrate ratio of the two lines. By designing proper resistance for the two lines, desired flowrates can be obtained for both lines.
Stage 1
This is a working stage. As presented in Figure 6(a), the main control line is connecting the power source to the load; the auxiliary line is disconnected; and the system is actuating the load. The capacity is connected with the tank and the inlet pressure is equal to tank pressure.
Stage 2
When there is a need to stop the load, stage 2 is started: valve 2 and valve 3 are switched on to connect the capacity and conduit 2 parallel to the main control line. By switching from the tank to the power source at inlet of the capacity, it makes a large pressure difference to the capacity, which fulfills the condition for the flowrate at inlet of capacity to accelerate. As a result, the flowrate through the auxiliary line rises up until the flowrate through the main control line drops to minimum. Since the capacity is large enough and there is an inductance at the outlet of the capacity, flowrate through valve 3 stays zero for some time which can be long enough for valve 3 to switch off at zero flowrate.
Stage 3
At the beginning of this stage, the flowrate through the main control line has already been minimum, when is the best time to switch off valve 1. After valve 1 is switched off, almost all the flowrate from the power source goes to the capacity. Due to the effect of inductance, flowrate through valve 3 keeps zero in this stage.
Stage 4
After valve 3 is switched off, both the main control line and the auxiliary control line have been disconnected; in the same time, the inlet port of capacity is still connected with the power source. It takes some time for the capacity to finish relieving and the inlet pressure to achieve stabilization.
Stage 5
When inlet pressure of the capacity has achieved stabilization, the inlet flowrate (which is also the flowrate through valve 2) becomes zero, accordingly; then valve 2 can be switched off at zero flowrate. As Figure 6(e) shows, valve 2 now is connected to the tank allowing capacity pressure to relieve, which is to make sure there will be enough pressure difference for next switching-off process. The working states of the three valves in 5 stages are presented in Table 1.

IV. Validation of the Improved Flowrate-switching Method

In this paper, commercial software AMESim is used to build models for hydraulic systems. Before the presentation of a complete hydraulic system, AMESim submodels will be introduced; with these submodels, the characteristics of a capacity to generate flowrate pulse with step pressure input will be investigated; based on which, the performance of a switching control line with 2 parallel branches (one is the main control line and the other is the auxiliary line) excited by step input pressure is analyzed. The results show feasibility of the mechanism of a zero-flowrate switching technology. With these validations, an optimization design of a zero-flowrate switching actuator is obtained and a complete actuation model will be built and investigated for power loss and efficiency performance in next section.

A. AMESim Submodels for Hydraulic Conduits

Ideally, the inductance conduit is purely an inductive element. However, friction effect existing in a physical tube yields resistance; compressibility of the fluid makes capacitive effects. Capacitance and resistance are distributed along the conduits throughout a physical system. In this simulation, capacitance, resistance, and inductance through the conduits are modeled with different lumped submodels according to the main effects of the components. The AMESim submodels of hydraulic conduits applied in this work are HL0000 (C), HL0000 (C-R), HL002 (R-C), HL002 (R-C-R), HL0002 (R-C-R), HL0040 (C-IR-**-C-IR). The description of these submodels can be checked in help file from AMESim software. In all these submodels, capacity is modeled as a pure capacitive element. Take submodel HL002 (R-C-R) for example, to compute capacitive effect, the formula for computing the pressure derivative at center of the pipe is
∂ P ∂ t = − B A · ∂ Q ∂ x
where P/Q is pressure/flowrate at center of the pipe, x is the axial position along the pipe; A is the cross-sectional area of the pipe and B is the effective bulk modulus of the pipe/fluid combination. Capacitance in all the 6 submodels share principle of (3), only the input/outputs are different. According to (3), a pressure difference at inlet port of capacity causes flowrate pulse; larger cross-section area of the pipe (which is actually the dimension of inlet port) leads to larger flowrate difference.
In some occasions, resistance and inductance of the conduit can be ignored, where submodel HL0000 (C) match the applications; while in some other occasions, resistance is necessary to be included in a conduit model. AMESim provides different submodels considering about both resistance and capacitance. For example, submodel HL002 (R-C-R) contains a capacitance and 2 resistive elements at either end, and each resistive element represents the resistance of one half of the total conduit length. The flowrate at port 1 and port 2 takes into account geometry and are calculated with
v = 2 · d i a m · | Δ P − ρ · L · 9.81 · sin ( θ ) | L · ρ · f f
where v is the mean fluid velocity, diam the pipe hydraulic diameter, ΔP the pressure drop, ρ the density, L the pipe length, θ the inclination of the pipe and ff the friction factor. According to (4), by adjusting the length and diameter of the pipe, the desired mean fluid velocity (which is actually the flowrate) can be obtained. Friction factor has an effect on the mean fluid velocity, thus, different material of the inner wall of the pipe lead to different resistive effect. In this work, standard material is utilized and further investigation of friction effect is not included here.
In some occasions, inductance/inertia has an effect on flowrate/pressure response, where AMESim submodel HL 0040 (C-IR-**-C-IR) can be applied. HL 0040 (C-IR-**-C-IR) is a complex distributed parameter submodel for a hydraulic conduit taking into account of compressibility of the fluid, expansion of the pipe/hose wall with pressure, and inertia of the fluid in several lumped elements along the line; in the related submodels, the flowrate derivatives can be corrected by a term corresponding to frequency dependent friction. More description of this submodel can be checked with AMESim help file.

B. Validation Models

Before develop a complete model for a zero-flowrate switching actuator, two models should be validated. The first model is a capacity applied in a simple line with step input pressure, which validate the feasibility of capacity in this novel method; the second model is basic schematic of a zero-flowrate switching controller, which validates the feasibility of the principle of this novel method.
1)
Performance of a Capacity with Step Pressure Source
In the model presented in Figure 7, a step pressure source is used to supply a line where there is only a capacity and a tank. The pressure/flowrate of the tank is zero. AMESim submodel HL0002 R-C-R is used before and after the capacity to simulate the effect of the fluid compressibility and friction of conduit walls of the conduits. Before the capacity, flowrate changes as pressure varies. Theoretically, a step pressure input will result in maximum flowrate pulse at inlet of capacity. The result in Figure 8 shows that flowrate is up to 464 L/min at the moment when input pressure rises from 0 bar to 2 bar, and finally keeps at 373 L/min. According to the explanation in Figure 4, a flowrate of 373 L/min through the auxiliary line is able to make the flowrate through the main control line zero in the conditions where load flowrate is under 373 L/min, which is quite large in most cases. Thus applying such a capacity in this novel method is feasible.
2)
A basic Schematic for Zero-flowrate Switching Controller
In the model presented in Figure 9, a basic schematic for improved zero-flowrate switching controller is simulated to validate the feasibility of the novel method. As Figure 5 shows, there are two parallel lines, one is the main control line, and the other is the auxiliary control line. The main control line is the same one as shown in Figure 7; the conduit through the line is modeled with AMESim submodel HL002 R-C-R. By adding a parallel auxiliary control line which consists of a capacity and two conduit models, it is possible to investigate the flowrate response and validate the feasibility of the novel method. To make flowrates of the two lines as required, total resistance and capacitance through the two lines are considered with proper submodels and parameters: capacity is 0.8 L; submodels and parameters for conduit pieces 1, 2, 3, and 4 are presented in Table 2.
Figure 10 shows simulation results for this model. The input pressure source is the same as that in former model. Flowrate before capacity and through the main control line are presented in Figure 10. When input pressure increases, the flowrate through the main control line rises up to 13.6 L/min; the flowrate through the auxiliary control line first blow up to 281.3 L/min and then drops to 13.6 L/min, and finally stabilizes at this value.

V. Simulation Model of an Improved Zero-Flowrate Switching (IZFS) Controller

A simulation model (as presented in Figure 11) is now developed to validate the effectiveness of a zero-flowrate switching control method applied in a complete hydraulic actuation system. The power source is modelled with small pressure waves as a normal piston pump outputs for better accuracy; the load is a hydraulic cylinder moving at a constant speed; the hydraulic control unit is a zero-flowrate switching controller; check valve 1 is to prevent flowrate from flowing from the capacity to the main control line; check valve 2 is to prevent flowrate flowing from load to the main control line and auxiliary line.
A contrast system is designed as Figure 12 presents. The power unit and load unit are the same as those in a zero-flowrate switching control system, while there is only one switching valve applied in the hydraulic control unit. When there is a need to switch off the load system, the zero-flowrate switching control system will start a switching-off process as Figure 6 explains, and the contrast system is to switch off the switching valve directly. The authors will analyze the performances and compare efficiencies of the two system to validate the feasibility of improved zero-flowrate switching control method proposed in this work. More details about the two systems will be explained as follows.

A. The Power Unit

The model of a power unit is to simulate a real power source considering about some dynamic characteristics. A real power source normally outputs small pressure waves instead of ideal constant pressure. This is due to periodicity movement of the pistons inside the pump. for example, there are 9 pistons within a pump, and the rotation speed of the pump is 1000 r/s, thus the piston frequency is 111 Hz which is also the frequency of output flowrates and pressures. In this simulation, a capacity is applied to regulate flowrates between two branches. Since a capacity is possible to generate vibrations with pressure waves, a power unit with small pressure waves are modeled for better accuracy of the evaluation of the system performance. In the simulation model, hydraulic submodels like pump (PU001) and relief valve (RV010) are used to offer a constant pressure 100 bar. An expanded algebra model is built to add a wave characteristic to output pressure of the pump. The output pressure of the pump unit is a sine wave whose frequency, amplitude and average pressure are 111 Hz, 1 bar, and 100 bar, respectively.

B. The Load Unit

To better simulate the physical application, a hydraulic cylinder is used to generate a constant flowrate of 8 L/min; a closed-loop electronic controller is applied to keep the cylinder moving at a constant speed.

C. The Conduits

As shown in Figure 8, a pressure drop of 2 bar is able to excite a flowrate increase of 373 L/min. Actually, it is not necessary to obtain such a large flowrate change in most applications. In this simulation model, conduits with proper diameters and lengths are used in both main control line and auxiliary control line to regulate flowrates for the two branches. Conduits 1-5 are applied to offer capacity, resistance, and inductance for the two branches. Conduit 1 is before valve 1, submodel HL0000 C is used here to simulate the capacity at inlet of valve 1; conduit 2 connects valve 1 and the load, submodel HL0001 C-R is used to simulate the capacitance and resistance of the conduit; conduit 3 connects check valve 1 and valve 2, submodel HL0000 C is used to simulate effect of capacitance at inlet of valve 2; conduit 4 is actually the inlet of capacity, here submodel HL0040 C-IR***C-IR is used to simulate inertia, capacitance and resistance of the inlet; conduit 5 is an inductance pipe after the capacity to stablize flowrate and pressure, where submodel HL0040 C-IR***C-IR is used to simulate inductance, as well as resistance and capacitance of the pipe. Parameters of the 5 pieces of conduits are presented in Table 3.

D. The Model of an Improved Zero-Flowrate Switching (IZFS) Actuator

In the simulation model, an improved zero-flowrate switching control method explained in Figure 6 is applied to switch off the control line at minimum cost. The following model is designed to realize such a control method: a switching valve is used to control the main control line; a parallel auxiliary line is designed with a capacity volume in series for flowrate regulation. The capacity volume is a chamber with separate inlet/outlet ports instead of an accumulator whose inlet and outlet ports share the same port. When there are varies pressures at inlet and a constant pressure at outlet, there can be varies flowrates at inlet and stabilization of flowrate at outlet. Two switching valves are connected before/after the capacity to connect/disconnect the capacity with the system. In stage 4 of the switching process, the pressure of the capacity might be higher than that of power source, thus, check valve 1 is applied to prevent flowrate from the capacity back to pump or the main control line. During switching off process, there might be some oscillation in the cylinder, check valve 2 will make flowrates at the end of auxiliary control line more stable at zero, which makes switching power loss of valve 3 absolutely zero.
As explained in Figure 13 and Figure 14, an electronic controller of a zero-flowrate switching control system is needed to send null signals to valves when relating flowrate is zero. In the simulation model, the zero-flowrate times for valve 1, valve 2, and valve 3 are 1.02 s, 1.086 s, and 1.019 s, respectively; the closing time of the valves is 20 ms. As explained in Figure 13, a linear piecewise signal is designed to control valves. When 0.5⩽command<1, valve 1 is connected in the system and the load is working; when Command=1, the switching-off period starts: firstly, valve 2 and valve 3 switch on; then valve 1, 2 and 3 switch off at T1, T2, and T3 when flowrates are zero, respectively. When all the valves have switched off, the switching-off period is over.
Logic controller 1, 2, and 3 are designed to convert the command to proper control signals for valve 1, 2, and 3. Take Logic control 1 for example, two AMESim submodels SWITCH01 are used to send out different signals on different conditions. When command<0.5, SWITCH1 outputs 0; when command⩾0.5, SWITCH1outputs signals from SWITCH2; when command<1.02, SWITCH2=1; when command⩾1.02, SWITCH2=0. Logic controller 2 and 3 are designed in similar principles as explained in Figure 14.

E. A Hard Switching (HS) System

A hard switching control system is designed as a contrast. As presented in Figure 13, a step signal is used to send null signal to the switching valve when it is time to switch off the control line. The power source and the load are the same as those in an improved zero-flowrate switching control actuator system.

VI. Simulation Results

As explained above, simulations of the improved zero-flowrate switching system and hard switching system were conducted. Switching power losses of each valve have been calculated as the production of the pressure drop and flowrates of the valves. total switching power losses is also accumulated. Simulation results are presented in Figure 15, Figure 16, Figure 17, Figure 18 and Figure 19. As presented in Figure 16, when electronic controller starts to send out command at 0 s, the displacement of the cylinder starts to increase from 0 m linearly until the command signal becomes 1 at 1 s; then the cylinder piston stops at 0.01 m without any vibration, which validates that the displacement response of the zero-flowrate switching system is accurate and smooth. At 1 s, valve 2 and valve 3 switch on, the flowrate Q2 of valve 2 increases immediately, and the flowrate Q1 of valve 1 responses to drop down; in the same time, the profile of flowrate Q3 through valve 3 sinks for a little bit due to the effect of capacity; after a little while Q3 goes back to zero at 1.04 s. This is due to the effect of capacity. Before valve 2 and valve 3 switch on, the pressure of capacity is tank pressure (0 bar); after the two valves switch on, the capacity starts to absorb fluid from both inlet/outlet ports until outlet pressure achieves load pressure when flowrate Q3 is back to zero at 1.019 s, which is the time to switch off valve 3. When Q1 is equal to 0.8 L/min at 1.02 s, valve 1 starts to switch off; in the same time Q2 through valve 2 keeps increasing until Q2 achieves 10 L/min, then Q2 keeps at this value until t=1.085 s, which is due to the effect of capacity. Then the capacity discharges fluid quickly and Q2 becomes zero at 1.086 s, when valve 2 starts to switch off.
Figure 17 presents switching power loss of valve 1, valve 2, and valve 3 in the IZFS system. After switching-off command is sent out at 1 s, switching power loss for valve 1 firstly peaks up to 0.4 W and then drops to 0 W quickly. Switching power loss for valve 2 and valve 3 are both 0 W.
As a contrast, a HS system also shows smooth and accurate displacement response as presented in Figure 18. Average flowrate through the valve keeps at 8 L/min.
Figure 19 shows that the switching power loss of a HS system blows up to 163.62 W and then back to 0 W at 1.02 s, when the switching valve is totally switched off.
By comparing switching energy losses between IZFS and HS systems in Figure 20, an IZFS system consumes 0.055% of switching energy loss that is consumed by a HS system. In another word, a IZFS system makes switching energy loss almost 0 J.

VII. Discussion

With the characteristics of capacity investigated, optimization design of resistance, inductance and capacitance can be obtained and guide design optimization of a complete zero-flowrate switching actuator. Generally, it can be seen that capacitance through the auxiliary line absorbs the flowrate that is supposed to flow through the main control line; thus, the capacitance should match the actual flowrates of the main control line. the inductance after the capacity works to stable the flowrate at the end of the capacity at zero for long enough until the valve after it closes totally. Furthermore, the resistance through the two control lines helps to regulate the flowrates through each lines. By changing the ratio of resistances of the two lines, the ratio of flowrates through the two lines can be changed. It can be concluded as follows:
a)
A capacity is able to generate flowrate pulses. When there is a proper capacity in the line, it is able to generate flowrate pulse which is up to 373.041 L/min when pressure pulse is 2 bar.
b)
Flowrates for the main control line and auxiliary line can be regulated as desired by designing proper resistance and capacitance in each line. None of hydraulic components is a pure capacitance or resistance. A capacity chamber is mostly a capacitive element while there is resistance at inlet/outlet ports; a piece of conduit is a typical resistive element while it also shows capacitive effect due to elastic wall and compressibility of the fluid. To explain the effect of these capacitance and resistance in the system, lumped models presented in Figure 21 are used to simulate different hydraulic components. The resistance through the main control line is regarded as a resistive element R1; the resistance through the auxiliary line is regarded as a resistive element R2; the total capacitance (capacitance of the capacity chamber and conduits) through the main control line is regarded as a capacitive element C. With pressure pulse and capacitance C, it is able to generate large flowrate pulses in the auxiliary line; with proper values of resistance R1 and R2, proper values of Q1 and Q2 can be obtained.
a)
In this work, the author chose to switch off valve 1 at 1.02 s when flowrate Q1 is 0.8 L/min. The total switching energy loss is 7.1301e-04 J which is almost 0 J. It is possible to switch off valve 1 when Q1 is absolutely 0 L/min at 1.031 s; as a result, the switching-off time is delayed by 11 ms. Therefore, it is a trade-off between smaller flowrate and larger switching-off time. In a real application, the decision can be made depending on actual requirements of the application.

VIII. Conclusion

This paper presents basic principle and performance of an improved zero-flowrate switching method which is supposed to make switching energy loss almost zero. models with a capacity applied in a simple line are built to validate the feasibility of a capacity with 2 independent inlet/outlet ports proposed in this novel method; based on the validation, a complete zero-flowrate switching controller applied in a constant-speed actuator is modeled with hydraulic inductance, resistance and capacitance described as lumped models. With optimization of resistance, inductance and resistance, simulation results shows that this novel control method is feasible to save switching energy by 99.945% as expected.

References

  1. M. Linjama, “Digital fluid power – state of the art,” The Twelfth Scandinavian International Conference on Fluid Power, May 18-20, 2011, Tampere, Finland.
  2. J. Andruch and J. H. Lumkes, “Regenerative hydraulic topographies using high speed valves,” SAE Commercial Vehicle Engineering Congress and Exhibition, 2009, Rosemont, Illinois, USA. [CrossRef]
  3. S. Liu and B. Yao, “Coordinate control of energy saving programmable valves,” IEEE Transactions on Control Systems technology, Vol. 16, No. 1, January 2008. [CrossRef]
  4. B. Xu, R. Ding, and J. Zhang, “Experiment research on individual metering systems of mobile machinery based on coordinate control of pump and valves,” Journal of Zhejiang University (Engineering Science), Vol. 49, No. 1, 2015.
  5. Q. Gao, Y. Zhu, Z. Luo, R. Wang, Y. Song and X. Chen, “Hardware-in-the-loop simulation for position control of hydraulic cylinder using high speed on/off valve,” Transactions of Beijing Institute of Technology, Vol. 39, No. 10, October, 2019. [CrossRef]
  6. Z. Jiao, C. Peng, and S. Wu, “Progress in construction machinery multi-way valve and future trends,” Chinese Hydraulics & Pneumatics, 2013(1). [CrossRef]
  7. J. Cao, L. Gu, F. Wang, and M. Qiu, “Switch-mode hydraulic power supply theory,” Proceedings of the 2005 ASME-IMECE, 2005, Paper No. IMECE2005–79019. [CrossRef]
  8. M. B. Rannow et al, “Software enabled variable displacement pumps—experimental studies,” Proceedings of the 2006 ASME-IMECE, Chicago, IL, Nov. 5–10, 2006, Paper No. IMECE2006-14973. [CrossRef]
  9. P. Y. Tu et al, “High speed rotary pulse width modulated on/off valve,” Proceedings of the 2007 ASME-IMECE, Seattle, WA, Nov. 11–15, 2007, Paper No. IMECE2007–42559. [CrossRef]
  10. J. D. Van de Ven, “On fluid compressibility in switch-mode hydraulic circuits-Part I: modeling and analysis,” Journal of Dynamic Systems, Measurement, and Control, Vol, 135/021013-1, March 2013. [CrossRef]
  11. M. B. Rannow and P. Y. Li, “Soft switching approach to reducing transition losses in an on/off hydraulic valve,” Journal of Dynamic Systems, Measurement, and Control, November 2012, Vol. 134/064501-1. [CrossRef]
  12. S. Peng, “The concept of a zero-flowrate-switching controller,” The Eighth Workshop on Digital Fluid Power, May 24 – 25, 2016, Tampere, Finland.
  13. S. Peng, “Modeling and theoretical analysis of zero-flowrate-switching control method for a dynamic load system to reduce switching power loss of control valves,” Actuators, Vol. 12, No. 183, 2023. [CrossRef]
  14. G. Hua and F. C. Lee, “Soft-switching techniques in PWM vonverters,” IEEE Transactions on Industrial Electronics, Vol. 42, No. 6, December 1995. [CrossRef]
Preprints 100735 g001
Figure 1. An actuation system with a switching valve.
Figure 1. An actuation system with a switching valve.
Preprints 100735 g001
Preprints 100735 g002
Figure 2. Pressure/Flowrate response in a zero-flowrate switching system.
Figure 2. Pressure/Flowrate response in a zero-flowrate switching system.
Preprints 100735 g002
Preprints 100735 g003
Figure 3. Switching power loss for a ZFS system.
Figure 3. Switching power loss for a ZFS system.
Preprints 100735 g003
Preprints 100735 g004
Figure 4. Principle of a ZFS system.
Figure 4. Principle of a ZFS system.
Preprints 100735 g004
Preprints 100735 g005
Figure 5. Schematic of an improved zero-flowrate switching controller.
Figure 5. Schematic of an improved zero-flowrate switching controller.
Preprints 100735 g005
Preprints 100735 g006aPreprints 100735 g006b
Figure 6. Sequence of operation of a zero-flowrate switching system.
Figure 6. Sequence of operation of a zero-flowrate switching system.
Preprints 100735 g006aPreprints 100735 g006b
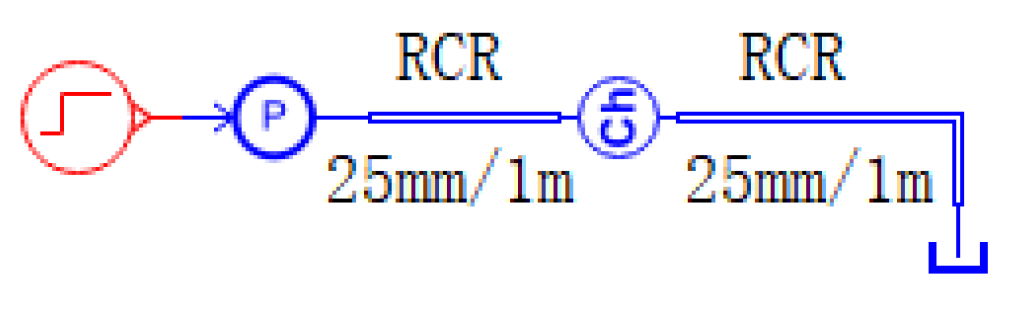
Preprints 100735 g007
Figure 7. A System with a step pressure source and a capacity in the line.
Figure 7. A System with a step pressure source and a capacity in the line.
Preprints 100735 g007
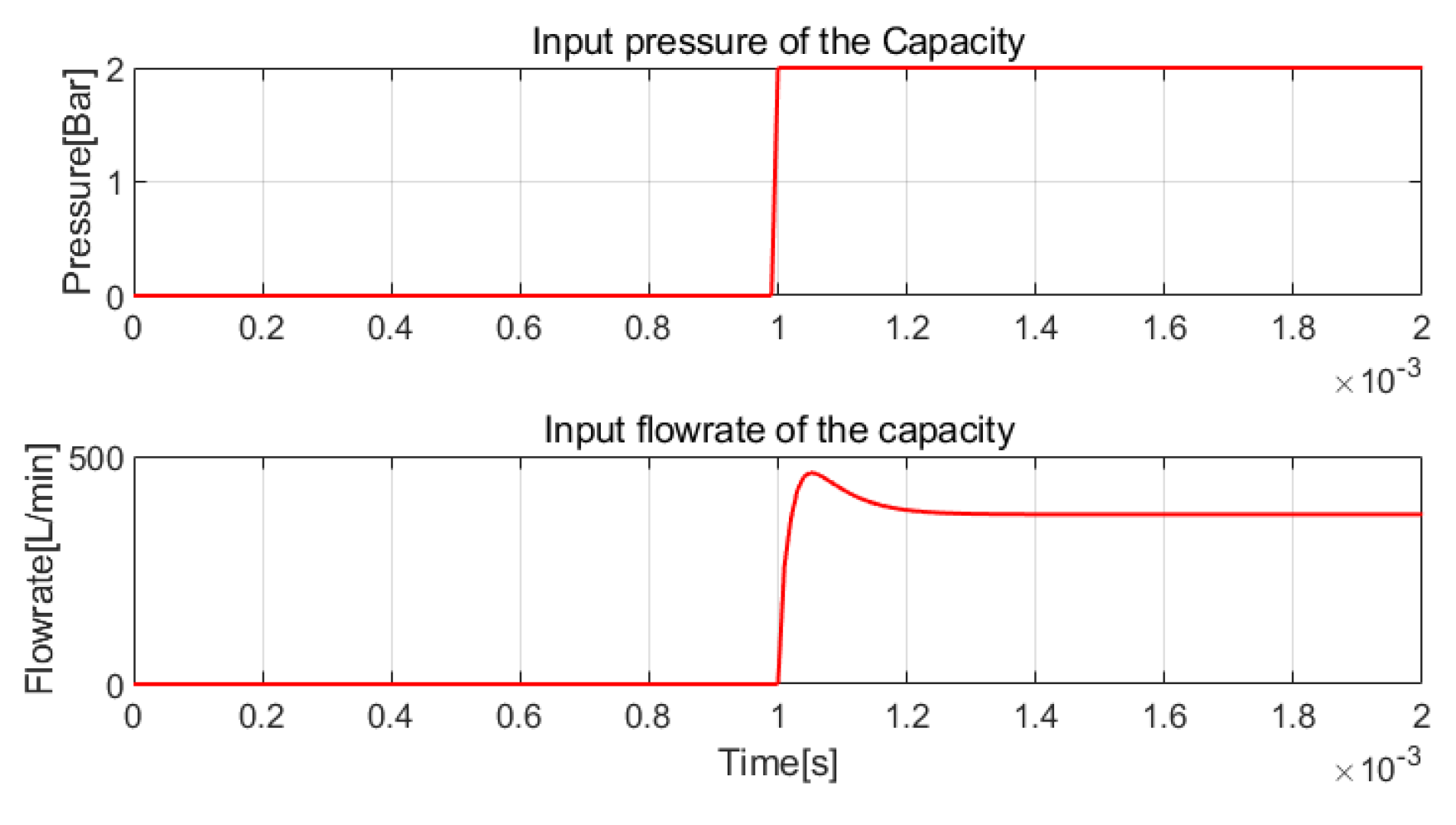
Preprints 100735 g008
Figure 8. Pressure/Flowrate response of a system with a step pressure source and a capacity in the line.
Figure 8. Pressure/Flowrate response of a system with a step pressure source and a capacity in the line.
Preprints 100735 g008
Preprints 100735 g009
Figure 9. An improved zero-flowrate switching control line.
Figure 9. An improved zero-flowrate switching control line.
Preprints 100735 g009
Preprints 100735 g010
Figure 10. Simulation results for an improved zero-flowrate switching control line: step pressure power source; flowrate response through the main control line; flowrate response through the auxiliary control line.
Figure 10. Simulation results for an improved zero-flowrate switching control line: step pressure power source; flowrate response through the main control line; flowrate response through the auxiliary control line.
Preprints 100735 g010
Preprints 100735 g011
Figure 11. The simulation model of an improved zero-flowrate switching system in AMESim software.
Figure 11. The simulation model of an improved zero-flowrate switching system in AMESim software.
Preprints 100735 g011
Preprints 100735 g012
Figure 12. The simulation model of a hard switching system in AMESim software.
Figure 12. The simulation model of a hard switching system in AMESim software.
Preprints 100735 g012
Preprints 100735 g013
Figure 13. Command signals of the electronic controller.
Figure 13. Command signals of the electronic controller.
Preprints 100735 g013
Preprints 100735 g014
Figure 14. Description of logic controller 1, 2, and 3.
Figure 14. Description of logic controller 1, 2, and 3.
Preprints 100735 g014
Preprints 100735 g015
Figure 15. Displacement/Flowrate performance of IZFS system.
Figure 15. Displacement/Flowrate performance of IZFS system.
Preprints 100735 g015
Preprints 100735 g016
Figure 16. Switching power loss of IZFS system.
Figure 16. Switching power loss of IZFS system.
Preprints 100735 g016
Preprints 100735 g017
Figure 17. Displacement/Flowrate performance of HS system.
Figure 17. Displacement/Flowrate performance of HS system.
Preprints 100735 g017
Preprints 100735 g018
Figure 18. Switching power loss of HS system.
Figure 18. Switching power loss of HS system.
Preprints 100735 g018
Preprints 100735 g019
Figure 19. Compare of switching energy losses between IZFS/HS systems.
Figure 19. Compare of switching energy losses between IZFS/HS systems.
Preprints 100735 g019
Preprints 100735 g020
Figure 20. Hydraulic schematic with lumped resistance and capacitance.
Figure 20. Hydraulic schematic with lumped resistance and capacitance.
Preprints 100735 g020
Table 1. Working States of Three Valves in Five Stages.
Table 1. Working States of Three Valves in Five Stages.
Valve 1 Valve 2 Valve 3
Stage 1 ON OFF OFF
Stage 2 ON ON ON
Stage 3 OFF ON ON
Stage 4 OFF ON OFF
Stage 5 OFF OFF OFF
Table 2. Submodels and Parameters of Conduits in the Main Control Line/Auxiliary Control Line from load to the control unit.
Table 2. Submodels and Parameters of Conduits in the Main Control Line/Auxiliary Control Line from load to the control unit.
CONDUIT PIECE SUBMODEL DIAMETER (mm) LENGTH(m)
1 HL002, R-C-R 25 0.01
2 HL002, R-C-R 25 1
3 HL002, R-C-R 8 0.2
4 HL002, R-C-R 10 1
Table 3. Parameters of the Conduits.
Table 3. Parameters of the Conduits.
Conduit Submodel Diameter (mm) Length (m)
① HL0000 C 25 0.01
② HL0001 C-R 4 0.1
③ HL000 C 25 0.01
④ HL0040 C-IR***C-IR 25 0.01
⑤ HL0040C-IR***C-IR 8 0.2
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content.
Copyright: This open access article is published under a Creative Commons CC BY 4.0 license, which permit the free download, distribution, and reuse, provided that the author and preprint are cited in any reuse.
Prerpints.org logo

Preprints.org is a free preprint server supported by MDPI in Basel, Switzerland.

facebook logotwitter logolinkedin logo
MDPI Initiatives
Important Links
Subscribe

Disclaimer

Terms of Use

Privacy Policy

© 2024 MDPI (Basel, Switzerland) unless otherwise stated